A Conjugate Linearly Polarized Light Wave Along an Optical Fiber with the Berry Phase Model and Its Magnetic Trajectories According to the Conjugate Frame
Abstract
1. Introduction
2. Preliminaries
3. Berry Phase Model of the
4. Electromagnetic Curves of Along an Optical Fiber
4.1. The Lie Reduction
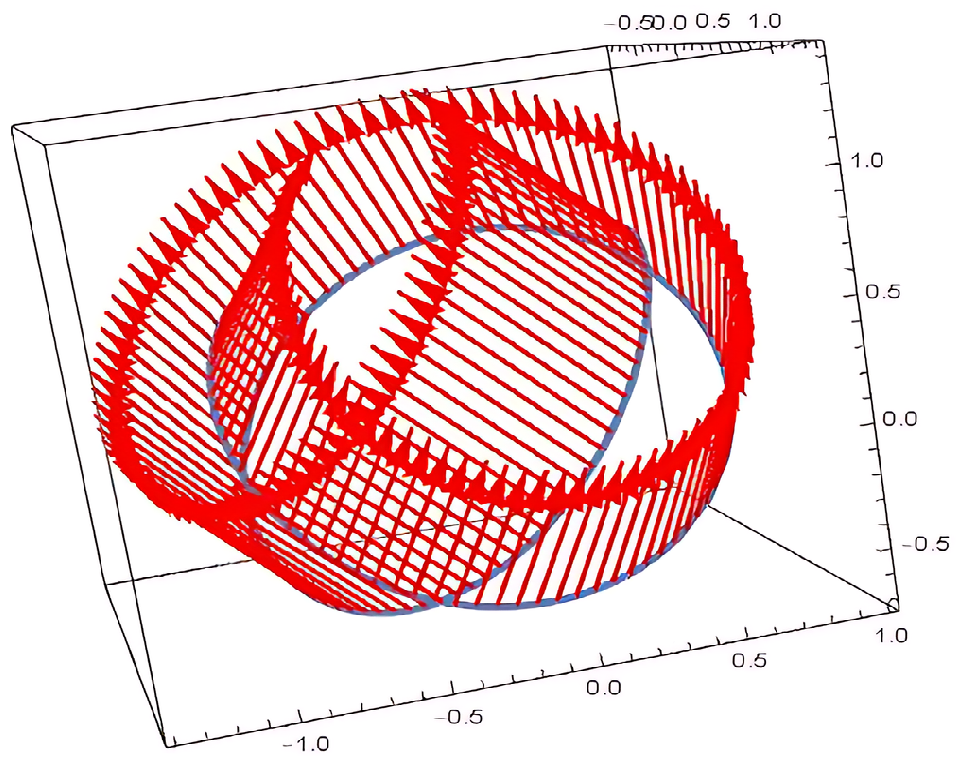
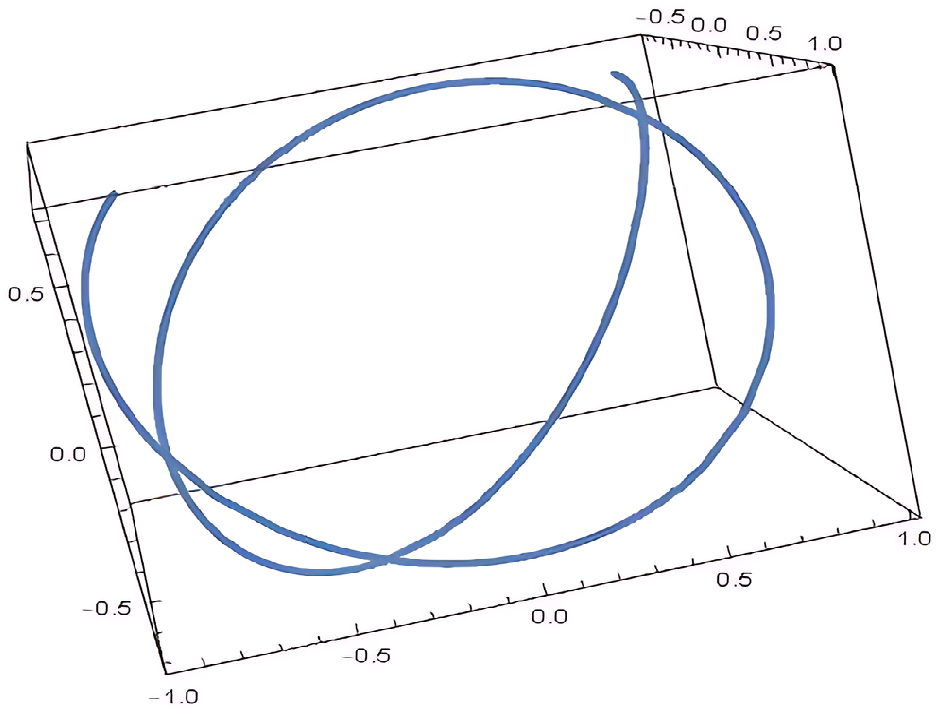
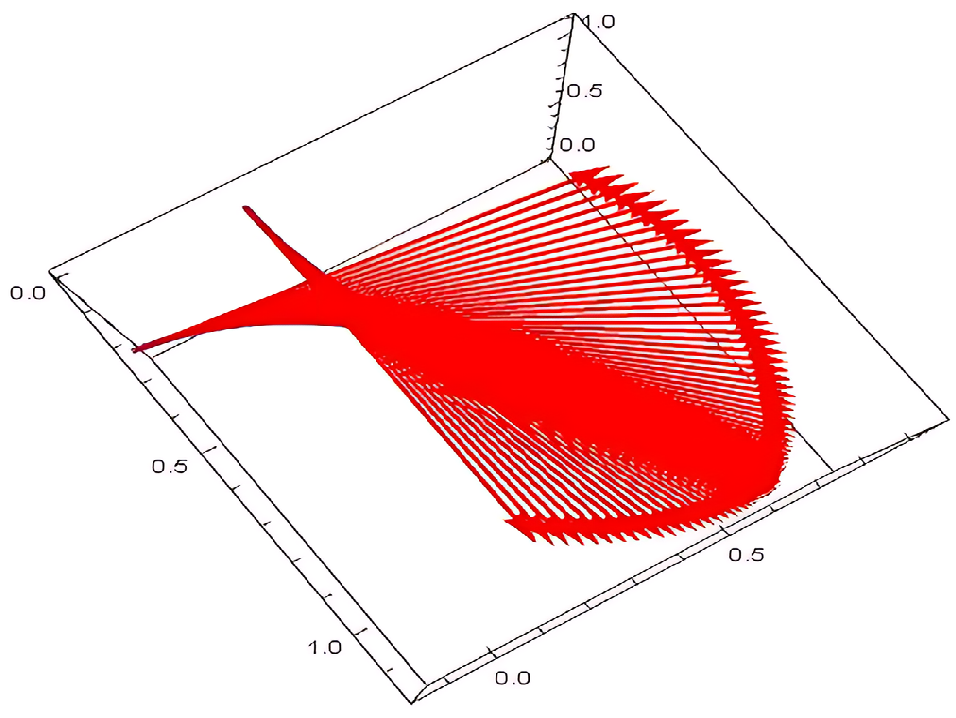
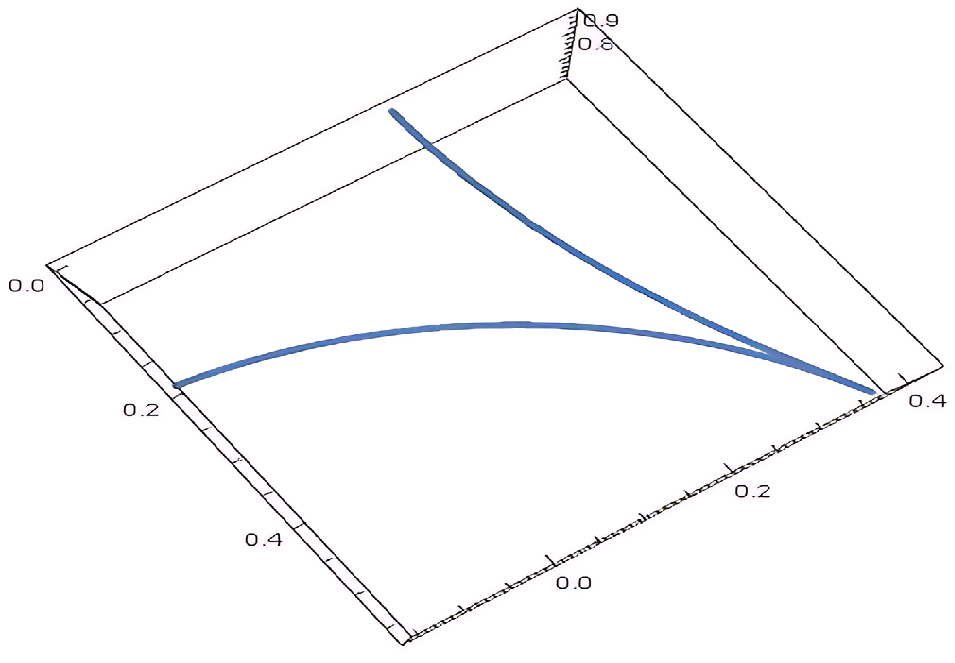
4.2. Magnetic Trajectories of Electromagnetic Curves
5. Discussion and Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Comtet, A. On the Landau levels on the hyperbolic plane. Ann. Phys. 1987, 173, 185–209. [Google Scholar] [CrossRef]
- Druţă-Romaniuc, S.L.; Munteanu, M.I. Killing magnetic curves in a Minkowski 3-space. Nonlinear Anal. Real World Appl. 2013, 14, 383–396. [Google Scholar] [CrossRef]
- Efimov, D.I. The magnetic geodesic flow on a homogeneous symplectic manifold. Sib. Math. J. 2005, 46, 83–93. [Google Scholar] [CrossRef]
- Munteanu, M.I.; Nistor, A.I. The classification of Killing magnetic curves in S2 × . J. Geom. Phys. 2012, 62, 170–182. [Google Scholar] [CrossRef]
- Novikov, S.P. The Hamiltonian formalism and a many-valued analogue of Morse theory. Russ. Math. Surv. 1982, 37, 1. [Google Scholar] [CrossRef]
- Yazıcı, B.D.; Okuyucu, O.Z.; Tosun, M. Framed curves in three-dimensional Lie groups and a Berry phase model. J. Geom. Phys. 2022, 182, 104682. [Google Scholar] [CrossRef]
- Sunada, T. Magnetic flows on a Riemannian surface. In Proceedings of the KAIST Mathematics Workshop Analysis and Geometry, Taejeon, Republic of Korea, 3–6 January 1993; Volume 8, pp. 93–108. [Google Scholar]
- Körpinar, T. Bianchi type-I cosmological models for inextensible flows of biharmonic particles by using curvature tensor field in spacetime. Int. J. Theor. Phys. 2015, 54, 1762–1774. [Google Scholar] [CrossRef]
- Özdemir, Z. A new calculus for the treatment of Rytov’s law in the optical fiber. Optik 2020, 216, 164892. [Google Scholar] [CrossRef]
- Gürbüz, N.E. The pseudo-null geometric phase along optical fiber. Int. J. Geom. Methods Mod. Phys. 2021, 18, 2150230. [Google Scholar] [CrossRef]
- Gürbüz, N.E. The variation of the electric field along optic fiber for null Cartan and pseudo-null frames. Int. J. Geom. Methods Mod. Phys. 2021, 18, 2150122. [Google Scholar] [CrossRef]
- Gürbüz, N.E. The null geometric phase along optical fiber for anholonomic coordinates. Optik 2022, 258, 168841. [Google Scholar] [CrossRef]
- Berry, M.V. The adiabatic phase and Pancharatnam’s phase for polarized light. J. Mod. Opt. 1987, 34, 1401–1407. [Google Scholar] [CrossRef]
- Nurkan, S.K.; Ceyhan, H.; Özdemir, Z.; Gök, İ. Electromagnetic curves and Rytov’s law in the optical fiber with Maxwellian evolution via alternative moving frame. Rev. Mex. Fís. 2023, 69, 061301-1. [Google Scholar] [CrossRef]
- Körpinar, T.; Demirkol, R.C. Electromagnetic curves of the linearly polarized light wave along an optical fiber in a 3D Riemannian manifold with Bishop equations. Optik 2020, 200, 163334. [Google Scholar] [CrossRef]
- Körpinar, T.; Demirkol, R.C. Berry phase of the linearly polarized light wave along an optical fiber and its electromagnetic curves via quasi adapted frame. Waves Random Complex Media 2022, 32, 1497–1516. [Google Scholar] [CrossRef]
- Kugler, M.; Shtrikman, S. Berry’s phase, locally inertial frames, and classical analogues. Phys. Rev. D 1988, 37, 934. [Google Scholar] [CrossRef]
- Ross, J.N. The rotation of the polarization in low birefringence monomode optical fibres due to geometric effects. Opt. Quantum Electron. 1984, 16, 455–461. [Google Scholar] [CrossRef]
- Ceyhan, H.; Özdemir, Z.; Gök, İ.; Ekmekci, F.N. Electromagnetic curves and rotation of the polarization plane through alternative moving frame. Eur. Phys. J. Plus 2020, 135, 867. [Google Scholar] [CrossRef]
- Casas-Alvero, E. Singularities of Plane Curves; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- El Kahoui, M.H.; Moussa, Z.Y. An algorithm to compute the adjoint ideal of an affine plane algebraic curve. Math. Comput. Sci. 2014, 8, 289–298. [Google Scholar] [CrossRef]
- Gorenstein, D. An arithmetic theory of adjoint plane curves. Trans. Am. Math. Soc. 1952, 72, 414–436. [Google Scholar] [CrossRef]
- Zymaris, A.S.; Papadimitriou, D.I.; Giannakoglou, K.C.; Othmer, C. Adjoint wall functions: A new concept for use in aerodynamic shape optimization. J. Comput. Phys. 2010, 229, 5228–5245. [Google Scholar] [CrossRef]
- Hunt, B. Differential Geometry, Curves–Surfaces–Manifolds; AMS: Providence, RI, USA, 2006. [Google Scholar]
- Nurkan, S.K.; Güven, İ.A.; Karacan, M.K. Characterizations of adjoint curves in Euclidean 3-space. Sect. A Phys. Sci. 2019, 89, 155–161. [Google Scholar] [CrossRef]
- Sariaydin, M.T.; Korpinar, T. An Approach for Vectorial Moments in Euclidean 3-Space. Honam Math. J. 2020, 42, 187–195. [Google Scholar]
- Güler, F. Surface Pencil with a Common Timelike Adjoint Curve. Palest. J. Math. 2024, 13, 302–309. [Google Scholar]
- Güler, F. Construction of surface pencil with a given spacelike adjoint curve. Adv. Stud. Euro-Tbil. Math. J. 2022, 15, 1–11. [Google Scholar] [CrossRef]
- Crouch, P.; Leite, F.S. The dynamic interpolation problem: On Riemannian manifolds, Lie groups, and symmetric spaces. J. Dyn. Control. Syst. 1995, 1, 177–202. [Google Scholar] [CrossRef]
- Al-Jedani, A.; Abdel-Baky, R. Sweeping surfaces due to conjugate Bishop frame in 3-dimensional Lie group. Symmetry 2023, 15, 910. [Google Scholar] [CrossRef]
- Keskin, Ö.; Yaylı, Y. Normal Fermi-walker derivative. Math. Sci. Appl. E-Notes 2017, 5, 1–8. [Google Scholar] [CrossRef]
- Karakuş, F.; Yayli, Y. The Fermi–Walker derivative in Lie groups. Int. J. Geom. Methods Mod. Phys. 2013, 10, 1320011. [Google Scholar] [CrossRef]
- Markovski, B.; Vinitsky, S.I. Topological Phases in Quantum Theory; World Scientific: Dubna, Russia, 1989. [Google Scholar]
- Kravtsov, Y.A.; Orlov, Y.I. Geometrical Optics of Inhomogeneous Media; Springer: Berlin/Heidelberg, Germany, 1990. [Google Scholar]
- Munteanu, M.I. Magnetic curves in a Euclidean space: One example, several approaches. Publ. de L’Inst. Math. 2013, 94, 141–150. [Google Scholar] [CrossRef]
- Barros, M.; Cabrerizo, J.L.; Fernández, M.; Romero, A. Magnetic vortex filament flows. J. Math. Phys. 2007, 48, 082904. [Google Scholar] [CrossRef]
- Noakes, L. Null cubics and Lie quadratics. J. Math. Phys. 2003, 44, 1436–1448. [Google Scholar] [CrossRef]
- Popiel, T.; Noakes, L. Elastica in SO3. J. Aust. Math. Soc. 2007, 83, 105–124. [Google Scholar] [CrossRef]
- Turhan, T. Magnetic trajectories in three-dimensional Lie groups. Math. Methods Appl. Sci. 2020, 43, 2747–2758. [Google Scholar] [CrossRef]


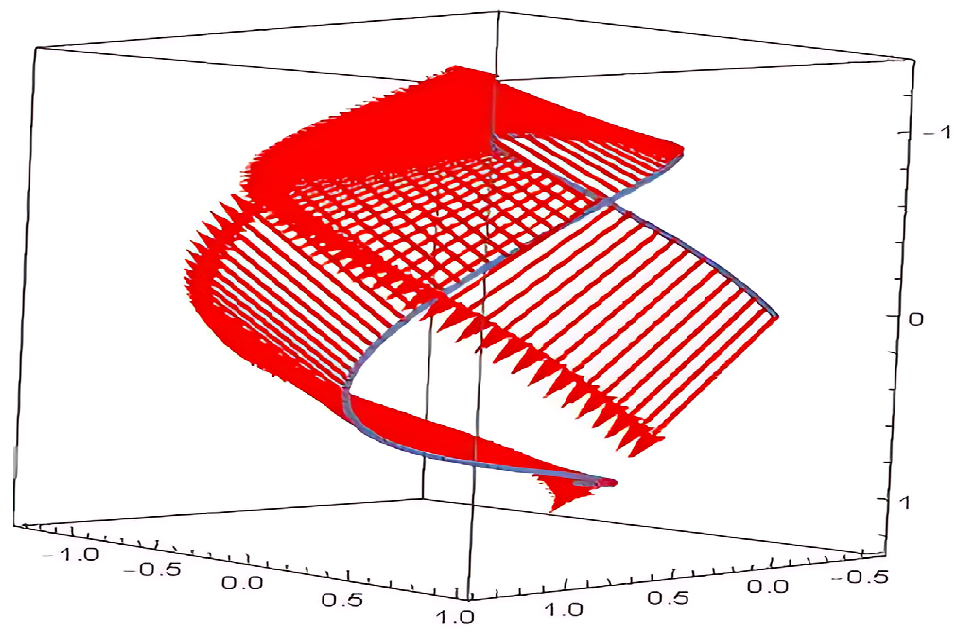
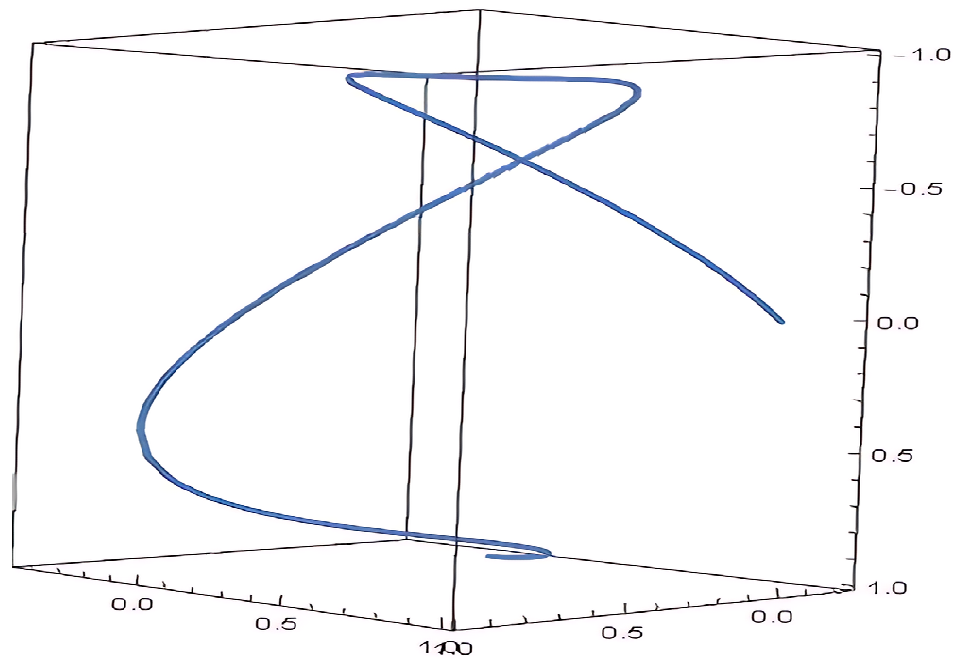
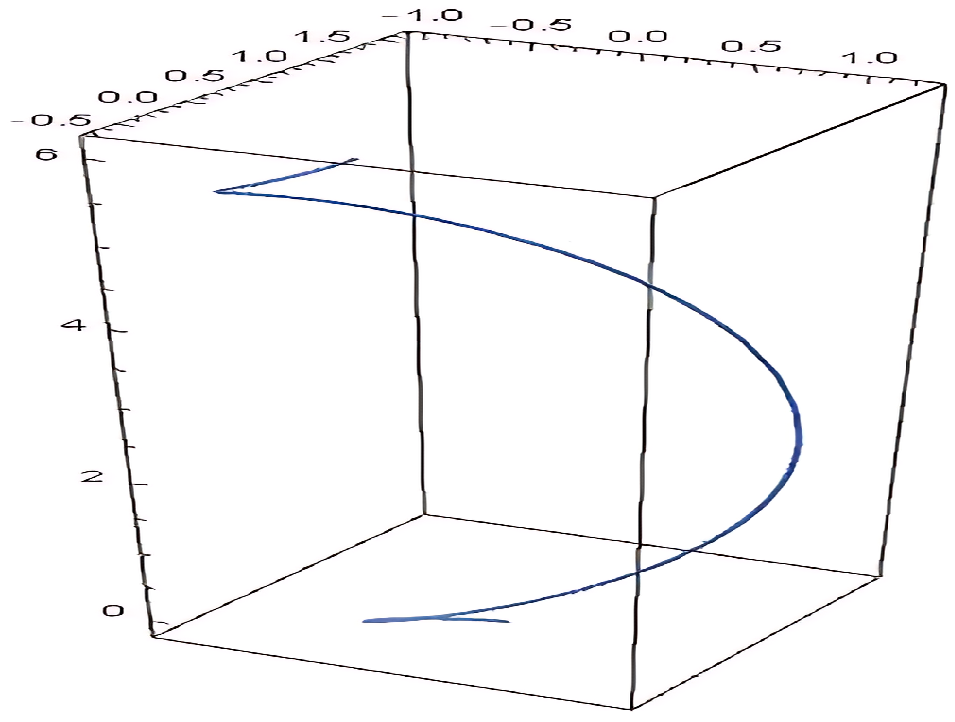
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sariaydin, M.T. A Conjugate Linearly Polarized Light Wave Along an Optical Fiber with the Berry Phase Model and Its Magnetic Trajectories According to the Conjugate Frame. Symmetry 2024, 16, 1518. https://doi.org/10.3390/sym16111518
Sariaydin MT. A Conjugate Linearly Polarized Light Wave Along an Optical Fiber with the Berry Phase Model and Its Magnetic Trajectories According to the Conjugate Frame. Symmetry. 2024; 16(11):1518. https://doi.org/10.3390/sym16111518
Chicago/Turabian StyleSariaydin, Muhammed Talat. 2024. "A Conjugate Linearly Polarized Light Wave Along an Optical Fiber with the Berry Phase Model and Its Magnetic Trajectories According to the Conjugate Frame" Symmetry 16, no. 11: 1518. https://doi.org/10.3390/sym16111518
APA StyleSariaydin, M. T. (2024). A Conjugate Linearly Polarized Light Wave Along an Optical Fiber with the Berry Phase Model and Its Magnetic Trajectories According to the Conjugate Frame. Symmetry, 16(11), 1518. https://doi.org/10.3390/sym16111518





