Review of Chaotic Intermittency
Abstract
1. Introduction
2. Types of Chaotic Intermittency
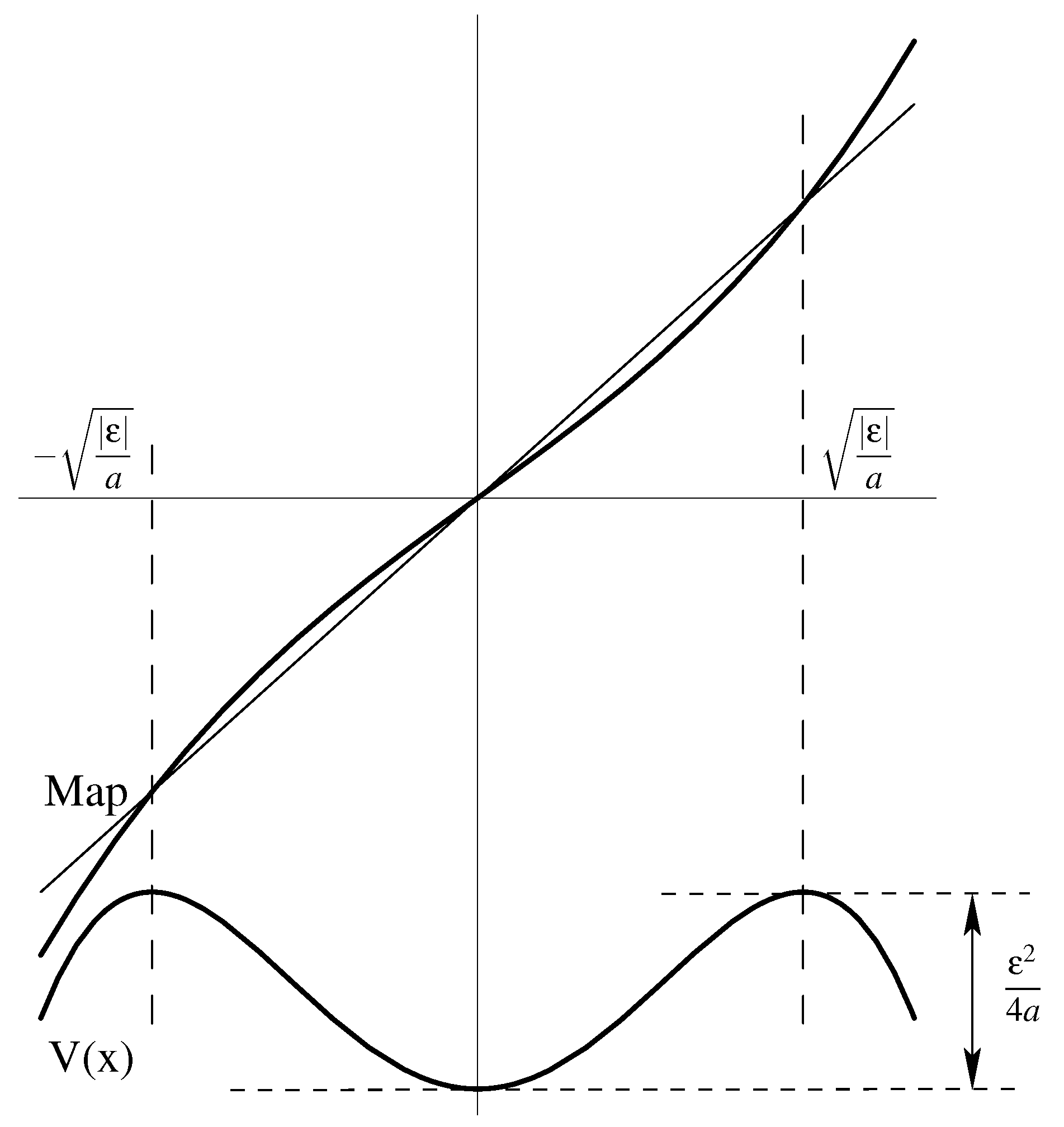
2.1. Type-I Intermittency
2.2. Type-III Intermittency
- . There are three fixed points: and . When loses its stability, the other two fixed points are stable and attract the trajectories. There is a supercritical pitchfork bifurcation, and intermittency does not occur.
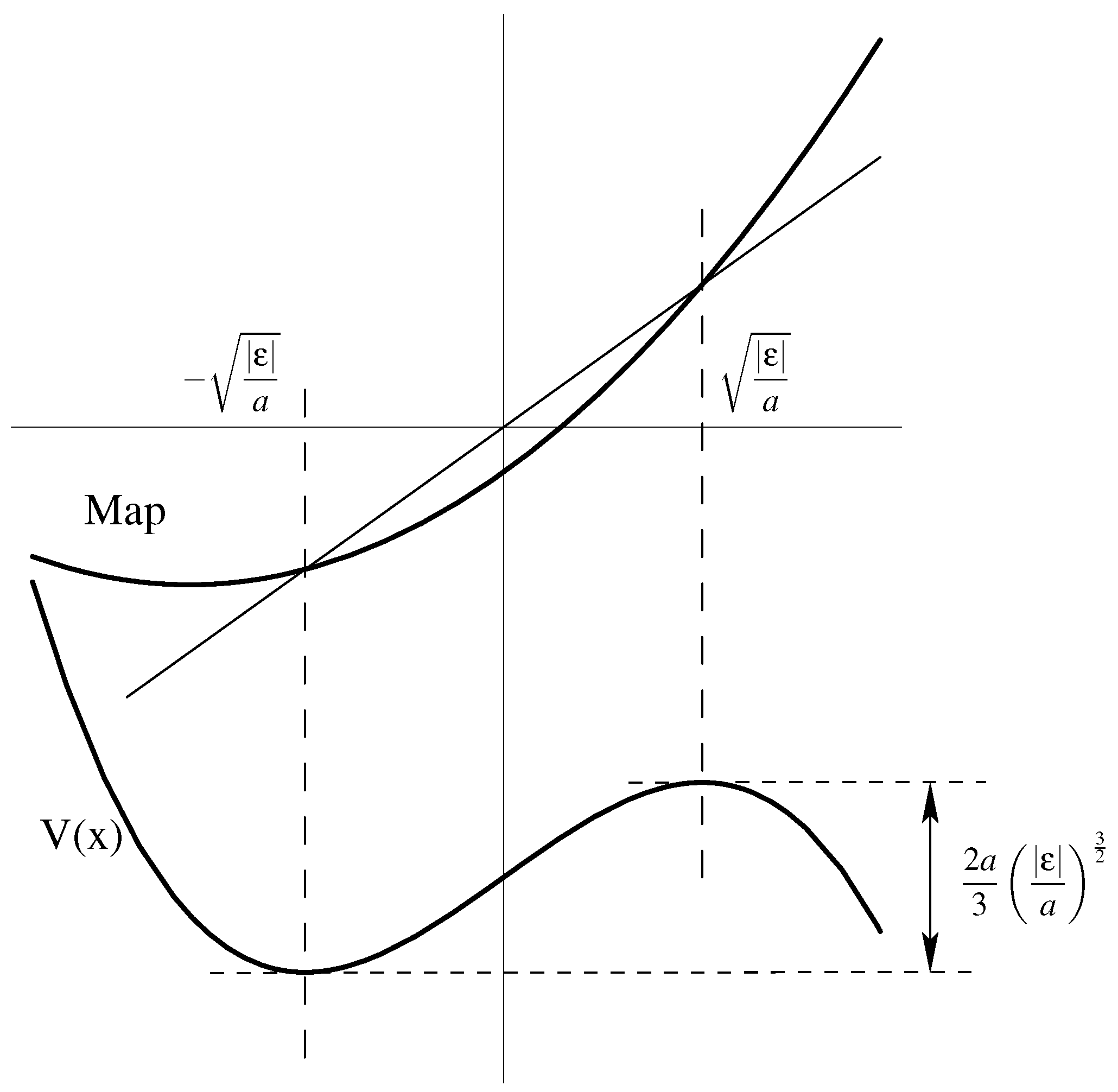
- . For , is the only real fixed point, which is unstable. If there is a reinjection mechanism, type-III intermittency occurs. It is related to a sub-critical pitchfork bifurcation of , or associated with a sub-critical period-doubling bifurcation of . Note that implies . For this reason, several studies utilize Equation (14) as a local map instead of Equations (12) or (13).
2.3. Type-II Intermittency
2.4. Type-V Intermittency
2.5. Type-X Intermittency
2.6. On–Off Intermittency
2.7. Eyelet Intermittency
2.8. Spatiotemporal Intermittency
2.9. Crisis-Induced Intermittency
2.10. Fine Structure in Intermittency
2.11. Two-Dimensional Intermittency
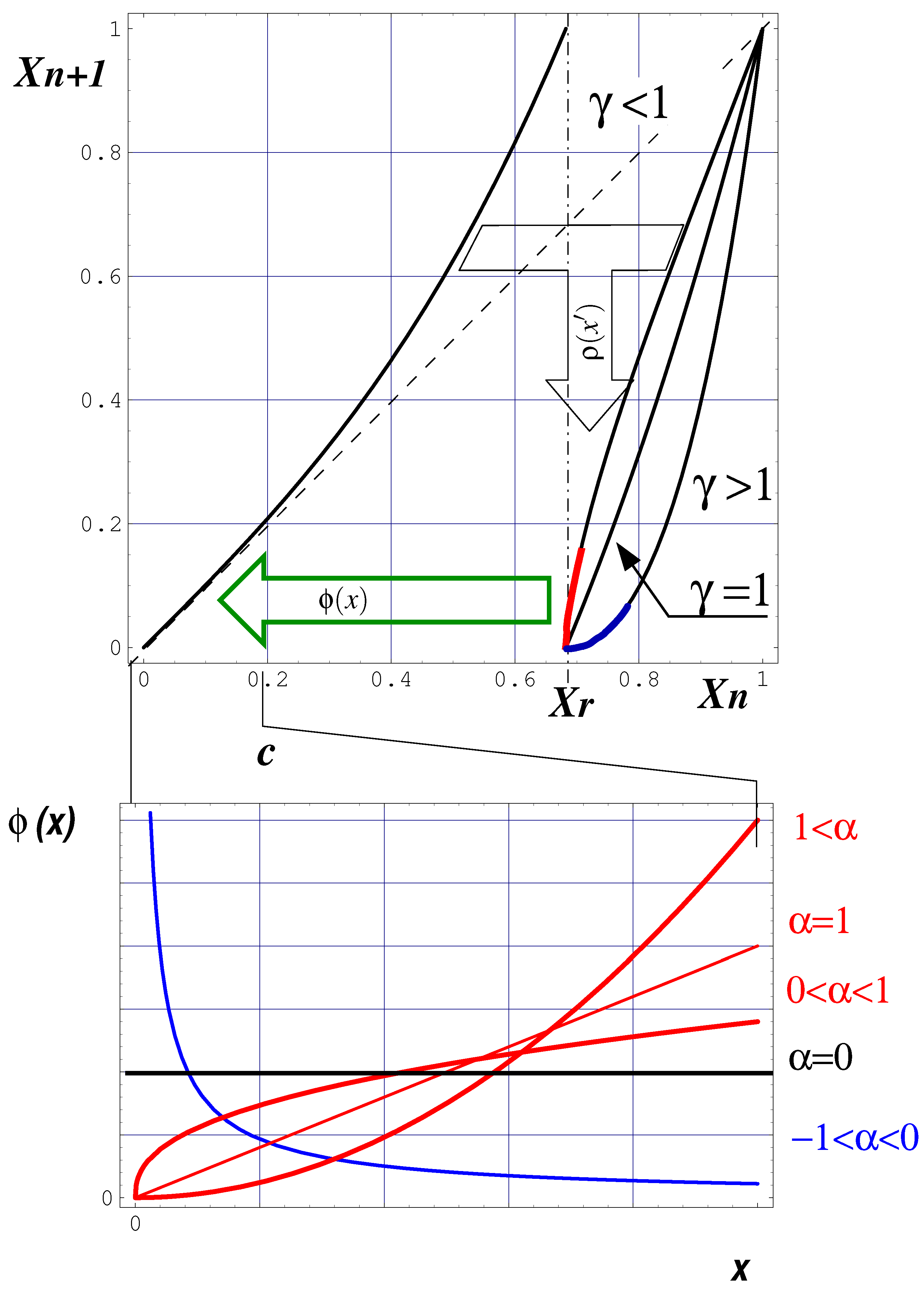
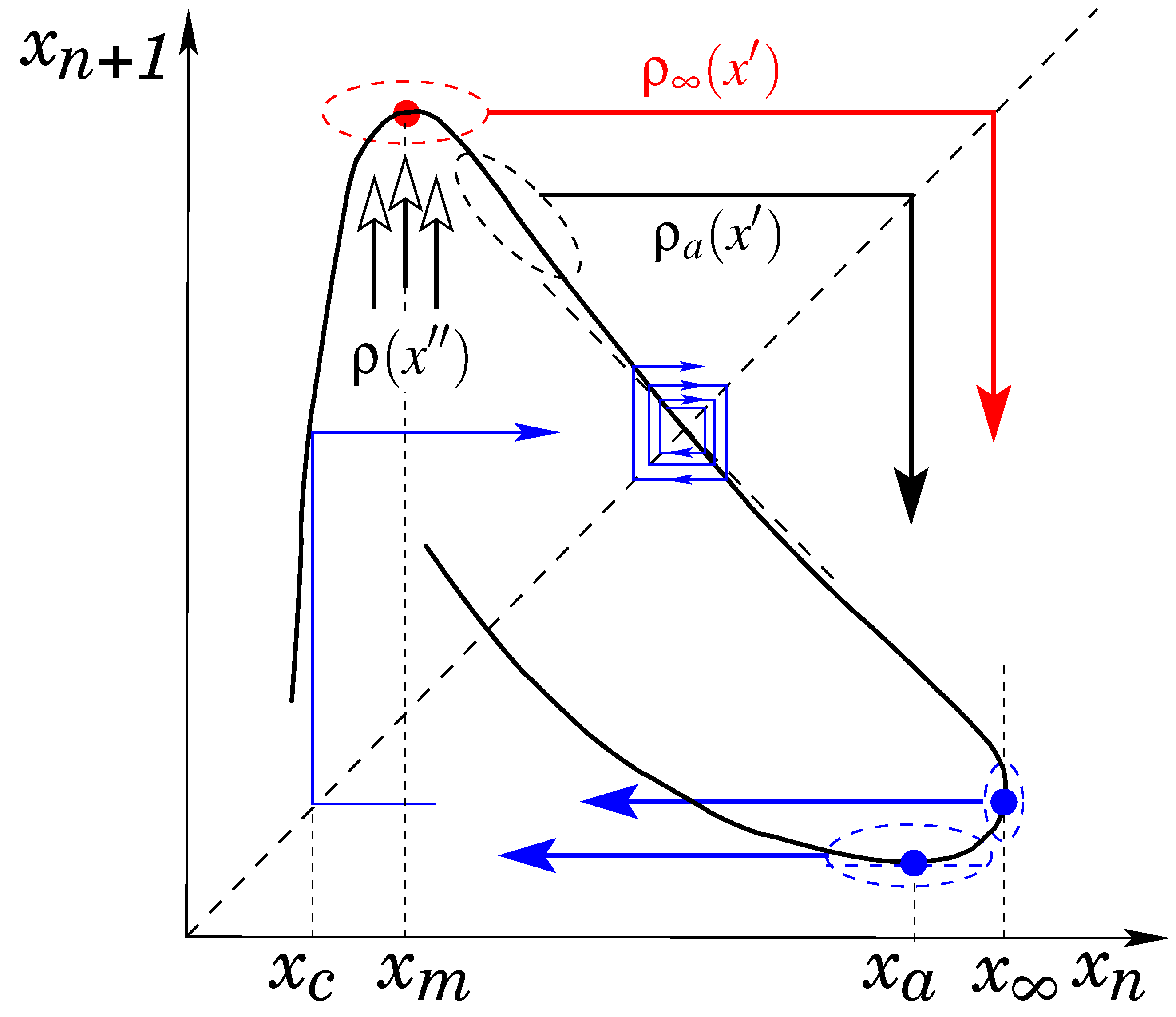
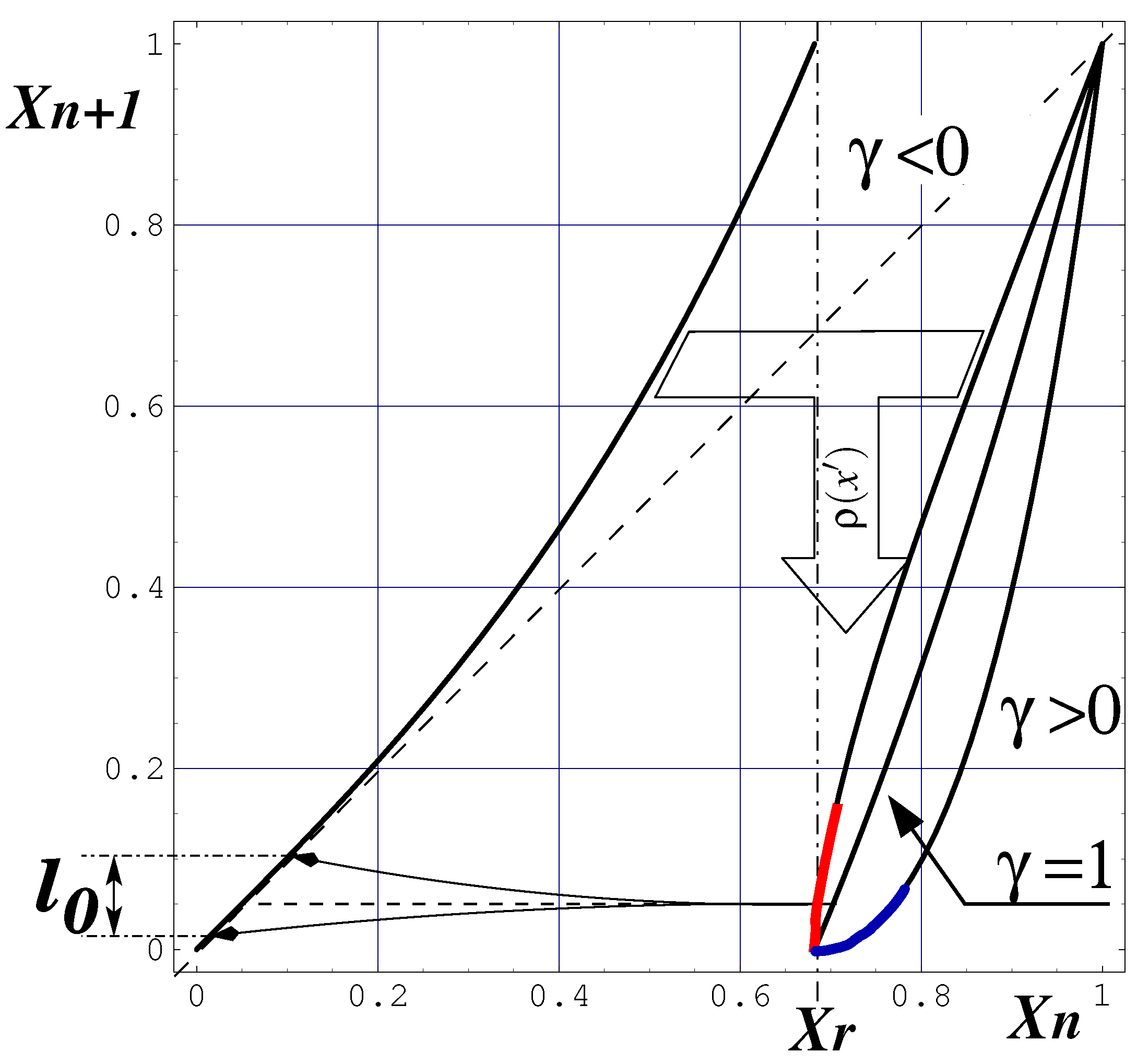
3. New Formulation of the Chaotic Intermittency
3.1. The Reinjection Probability Density Function (RPD)
3.1.1. RPD from Data Series
3.1.2. RPD from the Analytical Definition of the Map
3.2. Type-II Intermittency
3.2.1. Length of Laminar Phase
3.2.2. Characteristic Relations
- (i)
- (ii)
- is bounded.
- Case A:
- −
- A1: or equivalent .
- −
- A2: or equivalent . We have, in this case:
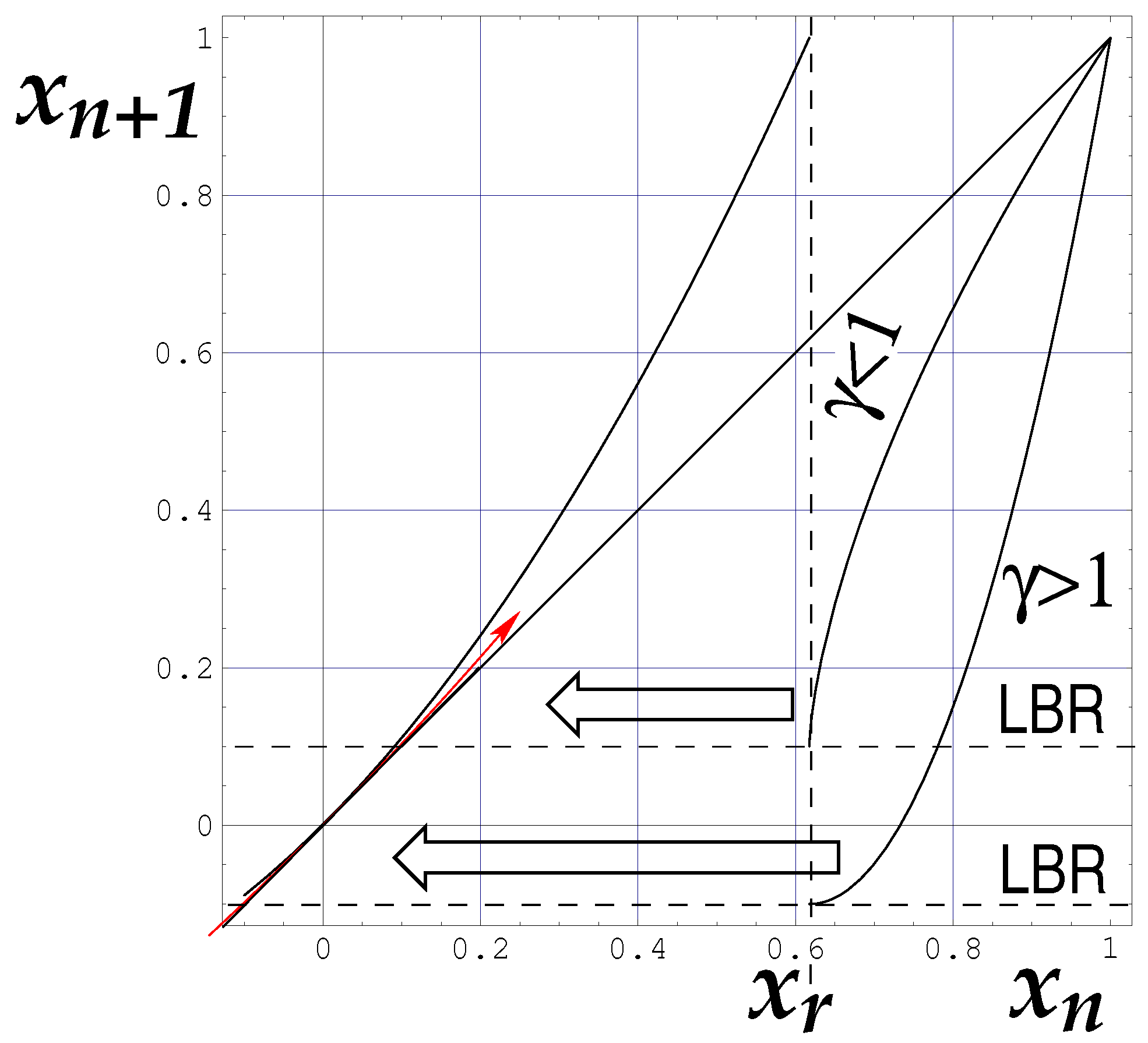
- Case B: . There is an upper cut-off for l. In this case, with the limit , the value of practically remains constant, hence:
- Case C: .as in the uniform reinjection.
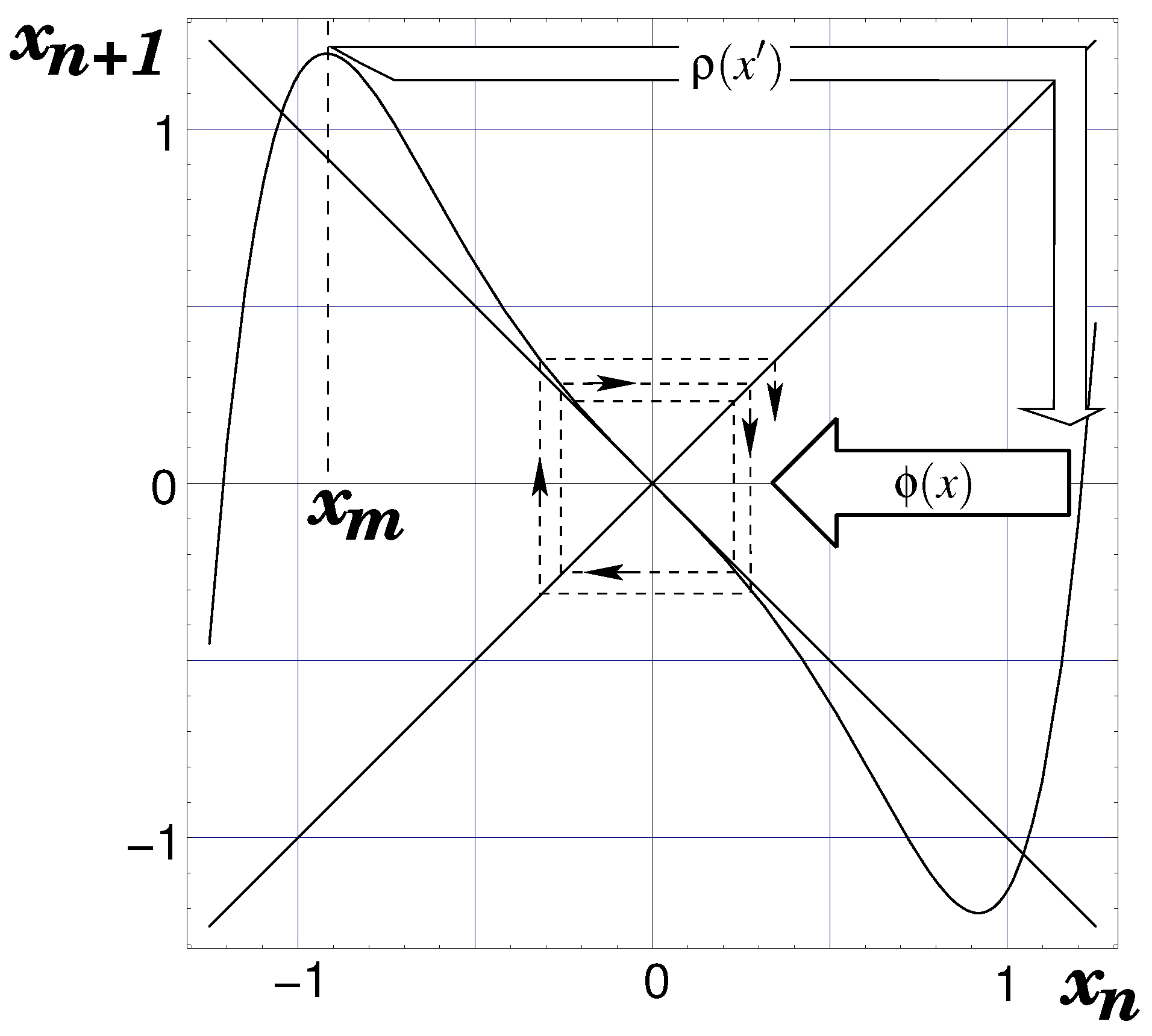
3.3. Type-III Intermittency
Length of Laminar Phase
3.4. Type-I Intermittency
3.4.1. Length of Laminar Phase
3.4.2. Characteristic Relations
3.5. Remarks on the Characteristic Exponent
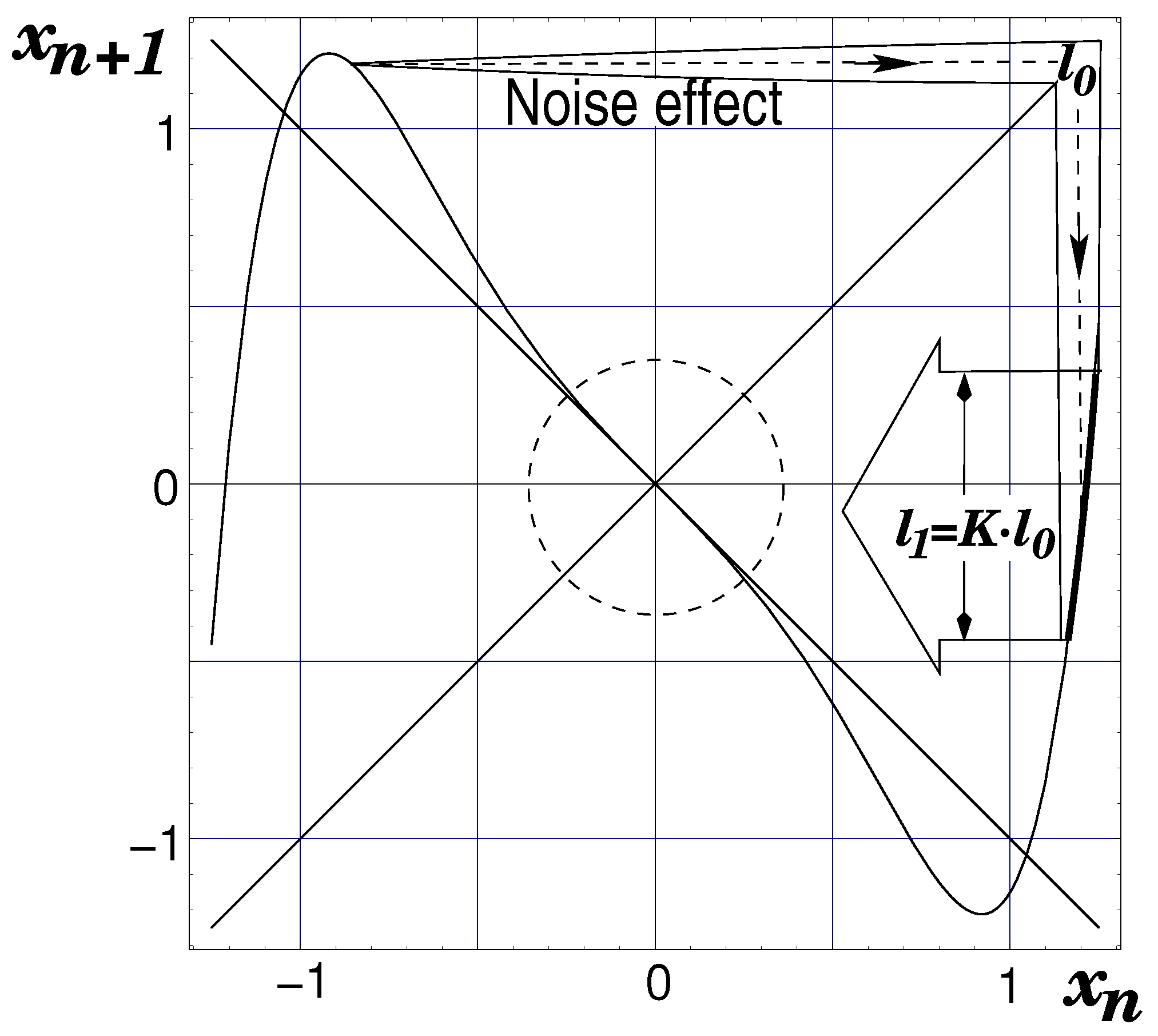
4. Classical Theory about Noise Effects in Chaotic Intermittency
4.1. Noise Effect —Fokker–Plank Approach
4.2. Type-I Intermittency
4.3. Type-II and III Intermittency
4.4. Renormalization Group and Scaling Theory
4.5. Exact Solution for Renormalization Group Equation
5. New Formulation of the Noise Effects in Chaotic Intermittency
5.1. Noisy Reinjection Probability Density Function (NRPD)
5.2. Noise Effect on Type-II Intermittency
5.3. Noise Effect on Type-III Intermittency
5.4. Noise Effect in Type-I Intermittency
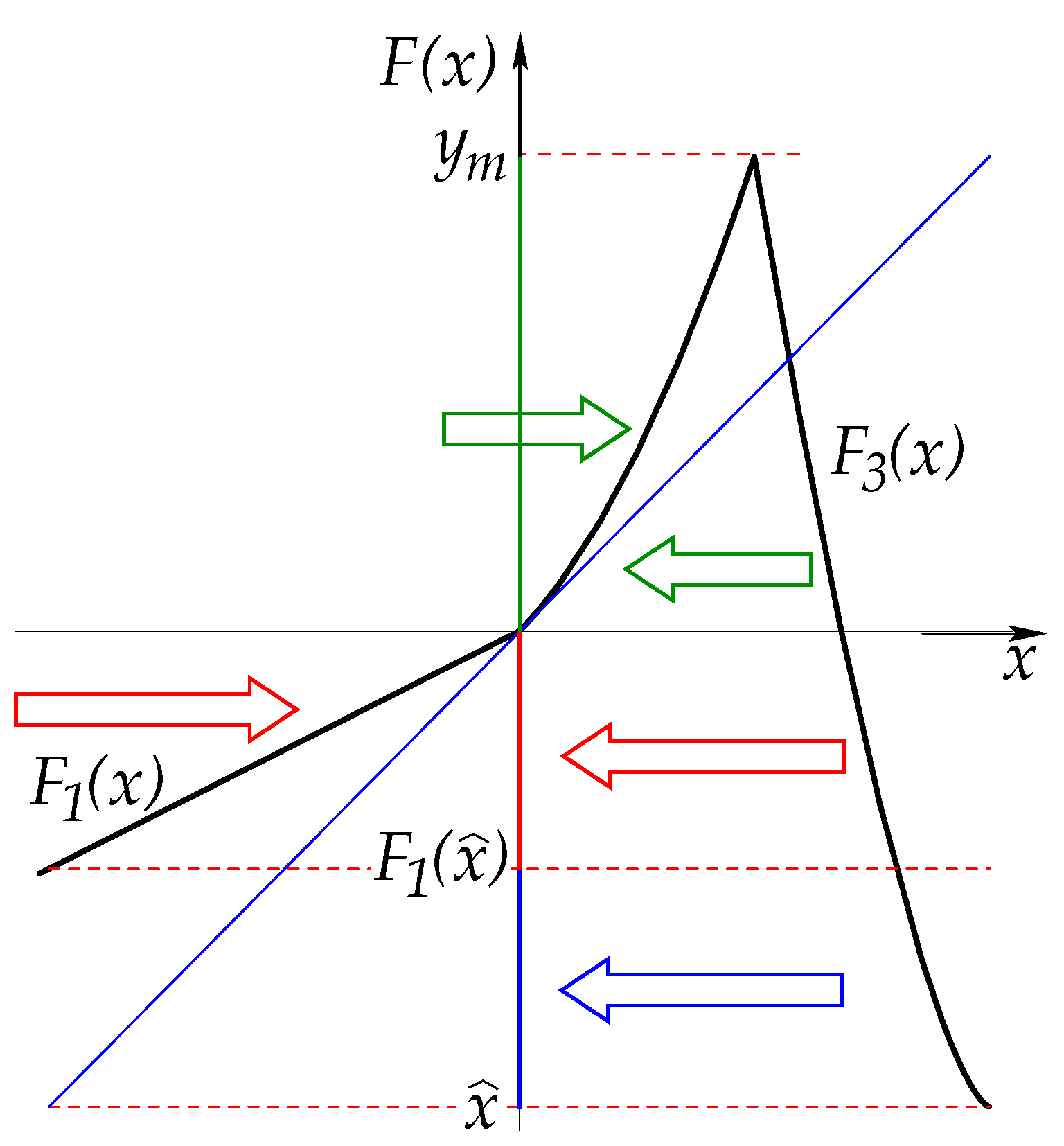
6. Statistical Properties of Intermittency Using the Perron–Frobenius Operator
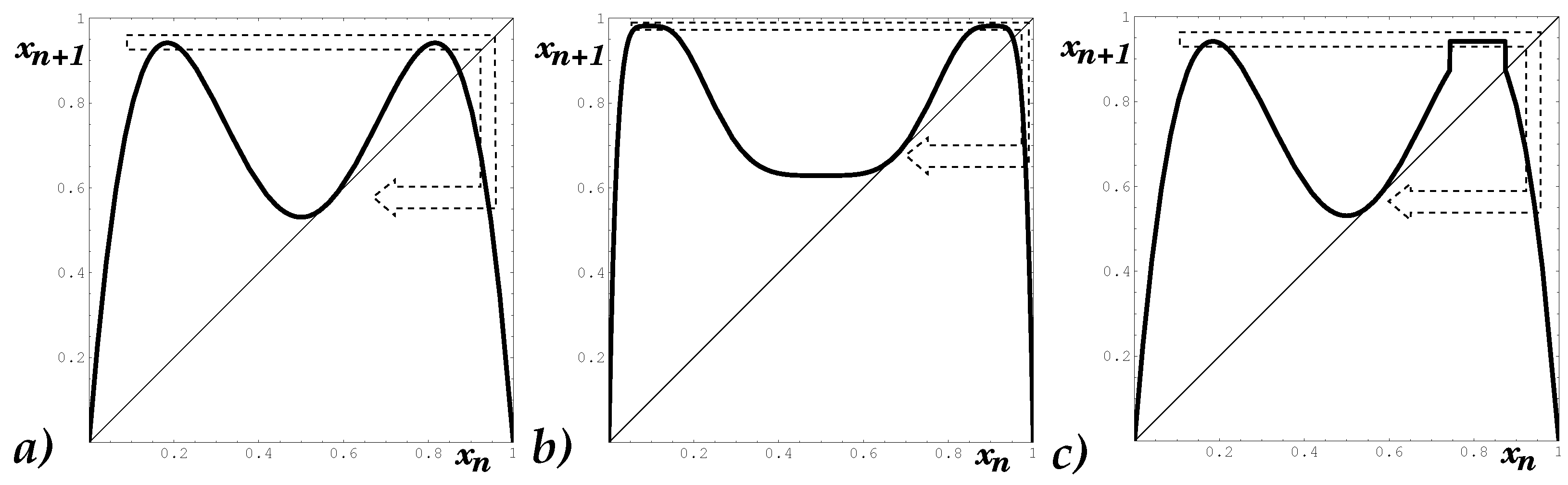
6.1. Piecewise Monotonic Map with Three Subintervals
6.2. Map with Nonlinear Function
6.3. Type-V Intermittency
6.3.1. Continuous RPD
6.3.2. Discontinuous RPD
7. Discussion and Conclusions
- To include noise in the mathematical model introduced in Section 6.
- To analyze the noisy theory due to the discrepancies between the experimental data in electronic circuits and the analytical results.
- To study the density evolution in maps with derivatives equal to zero or tending to infinity.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CDF | Channel Distribution Function |
| DHS | d-Dimensional Diagonal Hyper Surface |
| FPE | Backward Fokker–Plank Equation |
| LBR | Lower Boundary of Reinjection |
| MFPT | Mean First-Passage Time |
| NRPD | Noisy Reinjection Probability Density function |
| RGT | Renormalization Group Theory |
| RPD | Reinjection Probability Density function (form the chaotic region into the laminar one) |
References
- Batchelor, G.; Townsend, C. The nature of turbulent motion at large wave-number. Proc. R. Soc. London Ser. A 1949, 199, 238–255. [Google Scholar]
- Irimiciuc, S.; Saviuc, A.; Tudose-Sandu-Ville, F.; Toma, S.; Nedeff, F.; Marcela Rusu, C.; Agop, M. Non-Linear Behaviors of Transient Periodic Plasma Dynamics in a Multifractal Paradigm. Symmetry 2020, 12, 1356. [Google Scholar] [CrossRef]
- Chertovskih, R.; Rempel, E; Chimanski, E. Magnetic field generation by intermittent convection. Phys. Lett. 2017, 381, 3300–3306. [Google Scholar] [CrossRef]
- Belyaev, I.; Biryukov, D.; Gerasimov, D.; Yurin, E. On-off intermittency and hard turbulence in the flow of fluid in the magnetic field. Chaos Interdiscip. J. Nonlinear Sci. 2019, 29, 083119. [Google Scholar] [CrossRef] [PubMed]
- Goldman, M. Plasma Wave Turbulence and Electromagnetic Radiation Caused by Electron Beams; Grant 84-0007. AFOSR-TR-86-2062; Air Office Scientific Research: Arlington, Virginia, 1986. [Google Scholar]
- Schmiegel, J.; Pons, F. Stochastic Intermittency Fields in a von Kármán Experiment. Symmetry 2021, 13, 1752. [Google Scholar] [CrossRef]
- Manasseh, R. Breakdown regimes of inertia waves in a precessing cylinder. J. Fluid Mech. 1992, 243, 261–296. [Google Scholar] [CrossRef]
- Loiseau, J.; Robinet, J.; Leriche, E. Intermittency and transition to chaos in the cubical lid-driven cavity flow. Fluid Dyn. Res. 2016, 48, 061421. [Google Scholar] [CrossRef]
- Gao, J.; Zheng, Z.; Ma, J. Controlling turbulence via target waves generated by local phase space compression. Int. J. Mod. Phys. B 2008, 22, 3855–3863. [Google Scholar] [CrossRef]
- Malm, A.; Waigh, T. Elastic turbulence in entangled semi-dilute DNA solutions measured with optical coherence tomography velocimetry. Sci. Rep. 2017, 7, 1186. [Google Scholar] [CrossRef]
- Malasoma, J.; Werny, P.; Boiron, M. Multichannel type-I intermittency in two models of Rayleigh-Benard convection. Phys. Rev. Lett. 2004, 51, 487–500. [Google Scholar] [CrossRef]
- Stavrinides, S.; Miliou, A.; Laopoulos, T.; Anagnostopoulos, A. The intermittency route to chaos of an electronic digital oscillator. Int. J. Bifurc. Chaos 2008, 18, 1561–1566. [Google Scholar] [CrossRef]
- Elaskar, S.; del Rio, E.; Elaskar, S. Intermittency Reinjection in the Logistic Map. Symmetry 2022, 14, 281. [Google Scholar] [CrossRef]
- Sanmartín, J.; López-Rebollal, O.; del Río, E.; Elaskar, S. Hard transition to chaotic dynamics in Alfven wave-fronts. Phys. Plasmas 2004, 11, 2026–2035. [Google Scholar] [CrossRef]
- Sánchez-Arriaga, G.; Sanmartín, J.; Elaskar, S. Damping models in the truncated derivative nonlinear Schrödinger equation. Phys. Plasmas 2007, 14, 082108. [Google Scholar] [CrossRef]
- Pizza, G.; Frouzakis, C.; Mantzaras, J. Chaotic dynamics in premixed Hydrogen/air channel flow combustion. Combust. Theor. Model 2012, 16, 275–299. [Google Scholar] [CrossRef]
- Manneville, P.; Pomeau, Y. Intermittency and Lorenz model. Phys. Lett. A 1979, 75, 1–2. [Google Scholar] [CrossRef]
- Casagrande, V.; Mikhailov, A. Birhythmicity, synchronization, and turbulence in an oscillatory system with nonlocal inertial coupling. Phys. D Nonlinear Phenom. 2005, 205, 154–169. [Google Scholar] [CrossRef]
- Saha, A.; Feudel, U. Characteristics of in-out intermittency in delay-coupled FitzHugh–Nagumo oscillators. Eur. Phys. J. Spec. Top. 2018, 227, 1205–1219. [Google Scholar] [CrossRef]
- Gil, S.; Mikhailov, A. Networks on the edge of chaos: Global feedback control of turbulence in oscillator networks. Phys. Rev. E 2009, 79, 026219. [Google Scholar] [CrossRef]
- Hu, B.; Zheng, Z. Phase synchronizations: Transitions from high-to low-dimensional tori through chaos. Int. J. Bifurc. Chaos 2000, 10, 2399–2414. [Google Scholar] [CrossRef]
- Elnashaie, S.; Abashar, M.; Teymour, F. Bifurcation, instability and chaos in fluidized bed catalytic reactors with consecutive exothermic chemical reactions. Chaos Solitons Fractals 1993, 3, 1–33. [Google Scholar] [CrossRef]
- Li, H.-H.; Xiao, J.-H.; Hu, G.; Hu, B. Intermittencies in complex Ginzburg–Landau equation by varying system size. Chin. Phys. B 2010, 19, 050516. [Google Scholar]
- Serre, T.; Nesme-Ribes, E. Nonlinear analysis of solar cycles. Astron. Astrophys. 2000, 360, 319–330. [Google Scholar]
- Coulibaly, S.; Clerc, M.; Selmi, F.; Barbay, S. Extreme events following bifurcation to spatiotemporal chaos in a spatially extended microcavity laser. Phys. Rev. A 2017, 95, 023816. [Google Scholar] [CrossRef]
- Pavlos, G.; Iliopoulos, A.; Tsoutsouras, V.; Karakatsanis, L.; Pavlos, E. Spatiotemporal chaos in distributed systems: Theory and practice. In Chaotic Systems: Theory and Applications; World Scientific: Singapore, 2010; pp. 268–283. [Google Scholar]
- Sujith, R.; Unni, V. Complex system approach to investigate and mitigate thermoacoustic instability in turbulent combustors. Phys. Fluids 2020, 32, 061401. [Google Scholar] [CrossRef]
- Zambrano, S.; Mariño, I.P.; Sanjuán, M. Controlling crisis-induced intermittency using its relation with a boundary crisis. New J. Phys. 2009, 11, 023025. [Google Scholar] [CrossRef]
- Chian, A. Complex System Approach to Economic Dynamics. Lecture Notes in Economics and Mathematical Systems; Springer: Berlin, Germany, 2007. [Google Scholar]
- Bhansali, R.; Holland, M.; Kokoszka, P. Intermittency, long-memory and financial returns. In Long Memory in Economics; Springer: Berlin, Germany, 2007; pp. 39–68. [Google Scholar]
- Zebrowski, J.; Baranowski, R. Type-I intermittency in nonstationary systems: Models and human heart-rate variability. Physics A 2004, 336, 74–86. [Google Scholar] [CrossRef]
- Velazquez, J.; Khosravani, H.; Lozano, A.; Bardakjian, B.; Carlen, P.; Wennberg, R. Type III intermittency in human partial epilepsy. Eur. J. Neurosci. 1999, 11, 2571–2576. [Google Scholar] [CrossRef]
- Paradisi, P.; Allegrini, P.; Gemignani, A.; Laurino, M.; Menicucci, D.; Piarulli, A. Scaling and intermittency of brains events as a manifestation of consciousness. AIP Conf. Proc. 2012, 1510, 151–161. [Google Scholar]
- Bashkirtseva, I.; Nasyrova, V.; Ryashko, L. Scaling and intermittency of brains events as a manifestation of consciousness. Chaos Solitons Fractals 2018, 110, 76–81. [Google Scholar] [CrossRef]
- Suzuki, Y.; Lu, M.; Ben-Jacob, E.; Onuchic, J. Periodic, quasi-periodic and chaotic dynamics in simple gene elements with time delays. Sci. Rep. 2016, 6, 21037. [Google Scholar] [CrossRef] [PubMed]
- Gardiner, J.; Atema, J. The function of bilateral odor arrival time differences in olfactory orientation of sharks. Curr. Biol. 2010, 20, 1187–1191. [Google Scholar] [CrossRef] [PubMed]
- Atema, J.; Brönmark, C.; Hansson, L. Aquatic odor dispersal fields: Opportunities and limits of detection, communication and navigation. In Chemical Ecology in Aquatic Systems; Oxford University Press: New York, NY, USA, 2012; pp. 1–18. [Google Scholar]
- Nayfeh, A.; Balachandran, B. Applied Nonlinear Dynamics; Wiley: New York, NY, USA, 1995. [Google Scholar]
- Elaskar, S.; del Rio, E. New Advances on Chaotic Intermittency and Applications; Springer: New York, NY, USA, 2017; ISBN 978–3-319-47836-4. [Google Scholar]
- Manneville, P. Intermittency, self-similarity and 1/f spectrum in dissipative dynamical systems. J. Phys. 1980, 41, 1235–1243. [Google Scholar] [CrossRef]
- Hirsch, J.; Huberman, B.; Scalapino, D. Theory of intermittency. Phys. Rev. Lett. 1982, 25, 519–532. [Google Scholar] [CrossRef]
- Schuster, H.; Just, W. Deterministic Chaos; Wiley VCH: Mörlenbach, Germany, 2005. [Google Scholar]
- Kaplan, H. Return to type-I intermittency. Phys. Rev. Lett. 1992, 68, 553–557. [Google Scholar] [CrossRef] [PubMed]
- Price, T.; Mullin, P. An experimental observation of a new type of intermittency. Phys. D 1991, 48, 29–52. [Google Scholar] [CrossRef]
- Platt, N.; Spiegel, E.; Tresser, C. On-off intermittency: A mechanism for bursting. Phys. Rev. Lett. 1993, 70, 279–282. [Google Scholar] [CrossRef]
- Pikovsky, A.; Osipov, G.; Rosenblum, M.; Zaks, M.; Kurths, J. Attractor–repeller collision and eyelet intermittency at the transition to phase synchronization. Phys. Rev. Lett. 1997, 79, 47–50. [Google Scholar] [CrossRef]
- Lee, K.; Kwak, Y.; Lim, T. Phase jumps near a phase synchronization transition in systems of two coupled chaotic oscillators. Phys. Rev. Lett. 1998, 81, 321–324. [Google Scholar] [CrossRef]
- Hramov, A.; Koronovskii, A.; Kurovskaya, M.; Boccaletti, S. Ring intermittency in coupled chaotic oscillators at the boundary of phase synchronization. Phys. Rev. Lett. 2006, 97, 114101. [Google Scholar] [CrossRef]
- Guckenheimer, J.; Holmes, P. Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Field; Springer: New York, NY, USA, 1983. [Google Scholar]
- Bai-lin, H. Elementary Simbolic Dynamics and Chaos in Dissipative Systems; World Scientific: Singapure, 1989. [Google Scholar]
- Wiggins, S. Introduction to Applied Nonlinear Dynamical Systems and Chaos; Springer: New York, NY, USA, 2003. [Google Scholar]
- Laugesen, J.; Carlsson, N.; Mosckilde, E.; Bountis, T. Anomalous statistics for type-III intermittency. Open Syst. Inf. Dyn. 1998, 4, 393–405. [Google Scholar] [CrossRef]
- Marek, M.; Schreiber, I. Chaotic Behaviour of Deterministic Dissipative Systems; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Kye, W.; Kim, C. Characteristic relations of type-I intermittency in presence of noise. Phys. Rev. E 2000, 62, 6304–6307. [Google Scholar] [CrossRef] [PubMed]
- Kye, W.; Rim, S.; Kim, C.; Lee, J.; Ryu, J.; Yeom, B.; Park, Y. Experimental observation of characteristic relations of type-III intermittency in the presence of noise in a simple electronic circuit. Phys. Rev. E 2003, 68, 036203. [Google Scholar] [CrossRef]
- del Rio, E.; Velarde, M.; Rodríguez-Lozano, A. Long time data series and difficulties with the characterization of chaotic attractors: A case with intermittency III. Chaos Solitons Fractals 1994, 4, 2169–2179. [Google Scholar] [CrossRef]
- del Rio, E.; Elaskar, S. New characteristic relation in type-II intermittency. Int. J. Bifurc. Chaos 2010, 20, 1185–1191. [Google Scholar] [CrossRef]
- Elaskar, S.; del Rio, E.; Donoso, J. Reinjection probability density in type-III intermittency. Phys. A 2011, 390, 2759–2768. [Google Scholar] [CrossRef]
- del Rio, E.; Elaskar, S.; Makarov, S. Theory of intermittency applied to classical pathological cases. Chaos 2013, 23, 033112. [Google Scholar] [CrossRef]
- del Rio, E.; Elaskar, S.; Donoso, J. Laminar length and characteristic relation in type-I intermittency. Commun. Numer. Simul. Nonlinear Sci. 2014, 19, 967–976. [Google Scholar] [CrossRef]
- Krause, G.; Elaskar, S.; del Rio, E. Type-I intermittency with discontinuous reinjection probability density in a truncation model of the derivative nonlinear Schrödinger equation. Nonlinear Dyn. 2014, 77, 455–466. [Google Scholar] [CrossRef]
- Krause, G.; Elaskar, S.; del Rio, E. Noise effect on statistical properties of type-I intermittency. Phys. A 2014, 402, 318–329. [Google Scholar] [CrossRef]
- Elaskar, S.; del Rio, E.; Krause, G.; Costa, A. Effect of the lower boundary of reinjection and noise in type-II intermittency. Nonlinear Dyn. 2015, 79, 1411–1424. [Google Scholar] [CrossRef]
- Elaskar, S.; del Rio, E.; Grioni, M. Chaotic intermittency with non-differentiable M(x) function. REDIM Rev. Fac. Ing. 2023, in press. [Google Scholar] [CrossRef]
- Elaskar, S.; del Rio, E.; Zapico, E. Evaluation of the statistical properties for type-II intermittency using the Perron-Frobenius operator. Nonlinear Dyn. 2016, 86, 1107–1116. [Google Scholar] [CrossRef]
- Elaskar, S.; del Rio, E.; Schulz, W. Analysis of the Type V Intermittency Using the Perron-Frobenius Operator. Symmetry 2022, 14, 2519. [Google Scholar] [CrossRef]
- Elaskar, S.; del Rio, E.; Lorenzón, D. Calculation of the Statistical Properties in Intermittency Using the Natural Invariant Density. Symmetry 2021, 13, 935. [Google Scholar] [CrossRef]
- del Rio, E.; Sanjuan, M.; Elaskar, S. Effect of noise on the reinjection probability density in intermittency. Commun. Numer. Simul. Nonlinear Sci. 2012, 17, 3587–3596. [Google Scholar] [CrossRef]
- Elaskar, S.; del Rio, E.; Costa, A. Reinjection probability density for type-III intermittency with noise and lower boundary of reinjection. J. Comput. Nonlinear Dyn. ASME 2017, 12, 031020. [Google Scholar] [CrossRef]
- Elaskar, S.; del Rio, E.; Gutiérrez Marcantoni, L. Non-uniform reinjection probability density function in type V intermittency. Nonlinear Dyn. 2018, 92, 683697. [Google Scholar] [CrossRef]
- Arnold, V. Geometrical Methods in the Theory of Differential Equations; Springer: Berlin, Germany, 1988. [Google Scholar]
- Rasband, S. Chaotic Dynamics of Nonlinear Dynamics; John Wiley & Sons: New York, NY, USA, 1990. [Google Scholar]
- Bauer, M.; Habip, S.; He, D.; Martiessen, W. New type of intermittency in discontinuous maps. Phys. Rev. Lett. 1992, 68, 1625–1628. [Google Scholar] [CrossRef]
- He, D.; Bauer, M.; Habip, S.; Kruger, U.; Martiessen, W.; Christiansen, B.; Wang, B. Type V intermittency. Phys. Lett. A 1992, 171, 61–65. [Google Scholar] [CrossRef]
- Fan, J.; Ji, F.; Guan, S.; Wang, B.; He, D. The distribution of laminar lenghts in type V intermittency. Phys. Lett. A 1993, 182, 232–237. [Google Scholar] [CrossRef]
- Heagy, J.; Platt, N.; Hammel, S. Characterization of on-off intermittency. Phys. Rev. E 1994, 49, 1140–1150. [Google Scholar] [CrossRef] [PubMed]
- Pisarchik, A.N.; Feudel, U. Control of multistability. Phys. Rep. 2014, 540, 167–218. [Google Scholar] [CrossRef]
- Pisarchik, A.N.; Jaimes-Reátegui, R.; Villalobos-Salazar, J.R.; Garcia-Lopez, J.H.; Boccaletti, S. Synchronization of chaotic systems with coexisting attractors. Phys. Rev. Lett. 2006, 96, 244102. [Google Scholar] [CrossRef] [PubMed]
- Reategui, R.J.; Pisarchik, A.N. Control of on-off intermittency by slow parametric modulation. Phys. Rev. E 2004, 69, 067203. [Google Scholar] [CrossRef]
- Kurovskaya, M. Distribution of laminar phases at eyelet-type intermittency. Tech. Phys. Lett. 2008, 34, 1063–1065. [Google Scholar] [CrossRef]
- Pikovsky, A.; Rosenblum, M.; Kurths, J. Synchronization—A universal concept in Nonlinear Sciences; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Ott, E. Chaos in Dynamical Systems; Cambridge University Press: Cambridge, UK, 1994. [Google Scholar]
- Osipov, G.; Kurths, J.; Zhou, C. Synchronization in Oscillatory Networks; Springer: Berlin, Germany, 2007. [Google Scholar]
- Hramov, A.; Koronovskii, A.; Kurovskaya, M.; Moskalenko, O. Type-I intermittency with noise versus eyelet intermittency. Phys. Lett. A 2011, 375, 1646–1652. [Google Scholar] [CrossRef]
- Boccaletti, S.; Allaria, E.; Meucci, R.; Arecchi, F. Experimental characterization of the transition to phase synchronization of chaotic CO2 laser systems. Phys. Rev. Lett. 2002, 89, 194101. [Google Scholar] [CrossRef]
- White, F. Viscous Fluid Flow; McGraw-Hill Inc.: New York, NY, USA, 1991. [Google Scholar]
- Pope, S. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Malvern, L. Introduction to the Mechanics of A Continuous Medium; Prentice-Hall Inc.: Hoboken, NJ, USA, 1969. [Google Scholar]
- Aris, R. Vectors, Tensors and the Basic Equations of Fluid Mechanics; Dover Publications: New York, NY, USA, 1962. [Google Scholar]
- Gollubm, J. Order and disorder in fluid motion. Proc. Natl. Acad. Sci. USA 1995, 92, 6705–6711. [Google Scholar] [CrossRef]
- Lepiller, V.; Prigent, A.; Dumochel, F.; Mutabazi, I. Transition to turbulence in a tall annulus submitted to a radial temperature gradient. Phys. Fluids 2007, 19, 054101. [Google Scholar] [CrossRef]
- Lai, Y.; Tei, T. Transient Chaos; Springer: New York, NY, USA, 2001. [Google Scholar]
- Chaté, H.; Manneville, P. Transition to turbulence via spatiotemporal intermittency. Phys. Rev. Lett. 1987, 58, 112–115. [Google Scholar] [CrossRef]
- Kaneko, K. Pattern dynamics in spatiotemporal chaos. Physics D 1989, 34, 1–41. [Google Scholar] [CrossRef]
- Qu, Z.; Hu, G. Spatiotemporal periodic states, periodic windows, and intermittency in coupled-map lattices. Phys. Rev. E 1994, 49, 1099–1108. [Google Scholar]
- Keeler, J.; Farmer, J. Robust sapce-time intermittency and 1/f noise. Phys. D 1986, 23, 413–435. [Google Scholar] [CrossRef]
- Kaneko, K.; Tsuda, I. Complex System, Chaos and Beyond, a Constructive Approach with Applications om Life Sciences; Springer: Berlin, Germany, 2000. [Google Scholar]
- Rupp, P.; Richter, R.; Rehberg, I. Critical exponents of directed percolation measured in spatiotemporal intermittency. Phys. Rev. E 2003, 67, 036209. [Google Scholar] [CrossRef]
- Grebogi, C.; Ott, E.; Yorke, J. Crisis, sudden changes in chaotic attractors and transient chaos. Physics D 1983, 7, 181–200. [Google Scholar] [CrossRef]
- Grebogi, C.; Ott, E.; Romeiras, F.; Yorke, J. Critical exponents for crisis-induced intermittency. Phys. Rev. E 1987, 36, 5365–5380. [Google Scholar] [CrossRef]
- Cavalcante, d.S.H.; Rios Leite, J. Fine structure in scaling of type-I intermittency bifurcation. Physics A 2004, 342, 356–362. [Google Scholar] [CrossRef]
- Cavalcante, d.S.H.; Rios Leite, J. Logarithmic periodicities in the bifurcations of type-I intermittent chaos. Phys. Rev. Lett. 2004, 92, 254102. [Google Scholar] [CrossRef]
- Kim, C.; Kye, W. Two-dimensional intermittency. Phys. Rev. E 2001, 63, 037202. [Google Scholar] [CrossRef] [PubMed]
- Dubois, M.; Rubio, M.; Berge, P. Experimental evidence of intermittencies associated with a subharmonic bifurcation. Phys. Rev. E 1983, 51, 1446–1449. [Google Scholar]
- Pikovsky, A. A new type of intermittent transition to chaos. J. Phys. A 1983, 16, L109–L112. [Google Scholar] [CrossRef]
- Kim, C.; Kwon, O.; Lee, E.; Lee, H. New characteristic relations in type-I intermittency. Phys. Rev. Lett. 1994, 73, 525–528. [Google Scholar] [CrossRef]
- Kim, C.; Yim, G.; Ryu, J.; Park, Y. Characteristic relations of type-III intermittency in an electronic circuit. Phys. Rev. Lett. 1998, 80, 5317–5320. [Google Scholar] [CrossRef]
- Kim, C.; Yim, G.; Kim, Y.; Kim, J.; Lee, H. Experimental evidence of characteristic relations of type-I intermittency in an electronic circuit. Phys. Rev. E 1997, 56, 2573–2577. [Google Scholar] [CrossRef]
- Cho, J.; Ko, M.; Park, Y.; Kim, C. Experimental observation of the characteristic relations of type-I intermittency in the presence of noise. Phys. Rev. E 2002, 65, 036222. [Google Scholar] [CrossRef]
- Ono, Y.; Fukushima, K.; Yazaki, T. Critical behavior for the onset of type-III intermittency observed in an electronic circuit. Phys. Rev. E 1995, 52, 4520–4522. [Google Scholar] [CrossRef]
- del Rio, E.; Elaskar, S. On the theory of intermittency in 1D maps. Int. J. Bifurc. Chaos 2016, 26, 1650228. [Google Scholar] [CrossRef]
- del Rio, E.; Elaskar, S. Experimental evidence of power law reinjection in chaotic intermittency. Commun. Numer. Simul. Nonlinear Sci. 2018, 64, 122–134. [Google Scholar]
- Kwon, O.; Kim, C.; Lee, E.; Lee, H. Effects of reinjection on the scaling property of intermittency. Phys. Rev. E 1996, 53, 1253–1256. [Google Scholar] [CrossRef] [PubMed]
- Lichtenberg, A.; Lieberman, M. Regular and Stochastic Motion; Springer: New York, NY, USA, 1983. [Google Scholar]
- del Rio, E.; Elaskar, S. Type III intermittency without characteristic relation. Chaos 2021, 31, 043127. [Google Scholar] [CrossRef] [PubMed]
- Abramowitz, M.; Stegun, I. Handbook of Mathematical Functions; Dover: New York, NY, USA, 1970. [Google Scholar]
- Hirsch, J.; Nauenberg, M.; Scalapino, D. Intermittency in presence of noise: A renormalization group formulation. Phys. Lett. A 1982, 87, 391–393. [Google Scholar] [CrossRef]
- Kodama, H.; Sato, S.; Honda, K. Reconsideration of the renormalization-group theory on intermittent chaos. Phys. Lett. A 1991, 157, 354–356. [Google Scholar] [CrossRef]
- Lasota, A.; Mackey, M. Chaos, Fractals, and Noise: Stochastic Aspects of Dynamics; Springer Science & Business Media: New York, NY, USA, 1998; Volume 97. [Google Scholar]
- Beck, C.; Schogl, F. Thermodynamics of Chaotic Systems; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
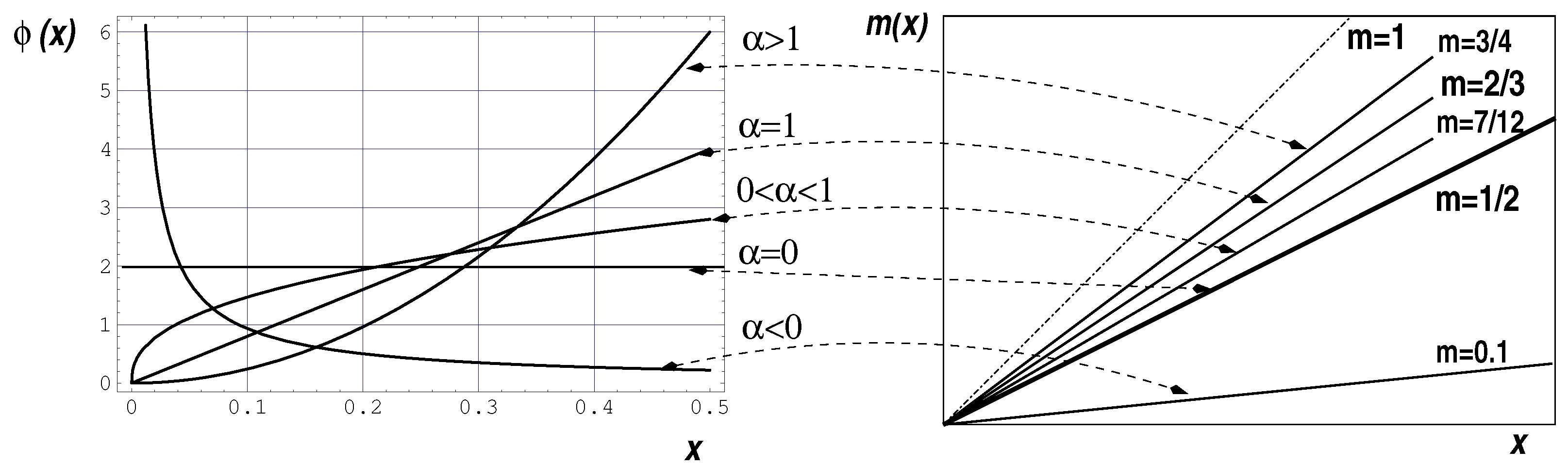

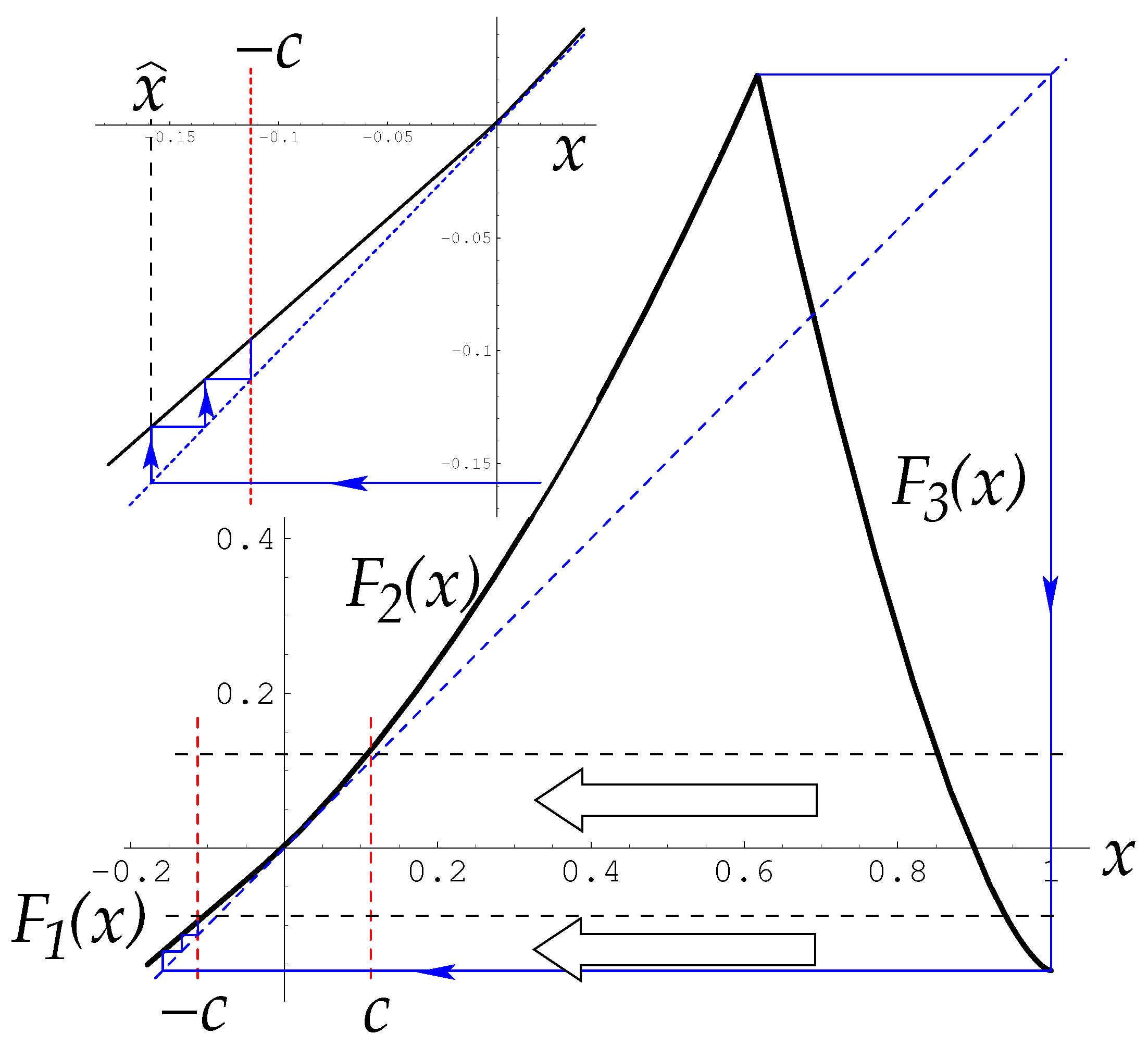
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elaskar, S.; del Río, E. Review of Chaotic Intermittency. Symmetry 2023, 15, 1195. https://doi.org/10.3390/sym15061195
Elaskar S, del Río E. Review of Chaotic Intermittency. Symmetry. 2023; 15(6):1195. https://doi.org/10.3390/sym15061195
Chicago/Turabian StyleElaskar, Sergio, and Ezequiel del Río. 2023. "Review of Chaotic Intermittency" Symmetry 15, no. 6: 1195. https://doi.org/10.3390/sym15061195
APA StyleElaskar, S., & del Río, E. (2023). Review of Chaotic Intermittency. Symmetry, 15(6), 1195. https://doi.org/10.3390/sym15061195





