Stability of Peakons and Periodic Peakons for the mCH–Novikov–CH Equation
Abstract
:1. Introduction
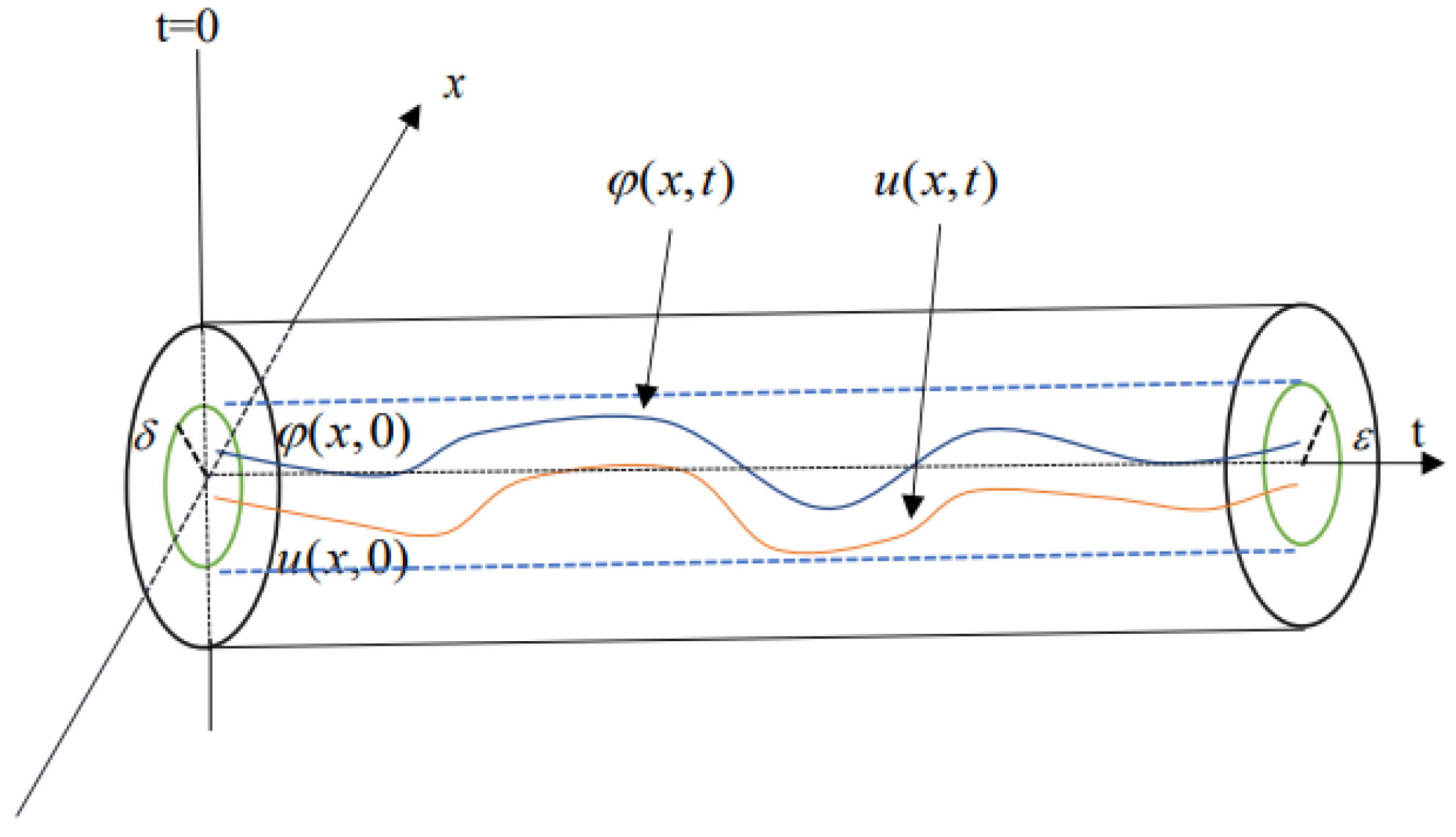
2. Preliminary
3. Stability
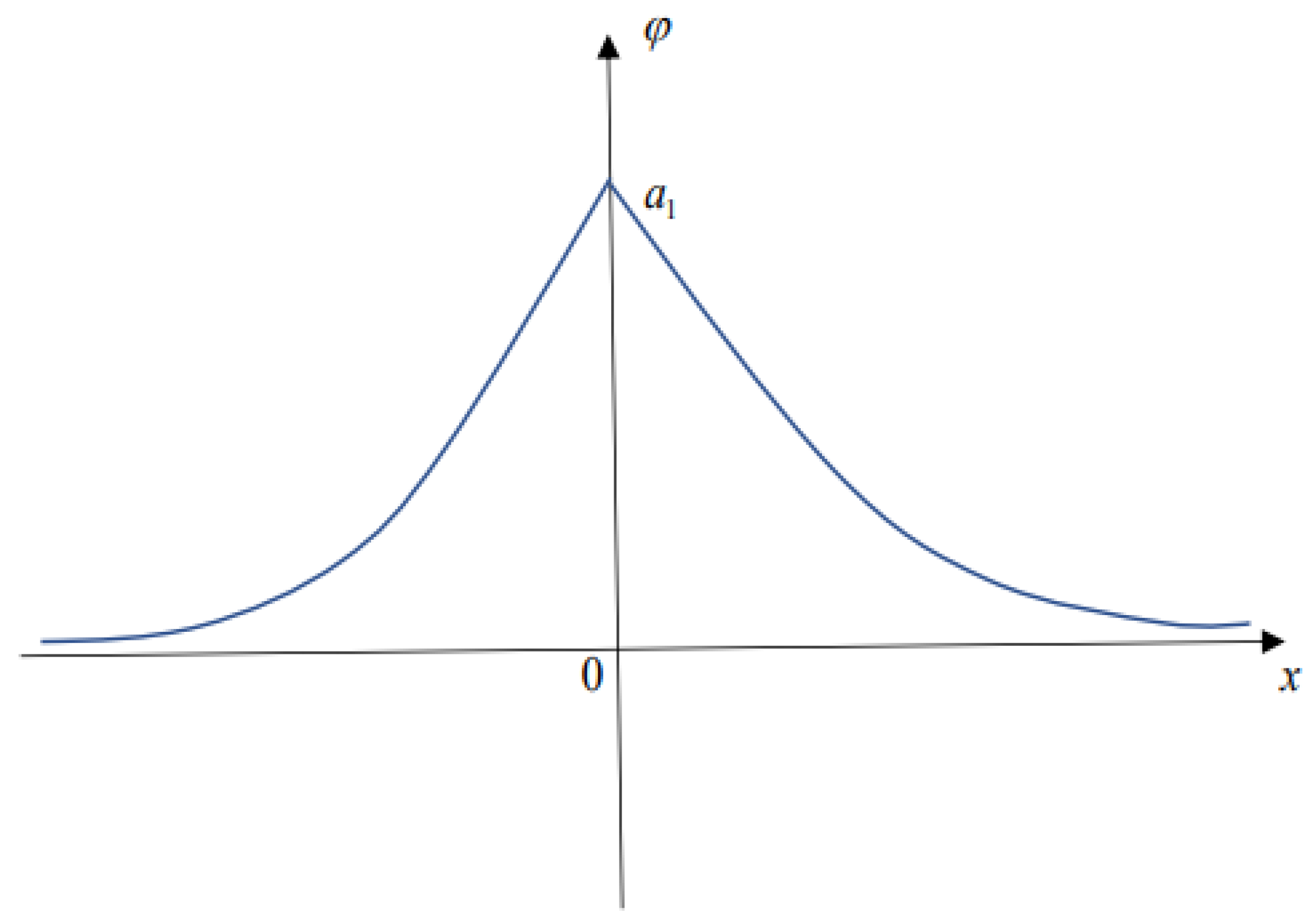
3.1. Stability of Peakons
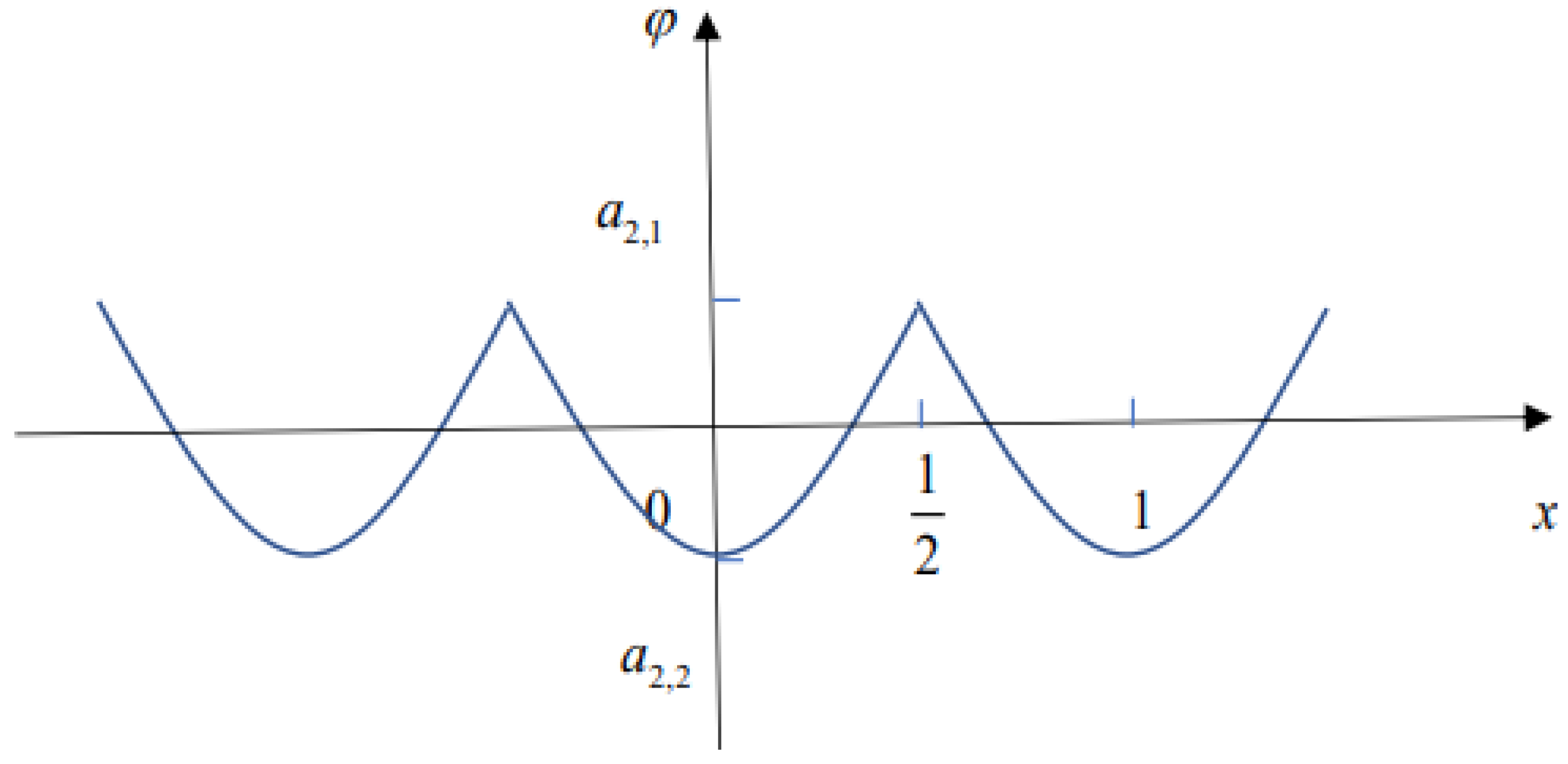
3.2. Stability of Periodic Peakons
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Qin, G.; Yan, Z.; Guo, B. The cauchy problem and multi-peakons for the mCH–Novikov–CH equation with quadratic and cubic nonlinearities. J. Dyn. Differ. Equ. 2022, 2022, 1–60. [Google Scholar] [CrossRef]
- Camassa, R.; Holm, D. An integrable shallow water equation with peaked solitons. Phys. Rev. Lett. 1993, 71, 1661–1664. [Google Scholar] [CrossRef]
- Constantin, A.; Lannes, D. The hydrodynamical relevance of the Camassa–Holm and Degasperis-Procesi equations. Arch. Ration. Mech. Anal. 2009, 192, 165–186. [Google Scholar] [CrossRef]
- Camassa, R.; Holm, D.; Hyman, J. A new integrable shallow water equation. Adv. Appl. Mech. 1994, 31, 1–33. [Google Scholar]
- Fuchssteiner, B.; Fokas, A. Symplectic structures, their Bäcklund transformations and hereditary symmetries. Physics D 1982, 4, 47–66. [Google Scholar] [CrossRef]
- Alber, M.; Camassa, R.; Holm, D. The geometry of peaked solitons and billiard solutions of a class of integrable PDE’s. Lett. Math. Phys. 1994, 32, 137–151. [Google Scholar] [CrossRef]
- Constantin, A.; Escher, J. Wave breaking for nonlinear nonlocal shallow water equations. Acta Math. 1998, 181, 229–243. [Google Scholar] [CrossRef]
- Constantin, A.; Escher, J. Global existence and blow-up for a shallow water equation. Ann. Sci. Norm. Super. 1998, 26, 303–328. [Google Scholar]
- Li, Y.; Olver, P. Well-posedness and blow-up solutions for an integrable nonlinearly dispersive model wave equation. J. Differ. Equ. 2000, 162, 27–63. [Google Scholar] [CrossRef]
- Constantin, A.; Strauss, W. Stability of peakons. Commun. Pure Appl. Math. 2000, 53, 603–610. [Google Scholar] [CrossRef]
- Lenells, J. Stability of periodic peakons. Int. Math. Res. Not. 2004, 10, 485–499. [Google Scholar] [CrossRef]
- Constantin, A.; Molinet, L. Orbital stability of solitary waves for a shallow water equation. Physics D 2001, 157, 75–89. [Google Scholar] [CrossRef]
- Dika, K.; Molinet, L. Stability of multipeakons. Ann. I. H. Poincaré-An. 2009, 26, 1517–1532. [Google Scholar] [CrossRef]
- Wang, T.; Han, X.; Lu, Y. On the solutions of the b-family of Novikov equation. Symmetry 2021, 13, 1765. [Google Scholar] [CrossRef]
- Ray, S.S.; Sahoo, S. Traveling wave solutions to Riesz time-fractional Camassa–Holm equation in modeling for shallow-water waves. J. Comput. 2015, 10, 061026. [Google Scholar]
- Lin, Z.; Liu, Y. Stability of peakons for the Degasperis-Procesi equation. Commun. Pure. Appl. Math. 2009, 62, 125–146. [Google Scholar] [CrossRef]
- Liu, X.; Liu, Y.; Qu, C. Stability of peakons for the Novikov equation. J. Math. Pure Appl. 2014, 101, 172–187. [Google Scholar] [CrossRef]
- Wang, Y.; Tian, L. Stability of periodic peakons for the Novikov equation. arXiv 2018, arXiv:1811.05835v1. [Google Scholar]
- Moon, B. Single peaked traveling wave solutions to a generalized μ-Novikov equation. Adv. Nonlinear Anal. 2021, 10, 66–75. [Google Scholar] [CrossRef]
- Olver, P.; Rosenau, P. Tri-hamiltonian duality between solitons and solitary-wave solutions having compact support. Phys. Rev. E 1996, 53, 1900–1906. [Google Scholar] [CrossRef]
- Qu, C.; Liu, X.; Liu, Y. Stability of peakons for an integrable modified Camassa–Holm equation with cubic nonlinearity. Commun. Math. Phys. 2013, 322, 967–997. [Google Scholar] [CrossRef]
- Liu, X.; Liu, Y.; Olver, P.J.; Qu, C. Orbital stability of peakons for a generalization of the modified Camassa–Holm equation. Nonlinearity 2014, 27, 2297–2319. [Google Scholar] [CrossRef]
- Moon, B. Orbital stability of periodic peakons for the generalized modified Camassa–Holm equation. Discrete. Contin. Dyn. Syst. Ser. A 2021, 14, 4409–4437. [Google Scholar] [CrossRef]
- Chen, R.; Di, H.; Liu, Y. Stability of peaked solitary waves for a class of cubic quasilinear shallow-water equations. Int. Math. Res. Not. 2022, 2022, rnac032. [Google Scholar] [CrossRef]
- Chen, A.; Deng, T.; Qiao, Z. Stability of peakons and periodic peakons for a nonlinear quartic Camassa–Holm equation. Mon. Hefte. Math. 2022, 198, 251–288. [Google Scholar] [CrossRef]
- Hwang, G.; Moon, B. Periodic peakons to a generalized μ-Camassa–Holm-Novikov equation. Appl. Anal. 2021, 2021, 1–11. [Google Scholar] [CrossRef]
- Izgi, Z.P.; Saglam, F.N.; Sahoo, S. A partial offloading algorithm based on intelligent sensing. Int. J. Mod. Phys. B 2022, 36, 2500977. [Google Scholar] [CrossRef]
- Sahoo, S.; Saha, R.S. New soliton solutions of fractional Jaulent-Miodek system with symmetry analysis. Symmetry 2020, 12, 1001. [Google Scholar] [CrossRef]
- Tripathy, A.; Sahoo, S. New optical behaviours of the time-fractional Radhakrishnan-Kundu-Lakshmanan model with Kerr law nonlinearity arise in optical fibers. Opt. Quantum Electron. 2022, 54, 232. [Google Scholar] [CrossRef]
- Drazin, P.G.; Johnson, R.S. Solitons: An Introduction; Cambridge University Press: Cambridge, UK, 1989. [Google Scholar]
- Constantin, A.; Escher, J. Analyticity of periodic traveling free surface water waves with vorticity. Ann. Math. 2011, 173, 559–568. [Google Scholar] [CrossRef]
- Sahoo, S.; Saha, R.S. Invariant analysis with conservation law of time fractional coupled Ablowitz–Kaup–Newell–Segur equations in water waves. Waves Random Complex Media 2020, 30, 530–543. [Google Scholar] [CrossRef]
- Sahoo, S.; Saha, R.S. On the conservation laws and invariant analysis for time-fractional coupled Fitzhugh-Nagumo equations using the Lie symmetry analysis. Eur. Phys. J. Plus 2019, 134, 83. [Google Scholar] [CrossRef]
- Sahoo, S.; Saha, R.S. Lie symmetries analysis and conservation laws for the fractional Calogero–Degasperis–Ibragimov–Shabat equation. Int. J. Geom. Methods Mod. Phys. 2018, 15, 1–11. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, K.; Yu, J.; Tang, S. Stability of Peakons and Periodic Peakons for the mCH–Novikov–CH Equation. Symmetry 2022, 14, 1702. https://doi.org/10.3390/sym14081702
Zhang K, Yu J, Tang S. Stability of Peakons and Periodic Peakons for the mCH–Novikov–CH Equation. Symmetry. 2022; 14(8):1702. https://doi.org/10.3390/sym14081702
Chicago/Turabian StyleZhang, Kelei, Jianguo Yu, and Shengqiang Tang. 2022. "Stability of Peakons and Periodic Peakons for the mCH–Novikov–CH Equation" Symmetry 14, no. 8: 1702. https://doi.org/10.3390/sym14081702
APA StyleZhang, K., Yu, J., & Tang, S. (2022). Stability of Peakons and Periodic Peakons for the mCH–Novikov–CH Equation. Symmetry, 14(8), 1702. https://doi.org/10.3390/sym14081702





