Abstract
In this paper, the Sharma-Tasso-Olver-Burgers (STOB) system is analyzed by the Lie point symmetry method. The hypergeometric wave solution of the STOB equation is derived by symmetry reductions. In the meantime, the consistent tanh expansion (CTE) method is applied to the STOB equation. An nonauto-Bäcklund (BT) theorem that includes the over-determined equations and the consistent condition is obtained by the CTE method. By using the nonauto-BT theorem, the interactions between one-soliton and the cnoidal wave, and between one-soliton and the multiple resonant soliton solutions, are constructed. The dynamics of these novel interaction solutions are shown both in analytical and graphical forms. The results are potentially useful for explaining ocean phenomena.
1. Introduction
The study of exact solutions of integrable systems has been extensively investigated. A number of mathematical physical equations have been investigated by various classical methods, such as the inverse scattering transformation [], the Painlevé property [], the Darboux transformation [], the Bäcklund transformation (BT) [], the Hirota bilinear method [], and the variable separation method [], etc. [,,,,,,,]. Among these methods, the Lie point symmetry approach plays key roles in studying the nonlinear partial differential equation (NPDE) [,]. It can reduce the number of variables for the NPDE and the order of the ordinary differential equation (ODE). One can use the symmetry to obtain the explicit solutions. The group invariant solutions are obtained by the symmetry reduction equation.
On the other hand, in order to construct various interaction solutions between different types of excitations, it is necessary to develop some methods for studying nonlinear systems. The soliton solutions on a cnoidal waves background, which can treated as a nonautonomous soliton solution, have been extensively investigated []. The interaction between solitons and the cnoidal waves can be obtained by the Darboux transformation [], the symmetry reductions related by the nonlocal symmetry and the consistent tanh expansion (CTE) method [,,,,,,]. The CTE method is a relatively simple method to obtain various interaction solutions between different types of excitations. The exploration of the Lie point symmetry method and the CTE method for the NPDE is an interesting topic []. These methods have been not applied to the Sharma-Tasso-Olver-Burgers (STOB) equation. In this paper, the purpose of this study is mainly to apply the Lie point symmetry approach and the CTE method to the STOB equation.
The STOB equation has the following form:
where and are arbitrary constants. It is obvious that (1) will degenerate to the usual Burgers system with and the STO system with , respectively. The integrability properties and the nonlinear waves of these three integrable systems have been investigated by using different approaches. Super extension of the Burgers equation is studied by the bosonization approach [,]. The soliton fission and fusion of the STO equation is studied by the symmetry reduction procedure []. The nonlocal symmetry and the CTE method are applied to the STO equation [,]. Non-topological, topological and rogue waves for the STO equation are constructed by the solitary wave ansatz approach []. For the STOB equation, lump and diverse interaction solutions are obtained by means of the corresponding bilinear form []. Soliton molecules of the STOB equation are constructed by means of the velocity resonant mechanism []. Conservation laws and the kink solitons are studied by the formal Lagrangian, the Kudryashov and exponential methods [].
The paper is organized as follows. The preliminaries of the Lie point symmetry and the CTE method are introduced in Section 2. In Section 3, the STOB equation is studied by the Lie point symmetry method. In Section 4, the CTE method is applied to the STOB equation. Some novel interaction solutions are constructed using the nonauto-BT theorem. Section 5 is a simple summary and discussion.
2. Preliminaries of Lie Point Symmetry and CTE Method
2.1. Method of Lie Point Symmetry
One supposes the form of a derivative nonlinear polynomial equation as
A one-parameter Lie group of infinitesimal transformations on the system (2) reads
where is the parameter of the transformation and and are the infinitesimals of the transformations, respectively. A symmetry of (2) is defined as a solution of its linearized equation
The general Lie point symmetry has the form
The corresponding vector with the group of transformations can be written as
2.2. Method of CTE Method
According to the CTE method [], the expansion form of Equation (2) reads as
where the positive integer is determined by the leading order analysis, and is an arbitrary function of . Substituting Equation (7) into Equation (2) leads to
where are dependent on the models, and are functions of and their derivatives. By vanishing different powers of , we obtain the over-determined system
3. Lie Point Symmetry and Similarity Reductions of STOB Equation
Based on the Lie symmetry method [], the STOB equation is invariant under transformation
The general vector field is given as
where and U are the functions of and u. The symmetry equation for is expressed as a solution of the linearized system (1)
The symmetry has the form
Over-determined equations of the STOB system can be obtained by substituting Equation (13) into the symmetry Equation (12) and letting u satisfy the STOB system. Solving the over-determined equations leads to the infinitesimals
where are arbitrary constants. We can find the group invariant solutions by solving the characteristic equation []
The general symmetry reductions related with Equation (14) are studied in detail. There are three cases for symmetry reductions.
Case I. By solving the characteristic Equation (15), the similarity solution is given as the following form
with the similarity variable and the group invariant function . By substituting Equation (16) into the system (1), the invariant function satisfies the variable coefficient of the ODE
It is obvious that once the solution is solved by Equation (17), the the similarity solution is given by using Equation (16).
By means of the MAPLE technique, the hypergeom solution of can be obtained by solving Equation (17) directly
where H denotes the generalized hypergeometric function. The solution of the STOB Equation (1) can be obtained by using Equations (16) and (18). The type solution of the hypergeometric function can be also obtained by means of the Hopf–Cole transformation [].
Case II. . The group invariant solution reads as the following form after solving the characteristic Equation (15)
with the similarity variable and the group invariant function . Substituting Equation (19) into Equation (1), the invariant function satisfies the reduction system
As a similar procedure, the solution of the STOB equation can be derived by solving the reduction system (20).
Case III. . The group invariant solution reads as the following form after solving out the characteristic Equation (15),
The group invariant function satisfies the following reduction system
The field of u can be obtained by solving the above reduction system.
4. CTE Solvability and Interaction Solutions of STOB Equation
Based on the CTE method [], the generalized truncated tanh expansion of the STOB equation is
where , and w are arbitrary functions of x and t. By substituting Equation (23) into the STOB system (1) and vanishing the coefficients of the powers of , one obtains two classes solutions.
Case I. The functions and read as
and w satisfies the following over-determined equations
where and K satisfies the Schwarzian derivative [].
Case II. The solutions of and are
and the consistent condition is
From the above detailed calculations, an nonauto-BT theorem can be constructed.
Nonauto-BT theorem. One finds the solution w of the over-determined Equations (25) and (26) or the consistent condition (28); then, the following forms of u are also a solution of the STOB system
and
respectively.
By using the above nonauto-BT theorem, some interactions between solitons and other types of nonlinear waves are derived. One lists some novel interactions as follows. The interaction between one-soliton and the cnoidal wave for the over-determined Equations (25) and (26) is assumed
where , , and are all free constants. Substituting Equation (31) into Equation (25), one obtains the over-determined equations of :
with
and
where and are arbitrary constants. Equation (32) is the standard elliptic function equation, while Equation (33) becomes the identical equation with the constraint as
It indicates that the interaction between one soliton and the cnoidal wave is obtained by solving the over-determined equations. This type of interaction solution is discussed via the CTE method [,].
Besides solving over-determined equations, the interaction between one-soliton and the cnoidal wave can be obtained by solving the consistent condition (28). The corresponding solution form assumes
where and are the third kind of elliptic integral and the Jacobi elliptic function, respectively. Substituting Equation (35) into Equation (28), one obtains
where and represent the Jacobi elliptic functions of and , respectively. Vanishing the coefficients of the powers of , the non-trivial constants are
By selecting the parameters as
the interaction solution (38) becomes
with the first kind of incomplete elliptic integral of . Figure 1 displays the three-dimensional and the density plots of interaction solution (40).
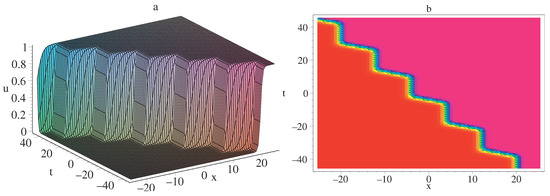
Figure 1.
The graph of the propagation for u. (a) Three-dimensional plotting. (b) Density plotting.
The interaction between one soliton and multiple resonant soliton solutions of the consistent condition (28) has the form
where and are arbitrary constants and and satisfy the relations
By substituting Equations (41) and (42) into Equation (30), the interaction between one-soliton and the multiple resonant soliton solutions can be written as
with . We show this type of solution in Figure 2 by selecting the parameters as
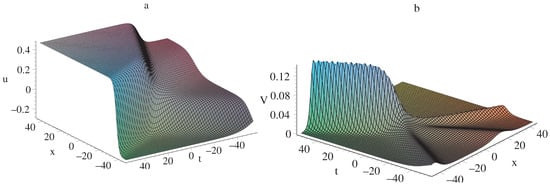
Figure 2a,b plot the solution of u and the potential of u, i.e., , respectively. It is obvious that three solitary waves become a single wave with the time evolution. This is called the fusion phenomena of the solitary waves, which have been studied both theoretically and experimentally [].
These types of interaction between solitons and the cnoidal periodic waves, and interaction between solitons and the multiple resonant soliton solutions, may happen in the ocean []. The results are useful for explaining ocean phenomena.
5. Conclusions and Discussion
In summary, the Lie point symmetry approach and the CTE method are applied for solving the STOB equation. One obtains three classes of symmetry reduction equations based on the infinitesimal generators. Some explicit solutions are derived by solving the symmetry reduction equations. In addition, a nonauto-BT theorem that includes the types of the over-determined Equations (25) and (26) and the consistent condition (28) is obtained by the CTE method. The interaction between one-soliton and the cnoidal wave can be obtained by solving the over-determined equations and the consistent condition. The interaction between one-soliton and the multiple resonant soliton solutions is derived by means of the consistent condition. These novel solutions are studied both in analytical and graphical ways. The fusion phenomena of the solitary waves are shown in Figure 2. The results are helpful in understanding some physical phenomena including fluid dynamics, oceanography and related disciplines.
Besides the Lie point symmetry, the nonlocal symmetry is widely studied by the Painlevé analysis, the Lax pair and so on [,,]. Based on the the Painlevé analysis, the solution of the STOB equation can be expanded as the following form about the singularity manifold
where and are functions with respect to x and t. By substituting Equation (45) into Equation (1) and vanishing the coefficients of the powers of independently. We obtain
This type of nonlocal symmetry, which is named the residual symmetry, can be read out by the residual of the singularity manifold []. The nonlocal symmetry of the STOB equation is written as from the expression (46). The field of f satisfies the following equation
The symmetry reductions related tp the nonlocal symmetry and the infinite many nonlocal symmetries are worth studying.
Author Contributions
Conceptualization, B.R.; formal analysis, J.Y., B.R. and W.-L.W.; investigation, J.Y. and B.R.; writing—original draft preparation, B.R. All authors have read and agreed to the published version of the manuscript.
Funding
The publishing of this paper is financially supported by the National Natural Science Foundation of China under Grant Nos. 11775146, 11975156 and 12105243, and Zhejiang Provincial Natural Science Foundation of China under Grant No. LQ22A050002.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
The data presented in this study are available on request from the corresponding author.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Gardner, C.S.; Greene, J.M.; Kruskal, M.D.; Miura, R.M. Method for solving the Korteweg-deVries equation. Phys. Rev. Lett. 1967, 19, 1095–1097. [Google Scholar] [CrossRef]
- Weiss, J.; Tabor, M.; Carnevale, G. The Painlevé property for partial differential equations. J. Math. Phys. 1983, 24, 522–526. [Google Scholar] [CrossRef]
- Matveev, V.B.; Salle, M.A. Darboux Transformations and Solitons; Springer: Berlin, Germany, 1991. [Google Scholar]
- Rogers, C.; Schief, W.K. Bäcklund and Darboux transformations geometry and modern applications in soliton theory. In Cambridge Texts in Applied Mathematics; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Hirota, R. The Direct Method in Soliton Theory; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Tang, X.Y.; Lou, S.Y.; Zhang, Y. Localized excitations in (2+1)-dimensional systems. Phys. Rev. E 2002, 66, 046601. [Google Scholar] [CrossRef]
- Jin, X.W.; Shen, S.J.; Yang, Z.Y.; Lin, J. Magnetic lump motion in saturated ferromagnetic films. Phys. Rev. E 2022, 105, 014205. [Google Scholar] [CrossRef]
- Dai, C.Q.; Chen, R.P.; Wang, Y.Y.; Fan, Y. Dynamics of light bullets in inhomogeneous cubic-quintic-septimal nonlinear media with PT-symmetric potentials. Nonlinear Dyn. 2017, 87, 1675–1683. [Google Scholar] [CrossRef]
- Fang, Y.; Wu, G.Z.; Wang, Y.Y.; Dai, C.Q. Data-driven femtosecond optical soliton excitations and parameters discovery of the high-order NLSE using the PINN. Nonlinear Dyn. 2021, 105, 603–616. [Google Scholar] [CrossRef]
- Dai, C.Q.; Zhang, J.F. Controlling effect of vector and scalar crossed double-Ma breathers in a partially nonlocal nonlinear medium with a linear potential. Nonlinear Dyn. 2020, 100, 1621–1628. [Google Scholar] [CrossRef]
- Dai, C.Q.; Wang, Y.Y.; Fan, Y.; Zhang, J.F. Interactions between exotic multi-valued solitons of the (2+1)-dimensional Korteweg-de Vries equation describing shallow water wave. Appl. Math. Model. 2020, 80, 506–515. [Google Scholar] [CrossRef]
- Dai, C.Q.; Fan, Y.; Wang, Y.Y. Three-dimensional optical solitons formed by the balance between different-order nonlinearities and high-order dispersion/diffraction in parity-time symmetric potentials. Nonlinear Dyn. 2019, 98, 489–499. [Google Scholar] [CrossRef]
- Olver, P.J. Application of Lie Group to Differential Equation; Springer: Berlin, Germany, 1986. [Google Scholar]
- Bluman, G.W.; Anco, S.C. Symmetry and Integration Methods for Differential Equations; Springer: New York, NY, USA, 2002. [Google Scholar]
- Jiang, H.J.; Xiang, J.J.; Dai, C.Q.; Wang, Y.Y. Nonautonomous bright soliton solutions on continuous wave and cnoidal wave backgrounds in blood vessels. Nonlinear Dyn. 2014, 75, 201–207. [Google Scholar] [CrossRef]
- Shin, H.J. Multisoliton complexes moving on a cnoidal wave background. Phys. Rev. E 2005, 71, 036628. [Google Scholar] [CrossRef]
- Gao, X.N.; Lou, S.Y.; Tang, X.Y. Bosonization, singularity analysis, nonlocal symmetry reductions and exact solutions of supersymmetric KdV equation. J. High Energy Phys. 2013, 2013, 29. [Google Scholar] [CrossRef]
- Cheng, X.P.; Lou, S.Y.; Chen, C.L.; Tang, X.Y. Interactions between solitons and other nonlinear Schrödinger waves. Phys. Rev. E 2014, 89, 043202. [Google Scholar] [CrossRef] [PubMed]
- Ren, B. Interaction solutions for mKP equation with nonlocal symmetry reductions and CTE method. Phys. Scr. 2015, 90, 065206. [Google Scholar] [CrossRef]
- Ren, B.; Cheng, X.P.; Lin, J. The (2+1)-dimensional Konopelchenko-Dubrovsky equation: Nonlocal symmetries and interaction solutions. Nonlinear Dyn. 2016, 86, 1855. [Google Scholar] [CrossRef]
- Ren, B. Painlevé analysis, nonlocal symmetry and explicit interaction solutions for supersymmetric mKdVB equation. AIP Adv. 2016, 6, 085205. [Google Scholar] [CrossRef]
- Ren, B.; Lou, Z.M.; Liang, Z.F.; Tang, X.Y. Nonlocal symmetry and explicit solutions for Drinfel’d-Sokolov-Wilson system. Eur. Phys. J. Plus 2016, 131, 441. [Google Scholar] [CrossRef]
- Ren, B. Symmetry reduction related with nonlocal symmetry for Gardner equation. Commun. Nonlinear Sci. Numer. Simulat. 2017, 42, 456–463. [Google Scholar] [CrossRef]
- Ren, B. Painlevé analysis and exact solutions of bosonized N = 1 supersymmetric Burgers equation. Open Phys. 2015, 13, 205–209. [Google Scholar] [CrossRef]
- Ren, B.; Gao, X.N.; Yu, J.; Liu, P. Exact solutions of supersymmetric Burgers equation with bosonization procedure. Open Math. 2015, 13, 502–509. [Google Scholar] [CrossRef]
- Lian, Z.J.; Lou, S.Y. Symmetries and exact solutions of the Sharma-Tass-Olver equation. Nonlinear Anal. 2005, 63, e1167–e1177. [Google Scholar] [CrossRef]
- Hu, H.C.; Li, Y.Y.; Zhu, H.D. Residual symmetry, interaction solutions and consistent tanh expansion solvability for the third-order Burgers equation. Chaos Soliton Fractals 2018, 108, 77–81. [Google Scholar] [CrossRef]
- Pu, H.; Jia, M. CTE Solvability, Exact Solutions and nonlocal Symmetries of the Sharma-Tasso-Olver equation. Commun. Theor. Phys. 2015, 64, 623–629. [Google Scholar] [CrossRef]
- Zafar, Z.U.A.; Akinyemi, L.; Rezazadeh, H.; Inc, M. Non-topological, topological and rogue wave Soliton solutions for Sharma Tasso Olver equation. J. Ocean. Eng. Sci. 2022; in press. [Google Scholar] [CrossRef]
- Miao, Z.W.; Hu, X.R.; Chen, Y. Interaction phenomenon to (1+1)-dimensional Sharma-Tasso-Olver-Burgers equation. Appl. Math. Lett. 2021, 112, 106722. [Google Scholar] [CrossRef]
- Yan, Z.W.; Lou, S.Y. Soliton molecules in Sharma-Tasso-Olver-Burgers equation. Appl. Math. Lett. 2020, 104, 106271. [Google Scholar] [CrossRef]
- Hosseini, K.; Akbulut, A.; Baleanu, D.; Salahshour, S. The Sharma-Tasso-Olver-Burgers equation: Its conservation laws and kink solitons. Commun. Theor. Phys. 2022, 74, 025001. [Google Scholar] [CrossRef]
- Wang, G.W.; Kara, A.H. Nonlocal symmetry analysis, explicit solutions and conservation laws for the fourth-order Burgers’ equation. Chaos Soliton Fractals 2015, 81, 290–298. [Google Scholar] [CrossRef]
- Jin, X.W.; Lin, J. Rogue wave, interaction solutions to the KMM system. J. Magn. Magn. Mater. 2020, 502, 166590. [Google Scholar] [CrossRef]
- Cheng, W.; Li, B. CRE solvability, exact soliton-cnoidal wave interaction solutions, and nonlocal symmetry for the modified Boussinesq equation. Adv. Math. Phys. 2016, 2016, 4874392. [Google Scholar] [CrossRef]
- Wang, S.; Tang, X.Y.; Lou, S.Y. Soliton fission and fusion: Burgers equation and Sharma-Tasso-Olver equation. Chaos Soliton Fractals 2004, 21, 231–239. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).