Three-Dimensional Investigation of Hydraulic Properties of Vertical Drop in the Presence of Step and Grid Dissipators
Abstract
1. Introduction
2. Materials and Methods
2.1. Turbulence Model
- Large Vortex Simulation Model (LES),
- Model of two equations (k-ɛ),
- Model of Re-Normalized Group (RNG),
- Prandtl mixing length.
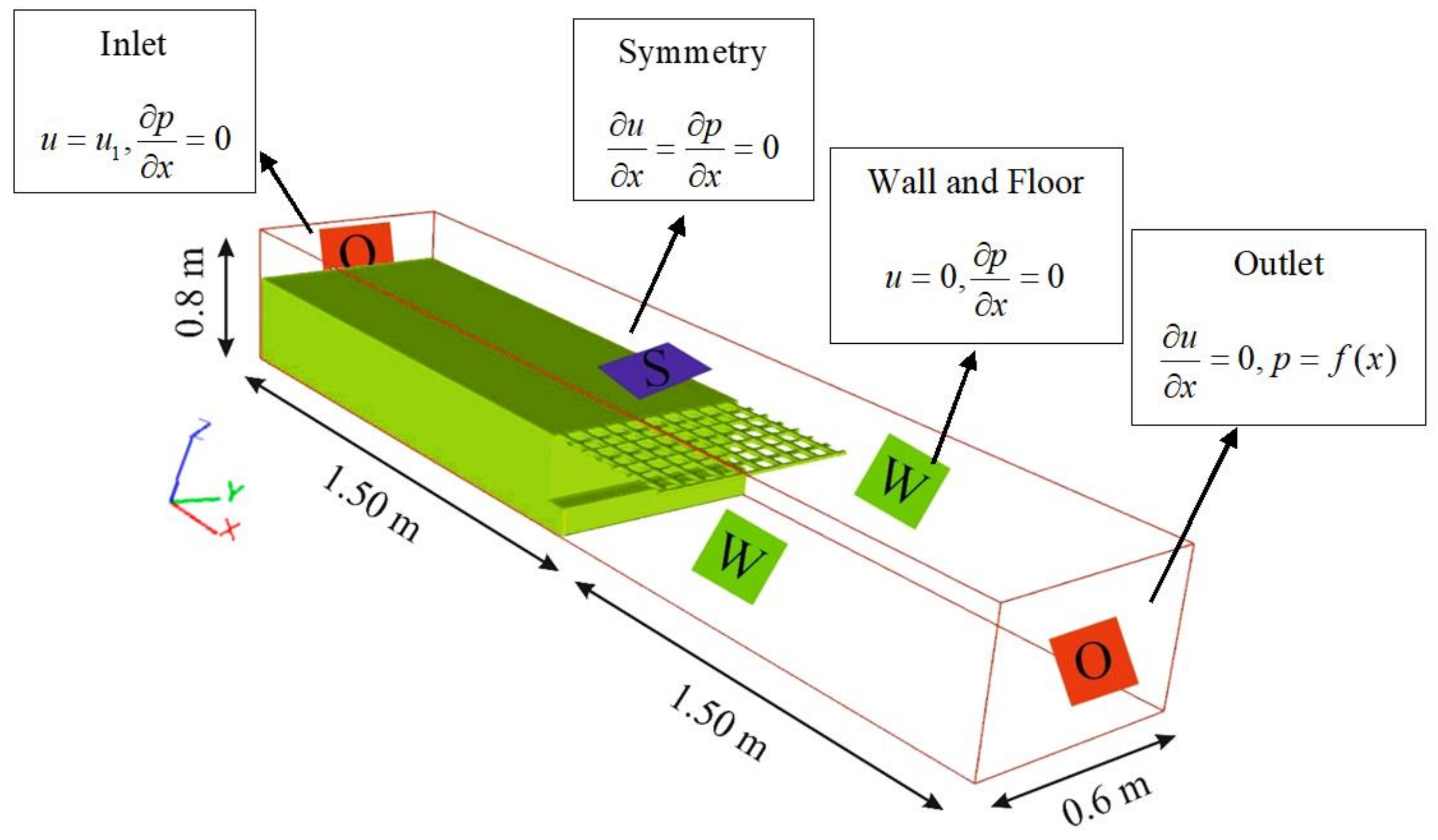
2.2. Simulation Specification and Solution Field Network
- (i)
- inlet boundary = volume flow rate (VFR),
- (ii)
- outlet boundary = outflow,
- (iii)
- the bottom and side, boundary = Wall, v: the top boundary = symmetry.
2.3. Dimensional Analysis
2.4. Classical Hydraulic Equations and Evaluation Criteria
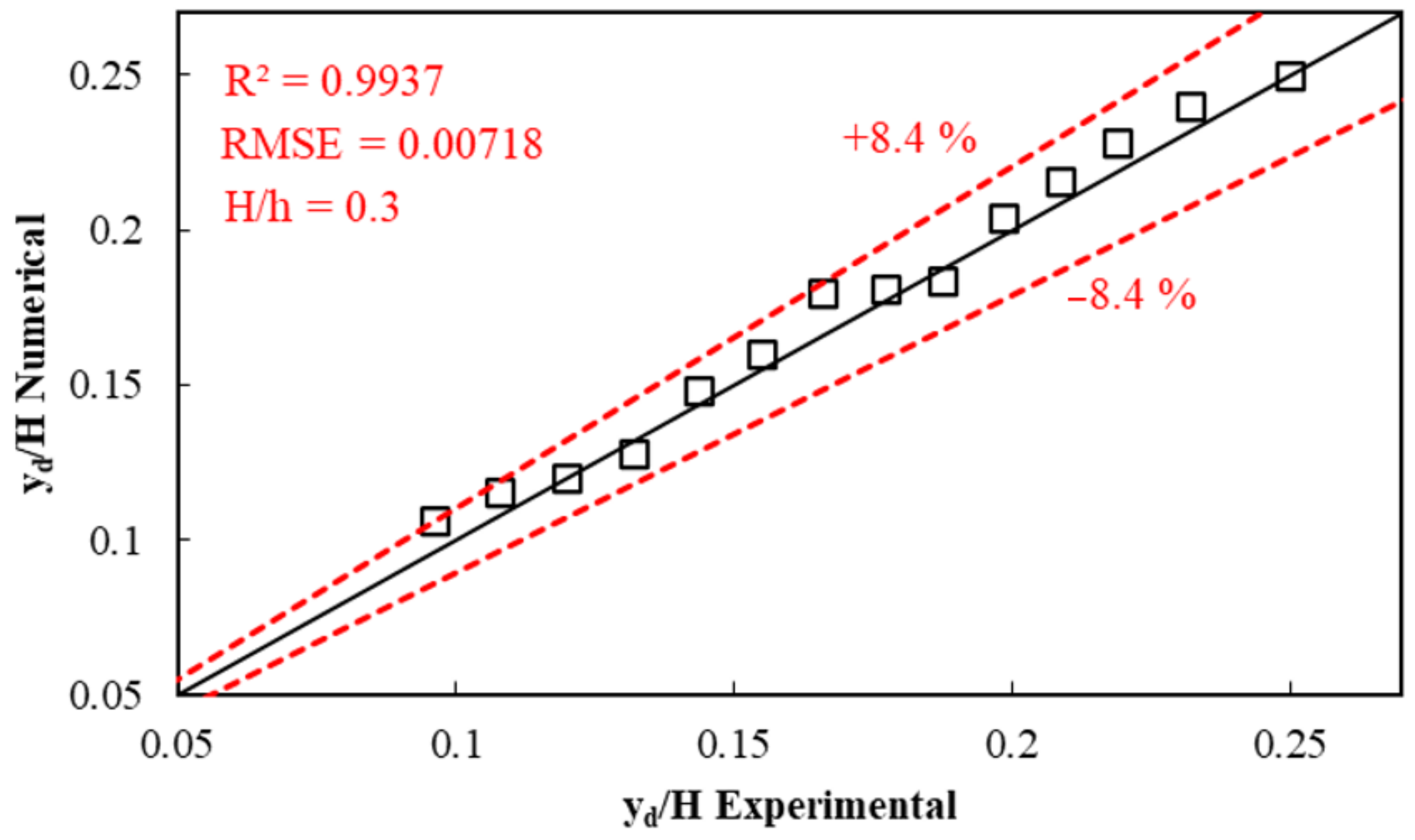
2.5. Validation
3. Results and Discussion
3.1. Water Surface Profile
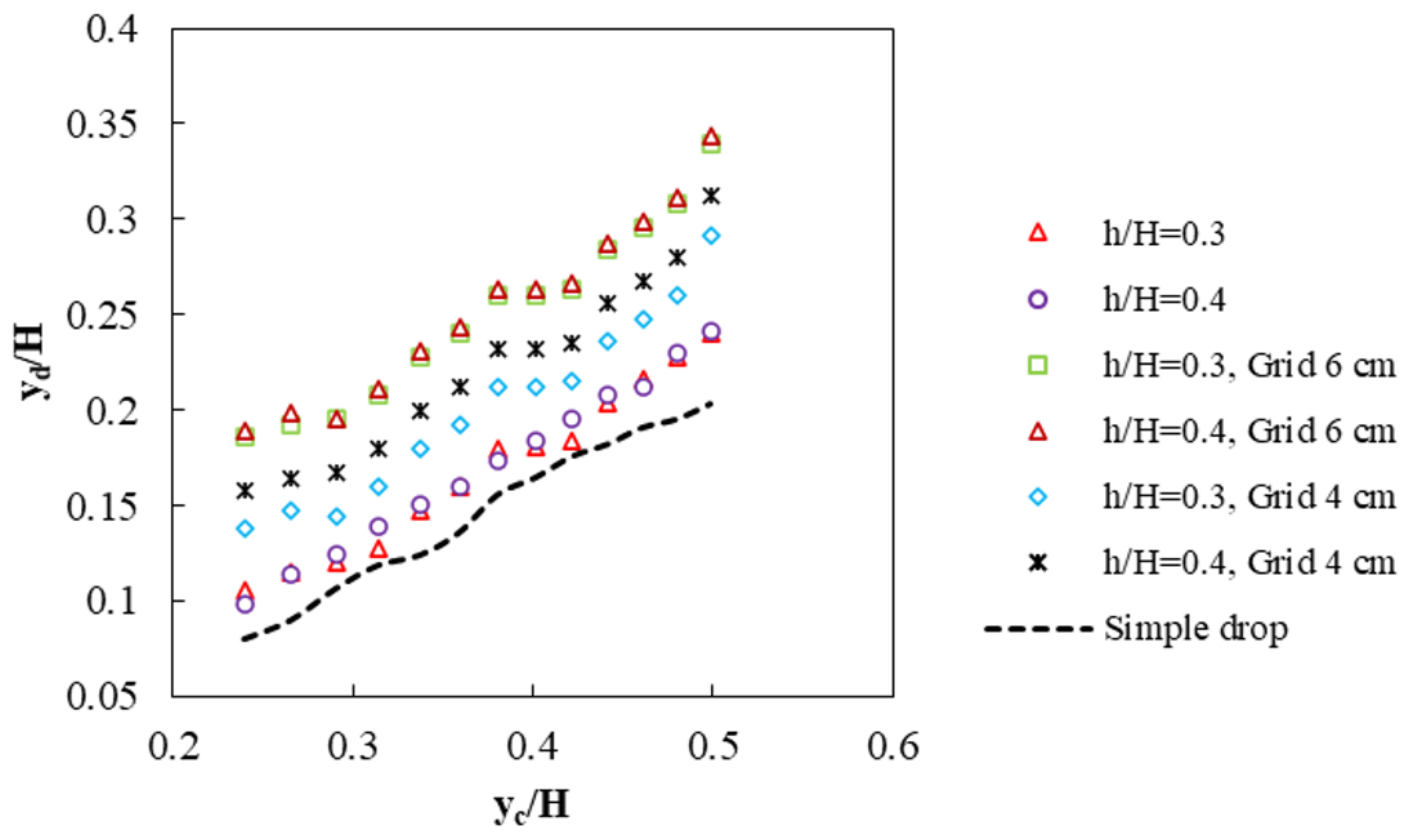
3.2. Relative Downstream Depth
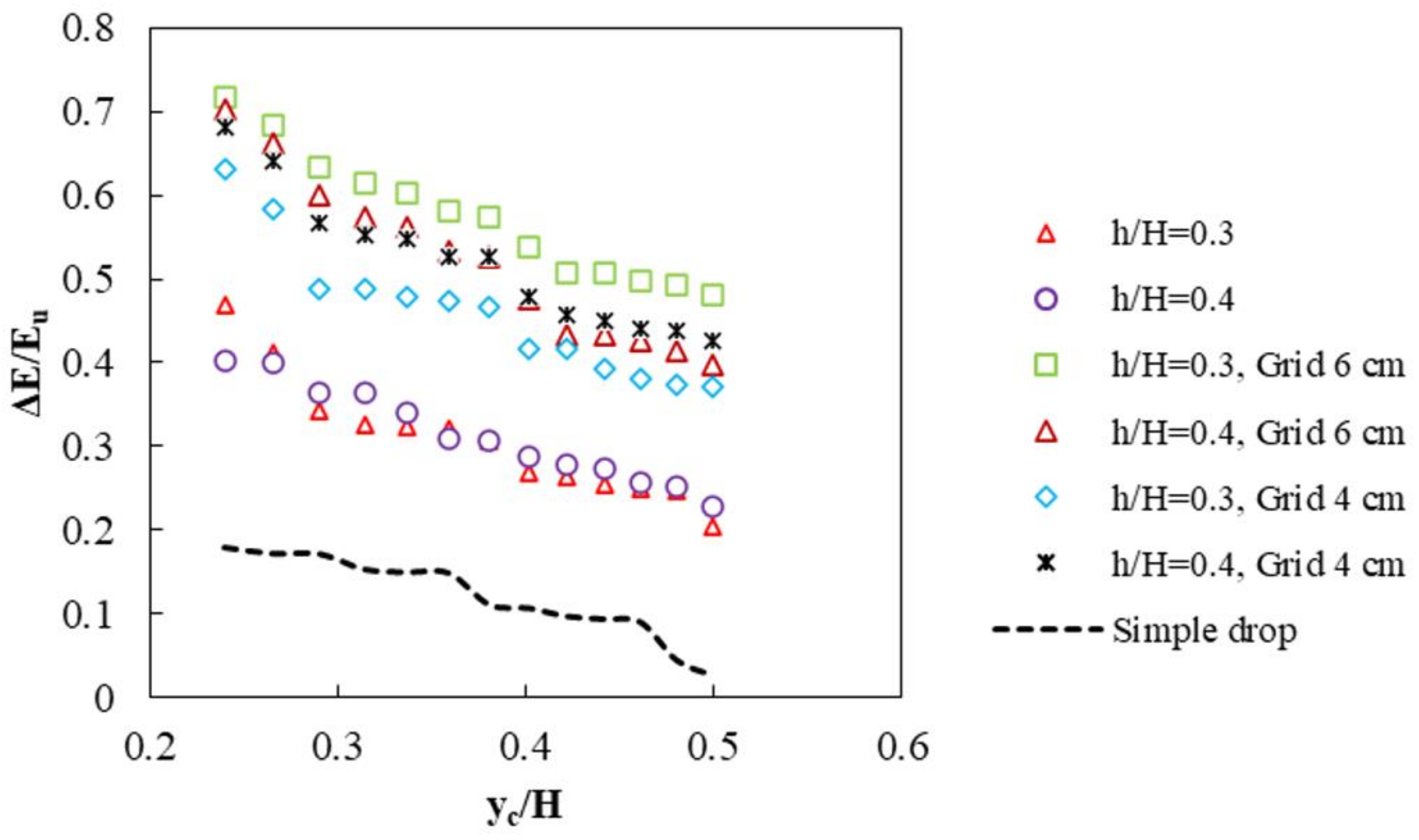
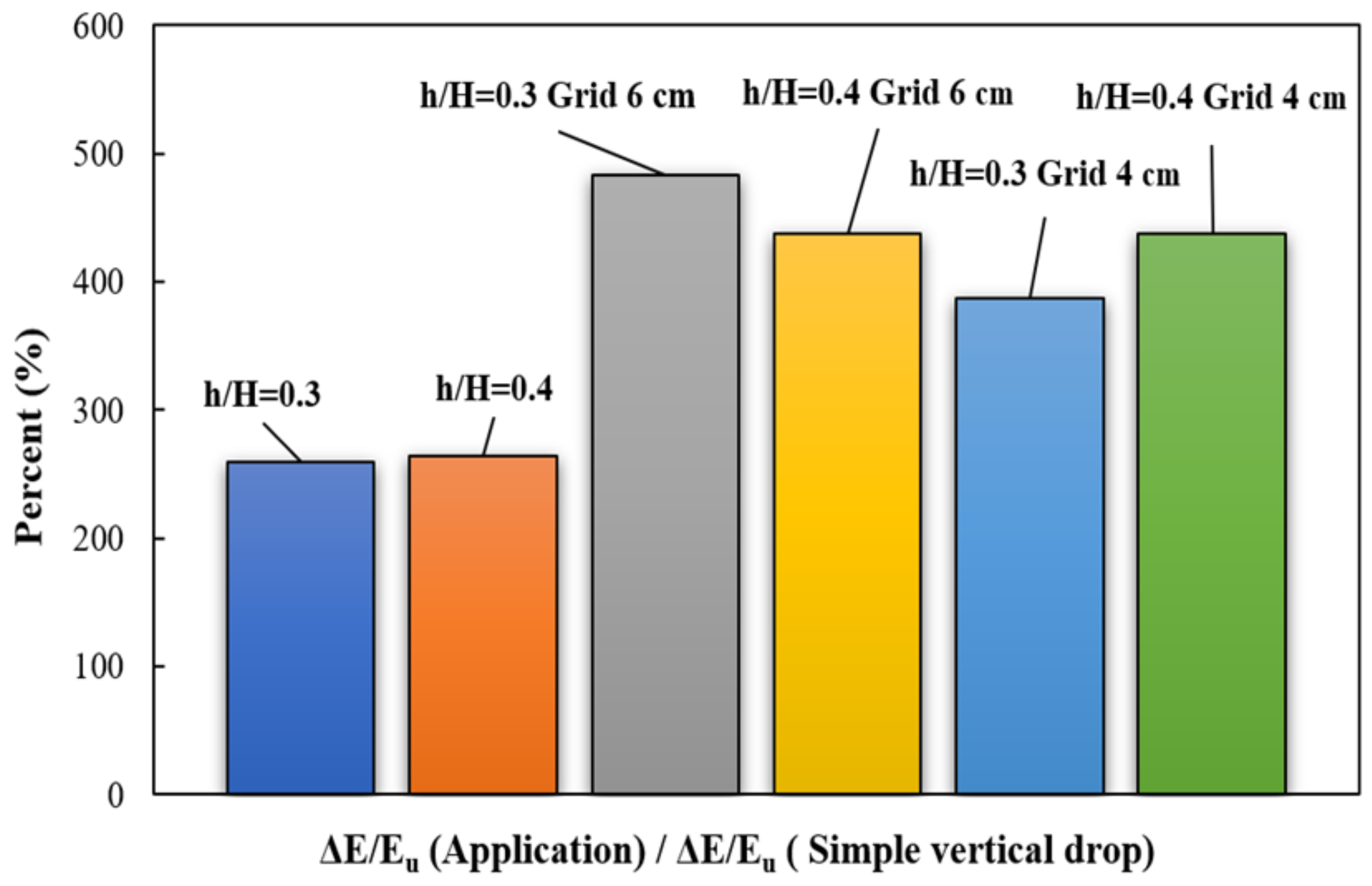
3.3. Relative Energy Dissipation
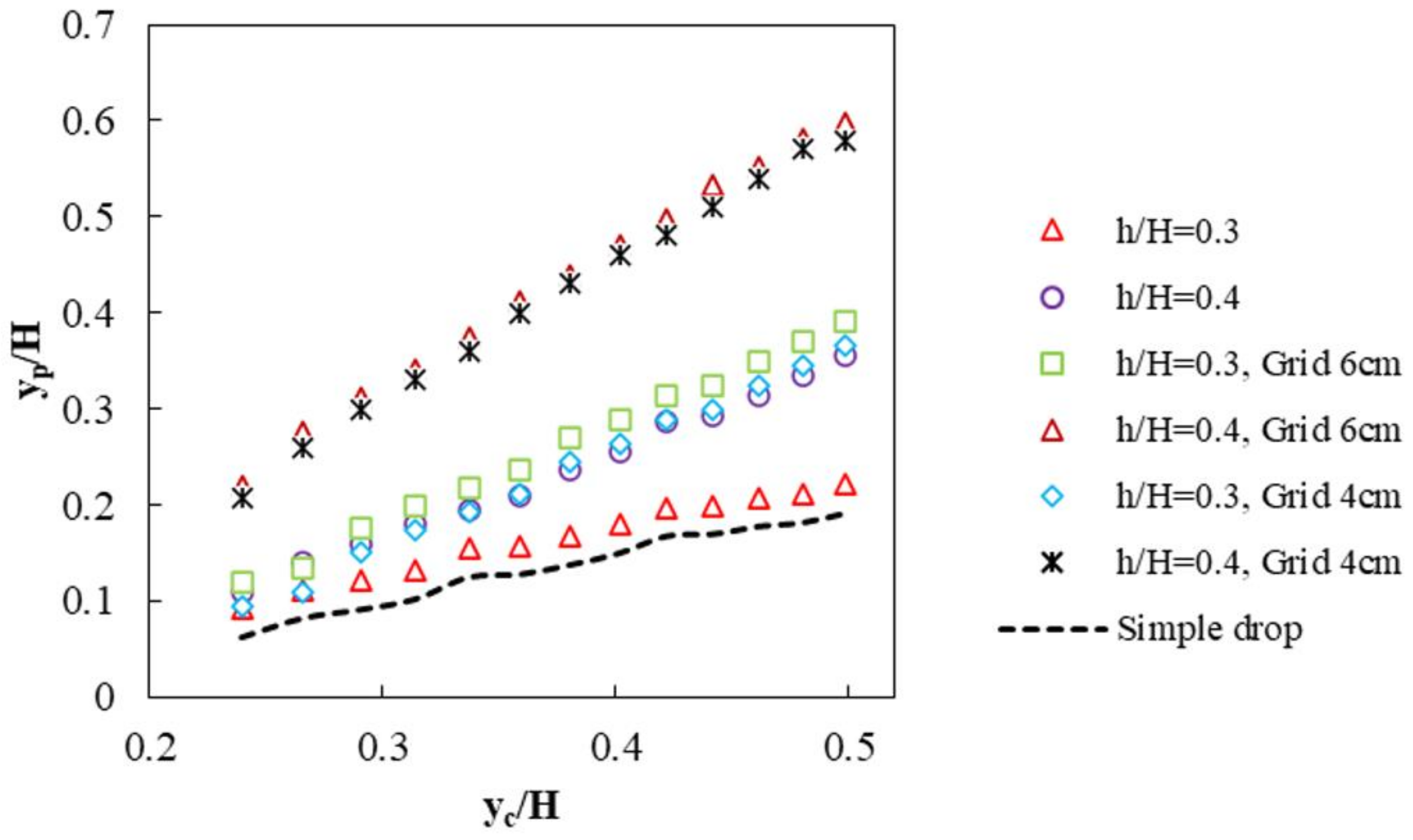
3.4. Relative Pool Depth
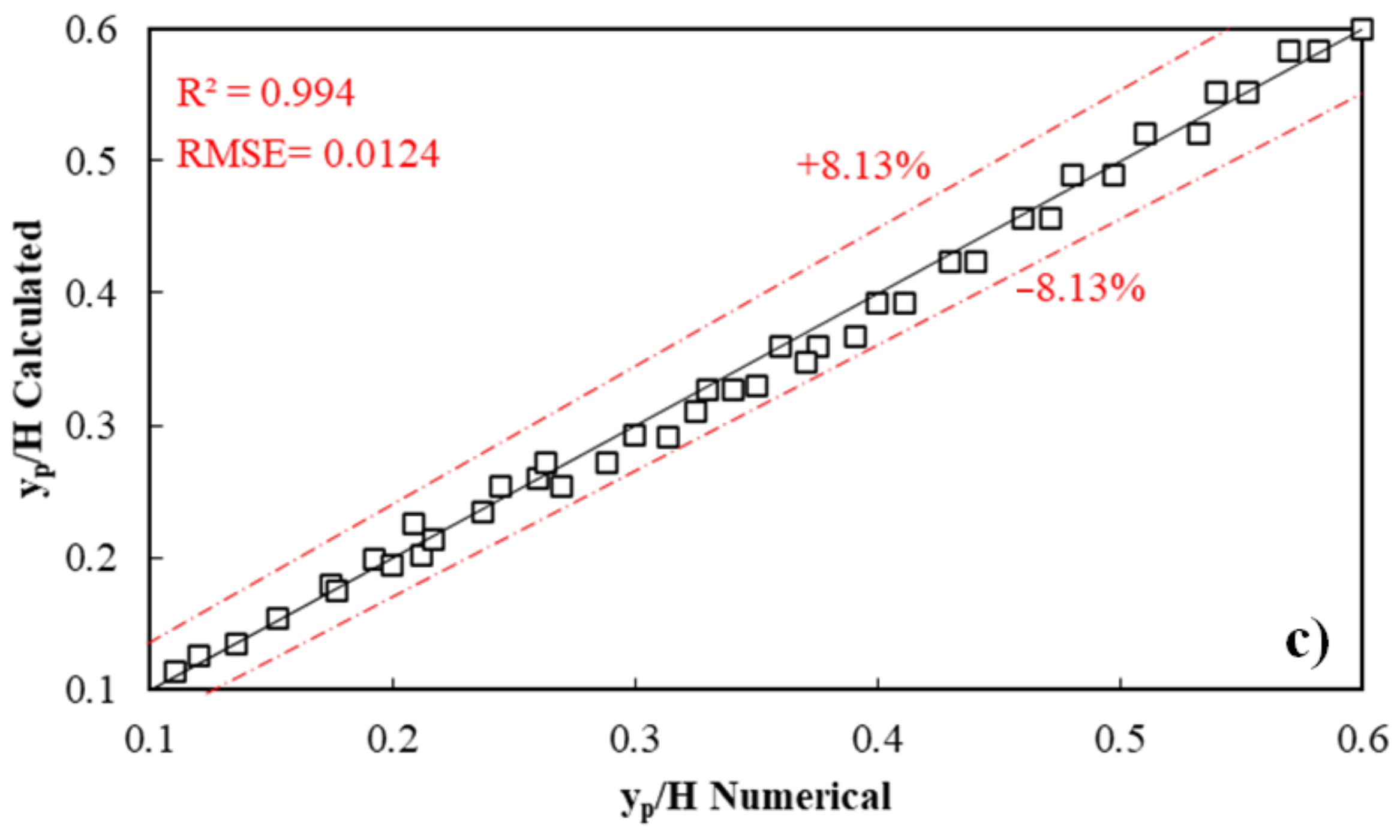
3.5. Equations
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Daneshfaraz, R.; Majedi Asl, M.; Bazyar, A.; Abraham, J.; Norouzi, R. The laboratory study of energy dissi-pation in inclined drops equipped with a screen. J. Appl. Water Eng. Res. 2020, 1–10. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Bagherzadeh, M.; Esmaeeli, R.; Norouzi, R.; Abraham, J. Study of the performance of support vector machine for predicting vertical drop hydraulic parameters in the presence of dual horizontal screens. Water Supply 2020, 21, 217–231. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Bagherzadeh, M.; Ghaderi, A.; Di Francesco, S.; Asl, M.M. Experimental investigation of gabion inclined drops as a sustainable solution for hydraulic energy loss. Ain Shams Eng. J. 2021. [Google Scholar] [CrossRef]
- Esen, I.I.; Alhumoud, J.M.; Hannan, K.A. Energy Loss at a Drop Structure with a Step at the Base. Water Int. 2004, 29, 523–529. [Google Scholar] [CrossRef]
- Rajaratnam, N.; Chamani, M.R. Energy loss at drops. J. Hydraul. Res. 1995, 33, 373–384. [Google Scholar] [CrossRef]
- Kabiri-Samani, A.; Bakhshian, E.; Chamani, M. Flow characteristics of grid drop-type dissipators. Flow Meas. Instrum. 2017, 54, 298–306. [Google Scholar] [CrossRef]
- Sharif, M.; Kabiri-Samani, A. Flow regimes at grid drop-type dissipators caused by changes in tail-water depth. J. Hydraul. Res. 2018, 56, 505–516. [Google Scholar] [CrossRef]
- Moore, W.L. Energy Loss at the Base of a Free Overfall. Trans. Am. Soc. Civ. Eng. 1943, 108, 1343–1360. [Google Scholar] [CrossRef]
- Paik, J.; Escauriaza, C.; Sotiropoulos, F. Coherent structure dynamics in turbulent flows past in-stream structures: Some insights gained via numerical simulation. J. Hydraul. Eng. 2010, 136, 981–993. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Sadeghfam, S.; Ghahramanzadeh, A. Three-dimensional numerical investigation of flow through screens as energy dissipators. Can. J. Civ. Eng. 2017, 44, 850–859. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Asl, M.M.; Razmi, S.; Norouzi, R.; Abraham, J. Experimental investigation of the effect of dual horizontal screens on the hydraulic performance of a vertical drop. Int. J. Environ. Sci. Technol. 2020, 17, 2927–2936. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Ghaderi, A.; Di Francesco, S.; Khajei, N. Experimental study of the effect of horizontal screen diameter on hydraulic parameters of vertical drop. Water Supply 2021. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Aminvash, E.; Ghaderi, A.; Abraham, J.; Bagherzadeh, M. SVM Performance for Predicting the Effect of Horizontal Screen Diameters on the Hydraulic Parameters of a Vertical Drop. Appl. Sci. 2021, 11, 4238. [Google Scholar] [CrossRef]
- Ghaderi, A.; Dasineh, M.; Aristodemo, F.; Aricò, C. Numerical Simulations of the Flow Field of a Submerged Hydraulic Jump over Triangular Macroroughnesses. Water 2021, 13, 674. [Google Scholar] [CrossRef]
- Ghaderi, A.; Abbasi, S.; Abraham, J.; Azamathulla, H.M. Efficiency of Trapezoidal Labyrinth Shaped stepped spillways. Flow Meas. Instrum. 2020, 72, 101711. [Google Scholar] [CrossRef]
- Akoz, M.S.; Soydan, N.G.; Simsek, O. Numerical validation of open channel flow over a curvilinear broad-crested weir. Prog. Comput. Fluid Dyn. Int. J. 2016, 16, 364. [Google Scholar] [CrossRef]
- Ghaderi, A.; Dasineh, M.; Aristodemo, F.; Ghahramanzadeh, A. Characteristics of free and submerged hydraulic jumps over different macroroughnesses. J. Hydroinformatics 2020, 22, 1554–1572. [Google Scholar] [CrossRef]
- Lai, Y.G.; Wu, K. A Three-Dimensional Flow and Sediment Transport Model for Free-Surface Open Channel Flows on Unstructured Flexible Meshes. Fluids 2019, 4, 18. [Google Scholar] [CrossRef]
- Ghaderi, A.; Abbasi, S. Experimental and Numerical Study of the Effects of Geometric Appendance Elements on Energy Dissipation over Stepped Spillway. Water 2021, 13, 957. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Aminvash, E.; Esmaeli, R.; Sadeghfam, S.; Abraham, J. Experimental and numerical investigation for energy dissipation of supercritical flow in sudden contractions. J. Groundw. Sci. Eng. 2020, 8, 396–406. [Google Scholar]
- Ghaderi, A.; Abbasi, S.; Di Francesco, S. Numerical Study on the Hydraulic Properties of Flow over Different Pooled Stepped Spillways. Water 2021, 13, 710. [Google Scholar] [CrossRef]
- Yakhot, V.; Orszag, S.A.; Thangam, S.; Gatski, T.B.; Speziale, C.G. Development of turbulence models for shear flows by a double expansion technique. Phys. Fluids A Fluid Dyn. 1992, 4, 1510–1520. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Ghaderi, A.; Akhtari, A.; Di Francesco, S. On the Effect of Block Roughness in Ogee Spill-ways with Flip Buckets. Fluids 2020, 5, 182. [Google Scholar] [CrossRef]
- Ghaderi, A.; Daneshfaraz, R.; Dasineh, M.; Di Francesco, S. Energy Dissipation and Hydraulics of Flow over Trapezoidal–Triangular Labyrinth Weirs. Water 2020, 12, 1992. [Google Scholar] [CrossRef]
- Abbasi, S.; Fatemi, S.; Ghaderi, A.; Di Francesco, S. The Effect of Geometric Parameters of the Antivortex on a Triangular Labyrinth Side Weir. Water 2020, 13, 14. [Google Scholar] [CrossRef]
- Shamloo, H.; Rajaratnam, N.; Katopodis, C. Hydraulics of simple habitat structures. J. Hydraul. Res. 2001, 39, 351–366. [Google Scholar] [CrossRef]
- Sousa, J.L.; Carter, B.J.; Ingraffea, A.R. Numerical simulation of 3D hydraulic fracture using Newtonian and power-law fluids. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 1265–1271. [Google Scholar]
- Bradbrook, K.F.; Lane, S.N.; Richards, K.S. Numerical simulation of three-dimensional, time-averaged flow structure at river channel confluences. Water Resour. Res. 2000, 36, 2731–2746. [Google Scholar] [CrossRef]
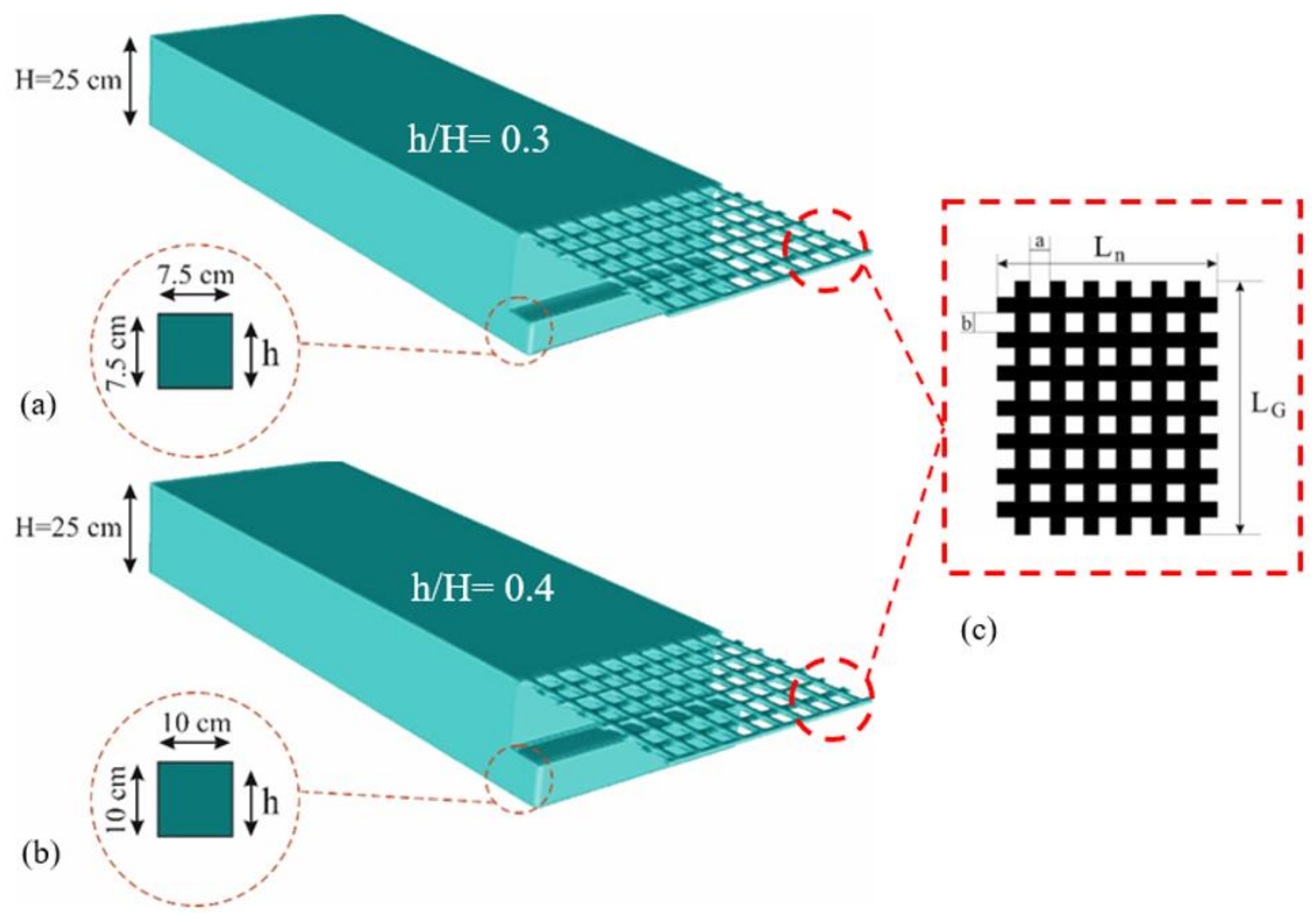



| Dissipator | N4 × 4 | N6 × 6 |
|---|---|---|
| a (cm) | 4 | 6 |
| b (cm) | 4 | 6 |
| Ln (cm) | 60 | 60 |
| LG (cm) | 40 | 40 |
| a/b | 1.0 | 1.0 |
| ɛ (%) | 44 | 56 |
| h/H | Dissipator Size (cm) | Variables | ||||
|---|---|---|---|---|---|---|
| yd (cm) | yc (cm) | Q (Lit/s) | Frd | Reu (×104) | ||
| Without step | - | 2.00–6.00 | 6.00–12.50 | 27.60–64.50 | 3.83–5.20 | 16–39.9 |
| 0.3 | - | 2.60–6.00 | 2.90–3.85 | |||
| 0.4 | 2.45–6.00 | 2.90–3.83 | ||||
| 0.3 | 6 × 6 | 4.65–7.00 | 1.46–2.00 | |||
| 0.4 | 4.73–8.60 | 1.43–2.00 | ||||
| 0.3 | 4 × 4 | 3.45–7.30 | 2.20–2.90 | |||
| 0.4 | 3.95–7.80 | 1.87–2.36 | ||||
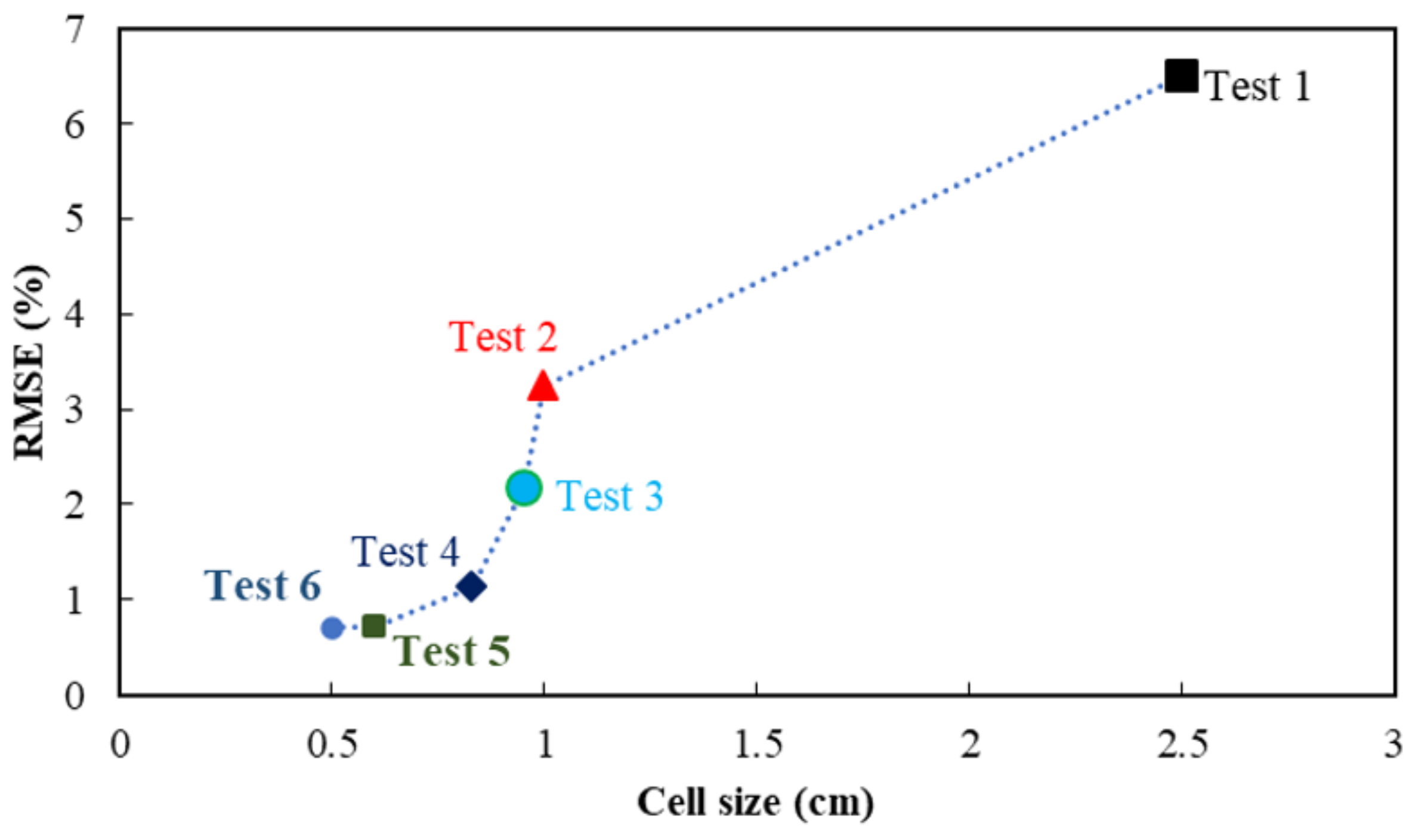
| Test No. | Turbulence Model | Cell Size (cm) | Total Number of Cells | RMSE (%) | R2 |
|---|---|---|---|---|---|
| Test 1 | RNG (k-ɛ) | 2.5 | 46,080 | 6.504 | 0.74 |
| Test 2 | RNG (k-ɛ) | 1.0 | 720,000 | 3.251 | 0.776 |
| Test 3 | RNG (k-ɛ) | 0.95 | 836,136 | 2.186 | 0.802 |
| Test 4 | RNG (k-ɛ) | 0.83 | 1,856,200 | 1.136 | 0.89 |
| Test 5 | RNG (k-ɛ) | 0.60 | 3,350,000 | 0.718 | 0.993 |
| Test 6 | RNG (k-ɛ) | 0.50 | 5,253,000 | 0.708 | 0.995 |
| h/H | Grid Cell Size (mm) | Froude Number (Downstream) |
|---|---|---|
| Without step | Without dissipator | 3.83–2.50 |
| 0.3 | Without dissipator | 2.90–3.85 |
| 0.4 | Without dissipator | 2.90–3.83 |
| 0.3 | 60 × 60 | 1.46–2.00 |
| 0.4 | 1.43–2.00 | |
| 0.3 | 40 × 40 | 2.20–2.90 |
| 0.4 | 1.87–2.36 |
| Dependent Parameters | Constant Parameters | Criteria Parameters | |||||
|---|---|---|---|---|---|---|---|
| a | b | c | d | e | RMSE | R2 | |
| 0.4565 | −0.2808 | 0.3487 | 1.2620 | 1.4209 | 0.0223 | 0.980 | |
| 0.3226 | 0.8063 | 0.6159 | 0.3669 | 1.3921 | 0.0228 | 0.973 | |
| 5.325 | 1.370 | 1.796 | - | 0.0124 | 0.993 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Daneshfaraz, R.; Aminvash, E.; Ghaderi, A.; Kuriqi, A.; Abraham, J. Three-Dimensional Investigation of Hydraulic Properties of Vertical Drop in the Presence of Step and Grid Dissipators. Symmetry 2021, 13, 895. https://doi.org/10.3390/sym13050895
Daneshfaraz R, Aminvash E, Ghaderi A, Kuriqi A, Abraham J. Three-Dimensional Investigation of Hydraulic Properties of Vertical Drop in the Presence of Step and Grid Dissipators. Symmetry. 2021; 13(5):895. https://doi.org/10.3390/sym13050895
Chicago/Turabian StyleDaneshfaraz, Rasoul, Ehsan Aminvash, Amir Ghaderi, Alban Kuriqi, and John Abraham. 2021. "Three-Dimensional Investigation of Hydraulic Properties of Vertical Drop in the Presence of Step and Grid Dissipators" Symmetry 13, no. 5: 895. https://doi.org/10.3390/sym13050895
APA StyleDaneshfaraz, R., Aminvash, E., Ghaderi, A., Kuriqi, A., & Abraham, J. (2021). Three-Dimensional Investigation of Hydraulic Properties of Vertical Drop in the Presence of Step and Grid Dissipators. Symmetry, 13(5), 895. https://doi.org/10.3390/sym13050895










