Some Higher-Degree Lacunary Fractional Splines in the Approximation of Fractional Differential Equations

Abstract

In this article, we begin by introducing two classes of lacunary fractional spline functions by using the Liouville–Caputo fractional Taylor expansion. We then introduce a new higher-order lacunary fractional spline method. We not only derive the existence and uniqueness of the method, but we also provide the error bounds for approximating the unique positive solution. As applications of our fundamental findings, we offer some Liouville–Caputo fractional differential equations (FDEs) to illustrate the practicability and effectiveness of the proposed method. Several recent developments on the the theory and applications of FDEs in (for example) real-life situations are also indicated.

1. Introduction

In recent years, the subject of fractional calculus (that is, the calculus of integrals and derivatives of any arbitrary real or complex order) has gained considerable popularity and importance due mainly to its demonstrated applications in the mathematical modelling of numerous seemingly diverse and widespread real-life problems in the fields of mathematical, physical, engineering and statistical sciences. Such operators of fractional-order derivatives as (for example) the Riemann–Liouville fractional derivative and the Liouville–Caputo fractional derivative are found to be potentially useful in the mathematical modelling of many of these problems (see, for example, [1,2,3,4,5,6,7]).

Motivated essentially by some recent developments (see, for example, [8]; see also [9,10] as well as the references to the related earlier works cited therein), we introduce and investigate here two classes of higher-order lacunary fractional spline functions based upon the Liouville–Caputo fractional derivative. For this purpose, we make use of a (presumably new) lacunary fractional spline method in order to investigate the above-mentioned classes of higher-order lacunary fractional spline functions by applying the Liouville–Caputo fractional Taylor expansion. We not only prove the existence and uniqueness of the method on each of the classes, but we also find the error bounds of the method via the modulus of continuity. Furthermore, with a view to illustrating our theoretical results, we successfully solve several Liouville–Caputo fractional differential eauations (FDEs) by using the method which we have introduced in this paper. Furthermore, finally, we graphically illustrate the numerical solutions which are presented here.

2. Definitions and Preliminaries

In this section, we revisit and recall all of the needed concepts and results involving fractional calculus and integral equations. We choose to divide this section into the following subsections.

2.1. Fractional Calculus

There are many definitions of fractional integrals and fractional derivatives, so it is always necessary to specify which definition is being used by us. In this article, we need the left Riemann–Liouville (L-RL) fractional integral and fractional derivative, as well as the left Liouville–Caputo (L-LC) derivative, which are defined as follows.

Definition 1

([11,12,13]). For any $L^1$ function Υ defined on $[b_1,b_2]$, the νth L-RL fractional integral of $\mathsf{{\rm Y}}\left(\eta \right)$ is defined for $\Re \left(\nu \right)>0$ as follows:

$${{}^{\mathrm{RL}}\mathcal{I}}_{b_1+}^{\nu }\mathsf{{\rm Y}}\left(\eta \right):=\frac{1}{\mathsf{\Gamma }\left(\nu \right)}{\int }_{b_1}^{\eta }{(\eta -\xi )}^{\nu -1}\mathsf{{\rm Y}}\left(\xi \right)\mathrm{d}\xi (\eta \in [b_1,b_2]).$$

(1)

For any $C^n$ function Υ defined on $[b_1,b_2]$, the νth L-RL and L-LC fractional derivatives of $\mathsf{{\rm Y}}\left(\eta \right)$ are defined for $n-1\leqq \Re \left(\nu \right)<n(n\in \mathbb{N})$ as follows:

$$\begin{array}{c}\hfill {{}^{\mathrm{RL}}\mathcal{D}}_{b_1+}^{\nu }\mathsf{{\rm Y}}\left(\eta \right):=\frac{{\mathrm{d}}^n}{\mathrm{d}{\eta }^n}{{}^{\mathrm{RL}}\mathcal{I}}_{b_1+}^{n-\nu }\mathsf{{\rm Y}}\left(\eta \right)(\eta \in [b_1,b_2])\end{array}$$

(2)

and

$$\begin{array}{c}\hfill {{}^{\mathrm{LC}}\mathcal{D}}_{b_1+}^{\nu }\mathsf{{\rm Y}}\left(\eta \right):={{}^{\mathrm{RL}}\mathcal{I}}_{b_1+}^{n-\nu }\frac{{\mathrm{d}}^n}{\mathrm{d}{\eta }^n}\mathsf{{\rm Y}}\left(\eta \right)(\eta \in [b_1,b_2]),\end{array}$$

(3)

respectively, $\mathbb{N}$ being the set of positive integers.

Lemma 1

(see [11,12,13,14]). Let $\mu >-1,\eta >b_1$ and $\Re \left(\nu \right)>0$.Then the L-RL fractional integral of the power function satisfies the following result:

$${{}^{\mathrm{RL}}\mathcal{I}}_{b_1+}^{\nu }{(\eta -b_1)}^{\mu }=\frac{\mathsf{\Gamma }(\mu +1)}{\mathsf{\Gamma }(\mu +1+\nu )}{(\eta -b_1)}^{\mu +\nu }.$$

Moreover, the L-RL and L-LC fractional derivatives of a constant $\mathfrak{C}$ are given by

$$\begin{array}{c}\hfill {{}^{\mathrm{RL}}\mathcal{D}}_{b_1+}^{\nu }\mathfrak{C}=\mathfrak{C}\frac{\mathsf{\Gamma }(\mu +1)}{\mathsf{\Gamma }(1-\nu )}{(\eta -b_1)}^{-\nu }(0<\nu \leqq 1)\end{array}$$

and

$$\begin{array}{c}\hfill {{}^{\mathrm{LC}}\mathcal{D}}_{b_1+}^{\nu }\mathfrak{C}=0(0<\nu \leqq 1),\end{array}$$

respectively.

Definition 2

(see [15,16]). Let $\mathsf{\Omega }\subset \mathbb{R}$ denote an interval with $b_1\in \mathsf{\Omega }$ and $b_1\leqq \eta$ for all $\eta \in \mathsf{\Omega }$. Furthermore, let $\delta \in {\mathbb{R}}^{+}$. We then define the sets ${{}_{b_1}\mathbf{I}}_{\delta }$ and ${{}_{b_1}\mathbf{D}}_{\delta }$ of functions as follows:

$$\begin{array}{c}\hfill {{}_{b_1}\mathbf{I}}_{\delta }=\left\{\mathsf{{\rm Y}}:\mathsf{{\rm Y}}\left(\eta \right)\in C\left(\mathsf{\Omega }\right)\mathrm{and}{{}^{\mathrm{RL}}\mathcal{I}}_{b_1+}^{\delta }\mathsf{{\rm Y}}\left(\eta \right)\mathit{exists}\mathit{and}\mathit{is}\mathit{finite}\mathit{in}\mathsf{\Omega }\right\}\end{array}$$

and

$$\begin{array}{c}\hfill {{}_{b_1}\mathbf{D}}_{\delta }=\left\{\mathsf{{\rm Y}}:\mathsf{{\rm Y}}\left(\eta \right)\in C\left(\mathsf{\Omega }\right)\mathrm{and}{{}^{\mathrm{LC}}\mathcal{D}}_{b_1+}^{\delta }\mathsf{{\rm Y}}\left(\eta \right)\mathit{exists}\mathit{and}\mathit{is}\mathit{finite}\mathit{in}\mathsf{\Omega }\right\}.\end{array}$$

Theorem 1

(see [15,16]). Let $\delta \in (0,1]$ and $\ell \in \mathbb{N}$. Furthermore, let $\mathsf{{\rm Y}}\left(\eta \right)$ be a continuous function on $[b_1,b_2]$ satisfying the following conditions:

(1)

${{}^{\mathrm{LC}}\mathcal{D}}_{b_1+}^{m\delta }\mathsf{{\rm Y}}\in C\left([b_1,b_2]\right)$ and ${{}^{\mathrm{LC}}\mathcal{D}}_{b_1+}^{m\delta }\mathsf{{\rm Y}}\in {{}_{b_1}\mathbf{I}}_{\delta }\left([b_1,b_2]\right)$ for all $m=1,2,\cdots ,\ell$.

(2)

${{}^{\mathrm{LC}}\mathcal{D}}_{b_1+}^{(\ell +1)\delta }\mathsf{{\rm Y}}\left(\eta \right)$ is continuous on $[b_1,b_2]$.

Then, for each $\eta \in [b_1,b_2],$

$$\begin{array}{c}\hfill \mathsf{{\rm Y}}\left(\eta \right)=\sum _{m=0}^{\ell }{{}^{\mathrm{LC}}\mathcal{D}}_{b_1+}^{m\delta }\mathsf{{\rm Y}}\left(a\right)\frac{{(\eta -b_1)}^{m\delta }}{\mathsf{\Gamma }(m\delta +1)}+R_{\ell }(\eta ,b_1),\end{array}$$

where

$$R_{\ell }(\eta ,b_1)=\frac{{(\eta -b_1)}^{(\ell +1)\delta }}{\mathsf{\Gamma }\left(\right(\ell +1)\delta +1)}{{}^{\mathrm{LC}}\mathcal{D}}_{b_1+}^{(\ell +1)\delta }\mathsf{{\rm Y}}\left(\xi \right)(a\leqq \xi \leqq \eta ).$$

Definition 3.

(see [17]). Let $\mathsf{{\rm Y}}:\mathcal{I}\subset \mathbb{R}\to \mathbb{R}$ be a continuous function. Then, the modulus of a continuity of Υ is defined by

$$\begin{array}{c}\hfill {\omega }_{\delta }\left(h\right):=\omega (\delta ;\mathsf{{\rm Y}})=\underset{\left|h\right|<\delta }{sup}|\mathsf{{\rm Y}}(\eta +h)-\mathsf{{\rm Y}}\left(\eta \right)|,\forall \eta \in \mathcal{I}.\end{array}$$

2.2. Formulation of the Problem

Given the mesh points: $\mathsf{\Delta }:0={\eta }_0<{\eta }_1<\cdots <{\eta }_n=1$, with ${\eta }_i=\frac{i}{n},i=0,1,\cdots ,n-1,$$h=\frac{1}{n}$, and the real numbers ${\left\{{\mathsf{{\rm Y}}}_i,{{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}{\mathsf{{\rm Y}}}_i,{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_i,{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_i\right\}}_{i=0}^n$ associated with the knots, our problem is to find s in a suitable class such that

$${\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^m{s}_{\mathsf{\Delta }}\left({\eta }_i\right)={\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^m{\mathsf{{\rm Y}}}_i(i=0,1,\cdots ,n;m=0,1,2,4).$$

(4)

3. The First Class of Lacunary Fractional Spline

This section is being divided into the following subsections.

3.1. Existence and Uniqueness

Here, in this subsection, we define the class $S_{n,6}^{2\delta }$ of lacunary fractional spline functions as detailed below.

Definition 4.

We say that $s_{\mathsf{\Delta }}$ in $S_{n,6}^{2\delta }$ if the following conditions are fulfilled:

$${{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta m}{s}_{\mathsf{\Delta }}\in C\left([0,1]\right)\left(m=0,1;\delta =\frac{1}{2}\right)$$

(5)

and

$$s_{\mathsf{\Delta }}=\left\{\begin{array}{cc}{\displaystyle \sum _{i=0}^6}{a}_i{(\eta -{\eta }_i)}^{i/2}\hfill & \left(\eta \in [{\eta }_i,{\eta }_{i+1}]\forall i=0,1,\cdots ,n-2\right)\hfill \\ \\ {\displaystyle \sum _{i=0}^7}{a}_i{(\eta -{\eta }_i)}^{i/2}\hfill & \left(\eta \in [{\eta }_{n-1},{\eta }_n]\right).\hfill \end{array}\right.$$

(6)

We construct $s_{\mathsf{\Delta }}$ in order that it is a solution of (4) for functions ${\left(D\right)}^{\delta m}{S}_{\mathsf{\Delta }}\in C\left([0,1]\right)$ for $m=0,1,\cdots ,6$ and $\delta =\frac{1}{2}$. For this purpose, we set

$$s_{\mathsf{\Delta }}=\left\{\begin{array}{cc}{s}_i\left(\eta \right)\hfill & \left({\eta }_i\leqq \eta \leqq {\eta }_{i+1};\forall i=0,1,\cdots ,n-2\right)\hfill \\ \\ s_{n-1}\left(\eta \right)\hfill & \left({\eta }_{n-1}\leqq \eta \leqq {\eta }_n\right).\hfill \end{array}\right.$$

Owing to (4) and (6), we can write

$$\begin{array}{c}{s}_i\left(\eta \right)=\sum _{m=0}^2\frac{1}{\mathsf{\Gamma }(\frac{1}{2}m+1)}{(\eta -{\eta }_i)}^{\frac{1}{2}m}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^m{\mathsf{{\rm Y}}}_i+\frac{4}{3\sqrt{\pi }}{(\eta -{\eta }_i)}^{3/2}{a}_{i,\frac{3}{2}}\hfill \\ \hfill +\frac{1}{2}{(\eta -{\eta }_i)}^2{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_i+\sum _{m=5}^6\frac{1}{\mathsf{\Gamma }(\frac{1}{2}m+1)}{(\eta -{\eta }_i)}^{\frac{1}{2}m}{a}_{i,\frac{m}{2}},\end{array}$$

(7)

and

$$\begin{array}{c}{s}_{n-1}\left(\eta \right)=\sum _{m=0}^2\frac{1}{\mathsf{\Gamma }(\frac{1}{2}m+1)}{(\eta -{\eta }_{n-1})}^{\frac{1}{2}m}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^m{\mathsf{{\rm Y}}}_{n-1}+\frac{4}{3\sqrt{\pi }}{(\eta -{\eta }_{n-1})}^{3/2}{a}_{n-1,\frac{3}{2}}\hfill \\ \hfill +\frac{1}{2}{(\eta -{\eta }_{n-1})}^2{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_{n-1}+\sum _{m=5}^7\frac{1}{\mathsf{\Gamma }(\frac{1}{2}m+1)}{(\eta -{\eta }_{n-1})}^{\frac{1}{2}m}{a}_{n-1,\frac{m}{2}}.\end{array}$$

(8)

Then the coefficients are determined by

$$\begin{array}{cc}\hfill a_{i,\frac{3}{2}}& =\frac{\sqrt{\pi }(415{\beta }_i^1+128{\beta }_i^2-543{\beta }_i^3)}{4(60\pi +287)},\hfill \\ \hfill h{a}_{i,\frac{5}{2}}& =-\frac{\frac{225}{2}({\beta }_i^3-{\beta }_i^1)\pi +240{\beta }_i^1+240{\beta }_i^2-128{\beta }_i^3}{\sqrt{\pi }(60\pi +287)},\hfill \\ \hfill h^{\frac{3}{2}}{a}_{i,3}& =6{\beta }_i^3-5{\beta }_i^1+\frac{4(415{\beta }_i^1+128{\beta }_i^2-543{\beta }_i^3)}{60\pi +287},\hfill \end{array}$$

$$\begin{array}{cc}\hfill a_{n-1,\frac{3}{2}}& =\frac{2776}{3485}{\beta }_{n-1}^1+\frac{8}{697}{\beta }_{n-1}^2-\frac{5612}{3485}{\beta }_{n-1}^3+\frac{2796}{3485}{\beta }_{n-1}^4,\hfill \\ \hfill h{a}_{n-1,\frac{5}{2}}& =\frac{1}{697}(-2064{\beta }_{n-1}^1-90{\beta }_{n-1}^2+4960{\beta }_{n-1}^3-2806{\beta }_{n-1}^4),\hfill \\ \hfill h^{\frac{3}{2}}{a}_{n-1,3}& =\frac{32}{697\sqrt{\pi }}(-3{\beta }_{n-1}^1+10{\beta }_{n-1}^2-9{\beta }_{n-1}^3+4858{\beta }_{n-1}^4),\hfill \\ \hfill h^2{a}_{n-1,\frac{7}{2}}& =\frac{1}{697}(3168{\beta }_{n-1}^1-105{\beta }_{n-1}^2-7224{\beta }_{n-1}^3+4858{\beta }_{n-1}^4),\hfill \end{array}$$

where

$$\begin{array}{cc}\hfill h^{\frac{3}{2}}{\beta }_i^1& =6\left({\mathsf{{\rm Y}}}_{i+1}-{\mathsf{{\rm Y}}}_i-\frac{2}{\sqrt{\pi }}{{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}{\mathsf{{\rm Y}}}_i-h{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_i-\frac{1}{2}{h}^2{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_i\right),\hfill \\ \hfill h{\beta }_i^2& =\frac{15\sqrt{\pi }}{8}\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}{\mathsf{{\rm Y}}}_{i+1}-{{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}{\mathsf{{\rm Y}}}_i-h^{1/2}\frac{2}{\sqrt{\pi }}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_i-\frac{4}{3\sqrt{\pi }}{h}^{3/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_i\right),\hfill \\ \hfill h^{\frac{1}{2}}{\beta }_i^3& =2\left({\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_{i+1}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_i-\frac{2}{\sqrt{\pi }}{h}^{1/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_i\right),\hfill \end{array}$$

$$\begin{array}{cc}\hfill h^{\frac{3}{2}}{\beta }_{n-1}^1& =\frac{105\sqrt{\pi }}{16}\left({\mathsf{{\rm Y}}}_n-{\mathsf{{\rm Y}}}_{n-1}-h^{1/2}\frac{2}{\sqrt{\pi }}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_{n-1}-\frac{1}{2}{h}^2{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_{n-1}\right),\hfill \\ \hfill h{\beta }_{n-1}^2& =6({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}{\mathsf{{\rm Y}}}_n-{{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}{\mathsf{{\rm Y}}}_{n-1}-h^{1/2}\frac{2}{\sqrt{\pi }}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_{n-1}\hfill \\ \hfill & -\frac{4}{3\sqrt{\pi }}{h}^{3/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_{n-1}),\hfill \\ \hfill h^{\frac{1}{2}}{\beta }_{n-1}^3& =\frac{15\sqrt{\pi }}{8}\left({\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_n-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_{n-1}-\frac{2}{\sqrt{\pi }}{h}^{1/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_{n-1}\right),\hfill \end{array}$$

and

$$\begin{array}{c}\hfill {\beta }_{n-1}^4=\frac{3\sqrt{\pi }}{4}{h}^{\frac{1}{2}}\left({\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_n-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_{n-1}\right).\end{array}$$

Hence, clearly, we have proved Theorem 2 below.

Theorem 2.

For a uniform partition Δ of the interval $I=[0,1],$ there exists a unique fractional spline function $s_{\mathsf{\Delta }}\in S_{n,6}^{2\delta }$ which is a solution of the problem involving (4).

3.2. Error Bounds

In this subsection, we first give the following main lemmas to work on our error bound theorem.

Lemma 2.

Assume that Υ satisfies the hypothesis of Theorem 1 for $m=j=3,5,6$. For each $i=0,1,\cdots ,n-2,$ it is asserted that

$$\begin{array}{cc}\hfill \left|a_{i,\frac{j}{2}}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^j\mathsf{{\rm Y}}\left({\eta }_i\right)\right|& \leqq c_{i,\frac{j}{2}}{h}^{3-\frac{j}{2}}{\omega }_{6\delta }\left(h\right)(j=3,5),\hfill \\ \hfill \left|a_{i,3}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_i\right)\right|& \leqq 6\left(\frac{60\pi +694}{60\pi +287}\right){\omega }_{6\delta }\left(h\right)\left({\eta }_i\leqq \eta \leqq {\eta }_{i+1}\right),\hfill \end{array}$$

where

$$c_{i,\frac{3}{2}}=\frac{543\sqrt{\pi }}{4(60\pi +287)}$$

and

$$c_{i,\frac{5}{2}}=\frac{225\pi +960}{2\sqrt{\pi }(60\pi +287)}.$$

Proof. 

Suppose that ${\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\in C\left(I\right)$. Then, by using Theorem 1, we have

$$\begin{array}{cc}\hfill {\beta }_i^1& =\frac{8}{\sqrt{\pi }}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_i+\frac{16h}{5\sqrt{\pi }}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_i+h^{3/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\xi }_i\right),\hfill \\ \hfill {\beta }_i^2& =\frac{15\sqrt{\pi }}{8}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_i+\frac{15\sqrt{\pi }h}{16}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_i+h^{3/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_i\right),\hfill \end{array}$$

and

$$\begin{array}{cc}\hfill {\beta }_i^3& =\frac{4}{\sqrt{\pi }}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_i+\frac{8h}{3\sqrt{\pi }}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_i+h^{3/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_i\right),\hfill \end{array}$$

where ${\eta }_i<{\xi }_i,{\eta }_i,{\theta }_i<{\eta }_{i+1}$ for $i=0,1,\cdots ,n-2$. We thus find that

$$\begin{array}{c}\left|a_{i,\frac{3}{2}}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_i\right|=\left|\frac{\sqrt{\pi }}{4(60\pi +287)}\left[415{\beta }_i^1+128{\beta }_i^2-543{\beta }_i^3\right]-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_i\right|\hfill \\ =\frac{\sqrt{\pi }}{4(60\pi +287)}{h}^{3/2}|415\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\xi }_i\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_i\right)\right]\\ \hfill +128\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_i\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_i\right)\right]|\leqq \frac{543\sqrt{\pi }}{4(60\pi +287)}{h}^{3/2}{\omega }_{6\delta }\left(h\right),\end{array}$$

$$\begin{array}{c}\left|a_{i,\frac{5}{2}}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_i\right|=\frac{h^{3/2}{h}^{-1}}{\sqrt{\pi }(60\pi +287)}|\frac{225}{2}\pi \left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_i\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\xi }_i\right)\right]\hfill \\ +240\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\xi }_i\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_i\right)\right]+240\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_i\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_i\right)\right]|\\ \hfill \leqq \frac{225\pi +960}{2\sqrt{\pi }(60\pi +287)}{h}^{1/2}{\omega }_{6\delta }\left(h\right),\end{array}$$

and, finally, we get

$$\begin{array}{c}\left|a_{i,3}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6{\mathsf{{\rm Y}}}_i\right|=\left|6{\beta }_i^3-5{\beta }_i^1+\frac{4(415{\beta }_i^1+128{\beta }_i^2-543{\beta }_i^3)}{60\pi +287}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6{\mathsf{{\rm Y}}}_i\right|\hfill \\ \leqq 5\left|{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_i\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\xi }_i\right)\right|+\frac{1660}{60\pi +287}\left|{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\xi }_i\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_i\right)\right|\\ \hfill +\frac{512}{60\pi +287}\left|{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_i\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_i\right)\right|\leqq 6\left(\frac{60\pi +694}{60\pi +287}\right){\omega }_{6\delta }\left(h\right),\end{array}$$

The proof of Lemma 2 is completed. □

Lemma 3.

Assume that Υ satisfies the hypothesis of Theorem 1 for $m=0,1,\cdots ,6$. The following estimates are valid:

$$\begin{array}{cc}\hfill \left|a_{n-1,\frac{j}{2}}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^j\mathsf{{\rm Y}}\left({\eta }_{n-1}\right)\right|& \leqq c_{n-1,\frac{j}{2}}{h}^{3-\frac{j}{2}}{\omega }_{6\delta }\left(h\right)(j=3,5),\hfill \\ \hfill \left|a_{n-1,3}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left(\eta \right)\right|& \leqq \left(\frac{1072}{697}\right){\omega }_{6\delta }\left(h\right)\left({\eta }_{n-1}\leqq \eta \leqq {\eta }_n\right),\hfill \\ \hfill \left|a_{n-1,\frac{7}{2}}\right|& \leqq \left(\frac{14217\sqrt{\pi }}{1394}\right)h^{-1/2}{\omega }_{6\delta }\left(h\right),\hfill \end{array}$$

where

$$c_{n-1,\frac{3}{2}}=\frac{63135\sqrt{\pi }}{41820}$$

and

$$c_{n-1,\frac{5}{2}}=\frac{4650\sqrt{\pi }}{697}.$$

Proof. 

Let ${\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^m\mathsf{{\rm Y}}\in C\left(I\right)$ for $m=0,1,\cdots ,6$. Then, by applying Theorem 1, we obtain

$$\begin{array}{cc}\hfill {\beta }_{n-1}^1& =\frac{35}{4}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_{n-1}+\frac{7}{2}h{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_{n-1}+\frac{105\sqrt{\pi }}{96}{h}^{3/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\xi }_{n-1}\right),\hfill \\ \hfill {\beta }_{n-1}^2& =6{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_{n-1}+3h{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_{n-1}+\frac{16}{5\sqrt{\pi }}{h}^{3/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_{n-1}\right),\hfill \end{array}$$

$$\begin{array}{cc}\hfill {\beta }_{n-1}^3& =\frac{15}{4}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_{n-1}+\frac{5}{2}h{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_{n-1}+\frac{15\sqrt{\pi }}{16}{h}^{3/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_{n-1}\right),\hfill \end{array}$$

and

$$\begin{array}{cc}\hfill {\beta }_{n-1}^4& =\frac{3}{2}h{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_{n-1}+\frac{3\sqrt{\pi }}{4}{h}^{3/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\lambda }_{n-1}\right),\hfill \end{array}$$

where ${\eta }_{n-1}<{\xi }_{n-1},{\eta }_{n-1},{\theta }_{n-1},{\lambda }_{n-1}<{\eta }_n$. We thus find that

$$\begin{array}{c}\left|a_{n-1,\frac{3}{2}}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_{n-1}\right|=|\frac{1}{3485}\left[2776{\beta }_{n-1}^1+40{\beta }_{n-1}^2-5612{\beta }_{n-1}^3+2796{\beta }_{n-1}^4\right]\hfill \\ -{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_{n-1}|\\ \leqq \frac{\sqrt{\pi }}{\left(3485\right)\left(12\right)}{h}^{3/2}|36435\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\xi }_{n-1}\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_{n-1}\right)\right]\\ +1536\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_{n-1}\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_{n-1}\right)\right]\\ \hfill +25164\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_{n-1}\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_{n-1}\right)\right]|\leqq \frac{63135\sqrt{\pi }}{41820}{h}^{3/2}{\omega }_{6\delta }\left(h\right),\end{array}$$

$$\begin{array}{c}\left|a_{n-1,\frac{5}{2}}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_{n-1}\right|=\frac{h^{1/2}}{697}\frac{\sqrt{\pi }}{16}|-36120{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\xi }_{n-1}\right)\hfill \\ -4608{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_{n-1}\right)+74400{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_{n-1}\right)\\ \hfill -33672{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\lambda }_{n-1}\right)|\leqq \frac{4650\sqrt{\pi }}{697}{h}^{1/2}{\omega }_{6\delta }\left(h\right),\end{array}$$

$$\begin{array}{c}\left|a_{n-1,3}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6{\mathsf{{\rm Y}}}_{n-1}\right|=|\frac{32}{697\sqrt{\pi }}\left[-3{\beta }_{n-1}^1+10{\beta }_{n-1}^2-9{\beta }_{n-1}^3+2{\beta }_{n-1}^4\right]\hfill \\ -{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6{\mathsf{{\rm Y}}}_{n-1}|\\ \leqq \frac{1}{697}|105\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_{n-1}\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\xi }_{n-1}\right)\right]\\ +276\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_{n-1}\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\theta }_{n-1}\right)\right]\\ +673\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_{n-1}\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_{n-1}\right)\right]\\ \hfill +24\left[{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\lambda }_{n-1}\right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6\mathsf{{\rm Y}}\left({\eta }_{n-1}\right)\right]\leqq \frac{1072}{697}{\omega }_{6\delta }\left(h\right),\end{array}$$

and, finally, we get

$$\begin{array}{cc}\hfill \left|a_{n-1,\frac{7}{2}}\right|& =\frac{h^{-2}}{697}|3168{\beta }_{n-1}^1-105{\beta }_{n-1}^2-7224{\beta }_{n-1}^3+4858{\beta }_{n-1}^4|\leqq \frac{14217\sqrt{\pi }}{1349}{h}^{-1/2}{\omega }_{6\delta }\left(h\right).\hfill \end{array}$$

This completes the proof of Lemma 3. □

We now state and proof our result on the error bound.

Theorem 3

(Error bound). Let $s_{\mathsf{\Delta }}\in S_{n,6}^{2\delta }$ be the solution of the problem involving (4). Then, for any

$${\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^m\mathsf{{\rm Y}}\in C\left([0,1]\right)\left(m=0,1,\cdots ;\delta =\frac{1}{2}\right),$$

the following error bound holds true:

$$\left|{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^m{s}_i-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^m\mathsf{{\rm Y}}\left(\eta \right)\right|\leqq c_{i,\delta m}{h}^{3-\delta m}{\omega }_{6\delta }\left(h\right)(i=0,1,\cdots ,n-1),$$

(9)

where ${\omega }_{6\delta }\left(h\right)$ is the modulus of continuity of ${\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^6\mathsf{{\rm Y}}\left(\eta \right)$ and the constants $c_{i,\delta m}$ are given in Table 1.

Table 1. The constants $c_{i,\delta m}$.

	${\mathit{c}}_{\mathit{i},0}$	${\mathit{c}}_{\mathit{i},\frac{1}{2}}$	${\mathit{c}}_{\mathit{i},1}$	${\mathit{c}}_{\mathit{i},\frac{3}{2}}$	${\mathit{c}}_{\mathit{i},2}$	${\mathit{c}}_{\mathit{i},\frac{5}{2}}$	${\mathit{c}}_{\mathit{i},3}$
$0\leqq i\leqq n-2$	$\frac{3909}{491}$	$\frac{707}{704}$	$\frac{1549}{145}$	$\frac{8776}{889}$	$\frac{1997}{163}$	$\frac{4378}{323}$	$\frac{3775}{339}$
$i=n-1$	$\frac{716}{97}$	$\frac{2211}{122}$	$\frac{2308}{107}$	$\frac{8861}{934}$	$\frac{19,850}{697}$	$\frac{22,051}{697}$	$\frac{15,289}{697}$

Proof. 

Let us first assume that ${\eta }_i\leqq \eta \leqq {\eta }_{i+1}$ for $i=0,1,\cdots ,n-2$. Then, from Equation (7) and by using Theorem 1, we have

$$\begin{array}{cc}\hfill & s_i^{\left(m\right)}\left(\eta \right)-{\mathsf{{\rm Y}}}^{\left(m\right)}\left(\eta \right)=\hfill \\ \hfill & \left\{\begin{array}{c}\frac{{(\eta -{\eta }_i)}^{3-\frac{m}{2}}}{\mathsf{\Gamma }\left(4-\frac{m}{2}\right)}\left[a_{i,\frac{3}{2}}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^3{\mathsf{{\rm Y}}}_i\right]+\frac{{(\eta -{\eta }_i)}^{5-\frac{m}{2}}}{\mathsf{\Gamma }\left(6-\frac{m}{2}\right)}\left[a_{i,\frac{5}{2}}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_i\right]\hfill \\ +\frac{{(\eta -{\eta }_i)}^{6-\frac{m}{2}}}{\mathsf{\Gamma }\left(7-\frac{m}{2}\right)}\left[a_{i,3}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6{\mathsf{{\rm Y}}}_i\right]\hfill & (m=0,1,\cdots ,3);\hfill \\ \frac{{(\eta -{\eta }_i)}^{5-\frac{m}{2}}}{\mathsf{\Gamma }\left(6-\frac{m}{2}\right)}\left[a_{i,\frac{5}{2}}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^5{\mathsf{{\rm Y}}}_i\right]+\frac{{(\eta -{\eta }_i)}^{6-\frac{m}{2}}}{\mathsf{\Gamma }\left(7-\frac{m}{2}\right)}\left[a_{i,3}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6{\mathsf{{\rm Y}}}_i\right]\hfill & (m=4,5);\hfill \\ a_{i,3}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^6{\mathsf{{\rm Y}}}_i\hfill & (m=6).\hfill \end{array}\right.\hfill \end{array}$$

Now, by making use of the estimates asserted by Lemma 2, we can obtain the result for $i=0,1,\cdots ,n-2$. In addition, by making use of Lemma 3, we can similarly obtain the rest of the results for ${\eta }_{n-1}\leqq \eta \leqq {\eta }_n$. This completes the proof of Theorem 3. □

4. The Second Class of Lacunary Fractional Spline

Here, in this connection, we consider the class of the lacunary fractional spline functions $S_{n,7}^{(2\delta ,g)}$ and we denote this class of functions by $G_{\mathsf{\Delta }}\left(\eta \right)$ such that

$$\begin{array}{cc}\hfill & {\left(D\right)}^{\delta m}{G}_{\mathsf{\Delta }}\in C\left([0,1]\right)\left(m=0,1,2;\delta =\frac{1}{2}\right),\hfill \end{array}$$

(10)

$$\begin{array}{cc}\hfill & G_{\mathsf{\Delta }}=\sum _{i=0}^7{a}_i{(\eta -{\eta }_i)}^{i/2}\left(\eta \in [{\eta }_i,{\eta }_{i+1}]\forall i=0,1,2,\cdots ,n-1\right),\hfill \end{array}$$

(11)

$$\begin{array}{cc}\hfill & {\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{G}_{\mathsf{\Delta }}({\eta }_i+0)=G_{\mathsf{\Delta }}({\eta }_i-0)(i=0,1,\cdots ,n-1).\hfill \end{array}$$

(12)

We construct $G_{\mathsf{\Delta }}$ such that it is a solution of the problem involving (4) for

$${\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^m\mathsf{{\rm Y}}\in C\left([0,1]\right)(m=0,1,\cdots ,7).$$

For this purpose, we set

$$G_{\mathsf{\Delta }}=G_i\left(\eta \right)(i=0,1,\cdots ,n-1).$$

Owing to (4) and (11), we can write

$$\begin{array}{c}{G}_i\left(\eta \right)=\sum _{m=0}^2\frac{1}{\mathsf{\Gamma }(\frac{1}{2}m+1)}{(\eta -{\eta }_i)}^{\frac{1}{2}m}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^m{\mathsf{{\rm Y}}}_i+\frac{4}{3\sqrt{\pi }}{(\eta -{\eta }_i)}^{3/2}{b}_{i,\frac{3}{2}}\hfill \\ \hfill +\frac{1}{2}{(\eta -{\eta }_i)}^2{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_i+\sum _{m=5}^7\frac{1}{\mathsf{\Gamma }(\frac{1}{2}m+1)}{(\eta -{\eta }_i)}^{\frac{1}{2}m}{b}_{i,\frac{m}{2}}.\end{array}$$

(13)

The constants can be determined, after using the conditions (10) to (12), as follows:

$$\begin{array}{cc}\hfill b_{i,\frac{3}{2}}& =\frac{1}{3485}(2776{\beta }_i^1+40{\beta }_i^2-5612{\beta }_i^3+2796{\beta }_i^4),\hfill \\ \hfill h{b}_{i,\frac{5}{2}}& =\frac{1}{697}(-2064{\beta }_i^1-90{\beta }_i^2+4960{\beta }_i^3-2806{\beta }_i^4),\hfill \\ \hfill h^{\frac{3}{2}}{b}_{i,3}& =\frac{32}{697\sqrt{\pi }}(-3{\beta }_i^1+10{\beta }_i^2-9{\beta }_i^3+4858{\beta }_i^4),\hfill \\ \hfill h^2{b}_{i,\frac{7}{2}}& =\frac{1}{697}(3168{\beta }_i^1-105{\beta }_i^2-7224{\beta }_i^3+4858{\beta }_i^4),\hfill \end{array}$$

where

$$\begin{array}{cc}\hfill h^{\frac{3}{2}}{\beta }_i^1& =\frac{105\sqrt{\pi }}{16}\left({\mathsf{{\rm Y}}}_{i+1}-{\mathsf{{\rm Y}}}_i-h^{1/2}\frac{2}{\sqrt{\pi }}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_i-\frac{1}{2}{h}^2{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_i\right),\hfill \\ \hfill h{\beta }_i^2& =6\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}{\mathsf{{\rm Y}}}_{i+1}-{{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}{\mathsf{{\rm Y}}}_i-h^{1/2}\frac{2}{\sqrt{\pi }}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_i-\frac{4}{3\sqrt{\pi }}{h}^{3/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_i\right),\hfill \\ \hfill h^{\frac{1}{2}}{\beta }_i^3& =\frac{15\sqrt{\pi }}{8}\left({\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_{i+1}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^2{\mathsf{{\rm Y}}}_i-\frac{2}{\sqrt{\pi }}{h}^{1/2}{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_i\right),\hfill \\ \hfill {\beta }_i^4& =\frac{3\sqrt{\pi }}{4}{h}^{\frac{1}{2}}\left({\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_{i+1}-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{1/2}\right)}^4{\mathsf{{\rm Y}}}_i\right).\hfill \end{array}$$

One can now observe that $G_{\mathsf{\Delta }}$ is a unique element of $S_{n,7}^{(2\delta ,g)}$ which is the solution of the interpolation problem (4). Then, by following the same technique which we used for the previous class, we are easily led the following theorem.

Theorem 4

(Error bound). Let $s_{\mathsf{\Delta }}\in S_{n,6}^{(2\delta ,g)}$ be the solution of the interpolation problem involving (4). Then, for any

$${\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^m\mathsf{{\rm Y}}\in C\left([0,1]\right),m=0,1,\cdots ,7,\delta =\frac{1}{2},\mathrm{and}i=0,1,\cdots ,n-1,$$

we have

$$\left|{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^m{s}_i-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^m\mathsf{{\rm Y}}\left(\eta \right)\right|\leqq c_{i,\delta m}{h}^{3.5-\delta m}{\omega }_{6\delta }\left(h\right),$$

(14)

where ${\omega }_{7\delta }\left(h\right)$ is the modulus of continuity of ${\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^7\mathsf{{\rm Y}}\left(\eta \right)$, and the constants $c_{i,\delta m}$ are given in Table 2.

Table 2. The constants $c_{i,\delta m}$.

	${\mathit{c}}_{\mathit{i},0}$	${\mathit{c}}_{\mathit{i},\frac{1}{2}}$	${\mathit{c}}_{\mathit{i},1}$	${\mathit{c}}_{\mathit{i},\frac{3}{2}}$	${\mathit{c}}_{\mathit{i},2}$	${\mathit{c}}_{\mathit{i},\frac{5}{2}}$	${\mathit{c}}_{\mathit{i},3}$	${\mathit{c}}_{\mathit{i},\frac{7}{2}}$
$0\leqq i\leqq n-1$	$\frac{213}{49}$	$\frac{2894}{403}$	$\frac{5719}{530}$	$\frac{7565}{514}$	$\frac{5951}{350}$	$\frac{5277}{278}$	$\frac{808}{61}$	$\frac{8026}{697}$

5. Applications

In what follows, we provide some numerical examples to verify the validity of the proposed method.

Example 1.

Consider the following FDE [8]:

$${{}^{\mathrm{LC}}\mathcal{D}}_{0+}^2\mathsf{{\rm Y}}\left(\eta \right)+2{{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\mathsf{{\rm Y}}\left(\eta \right)+\mathsf{{\rm Y}}\left(\eta \right)=2\eta +{\displaystyle \frac{4}{\mathsf{\Gamma }(4-\delta )}}{\eta }^{3-\delta }+{\displaystyle \frac{1}{3}}{\eta }^3(0<\delta \leqq 1),$$

(15)

subject to the initial condition given by

$$\mathsf{{\rm Y}}\left(0\right)={\mathsf{{\rm Y}}}^{\prime }\left(0\right)=0.$$

It is easily verified that the exact solution of this problem is

$$\mathsf{{\rm Y}}\left(\eta \right)=\frac{1}{3}{\eta }^3.$$

The maximal absolute errors and their fractional derivatives, which are obtained for $0\leqq \eta \leqq 1$, are shown in Table 3. We note that

$$\left|e^{m\delta }\left(\eta \right)\right|=\left|{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^m{S}_i\left(\eta \right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^m\mathsf{{\rm Y}}\left(\eta \right)\right|,$$

Table 3. The observed maximum absolute errors for Example 1.

Methods	h	$\left|\mathit{e}\right(\mathit{\eta }\left)\right|$	$|{\mathit{e}}^{\mathit{\delta }}\left(\mathit{\eta }\right)|$	$|{\mathit{e}}^{2\mathit{\delta }}\left(\mathit{\eta }\right)|$	$|{\mathit{e}}^{3\mathit{\delta }}\left(\mathit{\eta }\right)|$	$|{\mathit{e}}^{4\mathit{\delta }}\left(\mathit{\eta }\right)|$
	$0.1$	$4865\times {10}^{-2}$	$9856\times {10}^{-1}$	$1211\times {10}^0$	$1145\times {10}^0$	$2100\times {10}^0$
Our method	$0.01$	$1001\times {10}^{-6}$	$9987\times {10}^{-5}$	$6413\times {10}^{-4}$	$1120\times {10}^{-2}$	$8997\times {10}^{-2}$
	$0.001$	$3544\times {10}^{-9}$	$8770\times {10}^{-8}$	$9948\times {10}^{-6}$	$5189\times {10}^{-5}$	$1102\times {10}^{-3}$
	$0.1$	$4211\times {10}^{-1}$	$1139\times {10}^0$	$3832\times {10}^0$	$1560\times {10}^0$	$2546\times {10}^0$
Method in [8]	$0.01$	$4211\times {10}^{-4}$	$3603\times {10}^{-3}$	$3832\times {10}^{-2}$	$4936\times {10}^{-2}$	$2546\times {10}^{-1}$
	$0.001$	$1331\times {10}^{-6}$	$1139\times {10}^{-5}$	$3832\times {10}^{-4}$	$1560\times {10}^{-3}$	$2546\times {10}^{-2}$

where

$$m=0,1,\cdots ,4,i=0,1,\cdots ,n-1,\mathrm{and}\delta =\frac{1}{2}.$$

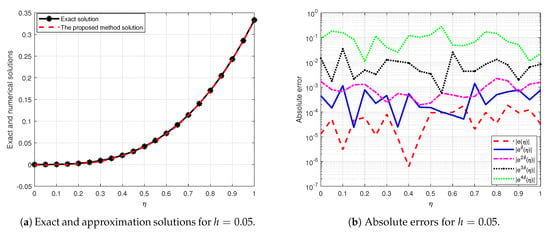
Figure 1a shows the exact and approximation solutions for $h=0.05$. Also, the absolute error is shown in Figure 1b for $h=0.05$.

Figure 1. Plot illustrations for Example 1.

Example 2.

Consider the following FDE [8]:

$${{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\mathsf{{\rm Y}}\left(\eta \right)={\eta }^4-\frac{1}{2}{\eta }^3+\frac{24}{\mathsf{\Gamma }(4-\delta )}{\eta }^{3-\delta }+\frac{3}{\mathsf{\Gamma }(5-\delta )}{\eta }^{4-\delta }-\mathsf{{\rm Y}}\left(\eta \right)(0<\delta <1),$$

(16)

With the initial condition $\mathsf{{\rm Y}}\left(0\right)=0$. The exact solution is given by

$$\mathsf{{\rm Y}}\left(\eta \right)={\eta }^4-\frac{1}{2}{\eta }^3.$$

Together with

$$\left|e^{m\delta }\left(\eta \right)\right|=\left|{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^m{S}_i\left(\eta \right)-{\left({{}^{\mathrm{LC}}\mathcal{D}}_{0+}^{\delta }\right)}^m\mathsf{{\rm Y}}\left(\eta \right)\right|,$$

where

$$m=0,1,\cdots ,4,i=0,1,\cdots ,n-1\mathrm{and}\delta =\frac{1}{2},$$

we have derived the maximal absolute errors and their fractional derivatives. These results are shown in Table 4.

Table 4. The observed maximum absolute errors for Example 2.

Methods	h	$\left|\mathit{e}\right(\mathit{\eta }\left)\right|$	$|{\mathit{e}}^{\mathit{\delta }}\left(\mathit{\eta }\right)|$	$|{\mathit{e}}^{2\mathit{\delta }}\left(\mathit{\eta }\right)|$	$|{\mathit{e}}^{3\mathit{\delta }}\left(\mathit{\eta }\right)|$	$|{\mathit{e}}^{4\mathit{\delta }}\left(\mathit{\eta }\right)|$
	$0.1$	$9929\times {10}^{-2}$	$5186\times {10}^{-1}$	$2464\times {10}^0$	$1063\times {10}^1$	$3883\times {10}^1$
Our method	$0.01$	$9929\times {10}^{-6}$	$1640\times {10}^{-4}$	$2464\times {10}^{-3}$	$3361\times {10}^{-2}$	$3883\times {10}^{-1}$
	$0.001$	$9929\times {10}^{-10}$	$5186\times {10}^{-8}$	$2464\times {10}^{-6}$	$1063\times {10}^{-4}$	$3883\times {10}^{-3}$
	$0.1$	$7412\times {10}^0$	$1487\times {10}^0$	$3659\times {10}^0$	$8398\times {10}^1$	$2700\times {10}^1$
Method in [8]	$0.01$	$7412\times {10}^{-3}$	$4702\times {10}^{-2}$	$3659\times {10}^{-1}$	$2655\times {10}^0$	$2700\times {10}^0$
	$0.001$	$7412\times {10}^{-6}$	$1487\times {10}^{-4}$	$3659\times {10}^{-3}$	$8398\times {10}^{-2}$	$2700\times {10}^{-1}$

Figure 2a shows the exact and approximation solutions for $h=0.05$. Also, the absolute error is shown in Figure 2b for $h=0.05$.

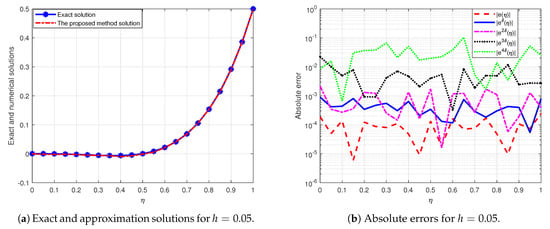
Figure 2. Plot illustrations for Example 2.

All of the tables, which we have presented in this paper, show that our method is more accurate than the existing fractional spline methods.

6. Conclusions

The content of this article can be summarized as follows:

• Two classes of higher-order lacunary fractional spline functions are introduced.
• A new lacunary fractional spline method is obtained for the above-mentioned classes by using the Liouville–Caputo fractional Taylor expansion.
• The existence and uniqueness of the method on each of the classes is proved.
• The error bounds of the method is shown via the modulus of continuity.
• Some Liouville–Caputo FDEs are solved by using the new method in order to illustrate our theoretical results.
• The numerical solutions are also illustrated graphically.

In conclusion, we remark that we have chosen to include some recent works (see, for example, [9,10,18,19,20,21] which will attract researchers and motivate them for further developments along these lines.