Probing the Nuclear Equation of State from the Existence of a ∼2.6 M⊙ Neutron Star: The GW190814 Puzzle
Abstract
1. Introduction
2. The MDI-APR Model and the Rapidly Rotating Neutron Star
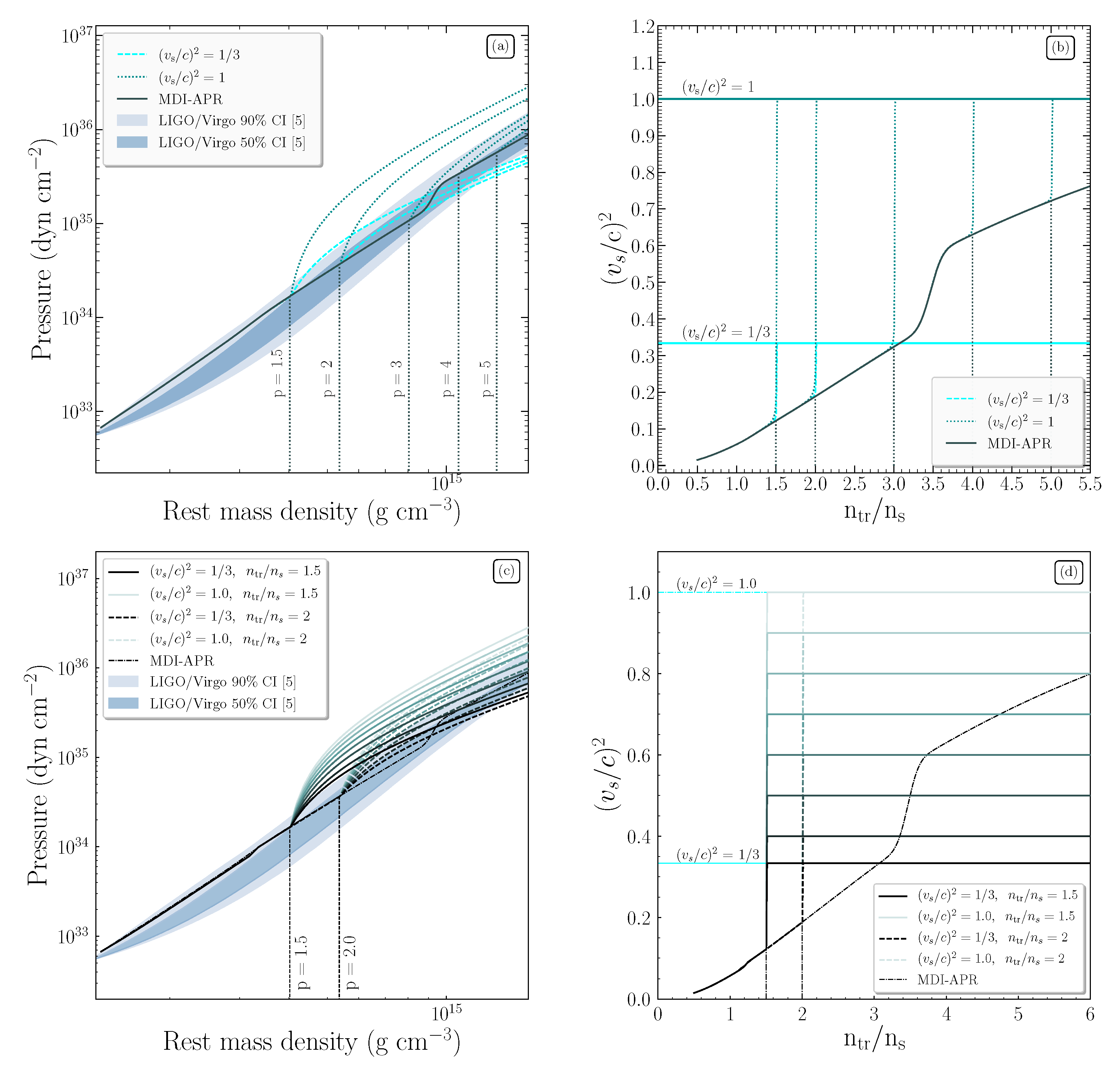
3. Speed of Sound Formalism and Stiffness of Equation of State
4. Tidal Deformability
5. Results and Discussion
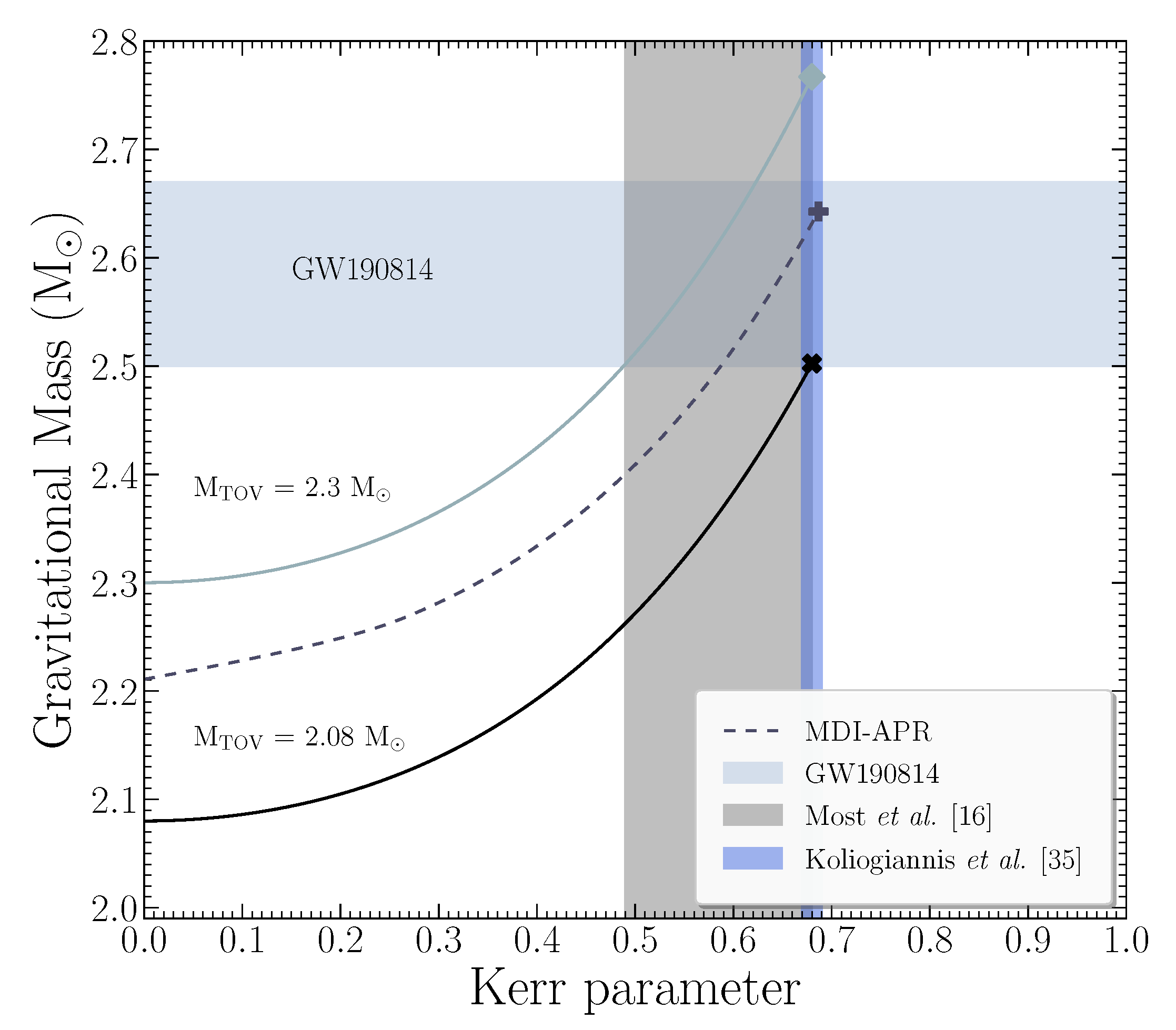
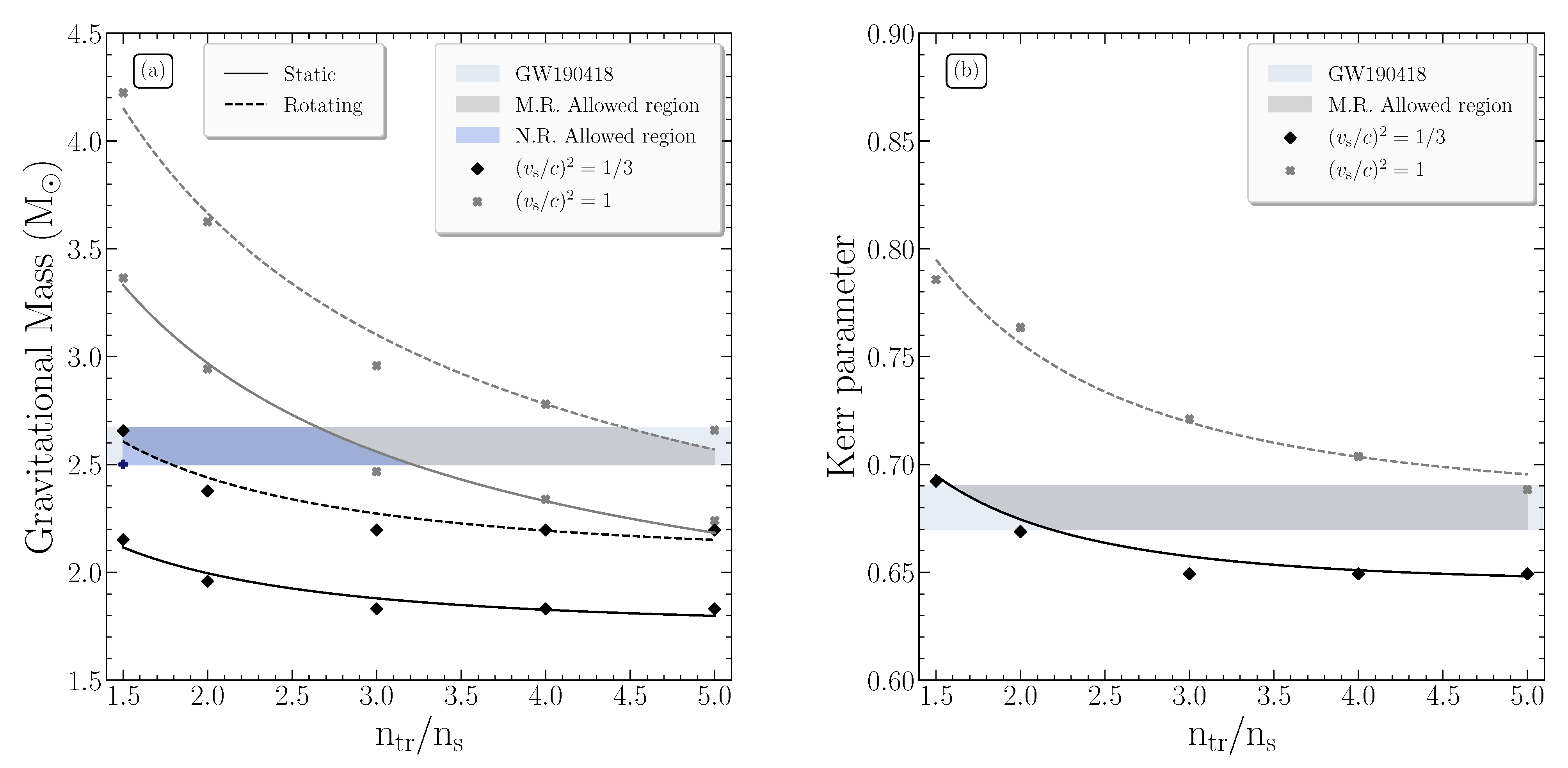
5.1. Slow/Rapid Rotation: Implications to Neutron Star Properties
5.2. Tidal Effects and Speed of Sound: A Very Massive Neutron Star Hypothesis
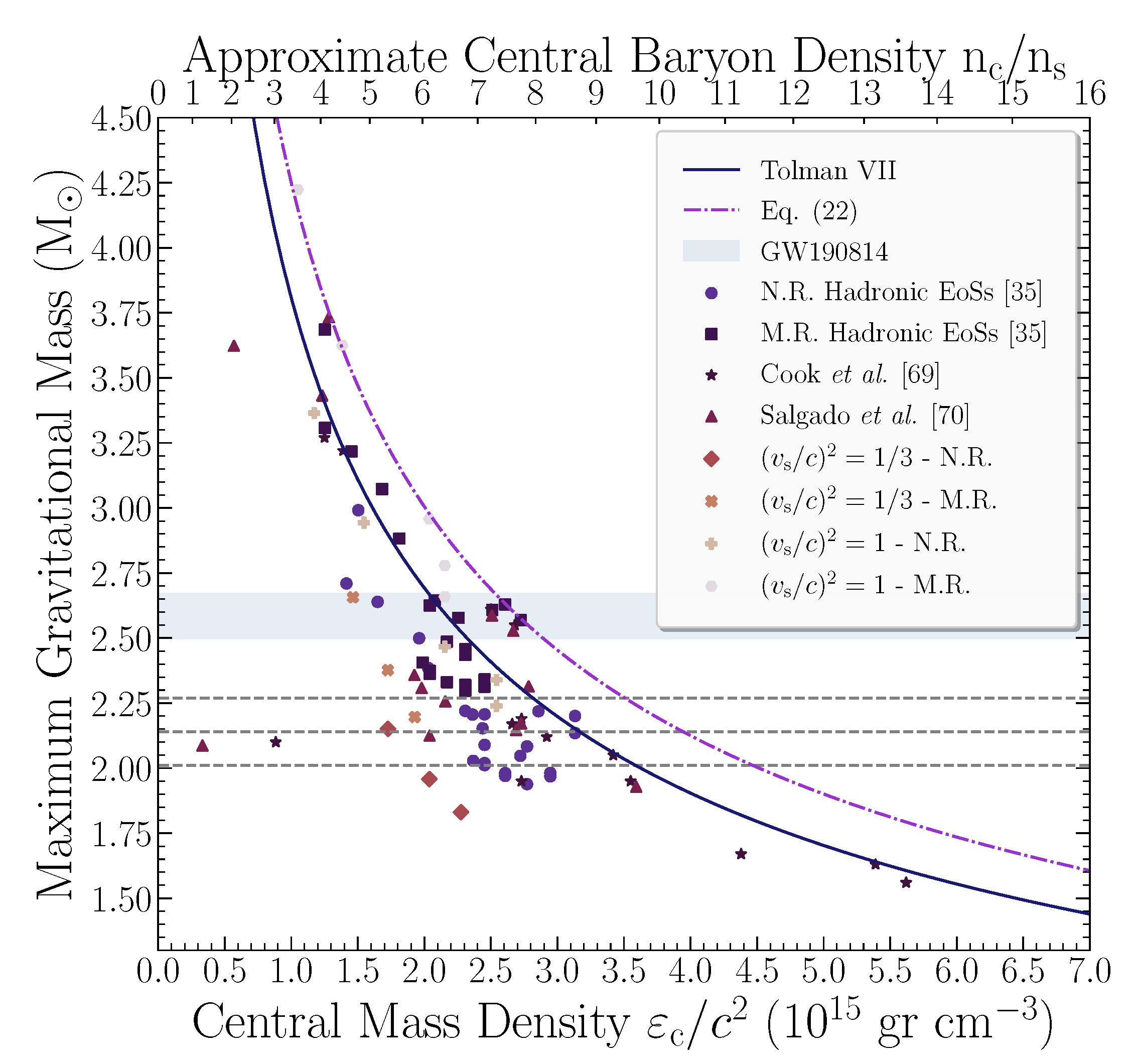
5.2.1. Isolated Non-Rotating Neutron Star
5.2.2. A Very Massive Neutron Star Component
6. Concluding Remarks
7. Materials and Methods
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| EoS | Equation of state |
| NS | Neutron star |
| QCD | Quantum chromodynamics |
| MDI | Momentum dependent interaction |
| APR | Akmal, Pandharipande and Ravenhall |
| SNM | Symmetric Nuclear Matter |
| N.R. | Non-rotating configuration |
| M.R. | Maximally-rotating configuration |
References
- Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; Agathos, M.; et al. GW190814: Gravitational Waves from the Coalescence of a 23 Solar Mass Black Hole with a 2.6 Solar Mass Compact Object. Astrophys. J. Lett. 2020, 896, L44. [Google Scholar] [CrossRef]
- Datta, S.; Phukon, K.S.; Bose, S. Recognizing black holes in gravitational-wave observations: Telling apart impostors in mass-gap binaries. arXiv 2020, arXiv:gr-qc/2004.05974. [Google Scholar]
- Alsing, J.; Silva, H.O.; Berti, E. Evidence for a maximum mass cut-off in the neutron star mass distribution and constraints on the equation of state. Mon. Not. R. Astron. Soc. 2018, 478, 1377–1391. [Google Scholar] [CrossRef]
- Farr, W.M.; Chatziioannou, K. A Population-Informed Mass Estimate for Pulsar J0740+6620. Res. Notes AAS 2020, 4, 65. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. (The LIGO Scientific Collaboration and the Virgo Collaboration) GW170817: Measurements of Neutron Star Radii and Equation of State. Phys. Rev. Lett. 2018, 121, 161101. [Google Scholar] [CrossRef]
- Tsokaros, A.; Ruiz, M.; Shapiro, S.L. GW190814: Spin and equation of state of a neutron star companion. Astrophys. J. 2020, 905, 48. [Google Scholar] [CrossRef]
- Huang, K.; Hu, J.; Zhang, Y.; Shen, H. The Possibility of the Secondary Object in GW190814 as a Neutron Star. Astrophys. J. 2020, 904, 39. [Google Scholar] [CrossRef]
- Zevin, M.; Spera, M.; Berry, C.; Kalogera, V. Exploring the Lower Mass Gap and Unequal Mass Regime in Compact Binary Evolution. Astrophys. J. Lett. 2020, 899, L1. [Google Scholar] [CrossRef]
- Fattoyev, F.J.; Horowitz, C.J.; Piekarewicz, J.; Reed, B. GW190814: Impact of a 2.6 solar mass neutron star on nucleonic equations of state. Phys. Rev. C 2020, 102, 065805. [Google Scholar] [CrossRef]
- Essick, R.; Landry, P. Discriminating between Neutron Stars and Black Holes with Imperfect Knowledge of the Maximum Neutron Star Mass. Astrophys. J. 2020, 904, 80. [Google Scholar] [CrossRef]
- Safarzadeh, M.; Loeb, A. Formation of Mass Gap Objects in Highly Asymmetric Mergers. Astrophys. J. Lett. 2020, 899, L15. [Google Scholar] [CrossRef]
- Godzieba, D.A.; Radice, D.; Bernuzzi, S. On the maximum mass of neutron stars and GW190814. arXiv 2020, arXiv:astro-ph.HE/2007.10999. [Google Scholar]
- Sedrakian, A.; Weber, F.; Li, J.J. Confronting GW190814 with hyperonization in dense matter and hypernuclear compact stars. Phys. Rev. D 2020, 102, 041301. [Google Scholar] [CrossRef]
- Biswas, B.; Nandi, R.; Bose, S.; Stergioulas, N. GW190814: On the properties of the secondary component of the binary. arXiv 2020, arXiv:astro-ph.HE/2010.02090. [Google Scholar]
- Zhang, N.B.; Li, B.A. GW190814’s Secondary Component with Mass 2.50–2.67 M⊙ as a Superfast Pulsar. Astrophys. J. 2020, 902, 38. [Google Scholar] [CrossRef]
- Most, E.R.; Papenfort, L.J.; Weih, L.R.; Rezzolla, L. A lower bound on the maximum mass if the secondary in GW190814 was once a rapidly spinning neutron star. Mon. Not. R. Astron. Soc. 2020, 499, L82–L86. [Google Scholar] [CrossRef]
- Tan, H.; Hostler-Noronha, J.; Yunes, N. Neutron Star Equation of State in light of GW190814. Phys. Rev. Lett. 2020, 125, 261104. [Google Scholar] [CrossRef]
- Zhang, C.; Mann, R.B. Unified Interacting Quark Matter and its Astrophysical Implications. arXiv 2020, arXiv:astro-ph.HE/2009.07182. [Google Scholar]
- Bombaci, I.; Drago, A.; Logoteta, D.; Pagliara, G.; Vidana, I. Was GW190814 a black hole–strange quark star system? arXiv 2020, arXiv:nucl-th/2010.01509. [Google Scholar]
- Demircik, T.; Ecker, C.; Jarvinen, M. Rapidly Spinning Compact Stars with Deconfinement Phase Transition. arXiv 2020, arXiv:astro-ph.HE/2009.10731. [Google Scholar]
- Cao, Z.; Chen, L.W.; Chu, P.C.; Zhou, Y. GW190814: Circumstantial Evidence for Up-Down Quark Star. arXiv 2020, arXiv:astro-ph.HE/2009.00942. [Google Scholar]
- Dexheimer, V.; Gomes, R.O.; Klahn, T.; Han, S.; Salinas, M. GW190814 as a massive rapidly-rotating neutron star with exotic degrees of freedom. arXiv 2020, arXiv:astro-ph.HE/2007.08493. [Google Scholar]
- Roupas, Z.; Panotopoulos, G.; Lopes, I. QCD color superconductivity in compact stars: Color-flavor locked quark star candidate for the gravitational-wave signal GW190814. arXiv 2020, arXiv:astro-ph.HE/2010.11020. [Google Scholar]
- Rather, I.A.; Usmani, A.A.; Patra, S.K. Hadron-Quark phase transition in the context of GW190814. arXiv 2020, arXiv:nucl-th/2011.14077. [Google Scholar]
- Moffat, J.W. Modified Gravity (MOG) and Heavy Neutron Star in Mass Gap. arXiv 2020, arXiv:gr-qc/2008.04404. [Google Scholar]
- Astashenok, A.V.; Capozziello, S.; Odintsov, S.D.; Oikonomou, V.K. Extended gravity description for the GW190814 supermassive neutron star. Phys. Lett. B 2020, 811, 135910. [Google Scholar] [CrossRef]
- Nunes, R.C.; Coelho, G.G.; Araujo, J.C.N. Weighing massive neutron star with screening gravity: A look on PSR J0740 + 6620 and GW190814 secondary component. Eur. Phys. J. C 2020, 80, 1115. [Google Scholar] [CrossRef]
- Annala, E.; Gorda, T.; Kurkela, A.; Nättilä, J.; Vuorinen, A. Evidence for quark-matter cores in massive neutron stars. Nat. Phys. 2020, 16, 907–910. [Google Scholar] [CrossRef]
- Bombaci, I. The Hyperon Puzzle in Neutron Stars. JPS Conf. Proc. 2017, 17, 101002. [Google Scholar]
- Shapiro, S.L.; Teukolsky, S.A. Black Holes, White Dwarfs, and Neutron Stars; John Wiley and Sons: Hoboken, NJ, USA; New York, NY, USA, 1983. [Google Scholar]
- Glendenning, N.K. Compact Stars: Nuclear Physics, Particle Physics, and General Relativity; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Haensel, P.; Potekhin, A.Y.; Yakovlev, D.G. Neutron Stars 1: Equation of State and Structure; Springer: New York, NY, USA, 2007. [Google Scholar]
- Weinberg, S. Gravitational and Cosmology: Principle and Applications of the General Theory of Relativity; Wiley: New York, NY, USA, 1972. [Google Scholar]
- Zel’dovich, Y.B.; Novikov, I.D. Stars and Relativity; Dover Publications, Inc.: New York, NY, USA, 1971. [Google Scholar]
- Koliogiannis, P.S.; Moustakidis, C.C. Effects of the equation of state on the bulk properties of maximally rotating neutron stars. Phys. Rev. C 2020, 101, 015805. [Google Scholar] [CrossRef]
- Prakash, M.; Bombaci, I.; Prakash, M.; Ellis, P.J.; Lattimer, J.M.; Knorren, R. Composition and structure of protoneutron stars. Phys. Rep. 1997, 280, 1. [Google Scholar] [CrossRef]
- Moustakidis, C.C. Effects of the nuclear equation of state on the r-mode instability and evolution of neutron stars. Phys. Rev. C 2015, 91, 035804. [Google Scholar] [CrossRef]
- Gale, C.; Bertsch, G.; Das Gupta, S. Heavy-ion collision theory with momentum-dependent interactions. Phys. Rev. C 1987, 35, 1666. [Google Scholar] [CrossRef] [PubMed]
- Gale, C.; Welke, G.M.; Prakash, M.; Lee, S.J.; Das Gupta, S. Transverse momenta, nuclear equation of state, and momentum-dependent interactions in heavy-ion collisions. Phys. Rev. C 1990, 41, 1545. [Google Scholar] [CrossRef] [PubMed]
- Akmal, A.; Pandharipande, V.R.; Ravenhall, D.G. Equation of state of nucleon matter and neutron star structure. Phys. Rev. C 1998, 58, 1804. [Google Scholar] [CrossRef]
- Antoniadis, J.; Freire, P.C.; Wex, N.; Tauris, T.M.; Lynch, R.S.; van Kerkwijk, M.H.; Kramer, M.; Bassa, C.; Dhillon, V.S.; Driebe, T.; et al. A Massive Pulsar in a Compact Relativistic Binary. Science 2013, 340, 1233232. [Google Scholar] [CrossRef]
- Cromartie, H.T.; Fonseca, E.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Blumer, H.; Brook, P.R.; DeCesar, M.E.; Dolch, T.; Ellis, J.A.; et al. Relativistic Shapiro delay measurements of an extremely massive millisecond pulsar. Nat. Astron. 2020, 4, 72. [Google Scholar] [CrossRef]
- Linares, M.; Shahbaz, T.; Casares, J. Peering into the Dark Side: Magnesium Lines Establish a Massive Neutron Star in PSR J2215+5135. Astrophys. J. 2018, 859, 54. [Google Scholar] [CrossRef]
- Stergioulas, N. 1996. Available online: http://www.gravity.phys.uwm.edu/rns/ (accessed on 15 December 2020).
- Breu, C.; Rezzolla, L. Maximum mass, moment of inertia and compactness of relativistic stars. Mon. Not. R. Astron. Soc. 2016, 459, 646. [Google Scholar] [CrossRef]
- Margaritis, C.; Koliogiannis, P.S.; Moustakidis, C.C. Speed of sound constraints on maximally rotating neutron stars. Phys. Rev. D 2020, 101, 043023. [Google Scholar] [CrossRef]
- Rhoades, C.E.; Ruffini, R. Maximum Mass of a Neutron Star. Phys. Rev. Lett. 1974, 32, 324. [Google Scholar] [CrossRef]
- Kalogera, V.; Baym, G. The Maximum Mass of a Neutron Star. Astrophys. J. 1996, 470, L61. [Google Scholar] [CrossRef]
- Koranda, S.; Stergioulas, N.; Friedman, J.L. Upper Limits Set by Causality on the Rotation and Mass of Uniformly Rotating Relativistic Stars. Astrophys. J. 1997, 488, 799. [Google Scholar] [CrossRef]
- Chamel, N.; Haensel, P.; Zdunik, J.L.; Fantina, A.F. On the maximum mass of neutron stars. Int. J. Mod. Phys. E 2013, 22, 1330018. [Google Scholar] [CrossRef]
- Podkowka, D.M.; Mendes, R.F.P.; Poisson, E. Trace of the energy-momentum tensor and macroscopic properties of neutron star. Phys. Rev. D 2018, 98, 064057. [Google Scholar] [CrossRef]
- Xia, C.; Zhu, Z.; Zhou, X.; Li, A. Sound velocity in dense stellar matter with strangeness and compact stars. arXiv 2019, arXiv:nucl-th/1906.00826. [Google Scholar]
- Feynman, R.P.; Metropolis, N.; Teller, E. Equations of State of Elements Based on the Generalized Fermi-Thomas Theory. Phys. Rev. 1949, 75, 1561. [Google Scholar] [CrossRef]
- Baym, G.; Pethik, C.; Sutherland, P. The Ground State of Matter at High Densities: Equation of State and Stellar Models. Astrophys. J. 1971, 170, 299. [Google Scholar] [CrossRef]
- Tews, I.; Carlson, J.; Gandolfi, S.; Reddy, S. Constraining the Speed of Sound inside Neutron Stars with Chiral Effective Field Theory Interactions and Observations. Astrophys. J. 2018, 860, 149. [Google Scholar] [CrossRef]
- Postnikov, S.; Prakash, M.; Lattimer, J.M. Tidal Love numbers of neutron and self-bound quark stars. Phys. Rev. D 2010, 82, 024016. [Google Scholar] [CrossRef]
- Baiotti, L. Gravitational waves from neutron star mergers and their relation to the nuclear equation of state. Prog. Part. Nucl. Phys. 2019, 109, 103714. [Google Scholar] [CrossRef]
- Flanagan, E.E.; Hinderer, T. Constraining neutron-star tidal Love numbers with gravitational-wave detectors. Phys. Rev. D 2008, 77, 021502(R). [Google Scholar] [CrossRef]
- Hinderer, T. Tidal Love Numbers of Neutron Stars. Astrophys. J. 2008, 677, 1216. [Google Scholar] [CrossRef]
- Damour, T.; Nagar, A. Relativistic tidal properties of neutron stars. Phys. Rev. D 2009, 80, 084035. [Google Scholar] [CrossRef]
- Hinderer, T.; Lackey, B.D.; Lang, R.N.; Read, J.S. Tidal deformability of neutron stars with realistic equations of state and their gravitational wave signatures in binary inspiral. Phys.Rev. D 2010, 81, 123016. [Google Scholar] [CrossRef]
- Fattoyev, F.J.; Carvajal, J.; Newton, W.G.; Li, B.A. Constraining the high-density behavior of the nuclear symmetry energy with the tidal polarizability of neutron stars. Phys. Rev. C 2013, 87, 015806. [Google Scholar] [CrossRef]
- Lackey, B.D.; Wade, L. Reconstructing the neutron-star equation of state with gravitational-wave detectors from a realistic population of inspiralling binary neutron stars. Phys. Rev. D 2015, 91, 043002. [Google Scholar] [CrossRef]
- Takátsy, J.; Kovács, P. Comment on “Tidal Love numbers of neutron and self-bound quark stars”. Phys. Rev. D 2020, 102, 028501. [Google Scholar] [CrossRef]
- Thorne, K.S. Tidal stabilization of rigidly rotating, fully relativistic neutron stars. Phys. Rev. D 1998, 58, 124031. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. Properties of the Binary Neutron Star Merger GW170817. Phys. Rev. X 2019, 9, 011001. [Google Scholar] [CrossRef]
- Koliogiannis, P.S.; Moustakidis, C.C. Thermodynamical description of hot rapidly rotating neutron stars and neutron stars merger remnant. arXiv 2020, arXiv:astro-ph.HE/2007.10424. [Google Scholar]
- Cook, G.B.; Shapiro, S.L.; Teukolsky, S.A. Rapidly Rotating Neutron Stars in General Relativity: Realistic Equations of State. Astrophys. J. 1994, 424, 823. [Google Scholar] [CrossRef]
- Salgado, M.; Bonazzola, S.; Gourgoulhon, E.; Haensel, P. High precision rotating neutron star models 1: Analysis of neutron star properties. Astron. Astrophys. 1994, 291, 155. [Google Scholar]
- Kanakis-Pegios, A.; Koliogiannis, P.S.; Moustakidis, C.C. Speed of sound constraints from tidal deformability of neutron stars. Phys. Rev. C 2020, 102, 055801. [Google Scholar] [CrossRef]
- Zhao, T.; Lattimer, J.M. Tidal deformabilities and neutron star mergers. Phys. Rev. D 2018, 98, 063020. [Google Scholar] [CrossRef]
- De, S.; Finstad, D.; Lattimer, J.M.; Brown, D.A.; Berger, E.; Biwer, C.M. Tidal Deformabilities and Radii of Neutron Stars from the Observation of GW170817. Phys. Rev. Lett. 2018, 121, 091102. [Google Scholar] [CrossRef]
- Tsang, C.Y.; Tsang, M.B.; Danielewicz, P.; Fattoyev, F.J.; Lynch, W.G. Insights on Skyrme parameters from GW170817. Phys. Lett. B 2019, 796, 1. [Google Scholar] [CrossRef]
- Wei, J.-B.; Lu, J.-J.; Burgio, G.F.; Li, Z.-H.; Schulze, H.-J. Are nuclear matter properties correlated to neutron star observables? Eur. Phys. J. A 2020, 56, 63. [Google Scholar] [CrossRef]
- Raithel, C.A.; Özel, F.; Psaltis, D. Tidal Deformability from GW170817 as a Direct Probe of the Neutron Star Radius. Astrophys. J. Lett. 2018, 857, L23. [Google Scholar] [CrossRef]
- Stergioulas, N.; Friedman, J.L. Comparing Models of Rapidly Rotating Relativistic Stars Constructed by Two Numerical Methods. Astrophys. J. 1995, 444, 306. [Google Scholar] [CrossRef]
- Komatsu, H.; Eriguchi, Y.; Hachisu, I. Rapidly rotating general relativistic stars. I - Numerical method and its application to uniformly rotating polytropes. Mon. Not. R. Astron. Soc. 1989, 237, 355. [Google Scholar] [CrossRef]
- Cook, G.B.; Shapiro, S.L.; Teukolsky, S.A. Rapidly Rotating Polytropes in General Relativity. Astrophys. J. 1994, 422, 227. [Google Scholar] [CrossRef]

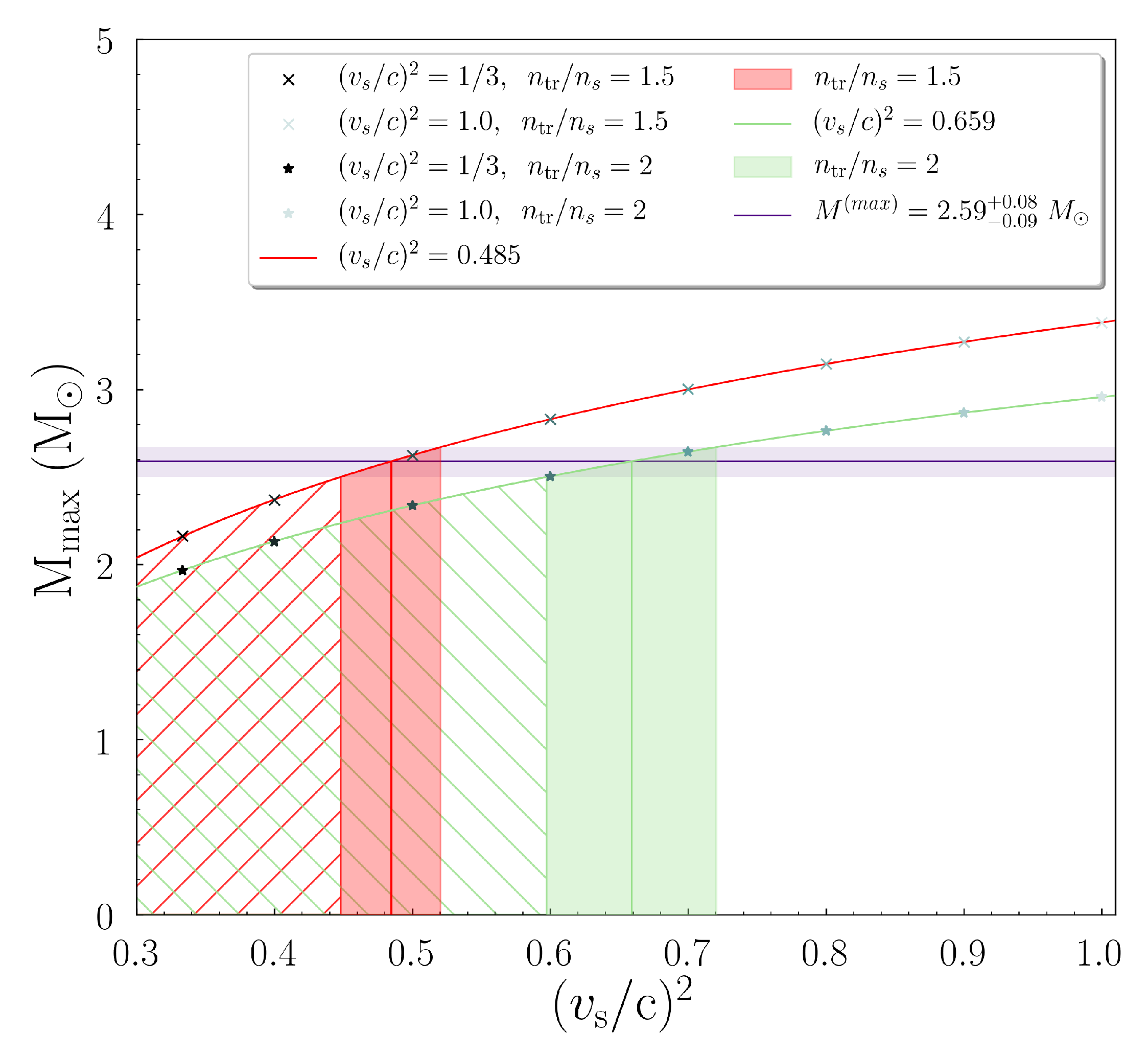
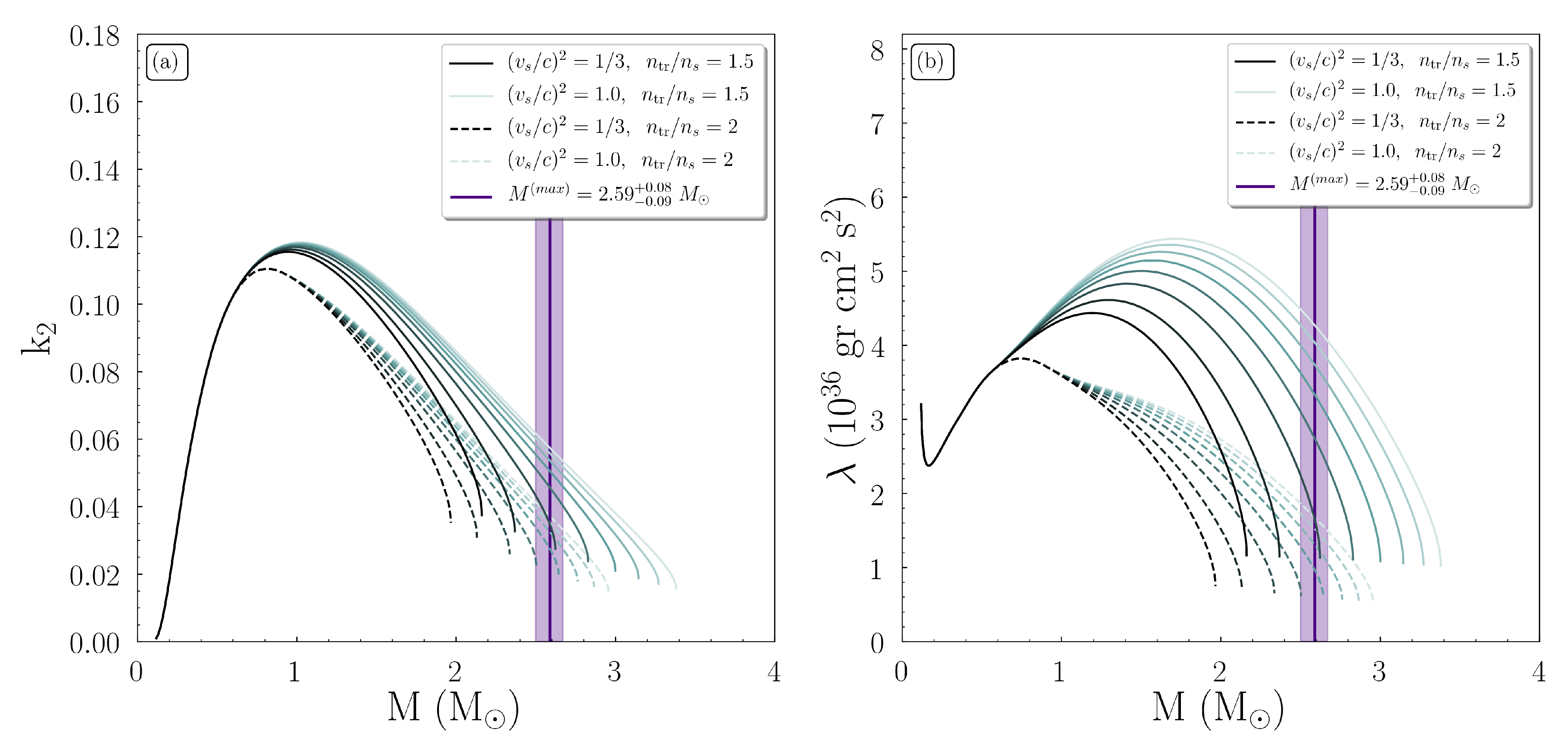

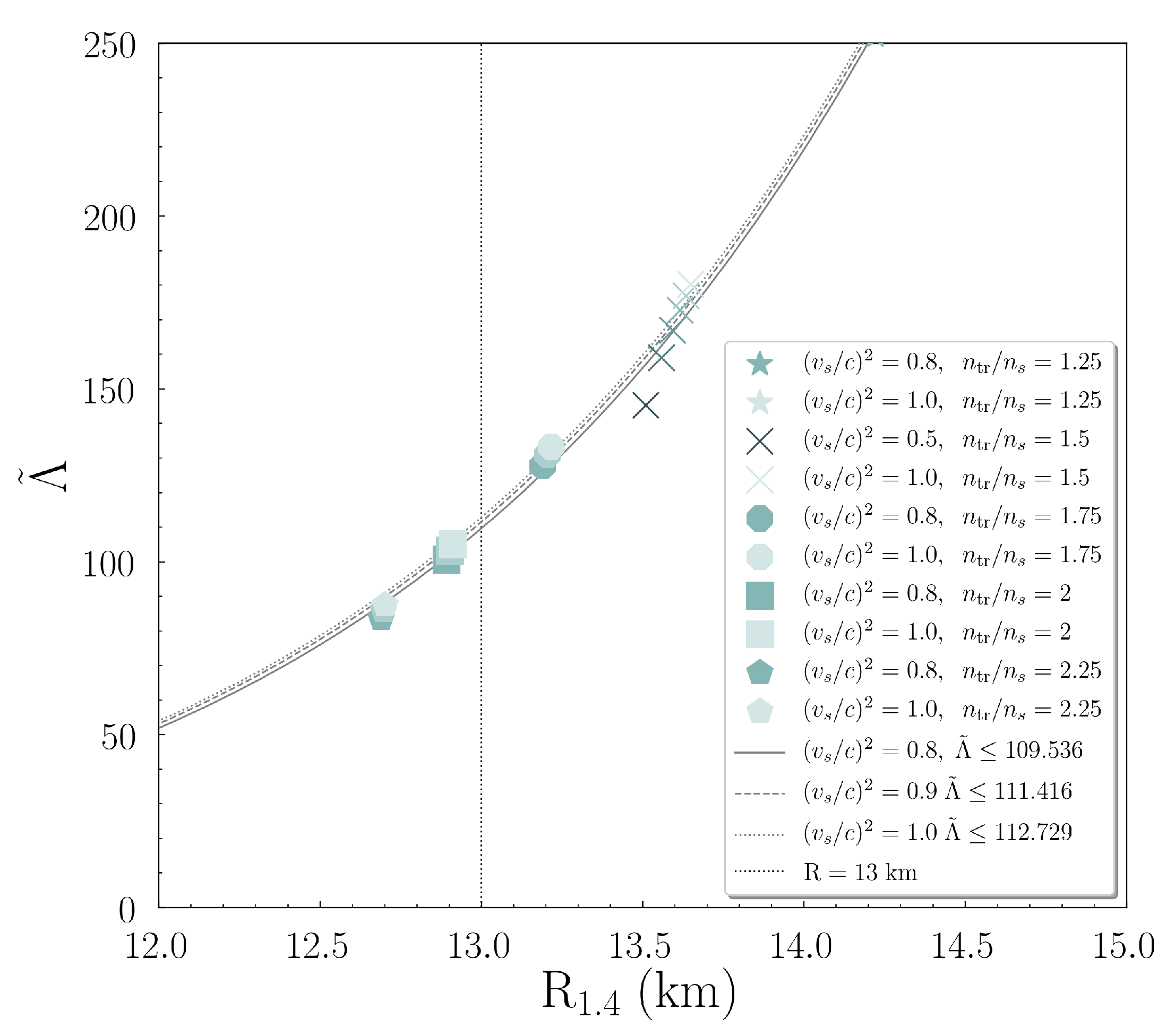
| Speed of Sound Bounds | ||||||||
|---|---|---|---|---|---|---|---|---|
| N.R. | M.R. | N.R. | M.R. | N.R. | M.R. | N.R. | M.R. | |
| c | 1.665 | 1.689 | 0.448 | 0.352 | – | 0.683 | – | 1.053 |
| 1.751 | 2.069 | 0.964 | 0.883 | – | 0.645 | – | 1.348 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kanakis-Pegios, A.; Koliogiannis, P.S.; Moustakidis, C.C. Probing the Nuclear Equation of State from the Existence of a ∼2.6 M⊙ Neutron Star: The GW190814 Puzzle. Symmetry 2021, 13, 183. https://doi.org/10.3390/sym13020183
Kanakis-Pegios A, Koliogiannis PS, Moustakidis CC. Probing the Nuclear Equation of State from the Existence of a ∼2.6 M⊙ Neutron Star: The GW190814 Puzzle. Symmetry. 2021; 13(2):183. https://doi.org/10.3390/sym13020183
Chicago/Turabian StyleKanakis-Pegios, Alkiviadis, Polychronis S. Koliogiannis, and Charalampos C. Moustakidis. 2021. "Probing the Nuclear Equation of State from the Existence of a ∼2.6 M⊙ Neutron Star: The GW190814 Puzzle" Symmetry 13, no. 2: 183. https://doi.org/10.3390/sym13020183
APA StyleKanakis-Pegios, A., Koliogiannis, P. S., & Moustakidis, C. C. (2021). Probing the Nuclear Equation of State from the Existence of a ∼2.6 M⊙ Neutron Star: The GW190814 Puzzle. Symmetry, 13(2), 183. https://doi.org/10.3390/sym13020183







