Abstract
This paper investigates the fixed-time formation (FixF) control problem for second-order multi-agent systems (MASs), where each agent is subject to disturbance and the communication network is general directed. First, a FixF protocol is presented based on the backstepping technique, where the distributed cooperative variable structure control method is utilized to handle the bounded disturbances. Then, to remove the dependence of control gains on the global information, a practical adaptive FixF control is presented, where the MASs can achieve formation with a bounded error within fixed time. Finally, a numerical example is presented to validate the theoretical result.
1. Introduction
Formation control is one of the most actively investigated topics on the cooperation of multi-agent systems (MASs), such as multiple vehicle [] and quadrotor aircraft []. Generally speaking, each agent is driven to satisfy predefined relative state constraints with respect to its neighbors in a formation control task.
A key point in the study of the formation control problem is the convergence rate, which indicates how efficient a proposed control protocol could be. The formation tracking problem for the general linear MASs was studied in [] over fixed and switching topologies, where the formation can be achieved asymptotically. Compared to the asymptotic controllers, designing a formation controller with finite-time convergence is more desirable as it indeed guarantees faster convergence rate as well as improved system performance [,,]. The formation control problems with finite-time convergence rate for linear and nonlinear first-order MASs were studied in [,] over undirected and directed graphs, respectively, while in [,], such problems were studied for multiple vehicles and multiple nonholonomic mobile robots, where the dynamics of the systems in [,] were second-order. The authors of [] proposed the adaptive formation control protocol for double integrator disturbed MASs with a general directed networks, which is fully distributed.
Having evolved from finite-time stability, fixed-time stability (FixS) [] inherits the advantage of finite-time convergence. Meanwhile, the convergence time is uniformly bounded with respect to the initial conditions. After that, FixS was well studied for single systems [], and extended to single integrator [,,,] and double integrator MASs [,,,,,,,,,,]. Specifically, the works in [,,,] all studied the leader–follower FixF control problem. Compared with the leader–follower case, it is more challenging to study the leaderless formation control problem because the agreed trajectory is not known in advance, and thus is not available to any agent. Furthermore, disturbances usually exist in real control networked systems [,].
Note that the communication topology discussed in most of the above-mentioned works is undirected. It is nontrivial to generalize these results to the formation problem with general directed topologies. The main challenge lies in the asymmetry property of directed topologies. In addition, global information is usually utilized in the control design in most existing works. Bearing the observation in mind, we will discover the FixF control problem for a kind of disturbed second-order MASs, where the communication graph is directed. We summarized the main contribution as follows. First, a FixF control protocol is proposed by utilizing the backstepping technique. The distributed cooperative variable structure control is utilized to handle the bounded disturbances. Then, to remove the dependence of control gains on the global information, a practical adaptive FixF control is presented, where the MASs can achieve formation with a bounded error within fixed time.
The rest of the paper consists of seven parts in total. Some concepts in graph theory and problem statement are provided in Section 2. Main results are given in Section 3. Section 4 provides a numerical example to verify the effectiveness of the theoretical results, and the paper is concluded in Section 5.
2. Preliminaries and Problem Statement
Before continuing, necessary notations used in this paper are introduced below.
Notations: The transposition of a vector or a matrix is marked by the superscript T; the eigenvalues of the symmetric and real matrix Q are arranged as . For any , is the signum function. We further define and , where . Symbol denotes the 2-norm of ; denote .
2.1. Graph Theory
The concepts for graph are given below, which can be found in [].
A directed graph is denoted as with , being the node set and edge set, respectively, and is the adjacency matrix of the graph with non-negative elements. An edge rooted at spacecraft j and ending at spacecraft i is denoted by . The weight . Furthermore, it is assumed that no self-loop exists. The corresponding Laplacian matrix is defined by for and for . Denote and the inner and outer neighbors of agent i, respectively, and is the number of the inner neighbors of agent i.
Assumption 1.
The graph is strongly connected.
2.2. Supporting Lemmas
First, we record the following lemma about the properties of directed graphs.
Lemma 1.
[,] Suppose Assumption 1 holds. Denote the Laplacian matrix associated with the graph . Then, , and there exists a vector with and such that . Furthermore, let the matrix with . Let be any positive vector. Then, where is the second smallest eigenvalue of .
One lemma for FixS and several lemmas about some inequalities are introduced, which will be used to prove the main result.
Lemma 2.
[] We consider the system
with its equilibrium point being the origin. If there exists a continuous radially unbounded function (CRUF) such that and for some positive constants and satisfying Then, the system (1) is said FixS with the settling time estimated by .
Lemma 3.
Lemma 4.
[] For , it always holds that
Lemma 5.
[] For and , it holds that
Lemma 6.
[] Given , it holds that
Lemma 7.
[] For , and , the following inequalities hold:
2.3. Problem Formulation
We consider the second-order MASs with disturbances.
where represent the position and the velocity states of the ith agent, stands for the disturbance, and is a control input.
Definition 1.
Formation information can be represented by a time-dependent vector . The FixS formation for the second-order MASs (2) is said to be achieved if there exists a settling time , which is uniformly bounded with regard to the initial state, viz, there exists a fixed constant with , such that
Here, is simplified as
The following assumptions are introduced which will be used in the proof of the main result.
Assumption 2.
Suppose that the unknown disturbances are bounded:
where is a constant.
Assumption 3.
Suppose the second derivative of is bounded, that is,
where is a constant.
In the following, we will design a distributed control protocol by invoking local information to drive the system (2) to achieve the formation with fixed-time convergence.
3. Main Results
3.1. Fixed-Time Formation Control Protocol
In this subsection, a FixF control protocol will be designed via backstepping method. Let and . The error dynamics can be given:
where , satisfying .
Define and . Then, we have and .
The formation control protocol design procedure is illustrated as follows.
(1) First, we consider the following systems:
A virtual control input for system (7) is designed as
where and . Furthermore, a virtual control law for is designed as
where and .
For further analysis, we construct a Lyapunov function candidate as follows:
where is given in Lemma 1.
The time derivative of can be computed as follows:
where the definition of can be found in Lemma 1, and .
As inspired by the work in [], we introduce the following indicative function:
with . By Lemma 1, one obtains . It follows that
Thus, , where . One can observe that is a positive vector. By Lemma 1, we have
On the other hand,
where , and is utilized based on Lemma 4 to derive the last inequality. From (10)–(13), one obtains
(2) Design of control law
Consider the dynamics of :
Then, the control protocol is designed as follows:
where , and the constant coefficients and will be given in the next Theorem.
To this end, we summarize the main result and provide the corresponding stability analysis.
Theorem 1.
Proof.
Consider the Lyapunov function candidate
with
where is defined in Lemma 1. It is not difficult to verify that V is differentiable, positive definite, and proper. Denote and .
The time derivative of is given as
Let and , then one obtains
Based on Lemma 4, one obtains
and
Furthermore,
where and
Taking the control protocol (15) into consideration and one has
where and .
Thus, the parameters can be chosen as
such that and are all positive.
Then, from (25), one obtains
where and . The last inequality is because due to .
On the other hand, from (18) one has
with and .
According to Lemma 7 and from (26), (27), one has
where the Lemma 5 is utilized for the last inequality by noting , and with .
Because , by using Lemma 2, it can be obtained that converges to 0 within a fixed T satisfying Furthermore, , which means the FixF is achieved. The proof is completed. □
Remark 1.
We claim that the control input (15) is globally bounded. In fact, from (26) in the paper, one obtains that and are bounded, which implies that are bounded. From (8), we have , which means that is bounded. Besides, is bounded by noting that . By noting the expression of controller (15), it is known that the control input is globally bounded.
Remark 2.
From Theorem 1, it can be observed that the parameters in (8), (9) and in (15) can be chosen as any positive numbers. However, from (16), it can be seen that the control gains relies on the global information, viz., the spectrum of the Laplacian matrix and the outer degree. To remove such constraint, a new adaptive practical fixed-time controller will be proposed in the next subsection.
3.2. Adaptive Practical Fixed-Time Formation Control Protocol
In this subsection, an adaptive practical FixF controller will be designed to adjust the control gain online.
An adaptive FixF control protocol is designed as follows:
where are any positive constants, , , and are defined in the above subsection. Moreover, are dynamic control gains, which are defined as
Theorem 2.
Proof.
Consider the Lyapunov function candidate
in which V is defined in (17) and with being some positive constants to be designed later.
Following the same procedures in the proof of Theorem 1, one obtains
The last inequality is due to
One can choose and let . Then, following the same steps in the proof of Theorem 1, one has
where and are designed as before.
Because and , one obtains
By noticing that are constants, it can be concluded that W is bounded, which implies is bounded, viz., there exists a constant such that .
Moreover,
where
Based on Lemma 3, we can conclude that the system in (6) is practical FixS. Furthermore, we calculate the residual set as with . The time needed to reach the residual set is bounded as . The proof is completed. □
Remark 3.
By invoking the tools of Kronecker product, the theoretical result obtained here can be extended to MASs with multidimensional dynamics directly.
Remark 4.
In this work, some practical factors are considered such as disturbance, convergence time, and the dependence of global information. In fact, there are many other practical but important factors worth considering. For example, actuator failure, communication switching, collision, and deadlock [,,,]. We will consider these interesting problems in our future research.
4. Simulations
In this section, a numerical simulation is provided to show the performance of the proposed adaptive control protocol.
Consider a MASs with six agents, where and The interaction topology is represented by the strongly connected directed graph shown in Figure 1. The desired formation is a regular hexagon and the desired trajectory of the formation center is “O”: . Furthermore, in the desired formation, the relative displacements of each agent to the formation center are given as , , , , , . Control parameters are chosen as Figure 2 depicts the adaptive gains. It can be observed that all the adaptive gains will be bounded. The trajectories of the 6 agents are depicted in Figure 3. From Figure 3, it is seen that when , the formation is not formed; when , the formation is achieved and maintained. The formation trajectory tracking errors and are shown in Figure 4 and Figure 5, where and reach the same value very fast. Another interesting thing is that converges to zero finally, while converges to some steady but nonzero value. In fact, what matters most is . is not required to reach zero.
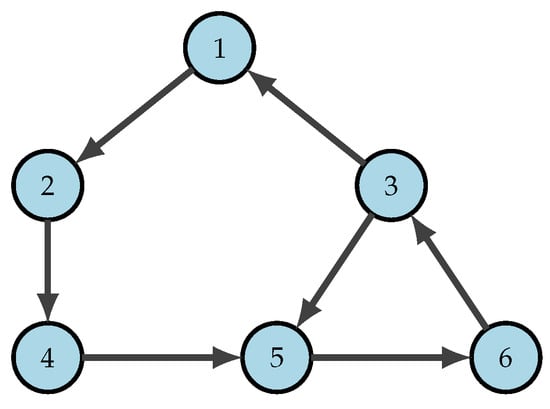
Figure 1.
The communication graph .
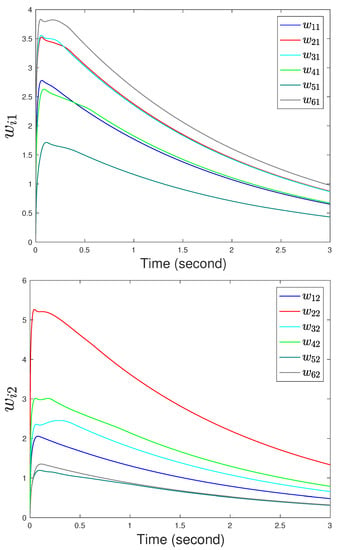
Figure 2.
The trajectory of adaptive control gain. Above: the first dimension ; below: the second dimension .
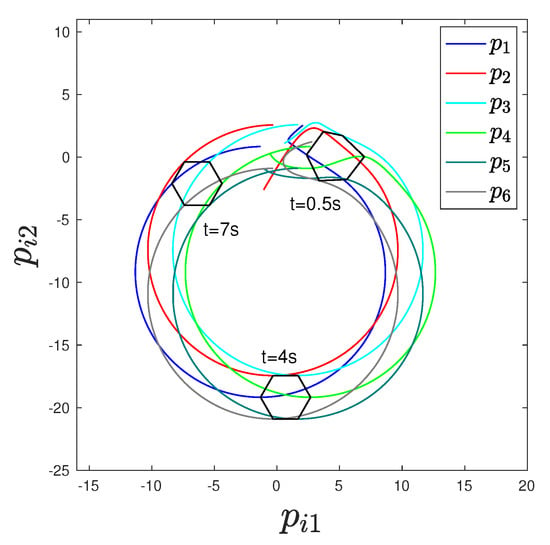
Figure 3.
The trajectories of agents.
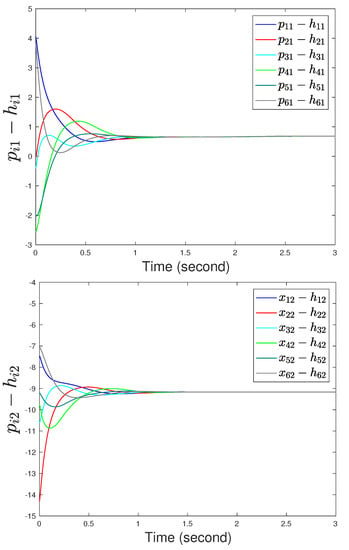
Figure 4.
Formation trajectory tracking error: . Above: the first dimension ; below: the second dimension .
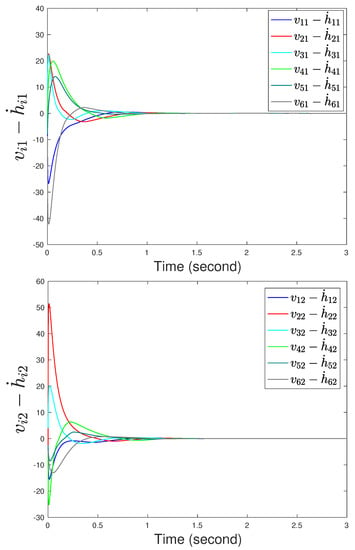
Figure 5.
Formation trajectory tracking error: . Above: the first dimension ; below: the second dimension .
5. Conclusions
The FixF problem for a kind of second-order MASs with disturbances over general directed graphs has been resolved in this paper. A novel FixF control protocol has been designed based on backstepping method, and the convergence time can be estimated directly, which is uniformly bounded. Then, to remove the dependence of control gains on global conditions, an adaptive practical FixF control protocol has been presented, which is fully distributed. In fact, the results can be generalized to more general directed graphs that just contain a directed spanning tree []. Future work includes considering FixF of general nonlinear MASs with directed communication topologies. Another research direction is to design a fully distributed FixF control protocol to achieve an accurate convergence for second-order or higher-order MASs by noticing that the errors converge to a small region in this paper.
Author Contributions
Conceptualization, H.H. and H.W.; methodology, H.H. and H.W.; validation, H.H. and H.W.; formal analysis, H.H.; investigation, H.H. and H.W.; writing—original draft preparation, H.H.; writing—review and editing, H.H. and H.W.; visualization, H.H. and H.W.; supervision, H.W.; project administration, H.H. and H.W.; funding acquisition, H.H. and H.W. All authors have read and agreed to the published version of the manuscript.
Funding
This research was funded by the Natural Science Foundation of Jiangsu Province under grant numbers BK20210591 and BK20210216, the University Science Research Project of Jiangsu Province under grant number 21KJB510044, the Doctor of Entrepreneurship and Innovation in Jiangsu Province under grant numbers JSSCBS20210509 and JSSCBS20210118, by the Scientific Foundation of Nanjing University of Posts and Telecommunications under grant number NY220147, by the Guangdong Basic and Applied Basic Research Foundation under grant number 2020A1515110148, and by the Shandong Province Key R&D Program under grant number 2020RKB01059.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Liu, Y.; Zhao, Y.; Wen, G. Finite-time formation tracking control for multiple vehicles: A motion planning approach. Int. J. Robust Nonlinear Control 2016, 26, 3130–3149. [Google Scholar] [CrossRef]
- Du, H.; Zhu, W.; Wen, G.; Wu, D. Finite-time formation control for a group of quadrotor aircraft. Aerosp. Sci. Technol. 2017, 69, 609–616. [Google Scholar] [CrossRef]
- Sun, T.; Liu, H.; Yao, Y.; Li, T.; Cheng, Z. Distributed adaptive formation tracking control under fixed and switching topologies: Application on general linear multi-agent systems. Symmetry 2021, 13, 941. [Google Scholar] [CrossRef]
- Hou, H.; Yu, X.; Xu, L.; Restam, K.; Cao, Z. Finite-time continuous terminal sliding mode control of servo motor systems. IEEE Trans. Ind. Electron. 2020, 67, 5647–5656. [Google Scholar] [CrossRef]
- Hong, H.; Wen, G.; Yu, X.; Yu, W. Robust distributed average tracking for disturbed second-order multiagent systems. IEEE Trans. Syst. Man Cybern. Syst. 2021. [Google Scholar] [CrossRef]
- Xiao, F.; Wang, L.; Chen, J.; Gao, Y. Finite-time formation control for multi-agent systems. Automatica 2009, 45, 2605–2611. [Google Scholar] [CrossRef]
- Lü, J.; Chen, F.; Chen, G. Nonsmooth leader-following formation control of nonidentical multi-agent systems with directed communication topologies. Automatica 2016, 64, 112–120. [Google Scholar] [CrossRef]
- Ou, M.; Du, H.; Li, S. Finite-time formation control of multiple nonholonomic mobile robots. Int. J. Robust Nonlinear Control 2014, 24, 140–165. [Google Scholar] [CrossRef]
- Mei, F.; Wang, H.; Yao, Y.; Fu, J.; Yuan, X.; Yu, W. Robust second-order finite-time formation control of heterogeneous multi-agent systems on directed communication graphs. IET Control Theory Appl. 2020, 14, 816–823. [Google Scholar] [CrossRef]
- Polyakov, A. Nonlinear feedback design for fixed-time stabilization of linear control systems. IEEE Trans. Autom. Control 2012, 57, 2106–2110. [Google Scholar] [CrossRef] [Green Version]
- Chen, Q.; Xie, S.; Sun, M.; He, X. Adaptive nonsingular fixed-time attitude stabilization of uncertain spacecraft. IEEE Trans. Aerosp. Electron. Syst. 2018, 54, 2937–2950. [Google Scholar] [CrossRef]
- Parsegov, S.; Polyakov, A.; Schcherbakov, P. Fixed-time consensus algorithm for multi-agent systems with integrator dynamics. IFAC Proc. Vol. 2013, 46, 110–115. [Google Scholar] [CrossRef] [Green Version]
- Hong, H.; Yu, W.; Wen, G.; Yu, X. Distributed robust fixed-time consensus for nonlinear and disturbed multi-agent systems. IEEE Trans. Syst. Man Cybern. Syst. 2017, 47, 1464–1473. [Google Scholar] [CrossRef]
- Wang, H.; Yu, W.; Wen, G.; Chen, G. Fixed-time consensus of nonlinear multi-agent sytems with general directed topologies. IEEE Trans. Circuits Syst. II Exp. Briefs 2019, 66, 1587–1591. [Google Scholar] [CrossRef]
- Wang, C.; Tnunay, H.; Zuo, Z.; Lennox, B.; Ding, Z. Fixed-time formation control of multirobot systems: Design and experiments. IEEE Trans. Ind. Electron. 2018, 66, 6292–6301. [Google Scholar] [CrossRef] [Green Version]
- Zuo, Z. Nonsingular fixed-time consensus tracking for second-order multi-agent networks. Automatica 2015, 54, 305–309. [Google Scholar] [CrossRef]
- Wen, G.; Yu, X.; Fu, J.; Wang, H.; Yu, W. Fast distributed average tracking in multi-agent networks: The case with general linear agent dynamics. IEEE Trans. Control Netw. Syst. 2020. [Google Scholar] [CrossRef]
- Fu, J.; Wang, J. Fixed-time coordinated tracking for second-order multi-agent systems with bounded input uncertainties. Syst. Control Lett. 2016, 93, 1–12. [Google Scholar] [CrossRef]
- Hong, H.; Yu, W.; Fu, J.; Yu, X. A novel class of distributed fixed-time consensus protocols for second-order nonlinear and disturbed multi-agent systems. IEEE Trans. Netw. Sci. Eng. 2019, 6, 760–772. [Google Scholar] [CrossRef]
- Zou, A.; Fan, Z. Distributed fixed-time attitude coordination control for multiple rigid spacecraft. Int. J. Robust Nonlinear Control 2020, 30, 266–281. [Google Scholar] [CrossRef]
- Ni, J.; Liu, L.; Liu, C.; Liu, J. Fixed-time leader-following consensus for second-order multi-agent systems with input delay. IEEE Trans. Ind. Electron. 2017, 64, 8635–8646. [Google Scholar] [CrossRef]
- Hong, H.; Anderson, B.D.O. Distributed fixed-time attitude tracking consensus for rigid spacecraft systems under directed graphs. IEEE Control Syst. Lett. 2020, 4, 698–703. [Google Scholar] [CrossRef]
- Li, Q.; Wei, J.; Gou, Q.; Niu, Z. Distributed adaptive fixed-time formation control for second-order multi-agent systems with collision avoidance. Inf. Sci. 2021, 564, 27–44. [Google Scholar] [CrossRef]
- Xiong, T.; Gu, Z. Observer-based adaptive fixed-time formation control for multi-agent systems with unknown uncertainties. Neurocomputing 2021, 423, 506–517. [Google Scholar] [CrossRef]
- Gao, Z.; Guo, G. Fixed-time sliding mode formation control of AUVs based on a disturbance observer. IEEE/CAA J. Autom. Sin. 2020, 7, 539–545. [Google Scholar] [CrossRef]
- Chang, S.; Wang, Y.; Zuo, Z.; Yang, H. Fixed-time formation control for wheeled mobile robots with prescribed performance. IEEE Trans. Contr. Syst. Technol. 2021. [Google Scholar] [CrossRef]
- Yu, W.; Wang, H.; Hong, H.; Wen, G. Distributed cooperative anti-disturbance control of multi-agent systems: An overview. Sci. China Inf. Sci. 2017, 60, 110202. [Google Scholar] [CrossRef] [Green Version]
- Hou, H.; Yu, X.; Fu, Z. Sliding mode control of networked control systems: An auxiliary matrices-based approach. IEEE Trans. Autom. Control 2021. [Google Scholar] [CrossRef]
- Godsil, C.; Royle, G. Algebraic Graph Theory; Springer: New York, NY, USA, 2001. [Google Scholar]
- Yu, W.; Chen, G.; Cao, M.; Kurths, J. Second-order consensus for multiagent systems with directed topologies and nonlinear dynamics. IEEE Trans. Syst. Man Cybern. Cybern. 2010, 40, 881–891. [Google Scholar]
- Mei, J.; Ren, W.; Chen, J. Distributed consensus of second-order multi-agent systems with heterogeneous unknown inertias and control gains under a directed graph. IEEE Trans. Autom. Control 2016, 61, 2019–2034. [Google Scholar] [CrossRef]
- Jiang, B.; Hu, Q.; Friswell, M.I. Fixed-time attitude controlfor rigid spacecraft with actuator saturation and faults. IEEE Trans. Contr. Syst. Technol. 2016, 24, 1892–1898. [Google Scholar] [CrossRef]
- Wang, H.; Yu, W.; Ren, W.; Lü, J. Distributed adaptive finite-time consensus for second-order multiagent systems with mismatched disturbances under directed networks. IEEE Trans. Cybern. 2021, 51, 1347–1358. [Google Scholar] [CrossRef]
- Goldberg, M. Equivalence constants for lp norms of matrices. Lin. Multilin. Algebra 1987, 21, 173–179. [Google Scholar] [CrossRef]
- Qian, C.; Lin, W. A continuous feedback approach to global strong stabilization of nonlinear systems. IEEE Trans. Autom. Control 2001, 46, 1061–1079. [Google Scholar] [CrossRef]
- Yu, C.; Wang, H.; Yu, W. Distributed average tracking problem under directed networks: A distributed estimator-based design. IEEE Trans. Control Netw. Syst. 2021. [Google Scholar] [CrossRef]
- Tao, G.; Chen, S.; Joshi, S.M. An adaptive actuator failure compensation controller using output feedback. IEEE Trans. Autom. Control 2002, 47, 506–511. [Google Scholar] [CrossRef]
- Wen, G.; Zheng, W. On constructing multiple Lyapunov functions for tracking control of multiple agents with switching topologies. IEEE Trans. Autom. Control 2018, 64, 3796–3803. [Google Scholar] [CrossRef]
- Damasceno, B.C.; Xie, X. Deadlock-free scheduling of manufacturing systems using Petri nets and dynamic programming. IFAC Proc. Vol. 1999, 32, 4870–4875. [Google Scholar] [CrossRef]
- Foumani, M.; Gunawan, I.; Smith-Miles, K. Resolution of deadlocks in a robotic cell scheduling problem with post-process inspection system: Avoidance and recovery scenarios. In Proceedings of the 2015 IEEE International Conference on Industrial Engineering and Engineering Management (IEEM), Singapore, 6–9 December 2015; pp. 1107–1111. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).