Developing a Strategy to Improve Handling Behaviors of a Medium-Size Electric Bus Using Active Anti-Roll Bar
Abstract
1. Introduction
2. Research Background
2.1. Specifications of the Simulation Model
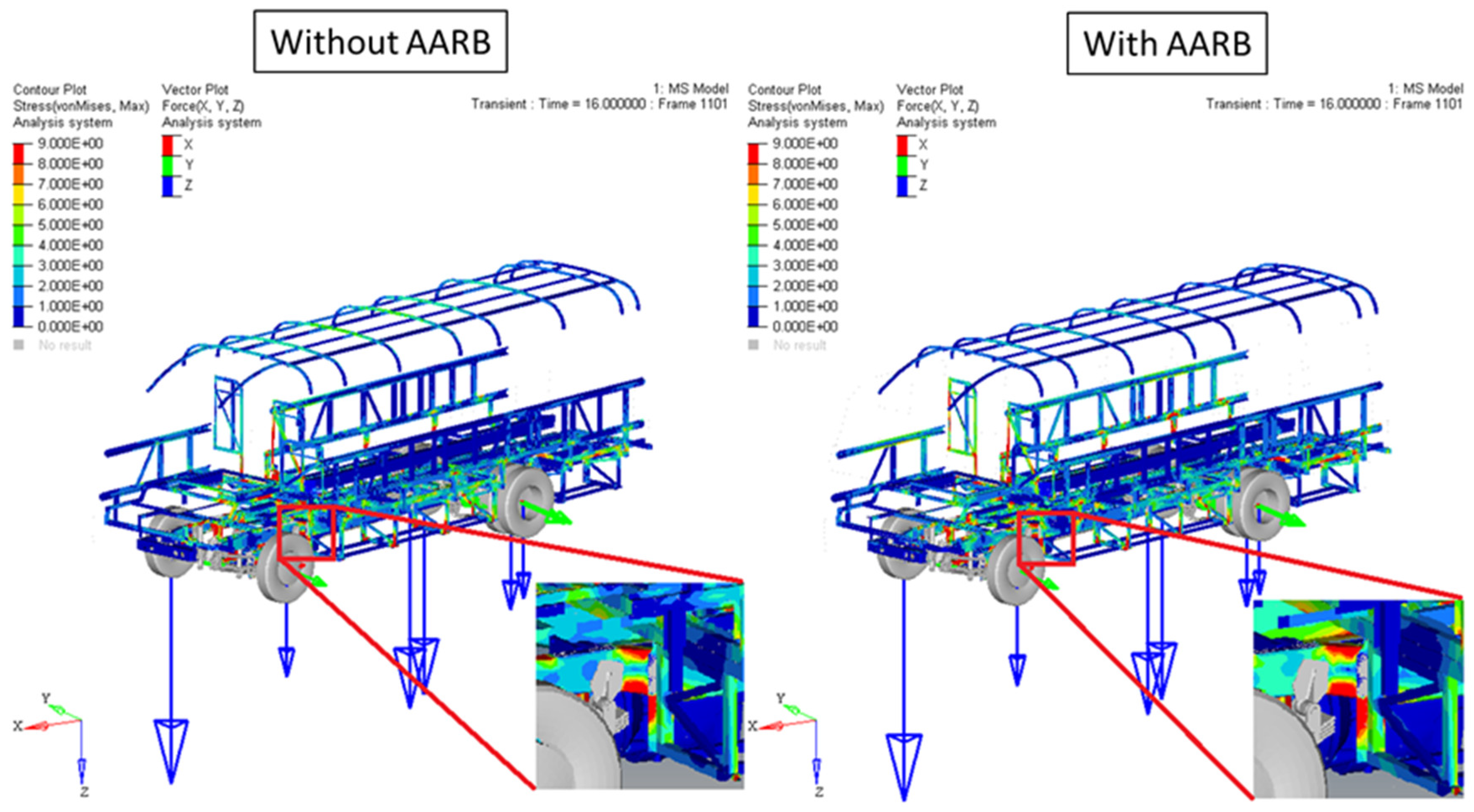
2.2. Flexible Body Model
2.3. The Multi-Body Dynamic Model of the Chassis
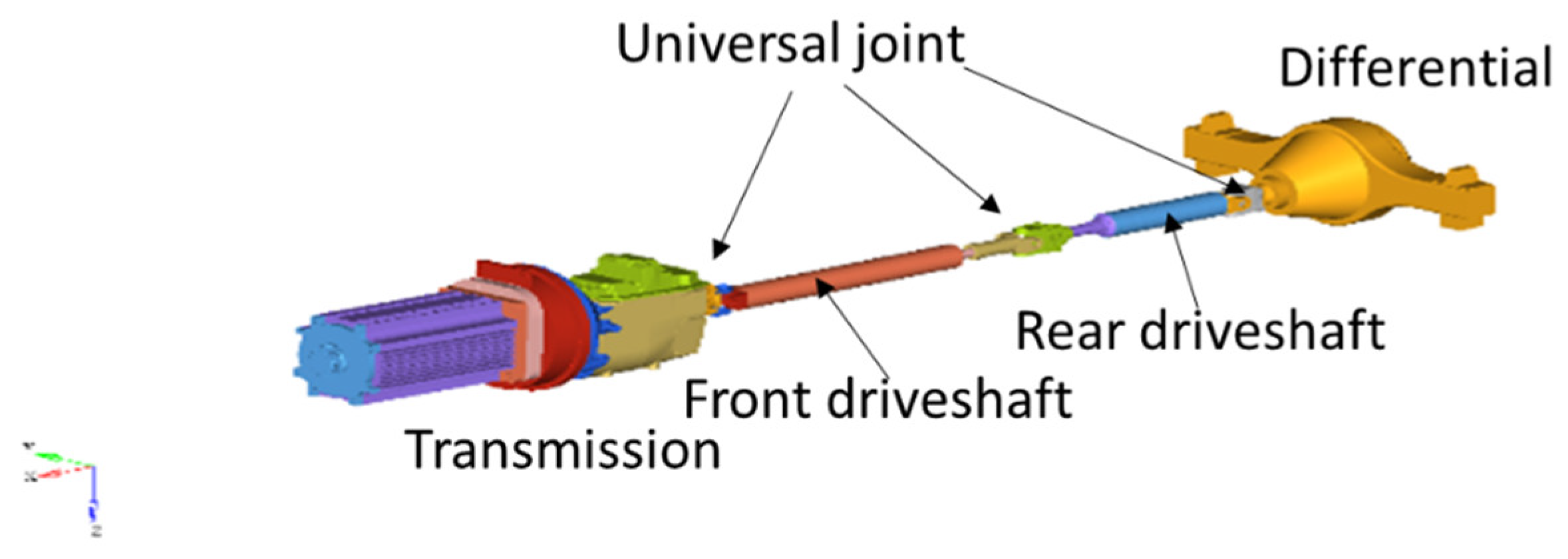
2.3.1. Transmission System
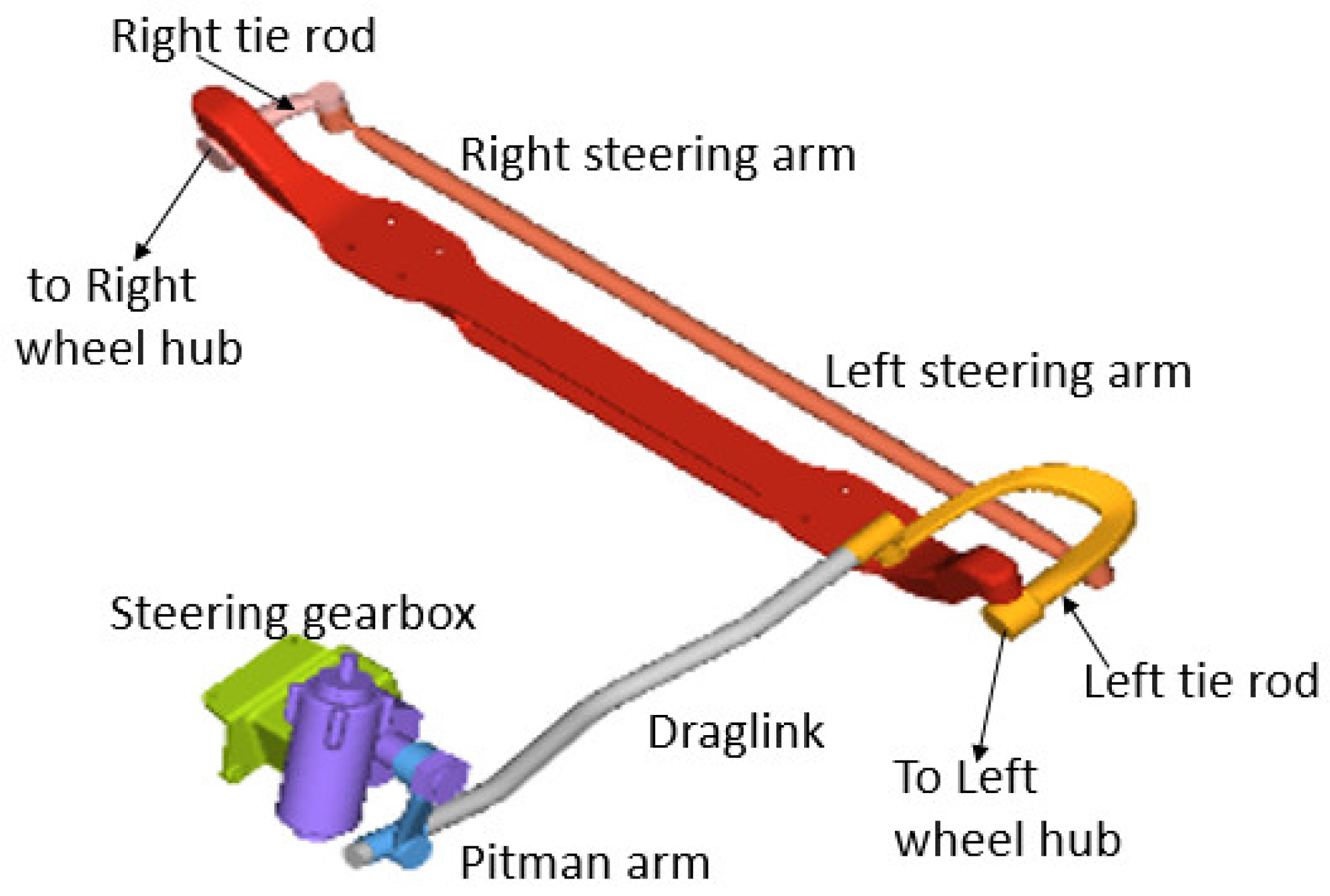
2.3.2. Steering System
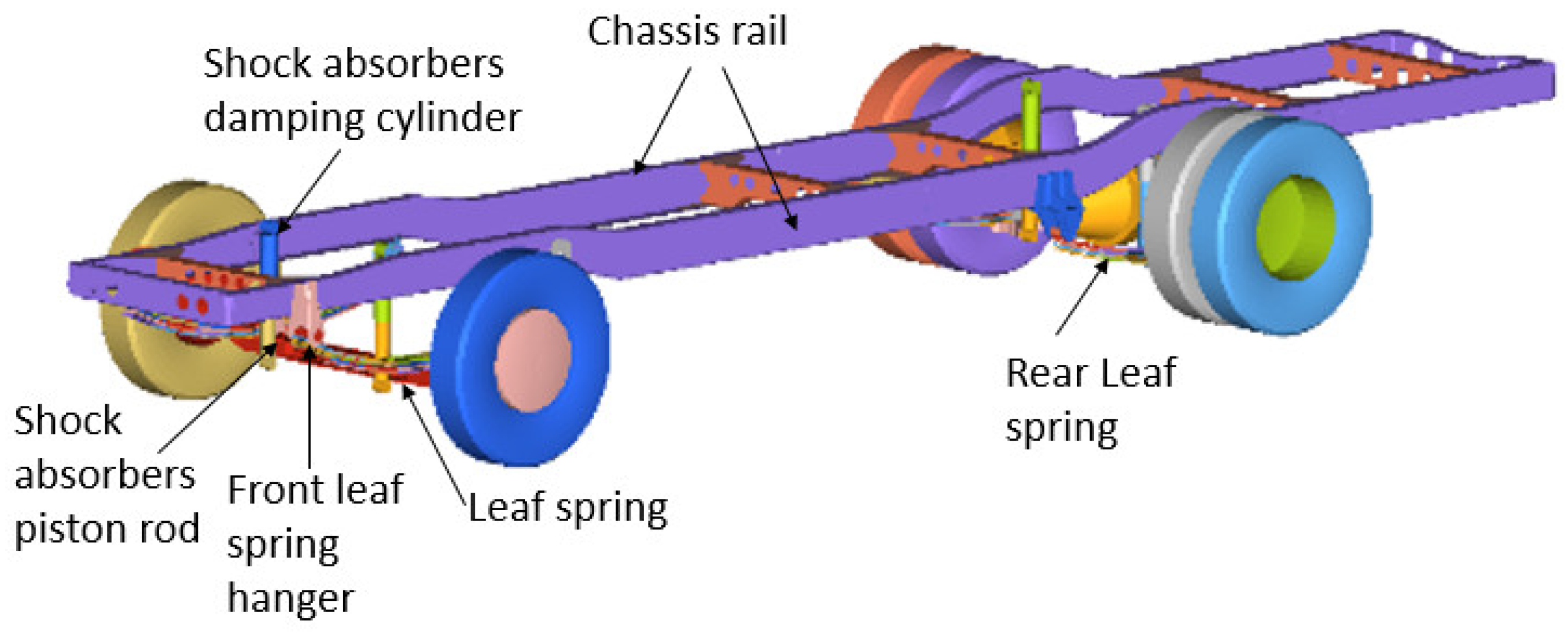
2.3.3. Suspension System
2.4. Model of the Tires and Road Surface
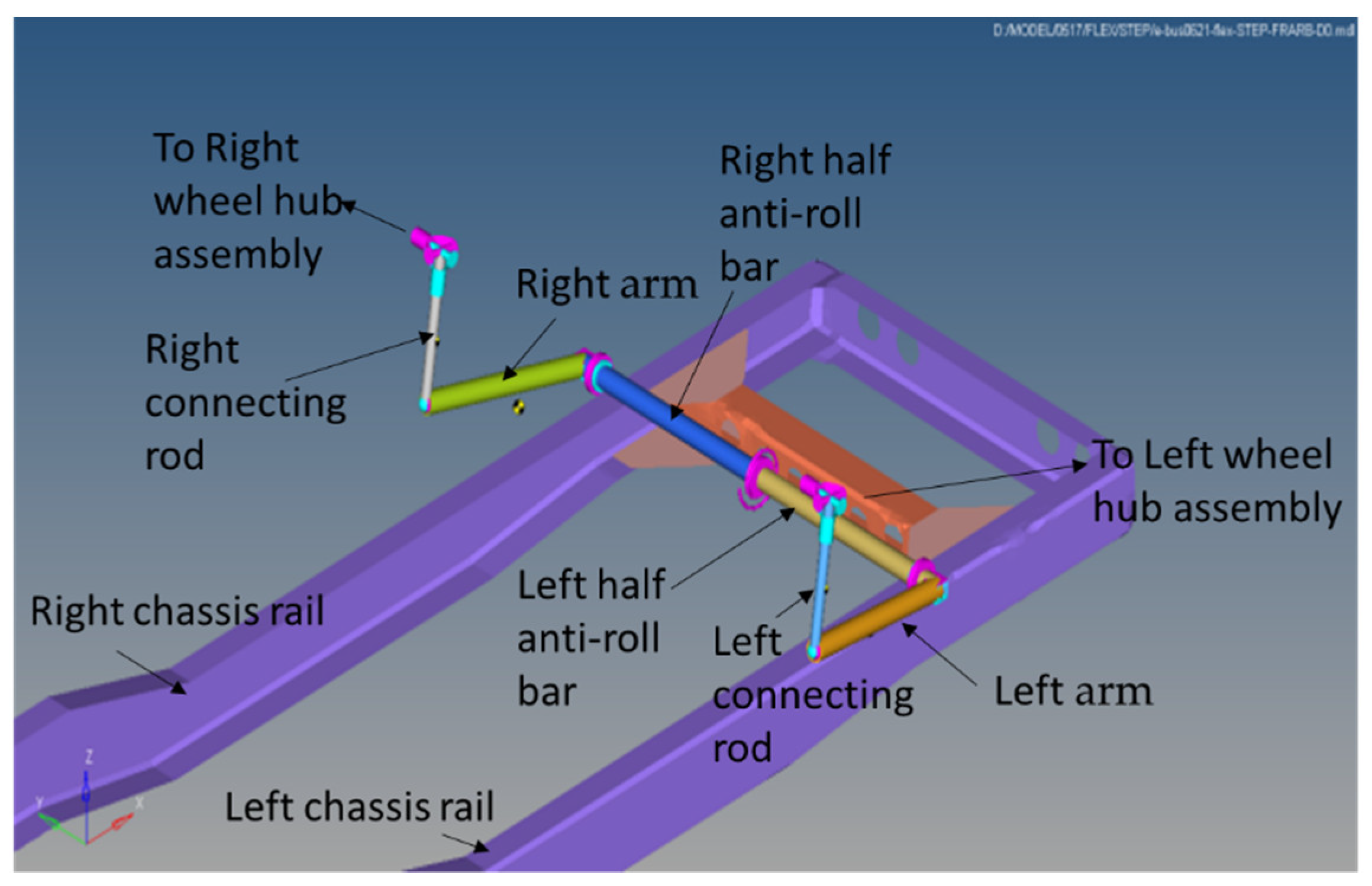
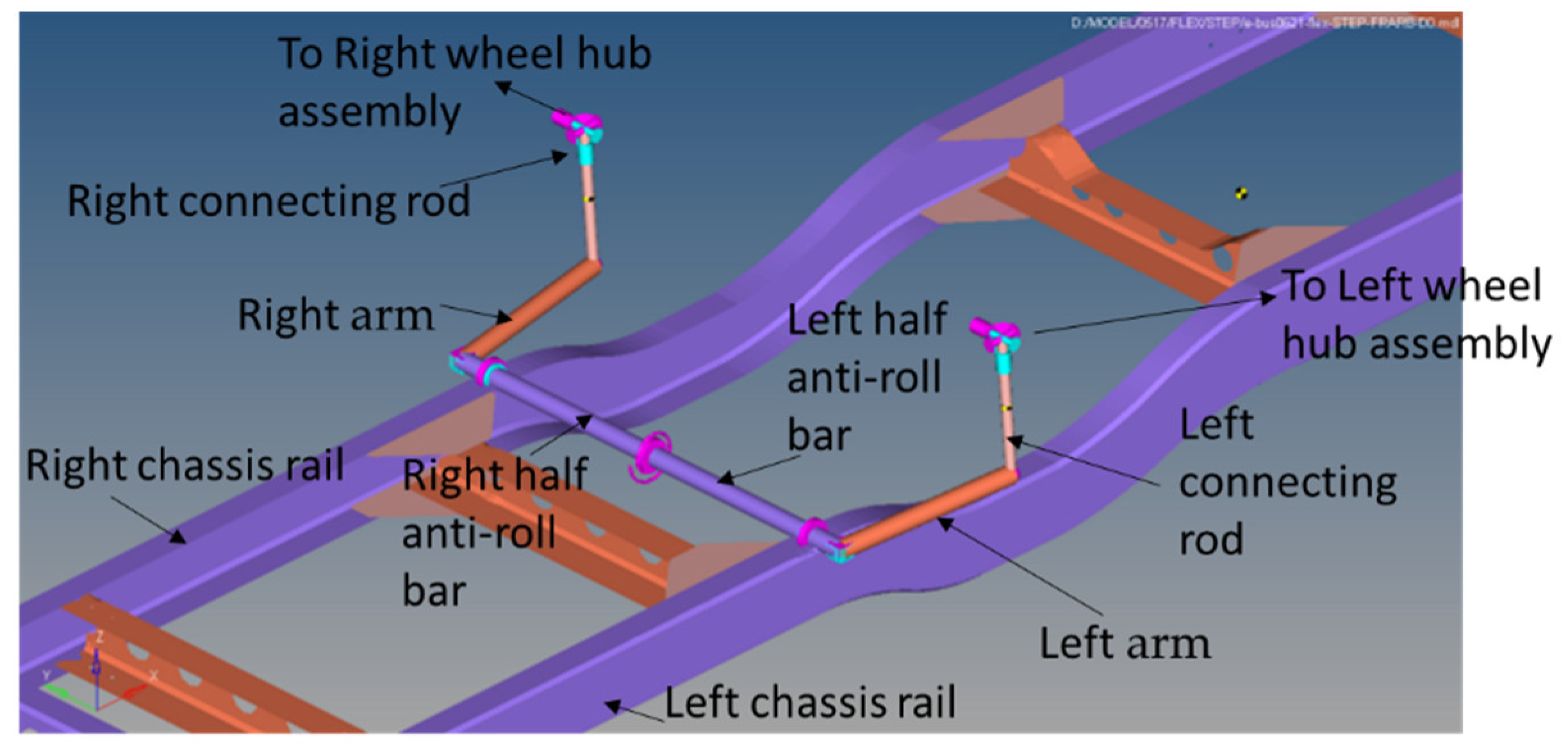
2.5. Model of the Anti-Roll Bar
2.6. Simulation Testing Method
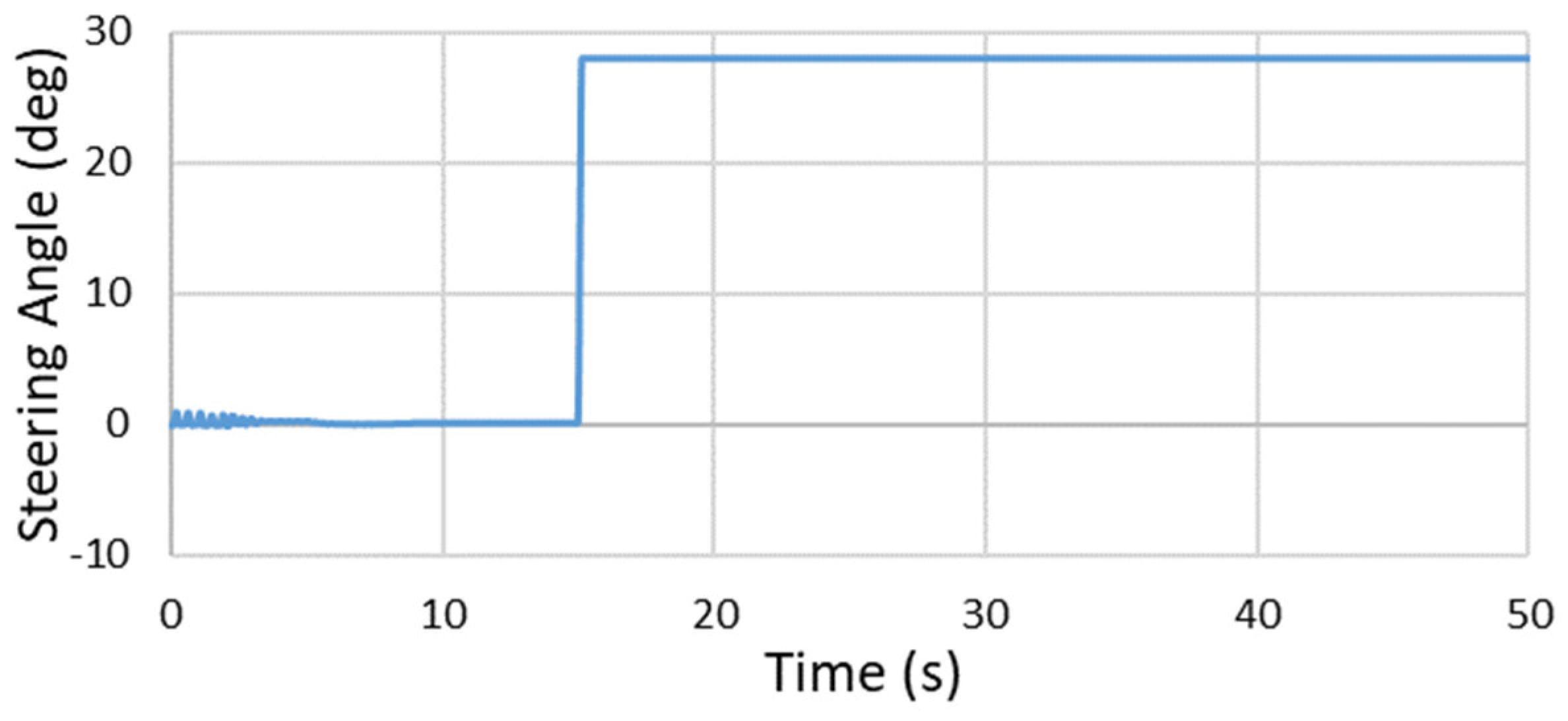
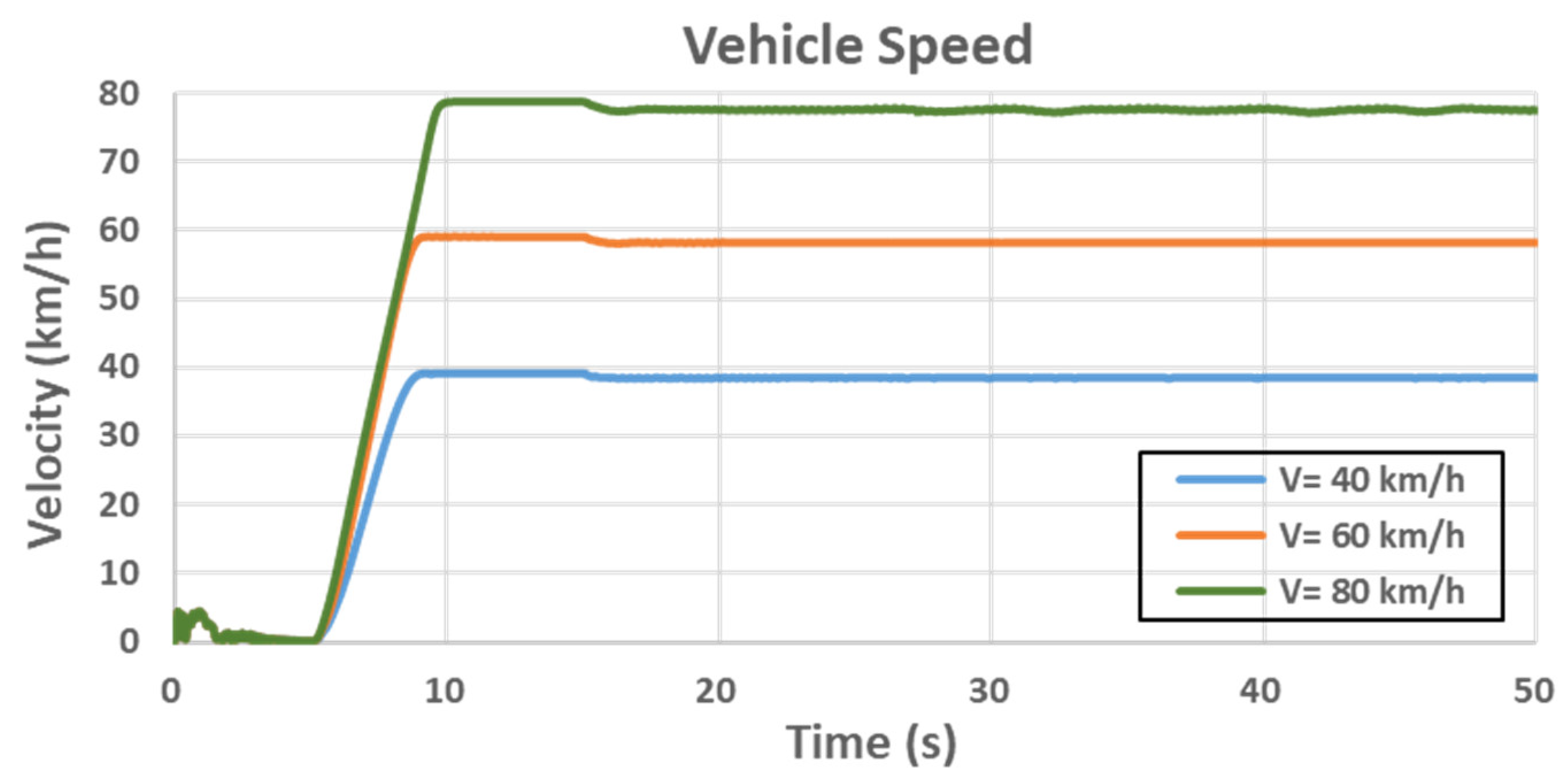
2.6.1. Step Steering Input Test
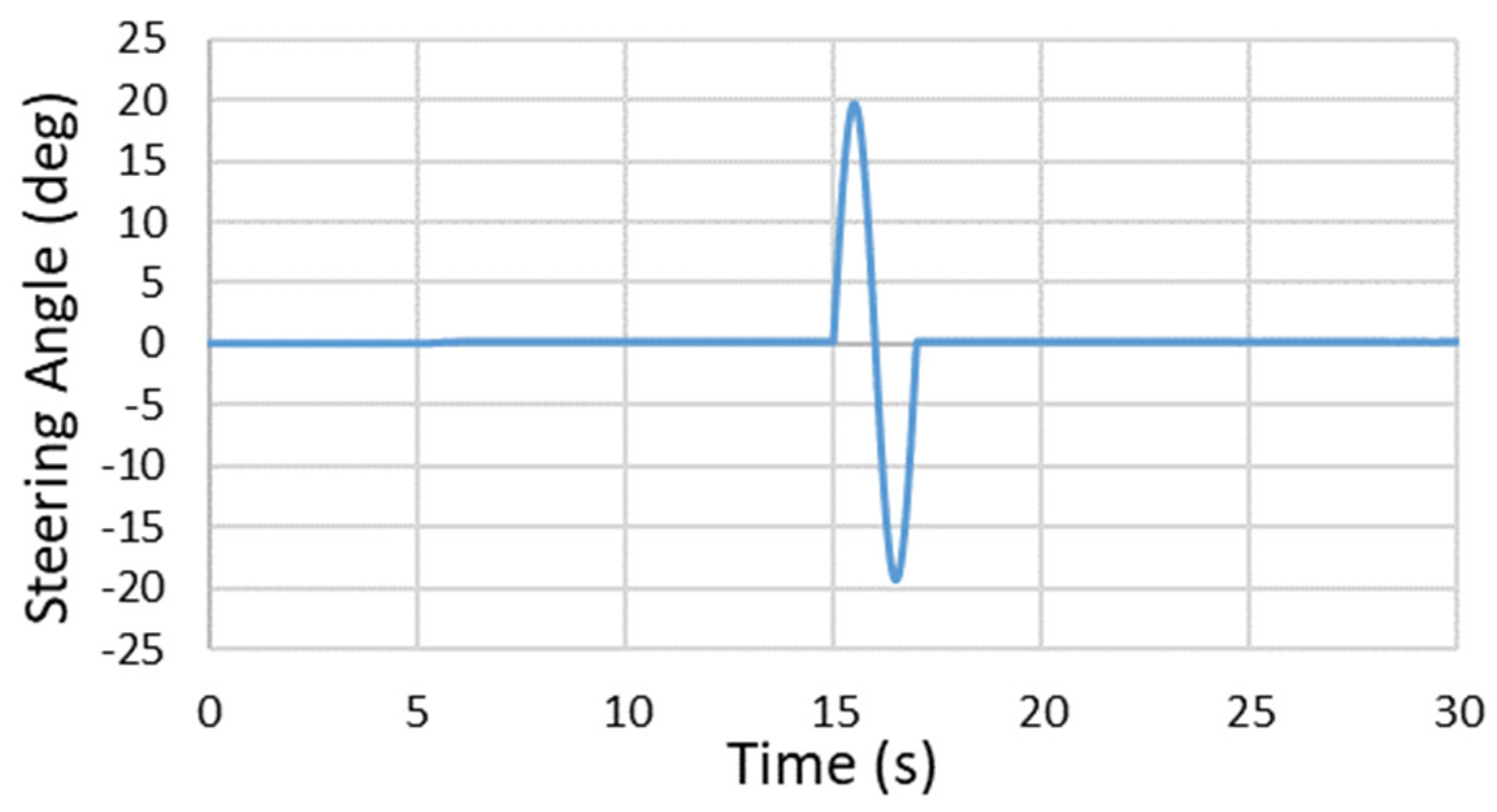
2.6.2. Single Sinusoidal Steering Input Test
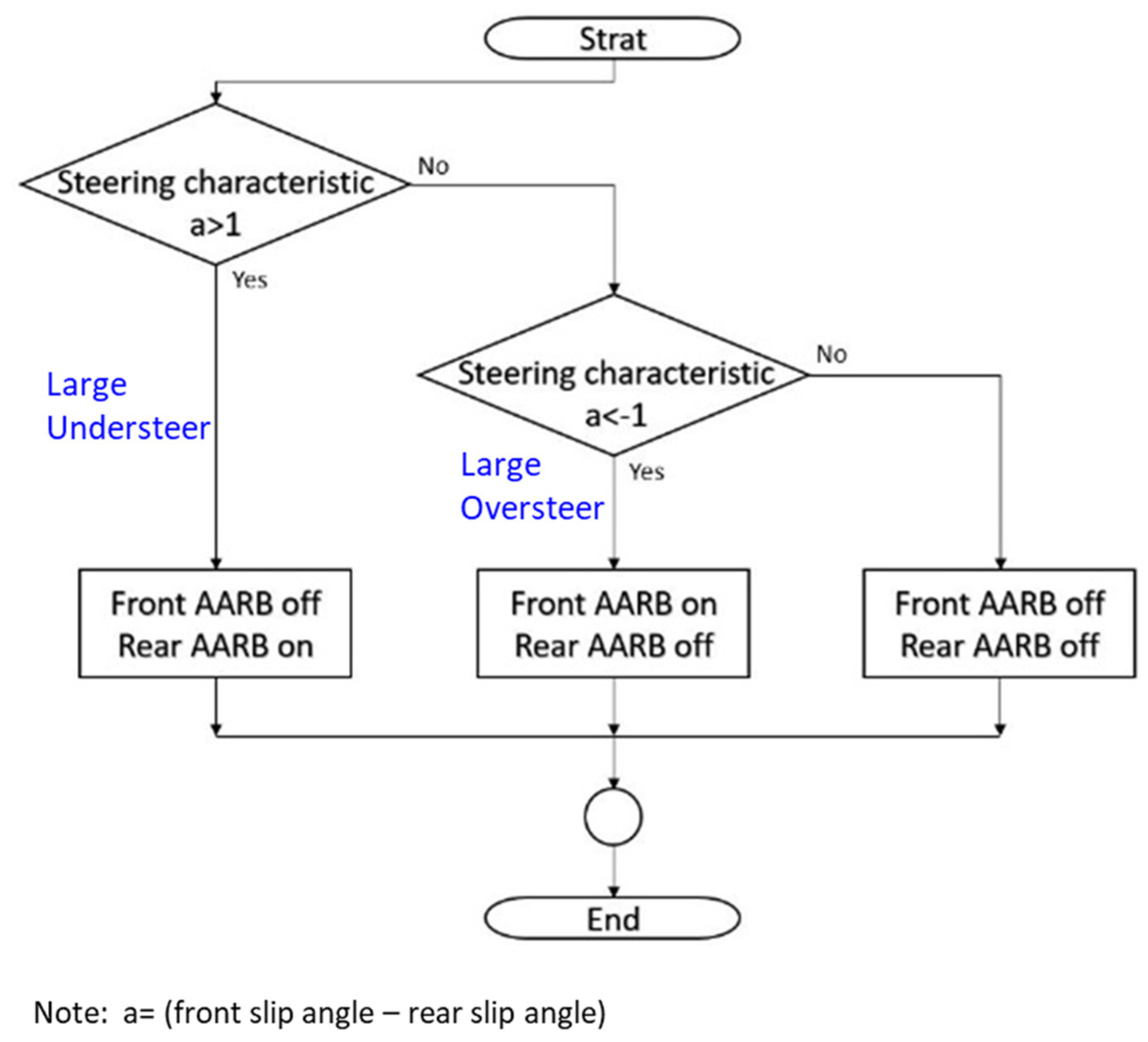
3. Active Anti-Roll Bar (AARB) Control Strategy
4. Results
4.1. Analysis of the Step Steering Input Test
4.2. Analysis of the Single Sinusoidal Steering Input Test
5. Conclusions
- (1)
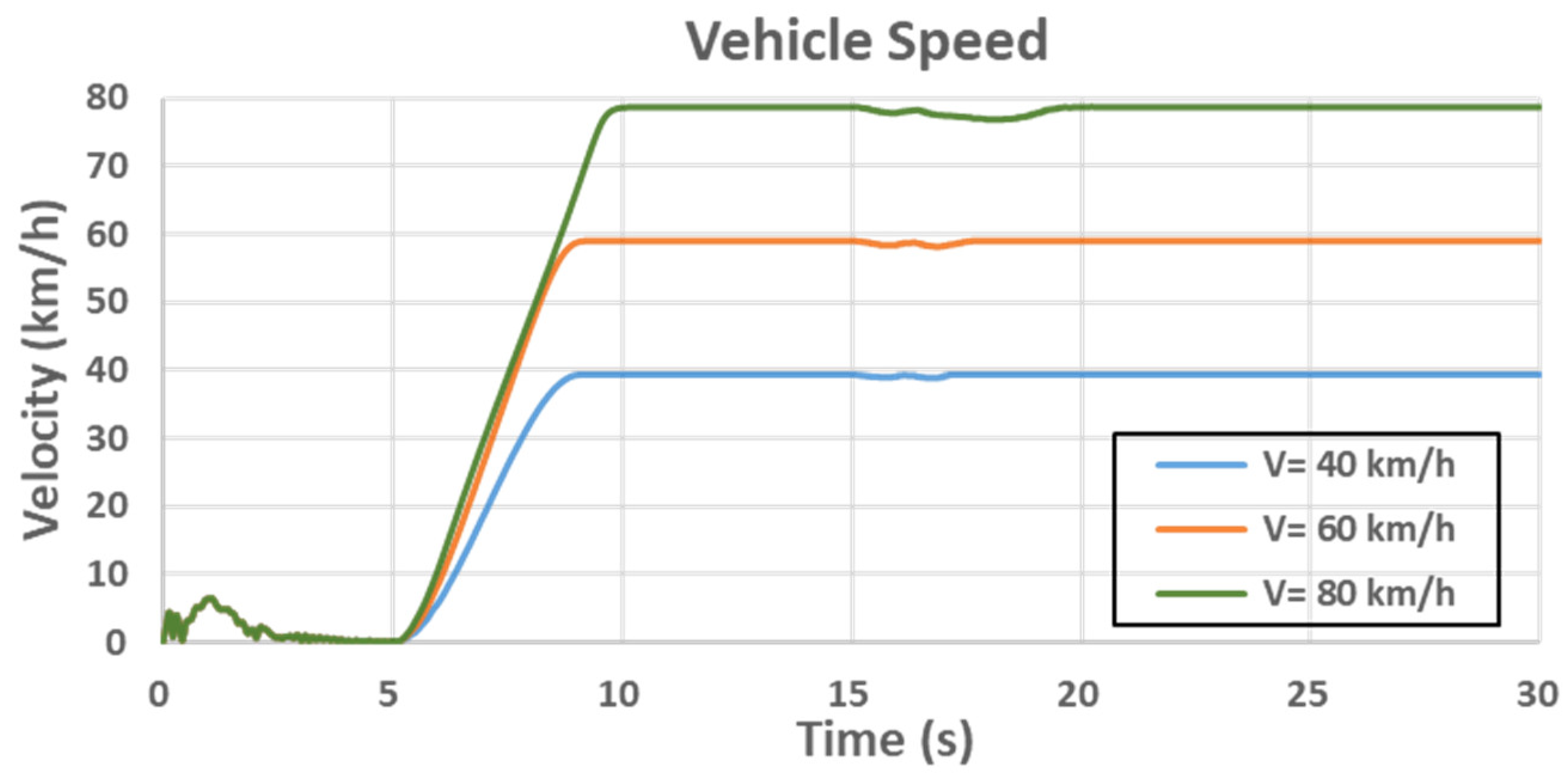
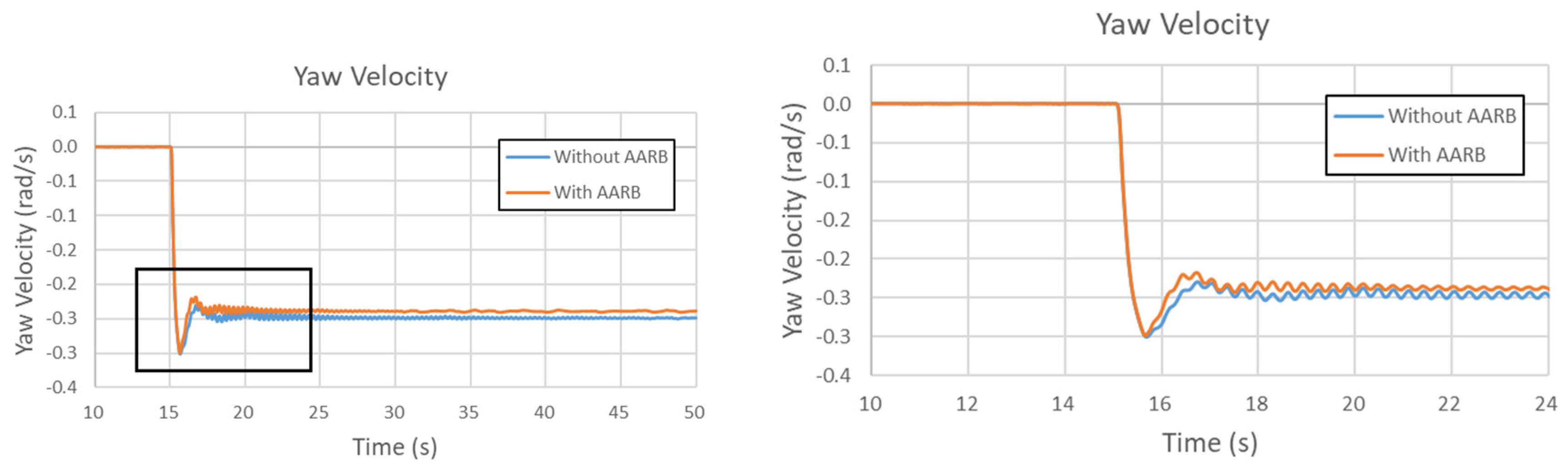
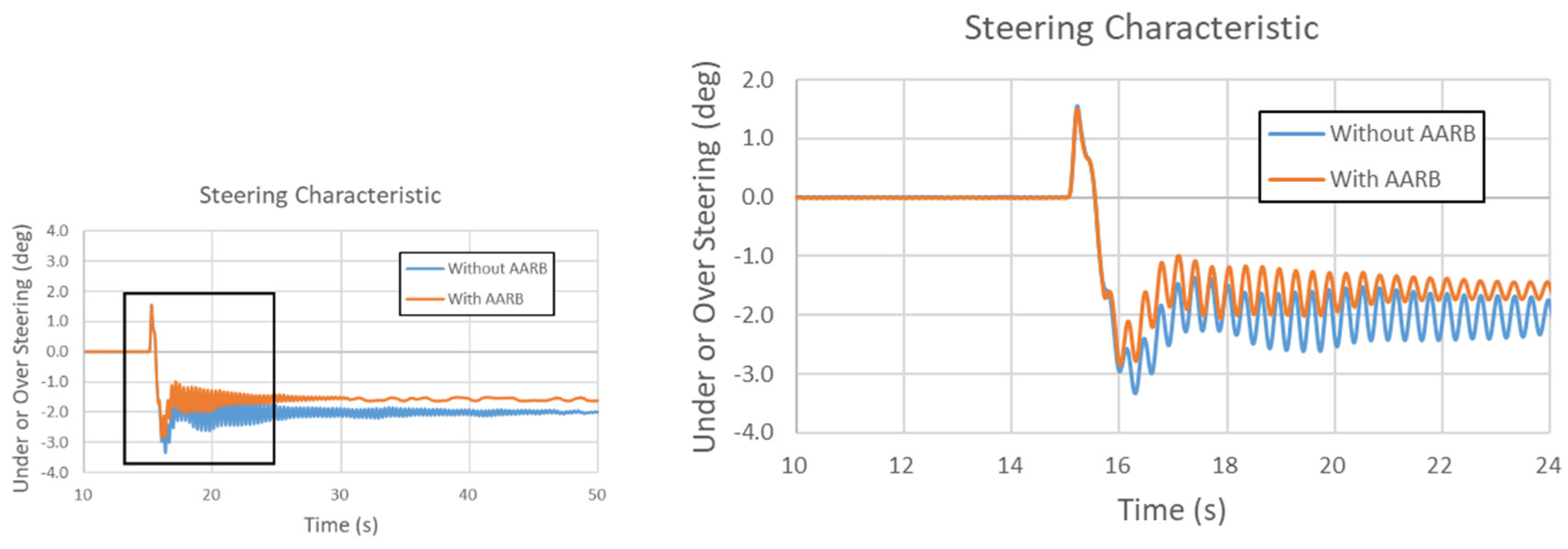
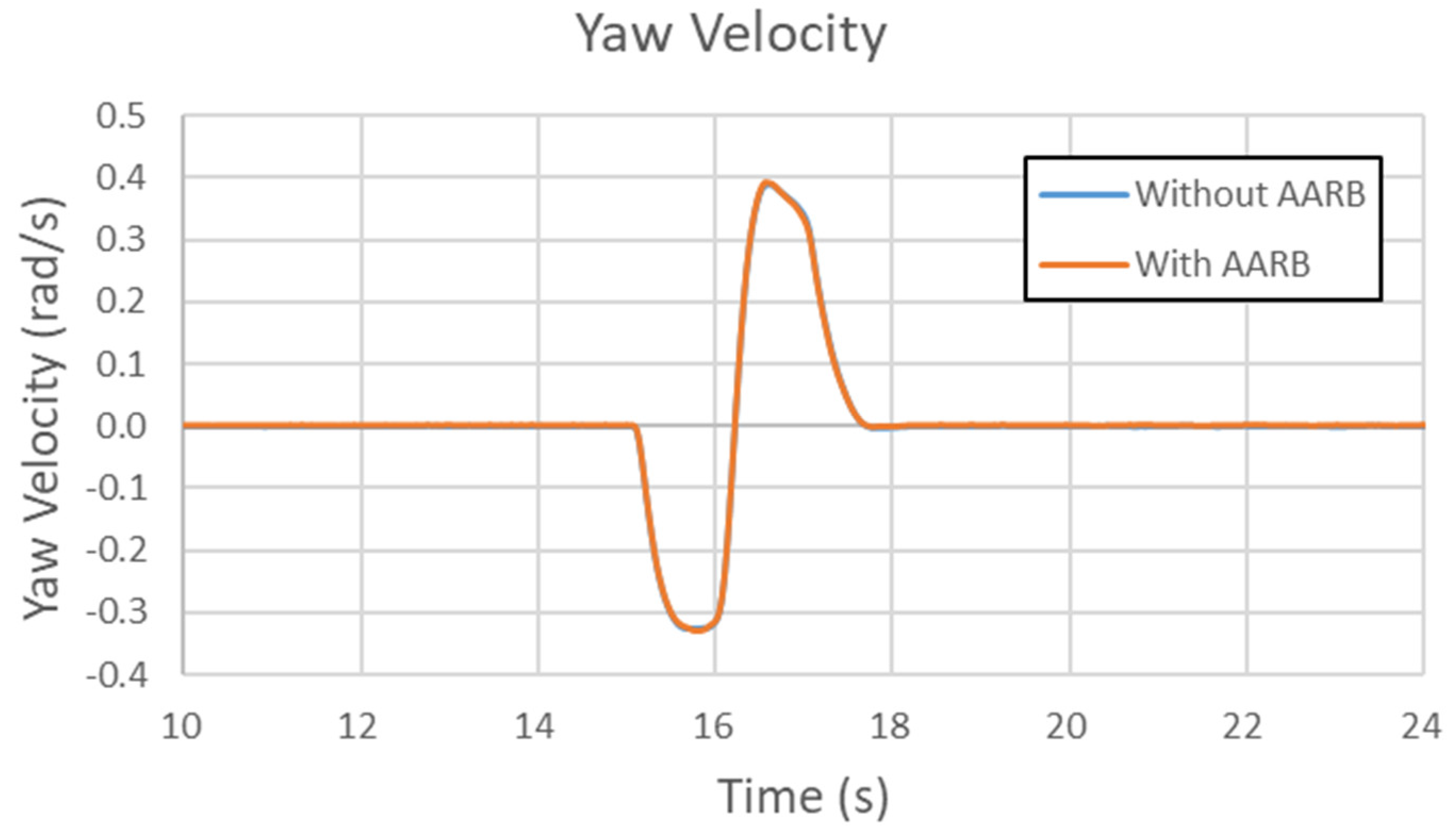
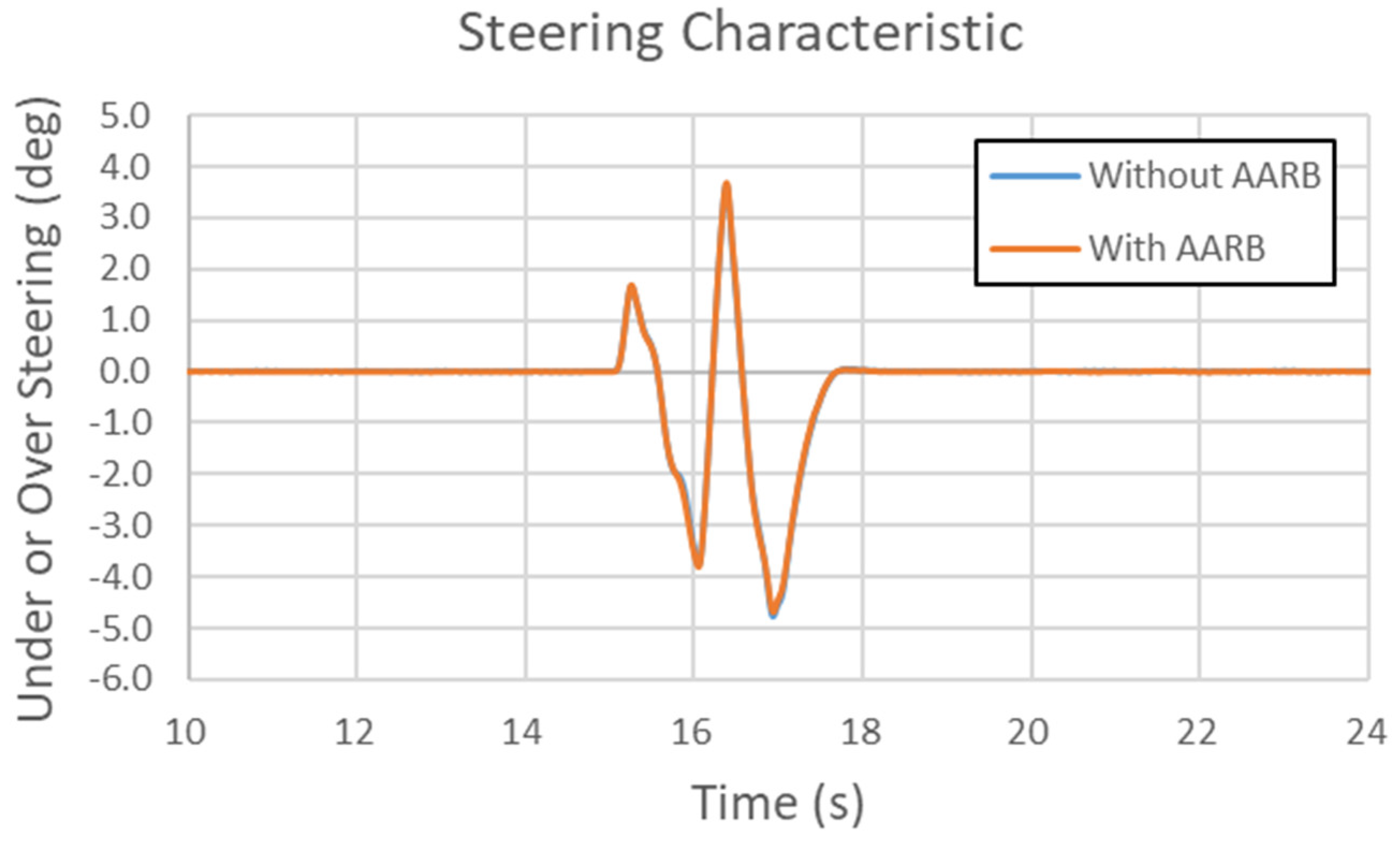
- In the step steering input test, for this medium-size electric bus, understeering occurred at 40 km/h and oversteering occurred at 60 and 80 km/h in both the original case without AARB and the improved case with AARB. In the original case, the time of stabilization for 40 km/h was 6.23 s. The time of stabilization for 60 km/h was 33.67 s. However, for a speed of 80 km/h, the steering characteristic continued changing and was unstable, where the inner wheel lifting occurred which is unwanted unsteady situation.
- (2)
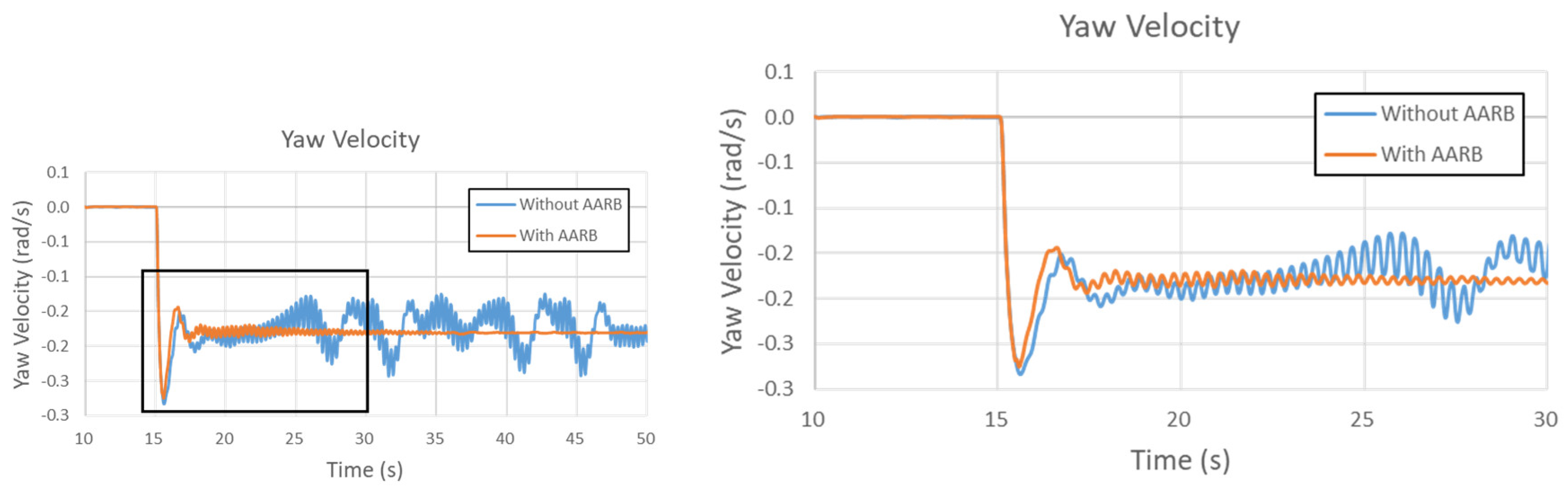
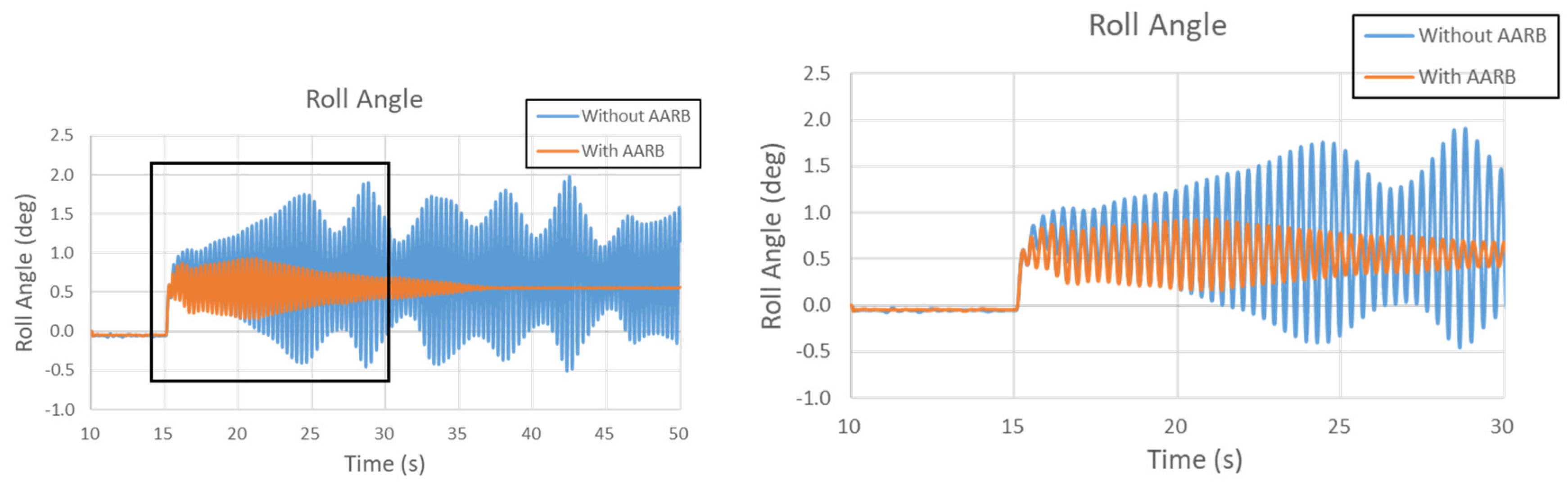
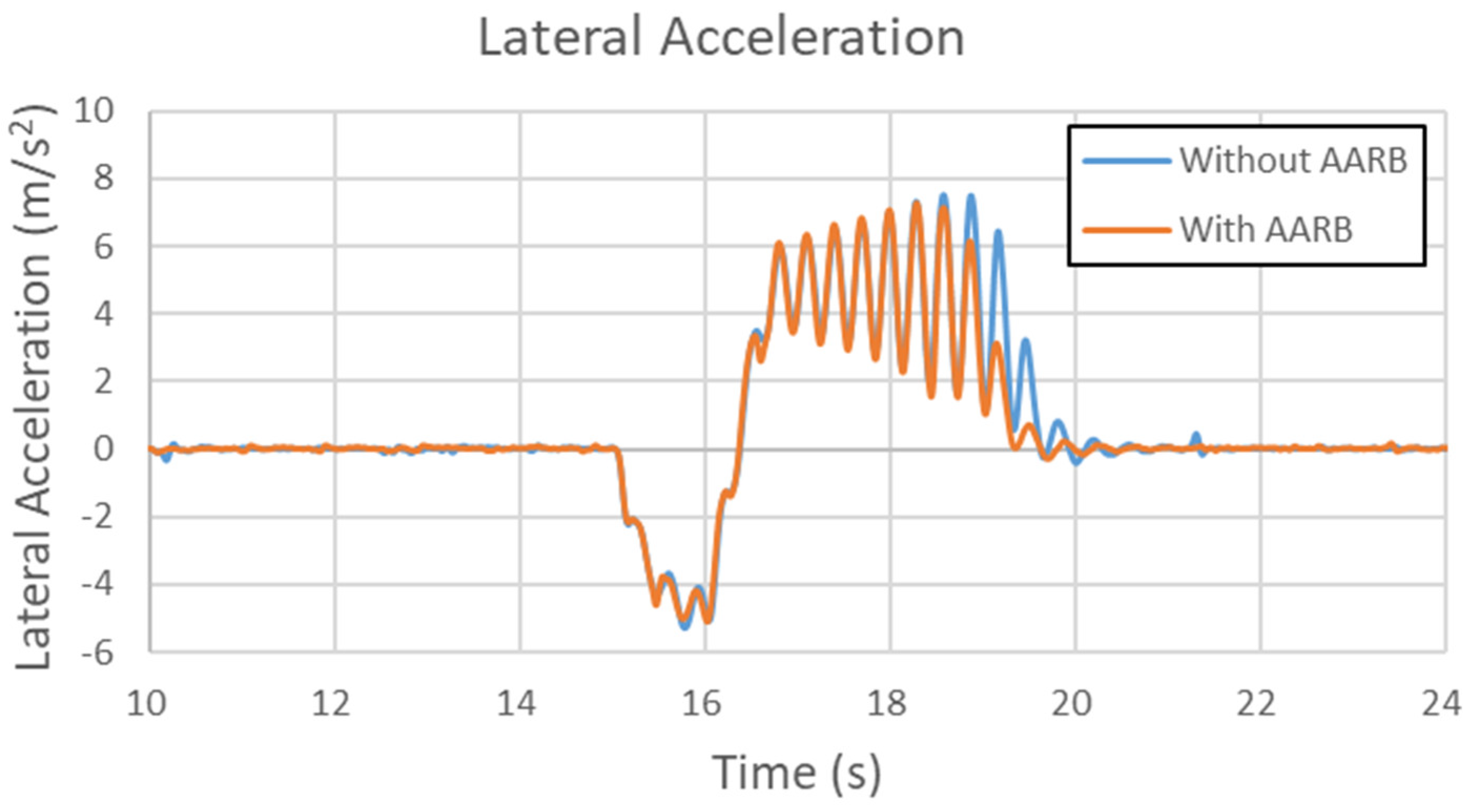
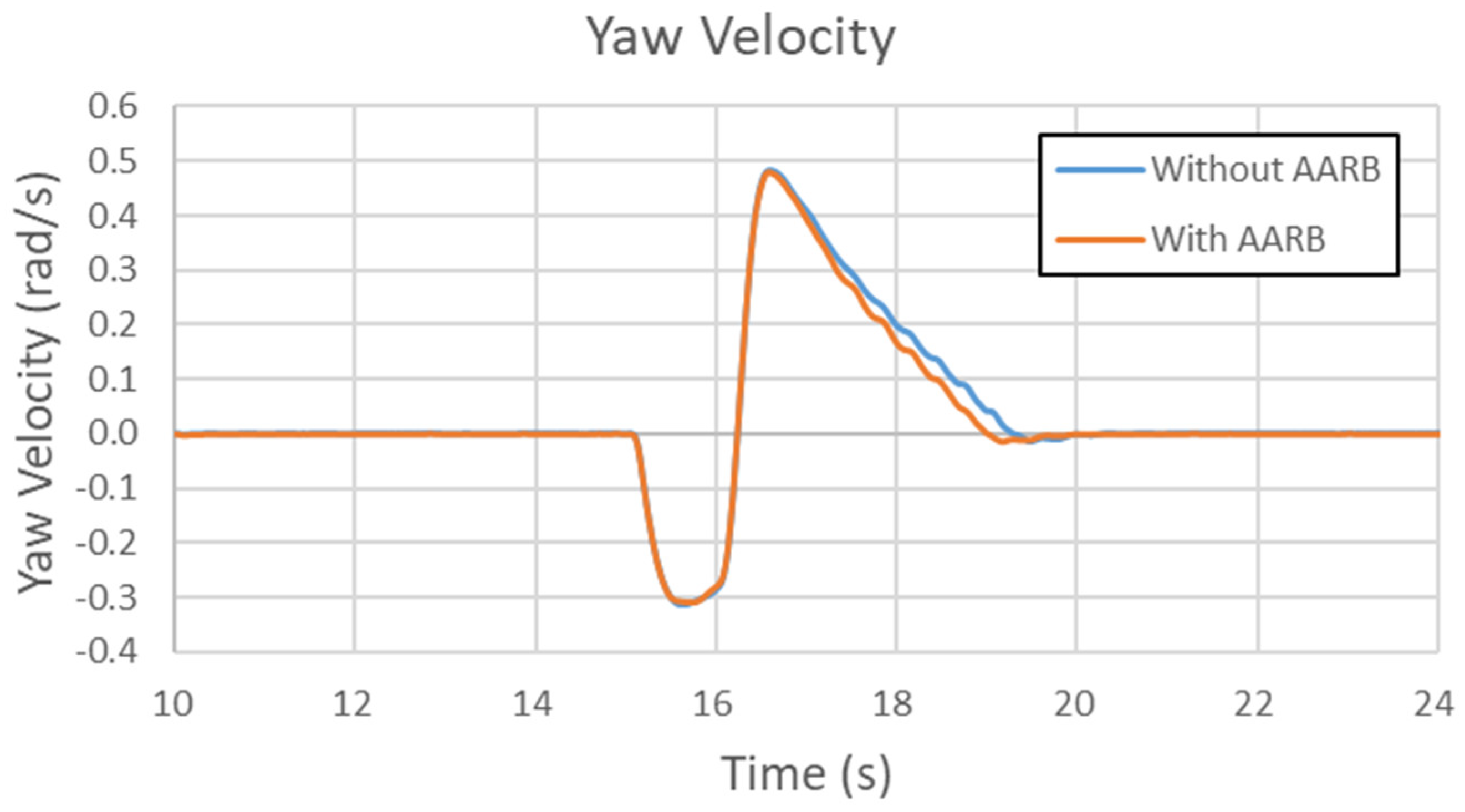
- In the single sinusoidal steering input test of the original vehicle model, the time of stabilization increased with the increase of the vehicle speed. In the 80 km/h test, the steady state restored within three to four seconds after the turn signal ended, and the unsteady situation of the inner wheel lifting also occurred.
- (3)
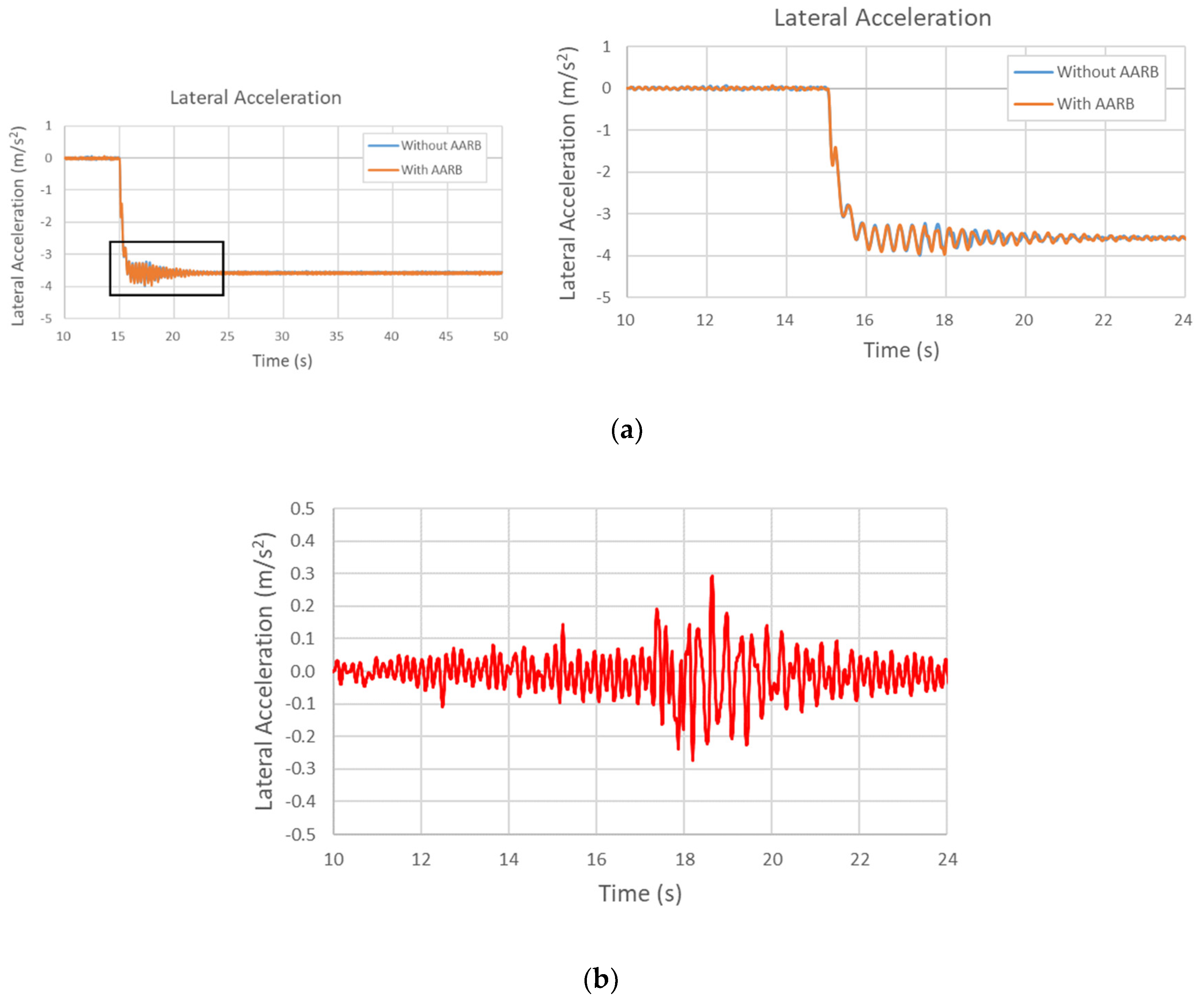
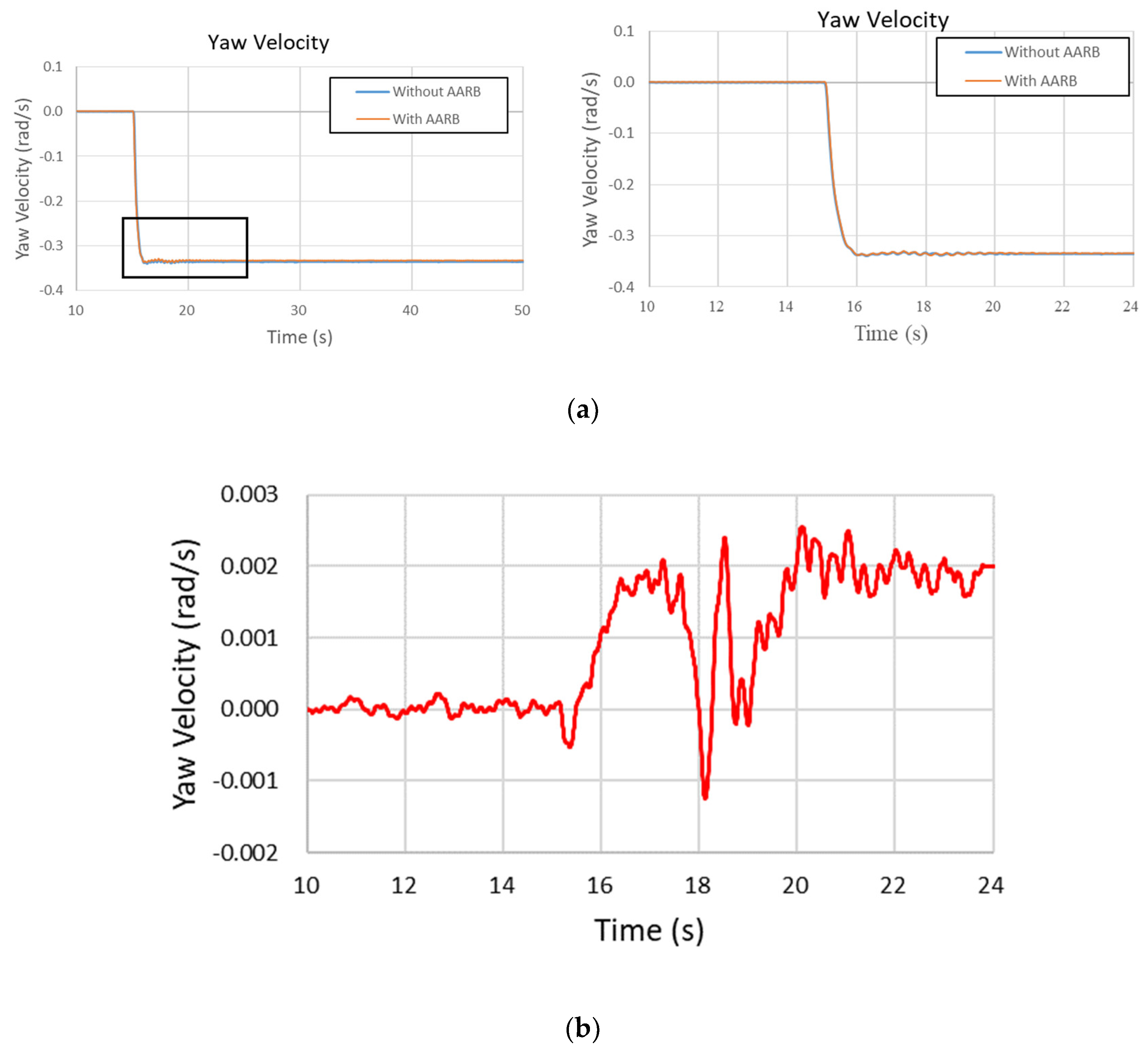
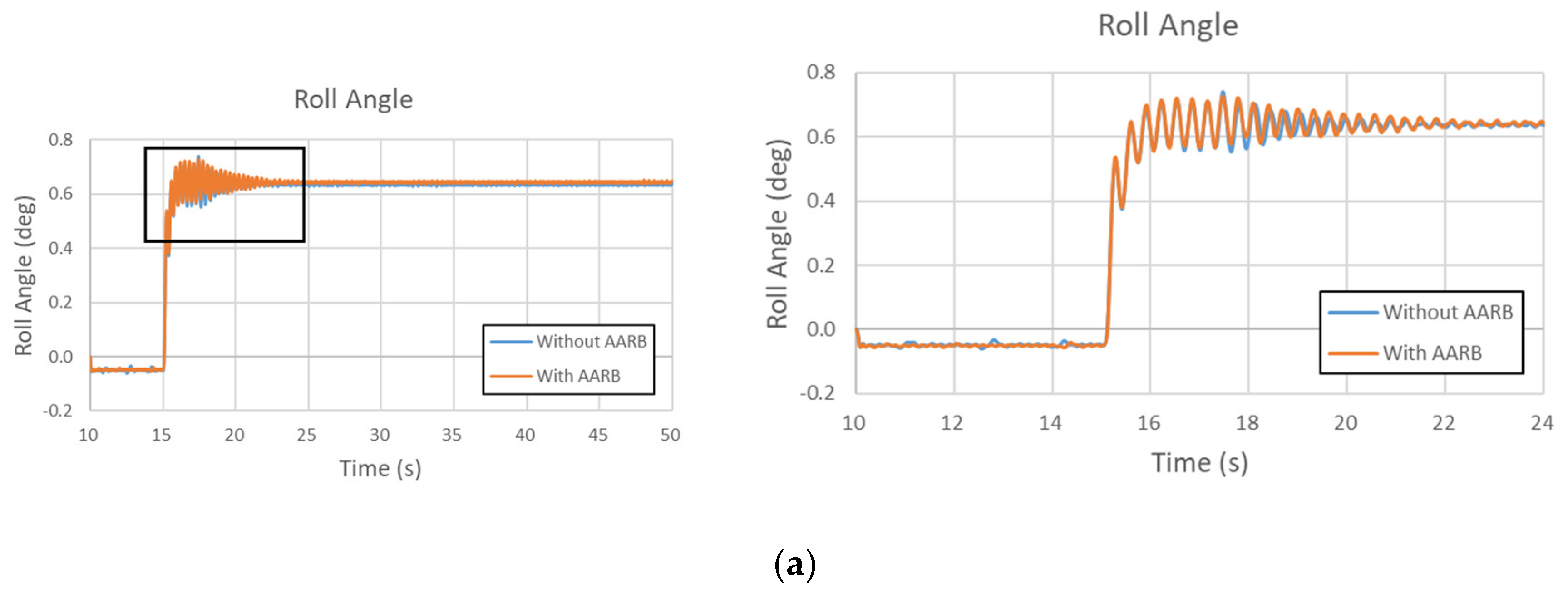
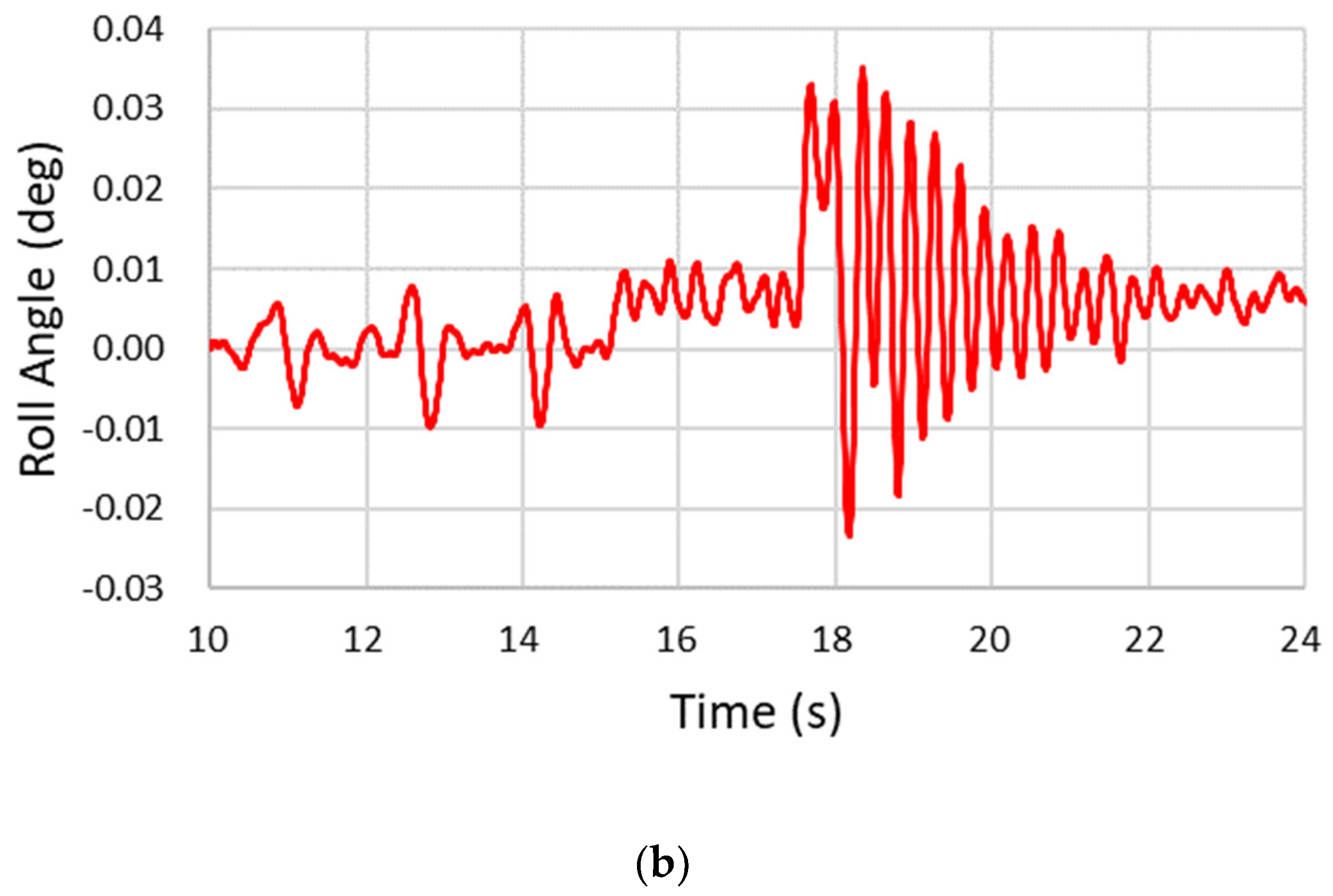
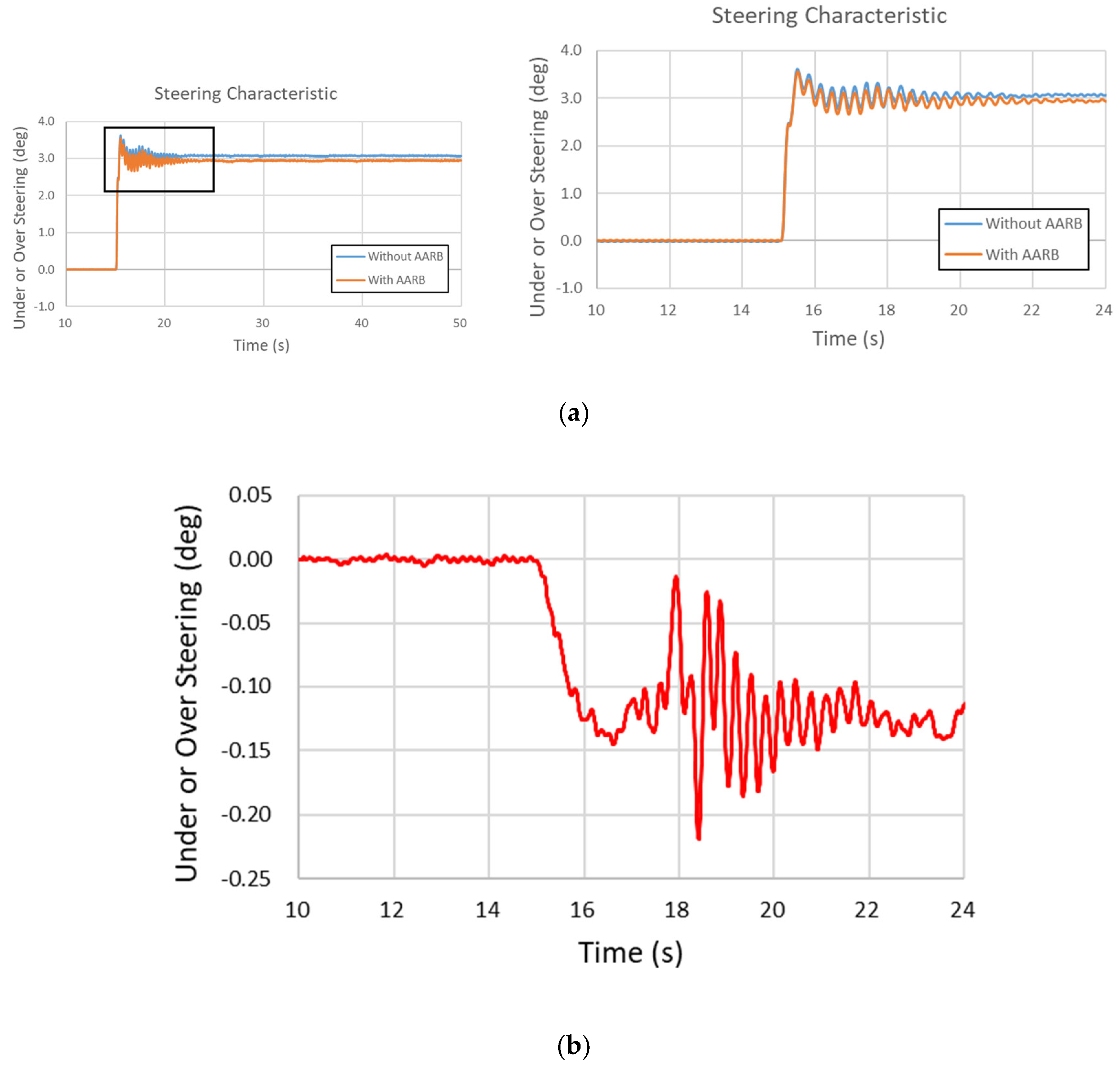
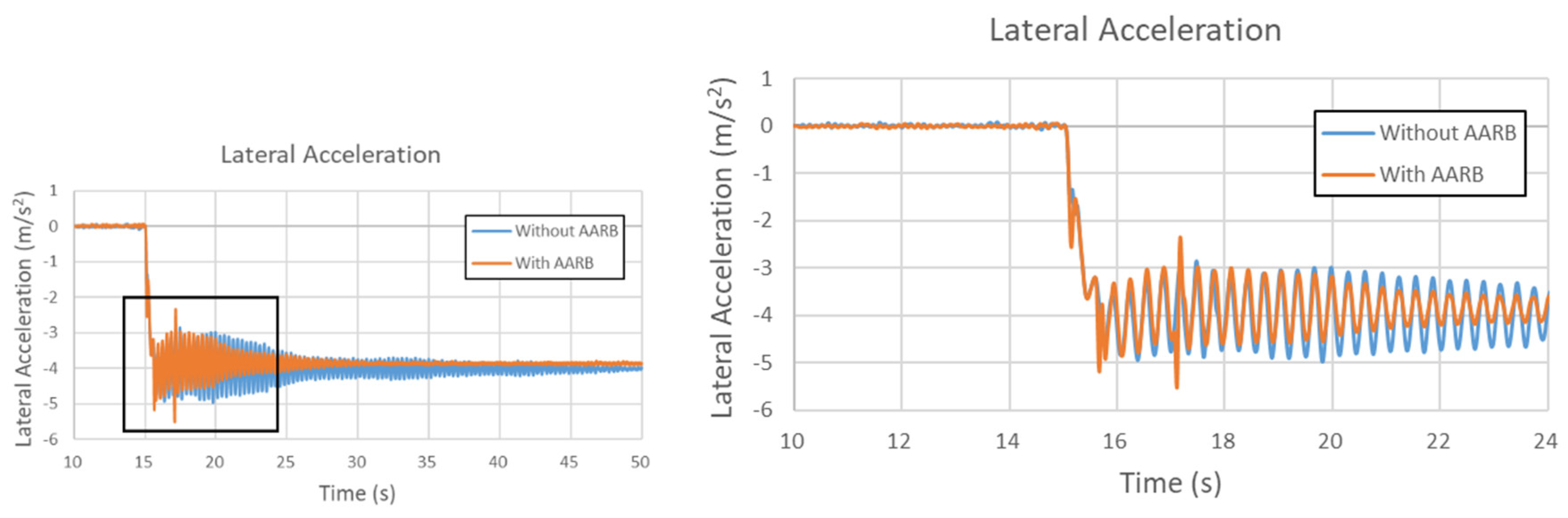
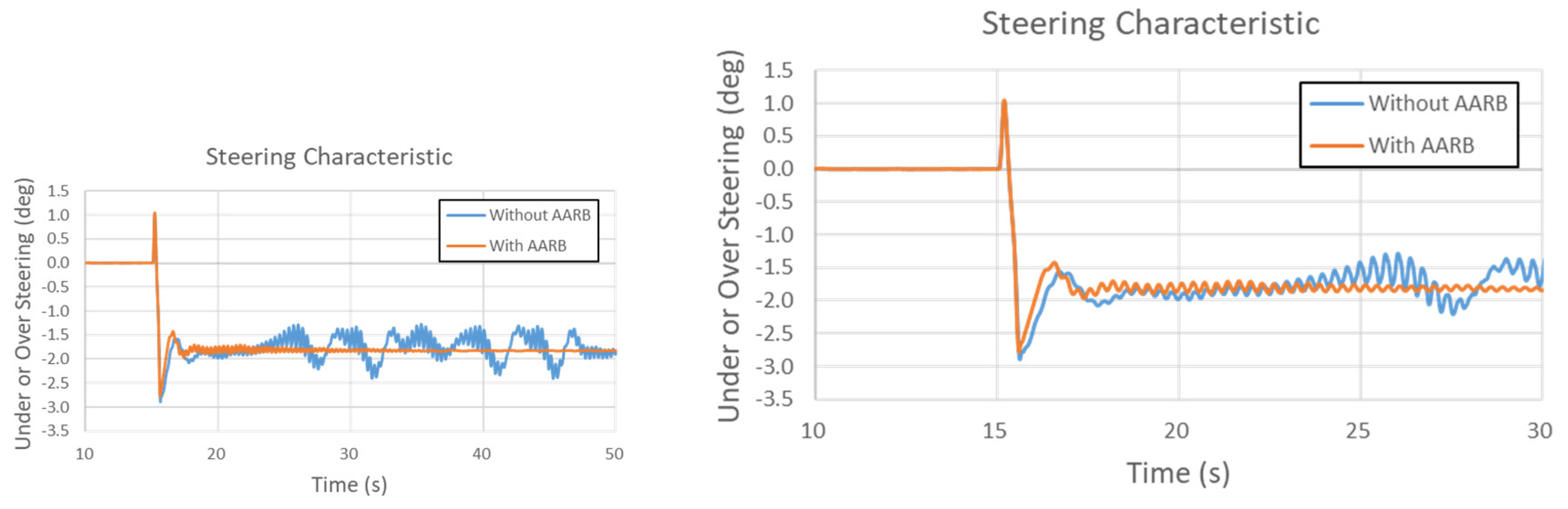
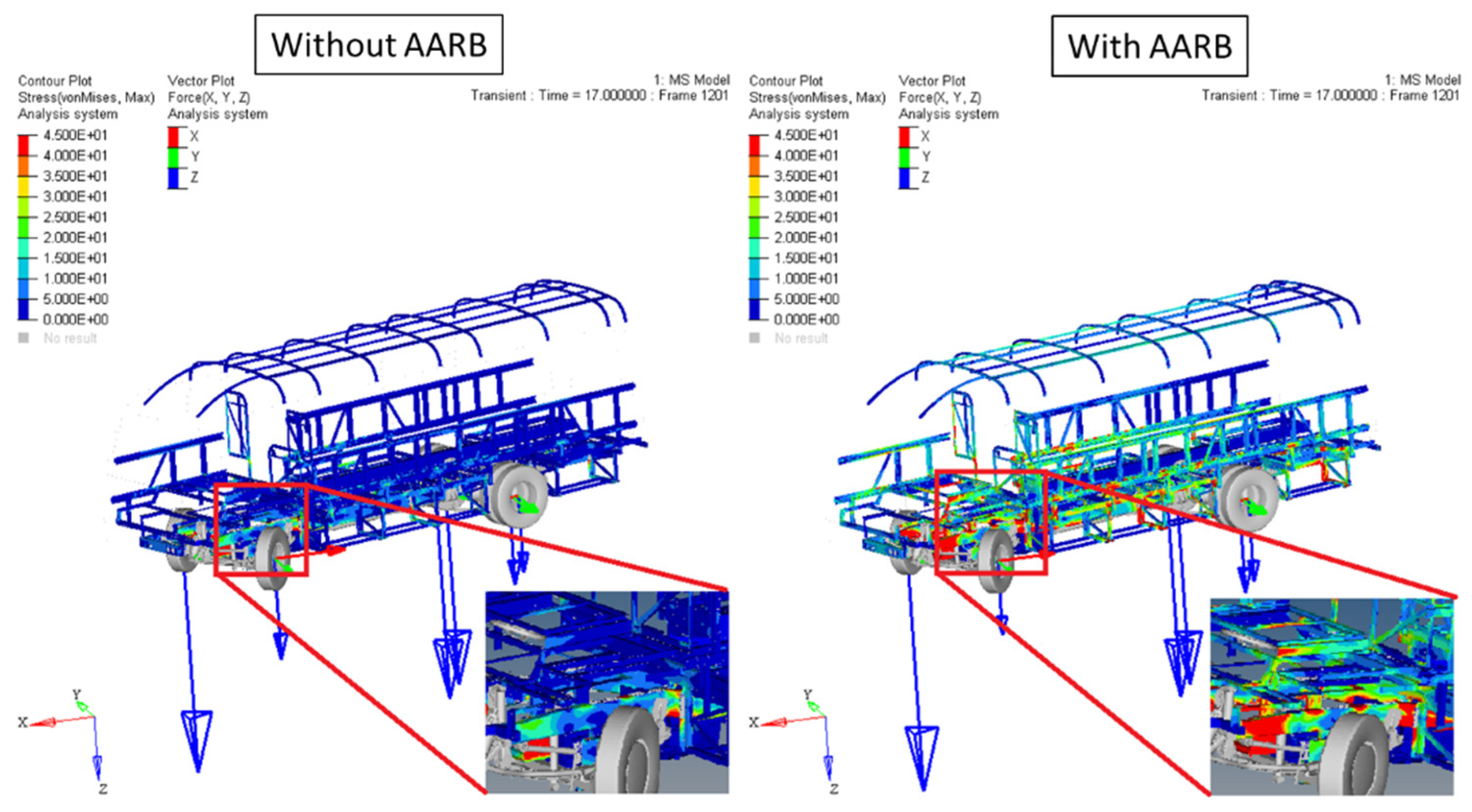
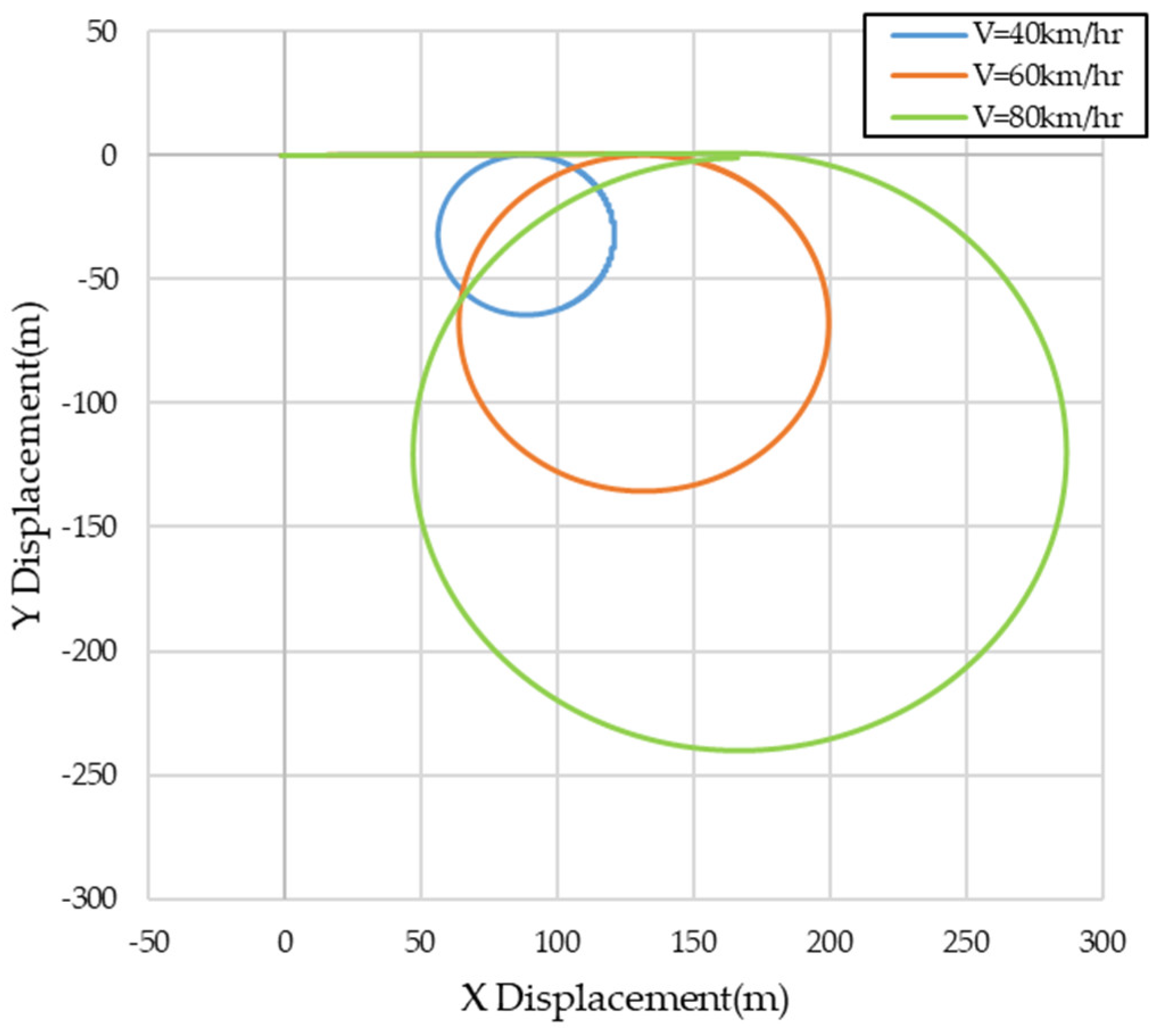
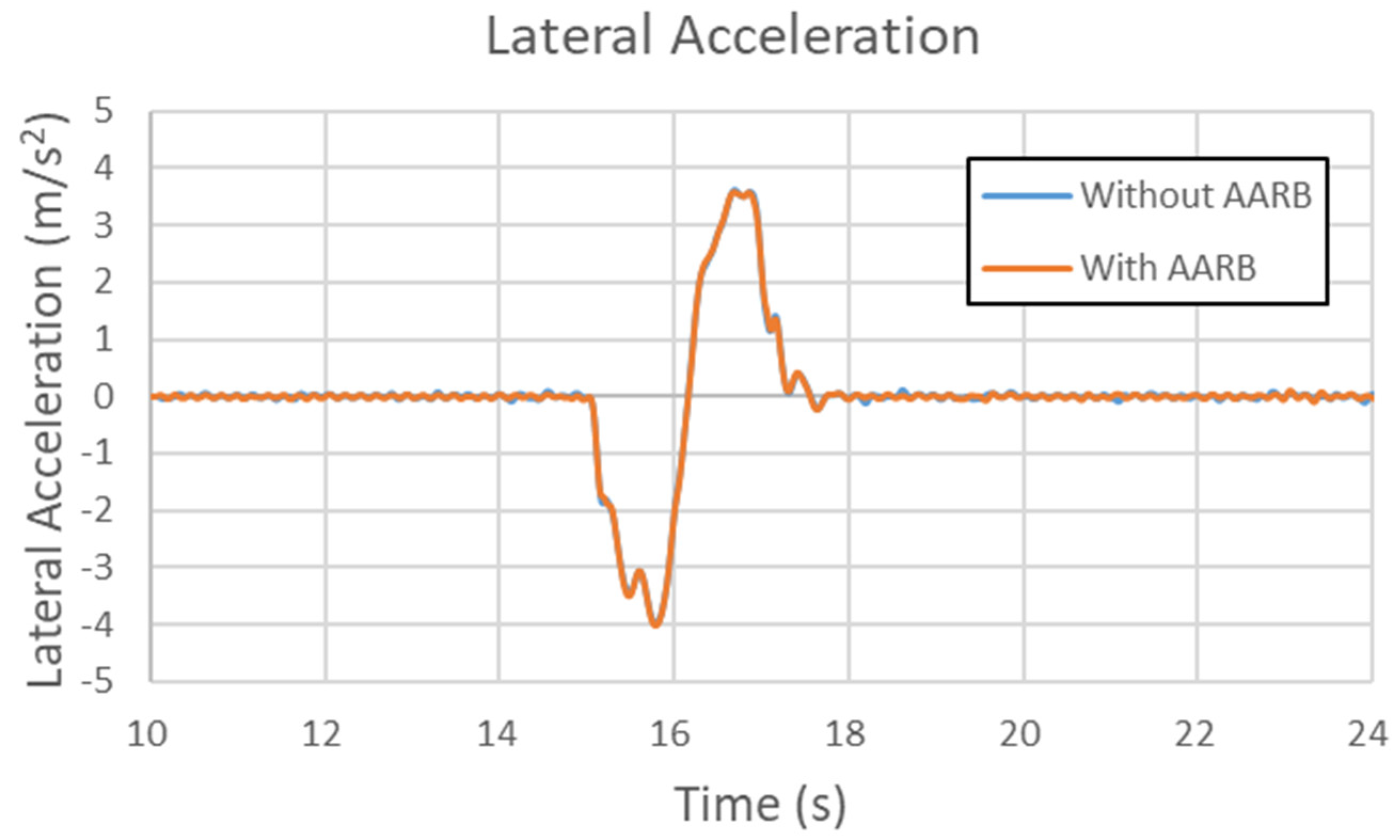
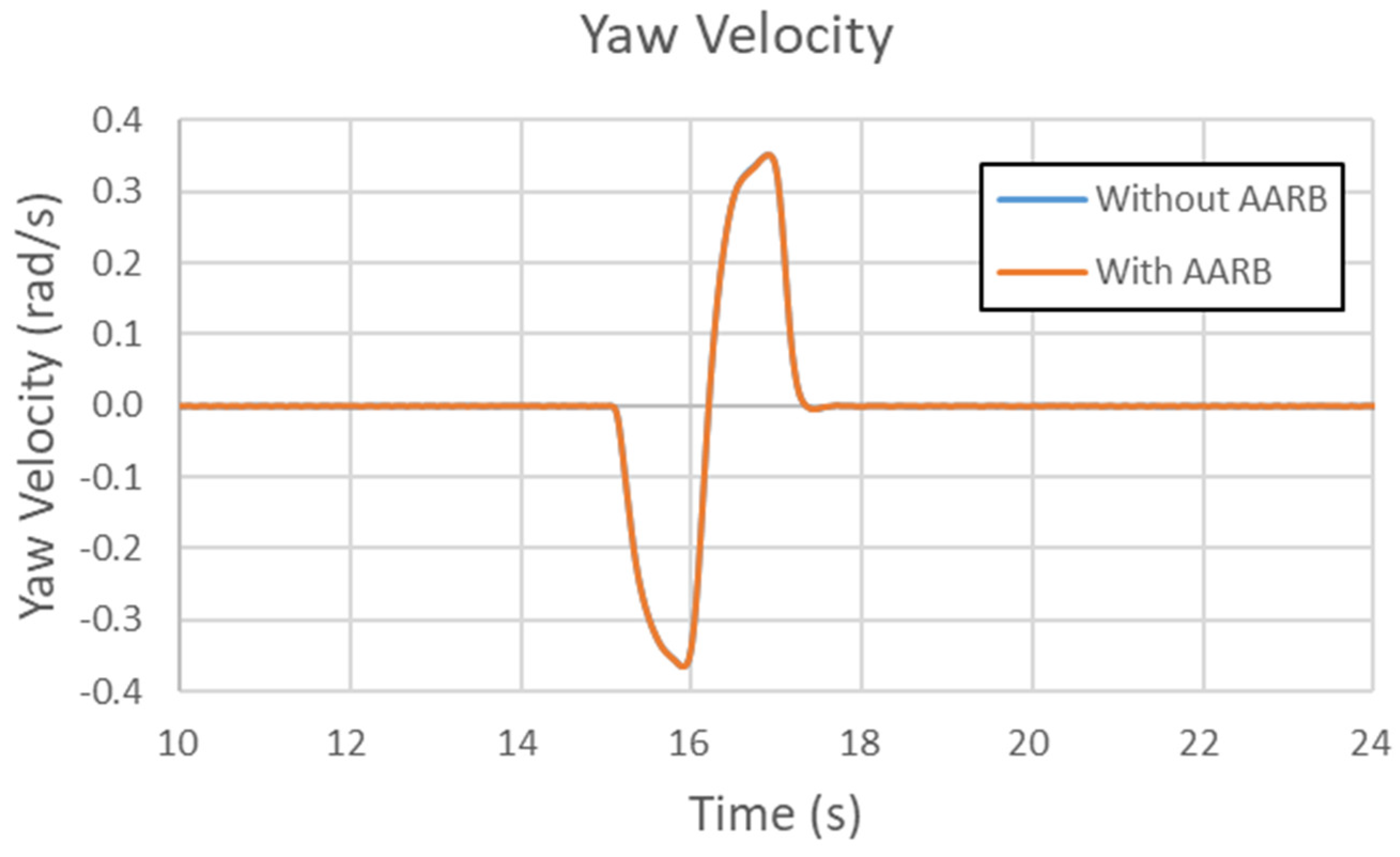
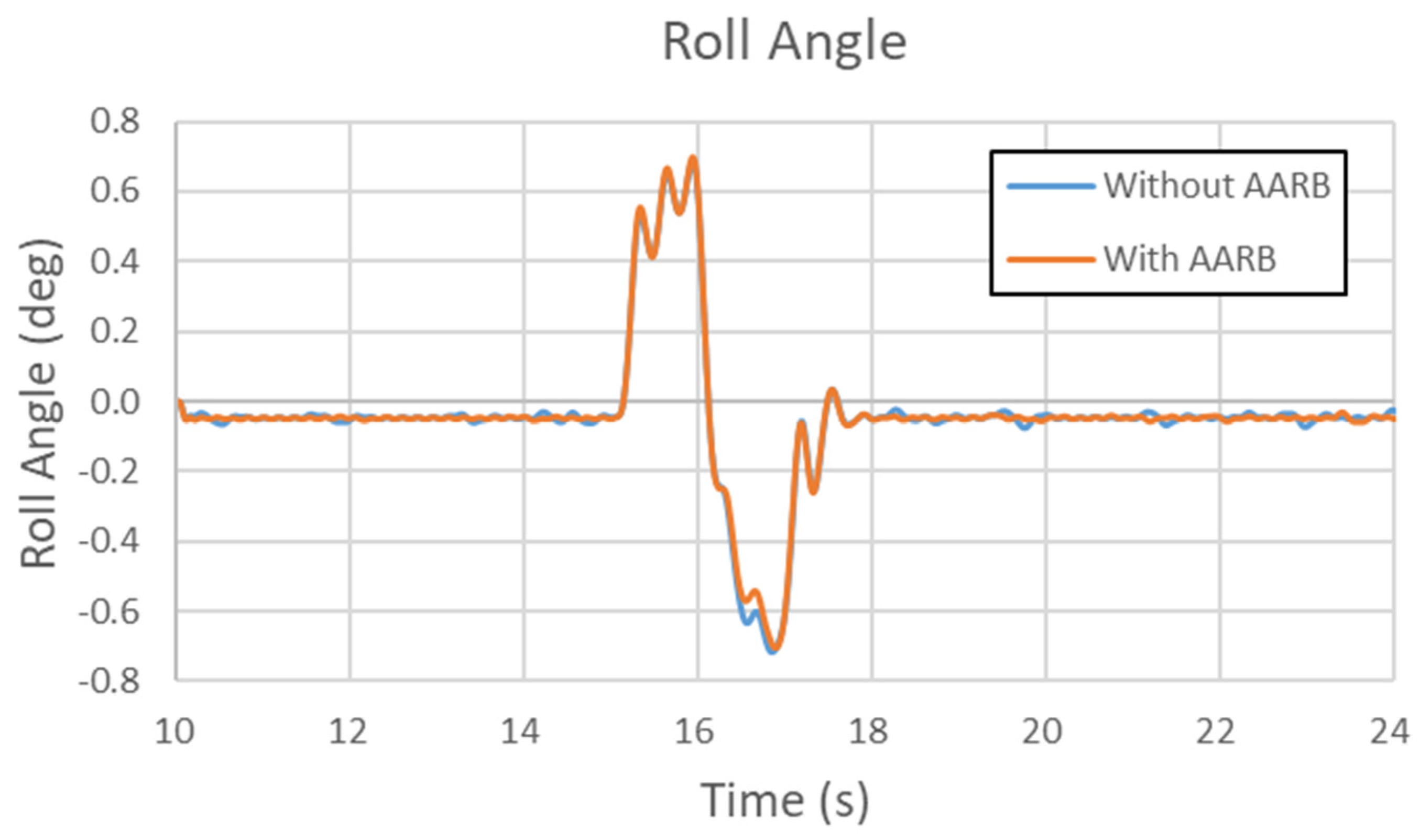
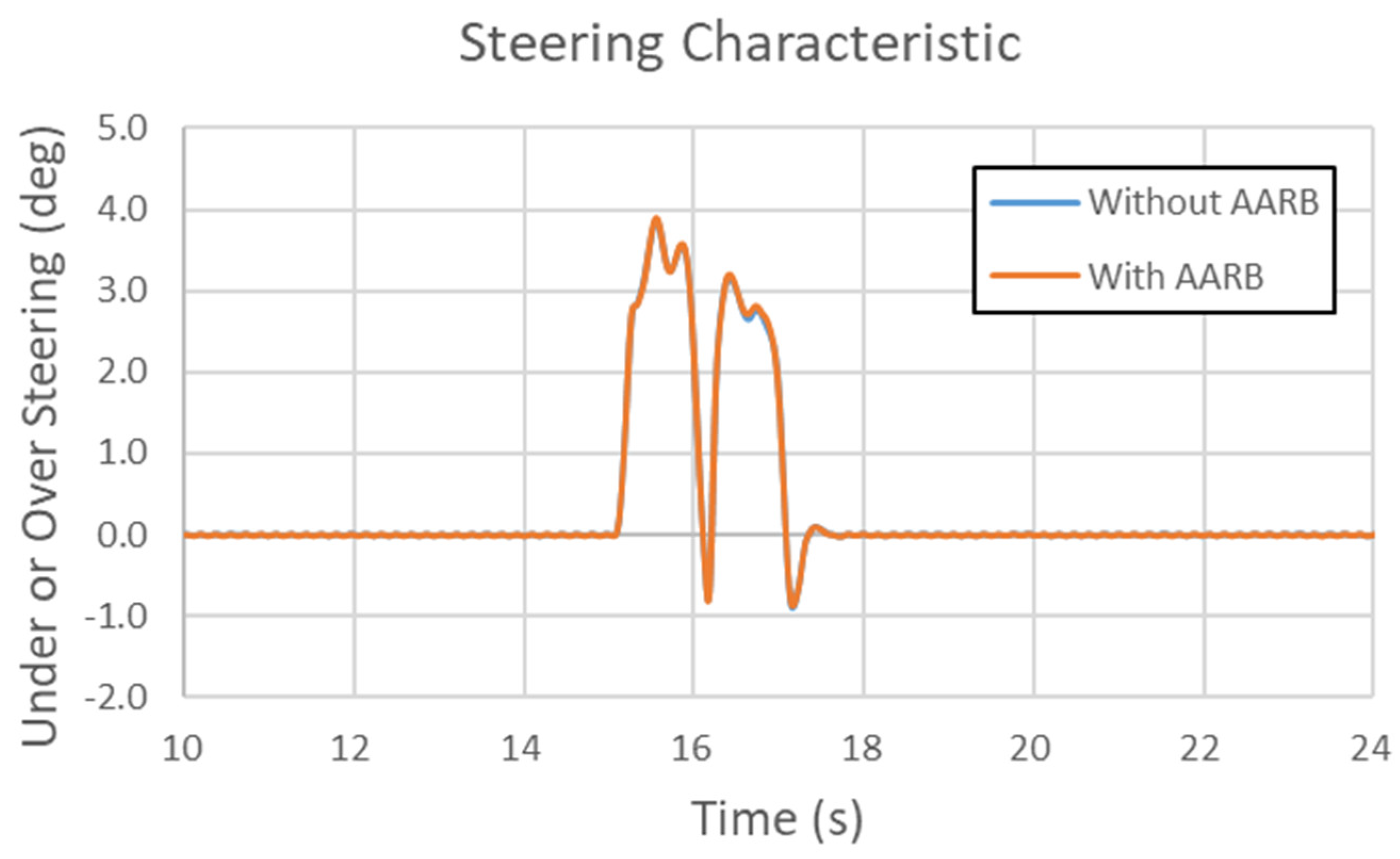
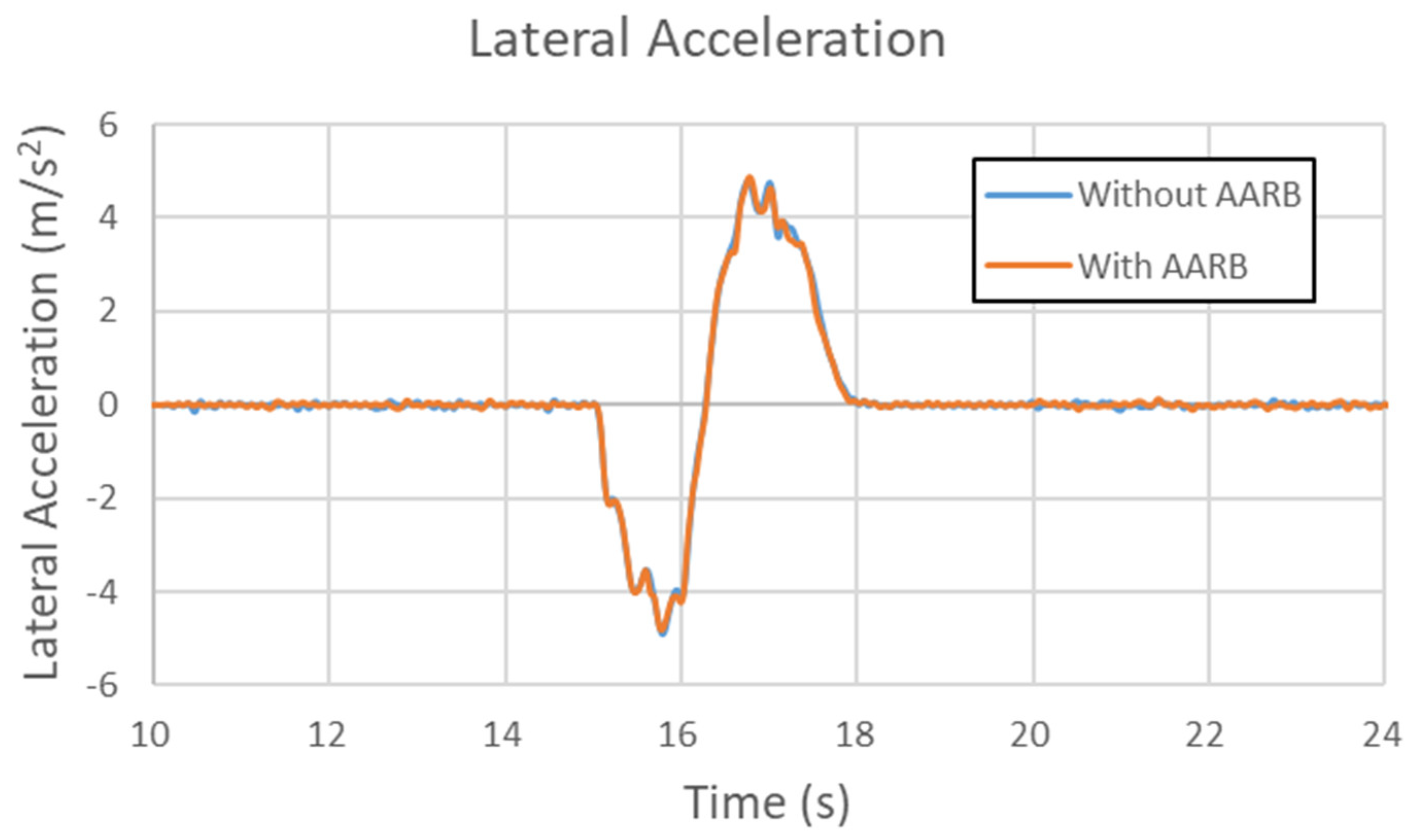
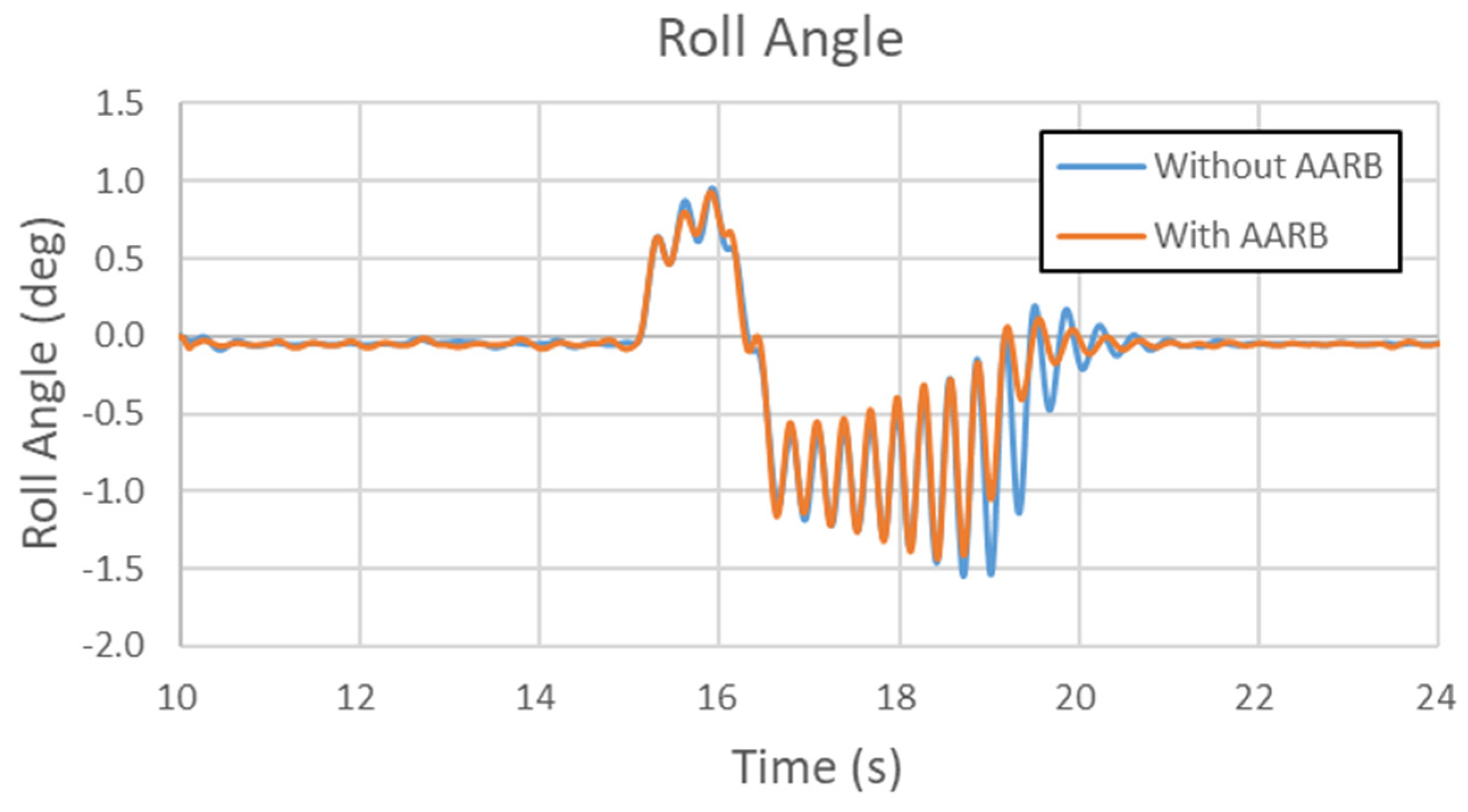
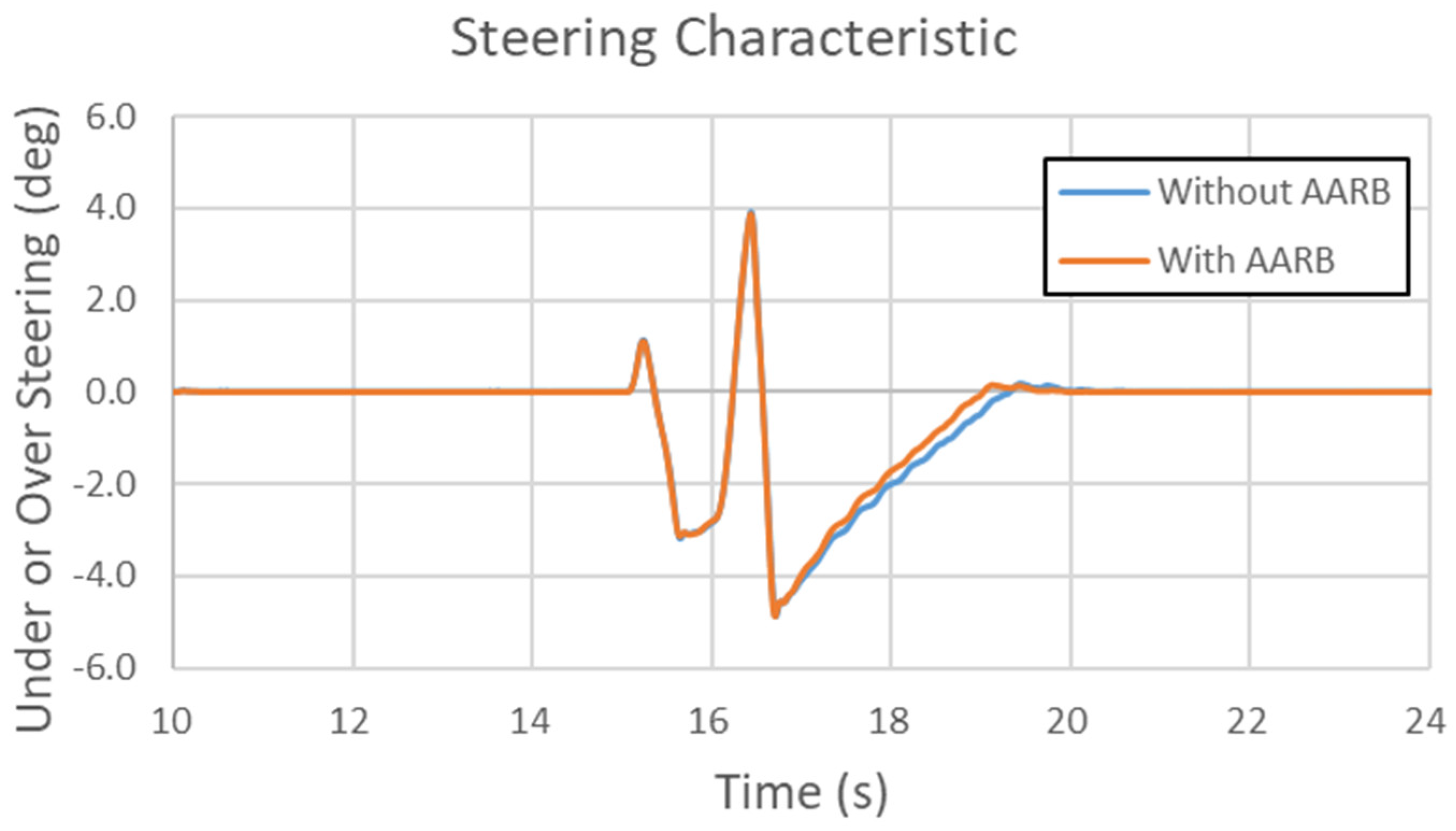
- After the improvement made with the active anti-roll bar system designed by this research, most of the data changed during the step steering input test. In the 40 km/h step steering input test, the yaw velocity reduced 0.002 rad/s, which was about 0.60%; the understeering characteristic was effectively suppressed by 0.12 degree, which is about 3.91%; and the total time of stabilization increased by 0.61 s, which is about 9.79%. In the 60 km/h case, the yaw velocity reduced 0.009 rad/s, which was about 3.63%; the oversteering characteristic was effectively suppressed by 0.45 degree, which was about 22.5%; and the total stabilization time reduced 18.21 s, which is about 54.08%. In the 80 km/h case, the phenomenon of the inner wheel lifting, occurred in the original vehicle, was effectively suppressed and altered the original unstable and dangerous condition to stable. Since the original vehicle is not stable in the 80 km/h step steering input test, an average mean of each performance value was calculated for comparison. In comparison with the mean of original design, the design with active anti-roll bar had a lower yaw velocity than the original one by 1.09%. The diameter of the circle path was reduced by 2.65%.
- (4)
- With the active anti-roll bar design, there was no significant difference from the original one in the yaw velocity and the total stabilization time for the 40 km/h single sinusoidal steering input test. The yaw angle changed between the steering turned and the end of test. In the original design, the change of yaw angle was 0.6 degree, while it was 0.7 degree in the proposed design, increased 0.1 degree, which was about 16.7% to the baseline value. In the test of 60 km/h, there was no significant difference in the yaw velocity and the total stabilization time. In the original design, the change of yaw angle was 4.0 degrees, while it was 3.6 degrees in the proposed design, reduced by 0.4 degrees, which was about 10%. In the test of 80 km/h, the time of stabilization was reduced by 8.43%. In the original design, the change of yaw angle was 26.0 degrees, while it was 22.2 degrees in the proposed design, reduced by 3.8 degrees, which was about 14.6%.
Author Contributions
Funding
Conflicts of Interest
References
- Ambrósio, J.A.C.; Gonçalves, J.P.C. Complex Flexible Multibody Systems with Application to Vehicle Dynamics. Multibody Syst. Dyn. 2001, 6, 163–182. [Google Scholar] [CrossRef]
- Suh, K.H.; Lee, Y.K.; Yoon, H.S. Dynamic Stress Analysis of a Vehicle Frame by Flexible Multibody Dynamics Simulation. In Proceedings of the SAE 2001 World Congress, Detroit, MI, USA, 5–8 March 2010. [Google Scholar]
- Ma, T.; Zuo, A.; Wang, D.; Chen, S.; Dong, J. Ride Comfort Analysis of Commercial Vehicle Using Flexible Multi-body and Finite Element Methods. In Proceedings of the 2010 International Conference on Mechanical and Electrical Technology, Singapore, 10–12 September 2010; pp. 295–299. [Google Scholar]
- Gonçalves, J.P.C.; Ambrósio, J.A.C. Road vehicle modeling requirements for optimization of ride and handling. Multibody Syst. Dyn. 2005, 13, 3–23. [Google Scholar]
- Mirone, G. Multi-body elastic simulation of a go-kart: Correlation between frame stiffness and dynamic performance. Int. J. Automot. Technol. 2010, 11, 461–469. [Google Scholar] [CrossRef]
- Shiiba, T.; Ashari, M.F.B.; Yano, M.; Takada, Y. Evaluations of the Racing Kart Dynamic Behavior with Flexible Multibody Dynamic Analysis. In Proceedings of the ASME 2011 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Washington, DC, USA, 28–31 August 2011; Volume 4, pp. 609–615. [Google Scholar]
- Guo, D.L.; Hu, H.Y.; Yi, J.Q. Neural Network Control for a Semi-Active Vehicle Suspension with a Magnetorheological Damper. J. Vib. Control. 2004, 10, 461–471. [Google Scholar] [CrossRef]
- Azadi, S.; Vaziri, M.; Hoseini, M. Vehicle dynamic control of a passenger car applying flexible body model. Veh. Syst. Dyn. 2010, 48, 587–617. [Google Scholar] [CrossRef]
- Zhang, J.Y.; Wang, S.F. The virtual experiment on vehicle handling stability. In Proceedings of the 2007 14th Asia Pacific Automotive Engineering Conference, Hollywood, CA, USA, 5–8 August 2007. [Google Scholar]
- Angel, L.M.; Antonio, J.N.; Jose’, M.C.; Publio, P. A Semi-Active Vehicle Suspension Based on Pneumatic Springs and Magnetorheological Dampers. J. Vib. Control. 2010, 24, 808–821. [Google Scholar]
- Darling, J.; Hickson, L.R. An Experimental Study of a Prototype Active Anti-Roll Suspension System. Veh. Syst. Dyn. 2007, 29, 309–329. [Google Scholar] [CrossRef]
- Cronje’, P.H.; Els, P.S. Improving off-road vehicle handling using an active anti-roll bar. J. Terramechanics 2010, 47, 179–189. [Google Scholar] [CrossRef]
- Vu, V.; Sename, O.; Dugard, L.; Gáspár, P. Active anti-roll bar control using electronic servo valve hydraulic damper on single unit heavy vehicle. IFAC-PapersOnLine 2016, 49, 418–425. [Google Scholar] [CrossRef]
- Danesin, D.; Krief, P.; Sorniotti, A.; Velardocchia, M. Active Roll Control to Increase Handling and Comfort. SAE Trans. 2003, 1007–1017, SAE paper 2003-01-0962. [Google Scholar]
- Struss, F.J.; Urbach, B.A. Variable Stiffness Anti-Roll Bar. U.S. 122571, 24 July 1998. [Google Scholar]
- Lin, Y.L. Vibration Reduction of Electric Bus Driveline System. Master’s Thesis, National Taipei University of Technology, Taipei, Taiwan, 2014. [Google Scholar]
- Yang, C.N. Simulation and Analysis of Steering Test with Flex-Body Dynamics Model. Master’s Thesis, National Taipei University of Technology, Taipei, Taiwan, 2015. [Google Scholar]
- Craig, R.R.; Bampton, M.C. Coupling of Substructures for Dynamic Analysis. AIAA J. 1968, 6, 1313–1319. [Google Scholar] [CrossRef]
- Krattiger, D.; Wu, L.; Zacharczuk, M.; Buck, M.; Kuether, R.J.; Allen, M.S.; Tiso, P.; Brake, M.R. Interface Reduction for Hurty/Craig-Bampton Substructured Models: Review and Improvements. Mech. Syst. Signal Process. 2019, 114, 579–603. [Google Scholar] [CrossRef]
- Medium Bus RAC-300. Available online: www.racev.com.tw/product/k5 (accessed on 29 October 2019).
- ISO 7401:2011 Road Vehicles—Lateral Transient Response Test Methods—Open-Loop Test Methods. Available online: Iso.org/standard/54144.html (accessed on 29 October 2019).
- ISO/TR 8725:1988 Road vehicles—Transient Open-Loop Response Test Method with One Period of Sinusoidal Input. Available online: Iso.org/standard/16128.html (accessed on 29 October 2019).
- Gillespie, T. Fundamentals of Vehicle Dynamics; SAE: Warrendale, PA, USA, 1992. [Google Scholar]








| Parameters | RAC-300 |
|---|---|
| Dimensions L*W*H (mm mm mm) | 7000 × 2135 × 2603 |
| Wheel Base (mm) | 3800 |
| Curb Weight (N) | 58,958 |
| Vehicle Load (N) | 16,579 |
| GVWR (N) | 75,537 |
| Front Axle Load (N) | 30,794 |
| Rear Axle Load (N) | 44,773 |
| Passengers (seat/stand) | 19 + 1/10 |
| Top Speed (km/h) | >100 |
| Max. Climbing Angle (%) | >30% (full load) |
| Charging Time (h) | 6–7 h (30 Kw) |
| Battery Type | Yttrium Lithium Battery |
| Battery Capacity (kWh) | 107 |
| Motor Type | AC Induction Air-cooled |
| Motor Power (kW) | Rated 75/Peak 150 |
| Motor Torque (Nm) | Rated 284.5/Max 706 |
| Front/Rear Axle | 2500/5000 |
| Braking Systems | Air Brake + WABCO ABS |
| Assisted Braking | Regenerative Braking |
| Gearbox | 6-Speed MT |
| Vehicle Operating System | BMS/VCU |
| Component 1 | Component 2 | Connect Method |
|---|---|---|
| Transmission | Front driveshaft | Y-Z Universal joint |
| Front driveshaft | Rear driveshaft | Y-Z Universal joint |
| Rear driveshaft | Differential | Y-Z Universal joint |
| Component 1 | Component 2 | Connect Method |
|---|---|---|
| Steering gearbox output | Pitman arm | Y rotational joint |
| Pitman arm | Drag link | Y-Z Universal joint |
| Drag link | Left steering arm | Y-Z Universal joint |
| Left steering arm | Left Tie rod | Z rotational joint |
| Left tie rod | Left wheel hub | Z rotational joint (left-front Kingpin) |
| Right steering arm | Right tie rod | Z rotational joint |
| Right tie rod | Right wheel hub | Z rotational joint (right-front Kingpin) |
| Materials Properties | AISI5160 Manganese Steel |
|---|---|
| Elastic coefficient E (MPa) | 205,000 |
| Poisson’s ratio | 0.29 |
| Yield strength (MPa) | 275 |
| Component 1 | Component 2 | Connect Method |
|---|---|---|
| Chassis rail | Front leaf spring hanger | Fixed joint |
| Front leaf spring hanger | Leaf spring 1 | Y rotational joint |
| Leaf spring n (n = 1–31) | Leaf spring n + 1 | Fixed joint |
| Leaf spring 29 | Wheel hub | Fixed joint |
| Leaf spring 32 | Leaf spring shackle | Y rotational joint |
| Leaf spring shackle | Rear leaf spring hanger | Y rotational joint |
| Rear leaf spring hanger | Chassis rail | Fixed joint |
| Chassis rail | Shock absorbers damping cylinder | Y rotational joint |
| Shock absorbers damping cylinder | Shock absorbers piston rod | Z cylindrical joint (damper) |
| Shock absorbers piston rod | Wheel hub | Y rotational joint |
| Equivalent vertical stiffness of the front leaf spring | 490.15 N/mm |
| Equivalent vertical stiffness of the rear leaf spring | 146.96 N/mm |
| Damping coefficient of the front shock absorbers | 315 Ns/mm |
| Damping coefficient of the rear shock absorbers | 525 Ns/mm |
| Component 1 | Component 2 | Connect Method |
|---|---|---|
| Left connecting rod | Left wheel hub assembly | Y-Z universal joint |
| Left arm | Left connecting rod | Ball joint |
| Left half anti-roll bar | Left arm | Fixed joint |
| Left half anti-roll bar | Left chassis rail | Y revolute joint |
| Left half anti-roll bar | Right half anti-roll bar | Y revolute joint (with Torsion spring-damper) |
| Right half anti-roll bar | Right chassis rail | Y revolute joint |
| Right half anti-roll bar | Right arm | Fixed joint |
| Right arm | Right connecting rod | Ball joint |
| Right connecting rod | Right wheel hub assembly | Y-Z universal joint |
| Items | Lateral Acceleration (m/s2) | Yaw Velocity (rad/s) | Roll Angle (deg) | Steering Characteristic (deg) |
|---|---|---|---|---|
| Without AARB (A) | −3.58 | −0.335 | 0.637 | +3.07 |
| With AARB (B) | −3.59 | −0.333 | 0.643 | +2.95 |
| Difference (BA)/A100 (%) | +0.28 | −0.60 | +0.94 | −3.91 |
| Stabilization Time | Lateral Acceleration Stabilization Time (s) | Yaw Velocity Stabilization Time (s) | Roll Angle Stabilization Time (s) | Steering Characteristic Stabilization Time (s) | Total Stabilization Time (s) |
|---|---|---|---|---|---|
| Without AARB (A) | 6.23 | 0.87 | 6.22 | 5.39 | 6.23 |
| With AARB (B) | 6.83 | 0.85 | 6.84 | 6.13 | 6.84 |
| Difference (BA)/A100 (%) | 9.63 | −2.30 | 9.97 | 13.73 | 9.79 |
| Items | Lateral Acceleration (m/s2) | Yaw Velocity (rad/s) | Roll Angle (deg) | Steering Characteristic (deg) |
|---|---|---|---|---|
| Without AARB (A) | −4.01 | −0.248 | 0.713 | −2.00 |
| With AARB (B) | −3.87 | −0.239 | 0.615 | −1.55 |
| Difference (BA)/A100 (%) | −3.49 | −3.63 | −13.74 | −22.5 |
| Stabilization Time | Lateral Acceleration Stabilization Time (s) | Yaw Velocity Stabilization Time (s) | Roll Angle Stabilization Time (s) | Steering Characteristic Stabilization Time (s) | Total Stabilization Time (s) |
|---|---|---|---|---|---|
| Without AARB (A) | 33.50 | 8.64 | 33.67 | 32.37 | 33.67 |
| With AARB (B) | 14.30 | 5.51 | 15.46 | 13.97 | 15.46 |
| Difference (BA)/A100 (%) | −57.31 | −36.23 | −54.08 | −56.84 | −54.08 |
| Items | Lateral Acceleration (m/s2) | Yaw Velocity (rad/s) | Roll Angle (deg) | Steering Characteristic (deg) |
|---|---|---|---|---|
| Without AARB Oscillation mean (A) | −3.76 * | −0.183 * | 0.707 * | −1.71 * |
| With AARB (B) | −3.91 | −0.181 | 0.555 | −1.81 |
| Difference (BA)/A100 (%) | 3.99 | −1.09 | −21.50 | 5.85 |
| Stabilization Time | Lateral Acceleration Stabilization Time (s) | Yaw Velocity Stabilization Time (s) | Roll Angle Stabilization Time (s) | Steering Characteristic Stabilization Time (s) | Total Stabilization Time (s) |
|---|---|---|---|---|---|
| Without AARB | NA | NA | NA | NA | NA |
| With AARB | 21.77 | 13.33 | 21.91 | 10.94 | 21.91 |
| Items | V = 40 km/h | V = 60 km/h | V = 80 km/h | |||
|---|---|---|---|---|---|---|
| Steering Characteristic (deg) | Stabilization Time (s) | Steering Characteristic (deg) | Stabilization Time (s) | Steering Characteristic (deg) | Stabilization Time (s) | |
| Without AARB (A) | +3.07 | 6.23 | −2.0 | 33.67 | −1.71 * | NA |
| With AARB (B) | +2.95 | 6.84 | −1.55 | 15.46 | −1.81 | 21.91 |
| Difference (BA)/A100 (%) | −3.91 | 9.79 | −22.5 | −54.08 | 5.85 | NA |
| Path Diameter | V = 40 km/h Path Diameter (m) | V = 60 km/h Path Diameter (m) | V = 80 km/h Path Diameter (m) |
|---|---|---|---|
| Without AARB (A) | 63.7 | 130.3 | 245.6 |
| With AARB (B) | 64.7 | 135.6 | 239.1 |
| Difference (BA)/A100 (%) | +1.57% | +4.07% | −2.65% |
| Stabilization Time | Lateral Acceleration Stabilization Time (s) | Yaw Velocity Stabilization Time (s) | Roll Angle Stabilization Time (s) | Steering Characteristic Stabilization Time (s) | Total Stabilization Time (s) |
|---|---|---|---|---|---|
| Without AARB (A) | 0.71 | 0.74 | 0.97 | 0.34 | 0.97 |
| With AARB (B) | 0.71 | 0.74 | 0.97 | 0.34 | 0.97 |
| Difference (BA)/A100 (%) | 0.00% | 0.00% | 0.00% | 0.00% | 0.00% |
| Stabilization Time | Lateral Acceleration Stabilization Time (s) | Yaw Velocity Stabilization Time (s) | Roll Angle Stabilization Time (s) | Steering Characteristic Stabilization Time (s) | Total Stabilization Time (s) |
|---|---|---|---|---|---|
| Without AARB (A) | 0.99 | 0.85 | 1.09 | 0.64 | 1.09 |
| With AARB (B) | 0.91 | 0.85 | 1.06 | 0.63 | 1.06 |
| Difference (BA)/A100 (%) | −8.08% | 0.00% | −2.75% | −1.56% | −2.75% |
| Stabilization Time | Lateral Acceleration Stabilization Time (s) | Yaw Velocity Stabilization Time (s) | Roll Angle Stabilization Time (s) | Steering Characteristic Stabilization Time (s) | Total Stabilization Time (s) |
|---|---|---|---|---|---|
| Without AARB (A) | 3.45 | 2.86 | 3.56 | 2.84 | 3.56 |
| With AARB (B) | 3.14 | 2.55 | 3.26 | 2.54 | 3.26 |
| Difference (BA)/A100 (%) | −8.99% | −10.84% | −8.43% | −10.56% | −8.43% |
| Total Stabilization Time | V = 40 km/h Total Stabilization Time (s) | V = 60 km/h Total Stabilization Time (s) | V = 80 km/h Total Stabilization Time (s) |
|---|---|---|---|
| Without AARB (A) | 0.97 | 1.09 | 3.56 |
| With AARB (B) | 0.97 | 1.06 | 3.26 |
| Difference (BA)/A100 (%) | 0 | −2.75 | −8.43 |
| Yaw Angle | V = 40 km/h Yaw Angle (deg) | V = 60 km/h Yaw Angle (deg) | V = 80 km/h Yaw Angle (deg) |
|---|---|---|---|
| Without AARB (A) | −0.6 | +4.0 | +26.0 |
| With AARB (B) | −0.7 | +3.6 | +22.2 |
| Difference (BA)/A100 (%) | +16.7% | −10% | −14.6% |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hwang, H.-Y.; Lan, T.-S.; Chen, J.-S. Developing a Strategy to Improve Handling Behaviors of a Medium-Size Electric Bus Using Active Anti-Roll Bar. Symmetry 2020, 12, 1334. https://doi.org/10.3390/sym12081334
Hwang H-Y, Lan T-S, Chen J-S. Developing a Strategy to Improve Handling Behaviors of a Medium-Size Electric Bus Using Active Anti-Roll Bar. Symmetry. 2020; 12(8):1334. https://doi.org/10.3390/sym12081334
Chicago/Turabian StyleHwang, Hsiu-Ying, Tian-Syung Lan, and Jia-Shiun Chen. 2020. "Developing a Strategy to Improve Handling Behaviors of a Medium-Size Electric Bus Using Active Anti-Roll Bar" Symmetry 12, no. 8: 1334. https://doi.org/10.3390/sym12081334
APA StyleHwang, H.-Y., Lan, T.-S., & Chen, J.-S. (2020). Developing a Strategy to Improve Handling Behaviors of a Medium-Size Electric Bus Using Active Anti-Roll Bar. Symmetry, 12(8), 1334. https://doi.org/10.3390/sym12081334






