Neutrino Oscillations in the Presence of Matter and Continuous Non-Selective Measurement
Abstract
1. Introduction
1.1. Neutrino Oscillation of Three Flavors
1.2. Continuous Non-Selective Measurement
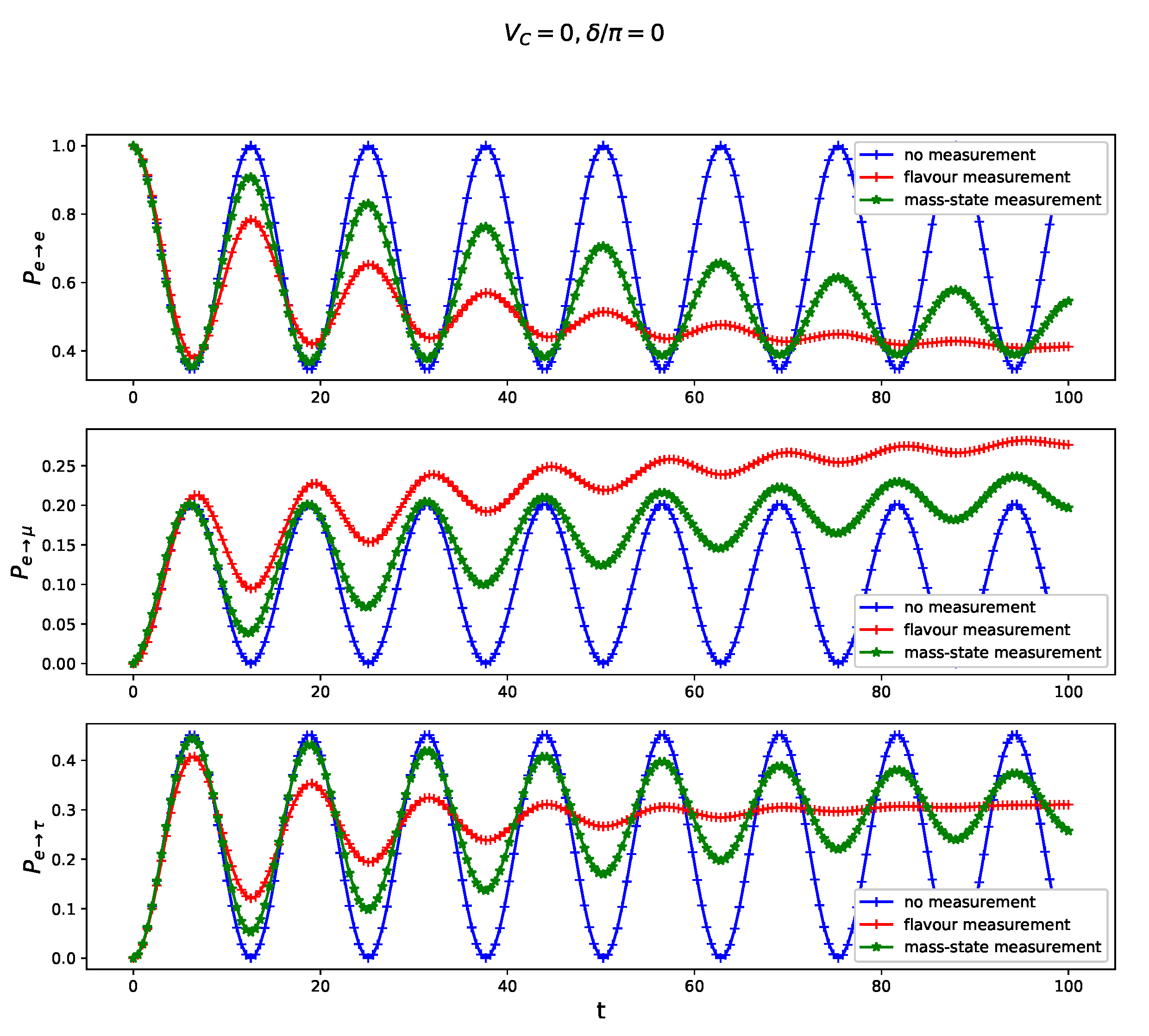
2. Results
3. Discussion
4. Conclusions
5. Materials and Methods
Author Contributions
Funding
Conflicts of Interest
References
- Giunti, C.; Wook, K.C. Fundamentals of Neutrino Physics and Astrophysics; Oxford Univ.: Oxford, UK, 2007. [Google Scholar]
- Bilenky, S. Neutrino oscillations: From a historical perspective to the present status. Nucl. Phys. B 2016, 908, 2–13. [Google Scholar] [CrossRef]
- Giganti, C.; Lavignac, S.; Zito, M. Neutrino oscillations: The rise of the PMNS paradigm. Prog. Part. Nucl. Phys. 2018, 98, 1–54. [Google Scholar] [CrossRef]
- Benatti, F.; Floreanini, R. Massless neutrino oscillations. Phys. Rev. 2001, D64, 085015. [Google Scholar] [CrossRef]
- Banerjee, S.; Alok, A.K.; Srikanth, R.; Hiesmayr, B.C. A quantum information theoretic analysis of three flavor neutrino oscillations. Eur. Phys. J. 2015, C75, 487. [Google Scholar] [CrossRef] [PubMed]
- Molfetta, G.D.; Pérez, A. Quantum walks as simulators of neutrino oscillations in a vacuum and matter. New J. Phys. 2016, 18, 103038. [Google Scholar] [CrossRef]
- Carpio, J.; Massoni, E.; Gago, A.M. Revisiting quantum decoherence in the matter neutrino oscillation framework. arXiv 2017, arXiv:1711.03680. [Google Scholar]
- Oliveira, R.L.N.; Guzzo, M.M. Quantum dissipation in vacuum neutrino oscillation. Eur. Phys. J. C 2010, 69, 493–502. [Google Scholar] [CrossRef]
- Lisi, E.; Marrone, A.; Montanino, D. Probing Possible Decoherence Effects in Atmospheric Neutrino Oscillations. Phys. Rev. Lett. 2000, 85, 1166–1169. [Google Scholar] [CrossRef]
- Bakhti, P.; Farzan, Y.; Schwetz, T. Revisiting the quantum decoherence scenario as an explanation for the LSND anomaly. J. High Energy Phys. 2015, 2015, 7. [Google Scholar] [CrossRef]
- Gago, A.M.; Santos, E.M.; Teves, W.J.C.; Zukanovich Funchal, R. Quantum dissipative effects and neutrinos: Current constraints and future perspectives. Phys. Rev. D 2001, 63, 073001. [Google Scholar] [CrossRef]
- Balieiro Gomes, G.; Guzzo, M.M.; de Holanda, P.C.; Oliveira, R.L.N. Parameter limits for neutrino oscillation with decoherence in KamLAND. Phys. Rev. D 2017, 95, 113005. [Google Scholar] [CrossRef]
- Gangopadhyay, D.; Home, D.; Roy, A.S. Probing the Leggett-Garg Inequality for Oscillating Neutral Kaons and Neutrinos. Phys. Rev. 2013, A88, 022115. [Google Scholar] [CrossRef]
- Alok, A.K.; Banerjee, S.; Sankar, S.U. Quantum correlations in terms of neutrino oscillation probabilities. Nucl. Phys. 2016, B909, 65–72. [Google Scholar] [CrossRef]
- Blasone, M.; Dell’Anno, F.; Siena, S.D.; Illuminati, F. Entanglement in neutrino oscillations. EPL Europhys. Lett. 2009, 85, 50002. [Google Scholar] [CrossRef]
- Richter, M.; Dziewit, B.; Dajka, J. Leggett-Garg K3 quantity discriminates between Dirac and Majorana neutrinos. Phys. Rev D. 2017, 96, 076008. [Google Scholar] [CrossRef]
- Jacobs, K.; Steck, D.A. A straightforward introduction to continuous quantum measurement. Contemp. Phys. 2006, 47, 279–303. [Google Scholar] [CrossRef]
- Capozzi, F.; Di Valentino, E.; Lisi, E.; Marrone, A.; Melchiorri, A.; Palazzo, A. Global constraints on absolute neutrino masses and their ordering. Phys. Rev. D 2017, 95, 096014. [Google Scholar] [CrossRef]
- Blennow, M.; Smirnov, A.Y. Neutrino propagation in matter. Adv. High Energy Phys. 2013, 2013, 972485. [Google Scholar] [CrossRef]
- Dajka, J.; Syska, J.; Luczka, J. Geometric phase of neutrino propagating through dissipative matter. Phys. Rev. 2011, D83, 097302. [Google Scholar] [CrossRef]
- Richter-Laskowska, M.; Łobejko, M.; Dajka, J. Quantum contextuality of a single neutrino under interactions with matter. New J. Phys. 2018, 20, 063040. [Google Scholar] [CrossRef]
- Wiesman, H.; Milburn, G. Quantum Measurment and Control; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- Jacobs, K. Quantum Measurement Theory and its Applications; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Alicki, R.; Lendi, K. Quantum Dynamical Semigroups and Applications; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Breuer, H.P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: Oxford, UK, 2002. [Google Scholar]
- Facchi, P.; Pascazio, S. Quantum Zeno dynamics: Mathematical and physical aspects. J. Phys. A Math. Theor. 2008, 41, 493001. [Google Scholar] [CrossRef]
- Rivas, A.; Huelga, S.F. Open Quantum Systems. An introduction; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Gardiner, C.; Zoller, P. Quantum Noise: A Handbook of Markovian and Non-Markovian Quantum Stochastic Methods with Applications to Quantum Optics; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Johansson, J.; Nation, P.; Nori, F. QuTiP 2: A Python framework for the dynamics of open quantum systems. Comput. Phys. Commun. 2013, 184, 1234–1240. [Google Scholar] [CrossRef]
- Johansson, J.; Nation, P.; Nori, F. QuTiP: An open-source Python framework for the dynamics of open quantum systems. Comput. Phys. Commun. 2012, 183, 1760–1772. [Google Scholar] [CrossRef]







© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, F.; Dajka, J. Neutrino Oscillations in the Presence of Matter and Continuous Non-Selective Measurement. Symmetry 2020, 12, 1296. https://doi.org/10.3390/sym12081296
Khan F, Dajka J. Neutrino Oscillations in the Presence of Matter and Continuous Non-Selective Measurement. Symmetry. 2020; 12(8):1296. https://doi.org/10.3390/sym12081296
Chicago/Turabian StyleKhan, Fazeel, and Jerzy Dajka. 2020. "Neutrino Oscillations in the Presence of Matter and Continuous Non-Selective Measurement" Symmetry 12, no. 8: 1296. https://doi.org/10.3390/sym12081296
APA StyleKhan, F., & Dajka, J. (2020). Neutrino Oscillations in the Presence of Matter and Continuous Non-Selective Measurement. Symmetry, 12(8), 1296. https://doi.org/10.3390/sym12081296




