The Acid-Base Through-the-Cage Interaction as an Example of an Inversion in a Cage Isomerism
Abstract
1. Introduction
2. Calculations
3. Results and Discussion
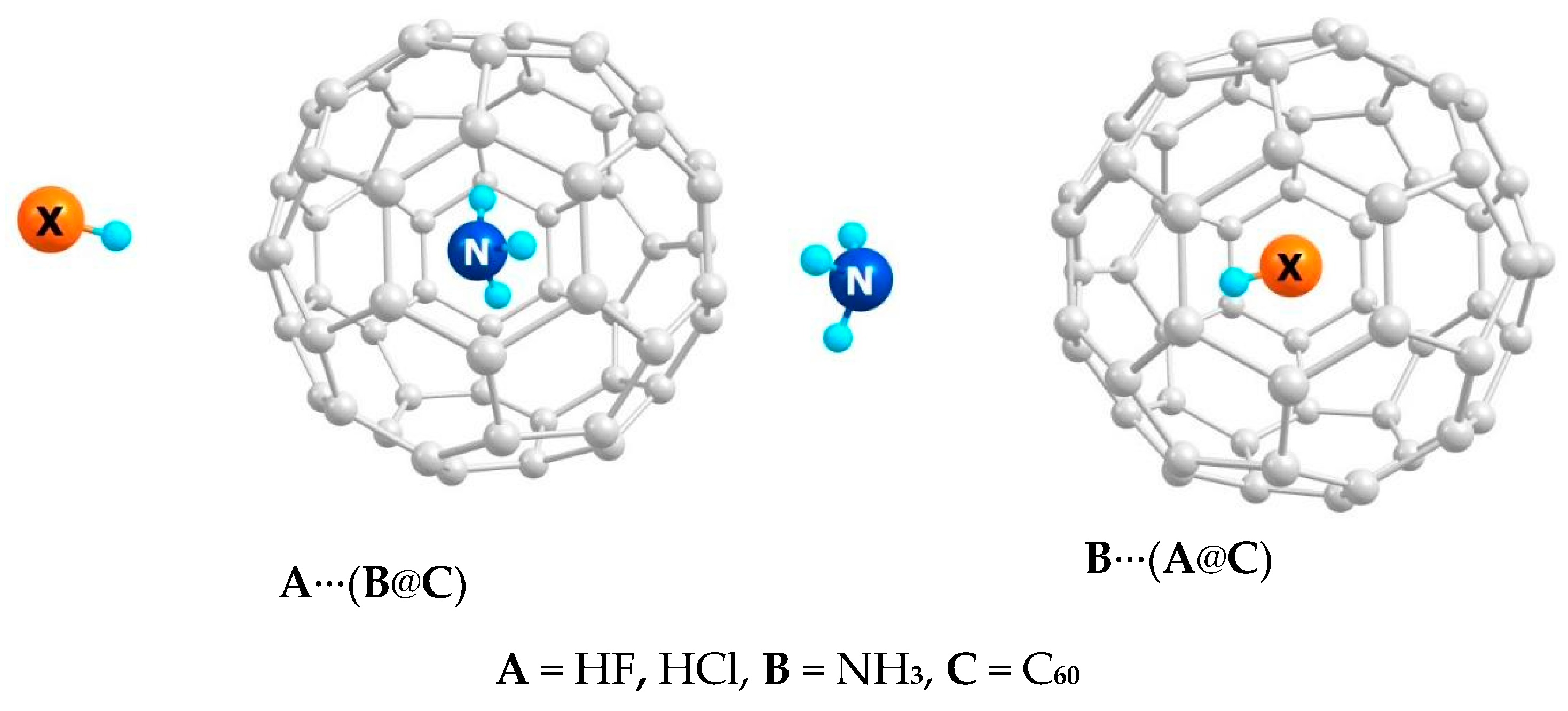
3.1. Inversion in a Cage Isomerism
3.2. Computational Example of the Inversion in a Cage Isomers
3.3. Acids and Bases in the Inversion in a Cage Isomer
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
Appendix C
| Block | System | ωB97XD/Def2TZVP | ωB97XD/6-31G** | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| ΔE7 | ΔE | ΔECP | ΔEdef | BSSE | ΔE7 | ΔE | ΔECP | ΔEdef | BSSE | ||
| I | FH⋯NH3 | −13.07 | −13.92 | −13.90 | 0.83 | 0.85 | −13.40 | −14.70 | −13.96 | 0.56 | 1.30 |
| ClH⋯NH3 | −9.33 | −10.36 | −10.04 | 0.71 | 1.03 | −12.30 | −14.11 | −13.84 | 1.55 | 1.81 | |
| II | FH⋯C60 | −2.44 | −3.29 | −2.48 | 0.04 | 0.86 | −0.98 | −4.34 | −1.00 | 0.02 | 3.36 |
| ClH⋯C60 | −2.27 | −2.77 | −2.28 | 0.01 | 0.50 | −2.55 | −3.31 | −2.57 | 0.02 | 0.76 | |
| H3N⋯C60 | −2.22 | −2.92 | −2.22 | 0.00 | 0.71 | −1.86 | −3.46 | −1.86 | 0.00 | 1.60 | |
| III | HF@C60 | −10.75 | −12.57 | −10.79 | 0.04 | 1.82 | −8.13 | −13.62 | −8.19 | 0.06 | 5.49 |
| HCl@C60 | −9.29 | −10.98 | −9.33 | 0.04 | 1.69 | −10.48 | −12.91 | −10.53 | 0.05 | 2.43 | |
| NH3@C60 | −15.92 | −17.84 | −15.97 | 0.05 | 1.92 | −15.61 | −20.50 | −15.66 | 0.05 | 4.89 | |
| IV | H3N⋯(HF@C60) | −13.14 | −15.68 | −13.18 | 0.04 | 2.54 | −10.12 | −17.22 | −10.15 | 0.03 | 7.09 |
| H3N⋯(HCl@C60) | −11.68 | −14.09 | −11.70 | 0.02 | 2.41 | −12.46 | −16.52 | −12.50 | 0.04 | 4.05 | |
| FH⋯(NH3@C60) | −18.61 | −21.36 | −18.69 | 0.08 | 2.75 | −16.84 | −25.06 | −16.90 | 0.06 | 8.23 | |
| ClH⋯(NH3@C60) | −18.32 | −20.73 | −18.38 | 0.06 | 2.41 | −18.40 | −24.05 | −18.46 | 0.06 | 5.65 | |
| V | H3N⋯(HF@C60) | −2.39 | −3.11 | −2.42 | 0.03 | 0.72 | −2.02 | −3.60 | −2.03 | 0.01 | 1.58 |
| H3N⋯(HCl@C60) | −2.37 | −3.37 | −3.08 | 0.01 | 0.71 | −2.02 | −3.62 | −2.03 | 0.01 | 1.59 | |
| FH⋯(NH3@C60) | −2.69 | −3.52 | −2.74 | 0.05 | 0.83 | −1.11 | −4.57 | −1.14 | 0.03 | 3.46 | |
| ClH ⋯(NH3@C60) | −2.40 | −2.89 | −2.42 | 0.02 | 0.50 | −2.71 | −3.55 | −2.73 | 0.02 | 0.84 | |
| VI | H3N⋯(HF@C60) | 0.75 * | −1.76 * | −12.59 * | 13.34 * | 2.51 * | 4.66 | −2.51 | −9.46 | 14.12 | 7.18 |
| H3N⋯(HCl@C60) | −1.69 | −4.05 | −11.27 | 9.58 | 2.37 | 1.43 | −2.68 | −11.92 | 13.35 | 4.11 | |
| FH⋯(NH3@C60) | −4.75 | −7.44 | −18.1 | 13.35 | 2.69 | −2.13 | −10.36 | −16.56 | 14.43 | 8.19 | |
| ClH⋯(NH3@C60) | −8.33 | −10.69 | −18.02 | 9.69 | 2.36 | −4.53 | −10.20 | −17.95 | 13.42 | 5.67 | |
| VII | H3N⋯(HF@C60) | −10.93 | −12.75 | −10.99 | 0.06 | 1.83 | −8.25 | −13.77 | −8.28 | 0.03 | 5.52 |
| H3N⋯(HCl@C60) | −9.46 | −11.17 | −9.51 | 0.05 | 1.71 | −10.60 | −13.07 | −10.64 | 0.04 | 2.48 | |
| FH⋯(NH3@C60) | −16.15 | −18.06 | −16.20 | 0.05 | 1.92 | −15.78 | −20.73 | −15.83 | 0.05 | 4.94 | |
| ClH⋯(NH3@C60) | −16.04 | −17.96 | −16.10 | 0.06 | 1.92 | −15.85 | −20.74 | −15.89 | 0.04 | 4.89 | |
| System | ωB97XD/Def2TZVP | ωB97XD/6-31G** | ||||
|---|---|---|---|---|---|---|
| Total | ZPE | Gibbs Free | Total | ZPE | Gibbs Free | |
| HF | −100.462986 | −100.453491 | −100.469888 | −100.404833 | −100.395270 | −100.411669 |
| HCl | −460.817098 | −460.810283 | −460.828159 | −460.782991 | −60.776154 | −460.794033 |
| NH3 | −56.564335 | −56.529785 | −56.548845 | −56.538783 | −56.503923 | −56.522991 |
| C60 | −2286.129284 | −2285.746652 | −2285.789339 | −2285.455680 | −2285.072455 | −2285.115257 |
| FH⋯NH3 | −157.049486 | −157.000710 | −157.025302 | −156.967034 | −156.917986 | −156.942771 |
| ClH⋯NH3 | −517.397416 | −517.352302 | −517.378181 | −517.343823 | −517.298097 | −517.323707 |
| FH⋯C60 | −2386.597516 | −2386.204068 | −2386.253997 | −2385.867420 | −2385.473283 | −2385.520938 |
| ClH⋯C60 | −2746.950794 | −2746.560299 | −2746.610222 | −2746.243940 | −2745.852699 | −2745.902481 |
| H3N⋯C60 | −2342.698277 | −2342.280249 | −2342.332021 | −2341.999973 | −2341.581128 | −2341.633465 |
| HF@C60 | −2386.612290 | −2386.216769 | −2386.261739 | −2385.882203 | −2385.486287 | −2385.532132 |
| HCl@C60 | −2746.963914 | −2746.570802 | −2746.615744 | −2746.259229 | −2745.866648 | −2745.912129 |
| NH3@C60 | −2342.722027 | −2342.299986 | −2342.344821 | −2342.027106 | −2341.604915 | −2341.649989 |
| H3N⋯(HF@C60) | −2443.181575 * | −2442.749552 * | −2442.800353 * | −2442.426715 | −2441.995066 | −2442.050334 |
| H3N⋯(HCl@C60) | −2803.533157 | −2803.104201 | −2803.157997 | −2802.803770 | −2802.375135 | −2802.429715 |
| FH⋯(NH3@C60) | −2443.190621 | −2442.756905 | −2442.808853 | −2442.439213 | −2442.006495 | −2442.056459 |
| ClH⋯(NH3@C60) | −2803.543733 | −2803.114174 | −2803.166803 | −2802.815748 | −2802.385858 | −2802.438281 |
| HF ic isomers | −5.676 * | −4.614 * | −5.334 * | −7.843 | −7.172 | −3.843 |
| HCl ic isomers | −6.637 | −6.258 | −5.526 | −7.516 | −6.729 | −5.375 |
References
- Berzelius, J.J. Ueber die Zusmmensetzung der Weinsäure und Traubensäure (John’s Säure aus den Vogesen), über das Atomengewicht das Bleioxyds, nebst allgemeinen Bemerkungen über soche Körper, die gleiche Zusammensetzung, aber ungleiche Eigenschaften besitzen. Ann. Phys. Chem. 1830, 19, 305–335. [Google Scholar] [CrossRef]
- Rouvray, D.H. Isomer Enumeration Methods. Chem. Soc. Rev. 1974, 3, 355–372. [Google Scholar] [CrossRef]
- Slanina, Z. Contemporary Theory of Chemical Isomerism; Academia: Praha, Czech Republic, 1986. [Google Scholar]
- Fowler, P.W.; Manolopoulos, D.E. An Atlas of Fullerenes; Clarendon Press: Oxford, UK, 1995. [Google Scholar]
- Babić, D.; Klein, D.J.; Sah, C.H. Symmetry of fullerenes. Chem. Phys. Lett. 1993, 211, 235–241. [Google Scholar] [CrossRef]
- Vostrowsky, O.; Hirsch, A. Heterofullerenes. Chem. Rev. 2006, 106, 5191–5207. [Google Scholar] [CrossRef]
- Hummelen, J.C.; Bellavia-Lund, C.; Wudl, F. Heterofullerenes. In Fullerenes and Related Structures; Hirsch, A., Ed.; Springer: Berlin/Heidelberg, Germany, 1999; Volume 199, pp. 93–134. [Google Scholar]
- Ashrafi, A.R.; Ghorbani, M. Enumeration of a class of IPR hetero-fullerenes. J. Serb. Chem. Soc. 2010, 75, 361–368. [Google Scholar] [CrossRef]
- Weiske, T.; Böhme, D.K.; Hrušák, J.; Krätschmer, W.; Schwarz, H. Endohedral cluster compounds—Inclusion of helium within C60·+ and C70·+ through collision experiments. Angew. Chem. Int. Ed. 1991, 30, 884–886. [Google Scholar] [CrossRef]
- Cioslowski, J.; Fleischmann, E.D. Endohedral complexes: Atoms and ions inside the C60 cage. J. Chem. Phys. 1991, 94, 730–3734. [Google Scholar] [CrossRef]
- Popov, A.A.; Yang, S.; Dunsch, L. Endohedral Fullerenes. Chem. Rev. 2013, 113, 5989–6113. [Google Scholar] [CrossRef]
- Matsuo, Y.; Okada, H.; Ueno, H. Endohedral Lithium-Containing Fullerenes; Preparation, Derivatization, and Application; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Popov, A.A. (Ed.) Endohedral Fullerenes: Electron Transfer and Spin; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Filippone, S.; Nazario, M. Exohedral Fullerenes. In Encyclopedia of Polymeric Nanomaterials; Kobayashi, S., Müllen, K., Eds.; Springer: Berlin/Heidelberg, Germany, 2014; pp. 1–16. [Google Scholar]
- Ohtsuki, T.; Ohno, K.; Shiga, K.; Kawazoe, Y.; Maruyama, Y.; Masumoto, K. Insertion of Xe and Kr Atoms into C60 and C70 Fullerenes and the Formation of Dimers. Phys. Rev. Lett. 1998, 81, 967–970. [Google Scholar] [CrossRef]
- Iglesias-Groth, S.; Breton, J.; Girardet, C. Structure of the Van der Waals rare gas–C60 exohedral complexes [(C60)(RG)n; n=1, 2]. Chem. Phys. 1998, 237, 285–293. [Google Scholar] [CrossRef]
- Ichimura, K.; Imaeda, K.; Inokuchi, H. Bonding States of He, Ne and Ar in Solid C60. Chem. Lett. 2000, 29, 196–197. [Google Scholar] [CrossRef]
- Watanabe, S.; Katabuchi, T.; Ishioka, N.; Matsuhashi, S.; Muramatsu, H. Production of endohedral 133Xe-higher fullerenes by ion implantation. J. Radioanal. Nuclear Chem. 2007, 272, 467–469. [Google Scholar] [CrossRef]
- Kuganathan, N.; Arya, A.K.; Rushton, M.J.D.; Grimes, R.W. Trapping of volatile fission products by C60. Carbon 2018, 132, 477–485. [Google Scholar] [CrossRef]
- Kuganathan, N.; Ratnasothy Srikaran, R.; Chroneos, A. Stability of Coinage Metals Interacting with C60. Nanomaterials 2019, 9, 1484. [Google Scholar] [CrossRef]
- Simmons, H.E. 20th National Organic Chemistry Symposium Program Book; Division of Organic Chemistry of the American Chemical Society: Burlington, VT, USA, 1967; pp. 12–13. Available online: http://organicdivision.org/noshistory (accessed on 12 March 2017).
- Park, C.H.; Simmons, H.E.; Uyeda, R.T.; Habibi, M.F. Macrobicyclic amines. Trans. N. Y. Acad. Sci. 1970, 32, 521–534. [Google Scholar]
- Dietrich, B.; Lehn, J.M.; Sauvage, J.P.; Blanzat, J. Cryptates-X. Syntheses et Proprietes Physiques de Systemes Diaza-Polyoxa-Macrobicycliques. Tetrahedron 1973, 29, 1629–1645. [Google Scholar] [CrossRef]
- Alder, R.W.; East, S.P. In/Out Isomerism. Chem. Rev. 1996, 96, 2097–2112. [Google Scholar] [CrossRef]
- Cross, L.C.; Klyne, W. Rules for the Nomenclature of Organic Chemistry: Section E: Stereochemistry (Recommendations 1974). Pure Appl. Chem. 1976, 45, 11–30. [Google Scholar]
- Prelog, V.; Helmchen, G. Basic Principles of the CIP-System and Proposals for a Revision. Angew. Chem. Int. Ed. Engl. 1982, 21, 567–583. [Google Scholar] [CrossRef]
- Dodziuk, H.; Nowinski, K.S. ‘Horror vacui’ or topological in-out isomerism in perhydrogenated fullerenes. Part 1. C60H60 and monoalkylated perhydrogenated fullerenes. Chem. Phys. Lett. 1996, 249, 406–412. [Google Scholar] [CrossRef]
- Dodziuk, H.; Lukin, O.; Nowiński, K.S. Topological “In-Out” Isomerism in Perhydrogenated fullerenes Revisited. C60H60R1R2 with Two R Groups “In” (R1,R2=H, Me). Pol. J. Chem. 1999, 73, 299–306. [Google Scholar]
- Grimme, S. Semiempirical GGA-Type Density Functional Constructed with a Long-Range Dispersion Correction. J. Comput. Chem. 2006, 27, 1787–1799. [Google Scholar] [CrossRef] [PubMed]
- Ditchfield, R.; Hehre, W.J.; Pople, J.A. Self-Consistent Molecular Orbital Methods. 9. Extended Gaussian-type basis for molecular-orbital studies of organic molecules. J. Chem. Phys. 1971, 54, 724–728. [Google Scholar] [CrossRef]
- Weigend, F.; Ahlrichs, R. Balanced basis sets of split valence, triple zeta valence and quadruple zeta valence quality for H to Rn: Design and assessment of accuracy. Phys. Chem. Chem. Phys. 2005, 7, 3297–3305. [Google Scholar] [CrossRef] [PubMed]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09, Revision D.01; Gaussian Inc.: Wallingford, CT, USA, 2013.
- Chai, J.-D.; Head-Gordon, M. Long-Range Corrected Double-Hybrid Density Functionals. J. Chem. Phys. 2009, 131, 174105. [Google Scholar] [CrossRef]
- Ou, Q.; Subotnik, J.E. Electronic Relaxation in Benzaldehyde Evaluated via TD-DFT and Localized Diabatization: Intersystem Crossings, Conical Intersections, and Phosphorescence. J. Phys. Chem. C 2013, 117, 19839–19849. [Google Scholar] [CrossRef]
- Chai, J.-D.; Head-Gordon, M. Long-range corrected hybrid density functionals with damped atom-atom dispersion corrections. Phys. Chem. Chem. Phys. 2008, 10, 6615–6620. [Google Scholar] [CrossRef]
- Dobrowolski, J.C.; Lipiński, P.F.J.; Karpińska, G. Substituent Effect in the First Excited Singlet State of Monosubstituted Benzenes. J. Phys. Chem. A 2018, 122, 4609–4621. [Google Scholar] [CrossRef]
- Dobrowolski, J.C.; Karpińska, G. Substituent Effect in the First Excited Triplet State of Monosubstituted Benzenes. ACS Omega 2020, 5, 9477–9490. [Google Scholar] [CrossRef]
- Boys, S.F.; Bernardi, F. The calculation of small molecular interactions by the differences of separate total energies. Some procedures with reduced errors. Mol. Phys. 1970, 19, 553–566. [Google Scholar] [CrossRef]
- Turi, L.; Dannenberg, J.J. Correcting for basis set superposition error in aggregates containing more than two molecules: Ambiguities in the calculation of the counterpoise correction. J. Phys. Chem. 1993, 97, 2488–2490. [Google Scholar] [CrossRef]
- Rode, J.E.; Dobrowolski, J.C. Theoretical studies on the Oxetane⋯HCl and Thietane⋯HCl complexes. Chem. Phys. Lett. 2002, 360, 123–132. [Google Scholar] [CrossRef]
- Ostrowski, S.; Garnuszek, P.; Dobrowolski, J.C. Endohedral Isomerism in Model Achiral and Chiral La@C58N2 Systems. Spectrochim. Acta Part A 2020, 231, 117791. [Google Scholar] [CrossRef] [PubMed]
- Dodziuk, H. The classification scheme of isomers of organic molecules. Tetrahedron 1996, 52, 12941–12946. [Google Scholar] [CrossRef]
- Ayers, P.L.; Boyd, R.J.; Bultinck, P.; Caffarel, M.; Carbó-Dorca, R.; Causá, M.; Cioslowski, J.; Contreras-Garcia, J.; Cooper, D.L.; Coppens, P.; et al. Six questions on topology in theoretical chemistry. Comput. Theor. Chem. 2015, 1053, 2–16. [Google Scholar] [CrossRef]
- Testa, B. Organic stereochemistry. Part 3: Other stereogenic elements: Axes of chirality, planes of chirality, helicity, and (E,Z)-diastereoisomerism. Helv. Chim. Acta 2013, 96, 351–374. [Google Scholar] [CrossRef]
- Nori-Shargh, D.; Soltani, B.; Deyhimi, F. Ab initio study of configurational and conformational properties of cyclododeca-1,2,4,5,7,8,10,11-octaene. J. Mol. Struct. THEOCHEM 2002, 585, 257–263. [Google Scholar] [CrossRef]
- Jamróz, M.E.; Gałka, S.; Dobrowolski, J.C. On dicyclopentadiene isomers. J. Mol. Struct. THEOCHEM 2003, 634, 225–233. [Google Scholar] [CrossRef]
- Dobrowolski, J.C.; Mazurek, A.P. On the C60 Cyclized Hook and Ladder Carbynes. Int. J. Quantum. Chem. 2000, 80, 1087–1098. [Google Scholar] [CrossRef]
- Dobrowolski, J.C. On the Belt and Moebius Isomers of the Coronene Molecule. J. Chem. Inf. Comp. Sci. 2002, 42, 490–499. [Google Scholar] [CrossRef]
- Dobrowolski, J.C.; Mazurek, A.P. Model Carbyne Knots vs. Ideal Knots. J. Chem. Inf. Comp. Sci. 2003, 43, 861–869. [Google Scholar] [CrossRef] [PubMed]
- Dobrowolski, J.C.; Mazurek, A.P. Model Carbyne vs. Ideal and DNA Catenanes. J. Chem. Inf. Model. 2005, 45, 1030–1038. [Google Scholar] [CrossRef] [PubMed]
- Dobrowolski, J.C. On the Classification of Topological Isomers: Knots, Links, Rotaxanes, etc. Croat. Chem. Acta 2003, 76, 145–152. [Google Scholar]
- Kroto, H.W. Carbon onions introduce new flavour to fullerene studies. Nature 1992, 359, 670–671. [Google Scholar] [CrossRef]
- Sloan, J.S.; Dunin-Borkowski, R.E.; Hutchison, J.L.; Coleman, K.S.; Clifford Williams, V.C.; John, B.; Claridge, J.B.; York, A.P.E.; Xu, C.; Bailey, S.R.; et al. The size distribution, imaging and obstructing properties of C60 and higher fullerenes formed within arc-grown single walled carbon nanotubes. Chem. Phys. Lett. 2000, 316, 191–198. [Google Scholar] [CrossRef]
- Kawase, T.; Tanaka, K.; Shiono, N.; Seirai, Y.; Oda, M. Onion-Type Complexation Based on Carbon Nanorings and a Buckminsterfullerene. Angew. Chem. Int. Ed. 2004, 43, 1722–1724. [Google Scholar] [CrossRef]
- Sun, B.-Y.; Sato, Y.; Suenaga, K.; Okazaki, T.; Kishi, N.; Sugai, T.; Bandow, S.; Iijima, S.; Shinohara, H. Entrapping of exohedral metallofullerenes in carbon nanotubes: (CsC60)n@SWNT nano-peapods. J. Am. Chem. Soc. 2005, 127, 17972–17973. [Google Scholar] [CrossRef]
- Yang, W.L.; Xu, Z.Y.; Wei, H.; Feng, M.; Suter, D. Quantum–information–processing architecture with endohedral fullerenes in a carbon nanotube. Phys. Rev. A 2010, 81, 032303. [Google Scholar] [CrossRef]
- Zhao, H.; Shi, Q.; Han, Z.; Gong, H.; Zhang, Z.; Wu, S.; Wu, J. Anomalous thermal stability in supergiant onion-like carbon fullerenes. Carbon 2018, 138, 243–256. [Google Scholar] [CrossRef]
- Hesselmann, A.; Korona, T. On the accuracy of DFT-SAPT, MP2, SCS-MP2, MP2C, and DFT+Disp methods for the interaction energies of endohedral complexes of the C60 fullerene with a rare gas atom. Phys. Chem. Chem. Phys. 2011, 13, 732–743. [Google Scholar] [CrossRef]
- Malani, H.; Zhang, D. Theoretical Insight for the Metal Insertion Pathway of Endohedral Alkali Metal Fullerenes. J. Phys. Chem. A 2013, 117, 3521–3528. [Google Scholar] [CrossRef] [PubMed]
- Kaiser, A.; Leidlmair, C.; Bartl, P.; Zöttl, S.; Denifl, S.; Mauracher, A.; Probst, M.; Scheier, P.; Echt, O. Adsorption of hydrogen on neutral and charged fullerene: Experiment and theory. J. Chem. Phys. 2013, 138, 074311. [Google Scholar] [CrossRef] [PubMed]
- Whitener, K.E.; Frunzi, M.; Iwamatsu, S.; Murata, S.; Cross, R.J.; Saunders, M. Putting ammonia into a chemically opened fullerene. J. Am. Chem. Soc. 2008, 130, 13996–13999. [Google Scholar] [CrossRef] [PubMed]
- Christopher, M.; Stanisky, R.; Cross, J.; Saunders, M. Putting Atoms and Molecules into Chemically Opened Fullerenes. J. Am. Chem. Soc. 2009, 131, 3392–3395. [Google Scholar]
- Lakhlifi, A.; Dahoo, P.R. Enhanced localization of tunneling of the NH3 umbrella mode in nano-cage fullerene. Chem. Phys. 2011, 386, 73–80. [Google Scholar] [CrossRef]
- Krachmalnicoff, A.; Bounds, R.; Mamone, S.; Alom, S.; Concistrè, M.; Meier, B.; Kouřil, K.; Light, M.E.; Johnson, M.R.; Rols, S.; et al. The dipolar endofullerene HF@C60. Nat. Chem. 2016, 8, 953–957. [Google Scholar] [CrossRef]
- Vidal, S.; Izquierdo, M.; Alom, S.; Garcia-Borràs, M.; Filippone, S.; Osuna, S.; Solà, M.; Whitby, R.J.; Martín, N. Effect of incarcerated HF on the exohedral chemical reactivity of HF@C60. Chem. Comm. 2017, 53, 10993–10996. [Google Scholar] [CrossRef]
- Bloodworth, S.; Gräsvik, J.; Alom, S.; Kouřil, K.; Elliott, S.J.; Wells, N.J.; Horsewill, A.J.; Mamone, S.; Jiménez-Ruiz, M.; Rols, S.; et al. Synthesis and Properties of Open Fullerenes Encapsulating Ammonia and Methane. ChemPhysChem 2018, 19, 266–276. [Google Scholar] [CrossRef]
- Bloodworth, S.; Sitinova, G.; Alom, S.; Vidal, S.; Bacanu, G.R.; Elliott, S.J.; Light, M.E.; Herniman, J.M.; Langley, G.J.; Levitt, M.H.; et al. First Synthesis and Characterization of CH4@C60. Angew. Chem. Int. Ed. Engl. 2019, 58, 5038–5043. [Google Scholar] [CrossRef]
- Ma, F.; Li, Z.R.; Xu, H.L.; Li, Z.J.; Wu, D.; Li, Z.S.; Gu, F.L. Proton transfer in the complex H3N⋯HCl catalyzed by encapsulation into a C60 cage. ChemPhysChem 2009, 10, 1112–1116. [Google Scholar] [CrossRef]
- Zhang, R.; Murata, M.; Wakamiya, A.; Shimoaka, T.; Hasegawa, T.; Murata, Y. Isolation of the simplest hydrated acid. Sci. Adv. 2017, 3, e1602833. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Z.-J.; Li, X.-P.; Liu, Z.-B.; Li, Z.-R.; Huang, X.-R.; Sun, C.-C. Electric Field-Driven Acid-Base Chemistry: Proton Transfer from Acid (HCl) to Base (NH3/H2O). J. Phys. Chem. A 2011, 115, 1418–1422. [Google Scholar] [CrossRef] [PubMed]
- Srivastava, A.K.; Pandey, S.K.; Pandey, A.K.; Misra, N. C60 as Electron Acceptor and Donor: A Comparative DFT Study of Li@C60 and F@C60. Aust. J. Chem. 2018, 71, 953–956. [Google Scholar] [CrossRef]
- Jaroš, A.; Badri, Z.; Bora, P.L.; Bonab, E.F.; Marek, R.; Straka, M.; Foroutan-Nejad, C. How Does a Container Affect Acidity of its Content: Charge Depletion Bonding Inside Fullerenes. Chem. Eur. J. 2018, 24, 4245–4249. [Google Scholar] [CrossRef]
- Muller, P. Glossary of terms used in physical organic chemistry (IUPAC Recommendations 1994). Pure Appl. Chem. 1994, 66, 1077–1184. [Google Scholar] [CrossRef]
- Hunter, E.P.L.; Lias, S.G. Evaluated Gas Phase Basicities and Proton Affinities of Molecules: An Update. J. Phys. Chem. Ref. Data 1998, 27, 413–656. [Google Scholar] [CrossRef]
- Hunter, E.P.L.; Lias, S.G. Proton Affinity Evaluation. In NIST Chemistry WebBook, NIST Standard Reference Database No. 69; Linstrom, P.J., Mallard, W.G., Eds.; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2014. Available online: http://webbook.nist.gov/chemistry (accessed on 10 July 2015).
- Raczyńska, E.D.; Gal, J.-F.; Maria, P.-C. Enhanced Basicity of Push–Pull Nitrogen Bases in the Gas Phase. Chem. Rev. 2016, 116, 13454–13511. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Gal, J.-F.; Maria, P.-C.; Szeląg, M. Proton Transfer Chemistry in the Gas Phase. Is a Spontaneous ‘Neutralization’ Reaction a Myth or a Reality? Croat. Chem. Acta 2009, 82, 87–103. [Google Scholar]
- Bohme, D.K. Buckminsterfullerene cations: New dimensions in gas-phase ion chemistry. Mass Spectrom. Rev. 2009, 28, 672–693. [Google Scholar] [CrossRef]
- Kroto, H.W.; Jura, M. Circumstellar and interstellar fullerenes and their analogues. Astron. Astrophys. 1992, 263, 275–280. [Google Scholar]
- Palotás, J.; Martens, J.; Berden, G.; Oomens, J. The infrared spectrum of protonated buckminsterfullerene C60H+. Nat. Astron. 2019. [Google Scholar] [CrossRef]
| Block | Interaction | System | ωB97XD/Def2TZVP | ||||
|---|---|---|---|---|---|---|---|
| ΔE7 | ΔE | ΔECP | ΔEdef | BSSE | |||
| I | acid-base | FH⋯NH3 | −13.07 | −13.92 | −13.90 | 0.83 | 0.85 |
| ClH⋯NH3 | −10.04 2 | −9.33 | −10.36 | 1.03 | 0.71 | ||
| II | external with the empty cage | FH⋯C60 | −2.44 | −3.29 | −2.48 | 0.04 | 0.86 |
| ClH⋯C60 | −2.27 | −2.77 | −2.28 | 0.01 | 0.50 | ||
| H3N⋯C60 | −2.22 | −2.92 | −2.22 | 0.00 | 0.71 | ||
| III | guest-cage | HF@C60 | −10.75 | −12.57 | −10.79 | 0.04 | 1.82 |
| HCl@C60 | −9.29 | −10.98 | −9.33 | 0.04 | 1.69 | ||
| NH3@C60 | −15.92 | −17.84 | −15.97 | 0.05 | 1.92 | ||
| IV | ternary | H3N⋯(HF@C60) | −13.14 | −15.68 | −13.18 | 0.04 | 2.54 |
| H3N⋯(HCl@C60) | −11.68 | −14.09 | −11.70 | 0.02 | 2.41 | ||
| FH⋯(NH3@C60) | −18.61 | −21.36 | −18.69 | 0.08 | 2.75 | ||
| ClH⋯(NH3@C60) | −18.32 | −20.73 | −18.38 | 0.06 | 2.41 | ||
| V | external with the filled cage | H3N⋯(HF@C60) | −2.39 | −3.11 | −2.42 | 0.03 | 0.72 |
| H3N⋯(HCl@C60) | −2.37 | −3.37 | −3.08 | 0.01 | 0.71 | ||
| FH⋯(NH3@C60) | −2.69 | −3.52 | −2.74 | 0.05 | 0.83 | ||
| ClH ⋯(NH3@C60) | −2.40 | −2.89 | −2.42 | 0.02 | 0.50 | ||
| VI | acid-base through-the-cage | H3N⋯(HF@C60) | 0.75 3 | −1.76 3 | −12.59 3 | 13.34 3 | 2.51 3 |
| H3N⋯(HCl@C60) | −1.69 | −4.05 | −11.27 | 9.58 | 2.37 | ||
| FH⋯(NH3@C60) | −4.75 | −7.44 | −18.10 | 13.35 | 2.69 | ||
| ClH⋯(NH3@C60) | −8.33 | −10.69 | −18.02 | 9.69 | 2.36 | ||
| VII | guest-cage with the surrounding | H3N⋯(HF@C60) | −10.93 | −12.75 | −10.99 | 0.06 | 1.83 |
| H3N⋯(HCl@C60) | −9.46 | −11.17 | −9.51 | 0.05 | 1.71 | ||
| FH⋯(NH3@C60) | −16.15 | −18.06 | −16.20 | 0.05 | 1.92 | ||
| ClH⋯(NH3@C60) | −16.04 | −17.96 | −16.10 | 0.06 | 1.92 | ||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dobrowolski, J.C.; Ostrowski, S. The Acid-Base Through-the-Cage Interaction as an Example of an Inversion in a Cage Isomerism. Symmetry 2020, 12, 1291. https://doi.org/10.3390/sym12081291
Dobrowolski JC, Ostrowski S. The Acid-Base Through-the-Cage Interaction as an Example of an Inversion in a Cage Isomerism. Symmetry. 2020; 12(8):1291. https://doi.org/10.3390/sym12081291
Chicago/Turabian StyleDobrowolski, Jan Cz., and Sławomir Ostrowski. 2020. "The Acid-Base Through-the-Cage Interaction as an Example of an Inversion in a Cage Isomerism" Symmetry 12, no. 8: 1291. https://doi.org/10.3390/sym12081291
APA StyleDobrowolski, J. C., & Ostrowski, S. (2020). The Acid-Base Through-the-Cage Interaction as an Example of an Inversion in a Cage Isomerism. Symmetry, 12(8), 1291. https://doi.org/10.3390/sym12081291







