MHD Bioconvection Flow and Heat Transfer of Nanofluid through an Exponentially Stretchable Sheet
Abstract
1. Introduction
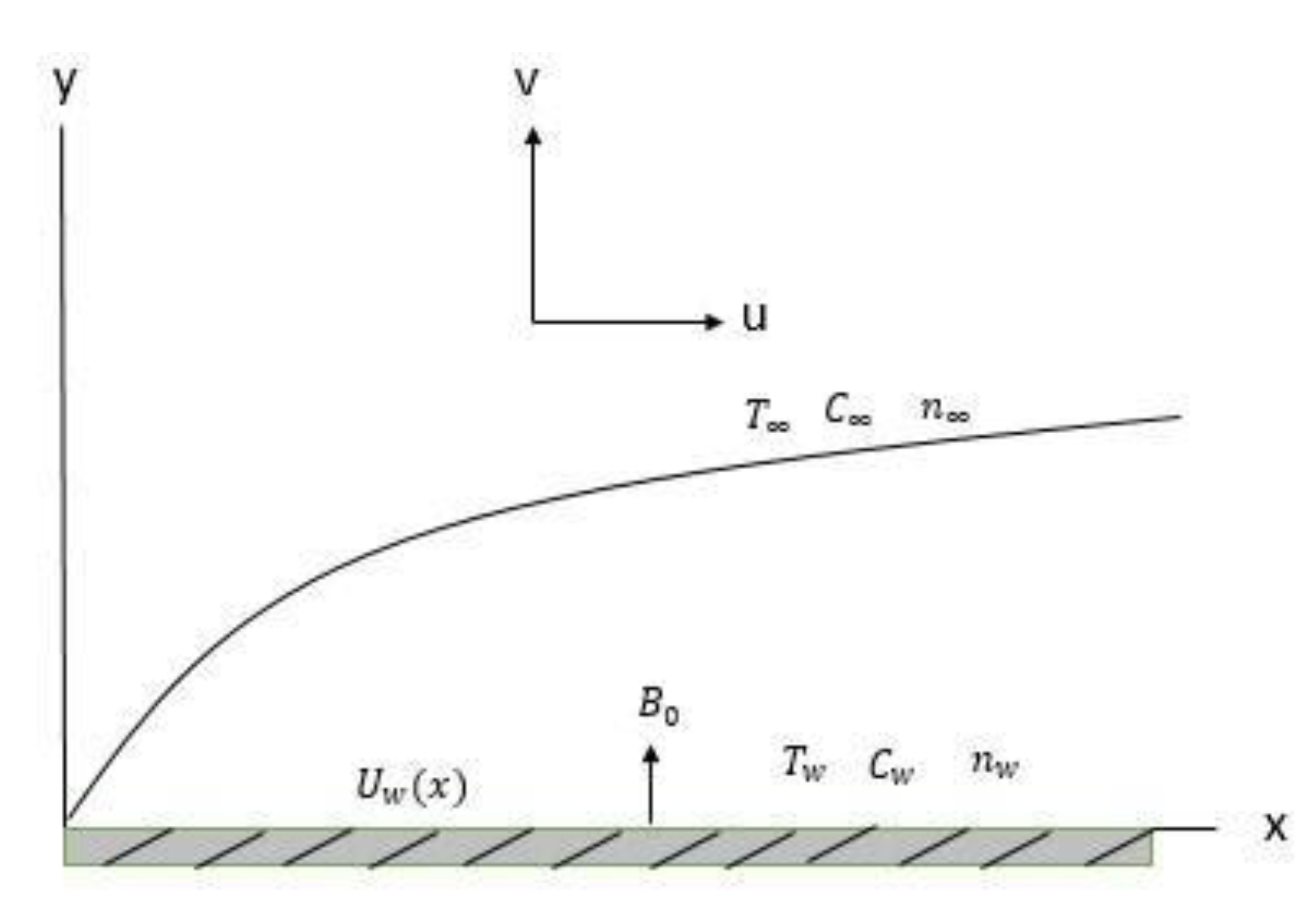
2. Mathematical Modeling
3. Spectral Relaxation Method
| Algorithm 1: Spectral Relaxation Method |
| Step 1: Introducing the transformation: . Step 2: Depict the original equation in terms of to decrease the order of the momentum equation for . Step 3: Assume that , , , are similar to the former iteration (indicated by , , , and ). Step 4: Construct an iteration scheme for , , , and . Step 5: Assume that only linear terms in , , , and are to be estimated at the present iteration scale (indicated by , , , ), and all other terms are presumed to be similar to the former iteration. |
4. Results and Discussion
5. Conclusions
- The comparison values of heat rate transfer were in good agreement with the former study, and hence led to the confidence of the present results to be reported further.
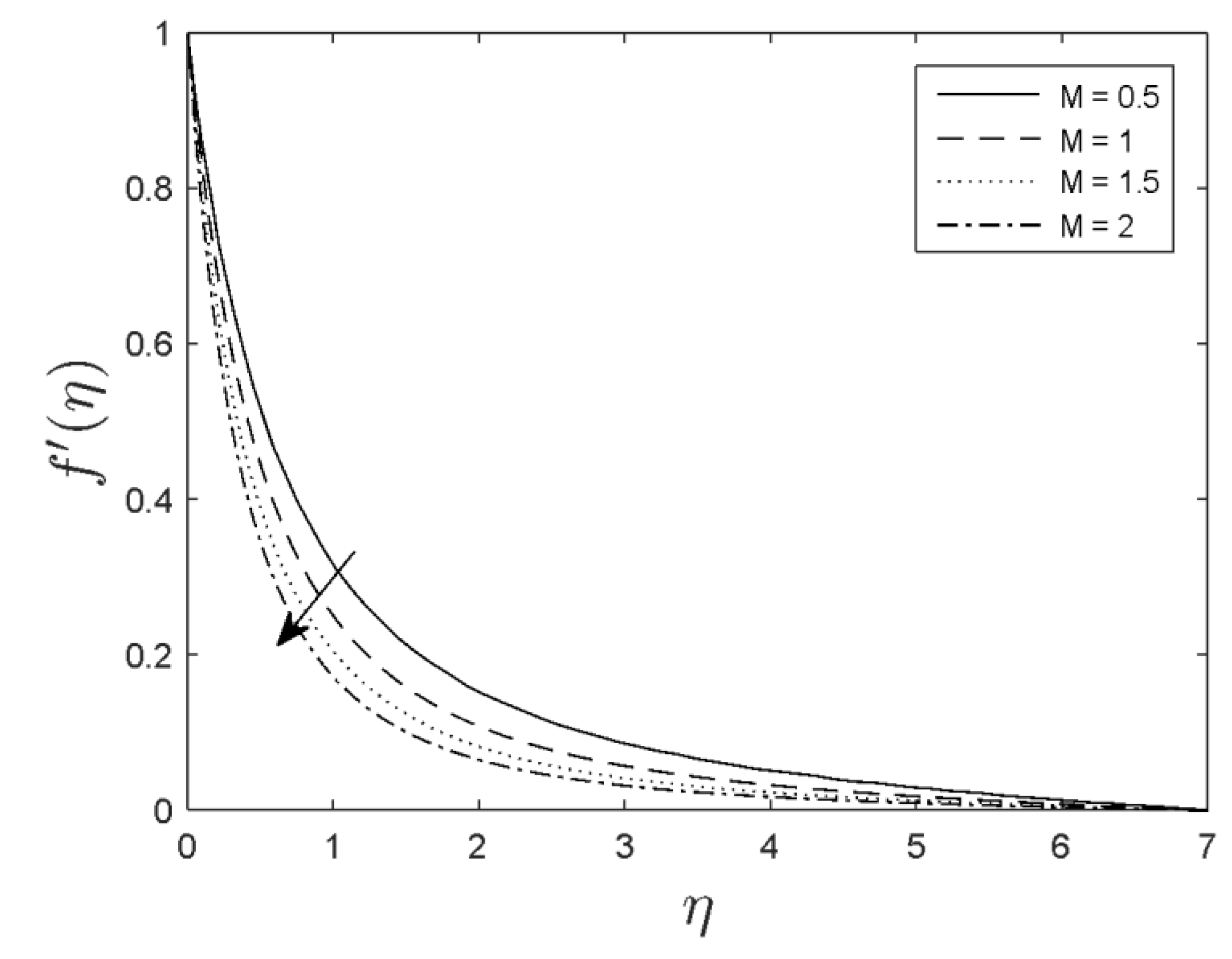
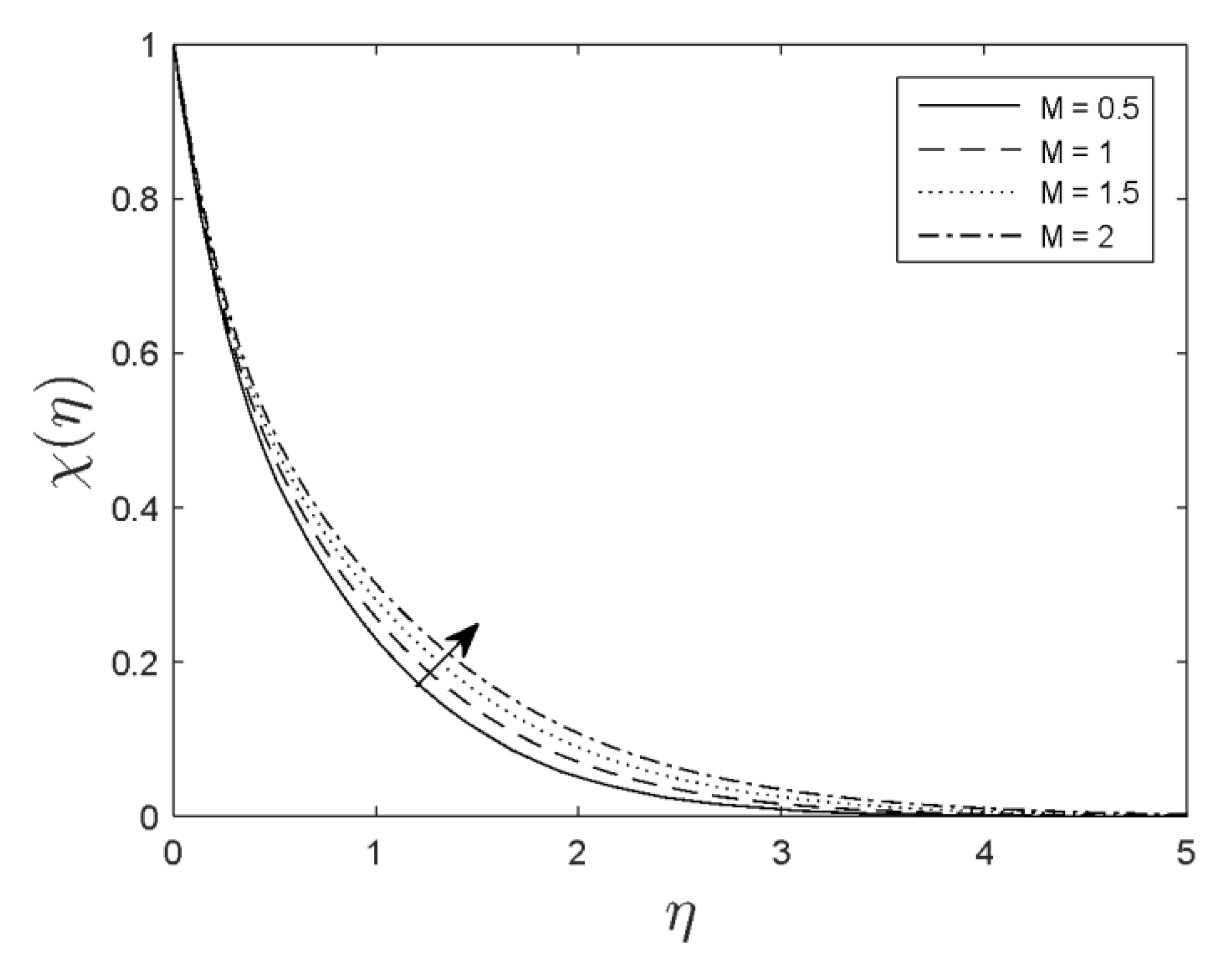
- The resultant velocity diminished with the increments in the magnetic parameter.
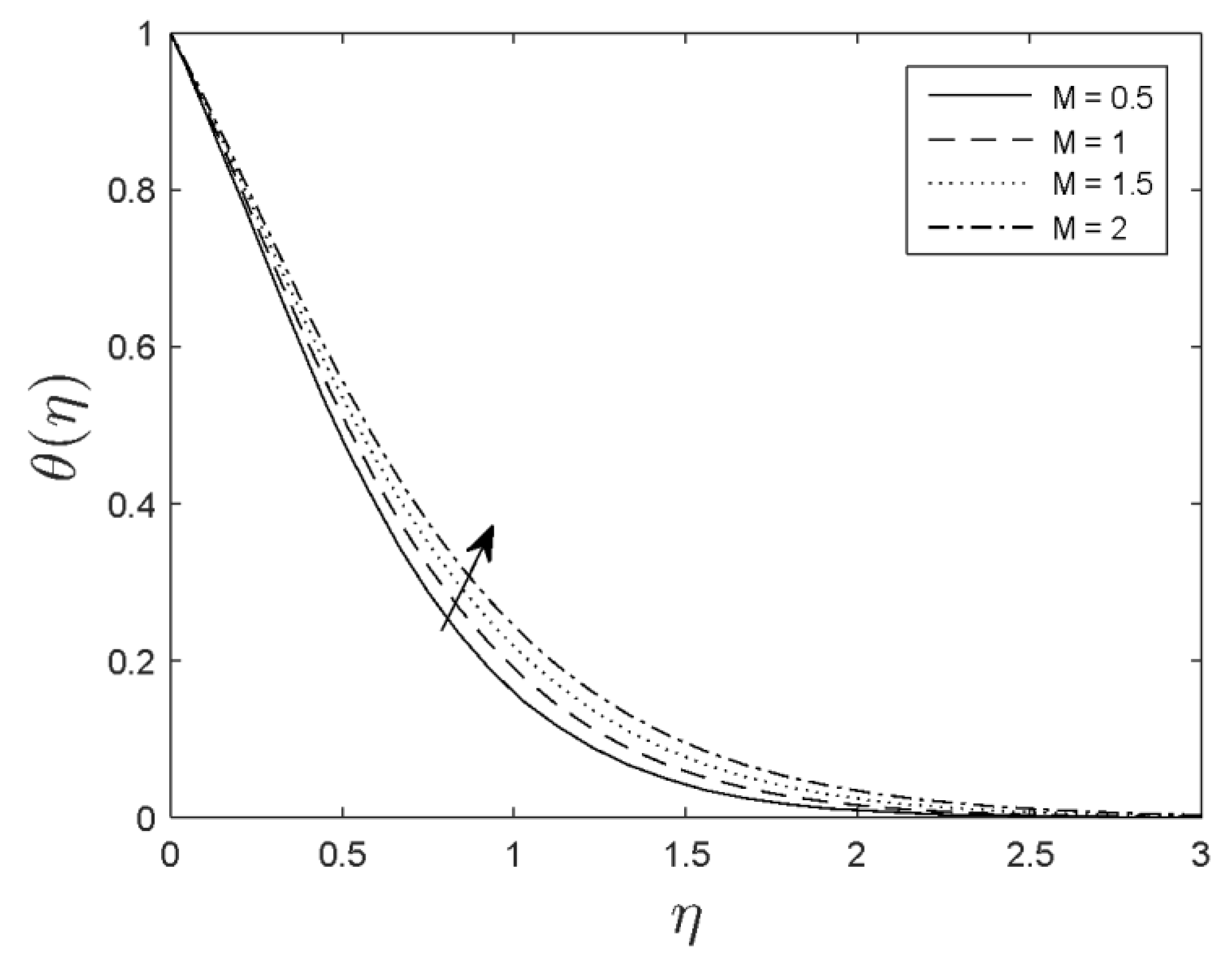
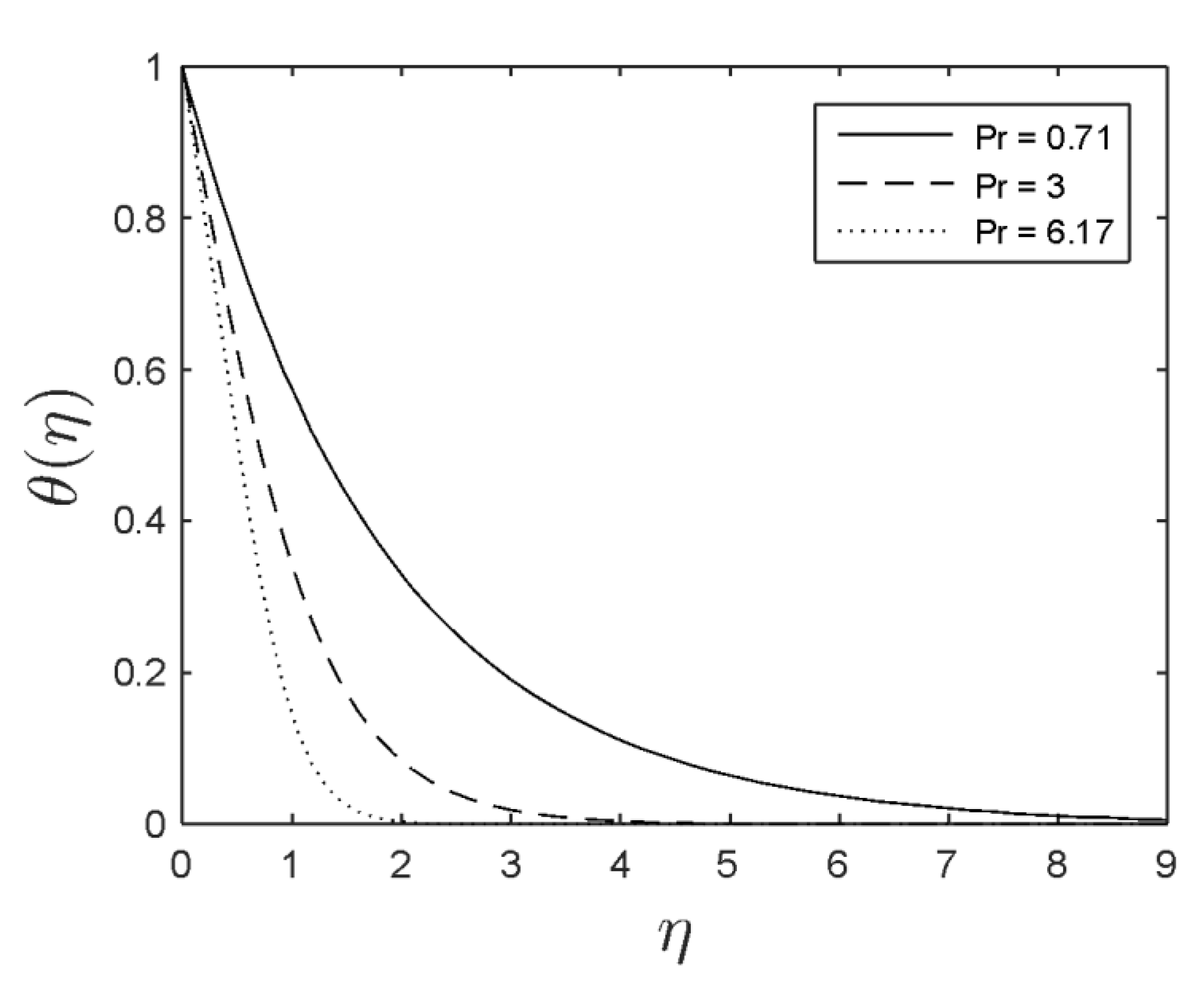
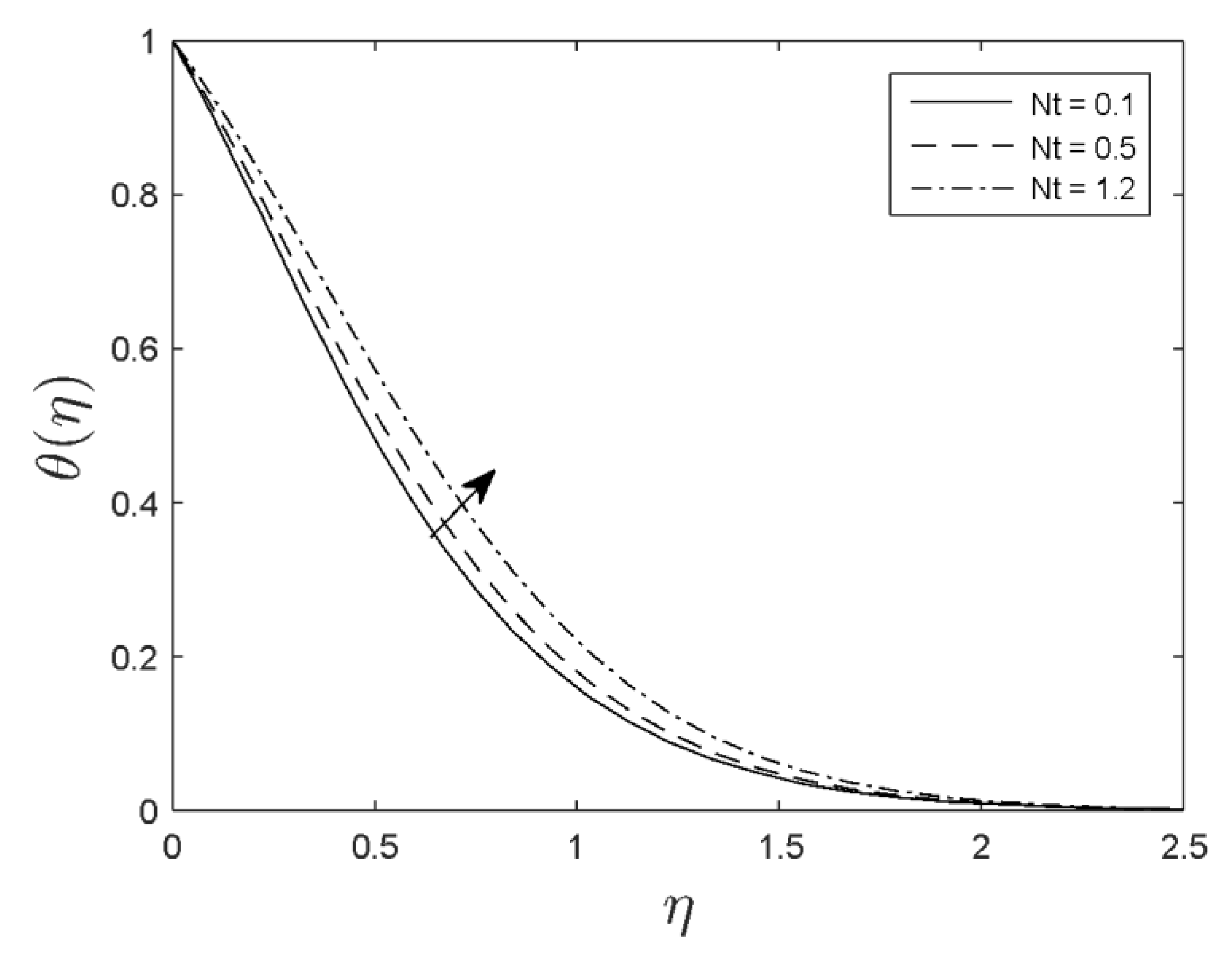
- Fluid temperature increased as the magnetic parameter, thermophoresis, and Brownian motion parameters increased.
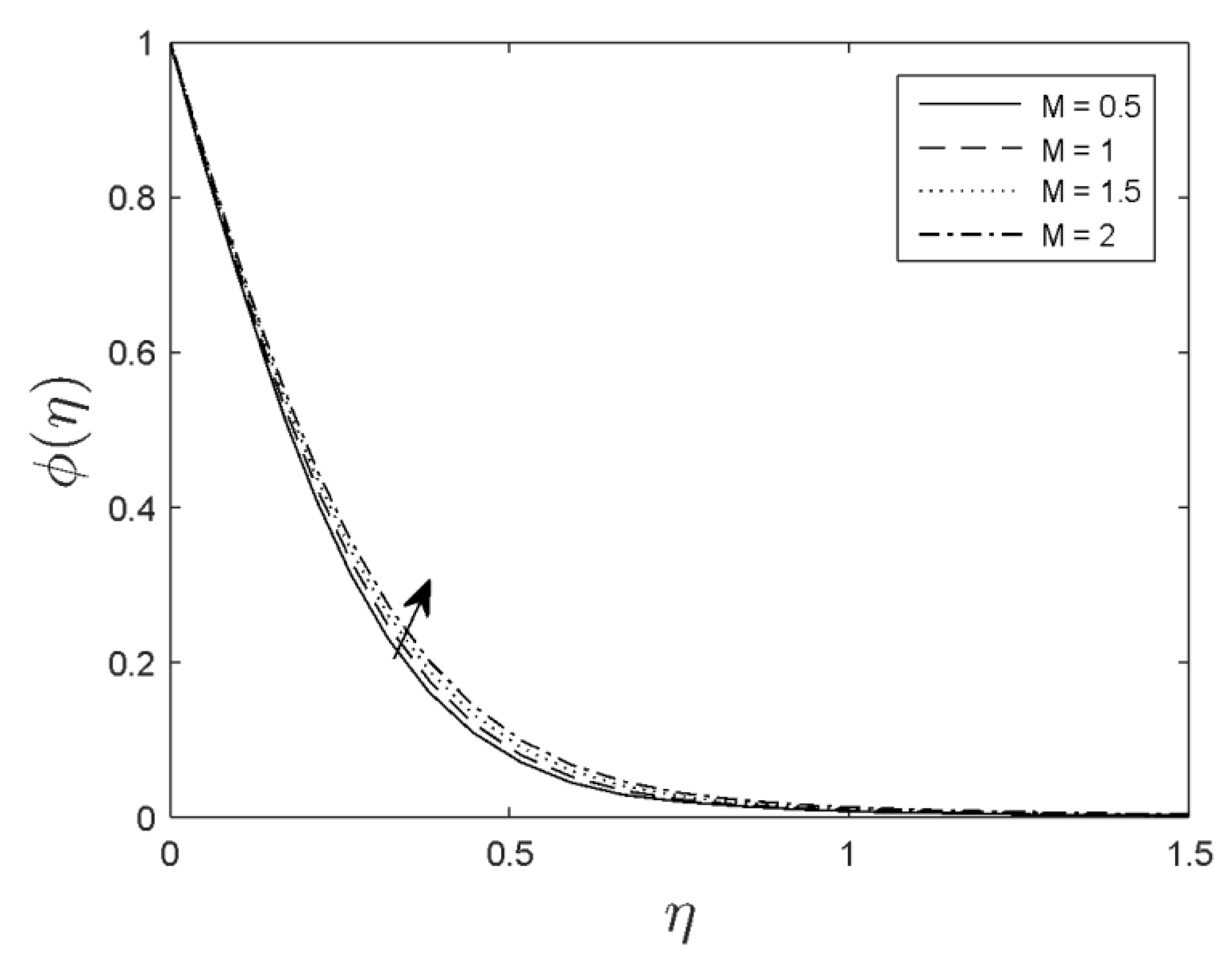
- The concentration was reduced with the boost in the Lewis number and Brownian motion parameter.
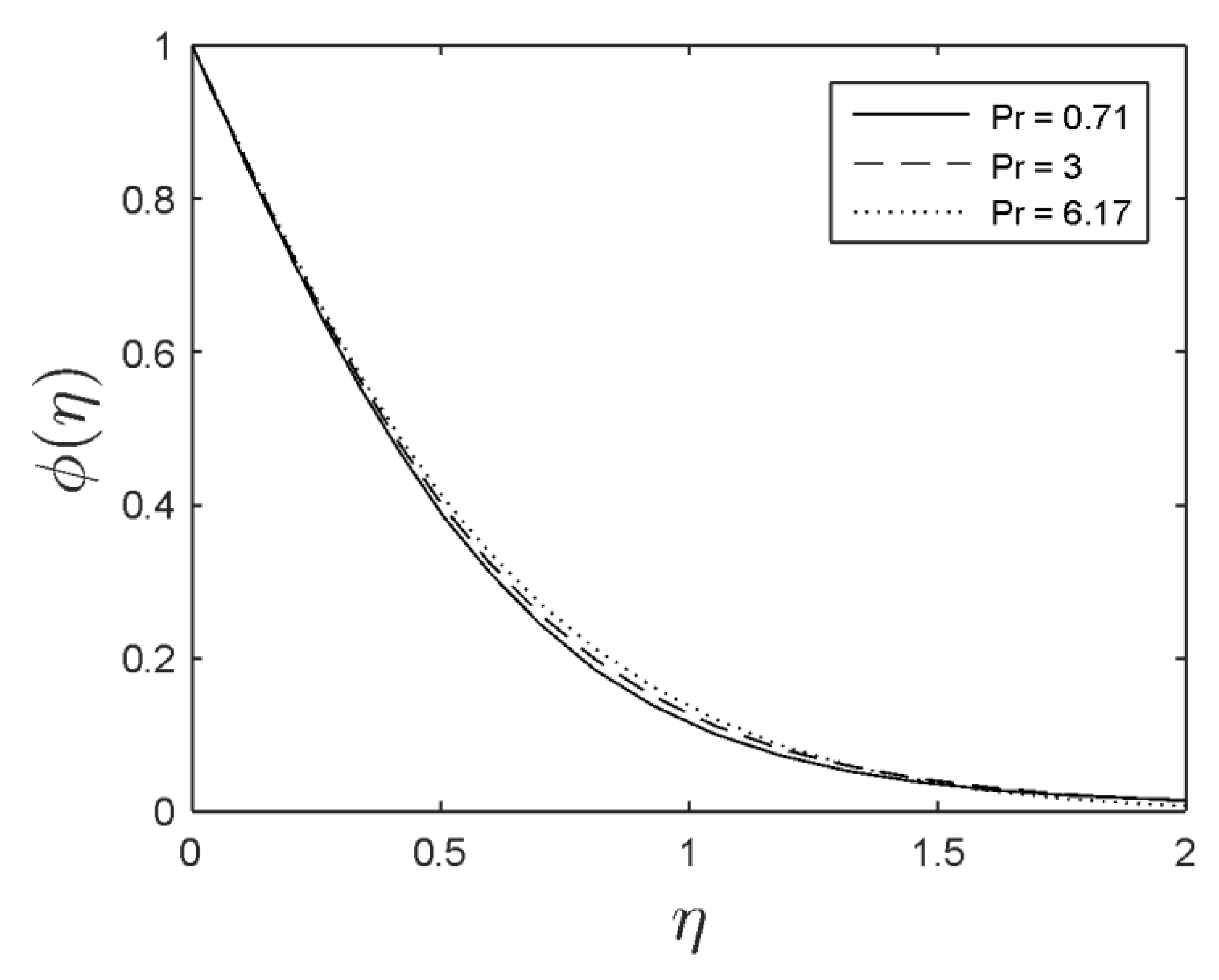
- The concentration was increased with the increment in the Prandtl number, thermophoresis, and magnetic parameters.
- The density of the motile microorganism is a decreasing function of the Prandtl number, Lewis number, Peclet number, bioconvection Lewis number, and bioconvection parameter.
- The residual errors of , , , and were iteration dependent.
- For future research, it is suggested for the present study to consider all possible multiple solutions or dual solutions. This is driven by the fact that the multiple solutions cannot be seen experimentally and can only be obtained by using numerical simulation.
- It was also proposed for the stability of multiple solutions to be included as one of the main objective studies for future work. Stability analysis is important for identifying the reliability of the multiple solutions, which depend on the assumptions of the physical model.
Author Contributions
Funding
Conflicts of Interest
References
- Choi, S.U.S.; Singer, D.A.; Wang, H.P. Developments and applications of non-Newtonian flows. ASME Fed. 1995, 66, 99–105. [Google Scholar]
- Hadavand, M.; Yousefzadeh, S.; Akbari, O.A.; Pourfattah, F.; Nguyen, H.M.; Asadi, A. A numerical investigation on the effects of mixed convection of Ag-water nanofluid inside a sim-circular lid-driven cavity on the temperature of an electronic silicon chip. Appl. Therm. Eng. 2019, 162, 114298. [Google Scholar] [CrossRef]
- Pourfattah, F.; Arani, A.A.A.; Babaie, M.R.; Nguyen, H.M.; Asadi, A. On the thermal characteristics of a manifold microchannel heat sink subjected to nanofluid using two-phase flow simulation. Int. J. Heat Mass Transf. 2019, 143, 118518. [Google Scholar] [CrossRef]
- Bazdar, H.; Toghraie, D.; Pourfattah, F.; Akbari, O.A.; Nguyen, H.M.; Asadi, A. Numerical investigation of turbulent flow and heat transfer of nanofluid inside a wavy microchannel with different wavelengths. J. Therm. Anal. Calorim. 2020, 139, 2365–2380. [Google Scholar] [CrossRef]
- Asadi, A.; Pourfattah, F.; Szilágyi, I.M.; Afrand, M.; Żyłag, G.; Ahn, H.S.; Wongwises, S.; Nguyen, H.M.; Arabkoohsar, A.; Mahian, O. Effect of sonication characteristics on stability, thermophysical properties, and heat transfer of nanofluids: A comprehensive review. Ultrason. Sonochem. 2019, 58, 104701. [Google Scholar] [CrossRef]
- Asadi, A.; Aberoumand, S.; Moradikazerouni, A.; Pourfattah, F.; Żyła, G.; Estellé, P.; Mahian, O.; Wongwises, S.; Nguyen, H.M.; Arabkoohsar, A. Recent advances in preparation methods and thermophysical properties of oil-based nanofluids: A state-of-the-art review. Powder Technol. 2019, 352, 209–226. [Google Scholar] [CrossRef]
- Alarifi, I.M.; Nguyen, H.M.; Bakhtiyari, A.N.; Asadi, A. Feasibility of ANFIS-PSO and ANFIS-GA Models in Predicting Thermophysical Properties of Al2O3-MWCNT/Oil Hybrid Nanofluid. Materials 2019, 12, 3628. [Google Scholar] [CrossRef]
- Asadi, A.; Alarifi, I.M.; Ali, V.; Nguyen, H.M. An experimental investigation on the effects of ultrasonication time on stability and thermal conductivity of MWCNT-water nanofluid: Finding the optimum ultrasonication time. Ultrason. Sonochem. 2019, 58, 104639. [Google Scholar] [CrossRef]
- Nabwey, H.A.; Boumazgour, M.; Rashad, A.M. Group method analysis of mixed convection stagnation-point flow of non-Newtonian nanofluid over a vertical stretching surface. Indian J. Phys. 2017, 91. [Google Scholar] [CrossRef]
- Tlili, I.; Nabwey, H.A.; Ashwinkumar, G.P.; Sandeep, N. 3-D magnetohydrodynamic AA7072-AA7075/methanol hybrid nanofluid flow above an uneven thickness surface with slip effect. Sci. Rep. 2020, 10, 1–13. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Rashad, A.M.; Alsabery, A.I.; Abdelrahman, Z.M.A.; Nabwey, H.A. Impact of Partial Slip on Magneto-Ferrofluids Mixed Convection Flow in Enclosure. J. Therm. Sci. Eng. Appl. 2020, 12, 051002. [Google Scholar] [CrossRef]
- Ferdows, M.; Nabwey, H.A.; Rashad, A.M.; Uddin, M.J.; Alzahrani, F. Boundary layer flow of a nanofluid past a horizontal flat plate in a Darcy porous medium: A Lie group approach. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019. [Google Scholar] [CrossRef]
- Nabwey, H.A.; El-Mky, H.A. Lie group analysis of thermophoresis on a vertical surface in a porous medium. J. King Saud Univ.-Sci. 2019, 31, 1048–1055. [Google Scholar] [CrossRef]
- Mahdy, A.E.N.; Hady, F.M.; Nabwey, H.A. Unsteady homogeneous-heterogeneous reactions in MHD nanofluid mixed convection flow past a stagnation point of an impulsively rotating sphere. Therm. Sci. 2019, 388. [Google Scholar] [CrossRef]
- Nabwey, H.A.; EL-Kabeir, S.M.M.; Rashad, A.M. Lie group analysis of effects of radiation and chemical reaction on heat and mass transfer by unsteady slip flow from a non-isothermal stretching sheet immersed in a porous medium. J. Comput. Theor. Nanosci. 2015, 12, 4056–4062. [Google Scholar] [CrossRef]
- Platt, J.R. Bioconvection Patterns in Cultures of Free-Swimming Organisms. Science 1961, 133, 1766–1767. [Google Scholar] [CrossRef]
- Pedley, T.J.; Hill, N.A.; Kessler, J.O. The growth of bioconvection patterns in a uniform suspension of gyrotactic micro-organisms. J. Fluid Mech. 1988, 195, 223–237. [Google Scholar] [CrossRef]
- Kuznetsov, A.V. Bio-thermal convection induced by two different species of microorganisms. Int. Commun. Heat Mass Transf. 2011, 38, 548–553. [Google Scholar] [CrossRef]
- Alloui, Z.; Nguyen, T.H.; Bilgen, E. Bioconvection of gravitactic microorganisms in a vertical cylinder. Int. Commun. Heat Mass Transf. 2005, 32, 739–747. [Google Scholar] [CrossRef]
- Uddin, M.J.; Kabir, M.N.; Bég, O.A. Computational investigation of Stefan blowing and multiple-slip effects on buoyancy-driven bioconvectionnanofluid flow with microorganisms. Int. J. Heat Mass Transf. 2016, 95, 116–130. [Google Scholar] [CrossRef]
- Dhanai, R.; Rana, P.; Kumar, L. Lie group analysis for bioconvection MHD slip flow and heat transfer of nanofluid over an inclined sheet: Multiple solutions. J. Taiwan Inst. Chem. Eng. 2016, 66. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Rashad, A.M.; Kameswaran, P.K.; Abdou, M.M.M. Radiation effects on natural bioconvection flow of a nanofluid containing gyrotactic microorganisms past a vertical plate with streamwise temperature variation. J. Nanofluids 2017, 6, 587–595. [Google Scholar] [CrossRef]
- Rashad, A.M.; Chamkha, A.; Mallikarjuna, B.; Abdou, M.M.M. Mixed bioconvection flow of a nanofluid containing gyrotactic microorganisms past a vertical slender cylinder. Front. Heat Mass Transf. 2018, 10. [Google Scholar] [CrossRef]
- Rashad, A.M.; Nabwey, H.A. Gyrotactic mixed bioconvection flow of a nanofluid past a circular cylinder with convective boundary condition. J. Taiwan Inst. Chem. Eng. 2019, 99, 9–17. [Google Scholar] [CrossRef]
- Babu, M.J.; Sandeep, N. Effect of nonlinear thermal radiation on non-aligned bio-convective stagnation point flow of a magnetic-nanofluid over a stretching sheet. Alex. Eng. J. 2016, 55, 1931–1939. [Google Scholar] [CrossRef]
- Khan, W.A.; Pop, I. Boundary-layer flow of a nanofluid past a stretching sheet. Int. J. Heat Mass Transf. 2010, 53, 2477–2483. [Google Scholar] [CrossRef]
- Motsa, S.S. A new spectral relaxation method for similarity variable nonlinear boundary layer flow systems. Chem. Eng. Commun. 2014, 201, 241–256. [Google Scholar] [CrossRef]
- Motsa, S.S.; Makukula, Z.G. On spectral relaxation method approach for steady von Kármán flow of a Reiner-Rivlin fluid with Joule heating, viscous dissipation and suction/injection. Cent. Eur. J. Phys. 2013, 11, 363–374. [Google Scholar] [CrossRef]
- Haroun, N.A.; Sibanda, P.; Mondal, S.; Motsa, S.S. On unsteady MHD mixed convection in a nanofluid due to a stretching/shrinking surface with suction/injection using the spectral relaxation method. Bound. Value Probl. 2015, 2015, 24. [Google Scholar] [CrossRef]
- Biliana, B.; Nazar, R. Numerical solution of the boundary layer flow over an exponentially stretching sheet with thermal radiation. Eur. J. Sci. Res. 2009, 33, 710–717. [Google Scholar]
- Magyari, E.; Keller, B. Heat and mass transfer in the boundary layers on an exponentially stretching continuous surface. J. Phys. D Appl. Phys. 1999, 32, 577–585. [Google Scholar] [CrossRef]
- El-Aziz, M.A. Viscous dissipation effect on mixed convection flow of a micropolar fluid over an exponentially stretching sheet. Can. J. Phys. 2009, 87, 359–368. [Google Scholar] [CrossRef]
- Loganthan, P.; Vimala, C. MHD flow of nanofluids over an exponentially stretching sheet embedded in a stratified medium with suction and radiation effects. J. Appl. Fluid Mech. 2015, 8, 85–93. [Google Scholar]

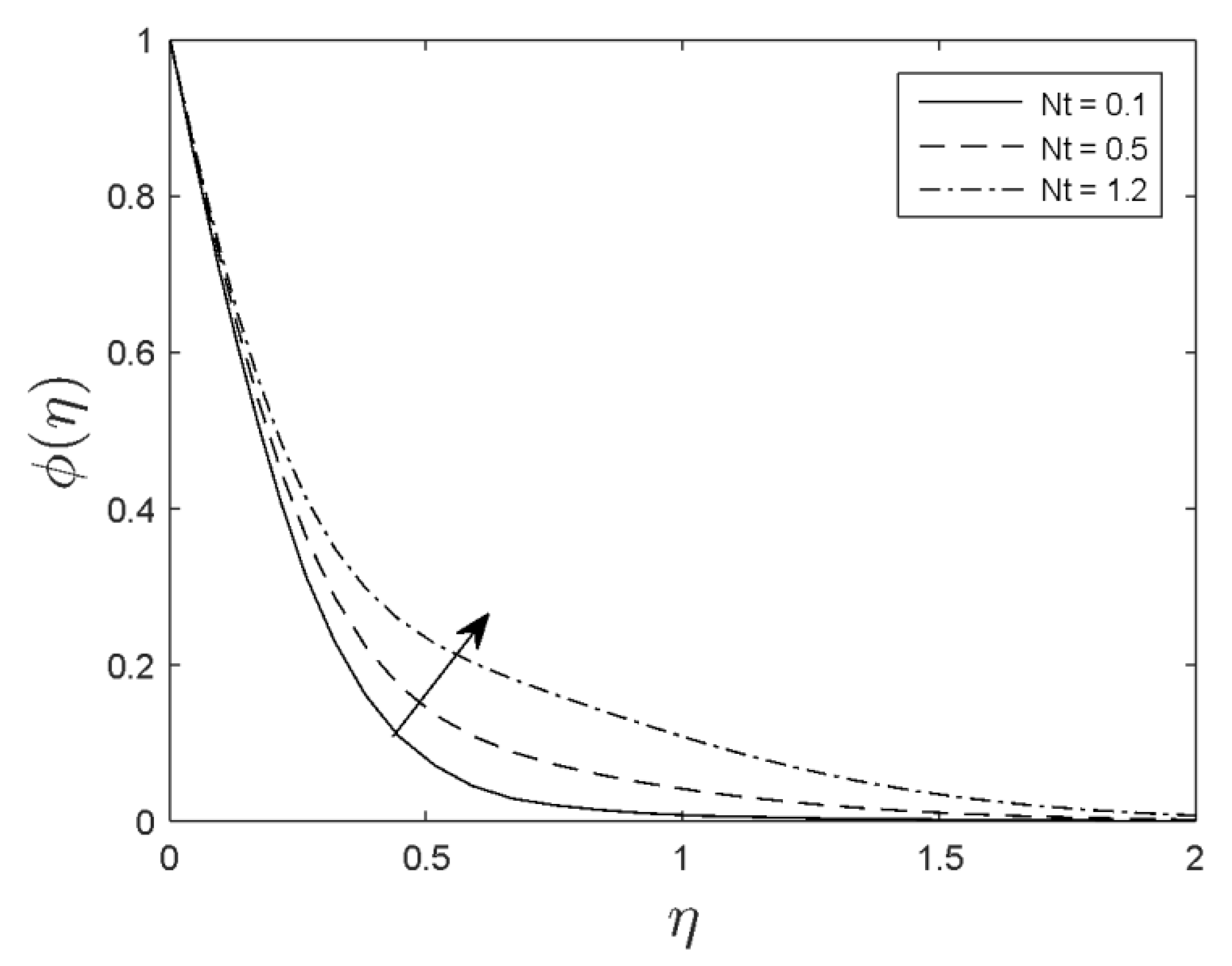
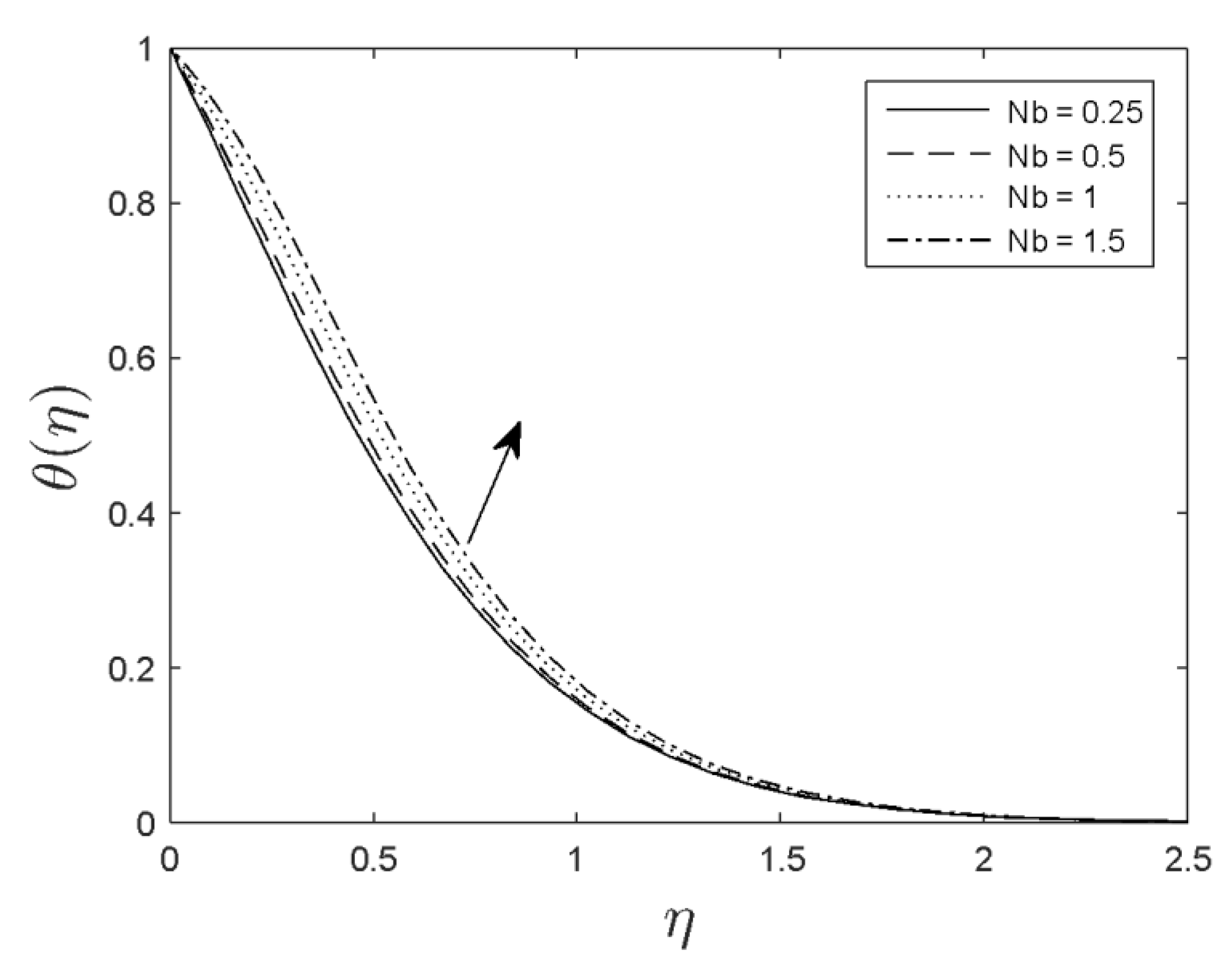
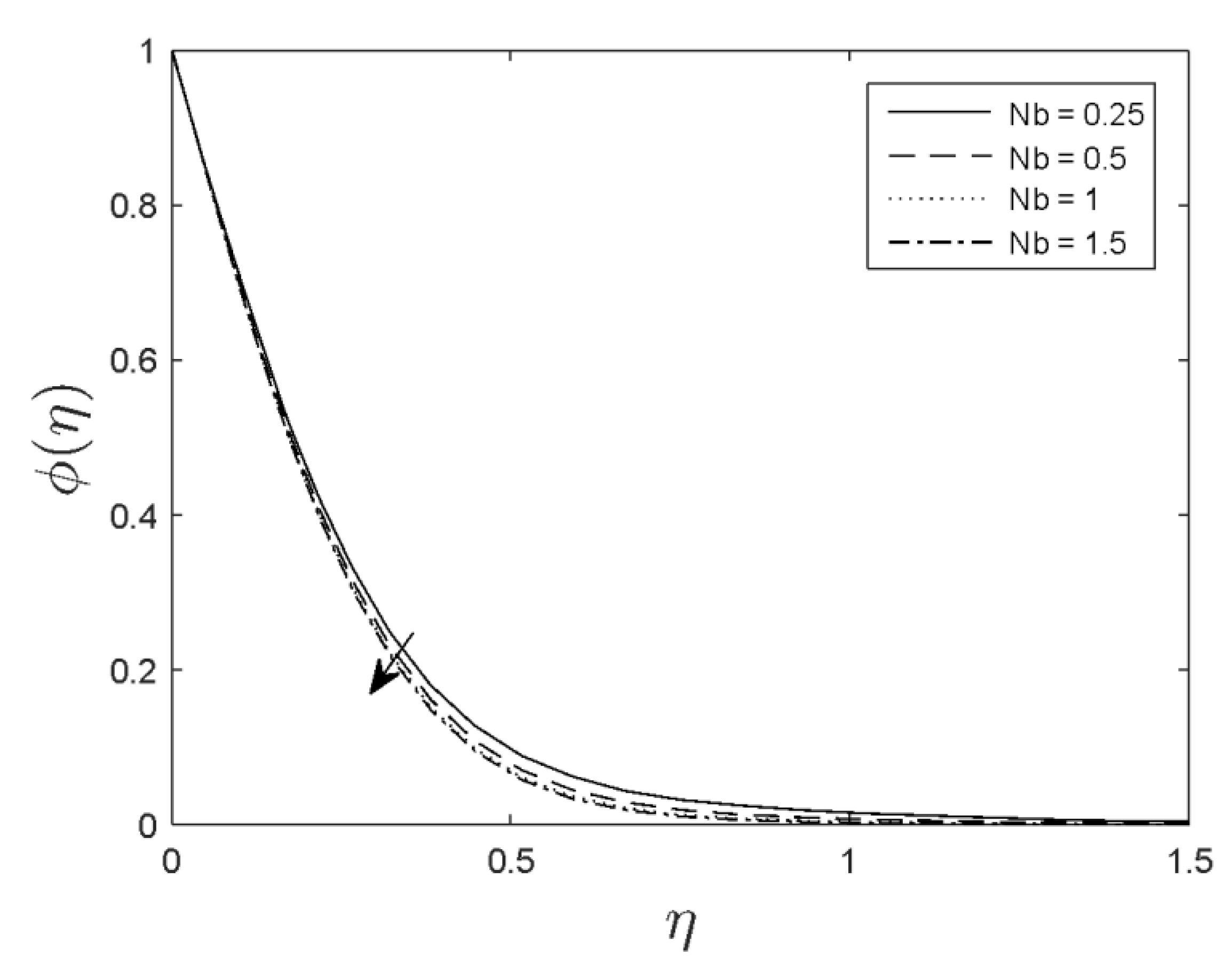
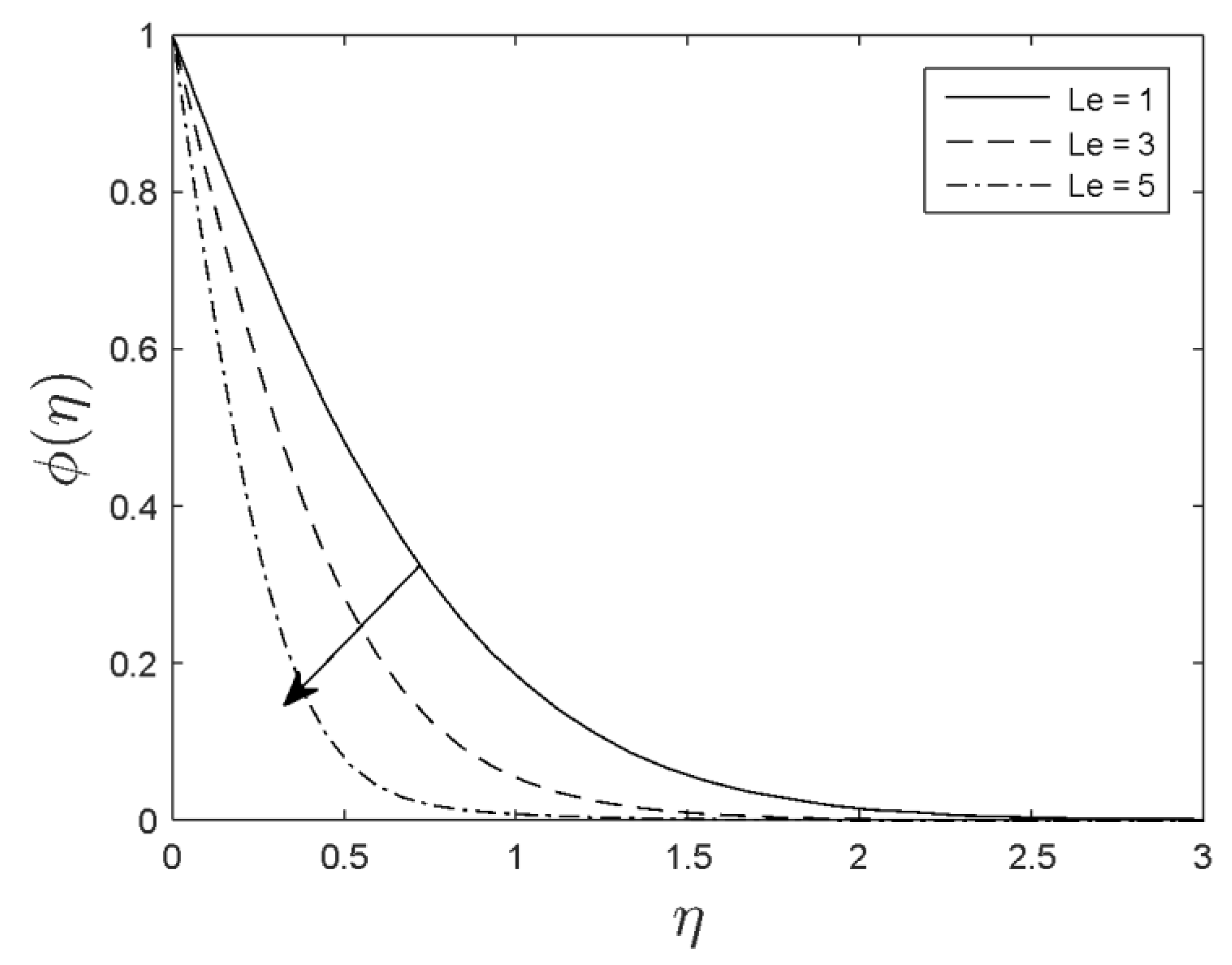
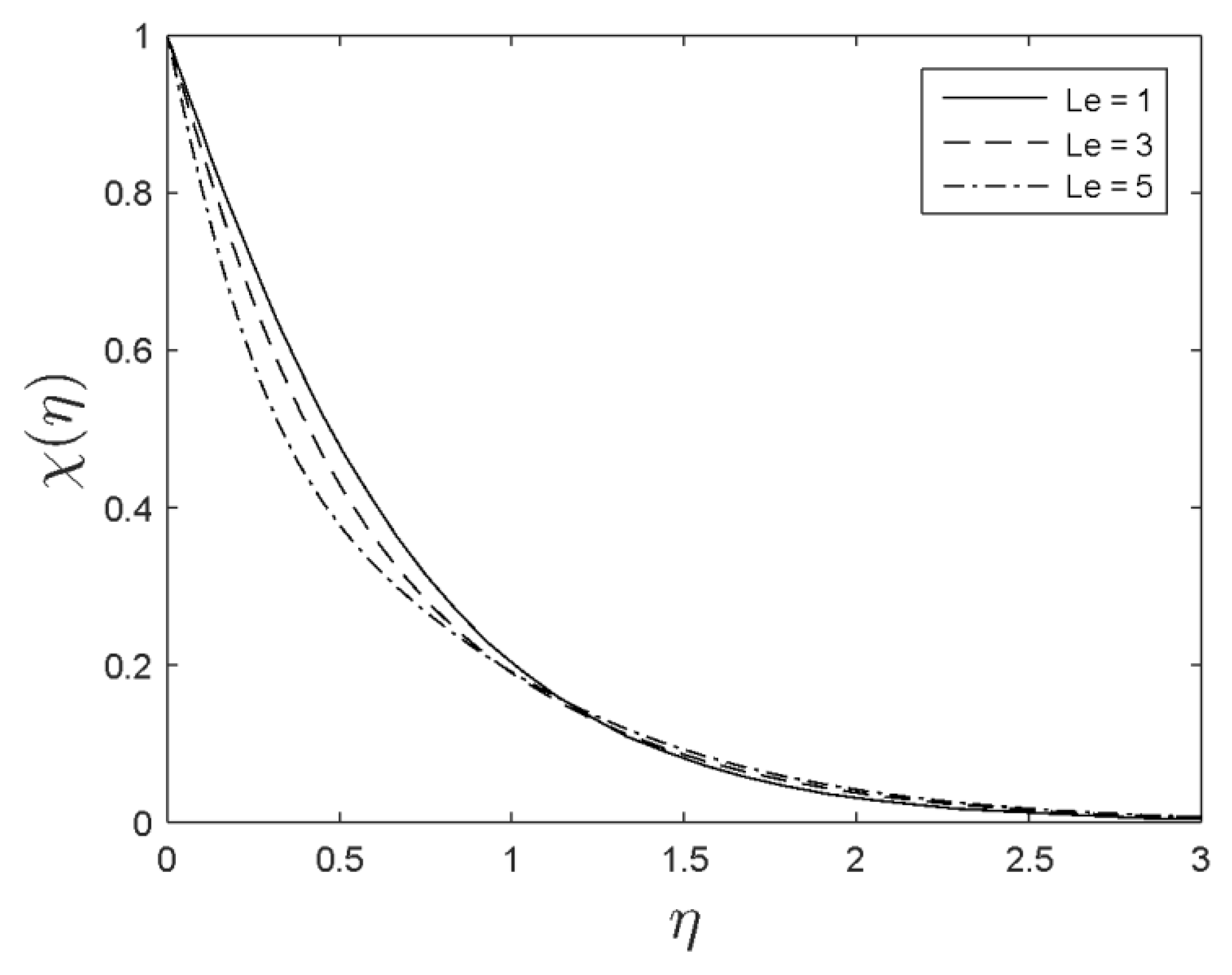
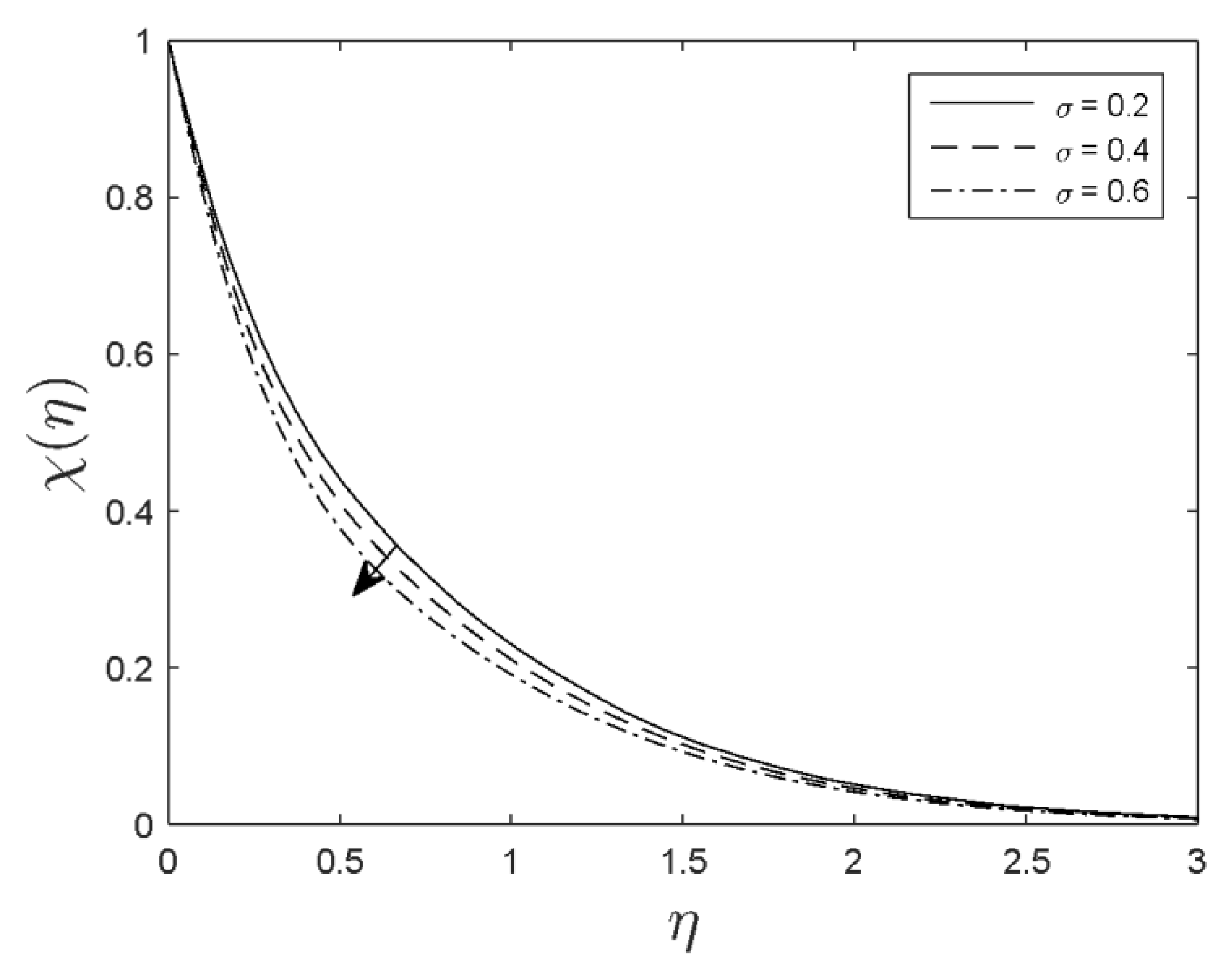

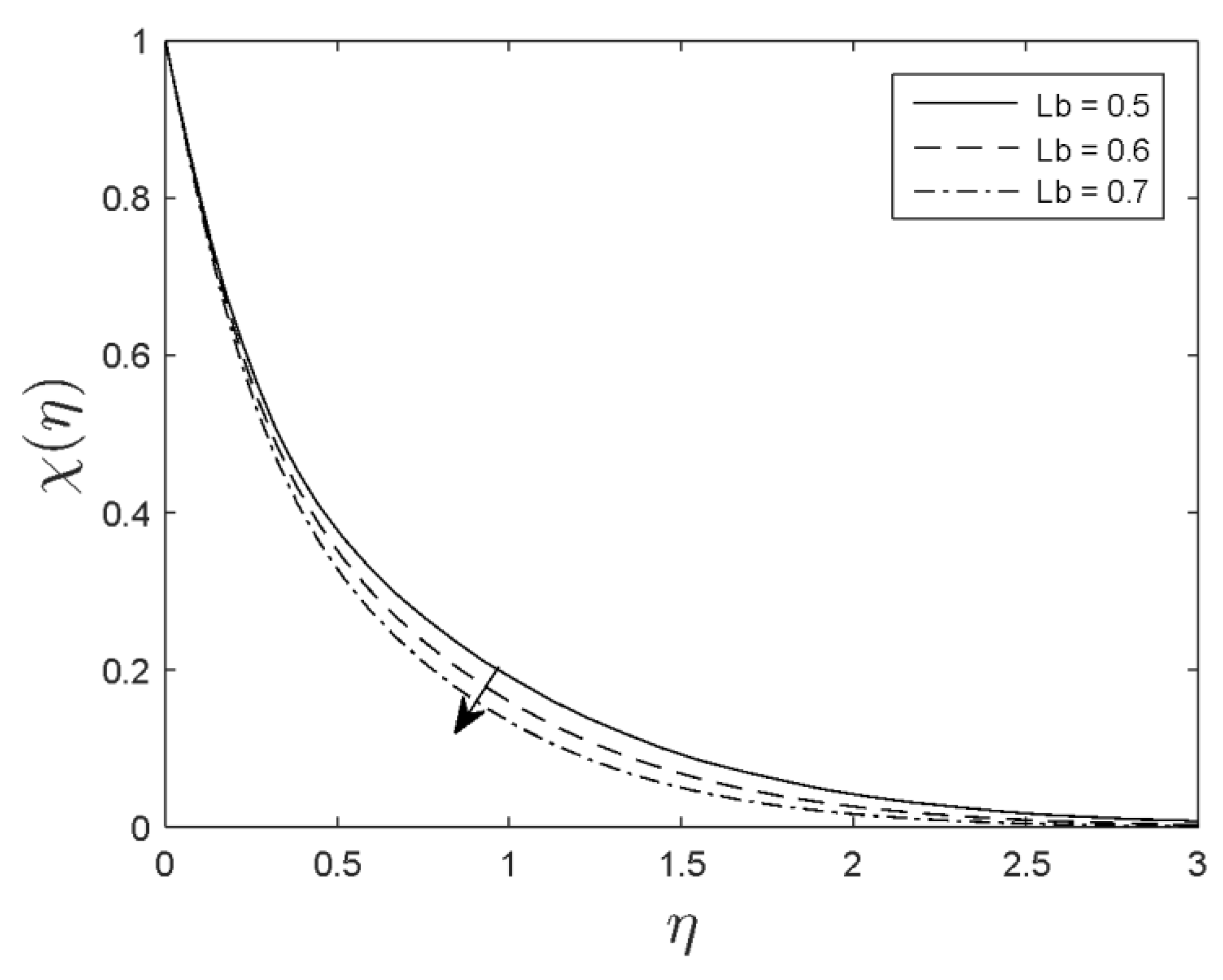
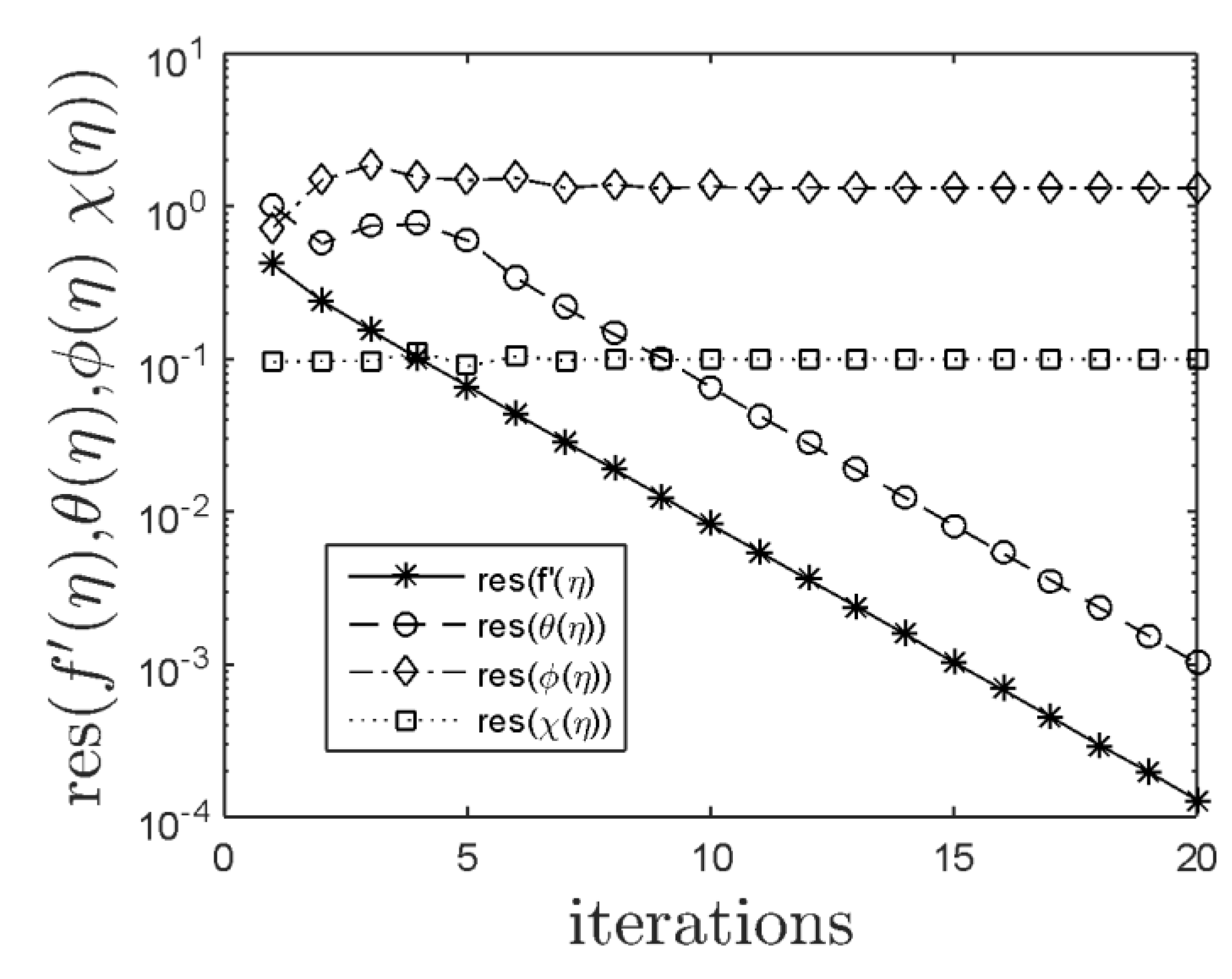
| Pr | Bidin and Nazar [30] | Magyari and Keller [31] | El-Aziz [32] | Loganthan and Vimala [33] | Present Study | |
|---|---|---|---|---|---|---|
| SRM | Bvp4c | |||||
| 1 | 0.9547 | 0.954782 | 0.954785 | 0.954955 | 0.9548 | 0.954782 |
| 1.5 | 1.2348 | 1.234755 | ||||
| 2 | 1.4714 | 1.4715 | 1.471460 | |||
| 2.5 | 1.6802 | 1.680229 | ||||
| 3 | 1.8691 | 1.869075 | 1.869074 | 1.869074 | 1.8691 | 1.869073 |
| 5 | 2.500135 | 2.500132 | 2.500184 | 2.5001 | 2.500131 | |
| 7 | 3.0133 | 3.013277 | ||||
| 10 | 3.660379 | 3.660372 | 3.660379 | 3.6604 | 3.660372 | |
| 20 | 5.3016 | 5.301625 | ||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ferdows, M.; Zaimi, K.; Rashad, A.M.; Nabwey, H.A. MHD Bioconvection Flow and Heat Transfer of Nanofluid through an Exponentially Stretchable Sheet. Symmetry 2020, 12, 692. https://doi.org/10.3390/sym12050692
Ferdows M, Zaimi K, Rashad AM, Nabwey HA. MHD Bioconvection Flow and Heat Transfer of Nanofluid through an Exponentially Stretchable Sheet. Symmetry. 2020; 12(5):692. https://doi.org/10.3390/sym12050692
Chicago/Turabian StyleFerdows, Mohammad, Khairy Zaimi, Ahmed M. Rashad, and Hossam A. Nabwey. 2020. "MHD Bioconvection Flow and Heat Transfer of Nanofluid through an Exponentially Stretchable Sheet" Symmetry 12, no. 5: 692. https://doi.org/10.3390/sym12050692
APA StyleFerdows, M., Zaimi, K., Rashad, A. M., & Nabwey, H. A. (2020). MHD Bioconvection Flow and Heat Transfer of Nanofluid through an Exponentially Stretchable Sheet. Symmetry, 12(5), 692. https://doi.org/10.3390/sym12050692







