Breather’s Properties within the Framework of the Modified Korteweg–de Vries Equation
Abstract
1. Introduction
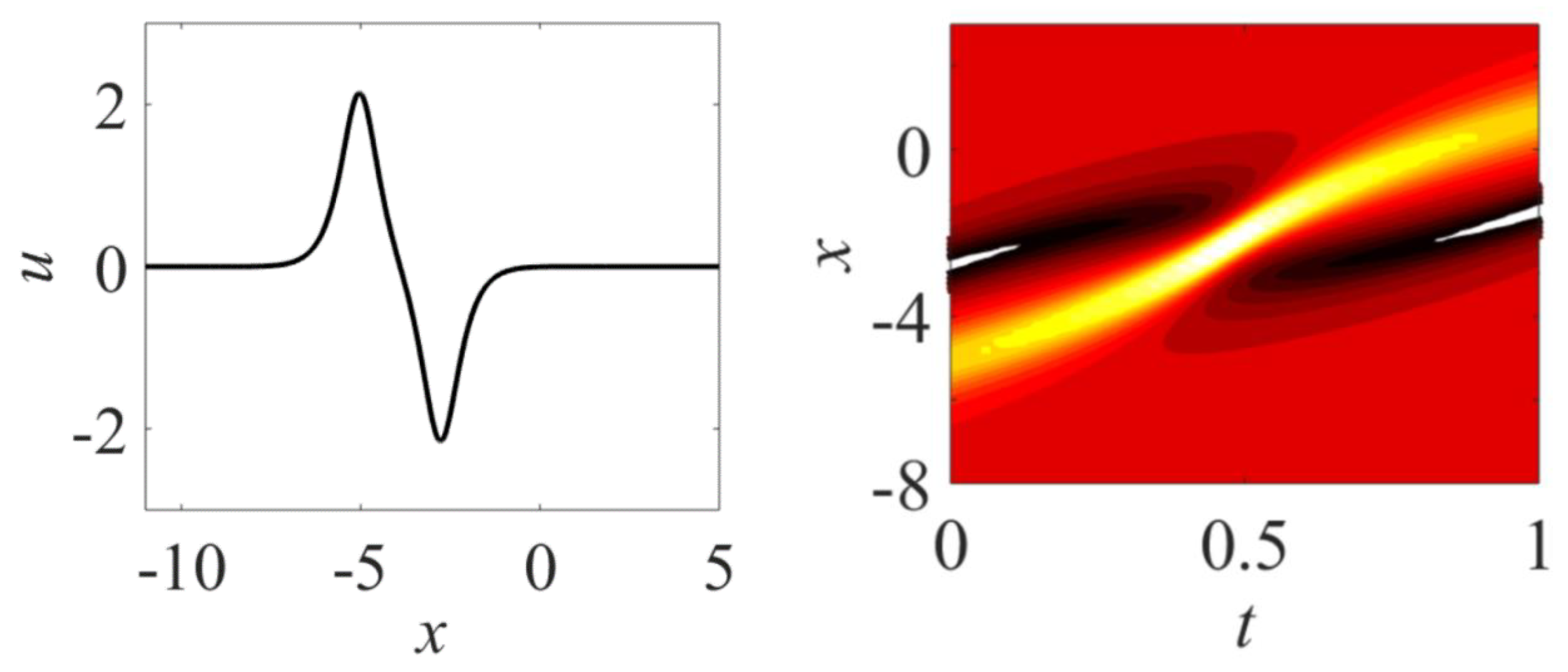
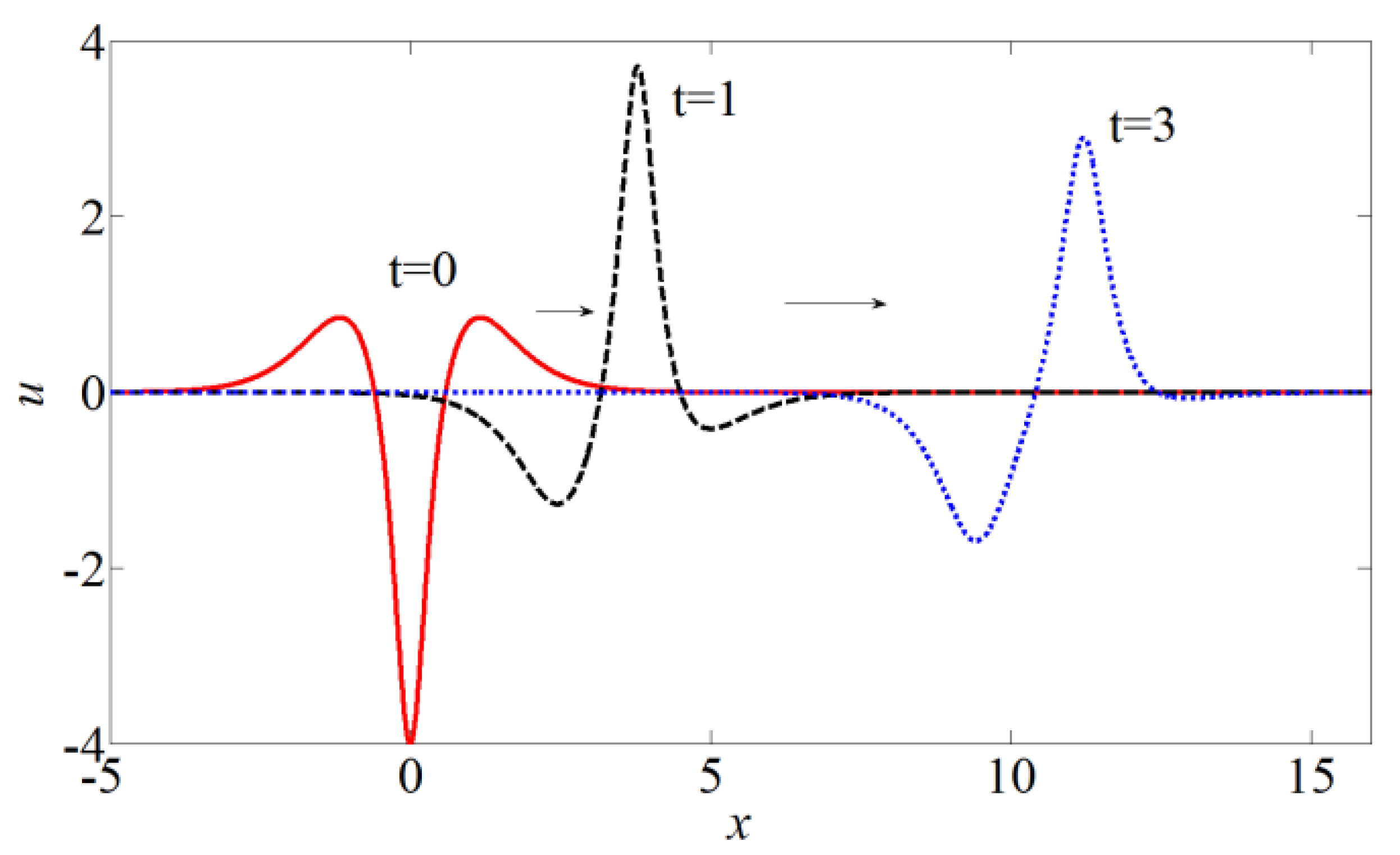
2. MKdV-Breather
- q << p: and as in the linear problem;
- q >> p: and as in the two-soliton solution;
- q = p, and as in the intermediate case.
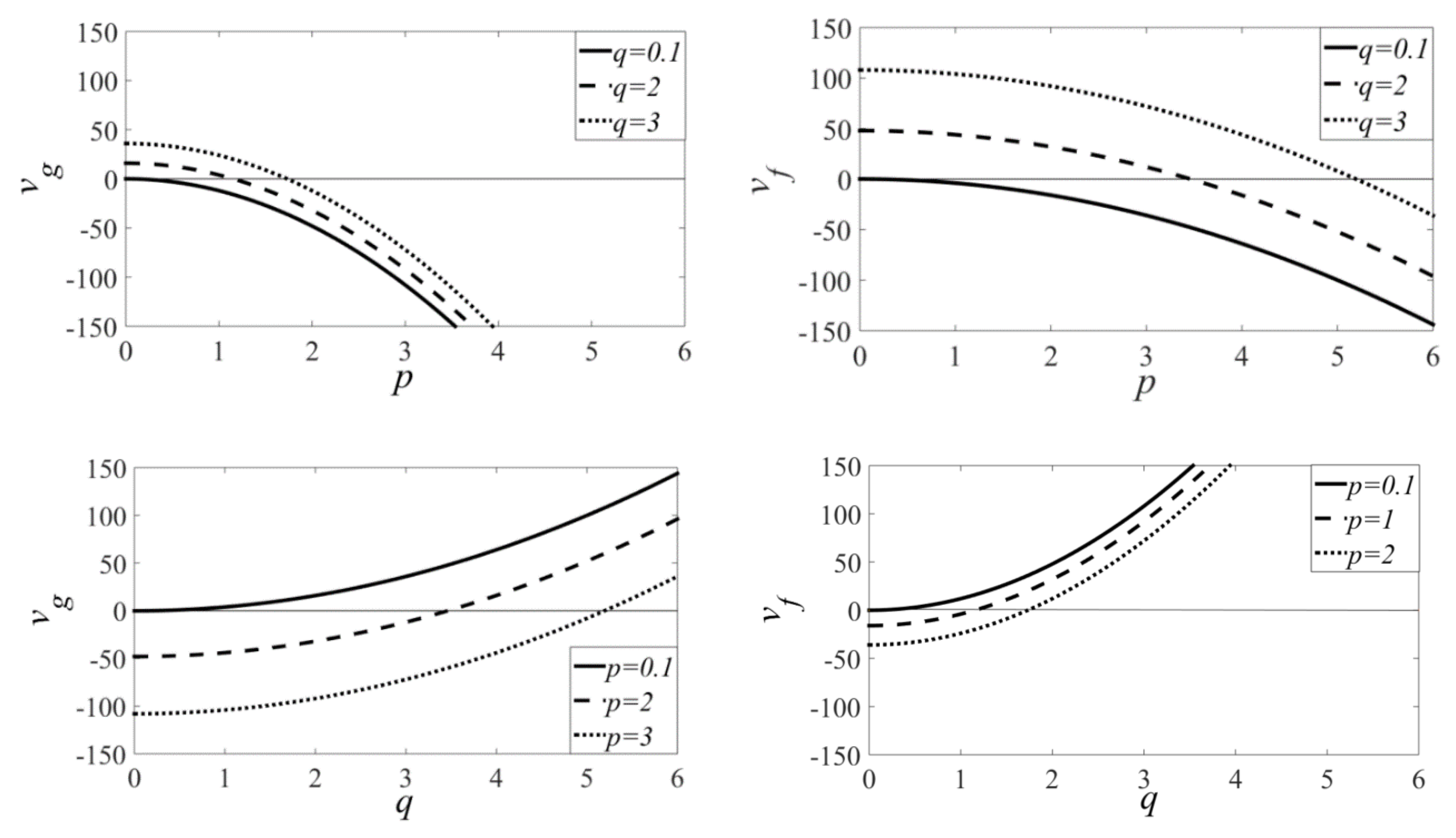
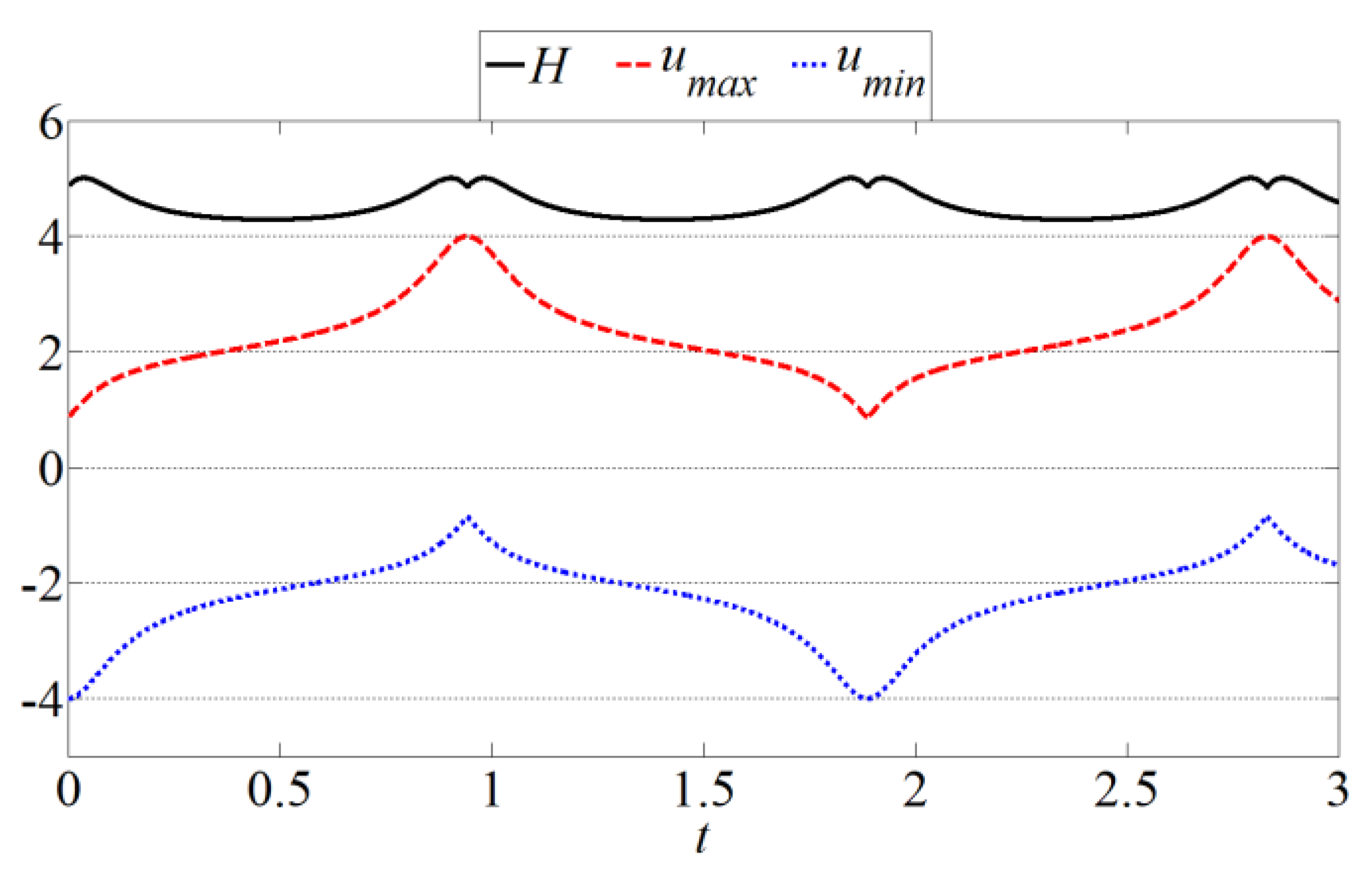
3. Breather’s Extrema
- (i)
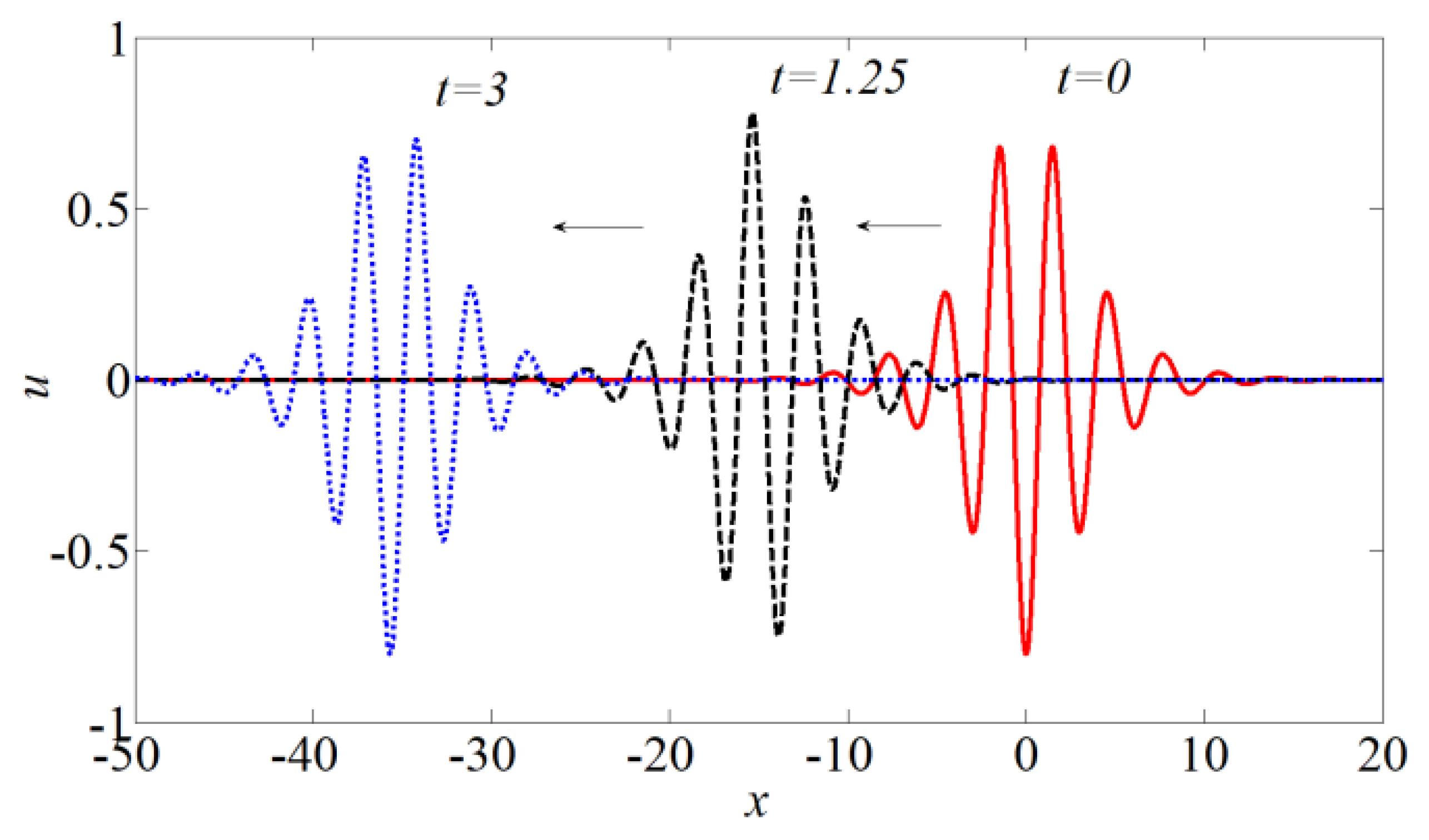
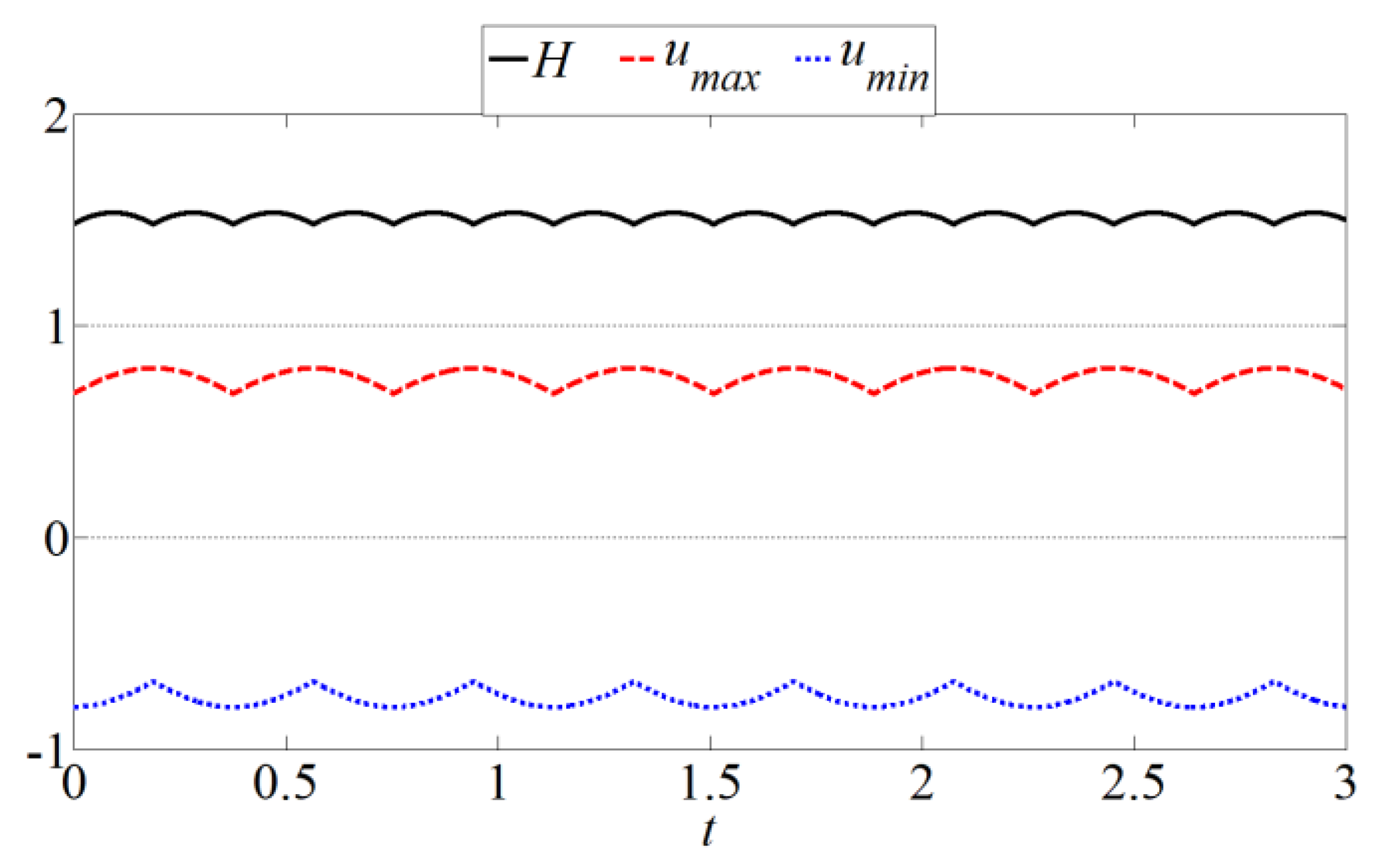
- case: q/p >> 1. Let us fix q = 1, p = 0.2 ().
- (ii)
- case: q/p << 1. Let us fix q = 0.2, p = 1 ().
- (iii)
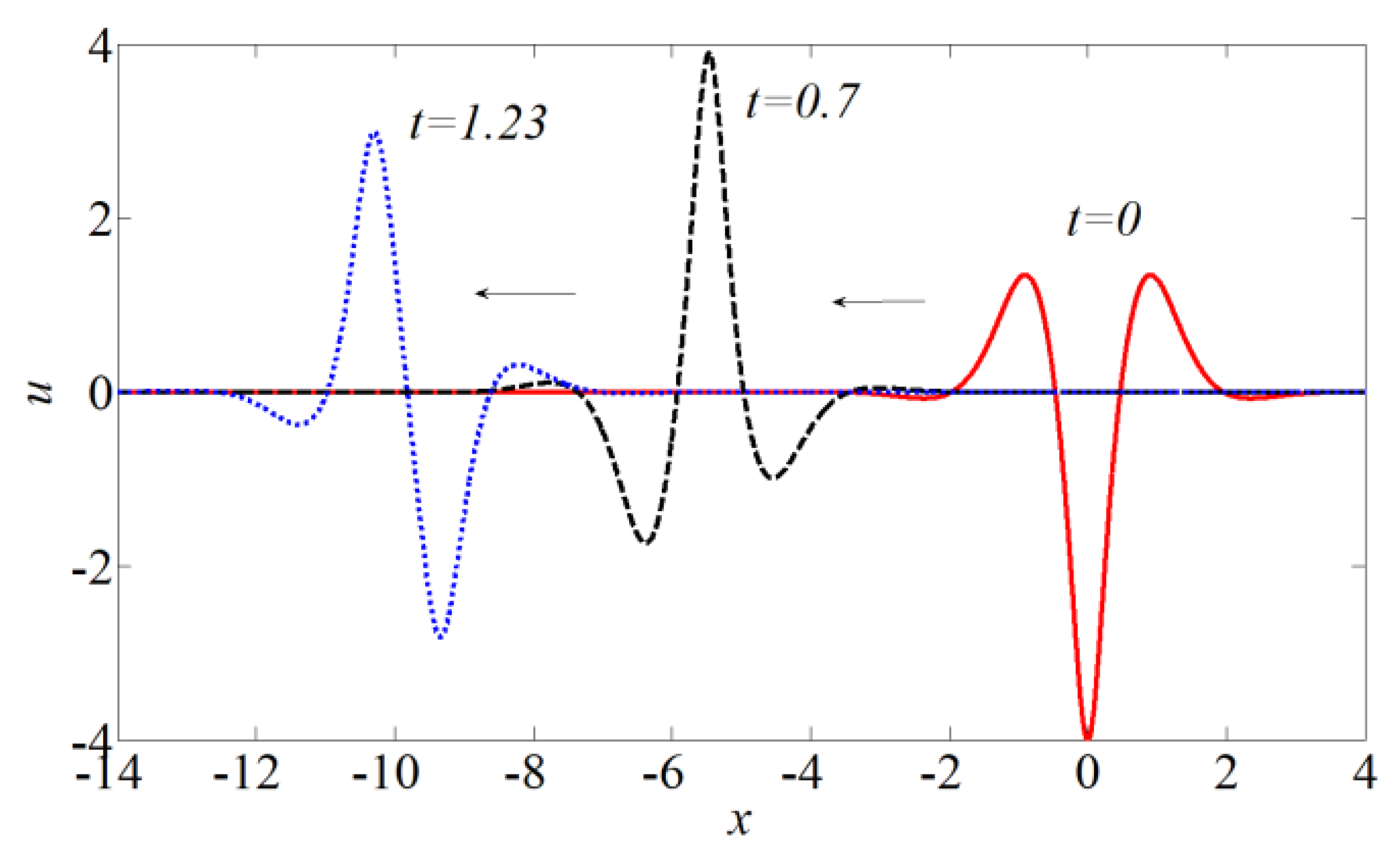
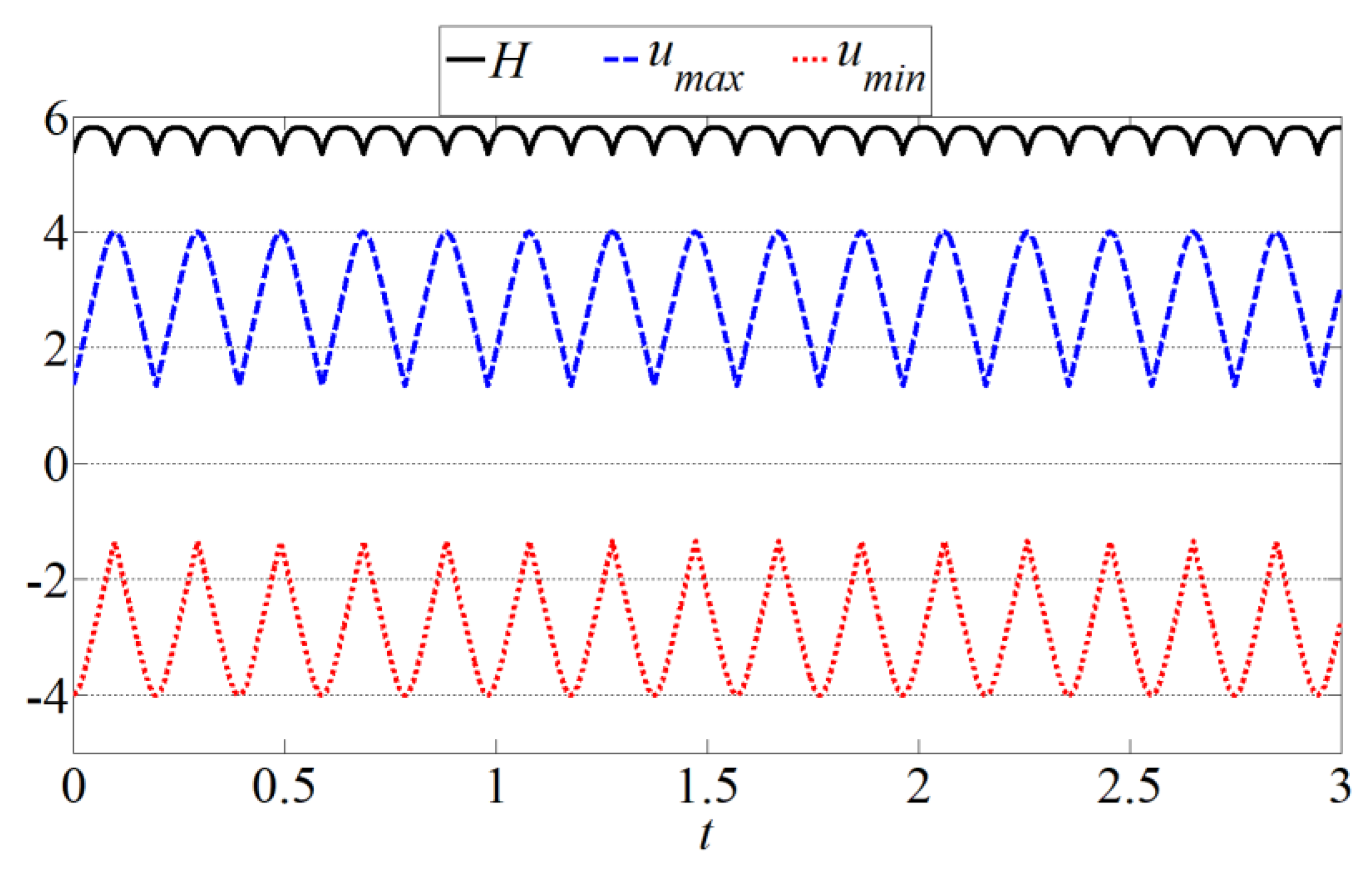
- case: q/p = 1. Let us fix q = 1, p = 1 ().
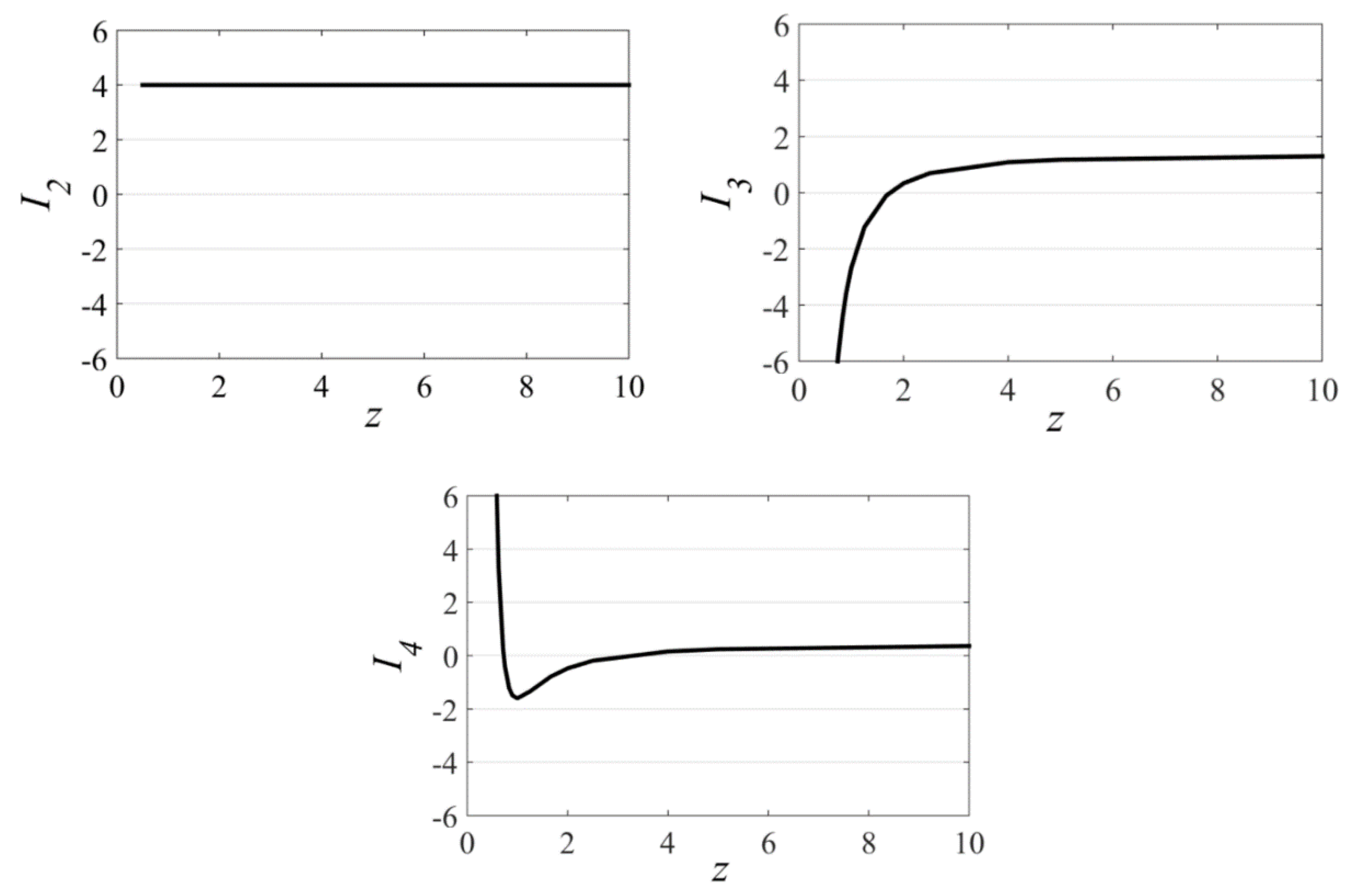
4. Breather’s Invariants
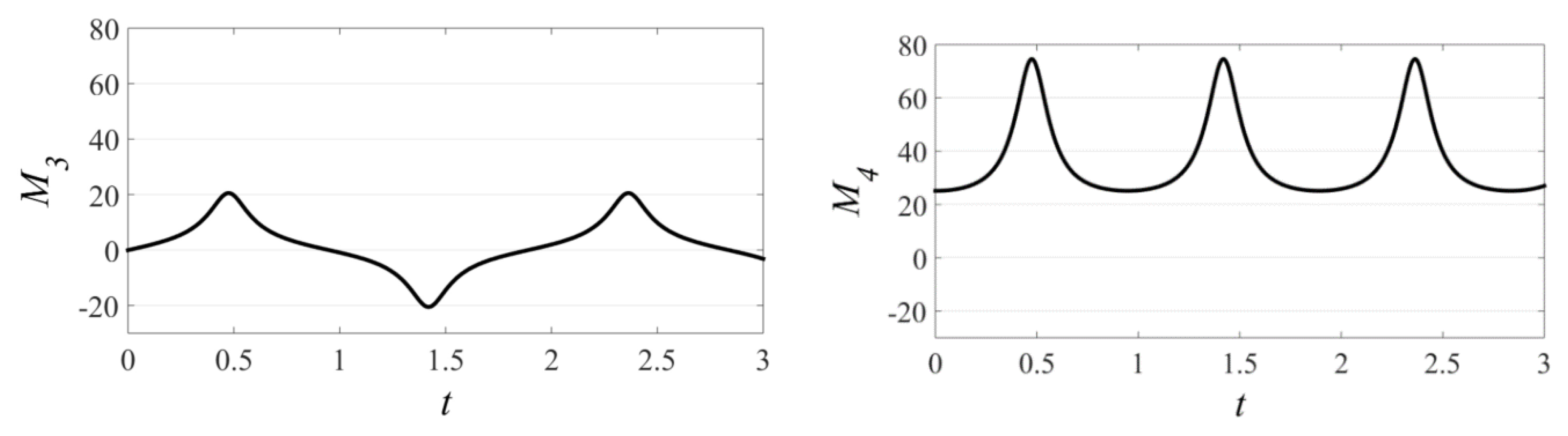
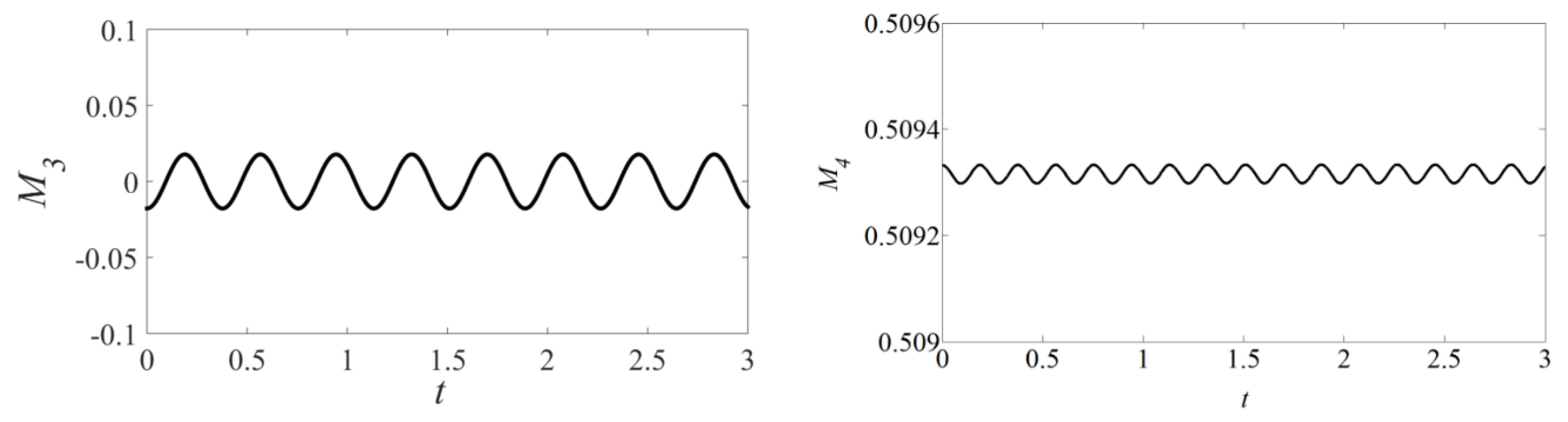
5. Breather’s Moments
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zakharov, V.E. Kinetic equation for solitons. Sov. Phys. JETP 1971, 60, 993–1000. [Google Scholar]
- El, G.A.; Kamchatnov, A.M. Kinetic equation for a dense soliton gas. Phys. Rev. Lett. 2005, 95, 1–4. [Google Scholar] [CrossRef]
- El, G.A.; Kamchatnov, A.M.; Pavlov, M.V.; Zykov, S.A. Kinetic equation for a soliton gas and its hydrodynamic reductions. J. Nonlinear Sci. 2011, 21, 151–191. [Google Scholar] [CrossRef]
- Gelash, A.; Agafontsev, D.; Zakharov, V.; El, G.; Randoux, S.; Suret, P. Bound state soliton gas dynamics underlying the spontaneous modulational instability. Phys. Rev. Lett. 2019, 123, 234102. [Google Scholar] [CrossRef] [PubMed]
- Agafontsev, D.S.; Zakharov, V.E. Integrable turbulence generated from modulational instability of cnoidal waves. Nonlinearity 2016, 29, 3551–3578. [Google Scholar] [CrossRef]
- Pelinovsky, E.; Shurgalina, E. KDV soliton gas: Interactions and turbulence. In Challenges in Complexity: Dynamics, Patterns, Cognition; Aronson, I., Pikovsky, A., Rulkov, N., Tsimring, L., Eds.; Series: Nonlinear Systems and Complexity; Springer: Berlin, Germany, 2017; Volume 20, pp. 295–306. [Google Scholar]
- Didenkulova, E.G.; Pelinovsky, E.N. The role of a thick soliton in the dynamics of the soliton gas within the framework of the Gardner equation. Radiophys. Quantum Electron. 2019, 61, 623–632. [Google Scholar] [CrossRef]
- Shurgalina, E.G.; Pelinovsky, E.N. Nonlinear dynamics of a soliton gas: Modified Korteweg-de Vries equation framework. Phys. Lett. A 2016, 380, 2049–2053. [Google Scholar] [CrossRef]
- El, G.A. Critical density of a soliton gas. Chaos 2016, 26, 023105. [Google Scholar] [CrossRef]
- Gelash, A.A.; Agafontsev, D.S. Strongly interacting soliton gas and formation of rogue waves. Phys. Rev. E 2018, 98, 1–11. [Google Scholar] [CrossRef]
- Pelinovsky, E.N.; Shurgalina, E.G. Formation of freak waves in a soliton gas described by the modified Korteweg–de Vries equation. Dokl. Phys. 2016, 61, 423–426. [Google Scholar] [CrossRef]
- Didenkulova (Shurgalina), E.G. Numerical modeling of soliton turbulence within the focusing Gardner equation: Rogue wave emergence. Phys. D 2019, 399, 35–41. [Google Scholar] [CrossRef]
- Dudley, J.M.; Dias, F.; Erkintalo, M.; Genty, G. Instabilities, breathers and rogue waves in optics. Nat. Photonics 2014, 8, 755–764. [Google Scholar] [CrossRef]
- Kharif, C.; Pelinovsky, E.; Slunyaev, A. Rogue Waves in the Ocean; Springer: Berlin, Germany, 2009; p. 216. [Google Scholar]
- Onorato, M.; Resitori, S.; Baronio, F. Rogue and Shock Waves in Nonlinear Dispersive Media; Lecture Notes in Physics; Springer International Publishing: New York, NY, USA, 2016. [Google Scholar]
- Slunyaev, A.V.; Pelinovsky, E.N. Role of multiple soliton interactions in the generation of rogue waves: The modified korteweg-de vries framework. Phys. Rev. Lett. 2016, 117, 214501. [Google Scholar] [CrossRef] [PubMed]
- Slunyaev, A. On the optimal focusing of solitons and breathers in long-wave models. Stud. Appl. Math. 2019, 142, 385–413. [Google Scholar] [CrossRef]
- Pelinovsky, E.N.; Shurgalina, E.G.; Sergeeva, A.V.; Talipova, T.G.; El, G.A.; Grimshaw, R.H.J. Two-soliton interaction as an elementary act of soliton turbulence in integrable systems. Phys. Lett. A 2013, 377, 272–275. [Google Scholar] [CrossRef]
- Pelinovsky, E.N.; Shurgalina, E.G. Two-soliton interaction in the frameworks of modified Korteweg–de Vries equation. Radiophys. Quantum Electron. 2015, 57, 737–744. [Google Scholar] [CrossRef]
- Shurgalina, E.G. The features of the paired soliton interactions within the framework of the Gardner equation. Radiophys. Quantum Electron. 2018, 60, 703–708. [Google Scholar] [CrossRef]
- Shurgalina, E.G. The mechanism of the formation of freak waves in the result of interaction of internal waves in stratified basin. Fluid Dyn. 2018, 53, 59–64. [Google Scholar] [CrossRef]
- Lamb, G.L. Elements of Soliton Theory; Wiley: New York, NY, USA, 1980; p. 289. [Google Scholar]
- Lamb, K.G.; Polukhina, O.; Talipova, T.; Pelinovsky, E.; Xiao, W.; Kurkin, A. Breather generation in fully nonlinear models of a stratified fluid. Phys. Rev. E 2007, 75, 046306. [Google Scholar] [CrossRef]
- Lee, J.H.; Lozovatsky, I.; Jang, S.-T.; Jang, C.J.; Hong, C.S.; Fernando, H.J.S. Episodes of nonlinear internal waves in the northern East China Sea. Geoph. Res. Lett. 2006, 33, L18601. [Google Scholar] [CrossRef]
- Shroyer, E.L.; Moum, J.N.; Nash, J.D. Mode 2 waves on the continental shelf: Ephemeral components of the nonlinear internal wavefield. JGR 2010, 115, C07001. [Google Scholar] [CrossRef]
- Vlasenko, V.; Stashchuk, N. Internal tides near the Celtic Sea shelf break: A new look at a well known problem. Deep Sea Res. I 2015, 103, 24–36. [Google Scholar] [CrossRef]
- Alejo, M.A.; Munoz, C. Nonlinear stability of mKdV breathers. Commun. Math. Phys. 2013, 324, 233–262. [Google Scholar] [CrossRef]
- Alejo, M.A.; Munoz, C.; Palacios, J.M. On the variational structure of Breather Solutions II: Periodic mKdV case. Electron. J. Diff. Eqns. 2017, 2017, 1–26. [Google Scholar]
- Chow, K.W.; Grimshaw, R.H.J.; Ding, E. Interactions of breathers and solitons in the extended Korteweg–de Vries equation. Wave Motion 2005, 43, 158–166. [Google Scholar] [CrossRef]
- Kachulin, D.; Dyachenko, A.; Gelash, A. Interactions of coherent structures on the surface of deep water. Fluids 2019, 4, 83. [Google Scholar] [CrossRef]
- Kachulin, D.; Gelash, A. On the phase dependence of the soliton collisions in the Dyachenko–Zakharov envelope equation. Nonlin. Process. Geophys. 2018, 25, 553–563. [Google Scholar] [CrossRef]
- Xu, G.; Gelash, A.; Chabchoub, A.; Zakharov, V.; Kibler, B. Breather wave molecules. Phys. Rev. Lett. 2019, 122, 084101. [Google Scholar] [CrossRef]
- Akhmediev, N.; Soto-Crespo, J.M.; Devine, N. Breather turbulence versus soliton turbulence: Rogue waves, probability density functions, and spectral features. Phys. Rev. E 2016, 94, 022212. [Google Scholar] [CrossRef]
- Soto-Crespo, J.M.; Devine, N.; Akhmediev, N. Integrable Turbulence and rogue waves: Breathers or solitons? Phys. Rev. Lett. 2016, 116, 103901. [Google Scholar] [CrossRef]
- Zakharov, V.E.; Kuznetsov, E.A. Multi-scale expansions in the theory of systems integrable by the inverse scattering transform. Phys. D 1986, 18, 455–463. [Google Scholar] [CrossRef]
- Ablowitz, M.J. Nonlinear Dispersive Waves: Asymptotic Analysis and Solitons; Cambridge University Press: Cambridge, UK, 2011; p. 364. [Google Scholar]
- Fronberg, B. A Practical Guide to Pseudospectral Methods; Cambridge University Press: Cambridge, UK, 1998; p. 231. [Google Scholar]
- Miura, R.M.; Gardner, C.S.; Kruskal, M.D. Korteweg-de Vries equation and generalizations, II. Existence of conservation laws and constants of motion. J. Math. Phys. 1968, 9, 1204–1209. [Google Scholar] [CrossRef]
- Monin, A.S.; Yaglom, A.M. Statistical Fluid Mechanics; MIT Press: Cambridge, MA, USA, 1971; p. 769. [Google Scholar]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Didenkulova, E.; Pelinovsky, E. Breather’s Properties within the Framework of the Modified Korteweg–de Vries Equation. Symmetry 2020, 12, 638. https://doi.org/10.3390/sym12040638
Didenkulova E, Pelinovsky E. Breather’s Properties within the Framework of the Modified Korteweg–de Vries Equation. Symmetry. 2020; 12(4):638. https://doi.org/10.3390/sym12040638
Chicago/Turabian StyleDidenkulova, Ekaterina, and Efim Pelinovsky. 2020. "Breather’s Properties within the Framework of the Modified Korteweg–de Vries Equation" Symmetry 12, no. 4: 638. https://doi.org/10.3390/sym12040638
APA StyleDidenkulova, E., & Pelinovsky, E. (2020). Breather’s Properties within the Framework of the Modified Korteweg–de Vries Equation. Symmetry, 12(4), 638. https://doi.org/10.3390/sym12040638





