Second Grade Bioconvective Nanofluid Flow with Buoyancy Effect and Chemical Reaction
Abstract
1. Introduction
2. Mathematical Modeling
3. Methodology
4. Analysis
5. Conclusions
- For larger Rayleigh numbers, the bio-convection restricts the upward movement of nanoparticles that are involved in nanofluids for the given buoyancy effect. Furthermore, larger buoyancy is instigated which certainly opposes the fluid flow and affects the concentration. Thus, a sufficient decline in flow movement is noticed for both parameters.
- The influence of grade-II fluid parameter is quite obvious on flow movement. There is an inverse relation in fluid parameter and fluid viscosity. For larger values of fluid parameters, the fluid viscosity faces a decline and certainly less restriction is faced by the fluid. As a result, fluid velocity increases.
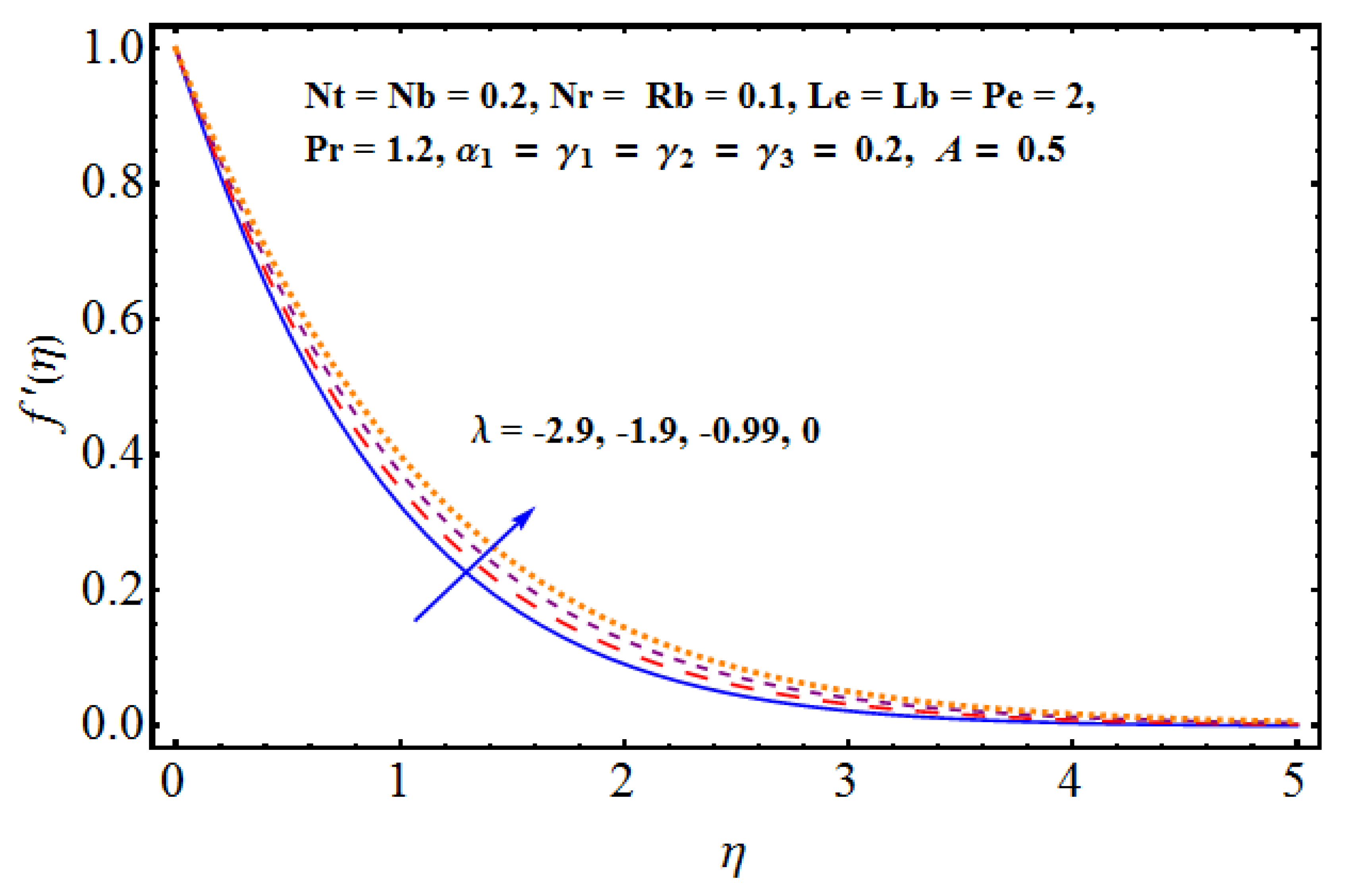
- The mixed convection parameter is responsible for the variations noticed in the velocity field. This phenomenon is discussed in lieu of (a) assisting and (b) opposing flows. In both assisting and opposing cases, we notice a certain rise in fluid motion.
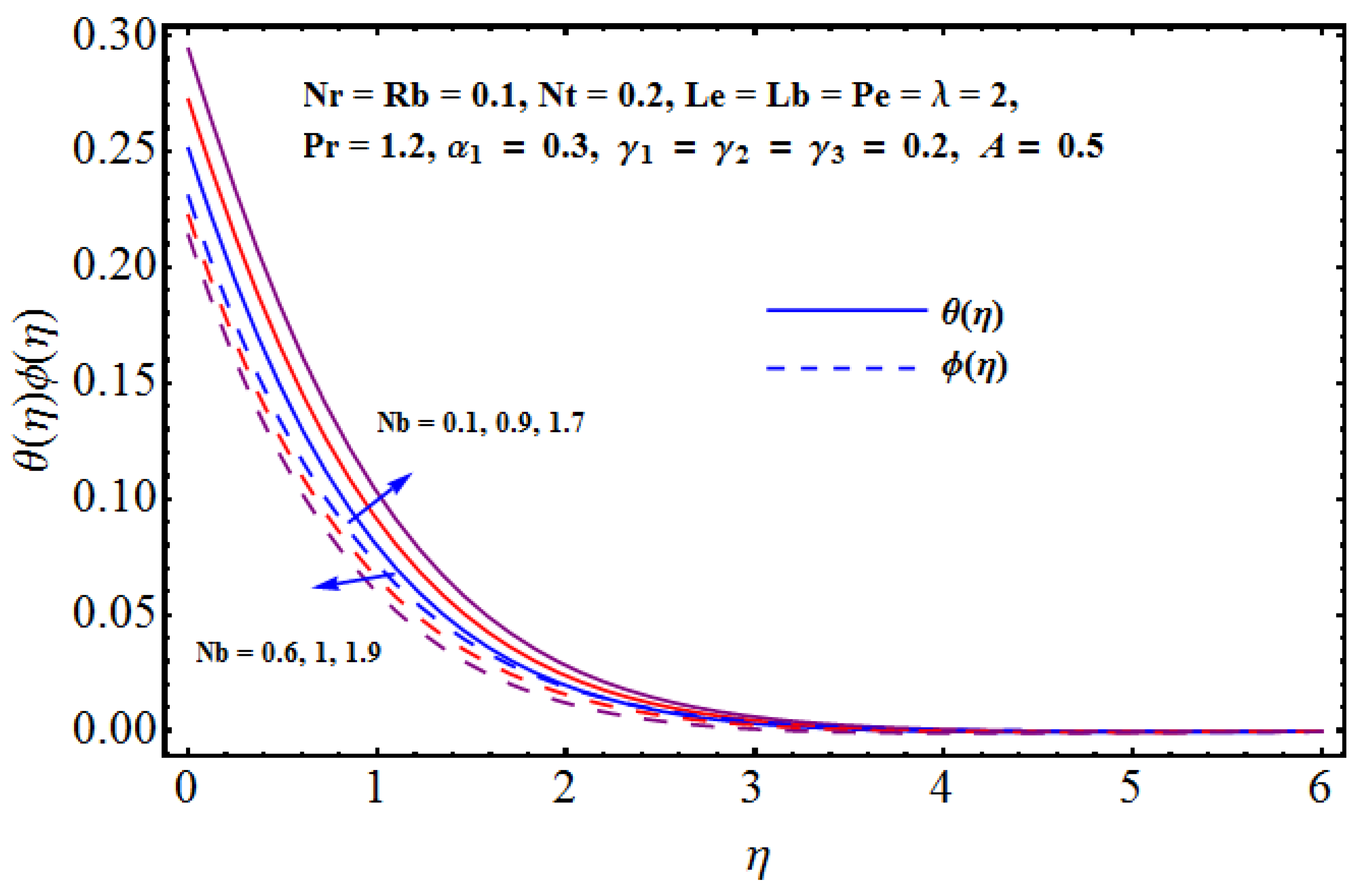
- The thermal layer receives enhancement for larger values of Brownian diffusion parameters. The random motion for stronger Brownian impact suddenly rises which abruptly enhances the heat production and consequently thermal distribution receives enhancement.
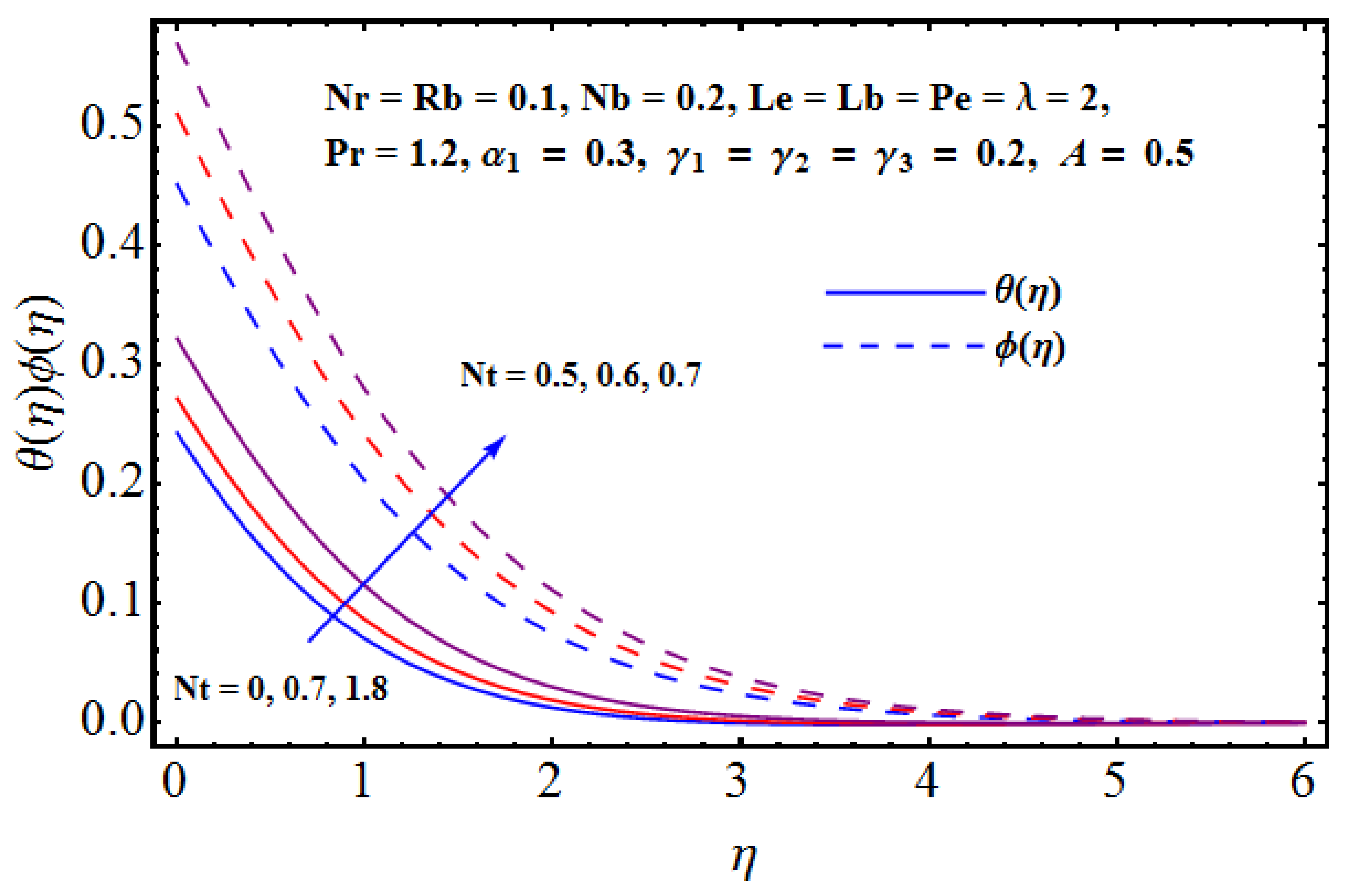
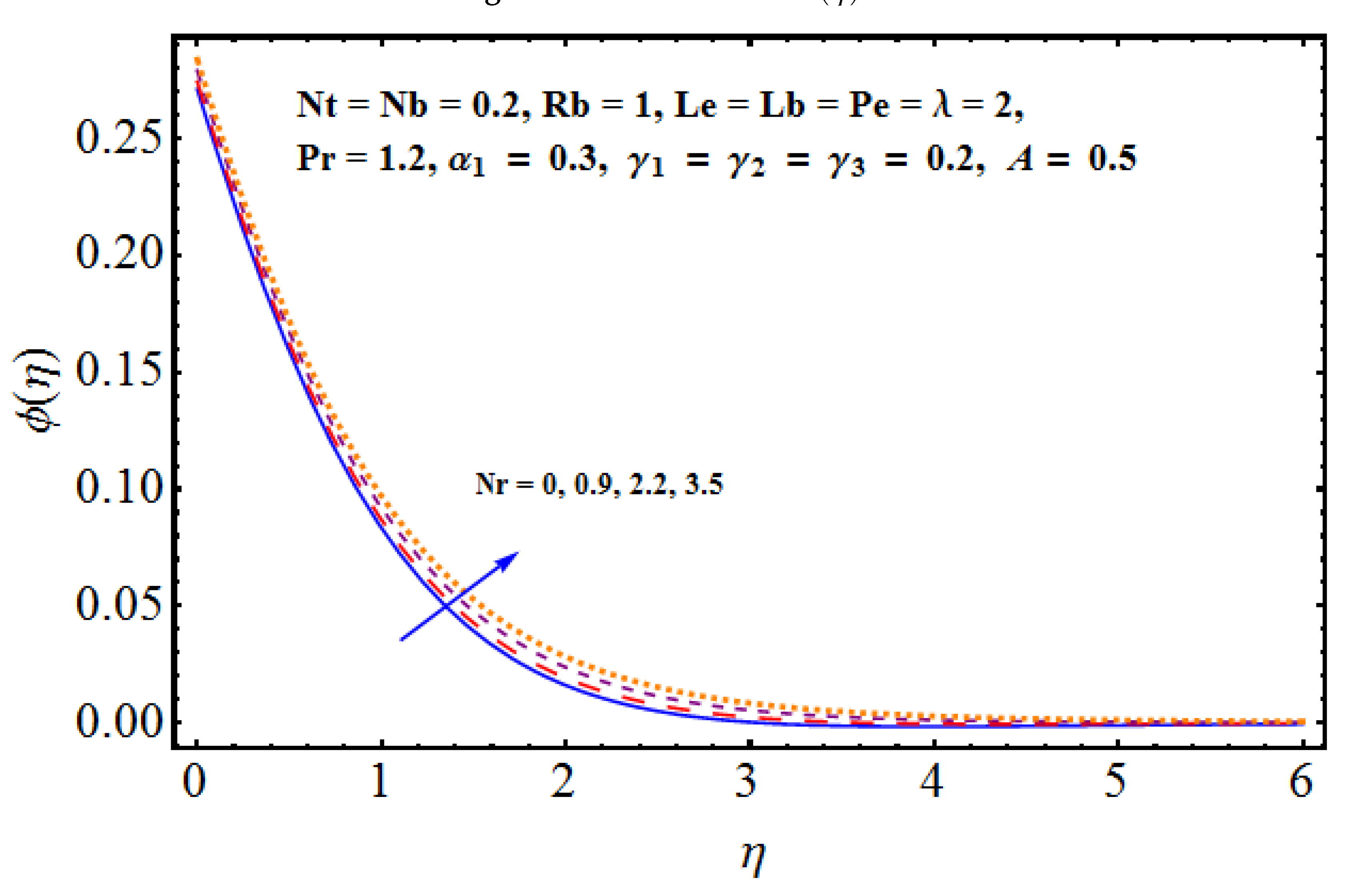
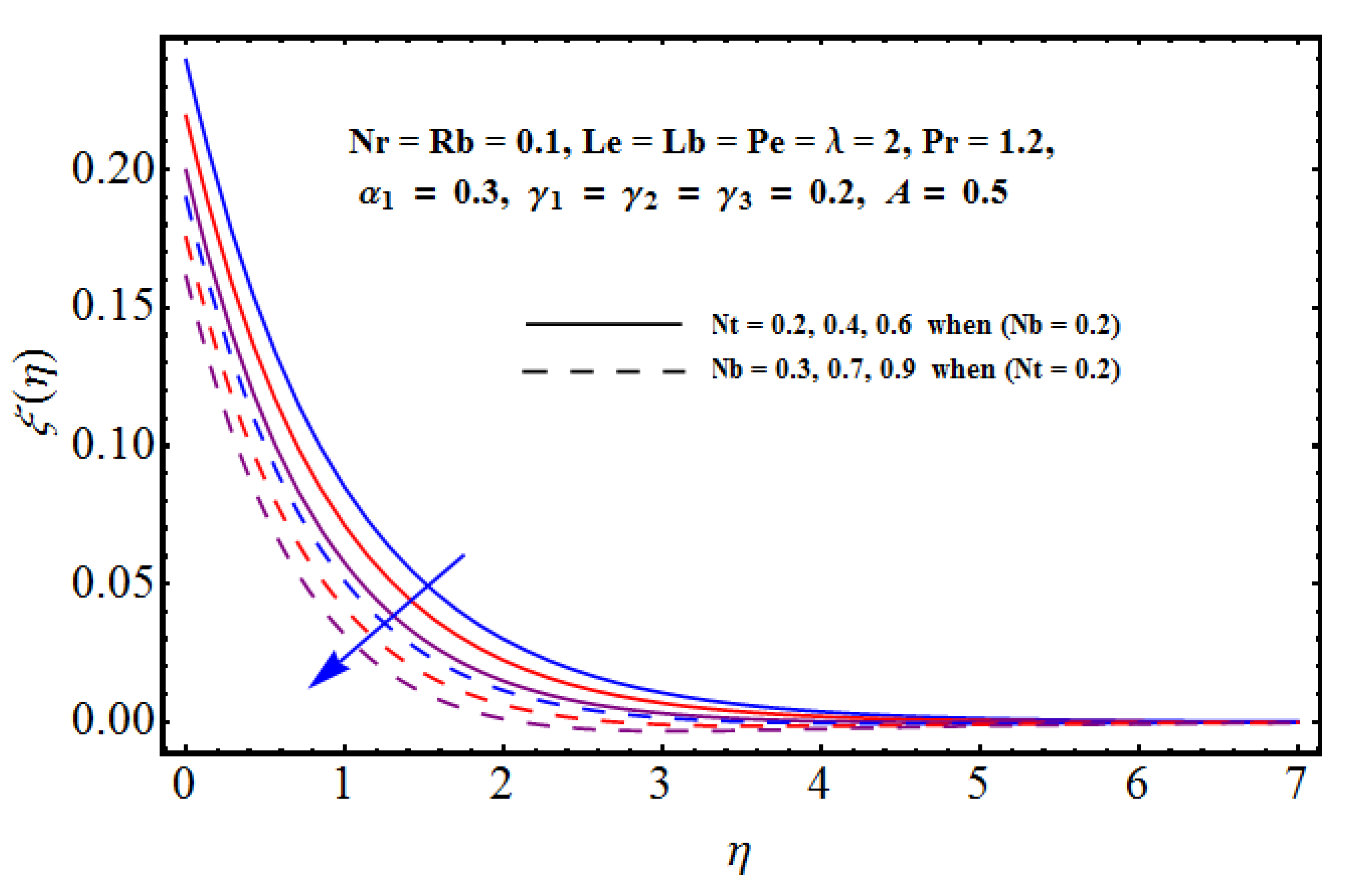
- An assistance is offered by the thermophoresis parameter for both the thermal and solute/nanoparticles diffusion. For positive and intensive thermophoresis, the plate is cold enough and, subsequently, the nanoparticles are transported from hot to colder regions which augments nanoparticle concentration.
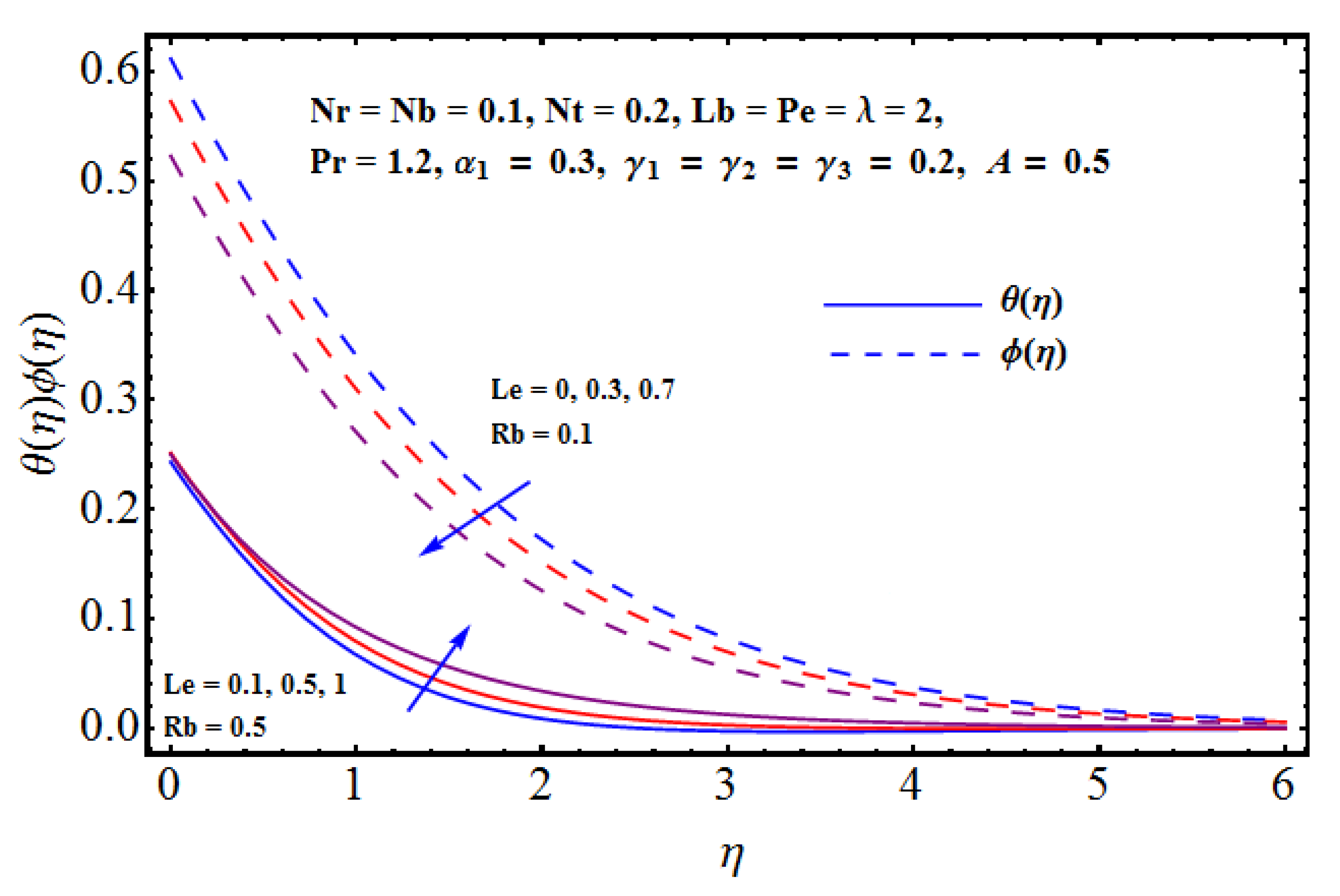
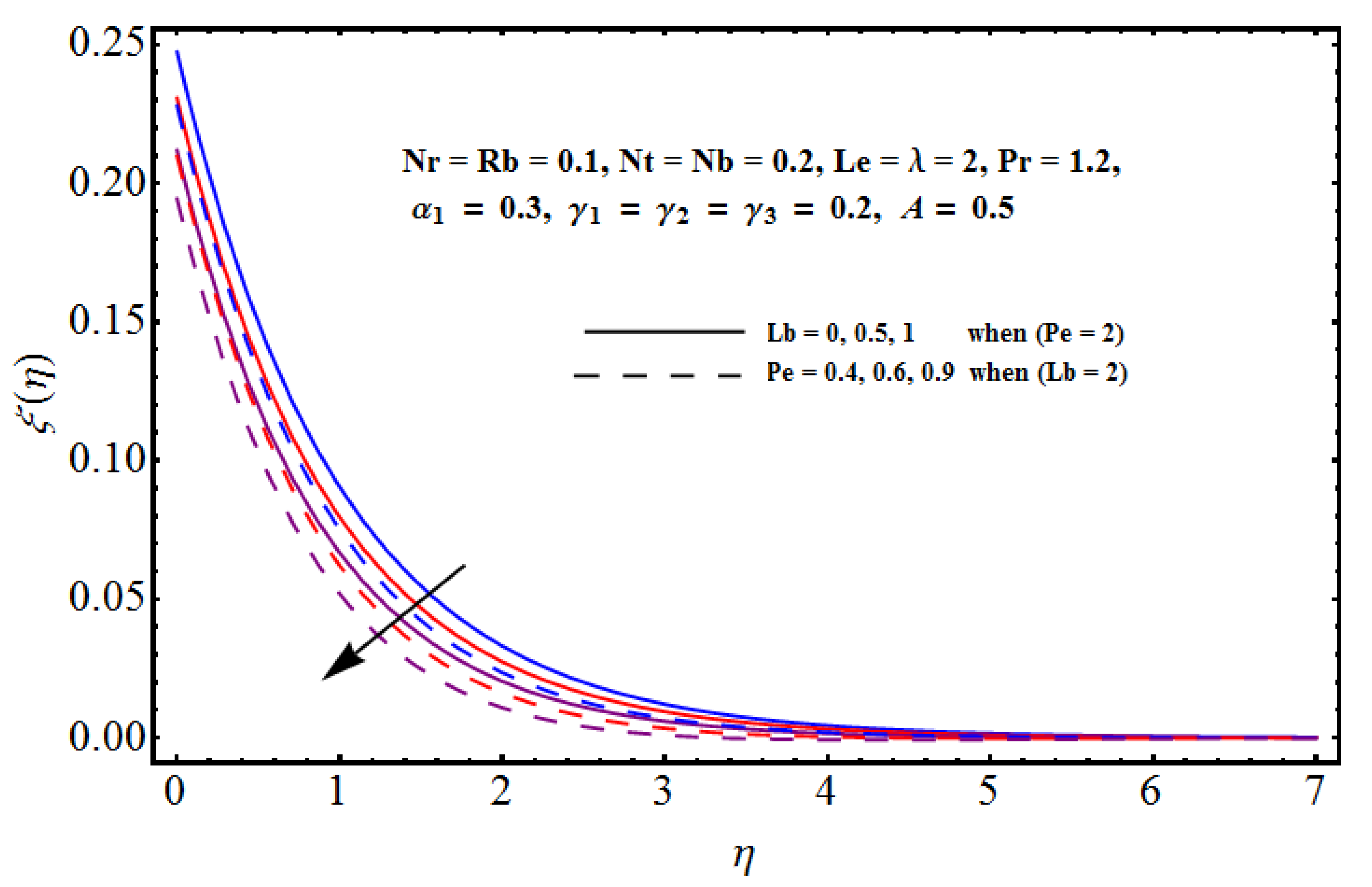
- Thermal distribution receives enhancement, whereas the decline is noticed in concentration distribution. Physically, the inverse relation between Brownian diffusion and Lewis factor is responsible for these variations.
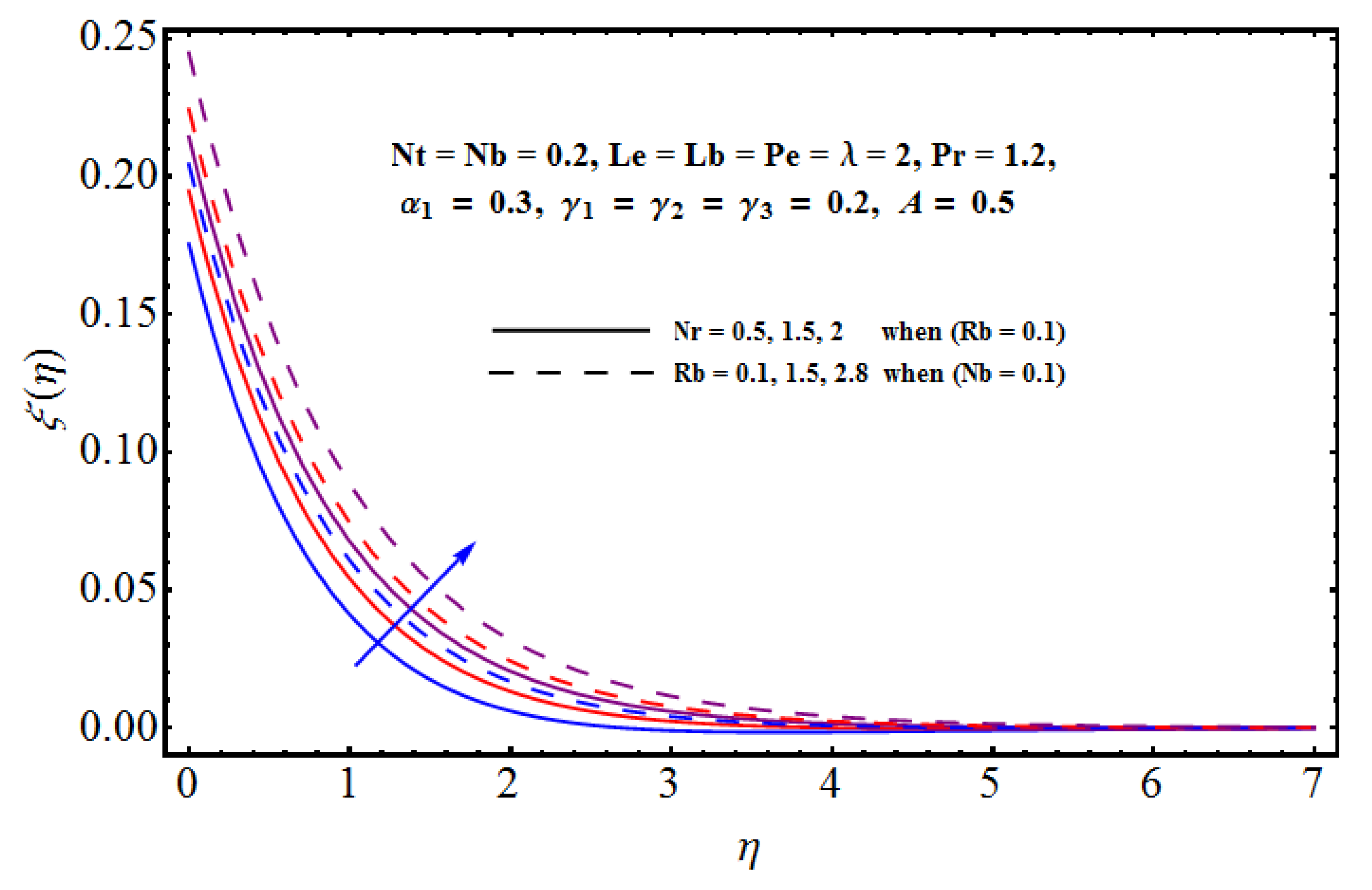
- The larger buoyancy force parameter augments the solute buoyancy force, therefore, the density increases. Further greater values of ) increase the buoyancy force because of the bio-convection process. This fact gives rise to the density of fluid.
- The motile density profile increases for larger for higher and intensive thermophoresis
- The motile density profile declines with the enhanced and . Higher values of and decrease the diffusivity of micro-organisms and, hence, the motile density of micro-organisms decreases.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Wang, L.; Fan, J. Nanofluids Research: Key Issue. Nanoscale Res. Lett. 2010, 5, 1241–1252. [Google Scholar] [CrossRef] [PubMed]
- Kuznetsov, A.V. The onset of nanofluid bioconvection in a suspension containing both nanoparticles and gyrotactic micro-organisms. Int. Commun. Heat Mass Trans. 2010, 37, 1421–1425. [Google Scholar] [CrossRef]
- Kuznetsov, A.V. Nanofluid bio-thermal convection: Simultaneous effects of gyrotactic and oxytactic micro-organisms. Fluid Dyn. Res. 2011, 43, 055505. [Google Scholar] [CrossRef]
- Aziz, A.; Khan, W.A.; Pop, I. Free convection boundary layer flow past a horizontal flat plate embedded in porous medium filled by nanofluid containing gyrotactic micro-organisms. Int. J. Therm. Sci. 2012, 56, 48–57. [Google Scholar] [CrossRef]
- Tham, L.; Nazar, R.; Pop, I. Mixed convection flow over a solid sphere embedded in a porous medium filled by a nanofluid containing gyrotactic micro-organisms. Int. J. Heat Mass Transf. 2013, 62, 647–660. [Google Scholar] [CrossRef]
- Xu, H.; Pop, I. Fully developed mixed convection flow in a horizontal channel filled by a nanofluid containing both nanoparticles and gyrotactic micro-organisms. Eur. J. Mech. B Fluids 2014, 46, 37–45. [Google Scholar] [CrossRef]
- Khan, W.A.; Uddin, M.J.; Ismail, A.I.M. Free convection of Non-Newtonian nanofluids in porous media with gyrotactic micro-organisms. Transp. Porous Med. 2013, 97, 241–252. [Google Scholar] [CrossRef]
- Mutuku, W.N.; Makinde, O.D. Hydromagnetic bioconvection of nanofluid over a permeable vertical plate due to gyrotactic micro-organisms. Comput. Fluids 2014, 95, 88–97. [Google Scholar] [CrossRef]
- Akbar, N.S. Bioconvection peristaltic flow in an asymmetric channel filled by nanofluid containing gyrotactic micro-organism: Bio nano engineering model. Int. J. Numer. Meth. Heat Fluid Flow 2015, 25, 214–224. [Google Scholar] [CrossRef]
- Mabood, F.; Khan, W.A.; Ismail, A.I.M. Analytical modelling of free convection of non-Newtonian nanofluids flow in porous media with gyrotactic micro-organisms using OHAM. AIP Conf. Proc. 2014, 1635, 131–137. [Google Scholar]
- Rasool, G.; Shafiq, A.; Khalique, C.M.; Zhang, T. Magnetohydrodynamic Darcy Forchheimer nanofluid flow over non-linear stretching sheet. Phys. Scr. 2019, 94, 105221. [Google Scholar] [CrossRef]
- Rasool, G.; Zhang, T.; Shafiq, A. Second grade nanofluidic flow past a convectively heated vertical Riga plate. Phys. Scr. 2019, 94, 125212. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Khan, I.; Raza, J.; Bakouri, M.; Tlili, I. Stability analysis of Darcy-Forchheimer flow of Casson type Nanofluid over an exponential sheet: Investigation of critical points. Symmetry 2019, 11, 412. [Google Scholar] [CrossRef]
- Rasool, G.; Zhang, T. Darcy-Forchheimer nanofluidic flow manifested with Cattaneo-Christov theory of heat and mass flux over non-linearly stretching surface. PLoS ONE 2019, 14, e0221302. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Khan, I.; Dero, S. Multiple solutions of Cu-C6 H9 NaO7 and Ag-C6 H9 NaO7 nanofluids flow over non-linear shrinking surface. J. Centr. South Univ. 2019, 26, 1283–1293. [Google Scholar] [CrossRef]
- Rasool, G.; Shafiq, A.; Tlili, I. Marangoni convective nano-fluid flow over an electromagnetic actuator in the presence of first order chemical reaction. Heat Transf.-Asian Res. 2019, 49, 274–289. [Google Scholar] [CrossRef]
- Rasool, G.; Shafiq, A.; Durur, H. Darcy-Forchheimer relation in Magnetohydrodynamic Jeffrey nanofluid flow over stretching surface. Discr. Contin. Dyn. Syst.-Ser. S 2019. accepted. [Google Scholar]
- Sohail, M.; Naz, R.; Abdelsalam, S.I. On the onset of entropy generation for a nanofluid with thermal radiation and gyrotactic micro-organisms through 3D flows. Phys. Scr. 2019. accepted. [Google Scholar]
- Shafiq, A.; Rasool, G.; Khalique, C.M. Significance of thermal slip and convective boundary conditions in three dimensional rotating Darcy-Forchheimer nanofluid flow. Symmetry 2020. accepted. [Google Scholar]
- Sohail, M.; Naz, R. Modified heat and mass transmission models in the magnetohydrodynamic flow of Sutterby nanofluid in stretching cylinder. Phys. A Stat. Mech. Its Appl. 2020, 124088. [Google Scholar] [CrossRef]
- Rasool, G.; Zhang, T. Characteristics of chemical reaction and convective boundary conditions in Powell-Eyring nanofluid flow along a radiative Riga plate. Heliyon 2019, 5, e01479. [Google Scholar] [CrossRef] [PubMed]
- Rasool, G.; Zhang, T.; Chamkha, A.J.; Shafiq, A.; Tlili, I.; Shahzadi, G. Entropy Generation and Consequences of Binary Chemical Reaction on MHD Darcy—Forchheimer Williamson Nanofluid Flow Over Non-Linearly Stretching Surface. Entropy 2020, 22, 18. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Ismael, M.; Kasaeipoor, A.; Armaghani, T. Entropy Generation and Natural Convection of CuO-Water Nanofluid in C-Shaped Cavity under Magnetic Field. Entropy 2016, 18, 50. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Khaled, A.-R.A. Similarity Solutions for Hydromagnetic Mixed Convection Heat and Mass Transfer for Hiemenz Flow Through Porous Media. Int. J. Numer. Methods Heat Fluid Flow 2000, 10, 94–115. [Google Scholar] [CrossRef]
- Rasool, G.; Shafiq, A.; Khan, I.; Baleanu, D.; Nisar, K.S.; Shahzadi, G. Entropy generation and consequences of MHD in Darcy-Forchheimer nanofluid flow bounded by non-linearly stretching surface. Symmetry 2020. accepted. [Google Scholar]
- Choi, S.U.S.; Eastman, J.A. Enhancing thermal conductivity of fluids with nanoparticles. ASME Publ.-Fed. 1995, 231, 99–106. [Google Scholar]
- Merkin, J.H. Natural convection boundary-layer flow on a vertical surface with Newtonian heating. Int. J. Heat Fluid Flow 1992, 15, 392–398. [Google Scholar] [CrossRef]
- Hayat, T.; Shafiq, A.; Mustafa, M.; Alsaedi, A. Boundary-layer flow of Walters’B fluid with Newtonian heating. Zeitschrift für Naturforschung A 2015, 70, 333–341. [Google Scholar] [CrossRef]
- Awais, M.; Hayat, T.; Nawaz, M.; Alsaedi, A. Newtonian heating, thermal diffusion and duffsion thermo effects in an axisymmetric flow of Jeffrey fluid over a stretching surface. Braz. J. Chem. Eng. 2015, 32, 555–561. [Google Scholar] [CrossRef][Green Version]
- Farooq, M.; Gull, N.; Alsaedi, A.; Hayat, T. MHD flow of a Jeffrey fluid with Newtonian heating. J. Mech. 2015, 31, 319–329. [Google Scholar] [CrossRef]
- Ramzan, M.; Farooq, M.; Alhothuali, M.S.; Malaikh, H.M.; Hayat, W.C.T. Three dimensional flow of an Oldroyd-B fluid with Newtonian Heating. Int. J. Numer. Meth. Heat Fluid Flow 2015, 25, 68–85. [Google Scholar] [CrossRef]
- Hayat, T.; Ali, S.; Awais, M.; Alhothuali, M.S. Newtonian heating in stagnation point flow of Burgers fluid. Appl. Math. Mech. 2015, 36, 61–68. [Google Scholar] [CrossRef]
- Kuznetsov, A.V.; Avramenko, A.A. Effect of small particles on the stability of bioconvection in a suspension of gyrotactic micro-organisms in a layer of finite depth. Int. Commun. Heat Mass Transf. 2014, 31, 1–10. [Google Scholar] [CrossRef]
- Geng, P.; Kuznetsov, A.V. Effect of small solid particles on the development of bioconvection plumes. Int. Commun. Heat Mass Transf. 2004, 31, 629–638. [Google Scholar] [CrossRef]
- Kuznetsov, A.V.; Geng, P. The interaction of bioconvection caused by gyrotactic micro-organisms and settling of small solid particles. Int. J. Numer. Methods Heat Fluid Flow 2005, 15, 328–347. [Google Scholar] [CrossRef]
- Uddin, M.J.; Khan, W.A.; Qureshi, S.R.; Beg, O.A. Bioconvection nanofluid slip flow past a wavy surface with applications in nano-biofuel cells. Chin. J. Phys. 2017, 55, 2048–2063. [Google Scholar] [CrossRef]
- Kuznetsov, A.V. Nanofluid bioconvection in water-based suspensions containing nanoparticles and oxytactic micro-organisms: Oscillatory instability. Nanoscale Res. Lett. 2011, 6, 100. [Google Scholar] [CrossRef]
- Waqas, H.; Khan, S.U.; Hassan, M.; Bhatti, M.M.; Imran, M. Analysis on the bioconvection flow of modified second-grade nanofluid containing gyrotactic micro-organisms and nanoparticles. J. Mol. Liq. 2019, 291, 111231. [Google Scholar] [CrossRef]
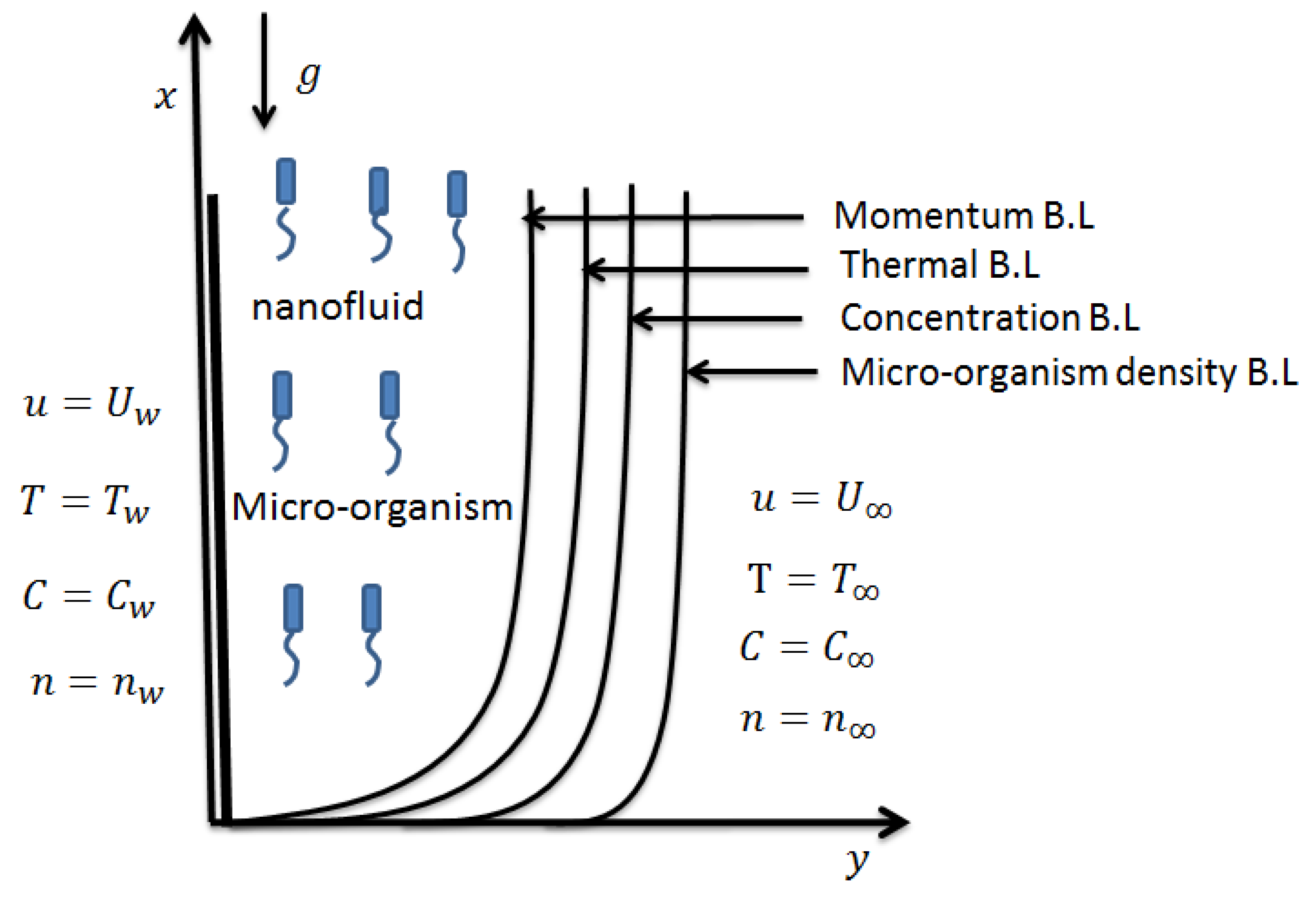
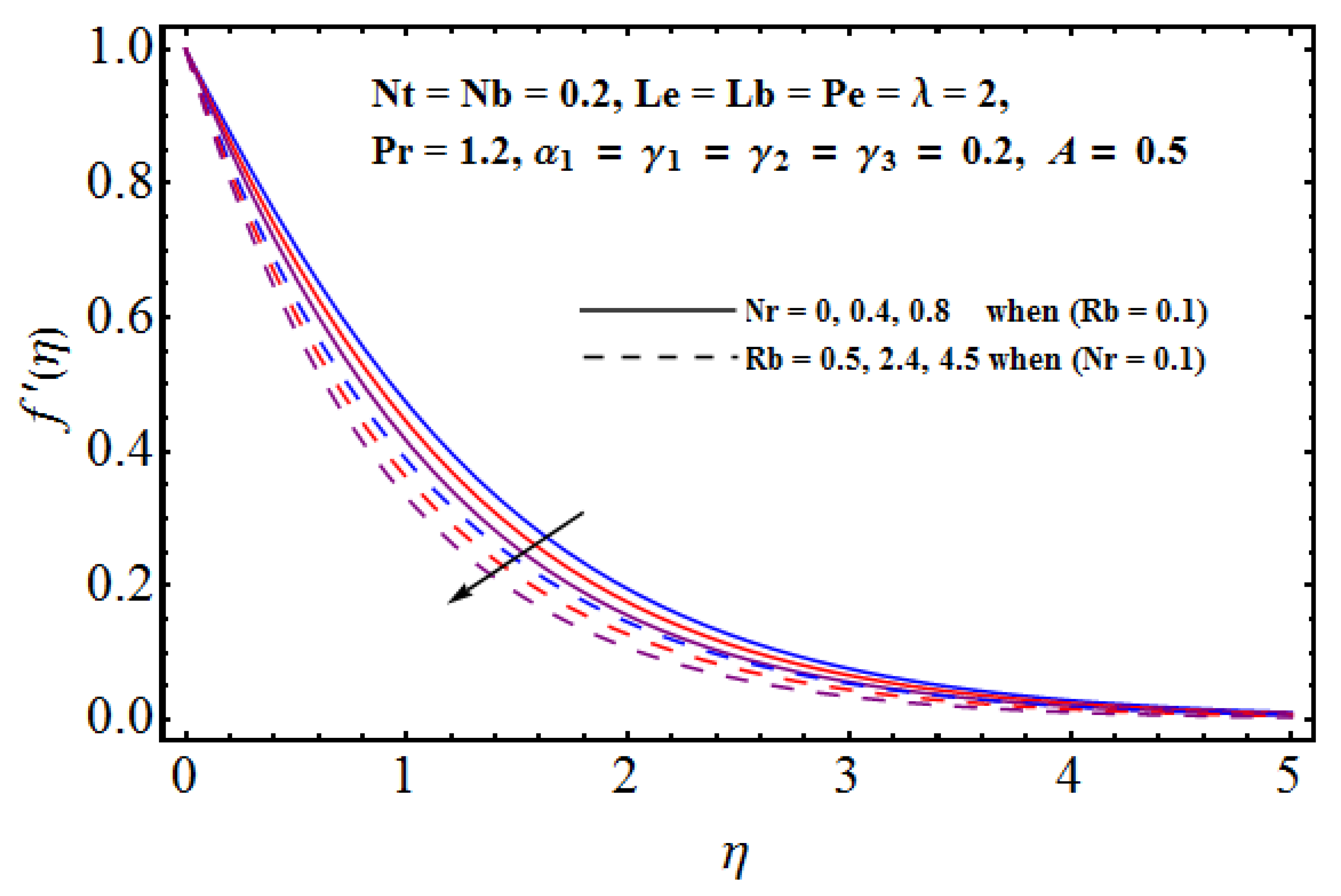
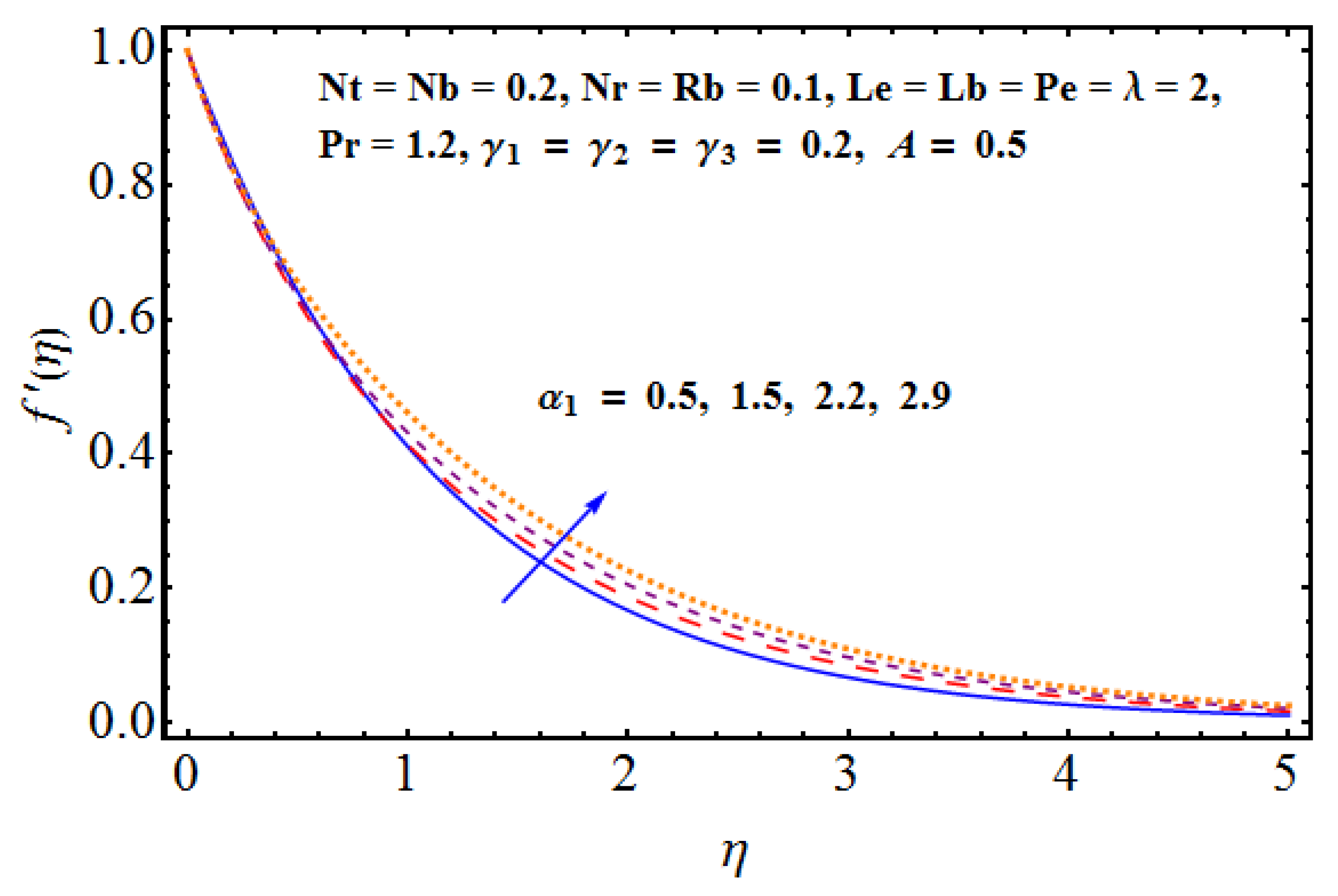
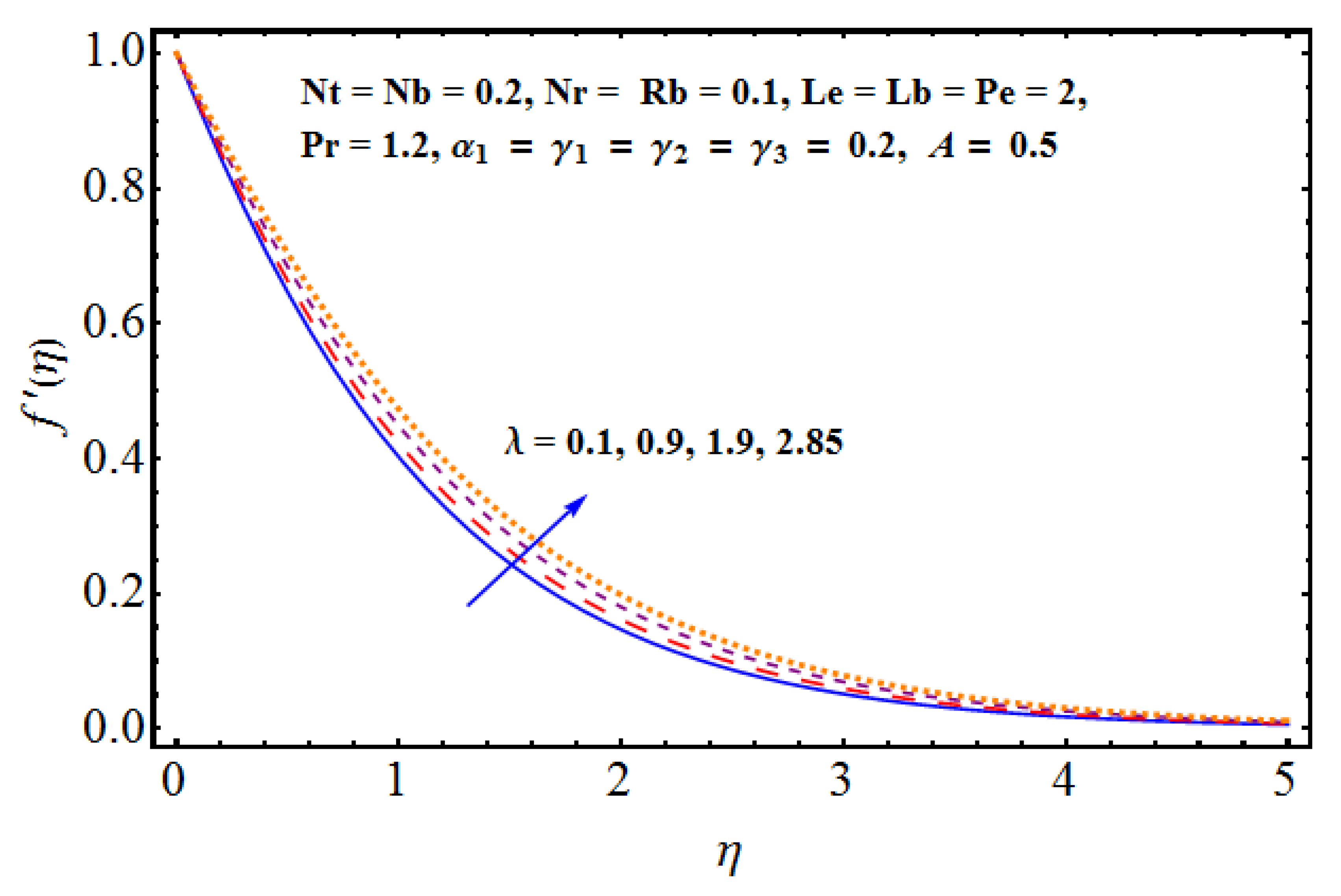



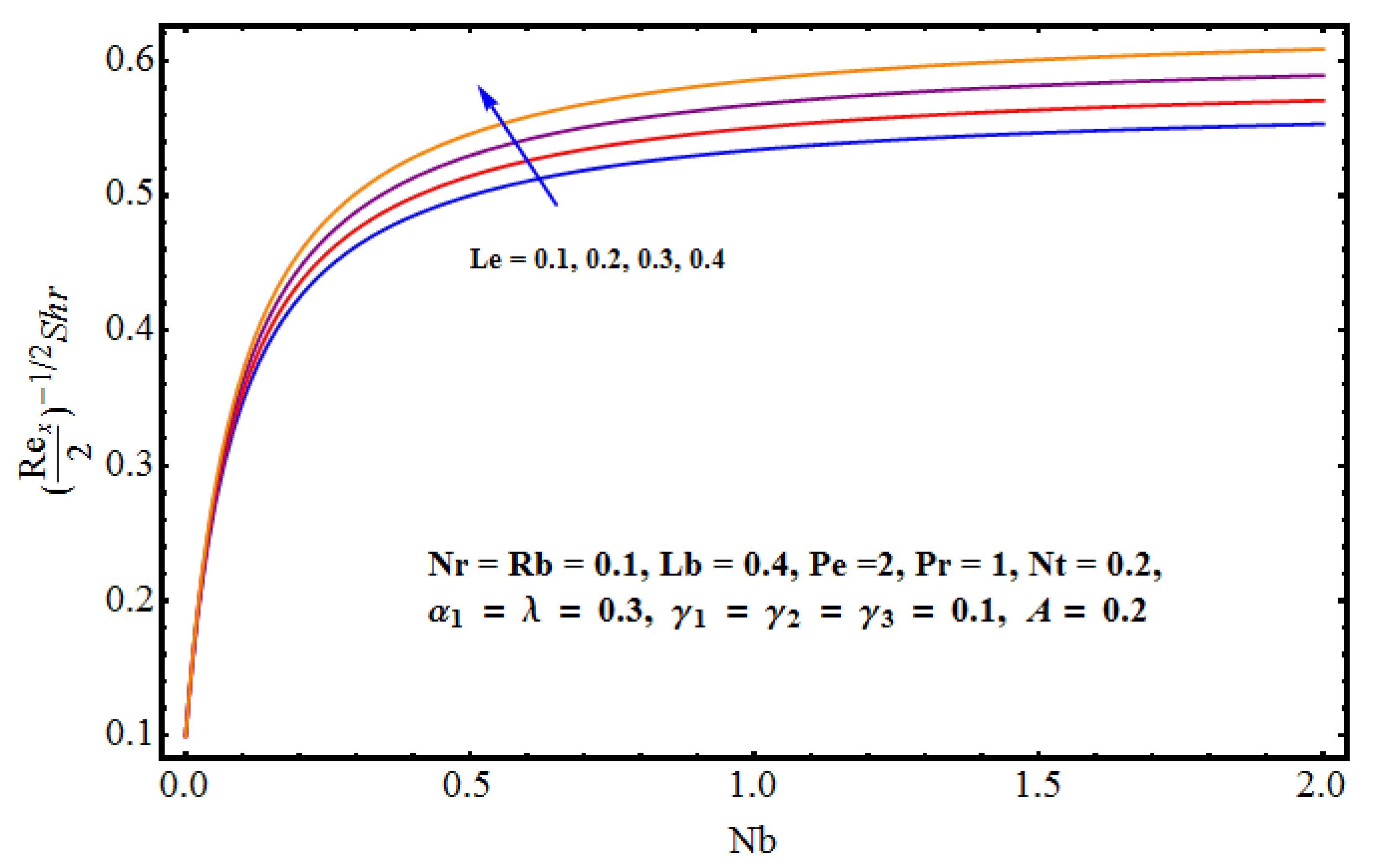
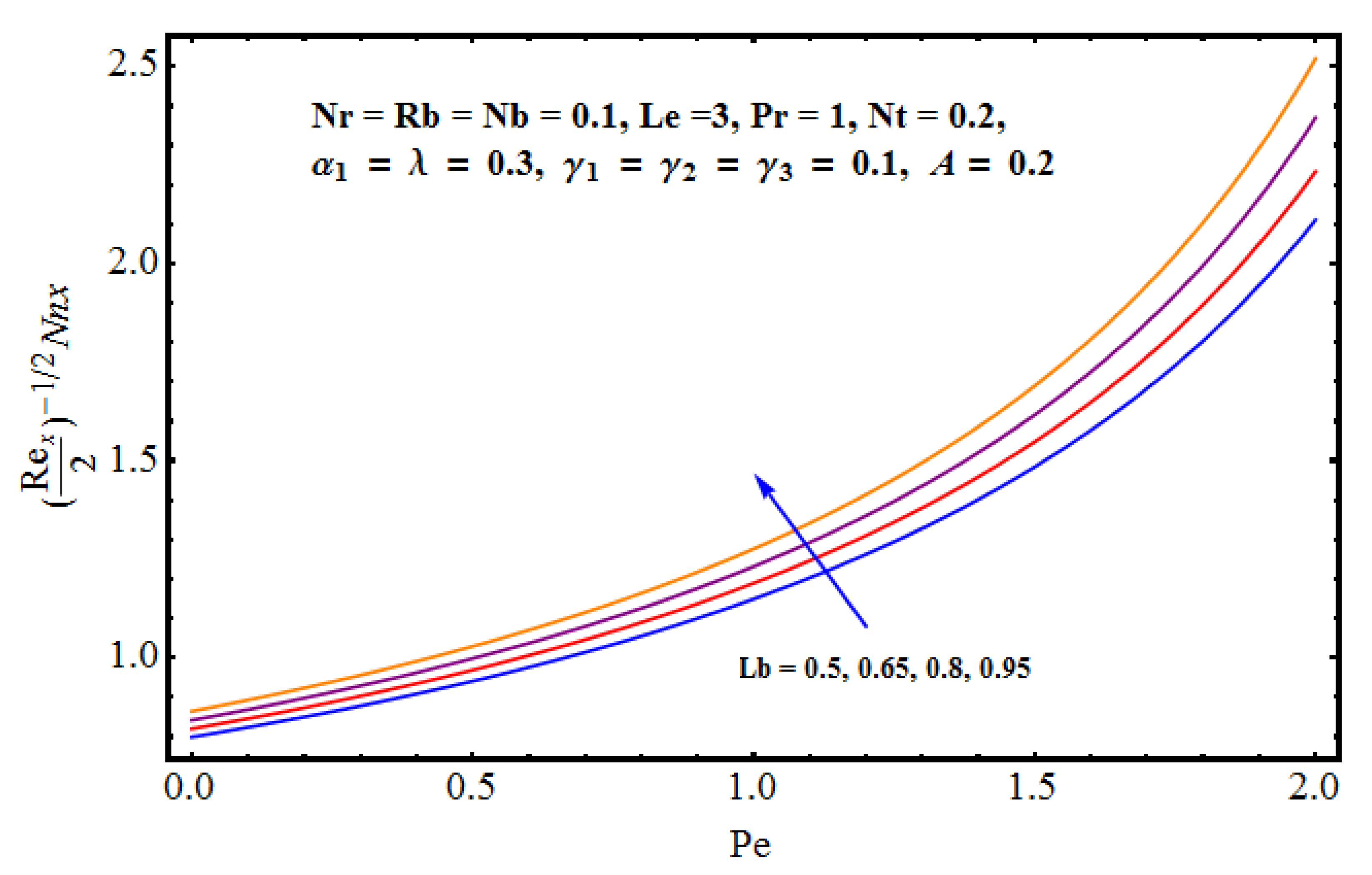
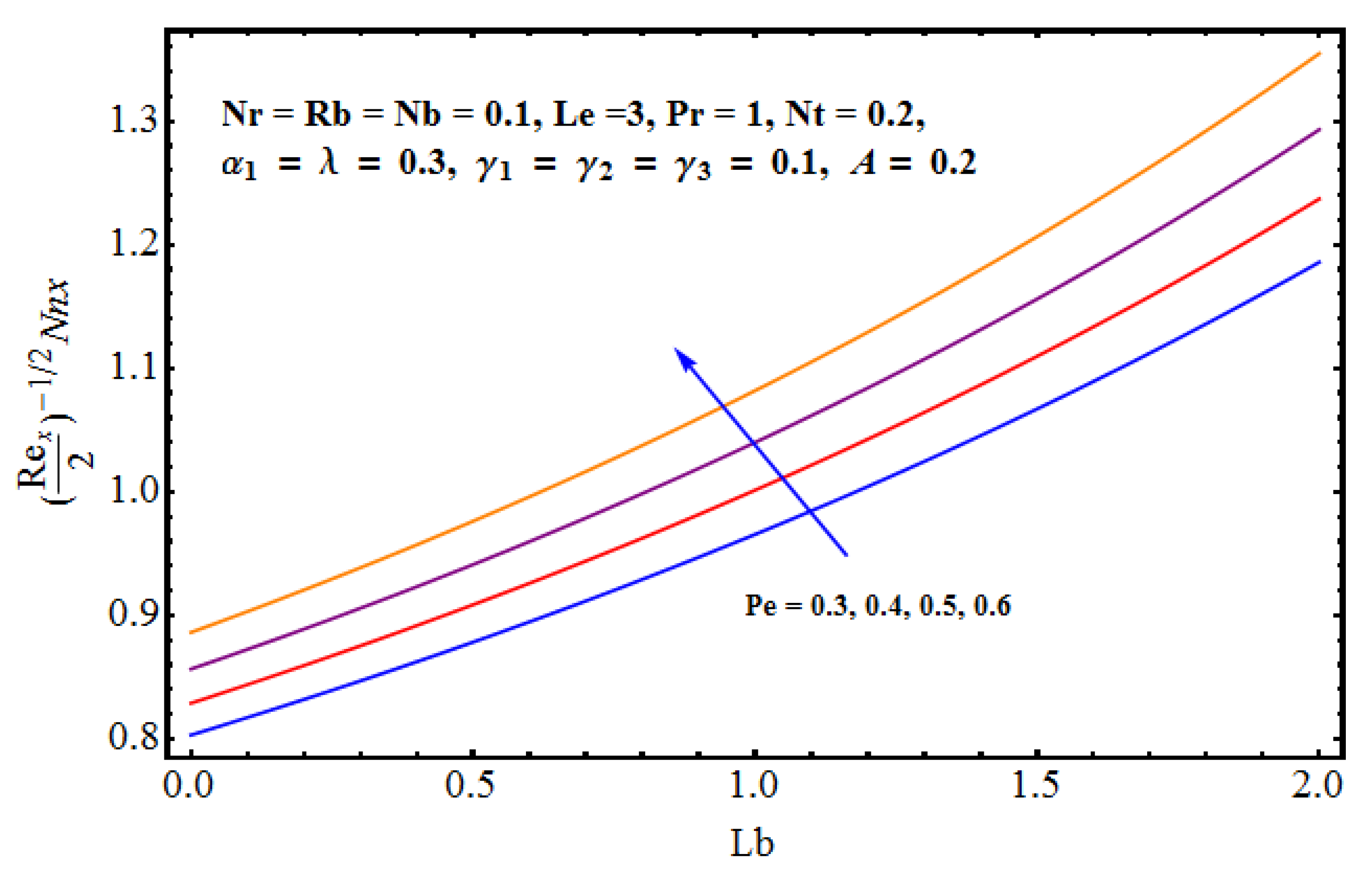

| Nr | Rb | Nb | λ | α1 | Nt | Le | |
|---|---|---|---|---|---|---|---|
| 0.1 | 0.1 | 0.1 | 0.5 | 0.3 | 0.1 | 2 | 1.2040 |
| 0.2 | 1.2118 | ||||||
| 0.3 | 1.2196 | ||||||
| 0.1 | 0.2 | 0.1 | 0.5 | 0.3 | 0.1 | 2 | 1.1937 |
| 0.3 | 1.1835 | ||||||
| 0.4 | 1.1785 | ||||||
| 0.1 | 0.1 | 0.12 | 0.5 | 0.3 | 0.1 | 2 | 1.2126 |
| 0.13 | 1.2123 | ||||||
| 0.14 | 1.2119 | ||||||
| 0.1 | 0.1 | 0.1 | 0.6 | 0.3 | 0.1 | 2 | 1.1877 |
| 0.65 | 1.1796 | ||||||
| 0.7 | 1.1715 | ||||||
| 0.1 | 0.1 | 0.1 | 0.5 | 0.35 | 0.1 | 2 | 1.3665 |
| 0.4 | 1.5360 | ||||||
| 0.45 | 1.7117 | ||||||
| 0.1 | 0.1 | 0.1 | 0.5 | 0.3 | 0.15 | 2 | 1.2046 |
| 0.2 | 1.2054 | ||||||
| 0.3 | 1.2068 | ||||||
| 0.1 | 0.1 | 0.1 | 0.5 | 0.3 | 0.1 | 2.5 | 1.2031 |
| 3 | 1.2027 | ||||||
| 3.5 | 1.2003 |
| Pr | Nb | Nt | λ | Le | |
|---|---|---|---|---|---|
| 1 | 0.1 | 0.1 | 0.3 | 2 | 0.7617 |
| 1.5 | 1.003 | ||||
| 1.7 | 1.090 | ||||
| 1 | 0.2 | 0.1 | 0.3 | 2 | 0.7575 |
| 0.3 | 0.7533 | ||||
| 0.4 | 0.7491 | ||||
| 1 | 0.1 | 0.2 | 0.3 | 2 | 0.7545 |
| 0.3 | 0.7473 | ||||
| 0.4 | 0.7401 | ||||
| 1 | 0.1 | 0.1 | 0.4 | 2 | 0.7630 |
| 0.5 | 0.7641 | ||||
| 0.6 | 0.7653 | ||||
| 1 | 0.1 | 0.1 | 0.3 | 2.5 | 0.7623 |
| 3 | 0.7628 | ||||
| 3.5 | 0.7632 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shafiq, A.; Rasool, G.; Khalique, C.M.; Aslam, S. Second Grade Bioconvective Nanofluid Flow with Buoyancy Effect and Chemical Reaction. Symmetry 2020, 12, 621. https://doi.org/10.3390/sym12040621
Shafiq A, Rasool G, Khalique CM, Aslam S. Second Grade Bioconvective Nanofluid Flow with Buoyancy Effect and Chemical Reaction. Symmetry. 2020; 12(4):621. https://doi.org/10.3390/sym12040621
Chicago/Turabian StyleShafiq, Anum, Ghulam Rasool, Chaudry Masood Khalique, and Sohail Aslam. 2020. "Second Grade Bioconvective Nanofluid Flow with Buoyancy Effect and Chemical Reaction" Symmetry 12, no. 4: 621. https://doi.org/10.3390/sym12040621
APA StyleShafiq, A., Rasool, G., Khalique, C. M., & Aslam, S. (2020). Second Grade Bioconvective Nanofluid Flow with Buoyancy Effect and Chemical Reaction. Symmetry, 12(4), 621. https://doi.org/10.3390/sym12040621








