Numerical Study of Bubble Behavior under Gradient Flows during Subcooled Flow Boiling in Vertical Flow Channel
Abstract
1. Introduction
2. Geometrical Configuration
3. Mathematical Formulation
3.1. Governing Equations
3.2. Numerical Method
4. Numerical Results and Discussions
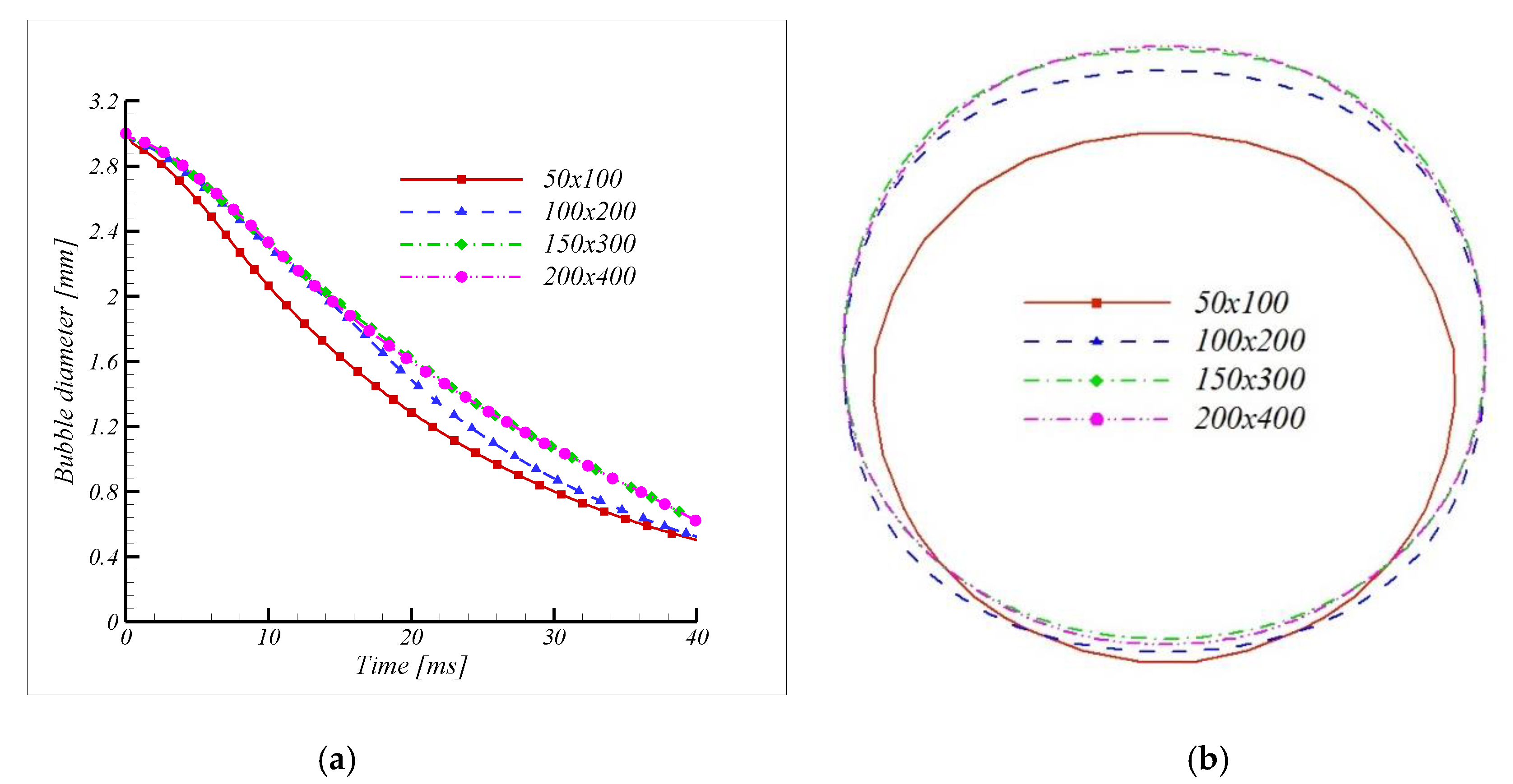
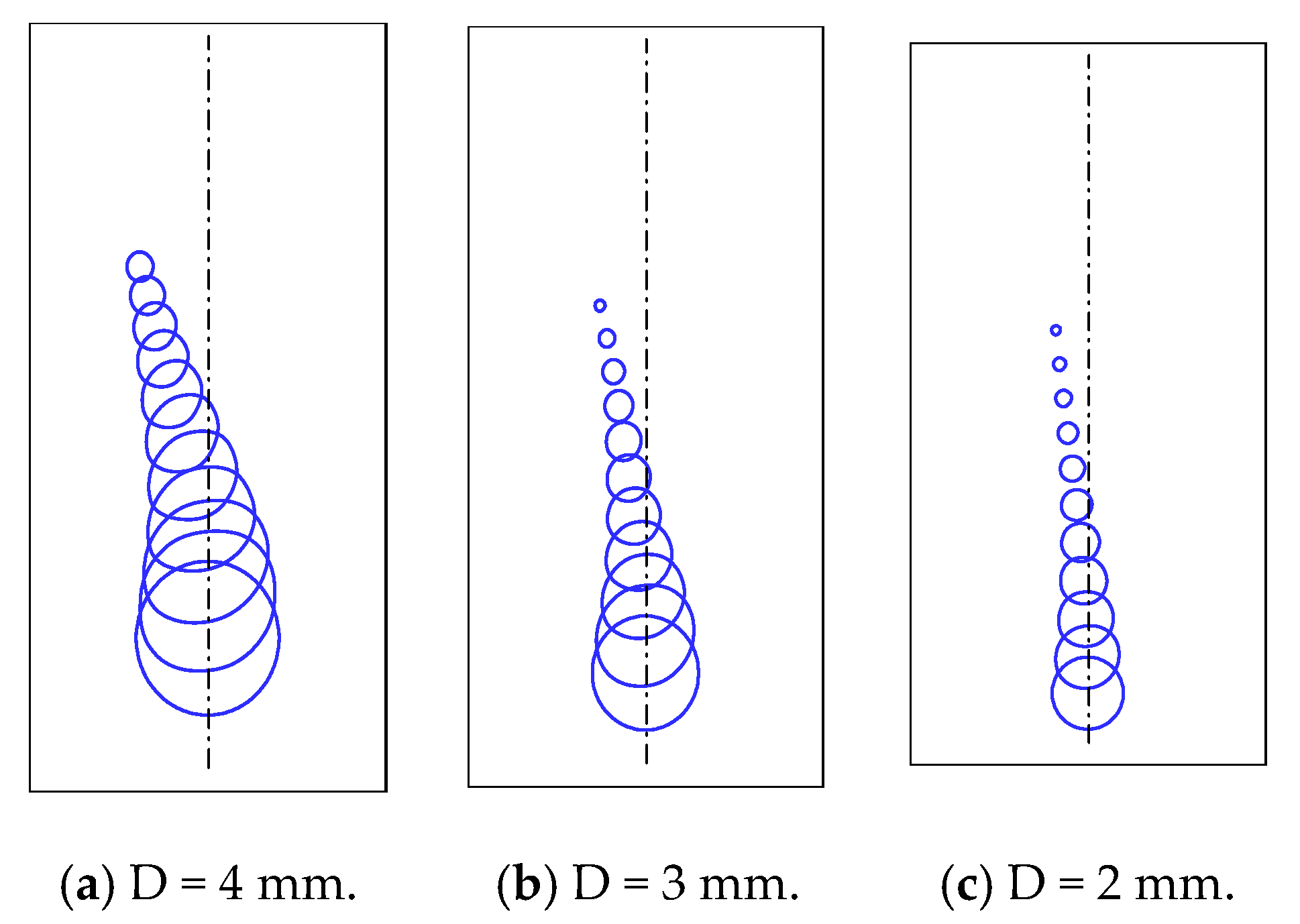
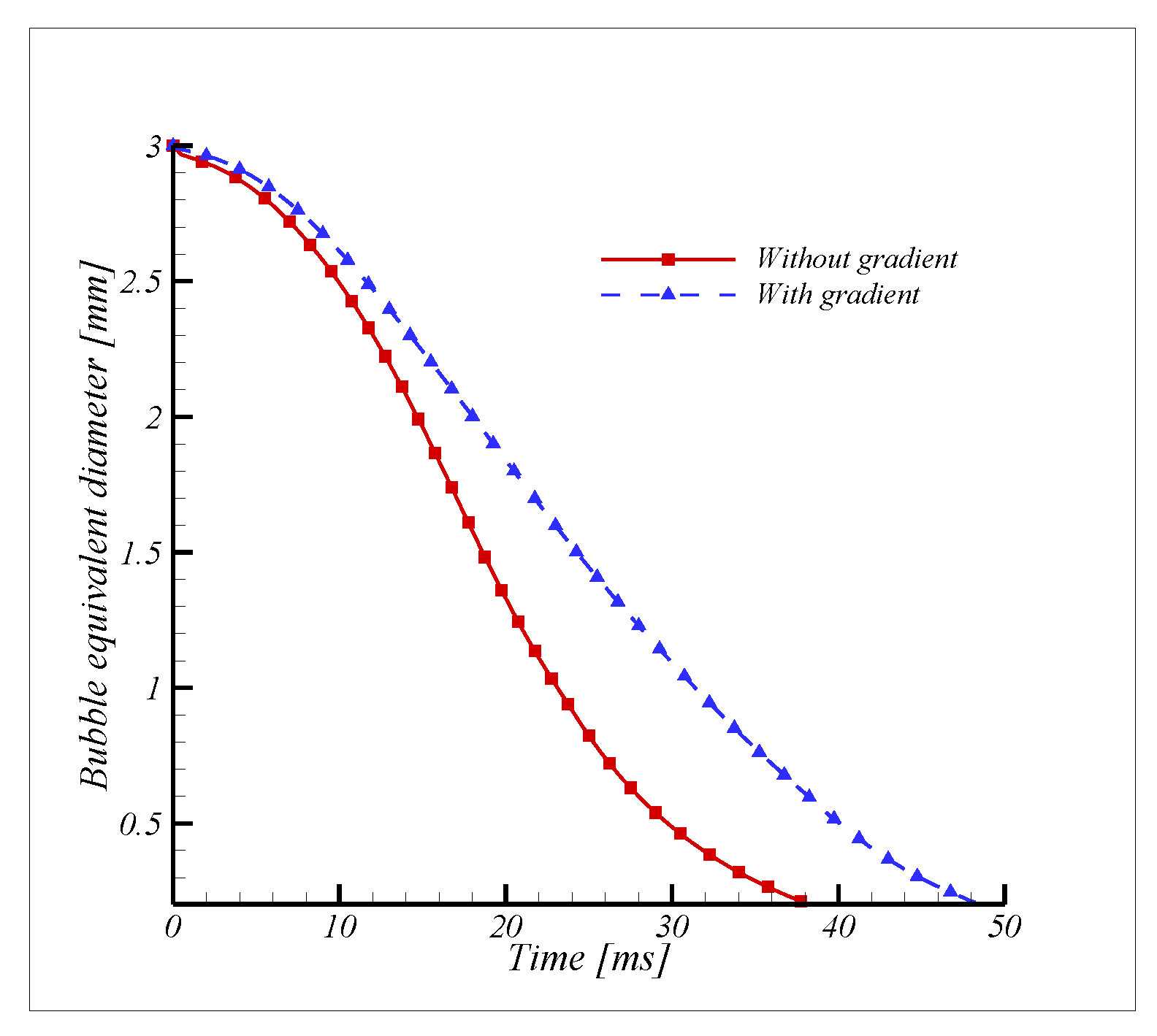
4.1. Geometrical Model Validation
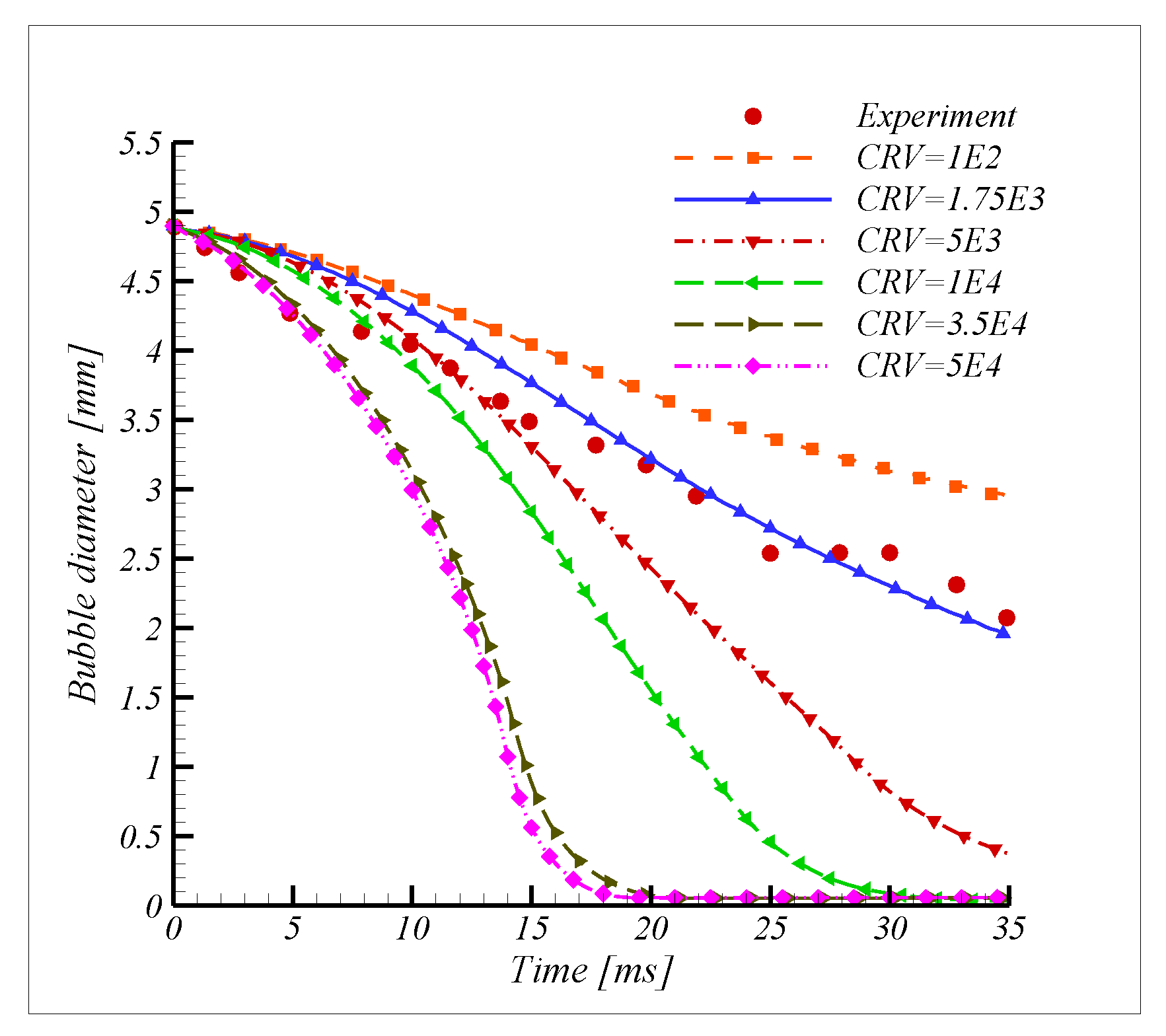
4.2. Mass Transfer Coefficient
4.3. Single-Bubble Rising Behavior
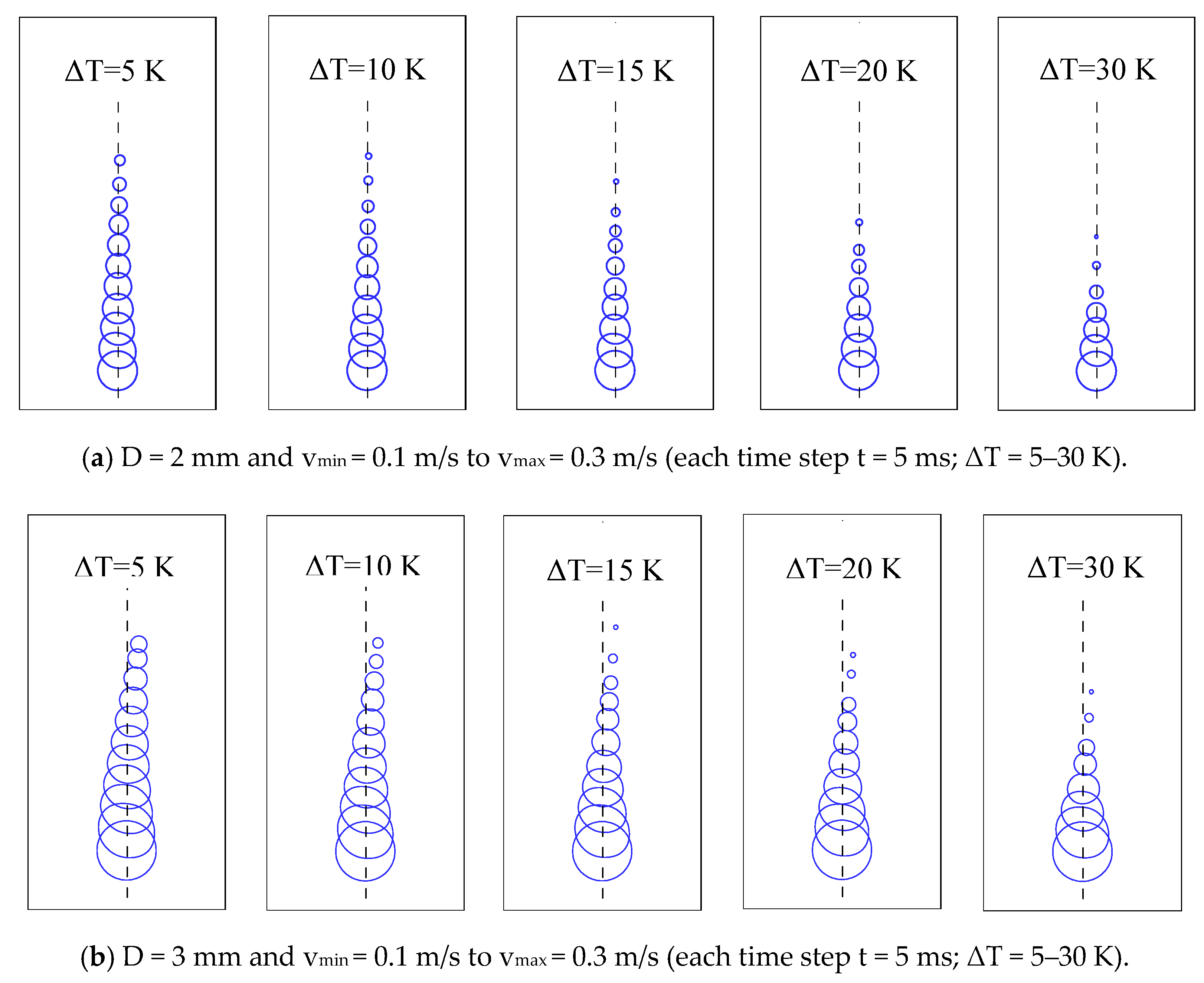
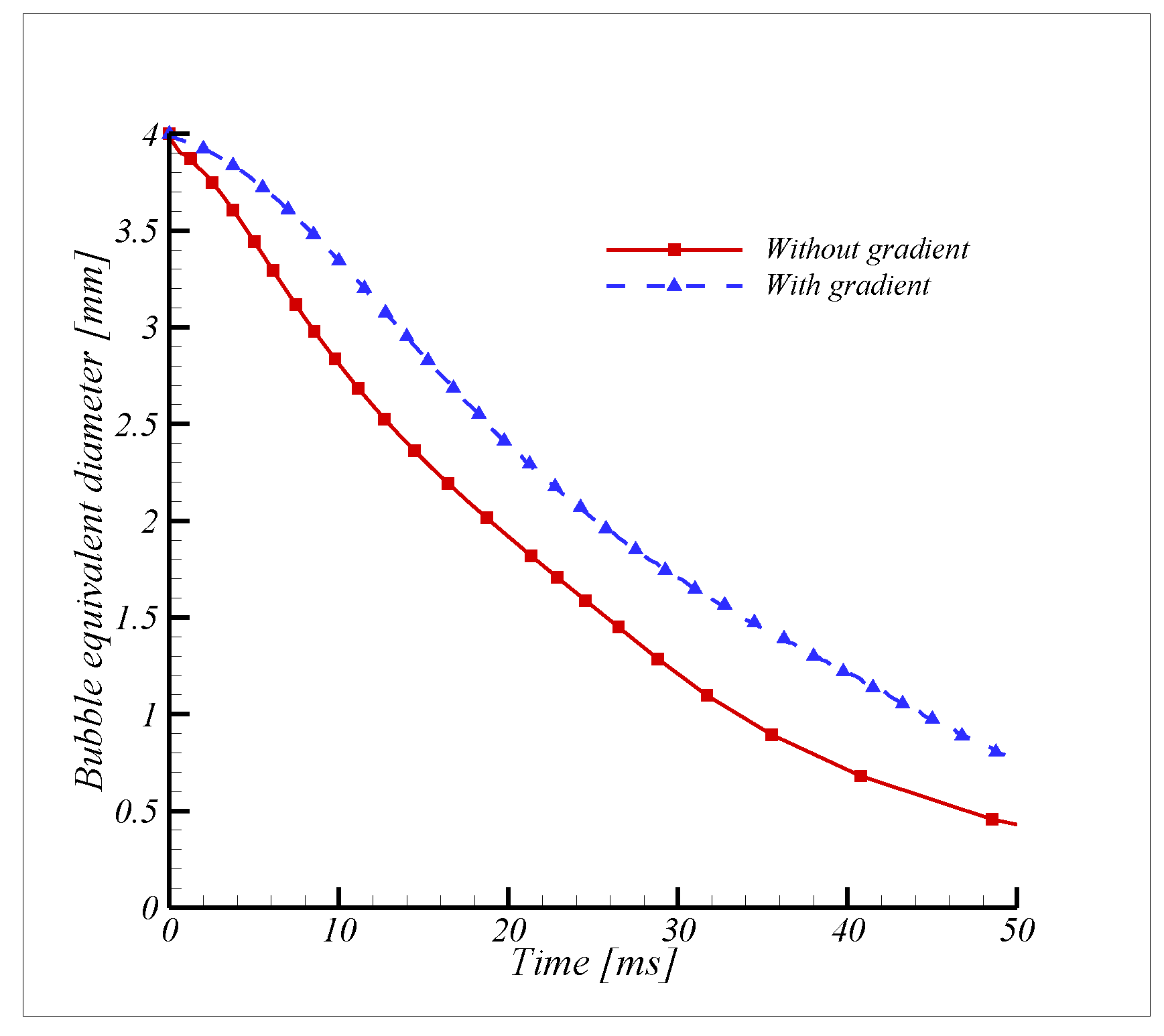
4.3.1. Velocity Gradient Flow
4.3.2. Temperature Gradient Flow
4.3.3. Velocity and Temperature Gradient Flow
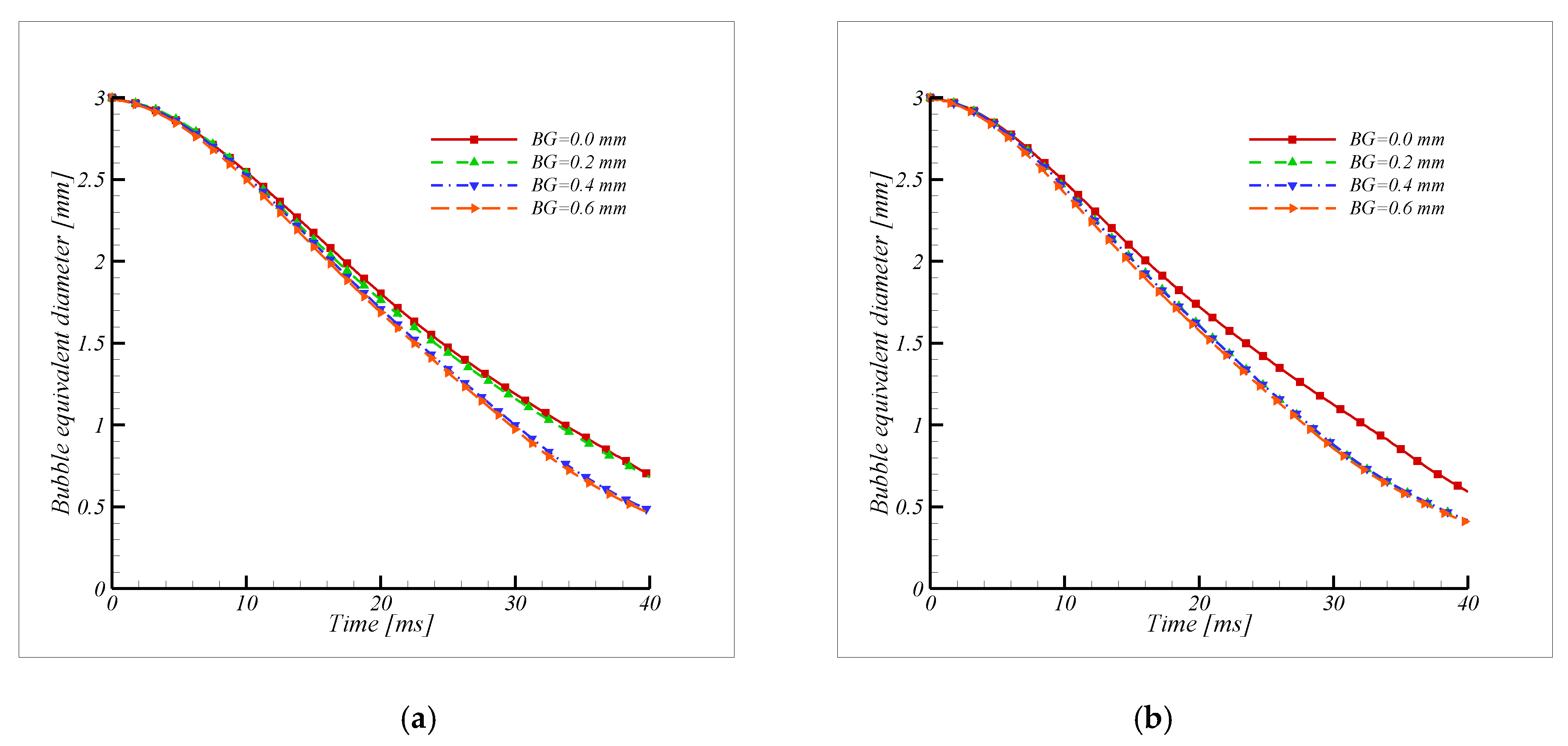
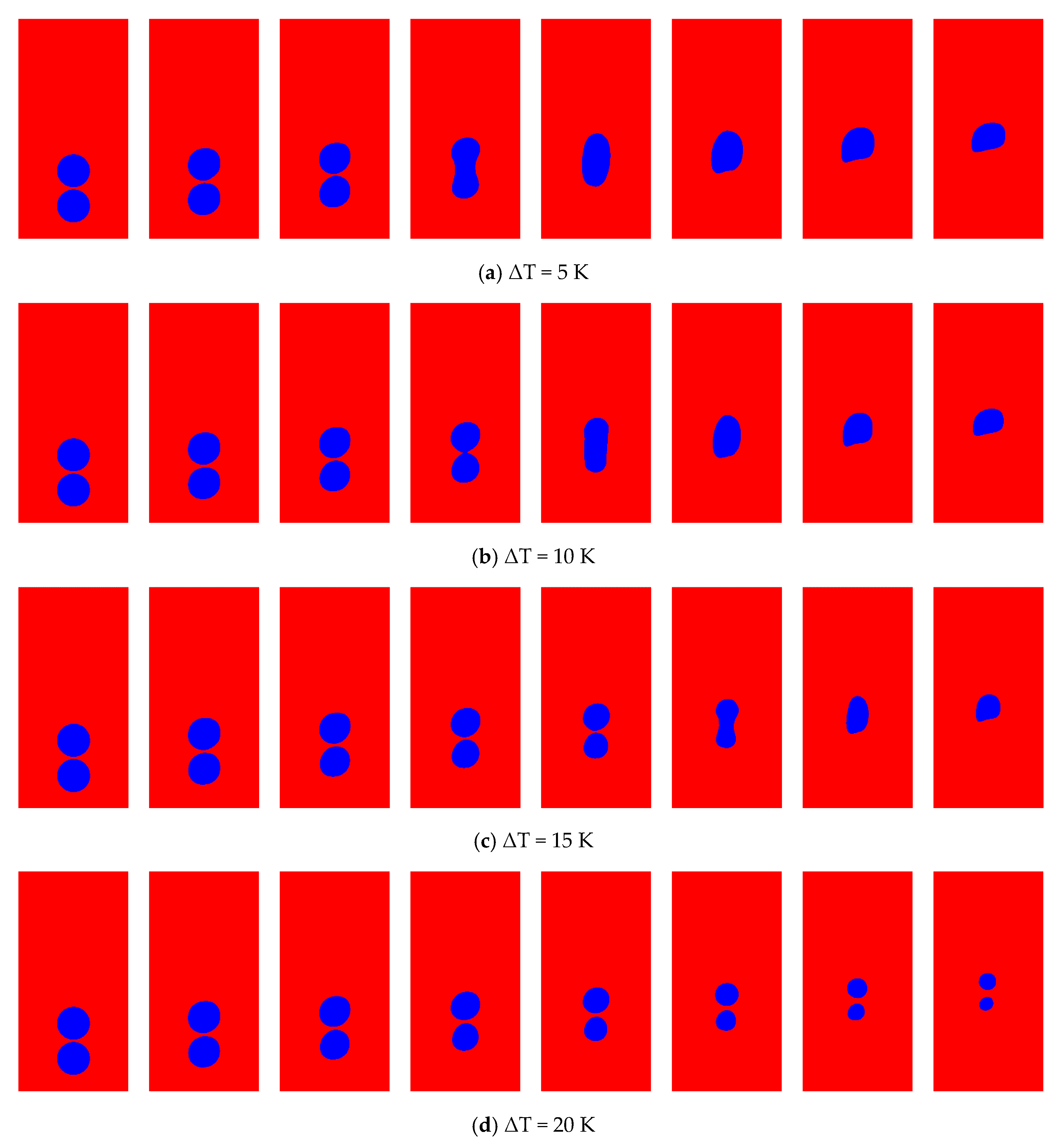
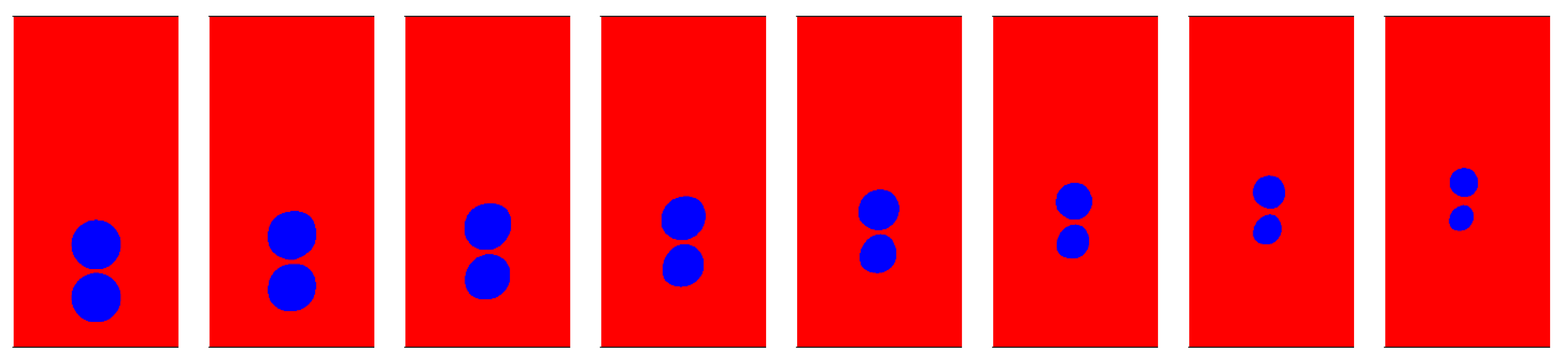
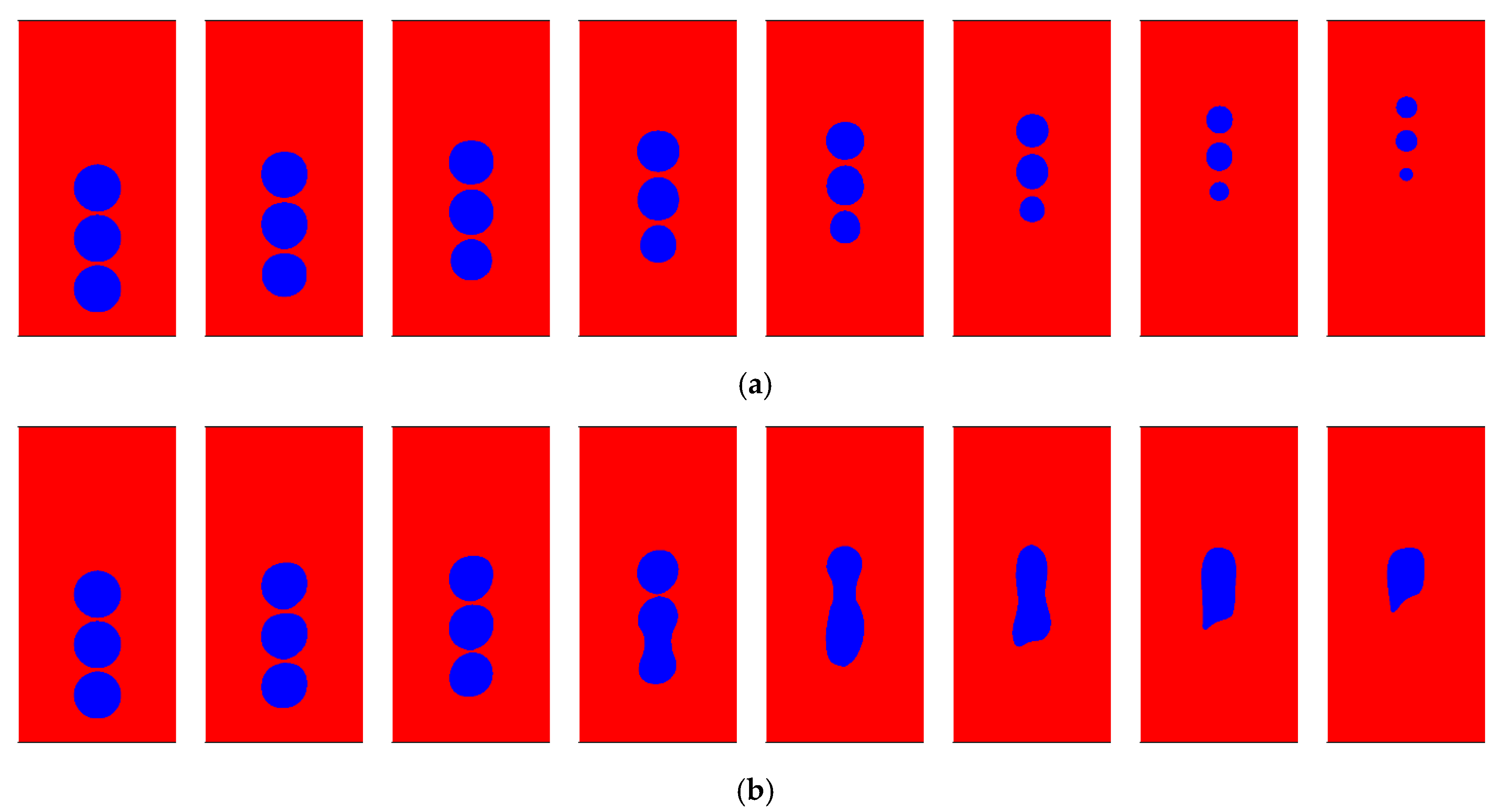
4.4. Multi-Bubble Rising Behavior in the Gradient Flow
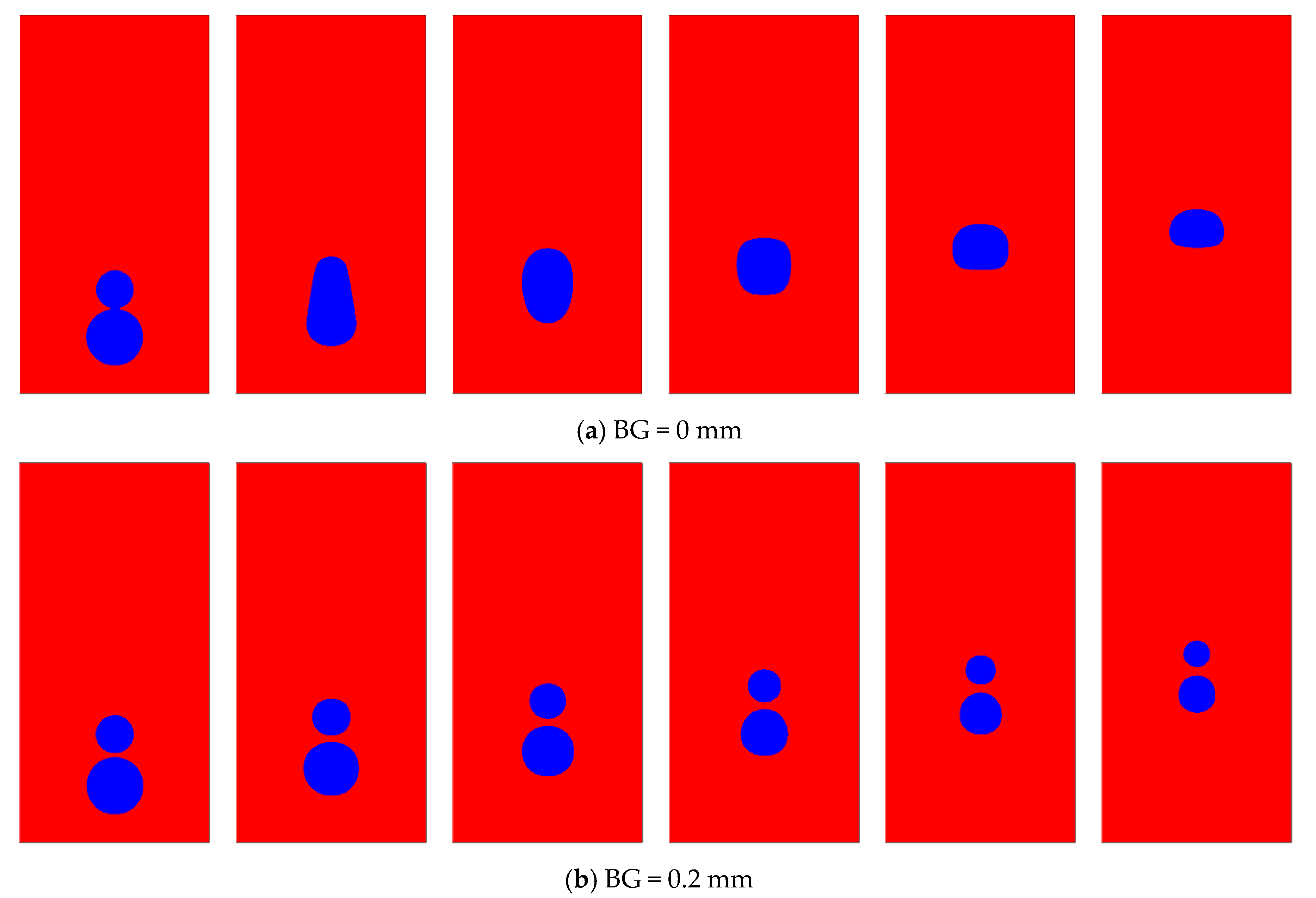
4.4.1. Two-Bubbles Rising Behavior
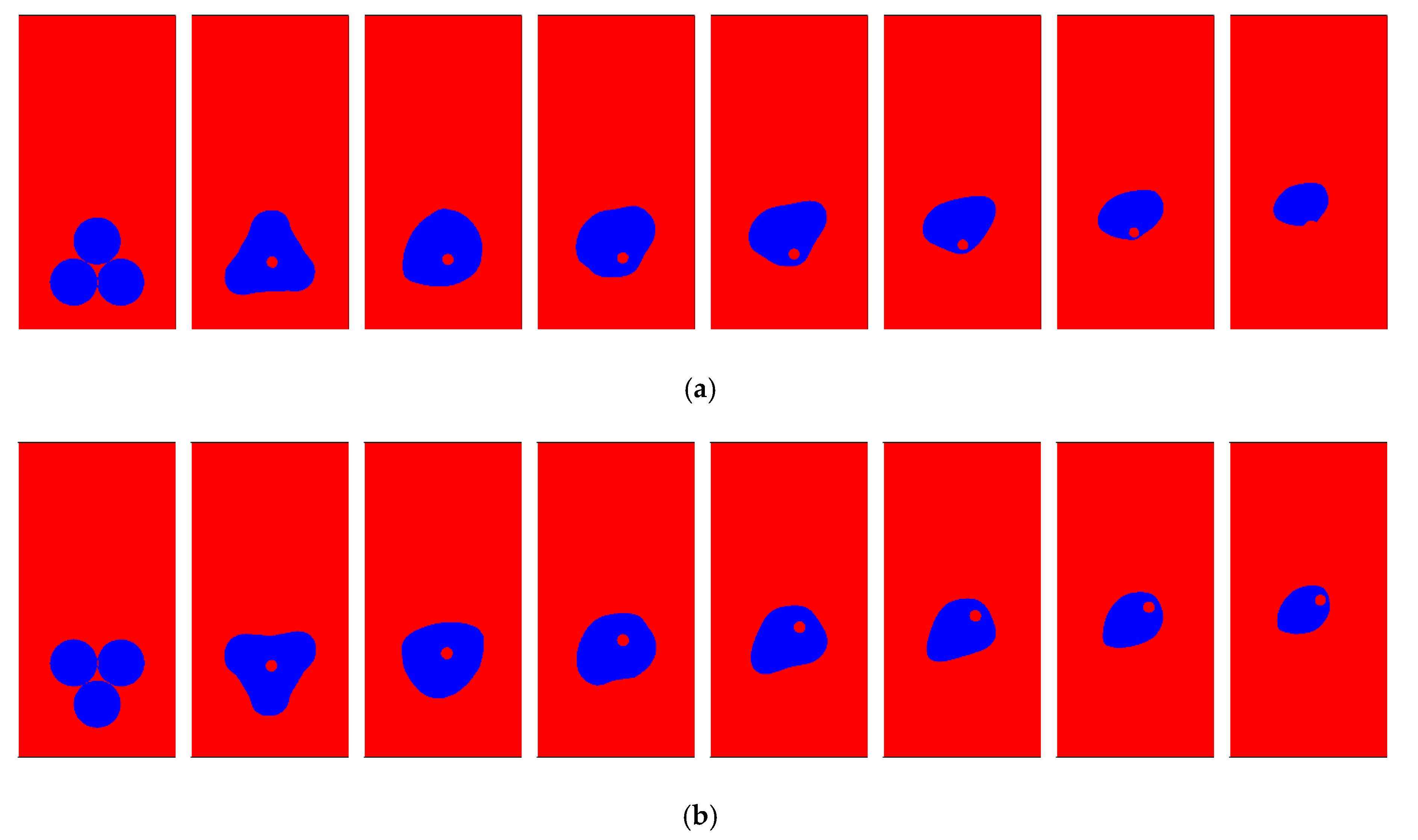
4.4.2. Three-Bubbles Rising Behavior
5. Conclusions
- When the bubble moved in the velocity gradient, it migrated toward the lower velocity side, thereby decreasing the bubble diameter and reducing the sinuous path. Similarly, in the temperature gradient, the bubble moved toward the lower temperature side and increased the condensation on one side of the bubble. In the case of velocity and temperature gradient flow, the bubble initially moved toward the higher velocity side; however, it again transformed and moved to the lower velocity and higher temperature side.
- A similar shape and size bubble merger occurred when the gap in the bubble was sufficiently small (BG = 0.2 mm). This occurred up to a certain subcooled temperature; however, it was interrupted beyond the subcooled temperature ΔT = 15 K.
- Compared to the three bubbles in a row condensation, the case without gradient flow condensation, the case with velocity and temperature gradient flow condensation affects the life of the bubble’s condensation, and this case causes the bubble merging process.
- The bubble pattern affects the bubble shapes and condensation rates during the bubble condensation because of the attraction with the neighboring bubble. From these cases, the bubbles heat and mass transfer depend on the number of bubbles within a particular BG and subcooled temperature in the defined shape and random arrangements of bubble flow inside the bubble column.
- When bubbles move vertically along the rising line, bubble coalescence did not occur because the lowest bubble condensed faster than the upper bubble due to the local perturbation were observed.
Author Contributions
Funding
Conflicts of Interest
References
- Zuber, N. The dynamics of vapor bubbles in nonuniform temperature fields. Int. J. Heat Mass Transf. 1960, 2, 83–98. [Google Scholar] [CrossRef]
- Okhotsimskii, A.D. The thermal regime of vapour bubble collapse at different Jacob numbers. Int. J. Heat Mass Transf. 1988, 31, 1569–1576. [Google Scholar] [CrossRef]
- Lucasa, D.; Prasserb, H.M. Steam Bubble Condensation in Sub-Cooled Water in Case of Co-Current Vertical Pipe Flow. Nucl. Eng. Des. 2007, 237, 497–508. [Google Scholar] [CrossRef]
- Brucker, G.G.; Sparrow, E.M. Direct contact condensation of steam bubbles in water at high pressure. Int. J. Heat Mass Transf. 1977, 20, 371–381. [Google Scholar] [CrossRef]
- Eames, I. Momentum Conservation and Condensing Vapor Bubbles. ASME J. Heat Transf. 2010, 132, 091501. [Google Scholar] [CrossRef]
- Kim, S.J.; Park, G.C. Interfacial heat transfer of condensing bubble in subcooled boiling flow at low pressure. Int. J. Heat Mass Transf. 2011, 54, 2962–2974. [Google Scholar] [CrossRef]
- Harada, T.; Nagakura, H.; Okawa, T. Dependence of bubble behavior in subcooled boiling on surface wettability. Nucl. Eng. Des. 2010, 240, 3949–3955. [Google Scholar] [CrossRef]
- Kamei, S.; Hirata, M. Condensing Phenomena of a Single Vapor Bubble into Subcooled Water. Exp. Heat Transf. 1990, 3, 173–182. [Google Scholar] [CrossRef]
- Tian, W.; Ishiwatari, Y.; Ikejiri, S.; Yamakawa, M.; Oka, Y. Numerical computation of thermally controlled steam bubble condensation using Moving Particle Semi-implicit (MPS) method. Ann. Nucl. Energy 2010, 37, 5–15. [Google Scholar] [CrossRef]
- Chena, R.H.; Wenxi, T.; Su, G.H.; Suizheng, Q.; Yuki, I.; Yoshiaki, O. Numerical investigation on bubble dynamics during flow boiling using moving particle semi-implicit method. Nucl. Eng. Des. 2010, 240, 3830–3840. [Google Scholar] [CrossRef]
- Ganguli, A.A.; Pandit, B.A.; Joshi, B.J. Bubble Dynamics of a Single Condensing Vapor Bubble from Vertically Heated Wall in Subcooled Pool Boiling System: Experimental Measurements and CFD Simulations. Int. J. Chem. Eng. 2012, 2012, 1–11. [Google Scholar] [CrossRef]
- Bahreini, M.; Ramiar, A.; Ranjbar, A.A. Numerical simulation of bubble behavior in subcooled flow boiling under velocity and temperature gradient. Nucl. Eng. Des. 2015, 293, 238–248. [Google Scholar] [CrossRef]
- Lucas, D.; Frank, T.; Lifante, C.; Zwart, P.; Burns, A. Extension of the Inhomogeneous MUSIG Model for Bubble Condensation. Nucl. Eng. Des. 2011, 241, 4359–4367. [Google Scholar] [CrossRef]
- Jeon, S.S.; Kim, S.J.; Park, G.C. Numerical study of condensing bubble in subcooled boiling flow using volume of fluid model. Chem. Eng. Sci. 2011, 66, 5899–5909. [Google Scholar] [CrossRef]
- Juanli, Z.; Wenxi, T.; Ronghua, C.; Suizheng, Q.; Guanghui, S. Two-Dimensional numerical simulation of single bubble rising behavior in liquid metal using moving particle semi-implicit method. Progress Nucl. Energy 2013, 64, 31–40. [Google Scholar] [CrossRef]
- Samkhaniani, N.; Ansari, M.R. Numerical simulation of bubble condensation using CF-VOF. Progress Nucl. Energy 2016, 89, 120–131. [Google Scholar] [CrossRef]
- Qua, X.; Revankar, S.T.; Tian, M. Numerical simulation of bubble formation and condensation of steam air mixture injected in subcooled pool. Nucl. Eng. Des. 2017, 320, 123–132. [Google Scholar] [CrossRef]
- Ha, C.-T.; Park, W.-G. Axisymmetric simulation of bubble condensation of pure steam and steam–Air mixture. Nucl. Eng. Des. 2018, 337, 193–204. [Google Scholar] [CrossRef]
- Kharangate, C.R.; Mudawar, I. Review of computational studies on boiling and condensation. Int. J. Heat Mass Transf. 2017, 108, 1164–1196. [Google Scholar] [CrossRef]
- Abdollahzadeh Jamalabadi, M.Y. Electromagnetohydrodynamic two-phase flow-Induced vibrations in vertical heated upward flow. J. Comput. Des. Eng. 2019, 6, 92–104. [Google Scholar] [CrossRef]
- Abdollahzadeh Jamalabadi, M.Y.; Ghasemi, M.; Alamian, R.; Wongwises, S.; Afrand, M.; Shadloo, M.S. Modeling of subcooled flow boiling with nanoparticles under the influence of a magnetic field. Symmetry 2019, 11, 1275. [Google Scholar] [CrossRef]
- Paramanantham S, S.; Ha, C.-T.; Park, W.-G. Numerical investigation of single and multiple bubble condensing behaviors in subcooled flow boiling based on homogeneous mixture model. Int. J. Mech. Sci. 2018, 136, 220–233. [Google Scholar] [CrossRef]
- Liu, Z.; Sunden, B.; Wu, H. Numerical Modeling of Multiple Bubbles Condensation in Subcooled Flow Boiling. J. Therm. Sci. Eng. Appl. 2015, 7, 1–9. [Google Scholar] [CrossRef]
- Owoeye, E.J.; Schubring, D. Computational modeling of bubble coalescence in a high-pressure steam-Water flow. Nucl. Eng. Des. 2017, 319, 28–39. [Google Scholar] [CrossRef]
- Chena, R.H.; Tiana, W.X.; Sua, G.H.; Qiu, S.Z.; Yuki, I.; Yoshiaki, O. Numerical investigation on coalescence of bubble pairs rising in a stagnant liquid. Chem. Eng. Sci. 2011, 66, 5055–5063. [Google Scholar] [CrossRef]
- Ha, C.T.; Kim, D.H.; Park, W.G.; Jung, C.M. A compressive interface-capturing scheme for computation of compressible multi-Fluid flows. Comput. Fluids 2017, 152, 164–181. [Google Scholar] [CrossRef]
- Merkle, C.L.; Feng, J.; Buelow, P.E.O. Computational modeling of the dynamics of sheet cavitation. In Proceedings of the 3rd International Symposium on Cavitation, Grenoble, France, 7–10 April 1998. [Google Scholar]
- Kunz, R.F.; Boger, D.A.; Stinebring, D.R.; Chyczewski, T.S.; Lindau, J.W.; Gibeling, H.J.; Venkateswaran, S.; Govindan, T.R. A preconditioned Navier–Stokes method for two-phase flows with application to cavitation prediction. Comput. Fluids 2000, 29, 849–875. [Google Scholar] [CrossRef]
- Owis, F.M.; Nayfeh, A.H. Numerical Simulation of Super- and PartiallyCavitating Flows over an Axisymmetric Projectile. AIAA 2001, 1042, 1–9. [Google Scholar]
- Lindau, J.; Venkateswaran, S.; Kunz, R.; Merkle, C. Development of a fully-Compressible multi-Phase Reynolds-Averaged Navier-Stokes model. AIAA Paper 2001, 2648, 1–12. [Google Scholar] [CrossRef]
- Ha, C.T.; Park, W.G. Evaluation of a new scaling term in preconditioning schemes for computations of compressible cavitating and ventilated flows. Ocean Eng. 2016, 126, 432–466. [Google Scholar] [CrossRef]
- Ha, C.T.; Park, W.G. Application of preconditioning to compressible multi-Phase mixture flow computation. In Proceedings of the 5th International Symposium on Fluids Machinery and Fluids Engineering, Jejum South Korea, Jeju, Korea, 24–27 October 2012; pp. 24–27. [Google Scholar]
- Jin, M.S.; Park, W.G.; Jung, C.M. Numerical analysis of cavitating flow past an axisymmetric cylinder with comparison to experiments. J. Mech. Sci. Technol. 2013, 27, 3673–3681. [Google Scholar] [CrossRef]
- Venkateswaran, S.; Lindau, J.W.; Kunz, R.; Merkle, C.L. Computation of multiphase mixture flows with compressibility effects. J. Computat. Phys. 2002, 180, 54–77. [Google Scholar] [CrossRef]
- Jin, M.S.; Ha, C.T.; Park, W.G. Numerical study on heat transfer effects of cavitating and flashing flows based on homogeneous mixture model. Int. J. Heat Mass Transf. 2017, 109, 1068–1083. [Google Scholar] [CrossRef]
- Phan, T.-H.; Won, S.-S.; Park, W.-G. Numerical simulation of air–steam mixture condensation flows in a vertical tube. Int. J. Heat Mass Transf. 2018, 127, 568–578. [Google Scholar] [CrossRef]
- Kretzschmar, H.J.; Wager, W. International Steam Tables-Properties of Water and Steam Based on the Industrial Formulation IAPWS-IF97; Springer: Berlin/Heidelberg, Germany, 2007; Part-A. [Google Scholar]
- Brackbill, J.U.; Kothe, D.B.; Zemach, C. A continuum method for modeling surface tension. J. Comput. Phys. 1992, 100, 335–354. [Google Scholar] [CrossRef]
- Ha, C.-T.; Park, W.-G.; Jung, C.-M. Numerical simulations of compressible flows using multi-Fluid models. Int. J. Multiph. Flow 2015, 74, 5–18. [Google Scholar] [CrossRef]
- Lee, W.H. A pressure iteration scheme for two-Phase flow modeling. In Multiphase Transport Fundamentals, Reactor Safety, Applications; Veziroglu, T.N., Ed.; Hemisphere Publishing: Washington, DC, USA, 1980; Volume 1. [Google Scholar]
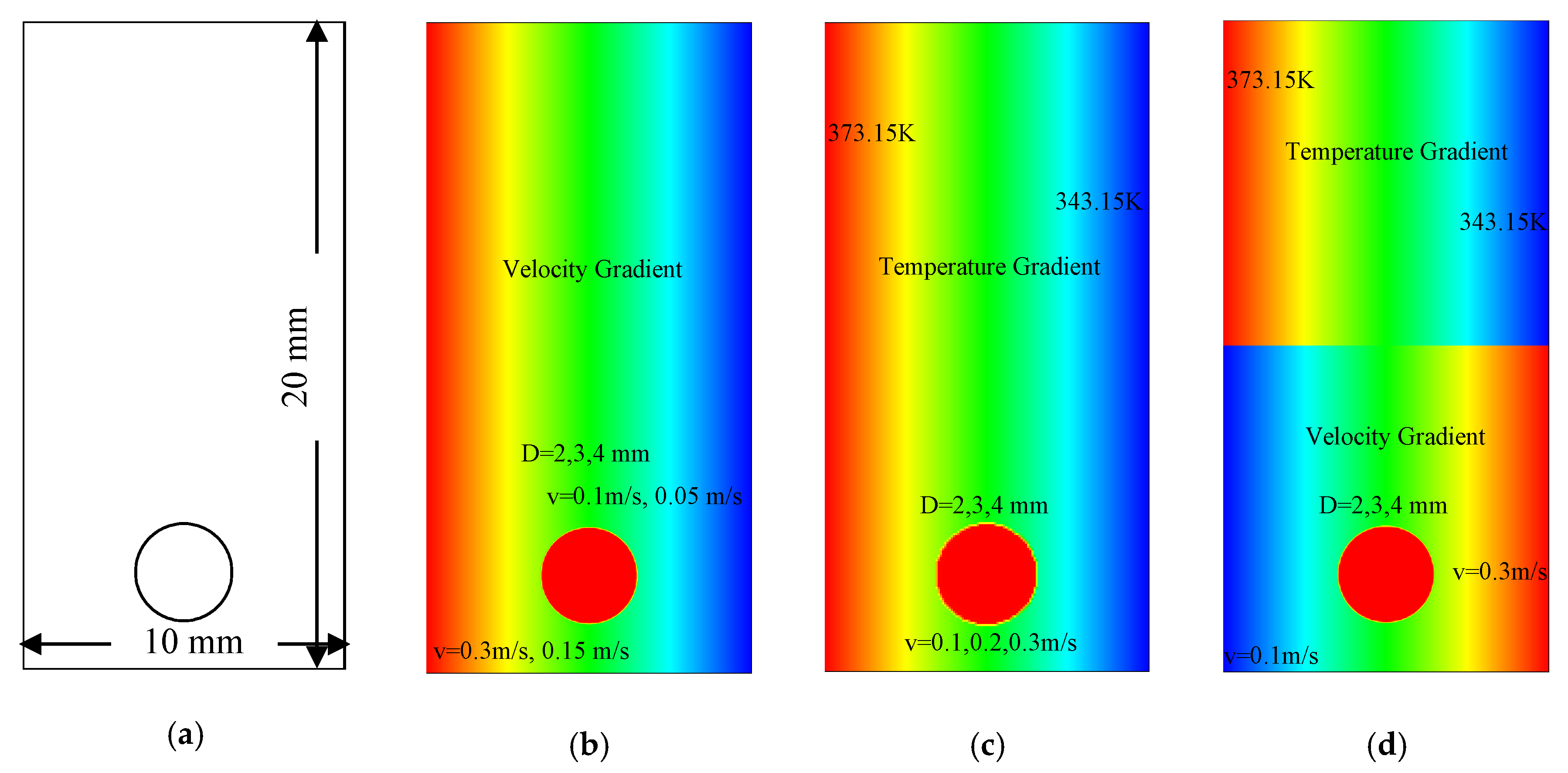









| Case No. | Bubble Diameter D (mm) | Subcooled Temp. ΔT (K) | Operating Pressure (MPa) | Vel. Gradient (m/s) | |
|---|---|---|---|---|---|
| Vmin | Vmax | ||||
| a | 2 | 5, 10, 15, 20, 30 | 0.101 | 0.1 | 0.3 |
| b | 3 | ||||
| c | 4 | ||||
| d | 4 | 0.05 | 0.15 | ||
| Case No. | Bubble Diameter D (mm) | Subcooled Temp. ΔT (K) | Velocity (m/s) | Temp. Gradient (K) | |
|---|---|---|---|---|---|
| Tmin | Tmax | ||||
| e | 4 | 0, 5 | 0.1, 0.2, 0.3 | 343.15 | 373.15 |
| Case No. | Bubble Diameter D (mm) | Subcooled Temp. ΔT (K) | Velocity Gradient (m/s) | Temp. Gradient (K) | ||
|---|---|---|---|---|---|---|
| Vmin | Vmax | Tmin | Tmax | |||
| f | 2 | 0, 5 | 0.1 | 0.3 | 343.15 | 373.15 |
| g | 3 | |||||
| h | 4 | |||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Paramanantham, S.S.; Kim, D.-H.; Park, W.-G. Numerical Study of Bubble Behavior under Gradient Flows during Subcooled Flow Boiling in Vertical Flow Channel. Symmetry 2020, 12, 611. https://doi.org/10.3390/sym12040611
Paramanantham SS, Kim D-H, Park W-G. Numerical Study of Bubble Behavior under Gradient Flows during Subcooled Flow Boiling in Vertical Flow Channel. Symmetry. 2020; 12(4):611. https://doi.org/10.3390/sym12040611
Chicago/Turabian StyleParamanantham, SalaiSargunan S, Dong-Hyun Kim, and Warn-Gyu Park. 2020. "Numerical Study of Bubble Behavior under Gradient Flows during Subcooled Flow Boiling in Vertical Flow Channel" Symmetry 12, no. 4: 611. https://doi.org/10.3390/sym12040611
APA StyleParamanantham, S. S., Kim, D.-H., & Park, W.-G. (2020). Numerical Study of Bubble Behavior under Gradient Flows during Subcooled Flow Boiling in Vertical Flow Channel. Symmetry, 12(4), 611. https://doi.org/10.3390/sym12040611






