On the Metric Dimension of Arithmetic Graph of a Composite Number
Abstract
1. Introduction
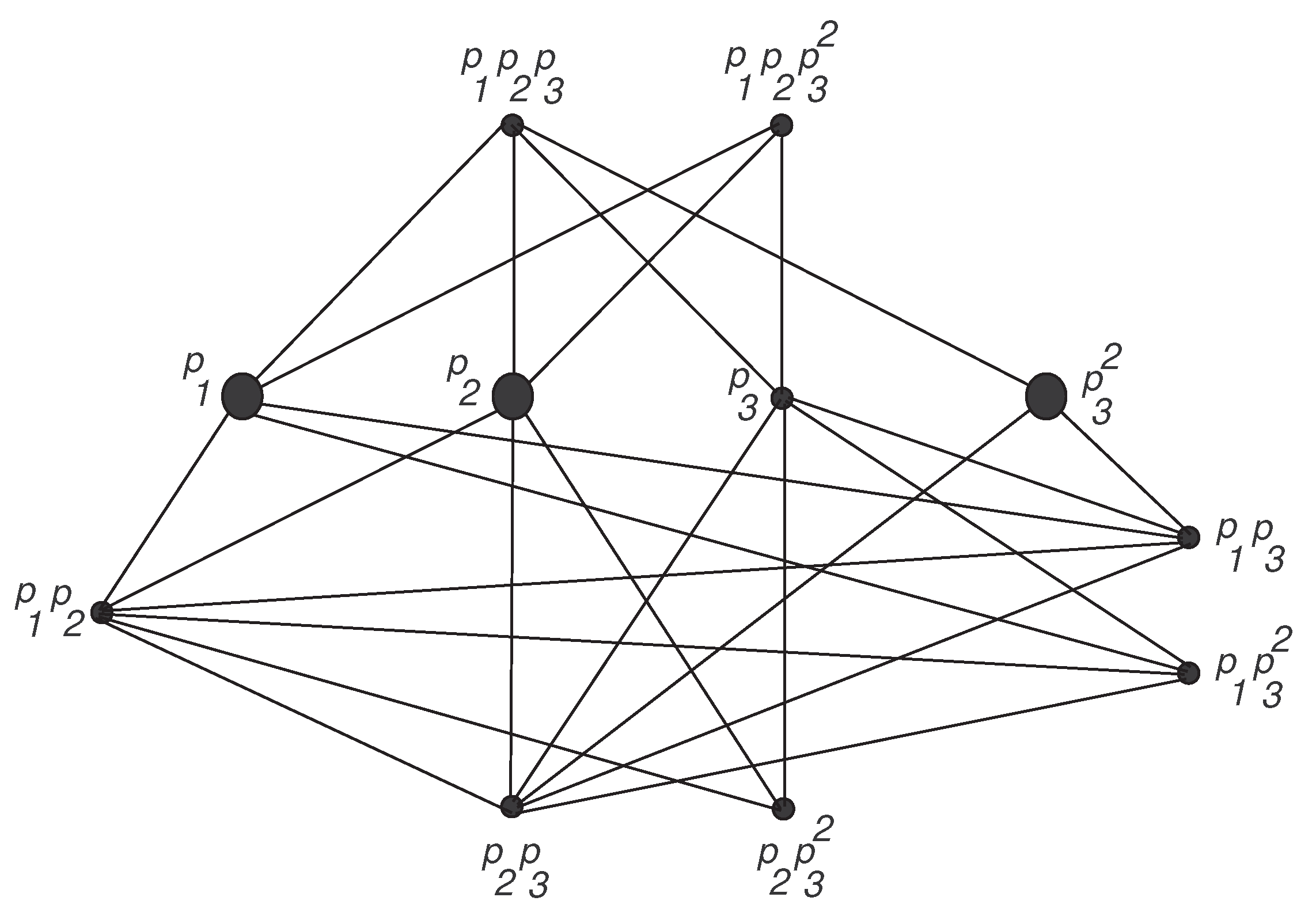
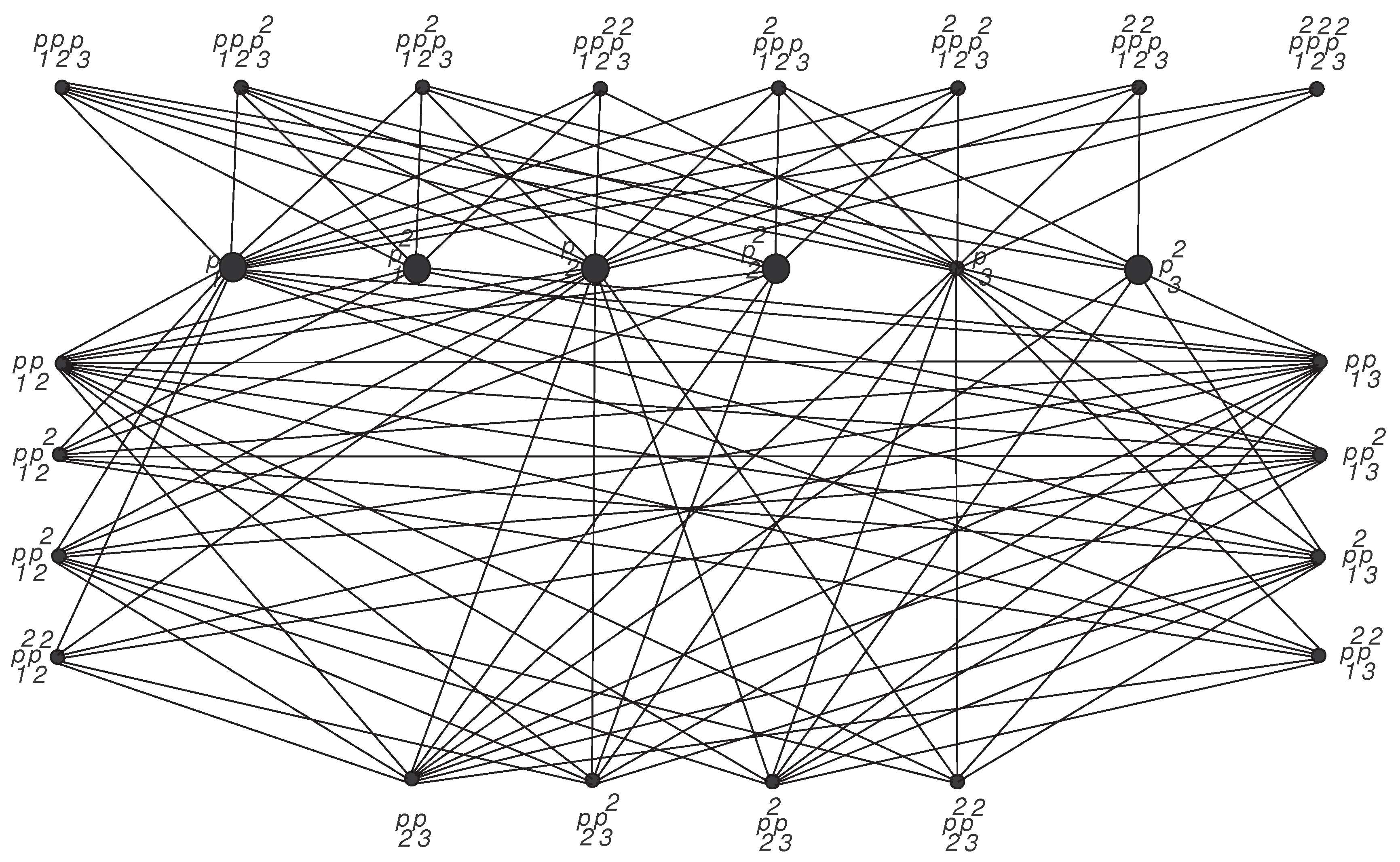
2. Results
- (i)
- if and only if .
- (ii)
- if and only if .
Metric Dimension of Arithmetic Graphs
- (i)
- For and , .
- (ii)
- For and , .
- (iii)
- For and , .
- (iv)
- For and , .
- (v)
- For , .
3. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| Arithmetic graph of a composite number m with at least two distinct primary divisors | |
| The diameter of a graph G | |
| The metric dimension of a graph G | |
| The degree of a vertex v | |
| The open neighborhood of a vertex v | |
| The distance between the vertices x and y |
References
- Berge, C. The Theory of Graphs and Its Applications; John Wiley and Sons: Hoboken, NJ, USA, 2019. [Google Scholar]
- Slater, P.J. Leaves of trees. Cong. Numer. 1975, 14, 549–559. [Google Scholar]
- Harary, F.; Melter, R.A. On the metric dimension of a graph. ARS Comb. 1976, 2, 191–195. [Google Scholar]
- Sebo, A.; Tannier, E. On metric generators of graphs. Math. Oper. Res. 2004, 29, 383–393. [Google Scholar] [CrossRef]
- Shapiro, H.; Sodeeberg, S. A combinatory detection problem. Am. Math. 1963, 70, 1066–1070. [Google Scholar]
- Chvatal, V. Mastermind. Combinatorica 1983, 3, 325–329. [Google Scholar] [CrossRef]
- Melter, R.A.; Tomescu, I. Metric bases in digital geometry. Comput. Vis. Graphics Image Process. 1984, 25, 113–121. [Google Scholar] [CrossRef]
- Chartrand, G.; Eroh, L.; Jhonson, M.; Oellermann, O. Resolviability in graph and metric dimension of a graph. Discret. App. Math. 2000, 105, 99–133. [Google Scholar] [CrossRef]
- Babai, L. On the complexity of canonical labeling of strongly regular graphs. SIAM J. Comput. 1980, 9, 212–216. [Google Scholar] [CrossRef]
- Beerliova, Z.; Eberhard, F.; Erlebach, T.; Hall, A.; Hoffmann, M.; Mihalak, M.; Ram, L. Network discovery and verification. IEEE J. Sel. Area Commun. 2006, 24, 2168–2181. [Google Scholar] [CrossRef]
- Bailey, R.F.; Caceres, J.; Garijo, D.; Gonzalez, A.; Marquez, A.; Meagher, K.; Puertas, M.L. Resolving sets for Johnson and Kneser graphs. Eur. J. Comb. 2013, 34, 736–751. [Google Scholar] [CrossRef]
- Bailey, R.F.; Cameron, P.J. Base size, metric dimension and other invariants of groups and graphs. Bull. Lond. Math. Soc. 2011, 43, 209–242. [Google Scholar] [CrossRef]
- Garey, M.R.; Johnson, D.S. Computers and Intractability. In A Guide to the Theory of NP-Completeness; Freeman: New York, NY, USA, 1979. [Google Scholar]
- Khuller, S.; Raghavachari, B.; Rosenfeld, A. Landmarks in graphs. Discret. Appl. Math. 1996, 70, 217–229. [Google Scholar] [CrossRef]
- Fehr, M.; Gosselin, S.; Oellermann, O.R. The metric dimension of Cayley digraphs. Discret. Math. 2006, 306, 31–41. [Google Scholar] [CrossRef]
- Shanmukha, B.; Sooryanarayana, B.; Harinath, K.S. Metric dimension of wheels. Far East J. Appl. Math. 2002, 8, 217–229. [Google Scholar]
- Poisson, C.; Zhang, P. The metric dimension of unicyclic graphs. J. Comb. Math. Comb. Comput. 2002, 40, 17–32. [Google Scholar]
- Caceres, J.; Hernando, C.; Mora, M.; Pelayo, I.M.; Puertas, M.L.; Seara, C.; Wood, D.R. On the metric dimension of cartesian products of graphs. SIAM J. Discret. Math. 2007, 21, 423–441. [Google Scholar] [CrossRef]
- Imran, S.; Siddiqui, M.K.; Imran, M.; Hussain, M.; Bilal, H.M.; Cheema, I.Z.; Tabraiz, A.; Saleem, Z. Computing the metric dimension of gear graphs. Symmetry 2018, 10, 209. [Google Scholar] [CrossRef]
- Imran, S.; Siddiqui, M.K.; Imran, M.; Hussain, M. On metric dimensions of symmetric graphs obtained by rooted product. Mathematics 2018, 6, 191. [Google Scholar] [CrossRef]
- Hussain, Z.; Munir, M.; Chaudhary, M.; Kang, M.N. Computing metric dimension and metric basis of 2D lattice of alpha-boron nanotubes. Symmetry 2018, 10, 300. [Google Scholar] [CrossRef]
- Ahmad, S.; Chaudhry, M.A.; Javaid, I.; Salman, M. On the metric dimension of generalized Petersen graphs. Quaest. Math. 2013, 36, 421–435. [Google Scholar] [CrossRef]
- Feng, M.; Ma, X.; Wang, K. The structure and metric dimension of the power graph of a finite group. Eur. J. Comb. 2015, 34, 82–97. [Google Scholar] [CrossRef]
- Jager, G.; Drewes, F. The metric dimension of is ⌊⌋. Comput. Sci. 2019, in press. [Google Scholar]
- Chaluvaraju, B.; Chaitra, V. Sign domination in arithmetic graphs. Gulf J. Math. 2016, 4, 49–54. [Google Scholar]
- Somer, L.; Křížek, M. On a connection of number theory with graph theory. Czechoslovak Math. J. 2004, 54, 465–485. [Google Scholar] [CrossRef]
- Yegnanarayanan, V. Analytic number theory for graph theory. Southeast Asian Bull. Math. 2011, 35, 717–733. [Google Scholar]
- Alon, N.; Erdos, P. An application of graph theory to additive number theory. Eur. J. Comb. 1985, 6, 201–203. [Google Scholar] [CrossRef]
- Saradhi, V. Vangipuram. Irregular graphs. Graph Theory Notes N. Y. 2001, 41, 33–36. [Google Scholar]
- Vasumathi, N.; Vangipuram, S. Existence of a graph with a given domination parameter. In Proceedings of the Fourth Ramanujan Symposium on Algebra and Its Applications, Madras, India, 1–3 February 1995; University of Madras: Madras, India, 1995; pp. 187–195. [Google Scholar]
- Suryanarayana Rao, K.V.; Sreenivansan, V. The split domination in arithmetic graphs. Int. J. Comput. Appl. 2011, 29, 46–49. [Google Scholar]
- Vasumathi, N.; Vangipuram, S. The annihilator domination in some standard graphs and arithmetic graphs. Int. J. Pure Appl. Math. 2016, 106, 123–135. [Google Scholar]
- Hernando, C.; Mora, M.; Pelaya, I.M.; Seara, C.; Wood, D.R. Extremal graph theory for metric dimension and diameter. Electronic Notes Discret. Math. 2007, 29, 339–343. [Google Scholar] [CrossRef]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rehman, S.u.; Imran, M.; Javaid, I. On the Metric Dimension of Arithmetic Graph of a Composite Number. Symmetry 2020, 12, 607. https://doi.org/10.3390/sym12040607
Rehman Su, Imran M, Javaid I. On the Metric Dimension of Arithmetic Graph of a Composite Number. Symmetry. 2020; 12(4):607. https://doi.org/10.3390/sym12040607
Chicago/Turabian StyleRehman, Shahid ur, Muhammad Imran, and Imran Javaid. 2020. "On the Metric Dimension of Arithmetic Graph of a Composite Number" Symmetry 12, no. 4: 607. https://doi.org/10.3390/sym12040607
APA StyleRehman, S. u., Imran, M., & Javaid, I. (2020). On the Metric Dimension of Arithmetic Graph of a Composite Number. Symmetry, 12(4), 607. https://doi.org/10.3390/sym12040607





