Kaonic Atoms to Investigate Global Symmetry Breaking
Abstract
1. Introduction
2. Low-Energy Strong Interaction Dynamics
2.1. Global Symmetries
2.2. Meson-Nucleon Sigma Terms
- There are channels opened below the threshold.
- There is a resonance, , just below threshold of .
- Uncertainties in the extrapolation procedure are present: the difference is larger than in the case, introducing additional uncertainty in the quantity of interest . In quantitative terms: let us refer to the case, where the “experimental” value is based on solid experimental data [9]. Here, at the C–D point is . The estimated difference [10] gives [11]; i.e., an uncertainty of about 10%.
3. Antikaon-Nucleon Scattering Lengths
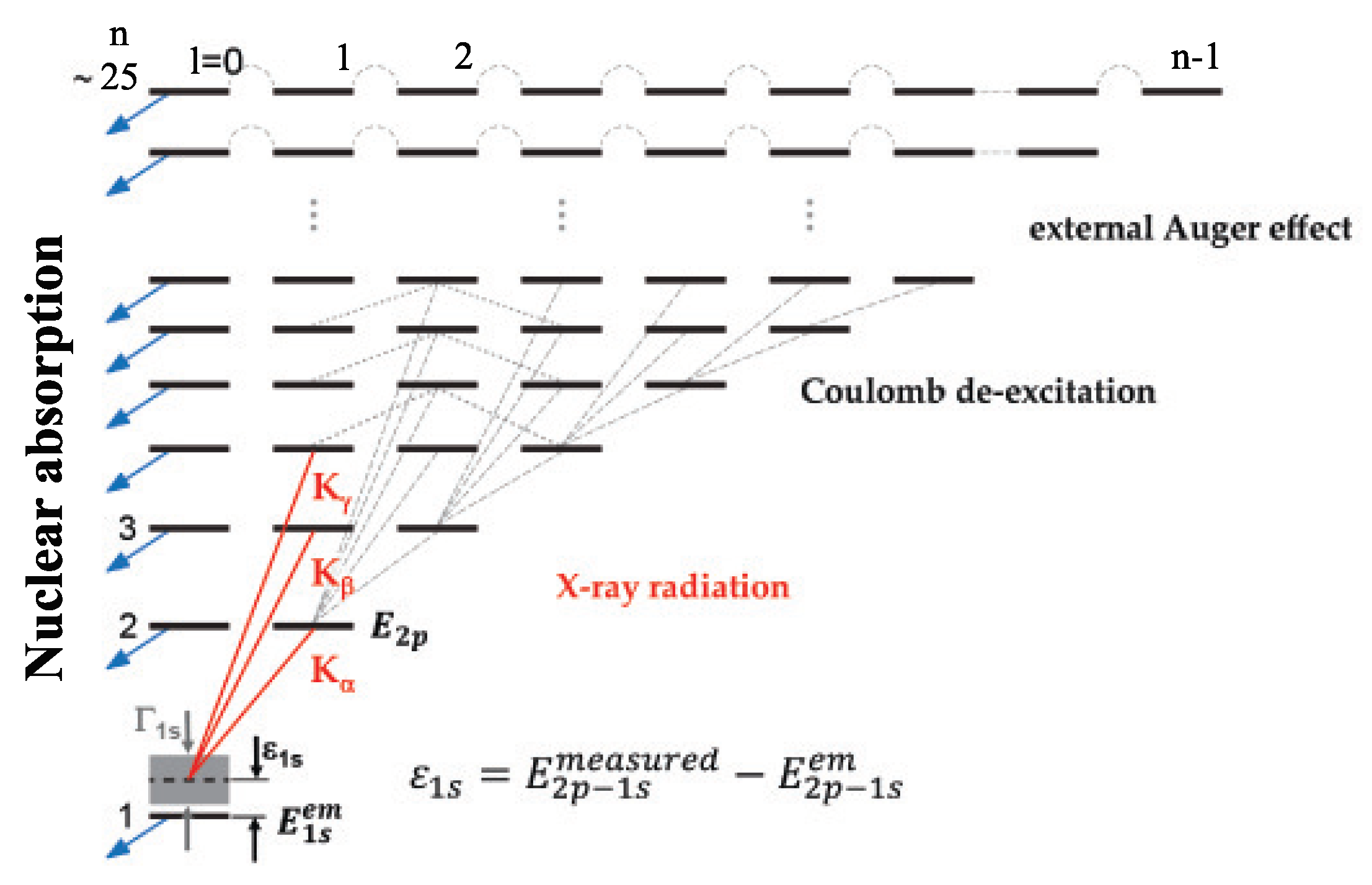
3.1. Formation of a Kaonic Atom
3.2. Antikaon-Nucleon Scattering Lengths
4. The SIDDHARTA Kaonic Hydrogen Measurement
5. Kaonic Deuterium Experiments
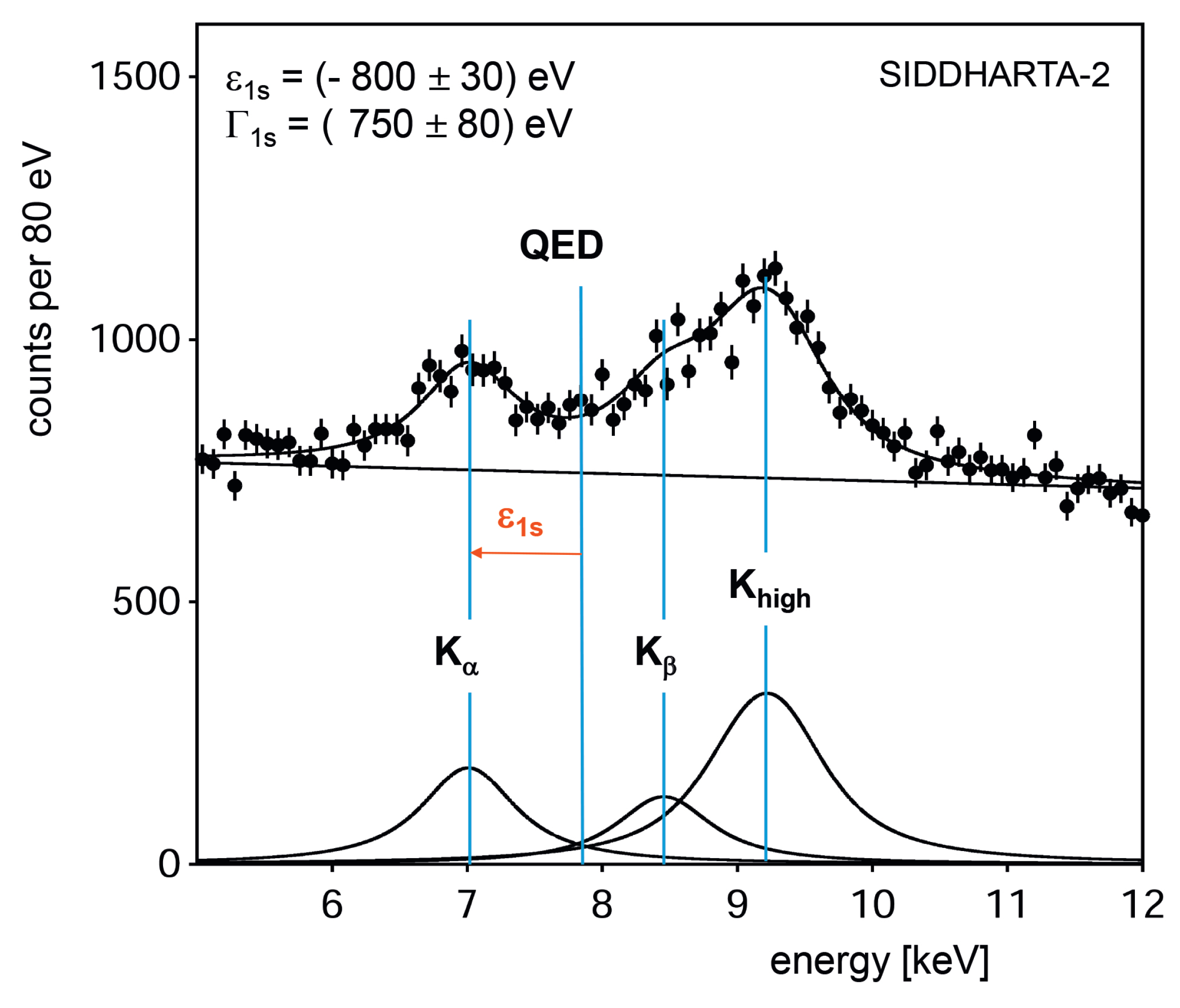
5.1. The SIDDHARTA-2 Experiment at LNF-INFN
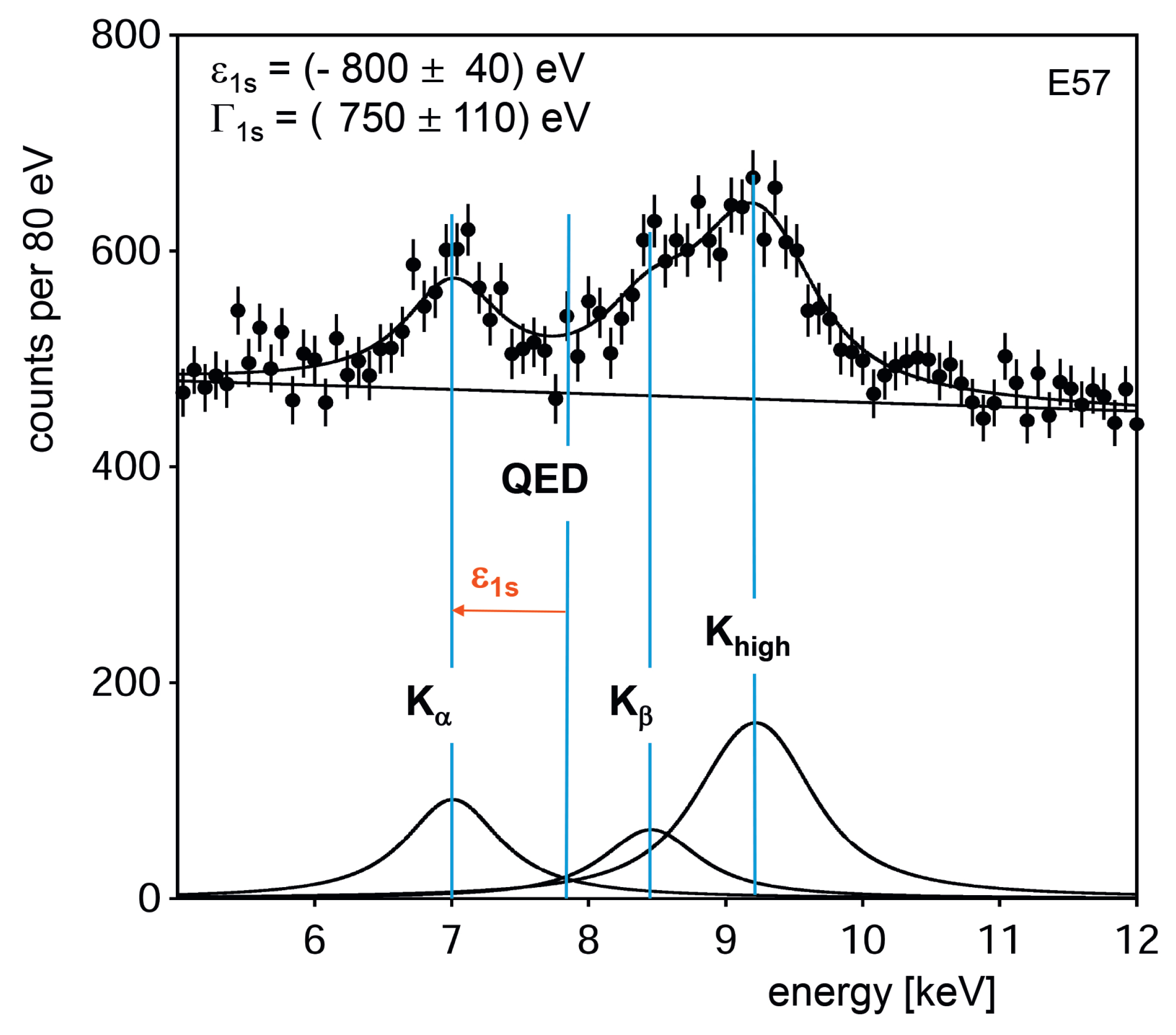
5.2. The E57 Experiment at J-PARC
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Guaraldo, C. The DEAR physics programme. Phys. Detect. DAΦNE 1999, XVI, 643–658. [Google Scholar]
- Reya, C. Chiral symmetry breaking and meson-nucleon sigma commutators: A Review. Rev. Mod. Phys. 1974, 46, 545–580. [Google Scholar] [CrossRef]
- Cheng, T.P.; Dashen, R.T. Experimental test of time-reversal invariance in the reaction np → γd. Phys. Rev. Lett. 1971, 26, 1659–1662. [Google Scholar]
- Altarelli, G.; Cabibbo, N.; Maiani, L. The sigma term and low-energy π-n scattering. Nucl. Phys. B 1971, 34, 621–631. [Google Scholar] [CrossRef]
- Gasser, J.; Sainio, M.E. Sigma term physics. Phys. Detect. DADAΦNE 1999, XVI, 659. [Google Scholar]
- Pavan, M.M.; Arndt, R.A.; Strakovsky, I.I.; Workman, R.I. New Result for the Pion-Nucleon Sigma Term from an Updated VPI/GW Pion-Nucleon Partial-Wave and Dispersion Relation Analysis. PiN Newslett. 1999, 15, 118–192. [Google Scholar]
- Bottino, A.; Donato, F.; Fornengo, N.; Scopel, S. Size of the neutralino nucleon cross-section in the light of a new determination of the pion nucleon sigma term. Astropart. Phys. 2002, 18, 205–211. [Google Scholar] [CrossRef][Green Version]
- Koch, R. A New Determination of the πN Sigma Term Using Hyperbolic Dispersion Relations in the (ν2, t) plane. Z. Phys. C 1982, 15, 161–168. [Google Scholar] [CrossRef]
- Jaffe, R.L. The Sigma Term Revisited. Phys. Rev. D 1980, 21, 3215–3224. [Google Scholar] [CrossRef]
- Pagels, H.J.; Pardee, W.J. Nonanalytic behavior of the sigma term in π-n scattering. Phys. Rev. D 1971, 4, 3335–3337. [Google Scholar] [CrossRef]
- Jaffe, R.L.; Korpa, C.L. The Pattern of Chiral Symmetry Breaking and the Strange Quark Content of the Proton. Comments Nucl. Part. Phys. 1987, 17, 163–175. [Google Scholar]
- Di Claudio, B.; Violini, G.; Rodríguez-Vergas, A.M. Uncertainties on the Determination of KNΣ Term Due to K−N Unphysical and Low-energy Region. Lett. Nuovo Cimento 1979, 26, 555–561. [Google Scholar] [CrossRef]
- Di Claudio, B.; Rodríguez-Vergas, A.M.; Violini, G. The Adler-Weisberger sum rule and the σ-commutator for the kaon-proton system. Z. Phys. C 1979, 3, 75–82. [Google Scholar] [CrossRef]
- Rodríguez-Vergas, A.M.; Violini, G. The Adler-Weisberger sum rule and the σ-commutator for the kaon-neutron system. Z. Phys. C 1980, 4, 135–139. [Google Scholar] [CrossRef]
- Martin, A.D.; Violini, G. The Zero Energy Kp Scattering Amplitude and the Evaluation of the Kaon-Nucleon Σ Terms. Lett. Nuovo Cimento 1981, 30, 105–110. [Google Scholar] [CrossRef]
- Chen, H.-X.; Dimitrasinovic, V.; Hosaka, A. Baryon fields with UL(3) × UR(3) chiral symmetry: Axial currents of nucleons and hyperons. Phys. Rev. D 2010, 81. [Google Scholar] [CrossRef]
- Chen, H.-X.; Dimitrasinovic, V.; Hosaka, A. Baryon fields with UL(3) × UR(3) chiral symmetry. IV. Interactions with chiral (8,1) ⨁ (1,8) vector and axial-vector mesons and anomalous magnetic moments. Phys. Rev. C 2012, 85, 055205(1)–055205(10). [Google Scholar] [CrossRef]
- Chen, H.-X.; Dimitrasinovic, V.; Hosaka, A.; Nagata, K.; Zhu, S.-L. Chiral properties of baryon fields with flavor SU(3) symmetry. Phys. Rev. D 2008, 78. [Google Scholar] [CrossRef]
- Alexandrou, C.; Drach, V.; Jansen, K.; Kallidonis, C.; Koutsou, G. Baryon spectrum with Nf = 2 + 1 + 1 twisted mass fermions. Phys. Rev. D 2014, 90, 074501(1)–074501(29). [Google Scholar] [CrossRef]
- Torrero, C. Computing the nucleon sigma terms at the physical point. In Proceedings of the 32nd International Symposium on Lattice Field Theory, New York, NY, USA, 23–28 June 2014. [Google Scholar]
- Dalitz, R.H.; McGinley, J.; Belyea, C.; Anthony, S. Theory of Low-energy Kaon-Nucleon Scattering. In Proceedings of the International Conference on Hypernuclear and Kaon Physics, Heidelberg, Germany, 20–24 June 1982; pp. 201–214. [Google Scholar]
- Zmeskal, J. From kaonic atoms to kaonic nuclei: A search for antikaon-mediated bound nuclear systems. Part. Nucl. Phys. 2008, 61, 512–550. [Google Scholar] [CrossRef]
- Iwasaki, M.; Bartlett, K.; Beer, G.A.; Gill, D.R.; Hayano, R.S.; Ito, T.M.; Lee, L.; Mason, G.; Nakamura, S.N.; Olin, A.; et al. Discovery of the repulsive energy shift of the kaonic hydrogen 1s state. Nucl. Phys. A 1998, 639, 501c–510c. [Google Scholar] [CrossRef]
- Deser, S.; Goldberger, M.L.; Baumann, K.; Thirring, W. Energy Level Displacements in Pi-Mesonic Atoms. Phys. Rev. 1954, 96, 774–776. [Google Scholar] [CrossRef]
- Trueman, T.L. Energy level shifts in atomic states of strongly-interacting particles. Nucl. Phys. 1961, 26, 57–67. [Google Scholar] [CrossRef]
- Meißner, U.-G.; Raha, U.; Rusetsky, A. Kaon-nucleon scattering lengths from kaonic deuterium experiments. Eur. Phys. J. C 2006, 47, 473–480. [Google Scholar] [CrossRef][Green Version]
- Meißner, U.-G.; Raha, U.; Rusetsky, A. Spectrum and decays of kaonic hydrogen. Eur. Phys. J. C 2004, 35, 349–357. [Google Scholar] [CrossRef]
- Bazzi, M.; Beer, G.; Bombelli, L.; Bragadireanu, A.; Cargnelli, M.; Corradi, G.; Curceanu (Petrascu), C.; d’Uffizi, A.; Fiorini, C.; Frizzi, T.; et al. A new measurement of kaonic hydrogen X-rays. Phys. Lett. B 2011, 704, 113–117. [Google Scholar] [CrossRef]
- Beer, G.; Bragadireanu, A.M.; Cargnelli, M.; Curceanu-Petrascu, C.; Egger, J.P.; Fuhrmann, H.; Guaraldo, C.; Iliescu, M.; Ishiwatari, T.; Itahashi, K.; et al. Measurement of the kaonic hydrogen X-ray spectrum. Phys. Rev. Lett. 2005, 94, 212302. [Google Scholar] [CrossRef]
- Hashimoto, T.; Ajimura, S.; Beer, G.; Bhang, H.; Bragadireanu, M.; Busso, L.; Cargnelli, M.; Choi, S.; Curceanu, C.; Enomoto, S.; et al. Search for the deeply bound K−pp state from the semi-inclusive forward-neutron spectrum in the in-flight K− reaction on helium-3. Prog. Theor. Exp. Phys. 2015, 2015. [Google Scholar] [CrossRef][Green Version]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Curceanu, C.; Guaraldo, C.; Sirghi, D.; Amirkhani, A.; Baniahmad, A.; Bazzi, M.; Bellotti, G.; Bosnar, D.; Bragadireanu, M.; Cargnelli, M.; et al. Kaonic Atoms to Investigate Global Symmetry Breaking. Symmetry 2020, 12, 547. https://doi.org/10.3390/sym12040547
Curceanu C, Guaraldo C, Sirghi D, Amirkhani A, Baniahmad A, Bazzi M, Bellotti G, Bosnar D, Bragadireanu M, Cargnelli M, et al. Kaonic Atoms to Investigate Global Symmetry Breaking. Symmetry. 2020; 12(4):547. https://doi.org/10.3390/sym12040547
Chicago/Turabian StyleCurceanu, Catalina, Carlo Guaraldo, Diana Sirghi, Aidin Amirkhani, Ata Baniahmad, Massimiliano Bazzi, Giovanni Bellotti, Damir Bosnar, Mario Bragadireanu, Michael Cargnelli, and et al. 2020. "Kaonic Atoms to Investigate Global Symmetry Breaking" Symmetry 12, no. 4: 547. https://doi.org/10.3390/sym12040547
APA StyleCurceanu, C., Guaraldo, C., Sirghi, D., Amirkhani, A., Baniahmad, A., Bazzi, M., Bellotti, G., Bosnar, D., Bragadireanu, M., Cargnelli, M., Carminati, M., Clozza, A., De Paolis, L., Del Grande, R., Fiorini, C., Iliescu, M., Iwasaki, M., King, P., Levi Sandri, P., ... Zmeskal, J. (2020). Kaonic Atoms to Investigate Global Symmetry Breaking. Symmetry, 12(4), 547. https://doi.org/10.3390/sym12040547






