Nonlocal Elasticity Response of Doubly-Curved Nanoshells
Abstract
1. Introduction
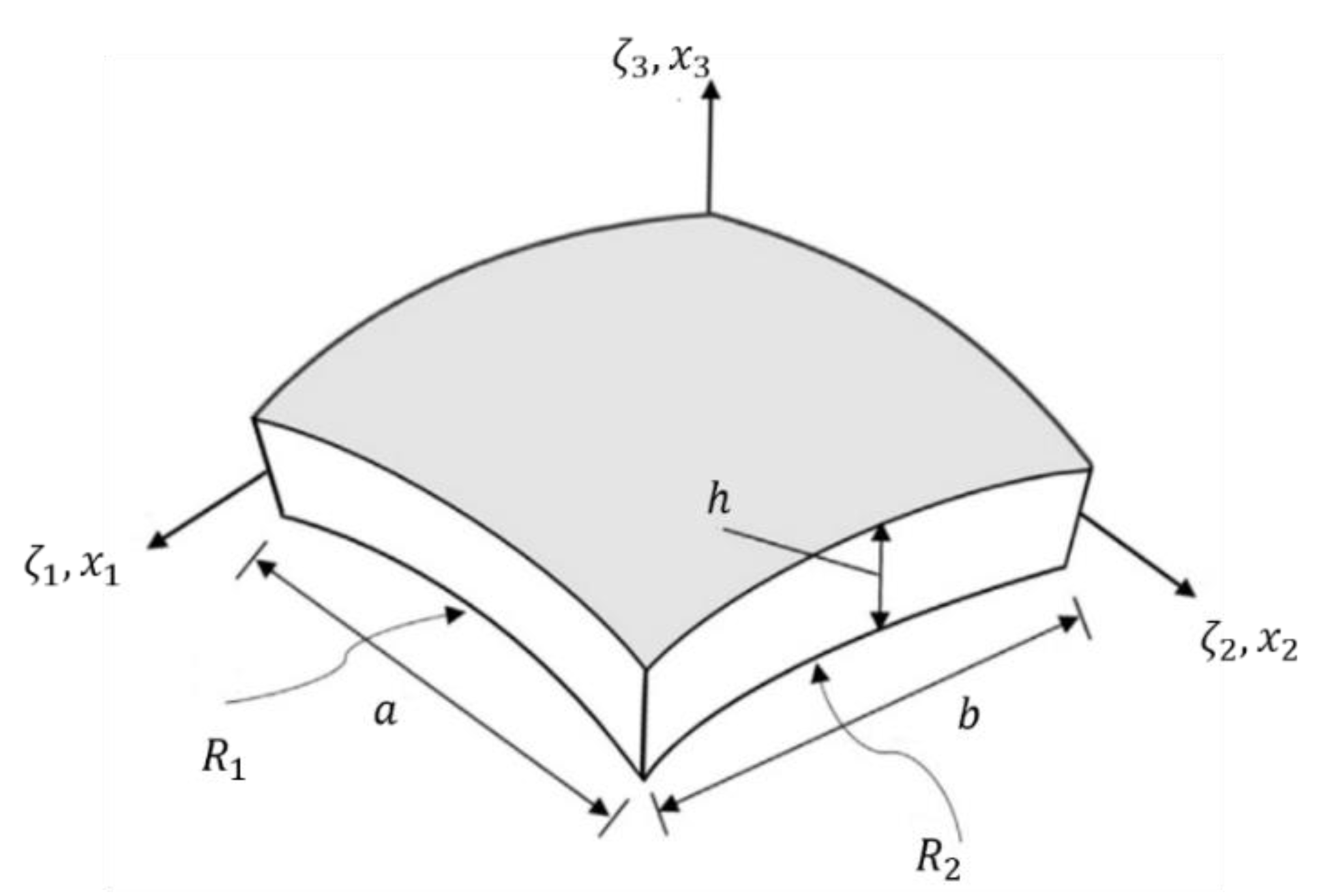
2. Governing Equations of Doubly-Curved Nanoshells
3. Solution Procedure
4. Numerical Results and Discussion
4.1. Comparison and Validation
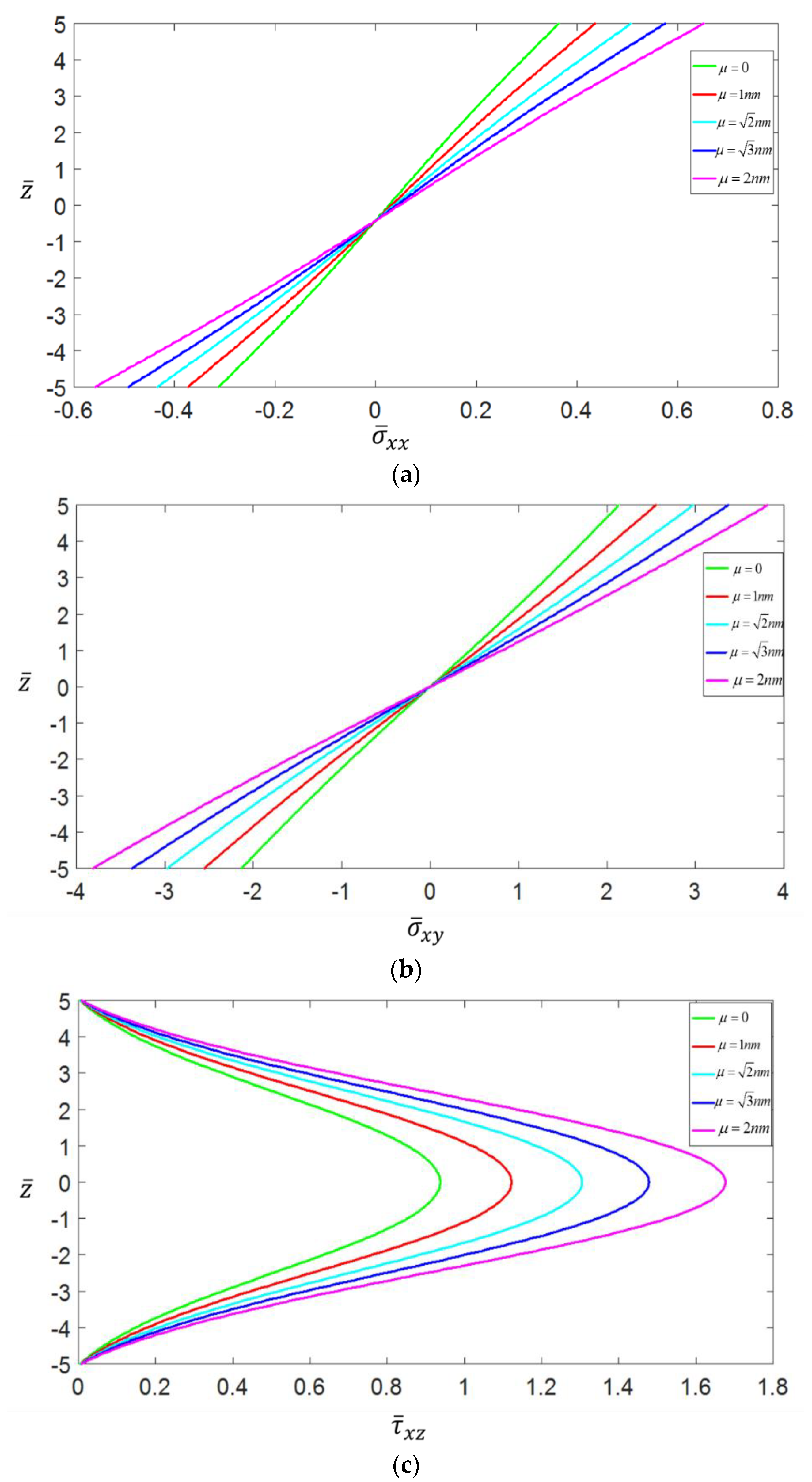
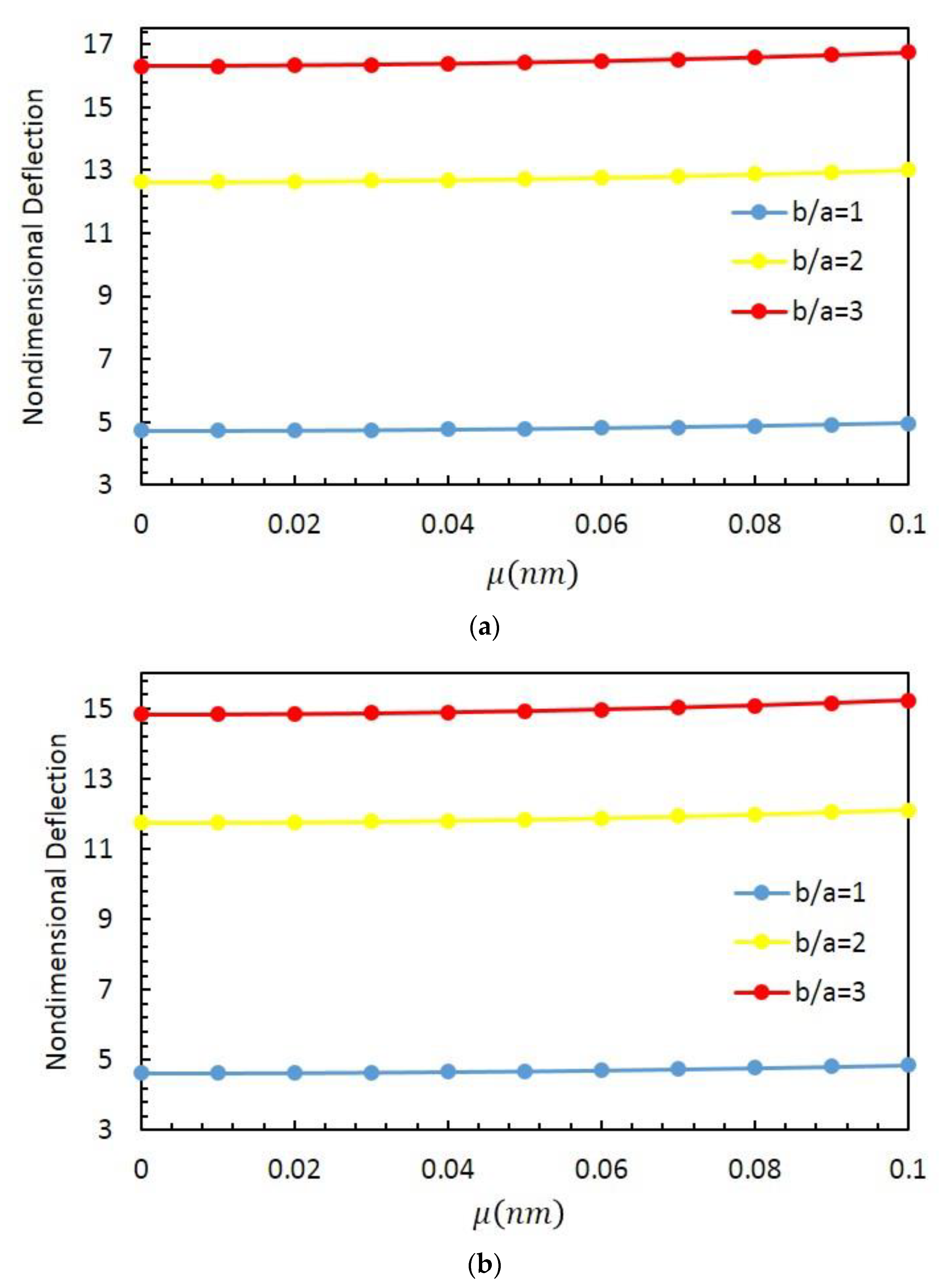
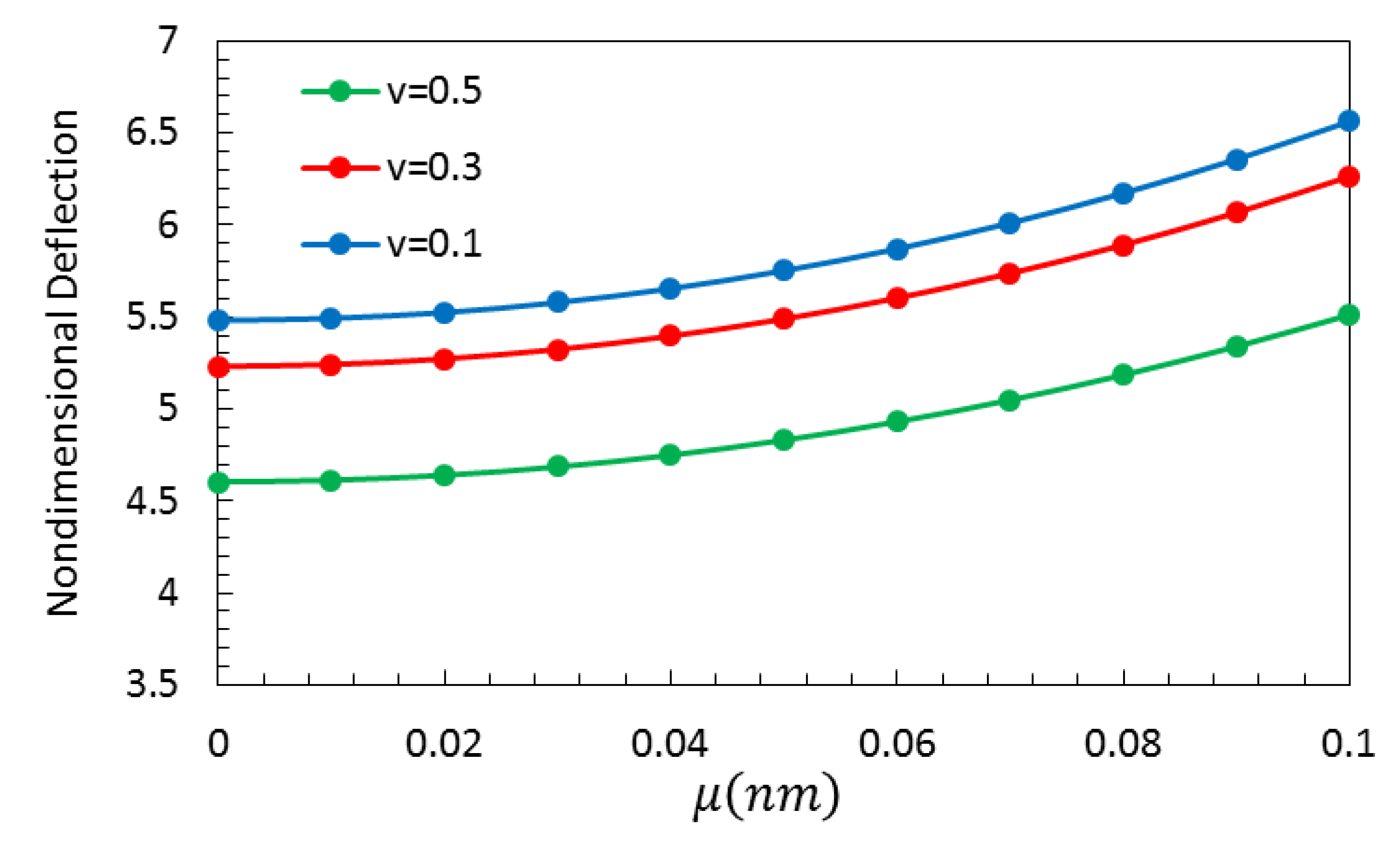
4.2. Static Analysis of Doubly-Curved Thin Nanoshells
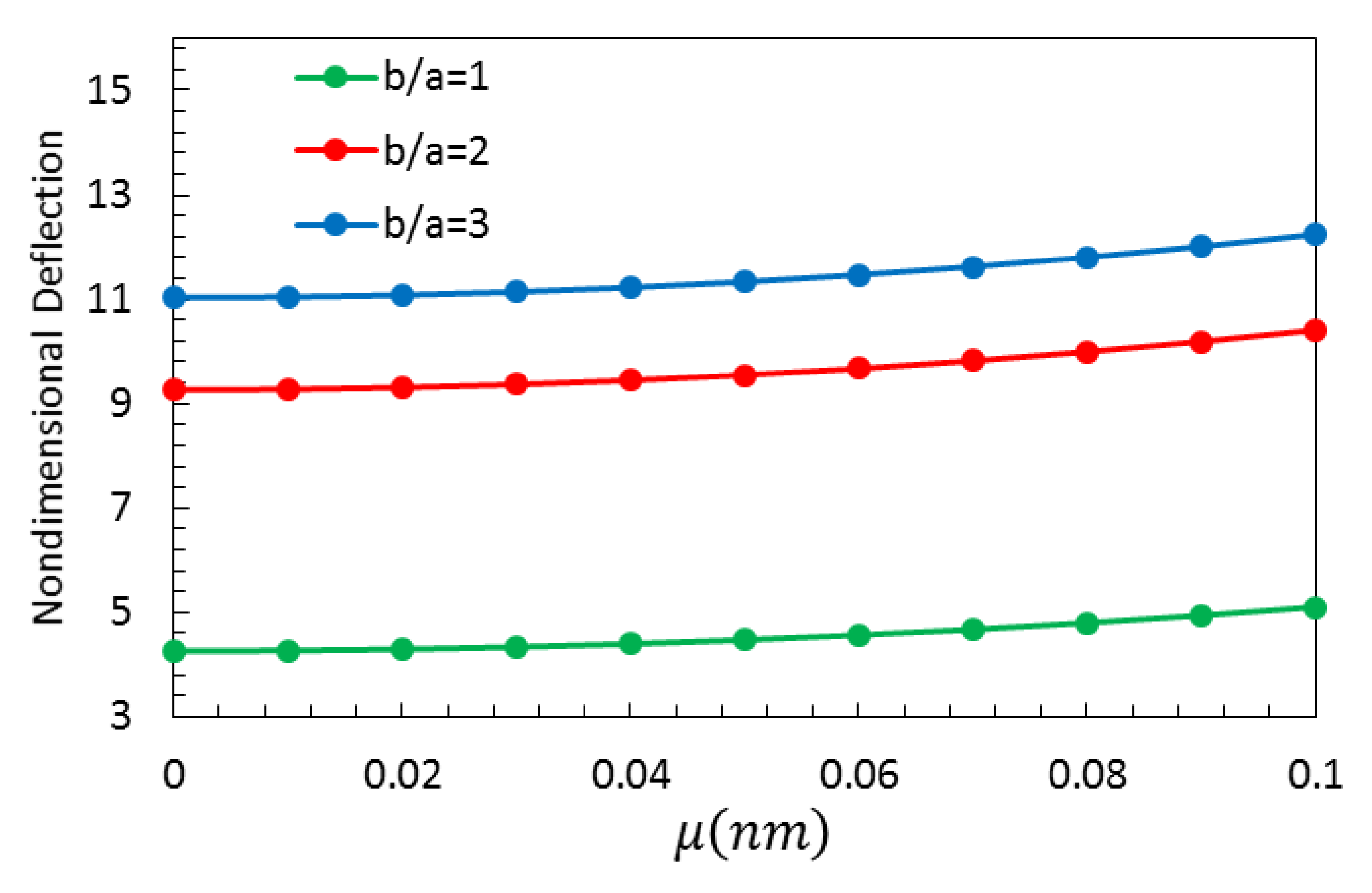
4.3. Static Analysis of Doubly-Curved Deep Nanoshells
5. Conclusions
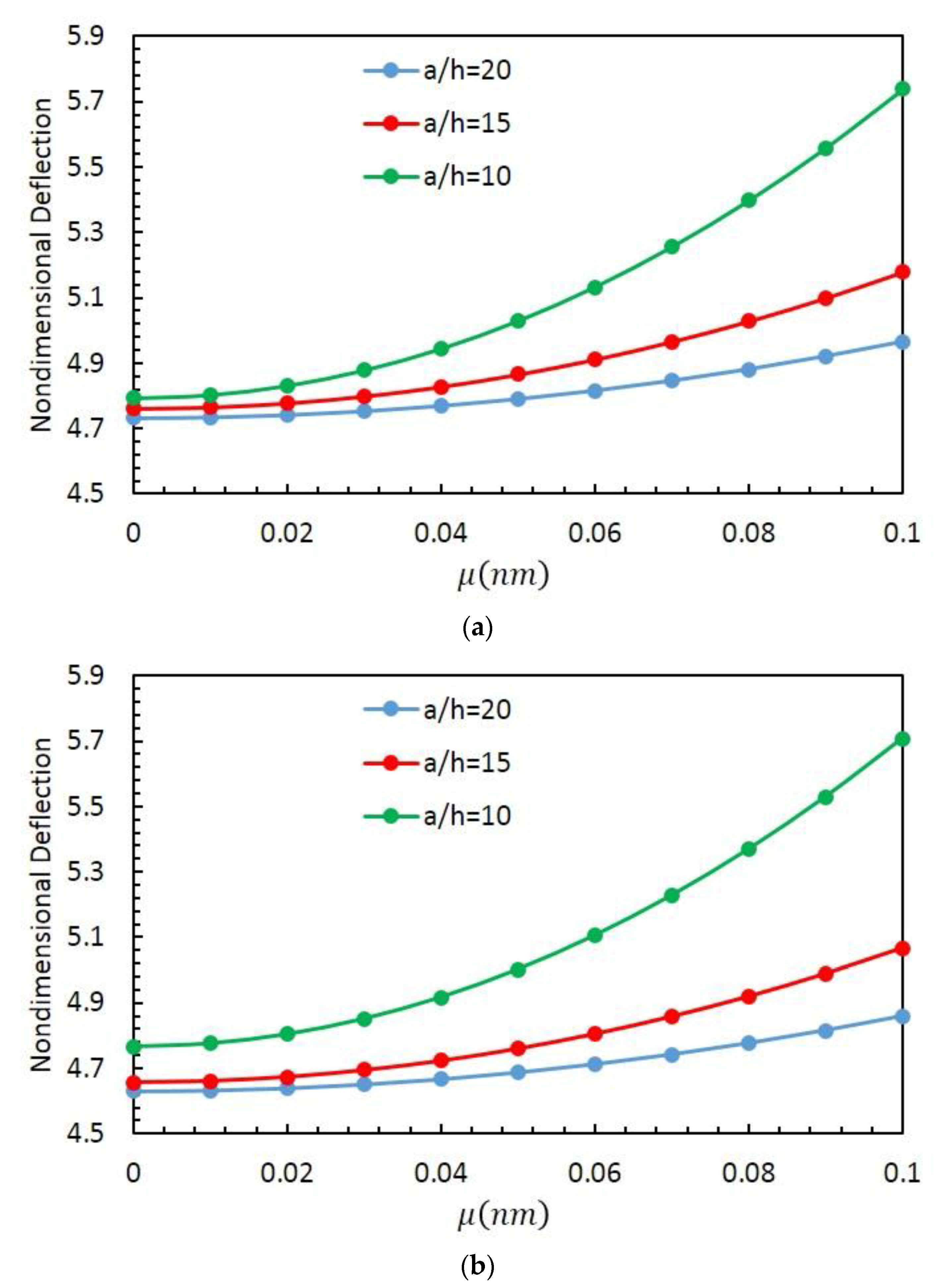
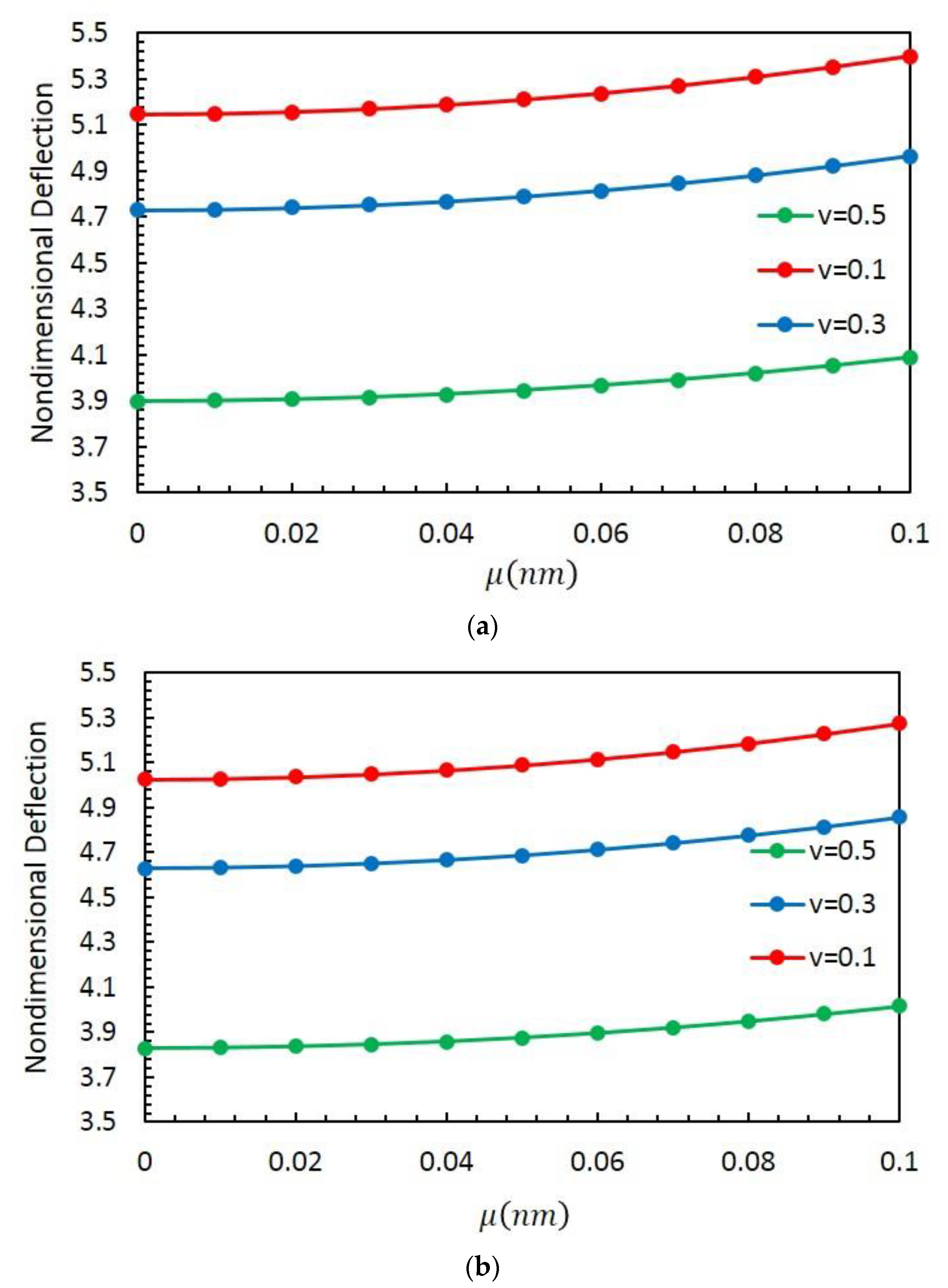
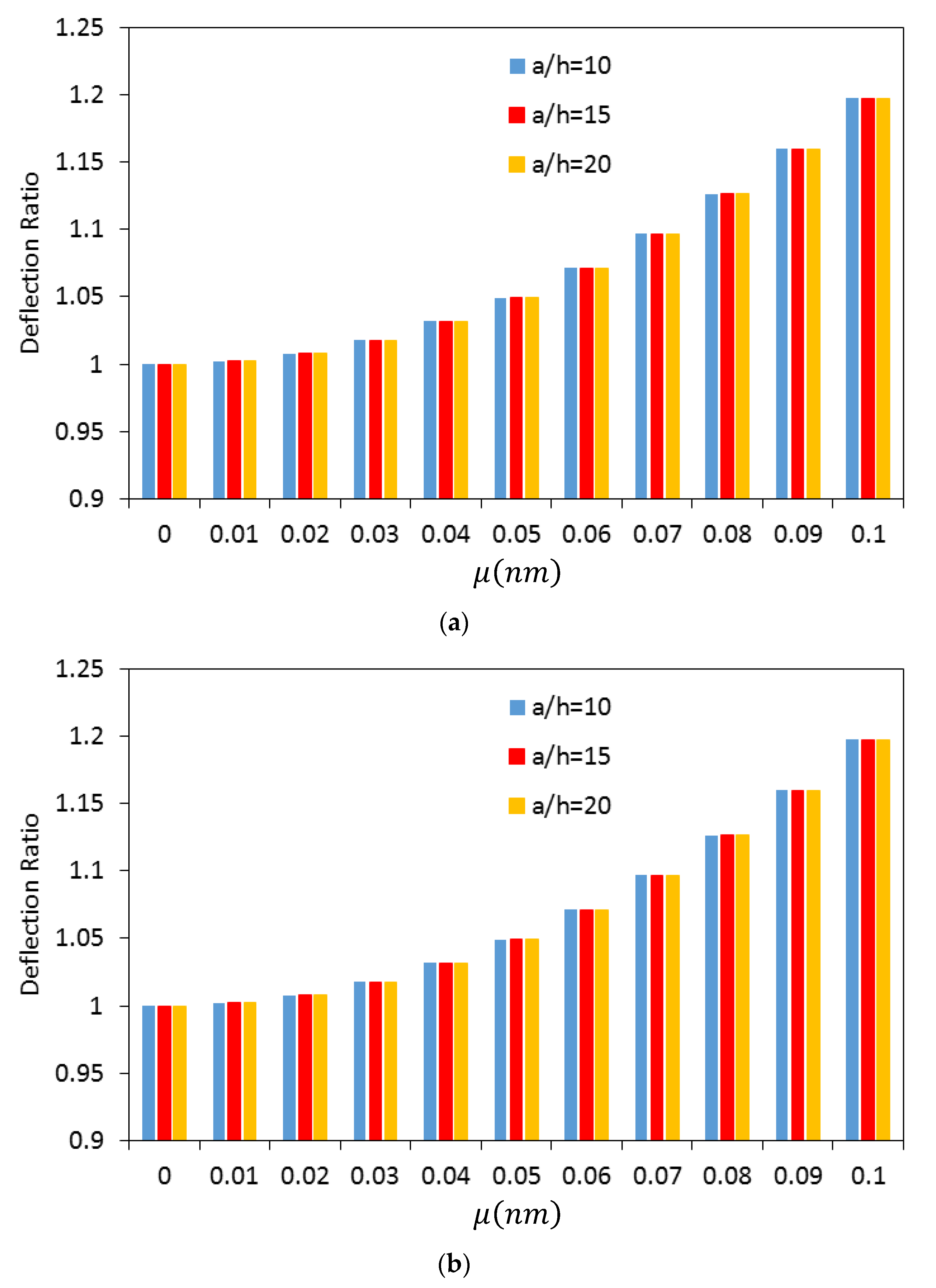
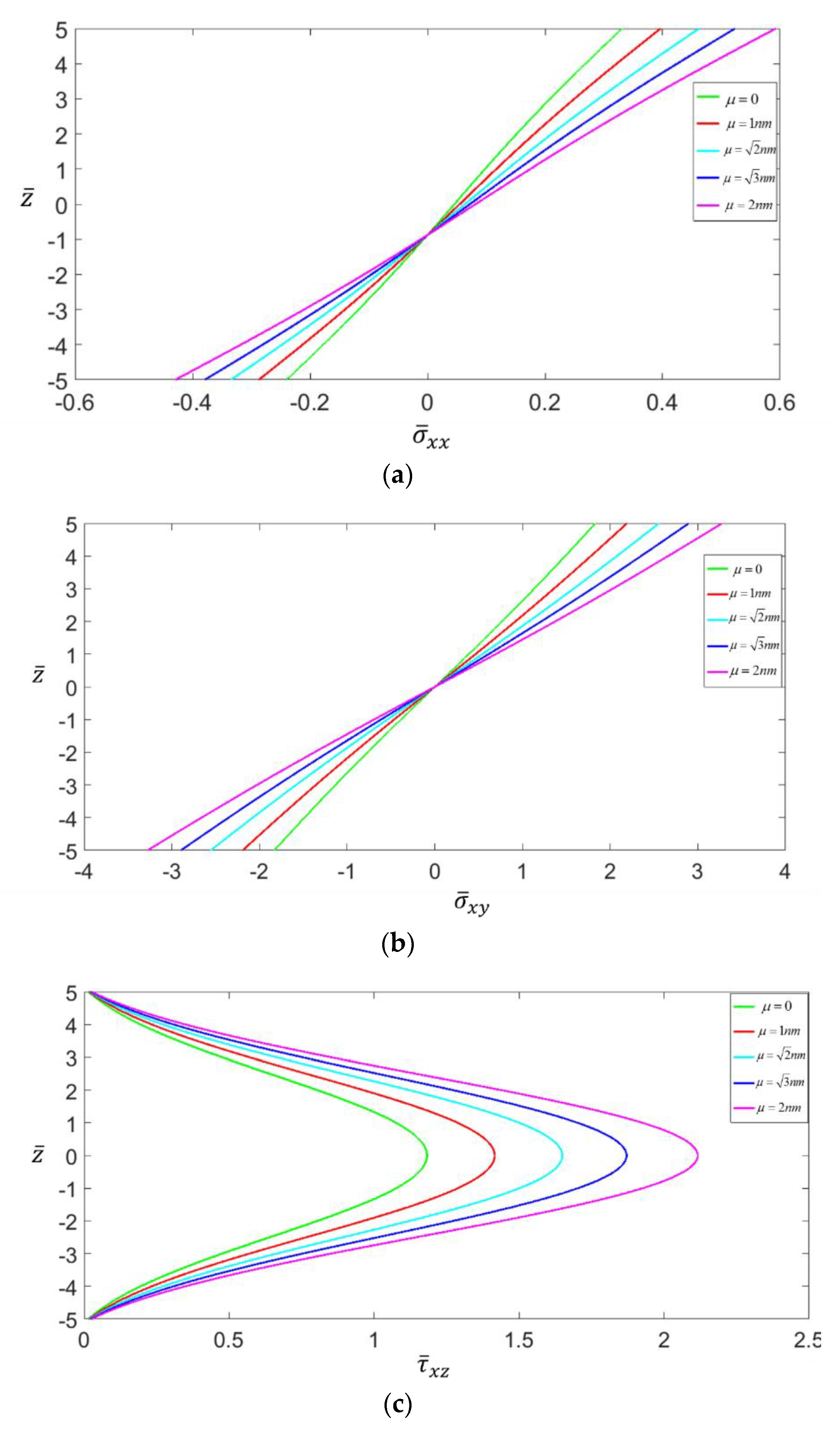
- An increased nonlocal parameter decreases the stiffness of the isotropic shallow and deep panels, along with a decreased natural frequency, an increased deflection of the nanostructure, and increased stress components.
- An increased value for the side-to-thickness ratio, Poisson’s ratio, and length-to-side ratio yields a reduced deflection in the isotropic shallow and deep panel.
- The side-to-thickness ratio does not significantly affect the deflection ratio of shallow and deep panels.
- The axial and longitudinal shear stress components at the top and bottom sides of shallow panels feature higher values than the deep ones.
- Shallow panels are more flexible than deep panels, as visible from their higher deformable response, when compared to deep panels.
Author Contributions
Funding
Conflicts of Interest
Appendix A
Appendix B
References
- Love, A.H. A Treatise on the Mathematical Theory of Elasticity; Cambridge University Press: Cambridge, UK, 1944; Volume 1, p. 952. [Google Scholar]
- Sokolnikoff, I.S.; Walker, M. Tensor analysis: Theory and applications. Am. J. Phys. 1952, 20, 249–250. [Google Scholar] [CrossRef]
- Sokolnikoff, I.S. Mathematical Theory of Elasticity; McGraw-Hill: New York, NY, USA, 1956; Volume 83. [Google Scholar]
- Timoshenko, S.P.; Woinowsky-Krieger, S. Theory of Plates and Shells; McGraw-Hill: New York, NY, USA, 1959. [Google Scholar]
- Leissa, A.W. Vibration of Shells; NASA: Washington, DC, USA, 1973.
- Niordson, F. Shell Theory; North-Holland: Amsterdam, The Nederland, 1985. [Google Scholar]
- Tornabene, F.; Fantuzzi, N. Mechanics of Laminated Composite Doubly-Curvel Shell Structures: The Generalized Differential Quadrature Method and the Strong Formulation Finite Element Method; Società Editrice Esculapio: Bologna, Italy, 2014. [Google Scholar]
- Carrera, E. Multilayered shell theories accounting for layerwise mixed description, Part 1: Governing equations. AIAA J. 1999, 37, 1107–1116. [Google Scholar] [CrossRef]
- Carrera, E. Historical review of zig-Zag theories for multilayered plates and shells. Appl. Mech. Rev. 2003, 56, 287–308. [Google Scholar] [CrossRef]
- Tornabene, F.; Viola, E.; Inman, D.J. 2-D differential quadrature solution for vibration analysis of functionally graded conical, cylindrical shell and annular plate structures. J. Sound Vib. 2009, 328, 259–290. [Google Scholar] [CrossRef]
- Duc, N.D.; Quan, T.Q. Transient responses of functionally graded double curved shallow shells with temperature-Dependent material properties in thermal environment. Eur. J. Mech.-A/Solids 2014, 47, 101–123. [Google Scholar] [CrossRef]
- Eringen, A.C. Nonlocal polar elastic continua. Int. J. Eng. Sci. 1972, 10, 1–16. [Google Scholar] [CrossRef]
- Eringen, A.C. On differential equations of nonlocal elasticity and solutions of screw dislocation and surface waves. J. Appl. Phys. 1983, 54, 4703–4710. [Google Scholar] [CrossRef]
- Eringen, A.C. Nonlocal Continuum Field Theories; Springer Science & Business Media: New York, NY, USA, 2002. [Google Scholar]
- Ramirez, F.; Heyliger, P.R.; Pan, E. Static analysis of functionally graded elastic anisotropic plates using a discrete layer approach. Compos. Part B Eng. 2006, 37, 10–20. [Google Scholar] [CrossRef]
- Merdaci, S.; Belghoul, H. High-Order shear theory for static analysis of functionally graded plates with porosities. Comptes Rendus Mécanique 2019, 347, 207–217. [Google Scholar] [CrossRef]
- Alibeigloo, A.; Nouri, V. Static analysis of functionally graded cylindrical shell with piezoelectric layers using differential quadrature method. Compos. Struct. 2010, 92, 1775–1785. [Google Scholar] [CrossRef]
- Kumar, R.; Mishra, B.; Jain, S. Static and dynamic analysis of smart cylindrical shell. Finite Elem. Anal. Des. 2008, 45, 13–24. [Google Scholar] [CrossRef]
- Frikha, A.; Dammak, F. Geometrically non-Linear static analysis of functionally graded material shells with a discrete double directors shell element. Comput. Methods Appl. Mech. Eng. 2017, 315, 1–24. [Google Scholar] [CrossRef]
- Mantari, J.L.; Oktem, A.S.; Soares, C.G. Static and dynamic analysis of laminated composite and sandwich plates and shells by using a new higher-Order shear deformation theory. Compos. Struct. 2011, 94, 37–49. [Google Scholar] [CrossRef]
- Sahmani, S.; Aghdam, M. Nonlinear instability of hydrostatic pressurized hybrid FGM exponential shear deformable nanoshells based on nonlocal continuum elasticity. Compos. Part B Eng. 2017, 114, 404–417. [Google Scholar] [CrossRef]
- Zeighampour, H.; Beni, Y.T.; Karimipour, I. Torsional vibration and static analysis of the cylindrical shell based on strain gradient theory. Arab. J. Sci. Eng. 2016, 41, 1713–1722. [Google Scholar] [CrossRef]
- Sahmani, S.; Aghdam, M. Size dependency in axial postbuckling behavior of hybrid FGM exponential shear deformable nanoshells based on the nonlocal elasticity theory. Compos. Struct. 2017, 166, 104–113. [Google Scholar] [CrossRef]
- Ke, L.; Wang, Y.; Reddy, J. Thermo-Electro-Mechanical vibration of size-Dependent piezoelectric cylindrical nanoshells under various boundary conditions. Compos. Struct. 2014, 116, 626–636. [Google Scholar] [CrossRef]
- Salehipour, H.; Shahsavar, A.; Civalek, O. Free vibration and static deflection analysis of functionally graded and porous micro/nanoshells with clamped and simply supported edges. Compos. Struct. 2019, 221, 110842. [Google Scholar] [CrossRef]
- Zeng, S.; Wang, B.; Wang, K. Static stability analysis of nanoscale piezoelectric shells with flexoelectric effect based on couple stress theory. Microsyst. Technol. 2018, 24, 2957–2967. [Google Scholar] [CrossRef]
- Arefi, M.; Mohammad-Rezaei Bidgoli, E.; Dimitri, R.; Tornabene, F. Free vibrations of functionally graded polymer composite nanoplates reinforced with graphene nanoplatelets. Aerosp. Sci. Technol. 2018, 81, 108–117. [Google Scholar] [CrossRef]
- Jouneghani, F.Z.; Dimitri, R.; Tornabene, F. Structural response of porous FG nanobeams under hygro-Thermo-Mechanical loadings. Compos. Part B Eng. 2018, 152, 71–78. [Google Scholar] [CrossRef]
- Arefi, M.; Mohammad-Rezaei Bidgoli, E.; Dimitri, R.; Bacciocchi, M.; Tornabene, F. Nonlocal bending analysis of curved nanobeams reinforced by graphene nanoplatelets. Compos. Part B Eng. 2019, 166, 1–12. [Google Scholar] [CrossRef]
- Arefi, M.; Bidgoli, E.M.-R.; Dimitri, R.; Tornabene, F.; Reddy, J.N. Size-Dependent free vibrations of FG polymer composite curved nanobeams reinforced with graphene nanoplatelets resting on Pasternak foundations. Appl. Sci. 2019, 9, 1580. [Google Scholar] [CrossRef]
- Karami, B.; Janghorban, M.; Shahsavari, D.; Dimitri, R.; Tornabene, F. Nonlocal Buckling Analysis of Composite Curved Beams Reinforced with Functionally Graded Carbon Nanotubes. Molecules 2019, 24, 2750. [Google Scholar] [CrossRef] [PubMed]
- Malikan, M.; Nguyen, V.B.; Dimitri, R.; Tornabene, F. Dynamic modeling of non-Cylindrical curved viscoelastic single-Walled carbon nanotubes based on the second gradient theory. Mater. Res. Express 2019, 6, 075041. [Google Scholar] [CrossRef]
- Arefi, M. Analysis of a doubly curved piezoelectric nano shell: Nonlocal electro-Elastic bending solution. Eur. J. Mech.-A/Solids 2018, 70, 226–237. [Google Scholar] [CrossRef]
- Tornabene, F.; Fantuzzi, N.; Viola, E.; Carrera, E. Static analysis of doubly-Curved anisotropic shells and panels using CUF approach, differential geometry and differential quadrature method. Compos. Struct. 2014, 107, 675–697. [Google Scholar] [CrossRef]
- Tornabene, F.; Viola, E. Static analysis of functionally graded doubly-Curved shells and panels of revolution. Meccanica 2013, 48, 901–930. [Google Scholar] [CrossRef]
- Viola, E.; Tornabene, F.; Fantuzzi, N. Static analysis of completely doubly-Curved laminated shells and panels using general higher-Order shear deformation theories. Compos. Struct. 2013, 101, 59–93. [Google Scholar] [CrossRef]
- Liew, K.; Lim, C. A higher-Order theory for vibration of doubly curved shallow shells. J. Appl. Mech. 1996, 63, 587–593. [Google Scholar] [CrossRef]
- Amabili, M.; Reddy, J. A new non-Linear higher-Order shear deformation theory for large-Amplitude vibrations of laminated doubly curved shells. Int. J. Non-Linear Mech. 2010, 45, 409–418. [Google Scholar] [CrossRef]
- Jouneghani, F.Z.; Dashtaki, P.M.; Dimitri, R.; Bacciocchi, M.; Tornabene, F. First-Order shear deformation theory for orthotropic doubly-Curved shells based on a modified couple stress elasticity. Aerosp. Sci. Technol. 2018, 73, 129–147. [Google Scholar] [CrossRef]
- Arefi, M.; Rabczuk, T. A nonlocal higher order shear deformation theory for electro-Elastic analysis of a piezoelectric doubly curved nano shell. Compos. Part B Eng. 2019, 168, 496–510. [Google Scholar] [CrossRef]
- Karami, B.; Janghorban, M.; Tounsi, A. Variational approach for wave dispersion in anisotropic doubly-Curved nanoshells based on a new nonlocal strain gradient higher order shell theory. Thin-Walled Struct. 2018, 129, 251–264. [Google Scholar] [CrossRef]
- Dindarloo, M.H.; Li, L. Vibration analysis of carbon nanotubes reinforced isotropic doubly-Curved nanoshells using nonlocal elasticity theory based on a new higher order shear deformation theory. Compos. Part B Eng. 2019, 175, 107170. [Google Scholar] [CrossRef]
- Karami, B.; Shahsavari, D.; Janghorban, M. On the dynamics of porous doubly-Curved nanoshells. Int. J. Eng. Sci. 2019, 143, 39–55. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Kabir, H.R. On analytical solutions to boundary-Value problems of doubly-Curved moderately-Thick orthotropic shells. Int. J. Eng. Sci. 1989, 27, 1325–1336. [Google Scholar] [CrossRef]
- Tornabene, F.; Fantuzzi, N.; Bacciocchi, M. On the mechanics of laminated doubly-Curved shells subjected to point and line loads. Int. J. Eng. Sci. 2016, 109, 115–164. [Google Scholar] [CrossRef]
- Ghayesh, M.H.; Farokhi, H. Nonlinear mechanics of doubly curved shallow microshells. Int. J. Eng. Sci. 2017, 119, 288–304. [Google Scholar] [CrossRef]
- Zaoui, F.Z.; Ouinas, D.; Tounsi, A. New 2D and quasi-3D shear deformation theories for free vibration of functionally graded plates on elastic foundations. Compos. Part B Eng. 2019, 159, 231–247. [Google Scholar] [CrossRef]
- Mantari, J.; Oktem, A.; Soares, C.G. A new higher order shear deformation theory for sandwich and composite laminated plates. Compos. Part B Eng. 2012, 43, 1489–1499. [Google Scholar] [CrossRef]
- Reddy, J.N. Mechanics of Laminated Composite Plates and Shells: Theory and Analysis; CRC Press: Boca Raton, FL, USA; London, UK; New York, NY, USA; Washington, DC, USA, 2003. [Google Scholar]
- Romano, G.; Barretta, R.; Diaco, M. On nonlocal integral models for elastic nano-Beams. Int. J. Mech. Sci. 2017, 131–132, 490–499. [Google Scholar] [CrossRef]
- Barretta, R.; Ali Faghidian, S.; Luciano, R.; Medaglia, C.M.; Penna, R. Free vibrations of FG elastic Timoshenko nano-Beams by strain gradient and stress-Driven nonlocal models. Compos. Part B Eng. 2018, 154, 20–32. [Google Scholar] [CrossRef]
- Romano, G.; Luciano, R.; Barretta, R.; Diaco, M. Nonlocal integral elasticity in nanostructures, mixtures, boundary effects and limit behaviours. Contin. Mechancis Thermodyn. 2018, 30, 641–655. [Google Scholar] [CrossRef]
- Pinnola, F.P.; Ali Faghidian, S.; Barretta, R.; Marotti de Sciarra, F. Variationally consistent dynamics of nonlocal gradient elastic beams. Int. J. Eng. Sci. 2020, 149, 103220. [Google Scholar] [CrossRef]
- Reddy, J.N. A simple higher-Order theory for laminated composite plates. J. Appl. Mech. 1984, 51, 745–752. [Google Scholar] [CrossRef]
- Reddy, J.; Liu, C. A higher-Order shear deformation theory of laminated elastic shells. Int. J. Eng. Sci. 1985, 23, 319–330. [Google Scholar] [CrossRef]
- Reddy, J.N. Energy and Variational Methods in Applied Mechanics: With an Introduction to the Finite Element Method; Wiley: New York, NY, USA, 1984. [Google Scholar]
- Reddy, J.N. An Introduction to the Finite Element Method; McGraw-Hill Book Company: New York, NY, USA, 1984. [Google Scholar]
- Ferreira, A.; Roque, C.; Martins, P. Analysis of composite plates using higher-Order shear deformation theory and a finite point formulation based on the multiquadric radial basis function method. Compos. Part B Eng. 2003, 34, 627–636. [Google Scholar] [CrossRef]
- Ferreira, A.; Roque, C.; Jorge, R. Analysis of composite plates by trigonometric shear deformation theory and multiquadrics. Comput. Struct. 2005, 83, 2225–2237. [Google Scholar] [CrossRef]
- Xiang, S.; Wang, K.-M.; Sha, Y.-D.; Shi, H. Analysis of isotropic, sandwich and laminated plates by a meshless method and various shear deformation theories. Compos. Struct. 2009, 91, 31–37. [Google Scholar] [CrossRef]
- Bhimaraddi, A. Three-Dimensional elasticity solution for static response of orthotropic doubly curved shallow shells on rectangular planform. Compos. Struct. 1993, 24, 67–77. [Google Scholar] [CrossRef]

| Method | |||
|---|---|---|---|
| 10 | Exact [56] | 4.791 | 0.2762 |
| Reddy [57] | 4.77 | 0.2899 | |
| Ferreira et al. [58] | 4.787 | 0.2739 | |
| Ferreira et al. [59] | 4.788 | 0.2762 | |
| Xiang et al. [60] | 4.609 | 0.288 | |
| present | 4.758 | 0.3193 | |
| 20 | Exact [56] | 4.625 | 0.2762 |
| Reddy [57] | 4.57 | 0.2683 | |
| Ferreira et al. [58] | 4.613 | 0.2737 | |
| Ferreira et al. [59] | 4.616 | 0.2749 | |
| Xiang et al. [60] | 4.442 | 0.276 | |
| present | 4.587 | 0.32 | |
| 50 | Exact [56] | 4.579 | 0.2762 |
| Reddy [57] | 4.496 | 0.2667 | |
| Ferreira et al. [58] | 4.575 | 0.2787 | |
| Ferreira et al. [59] | 4.578 | 0.2745 | |
| Xiang et al. [60] | 4.396 | 0.284 | |
| present | 4.55 | 0.3203 | |
| 100 | Exact [56] | 4.572 | 0.2762 |
| Reddy [57] | 4.482 | 0.2664 | |
| Ferreira et al. [58] | 4.573 | 0.2844 | |
| Ferreira et al. [59] | 4.5715 | 0.2744 | |
| Xiang et al. [60] | - | 0.282 | |
| present | 4.5455 | 0.3203 |
| Method | ||||
|---|---|---|---|---|
| 1 | Present | 98.1142 | 7.4312 | 3.877 |
| 3-D [61] | 100.59 | 8.7095 | 4.9497 | |
| PSDT [61] | 99.645 | 7.4751 | 3.8929 | |
| CST [61] | 99.644 | 7.3702 | 3.6979 | |
| 2 | Present | 392.8533 | 16.9774 | 6.8716 |
| 3-D [61] | 396.45 | 18.451 | 7.724 | |
| PSDT [61] | 394.37 | 17.013 | 6.9261 | |
| CST [61] | 394.37 | 16.48 | 6.3322 | |
| 3 | Present | 870.5856 | 22.1878 | 8.0018 |
| 3-D [61] | 875.36 | 23.381 | 8.5912 | |
| PSDT [61] | 872.02 | 22.277 | 8.094 | |
| CST [61] | 872 | 21.371 | 7.2945 | |
| 4 | Present | 1512.3 | 24.8495 | 8.4892 |
| 3-D [61] | 1518.3 | 25.785 | 8.9235 | |
| PSDT [61] | 1513.6 | 24.983 | 8.6017 | |
| CST [61] | 1513.6 | 23.849 | 7.7043 | |
| 5 | Present | 2294.2 | 26.3088 | 8.7352 |
| 3-D [61] | 2301.4 | 27.061 | 9.0755 | |
| PSDT [61] | 2295.4 | 26.471 | 8.8589 | |
| CST [61] | 2295.3 | 25.201 | 7.9099 | |
| 10 | Present | 7370.9 | 28.5417 | 9.0861 |
| 3-D [61] | 7383.1 | 28.91 | 9.2502 | |
| PSDT [61] | 7371.3 | 28.754 | 9.2267 | |
| CST [61] | 7370.2 | 27.262 | 8.2019 | |
| 20 | Present | 16,485 | 29.1602 | 9.1782 |
| 3-D [61] | 16,499 | 29.356 | 9.2666 | |
| PSDT [61] | 16,485 | 29.388 | 9.3235 | |
| CST [61] | 16,479 | 27.831 | 8.2783 | |
| Present | 28,039 | 29.3723 | 9.2094 | |
| 3-D [61] | 29,504 | 29.44 | 9.2352 | |
| PSDT [61] | 28,041 | 29.606 | 9.3562 | |
| CST [61] | 28,026 | 28.026 | 8.304 |
| 0.25 | 5 | 12.0386 | 11.0094 | 30.0962 | 28.7524 | 40.5842 | 38.0363 |
| 10 | 10.9468 | 6.7840 | 22.8961 | 19.6583 | 28.0741 | 27.3571 | |
| 20 | 10.7041 | 6.1901 | 21.6039 | 15.0639 | 26.0654 | 19.1014 | |
| 50 | 10.6381 | 6.0420 | 21.2679 | 14.1387 | 25.5534 | 17.6132 | |
| 100 | 10.6287 | 6.0214 | 21.2207 | 14.0157 | 25.4819 | 17.4193 | |
| plate | 10.6256 | 6.0146 | 21.2050 | 13.9752 | 25.4582 | 17.3556 | |
| 0.5 | 5 | 31.9859 | 18.7949 | 69.4048 | 42.6926 | 90.0968 | 54.7284 |
| 10 | 29.0851 | 11.5815 | 52.8006 | 29.1893 | 62.3243 | 39.3627 | |
| 20 | 28.4402 | 10.5675 | 49.8209 | 22.3674 | 57.8650 | 27.4840 | |
| 50 | 28.2648 | 10.3147 | 49.0459 | 20.9936 | 56.7285 | 25.3427 | |
| 100 | 28.2399 | 10.2796 | 48.9371 | 20.811 | 56.5698 | 25.0637 | |
| plate | 28.2316 | 10.2679 | 48.9010 | 20.7508 | 56.5171 | 24.9721 | |
| 0.75 | 5 | 65.2313 | 31.7707 | 134.9192 | 65.9262 | 172.6177 | 82.5485 |
| 10 | 59.3154 | 19.5772 | 102.6416 | 45.0744 | 119.4080 | 59.3719 | |
| 20 | 58.0004 | 17.8633 | 96.8491 | 34.540 | 110.8644 | 41.4551 | |
| 50 | 57.6426 | 17.4359 | 95.3426 | 32.4185 | 108.6870 | 38.2252 | |
| 100 | 57.5918 | 17.3765 | 95.1312 | 32.1365 | 108.3829 | 37.8044 | |
| plate | 57.5749 | 17.3567 | 95.0609 | 32.0436 | 108.2819 | 37.6662 | |
| 1 | 5 | 111.7749 | 49.9368 | 226.6394 | 98.4534 | 288.1471 | 121.4968 |
| 10 | 101.6379 | 30.7713 | 172.4189 | 67.3136 | 199.3252 | 87.3849 | |
| 20 | 99.3836 | 28.0773 | 162.6887 | 51.5815 | 185.0636 | 61.0145 | |
| 50 | 98.7715 | 27.4055 | 160.1579 | 48.4134 | 181.4289 | 56.2606 | |
| 100 | 98.6845 | 27.3121 | 159.8028 | 47.9923 | 180.9213 | 55.6413 | |
| plate | 98.6555 | 27.2811 | 159.6848 | 47.8535 | 180.7527 | 55.4379 | |
| 0.25 | 5 | 10.9468 | 6.784 | 21.4586 | 14.6529 | 25.5534 | 17.6132 |
| 10 | 10.7041 | 6.1901 | 21.2679 | 14.1387 | 25.4819 | 17.4193 | |
| 20 | 10.6451 | 6.0575 | 21.2207 | 14.0157 | 25.4641 | 17.3715 | |
| 50 | 10.6287 | 6.0214 | 21.2076 | 13.9816 | 25.4592 | 17.3582 | |
| 100 | 10.6264 | 6.0163 | 21.2057 | 13.9768 | 25.4584 | 17.3563 | |
| plate | 10.6256 | 6.0146 | 21.205 | 13.9752 | 25.4582 | 17.3556 | |
| 0.5 | 5 | 29.0851 | 11.5815 | 49.4858 | 21.7572 | 56.7285 | 25.3427 |
| 10 | 28.4402 | 10.5675 | 49.0459 | 20.9936 | 56.5698 | 25.0637 | |
| 20 | 28.2835 | 10.3412 | 48.9371 | 20.811 | 56.5303 | 24.9949 | |
| 50 | 28.2399 | 10.2796 | 48.9068 | 20.7604 | 56.5192 | 24.9757 | |
| 100 | 28.2337 | 10.2708 | 48.9024 | 20.7532 | 56.5176 | 24.973 | |
| plate | 28.2316 | 10.2679 | 48.901 | 20.7508 | 56.5171 | 24.9721 | |
| 0.75 | 5 | 59.3154 | 19.5772 | 96.1977 | 33.5977 | 108.687 | 38.2252 |
| 10 | 58.0004 | 17.8633 | 95.3426 | 32.4185 | 108.3829 | 37.8044 | |
| 20 | 57.6807 | 17.4807 | 95.1312 | 32.1365 | 108.3072 | 37.7006 | |
| 50 | 57.5918 | 17.3765 | 95.0721 | 32.0584 | 108.286 | 37.6717 | |
| 100 | 57.5791 | 17.3617 | 95.0637 | 32.0473 | 108.2829 | 37.6675 | |
| plate | 57.5749 | 17.3567 | 95.0609 | 32.0436 | 108.2819 | 37.6662 | |
| 1 | 5 | 101.6379 | 30.7713 | 161.5943 | 50.1744 | 181.4289 | 56.2606 |
| 10 | 99.3846 | 28.0773 | 160.1579 | 48.4134 | 180.9213 | 55.6413 | |
| 20 | 98.8368 | 27.4759 | 159.8028 | 47.9923 | 180.7948 | 55.4886 | |
| 50 | 98.6845 | 27.3121 | 159.7037 | 47.8757 | 180.7594 | 55.446 | |
| 100 | 98.6628 | 27.2889 | 159.6895 | 47.8591 | 180.7544 | 55.4399 | |
| plate | 98.6555 | 27.2811 | 159.6848 | 47.8535 | 180.7527 | 55.4379 | |
| 0 | 5.2322 | 4.2624 | 12.0728 | 9.2637 | 14.8348 | 11.0326 |
| 0.1 | 6.265 | 5.1038 | 13.5622 | 10.4066 | 16.4617 | 12.2425 |
| 0.2 | 9.3633 | 7.6279 | 18.0305 | 13.8352 | 21.3421 | 15.872 |
| 0.3 | 14.5273 | 11.8347 | 25.4776 | 19.5495 | 29.4762 | 21.9213 |
| 0.4 | 21.7568 | 17.7243 | 35.9036 | 27.5496 | 40.864 | 30.3903 |
| 0.5 | 31.0519 | 25.2966 | 49.3085 | 37.8354 | 55.5054 | 41.2791 |
| 0.6 | 42.4126 | 34.5516 | 65.6921 | 50.4069 | 73.4004 | 54.5875 |
| 0.7 | 55.8388 | 45.4894 | 85.0547 | 65.2642 | 94.5491 | 70.3157 |
| 0.8 | 71.3306 | 58.1099 | 107.4 | 82.4072 | 118.95 | 88.4636 |
| 0.9 | 88.888 | 72.4131 | 132.72 | 101.8359 | 146.61 | 109.0312 |
| 1 | 108.51 | 88.399 | 161.02 | 123.5504 | 177.52 | 132.0185 |
| 1.1 | 130.2 | 106.0677 | 192.29 | 147.5506 | 211.68 | 157.4255 |
| 1.2 | 153.95 | 125.4192 | 226.55 | 173.8365 | 249.1 | 185.2523 |
| 1.3 | 179.77 | 146.4533 | 263.79 | 202.4082 | 289.77 | 215.4988 |
| 1.4 | 207.66 | 169.1702 | 304.14 | 233.2656 | 333.69 | 248.165 |
| 1.5 | 237.61 | 193.5698 | 347.19 | 266.4088 | 380.87 | 283.2509 |
| 1.6 | 269.63 | 219.6522 | 393.37 | 301.8376 | 431.3 | 320.7565 |
| 1.7 | 303.71 | 247.4173 | 442.52 | 339.5522 | 484.99 | 360.6818 |
| 1.8 | 339.86 | 276.8651 | 494.65 | 379.5526 | 541.93 | 403.0269 |
| 1.9 | 378.07 | 307.9957 | 549.76 | 421.8386 | 602.12 | 447.7917 |
| 2 | 418.35 | 340.8089 | 607.84 | 466.4104 | 665.56 | 494.9762 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dindarloo, M.H.; Li, L.; Dimitri, R.; Tornabene, F. Nonlocal Elasticity Response of Doubly-Curved Nanoshells. Symmetry 2020, 12, 466. https://doi.org/10.3390/sym12030466
Dindarloo MH, Li L, Dimitri R, Tornabene F. Nonlocal Elasticity Response of Doubly-Curved Nanoshells. Symmetry. 2020; 12(3):466. https://doi.org/10.3390/sym12030466
Chicago/Turabian StyleDindarloo, Mohammad Hassan, Li Li, Rossana Dimitri, and Francesco Tornabene. 2020. "Nonlocal Elasticity Response of Doubly-Curved Nanoshells" Symmetry 12, no. 3: 466. https://doi.org/10.3390/sym12030466
APA StyleDindarloo, M. H., Li, L., Dimitri, R., & Tornabene, F. (2020). Nonlocal Elasticity Response of Doubly-Curved Nanoshells. Symmetry, 12(3), 466. https://doi.org/10.3390/sym12030466








