A Simplified Method to Avoid Shadows at Parabolic-Trough Solar Collectors Facilities
Abstract
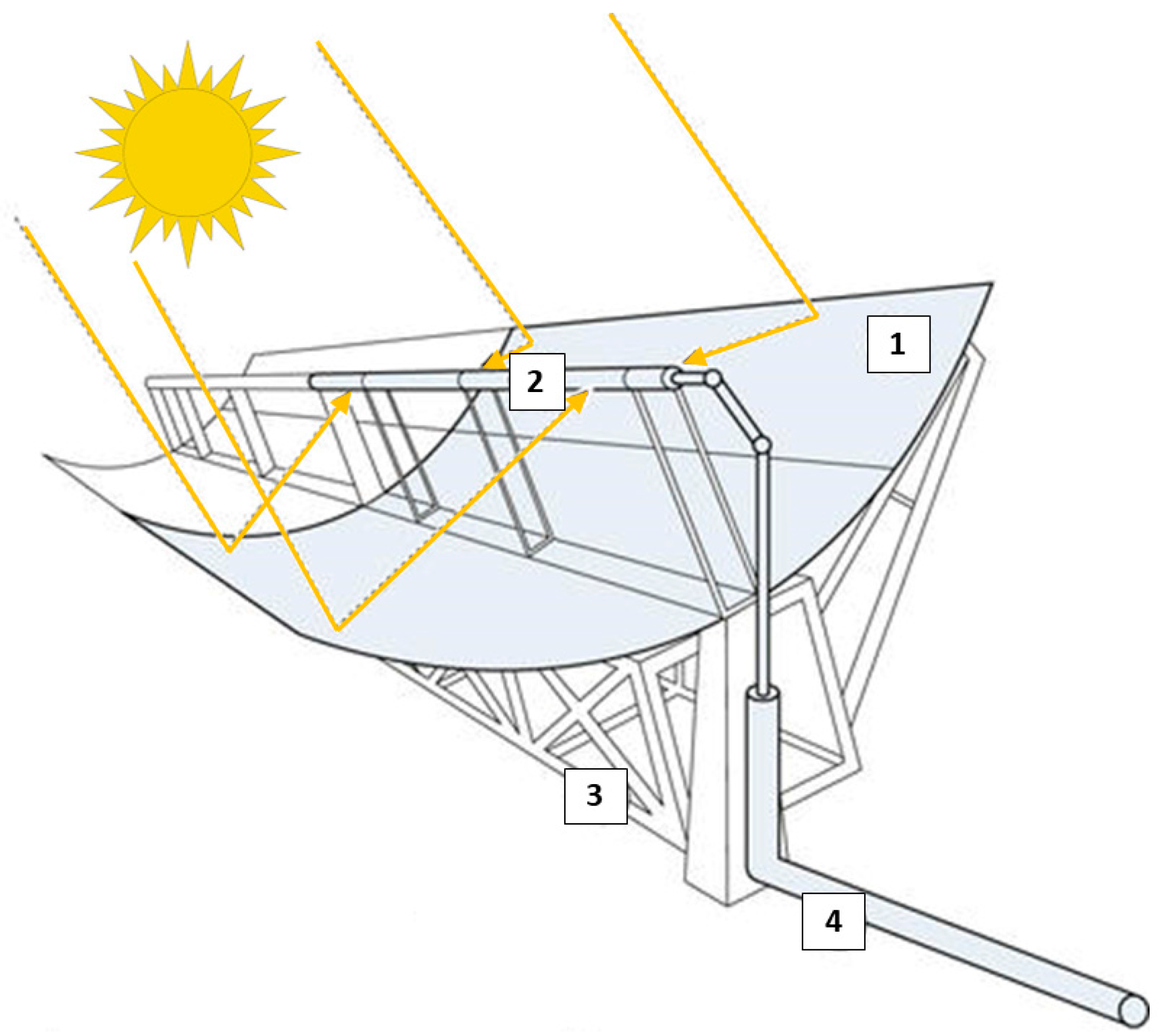
1. Introduction
2. Classical Methods for the Sizing of PTC: A Brief Overview
3. Standard Methods for Determining the Spacing between Collectors in PTC Facilities
3.1. Standard Method 1
3.2. Standard Method 2
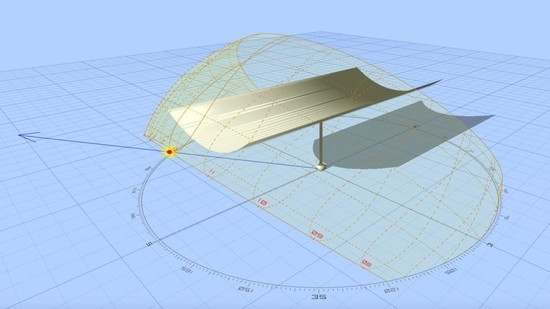
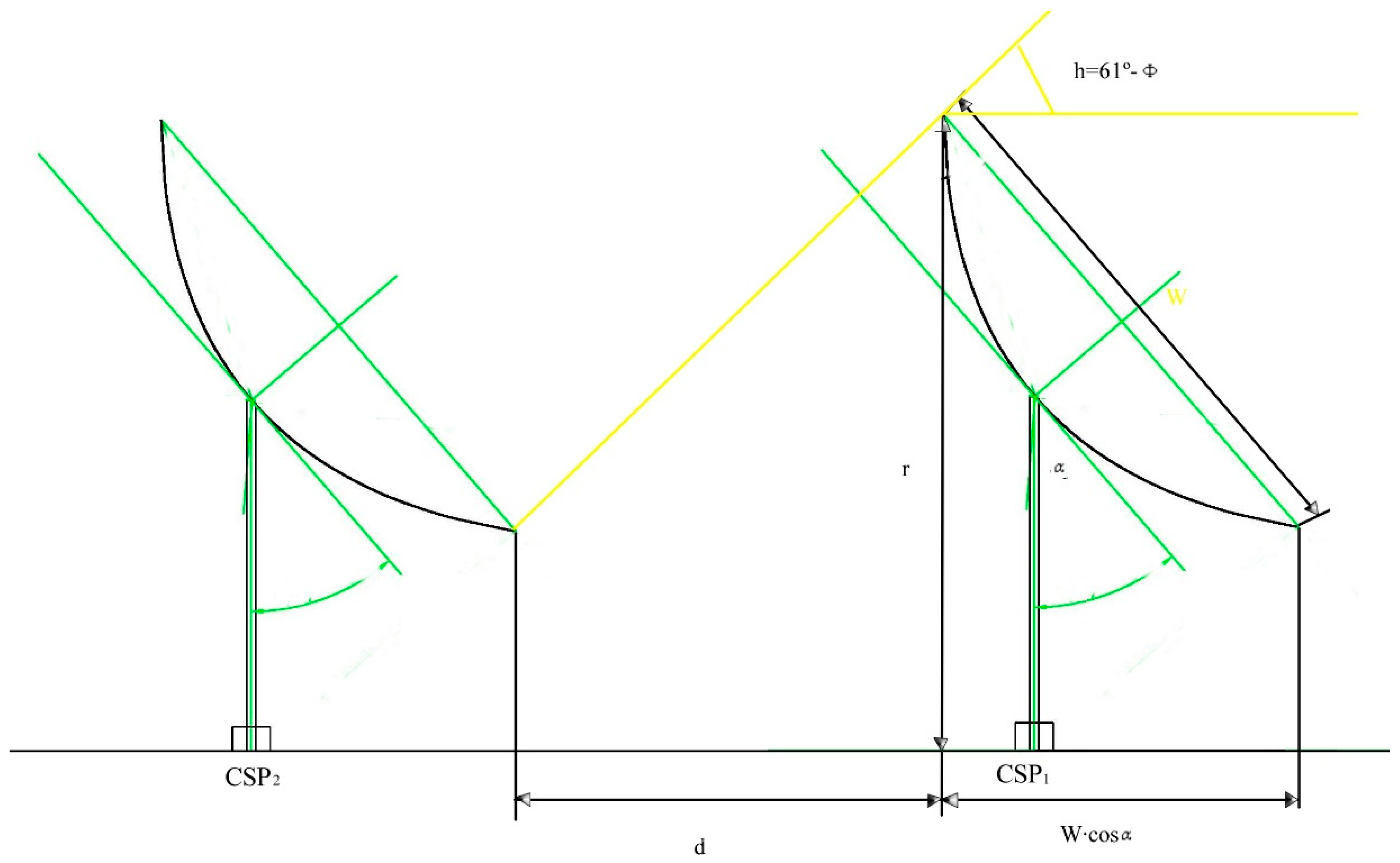
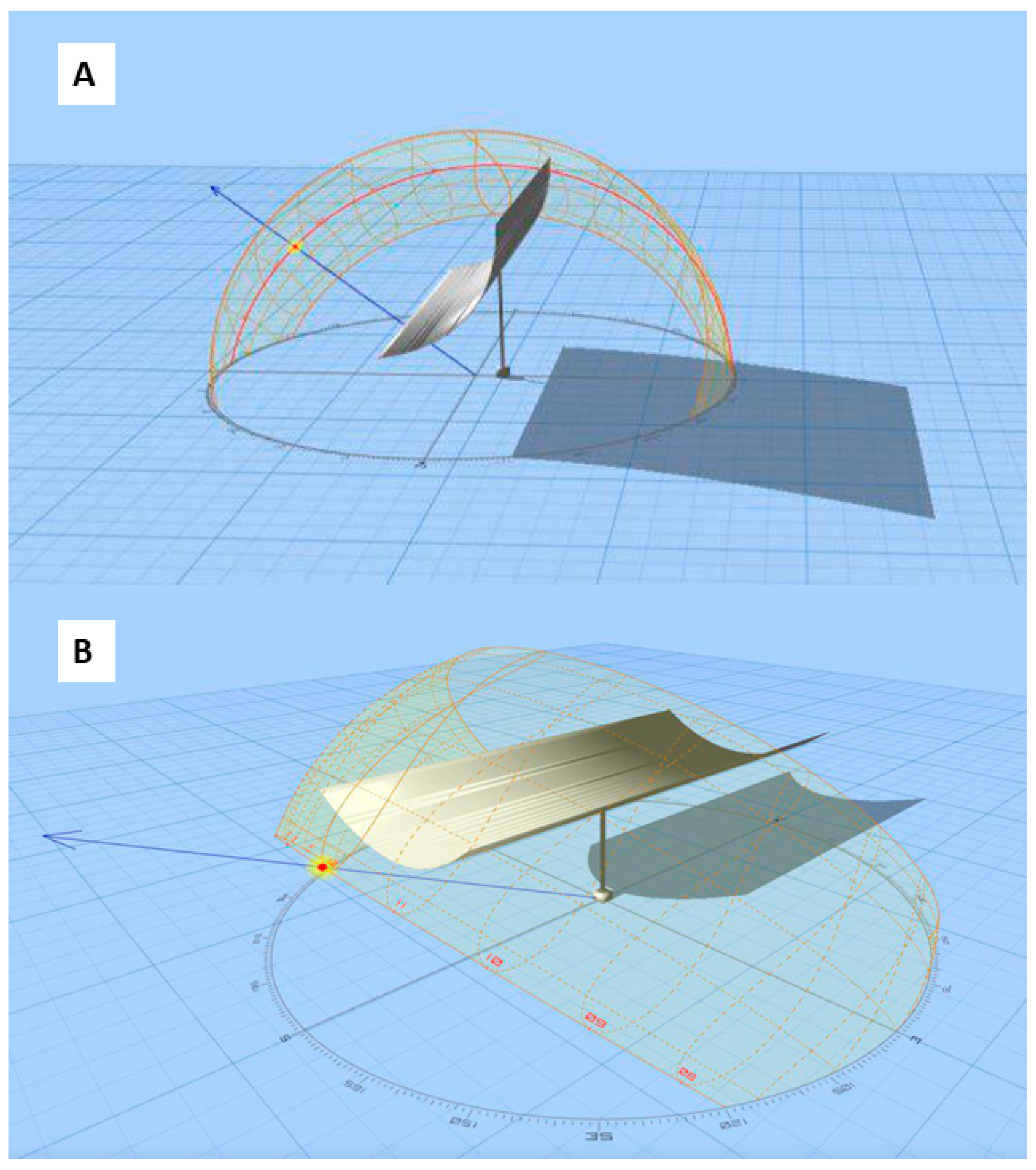
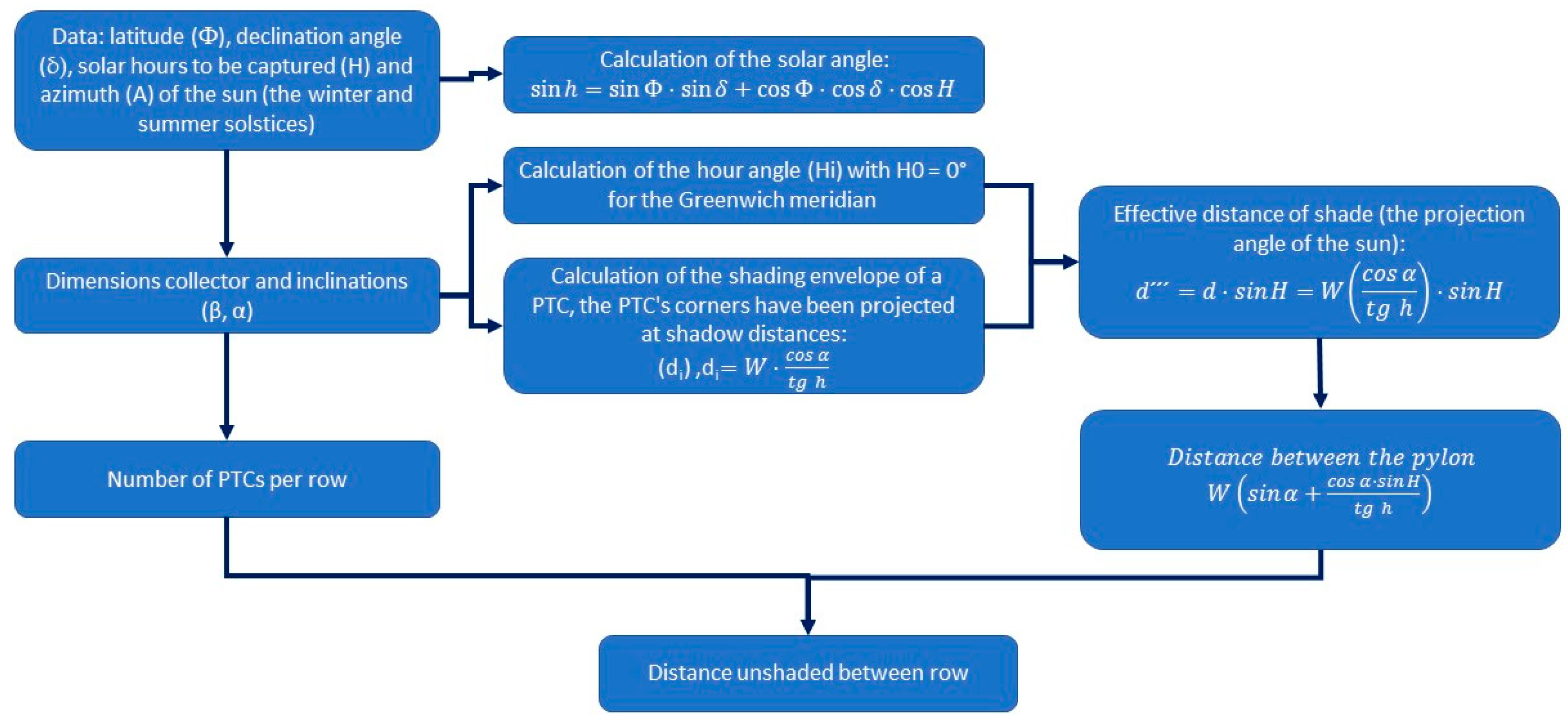
4. Proposed Method
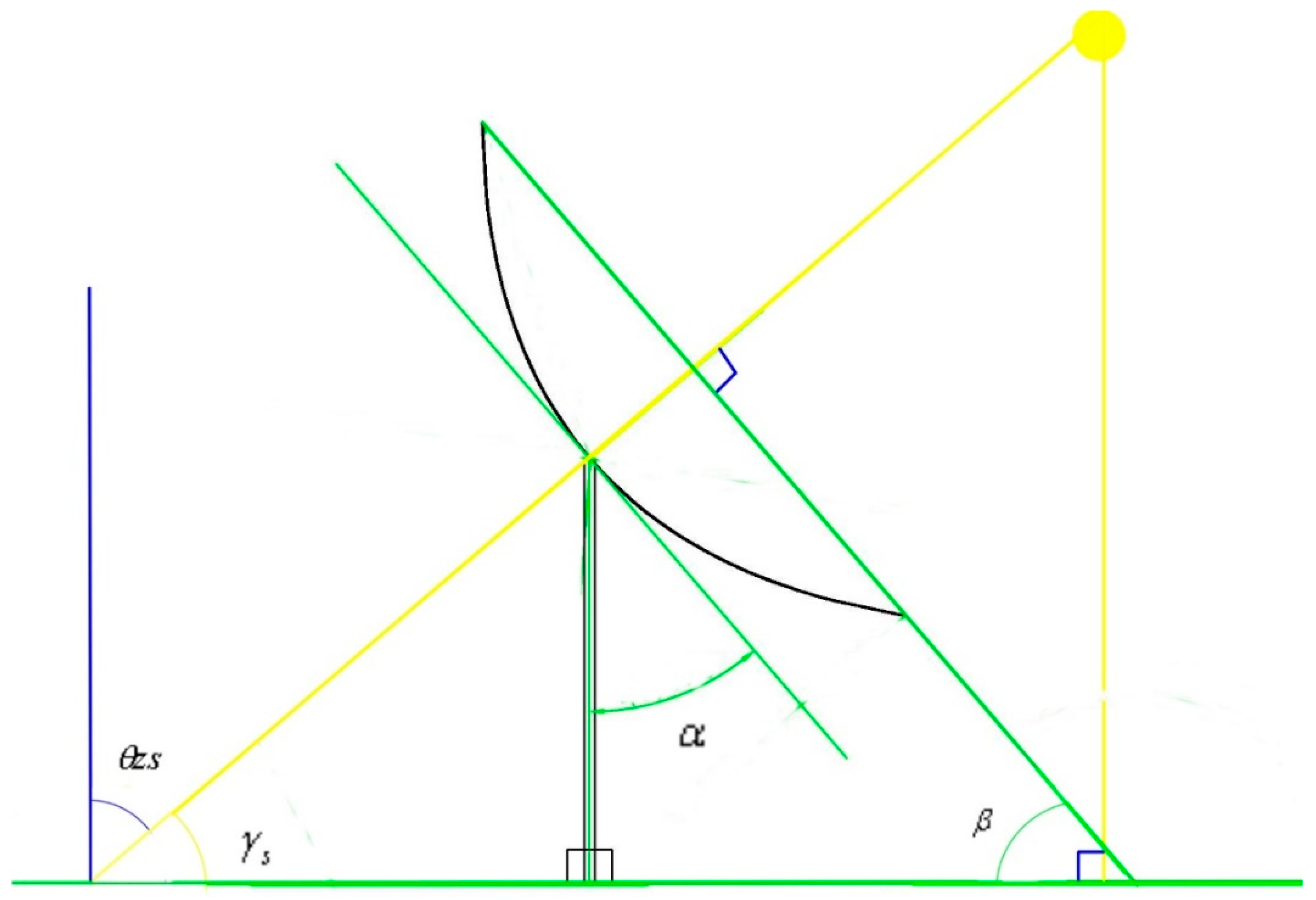
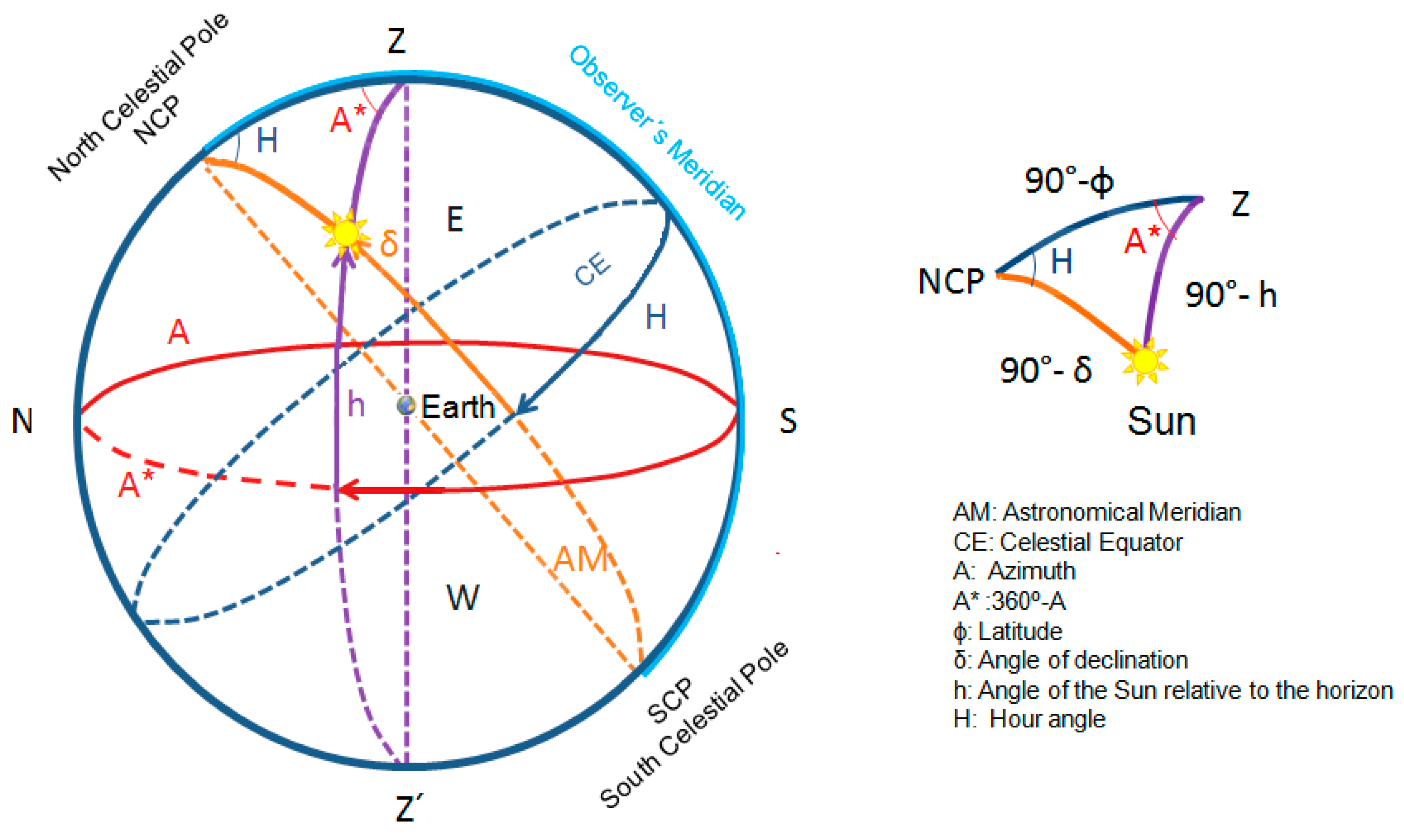
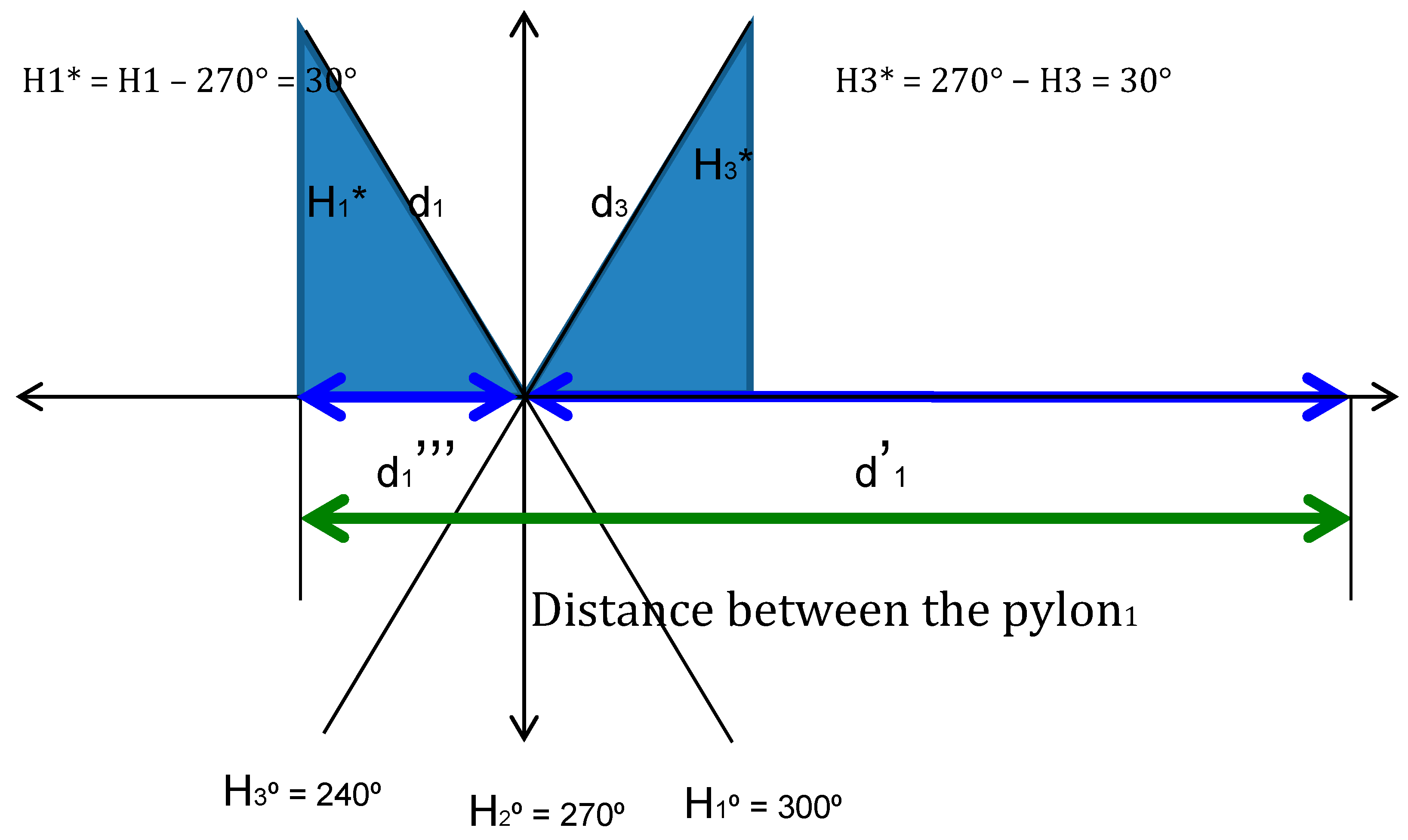
4.1. Solar Angle Calculation
- (90° − δ) ⇒ hour angle (H)
- (90° − Φ) ⇒ the azimuth supplement (A*)
- (90° − h) ⇒ solar height (h)
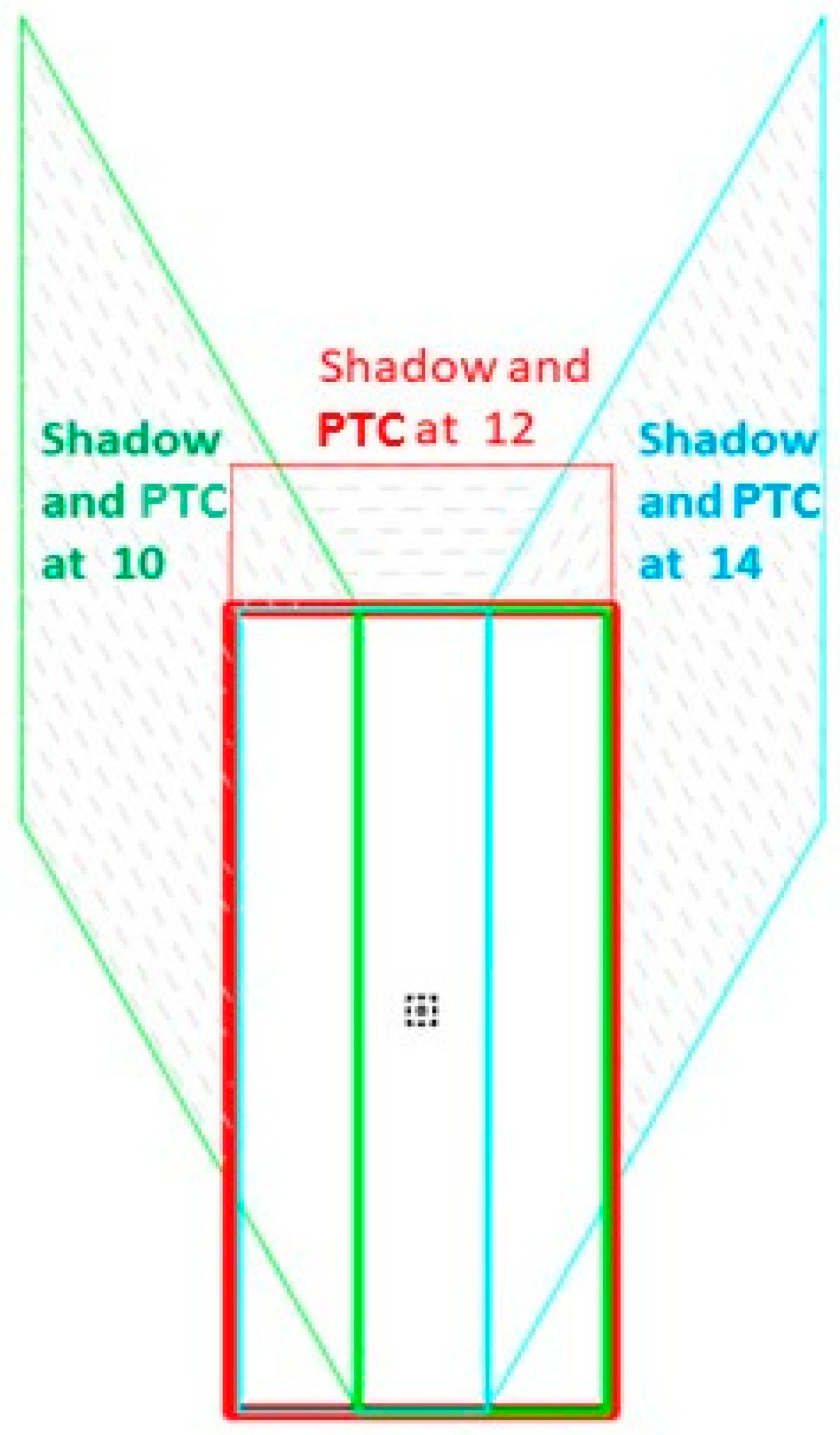
4.2. The Extent of the Shade
5. Results and Discussion
5.1. Case Study: Results
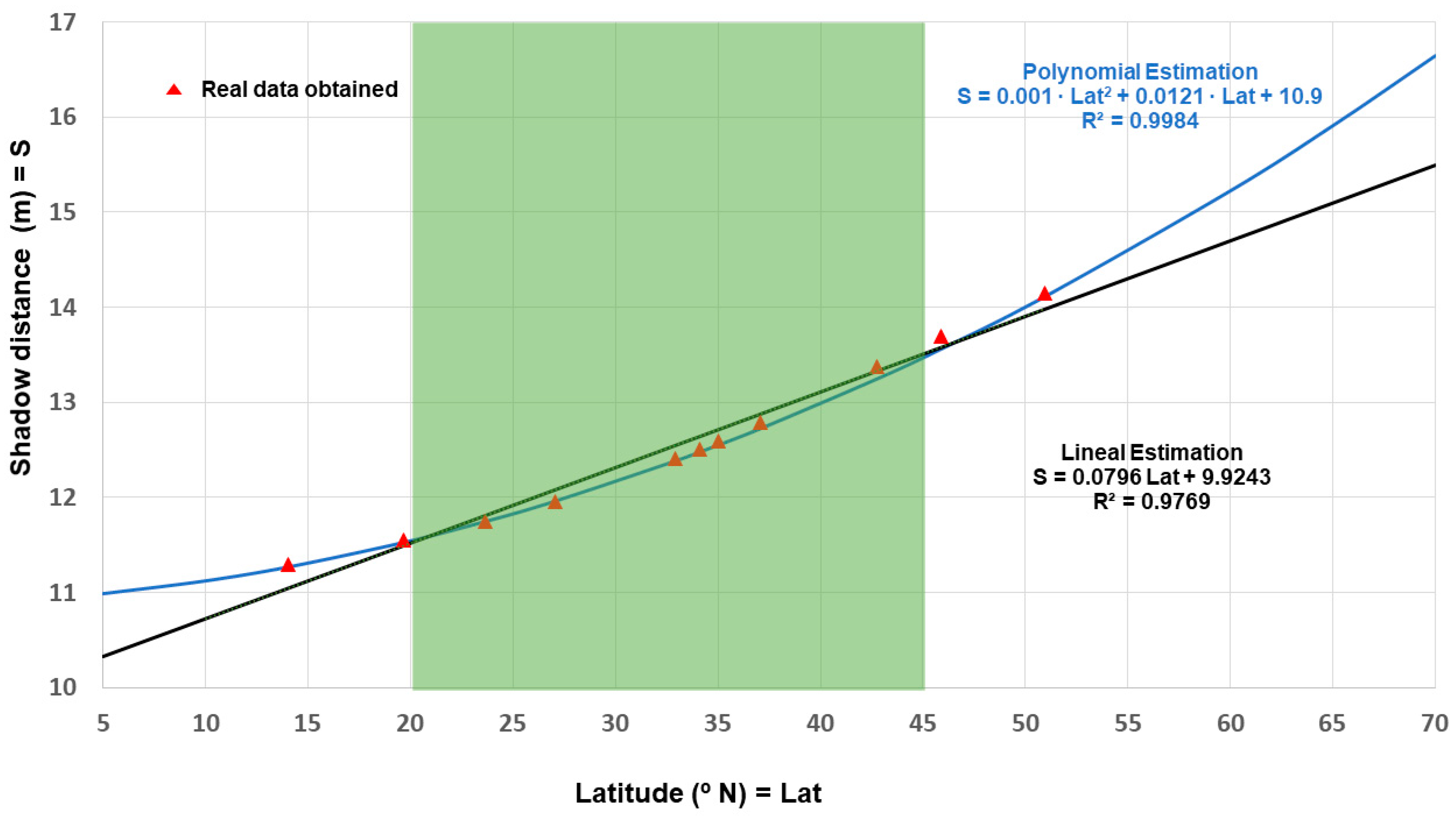
5.2. Extension of the Case Study to Worldwide
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Baños, R.; Manzano-Agugliaro, F.; Montoya, F.; Gil, C.; Alcayde, A.; Gómez, J. Optimization methods applied to renewable and sustainable energy: A review. Renew. Sustain. Energy Rev. 2011, 15, 1753–1766. [Google Scholar] [CrossRef]
- Schaeffer, M.; Hare, W.; Rahmstorf, S.; Vermeer, M. Long-term sea-level rise implied by 1.5 °C and 2 °C warming levels. Nat. Clim. Chang. 2012, 2, 867–870. [Google Scholar] [CrossRef]
- Meyers, S.; Schmitt, B.; Vajen, K. The future of low carbon industrial process heat: A comparison between solar thermal and heat pumps. Sol. Energy 2018, 173, 893–904. [Google Scholar] [CrossRef]
- Miao, C.; Fang, D.; Sun, L.; Luo, Q.; Yu, Q. Driving effect of technology innovation on energy utilization efficiency in strategic emerging industries. J. Clean. Prod. 2018, 170, 1177–1184. [Google Scholar] [CrossRef]
- Alfaris, F.; Juaidi, A.; Manzano-Agugliaro, F. Intelligent homes’ technologies to optimize the energy performance for the net zero energy home. Energy Build. 2017, 153, 262–274. [Google Scholar] [CrossRef]
- Massidda, L.; Marrocu, M. Decoupling Weather Influence from User Habits for an Optimal Electric Load Forecast System. Energies 2017, 10, 2171. [Google Scholar] [CrossRef]
- Massidda, L.; Marrocu, M. Use of Multilinear Adaptive Regression Splines and numerical weather prediction to forecast the power output of a PV plant in Borkum, Germany. Sol. Energy 2017, 146, 141–149. [Google Scholar] [CrossRef]
- Islam, T.; Huda, N.; Abdullah, A.; Saidur, R. A comprehensive review of state-of-the-art concentrating solar power (CSP) technologies: Current status and research trends. Renew. Sustain. Energy Rev. 2018, 91, 987–1018. [Google Scholar] [CrossRef]
- Hernandez-Escobedo, Q.; Rodriguez-Garcia, E.; Saldaña-Flores, R.; Fernández-García, A.; Manzano-Agugliaro, F. Solar energy resource assessment in Mexican states along the Gulf of Mexico. Renew. Sustain. Energy Rev. 2015, 43, 216–238. [Google Scholar] [CrossRef]
- Sansaniwal, S.K.; Sharma, V.; Mathur, J. Energy and exergy analyses of various typical solar energy applications: A comprehensive review. Renew. Sustain. Energy Rev. 2018, 82, 1576–1601. [Google Scholar] [CrossRef]
- Sengupta, M.; Xie, Y.; Lopez, A.; Habte, A.; Maclaurin, G.; Shelby, J. The National Solar Radiation Data Base (NSRDB). Renew. Sustain. Energy Rev. 2018, 89, 51–60. [Google Scholar] [CrossRef]
- Manzano-Agugliaro, F.; Alcayde, A.; Montoya, F.; Zapata-Sierra, A.J.; Gil, C. Scientific production of renewable energies worldwide: An overview. Renew. Sustain. Energy Rev. 2013, 18, 134–143. [Google Scholar] [CrossRef]
- Cruz-Peragón, F.; Palomar, J.; Casanova, P.; Dorado, M.; Manzano-Agugliaro, F. Characterization of solar flat plate collectors. Renew. Sustain. Energy Rev. 2012, 16, 1709–1720. [Google Scholar] [CrossRef]
- Behar, O.; Khellaf, A.; Mohammedi, K. A review of studies on central receiver solar thermal power plants. Renew. Sustain. Energy Rev. 2013, 23, 12–39. [Google Scholar] [CrossRef]
- Fernández-García, A.; Rojas, E.; Pérez, M.; Silva, R.; Hernandez-Escobedo, Q.; Manzano-Agugliaro, F.; Pérez-García, M. A parabolic-trough collector for cleaner industrial process heat. J. Clean. Prod. 2015, 89, 272–285. [Google Scholar] [CrossRef]
- Sutter, F.; Fernández-García, A.; Wette, J.; Reche-Navarro, T.J.; Martínez-Arcos, L. Acceptance criteria for accelerated aging testing of silvered-glass mirrors for concentrated solar power technologies. Sol. Energy Mater. Sol. Cells 2019, 193, 361–371. [Google Scholar] [CrossRef]
- Kasaeian, A.; Nouri, G.; Ranjbaran, P.; Wen, D. Solar collectors and photovoltaics as combined heat and power systems: A critical review. Energy Convers. Manag. 2018, 156, 688–705. [Google Scholar] [CrossRef]
- García-Segura, A.; Fernández-García, A.; Ariza, M.; Sutter, F.; Diamantino, T.; Martínez-Arcos, L.; Reche-Navarro, T.; Valenzuela, L. Influence of gaseous pollutants and their synergistic effects on the aging of reflector materials for concentrating solar thermal technologies. Sol. Energy Mater. Sol. Cells 2019, 200, 109955. [Google Scholar] [CrossRef]
- Jin, J.; Ling, Y.; Hao, Y. Similarity analysis of parabolic-trough solar collectors. Appl. Energy 2017, 204, 958–965. [Google Scholar] [CrossRef]
- Bellos, E.; Tzivanidis, C. Enhancing the performance of a parabolic trough collector with combined thermal and optical techniques. Appl. Therm. Eng. 2020, 164, 114496. [Google Scholar] [CrossRef]
- Asociación Española para la Promoción de la Industria Termosolar. Informe de Transición del sector Eléctrico Horizonte 2030. 2018. Available online: http://www.protermosolar.com (accessed on 10 December 2019).
- Bellos, E.; Tzivanidis, C. Alternative designs of parabolic trough solar collectors. Prog. Energy Combust. Sci. 2019, 71, 81–117. [Google Scholar] [CrossRef]
- Bellos, E.; Tzivanidis, C. Investigation of a nanofluid-based concentrating thermal photovoltaic with a parabolic reflector. Energy Convers. Manag. 2019, 180, 171–182. [Google Scholar] [CrossRef]
- Fernández-García, A.; Juaidi, A.; Sutter, F.; Martínez-Arcos, L.; Manzano-Agugliaro, F. Solar Reflector Materials Degradation Due to the Sand Deposited on the Backside Protective Paints. Energies 2018, 11, 808. [Google Scholar] [CrossRef]
- Sánchez-Lozano, J.; García-Cascales, M.; Lamata, M. Evaluation of suitable locations for the installation of solar thermoelectric power plants. Comput. Ind. Eng. 2015, 87, 343–355. [Google Scholar] [CrossRef]
- Kalogirou, S. Solar Energy Engineering, Processes and Systems; Academic Press: New York, NY, USA, 2014; 819p, ISBN 978-0-12-397270-5. [Google Scholar]
- IDEA (Instituto para la Diversificación y Ahorro de la Energía). Anexo III. Cálculo de pérdidas de radiación solar por sombras. In Instalaciones de Energía Solar Térmica, Pliego de Condiciones Técnicas de Instalaciones de Baja Temperatura; IDEA: Madrid, Spain, 2011; pp. 40–44. [Google Scholar]
- Castellano, N.N.; Parra, J.A.G.; Valls-Guirado, J.; Manzano-Agugliaro, F. Optimal displacement of photovoltaic array’s rows using a novel shading model. Appl. Energy 2015, 144, 1–9. [Google Scholar] [CrossRef]
- Fernández-García, A.; Zarza, E.; Valenzuela, L.; Perez, M. Parabolic-trough solar collectors and their applications. Renew. Sustain. Energy Rev. 2010, 14, 1695–1721. [Google Scholar] [CrossRef]
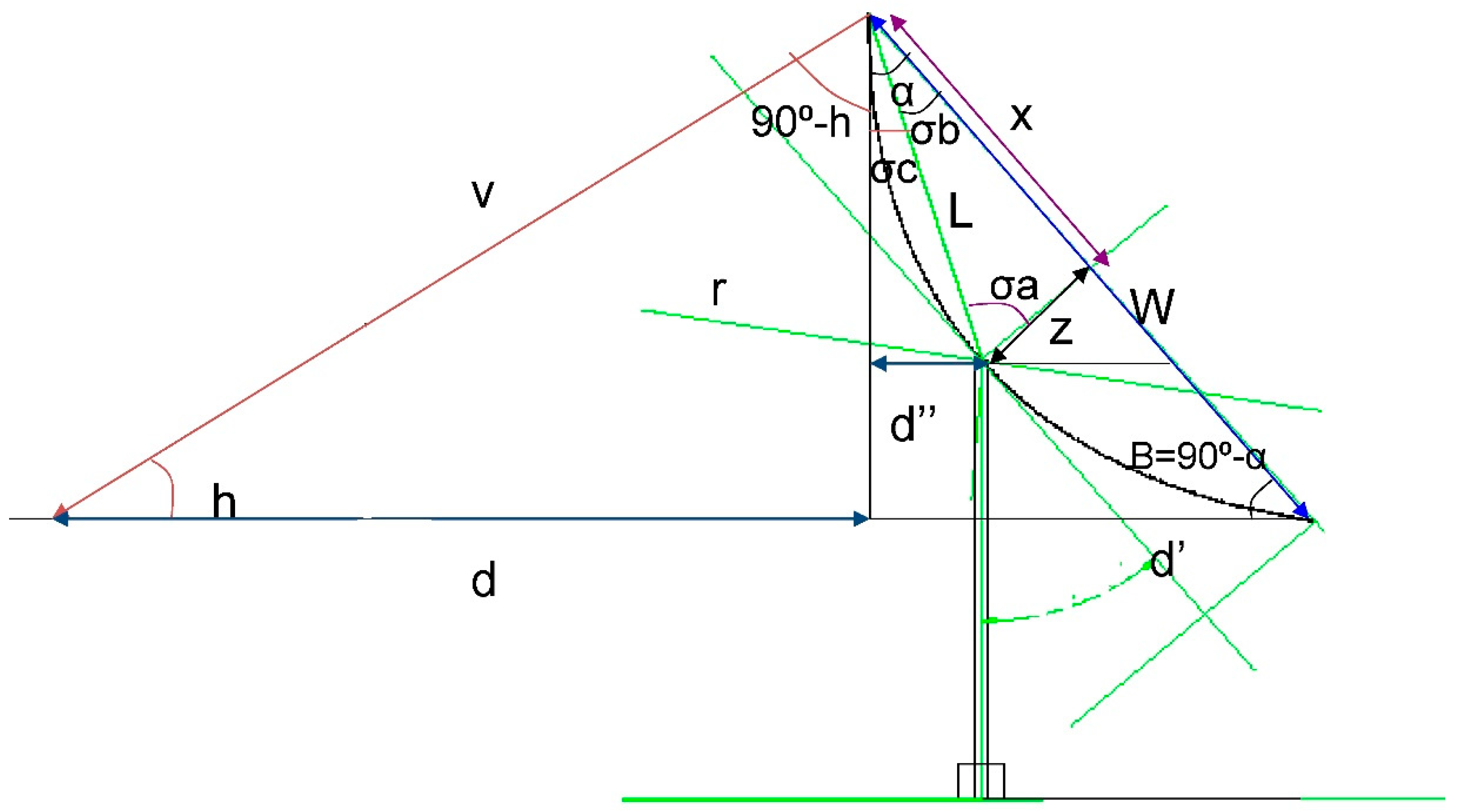

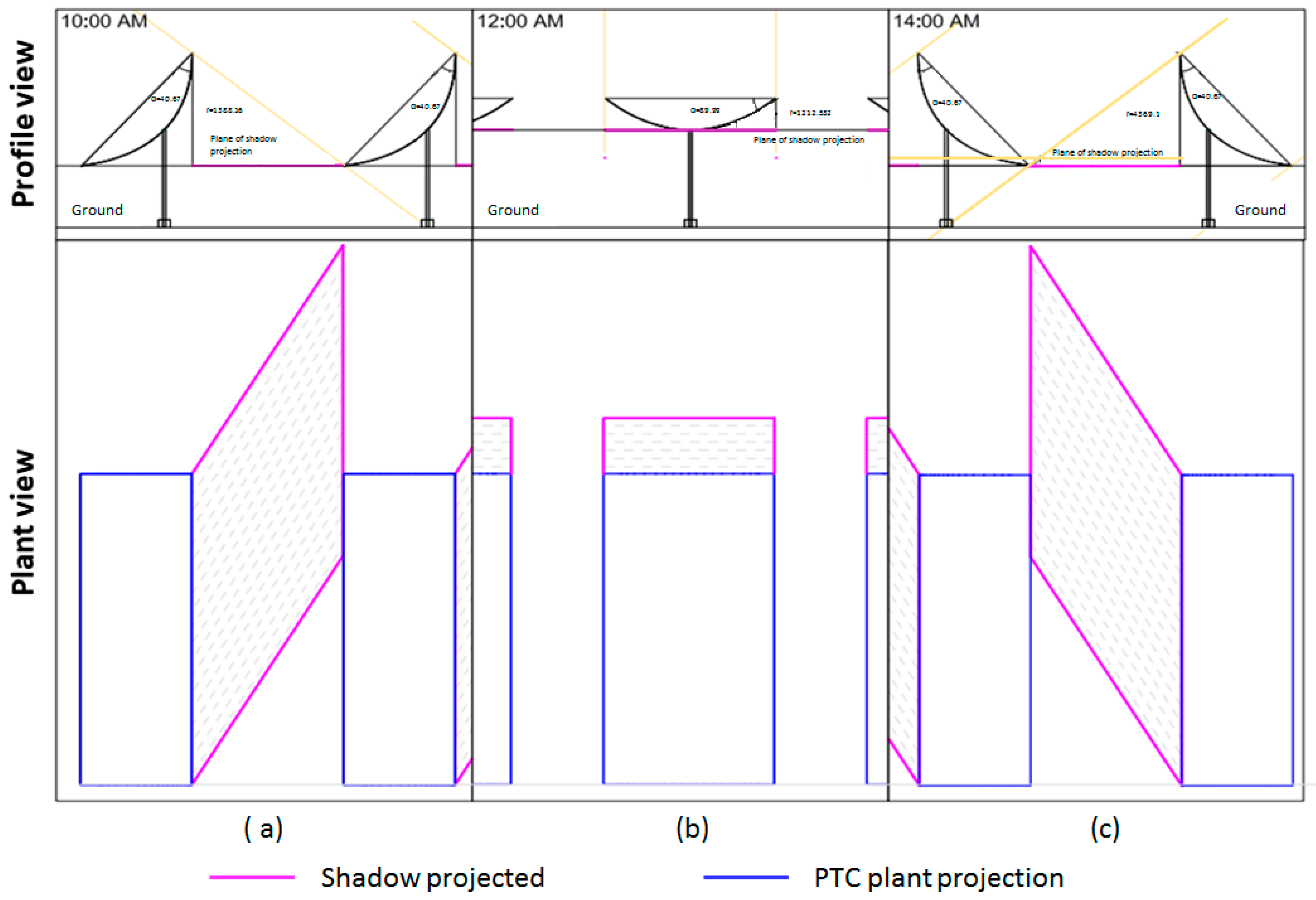
| Solar Hour | Time Angle (° (H) | Elevation or Solar Height (°) (h) | Flat Tilt Opening (°) (α) | Distance between Pylons (m) d‴ + d′ = W (sinα + cosα (sin H/tg h)) |
|---|---|---|---|---|
| 10:00:00 | 330.000 | 0.416 | 40.666 | 8.847 |
| 12:00:00 | 300.000 | 0.500 | 89.999 | 5.760 |
| 14:00:00 | 270.000 | 0.583 | 40.665 | 8.847 |
| Country | Emplacement | Latitude (° N) | Solar Hour (2 h after Sunrise) | Calculated Shadow Distance d‴ + d′ = W (sinα + cosα (sin H/tg h)) (m) |
|---|---|---|---|---|
| Thailand | Kanchanaburi | 14.022 | 8:25:07 | 11.294 |
| USA | Kailua-Kona (Hawai) | 19.639 | 8:35:43 | 11.546 |
| UEA | Medinat Zayed (Abu Dabi) | 23.660 | 8:43:00 | 11.749 |
| USA | Indiantown (Florida) | 27.027 | 8:51:07 | 11.957 |
| Algeria | HassiR’mel | 32.928 | 9:05:07 | 12.407 |
| Morocco | Ain Beni Mathar (Oujda) | 34.088 | 9:08:00 | 12.508 |
| USA | Mojave Desert (California) | 35.031 | 9:10:00 | 12.593 |
| Spain | Almeria | 37.051 | 9:16:25 | 12.787 |
| Italy | Massa Martana | 42.776 | 10:08:59 | 13.374 |
| Canada | Kingsey Falls (Québec) | 45.860 | 9:46:00 | 13.699 |
| Germany | Jülich | 50.922 | 10:08:59 | 14.147 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Novas, N.; Fernández-García, A.; Manzano-Agugliaro, F. A Simplified Method to Avoid Shadows at Parabolic-Trough Solar Collectors Facilities. Symmetry 2020, 12, 278. https://doi.org/10.3390/sym12020278
Novas N, Fernández-García A, Manzano-Agugliaro F. A Simplified Method to Avoid Shadows at Parabolic-Trough Solar Collectors Facilities. Symmetry. 2020; 12(2):278. https://doi.org/10.3390/sym12020278
Chicago/Turabian StyleNovas, Nuria, Aránzazu Fernández-García, and Francisco Manzano-Agugliaro. 2020. "A Simplified Method to Avoid Shadows at Parabolic-Trough Solar Collectors Facilities" Symmetry 12, no. 2: 278. https://doi.org/10.3390/sym12020278
APA StyleNovas, N., Fernández-García, A., & Manzano-Agugliaro, F. (2020). A Simplified Method to Avoid Shadows at Parabolic-Trough Solar Collectors Facilities. Symmetry, 12(2), 278. https://doi.org/10.3390/sym12020278