Breaking Symmetry: Fluctuating Asymmetry and Geometric Morphometrics as Tools for Evaluating Developmental Instability under Diverse Agroecosystems
Abstract
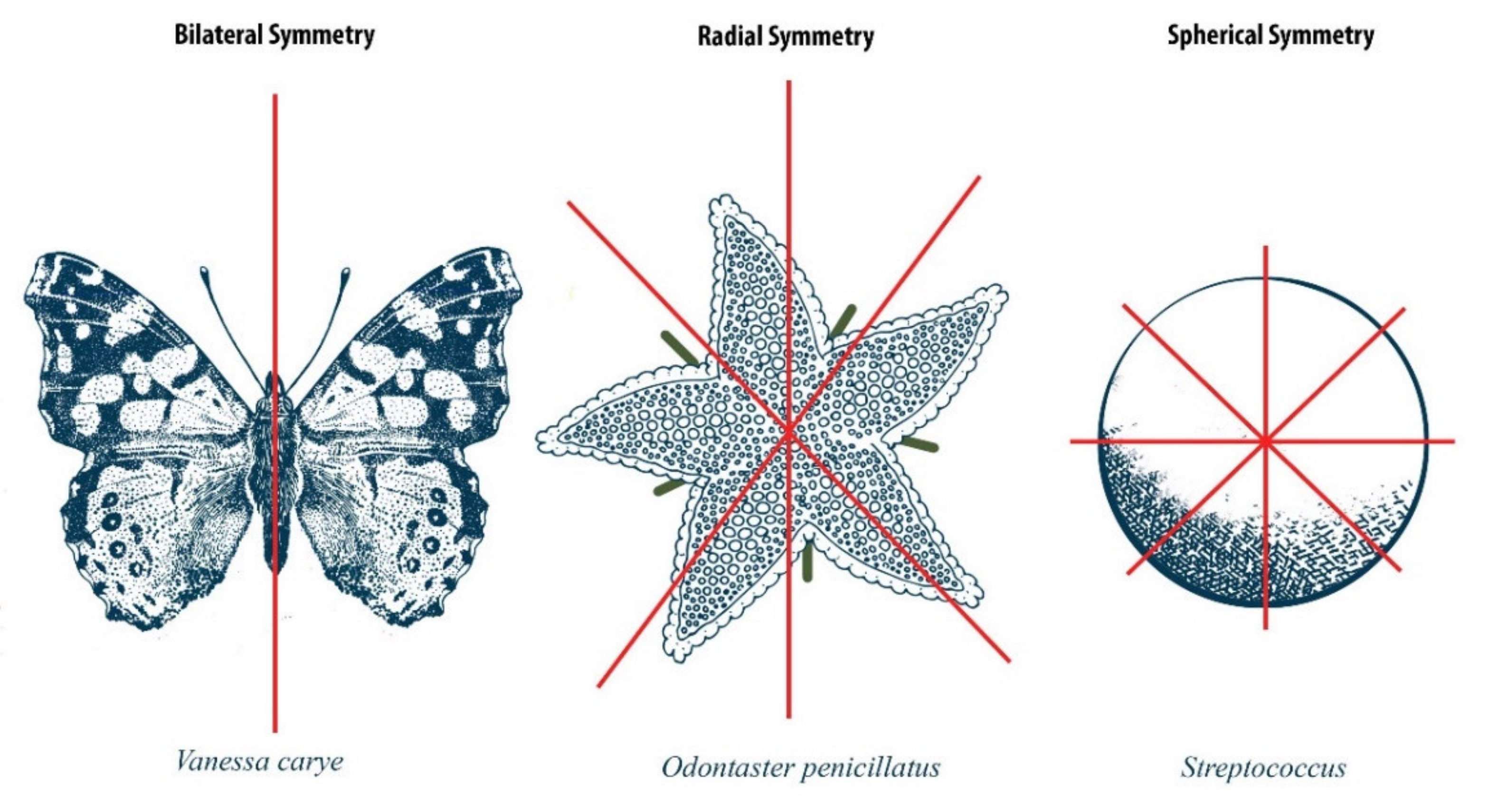
1. Introduction

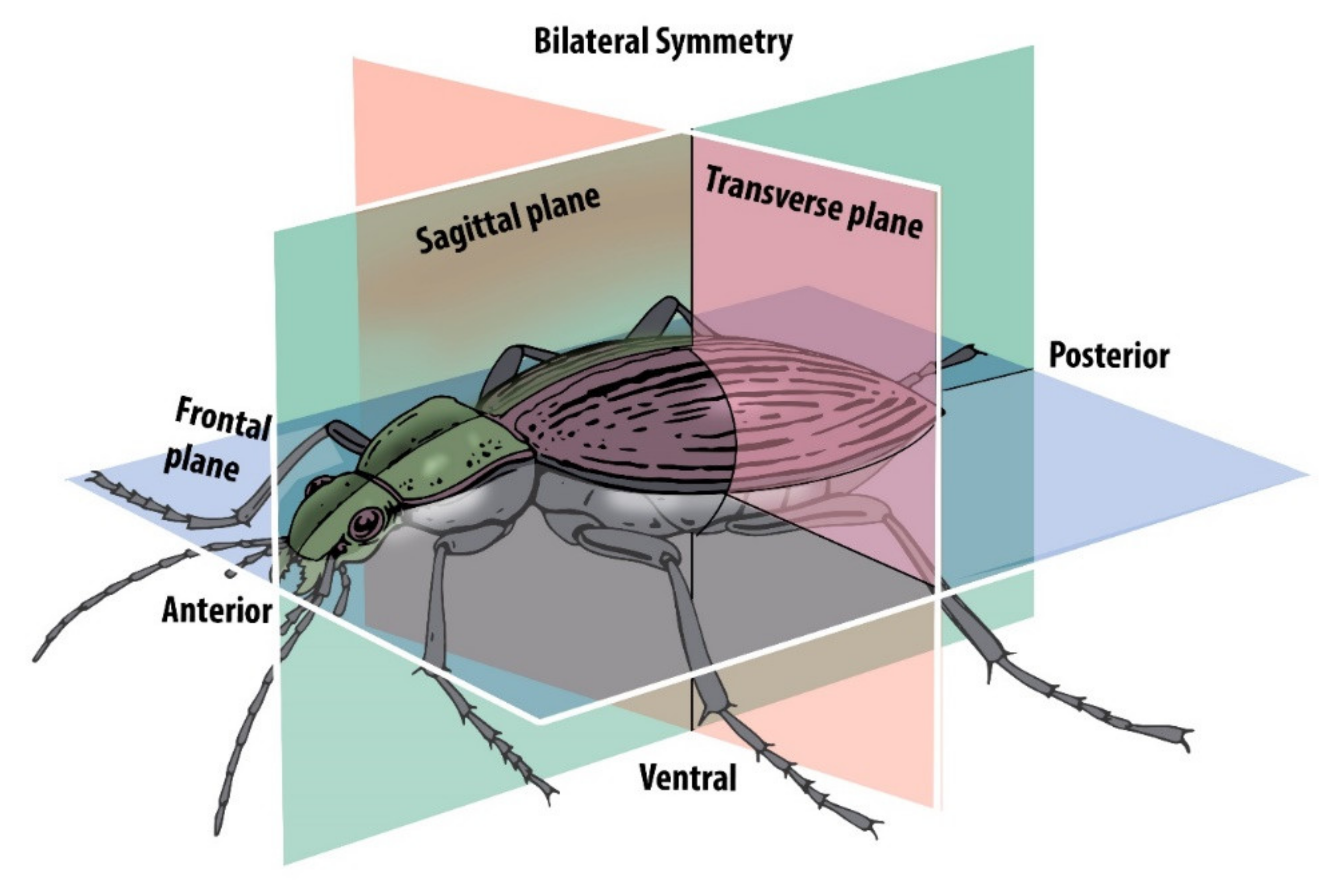
2. Traditional Morphometrics and Fluctuating Asymmetry
3. Fluctuating Asymmetry Using Geometric Morphometrics
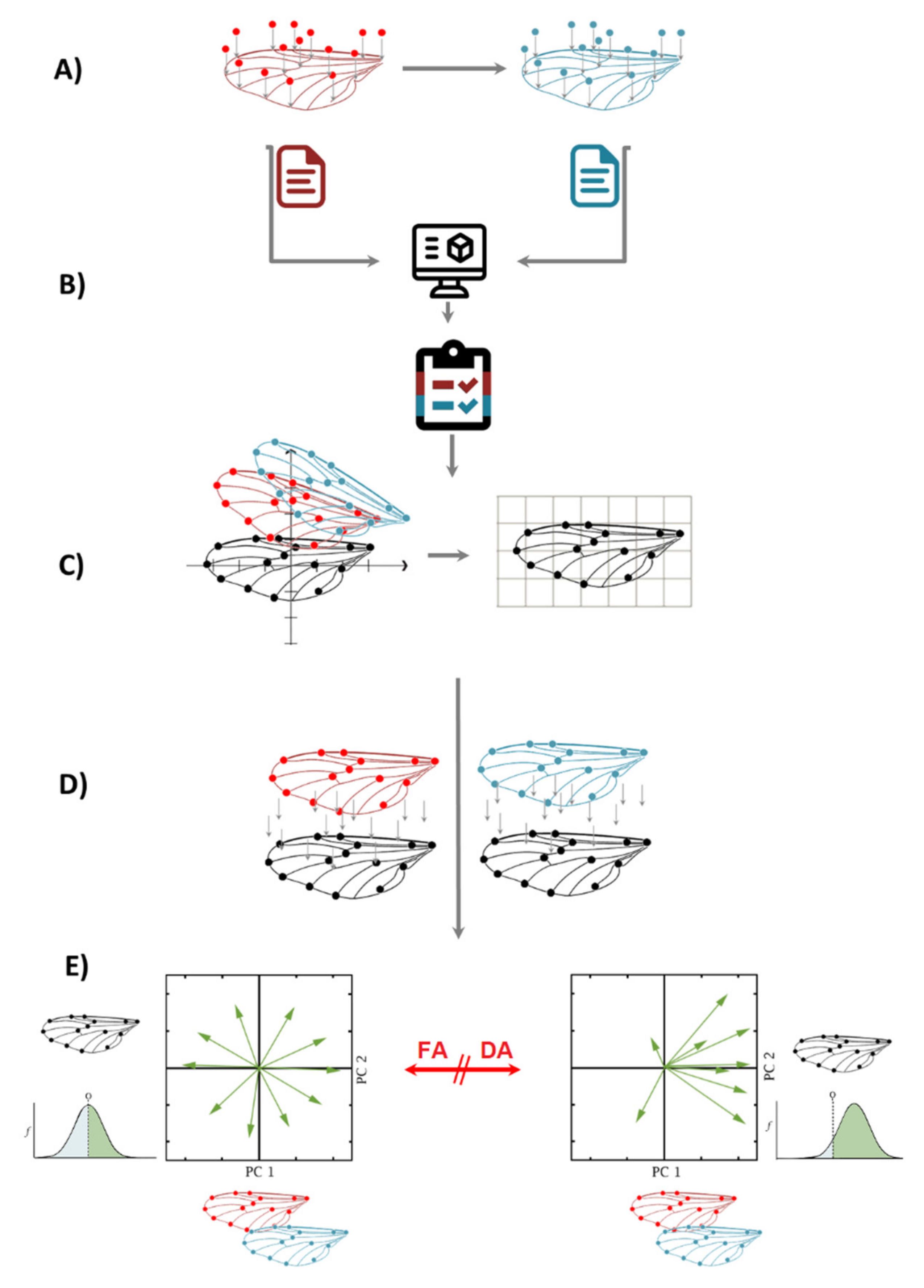
4. FA + GM, Step-by-Step Flow Chart
- (a)
- Two sets of independent images of the individuals are taken and landmarks are digitized, preferably not on the same day, to account for measurement error.
- (b)
- After digitizing the images, both independent datasets are combined in one single dataset. It is important to know that FA measurements of left and right sides are taken for each individual separately; therefore, classifiers of Individuals and Side (for matching asymmetry) need to be incorporated.
- (c)
- A Procrustes fit step is compulsory for all geometric morphometrics data. In this example, there are two matching symmetric wings; thus, the symmetries of paired structures are presented as separate, mirror-image copies, and the software automatically generates a reflection of each original landmark configuration to produce a reflected copy.
- (d)
- The shape asymmetry is calculated for individuals as the deviation from the original landmark configuration of the symmetric consensus. In this step, a Procrustes ANOVA is performed on the combined dataset, using the following classifiers: Individuals (in the Individual section) Side (in the Side section) and From Dataset (in the Error 1 section). It is important to note that the classifier “From Dataset” is the result of the combination of two repeated measurements, and it is compulsory for every fluctuating asymmetry analysis to calculate the measurement error as well.
- (e)
- When there is FA, the interaction of Individual × Side is significant in the Procrustes ANOVA; when there is directional asymmetry (DA), the classifier side is also significant. After this, there are two options to calculate the intensity of FA: one is extracting the MS values from the interaction of Individual × Side Procrustes ANOVA and comparing them between groups; the other is extracting the values of Mahalanobis or Procrustes FA scores from the dataset generated by the Procrustes ANOVA and comparing them between groups.
5. Fluctuating Asymmetry Using Geometric Morphometrics in Agroecosystems
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gilbert, S.F.; Bosch, T.C.; Ledón-Rettig, C. Eco-Evo-Devo: Developmental symbiosis and developmental plasticity as evolutionary agents. Nat. Rev. Genet. 2015, 16, 611–622. [Google Scholar] [CrossRef] [PubMed]
- Palmer, A.R.; Strobeck, C. Fluctuating Asymmetry—Measurement, Analysis, Patterns. Annu. Rev. Ecol. Syst. 1986, 17, 391–421. [Google Scholar] [CrossRef]
- Palmer, A.R. Fluctuating asymmetry analyses: A primer. In Developmental Instability: Its Origins and Evolutionary Implications; Springer: Berlin/Heidelberg, Germany, 1994; pp. 335–364. [Google Scholar]
- Klingenberg, C. Analyzing Fluctuating Asymmetry with Geometric Morphometrics: Concepts, Methods, and Applications. Symmetry 2015, 7, 843–934. [Google Scholar] [CrossRef]
- Savriama, Y.; Klingenberg, C.P. Beyond bilateral symmetry: Geometric morphometric methods for any type of symmetry. BMC Evol. Biol. 2011, 11. [Google Scholar] [CrossRef]
- Benítez, H.A.; Lemic, D.; Bažok, R.; Bravi, R.; Buketa, M.; Püschel, T. Morphological integration and modularity in Diabrotica virgifera virgifera LeConte (Coleoptera: Chrysomelidae) hind wings. Zool. Anz. A J. Comp. Zool. 2014, 253, 461–468. [Google Scholar] [CrossRef]
- Benítez, H.A.; Püschel, T.A. Modelando la Varianza de la Forma: Morfometría Geométrica Aplicaciones en Biología Evolutiva. Int. J. Morphol. 2014, 32, 998–1008. [Google Scholar] [CrossRef]
- Klingenberg, C.P. Developmental instability as a research tool: Using patterns of fluctuating asymmetry to infer the developmental origins of morphological integration. In Developmental Instability: Causes and Consequences; Polak, M., Ed.; Oxford University Press: Oxford, UK, 2003; pp. 427–442. [Google Scholar]
- Polak, M. Developmental Instability: Causes and Consequences; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Klingenberg, C.P. Morphological Integration and Developmental Modularity. In Annual Review of Ecology Evolution and Systematics; Annual Reviews: Palo Alto, CA, USA, 2008; Volume 39, pp. 115–132. [Google Scholar]
- Klingenberg, C.P.; Zaklan, S.D. Morphological integration between developmental compartments in the Drosophila wing. Evolution 2000, 54, 1273–1285. [Google Scholar] [CrossRef]
- Klingenberg, C.P.; Mebus, K.; Auffray, J.C. Developmental integration in a complex morphological structure: How distinct are the modules in the mouse mandible? Evol. Dev. 2003, 5, 522–531. [Google Scholar] [CrossRef]
- Graham, J.H.; Raz, S.; Hel-Or, H.; Nevo, E. Fluctuating asymmetry: Methods, theory, and applications. Symmetry 2010, 2, 466–540. [Google Scholar] [CrossRef]
- Van Dongen, S. Fluctuating asymmetry and developmental instability in evolutionary biology: Past, present and future. J. Evol. Biol. 2006, 19, 1727–1743. [Google Scholar] [CrossRef]
- Van Valen, L. A study of fluctuating asymmetry. Evolution 1962, 16, 125–142. [Google Scholar] [CrossRef]
- Babcock, L.E. Asymmetry in the fossil record. Eur. Rev. 2005, 13, 135. [Google Scholar] [CrossRef]
- Balzeau, A.; Gilissen, E.; Grimaud-Hervé, D. Shared pattern of endocranial shape asymmetries among great apes, anatomically modern humans, and fossil hominins. PLoS ONE 2012, 7, e29581. [Google Scholar] [CrossRef] [PubMed]
- Neubauer, S.; Gunz, P.; Scott, N.A.; Hublin, J.-J.; Mitteroecker, P. Evolution of brain lateralization: A shared hominid pattern of endocranial asymmetry is much more variable in humans than in great apes. Sci. Adv. 2020, 6, eaax9935. [Google Scholar] [CrossRef] [PubMed]
- Nijhout, H.; Davidowitz, G. Developmental Perspectives on Phenotypic Variation, Canalization, and Fluctuating Asymmetry; Oxford University Press: New York, NY, USA, 2003; pp. 3–13. [Google Scholar]
- Graham, J.H.; Shimizu, K.; Emlen, J.M.; Freeman, D.C.; Merkel, J. Growth models and the expected distribution of fluctuating asymmetry. Biol. J. Linn. Soc. 2003, 80, 57–65. [Google Scholar] [CrossRef]
- Alibert, P.; Moureau, B.; Dommergues, J.L.; David, B. Differentiation at a microgeographical scale within two species of ground beetle, Carabus auronitens and C-nemoralis (Coleoptera, Carabidae): A geometrical morphometric approach. Zool. Scr. 2001, 30, 299–311. [Google Scholar] [CrossRef]
- Bravi, R.; Benítez, H.A. Left-right asymmetries and shape analysis on Ceroglossus chilensis (Coleoptera: Carabidae). Acta Oecologica-Int. J. Ecol. 2013, 52, 57–62. [Google Scholar] [CrossRef]
- Benítez, H.A. Assessment of Patterns of Fluctuating Asymmetry and Sexual Dimorphism in Carabid Body Shape. Neotrop. Entomol. 2013, 42, 164–169. [Google Scholar] [CrossRef]
- Debat, V.; Begin, M.; Legout, H.; David, J.R. Allometric and nonallometric components of Drosophila wing shape respond differently to developmental temperature. Evolution 2003, 57, 2773–2784. [Google Scholar] [CrossRef]
- Parsons, P.A. Fluctuating asymmetry–a biological monitor of environmental and genomic stress. Heredity 1992, 68, 361–364. [Google Scholar] [CrossRef]
- Nattero, J.; Piccinali, R.V.; Gaspe, M.S.; Gürtler, R.E. Fluctuating asymmetry and exposure to pyrethroid insecticides in Triatoma infestans populations in northeastern Argentina. Infect. Genet. Evol. 2019, 74, 103925. [Google Scholar] [CrossRef]
- Nattero, J.; Malerba, R.; Rodríguez, C.S.; Crocco, L. Phenotypic plasticity in response to food source in Triatoma infestans (Klug, 1834) (Hemiptera, Reduviidae: Triatominae). Infect. Genet. Evol. 2013, 19, 38–44. [Google Scholar] [CrossRef] [PubMed]
- Benítez, H.; Briones, R.; Jerez, V. Fluctuating asymmetry in two populations of Ceroglossus chilensis (Eschscholtz, 1829) (Coleoptera: Carabidae) in agroecosystem of Pinus radiata d. Don, Bio-Bio region, Chile. Gayana 2008, 72, 131–139. [Google Scholar]
- Clarke, G. Developmental Stability and Fitness: The Evidence Is Not Quite So Clear. Am. Nat. 1998, 152, 762–766. [Google Scholar] [CrossRef] [PubMed]
- Garnier, S.; Gidaszewski, N.; Charlot, M.; Rasplus, J.-Y.; Alibert, P. Hybridization, developmental stability, and functionality of morphological traits in the ground beetle Carabus solieri (Coleoptera, Carabidae). Biol. J. Linn. Soc. 2006, 89, 151–158. [Google Scholar] [CrossRef]
- Hoffmann, A.; Woods, R. Associating environmental stress with developmental stability: Problems and patterns. In Developmental Instability: Causes and Consequences; Polak, M., Ed.; Oxford University Press: Oxford, UK, 2003; pp. 387–401. [Google Scholar]
- Markow, T.A. Evolutionary ecology and developmental instability. Annu. Rev. Entomol. 1995, 40, 105–120. [Google Scholar] [CrossRef]
- Chirichella, R.; Rocca, M.; Brugnoli, A.; Mustoni, A.; Apollonio, M. Fluctuating asymmetry in Alpine chamois horns: An indicator of environmental stress. Evol. Ecol. 2020, 34, 573–587. [Google Scholar] [CrossRef]
- Palmer, A.R.; Strobeck, C. Fluctuating asymmetry analyses revisited. In Developmental Instability: Causes and Consequences; Polak, M., Ed.; Oxford University Press: Oxford, UK, 2003; pp. 279–319. [Google Scholar]
- Windig, J.; Nylin, S. How to compare fluctuating asymmetry of different traits. J. Evol. Biol. 2000, 13, 29–37. [Google Scholar] [CrossRef]
- Angus, R. Quantifying Fluctuating Asymmetry: Not All Methods are Equivalent. Growth 1982, 46, 337–342. [Google Scholar]
- Graham, J.H.; Özener, B. Fluctuating asymmetry of human populations: A review. Symmetry 2016, 8, 154. [Google Scholar] [CrossRef]
- Klingenberg, C.P.; McIntyre, G.S. Geometric morphometrics of developmental instability: Analyzing patterns of fluctuating asymmetry with procrustes methods. Evolution 1998, 52, 1363–1375. [Google Scholar] [CrossRef] [PubMed]
- Klingenberg, C.P.; McIntyre, G.S.; Zaklan, S.D. Left-right asymmetry of fly wings and the evolution of body axes. Proc. R. Soc. B-Biol. Sci. 1998, 265, 1255–1259. [Google Scholar] [CrossRef] [PubMed]
- Auffray, J.; Debat, V.; Alibert, P. Shape asymmetry and developmental stability. In On Growth and Form: Spatio-Temporal Pattern Formation in Biology; Wiley: Chichester, UK, 1999; pp. 309–324. [Google Scholar]
- Zelditch, M.L.; Wood, A.R.; Swiderski, D.L. Building Developmental Integration into Functional Systems: Function-Induced Integration of Mandibular Shape. Evol. Biol. 2009, 36, 71–87. [Google Scholar] [CrossRef]
- Lawing, A.M.; Polly, P.D. Geometric morphometrics: Recent applications to the study of evolution and development. J. Zool. 2010, 280, 1–7. [Google Scholar] [CrossRef]
- Benítez, H.A.; Parra, L.E. Asimetría fluctuante: Una herramienta morfo-funcional para medir estabilidad del desarrollo. Int. J. Morphol. 2011, 29, 1459–1469. [Google Scholar] [CrossRef]
- Dryden, I.L.; Mardia, K.V. Statistical Shape Analysis; Wiley Chichester: New York, NY, USA, 1998; Volume 4. [Google Scholar]
- De Anna, E.B.; Bonisoli-Alquati, A.; Mousseau, T.A. The use of fluctuating asymmetry as a measure of environmentally induced developmental instability: A meta-analysis. Ecol. Indic. 2013, 30, 218–226. [Google Scholar]
- Ekrami, O.; Claes, P.; White, J.D.; Weinberg, S.M.; Marazita, M.L.; Walsh, S.; Shriver, M.D.; Dongen, S.V. A multivariate approach to determine the dimensionality of human facial asymmetry. Symmetry 2020, 12, 348. [Google Scholar] [CrossRef]
- Graham, J.H.; Emlen, J.M.; Freeman, D.C.; Leamy, L.J.; Kieser, J.A. Directional asymmetry and the measurement of developmental instability. Biol. J. Linn. Soc. 1998, 64, 1–16. [Google Scholar] [CrossRef]
- Dongen, S.V. How repeatable is the estimation of developmental stability by fluctuating asymmetry? Proc. R. Soc. Lond. Ser. B Biol. Sci. 1998, 265, 1423–1427. [Google Scholar] [CrossRef]
- Maestri, R.; Fornel, R.; Galiano, D.; de Freitas, T.R. Niche suitability affects development: Skull asymmetry increases in less suitable areas. PLoS ONE 2015, 10, e0122412. [Google Scholar] [CrossRef]
- Viscosi, V. Geometric morphometrics and leaf phenotypic plasticity: Assessing fluctuating asymmetry and allometry in European white oaks (Quercus). Bot. J. Linn. Soc. 2015, 179, 335–348. [Google Scholar] [CrossRef]
- Urošević, A.; Ljubisavljević, K.; Ivanović, A. Fluctuating asymmetry and individual variation in the skull shape of the common wall lizard (Podarcis muralis Laurenti, 1768) estimated by geometric morphometrics. Herpetol. J. 2015, 25, 177–186. [Google Scholar]
- Neustupa, J.; Woodard, K. Geometric morphometrics reveals increased symmetric shape variation and asymmetry related to lead exposure in the freshwater green alga Micrasterias compereana. Ecol. Indic. 2020, 111, 106054. [Google Scholar] [CrossRef]
- Sandner, T.M.; Zverev, V.; Kozlov, M.V. Can the use of landmarks improve the suitability of fluctuating asymmetry in plant leaves as an indicator of stress? Ecol. Indic. 2019, 97, 457–465. [Google Scholar] [CrossRef]
- Debat, V.; Debelle, A.; Dworkin, I. Plasticity, canalization, and developmental stability of the Drosophila wing: Joint effects of mutations and developmental temperature. Evol. Int. J. Org. Evol. 2009, 63, 2864–2876. [Google Scholar] [CrossRef]
- Klingenberg, C.P.; Barluenga, M.; Meyer, A. Shape analysis of symmetric structures: Quantifying variation among individuals and asymmetry. Evolution 2002, 56, 1909–1920. [Google Scholar] [CrossRef] [PubMed]
- Lehoux, C.; Cloutier, R. Building blocks of a fish head: Developmental and variational modularity in a complex system. J. Exp. Zool. Part. B Mol. Dev. Evol. 2015, 324, 614–628. [Google Scholar] [CrossRef]
- Villalobos-Leiva, A.; Benítez, H.A. Morfometría geométrica y sus nuevas aplicaciones en ecología y biología evolutiva. Parte 2. Int. J. Morphol. 2020, 38, 1818–1836. [Google Scholar]
- Klingenberg, C.P. MorphoJ: An integrated software package for geometric morphometrics. Mol. Ecol. Resour. 2011, 11, 353–357. [Google Scholar] [CrossRef]
- Hunter, M.D. Landscape structure, habitat fragmentation, and the ecology of insects. Agric. For. Entomol. 2002, 4, 159–166. [Google Scholar] [CrossRef]
- Tscharntke, T.; Klein, A.M.; Kruess, A.; Steffan-Dewenter, I.; Thies, C. Landscape perspectives on agricultural intensification and biodiversity–ecosystem service management. Ecol. Lett. 2005, 8, 857–874. [Google Scholar] [CrossRef]
- Geiger, F.; Bengtsson, J.; Berendse, F.; Weisser, W.W.; Emmerson, M.; Morales, M.B.; Ceryngier, P.; Liira, J.; Tscharntke, T.; Winqvist, C. Persistent negative effects of pesticides on biodiversity and biological control potential on European farmland. Basic Appl. Ecol. 2010, 11, 97–105. [Google Scholar] [CrossRef]
- Marchand, H.; Paillat, G.; Montuire, S.; Butet, A. Fluctuating asymmetry in bank vole populations (Rodentia, Arvicolinae) reflects stress caused by landscape fragmentation in the Mont-Saint-Michel Bay. Biol. J. Linn. Soc. 2003, 80, 37–44. [Google Scholar] [CrossRef]
- Leary, R.F.; Allendorf, F.W. Fluctuating asymmetry as an indicator of stress: Implications for conservation biology. Trends Ecol. Evol. 1989, 4, 214–217. [Google Scholar] [CrossRef]
- Clarke, G.M. Fluctuating asymmetry of invertebrate populations as a biological indicator of environmental quality. Environ. Pollut. 1993, 82, 207–211. [Google Scholar] [CrossRef]
- Naugler, C.T.; Leech, S.M. Fluctuating asymmetry and survival ability in the forest tent caterpillar moth Malacosoma disstria: Implications for pest management. Entomol. Exp. Appl. 1994, 70, 295–298. [Google Scholar] [CrossRef]
- Floate, K.; Fox, A. Flies under stress: A test of fluctuating asymmetry as a biomonitor of environmental quality. Ecol. Appl. 2000, 10, 1541–1550. [Google Scholar] [CrossRef]
- Ribeiro, B.; Guedes, R.; Corrêa, A.; Santos, C. Fluctuating asymmetry in insecticide-resistant and insecticide-susceptible strains of the maize weevil, Sitophilus zeamais (Coleoptera: Curculionidae). Arch. Environ. Contam. Toxicol. 2007, 53, 77–83. [Google Scholar] [CrossRef]
- Chang, X.; Zhai, B.; Wang, M.; Wang, B. Relationship between exposure to an insecticide and fluctuating asymmetry in a damselfly (Odonata, Coenagriidae). Hydrobiologia 2007, 586, 213–220. [Google Scholar] [CrossRef]
- Abaga, N.O.Z.; Alibert, P.; Dousset, S.; Savadogo, P.W.; Savadogo, M.; Sedogo, M. Insecticide residues in cotton soils of Burkina Faso and effects of insecticides on fluctuating asymmetry in honey bees (Apis mellifera Linnaeus). Chemosphere 2011, 83, 585–592. [Google Scholar] [CrossRef]
- Graham, J.H.; Freeman, D.C.; Emlen, J.M. Developmental stability: A sensitive indicator of populations under stress. Astm Spec. Tech. Publ. 1993, 1179, 136–158. [Google Scholar]
- Møller, A.; Sanotra, G.; Vestergaard, K. Developmental stability in relation to population density and breed of chickens Gallus gallus. Poult. Sci. 1995, 74, 1761–1771. [Google Scholar] [CrossRef] [PubMed]
- Møller, A.; Sanotra, G.; Vestergaard, K. Developmental instability and light regime in chickens (Gallus gallus). Appl. Anim. Behav. Sci. 1999, 62, 57–71. [Google Scholar] [CrossRef]
- Gest, T.; Siegel, M.; Anistranski, J. Increased fluctuating asymmetry in the long bones of neonatal rats stressed in cold, heat, and noise. Growth 1986, 50, 385–389. [Google Scholar]
- Newell-Morris, L.; Fahrenbruch, C.; Sackett, G. Prenatal psychological stress, dermatoglyphic asymmetry and pregnancy outcome in the pigtailed macaque (Macaca nemestrina). Neonatology 1989, 56, 61–75. [Google Scholar] [CrossRef] [PubMed]
- Eeva, T.; Tanhuanpaa, S.; Rabergh, C.; Airaksinen, S.; Nikinmaa, M.; Lehikoinen, E. Biomarkers and fluctuating asymmetry as indicators of pollution-induced stress in two hole-nesting passerines. Funct. Ecol. 2000, 14, 235–243. [Google Scholar] [CrossRef]
- Satterlee, D.; Cadd, G.; Jones, R. Developmental instability in Japanese quail genetically selected for contrasting adrenocortical responsiveness. Poult. Sci. 2000, 79, 1710–1714. [Google Scholar] [CrossRef]
- Yang, A.; Dunnington, E.; Siegel, P. Developmental stability in stocks of White Leghorn chickens. Poult. Sci. 1997, 76, 1632–1636. [Google Scholar] [CrossRef]
- Eriksen, M.; Haug, A.; Torjesen, P.; Bakken, M. Prenatal exposure to corticosterone impairs embryonic development and increases fluctuating asymmetry in chickens (Gallus gallus domesticus). Br. Poult. Sci. 2003, 44, 690–697. [Google Scholar] [CrossRef]
- Parés-Casanova, P.M.; Castel-Mas, L.; Jones-Capdevila, K.N. Asymmetries of Forelimb Digits of Young Cattle. Vet. Sci. 2020, 7, 83. [Google Scholar] [CrossRef]
- Parés Casanova, P.-M.; Crosby-Granados, R.; Muñoz, F.; Salamanca-Carreño, A. Marked directional skull asymmetry in the Araucan horse. Vet. Comp. Orthop. Traumatol. 2020, 3, 11–18. [Google Scholar] [CrossRef][Green Version]
- Rohlf, F.J.; Corti, M. Use of two-block partial least-squares to study covariation in shape. Syst. Biol. 2000, 49, 740–753. [Google Scholar] [CrossRef]
- Nattero, J.; Piccinali, R.V.; Lopes, C.M.; Hernández, M.L.; Abrahan, L.; Lobbia, P.A.; Rodríguez, C.S.; de la Fuente, A.L.C. Morphometric variability among the species of the Sordida subcomplex (Hemiptera: Reduviidae: Triatominae): Evidence for differentiation across the distribution range of Triatoma sordida. Parasites Vectors 2017, 10, 412. [Google Scholar] [CrossRef]
- Polak, M.; Taylor, P.W. A primary role of developmental instability in sexual selection. Proc. R. Soc. B Biol. Sci. 2007, 274, 3133–3140. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Benítez, H.A.; Lemic, D.; Villalobos-Leiva, A.; Bažok, R.; Órdenes-Claveria, R.; Pajač Živković, I.; Mikac, K.M. Breaking Symmetry: Fluctuating Asymmetry and Geometric Morphometrics as Tools for Evaluating Developmental Instability under Diverse Agroecosystems. Symmetry 2020, 12, 1789. https://doi.org/10.3390/sym12111789
Benítez HA, Lemic D, Villalobos-Leiva A, Bažok R, Órdenes-Claveria R, Pajač Živković I, Mikac KM. Breaking Symmetry: Fluctuating Asymmetry and Geometric Morphometrics as Tools for Evaluating Developmental Instability under Diverse Agroecosystems. Symmetry. 2020; 12(11):1789. https://doi.org/10.3390/sym12111789
Chicago/Turabian StyleBenítez, Hugo A., Darija Lemic, Amado Villalobos-Leiva, Renata Bažok, Rodrigo Órdenes-Claveria, Ivana Pajač Živković, and Katarina M. Mikac. 2020. "Breaking Symmetry: Fluctuating Asymmetry and Geometric Morphometrics as Tools for Evaluating Developmental Instability under Diverse Agroecosystems" Symmetry 12, no. 11: 1789. https://doi.org/10.3390/sym12111789
APA StyleBenítez, H. A., Lemic, D., Villalobos-Leiva, A., Bažok, R., Órdenes-Claveria, R., Pajač Živković, I., & Mikac, K. M. (2020). Breaking Symmetry: Fluctuating Asymmetry and Geometric Morphometrics as Tools for Evaluating Developmental Instability under Diverse Agroecosystems. Symmetry, 12(11), 1789. https://doi.org/10.3390/sym12111789









