Effect of the Direction of Uniform Horizontal Magnetic Field on the Linear Stability of Natural Convection in a Long Vertical Rectangular Enclosure
Abstract
1. Introduction
2. Governing Equations
3. Numerical Analyses of Basic Flows
4. Linear Stability Analyses
4.1. Linearized Disturbance Equations
4.2. Numerical Methodology
4.3. Verification of the Code Developed
4.4. Results for MHD Natural Convection
5. Discussion
6. Conclusions
Funding
Conflicts of Interest
Nomenclature
| bi | magnetic flux density = (bx, by, 0) (T) |
| Bi | dimensionless magnetic flux density = (BX, BY, 0) (-) |
| b0 | absolute value of magnetic flux density imposed (T) |
| C | constant (-) |
| g | gravitational acceleration (m/s2) |
| Gr | Grashof number (-) |
| Ha | Hartmann number (-) |
| i | imaginary unit (-) |
| ji | electric current density = (jx, jy, jz) (A/m2) |
| Ji | dimensionless electric current density = (JX, JY, JZ) (-) |
| k | dimensionless wavenumber (-) |
| l | characteristic length (m) |
| p | pressure (Pa) |
| P | dimensionless pressure (-) |
| Pr | Prandtl number (-) |
| S | complex eigenvalue (rad/s) |
| SI | angular frequency (rad/s) |
| SR | linear growth rate (rad/s) |
| t | time (s) |
| T | temperature (K) |
| Tc | temperature at cold wall (K) |
| Th | temperature at hot wall (K) |
| T0 | reference temperature = (Th + Tc)/2 (K) |
| ΔT | temperature difference between hot and cold walls = (Th - Tc) (K) |
| ui | velocity vector = (u, v, w) (m/s) |
| Ui | dimensionless velocity vector = (U, V, W) (-) |
| u | x-directional velocity component (m/s) |
| U | dimensionless X-directional velocity component (-) |
| v | y-directional velocity component (m/s) |
| V | dimensionless Y-directional velocity component (-) |
| w | z-directional velocity component (m/s) |
| W | dimensionless Z-directional velocity component (-) |
| xi | Cartesian coordinate (m) |
| Xi | dimensionless Cartesian coordinate (-) |
| x | x coordinate (m) |
| X | dimensionless x coordinate (-) |
| y | y coordinate (m) |
| Y | dimensionless y coordinate (-) |
| z | z coordinate (m) |
| Z | dimensionless z coordinate (-) |
| Greek symbols | |
| α | thermal diffusivity (m2/s) |
| β | volumetric coefficient of thermal expansion at T0 (1/K) |
| δij | Kronecker delta (-) |
| εijk | Levi-Civita symbol (-) |
| Θ | dimensionless temperature (-) |
| θ | angle between X-axis and magnetic field (rad) |
| ν | kinematic viscosity (m2/s) |
| ρ | density (kg/m3) |
| ρ0 | density at T0 (kg/m3) |
| σ | electric conductivity (1/(Ω·m)) |
| τ | dimensionless time (-) |
| φ | electric potential (V) |
| Φ | dimensionless electric potential (-) |
| Subscripts or superscripts | |
| infinitesimal disturbance | |
| basic state | |
| amplitude function | |
| I | imaginary part |
| R | real part |
| n | number of iterative steps |
References
- Moreau, R.J. Fluid Mechanics and Its Application. In Magnetohydrodynamics; Kluwer Academic Publishers: Dordrecht, The Netherlands; Norwell, MA, USA, 1990; Volume 3. [Google Scholar]
- Molokov, S.; Moreau, R.; Moffatt, H.K. Fluid Mechanics and Its Application. In Magnetohydrodynamics: Historical Evolution and Trends; Springer: Berlin/Heidelberg, Germany, 2007; Volume 80. [Google Scholar]
- Ozoe, H. Magnetic Convection; Imperial College Press: London, UK, 2005. [Google Scholar]
- Chandrasekhar, S. Hydrodynamic and Hydromagnetic Stability; Dover Publication: Mineola, NY, USA, 1961. [Google Scholar]
- Burr, U.; Müller, U. Rayleigh–Bénard convection in liquid metal layers under the influence of a vertical magnetic field. Phys. Fluids 2001, 13, 3247–3257. [Google Scholar] [CrossRef]
- Burr, U.; Müller, U. Rayleigh-Bénard convection in liquid metal layers under the influence of a horizontal magnetic field. J. Fluid Mech. 2002, 453, 345. [Google Scholar] [CrossRef]
- Mistrangelo, C.; Bühler, L. Magneto-convective instabilities in horizontal cavities. Phys. Fluids 2016, 28, 024104. [Google Scholar] [CrossRef]
- Vest, C.M.; Arpaci, V.S. Stability of natural convection in a vertical slot. J. Fluid Mech. 1969, 36, 1–15. [Google Scholar] [CrossRef]
- Hart, J.E. Stability of the flow in a differentially heated inclined box. J. Fluid Mech. 1971, 47, 547–576. [Google Scholar] [CrossRef]
- Bergholz, R.F. Instability of steady natural convection in a vertical fluid layer. J. Fluid Mech. 1978, 84, 743–768. [Google Scholar] [CrossRef]
- Choi, I.; Korpela, S.A. Stability of the conduction regime of natural convection in a tall vertical annulus. J. Fluid Mech. 1980, 99, 725–738. [Google Scholar] [CrossRef]
- Lee, Y.; Korpela, S. Multicellular natural convection in a vertical slot. J. Fluid Mech. 1983, 126, 91–121. [Google Scholar] [CrossRef]
- Chen, Y.M.; Pearlstein, A.J. Stability of free-convection flows of variable-viscosity fluids in vertical and inclined slots. J. Fluid Mech. 1989, 198, 513–541. [Google Scholar] [CrossRef]
- Fujimura, K.; Mizushima, J. Nonlinear equilibrium solutions for travelling waves in a free convection between vertical parallel plates. Eur. J. Mech. B Fluids 1991, 10, 25–30. [Google Scholar]
- McAllister, A.; Steinolfson, R.; Tajima, T. Vertical Slot Convection: A Linear Study (No. DOE/ET/53088-584; IFSR--584); Institute for Fusion Studies, Texas University: Austin, TX, USA, 1992. [Google Scholar]
- Lartigue, B.; Lorente, S.; Bourret, B. Multicellular natural convection in a high aspect ratio cavity: Experimental and numerical results. Int. J. Heat Mass Transf. 2000, 43, 3157–3170. [Google Scholar] [CrossRef]
- Bratsun, D.A.; Zyuzgin, A.V.; Putin, G.F. Non-linear dynamics and pattern formation in a vertical fluid layer heated from the side. Int. J. Heat Fluid Flow 2003, 24, 835–852. [Google Scholar] [CrossRef]
- Wright, J.L.; Jin, H.; Hollands, K.G.T.; Naylor, D. Flow visualization of natural convection in a tall, air-filled vertical cavity. Int. J. Heat Mass Transf. 2006, 49, 889–904. [Google Scholar] [CrossRef]
- Ganguli, A.A.; Pandit, A.B.; Joshi, J.B. Numerical predictions of flow patterns due to natural convection in a vertical slot. Chem. Eng. Sci. 2007, 62, 4479–4495. [Google Scholar] [CrossRef]
- Fogaing, M.T.; Nana, L.; Crumeyrolle, O.; Mutabazi, I. Wall effects on the stability of convection in an infinite vertical layer. Int. J. Therm. Sci. 2016, 100, 240–247. [Google Scholar] [CrossRef]
- Suslov, S.A.; Paolucci, S. Stability of natural convection flow in a tall vertical enclosure under non-Boussinesq conditions. Int. J. Heat Mass Transf. 1995, 38, 2143–2157. [Google Scholar] [CrossRef]
- Kitada, T.; Kato, Y.; Fujimura, K. Non-Boussinesq effects on the linear stability of thermal convection in an inclined slot. Trans. Jpn. Soc. Mech. Eng. Ser. B 2002, 68, 1002–1007. [Google Scholar] [CrossRef]
- Takashima, M. The stability of natural convection in a vertical layer of electrically conducting fluid in the presence of a transverse magnetic field. Fluid Dyn. Res. 1994, 14, 121. [Google Scholar] [CrossRef]
- Nagata, M. Nonlinear analysis on the natural convection between vertical plates in the presence of a horizontal magnetic field. Eur. J. Mech. B Fluids 1998, 17, 33–50. [Google Scholar] [CrossRef]
- Burr, U.; Barleon, L.; Jochmann, P.; Tsinober, A. Magnetohydrodynamic convection in a vertical slot with horizontal magnetic field. J. Fluid Mech. 2003, 475, 21. [Google Scholar] [CrossRef]
- Hudoba, A.; Molokov, S. Linear stability of buoyant convective flow in a vertical channel with internal heat sources and a transverse magnetic field. Phys. Fluids 2016, 28, 114103. [Google Scholar] [CrossRef]
- Wong, C.P.C.; Malang, S.; Sawan, M.; Dagher, M.; Smolentsev, S.; Merrill, B.; Youssef, M.; Reyes, S.; Sze, D.; Morley, N.; et al. An overview of dual coolant Pb–17Li breeder first wall and blanket concept development for the US ITER-TBM design. Fusion Eng. Des. 2006, 81, 461–467. [Google Scholar] [CrossRef]
- Soto, C.; Smolentsev, S.; García-Rosales, C. Mitigation of MHD phenomena in DCLL blankets by Flow Channel Inserts based on a SiC-sandwich material concept. Fusion Eng. Des. 2020, 151, 111381. [Google Scholar] [CrossRef]
- Tagawa, T.; Authié, G.; Moreau, R. Buoyant flow in long vertical enclosures in the presence of a strong horizontal magnetic field. Part 1. Fully-established flow. Eur. J. Mech. B Fluids 2002, 21, 383–398. [Google Scholar] [CrossRef]
- Authié, G.; Tagawa, T.; Moreau, R. Buoyant flow in long vertical enclosures in the presence of a strong horizontal magnetic field. Part 2. Finite enclosures. Eur. J. Mech. B Fluids 2003, 22, 203–220. [Google Scholar] [CrossRef]
- Lyubimov, D.V.; Lyubimova, T.P.; Perminov, A.B.; Henry, D.; Hadid, H.B. Stability of convection in a horizontal channel subjected to a longitudinal temperature gradient. Part 2. Effect of a magnetic field. J. Fluid Mech. 2009, 635, 297–319. [Google Scholar] [CrossRef][Green Version]
- Kitaura, T.; Tagawa, T. Linear stability analysis of thermal convection in an infinitely long vertical rectangular enclosure in the presence of a uniform horizontal magnetic field. J. Fluids 2014, 2014, 642042. [Google Scholar] [CrossRef][Green Version]
- Tagawa, T. Linear stability analysis of liquid metal flow in an insulating rectangular duct under external uniform magnetic field. Fluids 2019, 4, 177. [Google Scholar] [CrossRef]

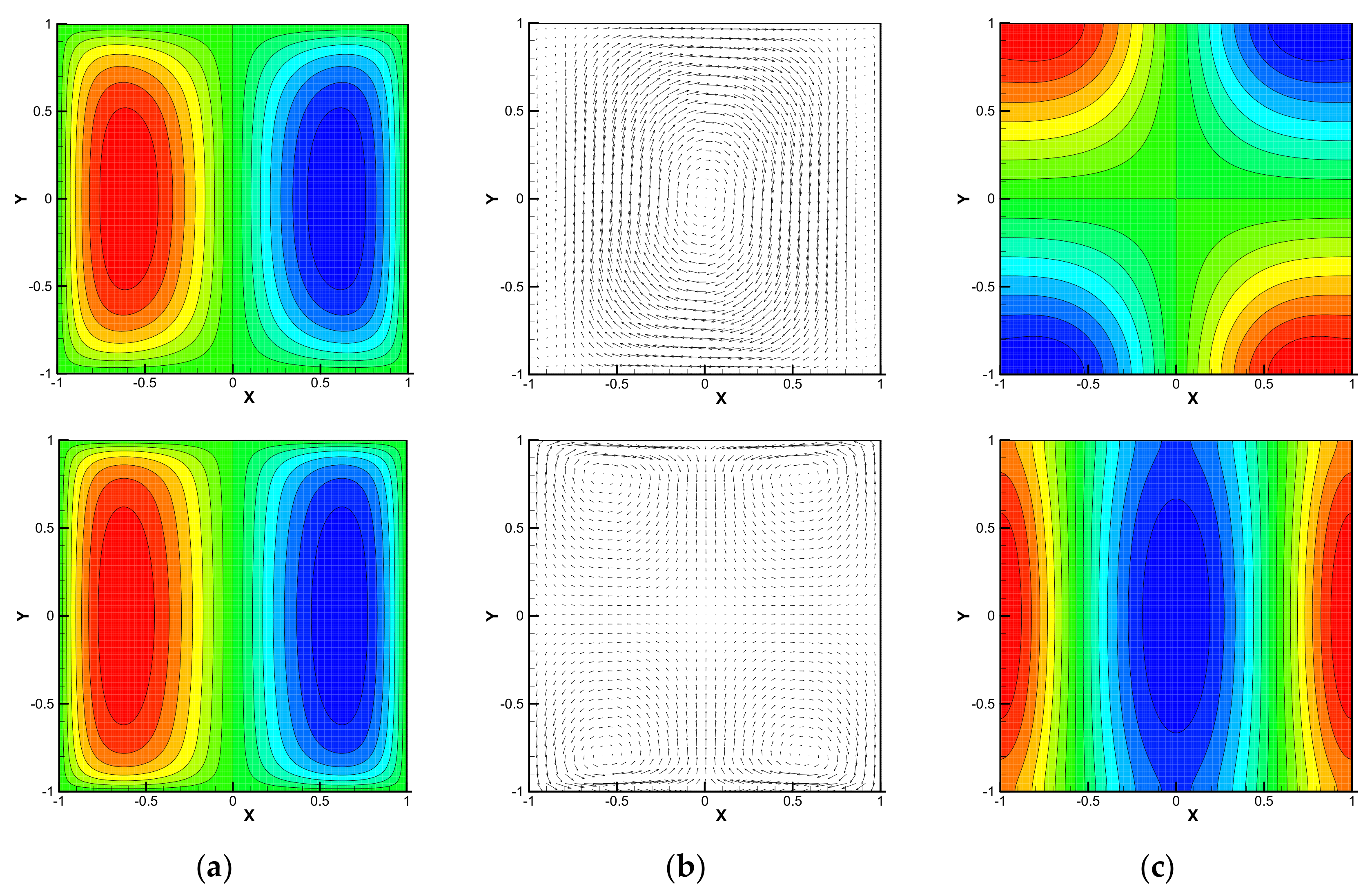
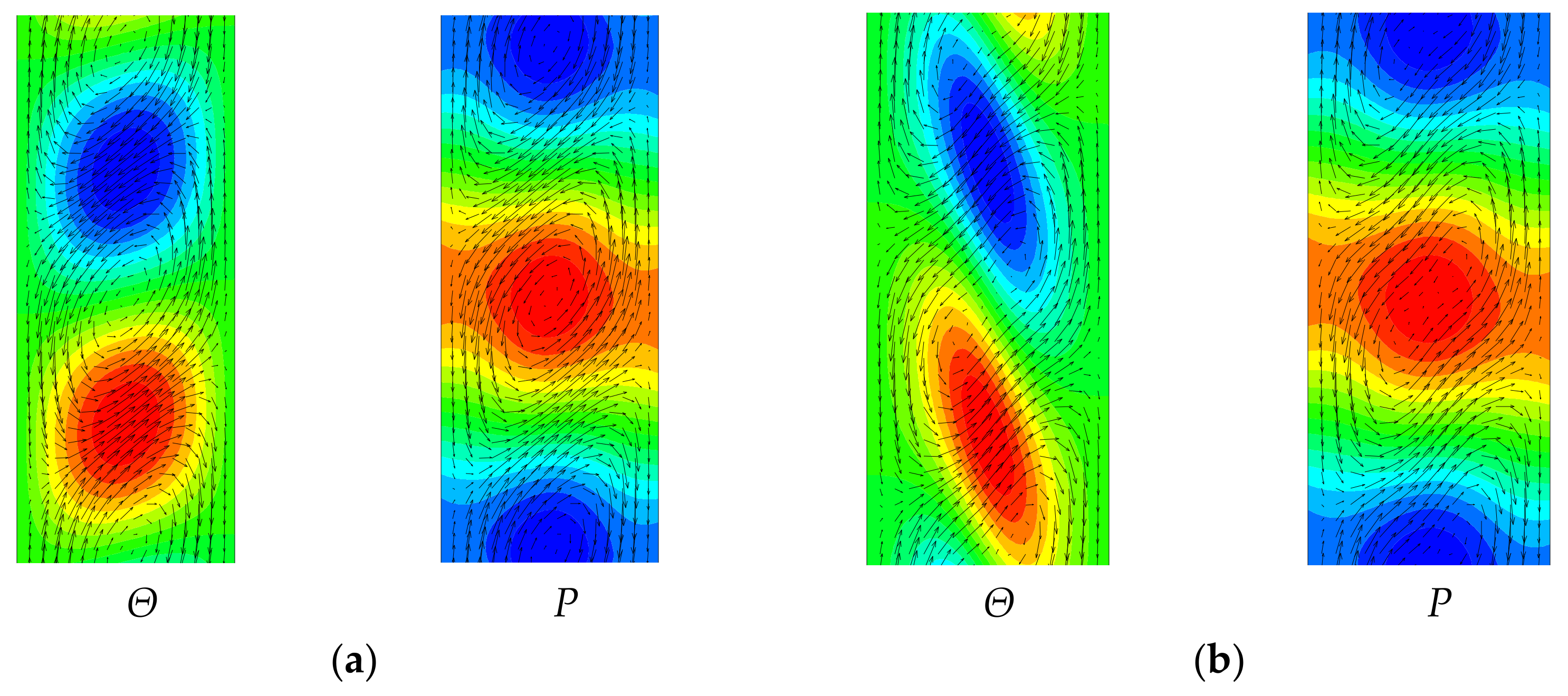
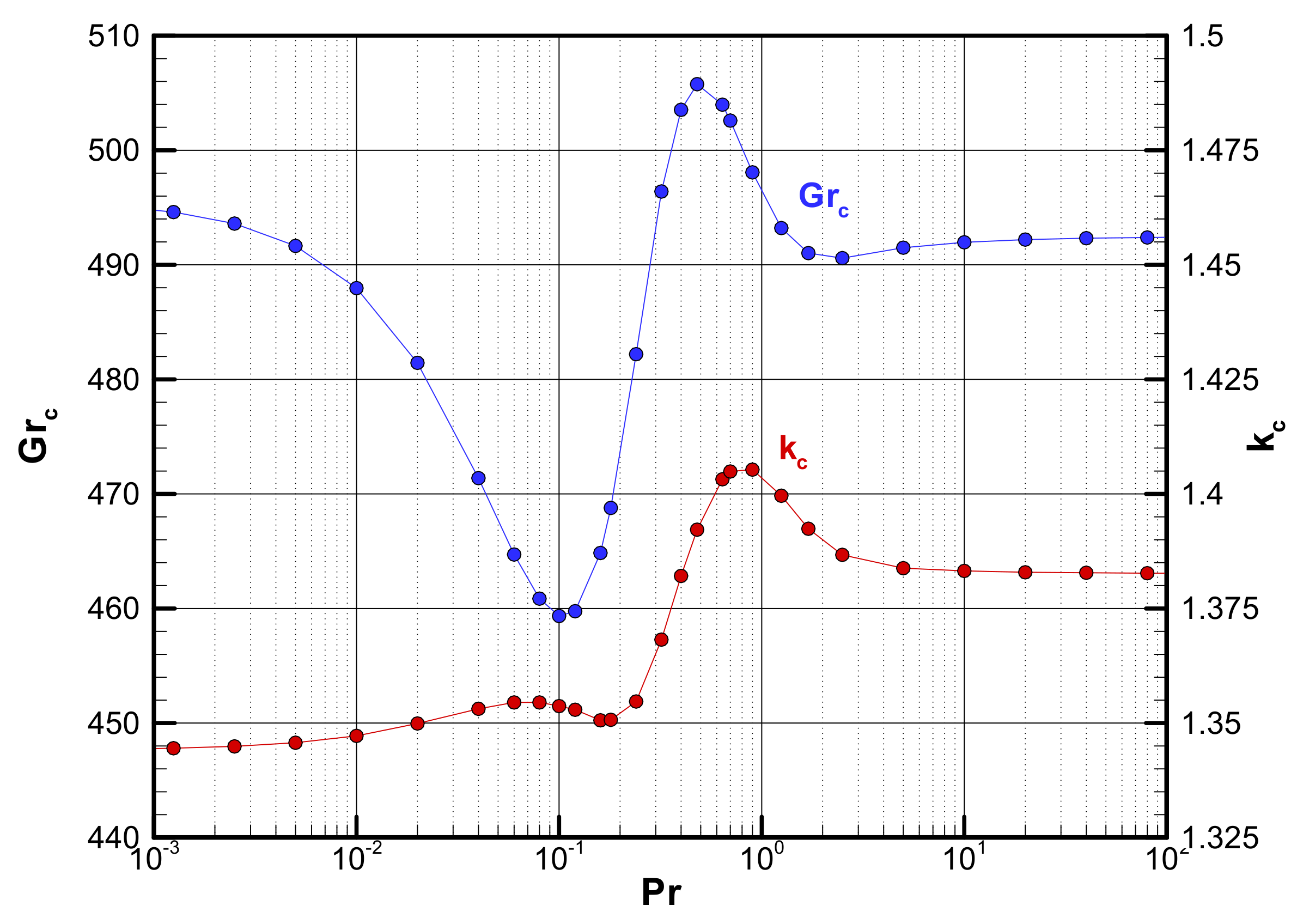

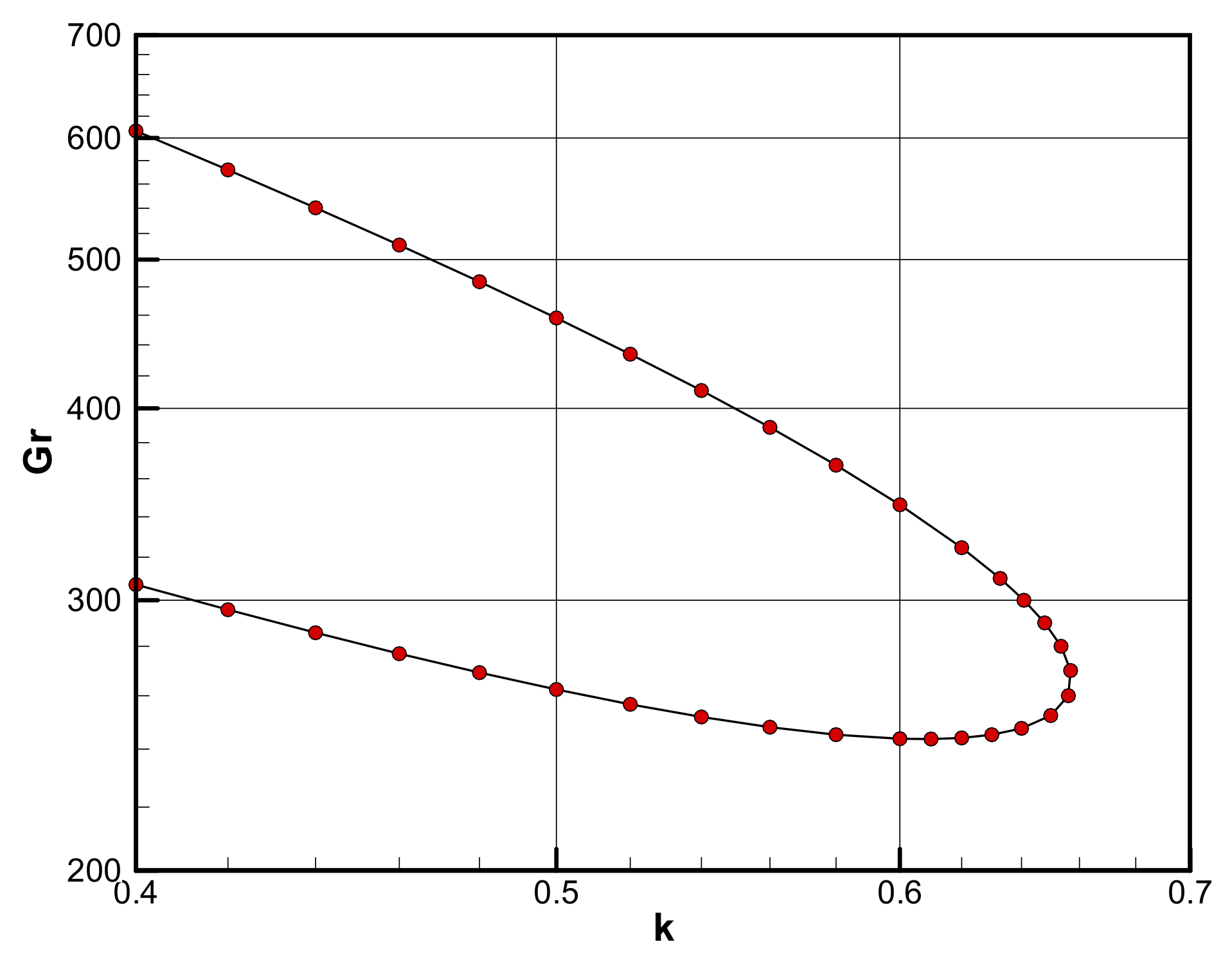

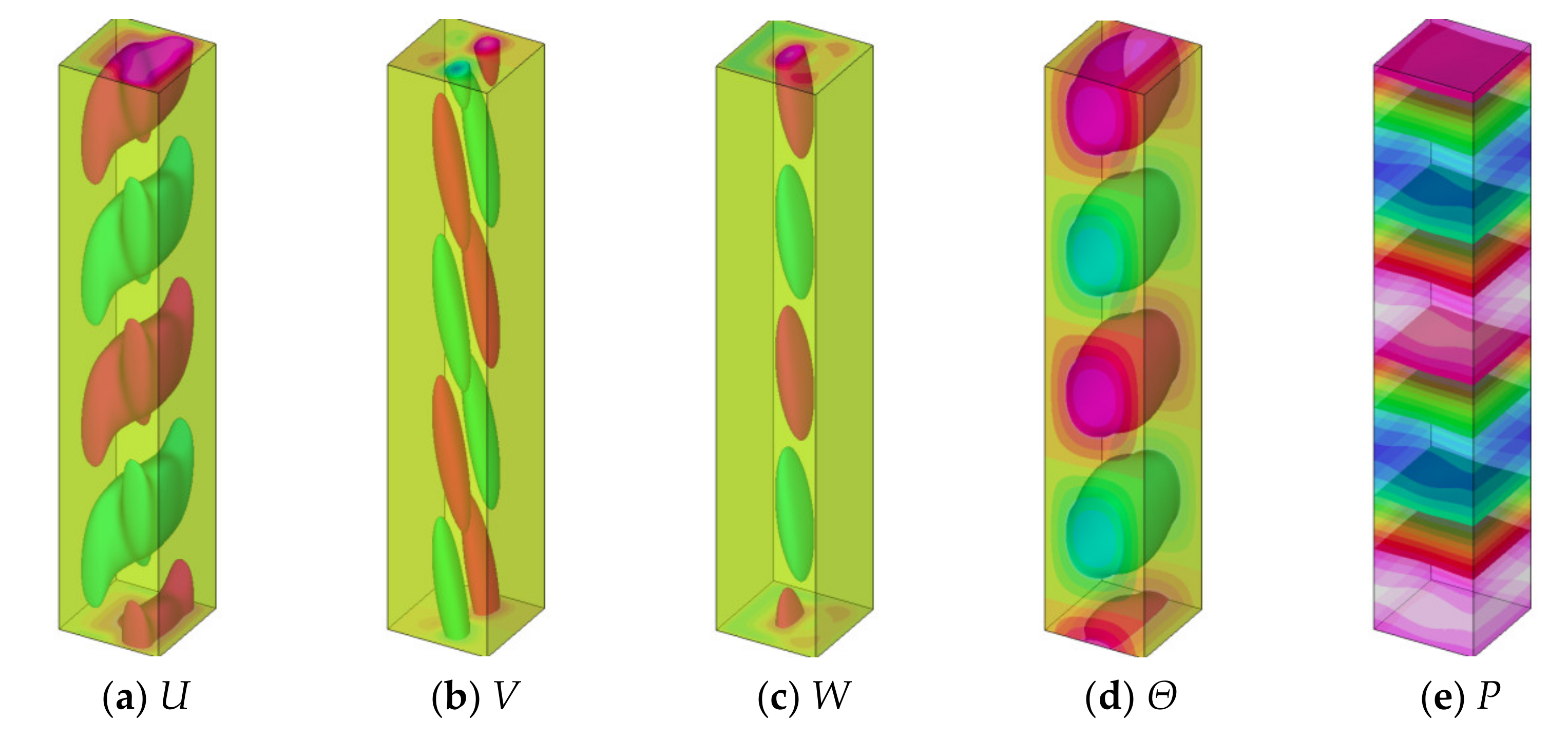
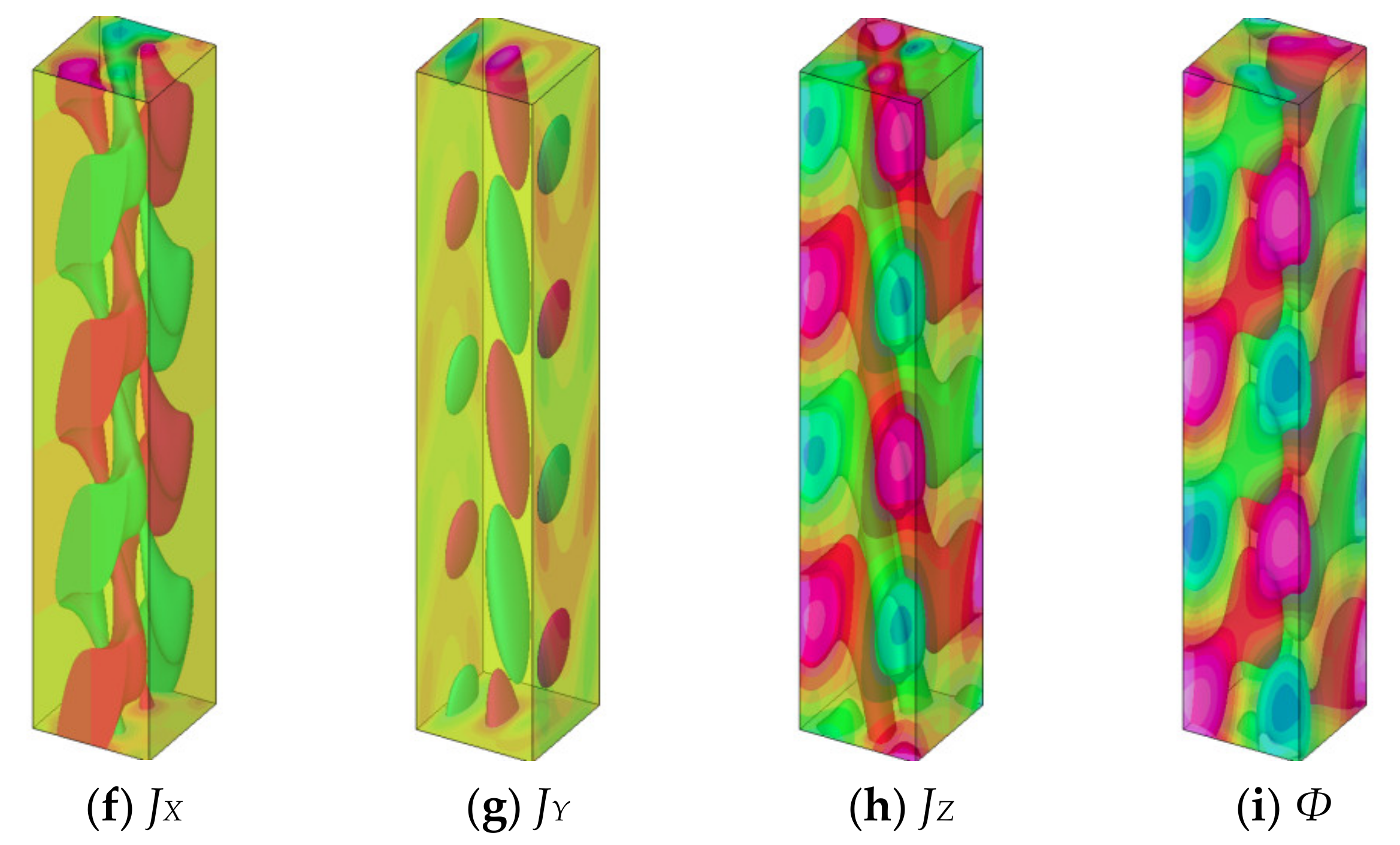
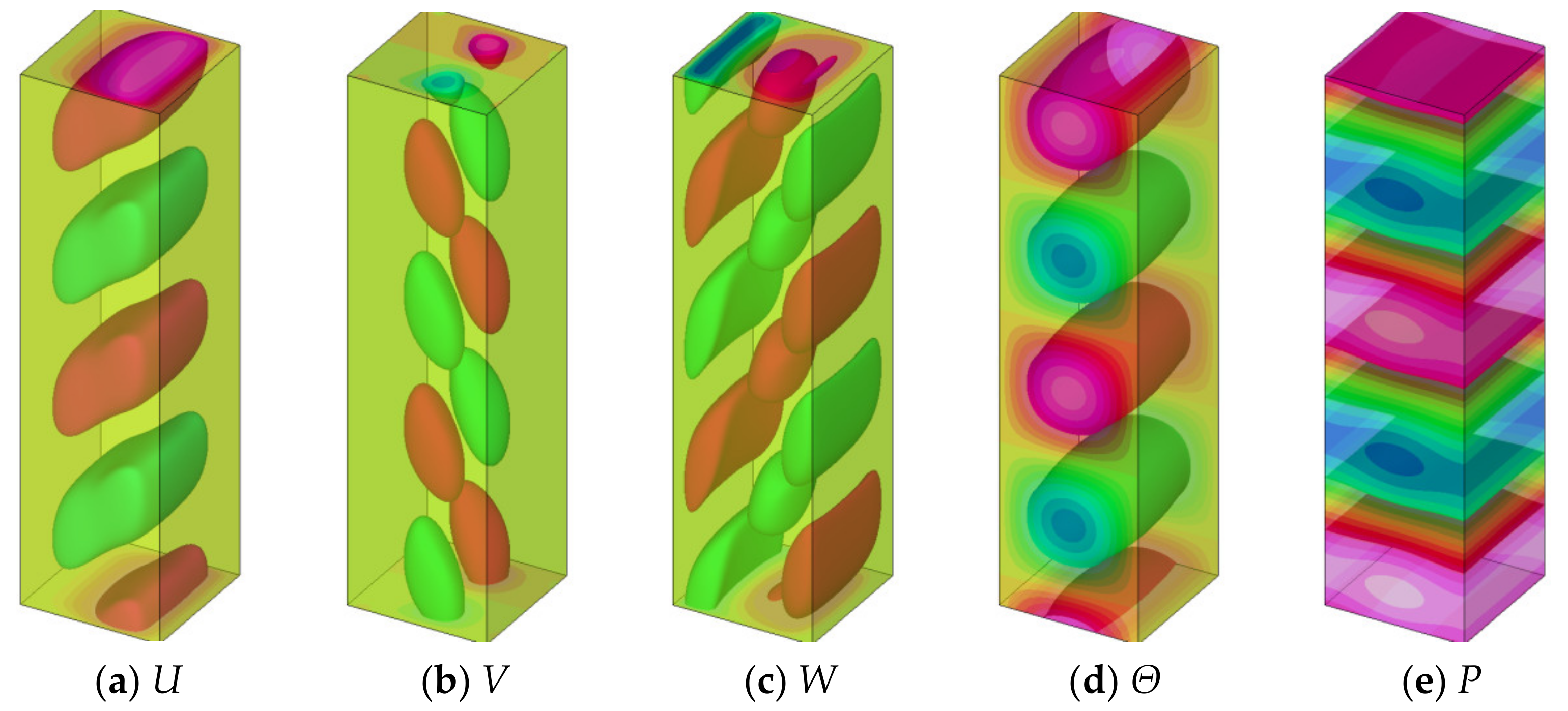


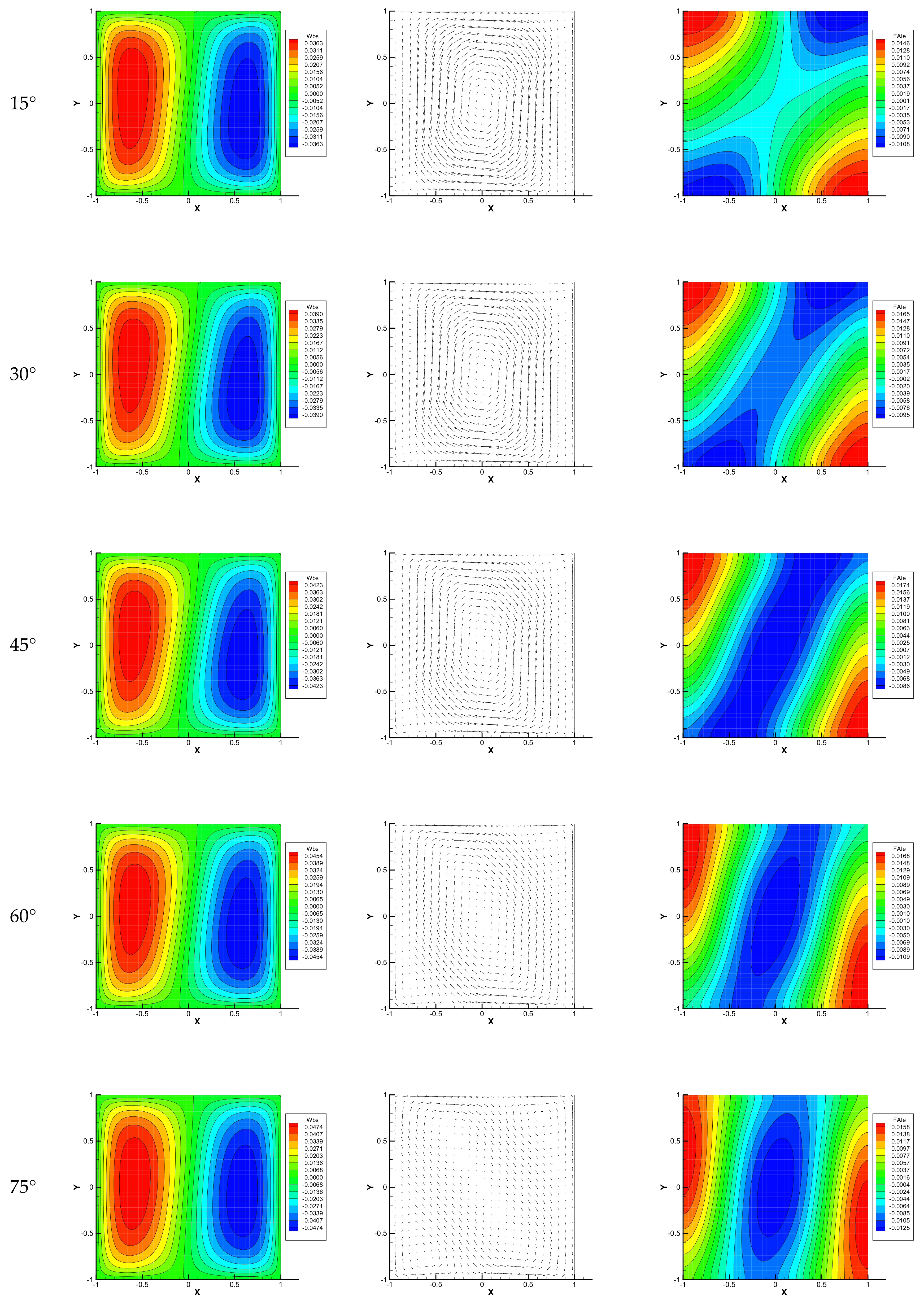

| Ha (Direction) | kc (50 × 50) | Grc (50 × 50) | Grn (100 × 100) |
|---|---|---|---|
| 0 | 0.7085 | 2501.77 | 2497.79 |
| 0.1 (X) | 0.7082 | 2506.12 | 2502.12 |
| 0.5 (X) | 0.6986 | 2612.84 | 2608.45 |
| 1.0 (X) | 0.6689 | 2977.45 | 2971.56 |
| 1.5 (X) | 0.6207 | 3708.12 | 3698.37 |
| 2.0 (X) | 0.5566 | 5072.35 | 5052.06 |
| 2.5 (X) | 0.4838 | 7705.94 | 7650.83 |
| 3.0 (X) | 0.4172 | 13,193.0 | 12,989.7 |
| 3.5 (X) | 0.3562 | 26,057.7 | 25,019.8 |
| 4.0 (X) | 0.3485 | 57,759.7 | 52,146.2 |
| 1 (Y) | 0.7212 | 2442.15 | 2438.47 |
| 2 (Y) | 0.7524 | 2310.72 | 2307.64 |
| 3 (Y) | 0.7887 | 2189.21 | 2186.67 |
| 4 (Y) | 0.8198 | 2124.65 | 2122.49 |
| 5 (Y) | 0.8413 | 2129.52 | 2127.56 |
| 7 (Y) | 0.8548 | 2338.08 | 2336.37 |
| 10 (Y) | 0.8196 | 3121.67 | 3121.36 |
| 15 (Y) | 0.6904 | 6149.12 | 6180.34 |
| 20 (Y) | 0.5687 | 14,081.9 | 14,435.9 |
| 25 (Y) | 0.4987 | 36,162.0 | 38,663.4 |
| Angle, θ rad | kc | Grc |
|---|---|---|
| 0 (X-mag.) | 0.4172 | 13,193 |
| π/12 | 0.4424 | 11,964 |
| π/6 | 0.5155 | 8240.4 |
| π/4 | 0.6082 | 4813.4 |
| π/3 | 0.7015 | 3112.7 |
| 5π/12 | 0.7662 | 2389.4 |
| π (Y-mag.) | 0.7887 | 2189.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tagawa, T. Effect of the Direction of Uniform Horizontal Magnetic Field on the Linear Stability of Natural Convection in a Long Vertical Rectangular Enclosure. Symmetry 2020, 12, 1689. https://doi.org/10.3390/sym12101689
Tagawa T. Effect of the Direction of Uniform Horizontal Magnetic Field on the Linear Stability of Natural Convection in a Long Vertical Rectangular Enclosure. Symmetry. 2020; 12(10):1689. https://doi.org/10.3390/sym12101689
Chicago/Turabian StyleTagawa, Toshio. 2020. "Effect of the Direction of Uniform Horizontal Magnetic Field on the Linear Stability of Natural Convection in a Long Vertical Rectangular Enclosure" Symmetry 12, no. 10: 1689. https://doi.org/10.3390/sym12101689
APA StyleTagawa, T. (2020). Effect of the Direction of Uniform Horizontal Magnetic Field on the Linear Stability of Natural Convection in a Long Vertical Rectangular Enclosure. Symmetry, 12(10), 1689. https://doi.org/10.3390/sym12101689





