Complex Patterns to the (3+1)-Dimensional B-type Kadomtsev-Petviashvili-Boussinesq Equation
Abstract
1. Introduction
2. The SGEM
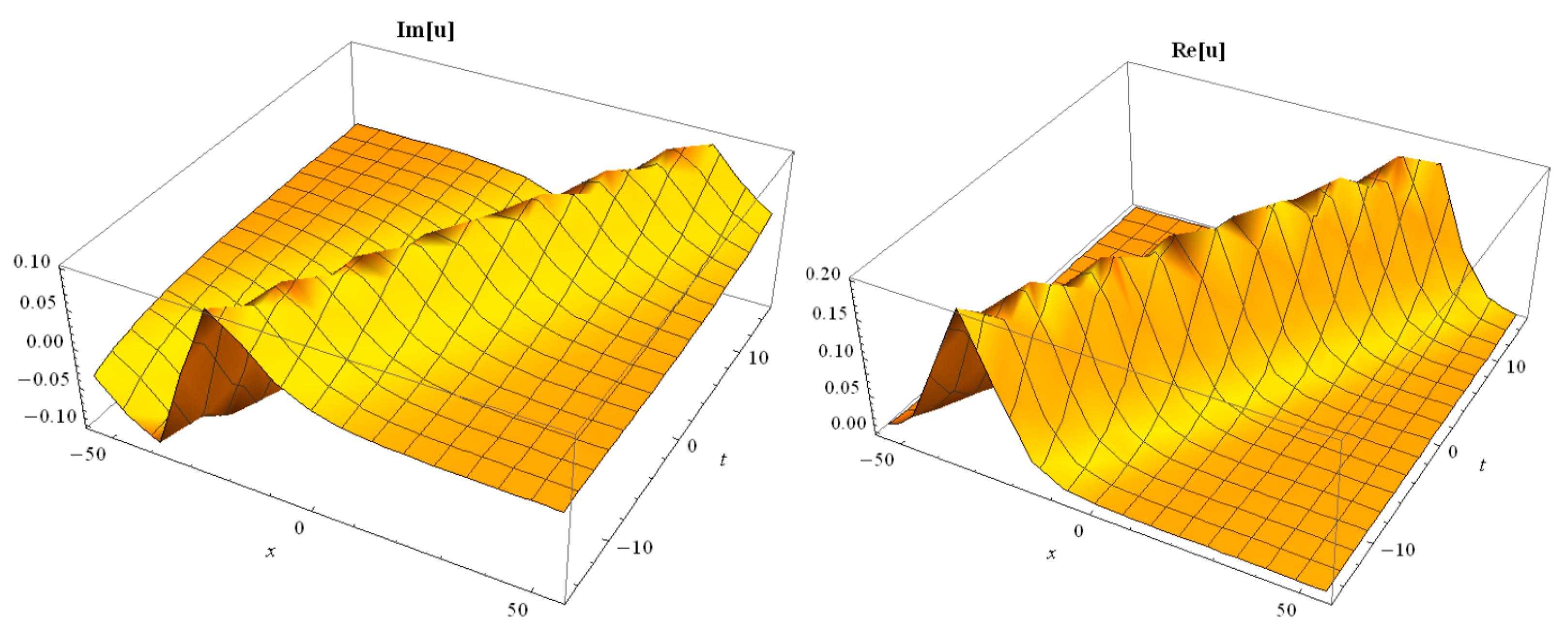
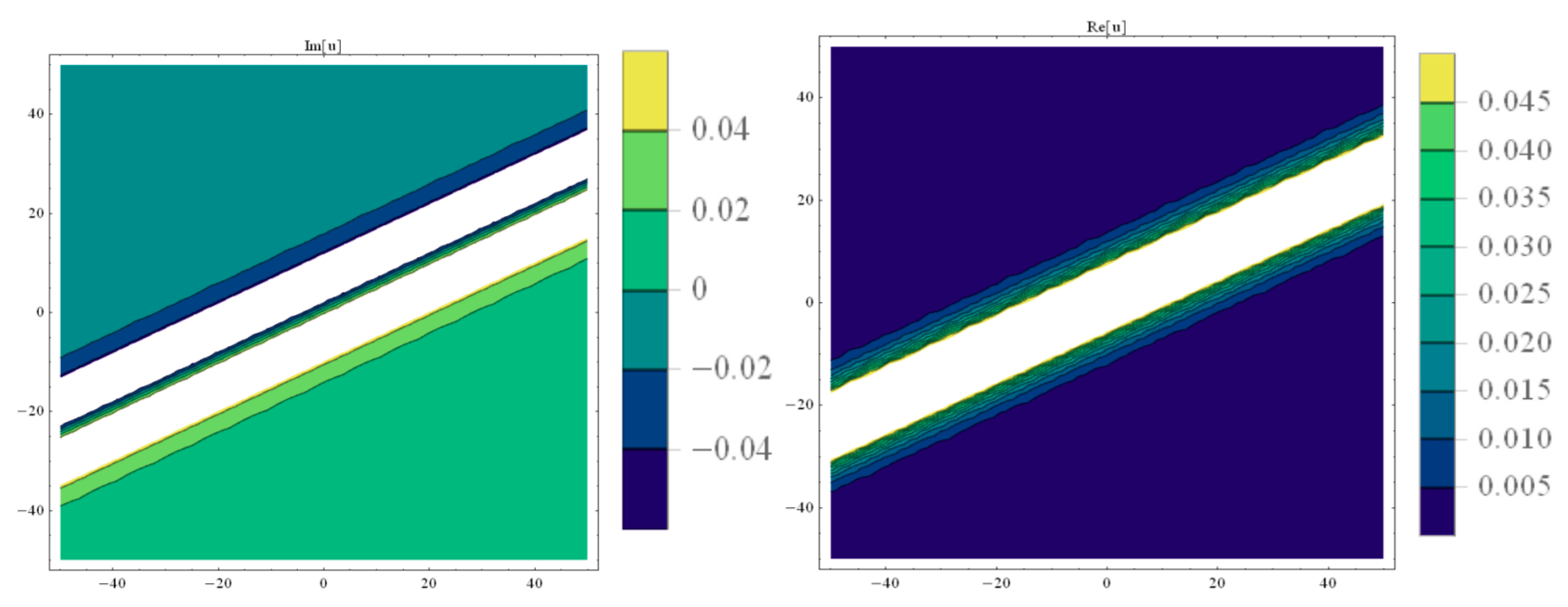
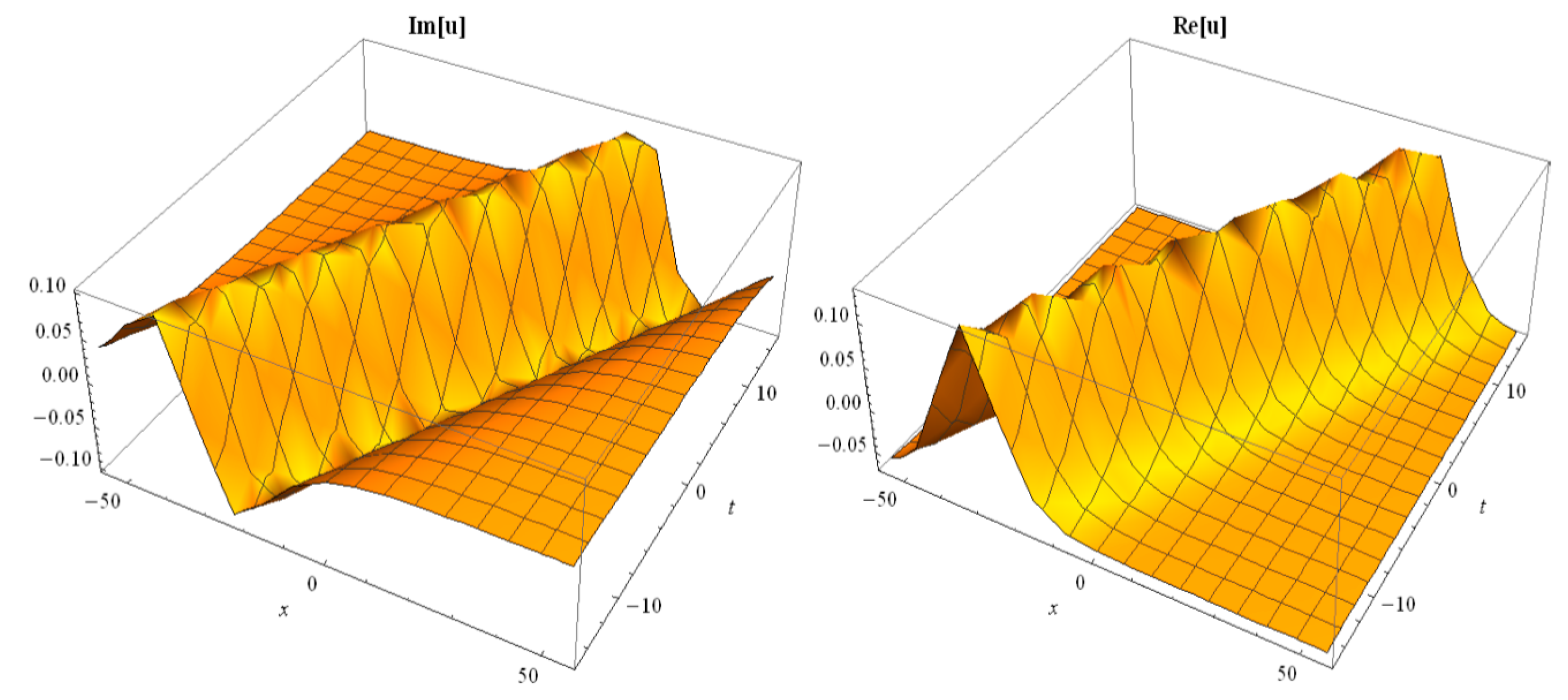
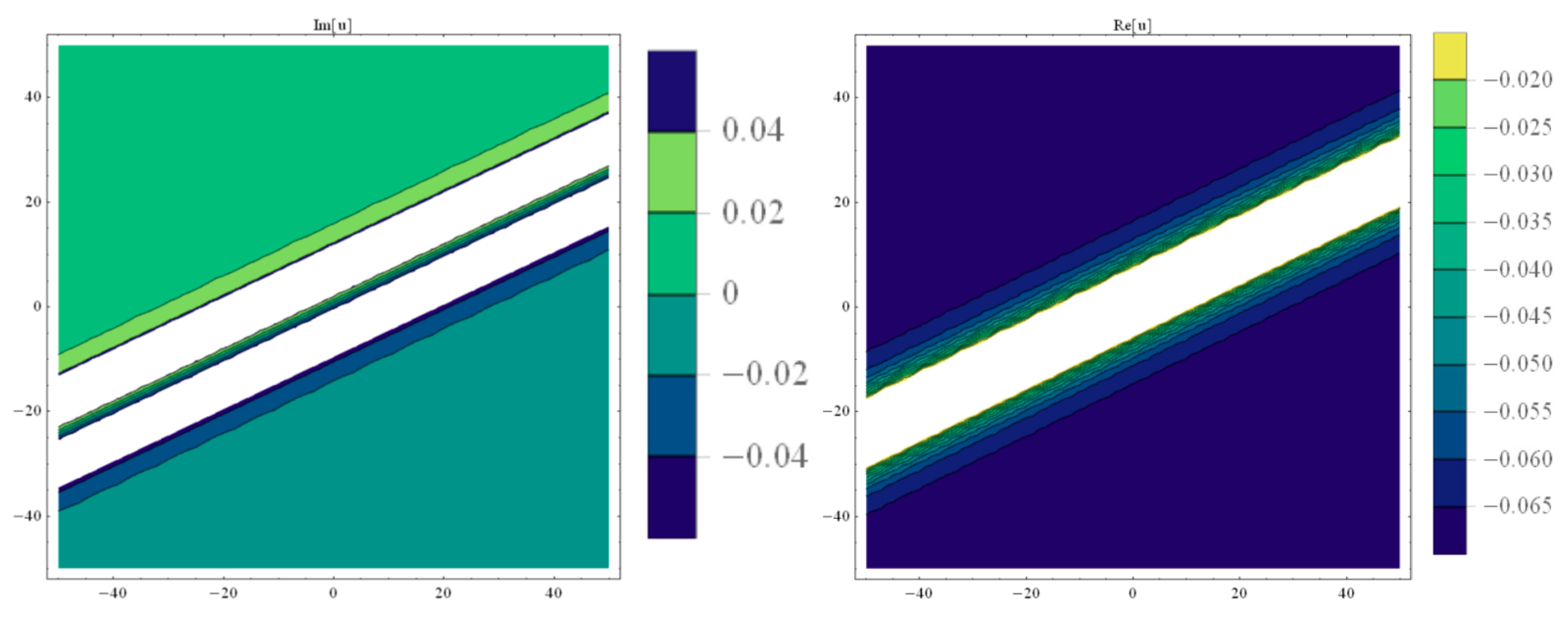
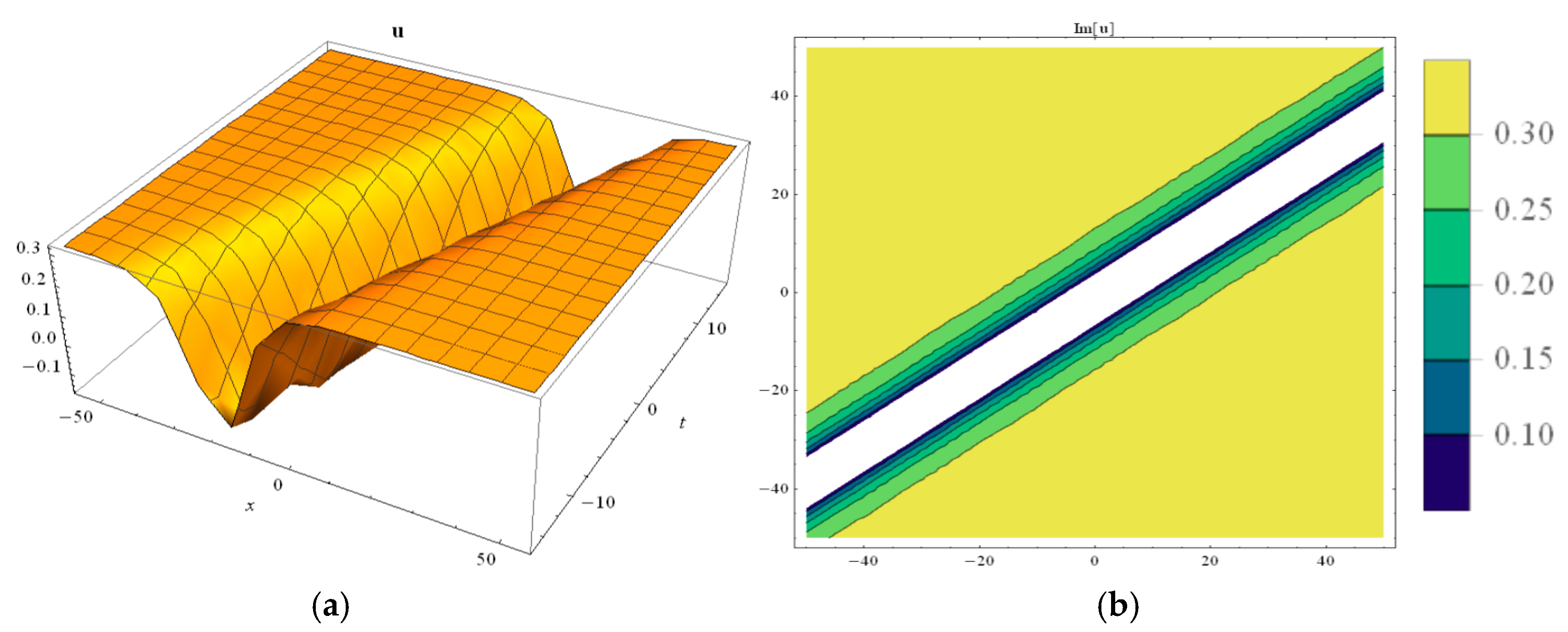
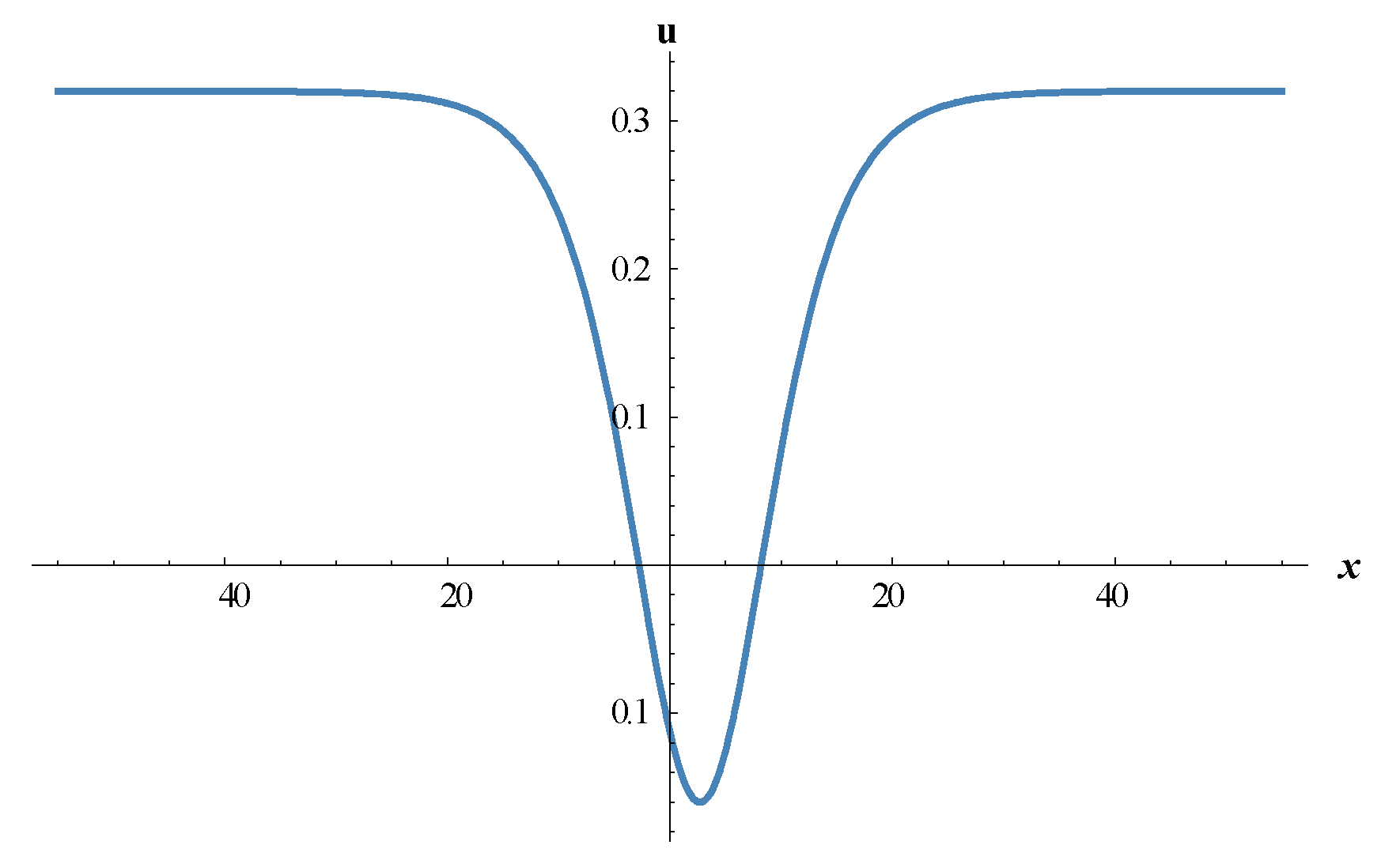
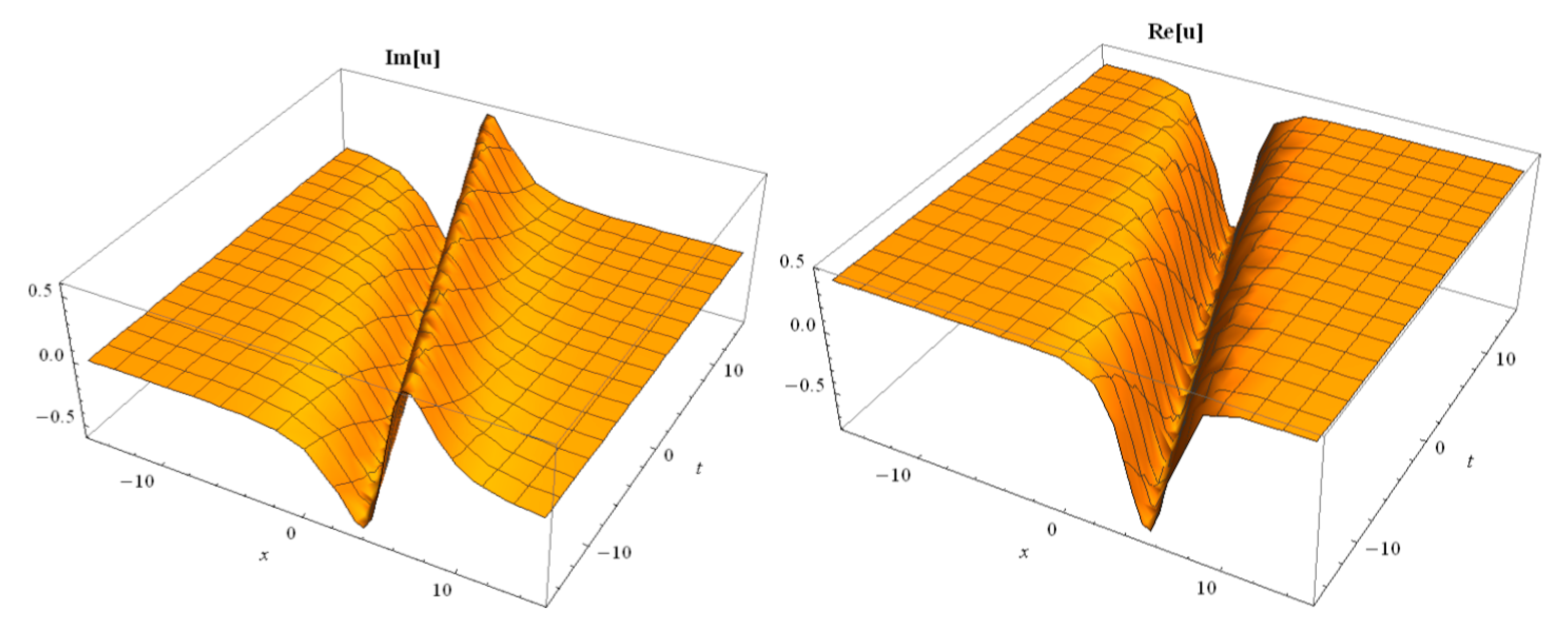
3. Application
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Biswas, A.; Yıldırım, Y.; Yaşar, E.; Zhou, Q.; Moshokoa, S.P.; Belic, M. Optical soliton solutions to Fokas-Lenells equations using some different methods. Optik 2018, 173, 21–31. [Google Scholar] [CrossRef]
- Wazwaz, A.M. New (3+1)-dimensional nonlinear evolution equations with mKdV equation constituting its main part: Multiple soliton solutions. Chaos Solitons Fractals 2015, 76, 93–97. [Google Scholar] [CrossRef]
- Abdullahi, A.R. Symbolic computation on exact solutions of a coupled Kadomtsev Petviashvili equation: Lie symmetry analysis and extended tanh method. Comput. Math. Appl. 2017, 74, 1897–1902. [Google Scholar]
- Wazwaz, A.M. New travelling wave solutions to the Boussinesq and the Klein Gordon equations. Commun. Nonlinear Sci. Numer. Simul. 2008, 13, 889–901. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The tanh method and the sine cosine method for solving the KP-MEW equation. Int. J. Comput. Math. 2007, 82, 235–246. [Google Scholar] [CrossRef]
- Vakhnenko, V.O.; Parkes, E.J.; Morrison, A.J. A Böcklund transformation and the inverse Scattering transform method for the generalized Vakhnenko equation. Chaos Solitons Fractals 2003, 17, 683–692. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Multiple complex and multiple real soliton solutions for the integrable sine Gordon equation. Optik 2018, 172, 622–627. [Google Scholar] [CrossRef]
- Khalique, C.M.; Mhlanga, I.E. Travelling waves and conservation laws of a (2+1)-dimensional coupling system with Korteweg-de Vries equation. Appl. Math. Nonlinear Sci. 2018, 3, 241–254. [Google Scholar] [CrossRef]
- Zhao, Z.; He, L. Multiple lump solutions of the (3+1)-dimensional potential Yu-Toda-Sasa-Fukuyama equation. Appl. Math. Lett. 2019, 95, 114–121. [Google Scholar] [CrossRef]
- Tala-Tebue, E.; Tsobgni-Fozap, D.C.; Kenfack-Jiotsa, A.; Kofane, T.C. Envelope periodic solutions for a discrete network with the Jacobi elliptic functions and the alternative (G’/G)-expansion method including the generalized Riccati equation. Eur. Phys. J. Plus 2014, 129, 136. [Google Scholar] [CrossRef]
- Kudryashov, N.A.; Sinelshchikov, D.I. New non-standard Lagrangians for the Lienard-type equations. Appl. Math. Lett. 2017, 63, 124–129. [Google Scholar] [CrossRef]
- Tala-Tebue, E.; Zayed, E.M.E. New Jacobi elliptic function solutions, solitons and other solutions for the (2+1)-dimensional nonlinear electrical transmission line equation. Eur. Phys. J. Plus 2018, 133, 1–10. [Google Scholar] [CrossRef]
- Fan, E. Extended tanh-function method and its applications to nonlinear equations. Phys. Lett. A 2000, 277, 212–219. [Google Scholar] [CrossRef]
- Parkes, E.J.; Duffy, B.R. An automated tanh-function method for finding solitary wave solutions to nonlinear evolution equations. Comput. Phys. Commun. 1998, 98, 288–300. [Google Scholar] [CrossRef]
- Baskonus, H.M.; Bulut, H.; Belgacem, F.B.M. Analytical solutions for nonlinear long-short wave interaction systems with highly complex structure. J. Comput. Appl. Math. 2017, 312, 257–266. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The extended tanh method for new solitons solutions for many forms of the fifth-order KdV equations. Appl. Math. Comput. 2007, 184, 1002–1014. [Google Scholar] [CrossRef]
- Qingling, G.; Xueqin, Z. A Generalized Tanh Method and its Application. Appl. Math. Sci. 2011, 5, 3789–3800. [Google Scholar]
- Whitham, G.B. Linear and Nonlinear Waves; Wiley: New York, NY, USA, 1974. [Google Scholar]
- Bulut, H.; Baskonus, H.M. On the complex structures of Kundu-Eckhaus equation via Improved Bernoulli sub-equation function method. Waves Random Complex Media 2015, 25, 720–728. [Google Scholar]
- Malfliet, W.; Hereman, W. The tanh method: II.Perturbation technique for conservative systems. Phys. Scr. 1996, 54, 569–575. [Google Scholar] [CrossRef]
- Malfliet, W. The tanh method a tool for solving certain classes of nonlinear evolution and wave equations. J. Comput. Appl. Math. 2004, 164–165, 529–541. [Google Scholar] [CrossRef]
- Wang, X.B.; Tian, S.F.; Yan, H.; Zhang, T.T. On the solitary waves, breather waves and rogue waves to a generalized (3+1)-dimensional Kadomtsev–Petviashvili equation. Comput. Math. Appl. 2017, 74, 556–563. [Google Scholar] [CrossRef]
- Gao, Y.T.; Tian, B. Generalized hyperbolic-function method with computerized Symbolic computation to construct the solitonic solutions to nonlinear equations of Mathematical physics. Comput. Phys. Commun. 2001, 133, 158–164. [Google Scholar] [CrossRef]
- Wu, X.Y.; Tian, B.; Chai, H.P.; Sun, Y. Rogue waves and lump solutions for a (3+1)-dimensional generalized B-type Kadomtsev Petviashvili equation in fluid mechanics. Mod. Phys. Lett. B 2017, 31, 1750122. [Google Scholar] [CrossRef]
- Kudryashov, N.A. A new note on exact complex travelling wave solutions for (2+1)-dimensional B-type Kadomtsev–Petviashvili equation. Appl. Math. Comput. 2010, 217, 2282–2284. [Google Scholar] [CrossRef]
- Wang, M.; Li, X.; Zhang, J. The (G’/G) expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Phys. Lett. A 2008, 372, 417–423. [Google Scholar] [CrossRef]
- Khalfallah, M. New Exact traveling wave solutions of the (2+1) dimensional Zakharov-Kuznetsov (ZK) equation. An. Stiintifice Ale Univ. Ovidius Constanta 2007, 15, 35–44. [Google Scholar]
- Raslan, K.R. Numerical Methods for Partial Differential Equations. Ph.D. Thesis, Al-Azhar University, Cairo, Egypt, 1999. [Google Scholar]
- Kudryashov, N.A. Traveling wave reduction of the modified KdV hierarchy: The Lax pair and the first integrals. Commun. Nonlinear Sci. Numer. Simul. 2019, 73, 472–480. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Solitary and periodic waves of the hierarchy for propagation pulse in optical fiber. Optik 2019, 194, 163060. [Google Scholar] [CrossRef]
- Kudryashov, N.A. On general solutions of two nonlinear ordinary differential equations. AIP Conf. Proc. 2019, 2116, 270002. [Google Scholar]
- Zhou, Y.; Cai, S.; Liu, Q. Bounded Traveling Waves of the (2+1)-Dimensional Zoomeron Equation. Math. Probl. Eng. 2015, 163597, 1–10. [Google Scholar] [CrossRef]
- Motsepa, T.; Khalique, C.M.; Gandarias, M.L. Symmetry Analysis and Conservation Laws of the Zoomeron Equation. Symmetry 2017, 9, 27. [Google Scholar] [CrossRef]
- Liua, S.; Fu, Z.; Liu, S. Exact solutions to sine-Gordon-type equations. Phys. Lett. A 2006, 351, 59–63. [Google Scholar] [CrossRef]
- Kaur, L.; Wazwaz, A.M. Dynamical Analysis of Lump Solutions for (3+1)-dimensional generalized KP-Boussinesq equation and Its Dimensionally Reduced equations. Phys. Scr. 2018, 93, 75–203. [Google Scholar] [CrossRef]
- Cheng, L.; Zhang, Y.; Ma, W.X. Pfaffians of B-type Kadomtsev–Petviashvili equation and complexitons to a class of nonlinear partial differential equations in (3++1) dimensions. Pramana 2019, 93, 1–10. [Google Scholar] [CrossRef]
- Malfliet, W. Solitary wave solutions of nonlinear wave equations. Am. J. Phys. 1992, 60, 650–654. [Google Scholar] [CrossRef]
- Al-Amr, M.O. Exact solutions of the generalized (2 + 1)-dimensional nonlinear evolution equations via the modified simple equation method. Comput. Math. Appl. 2014, 69, 390–397. [Google Scholar] [CrossRef]
- Pandey, P.K. Solution of two point boundary value problems, a numerical approach: Parametric difference method. Appl. Math. Nonlinear Sci. 2018, 3, 649–658. [Google Scholar] [CrossRef]
- Pandey, P.K.; Jaboob, S.S.A. A finite difference method for a numerical solution of elliptic boundary value problems. Appl. Math. Nonlinear Sci. 2018, 3, 311–320. [Google Scholar] [CrossRef]
- Raslan, K.R.; Evans, D.J. The tanh function method for solving some important non-linear partial differential equations. Int. J. Comput. Math. 2005, 82, 897–905. [Google Scholar]
- Raslan, K.R.; Ali, K.K.; Shallal, M.A. The modified extended tanh method with the Riccati equation for solving the space-time fractional EW and MEW equations. Chaos Solitons Fractals 2017, 103, 404–409. [Google Scholar] [CrossRef]
- Sirendaoreji. New exact travelling wave solutions to three nonlinear evolution equations. Appl. Math. A J. Chin. Univ. 2004, 19, 178–186. [Google Scholar]
- Zhang, Y.; Pang, J. Lump and Lump-Type Solutions of the Generalized (3+1)-Dimensional Variable -Coefficient B-Type Kadomtsev-Petviashvili Equation. J. Appl. Math. 2019, 7172860, 1–10. [Google Scholar] [CrossRef]
- Cattani, C.; Sulaiman, T.A.; Baskonus, H.M.; Bulut, H. On the soliton solutions to the Nizhnik-Novikov- Veselov and the Drinfel’d-Sokolov systems. Opt. Quantum Electron. 2018, 50, 138. [Google Scholar] [CrossRef]
- Zhao, Z.; Han, B. Lump solutions of a (3+1)-dimensional B-type KP equation and its dimensionally reduced equations. Anal. Math. Phys. 2019, 9, 119–130. [Google Scholar] [CrossRef]
- Pandey, P.K. A new computational algorithm for the solution of second order initial value problems in ordinary differential equations. Appl. Math. Nonlinear Sci. 2018, 3, 167–174. [Google Scholar] [CrossRef]
- Eskitascioglu, E.I.; Aktas, M.B.; Baskonus, H.M. New Complex and Hyperbolic Forms for Ablowitz-Kaup-Newell-Segur Wave Equation with Fourth Order. Appl. Math. Nonlinear Sci. 2019, 4, 105–112. [Google Scholar]
- Baskonus, H.M.; Bulut, H.; Sulaiman, T.A. New Complex Hyperbolic Structures to the Lonngren-Wave Equation by Using Sine-Gordon Expansion Method. Appl. Math. Nonlinear Sci. 2019, 4, 141–150. [Google Scholar] [CrossRef]
- Wazwaz, A.M.; El-Tantawy, S.A. Solving the (3+1)-dimensional KP- Boussinesq and BKP-Boussinesq. Equations by the simplified Hirota’s method. Nonlinear Dyn. 2017, 88, 3017–3021. [Google Scholar] [CrossRef]
- Deng, Y.S.; Tian, B.; Sun, Y.; Zhang, C.R.; Hu, C. Rational and semi-rational solutions for the (3+1)-dimensional B-type Kadomtsev Petviashvili Boussinesq equation. Mod. Phys. Lett. B 2019, 33, 1950296. [Google Scholar] [CrossRef]
- Bulut, H.; Baskonus, H.M. New wave behaviors of the system of equations for the ion Sound and Langmuir waves. Waves Random Complex Media 2016, 26, 613–625. [Google Scholar]
- Baskonus, H.M. New acoustic wave behaviors to the Davey-Stewartson equation with power-law nonlinearity arising in fluid dynamics. Nonlinear Dyn. 2016, 86, 177–183. [Google Scholar] [CrossRef]
- Weisstein, E.W. Concise Encyclopedia of Mathematics, 2nd ed.; CRC Press: New York, NY, USA, 2002. [Google Scholar]
- Nguyen, N.T.; Kalisch, H. Orbital stability of negative solitary waves. Math. Comput. Simul. 2009, 80, 139–150. [Google Scholar] [CrossRef][Green Version]

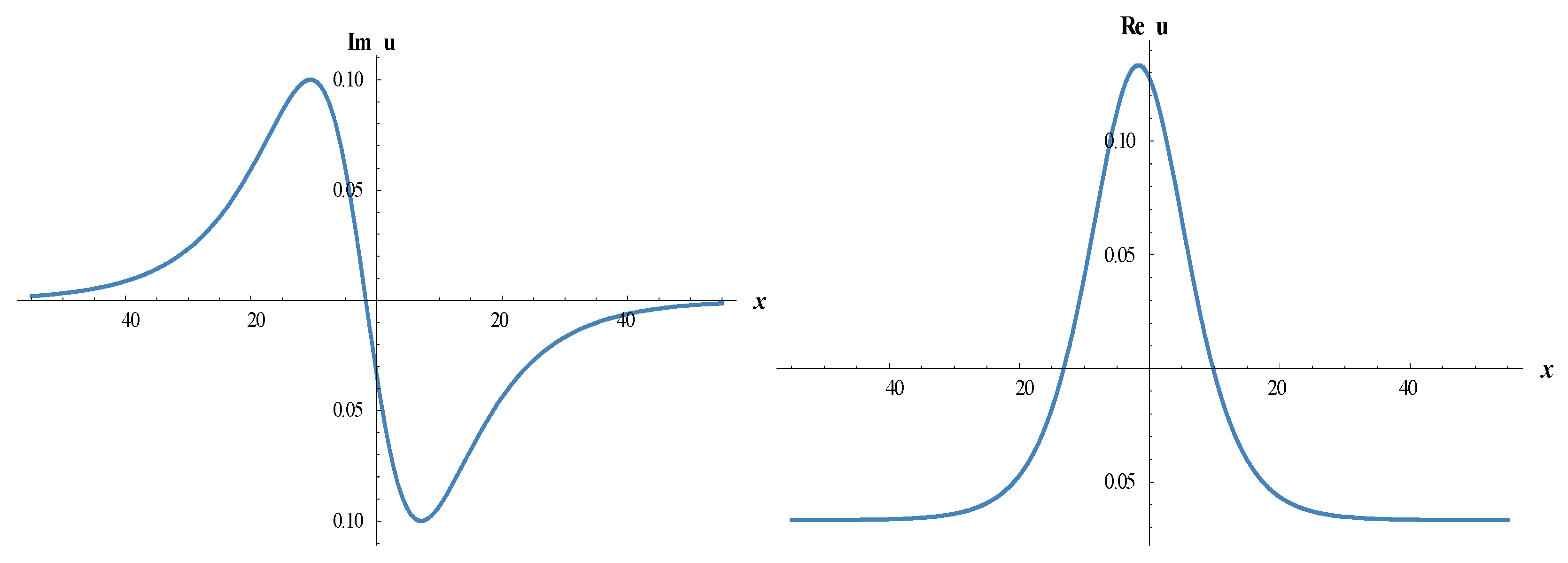


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
García Guirao, J.L.; Baskonus, H.M.; Kumar, A.; Rawat, M.S.; Yel, G. Complex Patterns to the (3+1)-Dimensional B-type Kadomtsev-Petviashvili-Boussinesq Equation. Symmetry 2020, 12, 17. https://doi.org/10.3390/sym12010017
García Guirao JL, Baskonus HM, Kumar A, Rawat MS, Yel G. Complex Patterns to the (3+1)-Dimensional B-type Kadomtsev-Petviashvili-Boussinesq Equation. Symmetry. 2020; 12(1):17. https://doi.org/10.3390/sym12010017
Chicago/Turabian StyleGarcía Guirao, Juan Luis, H. M. Baskonus, Ajay Kumar, M. S. Rawat, and Gulnur Yel. 2020. "Complex Patterns to the (3+1)-Dimensional B-type Kadomtsev-Petviashvili-Boussinesq Equation" Symmetry 12, no. 1: 17. https://doi.org/10.3390/sym12010017
APA StyleGarcía Guirao, J. L., Baskonus, H. M., Kumar, A., Rawat, M. S., & Yel, G. (2020). Complex Patterns to the (3+1)-Dimensional B-type Kadomtsev-Petviashvili-Boussinesq Equation. Symmetry, 12(1), 17. https://doi.org/10.3390/sym12010017






