Two-Phase Couette Flow of Couple Stress Fluid with Temperature Dependent Viscosity Thermally Affected by Magnetized Moving Surface
Abstract
1. Introduction
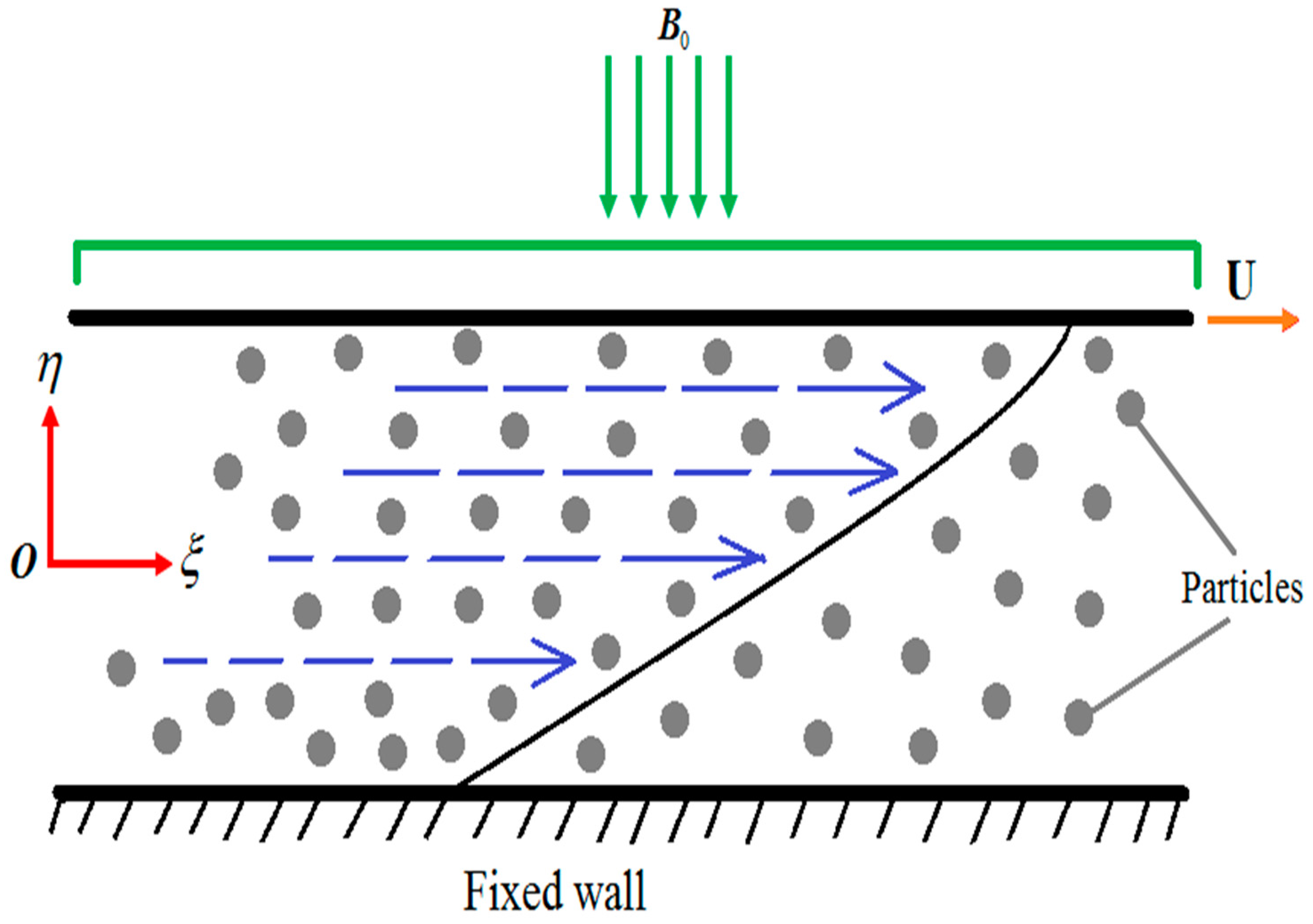
2. Mathematical Analysis
Boundary Conditions
3. Results and Discussion
3.1. Variable Viscosity
3.2. Numerical Procedure
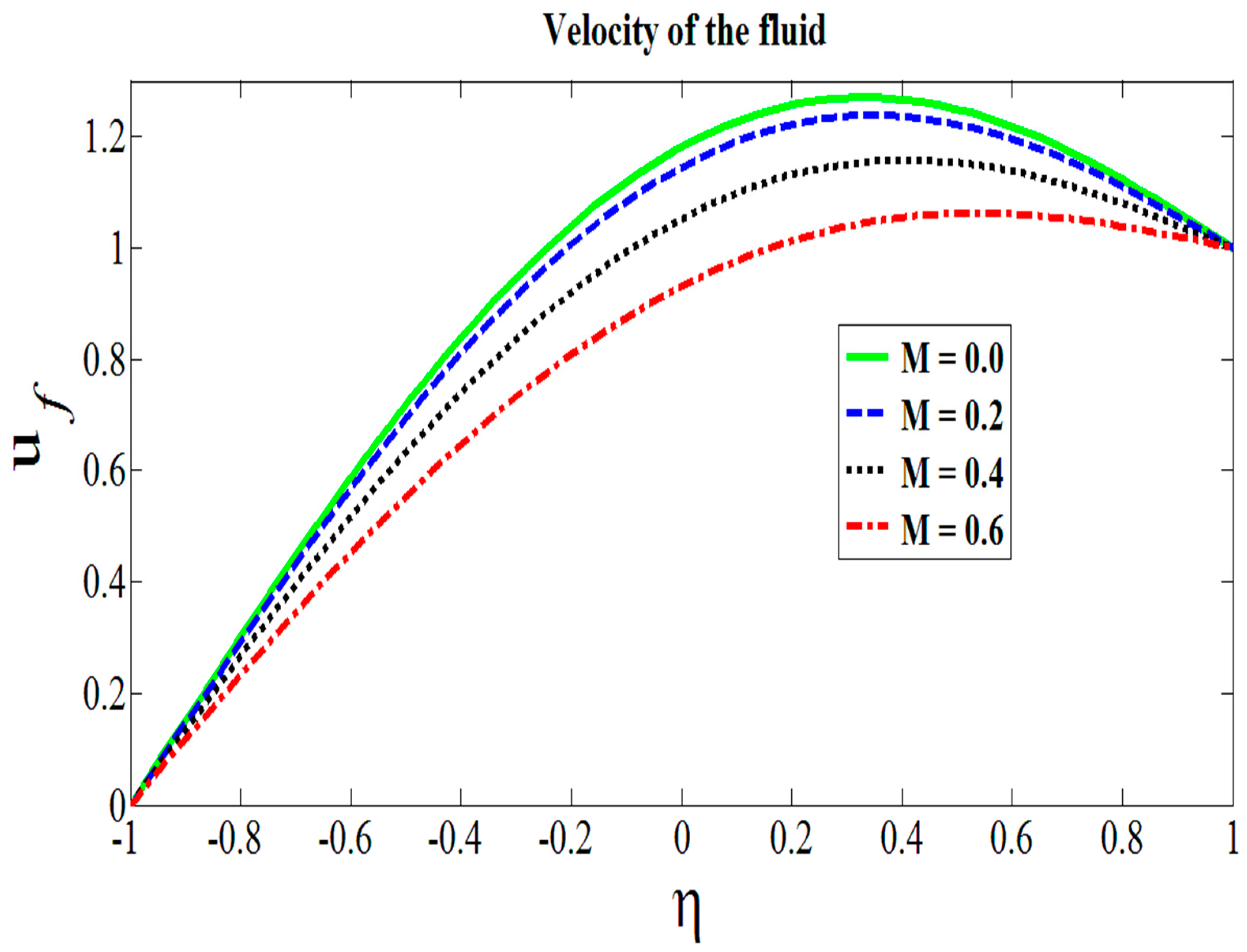
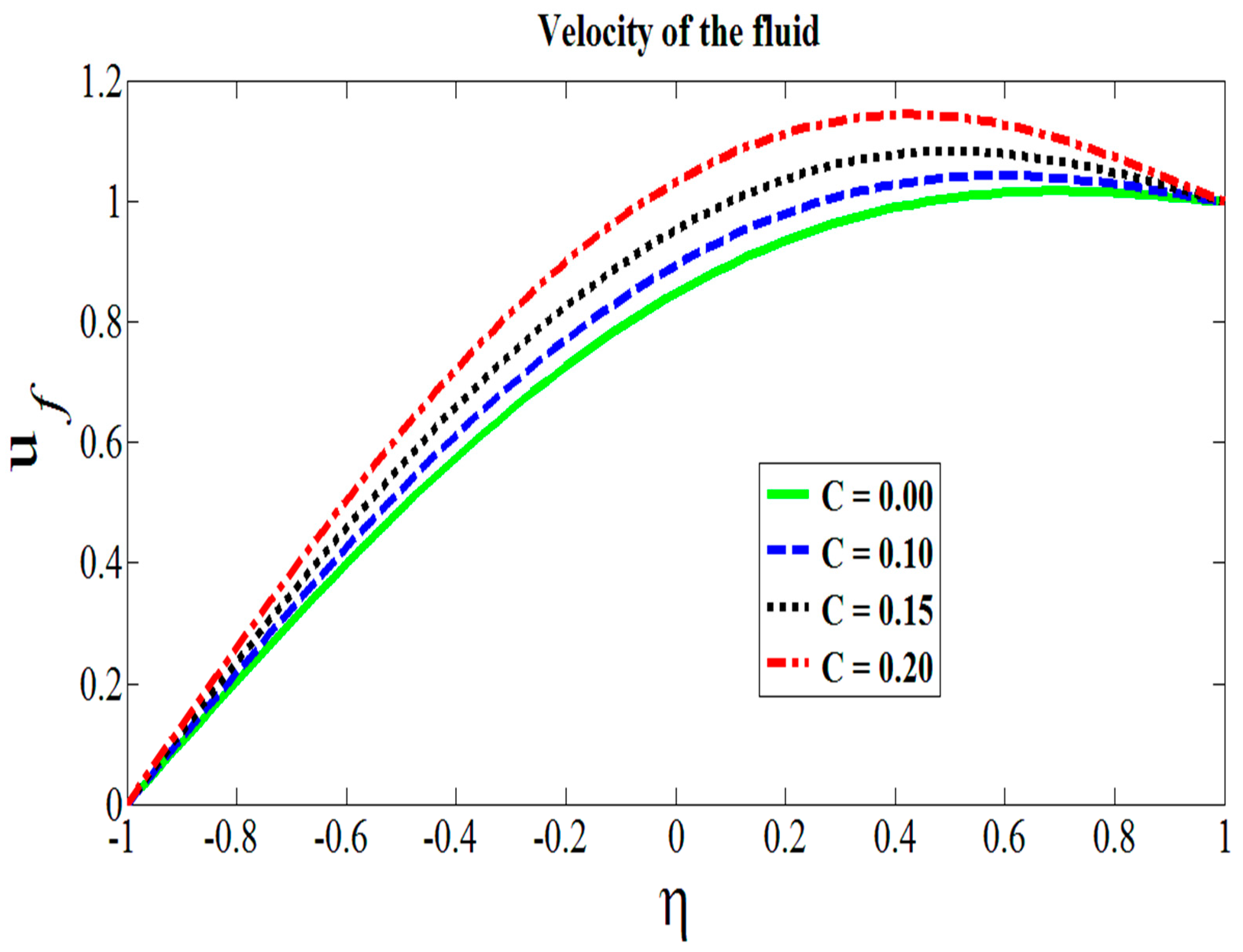
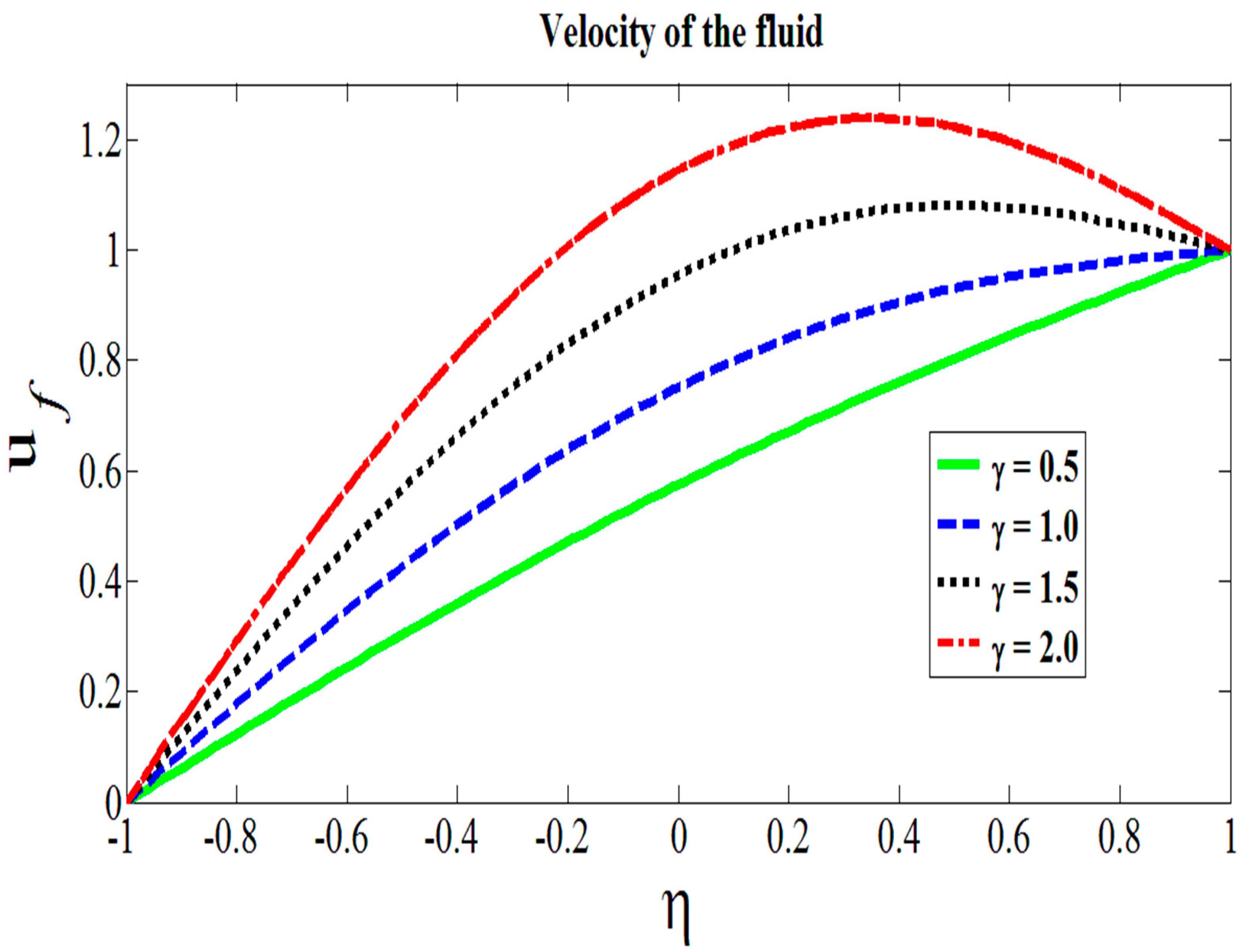
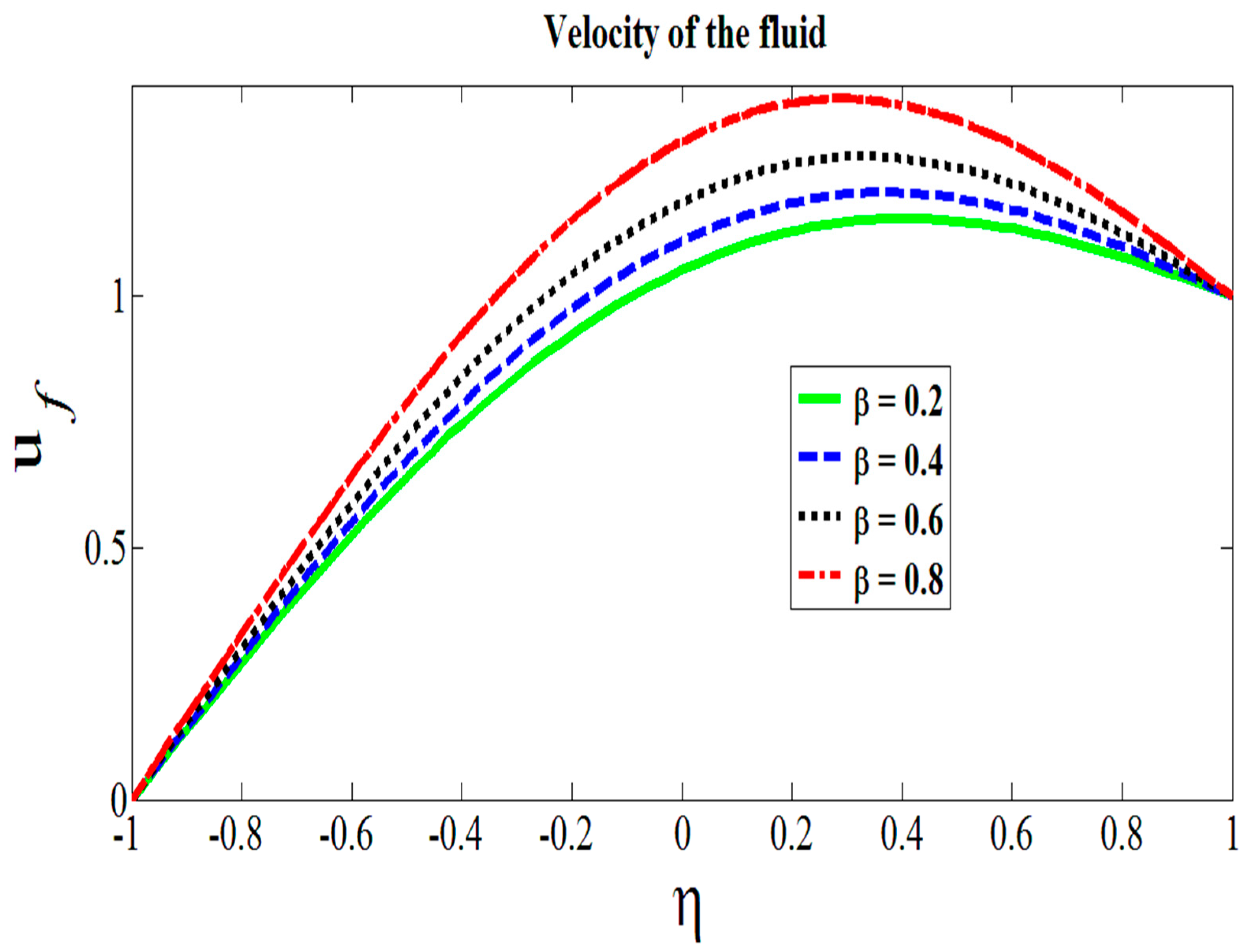
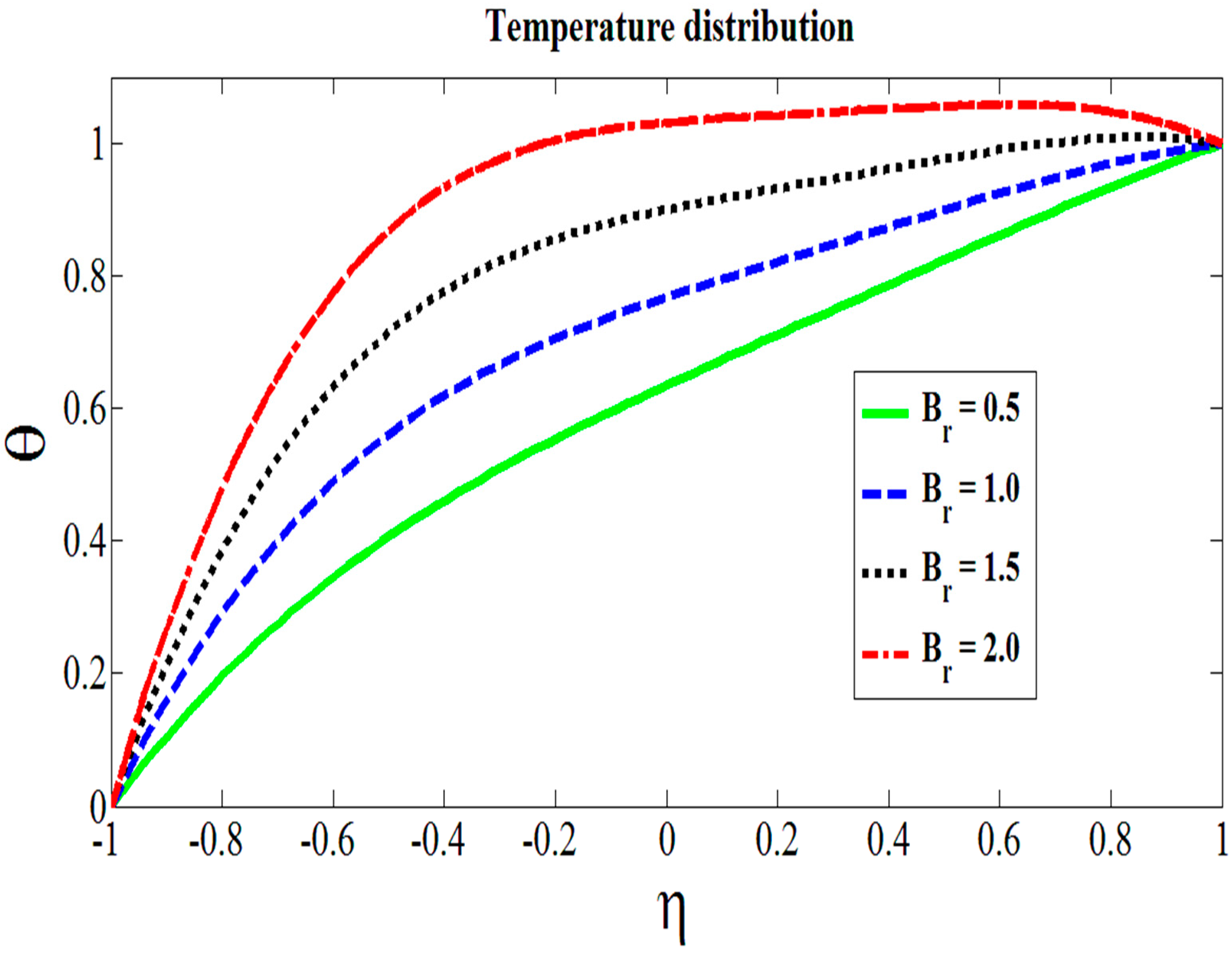
3.3. Graphical Illustration
3.4. Validation
4. Conclusions
- ⮚
- The flow of couple stress fluid resists for increasing values of Hartmann number.
- ⮚
- The temperature effectively variates the viscosity of the fluid to cause the shear thinning effects.
- ⮚
- The temperature of the flow mounts in response of higher values of Brinkman number.
- ⮚
- Attenuation of the viscosity results to expedite the flows.
- ⮚
- Viscosity parameter brings celerity in the velocity of bi-phase fluid due to high temperature difference.
- ⮚
- Molecules additives of base fluid reduce the force of friction and hence in both phases the velocity is galvanized.
- ⮚
- Due to the immense applications of multiphase flows in industrial and pharmaceutical, the proposed theoretical model is now available to vet relevant experimental investigations.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Farooq, M.; Rahim, M.T.; Islam, S.; Siddiqui, A.M. Steady Poiseuille flow and heat transfer of couple stress fluids between two parallel inclined plates with variable viscosity. J. Assoc. Arab Univ. Basic Appl. Sci. 2013, 14, 9–18. [Google Scholar] [CrossRef][Green Version]
- Mahabaleshwar, U.S.; Sarris, I.E.; Hill, A.; Lorenzini, G.; Pop, I. An MHD couple stress fluid due to a perforated sheet undergoing linear stretching with heat transfer. Int. J. Heat Mass. Transf. 2017, 105, 157–167. [Google Scholar] [CrossRef]
- Saad, H.; Ashmawy, E.A. Unsteady plane Couette flow of an incompressible couple stress fluid with slip boundary conditions. Int. J. Med. Health Sci. 2016, 3, 85–92. [Google Scholar] [CrossRef]
- Akhtar, S.; Shah, N.A. Exact solutions for some unsteady flows of a couple stress fluid between parallel plates. Ain Shams Eng. J. 2018, 9, 985–992. [Google Scholar] [CrossRef]
- Khan, N.A.; Khan, H.; Ali, S.A. Exact solutions for MHD flow of couple stress fluid with heat transfer. J. Egypt. Math. Soc. 2016, 24, 125–129. [Google Scholar] [CrossRef]
- Asghar, S.; Ahmad, A. Unsteady Couette flow of viscous fluid under a non-uniform magnetic field. Appl. Math. Lett. 2012, 25, 1953–1958. [Google Scholar] [CrossRef]
- Shaowei, W.; Mingyu, X. Exact solution on unsteady Couette flow of generalized Maxwell fluid with fractional derivative. Acta Mech. 2006, 187, 103–112. [Google Scholar] [CrossRef]
- Eegunjobi, A.S.; Makinde, O.D.; Tshehla, M.S.; Franks, O. Irreversibility analysis of unsteady Couette flow with variable viscosity. J. Hydrodyn. B 2015, 27, 304–310. [Google Scholar] [CrossRef]
- Ellahi, R.; Wang, X.; Hameed, M. Effects of heat transfer and nonlinear slip on the steady flow of Couette fluid by means of Chebyshev Spectral Method. Z. Naturforsch A. 2014, 69, 1–8. [Google Scholar] [CrossRef]
- Ellahi, R.; Shivanian, E.; Abbasbandy, S.; Hayat, T. Numerical study of magnetohydrodynamics generalized Couette flow of Eyring-Powell fluid with heat transfer and slip condition. Int. J. Numer. Method Heat Fluid Flow 2016, 26, 1433–1445. [Google Scholar] [CrossRef]
- Zeeshan, A.; Shehzad, N.; Ellahi, R. Analysis of activation energy in Couette-Poiseuille flow of nanofluid in the presence of chemical reaction and convective boundary conditions. Results Phys. 2018, 8, 502–512. [Google Scholar] [CrossRef]
- Shehzad, N.; Zeeshan, A.; Ellahi, R. Electroosmotic flow of MHD Power law Al2O3-PVC nanofluid in a horizontal channel: Couette-Poiseuille flow model. Commun. Theor. Phys. 2018, 69, 655–666. [Google Scholar] [CrossRef]
- Hussain, F.; Ellahi, R.; Zeeshan, A.; Vafai, K. Modelling study on heated couple stress fluid peristaltically conveying gold nanoparticles through coaxial tubes: A remedy for gland tumors and arthritis. J. Mol. Liq. 2018, 268, 149–155. [Google Scholar] [CrossRef]
- Ellahi, R.; Zeeshan, A.; Hussain, F.; Asadollahi, A. Peristaltic blood flow of couple stress fluid suspended with nanoparticles under the influence of chemical reaction and activation energy. Symmetry 2019, 11, 276. [Google Scholar] [CrossRef]
- Ellahi, R.; Bhatti, M.M.; Fetecau, C.; Vafai, K. Peristaltic flow of couple stress fluid in a non-uniform rectangular duct having compliant walls. Commun. Theor. Phys. 2016, 65, 66–72. [Google Scholar] [CrossRef]
- Poply, V.; Singh, P.; Yadav, A.K. A study of Temperature-dependent fluid properties on MHD free stream flow and heat transfer over a non-linearly stretching sheet. Procedia Eng. 2015, 127, 391–397. [Google Scholar] [CrossRef]
- Ellahi, R.; Raza, M.; Vafai, K. Series solutions of non-Newtonian nanofluids with Reynolds model and Vogel’s model by means of the homotopy analysis method. Math. Comput. Model. 2012, 55, 1876–1891. [Google Scholar] [CrossRef]
- Disu, A.B.; Dada, M.S. Reynolds model viscosity on radiative MHD flow in a porous medium between two vertical wavy walls. J. Taibah Univ. Sci. 2017, 11, 548–565. [Google Scholar] [CrossRef][Green Version]
- Nadeem, S.; Ali, M. Analytical solutions for pipe flow of a fourth-grade fluid with Reynolds and Vogel’s models of viscosities. Commun. Nonlin. Sci. Numer. Simulat. 2009, 14, 2073–2090. [Google Scholar] [CrossRef]
- Ellahi, R.; Zeeshan, A.; Hussain, F.; Abbas, T. Thermally charged MHD bi-phase flow coatings with non-Newtonian nanofluid and Hafnium particles through slippery walls. Coatings 2019, 9, 300. [Google Scholar] [CrossRef]
- Mahmoud, M.A. Chemical reaction and variable viscosity effects on flow and mass transfer of a non-Newtonian visco-elastic fluid past a stretching surface embedded in a porous medium. Meccanica 2010, 45, 835–846. [Google Scholar] [CrossRef]
- Makinde, O.D. Laminar falling liquid film with variable viscosity along an inclined heated plate. Appl. Math. Comput. 2006, 175, 80–88. [Google Scholar] [CrossRef]
- Jawad, M.; Shah, Z.; Islam, S.; Majdoubi, J.; Tlili, I.; Khan, W.; Khan, I. Impact of nonlinear thermal radiation and the viscous dissipation effect on the unsteady three-dimensional rotating flow of single-wall carbon nanotubes with aqueous suspensions. Symmetry 2019, 11, 207. [Google Scholar] [CrossRef]
- Ellahi, R. A study on the convergence of series solution of non-Newtonian third grade fluid with variable viscosity: By means of homotopy analysis method. Adv. Math. Phys. 2012, 2012, 634925. [Google Scholar] [CrossRef]
- Karimipour, A.; Orazio, A.D.; Shadloo, M.S. The effects of different nano particles of Al2O3 and Ag on the MHD nano fluid flow and heat transfer in a microchannel including slip velocity and temperature jump. Phys. E 2017, 86, 146–153. [Google Scholar] [CrossRef]
- Hosseini, S.M.; Safaei, M.R.; Goodarzi, M.; Alrashed, A.A.A.A.; Nguyen, T.K. New temperature, interfacial shell dependent dimensionless model for thermal conductivity of nanofluids. Int. J. Heat Mass Transf. 2017, 114, 207–210. [Google Scholar] [CrossRef]
- Nasiri, H.; Jamalabadi, M.Y.A.; Sadeghi, R.; Safaei, M.R.; Nguyen, T.K.; Shadloo, M.S. A smoothed particle hydrodynamics approach for numerical simulation of nano-fluid flows. J. Therm. Anal. Calorim. 2018, 1–9. [Google Scholar] [CrossRef]
- Safaei, M.R.; Ahmadi, G.; Goodarzi, M.S.; Shadloo, M.S.; Goshayeshi, H.R.; Dahari, M. Heat transfer and pressure drop in fully developed turbulent flows of graphene nanoplatelets–silver/water nanofluids. Fluids 2016, 1, 20. [Google Scholar] [CrossRef]
- Sadiq, M.A. MHD stagnation point flow of nanofluid on a plate with anisotropic slip. Symmetry 2019, 11, 132. [Google Scholar] [CrossRef]
- Rashidi, S.; Esfahani, J.A.; Ellahi, R. Convective heat transfer and particle motion in an obstructed duct with two side by side obstacles by means of DPM model. Appl. Sci. 2017, 7, 431. [Google Scholar] [CrossRef]
- Shehzad, N.; Zeeshan, A.; Ellahi, R.; Rashidid, S. Modelling study on internal energy loss due to entropy generation for non-Darcy Poiseuille flow of silver-water nanofluid: An application of purification. Entropy 2018, 20, 851. [Google Scholar] [CrossRef]
- Hassan, M.; Ellahi, R.; Bhatti, M.M.; Zeeshan, A. A comparative study of magnetic and non-magnetic particles in nanofluid propagating over a wedge. Can. J. Phys. 2019, 97, 277–285. [Google Scholar] [CrossRef]
- Zeeshan, A.; Shehzad, N.; Abbas, A.; Ellahi, R. Effects of radiative electro-magnetohydrodynamics diminishing internal energy of pressure-driven flow of titanium dioxide-water nanofluid due to entropy generation. Entropy 2019, 21, 236. [Google Scholar] [CrossRef]
- Ashrafi, N.; Khayat, R.E. A low-dimensional approach to nonlinear plane-Couette flow of viscoelastic fluids. Phys. Fluids. 2000, 12, 345–365. [Google Scholar] [CrossRef]
- Srivastava, L.M.; Srivastava, V.P. Peristaltic transport of a particle-fluid suspension. J. Biomech. Eng. 1989, 111, 157–165. [Google Scholar] [CrossRef]
- Tam, C.K.W. The drag on a cloud of spherical particles in a low Reynolds number flow. J. Fluid Mech. 1969, 38, 537–546. [Google Scholar] [CrossRef]
- Ellahi, R. The effects of MHD and temperature dependent viscosity on the flow of non-Newtonian nanofluid in a pipe: Analytical solutions. Appl. Math. Model. 2013, 37, 1451–1457. [Google Scholar] [CrossRef]
- Hossain, M.A.; Subba, R.; Gorla, R. Natural convection flow of non-Newtonian power-law fluid from a slotted vertical isothermal surface. Int. J Numer. Methods Heat Fluid Flow 2009, 19, 835–846. [Google Scholar] [CrossRef]
- Makinde, O.D.; Onyejekwe, O.O. A numerical study of MHD generalized Couette flow and heat transfer with variable viscosity and electrical conductivity. J. Magn. Magn. Mater. 2011, 323, 2757–2763. [Google Scholar] [CrossRef]
- Coelho, M.P.; Faria, J.S. On the generalized Brinkman number definition and its importance for Bingham fluids. J. Heat Transf. 2011, 133, 545051–545055. [Google Scholar] [CrossRef]
- Swarnalathamma, B.V.; Krishna, M.V. Peristaltic hemodynamic flow of couple stress fluid through a porous medium under the influence of magnetic field with slip effect. AIP Conf. Proc. 2016, 1728, 0206031–0206039. [Google Scholar] [CrossRef]
- Charm, S.E.; Kurland, G.S. Blood Flow and Microcirculation; Wiley: New York, NY, USA, 1974. [Google Scholar]

Newtonian Fluid | Couple Stress Fluid | Newtonian Fluid | Couple Stress Fluid | |
|---|---|---|---|---|
| −1.0 | 1.0000 | 1.0000 | 0.0000 | 0.0000 |
| −0.6 | 1.2000 | 1.3221 | 0.2000 | 0.3221 |
| −0.2 | 1.4000 | 1.5826 | 0.4000 | 0.5826 |
| 0.2 | 1.6000 | 1.7698 | 0.6000 | 0.7698 |
| 0.6 | 1.8000 | 1.8998 | 0.8000 | 0.8998 |
| 1.0 | 2.0000 | 2.0000 | 1.0000 | 1.0000 |
Single Phase | Solid–Liquid Phase | Solid–Liquid Phase | |
|---|---|---|---|
| −1.0 | 0.0000 | 1.0000 | 0.0000 |
| −0.6 | 0.2741 | 1.3221 | 0.3221 |
| −0.2 | 0.5117 | 1.5826 | 0.5826 |
| 0.2 | 0.7047 | 1.7698 | 0.7698 |
| 0.6 | 0.8618 | 1.8998 | 0.8998 |
| 1.0 | 1.0000 | 2.0000 | 1.0000 |
| −1.0 | 0.0000 | 0.0000 | 0.0000 | 0.0000 |
| −0.6 | 0.2000 | 0.3916 | 0.3512 | 0.3578 |
| −0.2 | 0.4000 | 0.6066 | 0.5629 | 0.5870 |
| 0.2 | 0.6000 | 0.7528 | 0.7095 | 0.7504 |
| 0.6 | 0.8000 | 0.8785 | 0.8446 | 0.8830 |
| 1.0 | 1.0000 | 1.0000 | 1.0000 | 1.0000 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ellahi, R.; Zeeshan, A.; Hussain, F.; Abbas, T. Two-Phase Couette Flow of Couple Stress Fluid with Temperature Dependent Viscosity Thermally Affected by Magnetized Moving Surface. Symmetry 2019, 11, 647. https://doi.org/10.3390/sym11050647
Ellahi R, Zeeshan A, Hussain F, Abbas T. Two-Phase Couette Flow of Couple Stress Fluid with Temperature Dependent Viscosity Thermally Affected by Magnetized Moving Surface. Symmetry. 2019; 11(5):647. https://doi.org/10.3390/sym11050647
Chicago/Turabian StyleEllahi, Rahmat, Ahmed Zeeshan, Farooq Hussain, and Tehseen Abbas. 2019. "Two-Phase Couette Flow of Couple Stress Fluid with Temperature Dependent Viscosity Thermally Affected by Magnetized Moving Surface" Symmetry 11, no. 5: 647. https://doi.org/10.3390/sym11050647
APA StyleEllahi, R., Zeeshan, A., Hussain, F., & Abbas, T. (2019). Two-Phase Couette Flow of Couple Stress Fluid with Temperature Dependent Viscosity Thermally Affected by Magnetized Moving Surface. Symmetry, 11(5), 647. https://doi.org/10.3390/sym11050647







