Numerical Analysis of Boundary Layer Flow Adjacent to a Thin Needle in Nanofluid with the Presence of Heat Source and Chemical Reaction
Abstract
1. Introduction
2. Governing Formula and Modeling
3. Stability Analysis
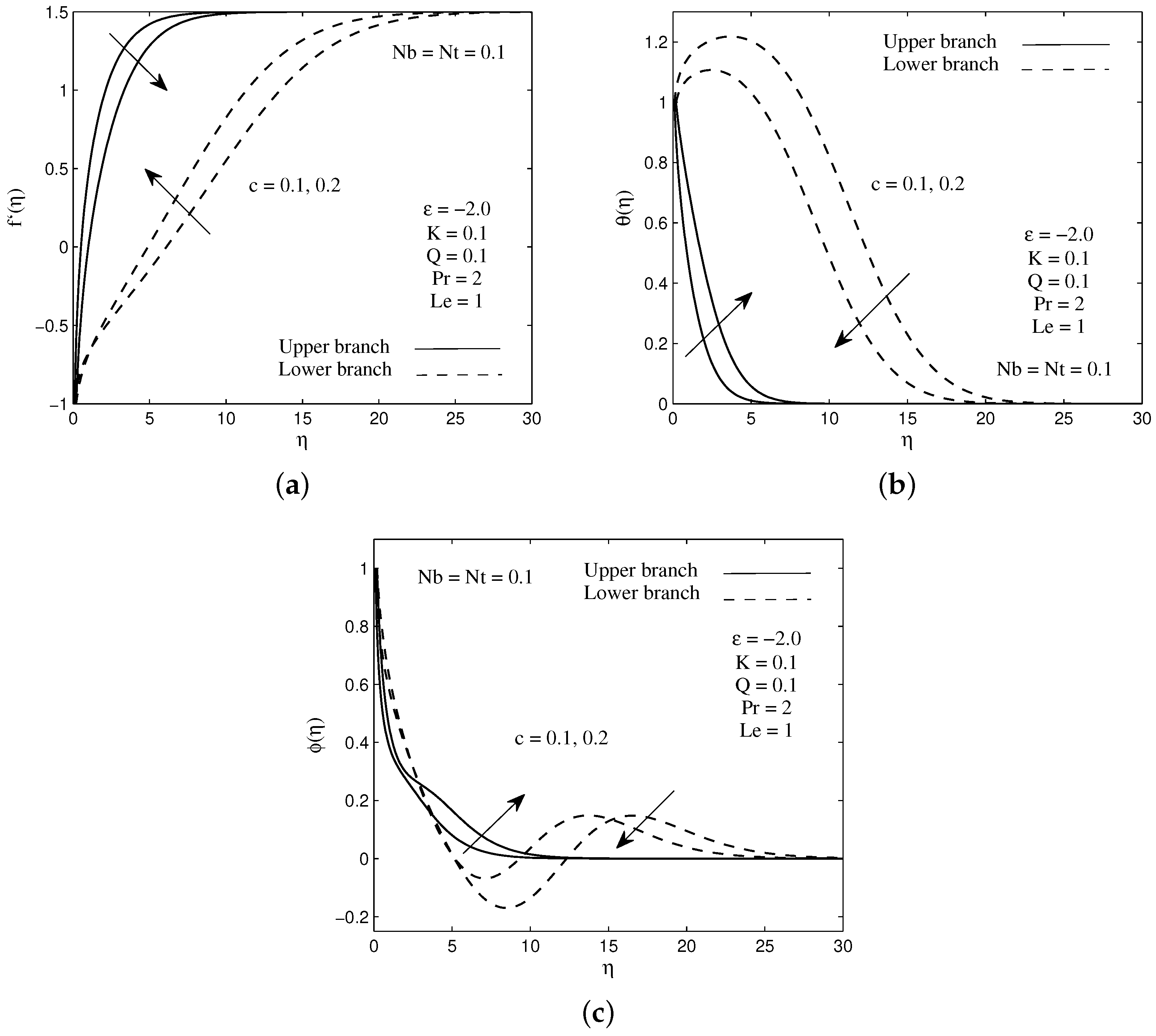
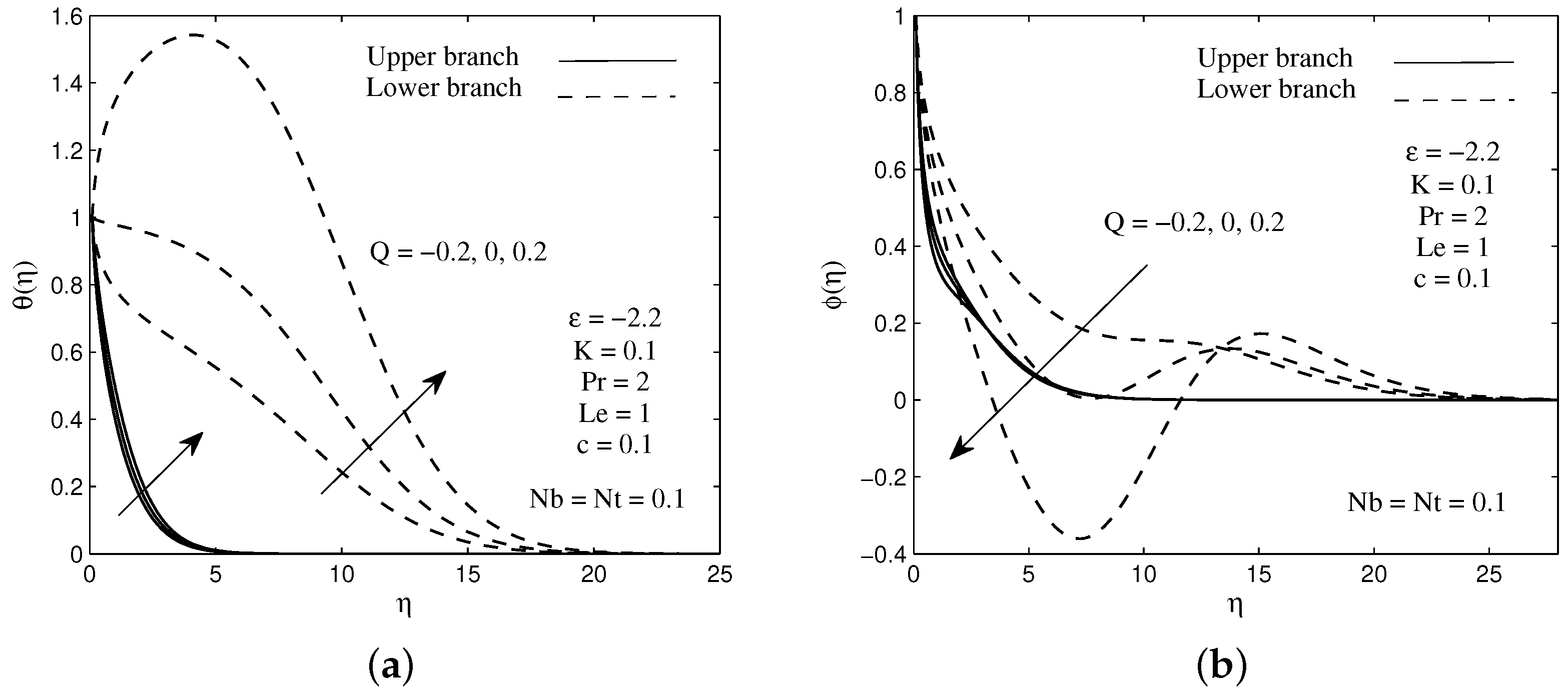
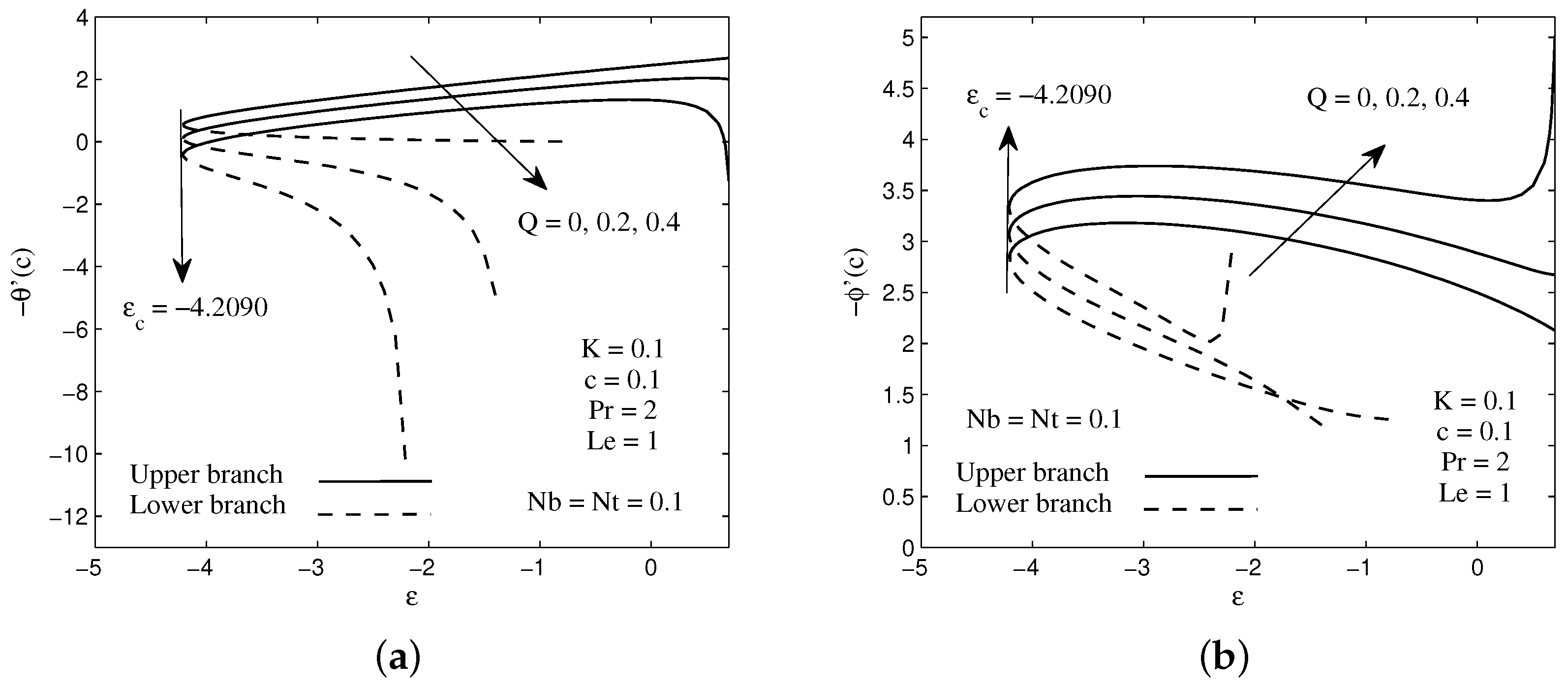
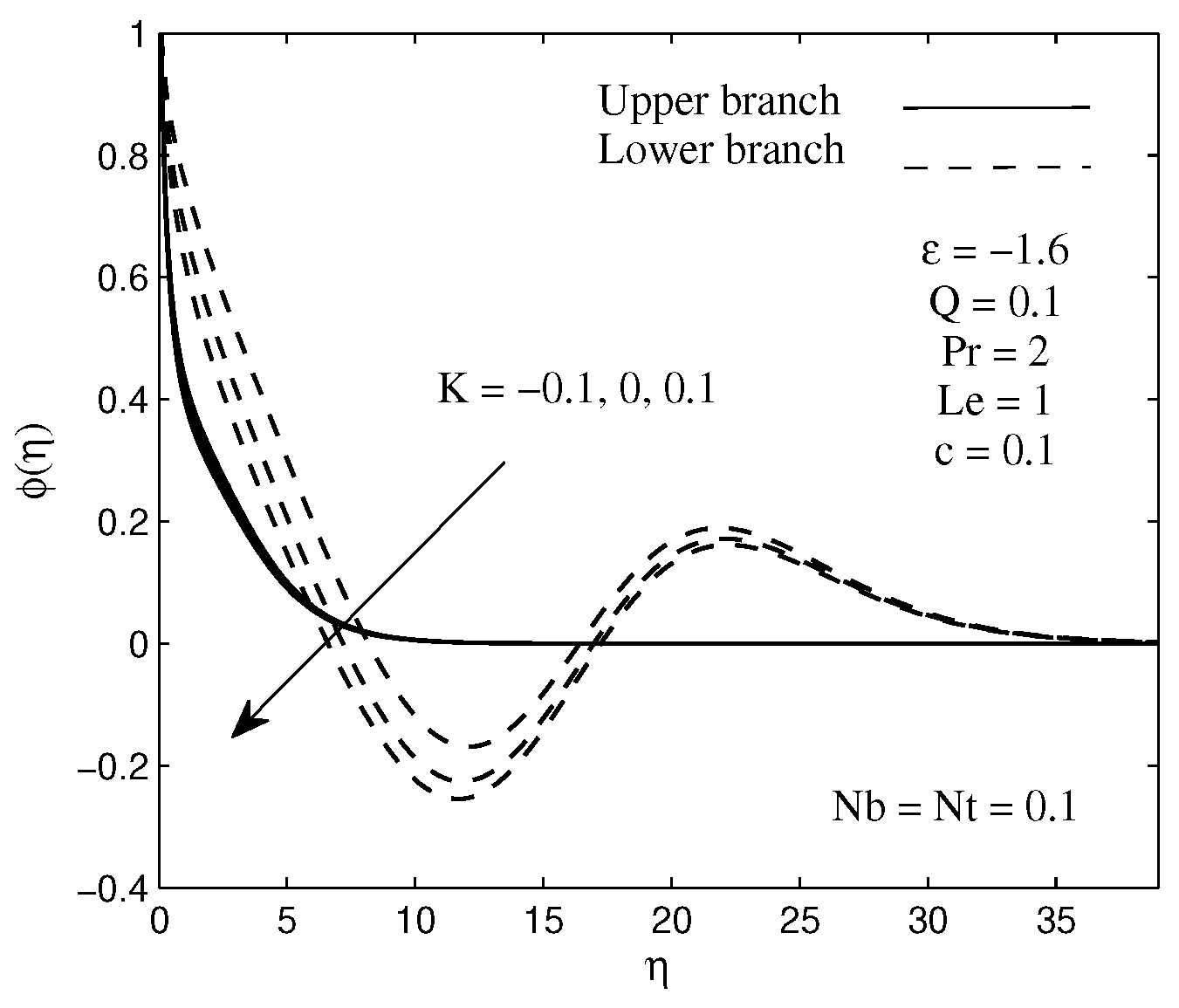
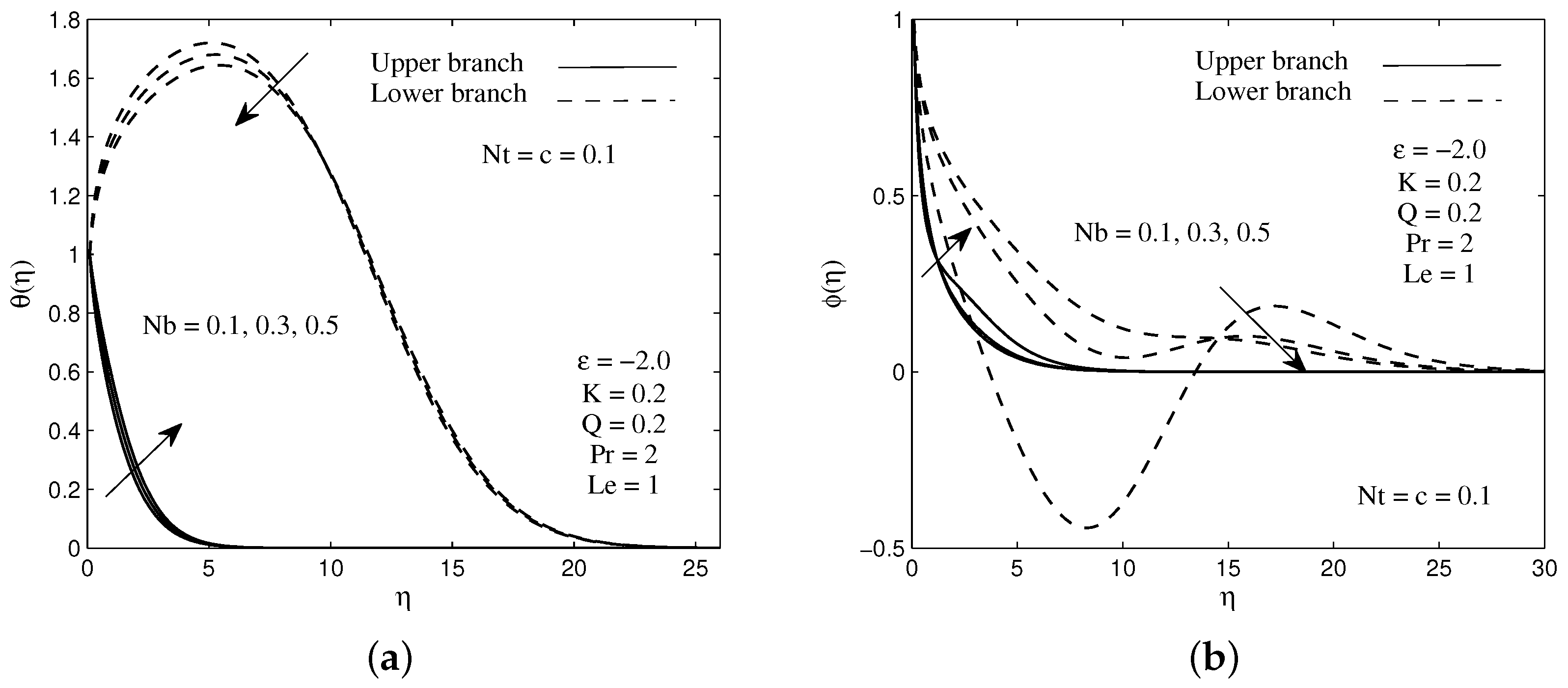
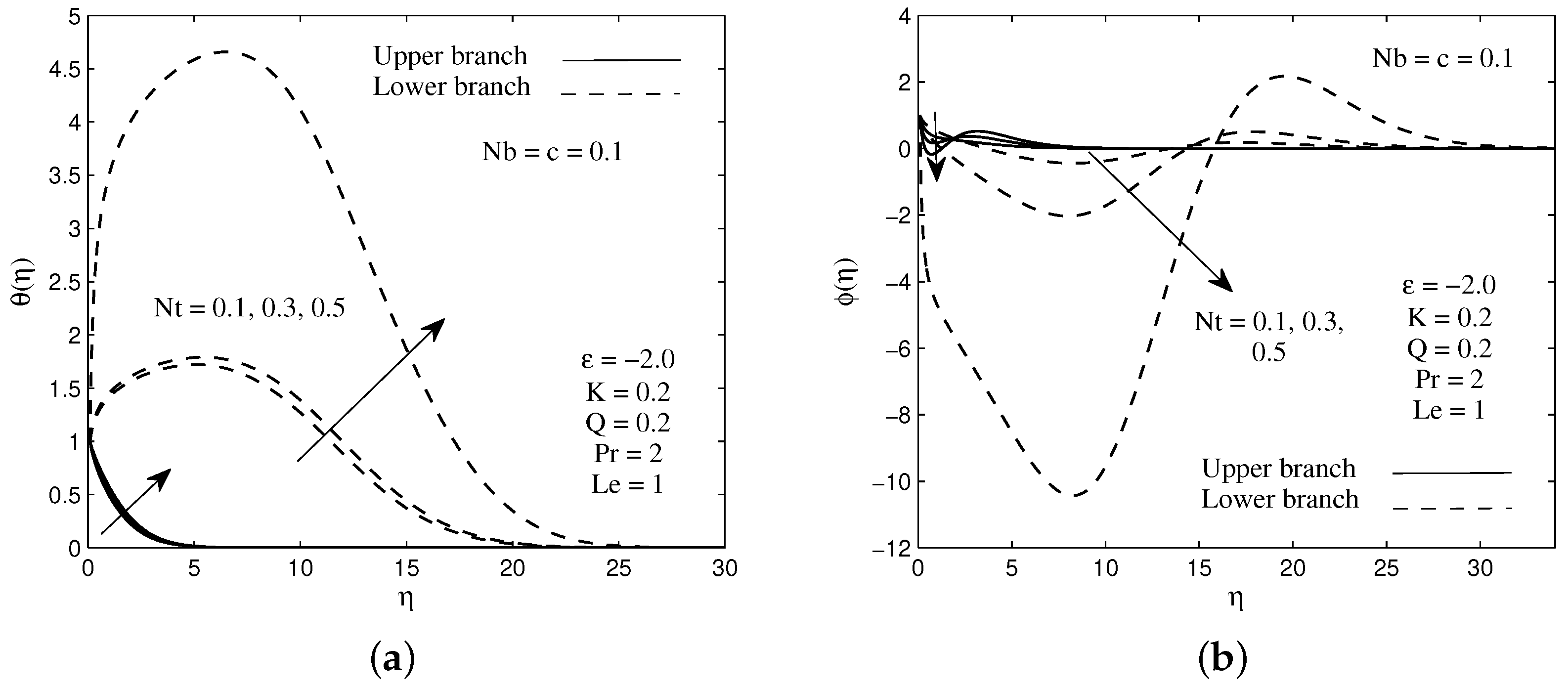
4. Graphical Results and Discussion
5. Final Remarks
- The heat generation parameter reduces the local heat flux as well as the rate of heat transfer.
- The presence of a chemical reaction increases the rate of mass transfer on the needle surface.
- The Brownian motion parameter diminishes the rate of heat and mass transfers from the needle surface to the flow.
- An increase in the thermophoresis parameter results in an increase in the mass transfer rate, while the reverse effect is noted for the heat transfer rate.
- An increment in the needle thickness leads to decrease the magnitudes of the surface shear stress, local heat flux and local mass flux.
- The dual solutions are likely to exist when the needle surface moves against the free-stream direction, .
- The upper branch solution exhibits stable flow (or solution) and lower branch solution exhibits unstable flow.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| c | Needle size |
| C | Fluid concentration (kg m) |
| Skin friction coefficient | |
| Ambient nanoparticle volume fraction | |
| Surface volume fraction | |
| Specific heat at constant pressure | |
| Brownian diffusion coefficient (m s) | |
| Thermophoretic diffusion coefficient (m s) | |
| f | Similarity function for velocity |
| K | Chemical reaction parameter |
| Chemical reaction coefficient | |
| Dimensionless reaction rate | |
| Lewis number | |
| Brownian motion parameter | |
| Thermophoresis parameter | |
| Local Nusselt number | |
| Prandtl number | |
| Q | Heat generation parameter |
| Heat generation coefficient | |
| Dimensionless heat generation | |
| r | Cartesian coordinate |
| Local Reynolds number | |
| Local Sherwood number | |
| T | Fluid temperature (K) |
| Wall temperature (K) | |
| Ambient temperature (K) | |
| U | Composite velocity (ms) |
| Wall velocity (ms) | |
| Ambient velocity (ms) | |
| u | Velocity in x direction (ms) |
| v | Velocity in r direction (ms) |
| x | Cartesian coordinate |
| Thermal diffusivity (m s) | |
| Similarity independent variable | |
| Dimensionless temperature | |
| Velocity ratio parameter | |
| Ratio of effective heat capacity of nanofluid | |
| Volumetric heat capacity (J K) | |
| Kinematic viscosity (m s) | |
| Dynamic viscosity (kg ms) | |
| Fluid density (kg m) | |
| Dimensionless solid volume fraction | |
| w | Condition at the wall |
| ∞ | Ambient condition |
| Differentiative with respect to |
References
- Choi, S.U.S. Enhancing thermal conductivity of fluids with nanoparticles. Am. Soc. Mech. Eng. Fluids Eng. Div. 1995, 231, 99–105. [Google Scholar]
- Wong, K.V.; Leon, O.D. Applications of Nanofluids: Current and Future. Adv. Mech. Eng. 2010, 2010, 519659. [Google Scholar] [CrossRef]
- Saidur, R.; Leong, K.Y.; Mohammad, H.A. A review on applications and challenges of nanofluids. Renew. Sustain. Energy Rev. 2011, 15, 1646–1668. [Google Scholar] [CrossRef]
- Huminic, G.; Huminic, A. Application of nanofluids in heat exchangers: A review. Renew. Sustain. Energy Rev. 2012, 16, 5625–5638. [Google Scholar] [CrossRef]
- Colangelo, G.; Favale, E.; Milanese, M.; Risi, A.D.; Laforgia, D. Cooling of electronic devices: Nanofluids contribution. Appl. Ther. Eng. 2017, 127, 421–435. [Google Scholar] [CrossRef]
- Buongiorno, J. Convective transport in nanofluids. J. Heat Trans. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Trans. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Khan, W.A.; Pop, I. Boundary-layer flow of a nanofluid past a stretching sheet. Int. J. Heat Mass Trans. 2010, 53, 2477–2483. [Google Scholar] [CrossRef]
- Makinde, O.D.; Aziz, A. Boundary layer flow of a nanofluid past a stretching sheet with a convective boundary condition. Int. J. Ther. Sci. 2011, 50, 1326–1332. [Google Scholar] [CrossRef]
- Bachok, N.; Ishak, A.; Pop, I. Unsteady boundary-layer flow and heat transfer of a nanofluid over a permeable stretching/shrinking sheet. Int. J. Heat Mass Trans. 2012, 55, 2102–2109. [Google Scholar] [CrossRef]
- Das, K.; Duari, P.R.; Kundu, P.K. Nanofluid flow over an unsteady stretching surface in presence of thermal radiation. Alex. Eng. J. 2014, 53, 737–745. [Google Scholar] [CrossRef]
- Mabood, F.; Khan, W.A.; Ismail, A.I.M. MHD boundary layer flow and heat transfer of nanofluids over a nonlinear stretching sheet: A numerical study. J. Magn. Magn. Mater. 2015, 374, 569–576. [Google Scholar] [CrossRef]
- Naramgari, S.; Sulochana, C. MHD flow over a permeable stretching/shrinking sheet of a nanofluid with suction/injection. Alex. Eng. J. 2016, 55, 819–827. [Google Scholar] [CrossRef]
- Pandey, A.K.; Kumar, M. Boundary layer flow and heat transfer analysis on Cu-water nanofluid flow over a stretching cylinder with slip. Alex. Eng. J. 2017, 56, 671–677. [Google Scholar] [CrossRef]
- Mustafa, M. MHD nanofluid flow over a rotating disk with partial slip effects: Buongiorno model. Int. J. Heat Mass Trans. 2017, 108, 1910–1916. [Google Scholar] [CrossRef]
- Jyothi, K.; Reddy, P.S.; Reddy, M.S. Influence of magnetic field and thermal radiation on convective flow of SWCNTs-water and MWCNTs-water nanofluid between rotating stretchable disks with convective boundary conditions. Adv. Powder Technol. 2018, 331, 326–337. [Google Scholar] [CrossRef]
- Bakar, N.A.A.; Bachok, N.; Arifin, N.M.; Pop, I. Stability analysis on the flow and heat transfer of nanofluid past a stretching/shrinking cylinder with suction effect. Results Phys. 2018, 9, 1335–1344. [Google Scholar] [CrossRef]
- Griffith, R.M. Velocity, temperature and concentration distributions during fiber spinning. Ind. Eng. Chem. Fundam. 1964, 3, 245–250. [Google Scholar] [CrossRef]
- Chin, D.T. Mass transfer to a continuousmoving sheet electrode. J. Electrochem. Soc. 1975, 122, 643–646. [Google Scholar] [CrossRef]
- Gorla, R.S.R. Unsteady mass transfer in the boundary layer on a continuous moving sheet electrode. J. Electrochem. Soc. 1978, 125, 865–869. [Google Scholar] [CrossRef]
- Damseh, R.A.; Al-Odat, M.Q.; Chamkha, A.J.; Shannak, B.A. Combined effect of heat generation or absorption and first-order chemical reaction on micropolar fluid flows over a uniformly stretched permeable surface. Int. J. Therm. Sci. 2009, 48, 1658–1663. [Google Scholar] [CrossRef]
- Magyari, E.; Chamkha, A.J. Combined effect of heat generation or absorption and first-order chemical reaction on micropolar fluid flows over a uniformly stretched permeable surface: The full analytical solution. Int. J. Therm. Sci. 2010, 49, 1821–1828. [Google Scholar] [CrossRef]
- Mabood, F.; Shateyi, S.; Rashidi, M.M.; Momoniat, E.; Freidoonimehr, N. MHD stagnation point flow heat and mass transfer of nanofluids in porous medium with radiation, viscous dissipation and chemical reaction. Adv. Powder Technol. 2016, 27, 742–749. [Google Scholar] [CrossRef]
- Eid, M.R. Chemical reaction effect on MHD boundary-layer flow of two-phase nanofluid model over an exponentially stretching sheet with a heat generation. J. Mol. Liq. 2016, 220, 718–725. [Google Scholar] [CrossRef]
- Ibrahim, S.M.; Lorenzini, G.; Vijaya Kumar, P.; Raju, C.S.K. Influence of chemical reaction and heat source on dissipative MHD mixed convection flow of a Casson nanofluid over a nonlinear permeable stretching sheet. Int. J. Heat Mass Trans. 2017, 111, 346355. [Google Scholar] [CrossRef]
- Nayak, M.K.; Akbar, N.S.; Tripathi, D.; Khan, Z.H.; Pandey, V.S. MHD 3D free convective flow of nanofluid over an exponentially stretching sheet with chemical reaction. Adv. Powder Technol. 2017, 28, 2159–2166. [Google Scholar] [CrossRef]
- Sithole, H.; Mondal, H.; Goqo, S.; Sibanda, P.; Motsa, S. Numerical simulation of couple stress nanofluid flow in magneto-porous medium with thermal radiation and a chemical reaction. Appl. Math. Comput. 2018, 339, 820–836. [Google Scholar] [CrossRef]
- Khan, M.; Shahid, A.; Malik, M.Y.; Salahuddin, T. Chemical reaction for Carreau-Yasuda nanofluid flow past a nonlinear stretching sheet considering Joule heating. Results Phys. 2018, 8, 1124–1130. [Google Scholar] [CrossRef]
- Zeeshan, A.; Shehzad, N.; Ellahi, R. Analysis of activation energy in Couette-Poiseuille flow of nanofluid in the presence of chemical reaction and convective boundary conditions. Results Phys. 2018, 8, 502–512. [Google Scholar] [CrossRef]
- Hayat, T.; Kiyani, M.Z.; Alsaedi, A.; Ijaz Khan, M.; Ahmad, I. Mixed convective three-dimensional flow of Williamson nanofluid subject to chemical reaction. Int. J. Heat Mass Trans. 2018, 127, 422–429. [Google Scholar] [CrossRef]
- Lee, L.L. Boundary layer over a thin needle. Phys. Fluids 1967, 10, 1820–1822. [Google Scholar] [CrossRef]
- Narain, J.P.; Uberoi, S.M. Combined forced and free-convection heat transfer from vertical thin needles in a uniform stream. Phys. Fluids 1973, 15, 1879–1882. [Google Scholar] [CrossRef]
- Wang, C.Y. Mixed convection on a vertical needle with heated tip. Phys. Fluids 1990, 2, 622–625. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Pop, I. Boundary layer flow over a continuously moving thin needle in a parallel free stream. Chin. Phys. Lett. 2007, 24, 2895–2897. [Google Scholar] [CrossRef]
- Ahmad, S.; Arifin, N.M.; Nazar, R.; Pop, I. Mixed convection boundary layer flow along vertical thin needles: Assisting and opposing flows. Int. Commun. Heat Mass Trans. 2008, 35, 157–162. [Google Scholar] [CrossRef]
- Afridi, M.I.; Qasim, M. Entropy generation and heat transfer in boundary layer flow over a thin needle moving in a parallel stream in the presence of nonlinear Rosseland radiation. Int. J. Therm. Sci. 2018, 123, 117–128. [Google Scholar] [CrossRef]
- Grosan, T.; Pop, I. Forced Convection Boundary Layer Flow Past Nonisothermal Thin Needles in Nanofluids. J. Heat Trans. 2011, 133. [Google Scholar] [CrossRef]
- Trimbitas, R.; Grosan, T.; Pop, I. Mixed convection boundary layer flow along vertical thin needles in nanofluids. Int. J. Numer. Methods Heat Fluid Flow 2014, 24, 579–594. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Farooq, M.; Yasmeen, T.; Alsaedi, A. Water-carbon nanofluid flow with variable heat flux by a thin needle. J. Mol. Liq. 2016, 224, 786–791. [Google Scholar] [CrossRef]
- Soid, S.K.; Ishak, A.; Pop, I. Boundary layer flow past a continuously moving thin needle in a nanofluid. Appl. Therm. Eng. 2017, 114, 58–64. [Google Scholar] [CrossRef]
- Krishna, P.M.; Sharma, R.P.; Sandeep, N. Boundary layer analysis of persistent moving horizontal needle in Blasius and Sakiadis magnetohydrodynamic radiative nanofluid flows. Nucl. Eng. Technol. 2017, 49, 1654–1659. [Google Scholar] [CrossRef]
- Ahmad, R.; Mustafa, M.; Hina, S. Buongiorno’s model for fluid flow around a moving thin needle in a flowing nanofluid: A numerical study. Chin. J. Phys. 2017, 55, 1264–1274. [Google Scholar] [CrossRef]
- Salleh, S.N.A.; Bachok, N.; Arifin, N.M.; Ali, F.M.; Pop, I. Magnetohydrodynamics flow past a moving vertical thin needle in a nanofluid with stability analysis. Energies 2018, 11, 3297. [Google Scholar] [CrossRef]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Rosca, A.V.; Pop, I. Flow and heat transfer over a vertical permeable stretching/shrinking sheet with a second order slip. Int. J. Heat Mass Trans. 2013, 60, 355–364. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]


| c | Ahmad et al. [42] | Salleh et al. [43] | Current Study |
|---|---|---|---|
| 0.01 | 8.4924360 | 8.4924452 | 8.4924453 |
| 0.1 | 1.2888171 | 1.2888299 | 1.2888300 |
| 0.15 | - | - | 0.9383388 |
| 0.2 | - | - | 0.7515725 |
| Heat Generation Parameter | Thermphoresis Parameter | |||
|---|---|---|---|---|
| 0.1 | 0.1 | 1.208880 | 0.959223 | 0.749362 |
| 0.3 | 0.989170 | 0.777343 | 0.601318 | |
| 0.5 | 0.805833 | 0.627349 | 0.480535 | |
| 0.2 | 0.1 | 1.078738 | 0.832762 | 0.628219 |
| 0.3 | 0.863788 | 0.656596 | 0.486510 | |
| 0.5 | 0.685682 | 0.512462 | 0.371923 | |
| Chemical Reaction Parameter | Thermphoresis Parameter | |||
|---|---|---|---|---|
| 0.1 | 0.1 | 2.005009 | 1.825444 | 1.781448 |
| 0.3 | 3.271205 | 2.343083 | 2.135890 | |
| 0.5 | 5.055702 | 2.990494 | 2.546082 | |
| 0.2 | 0.1 | 2.085015 | 1.898138 | 1.852426 |
| 0.3 | 3.362939 | 2.418572 | 2.207592 | |
| 0.5 | 5.144680 | 3.063527 | 2.615317 | |
| K = Q | c | Upper Branch | Lower Branch | |
|---|---|---|---|---|
| 0.1 | 0.1 | −4.1994 | 0.0471 | −0.0449 |
| −4.199 | 0.0481 | −0.0458 | ||
| −4.19 | 0.0668 | −0.0625 | ||
| 0.2 | −2.7424 | 0.0150 | −0.0147 | |
| −2.742 | 0.0175 | −0.0170 | ||
| −2.74 | 0.0265 | −0.0255 | ||
| 0.2 | 0.1 | −4.1246 | 0.1444 | −0.1254 |
| −4.124 | 0.1449 | −0.1258 | ||
| −4.12 | 0.1484 | −0.1284 | ||
| 0.2 | −2.7136 | 0.0793 | −0.0706 | |
| −2.713 | 0.0801 | −0.0713 | ||
| −2.71 | 0.0841 | −0.0744 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salleh, S.N.A.; Bachok, N.; Arifin, N.M.; Ali, F.M. Numerical Analysis of Boundary Layer Flow Adjacent to a Thin Needle in Nanofluid with the Presence of Heat Source and Chemical Reaction. Symmetry 2019, 11, 543. https://doi.org/10.3390/sym11040543
Salleh SNA, Bachok N, Arifin NM, Ali FM. Numerical Analysis of Boundary Layer Flow Adjacent to a Thin Needle in Nanofluid with the Presence of Heat Source and Chemical Reaction. Symmetry. 2019; 11(4):543. https://doi.org/10.3390/sym11040543
Chicago/Turabian StyleSalleh, Siti Nur Alwani, Norfifah Bachok, Norihan Md Arifin, and Fadzilah Md Ali. 2019. "Numerical Analysis of Boundary Layer Flow Adjacent to a Thin Needle in Nanofluid with the Presence of Heat Source and Chemical Reaction" Symmetry 11, no. 4: 543. https://doi.org/10.3390/sym11040543
APA StyleSalleh, S. N. A., Bachok, N., Arifin, N. M., & Ali, F. M. (2019). Numerical Analysis of Boundary Layer Flow Adjacent to a Thin Needle in Nanofluid with the Presence of Heat Source and Chemical Reaction. Symmetry, 11(4), 543. https://doi.org/10.3390/sym11040543






