MHD Flow and Heat Transfer over Vertical Stretching Sheet with Heat Sink or Source Effect
Abstract
1. Introduction
2. Problem Description
- is the electrical conductivity,
- is the magnetic permeability,
- is the temperature of free stream,
- g is acceleration due to gravity,
- is the volumetric coefficient of thermal expansion,
- k is the thermal conductivity,
- is the kinematic viscosity, and
- is the temperature of the sheet.
3. Scheme Analysis
4. Stability Analysis
5. Results and Discussion
6. Conclusions
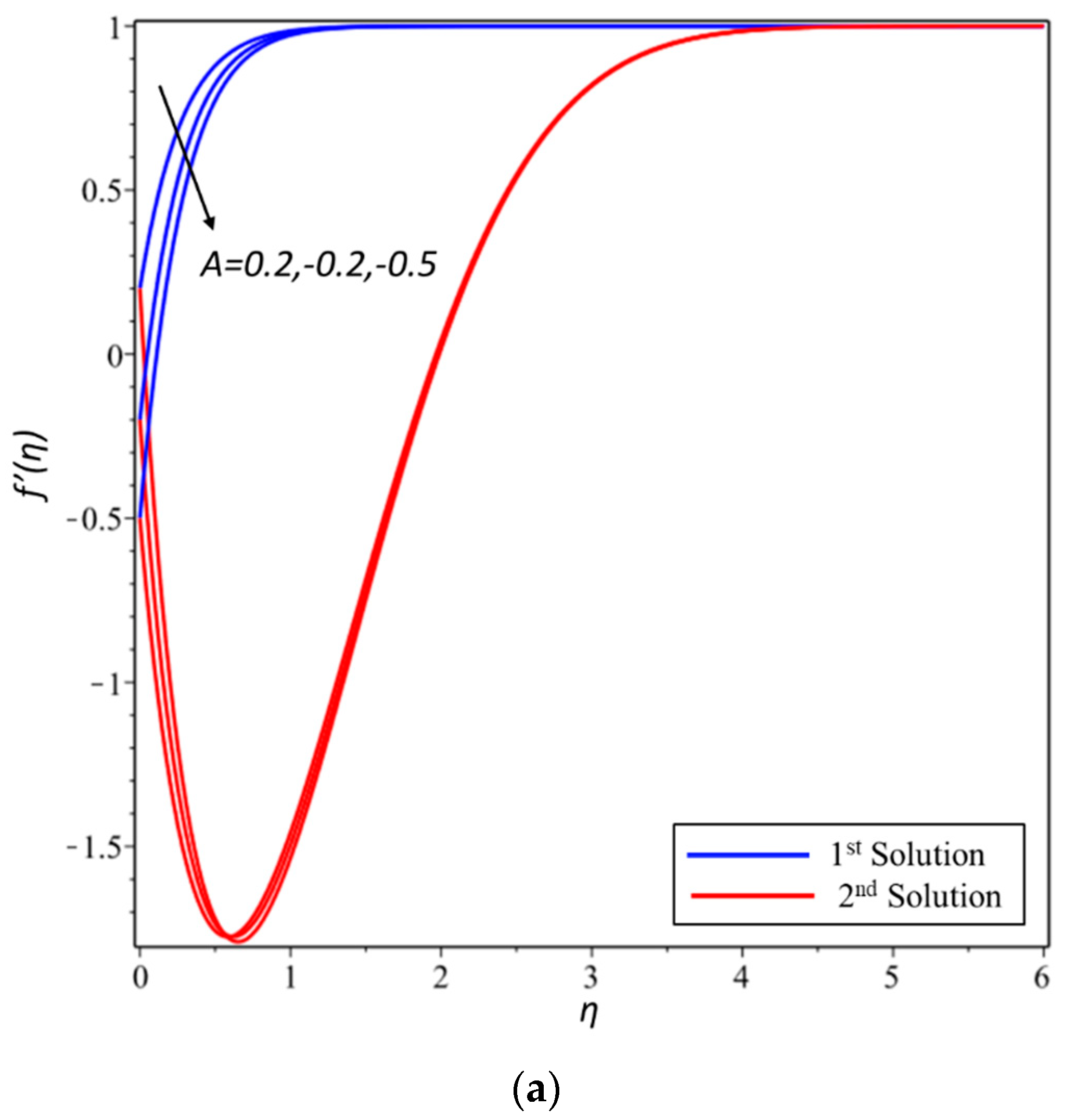
- It is perceived that, for assisting flow (λ > 0), the dimensionless velocity is the maximum at the superficial of the vertical stretching sheet and it gradually lessens to the minimum value , as it transfers to gone after the superficial, while for opposing flow (λ < 0), the dimensionless velocity is the lowest at the superficial of the vertical stretching sheet and gradually increases to the maximum value .
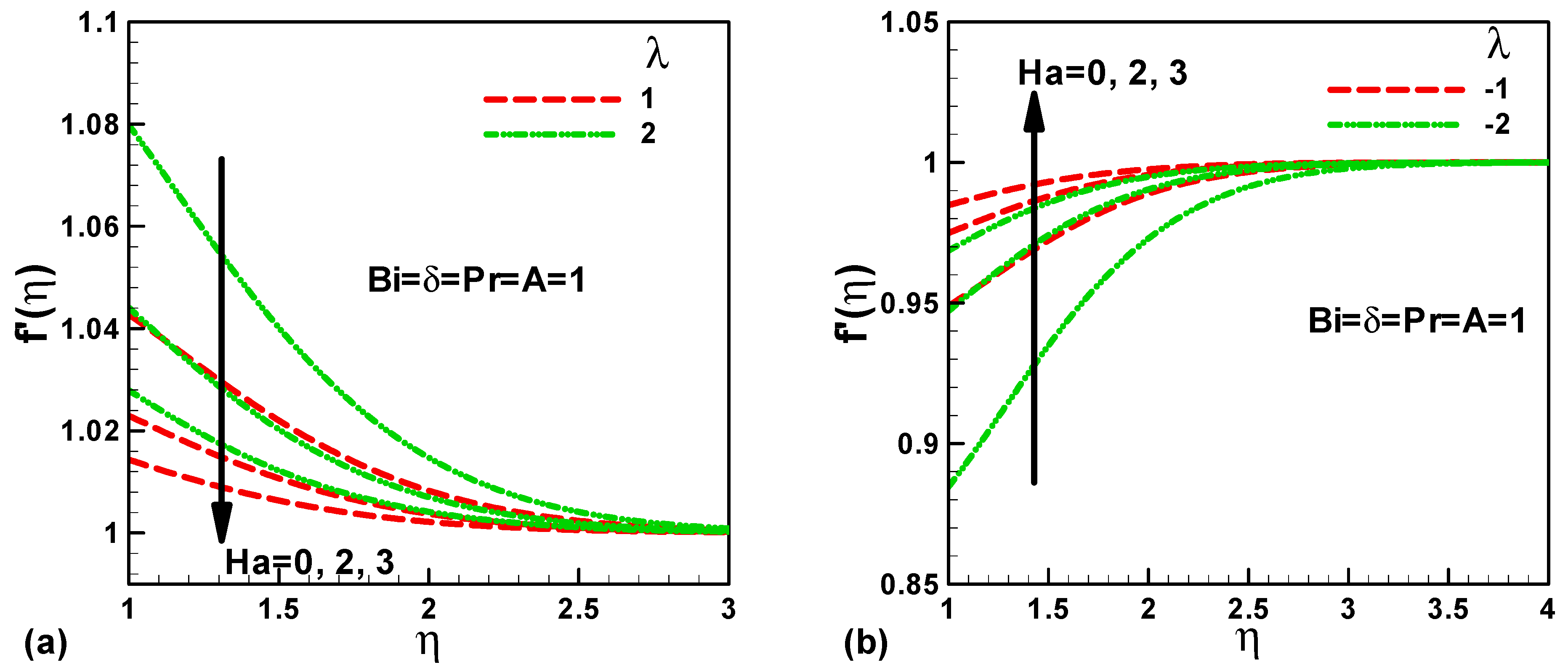
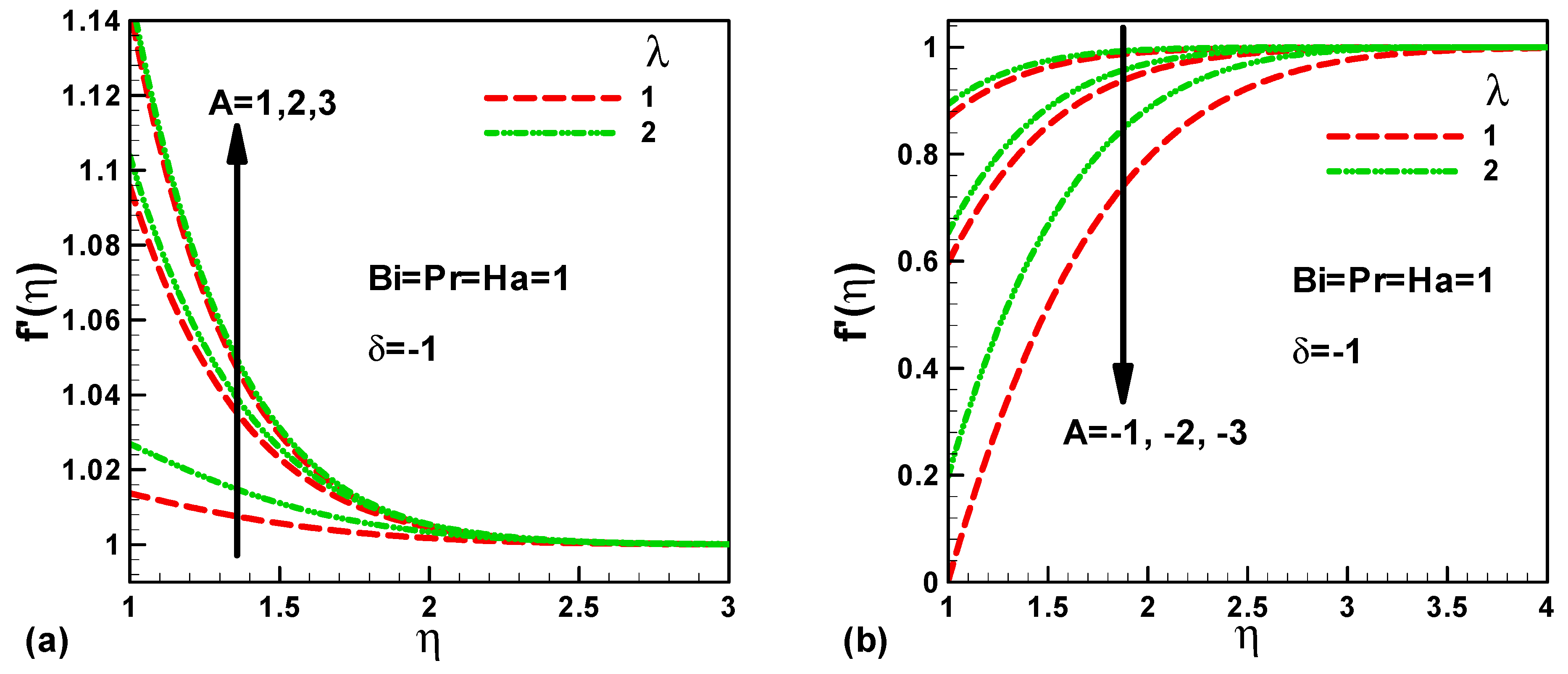
- The velocity profile augments with mixed convection parameter λ for both stretching in the flow direction and stretching in the opposite direction.
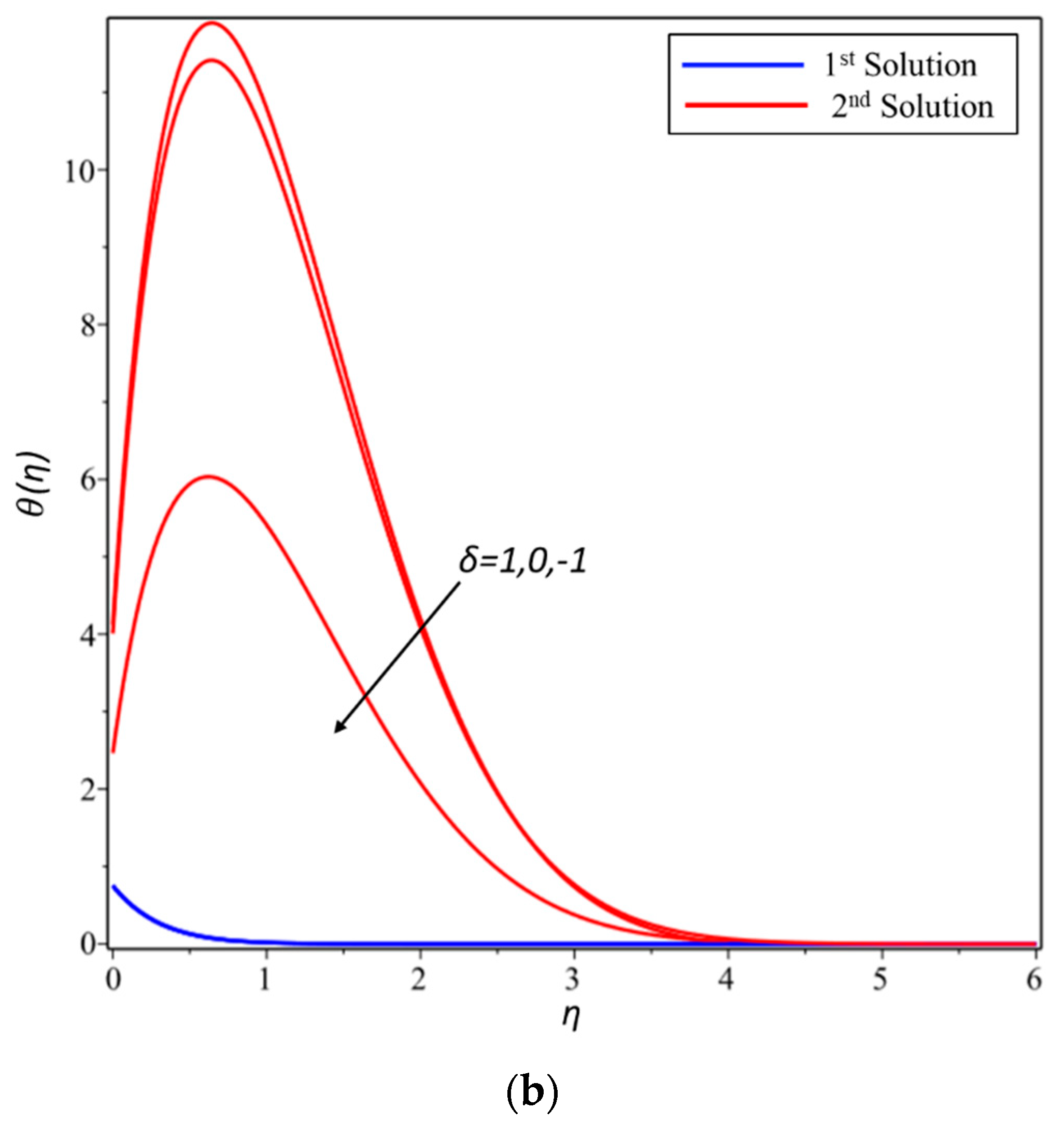
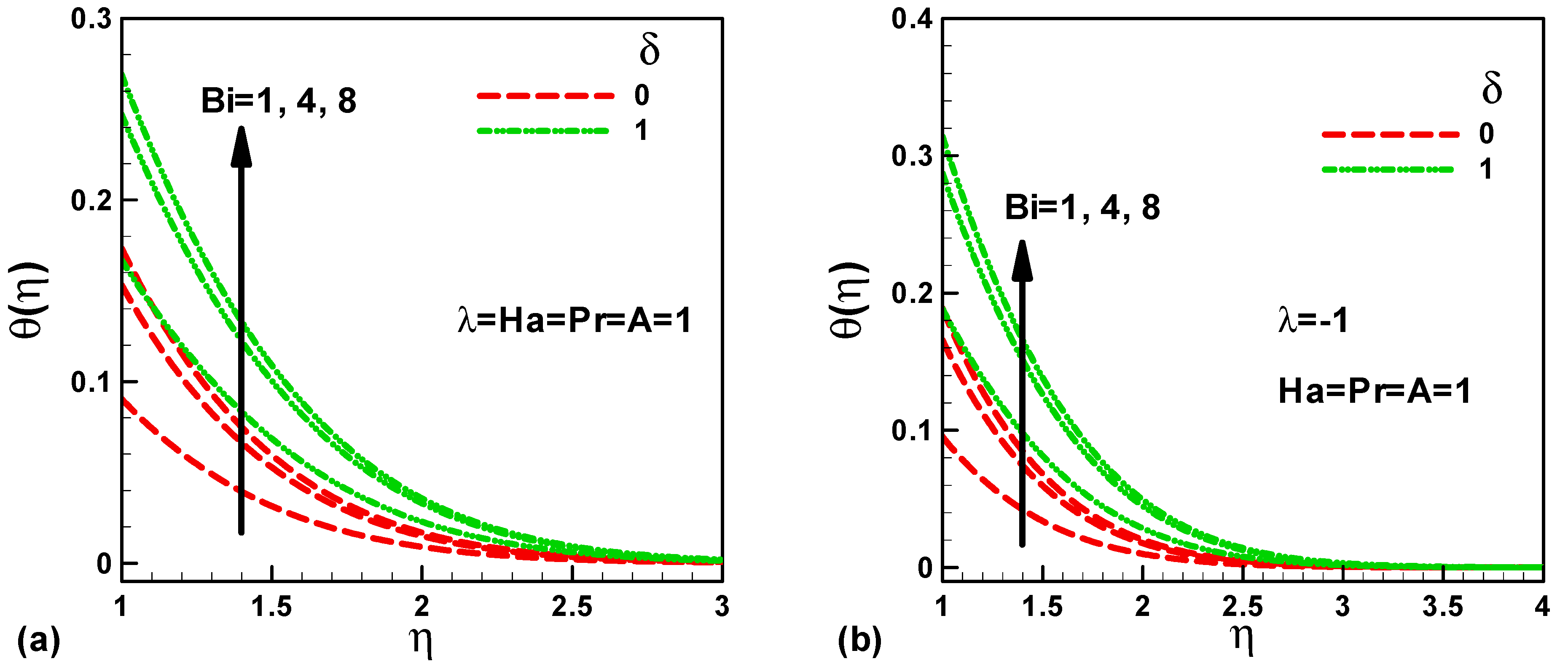
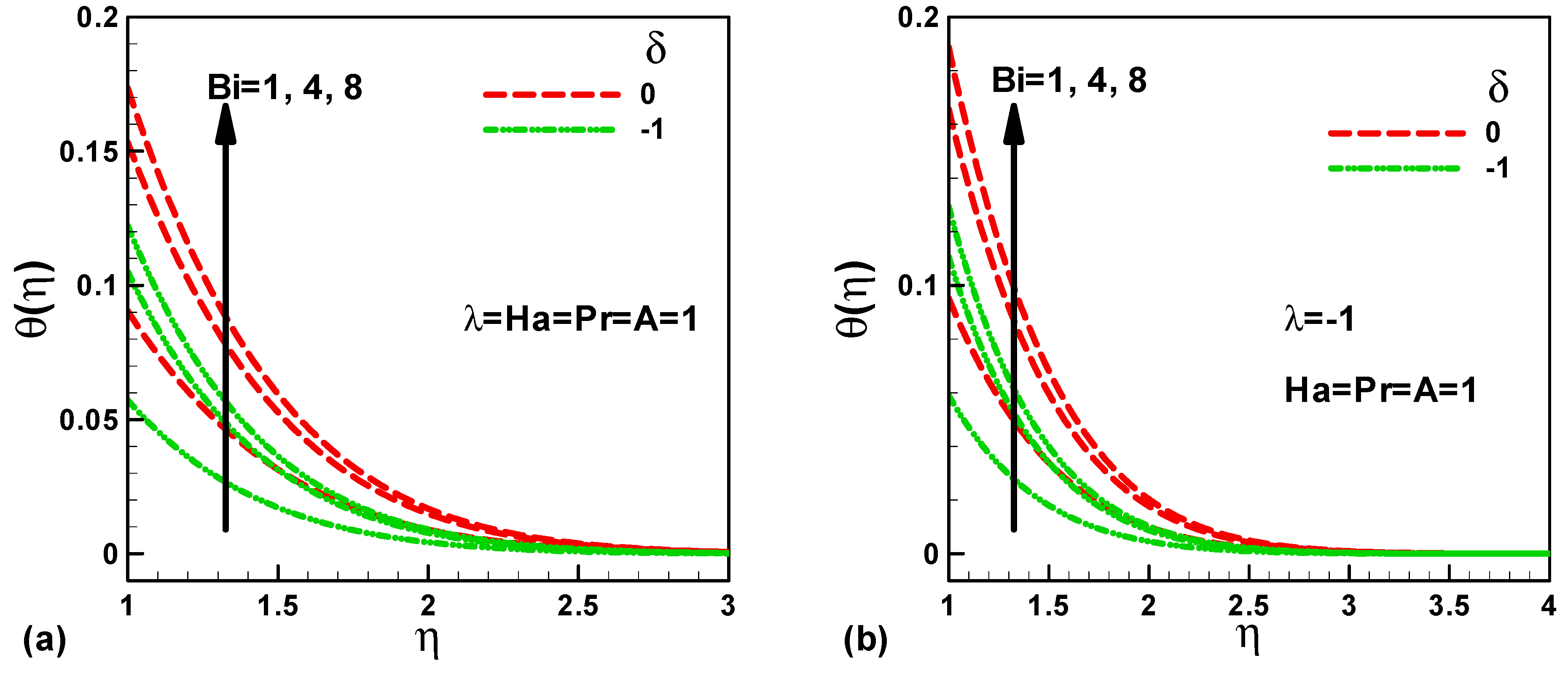
- The temperature profile is greater at the stretching sheet superficial then exponentially lessens along the streamwise path up to the zero value for both assisting and opposing flow.
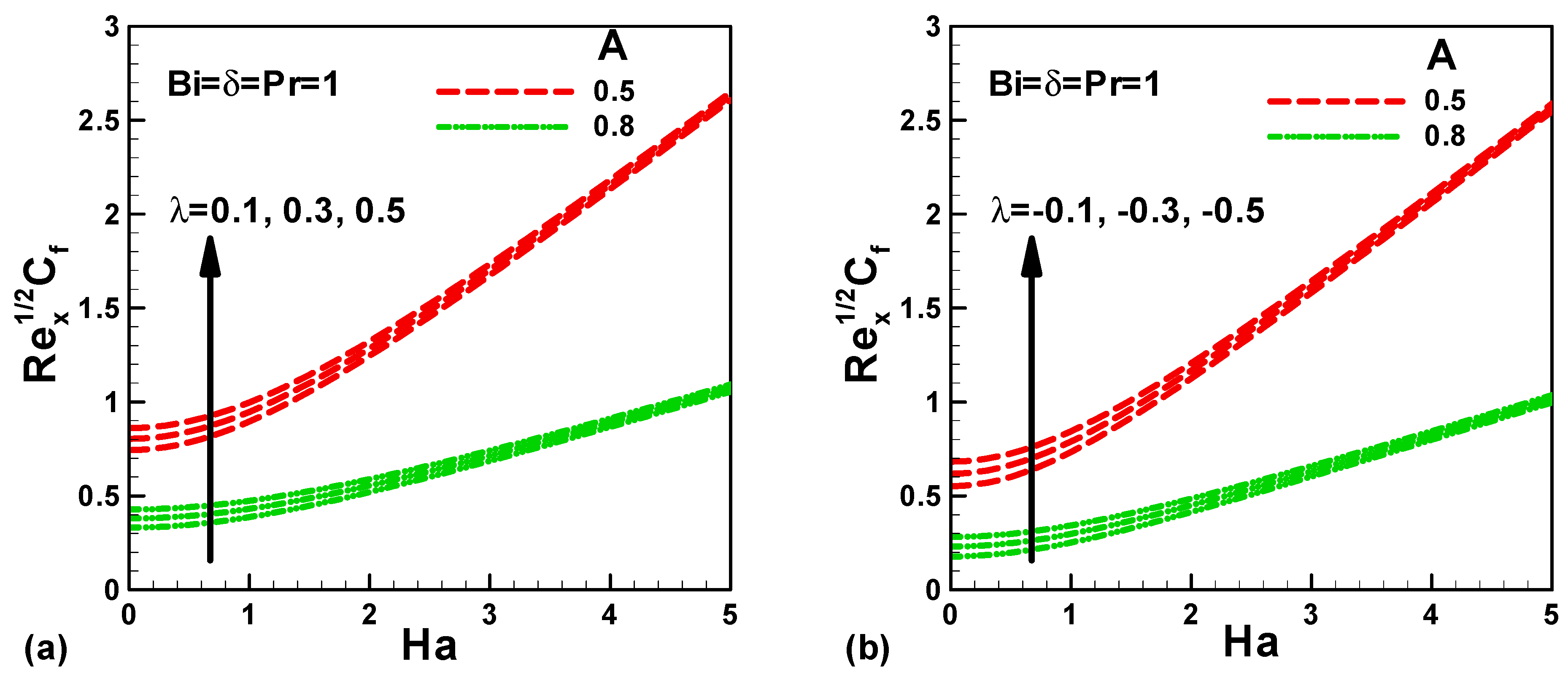
- Skin friction increase with a mixed convection parameter and Hartmann number Ha, though it declines by stretching speed ratio for together opposing and assisting flow.
- The effect of mixed convection parameter on the heat transfer rate is slightly perceptible. The Nusselt number at the sheet surface augments, because the Hartmann number, Hartmann number Ha, stretching velocity ratio A, and mixed convection parameter λ increase. Though, it declines according to heat generation/absorption coefficient δ.
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Mohammadein, S.A.; Raslan, K.; Abdel-Wahed, M.S.; Abedel-Aal, E.M. KKL-model of MHD CuO-nanofluid flow over a stagnation point stretching sheet with nonlinear thermal radiation and suction/injection. Results Phys. 2018, 10, 194–199. [Google Scholar] [CrossRef]
- Ibrahim, W. Magnetohydrodynamic (MHD) boundary layer stagnation point flow and heat transfer of a nanofluid past a stretching sheet with melting. Propuls. Power Res. 2017, 6, 214–222. [Google Scholar] [CrossRef]
- Sharma, P.R.; Sinha, S.; Yadav, R.S.; Filippov, A.N. MHD mixed convective stagnation point flow along a vertical stretching sheet with heat source/sink. Int. J. Heat Mass Transf. 2018, 117, 780–786. [Google Scholar] [CrossRef]
- SGhadikolaei, S.; Hosseinzadeh, K.H.; Ganji, D.D. MHD raviative boundary layer analysis of micropolar dusty fluid with graphene oxide (Go)-engine oil nanoparticles in a porous medium over a stretching sheet with joule heating effect. Powder Technol. 2018. [Google Scholar] [CrossRef]
- El-Mistikawy, T.M.A. MHD flow due to a linearly stretching sheet with induced magnetic field. Acta Mech. 2016, 227, 3049–3053. [Google Scholar] [CrossRef]
- Hussain, A.; Malik, M.Y.; Salahuddin, T.; Rubab, A.; Khan, M. Effects of viscous dissipation on MHD tangent hyperbolic fluid over a nonlinear stretching sheet with convective boundary conditions. Results Phys. 2017, 7, 3502–3509. [Google Scholar] [CrossRef]
- Tufail, M.N.; Butt, A.S.; Ali, A. Computational modeling of an MHD flow of a non-newtonian fluid over an unsteady stretching sheet with viscous dissipation effects. J. Appl. Mech. Tech. Phys. 2016, 57, 900–907. [Google Scholar] [CrossRef]
- Mabood, F.; Khan, W.A.; Ismail, A.I.M. MHD flow over exponential radiating stretching sheet using homotopy analysis method. J. King Saud Univ. Eng. Sci. 2017, 29, 68–74. [Google Scholar] [CrossRef]
- Hayat, T.; Qayyum, S.; Alsaedi, A.; Ahmad, B. Magnetohydrodynamic (MHD) nonlinear convective flow of Walters-B nanofluid over a nonlinear stretching sheet with variable thickness. Int. J. Heat Mass Transf. 2017, 110, 506–514. [Google Scholar] [CrossRef]
- Malik, M.Y.; Khan, M.; Salahuddin, T. Study of an MHD flow of the carreau fluid flow over a stretching sheet with a variable thickness by using an implicit finite difference scheme. J. Appl. Mech. Tech. Phys. 2017, 58, 1033–1039. [Google Scholar] [CrossRef]
- Agbaje, T.M.; Mondal, S.; Makukula, Z.G.; Motsa, S.S.; Sibanda, P. A new numerical approach to MHD stagnation point flow and heat transfer towards a stretching sheet. Ain Shams Eng. J. 2018, 9, 233–243. [Google Scholar] [CrossRef]
- Soid, S.K.; Ishak, A.; Pop, I. MHD flow and heat transfer over a radially stretching/shrinking disk. Chin. J. Phys. 2018, 56, 58–66. [Google Scholar] [CrossRef]
- Mahabaleshwar, U.S.; Sarris, I.E.; Hill, A.A.; Lorenzini, G.; Pop, I. An MHD couple stress fluid due to a perforated sheet undergoing linear stretching with heat transfer. Int. J. Heat Mass Transf. 2017, 105, 157–167. [Google Scholar] [CrossRef]
- Ferdows, M.; Khalequ, T.S.; Tzirtzilakis, E.E.; Sun, S. Effects of Radiation and Thermal Conductivity on MHD Boundary Layer Flow with Heat Transfer along a Vertical Stretching Sheet in a Porous Medium. J. Eng. Thermophys. 2017, 26, 96–106. [Google Scholar] [CrossRef]
- Hsiao, K.-L. Micropolar nanofluid flow with MHD and viscous dissipation effects towards a stretching sheet with multimedia feature. Int. J. Heat Mass Transf. 2017, 112, 983–990. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Salah, F. Thermal stratification effects on MHD radiative flow of nanofluid over nonlinear stretching sheet with variable thickness. J. Comput. Des. Eng. 2018, 5, 232–242. [Google Scholar] [CrossRef]
- Hayat, T.; Rashid, M.; Alsaedi, A. MHD convective flow of magnetite-Fe3O4 nanoparticles by curved stretching sheet. Results Phys. 2017, 7, 3107–3115. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Salah, F. Thermal radiation on unsteady electrical MHD flow of nanofluid over stretching sheet with chemical reaction. J. King Saud Univ. Sci. 2017, in press. [Google Scholar] [CrossRef]
- Seth, G.S.; Singha, A.K.; Mandal, M.S.; Banerjee, A.; Bhattacharyya, K. MHD stagnation-point flow and heat transfer past a non-isothermal shrinking/stretching sheet in porous medium with heat sink or source effect. Int. J. Mech. Sci. 2017, 134, 98–111. [Google Scholar] [CrossRef]
- Khan, M.; Hussain, A.; Malik, M.Y.; Salahuddin, T.; Khan, F. Boundary layer flow of MHD tangent hyperbolic nanofluid over a stretching sheet: A numerical investigation. Results Phys. 2017, 7, 2837–2844. [Google Scholar] [CrossRef]
- Madhu, M.; Kishan, N. MHD boundary-layer flow of a non-newtonian nanofluid past a stretching sheet with a heat source/sink. J. Appl. Mech. Tech. Phys. 2016, 57, 908–915. [Google Scholar] [CrossRef]
- Babu, M.J.; Sandeep, N. Three-dimensional MHD slip flow of nanofluids over a slendering stretching sheet with thermophoresis and Brownian motion effects. Adv. Powder Technol. 2016, 27, 2039–2050. [Google Scholar] [CrossRef]
- Khan, I.; Malik, M.Y.; Hussain, A.; Salahuddin, T. Effect of homogenous-heterogeneous reactions on MHD Prandtl fluid flow over a stretching sheet. Results Phys. 2017, 7, 4226–4231. [Google Scholar] [CrossRef]
- Seth, G.S.; Mishra, M.K. Analysis of transient flow of MHD nanofluid past a non-linear stretching sheet considering Navier’s slip boundary condition. Adv. Powder Technol. 2017, 28, 375–384. [Google Scholar] [CrossRef]
- Chen, X.; Ye, Y.; Zhang, X.; Zheng, L. Lie-group similarity solution and analysis for fractional viscoelastic MHD fluid over a stretching sheet. Comput. Math. Appl. 2018, 75, 3002–3011. [Google Scholar] [CrossRef]
- Ramchandran, N.; Chen, T.S.; Armaly, B.F. Mixed convection in stagnation flows adjacent to vertical surfaces. J. Heat Transf. 1988, 110, 373–377. [Google Scholar] [CrossRef]
- Lok, Y.Y.; Amin, N.; Pop, I. Unsteady mixed convection flow of a micropolar fluid near the stagnation point on a vertical surface. Int. J. Therm. Sci. 2006, 45, 1149–1152. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Pop, I. Dual solutions in mixed convection flow near a stagnation point on a vertical porous plate. Int. J. Therm. Sci. 2008, 47, 417–422. [Google Scholar] [CrossRef]
- Ali, F.M.; Nazar, R.; Arifin, N.M.; Pop, I. Mixed convection stagnation point flow on vertical stretching sheet with external magnetic field. Appl. Math. Mech. Engl. Ed. 2014, 35, 155–166. [Google Scholar] [CrossRef]
- Hassanien, I.A.; Gorla, R. Nonsimilar Solutions for Natural Convection in Micropolar Fluids on a Vertical Plate. Int. J. Fluid Mech. Res. 2003, 30, 4–14. [Google Scholar] [CrossRef]
- Li, J.; Zheng, L.; Liu, L. MHD viscoelastic flow and heat transfer over a vertical stretching sheet with Cattaneo-Christov heat flux effects. J. Mol. Liq. 2016, 221, 19–25. [Google Scholar] [CrossRef]
- Freidoonimehr, N.; Rahimi, A.B. Exact-solution of entropy generation for MHD nanofluid flow induced by a stretching/shrinking sheet with transpiration: Dual solution. Adv. Powder Technol. 2017, 28, 671–685. [Google Scholar] [CrossRef]
- Merkin, J.H. On dual solutions occurring in mixed convection in a porous medium. J. Eng. Math. 1986, 20, 171–179. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]
- Rahman, M.M.; Roşca, A.V.; Pop, I. Boundary layer flow of a nanofluid past a permeable exponentially shrinking/stretching surface with second order slip using Buongiorno’s model. Int. J. Heat Mass Transf. 2014, 77, 1133–1143. [Google Scholar] [CrossRef]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Roşca, A.V.; Pop, I. Flow and heat transfer over a vertical permeable stretching/shrinking sheet with a second order slip. Int. J. Heat Mass Transf. 2013, 60, 355–364. [Google Scholar] [CrossRef]

| Pr | Ramchandran et al. [26] | Hassanien and Gorla [30] | Lok et al. [27] | Ishak et al. [28] | Ali et al. [29] | Sharma et al. [3] | Present |
|---|---|---|---|---|---|---|---|
| 1 | - | - | - | 0.8708 | 0.8708 | 0.8707 | 0.87078 |
| 10 | - | 1.9446 | - | 1.9446 | 1.9448 | 1.94463 | 1.94465 |
| 20 | 2.4576 | - | 2.4577 | 2.4576 | 2.4579 | 2.4576 | 2.4577 |
| 40 | 3.1011 | - | 3.1023 | 3.1011 | 3.1017 | 3.1011 | 3.1015 |
| 60 | 3.5514 | - | 3.556 | 3.5514 | 3.5524 | 3.55142 | 3.55148 |
| 80 | 3.9055 | - | 3.9195 | 3.9095 | 3.9108 | 3.90949 | 3.90919 |
| 100 | 4.2116 | 4.2337 | 4.2289 | 4.2116 | 4.2133 | 4.21163 | 4.21135 |
| Ha | γ | - | |
|---|---|---|---|
| 1st Solution | 2nd Solution | ||
| 0.5 | 0.3 | 0.97533 | −0.09572 |
| 0.5 | 0.65753 | −0.06946 | |
| −0.5 | 0 | 1.34857 | −0.75392 |
| - | 0.5 | 1.02349 | −0.58327 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alarifi, I.M.; Abokhalil, A.G.; Osman, M.; Lund, L.A.; Ayed, M.B.; Belmabrouk, H.; Tlili, I. MHD Flow and Heat Transfer over Vertical Stretching Sheet with Heat Sink or Source Effect. Symmetry 2019, 11, 297. https://doi.org/10.3390/sym11030297
Alarifi IM, Abokhalil AG, Osman M, Lund LA, Ayed MB, Belmabrouk H, Tlili I. MHD Flow and Heat Transfer over Vertical Stretching Sheet with Heat Sink or Source Effect. Symmetry. 2019; 11(3):297. https://doi.org/10.3390/sym11030297
Chicago/Turabian StyleAlarifi, Ibrahim M., Ahmed G. Abokhalil, M. Osman, Liaquat Ali Lund, Mossaad Ben Ayed, Hafedh Belmabrouk, and Iskander Tlili. 2019. "MHD Flow and Heat Transfer over Vertical Stretching Sheet with Heat Sink or Source Effect" Symmetry 11, no. 3: 297. https://doi.org/10.3390/sym11030297
APA StyleAlarifi, I. M., Abokhalil, A. G., Osman, M., Lund, L. A., Ayed, M. B., Belmabrouk, H., & Tlili, I. (2019). MHD Flow and Heat Transfer over Vertical Stretching Sheet with Heat Sink or Source Effect. Symmetry, 11(3), 297. https://doi.org/10.3390/sym11030297









