1. Introduction
1.1. Theory of Uncertainty and Uncertainty Quantification
Uncertainty theory playsanimportant role in modeling sciences and engineering problems. However, there is a basic question regarding how we can define or use the uncertainty concept in our mathematical modeling. Researchers around the globe defined many approaches to defining them, and give their various recommendations to using uncertainty theory. There are several literaturestudiesthatclassify some basic uncertain parameters. It should be noted that there is no unique reorientation of the uncertain parameter. For the problem’s purpose or decision makers’ choice, it can be varied and presented as a different application. We now, here, give some info about uncertain parameters, and show how they differ from eachother using the concept of uncertainty using some definition, flowcharts, and diagrams. In this paper, we recommend the researcher to take the uncertain parameter as a parametric interval valued neutrosophic number.
Some basic differences between some uncertain parameters:
If we take Interval number [
1] then we can see,
If we take Fuzzy number [
2,
3], then we can see,
If we take Intuitionistic fuzzy number [
4], then we can see,
If we take Neutrosophic fuzzy number [
5], then we can see,
The concept of truthiness, falsity, and indeterminacy of the elements comes
The use of membership function for truthiness, falsity, and indeterminacy is present
Please follow the idea given in the flowchart below, as shown in
Figure 1:
1.2. Neutrosophic Number
Fuzzy systems (FSs) and Intuitionistic fuzzy systems (IFSs) cannot successfully deal with a situation where the conclusion is adequate, unacceptable, and decision-maker declaration is uncertain. Therefore, some novel theories are mandatory for solving the problem with uncertainty. The neutrosophic sets (NSs) [
5] reflect on the truth membership, indeterminacy membership, and falsity membership concurrently, which is more practical and adequate than FSs and IFSs in commerce, which areuncertain, incomplete, and inconsistent in sequence. Single-valued neutrosophic sets are an extension of NSs which were introduced by Wang et al. [
6]. Ye [
7] introduced simplify neutrosophic sets, and Peng et al. [
8,
9] definite their novel operations and aggregation operators. Finally, there are different extensions of NSs, such as interval neutrosophic set [
10], bipolar neutrosophic sets [
11], and multi-valued neutrosophic sets [
12,
13]. The decision-making problem [
14,
15,
16,
17,
18,
19,
20,
21,
22,
23,
24,
25,
26,
27,
28,
29,
30,
31,
32,
33,
34,
35,
36,
37,
38] is very important in study, when it is with uncertainty.
Although many researchers and scientists have worked in the recently developed neutrosophic method, and applied it in the field of decision making, there is, however, still some viewpoints regarding defining neutrosophic numbers in different forms, and their corresponding de-impreciseness is very important.
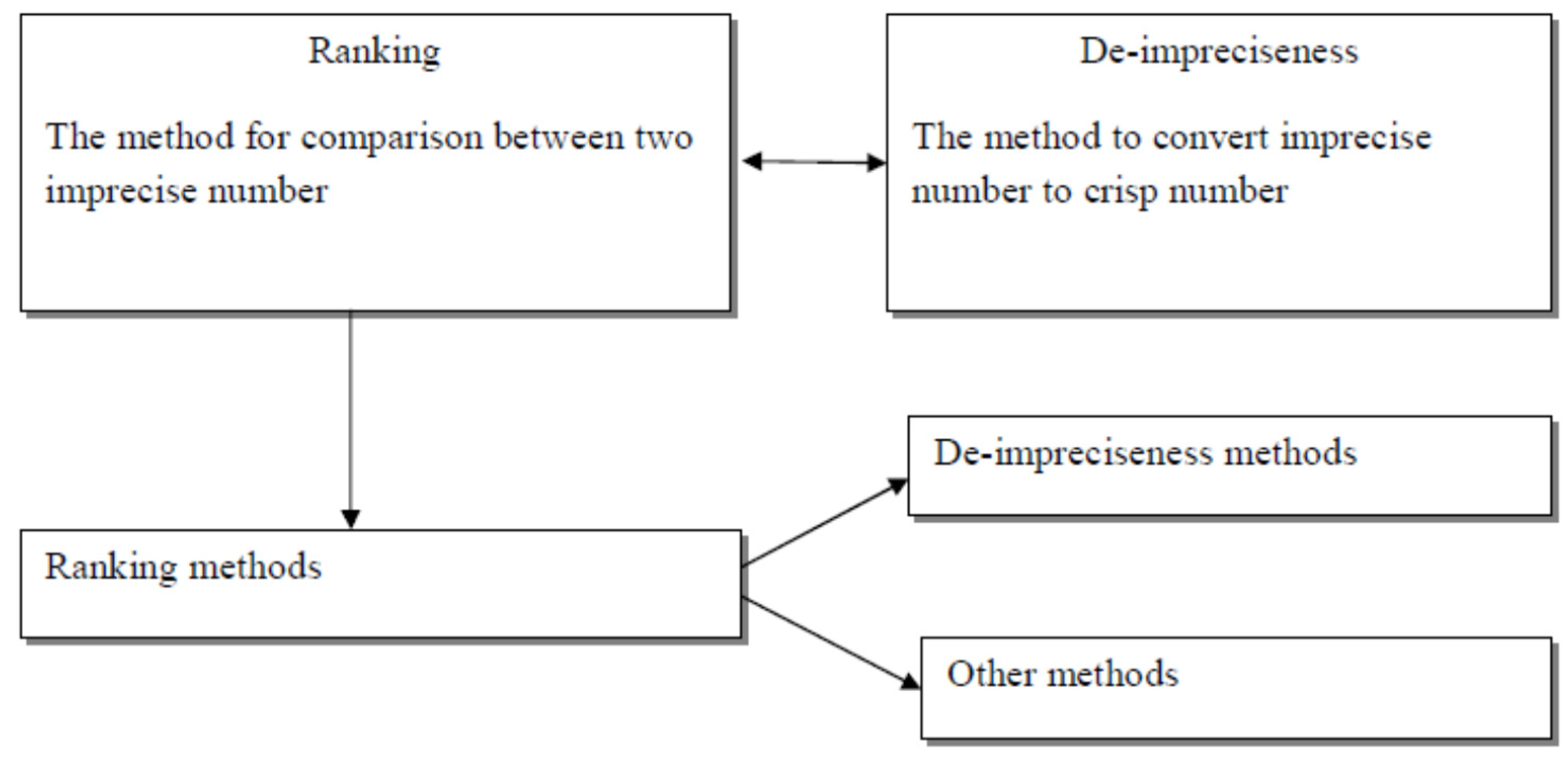
1.3. Ranking and De-Impreciseness
The ranking and de-impreciseness of the imprecise numbers are not a new concept.However, what is the basic concept of the above-said important results and what is the relation.
Figure 2 shows the flowchart for de-impreciseness and ranking.
Ranking is a concept where we can compare two imprecise numbers, and de-impreciseness is a technique where the imprecise number is converted to a crisp number. Somewhere, the decision maker takes the two concepts as the same. In this case, they convert the imprecise number into crisp number, and compares them on the basis of crisp value.
1.4. Structure of the Paper
The paper is organized as follows. In
Section 1, the basic concept on imprecise set theory and neutrosophic set theory are discussed.
Section 2 contains the preliminaries section.
Section 3 goes for the known definition of neutrosophic sets and numbers. Single valued linear neutrosophic number and its variation are showing in
Section 3. In
Section 4, we address the basic concept of neutrosophic non-linear number and generalized neutrosophic number. In
Section 5, the de-neutrosophication of linear neutrosophic triangular fuzzy number is performed. The PERT problem is considered in
Section 6. The application in assignment problem, considering aproblem, is taken in
Section 7. The conclusions are written in
Section 8.
2. Neutrosophic Number
Definition 1. (Neutrosophic Set) A setin the universal discourse, which is denoted generically by, is said to be a neutrosophic set if, whereis called the truth membership function which represents the degree of confidence,is called the indeterminacy membership function which represents the degree of uncertainty, andis called the falsity membership function which represents the degree of scepticism on the decision given the decision maker.
exhibits the following relation:
Definition 2. (Single Valued Neutrosophic Set) Neutrosophic setin the definition 2.3, is called a Single Valued Neutrosophic Setifis a single valued independent variable. Thus, whererepresents the truth, indeterminacy, and falsity membership function, respectively, as stated in definition 2.3, and also exhibits the same relationship as stated earlier.
If there exists three points, , for which , then the is called neut-normal.
A is said to be neut-convex, which implies that it is a subset of a real line, by satisfying the following conditions:
where, .
Definition 3. (Single Valued Neutrosophic Number)Single Valued Neutrosophic Numberis defined aswhere, the truth membership function, the indeterminacy membership function, and the falsity membership functionis given as: 3. Single Valued Linear Neutrosophic Number
Triangular Single Valued Neutrosophic number of Type 1: The quantity of the truth, indeterminacy and falsity are not dependent: A Triangular Single Valued Neutrosophic number of Type 1 is defined as
whose truth membership, indeterminacy and falsity membership is defined as follows:
and
and
where,
,
.
The parametric form of the above type number is
, where,
here,
,
,
and
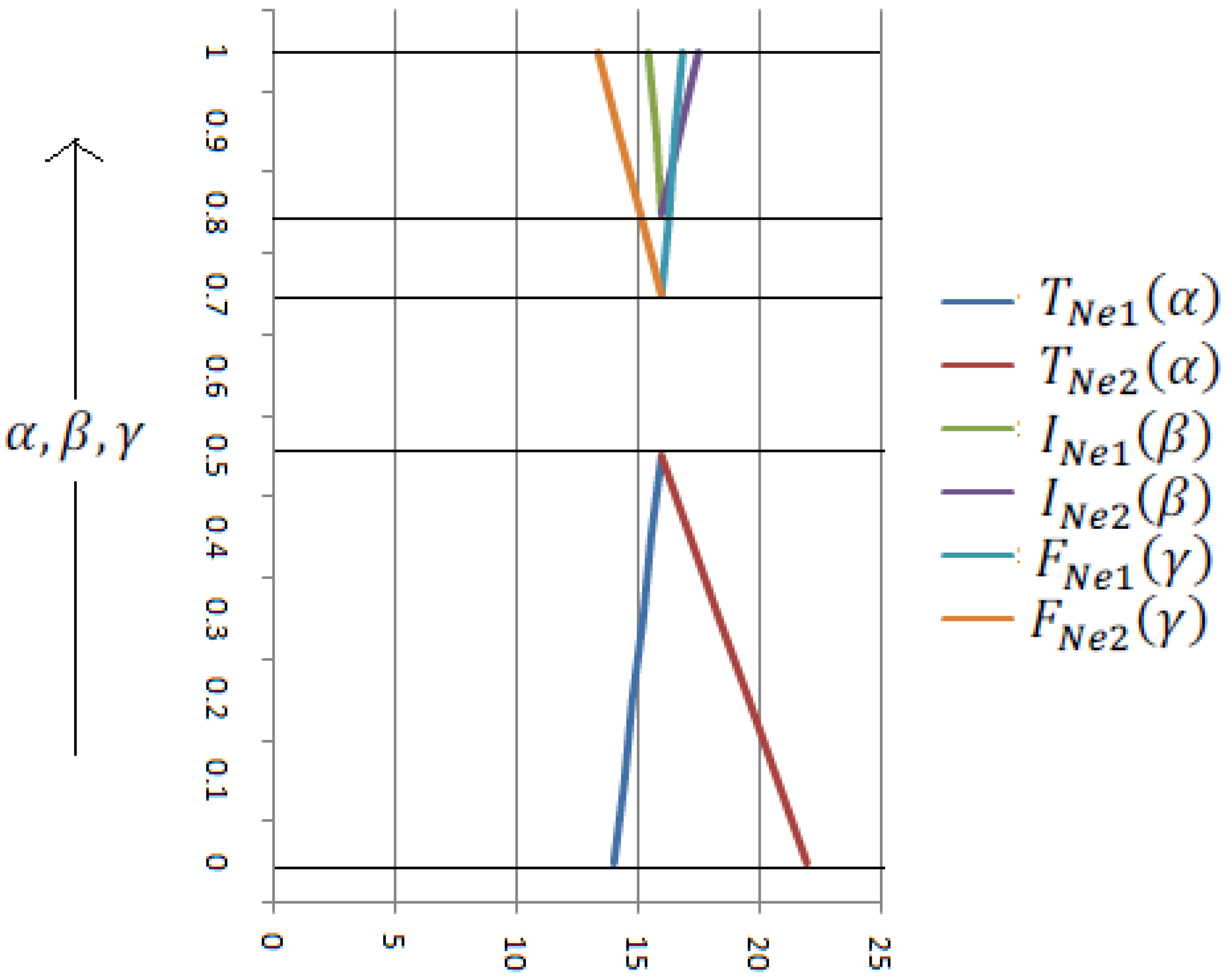
Example 1. Take.
The parametric representation is
Table 1 and
Figure 3 show the value of
,
,
,
,
, and
and graphical representation of triangular single valued neutrosophic numbers (TrSVNNs) respectively.
- 2.
Triangular Single Valued Neutrosophic Number of Type 2: The quantity of indeterminacy and falsity are dependent: A triangular single valued neutrosophic number (TrSVNN) of Type 2 is defined as
whose truth membership, indeterminacy, and falsity membership are defined as follows:
and
and
where,
,
.
The parametric form of the above type number is
, where
Here, , , and and .
Example 2. Take
The parametric representation is,
Table 2 and
Figure 4 show the value of
,
,
,
,
, and
and graphical representation of type-2 TrSVNNs.
- 3.
Triangular Single Valued Neutrosophic number of Type 3: The quantity of the truth, indeterminacy, and falsity are dependent: A TrSVNN of Type 3 is defined as
, whose truth membership, indeterminacy, and falsity membership are defined as follows:
and
and
where,
,
.
The parametric form of the above type number is
, where
Here, , , , and .
Example 3. Take
The parametric representation is,
Table 3 and
Figure 5 show the value of
,
,
,
,
and
. and Graphical representation of type-3 TrSVNNs
Different Operational Laws of Two Triangular Neutrosophic Numbers: If
and
are two single valued neutrosophic numbers with nine components having truthmembership
, indeterminacymembership
, and falsitymembership
, respectively, such as:
where
a, band
c are the scores given by the decision maker in the scale, ranging from lower limit
Ll to upper limit
Ul.
Multiplication by a constant
Divisions
Example 4. Ifandare two single valued neutrosophic numbers with independent truth, indeterminate, and false values in the scale of 0 to 25, then find thewhere k = 3.
Multiplication by a constant
4. Neutrosophic Non-Linear Number and Generalized Neutrosophic Number
4.1. Single Valued Non-Linear Triangular Neutrosophic Number with Nine Components
A single valued non-linear triangular neutrosophic number with nine components is defined as
, whose truth membership, indeterminacy, and falsity membership is defined as:
and
and
where,
,
.
Note. If , then single valued non-linear triangular neutrosophic number with nine components will be converted into single valued linear triangular neutrosophic number with nine components.
4.2. Single Valued Generalized Triangular Neutrosophic Number with Nine Components
A single valued triangular neutrosophic number with nine components is defined as
, whose truth membership, indeterminacy, and falsity membership is defined as:
and
and
where,
,
.
4.3. Single Valued Generalized Non-Linear Triangular Neutrosophic Number with Nine Components
A single valued non-linear triangular neutrosophic number with nine components is defined as
, whose truth membership, indeterminacy, and falsity membership is defined as:
and
and
where,
,
.
Note. if , then single valued generalized non-linear triangular neutrosophic number with nine components will be converted into single valued generalized linear triangular neutrosophic number with nine components.
5. De-Neutrosophication of Linear Neutrosophic Triangular Fuzzy Number
De-Neutrosophication Using Removal Area Method
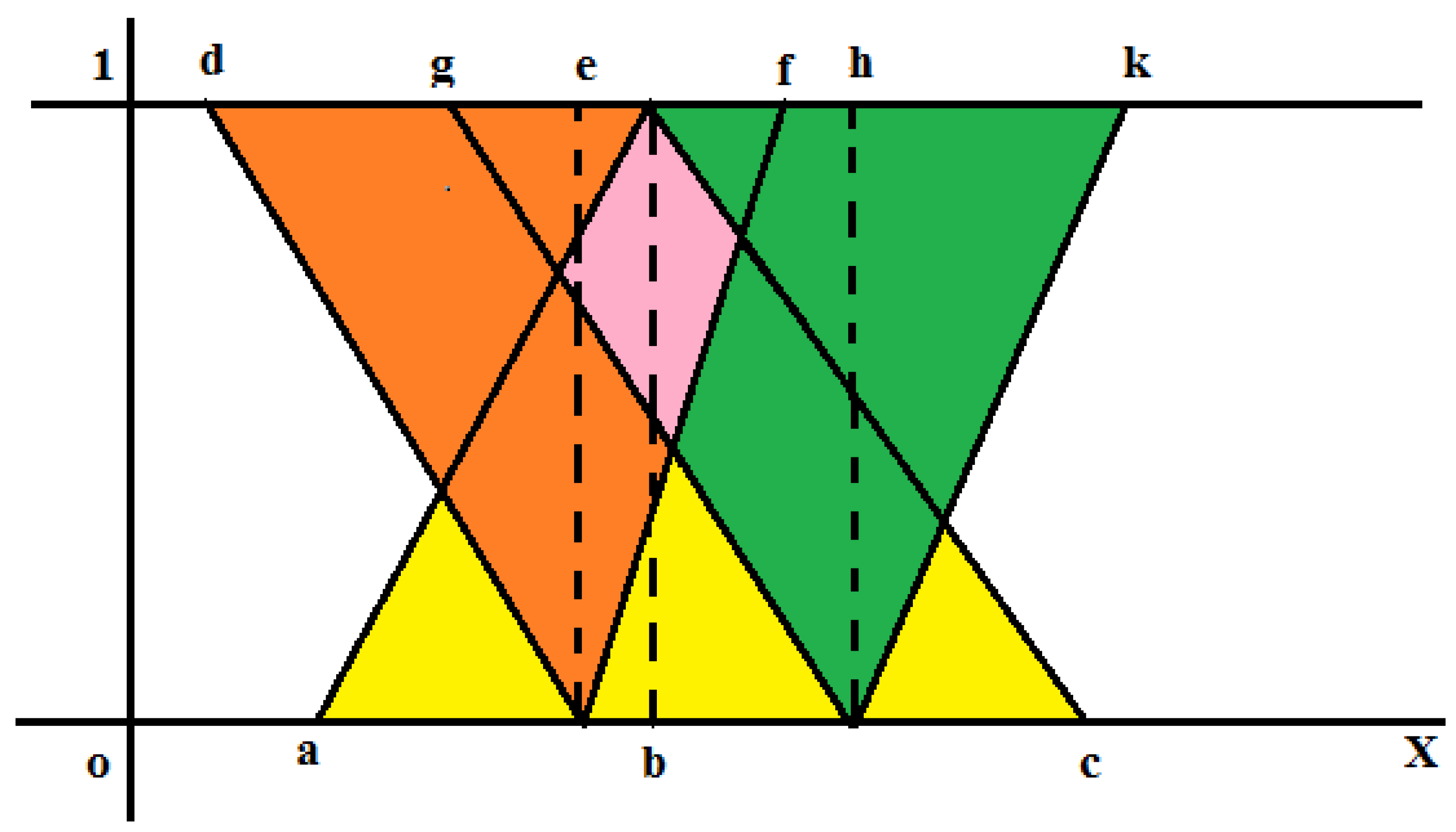
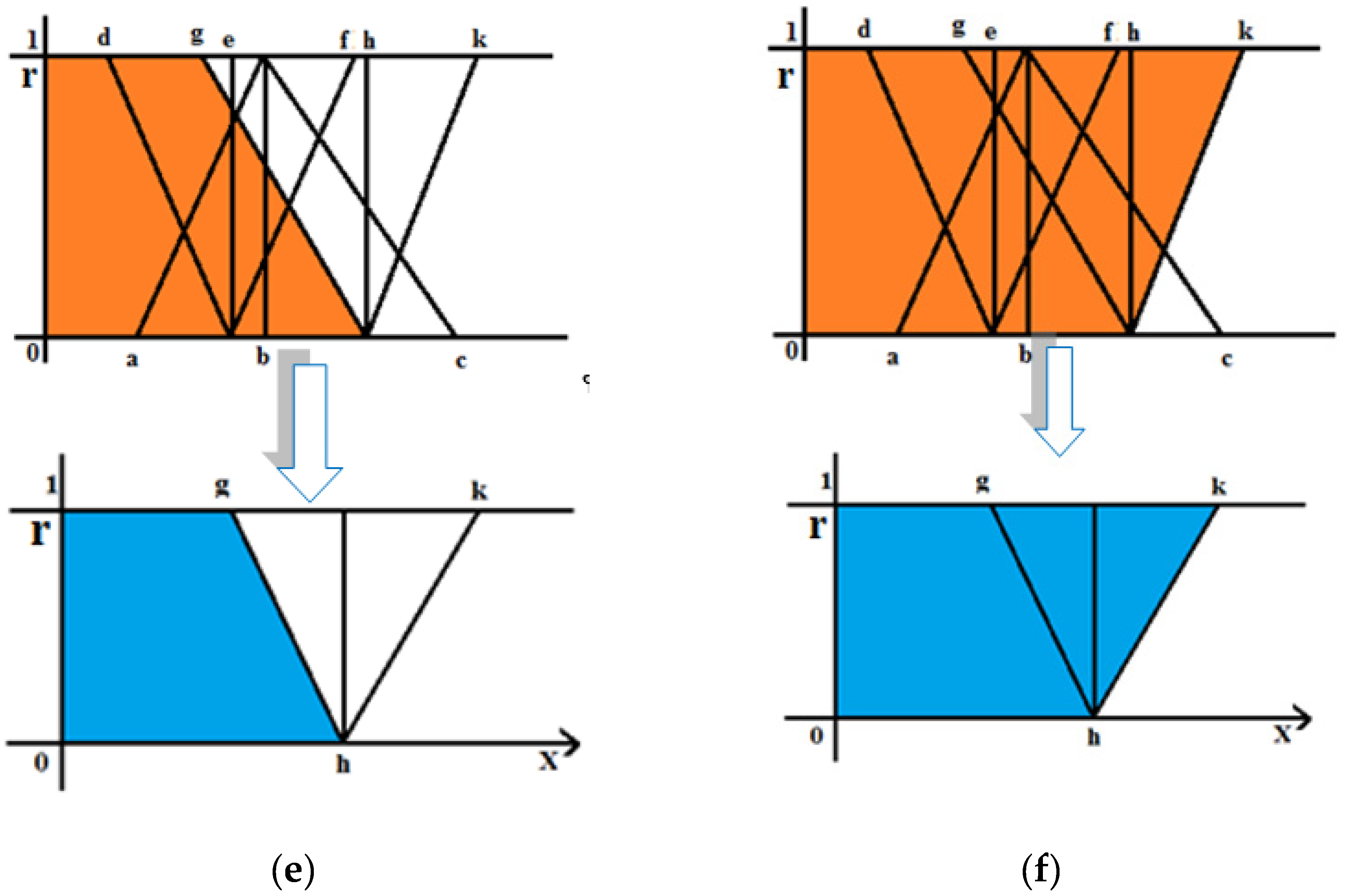
Let us consider a linear neutrosophic triangular fuzzy number as follows:
whose pictorial representation is as follows.
Firstly, we consider the graphical representation of linear neutrosophic triangular fuzzy number in
Figure 6.
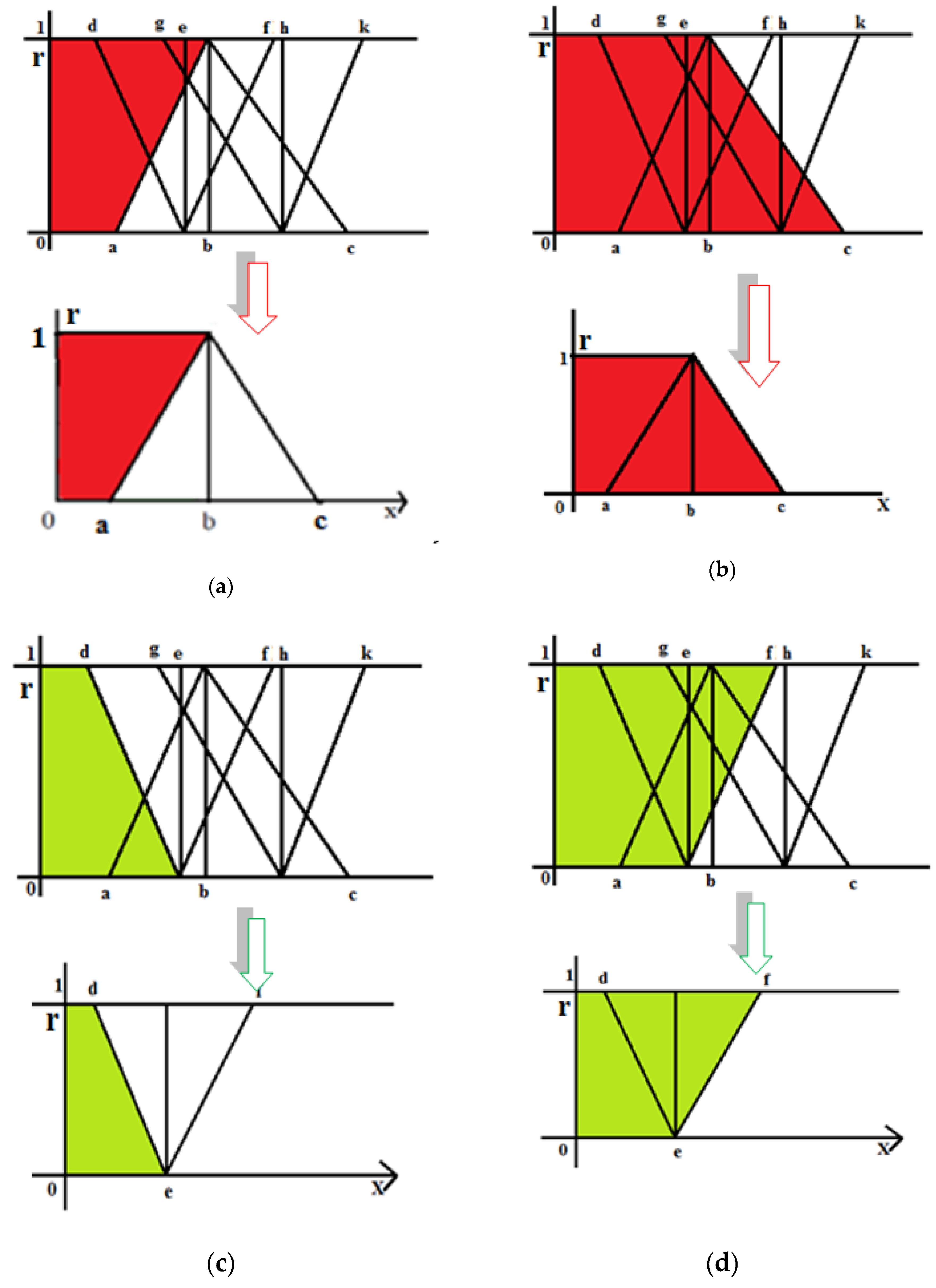
We consider an ordinary number and a fuzzy number for the lower triangle, then left side removal of with respect to k is , defined as the area bounded by k and the left side of the fuzzy number Similarly, the right side removal of with respect to k is Also consider an ordinary number and a fuzzy number for the left most upper triangle(def), then the left side removal of with respect to k is , defined as the area bounded by k and the left side of the fuzzy number Similarly, the right side removal of with respect to k is . A fuzzy number for the right most upper triangle(ghk), then left side removal of with respect to k is , defined as the area bounded by k and the left side of the fuzzy number Similarly, the right side removal of with respect to k is
Mean is defined as , , .
Then, we defined the defuzzification of a linear neutrosophic triangular fuzzy as .
We take .
Figure 7 shows the pictorial representation of de-neutrosophication.
Hence, , .
So, .
Example 5. Finding De-neutrosophication value of Neutrosophic number.
Table 4 shows the de-neutrosophication value of Neutrosophic number.
6. PERT in Triangular Neutrosophic Environment and the Proposed Model
The full form of PERT method is project evaluation and review technique, which is a project management tool used to schedule, organize, and coordinate tasks within a project. It is basically a method to analyze the tasks involved in completing a given project, especially the time needed to complete each task, and to identify the minimum time needed to complete the total project.
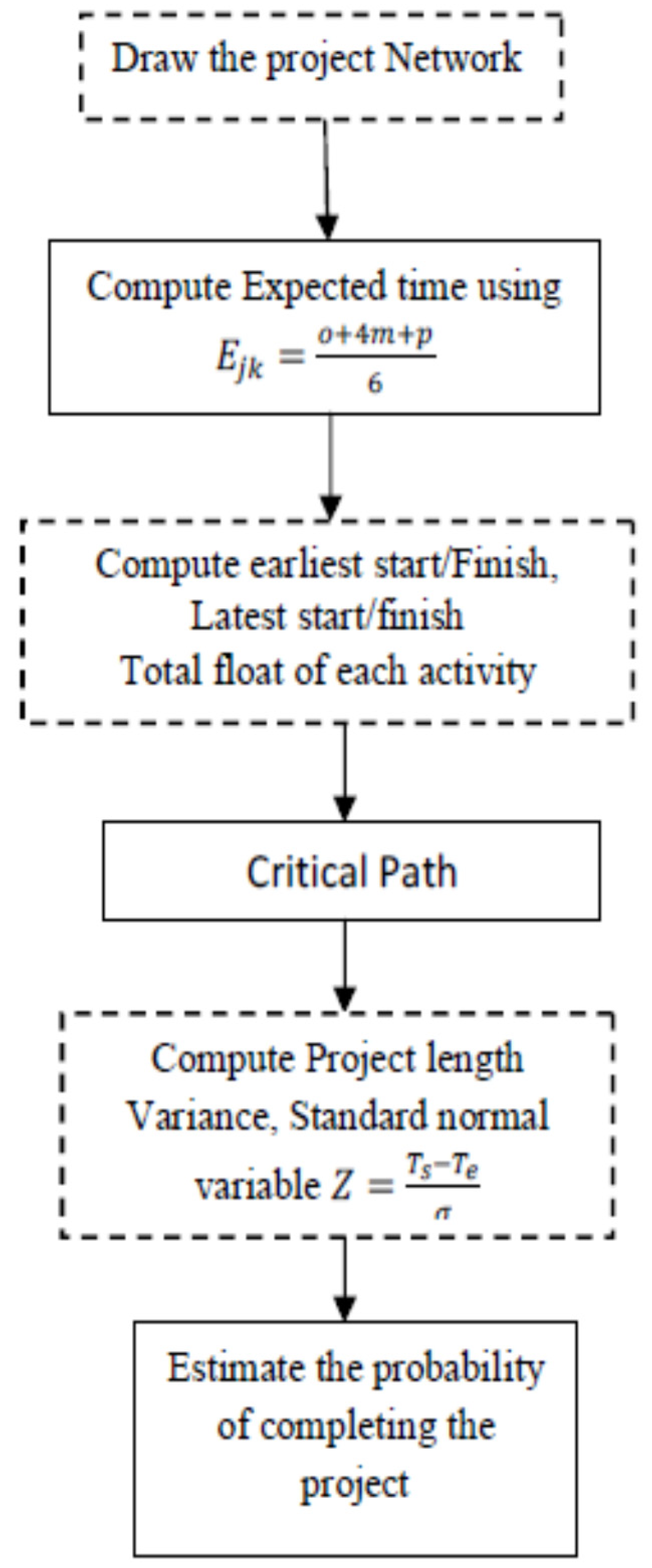
PERT planning involves the following steps:
Identify the specific activities and milestones.
Determine the proper sequence of the activities.
Construct a network diagram.
Estimate the time required for each activity.
Determine the critical path.
Update the PERT chart as the project progresses.
The main objective of PERT is to facilitate decision making and to reduce both the time and cost required to complete a project. PERT is intended for very large-scale, one-time, non-routine, complex projects with a high degree of dependency, projects which require a series of activities, some of which must be performed sequentially, and others that can be performed in parallel with other activities. PERT has been mainly used in new projects which have large uncertainty with respect to design of a structure, technology, and networking system. To take care of associated uncertainties, we introduced triangular neutrosophic environment for PERT activity duration.
The three time estimates for activity duration are as follows:
Optimistic time : Generally, the shortest time in which the activity can be completed. It is common practice to specify optimistic time to be three standards deviations from the mean so that there is approximately a 1% chance that the activity will be completed within the optimistic time.
Pessimistic time : Generally, the longest time that an activity might require. Three standard deviations from the mean are commonly used for the pessimistic time.
Most likely time : Generally, it is the completion time, in normal circumstances, having the highest probability. Note that this time is different from the expected time.
Note 2. In Ref. [
22], the authors introduced the concept of score and accuracy function to compute the crisp value of a trapezoidal neutrosophic number. In our proposed model, we choose all the three different times (optimistic, pessimistic, most likely) as triangular neutrosophic number.
To obtain the crisp value, we introduced the de-neutrosophication value of triangular neutrosophic number .
Now, the expected time and standard deviation can be calculated by the formula and , where o, p, and m are all crisp value of optimistic, pessimistic, and most likely time estimations, respectively.
Now, we use CPM method for further calculation of earliest/latest time, critical path, and float.
In forward pass, starting with a time of zero for the first event, the computation proceeds from left to right, up to the final event. For any activity , let denote the earliest time of event , then . If more than one activity enters an event, the earliest start time for that event is computed as for all activities emanating from node i entering into j.
In case of backward pass, starting with the final node, the computation proceeds from right to left, up to the initial event. For any activity , let denote the latest finished time of event i, then . If more than one activity enters an event, the latest finish time for that event is computed as for all activities emanating from node j entering into i.
After calculating the critical path, compute project length variance, which is the sum of the variances of all the critical activities. Next, calculate the standard normal variable
, where
is the scheduled time to complete the project, and
is the normal expected project length duration. Using a normal curve, we can estimate the probability of completing the project within a specified time. The steps of the said method are shown in
Figure 8. We also set the numerical value for the said problem to show the importance of our method in
Table 5.
Draw the project network and find the probability that the project is completed in 16 days.
Step-1.
Therefore, the expected project duration is 15.9 days.
Critical path ACGJK.
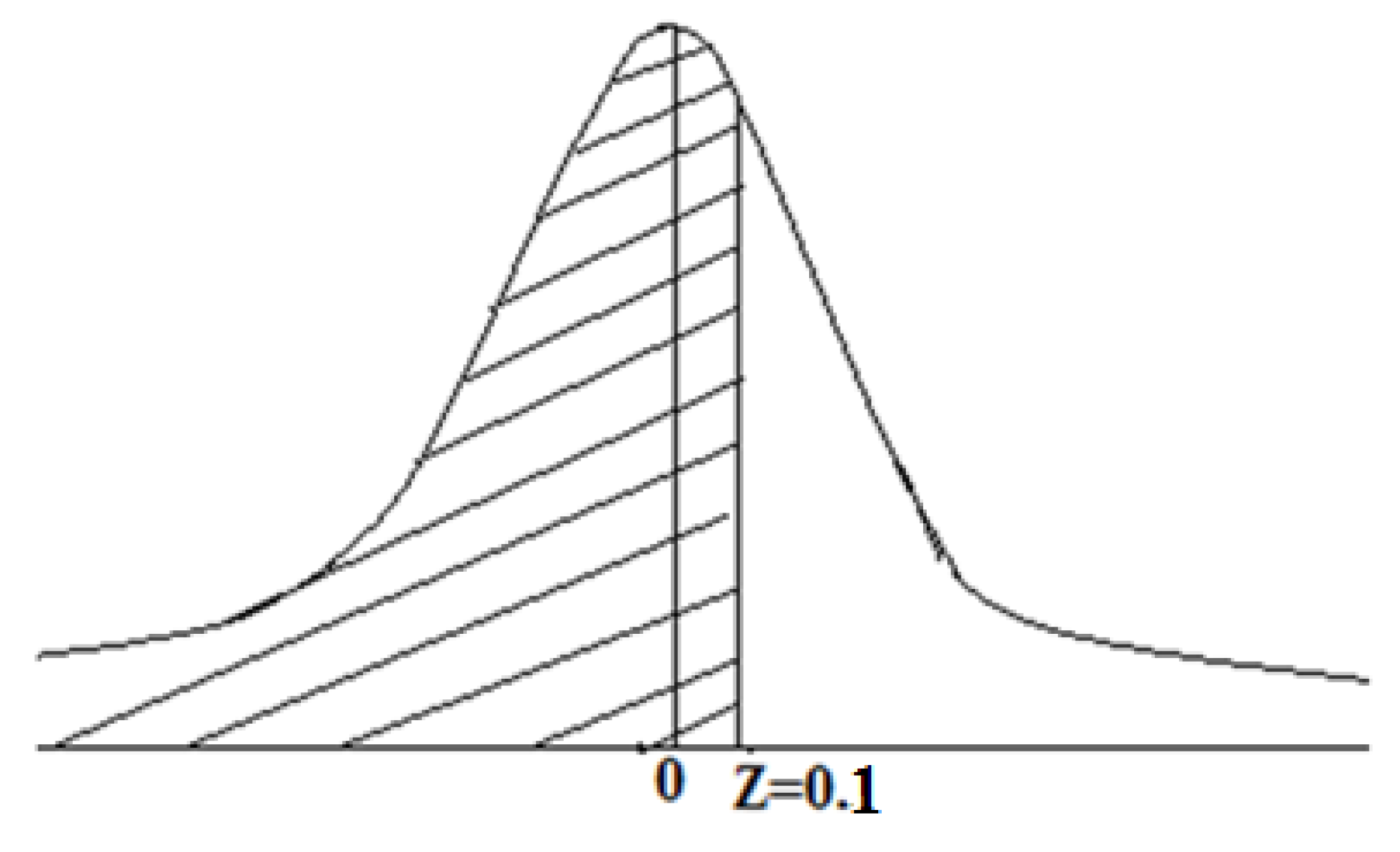
Project length variance , standard deviation0.98.
Probability that the project will be finished within 16 days is
Area under the normal curve = 0.5398
The related normal curve is drawn in
Figure 11.
7. Application of Triangular Neutrosophic Fuzzy Number in Assignment Problem Using De-Neutrosophic Value
The assignment problem is very important for transferring goods from one place to another place. In the assignment problem, if uncertainty occurs, then it is more complicated to solve. By the concept of impreciseness and its corresponding crispified value, we can easily handle the assignment problem. In this section, we take a route selection problem with neutrosophic cost data and solve the problem.
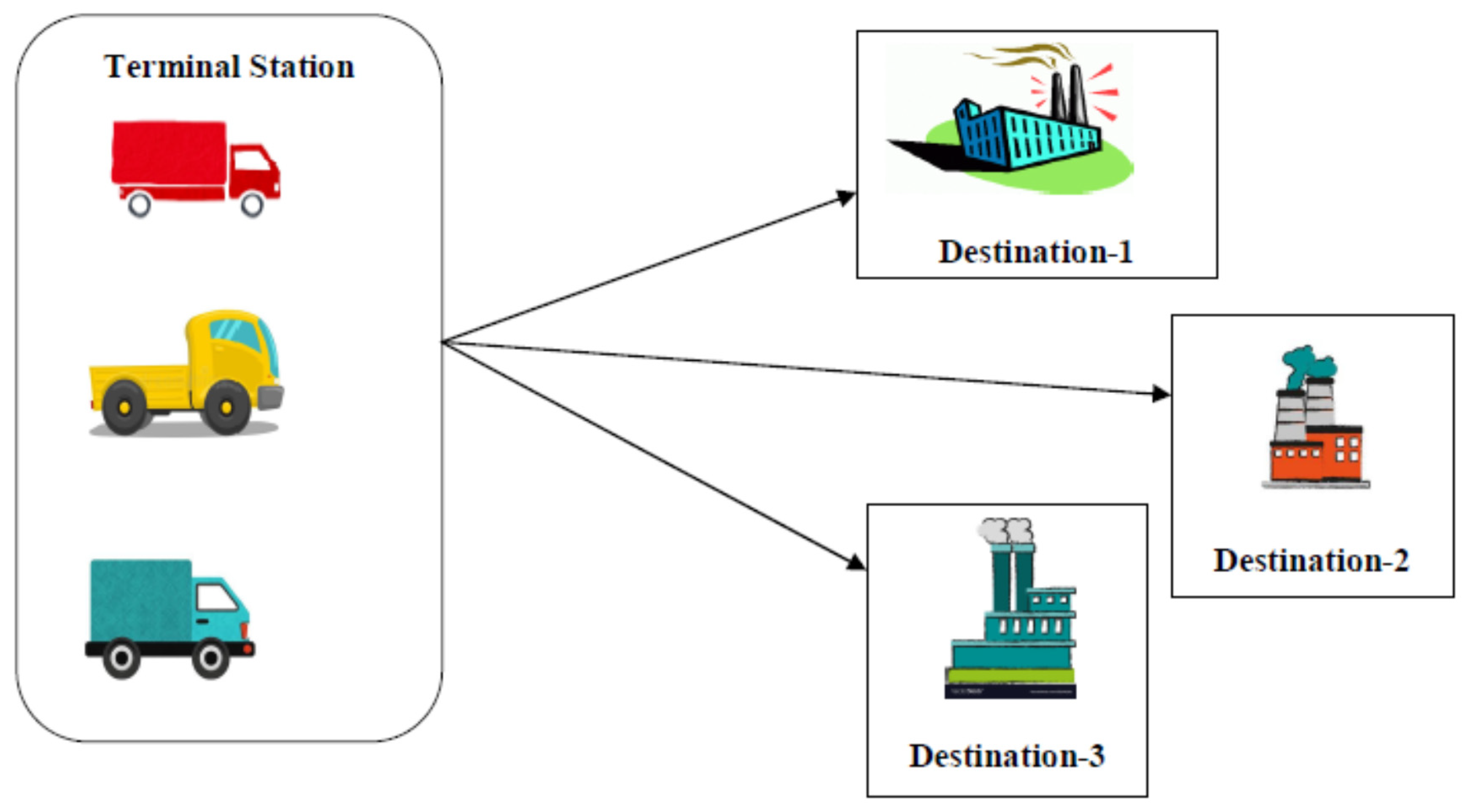
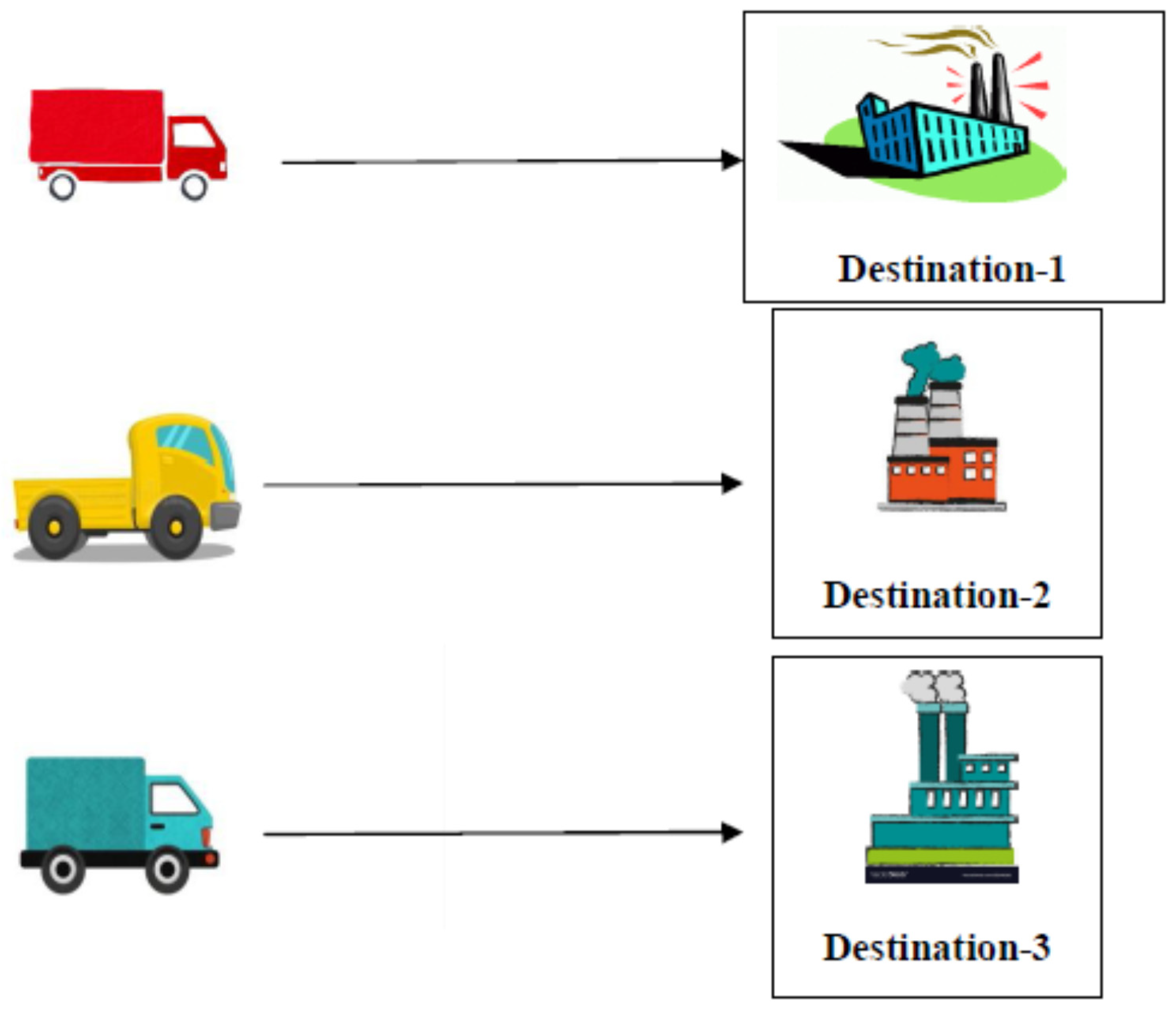
We consider a problem of assigning three different trucks to three different destinations. The assigning costs that are the travelling costs in rupees are given here. How should the trucks be dispatched so as to minimize the total travelling cost? Note, that all the costs are triangular neutrosophic numbers.
Let us consider that the transportation cost for the three trucks are neutrosophic in nature. For that viewpoint, we take that the cost of the three trucks are as follows in
Table 1, in units of dollar. Each component represents the moneys in units of dollars.
Here, red car denotes Truck 1, yellow car denotes Truck 2, and green car denotes Truck 3 as shown in the
Figure 12.
We apply the defuzzification result of triangular neutrosophic number from
Table 7.
to convert the numbers into a crisp number.
Then, we have the following
Table 8.
Now, we consider row minimum from each row, and subtract it from the other element (row-wise). Thus, we get
Table 9.
Now, we consider column minimum from each column and subtract it from the other element (column-wise). Thus, we get
Table 10.
Here, the minimum number of straight lines to cover all the zeros is 3 (which is also equal to the order of the matrix), as shown in
Table 11.
From the
Table 12, we see that if the Truck1 goes to Destination-3, Truck2 goes to Destination-2, and Truck3 goes to Destination-1, then the carrying is minimum.
That means from the
Figure 13 Truck-1
Destination-3, Truck-2
Destination-2, Truck-3
Destination-1.
The corresponding Min cost = (3.92 + 1.71 + 2.92) = 8.55 units of dollar.
Ye [
21] built up the concept of score function and accuracy function. The score function S and the accuracy function H are applied to compare the grades of triangular fuzzy numbers (TFNS). These functions show that greater is the value, the greater is the TFNS, and by using these, concept paths can be ranked.
We apply the result of triangular neutrosophic number.
Let, be a triangular neutrosophic fuzzy number, then the score function is defined as , and accuracy function is defined as .
In order to make comparisons between two triangular neutrosophic values, Ye [
21] presented the order relations between two triangular neutrosophic values.
Let and be two triangular neutrosophic values, then the ranking method is defined as follows.
- (i)
if , then
- (ii)
if and , then
We apply the score function result of triangular neutrosophic number to convert the numbers into a crisp number.
Then we have the following table, as shown in
Table 14.
Take the most negative cost (−1.42), add it with all the elements of the matrix we get
Table 15.
Now, we consider row minimum from each row and subtract it from the other elements (row-wise). Thus, we get
Table 16.
Now, we consider column minimum from each column, and subtract it from the other elements (column-wise). Thus, we get
Table 17.
Here, the minimum number of straight lines to cover all the zeros is 3(which is also equal to the order of the matrix), as shown in
Table 18.
From the
Table 19, we see that if the Truck1 goes to Destination-1, Truck2 goes to Destination-3, and Truck3 goes to Destination-2, then the carrying is minimum.
That means from the
Figure 14 the destination is as follows Truck1
Destination-1, Truck2
Destination-3, Truck3
Destination-2.
The corresponding Min cost = (−0.92 − 1.42 − 0.08) = −2.42 units of dollar.
Note: Since, using de-neutrosophic value, we observe that min cost is 8.55 units of dollar, whereas using score function, we get min cost in negative quantity that is loss, hence de-neutrosophication gives us a better result than the score function.
8. Conclusions
The theory of uncertainty plays a key role in applied mathematical modeling. The concept of neutrosophic number is very popular nowadays. The formation and de-neutrosophication of the corresponding number can be very important for the researcher who deals with uncertainty and decision-making problems. In this paper, we construct the concept triangular neutrosophic number from different viewpoints, which is not defined earlier. We use the concept of linear and non-linear form with generalization of the pick value of truth, falsity, and indeterminacy functions by considering triangular neutrosophic numbers, which are very important for uncertainty theory. We introduced the de-neutrosophication concept for triangular neutrosophic numbers. This concept helps us to convert a neutrosophic number into a crisp number, which is surely helpful for decision-making problems. In future, we can extend the concept into different types of neutrosophic numbers, which can be more applicable in modeling with uncertainty.