Binary Icosahedral Group and 600-Cell

Abstract

In this article, we have an explicit description of the binary isosahedral group as a 600-cell. We introduce a method to construct binary polyhedral groups as a subset of quaternions $\mathbb{H}$ via spin map of $SO(3)$. In addition, we show that the binary icosahedral group in $\mathbb{H}$ is the set of vertices of a 600-cell by applying the Coxeter–Dynkin diagram of $H_4$.

1. Introduction

The classification of finite subgroups in $S{L}_n(\mathbb{C})$ derives attention from various research areas in mathematics. Especially when $n=2$, it is related to McKay correspondence and ADE singularity theory [1].

The list of finite subgroups of $S{L}_2(\mathbb{C})$ consists of cyclic groups (${\mathbb{Z}}_n$), binary dihedral groups corresponded to the symmetry group of regular $2n$-gons, and binary polyhedral groups related to regular polyhedra. These are related to the classification of regular polyhedrons known as Platonic solids. There are five platonic solids (tetrahedron, cubic, octahedron, dodecahedron, icosahedron), but, as a regular polyhedron and its dual polyhedron are associated with the same symmetry groups, there are only three binary polyhedral groups (binary tetrahedral group $2T$, binary octahedral group $2O$, binary icosahedral group $2I$) related to regular polyhedrons. Moreover, it is a well-known fact that there is a correspondence between binary polyhedral groups and vertices of 4-polytopes as follows:

$$\begin{array}{ccc}\hfill 2T& \leftrightarrow & \mathrm{vertices}\mathrm{of}24\text{-}\mathrm{cell},\hfill \\ \hfill 2O& \leftrightarrow & \mathrm{vertices}\mathrm{of}\mathrm{dual}\mathrm{compound}\mathrm{of}24\text{-}\mathrm{cell},\hfill \\ \hfill 2I& \leftrightarrow & \mathrm{vertices}\mathrm{of}600\text{-}\mathrm{cell},\hfill \end{array}$$

where the dual compound of 24-cell means by the compound polytopes obtained from 24-cell and its dual polytope, which is also a 24-cell [2,3,4,5].

As the symmetries of polyhedrons are isometries, the related finite subgroups are also considered as the subgroups of $SU(2)$. As $SU(2)=Sp(1)$ is a spin group of $SO(3)$, we can regard $2T$, $2O$, and $2I$ as subgroups of quaternions $\mathbb{H}$. From this point of view, it is also well known that the vertices of 24 cell correspond to roots of $D_4$, and the set of vertices of the dual compound of 24-cell, which is the union of a 24-cell and a dual 24-cell forms a roots of $F_4$. The 600-cell is a complicated case of a reflection group of $H_4$-type [3,6].

The aim of this article is to provide explicit description of a binary icosahedron group $2I$ as a 600-cell. By applying spin covering map from $Sp(1)$ to $SO(3)$, we introduce a method to construct the binary polyhedral groups in terms of quaternions from the symmetries of regular polyhedrons. Then, by applying the theory of reflection groups along the Coxeter–Dynkin diagram, we show that the subgroup $2I$ in $\mathbb{H}$ is indeed the set of vertices of a 600-cell. We also discuss $2T$ related to 24-cell, but, because the dual compound of a 24-cell is not regular, its relation to $2O$ will be discussed in another article.

2. Binary Polyhedral Groups in Quaternions

Every finite subgroup of $S{L}_2(\mathbb{C})$ is conjugate to a finite subgroup of $SU(2)$ so that the classification of the finite subgroup of $S{L}_2(\mathbb{C})$ including binary polyhedral groups corresponds to the classification of the finite subgroup of $SU(2)$. As $SU(2)\simeq Sp(1)$, we can identify the binary polyhedral groups as certain subsets in quaternions $\mathbb{H}$. In fact, $Sp(1)$ is not only a unit sphere in $\mathbb{H}$ but also the spin group $Spin(3),$ which is a 2-covering map of $SO(3)$. In this section, we explain how an element in $SO(3)$ lifts to quaternions in $Sp(1)$.

The algebra of quaternions $\mathbb{H}$ is the four-dimensional vector space over $\mathbb{R}$ defined by

$$\mathbb{H}:=\left\{a+bi+cj+dk|a,b,c,d\in \mathbb{R}\right\}$$

satisfying relations $i^2=j^2=k^2=ijk=-1$. The quaternionic conjugate of $q=a+bi+cj+dk$ is defined by

$$\overline{q}:=a-bi-cj-dk$$

and the corresponding norm $\left|q\right|$ is also defined by $\left|q\right|:=\sqrt{q\overline{q}}=\sqrt{a^2+b^2+c^2+d^2}$. Along this norm, quaternions satisfy $\left|pg\right|=\left|p\right|\left|g\right|$, which implies that it is one of the normed algebras whose classification consists of real numbers $\mathbb{R}$, complex numbers $\mathbb{C}$, quaternions $\mathbb{H}$, and octonions $\mathbb{O}$. A quaternion q is called $real$ if $\overline{q}=q$ and is called $imaginary$ if $\overline{q}=-q$. According to these facts, we can divide $\mathbb{H}$ into a real part and an imaginary part:

$$\mathbb{H}\simeq {\mathbb{R}}^4\simeq Re(\mathbb{H})\oplus Im(\mathbb{H})=\mathbb{R}\oplus {\mathbb{R}}^3.$$

It is well known that the set of unit sphere $S^3=\left\{q\in \mathbb{H}\left|\left|q\right|=1\right.\right\}$ in $\mathbb{H}$ is a Lie group $Sp(1),$ which is also isomorphic to $SU(2)$ as follows:

$$\begin{array}{ccc}Sp(1)& \simeq & SU(2)=\left\{\left.\left(\begin{array}{cc}a& b\\ -\overline{b}& \overline{a}\end{array}\right)\right|a,b\in {\mathbb{C},|a|}^2+{|b|}^2=1\right\},\\ q=a+bj& ⟷& \left(\begin{array}{cc}a& b\\ -\overline{b}& \overline{a}\end{array}\right).\end{array}$$

Below, we use the identification between ${\mathbb{R}}^3$ and $Im(\mathbb{H})$. Along this, a vector $v=(v_1,v_2,v_3)$ in ${\mathbb{R}}^3$ (resp. a quaternion $q=a_{1}i+a_{2}j+a_{3}k$ in $Im(\mathbb{H})$) is corresponded to a quaternion ${\left(v\right)}^{\#}=v_{1}i+v_{2}j+v_{3}k$ in $Im(\mathbb{H})$ (resp. a vector $\overrightarrow{q}=(a_1,a_2,a_3)$ in ${\mathbb{R}}^3$).

Now, we define a map $\Phi ,$ which is given by an action of $Sp(1)$ on $Im(\mathbb{H})\cong {\mathbb{R}}^3$

$$\begin{array}{cccccc}\Phi :Sp(1)& \to & SO(3),& & & \\ x& \mapsto & \Phi \left(x\right):={\rho }_x& :Im(\mathbb{H})& \to & Im(\mathbb{H})\\ & & & v& \mapsto & xv\overline{x}.\end{array},$$

As a matter of fact, the map ${\rho }_x$ must be defined as

$$\begin{array}{cccc}{\rho }_x& :{\mathbb{R}}^3& \to & {\mathbb{R}}^3\\ & v& \mapsto & \overrightarrow{\left(x{v}^{\#}\overline{x}\right)}\end{array}$$

so that ${\rho }_x$ is in $SO(3)$. However, we use a simpler definition instead. It is well known that $\Phi$ is a 2-covering map, which is also a group homomorphism.

In the next section, we will consider the preimage of $\Phi$ to define the lifting of symmetry groups of polyhedrons in ${\mathbb{R}}^3,$ which are subgroups of $SO(3)$. For this purpose, we consider ${\rho }_x$ further in below.

We observe that the multiplication of two pure quaternions p, $q$ in $Im(\mathbb{H})$ can be written by the cross product × and the standard inner product · on ${\mathbb{R}}^3$:

$$pq=-\overrightarrow{p}·\overrightarrow{q}+{\left(\overrightarrow{p}\times \overrightarrow{q}\right)}^{\#}.$$

After we denote $x=x_0+x_{+}$ ($x_0\in$$Re(\mathbb{H}),$ $x_{+}\in$$Im(\mathbb{H})$), ${\rho }_x(v)$ can be written as

$$\begin{array}{cc}\hfill {\rho }_x(v)& =xv\overline{x}=(x_0+x_{+})v(x_0-x_{+})\hfill \\ & =\left(x_{0}v-\overrightarrow{v}·\overrightarrow{x_{+}}+{\left(\overrightarrow{x_{+}}\times \overrightarrow{v}\right)}^{\#}\right)(x_0-x_{+})\hfill \\ & =(x_0^2-|\overrightarrow{x_{+}}{|}^2)v+2\left(\overrightarrow{x_{+}}·\overrightarrow{v}\right)x_{+}+2x_0{\left(\overrightarrow{x_{+}}\times \overrightarrow{v}\right)}^{\#}.\hfill \end{array}$$

Here, since ${\left|x\right|}^2=x_0^2+{|\overrightarrow{x_{+}}|}^2$, we denote $x=cos\theta +sin\theta \frac{x_{+}}{\left|x_{+}\right|}$ where $cos\theta =x_0$ and $sin\theta =|\overrightarrow{x_{+}}{|}^2$ for some $\theta \in [0,\pi ).$

Now, to understand the meaning of ${\rho }_x(v)$, we consider two cases for $\overrightarrow{v}$ case (1) $\overrightarrow{v}\perp \overrightarrow{x_{+}}$ and case (2) $\overrightarrow{v}//\overrightarrow{x_{+}}$.

(1)

(Case $\overrightarrow{v}\perp \overrightarrow{x_{+}}$) Since $\overrightarrow{v}·\overrightarrow{x_{+}}$$=0$, we have

$$\begin{array}{cc}\hfill {\rho }_x(v)& =(x_0^2-|\overrightarrow{x_{+}}{|}^2)v+2x_0{\left(\overrightarrow{x_{+}}\times \overrightarrow{v}\right)}^{\#}\hfill \\ & =(x_0^2-|\overrightarrow{x_{+}}{|}^2)v+2x_0|\overrightarrow{x_{+}}|{\left({\displaystyle \frac{\overrightarrow{x_{+}}}{|\overrightarrow{x_{+}}|}}\times \overrightarrow{v}\right)}^{\#}\hfill \\ & =cos2\theta v+sin2\theta {\left({\displaystyle \frac{\overrightarrow{x_{+}}}{|\overrightarrow{x_{+}}|}}\times \overrightarrow{v}\right)}^{\#}.\hfill \end{array}$$

(2)

(Case $\overrightarrow{v}//\overrightarrow{x_{+}}$) After we denote $\overrightarrow{v}=t\overrightarrow{x_{+}}$ for some $t\in \mathbb{R}$,

$$\begin{array}{cc}\hfill {\rho }_x(v)& =(x_0^2-|\overrightarrow{x_{+}}{|}^2)t{x}_{+}+2\left(\overrightarrow{x_{+}}·t\overrightarrow{x_{+}}\right)x_{+}\hfill \\ & =\left(t(x_0^2-|\overrightarrow{x_{+}}{|}^2)+2\left(\overrightarrow{x_{+}}·t\overrightarrow{x_{+}}\right)\right)x_{+}\hfill \\ & =t(x_0^2+|\overrightarrow{x_{+}}{|}^2)x_{+}=t{x}_{+}=v.\hfill \end{array}$$

By the two cases above, we conclude ${\rho }_x(v)$ presents the rotation of vector $\overrightarrow{v}$ in ${\mathbb{R}}^3$ with respect to the axis $\overrightarrow{x_{+}}$ by $2{cos}^{-1}{x}_0$$\in [0,2\pi )$.

By applying the above, we have the following lemma.

Lemma 1.

For each element A in $SO(3)$ presenting a rotation with respect to a unit vector $\overrightarrow{a}$ for angle $\alpha \in [0,2\pi )$, the preimage of $\Phi :$ $Sp(1)\to SO(3)$ is given as

$${\Phi }^{-1}\left(A\right)=\left\{\pm \left(cos\frac{\alpha }{2}+sin\frac{\alpha }{2}{\left(\overrightarrow{a}\right)}^{\#}\right)\right\}\subset \mathbb{H}.$$

Note if we choose unit vector $-\overrightarrow{a}$ instead $\overrightarrow{a}$ in the lemma, then the rotation performed for angle $-\alpha$. Therefore, the related lifting is given by $cos\left(-\frac{\alpha }{2}\right)+sin\left(-\frac{\alpha }{2}\right){\left(-\overrightarrow{a}\right)}^{\#}$$=cos\frac{\alpha }{2}+sin\frac{\alpha }{2}{\left(\overrightarrow{a}\right)}^{\#}$. Hence ${\Phi }^{-1}\left(A\right)$ is well defined.

By applying Lemma 1, we can consider the preimage of any subset $G$ in $SO(3)$. We call the preimage ${\Phi }^{-1}\left(G\right)$ the lift of $G$ in $Sp(1)\subset \mathbb{H}$. When $G$ is one of the symmetry groups of regular polyhedrons, the lift ${\Phi }^{-1}\left(G\right)$ is called binary polyhedral group. In particular, we consider binary tetrahedral group $2T$, binary octahedral group $2O$, and binary icosahedral group $2I$, which are lifts of symmetry groups of tetrahedron, octahedron and icosahedron with order $24,48,120$, respectively.

2.1. Symmetry Groups of Regular Polyhedrons

A polyhedron considered here is convex and regular. According to convention, we denote a regular polyhedron by $\{p,q\},$ which means that the polyhedron has only one type of face which is a p-gon, and each vertex is contained in q faces. It is well known that there are only five regular polyhedrons, which are also called Platonic solids. Up to duality, we consider three classes of regular polyhedrons as follows:

$$\begin{array}{ccc}\hfill \mathrm{Tetrahedron}& \leftrightarrow & \mathrm{Tetrahedron}(\mathrm{self-dual}),\\ \hfill \mathrm{Octahedron}& \leftrightarrow & \mathrm{Cube},\\ \hfill \mathrm{Icosahedron}& \leftrightarrow & \mathrm{Dodecahedron}.\end{array}$$

As the special linear group $SO(3)$ is generated by rotations on ${\mathbb{R}}^3$, we consider rotations of ${\mathbb{R}}^3$ preserving a regular polyhedron to study the symmetry group of it. When the axis of the rotation crosses a vertex (the barycenter of an edge, the barycenter or a face resp.), we call the rotation vertex symmetry (edge symmetry, face symmetry resp.). For instance, the tetrahedron has two different types of axes of rotations. One is the line passing through a vertex and the barycenter of the opposite face, and the other is the line connecting barycenters of the edges at the opposite position. We also say that a symmetry has order $n$ if the order of the corresponding rotation is n. Note that the order of each edge symmetry is 2. One can figure all the possible orders of each type of symmetry for regular polyhedrons, as shown in

Table 1. Order of symmetries.

Polyhedron	Tetrahedron	Octahedron	Icosahedron
Point Symmetry	3	4, 2	5
Edge Symmetry	2	2	2
Face Symmetry	3	3	3

.

Construction of binary polyhedral groups

Now, we will introduce a construction which provides a way to find the elements of binary polyhedral groups related to regular polyhedrons when the set of vertices of regular polyhedrons are given.

Assume we have a regular polyhedron $\{p,q\}$ whose barycenter is the origin of ${\mathbb{R}}^3$ and let $\{P_i\}$ be the set of vertices of the regular polyhedron:

(1)

Find the barycenters of vertices, edges and faces (The barycenter of each vertex is itself).

(2)

For each barycenter, derive all the related symmetries in $SO(3)$ by identifying corresponding axis of rotations and its order.

(3)

For each symmetry obtained from step 2, we get related lifts in $\mathbb{H}$ by applying Lemma 1. It is useful to observe that we obtain the axis of rotation and its order instead of related angle where there can be more than one related angle.

(4)

The union of lifts is a subset of binary polyhedral groups. In fact, its union with $\{\pm 1\}$ is the binary polyhedral group by counting elements.

Note: From the above, it is clear that two regular polyhedrons which are dual to each other are associated with the same binary polyhedral groups.

For example, let $B$ be a barycenter of order 3. Then, there are two related angles ${\displaystyle \frac{\pi }{3}}$ and ${\displaystyle \frac{2\pi }{3}}$. Thus, the corresponding lift is

$$\left\{\pm \left(cos{\displaystyle \frac{\pi }{3}}+sin{\displaystyle \frac{\pi }{3}}{\displaystyle \frac{B}{|B|}}\right),\pm \left(cos{\displaystyle \frac{2\pi }{3}}+sin{\displaystyle \frac{2\pi }{3}}{\displaystyle \frac{B}{|B|}}\right)\right\}.$$

Here, we also observe that, if we begin with $-B$ instead of B, the corresponding lifts are still the same.

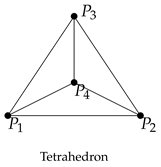
Binary tetrahedral group

We consider a tetrahedron consisting of vertices $\{P_1,P_2,P_3,P_4\}$.

(1)

Since vertex symmetry has order 3, each vertex symmetry has two angles ${\displaystyle \frac{\pi }{3}}$ and ${\displaystyle \frac{2\pi }{3}}$ and the union of lifts of vertex symmetries is

$$V_T:={\displaystyle \bigcup _i}\left\{\pm \left(cos{\displaystyle \frac{\pi }{3}}+sin{\displaystyle \frac{\pi }{3}}{\displaystyle \frac{P_i}{|P_i|}}\right),\pm \left(cos{\displaystyle \frac{2\pi }{3}}+sin{\displaystyle \frac{2\pi }{3}}{\displaystyle \frac{P_i}{|P_i|}}\right)\right\}.$$

Thus, we have

$$\left|V_T\right|=\left|\mathrm{vertices}\mathrm{of}\mathrm{a}\mathrm{tetrahedron}\right|\times 4=16.$$

(2)

As the edge symmetry has order 2, each edge symmetry has only one angle ${\displaystyle \frac{\pi }{2}}$ so that the related lift is

$$\left\{\pm \left(cos{\displaystyle \frac{\pi }{2}}+sin{\displaystyle \frac{\pi }{2}}{\displaystyle \frac{{\displaystyle \frac{P_i+P_j}{2}}}{\left|{\displaystyle \frac{P_i+P_j}{2}}\right|}}\right)\right\}$$

and the union is given as

$$E_T:={\displaystyle \bigcup _{i\ne j}}\left\{\pm \left({\displaystyle \frac{P_i+P_j}{\left|P_i+P_j\right|}}\right)\right\}.$$

Since $P_1+P_2+P_3+P_4=0$, two barycenters ${\displaystyle \frac{1}{2}}\left(P_1+P_2\right)$ and ${\displaystyle \frac{1}{2}}\left(P_3+P_4\right)$ of edges have the same lifts of edge symmetries. Similarly, the pairs of edges produce the same lifts of edge symmetries, and we get

$$\left|E_T\right|=\frac{\left|\mathrm{edges}\mathrm{of}\mathrm{a}\mathrm{tetrahedron}\right|}{2}\times 2=6.$$

(3)

For a barycenter of face consisting of $\left\{P_1,P_2,P_3\right\}$, we have a relation

$${\displaystyle \frac{P_1+P_2+P_3}{3}}=-{\displaystyle \frac{P_4}{3}}$$

since $P_1+P_2+P_3+P_4=0$. Thus, the related lift of face symmetry is the same as the lift of vertex lift for a vertex $P_4$. Similarly, each lift of face symmetry corresponds to the lift of vertex symmetry.

Finally, the union $V_T\cup E_T$ of lifts of symmetries of a tetrahedron is a subset binary tetrahedral group $2T$ in $Sp(1)$. Since

$$\left|V_T\cup E_T\cup \{\pm 1\}\right|=16+6+2=24=\left|2T\right|,$$

the union $V_T\cup E_T\cup \{\pm 1\}$ is a binary tetrahedral group, namely

$$2T=V_T\cup E_T\cup \{\pm 1\}.$$

If we choose vertices $\{P_1,P_2,P_3,P_4\}$ of a tetrahedron as

$$\left\{P_1={\displaystyle \frac{i+j+k}{2}},P_2={\displaystyle \frac{i-j-k}{2}},P_3={\displaystyle \frac{-i+j-k}{2}},P_4={\displaystyle \frac{-i-j+k}{2}}\right\},$$

the corresponding binary tetrahedral group is obtained as

$$2\widehat{T}=:\left\{\pm 1,\pm i,\pm j,\pm k,{\displaystyle \frac{1}{2}}(\pm 1\pm i\pm j\pm k)\right\}.$$

Remark: The subset $2\widehat{T}$ is the unit integral quaternions which is also known as Hurwitz integral quaternions. ([7,8])

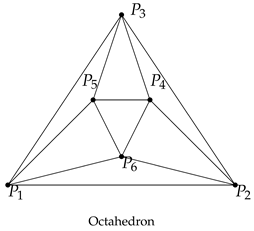
Binary Octahedral Group

We consider an octahedron consisting of vertices $\{P_{i}i=1,\dots ,8\}$ as below.

(1)

The possible orders of vertex symmetry are $2$ and 4. The vertex symmetry with order 4 has two angles ${\displaystyle \frac{\pi }{4}}$ and ${\displaystyle \frac{3\pi }{4}}$ and one with order $2$ has one angle ${\displaystyle \frac{\pi }{2}}$. Thus, the union of lifts of vertex symmetries is

$$V_O:={\displaystyle \bigcup _i}\left\{\pm \left(cos{\displaystyle \frac{\pi }{4}}+sin{\displaystyle \frac{\pi }{4}}{\displaystyle \frac{P_i}{|P_i|}}\right),\pm \left(cos{\displaystyle \frac{3\pi }{4}}+sin{\displaystyle \frac{3\pi }{4}}{\displaystyle \frac{P_i}{|P_i|}}\right),\pm \left(cos{\displaystyle \frac{\pi }{2}}+sin{\displaystyle \frac{\pi }{2}}{\displaystyle \frac{P_i}{|P_i|}}\right)\right\}.$$

Since two antipodal vertices produce the same lifts of vertex symmetries, we obtain that

$$\left|V_O\right|=\frac{\left|\mathrm{vertices}\mathrm{of}\mathrm{an}\mathrm{octahedron}\right|}{2}\times 6=18.$$

(2)

As the edge symmetry has order 2, each edge symmetry has only one angle ${\displaystyle \frac{\pi }{2}}$ so that the union of the related lift is

$$E_O:={\displaystyle \bigcup }\left\{\pm \left({\displaystyle \frac{P_i+P_j}{\left|P_i+P_j\right|}}\right)\right\},$$

where the union is performed for all the pairs of $P_i$ and $P_j$ form an edge. For the barycenter of an edge given by ${\displaystyle \frac{1}{2}}\left(P_i+P_j\right)$, there is exactly one edge whose barycenter is antipodal to ${\displaystyle \frac{1}{2}}\left(P_i+P_j\right)$. Moreover, the pair of edges produce the same lifts of edge symmetries. Therefore, we get

$$\left|E_O\right|=\frac{\left|\mathrm{edges}\mathrm{of}\mathrm{an}\mathrm{octahedron}\right|}{2}\times 2=12.$$

(3)

For a barycenter of face given as ${\displaystyle \frac{P_i+P_j+P_k}{3}}$, the face symmetry has order 3 and it is related to two angles ${\displaystyle \frac{\pi }{3}}$ and ${\displaystyle \frac{2\pi }{3}}$. Thus, the lifts of a face symmetry is

$$\left\{\pm \left(cos{\displaystyle \frac{\pi }{3}}+sin{\displaystyle \frac{\pi }{3}}{\displaystyle \frac{{\displaystyle \frac{P_i+P_j+P_k}{3}}}{\left|{\displaystyle \frac{P_i+P_j+P_k}{3}}\right|}}\right),\pm \left(cos{\displaystyle \frac{2\pi }{3}}+sin{\displaystyle \frac{2\pi }{3}}{\displaystyle \frac{{\displaystyle \frac{P_i+P_j+P_k}{3}}}{\left|{\displaystyle \frac{P_i+P_j+P_k}{3}}\right|}}\right)\right\}$$

and the union of lifting of face symmetries is

$$F_O:={\displaystyle \bigcup _{}}\left\{\pm \left(cos{\displaystyle \frac{\pi }{3}}+sin{\displaystyle \frac{\pi }{3}}{\displaystyle \frac{P_i+P_j+P_k}{|P_i+P_j+P_k|}}\right),\pm \left(cos{\displaystyle \frac{2\pi }{3}}+sin{\displaystyle \frac{2\pi }{3}}{\displaystyle \frac{P_i+P_j+P_k}{|P_i+P_j+P_k|}}\right)\right\}.$$

Since the octahedron is symmetric for origin, for the barycenter of a face given by ${\displaystyle \frac{1}{3}}\left(P_i+P_j+P_k\right)$, there is exactly one face whose barycenter is antipodal to ${\displaystyle \frac{1}{3}}\left(P_i+P_j+P_k\right)$, and the pair of faces produce the same lifts of face symmetries. Therefore, we deduce

$$\left|F_O\right|=\frac{\left|\mathrm{faces}\mathrm{of}\mathrm{an}\mathrm{octahedron}\right|}{2}\times 4=16.$$

Finally, the union $V_O\cup E_O$$\cup F_O$ of lifts of symmetries of an octahedron is a subset of the binary octahedral group $2O$ in $Sp(1)$. Since

$$\left|V_O\cup E_O\cup F_O\cup \{\pm 1\}\right|=18+12+16+2=48=\left|2O\right|,$$

the union $V_O\cup E_O\cup F_O\cup \{\pm 1\}$ is a binary octahedral group, namely

$$2O=V_O\cup E_O\cup F_O\cup \{\pm 1\}.$$

One can take $P_i$s as follows:

$$P_1=i,P_2=j,P_3=k,P_4=-i,P_5=-j,P_6=-k$$

so as to obtain

$$2\widehat{O}:=\left\{\begin{array}{c}\pm 1,\pm i,\pm j,\pm k,\frac{1}{2}\left(\pm 1\pm i\pm j\pm k\right),\\ \frac{1}{\sqrt{2}}\left(\pm 1\pm i\right),\frac{1}{\sqrt{2}}\left(\pm 1\pm j\right),\frac{1}{\sqrt{2}}\left(\pm 1\pm k\right),\frac{1}{\sqrt{2}}\left(\pm i\pm j\right),\frac{1}{\sqrt{2}}\left(\pm i\pm k\right),\frac{1}{\sqrt{2}}\left(\pm j\pm k\right)\end{array}\right\}.$$

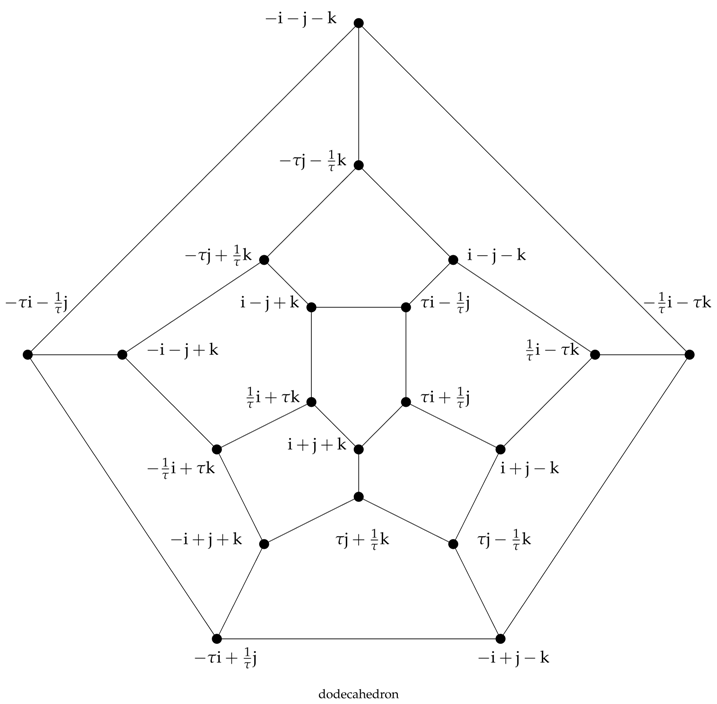
Binary Icosahedral Group

Since both the regular icosahedron and its dual regular dodecahedron produce the binary icosahedral group, we consider a regular dodecahedron in ${\mathbb{R}}^3$ instead of a regular icosahedron. Moreover, for the sake of convenience, one can choose specific coordinates of vertices of a dodecahedron in ${\mathbb{R}}^3$ such as

$$\left\{(\pm 1,\pm 1,\pm 1),(\pm \tau ,\pm {\displaystyle \frac{1}{\tau }},0),(0,\pm \tau ,\pm {\displaystyle \frac{1}{\tau }}),(\pm {\displaystyle \frac{1}{\tau }},0,\pm \tau )\right\},$$

where $\tau ={\displaystyle \frac{\sqrt{5}+1}{2}}=2cos{\displaystyle \frac{\pi }{5}}$ and ${\displaystyle \frac{1}{\tau }}={\displaystyle \frac{\sqrt{5}-1}{2}}=-2cos{\displaystyle \frac{2\pi }{5}}$. It is also useful to know $sin{\displaystyle \frac{\pi }{5}}={\displaystyle \frac{\sqrt{10-2\sqrt{5}}}{4}}$ and $sin{\displaystyle \frac{2\pi }{5}}={\displaystyle \frac{\sqrt{10+2\sqrt{5}}}{4}}$.

In the following diagram, we consider the given set of vertices as a subset in $Im\mathbb{H}={\mathbb{R}}^3$ and depict the configuration among the vertices.

For the above dodecahedron, we denote the set of vertices as $\{P_{i}i=1,\dots ,20\}$ without a specific choice of order.

(1)

Since the possible order of each vertex symmetry is 3, the vertex symmetry has two angles ${\displaystyle \frac{\pi }{3}}$ and ${\displaystyle \frac{2\pi }{3}}$. Thus, the union of lifts of vertex symmetries is

$$V_I:={\displaystyle \bigcup _i}\left\{\pm \left(cos{\displaystyle \frac{\pi }{3}}+sin{\displaystyle \frac{\pi }{3}}{\displaystyle \frac{P_i}{|P_i|}}\right),\pm \left(cos{\displaystyle \frac{2\pi }{3}}+sin{\displaystyle \frac{2\pi }{3}}{\displaystyle \frac{P_i}{|P_i|}}\right)\right\}.$$

Since the dodecahedron is symmetric for origin, each vertex and its antipodal vertex produce the same lifts of vertex symmetries. Thus, we obtain that

$$\left|V_I\right|=\frac{\left|\mathrm{vertices}\mathrm{of}\mathrm{a}\mathrm{dodecahedron}\right|}{2}\times 4=40.$$

(2)

As before, the edge symmetry has order 2, and each edge symmetry has only one angle ${\displaystyle \frac{\pi }{2}}$ so that the union of the related lifts is

$$E_I:={\displaystyle \bigcup }\left\{\pm \left({\displaystyle \frac{P_i+P_j}{\left|P_i+P_j\right|}}\right)\right\},$$

where the union is performed for all the pairs of $P_i$ and $P_j$ form an edge. Just like the lifts of edge symmetries for an octahedron, the pair of antipodal edges produce the same lifts of edge symmetries. Therefore, we get

$$\left|E_I\right|=\frac{\left|\mathrm{edges}\mathrm{of}\mathrm{a}\mathrm{dodecahedron}\right|}{2}\times 2=30.$$

(3)

For a barycenter of face given as ${\displaystyle \frac{1}{5}}\left(P_{i_1}+P_{i_2}+P_{i_3}+P_{i_4}+P_{i_5}\right)$ where $P_{i_l}$ ($l=1,2,3,4,5$) forms a face, the face symmetry has order 5 and it is related to four angles ${\displaystyle \frac{a\pi }{5}}(a=1,2,3,4)$. Thus, the lifts of a face symmetry are

$$\left\{\pm \left(cos{\displaystyle \frac{a\pi }{5}}+sin{\displaystyle \frac{a\pi }{5}}{\displaystyle \frac{{\displaystyle \frac{1}{5}}\left(P_{i_1}+P_{i_2}+P_{i_3}+P_{i_4}+P_{i_5}\right)}{\left|{\displaystyle \frac{1}{5}}\left(P_{i_1}+P_{i_2}+P_{i_3}+P_{i_4}+P_{i_5}\right)\right|}}\right)(a=1,2,3,4)\right\}$$

and the union of lifts of face symmetries is

$$F_I:={\displaystyle \bigcup _{}}\left\{\pm \left(cos{\displaystyle \frac{a\pi }{5}}+sin{\displaystyle \frac{a\pi }{5}}{\displaystyle \frac{P_{i_1}+P_{i_2}+P_{i_3}+P_{i_4}+P_{i_5}}{|P_{i_1}+P_{i_2}+P_{i_3}+P_{i_4}+P_{i_5}|}}\right)(a=1,2,3,4)\right\}.$$

Since a pair of antipodal faces produce the same lifts of face symmetries, we deduce

$$\left|F_I\right|=\frac{\left|\mathrm{faces}\mathrm{of}\mathrm{a}\mathrm{dodecahedron}\right|}{2}\times 8=48.$$

Finally, the union $V_I\cup E_I$$\cup F_I$ of lifts of symmetries of a dodecahedron is a subset of a binary icosahedral group $2I$ in $Sp(1)$. Since

$$\left|V_I\cup E_I\cup F_I\cup \{\pm 1\}\right|=40+30+48+2=120=\left|2I\right|,$$

the union $V_I\cup E_I$$\cup F_I\cup \{\pm 1\}$ is a binary icosahedral group, namely

$$2I=V_I\cup E_I\cup F_I\cup \{\pm 1\}.$$

For the given vertices we have, we can obtain

$$\begin{array}{cc}\hfill V_I& =\left\{{\displaystyle \frac{1}{2}}\left(\pm 1\pm i\pm j\pm k\right),\frac{1}{2}\left(\pm 1\pm \tau j\pm {\displaystyle \frac{1}{\tau }}k\right),\frac{1}{2}\left(\pm 1\pm {\displaystyle \frac{1}{\tau }}i\pm \tau k\right),\frac{1}{2}\left(\pm 1\pm \tau i\pm {\displaystyle \frac{1}{\tau }}j\right)\right\}\hfill \\ \hfill E_I& =\left\{\pm i,\pm j,\pm k,\frac{1}{2}\left(\pm i\pm {\displaystyle \frac{1}{\tau }}j\pm \tau k\right),\frac{1}{2}\left(\pm {\displaystyle \frac{1}{\tau }}i\pm \tau j\pm k\right),\frac{1}{2}\left(\pm \tau i\pm j\pm {\displaystyle \frac{1}{\tau }}k\right)\right\}\hfill \\ \hfill F_I& =\left\{\begin{array}{c}\frac{1}{2}\left(\pm {\displaystyle \frac{1}{\tau }}\pm \tau i\pm k\right),\frac{1}{2}\left(\pm \tau \pm {\displaystyle \frac{1}{\tau }}i\pm j\right),\frac{1}{2}\left(\pm \tau \pm {\displaystyle \frac{1}{\tau }}j\pm k\right),\\ \frac{1}{2}\left(\pm \tau \pm i\pm {\displaystyle \frac{1}{\tau }}k\right),\frac{1}{2}\left(\pm {\displaystyle \frac{1}{\tau }}\pm i\pm \tau j\right),\frac{1}{2}\left(\pm {\displaystyle \frac{1}{\tau }}\pm j\pm \tau k\right)\end{array}\right\}.\hfill \end{array}$$

As a result, we can identify all the elements of the binary icosahedral group as

$$2\widehat{I}:=\left\{\begin{array}{c}\pm 1,\pm i,\pm j,\pm k,{\displaystyle \frac{1}{2}}\left(\pm 1\pm i\pm j\pm k\right)\\ \frac{1}{2}\left(\pm i\pm {\displaystyle \frac{1}{\tau }}j\pm \tau k\right),\frac{1}{2}\left(\pm 1\pm \tau j\pm {\displaystyle \frac{1}{\tau }}k\right),\frac{1}{2}\left(\pm {\displaystyle \frac{1}{\tau }}\pm \tau i\pm k\right),\frac{1}{2}\left(\pm \tau \pm {\displaystyle \frac{1}{\tau }}i\pm j\right),\\ \frac{1}{2}\left(\pm {\displaystyle \frac{1}{\tau }}i\pm \tau j\pm k\right),\frac{1}{2}\left(\pm \tau \pm {\displaystyle \frac{1}{\tau }}j\pm k\right),\frac{1}{2}\left(\pm \tau \pm i\pm {\displaystyle \frac{1}{\tau }}k\right),\frac{1}{2}\left(\pm {\displaystyle \frac{1}{\tau }}\pm i\pm \tau j\right),\\ \frac{1}{2}\left(\pm \tau i\pm j\pm {\displaystyle \frac{1}{\tau }}k\right),\frac{1}{2}\left(\pm {\displaystyle \frac{1}{\tau }}\pm j\pm \tau k\right),\frac{1}{2}\left(\pm 1\pm {\displaystyle \frac{1}{\tau }}i\pm \tau k\right),\frac{1}{2}\left(\pm 1\pm \tau i\pm {\displaystyle \frac{1}{\tau }}j\right)\end{array}\right\}.$$

Theorem 1.

The finite subsets $2\widehat{T}$, $2\widehat{O}$ and $2\widehat{I}$ in $\mathbb{H}$ defined as above are a binary tetrahedral group, a binary octahedral group, and a binary icosahedral group, respectively.

Note that it is well known that the a subset $\left\{\pm 1,\pm i,\pm j,\pm k,{\displaystyle \frac{1}{2}}(\pm 1\pm i\pm j\pm k)\right\}$ in $2I$ is the vertices of 24-cell and the complementary subset in $2I$ is the vertices of a snub 24-cell.

3. 600-Cell

The Coxeter–Dynkin diagrams are the way of describing the group generated by reflections. For each graph, node represents a mirror (or a reflection hypersurface) and the label $m$ attached to a branch between nodes marks the dihedral angle $\frac{\pi }{m}$ between two mirrors. By convention, no label is attached to a branch if the corresponding dihedral angle is $\frac{\pi }{3}$. When all the dihedral angles are $\frac{\pi }{3}$, the diagram is called simply laced. Ringed nodes present so called active mirrors where there is a point $P$ not to sit in the hyperplanes of reflections corresponded to the mirrors. By successive applying the reflections in the diagram to the point P, we obtain a polytope whose symmetry group is the Weyl group generated by the Coxeter–Dynkin diagram. Moreover, the combinatorics of subpolytopes can also be decoded by the Coxeter–Dynkin diagram when it is simply laced with one ringed node (see [7,9,10]). In fact, a similar method can be applied for the diagram, which is not simply laced or has more than one ringed node.

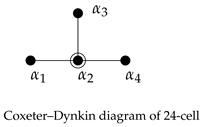
The Coxeter–Dynkin diagram of 24-cell is an example of simply laced with one ringed node.

The Weyl group associated with this diagram is $D_4$-type. In [7], the subpolytopes of 24-cell as shown in

Table 2. Subpolytopes of 24-cell.

Subpolytope	Vertices	Edges	Faces	Cells
total number	24	96	96 ($\{3\}$)	24 ($\{3,3\}$)

are described by using the Coxeter–Dynkin diagram.

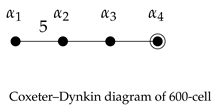
The Coxeter–Dynkin diagram of 600-cell is given by

whose Weyl group is $H_4$-type. Thus, the diagram is not simply laced and has one ringed node.

(1) Vertices

By removing a ringed node, we obtain the isotropy subgroup in the Weyl group of $H_4$ which fixed a vertex in the 600-cell. Here, the corresponding isotropy group is $H_3$ and we can compute the total number of vertices as

$${\displaystyle \frac{|H_4|}{|H_3|}}={\displaystyle \frac{14400}{120}}=120.$$

For the remaining diagram above, we ring a node connected to the removed node. Then, we obtain the Coxeter–Dynkin diagram of an Icosahedron, which implies that the vertex figure of $600$ cell is an icosahedron.

(2) Edges

For edges, we consider the ringed node that performs one reflection corresponding to an edge.

For the isotropy subgroup of the edge, we remove the unringed node connected to the ringed node. In addition, the remaining diagram generates the isotropy subgroup $H_2\times A_1$. Thus, we compute the total number of edges as

$${\displaystyle \frac{|H_4|}{|H_2||A_1|}}={\displaystyle \frac{14400}{10·2}}=720.$$

(3) Faces

For faces, we consider the ringed node and extend the diagram to unringed nodes so as to obtain a subdiagram of $A_2$-type. The subdiagram of $A_2$ with one ringed node generate $\{3\}$, namely a triangle. Thus, the faces of 600-cell are all triangles.

For the isotropy subgroup of a face, we remove any unringed node connected to the subdiagram of a face. The remaining subdiagram generates the isotropy subgroup of a face, which is $A_1\times A_2$. Thus, we compute the total number of faces as

$${\displaystyle \frac{|H_4|}{|A_1||A_2|}}={\displaystyle \frac{14400}{2!\dot{3}!}}=1200.$$

(4) Cells

To obtain a cell in a 600-cell, we consider an extended diagram from the ringed nodes to unringed nodes so as to obtain a subdiagram of $A_3$. The diagram of type $A_3$ with one ringed node on one side represents a tetrahedron.

For the isotropy subgroup of a cell, we consider that any unringed node connected to the subdiagram of $A_3$, and the subdiagram given by removing the node generates the isotropy subgroup, which is $A_3$. Thus, we compute the total number of cells,

Table 3. Subpolytopes of 600-cell.

Subpolytopes	Vertices	Edges	Faces	Cells
total number	120	720	$1200$($\{3\}$)	$600$($\{3,3\}$)

shows the subpolytopes of 600:

$${\displaystyle \frac{|H_4|}{|A_3|}}={\displaystyle \frac{14400}{4!}}=600.$$

4. Binary Polyhedral Groups as Polytopes

In this section, we show that the binary icosahedral group $2I$ in $\mathbb{H}$ is the set of vertices of a 600-cell. Thus, the convex hull of $2I$ in $\mathbb{H}$ is a 600-cell.

For each $\alpha$ in $\mathbb{H}$ with $\left|\alpha \right|=1$, we define a reflection on $\mathbb{H}$ as

$$\begin{array}{cccc}{\sigma }_{\alpha }:& \mathbb{H}& ⟶& \mathbb{H},\\ & x& \mapsto & {\sigma }_{\alpha }(x):=x-2\left(\overrightarrow{x}·\overrightarrow{\alpha }\right)\alpha .\end{array}$$

Since $\mathbb{H}$ is a normed division algebra, ${\sigma }_{\alpha }(x)$ is also written as ${\sigma }_a(x)=-a\overline{x}a$ via quaternionic multiplication (see Ref. [7]). Since ${\sigma }_a$ is a reflection for a vector $\alpha$, ${\sigma }_a$ has eigenvalues $\pm 1$ where $\alpha$ is an eigenvector of $-1$ and the hyperplane perpendicular to $\alpha$ is the eigenspace of 1. Moreover, it is not an element in $SO(3)$.

Binary tetrahedral group$2\widehat{T}$in$\mathbb{H}$and 24-cell

For 24-cell, we consider the Coxeter–Dynkin diagram of type $D_4$ given in Section 3, where

$${\alpha }_1=i,{\alpha }_2={\displaystyle \frac{1}{2}}(1+i+j+k),{\alpha }_3=j,{\alpha }_4=k.$$

In Ref. [7], the Weyl group generated by the Coxeter–Dynkin diagram acts on the binary tetrahedral group $2\widehat{T}$. Moreover, it is shown that $2\widehat{T}$ is the set of vertices of a 24-cell. In fact, it is also the subset of unit integral quaternions.

Binary icosahedral group$2\widehat{I}$in$\mathbb{H}$and 600-cell

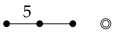
Similarly, for 600-cell, we consider the Coxeter–Dynkin diagram of Type $H_4$ given in Section 3, where

$${\alpha }_1=i,{\alpha }_2={\displaystyle \frac{1}{2}}\left(\tau i+j-\frac{1}{\tau }k\right),{\alpha }_3=j,{\alpha }_4={\displaystyle \frac{1}{2}}\left(-\frac{1}{\tau }+j+\tau k\right).$$

The Weyl group generated by the reflections $\left\{{\sigma }_{{\alpha }_i}i=1,2,3,4\right\}$ is denoted by $W_H$. In below, we want to show that (1) the Weyl group $W_H$ acts on the binary icosahedral group $2\widehat{I}$, and (2) $2\widehat{I}$ is a single orbit, where it corresponds to the set of vertices of 600-cell.

Lemma 2.

The Weyl group $W_H$ acts on the binary icosahedral group $2\widehat{I}$ in $\mathbb{H}$.

Proof. 

Since the Weyl group $W_H$ is generated by the reflections ${\sigma }_{{\alpha }_i}$ ($i=1,2,3,4$), we show that each ${\sigma }_{{\alpha }_i}$ acts on $2I$. For an arbitrary element $a+bi+cj+dk\in \mathbb{H}$, the reflections are written as follows:

$$\begin{array}{cc}\hfill {\sigma }_{{\alpha }_1}(a+bi+cj+dk)& =a-bi+cj+dk,\hfill \\ \hfill {\sigma }_{{\alpha }_2}(a+bi+cj+dk)& =a+\left(-\frac{1}{2\tau }b-\frac{\tau }{2}c+\frac{1}{2}d\right)i\hfill \\ & +\left(-\frac{\tau }{2}b+\frac{1}{2}c+\frac{1}{2\tau }d\right)j+\left(\frac{1}{2}b+\frac{1}{2\tau }c+\frac{\tau }{2}d\right)k,\hfill \\ \hfill {\sigma }_{{\alpha }_3}(a+bi+cj+dk)& =a+bi-cj+dk,\hfill \\ \hfill {\sigma }_{{\alpha }_4}(a+bi+cj+dk)& =\left(\frac{\tau }{2}a+\frac{1}{2\tau }c+\frac{1}{2}d\right)+bi\hfill \\ & +\left(\frac{1}{2\tau }a+\frac{1}{2}c-\frac{\tau }{2}d\right)j+\left(\frac{1}{2}a-\frac{\tau }{2}c-\frac{1}{2\tau }d\right)k.\hfill \end{array}$$

It is easy to see that ${\sigma }_{{\alpha }_1}$ and ${\sigma }_{{\alpha }_3}$ act on $2\widehat{I}$. By choosing $\left\{1,i,j,k\right\}$ as an ordered orthonormal basis of $\mathbb{H}$, ${\sigma }_{{\alpha }_2}$ and ${\sigma }_{{\alpha }_4}$ can be written as

$${\sigma }_{{\alpha }_2}=\left(\begin{array}{cccc}1& 0& 0& 0\\ 0& -\frac{1}{2\tau }& -\frac{\tau }{2}& \frac{1}{2}\\ 0& -\frac{\tau }{2}& \frac{1}{2}& \frac{1}{2\tau }\\ 0& \frac{1}{2}& \frac{1}{2\tau }& \frac{\tau }{2}\end{array}\right)\mathrm{and}{\sigma }_{{\alpha }_4}=\left(\begin{array}{cccc}\frac{\tau }{2}& 0& \frac{1}{2\tau }& \frac{1}{2}\\ 0& 1& 0& 0\\ \frac{1}{2\tau }& 0& \frac{1}{2}& -\frac{\tau }{2}\\ \frac{1}{2}& 0& -\frac{\tau }{2}& -\frac{1}{2\tau }\end{array}\right).$$

In addition, these are similar because ${\sigma }_{{\alpha }_4}=S^t{\sigma }_{{\alpha }_2}S,$ where $S$ is an orthogonal matrix

$$\left(\begin{array}{cccc}0& 1& 0& 0\\ 0& 0& 0& 1\\ 0& 0& 1& 0\\ 1& 0& 0& 0\end{array}\right).$$

In fact, S is an element in $SO(4)$ defined by

$$1\to k,i\to 1,j\to j,k\to i$$

and one can check that $S$ acts on $2\widehat{I}$ by simple calculation. Thus, it suffices to show that ${\sigma }_{{\alpha }_2}$ acts on $2I$ to check ${\sigma }_{{\alpha }_2}$ and ${\sigma }_{{\alpha }_4}$ act on $2\widehat{I}$. For ${\sigma }_{{\alpha }_2}$, we consider $3\times 3$ submatrix A of ${\sigma }_{{\alpha }_2}$ defined as

$$A:=\left(\begin{array}{ccc}-\frac{1}{2\tau }& -\frac{\tau }{2}& \frac{1}{2}\\ -\frac{\tau }{2}& \frac{1}{2}& \frac{1}{2\tau }\\ \frac{1}{2}& \frac{1}{2\tau }& \frac{\tau }{2}\end{array}\right).$$

This is a automorphism of $Im\mathbb{H}$ which satisfies $A^{t}A=Id$ and $detA=-1$. Moreover, $A$ also acts on

$$\left\{(\pm 1,\pm 1,\pm 1),(\pm \tau ,\pm {\displaystyle \frac{1}{\tau }},0),(0,\pm \tau ,\pm {\displaystyle \frac{1}{\tau }}),(\pm {\displaystyle \frac{1}{\tau }},0,\pm \tau )\right\},$$

which is our choice of the vertices of a dodecahedron. Since $A$ is a reflection, it is also a symmetry of the dodecahedron so that it also acts on the set of edges and the set of faces. According to the construction of binary icosahedral group $2\widehat{I}$ in Section 2.1, the action of $A$ on the icosahedron induces the action of ${\sigma }_{{\alpha }_2}$ on $2\widehat{I}$. For example, an edge symmetry given by an edge $P_i+P_j$ is sent to another given by $A{P}_i+A{P}_j$ because

$${\sigma }_{{\alpha }_2}\left({\displaystyle \frac{P_i+P_j}{\left|P_i+P_j\right|}}\right)={\displaystyle \frac{A{P}_i+A{P}_j}{\left|P_i+P_j\right|}}={\displaystyle \frac{A{P}_i+A{P}_j}{\left|A{P}_i+A{P}_j\right|}}.$$

Similarly, we conclude that ${\sigma }_{{\alpha }_2}$ acts on $2\widehat{I}$. ☐

By applying the above lemma, we obtain the following theorem.

Theorem 2.

The set $2\widehat{I}$ of a binary icosahedral group is an orbit of the Weyl group $W_H$, and it is the set of vertices of a 600-cell.

Proof. 

By Lemma 2, the Weyl group $W_H$ acts on $2I$. Now, we consider an element $1$ in $2\widehat{I}$ and its orbit $W_H\left\{1\right\}\subset$ $2\widehat{I}$. Since $1$ is perpendicular to ${\alpha }_1=i,{\alpha }_2={\displaystyle \frac{1}{2}}\left(\tau i+j-\frac{1}{\tau }k\right)$, ${\alpha }_3=j$ and $1·{\alpha }_4=\left(1,0,0,0\right)·\left(-\frac{1}{2\tau },0,\frac{1}{2},\frac{\tau }{2}\right)\ne 0$, the orbit $W_H\left\{1\right\}$ is given by the following Coxeter–Dynkin diagram 3 of 600-cell. By Section 3, we have $\left|W_H\left\{1\right\}\right|=120=\left|2\widehat{I}\right|$. Therefore, we conclude $W_H\left\{1\right\}=2\widehat{I}$ and this gives the theorem. ☐