A Study on Neutrosophic Cubic Graphs with Real Life Applications in Industries
Abstract
1. Introduction
2. Preliminaries
- (i)
- for all
- (ii)
- for all , for all .
- (iii)
- for all , for all
- (i)
- for all .
- (ii)
- for all for all
- (iii)
- for all for all
- (iv)
- for all for all
- (i)
- if
- (ii)
- if
- (iii)
- if
- (iv)
- if
- (v)
- if
- (vi)
- ( if .
- (i)
- if
- (ii)
- if
- (iii)
- if .
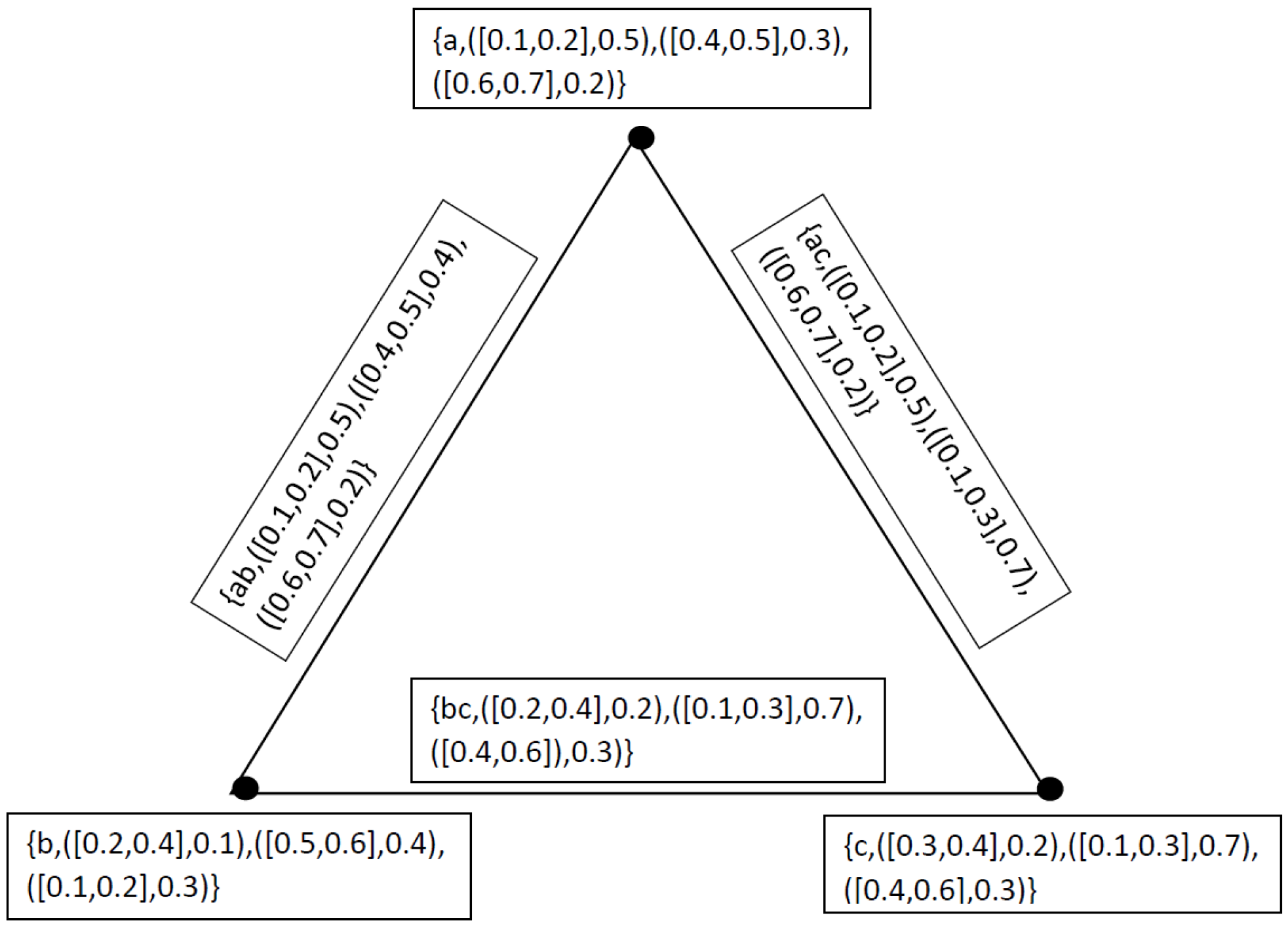
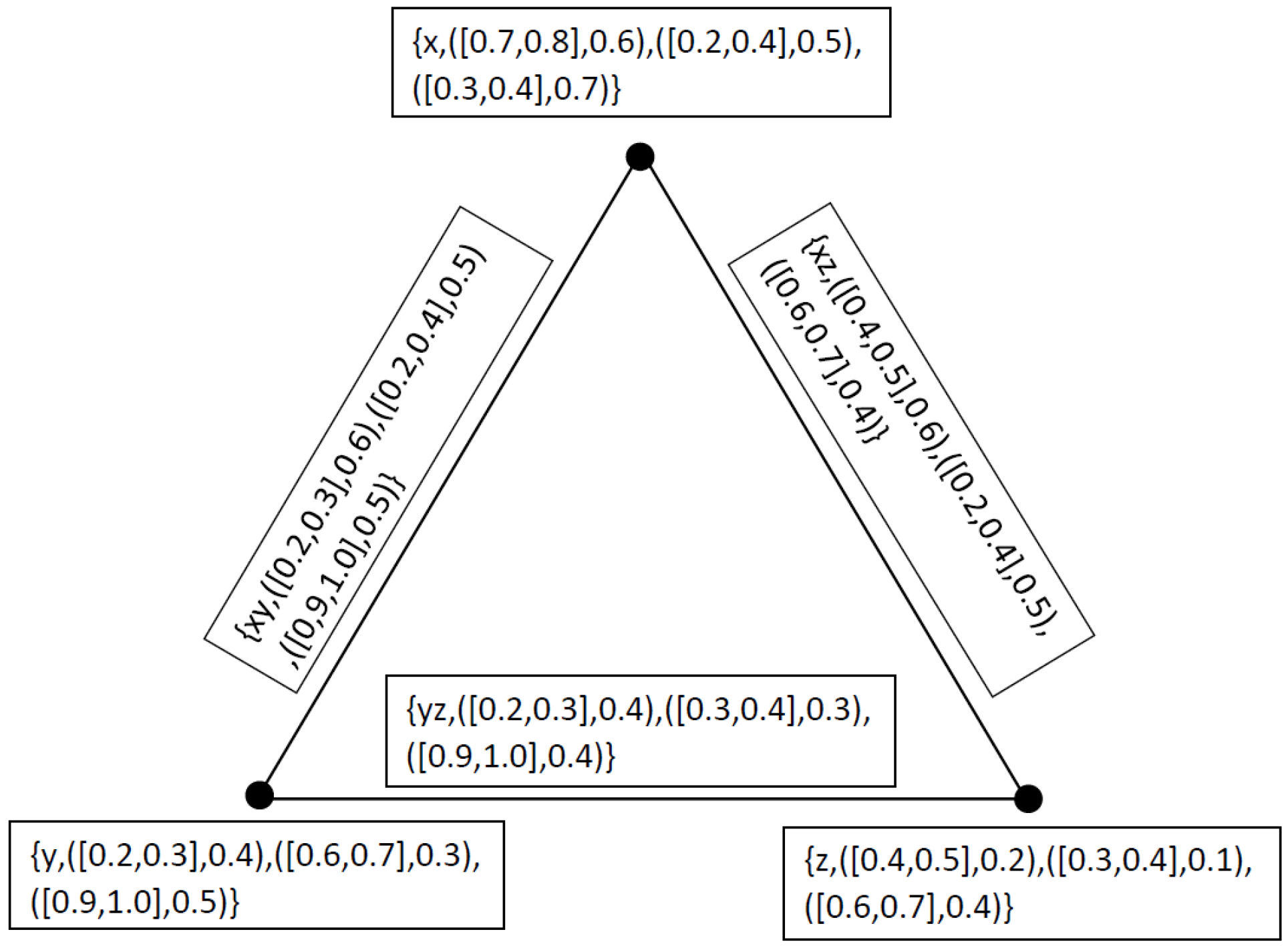
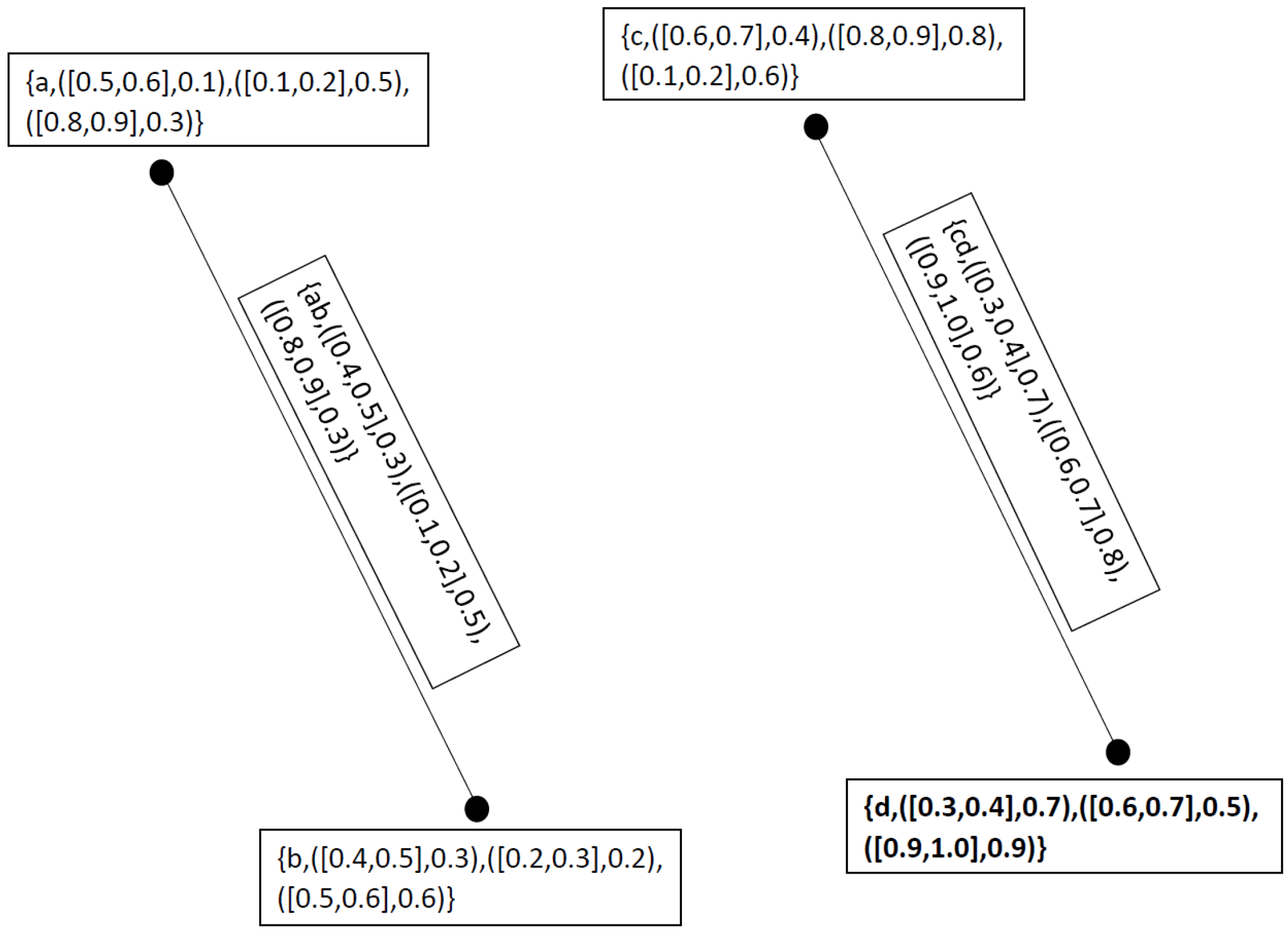
3. Neutrosophic Cubic Graphs
- (i)
- (ii)
- (iii)
- 1.
- If in the vertex set and in the set of edges then the graphs is a neutrosophic cubic polygon only when we join each vertex to the corresponding vertex through an edge.
- 2.
- If we have infinite elements in the vertex set and by joining the each and every edge with each other we get a neutrosophic cubic curve.
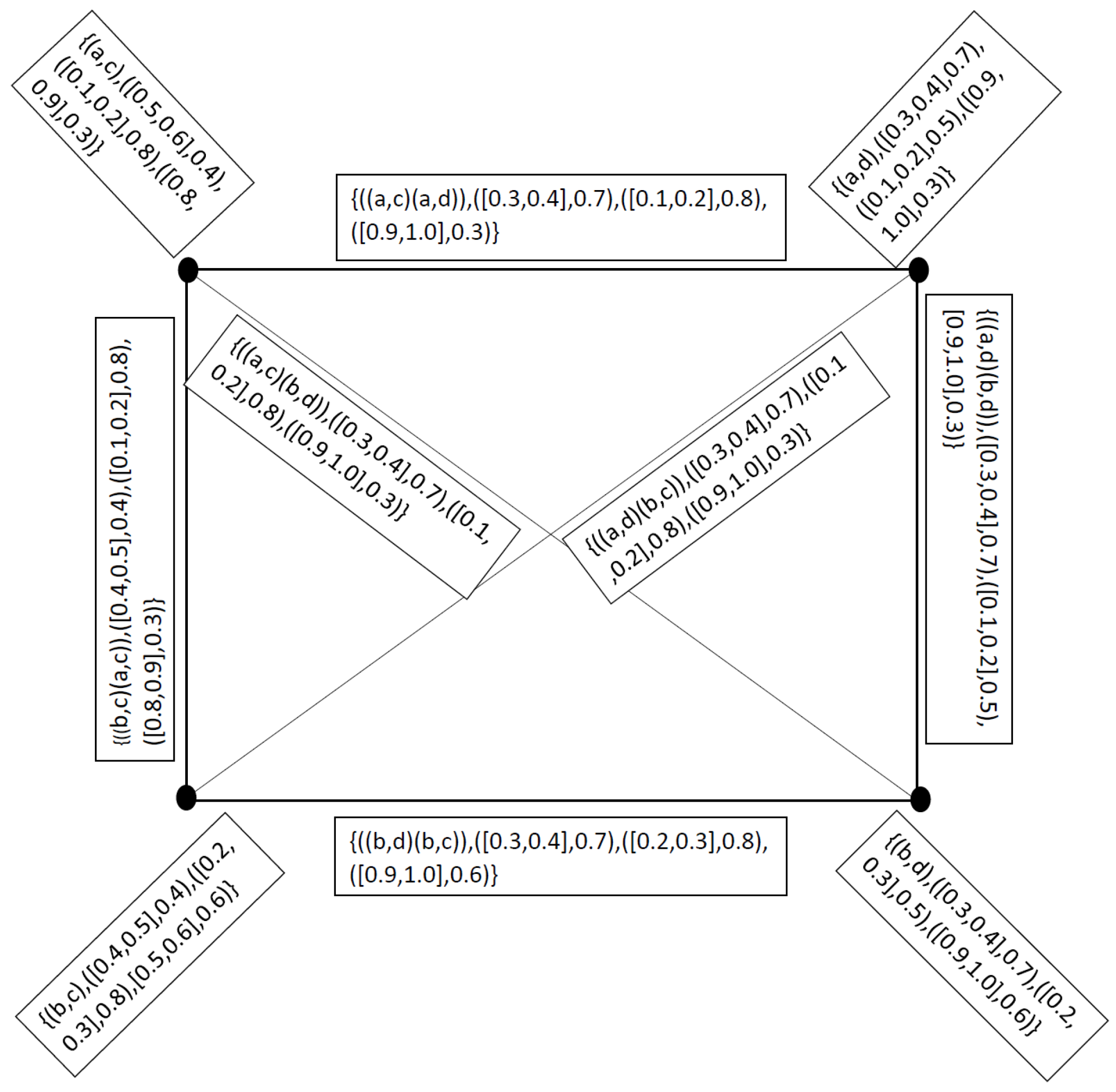
- (i)
- (ii)
- (iii)
- (iv)
- (v)
- (vi)
- (vii)
- (viii)
- (ix)
- ∀ for and for and for .
- (i)
- (ii)
- and
- (iii)
- and
- (iv)
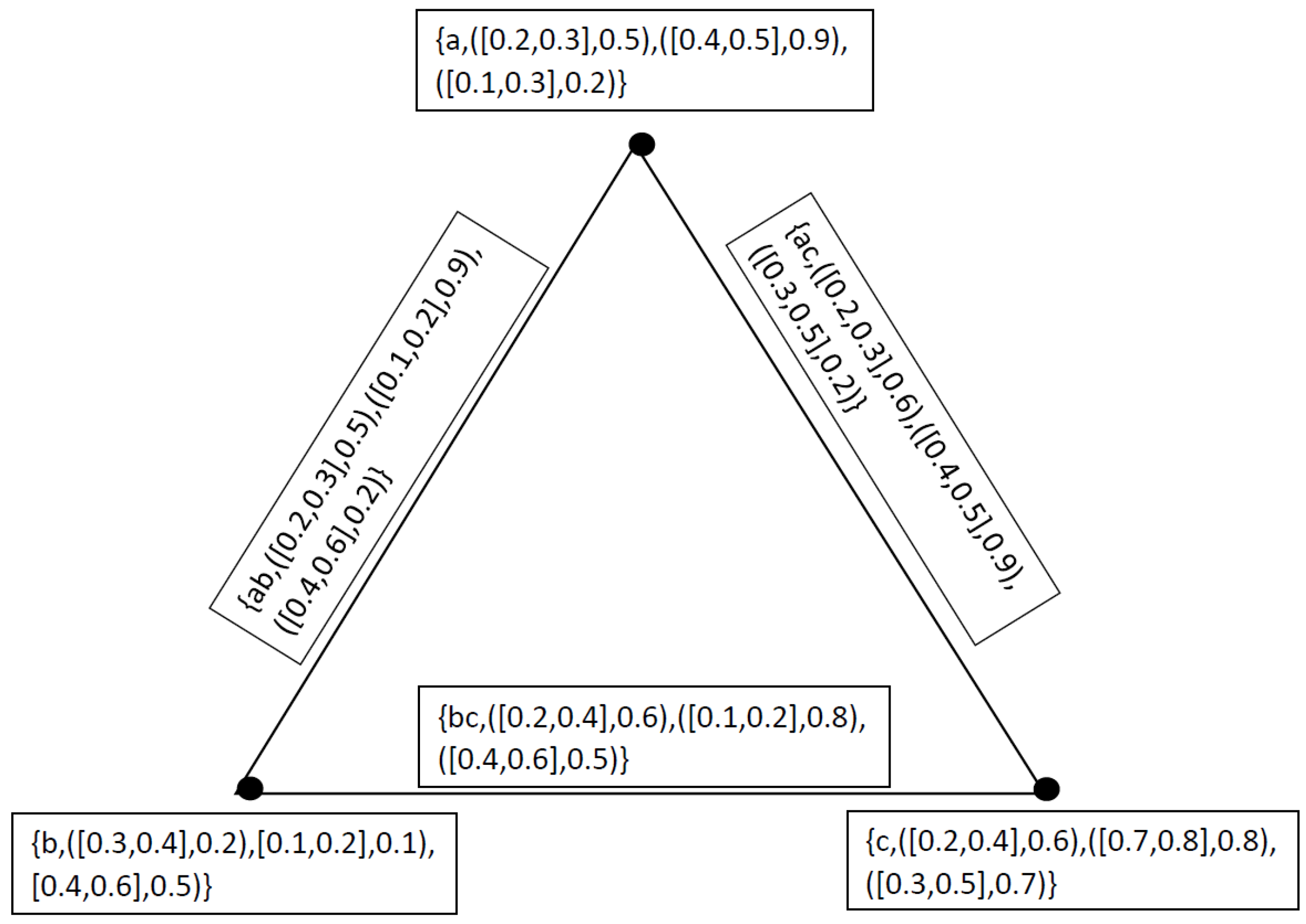
- (i)
- if
- (ii)
- if
- (iii)
- if , where is the set of all edges joining the vertices of and
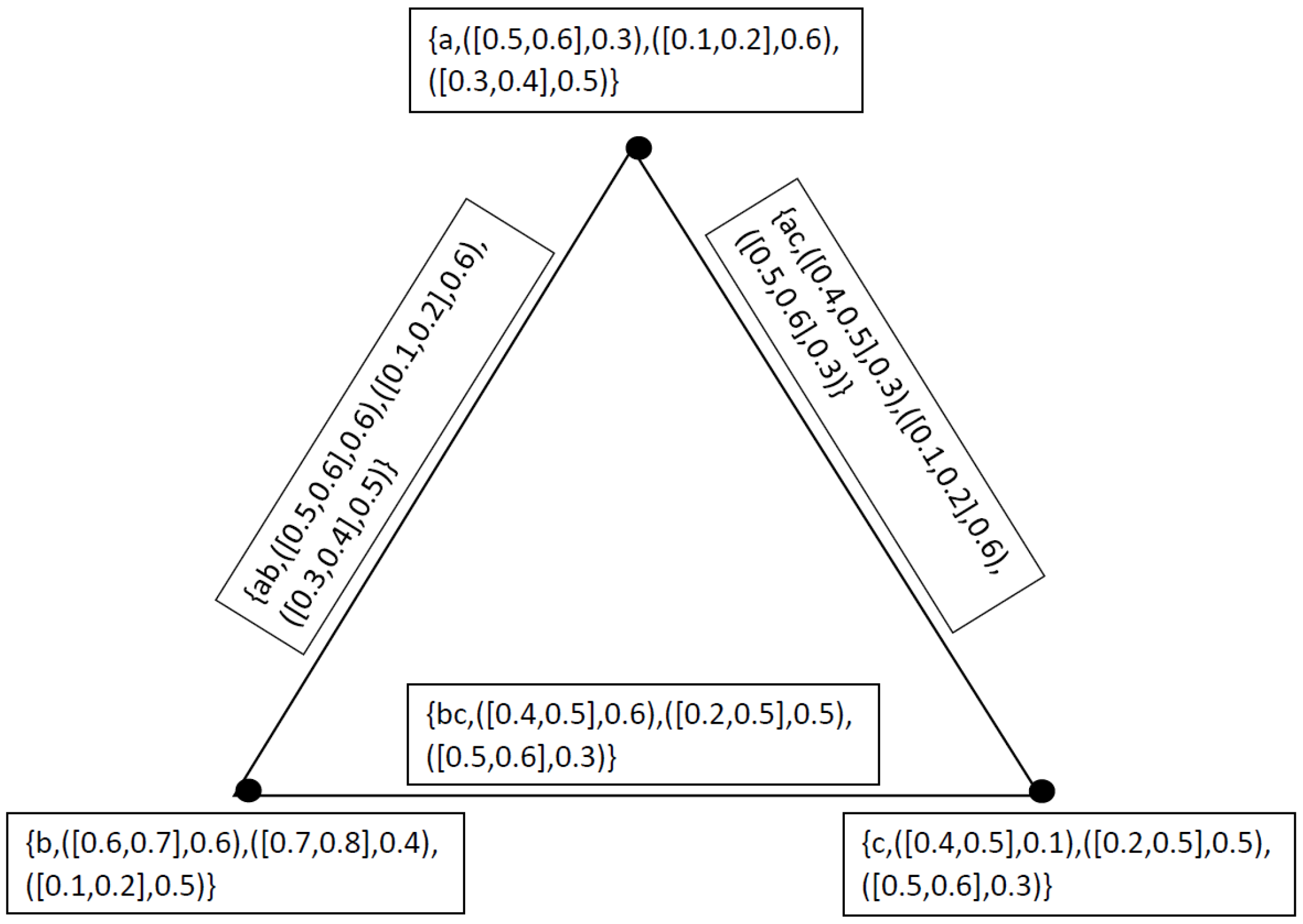
- (i)
- if
- (ii)
- if
- (iii)
- if , where is the set of all edges joining the vertices of and
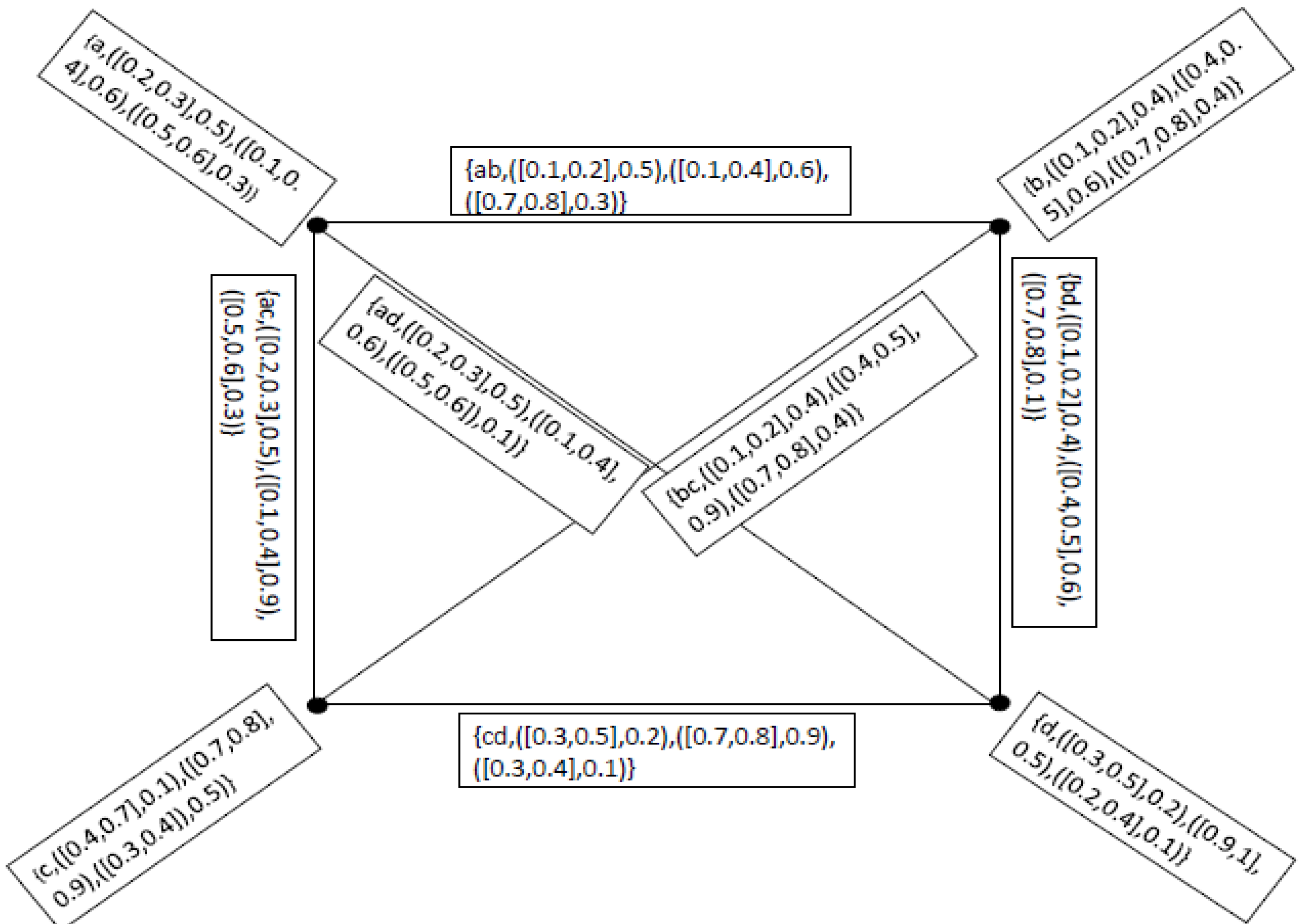
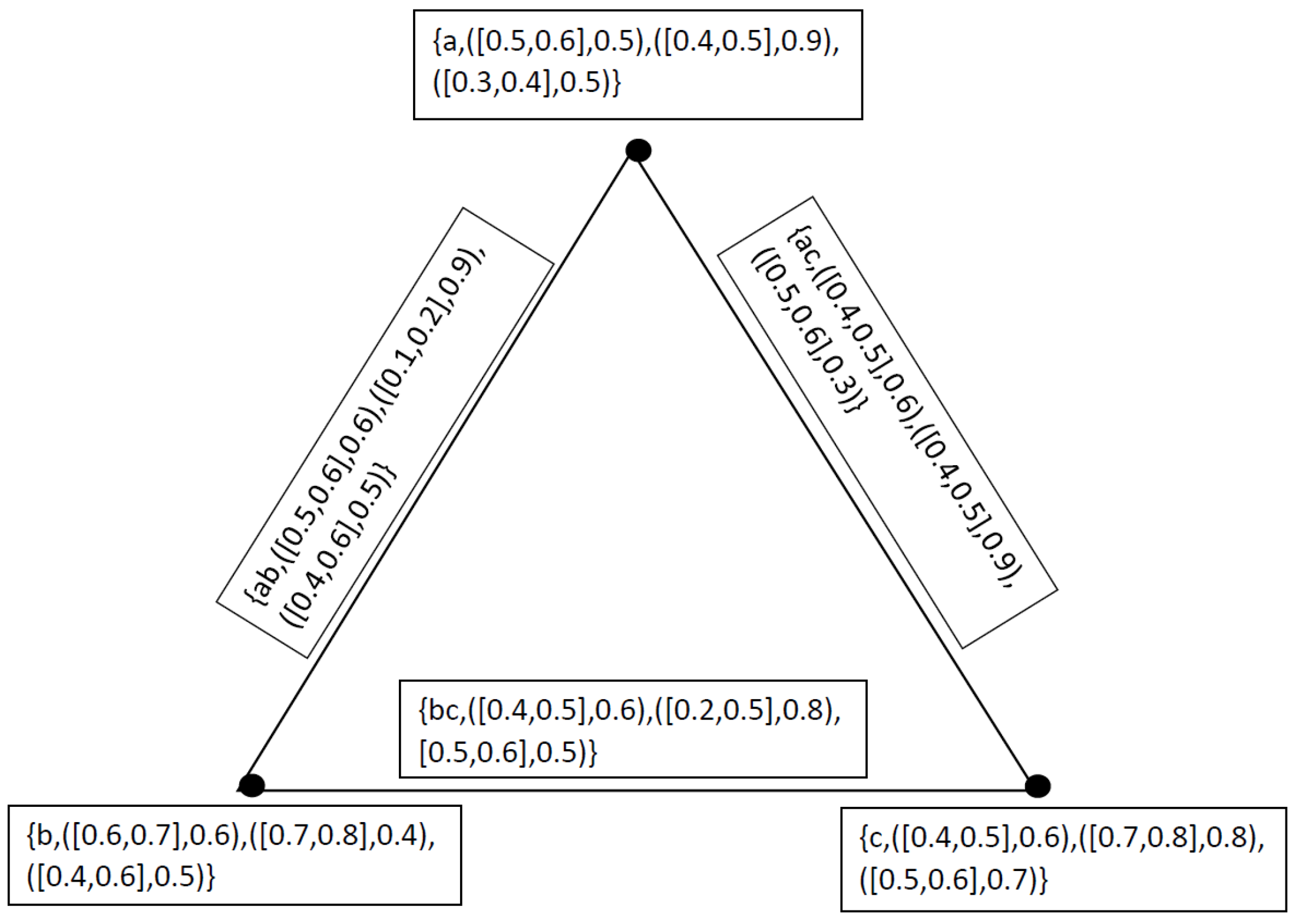
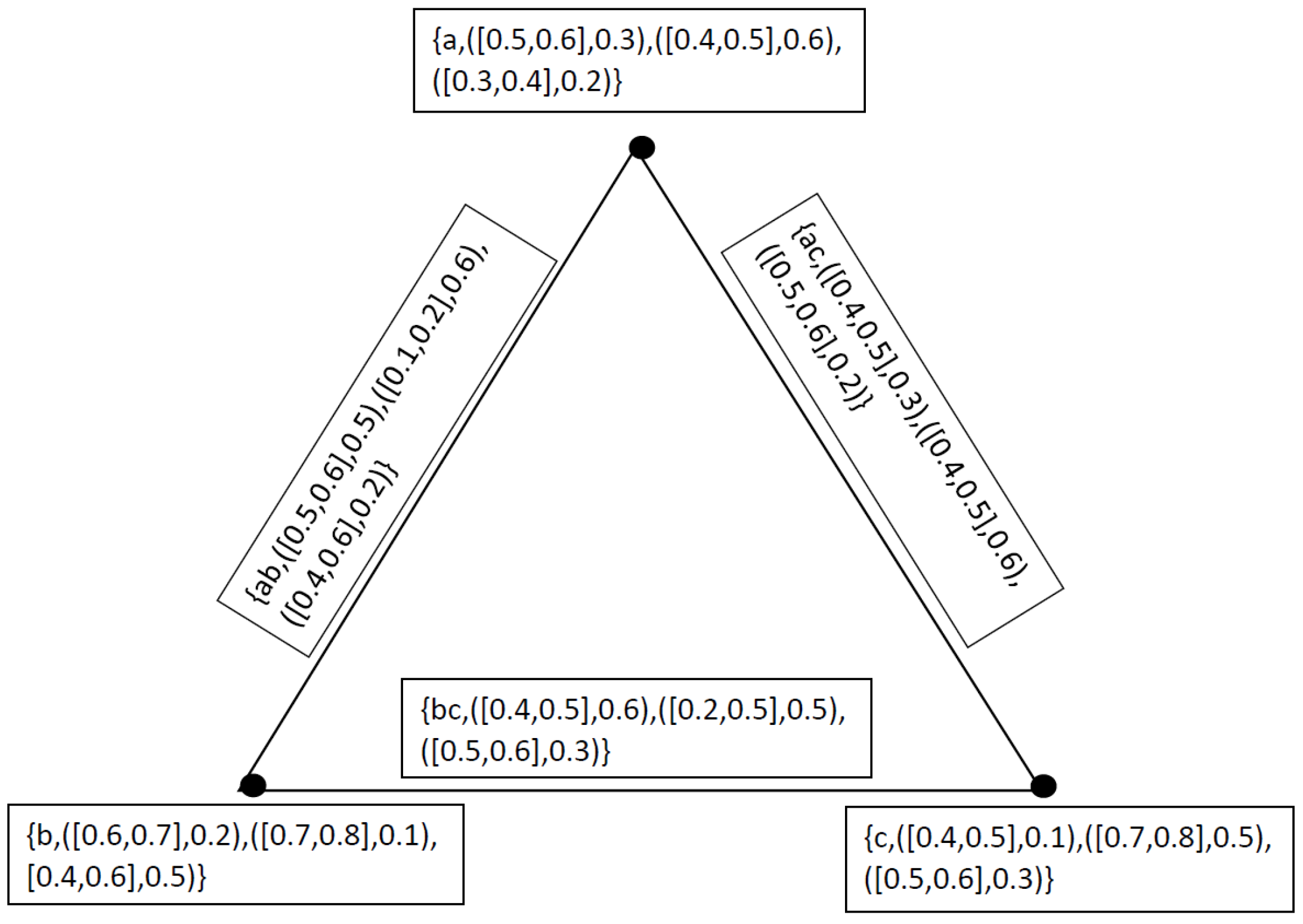
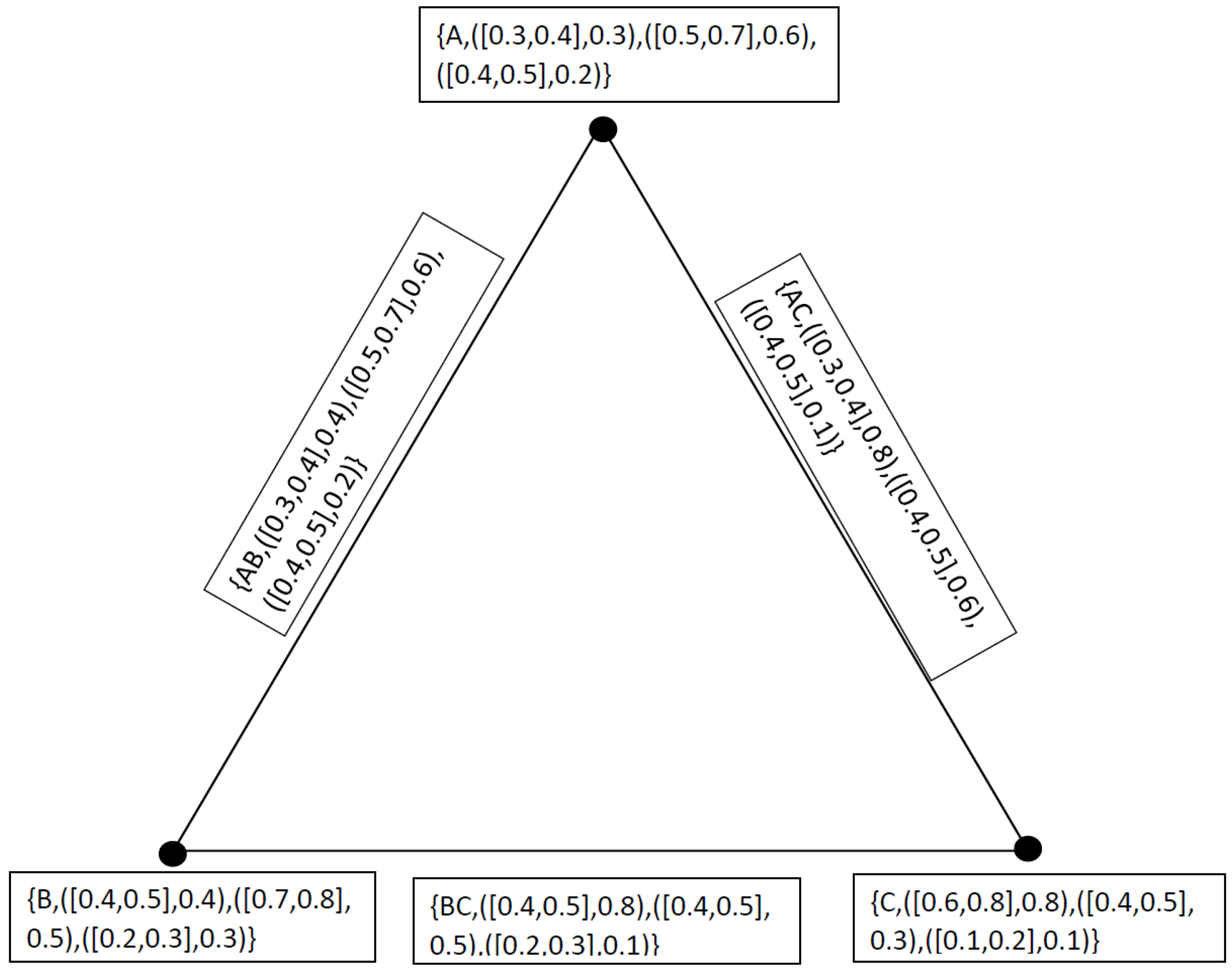
4. Applications
- 1.
- Technological Development degree of mechanization), (technical know-how), (product design
- 2.
- Quality of Human Resources ability of the worker), (willingness of the worker), (the environment under which he has to work
- 3.
- Availability of Finance advertisement campaign), (better working conditions to the workers), (up-keep of plant and machinery,
- 4.
- Managerial Talent devoted towards their profession), (Links with workers, customers and suppliers), (conceptual, human relations and technical skills
- 5.
- Government Policy Government Policy favorable conditions for saving), (investment), (flow of capital from one industrial sector to another
- 6.
- Natural Factors physical), (geographical(climatic exercise. As these factors affecting industrial productivity are inter-related and inter-dependent, it is a difficult task to evaluate the influence of each individual factor on the overall productivity of industrial units. The use of neutrosophic cubic graphs give us a more reliable information as under. Let we have a neutrosophic cubic set for the vertex set as under
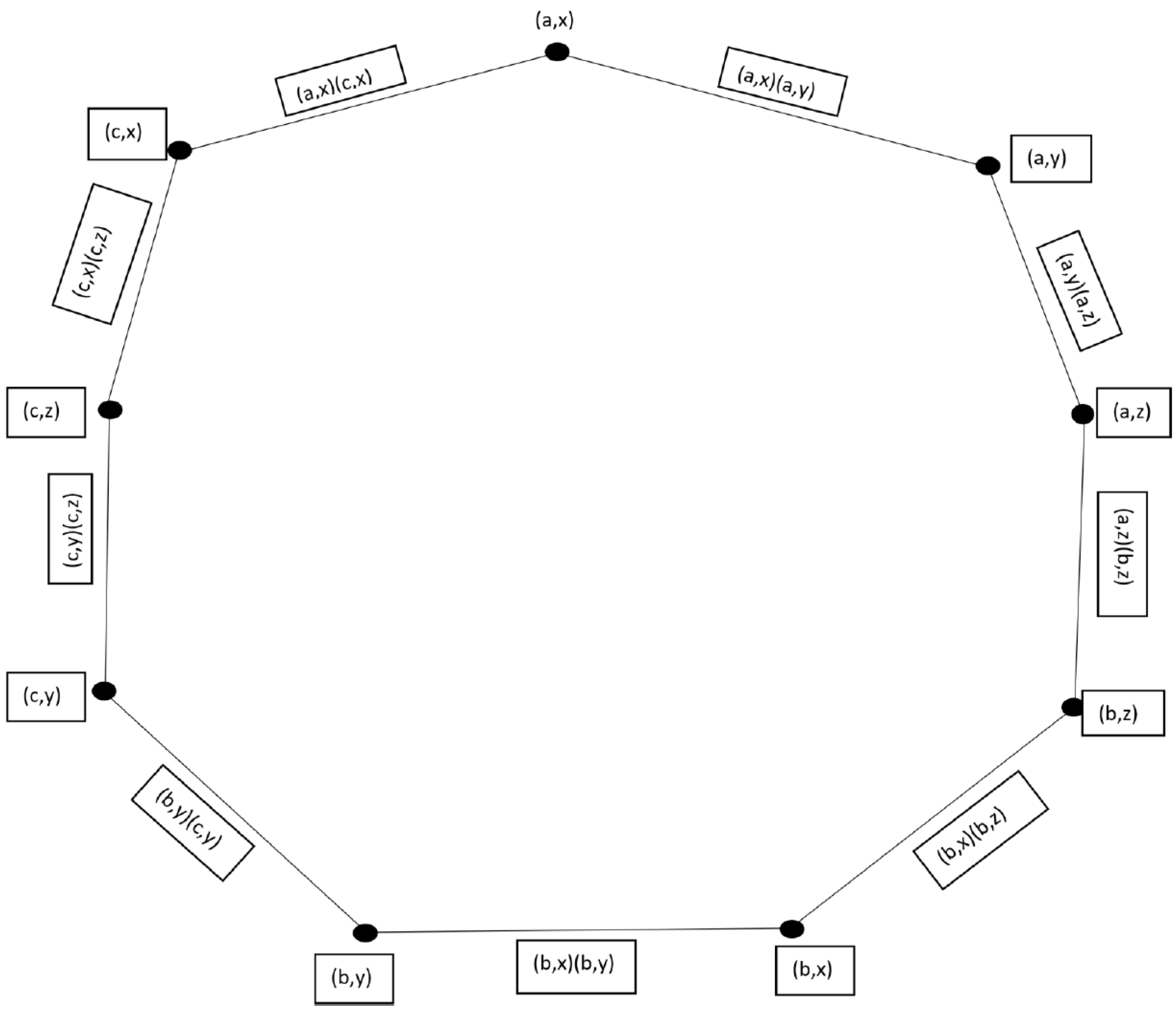
5. Comparison Analysis
6. Conclusions
Author Contributions
Conflicts of Interest
References
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar]
- Rosenfeld, A. Fuzzy Graphs, Fuzzy Sets and Their Applications; Academic Press: New York, NY, USA, 1975; pp. 77–95. [Google Scholar]
- Kauffman, A. Introduction a la Theorie des Sous-Emsembles Flous; Masson: Issy-les-Moulineaux, French, 1973; Volume 1. [Google Scholar]
- Bhattacharya, P. Some remarks on fuzzy graphs. Pattern Recognit. Lett. 1987, 6, 297–302. [Google Scholar]
- Akram, M.; Dudek, W.A. Interval-valued fuzzy graphs. Comput. Math. Appl. 2011, 61, 289–299. [Google Scholar]
- Akram, M. Interval-valued fuzzy line graphs. Neural Comput. Appl. 2012, 21, 145–150. [Google Scholar]
- Mordeson, J.N.; Nair, P.S. Fuzzy Graphs and Fuzzy Hypergraphs; Springer: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Sunitha, M.S.; Sameena, K. Characterization of g-self centered fuzzy graphs. J. Fuzzy Math. 2008, 16, 787–791. [Google Scholar]
- Borzooei, R.A.; Rashmanlou, H. Cayley interval-valued fuzzy threshold graphs, U.P.B. Sci. Bull. Ser. A 2016, 78, 83–94. [Google Scholar]
- Pal, M.; Samanta, S.; Rashmanlou, H. Some results on interval-valued fuzzy graphs. Int. J. Comput. Sci. Electron. Eng. 2015, 3, 2320–4028. [Google Scholar]
- Pramanik, T.; Pal, M.; Mondal, S. Inteval-valued fuzzy threshold graph. Pac. Sci. Rev. A Nat. Sci. Eng. 2016, 18, 66–71. [Google Scholar]
- Pramanik, T.; Samanta, S.; Pal, M. Interval-valued fuzzy planar graphs. Int. J. Mach. Learn. Cybern. 2016, 7, 653–664. [Google Scholar]
- Atanassov, K.T. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar]
- Atanassov, K.T. On Intuitionistic Fuzzy Graphs and Intuitionistic Fuzzy Relations. In Proceedings of the VI IFSA World Congress, Sao Paulo, Brazil, 22–28 July 1995; Volume 1, pp. 551–554. [Google Scholar]
- Atanassov, K.T.; Shannon, A. On a generalization of intuitionistic fuzzy graphs. Notes Intuit. Fuzzy Sets 2006, 12, 24–29. [Google Scholar]
- Karunambigai, M.G.; Parvathi, R. Intuitionistic Fuzzy Graphs. In Computational Intelligence, Theory and Applications; Springer: Berlin/Heidelberg, Germany, 2006; Volume 20, pp. 139–150. [Google Scholar]
- Shannon, A.; Atanassov, K.T. A first step to a theory of the intutionistic fuzzy graphs. In Proceedings of the 1st Workshop on Fuzzy Based Expert Systems, Sofia, Bulgaria, 26–29 June 1994; pp. 59–61. [Google Scholar]
- Mishra, S.N.; Rashmanlou, H.; Pal, A. Coherent category of interval-valued intuitionistic fuzzy graphs. J. Mult. Val. Log. Soft Comput. 2017, 29, 355–372. [Google Scholar]
- Parvathi, R.; Karunambigai, M.G.; Atanassov, K. Operations on Intuitionistic Fuzzy Graphs. In Proceedings of the IEEE International Conference on Fuzzy Systems, Jeju Island, Korea, 20–24 August 2009; pp. 1396–1401. [Google Scholar]
- Sahoo, S.; Pal, M. Product of intiutionistic fuzzy graphs and degree. J. Intell. Fuzzy Syst. 2017, 32, 1059–1067. [Google Scholar]
- Akram, M.; Davvaz, B. Strong intuitionistic fuzzy graphs. Filomat 2012, 26, 177–196. [Google Scholar]
- Zhang, W.R. Bipolar Fuzzy Sets and Relations: A Computational Framework for Coginitive Modeling and Multiagent Decision Analysis. In Proceedings of the IEEE Industrial Fuzzy Control and Intelligent Systems Conference, and the NASA Joint Technology Workshop on Neural Networks and Fuzzy Logic, Fuzzy Information Processing Society Biannual Conference, San Antonio, TX, USA, 18–21 December 1994; pp. 305–309. [Google Scholar]
- Akram, M. Bipolar fuzzy graphs. Inf. Sci. 2011, 181, 5548–5564. [Google Scholar]
- Akram, M. Bipolar fuzzy graphs with applications. Knowl. Based Syst. 2013, 39, 1–8. [Google Scholar]
- Akram, M.; Karunambigai, M.G. Metric in bipolar fuzzy graphs. World Appl. Sci. J. 2012, 14, 1920–1927. [Google Scholar]
- Smarandache, F. A Unifying Field in Logics: Neutrosophic Logic. Neutrosophy, Neutrosophic Set, Neutrosophic Probability; American Research Press: Rehoboth, NM, USA, 1999. [Google Scholar]
- Smarandache, F. Neutrosophic set-a generalization of the intuitionistic fuzzy set. Int. J. Pure Appl. Math. 2005, 24, 287–297. [Google Scholar]
- Wang, H.; Smarandache, F.; Zhang, Y.Q.; Sunderraman, R. Interval Neutrosophic Sets and Logic: Theory nd Applications in Computing; Hexis: phoenix, AZ, USA, 2005. [Google Scholar]
- Kandasamy, W.B.V.; Ilanthenral, K.; Smarandache, F. Neutrosophic Graphs: A New Dimension to Graph Theory; EuropaNova ASBL: Bruxelles, Belgium, 2015. [Google Scholar]
- Akram, M.; Rafique, S.; Davvaz, B. New concepts in neutrosophic graphs with application. J. Appl. Math. Comput. 2018, 57, 279–302. [Google Scholar]
- Akram, M.; Nasir, M. Concepts of Interval-Valued Neutrosophic Graphs. Int. J. Algebra Stat. 2017, 6, 22–41. [Google Scholar]
- Akram, M. Single-valued neutrosophic planar graphs. Int. J. Algebra Stat. 2016, 5, 157–167. [Google Scholar]
- Akram, M.; Shahzadi, S. Neutrosophic soft graphs with application. J. Intell. Fuzzy Syst. 2017, 32, 841–858. [Google Scholar]
- Jun, Y.B.; Kim, C.S.; Yang, K.O. Cubic Sets. Ann. Fuzzy Math. Inf. 2012, 4, 83–98. [Google Scholar]
- Jun, Y.B.; Kim, C.S.; Kang, M.S. Cubic subalgebras and ideals of BCK/BCI-algebras. Far East J. Math. Sci. 2010, 44, 239–250. [Google Scholar]
- Jun, Y.B.; Lee, K.J.; Kang, M.S. Cubic structures applied to ideals of BCI-algebras. Comput. Math. Appl. 2011, 62, 3334–3342. [Google Scholar]
- Kang, J.G.; Kim, C.S. Mappings of cubic sets. Commun. Commun. Korean Math. Soc. 2016, 31, 423–431. [Google Scholar]
- Rashid, S.; Yaqoob, N.; Akram, M.; Gulistan, M. Cubic Graphs with Application. Int. J. Anal. Appl. 2018, in press. [Google Scholar]
- Jun, Y.B.; Smarandache, F.; Kim, C.S. Neutrosophic cubic sets. New Math. Nat. Comput. 2017, 13, 41–54. [Google Scholar]
- Jun, Y.B.; Smarandache, F.; Kim, C.S. P-union and P-intersection of neutrosophic cubic sets. Anal. Univ. Ovid. Constant. Seria Mat. 2017, 25, 99–115. [Google Scholar]
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gulistan, M.; Yaqoob, N.; Rashid, Z.; Smarandache, F.; Wahab, H.A. A Study on Neutrosophic Cubic Graphs with Real Life Applications in Industries. Symmetry 2018, 10, 203. https://doi.org/10.3390/sym10060203
Gulistan M, Yaqoob N, Rashid Z, Smarandache F, Wahab HA. A Study on Neutrosophic Cubic Graphs with Real Life Applications in Industries. Symmetry. 2018; 10(6):203. https://doi.org/10.3390/sym10060203
Chicago/Turabian StyleGulistan, Muhammad, Naveed Yaqoob, Zunaira Rashid, Florentin Smarandache, and Hafiz Abdul Wahab. 2018. "A Study on Neutrosophic Cubic Graphs with Real Life Applications in Industries" Symmetry 10, no. 6: 203. https://doi.org/10.3390/sym10060203
APA StyleGulistan, M., Yaqoob, N., Rashid, Z., Smarandache, F., & Wahab, H. A. (2018). A Study on Neutrosophic Cubic Graphs with Real Life Applications in Industries. Symmetry, 10(6), 203. https://doi.org/10.3390/sym10060203





