A Novel Approach for Evaluation of Projects Using an Interval–Valued Fuzzy Additive Ratio Assessment (ARAS) Method: A Case Study of Oil and Gas Well Drilling Projects

Abstract

The beginning of the 21st-century resulted in a more developed multi-attribute decision-making (MADM) tool and inspired new application areas that have resulted in discoveries in sustainable construction and building life cycle analysis. Construction and civil engineering stand for the central axis of a body consisting of a multidisciplinary (multi-dimensional) world with ties to disciplines constituting the surface, and with the disciplines, as a consequence, tied to each other. When dealing with multi-attribute decision-making problems generally multiple solutions exist, especially when there is a large number of attributes, and the concept of Pareto-optimality is inefficient. The symmetry and structural regularity are essential concepts in many natural and man-made objects and play a crucial role in the design, engineering, and development of the world. The complexity and risks inherent in projects along with different effective indicators for success and failure may contribute to the difficulties in performance evaluation. In such situations, increasing the importance of uncertainty is observed. This paper proposes a novel integrated tool to find a balance between sustainable development, environmental impact and human well-being, i.e., to find symmetry axe with respect to goals, risks, and constraints (attributes) to cope with the complicated problems. The concept of “optimal solution” as the maximum degree of implemented goals (attributes) is very important. The model is built using the most relevant variables cited in the reviewed project literature and integrates two methods: the Step-Wise Weight Assessment Ratio Analysis (SWARA) method and a novel interval-valued fuzzy extension of the Additive Ratio Assessment (ARAS) method. This model was used to solve real case study of oil and gas well drilling projects evaluation. Despite the importance of oil and gas well drilling projects, there is lack of literature that describes and evaluates performance in this field projects. On the other hand, no structured assessment methodology has been presented for these types of projects. Given the limited research on performance evaluation in oil & gas well-drilling projects, the research identifies a set of performance criteria and proposes an evaluation model using fuzzy Delphi method. An illustrative example shows that the proposed method is a useful and alternative decision-making method.

1. Introduction

Today, projects have a significant role to play in the success of any company and the integration of activities leading to new products or services can improve its performance [1]. That is why many companies consider the use of project management as a key strategy for their survival in a competitive environment as well as for increasing the possibility of value creation in their businesses [2]. A project is a temporary attempt to produce a unique product or service and project management refers to the application of knowledge, skills, tools and techniques to carry out all activities of the project [3] as well as, project is a complex effort involving interconnected activities, with the purpose of achieving an objective [1]. A project performance evaluation dealing with evaluating and rating all tasks [4] help Decision Makers (‘DM’) through determining the status of the project and its weaknesses and strengths [5] and will help establish benchmarks of high performance projects for cross-learning and identify inefficiencies of low performance projects for potential improvement [6]. Therefore, the importance of project performance evaluation is inevitable and has long been confirmed by practitioners and academics from a variety of functional disciplines [7]. As a result, several project performance evaluation approach has been advanced that MCDM models is one of them. In a business environment, evaluation of the “best” project can be done by a decision committee, instead of a single DMs. Different DMs can bring their own points of view and knowledge, which must be resolved within a framework of understanding and mutual concessions [8,9]. In such situations, MCDM can help in finding a sufficiently good solution from a collection of alternatives and can address complex problems that involve high uncertainty, conflicting objectives, different forms of information, multiple interests and different perspectives [8]. MCDM methods solve a complex problem by converting it into several small problems and as smaller processes are weighted and re-aggregated, a general picture of decision makers is provided [10]. Therefore, given the inherent complexity, risks and uncertainties of projects and the diversity of success/failure criteria, this paper aims to introduce a novel assessment framework for projects using Interval-Valued Fuzzy Additive Ratio Assessment as a recent MADM approach dealing with uncertainties in decision making. In many practical situations, there exists information which is incomplete and uncertain so that decision makers cannot easily express their judgments on the candidates with exact and crisp values. As well as there are many real-life complex problems that need to involve a wide domain of knowledge. Therefore, fuzzy sets provide generally more adequate description to model real-life decision problems than real numbers [11]. However, it became apparent that the fuzzy sets are not sufficient for uncertain MCDM. Therefore, Zadeh (1975) [12] and Gorzałczany (1987) [13] developed the concept of interval-valued fuzzy sets, whereas Atanassov (1986) [14] proposed intuitionistic fuzzy sets [15]. Consequently, interval-valued fuzzy sets allow us to achieve a better imagination from environmental ambiguity and uncertainty [16].

On the other hand, Over the past two decades, infrastructure projects as an important category of projects have accounted for 3.8% of global GDP and this number is expected to rise to 1.4% by 2030 [5]. These type of projects include general fields of energy, transport, water, communications and social infrastructure such as hospitals [17]. Infrastructure projects are defined as long-term, large-scale and difficult-to-implement projects that can hardly be valued; therefore, evaluation of these projects is a complex and specialized activity [17].

Further, oil and gas well drilling projects are of great importance among major infrastructure projects because of a large volume of investment and the economic benefits of their proper implementation [4,18]. Difficulty in predicting operational costs due to inherent uncertainties in the economic evaluation of oil and gas exploration and development projects [19], costs of renting drilling rigs as well as drilling services required by these projects [20] are major parameters resulting in the need for high-volume investments. Like any costly projects for the highest quality and lowest cost and time, oil and gas drilling projects require appropriate decision-making procedures to face upcoming challenges and achieve the desired level of productivity [21]. A fast, continuous, timely and data-based decision-making process can lead to improved productivity; particularly as today’s global demand for energy and environmental constraints have forced oil and gas projects to enhance their activities in terms of efficiency and effectiveness [18]. In addition, similar to other projects, oil and gas well-drilling projects deal with inherent uncertainties [22] that are generally arisen from environmental factors, organizational complexities, as well as changes, deviations and events occurring in a project [23]. Accordingly, oil and gas projects face various risks and complexities that make the decision-making process so difficult [18].

However, a brief review of the literature shows a limited portion of structured performance evaluation models in these field and though a variety of project evaluation approaches like multi-criteria decision-making (MCDM) methods have been applied in other project fields [6] but there is a necessity for structured assessment methodology in the field of oil and gas well drilling projects. Therefore, we choised oil and gas well drilling projects as a case study and tried to identify an initial list of performance criteria based on review of literature and propose an evaluation model using Delphi method.

According to the above discussion, the purpose of this study is to provide an interval-valued MADM-based framework as a novel approach for evaluating the performance of projects that oil and gas well drilling projects was considered in order to remove the limitations noted in this type of projects. To this end, a review on the literature is provided and an initial list of evaluation criteria is extracted. Due to the research limitations in this context, the Fuzzy Delphi technique and expert panels are used to develop a more complete list of effective criteria. Next, the identified criteria are weighted by the SWARA method and finally the Interval-Valued Fuzzy Additive Ratio Assessment is employed to assess and rank active projects of a certain company in the field of oil and gas drilling.

The structure of this paper will be as follows. Section 2 presents the literature review and the initial list of criteria. Section 3 describes the research methodology, while Section 4 provides an experimental example using data from a set of seven oil and gas well drilling projects from a subsidiary of the National Iranian Oil Company (NIOC) in Iran. Finally, Section 5 concludes the study.

2. Literature Review

Decision making in a project context is a complex undertaking. The term complexity is an increasingly important point of reference when we are trying to understand the managerial demands of modern projects in general, and of the various situations encountered in projects [24,25]. On the one hand, a project is a temporary and transient organization surrounded by inherent uncertainty [24,26]. When complexity becomes too great, the possibilities and interrelations become so fuzzy that the system has to be assisted by appropriate tools and skills. Consequently, managers facing complex project need access to a decision-making aid model based on relevant performance evaluation [24]. Therefore the project performance evaluation is inevitable and necessary issue. So far, several decsion approach has been used to help project evaluation such as economic models, mathematical programming, artificial intelligence optimization methods, integrated models, data envelopment analysis (DEA) method, integrated the balanced scorecard (BSC) approach and MCDM models [27]. among these, MCDM models can help in finding a sufficiently good solution from a collection of alternatives and can address complex problems that involve high uncertainty, conflicting objectives, different forms of information, multiple interests and different perspectives [8]. In measuring the overall performance of projects, MCDM models have been used to aggregate multiple performance measures under various application contexts [6] and incidentally are widely used for energy projects [8]. Analytical Hierarchy Process (AHP) [28,29], Analytic Network Process (ANP) [29,30], TOPSIS [31], DEMATEL [32], ELECTRE [33] and some hybrid methods [4,8,34] are number of MCDM methods applied to projects evaluation.

On the other hand in many projects in practical situations, there exists information which is incomplete and uncertain so that decision makers cannot easily express their judgments on the candidates with exact and crisp values. As well as there are many real-life complex problems that need to involve a wide domain of knowledge. These conditions in which decisions are based on obscure and unreliable information or lake of knowledge and personal preferences of the experts can create difficulties in the decision-making process. These difficulties can lead to deceptive and uncertain decisions. Therefore, solving decision-making problems existing in real life and modeling them in the form of multi-criteria decision-making problems is still considered as a challenging topic [35,36,37] and fuzzy sets provide generally more adequate description to model real-life decision problems than real numbers [11] and presented to fix these challenges and provided a basis for development of a variety of fuzzy decision-making models. The development of the fuzzy concept has led to the provision of models which have the flexibility to control and display uncertainty and low accuracy due to lack of knowledge of experts and inadequate data [38,39]. Many developments have been made to better address inadequate and inaccurate data [14,40]. Atanassov developed intuitive fuzzy sets [14]. These sets included the membership function, the non-membership function and the hesitancy function [41]. Zadeh [12] introduced a type-2 fuzzy set which allowed expressing the membership of the components in the form of a fuzzy set. Further, the type-n fuzzy numbers were defined [42] which were the generalized form of type-2 fuzzy numbers and allowed the membership of elements to be in the form of a type-(n − 1) fuzzy set. Since the concept of fuzzy numbers with interval values has been presented, there has been an increasing interest of researchers in this field [43] so that it was successfully used in numerous decision-making issues in conditions of uncertainty. Briefly, there are two approaches to classify studies in this area: (1) Content Approach; (2) Applied Approach [44]. In the content approach, two main steps are considered for decision-making issues: aggregation of opinions [45,46,47] and method exploitation. In the applied approach, researches on fuzzy numbers with interval values can be classified into five main domains [48]: (1) basic operators in fuzzy space with interval values [49]; (2) group decision [50,51]; (3) combining decision making with linguistic variables [52,53]; (4) matrix of judgment based on priority relations [54] and (5) development of the model of dual interval-valued fuzzy sets [55]. In recent years, researches have been a growing trend in two domains; decision making with linguistic variables and development of basic operators in fuzzy space with interval values.

Given that this paper aims to prioritize the projects, it is related to the method exploitation step. In this category of methods, it is tried to find priority relations in non-preferred alternatives so that a set of options is ranked based on them. So far, various decision-making methods have been combined with fuzzy numbers with interval values [56] including the VIKOR method [57,58], the TOPSIS method [59], the MULTIMOORA method [15] and the TODIM method [60]. In this paper, we tried to use Additive Ratio Assessment (ARAS) method combined with fuzzy numbers with interval values which is addressed when we introduce fuzzy numbers with interval values and combined method steps.

In addition to the performance evaluation methodology, it is also necessary to define the performance evaluation criteria for each project. Evaluation criteria are quantitative or qualitative variables that measure the performances and the impacts of the analyzed alternatives [61]. Through a literature review, this section also seeks to develop an initial list of criteria as inputs for the fuzzy Delphi process. As discussed before, despite the importance of oil and gas well drilling projects, there is a limited research available on performance evaluation of these projects, by using some measures of these criteria. Below provides further details of some relevant research studies:

Dachyar and Pratama (2014) evaluated the efficiency of oil and gas well drilling projects using the MACBETH method [22]. The author introduced criteria such as implementation methods, time, cost, quality, risk and safety. Ahari and Niaki (2014) used a neuro-fuzzy network to assess the quality of oil and gas well drilling projects for a contractor selection problem [4,21]. The authors defined three criteria of time, cost and quality as the basic assessment objectives. Also, a set of five parameters were introduced as inputs; (1) the cost compliance percentage with plans; (2) the time compliance percentage with plans; (3) the percentage of quality failure in all operational failures; (4) the number of HSE incidences; and (5) the number of quality failures without non-productive time. The quality of work measure was defined as the model output. The authors followed the size of drilled holes as an important factor in the work package plan. Exploring the design of drilling contracts, Osmundsen et al. (2006) suggested safety and associated risks from the important indicators of a drilling project [20]. In other study (2010), the authors examined new incentive schemes in offshore drilling contracts to improve project performance. They highlighted higher cost and its rapid growth in drilling activity, especially for renting and drilling services, as well as the need to carefully examine effective criteria in drilling projects [62]. Liu et al. (2013) considered risks in drilling projects [63]. They identified a set of 25 risk factors in six categories of natural risks, R & D risks, management risks, drilling risks, equipment risks and security risk and environmental factors.

In addition to the related studies referred to above, in general, three main traditional criteria have always been considered; including time, cost, and quality. A project would be succeeded when it is implemented at a reasonable cost and quality during a planned period time, and meet stakeholders’ satisfaction [1]. This traditional approach—referred as the “Iron Triangle” [4]—merely deals with the economic dimension of projects, while ignoring other major aspects [64]. Further, these criteria are less flexible in evaluating project performance [65]. In order to overcome limitations of the traditional criteria, academic researchers suggest a variety of criteria for project performance evaluations. Some examples are safety of the project site [66,67,68], geographic location of projects [69], environmental impacts [70] and satisfaction of community, client or customer [71,72]. Moreover, the quality and variety of materials and goods used in a project [73,74], number of active labor force and salaries [75,76,77] and experience and scientific levels of employees in the project [78,79] are amongst other effective measures of project performance. Risks and operational risks, in particular, are also important indicators that many researchers have taken into account [23,63,80]. In addition, research and development (R & D) and related costs are identified as influential parameters in improving project performance [81,82].

Considering the generality of criteria mentioned above, these can be used in oil and gas wells drilling projects. As noted, there is a limited research available on performance evaluation of oil and gas well-drilling projects, however, a list of initial criteria for evaluating oil and gas well drilling projects can be found by reviewing the literature (

Table 1. Primary Criteria from Literature Review.

No.	Description	Source
1	Cost spent for drilling	[1,4,21,22]
2	Types of drilled wells in terms of number of holes	[4,32]
3	Time of drilling operations	[1,4,21,22]
4	Number of accidents caused by non-compliance with safety regulation or environmental factors	[4,20,21,22,63,66,67,68,70]
5	Actual cost compliance percentage with plans	[4,21]
6	Number of operational experts working on the project	[74,75,76]
7	Scientific levels of drilling specialists working in the project	[79]
8	Experience of drilling specialists working in Project	[78,79]
9	Average salaries of employees in the project	[74,75,76,77]
10	Types of wells drilled in operational risk	[20,22,23,63,69,81]
11	Number of operational failures	[4,21,63]
12	Employer/Senior Manager Satisfaction	[4,21,71,72]
13	Quality of materials and goods	[1,63,73,74]
14	R & D expenditure	[63,81,82]

). Obviously, these criteria are precisely related to the drilling of oil and gas wells and do not include all the relevant criteria in the entire oil and gas industry. This list will be completed in Section 4 using the Fuzzy Delphi method.

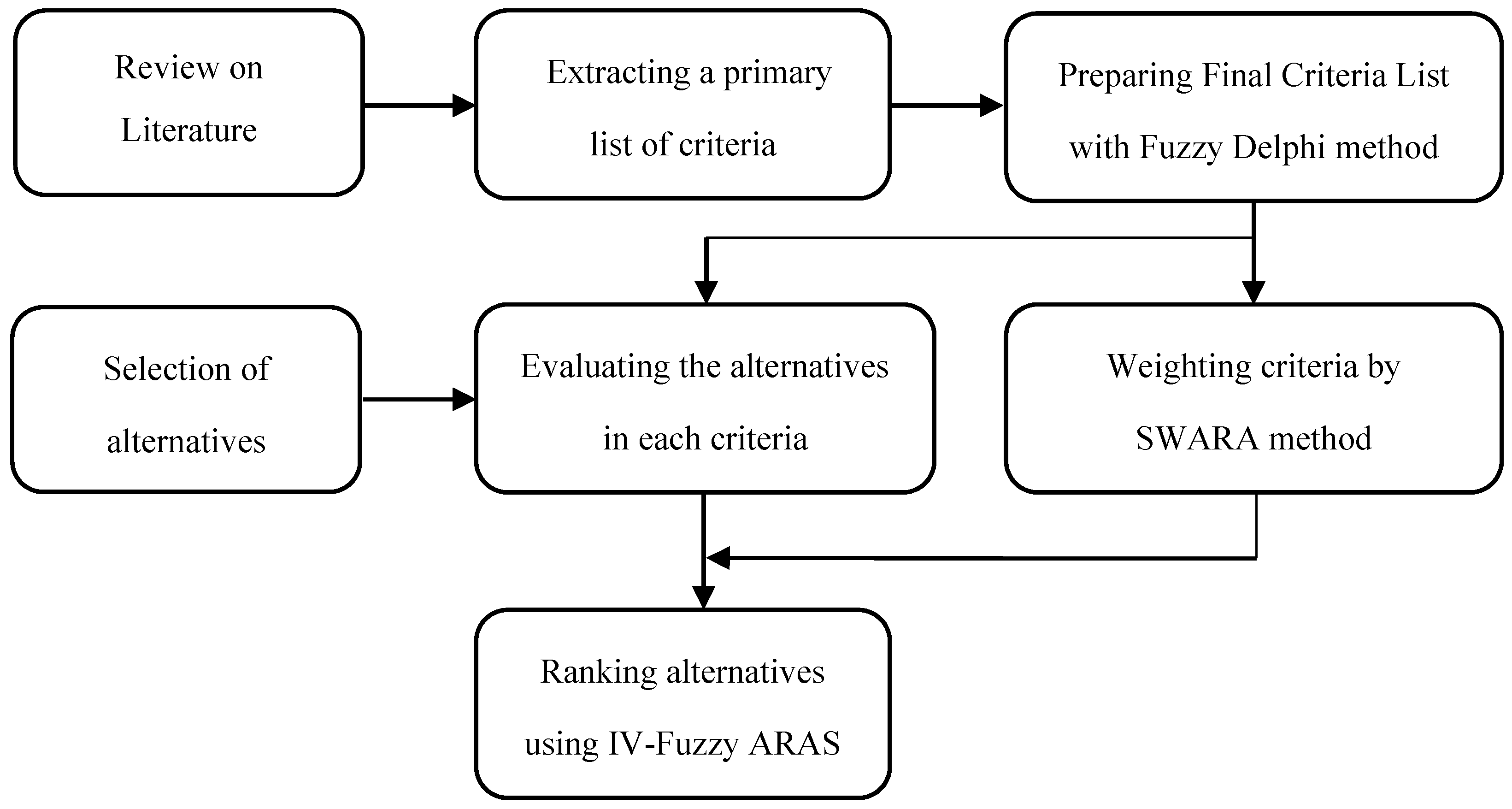
3. Research Methodology

First, a list of assessment criteria is derived from the literature available on the research subject. Then by using the Fuzzy Delphi method and expert opinions, the criteria are extended and the final list is obtained for oil and gas well-drilling proje cts (Table 4). The Step-Wise Weight Assessment Ratio Analysis (SWARA) method is employed to determine final criteria weights. According to the interval-values fuzzy Additive Ratio Assessment and the decision Table developed for a set of oil and gas projects, project performance is evaluated. Figure 1 presents the flowchart of the research.

3.1. Fuzzy Delphi Method

The traditional “Project DELPHI” was originally developed by Dalkey and Helmer (1963) to achieve the most reliable consensus among experts on a particular topic [83]; a theoretical consensus obtained through several rounds of extensive consultations through expert interviews [84]. The most important advantage of this method is to avoid direct confrontation of participants [85]. However, the Delphi method has been criticized for higher operational costs, lower degree of convergence and the possibility to remove some key ideas by organizers. Therefore, Murray et al. (1985) proposed the integration of the traditional Delphi method and fuzzy set theory to improve ambiguity and inconsistency in requirements [86,87]. A variety of techniques have been developed to determine metrics by using Delphi method. Here, based on the approach presented by Hsu and Yang (2000) and Kuo and Chen (2008), triangular fuzzy numbers are used to incorporate expert opinions and to implement the fuzzy Delphi technique. Maximum and minimum values obtained based on expert opinions are defined as two endpoints of triangular fuzzy numbers, while the geometric mean is expressed as the membership degree in triangular fuzzy numbers in order to avoid the portion of terminal values [86,88]. Kuo and Chen believed that this method enables decision-makers to achieve a better solution for the selection [86].

Further, the authors highlighted the simplicity and the lack of survey repetition, as well as the use of all expert opinions. Each fuzzy number (T_A) is defined as follows (1):

$$T_A=\left(L_A,M_A,U_A\right),L_A=min\left(X_{Ai}\right),U_A=max\left(X_{Ai}\right),M_A=\sqrt[n]{{{\displaystyle \prod }}_{i=1}^n{X}_{Ai}}$$

(1)

where, $X_{A_i}$ is the proposed value of the i-th decision-maker in terms of the critical factor A; (i = 1,2…). $L_A$, $U_A$ and $M_A$ represent the values of lower bound, upper bound and geometric mean for the critical factor A, respectively. In the next step, the Center of Area (COA) defuzzification process is performed using the model developed by Zheng and Teng (1993) [89]. The formula is presented as follows (2):

$$D{F}_k=\frac{\left(U_k-L_k\right)+\left(M_k-L_k\right)}{3}+L_k$$

(2)

where, the index k represents the number of criteria and $L_k$, $U_k$ and $M_k$ represent the values of lower bound, upper bound and geometric mean for the critical factor k.

The final step is to determine the threshold value for accepting or rejecting the criteria. To this end, the score of 0.7 is determined based on expert opinions. Finally, those criteria with a number less than the threshold value are removed from the list and the final list provides the necessary evaluation criteria.

3.2. SWARA Method

For a large number of multi-attribute decision-making problems, weighting indicators are included among the most important procedures in problem solution [90]. Accordingly, experts play a vital role in criteria evaluation and weighting and form inevitable part of the decision-making process. The Step-Wise Weight Assessment Ratio Analysis (SWARA) method newly proposed by Krešulienė et al. (2010) allows decision-makers to select, evaluate and weight the criteria [91]. The most important advantage of this approach is its potential for evaluating the accuracy of expert opinions about weights allocated by the process [91]. Further, expert consultations can yield more accurate results than other common methods of multi-criteria decision-making (MCDM) [92]. The main steps of determining the criteria weights based on the SWARA method are described below:

-

Step 1: Rank Criteria—First, the criteria determined by decision-makers are selected as the final criteria and then all the criteria are ranked in order of their importance. Accordingly, the most/least important criteria take the highest/lowest position of ranking.

-

Step 2: Determine Relative Importance for Criteria ($S_j$)—Now, the relative importance of each criterion is measured against the most important criterion. This value is represented by $S_j$.

-

Step 3: Calculate Coefficient Value of $K_j$—As a function of the relative importance for each criterion, the coefficient $K_j$ is determined using Equation (3).

$$K_j=S_j+1$$

(3)

-

Step 4: Calculate Initial Weights for Criteria—In this step, the initial weights of each criterion are calculated by Equation (4). Note that the initial weight for the first—i.e., the most important—criterion is generally considered equal to 1 ($q_1=1$).

$$q_j=\frac{q_{j-1}}{K_j}$$

(4)

-

Step 5: Calculate Final Normalized Weights—As the final step of SWARA, the final weights which is also known as the normalized weights are determined by Equation (5).

$$w_j=\frac{q_j}{{{\displaystyle \sum }}^{\text{}}{q}_j}$$

(5)

As mentioned before, SWARA is a newly established method for weighting which has been recently used by different studies [93].

3.3. Interval-Valued Fuzzy Additive Ratio Assessment

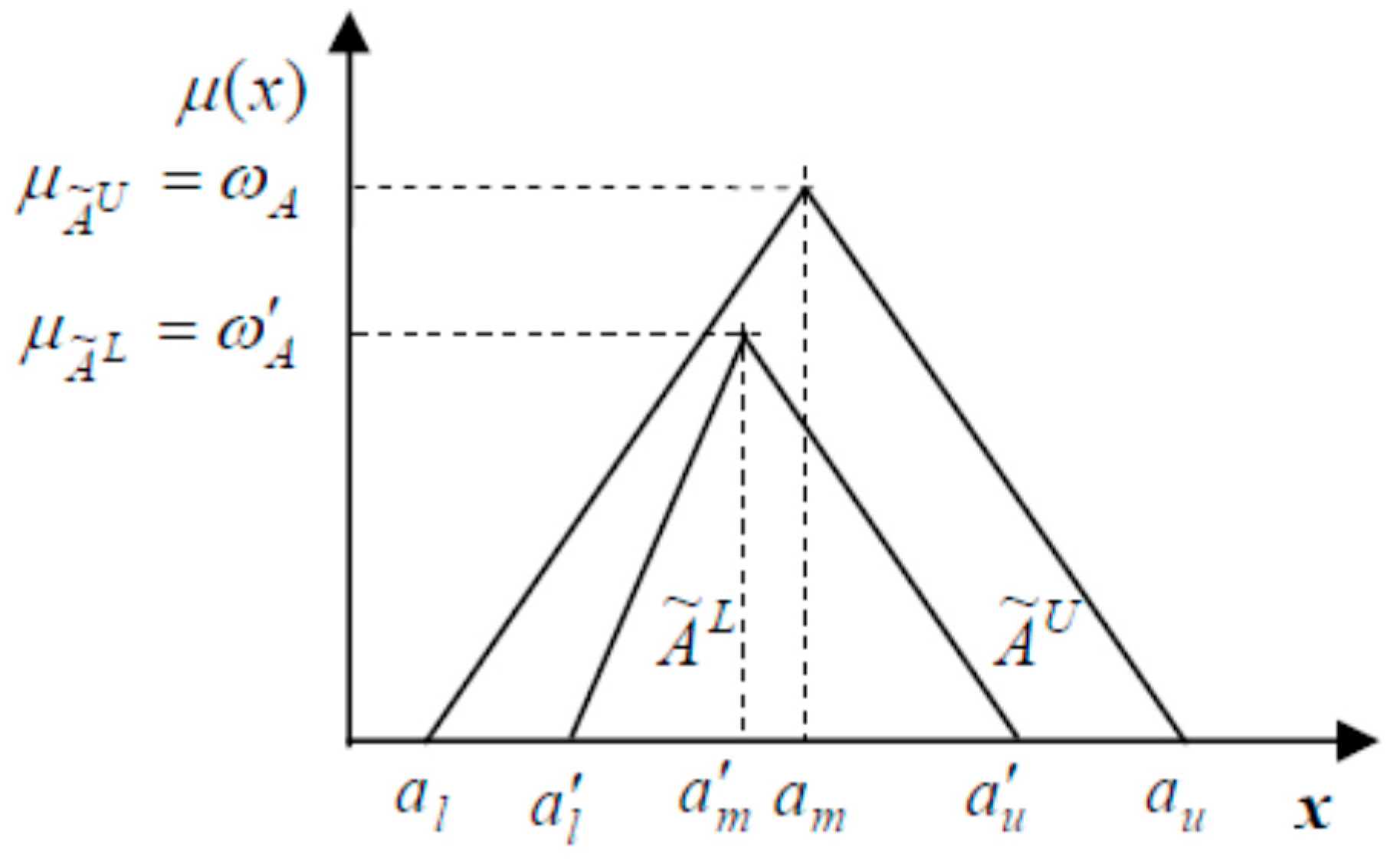
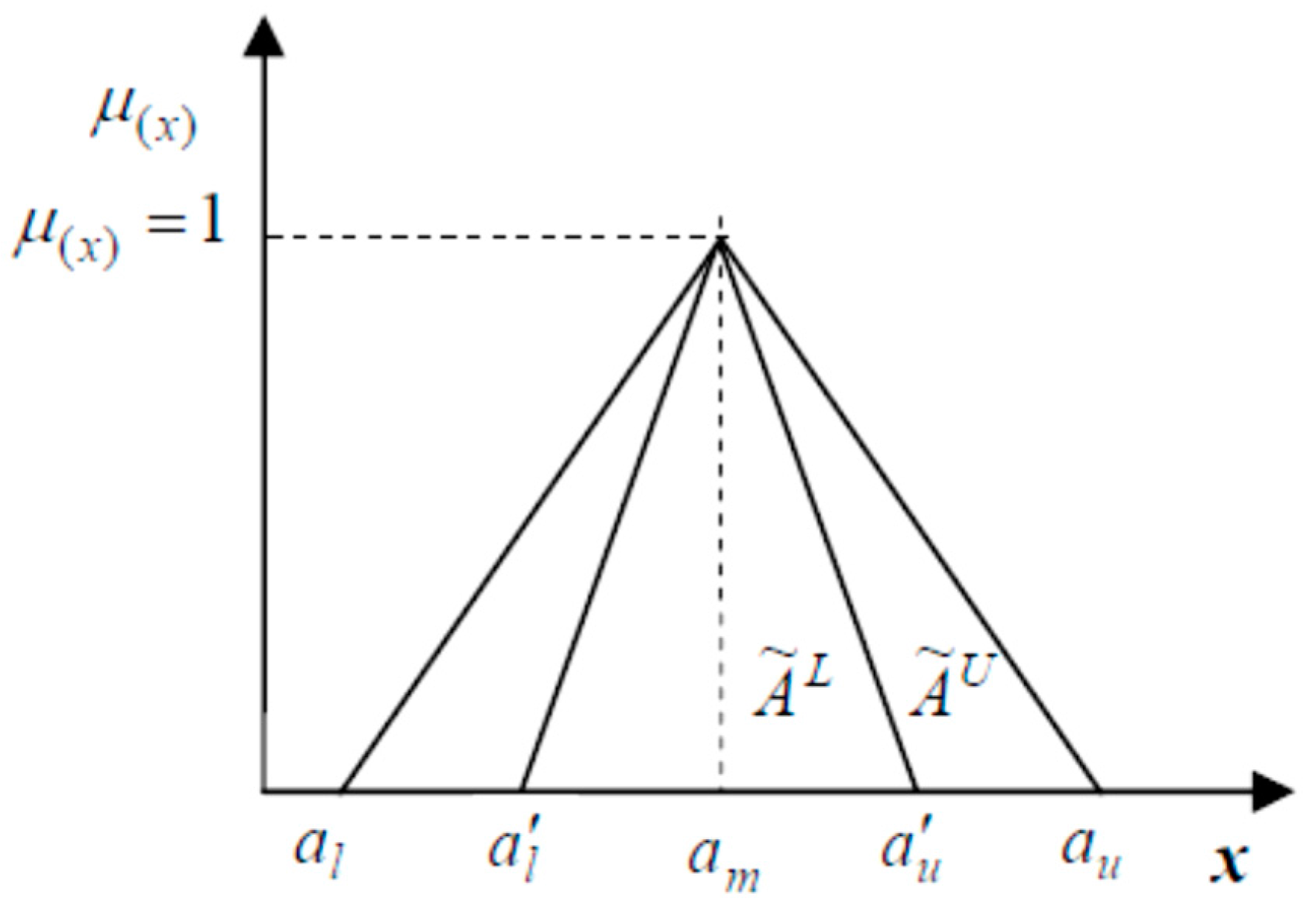
3.3.1. Generalized Fuzzy Numbers

A generalized fuzzy number à defined as (6),

$$\tilde{A}=\left(a,b,c,d;\omega \right),0\le a\le b\le c\le d\le 1,0\le \omega \le 1$$

(6)

It is a fuzzy subset of the real line Ʀ with the membership function (${\mu }_{\tilde{A}}$) which has the following features [94]:

• ${\mu }_{\tilde{A}}$ is a continuous mapping from Ʀ to the closed interval [0, 1].

  $$\forall x\in \left(-\infty ,a\right]\to {\mu }_{\tilde{A}}\left(x\right)=0$$
• ${\mu }_{\tilde{A}}\left(\mathrm{x}\right)$ is strictly increasing on [a, b].
• ${\mu }_{\tilde{A}}\left(\mathrm{x}\right)=\omega$ for all $x\in \left[b,c\right]$, where ω is a constant on [0, 1], $0\le \omega \le 1$.
• ${\mu }_{\tilde{A}}\left(\mathrm{x}\right)$ is strictly decreasing on [c, d].
• ${\mu }_{\tilde{A}}\left(\mathrm{x}\right)=0$ for all $x\in \left[d,+\infty \right]$.

If ${\mu }_{\tilde{A}}$ is linear on the intervals [a, b] and [c, d], then a generalized fuzzy number is called a generalized trapezoidal fuzzy number. Figure 2 shows a relationship between the generalized fuzzy number, $\tilde{B}$ and the normalized trapezoidal fuzzy number, Ã.

As seen from Figure 2, the normalized trapezoidal fuzzy numbers demonstrate certain cases of generalized fuzzy numbers, where ω = 1. Also, if b = c, then the trapezoidal fuzzy number becomes a triangular fuzzy number.

3.3.2. Interval-Valued Fuzzy Numbers

The interval-valued fuzzy numbers are special forms of generalized fuzzy numbers. Similar to generalized fuzzy numbers, these numbers can find a trapezoidal shape. Moreover, interval-valued triangular fuzzy numbers have a triangular shape. Figure 3 shows a graphical representation of an interval-valued triangular fuzzy number.

An interval-valued triangular fuzzy number can be defined as (7) [95]:

$$\tilde{A}=\left[{\tilde{A}}^L,{\tilde{A}}^U\right]=\left[\left(a_l^{\prime },a_m^{\prime },a_u^{\prime };{\omega }_A^{\prime }\right),\left(a_l,a_m,a_u;{\omega }_A\right)\right]$$

(7)

where Ã^L and Ã^U are the lower and upper triangular fuzzy numbers, Ã^L $\subset$ Ã^U and ${\mu }_{\tilde{A}}\left(\mathrm{x}\right)$ are their membership functions. However, ${\mu }_{{\tilde{A}}^L}\left(x\right)={\omega }_A^{\prime }$ and ${\mu }_{{\tilde{A}}^U}\left(x\right)={\omega }_A^{}$ denote the lower and upper membership functions.

Suppose $\tilde{A}=[{\tilde{A}}^L,{\tilde{A}}^U]$ and $\tilde{B}=[{\tilde{B}}^L,{\tilde{B}}^U]$ are two interval-valued triangular fuzzy numbers. Then, the basic arithmetic operations on these fuzzy numbers can be represented as (8) to (11):

$$\begin{gathered} \tilde{A}+\tilde{B}=\left[\left(a_l^{\prime }+b_l^{\prime },a_m^{\prime }+b_m^{\prime },a_u^{\prime }+b_u^{\prime };min\left({\omega }_A^{\prime },{\omega }_B^{\prime }\right)\right),\left(a_l^{}+b_l^{},a_m^{}+b_m^{},a_u^{} \\ +b_u^{};min\left({\omega }_A^{},{\omega }_B^{}\right)\right)\right] \end{gathered}$$

(8)

$$\begin{gathered} \tilde{A}-\tilde{B}=\left[\left(a_l^{\prime }-b_u^{\prime },a_m^{\prime }-b_m^{\prime },a_u^{\prime }-b_l^{\prime };min\left({\omega }_A^{\prime },{\omega }_B^{\prime }\right)\right),\left(a_l^{}-b_u^{},a_m^{}-b_m^{},a_u^{} \\ -b_l^{};min\left({\omega }_A^{},{\omega }_B^{}\right)\right)\right] \end{gathered}$$

(9)

$$\begin{gathered} \tilde{A}\times \tilde{B}=\left[\left(a_l^{\prime }\times b_l^{\prime },a_m^{\prime }\times b_m^{\prime },a_u^{\prime }\times b_u^{\prime };min\left({\omega }_A^{\prime },{\omega }_B^{\prime }\right)\right),\left(a_l^{}\times b_l^{},a_m^{}\times b_m^{},a_u^{} \\ \times b_u^{};min\left({\omega }_A^{},{\omega }_B^{}\right)\right)\right] \end{gathered}$$

(10)

$$\begin{gathered} \tilde{A}÷\tilde{B}=\left[\left(a_l^{\prime }÷b_u^{\prime },a_m^{\prime }÷b_m^{\prime },a_u^{\prime }÷b_l^{\prime };min\left({\omega }_A^{\prime },{\omega }_B^{\prime }\right)\right),\left(a_l^{}÷b_u^{},a_m^{}÷b_m^{},a_u^{} \\ ÷b_l^{};min\left({\omega }_A^{},{\omega }_B^{}\right)\right)\right] \end{gathered}$$

(11)

Figure 4 shows a certain case of generalized interval-valued fuzzy numbers, normalized with the same mode $\left(a_m^{\prime }=a_m^{}\right)$ and it can be represented as (12)

$$\tilde{A}=\left[{\tilde{A}}^L,{\tilde{A}}^U\right]=\left[\left(a_l,a_l^{\prime }\right),a_m,\left(a_u^{\prime },a_u\right)\right]$$

(12)

Suppose $\tilde{A}=\left[{\tilde{A}}^L,{\tilde{A}}^U\right]=\left[\left(a_l,a_l^{\prime }\right),a_m,\left(a_u^{\prime },a_u\right)\right]$ and $\tilde{B}=\left[{\tilde{B}}^L,{\tilde{B}}^U\right]=\left[\left(b_l,b_l^{\prime }\right),b_m,\left(b_u^{\prime },b_u\right)\right]$ denote two normalized interval-valued triangular fuzzy numbers with the same mode. Then, the basic arithmetic operations on these fuzzy numbers can be defined as (13) to (16) [96]:

$$\tilde{A}+\tilde{B}=\left[\left(a_l+b_l,a_l^{\prime }+b_l^{\prime }\right),a_m+b_m,\left(a_u^{\prime }+b_u^{\prime },a_u+b_u\right)\right]$$

(13)

$$\tilde{A}-\tilde{B}=\left[\left(a_l-b_u,a_l^{\prime }-b_u^{\prime }\right),a_m-b_m,\left(a_u^{\prime }-b_l^{\prime },a_u-b_l\right)\right]$$

(14)

$$\tilde{A}\times \tilde{B}=\left[\left(a_l\times b_l,a_l^{\prime }\times b_l^{\prime }\right),a_m\times b_m,\left(a_u^{\prime }\times b_u^{\prime },a_u\times b_u\right)\right]$$

(15)

$$\tilde{A}÷\tilde{B}=\left[\left(a_l÷b_u,a_l^{\prime }÷b_u^{\prime }\right),a_m÷b_m,\left(a_u^{\prime }÷b_l^{\prime },a_u÷b_l\right)\right]$$

(16)

In addition, the following unary operation defined on interval-valued triangular fuzzy numbers is of great importance. It is denoted as (17):

$$\frac{1}{k}\times \tilde{A}=\left[\left(\frac{1}{k}\times a_l,\frac{1}{k}\times a_l^{\prime }\right),\frac{1}{k}\times a_m,\left(\frac{1}{k}\times a_u^{\prime },\frac{1}{k}\times a_u\right)\right]$$

(17)

3.3.3. Linguistic Variables

The linguistic variables refer to variables whose values correspond to words or sentences in a natural or artificial language. A linguistic variable has a practical potential for dealing with many real-world decision-making problems, which are usually complex and relatively uncertain. A wide range of research studies have reported different linguistic variables with triangular fuzzy numbers [97,98,99]. Also, the literature provides linguistic variables based on the use of interval-valued fuzzy numbers. Wei and Chen (2009), for example, developed a scale of nine level linguistic terms using interval-valued trapezoidal fuzzy numbers [100]. Ashtiani et al. (2009) presented a seven-level linguistic terms scale based on interval-valued triangular fuzzy numbers.

Table 2. Linguistic variables for the weights of criteria.

Linguistic Variables	Triangular Fuzzy Number	Interval-Valued Triangular Fuzzy Number
Very low (VL)	(0.0, 0.0, 0.1)	[(0.00, 0.00), 0.0, (0.10, 0.15)]
Low (L)	(0.0, 0.1, 0.3)	[(0.00, 0.50), 0.1, (0.25, 0.35)]
Medium low (ML)	(0.1, 0.3, 0.5)	[(0.00, 0.15), 0.3, (0.45, 0.55)]
Medium (M)	(0.3, 0.5, 0.7)	[(0.25, 0.35), 0.5, (0.65, 0.75)]
Medium high (MH)	(0.5, 0.7, 0.9)	[(0.45, 0.55), 0.7, (0.80, 0.95)]
High (H)	(0.7, 0.7, 1.0)	[(0.55, 0.75), 0.9, (0.95, 1.00)]
Very high (VH)	(0.9, 1.0, 1.0)	[(0.85, 0.95), 1.0, (1.00, 1.00)]

and

Table 3. Linguistic variables for the performance ratings.

Linguistic Variables	Triangular Fuzzy Number	Interval-Valued Triangular Fuzzy Number
Very poor (VP)	(0.0, 0.0, 0.1)	[(0.00, 0.00), 0.0, (0.10, 0.15)]
Poor (P)	(0.0, 0.1, 0.3)	[(0.00, 0.50), 0.1, (0.25, 0.35)]
Medium poor (MP)	(0.1, 0.3, 0.5)	[(0.00, 0.15), 0.3, (0.45, 0.55)]
Fair (F)	(0.3, 0.5, 0.7)	[(0.25, 0.35), 0.5, (0.65, 0.75)]
Medium good (MG)	(0.5, 0.7, 0.9)	[(0.45, 0.55), 0.7, (0.80, 0.95)]
Good (G)	(0.7, 0.7, 1.0)	[(0.55, 0.75), 0.9, (0.95, 1.00)]
Very good (VG)	(0.9, 1.0, 1.0)	[(0.85, 0.95), 1.0, (1.00, 1.00)]

show the linguistic variables for the weights of criteria and performance ratings, based on the use of triangular fuzzy numbers and interval-valued triangular fuzzy numbers [57].

Since interval-valued fuzzy numbers are more complex than ordinary fuzzy numbers, the transformation of the ordinary fuzzy numbers into the corresponding interval-valued fuzzy numbers can raise some advantages. To transform their weights and performance ratings, the following equations are given:

$$l=\underset{k}{min}\left(l^k\right)$$

(18)

$$l^{\prime }={\left({\displaystyle \prod }_{k=1}^K{l}^k\right)}^{\frac{1}{K}}$$

(19)

$$m={\left({\displaystyle \prod }_{k=1}^K{m}^k\right)}^{\frac{1}{K}}$$

(20)

$$u^{\prime }={\left({\displaystyle \prod }_{k=1}^K{u}^k\right)}^{\frac{1}{K}}$$

(21)

$$u=\underset{k}{max}\left(u^k\right)$$

(22)

$\tilde{x}=\left[\left(l,l^{\prime }\right),m,\left(u^{\prime },u\right)\right]$ is the corresponding interval-valued triangular fuzzy number, while ${\tilde{x}}^k=\left(l^k,m^k,u^k\right)$ is the triangular fuzzy number obtained on the basis of opinion of kth decision maker. The parameters l and u denote the smallest and the greatest performance ratings among all stakeholders, respectively; which reflect the extreme attitudes provided by the experts. Unlike these parameters, other parameters of the interval-valued triangular fuzzy number reflect the expert opinions much more effectively. The reason is that these numbers are obtained as the geometric mean of attitudes from all experts.

3.3.4. Defuzzification of Interval-Valued Triangular Fuzzy Numbers

Since the results of arithmetic operations will be fuzzy numbers, they can be transformed into non-fuzzy numbers in order to rank and compare alternatives. Different procedures have been proposed for ranking fuzzy numbers and for their defuzzification but these procedures concern mainly the trapezoidal or triangular fuzzy numbers. By small changes, however, the same procedures can be used for the defuzzification of interval-valued triangular fuzzy numbers. Equations (23) and (24) are two general defuzzification equations for triangular fuzzy numbers (λ is a coefficient on [0, 1]):

$$gm(\tilde{A})=\frac{1}{2}\left[\left(1-\lambda \right)l+m+\lambda u\right]$$

(23)

$$gm(\tilde{A})=\frac{1+m+u}{3}$$

(24)

Moreover, (25) and (26) are proposed for defuzzification of interval-valued triangular fuzzy numbers:

$$gm(\tilde{B})=\frac{l+l^{\prime }+m+u^{\prime }+u}{5}$$

(25)

$$gm(\tilde{B})=\frac{\left(1-\lambda \right)l+\lambda l^{\prime }+m+\lambda u^{\text{'}}+\left(1-\lambda \right)u}{5}$$

(26)

where $\tilde{A}$ represents ordinary triangular fuzzy numbers, whereas $\tilde{B}$ presents interval-valued fuzzy numbers. λ is a coefficient on [0, 1]. Equation (25) is a simple extension of (24), providing an effective way for the defuzzification of known interval-valued fuzzy numbers, represented as the Best Non-Fuzzy Performance (BNP). In contrast, Equation (25) is relatively more complex but it has some advantages. For instance, varying the coefficient λ makes a greater importance to the parameters $l^{\prime }$ and $u^{\prime }$ against l and u and vice versa.

3.3.5. Additive Ratio Assessment (ARAS) Method

As a relatively new tool for MCDM, the ARAS method has received significant interested recently, still based on the theory that complex phenomena of the world could be accurately perceived trough simple relative comparisons [101,102,103]. The ARAS method uses the concept of optimality degree to find a ranking. It is the sum of normalized weighted values of the criteria with respect to each alternative divided by the sum of normalized weighted values of the best alternative.

- Step 1: First, a decision matrix is assembled as $m\times n$, where m denotes alternatives and n denotes criteria.

$$X=\left[\begin{array}{ccccc}{x}_{01}& \dots & x_{0j}& \dots & x_{0n}\\ ⋮& \ddots & ⋮& \ddots & ⋮\\ x_{i1}& \dots & x_{ij}& \dots & x_{in}\\ ⋮& \ddots & ⋮& \ddots & ⋮\\ x_{m1}& \dots & x_{mj}& \dots & x_{mn}\end{array}\right];i=\overline{0,m};j=\overline{1,n}$$

(27)

$x_{ij}$ is the performance measure of the i-th alternative on the j-th criterion. Also, $x_{0j}$ shows the optimum value for the j-th criterion. If the optimum value of the variable j is undetermined, then it can be determined as follows:

$$\begin{gathered} \mathrm{when}\underset{i}{max}{x}_{ij}\mathrm{is}\mathrm{optimal},x_{0j}=\underset{i}{max}{x}_{ij} \\ \mathrm{when}\underset{i}{min}{x}_{ij}^{*}\mathrm{is}\mathrm{optimal},x_{0j}=\underset{i}{min}{x}_{ij}^{*} \end{gathered}$$

(28)

In general, the evaluation values of alternatives with respect to criteria $(x_{ij})$ and the weights for each criterion ($w_j$) are given as the inputs in the decision matrix. Note that each criterion reflects its certain dimensions; therefore, a comparative analysis and preventing potential consequences from different dimensions require derive dimensionless quantities. To do this, the weighted values are simply divided by optimum obtained as (28). Numerous methods are available for deriving dimensionless useful dimensionless values which will be described below. Through normalization, the values of an original decision matrix are converted into the values on [0, 1] or on [0, ∞].

- Step 2: The primary inputs are normalized for all criteria, represented by ${\overline{x}}_{ij}$ and formed the matrix elements.

$$\overline{X}=\left[\begin{array}{ccccc}{\overline{x}}_{01}& \dots & {\overline{x}}_{0j}& \dots & {\overline{x}}_{0n}\\ ⋮& \ddots & ⋮& \ddots & ⋮\\ {\overline{x}}_{i1}& \dots & {\overline{x}}_{ij}& \dots & {\overline{x}}_{in}\\ ⋮& \ddots & ⋮& \ddots & ⋮\\ {\overline{x}}_{m1}& \dots & {\overline{x}}_{mj}& \dots & {\overline{x}}_{mn}\end{array}\right];i=\overline{0,m};j=\overline{1,n}$$

(29)

Since there are benefit type and cost type criteria, then the normalization is processed positively or negatively by using (30) and (31), respectively.

$${\overline{x}}_{ij}=\frac{x_{ij}}{{{\displaystyle \sum }}_{i=0}^m{x}_{ij}}$$

(30)

$$x_{ij}=\frac{1}{x_{ij}^{*}}{\overline{x}}_{ij}=\frac{x_{ij}}{{{\displaystyle \sum }}_{i=0}^m{x}_{ij}}$$

(31)

The achievement of dimensionless quantities provides a framework for comparing each criterion against all others.

- Step 3: Here, the weighted normalized decision matrix, $\widehat{X}$, is calculated by applying the weight values on the normalized decision matrix $\overline{X}$. The weights are determined by expert panels and should meet the following requirements:

$$\begin{gathered} 0<w_j<1,{{\displaystyle \sum }}_{j=1}^n{w}_j=1 \\ \widehat{X}=\left[\begin{array}{ccccc}{\widehat{x}}_{01}& \dots & {\widehat{x}}_{0j}& \dots & {\widehat{x}}_{0n}\\ ⋮& \ddots & ⋮& \ddots & ⋮\\ {\widehat{x}}_{i1}& \dots & {\widehat{x}}_{ij}& \dots & {\widehat{x}}_{in}\\ ⋮& \ddots & ⋮& \ddots & ⋮\\ {\widehat{x}}_{m1}& \dots & {\widehat{x}}_{mj}& \dots & {\widehat{x}}_{mn}\end{array}\right];i=\overline{0,m};j=\overline{1,n} \end{gathered}$$

(32)

$${\widehat{x}}_{ij}={\overline{x}}_{ij}{w}_j;i=\overline{0,m}$$

(33)

Again, $w_j$ denotes the weight value for the jth criterion and ${\overline{x}}_{ij}$ represents the normalized value for the ith alternative. Therefore, the value of an optimal function can be calculated as follows:

$$S_i={\displaystyle \sum }_{j=1}^n{\widehat{x}}_{ij};i=\overline{0,m}$$

(34)

According to the logic of ARAS, the best alternative is the only one with the greatest value for an optimal function. Clearly, the worst alternative obtains the value of minimum for the optimal function. To put it differently, the alternative ranking is determined based on the value of $S_i$.

The degree of utility can be measured by comparing each alternative against the best/optimal one with the best value, represented by $S_0$. The degree of utility, $K_i$, of the alternative $A_i$ follows Equation (35):

$$K_i=\frac{S_i}{S_0};i=\overline{0,m}$$

(35)

where, $S_0$ and $S_i$ are derived from Equation (34). Clearly, $K_i$ places on the interval [0, 1] and its value is used for ranking all alternatives.

3.3.6. An Extension of ARAS Method Based on Interval-Valued Triangular Fuzzy Numbers

- Step 1: Determine the optimal performance rating for each criterion

The first point to be considered is that the optimal performance rating for each criterion should be calculated as an interval-valued fuzzy number. Therefore, optimal interval-valued fuzzy performance ratings can be determined as follows:

$${\tilde{x}}_{0j}=\left[\left(l_{0j},l_{0j}^{\prime }\right),m_{0j},\left(u_{0j}^{\prime },u_{0j}\right)\right]$$

(36)

where, ${\tilde{x}}_{0j}$ represents the optimal interval-valued fuzzy performance rating of the jth criterion. Also, other criteria are defined as follows:

$$l_{0j}=\{\begin{array}{c}\underset{i}{max} l_{ij};j\in {\Omega }_{max}\\ \underset{i}{min} l_{ij};j\in {\Omega }_{min}\end{array}$$

(37)

$${l^{\prime }}_{0j}=\{\begin{array}{c}\underset{i}{max} {l^{\prime }}_{ij};j\in {\Omega }_{max}\\ \underset{i}{min} {l^{\prime }}_{ij};j\in {\Omega }_{min}\end{array}$$

(38)

$$m_{0j}=\{\begin{array}{c}\underset{i}{max} m_{ij};j\in {\Omega }_{max}\\ \underset{i}{min} m_{ij};j\in {\Omega }_{min}\end{array}$$

(39)

$${u^{\prime }}_{0j}=\{\begin{array}{c}\underset{i}{max} {u^{\prime }}_{ij};j\in {\Omega }_{max}\\ \underset{i}{min} {u^{\prime }}_{ij};j\in {\Omega }_{min}\end{array}$$

(40)

$$u_{0j}=\{\begin{array}{c}\underset{i}{max} u_{ij};j\in {\Omega }_{max}\\ \underset{i}{min} u_{ij};j\in {\Omega }_{min}\end{array}$$

(41)

${\Omega }_{max}$ denotes the benefit criteria, i.e., the higher the values are, the better it is; and ${\Omega }_{min}$ denotes the set of cost criteria, i.e., the lower the values are, the better it is.

- Step 2: Calculate the normalized decision matrix

To enable the use of these interval-valued fuzzy numbers, the normalization process requires some modifications. So, (34) can be replaced by (42):

$${\tilde{r}}_{ij}=\{\begin{array}{c}\left[\right(\frac{a_{ij}}{c_j^{+}},\frac{a_{ij}^{\prime }}{c_j^{+}}),\frac{b_{ij}}{c_j^{+}},(\frac{c_{ij}^{\prime }}{c_j^{+}},\frac{c_{ij}}{c_j^{+}}\left)\right];j\in {\Omega }_{max}\\ \left[\right(\frac{\frac{1}{a_{ij}}}{a_j^{-}},\frac{\frac{1}{a_{ij}^{\prime }}}{a_j^{-}}),\frac{\frac{1}{b_{ij}}}{a_j^{-}},(\frac{\frac{1}{c_{ij}^{\prime }}}{a_j^{-}},\frac{\frac{1}{c_{ij}}}{a_j^{-}}\left)\right];j\in {\Omega }_{min}\end{array}$$

(42)

Here, ${\tilde{r}}_{ij}$ is the optimal interval-valued fuzzy performance rating for the ith alternative on the jth criterion. Further,

$$a_j^{-}={{\displaystyle \sum }}_{i=0}^m\frac{1}{a_{ij}},c_j^{+}={{\displaystyle \sum }}_{i=0}^m{c}_{ij},i=0,1,\dots ,m$$

- Step 3: Calculate the normalized weighted interval-valued decision matrix

This is principally similar to the third step in the original ARAS method. The difference is that fuzzy numbers are to be now multiplied by using the multiplication operation on interval-valued triangular fuzzy numbers. Therefore, this can be expressed as follow:

$${\tilde{v}}_{ij}={\tilde{w}}_j.{\tilde{r}}_{ij}$$

(43)

where, ${\tilde{v}}_{ij}$ is the normalized weighted interval-valued fuzzy performance rating for the i-th alternative on the j-th criterion.

- Step 4: Compute the overall interval-valued fuzzy performance ratings

This step can be expressed using (44):

$${\tilde{S}}_i={\displaystyle \sum }_{j=1}^n{\tilde{v}}_{ij}$$

(44)

where, ${\tilde{S}}_i$ is the overall interval-valued fuzzy performance rating for the ith alternative.

- Step 5: Measure the degree of utility for each alternative

Since the result obtained from the previous step is provided as an interval-valued fuzzy numbers, the calculation process is often more complex with the overall degree of utility. Obviously, it should be transformed into a non-fuzzy number. The degree of utility can be calculated as follow:

$${\tilde{Q}}_i=\frac{{\tilde{S}}_i}{{\tilde{S}}_0}$$

(45)

Again, as the products of (45) are still interval-valued fuzzy numbers, these typically need to be defuzzified. The defuzzification process must be initiated prior of determining the degree of utility. There are a wide range of defuzzification methods with a variety of impacts on resultant outputs. Therefore, it is important how to choose an appropriate defuzzification technique.

- Step 6: Rank alternatives and select the most efficient one

This step follows the similar process as the original Additive Ratio Assessment method.

4. Results: A Case Study of Well-Drilling Projects

The case study of this research includes a company active in the Iranian drilling industry, implementing seven oil and gas well drilling projects. The locations of these projects are Iranian oil reservoirs which have a major portion of the country’s oil production. Concerns about environmental issues show other challenges related to such projects. Based on the opinion of the senior management, a group of three experienced professionals in the field of Iranian oil and gas drilling industry was established as the expert team to provide expertized views in different stages. In the first step, the initial questionnaire obtained from Table 1 was distributed among 15 experts in Iran’s oil and gas wells projects who were introduced by the expert team. The questionnaire consisted of two main parts: The first part included the importance evaluation of the criteria derived from previous studies (Table 1) based on a Likert scale. In the second part, the expert was asked to propose some measures important in the project evaluation but not included in the first part. As the first round of survey completed, a total of 13 criteria were confirmed by the Delphi method, while 14 new criteria were suggested by experts. Based on these results, the second questionnaire was formulated and its results were also analyzed as in the first stage. Finally, a list of 20 criteria was developed and categorized for evaluating oil and gas well drilling projects using the Fuzzy Delphi method. According to the expert opinions, these criteria were classified in six main criteria (

Table 4. Evaluation Criteria in Oil & Gas Well-Drilling Projects using Fuzzy Delphi method.

Criteria	Code	Sub-Criteria	Code
Materials & Equipment	A	Number of drilling rigs used	A1
		Type of drilling rigs	A2
		Quality of materials and goods used	A3
		Quality of drilling and support service	A4
Human Resource	B	Number of operational experts working on the project	B1
		Scientific levels of drilling specialists working in the project	B2
		Experience of drilling specialists working in Project (Ave.)	B3
		Average salaries of employees in the project (Million Rls)	B4
Planning	C	Type of drilled wells in terms of operational risk	C1
		Type of drilled wells in terms of depth	C2
		Type and number of fields under operation	C3
		Cost spent for drilling project (Billion Rls)	C4
		Status of cash flows in project	C5
Quality	D	Employer/Senior Manager Satisfaction	D1
		Waiting time percentage to total well drilling time	D2
		Number of failure reports	D3
		Number of accidents caused by non-compliance with safety regulation, or environmental factors	D4
		Actual cost compliance percentage with planned cost	D5
Number of planned wells	E	number of planned wells in a certain period of time	E
Number of drilled wells	F	number of drilled wells in a certain period of time	F

).

Next, the SWARA technique was implemented to determine weights of all criteria and sub-criteria based on the opinion of each expert. Also, the obtained values were integrated to calculate the final weights.

Table 5. Calculation of Criteria Weights for Expert #1.

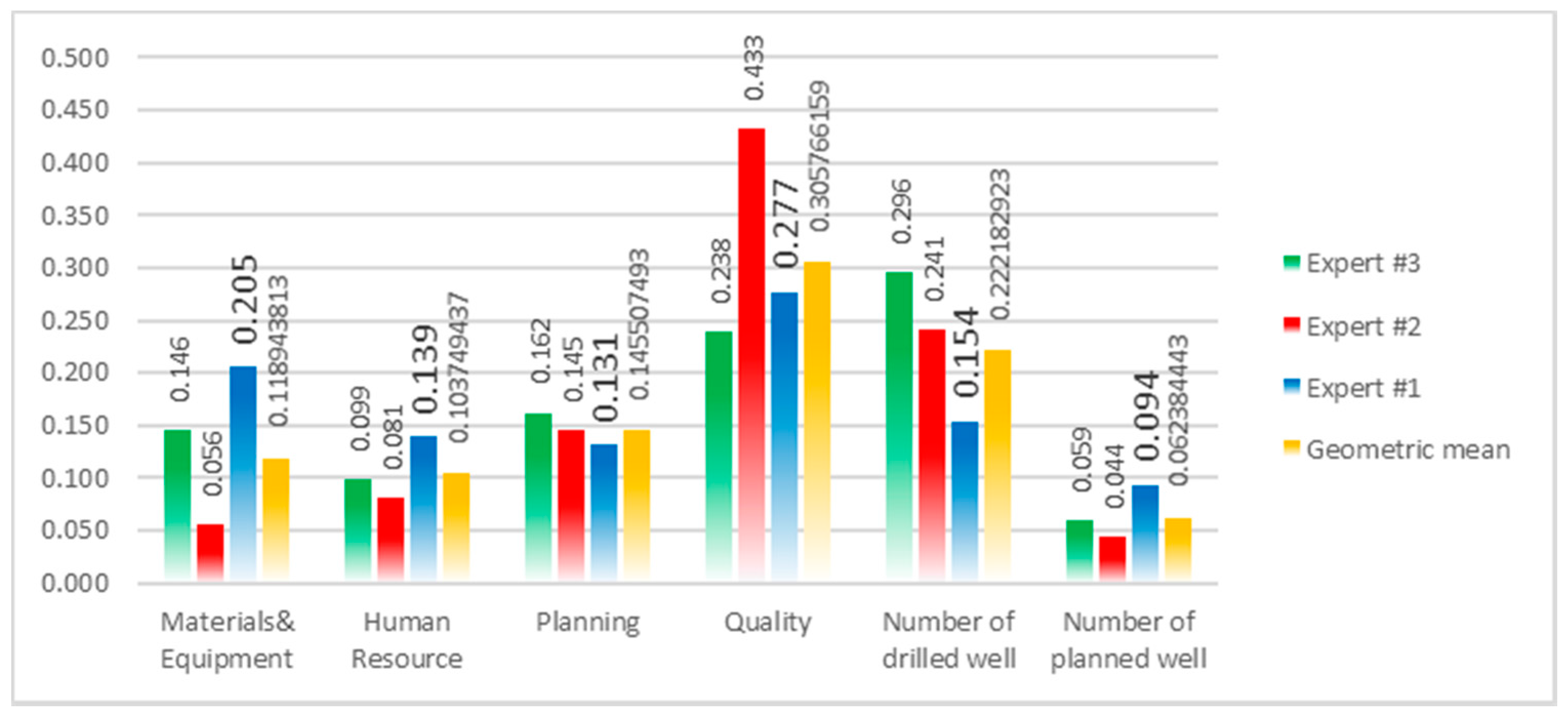
Code	Criterion	Sj	Kj (Kj = 1 + Sj)	Initial Weight	Normalized Final Weights
D	Quality	1	1	1	0.277
A	Materials & Equipment	0.35	1.35	0.741	0.205
E	Number of drilled wells	0.33	1.33	0.557	0.154
B	Human Resource	0.11	1.11	0.502	0.139
C	Planning	0.06	1.06	0.473	0.131
F	Number of planned wells	0.4	1.4	0.338	0.094

shows the results.

As seen in Table 5, based on the first step of the SWARA method, the expert was asked to rank the criteria in a descending order of importance. The results are displayed in the second column of Table 5. Also, the second, third and fourth steps of the SWARA method are presented in the columns 3, 4 and 5 of Table 5, respectively. By implementing the final step of the SWARA method to normalize the weights of criteria, the final weighting values are shown in the column 6 of Table 5. A similar procedure was carried out for the second and third experts. Moreover, for comparative analysis of the weights of all criteria according to expert opinions and the geometric means, the weights and the geometric means obtained from each expert are presented in Figure 5. The same was carried out for the sub-criteria. According to the results, the most important sub-criteria for the materials and equipment, human resource, planning and the quality criteria are types of drilling rigs, experience of drilling specialists working in project, cost spent for drilling project and actual cost compliance percentage with planned cost. Now, to determine the final weights of the sub-criteria, the weights of one sub-criterion obtained from each expert multiplied by the weights of the criteria corresponding to that sub-criterion. In order to aggregate expert opinions, the geometric means for the weights obtained for each sub-criterion from three experts were calculated, shown in the last column of

Table 6. Calculation of Sub-Criteria Weights for individual Experts and Normalized Final Weights.

Expert #1					Expert #2					Expert #3					Geometric Mean of Sub-Criteria Weight	Normalized Final Weights
Criterion Code	Criterion Weight	Sub-Criterion Code	Weight in Each Criterion	Final Weight of Sub-Criteria	Criterion Code	Criterion Weight	Sub-Criterion Code	Weight in Each Criterion	Final Weight of Sub-Criteria	Criterion Code	Criterion Weight	Sub-Criterion Code	Weight in Each Criterion	Final Weight of Sub-Criteria
A	0.205	A1	0.221	0.045	A	0.056	A1	0.262	0.015	A	0.146	A1	0.312	0.046	0.031	0.033
		A2	0.307	0.063			A2	0.445	0.025			A2	0.295	0.043	0.041	0.044
		A3	0.272	0.056			A3	0.114	0.006			A3	0.185	0.027	0.021	0.023
		A4	0.201	0.041			A4	0.178	0.010			A4	0.208	0.030	0.023	0.025
B	0.139	B1	0.236	0.033	B	0.081	B1	0.108	0.009	B	0.099	B1	0.301	0.030	0.020	0.022
		B2	0.278	0.039			B2	0.287	0.023			B2	0.193	0.019	0.026	0.028
		B3	0.286	0.040			B3	0.425	0.035			B3	0.389	0.038	0.038	0.040
		B4	0.200	0.028			B4	0.180	0.015			B4	0.117	0.012	0.017	0.018
C	0.131	C1	0.186	0.024	C	0.145	C1	0.348	0.050	C	0.162	C1	0.180	0.029	0.033	0.035
		C2	0.307	0.040			C2	0.170	0.025			C2	0.249	0.040	0.034	0.037
		C3	0.109	0.014			C3	0.132	0.019			C3	0.127	0.021	0.018	0.019
		C4	0.279	0.037			C4	0.264	0.038			C4	0.3	0.060	0.044	0.047
		C5	0.119	0.016			C5	0.087	0.013			C5	0.073	0.012	0.013	0.014
D	0.277	D1	0.248	0.069	D	0.433	D1	0.108	0.047	D	0.238	D1	0.278	0.066	0.060	0.064
		D2	0.199	0.055			D2	0.423	0.183			D2	0.177	0.042	0.075	0.080
		D3	0.129	0.036			D3	0.159	0.069			D3	0.135	0.032	0.043	0.046
		D4	0.136	0.038			D4	0.083	0.036			D4	0.076	0.018	0.029	0.031
		D5	0.288	0.080			D5	0.226	0.098			D5	0.334	0.080	0.085	0.091
E				0.154	E				0.241	E				0.296	0.222	0.237
F				0.094	F				0.044	F				0.059	0.062	0.067

. Finally, each element of the column was divided by the sum of all its elements to determine the normalized final weight of each sub-criterion. The results are shown in the last column of Table 6.

As found, some criteria such as the number of drilled wells and the sub-criteria such as the actual cost compliance percentage with planned costs and the percentage of waiting time to total well drilling time have been identified as the most important sub-criteria.

Table 7. Rankings of Sub-criteria in order of importance.

Code	Sub-Criteria	Final Weight
E	Number of drilled wells	0.237
D5	Actual cost compliance percentage with planned cost	0.091
D2	Waiting time percentage to total well drilling time	0.080
F	Number of planned wells	0.067
D1	Employer/Senior Manager Satisfaction	0.064
C4	Cost spent for drilling project (Billion Rls)	0.047
D3	Number of failure reports	0.046
A2	Type of drilling rigs	0.044
B3	Experience of drilling specialists working in Project	0.040
C2	Type of drilled wells in terms of depth	0.037
C1	Type of drilled wells in terms of operational risk	0.035
A1	Number of drilling rigs used	0.033
D4	Number of accidents caused by non-compliance with safety regulation	0.031
B2	Scientific levels of drilling specialists working in the project	0.028
A4	Quality of drilling and support service	0.025
A3	Quality of materials and goods used	0.023
B1	Number of operational experts working on the project	0.022
C3	Type and number of fields under operation	0.019
B4	Average salaries of employees in the project (Million Rls)	0.018
C5	Status of cash flows in project	0.014

shows the ranking of the criteria in terms of importance.

Now, as the weight and importance of the criteria determined, the evaluation of each alternative in terms of all criteria was carried out by the experts using the linguistic variables in Table 2. The results are presented in

Table 8. Initial Decision Matrix on Linguistic Variables.

Code	A1	A2	A3	A4	B1	B2	B3	B4	C1	C2	C3	C4	C5	D1	D2	D3	D4	D5	E	F
Weight	0.033	0.044	0.023	0.025	0.022	0.028	0.040	0.018	0.035	0.037	0.019	0.047	0.014	0.064	0.080	0.046	0.031	0.091	0.237	0.067
Sign	+	+	+	+	+	+	+	+	−	−	−	−	+	+	−	−	−	+	+	−
Expert #1
Project #1	G	G	G	VG	F	F	VG	G	F	VG	VG	F	G	G	F	F	F	G	P	G
Project #2	F	VG	F	G	G	G	G	P	P	G	F	G	F	G	P	P	F	VG	G	G
Project #3	F	G	VG	G	G	F	F	P	F	G	P	F	VG	F	F	G	VP	P	VP	G
Project #4	P	G	G	F	F	G	VG	F	G	F	F	VG	G	VG	P	P	G	G	F	VG
Project #5	G	F	F	G	F	G	G	F	VG	F	F	F	G	G	G	VG	G	VP	VG	F
Project #6	F	VG	P	VG	VG	VG	F	P	F	VG	G	P	F	F	VP	P	P	F	P	F
Project #7	G	G	G	F	G	F	F	P	VG	VG	VG	VG	G	VG	P	G	G	F	VP	G
Expert #2
Project #1	VG	G	VG	VG	P	F	VG	F	F	VG	VG	F	VG	G	F	F	F	VG	P	G
Project #2	F	VG	P	F	F	G	G	P	VP	G	F	G	F	VG	P	P	F	VG	G	G
Project #3	F	G	G	VG	G	F	P	P	G	G	P	P	VG	F	G	G	VP	P	VP	G
Project #4	P	P	F	P	F	G	VG	F	G	P	F	VG	G	VG	P	P	G	G	P	VG
Project #5	VG	F	F	F	P	G	G	F	VG	F	F	VG	G	G	VG	VP	VG	VP	VG	F
Project #6	F	VG	P	G	VG	VG	P	P	F	VG	G	P	P	P	VP	P	P	P	P	VG
Project #7	VG	G	G	P	G	F	F	P	VG	VG	VG	VG	G	VG	P	G	VG	P	VP	G
Expert #3
Project #1	G	G	F	VG	F	F	VG	G	P	VG	VG	F	G	VG	F	F	G	G	G	P
Project #2	F	VG	F	G	G	G	F	P	P	P	F	G	F	G	P	P	F	VG	G	G
Project #3	F	G	VG	F	F	F	P	P	F	G	P	F	VG	P	G	VG	VP	P	P	G
Project #4	VP	F	P	G	G	P	G	G	VG	VP	G	VG	G	G	P	P	VG	VG	G	VG
Project #5	VG	VP	F	G	P	G	G	F	G	VG	F	P	G	F	VG	VP	VG	VP	VG	F
Project #6	P	VG	P	VG	VG	VG	F	P	P	G	G	F	F	VP	VP	P	F	F	VG	VP
Project #7	G	G	G	P	G	F	VP	VP	G	VG	VG	G	G	VG	F	G	VG	G	VP	F

.

Then, the qualitative values were converted into quantitative values using Table 2, in order to establish the initial decision-making tables for the three experts.

Table 9. Initial Decision Matrix for Expert #1 with Quantitative Values.

Code	A1	A2	A3	A4	B1	B2	B3	B4	C1	C2
Weight	0.033	0.044	0.023	0.025	0.022	0.028	0.040	0.018	0.035	0.037
Sign	+	+	+	+	+	+	+	+	−	−
Project #1	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.9, 1, 1)
Project #2	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0, 0, 0.1)	(0.7, 0.9, 1)
Project #3	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.7, 0.9, 1)
Project #4	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0, 0.1, 0.3)
Project #5	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.3, 0.5, 0.7)
Project #6	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.9, 1, 1)
Project #7	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0.9, 1, 1)	(0.9, 1, 1)
Code	C3	C4	C5	D1	D2	D3	D4	D5	E	F
Weight	0.019	0.047	0.014	0.064	0.080	0.046	0.031	0.091	0.237	0.067
Sign	−	−	+	+	−	−	−	+	+	−
Project #1	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)
Project #2	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)
Project #3	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0, 0.1)	(0, 0.1, 0.3)	(0, 0, 0.1)	(0.7, 0.9, 1)
Project #4	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0.9, 1, 1)
Project #5	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0, 0, 0.1)	(0.9, 1, 1)	(0, 0, 0.1)	(0.9, 1, 1)	(0.3, 0.5, 0.7)
Project #6	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0, 0, 0.1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.9, 1, 1)
Project #7	(0.9, 1, 1)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0, 0, 0.1)	(0.7, 0.9, 1)

,

Table 10. Initial Decision Matrix for Expert #2 with Quantitative Values.

Code	A1	A2	A3	A4	B1	B2	B3	B4	C1	C2
Weight	0.033	0.044	0.023	0.025	0.022	0.028	0.040	0.018	0.035	0.037
Sign	+	+	+	+	+	+	+	+	−	−
Project #1	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.9, 1, 1)
Project #2	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0, 0, 0.1)	(0.7, 0.9, 1)
Project #3	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.7, 0.9, 1)
Project #4	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0, 0.1, 0.3)
Project #5	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.3, 0.5, 0.7)
Project #6	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.9, 1, 1)
Project #7	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0.9, 1, 1)	(0.9, 1, 1)
Code	C3	C4	C5	D1	D2	D3	D4	D5	E	F
Weight	0.019	0.047	0.014	0.064	0.080	0.046	0.031	0.091	0.237	0.067
Sign	−	−	+	+	−	−	−	+	+	−
Project #1	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)
Project #2	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)
Project #3	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0, 0.1)	(0, 0.1, 0.3)	(0, 0, 0.1)	(0.7, 0.9, 1)
Project #4	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0.9, 1, 1)
Project #5	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0, 0, 0.1)	(0.9, 1, 1)	(0, 0, 0.1)	(0.9, 1, 1)	(0.3, 0.5, 0.7)
Project #6	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0, 0, 0.1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.9, 1, 1)
Project #7	(0.9, 1, 1)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0, 0, 0.1)	(0.7, 0.9, 1)

and

Table 11. Initial Decision Matrix for Expert #3 with Quantitative Values.

Code	A1	A2	A3	A4	B1	B2	B3	B4	C1	C2
Weight	0.033	0.044	0.023	0.025	0.022	0.028	0.040	0.018	0.035	0.037
Sign	+	+	+	+	+	+	+	+	−	−
Project #1	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0.9, 1, 1)
Project #2	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0, 0.1, 0.3)
Project #3	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)
Project #4	(0, 0, 0.1)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0, 0, 0.1)
Project #5	(0.9, 1, 1)	(0, 0, 0.1)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.9, 1, 1)
Project #6	(0, 0.1, 0.3)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.9, 1, 1)	(0.9, 1, 1)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.7, 0.9, 1)
Project #7	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0, 0, 0.1)	(0, 0, 0.1)	(0.7, 0.9, 1)	(0.9, 1, 1)
Code	C3	C4	C5	D1	D2	D3	D4	D5	E	F
Weight	0.019	0.047	0.014	0.064	0.080	0.046	0.031	0.091	0.237	0.067
Sign	−	−	+	+	−	−	−	+	+	−
Project #1	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)
Project #2	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)
Project #3	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0, 0, 0.1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.7, 0.9, 1)
Project #4	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0, 0.1, 0.3)	(0, 0.1, 0.3)	(0.9, 1, 1)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)
Project #5	(0.3, 0.5, 0.7)	(0, 0.1, 0.3)	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0, 0.1)	(0.9, 1, 1)	(0, 0, 0.1)	(0.9, 1, 1)	(0.3, 0.5, 0.7)
Project #6	(0.7, 0.9, 1)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0, 0, 0.1)	(0, 0, 0.1)	(0, 0.1, 0.3)	(0.3, 0.5, 0.7)	(0.3, 0.5, 0.7)	(0.9, 1, 1)	(0, 0, 0.1)
Project #7	(0.9, 1, 1)	(0.7, 0.9, 1)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.3, 0.5, 0.7)	(0.7, 0.9, 1)	(0.9, 1, 1)	(0.7, 0.9, 1)	(0, 0, 0.1)	(0.3, 0.5, 0.7)

provide the quantitative values for the experts.

Next, using Equations (18)–(22), the fuzzy values in the decision matrix of three experts were converted into fuzzy numbers with interval values, as shown in

Table 12. Aggregated Decision Matrix as Fuzzy Numbers with Interval Values.

Code	A1	A2	A3	A4	B1	B2	B3	B4	C1	C2
Weight	0.033	0.044	0.023	0.025	0.022	0.028	0.040	0.018	0.035	0.037
Sign	+	+	+	+	+	+	+	+	−	−
Ideal Alternative (X0)	[(0.7, 0.8277), 0.9655, (1, 1)]	[(0.9, 0.9), 1, (1, 1)]	[(0.7, 0.8277), 0.9655, (1, 1)]	[(0.9, 0.9), 1, (1, 1)]	[(0.9, 0.9), 1, (1, 1)]	[(0.9, 0.9), 1, (1, 1)]	[(0.9, 0.9), 1, (1, 1)]	[(0.3, 0.5278), 0.7399, (0.8879, 1)]	[(0, 0), 0, (0.2080, 0.3)]	[(0, 0), 0, (0.2759, 0.7)]
Project #1	[(0.7, 0.76), 0.93, (1, 1)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.30, 0.57), 0.77, (0.89, 1)]	[(0.90, 0.90), 1, (1, 1)]	[(0, 0), 0.29, (0.53, 0.7)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.90, 0.90), 1, (1, 1)]	[(0.30, 0.53), 0.74, (0.89, 1)]	[(0, 0), 0.29, (0.53, 0.7)]	[(0.90, 0.90), 1, (1, 1)]
Project #2	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.90, 0.90), 1, (1, 1)]	[(0, 0), 0.29, (0.53, 0.7)]	[(0.30, 0.53), 0.74, (0.89, 1)]	[(0.30, 0.53), 0.74, (0.89, 1)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.30, 0.53), 0.74, (0.89, 1)]	[(0, 0), 0.10, (0.30, 0.30)]	[(0, 0), 0, (0.21, 0.30)]	[(0, 0), 0.43, (0.67, 1)]
Project #3	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.7, 0.83), 0.97, (1, 1)]	[(0.30, 0.57), 0.77, (0.89, 1)]	[(0.30, 0.53), 0.74, (0.89, 1)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0, 0), 0.17, (0.40, 0.7)]	[(0, 0), 0.10, (0.30, 0.30)]	[(0.30, 0.40), 0.61, (0.79, 1)]	[(0.7, 0.7), 0.90, (1, 1)]
Project #4	[(0, 0), 0, (0.21, 0.30)]	[(0, 0), 0.36, (0.59, 1)]	[(0, 0), 0.36, (0.59, 1)]	[(0, 0), 0.36, (0.59, 1)]	[(0.30, 0.40), 0.61, (0.79, 1)]	[(0, 0), 0.43, (0.67, 1)]	[(0.7, 0.83), 0.97, (1, 1)]	[(0.30, 0.40), 0.61, (0.79, 1)]	[(0.7, 0.76), 0.93, (1, 1)]	[(0, 0), 0, (0.28, 0.7)]
Project #5	[(0.7, 0.83), 0.97, (1, 1)]	[(0, 0), 0, (0.37, 0.7)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.30, 0.53), 0.74, (0.89, 1)]	[(0, 0), 0.17, (0.40, 0.7)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.7, 0.83), 0.97, (1, 1)]	[(0.30, 0.43), 0.63, (0.79, 1)]
Project #6	[(0, 0), 0.29, (0.53, 0.7)]	[(0.90, 0.90), 1, (1, 1)]	[(0, 0), 0.10, (0.30, 0.30)]	[(0.7, 0.83), 0.97, (1, 1)]	[(0.90, 0.90), 1, (1, 1)]	[(0.90, 0.90), 1, (1, 1)]	[(0, 0), 0.29, (0.53, 0.7)]	[(0, 0), 0.10, (0.30, 0.30)]	[(0, 0), 0.29, (0.53, 0.7)]	[(0.7, 0.83), 0.97, (1, 1)]
Project #7	[(0.7, 0.76), 0.93, (1, 1)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0, 0), 0.17, (0.40, 0.7)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0, 0), 0, (0.37, 0.7)]	[(0, 0), 0, (0.21, 0.30)]	[(0.7, 0.83), 0.97, (1, 1)]	[(0.90, 0.90), 1, (1, 1)]
Code	C3	C4	C5	D1	D2	D3	D4	D5	E	F
Weight	0.019	0.047	0.014	0.064	0.080	0.046	0.031	0.091	0.237	0.067
Sign	−	−	+	+	−	−	−	+	+	−
Ideal Alternative (X0)	[(0, 0), 0.1, (0.3, 0.3)]	[(0, 0), 0.1710, (0.3979, 0.7)]	[(0.9, 0.9), 1, (1, 1)]	[(0.9, 0.9), 1, (1, 1)]	[(0, 0), 0, (0.1, 0.1)]	[(0, 0), 0, (0.2154, 0.3)]	[(0, 0), 0, (0.1, 0.1)]	[(0.9, 0.9), 1, (1, 1)]	[(0.9, 0.9), 1, (1, 1)]	[(0, 0), 0, (0.4121, 0.7)]
Project #1	[(0.90, 0.90), 1, (1, 1)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.7, 0.76), 0.93, (1, 1)]	[(0.7, 0.76), 0.93, (1, 1)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.30, 0.40), 0.61, (0.79, 1)]	[(0.7, 0.76), 0.93, (1, 1)]	[(0, 0), 0.21, (0.45, 1)]	[(0, 0), 0.43, (0.67, 1)]
Project #2	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.7, 0.76), 0.93, (1, 1)]	[(0, 0), 0.10, (0.30, 0.30)]	[(0, 0), 0.10, (0.30, 0.30)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0.90, 0.90), 1, (1, 1)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.7, 0.7), 0.90, (1, 1)]
Project #3	[(0, 0), 0.10, (0.30, 0.30)]	[(0, 0), 0.29, (0.53, 0.7)]	[(0.90, 0.90), 1, (1, 1)]	[(0, 0), 0.29, (0.53, 0.7)]	[(0.30, 0.53), 0.74, (0.89, 1)]	[(0.7, 0.76), 0.93, (1, 1)]	[(0, 0), 0, (0.10, 0.10)]	[(0, 0), 0.10, (0.30, 0.30)]	[(0, 0), 0, (0.14, 0.30)]	[(0.7, 0.7), 0.90, (1, 1)]
Project #4	[(0.30, 0.40), 0.61, (0.79, 1)]	[(0.90, 0.90), 1, (1, 1)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.7, 0.83), 0.97, (1, 1)]	[(0, 0), 0.10, (0.30, 0.30)]	[(0, 0), 0.10, (0.30, 0.30)]	[(0.7, 0.76), 0.93, (1, 1)]	[(0.7, 0.76), 0.93, (1, 1)]	[(0, 0), 0.36, (0.59, 1)]	[(0.90, 0.90), 1, (1, 1)]
Project #5	[(0.30, 0.30), 0.50, (0.7, 0.7)]	[(0, 0), 0.37, (0.59, 1)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.30, 0.53), 0.74, (0.89, 1)]	[(0.7, 0.83), 0.97, (1, 1)]	[(0, 0), 0, (0.22, 1)]	[(0.7, 0.83), 0.97, (1, 1)]	[(0, 0), 0, (0.10, 0.10)]	[(0.90, 0.90), 1, (1, 1)]	[(0.30, 0.30), 0.50, (0.7, 0.7)]
Project #6	[(0.7, 0.7), 0.90, (1, 1)]	[(0, 0), 0.17, (0.40, 0.7)]	[(0, 0), 0.29, (0.53, 0.7)]	[(0, 0), 0, (0.28, 0.7)]	[(0, 0), 0, (0.10, 0.10)]	[(0, 0), 0.10, (0.30, 0.30)]	[(0, 0), 0.17, (0.40, 0.7)]	[(0, 0), 0.29, (0.53, 0.7)]	[(0, 0), 0.22, (0.45, 1)]	[(0, 0), 0, (0.41, 1)]
Project #7	[(0.90, 0.90), 1, (1, 1)]	[(0.7, 0.83), 0.97, (1, 1)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.90, 0.90), 1, (1, 1)]	[(0, 0), 0.17, (0.40, 0.7)]	[(0.7, 0.7), 0.90, (1, 1)]	[(0.7, 0.83), 0.97, (1, 1)]	[(0, 0), 0.36, (0.59, 1)]	[(0, 0), 0, (0.10, 0.10)]	[(0.30, 0.53), 0.74, (0.89, 1)]

.

Table 12 presents the ideal alternative using Equations (35)–(40). In the next step using Equation (41), the interval valued fuzzy decision matrix (Table 12) was normalized. The normalized matrix is shown in

Table 13. Normalized Decision Matrix as Fuzzy Numbers with Interval Values.

Code	A1	A2	A3	A4	B1	B2	B3	B4	C1	C2
Weight	0.033	0.044	0.023	0.025	0.022	0.028	0.040	0.018	0.035	0.037
Sign	+	+	+	+	+	+	+	+	−	−
Ideal Alternative (X0)	[(0.1169, 0.1169), 0.1299, (0.1299, 0.1299)]	[(0.1045, 0.1235), 0.1441, (0.1493, 0.1493)]	[(0.1169, 0.1169), 0.1299, (0.1299, 0.1299)]	[(0.1216, 0.1216), 0.1351, (0.1351, 0.1351)]	[(0.1268, 0.1268), 0.1408, (0.1408, 0.1408)]	[(0.1268, 0.1268), 0.1408, (0.1408, 0.1408)]	[(0.0612, 0.1077), 0.1510, (0.1812, 0.2041)]	[(0.2007, 0.2007), 0.2007, (0, 0)]	[(0.2687, 0.2687), 0.2687, (0, 0)]	[(0.5132, 0.5132), 0, (0, 0)]
Project #1	[(0.0909, 0.0909), 0.1169, (0.1299, 0.1299)]	[(0.0448, 0.0857), 0.1144, (0.1325, 0.1493)]	[(0.1169, 0.1169), 0.1299, (0.1299, 0.1299)]	[(0, 0), 0.0395, (0.0713, 0.0946)]	[(0.0423, 0.0423), 0.0704, (0.0986, 0.0986)]	[(0.1268, 0.1268), 0.1408, (0.1408, 0.1408)]	[(0.0612, 0.1077), 0.1510, (0.1812, 0.2041)]	[(0.1911, 0.1911), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]
Project #2	[(0.1169, 0.1169), 0.1299, (0.1299, 0.1299)]	[(0, 0), 0.0436, (0.0788, 0.1045)]	[(0.0390, 0.0685), 0.0961, (0.1153, 0.1299)]	[(0.0405, 0.0713), 0.1000, (0.1200, 0.1351)]	[(0.0986, 0.0986), 0.1268, (0.1408, 0.1408)]	[(0.0423, 0.0743), 0.1042, (0.1251, 0.1408)]	[(0, 0), 0.0204, (0.0612, 0.0612)]	[(0.3232, 0.3232), 0.3232, (0, 0)]	[(0.4326, 0.4326), 0, (0, 0)]	[(0, 0), 0, (0, 0)]
Project #3	[(0.0909, 0.0909), 0.1169, (0.1299, 0.1299)]	[(0.1045, 0.1235), 0.1441, (0.1493, 0.1493)]	[(0.0390, 0.0745), 0.0995, (0.1153, 0.1299)]	[(0.0405, 0.0713), 0.1000, (0.1200, 0.1351)]	[(0.0423, 0.0423), 0.0704, (0.0986, 0.0986)]	[(0, 0), 0.0241, (0.0560, 0.0986)]	[(0, 0), 0.0204, (0.0612, 0.0612)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.4868, 0.4868), 0, (0, 0)]
Project #4	[(0, 0), 0.0462, (0.0772, 0.1299)]	[(0, 0), 0.0531, (0.0887, 0.1493)]	[(0, 0), 0.0462, (0.0772, 0.1299)]	[(0.0405, 0.0538), 0.0822, (0.1065, 0.1351)]	[(0, 0), 0.0609, (0.0943, 0.1408)]	[(0.0986, 0.1166), 0.1360, (0.1408, 0.1408)]	[(0.0612, 0.0812), 0.1241, (0.1609, 0.2041)]	[(0, 0), 0, (0, 0)]	[(0.2987, 0.2987), 0.2987, (0, 0)]	[(0, 0), 0, (0, 0)]
Project #5	[(0, 0), 0, (0.0475, 0.0909)]	[(0.0448, 0.0448), 0.0746, (0.1045, 0.1045)]	[(0.0390, 0.0685), 0.0961, (0.1153, 0.1299)]	[(0, 0), 0.0231, (0.0538, 0.0946)]	[(0.0986, 0.0986), 0.1268, (0.1408, 0.1408)]	[(0.0986, 0.0986), 0.1268, (0.1408, 0.1408)]	[(0.0612, 0.0612), 0.1020, (0.1429, 0.1429)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]
Project #6	[(0.1169, 0.1169), 0.1299, (0.1299, 0.1299)]	[(0, 0), 0.0149, (0.0448, 0.0448)]	[(0.0909, 0.1075), 0.1254, (0.1299, 0.1299)]	[(0.1216, 0.1216), 0.1351, (0.1351, 0.1351)]	[(0.1268, 0.1268), 0.1408, (0.1408, 0.1408)]	[(0, 0), 0.0412, (0.0743, 0.0986)]	[(0, 0), 0.0204, (0.0612, 0.0612)]	[(0.2849, 0.2849), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]
Project #7	[(0.0909, 0.0909), 0.1169, (0.1299, 0.1299)]	[(0.1045, 0.1045), 0.1343, (0.1493, 0.1493)]	[(0, 0), 0.0222, (0.0517, 0.0909)]	[(0.0946, 0.0946), 0.1216, (0.1351, 0.1351)]	[(0.0423, 0.0423), 0.0704, (0.0986, 0.0986)]	[(0, 0), 0, (0.0515, 0.0986)]	[(0, 0), 0, (0.0425, 0.0612)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]
Code	C3	C4	C5	D1	D2	D3	D4	D5	E	F
Weight	0.019	0.047	0.014	0.064	0.080	0.046	0.031	0.091	0.237	0.067
Sign	−	−	+	+	−	−	−	+	+	−
Ideal Alternative (X0)	[(0.1992, 0.1992), 0, (0, 0)]	[(0.1216, 0.1216), 0.1351, (0.1351, 0.1351)]	[(0.1216, 0.1216), 0.1351, (0.1351, 0.1351)]	[(0.1584, 0.1584), 0.1584, (0, 0)]	[(0.1472, 0.1472), 0.1472, (0, 0)]	[(0.2969, 0.2969), 0.2969, (0, 0)]	[(0.1475, 0.1475), 0.1639, (0.1639, 0.1639)]	[(0.1406, 0.1406), 0.1563, (0.1563, 0.1563)]	[(0.2966, 0.2966), 0.2966, (0, 0)]
Project #1	[(0, 0), 0, (0, 0)]	[(0.0946, 0.1029), 0.1260, (0.1351, 0.1351)]	[(0.0946, 0.1029), 0.1260, (0.1351, 0.1351)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.1148, 0.1248), 0.1528, (0.1639, 0.1639)]	[(0, 0), 0.0325, (0.0700, 0.1563)]	[(0.2824, 0.2824), 0, (0, 0)]
Project #2	[(0, 0), 0, (0, 0)]	[(0.0405, 0.0405), 0.0676, (0.0946, 0.0946)]	[(0.0946, 0.1029), 0.1260, (0.1351, 0.1351)]	[(0.2550, 0.2550), 0, (0, 0)]	[(0.2370, 0.2370), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.1475, 0.1475), 0.1639, (0.1639, 0.1639)]	[(0.1094, 0.1094), 0.1406, (0.1563, 0.1563)]	[(0, 0), 0, (0, 0)]
Project #3	[(0.1889, 0.1889), 0, (0, 0)]	[(0.1216, 0.1216), 0.1351, (0.1351, 0.1351)]	[(0, 0), 0.0395, (0.0713, 0.0946)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.2816, 0.2816), 0.2816, (0, 0)]	[(0, 0), 0.0164, (0.0492, 0.0492)]	[(0, 0), 0, (0.0225, 0.0469)]	[(0, 0), 0, (0, 0)]
Project #4	[(0, 0), 0, (0, 0)]	[(0.0946, 0.0946), 0.1216, (0.1351, 0.1351)]	[(0.0946, 0.1118), 0.1305, (0.1351, 0.1351)]	[(0.1761, 0.1761), 0, (0, 0)]	[(0.1636, 0.1636), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.1148, 0.1248), 0.1528, (0.1639, 0.1639)]	[(0, 0), 0.0556, (0.0929, 0.1563)]	[(0, 0), 0, (0, 0)]
Project #5	[(0.3293, 0.3293), 0, (0, 0)]	[(0.0946, 0.0946), 0.1216, (0.1351, 0.1351)]	[(0.0405, 0.0713), 0.1000, (0.1200, 0.1351)]	[(0, 0), 0, (0, 0)]	[(0.2433, 0.2433), 0.2433, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0.0164, 0.0164)]	[(0.1406, 0.1406), 0.1563, (0.1563, 0.1563)]	[(0, 0), 0, (0, 0)]
Project #6	[(0.2827, 0.2827), 0, (0, 0)]	[(0, 0), 0.0395, (0.0713, 0.0946)]	[(0, 0), 0, (0.0373, 0.0946)]	[(0.2248, 0.2248), 0.2248, (0, 0)]	[(0.2089, 0.2089), 0, (0, 0)]	[(0.4215, 0.4215), 0, (0, 0)]	[(0, 0), 0.0479, (0.0865, 0.1148)]	[(0, 0), 0.0337, (0.0700, 0.1563)]	[(0.4210, 0.4210), 0.4210, (0, 0)]
Project #7	[(0, 0), 0, (0, 0)]	[(0.0946, 0.0946), 0.1216, (0.1351, 0.1351)]	[(0.1216, 0.1216), 0.1351, (0.1351, 0.1351)]	[(0.1858, 0.1858), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0.0583, (0.0974, 0.1639)]	[(0, 0), 0, (0.0156, 0.0156)]	[(0, 0), 0, (0, 0)]

.

Here, the weighting values obtained from the SWARA method normalized and aggregated in the final column of Table 6 and also Equation (43) were applied to achieve the normalized decision matrix with fuzzy intervals, as shown in

Table 14. Normalized weighted Decision Matrix with Interval-valued Fuzzy Numbers.

Code	A1	A2	A3	A4	B1	B2	B3	B4	C1	C2
Weight	0.033	0.044	0.023	0.025	0.022	0.028	0.040	0.018	0.035	0.037
Sign	+	+	+	+	+	+	+	+	−	−
Ideal Alternative (X0)	[(0.0036, 0.0043), 0.0050, (0.0052, 0.0052)]	[(0.0051, 0.0051), 0.0057, (0.0057, 0.0057)]	[(0.0024, 0.0028), 0.0033, (0.0034, 0.0034)]	[(0.0029, 0.0029), 0.0032, (0.0032, 0.0032)]	[(0.0027, 0.0027), 0.0030, (0.0030, 0.0030)]	[(0.0035, 0.0035), 0.0039, (0.0039, 0.0039)]	[(0.0051, 0.0051), 0.0056, (0.0056, 0.0056)]	[(0.0011, 0.0019), 0.0027, (0.0032, 0.0036)]	[(0.0071, 0.0071), 0.0071, (0, 0)]	[(0.0098, 0.0098), 0.0098, (0, 0)]
Project #1	[(0.0036, 0.0040), 0.0049, (0.0052, 0.0052)]	[(0.0040, 0.0040), 0.0051, (0.0057, 0.0057)]	[(0.0010, 0.0019), 0.0026, (0.0030, 0.0034)]	[(0.0029, 0.0029), 0.0032, (0.0032, 0.0032)]	[(0, 0), 0.0009, (0.0016, 0.0021)]	[(0.0012, 0.0012), 0.0019, (0.0027, 0.0027)]	[(0.0051, 0.0051), 0.0056, (0.0056, 0.0056)]	[(0.0011, 0.0019), 0.0027, (0.0032, 0.0036)]	[(0.0067, 0.0067), 0, (0, 0)]	[(0, 0), 0, (0, 0)]
Project #2	[(0.0016, 0.0016), 0.0026, (0.0036, 0.0036)]	[(0.0051, 0.0051), 0.0057, (0.0057, 0.0057)]	[(0, 0), 0.0010, (0.0018, 0.0024)]	[(0.0010, 0.0017), 0.0024, (0.0029, 0.0032)]	[(0.0009, 0.0016), 0.0022, (0.0026, 0.0030)]	[(0.0027, 0.0027), 0.0035, (0.0039, 0.0039)]	[(0.0017, 0.0030), 0.0042, (0.0050, 0.0056)]	[(0, 0), 0.0004, (0.0011, 0.0011)]	[(0.0114, 0.0114), 0.0114, (0, 0)]	[(0.0158, 0.0158), 0, (0, 0)]
Project #3	[(0.0016, 0.0016), 0.0026, (0.0036, 0.0036)]	[(0.0040, 0.0040), 0.0051, (0.0057, 0.0057)]	[(0.0024, 0.0028), 0.0033, (0.0034, 0.0034)]	[(0.0010, 0.0018), 0.0025, (0.0029, 0.0032)]	[(0.0009, 0.0016), 0.0022, (0.0026, 0.0030)]	[(0.0012, 0.0012), 0.0019, (0.0027, 0.0027)]	[(0, 0), 0.0010, (0.0022, 0.0040)]	[(0, 0), 0.0004, (0.0011, 0.0011)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]
Project #4	[(0, 0), 0, (0.0011, 0.0016)]	[(0, 0), 0.0020, (0.0034, 0.0057)]	[(0, 0), 0.0012, (0.0020, 0.0034)]	[(0, 0), 0.0011, (0.0019, 0.0032)]	[(0.0009, 0.0012), 0.0018, (0.0023, 0.0030)]	[(0, 0), 0.0017, (0.0026, 0.0039)]	[(0.0040, 0.0047), 0.0055, (0.0056, 0.0056)]	[(0.0011, 0.0015), 0.0022, (0.0029, 0.0036)]	[(0, 0), 0, (0, 0)]	[(0.0109, 0.0109), 0.0109, (0, 0)]
Project #5	[(0.0036, 0.0043), 0.0050, (0.0052, 0.0052)]	[(0, 0), 0, (0.0021, 0.0040)]	[(0.0010, 0.0010), 0.0017, (0.0024, 0.0024)]	[(0.0010, 0.0017), 0.0024, (0.0029, 0.0032)]	[(0, 0), 0.0005, (0.0012, 0.0021)]	[(0.0027, 0.0027), 0.0035, (0.0039, 0.0039)]	[(0.0040, 0.0040), 0.0051, (0.0056, 0.0056)]	[(0.0011, 0.0011), 0.0018, (0.0026, 0.0026)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]
Project #6	[(0, 0), 0.0015, (0.0027, 0.0036)]	[(0.0051, 0.0051), 0.0057, (0.0057, 0.0057)]	[(0, 0), 0.0003, (0.0010, 0.0010)]	[(0.0023, 0.0027), 0.0031, (0.0032, 0.0032)]	[(0.0027, 0.0027), 0.0030, (0.0030, 0.0030)]	[(0.0035, 0.0035), 0.0039, (0.0039, 0.0039)]	[(0, 0), 0.0017, (0.0030, 0.0040)]	[(0, 0), 0.0004, (0.0011, 0.0011)]	[(0.0100, 0.0100), 0, (0, 0)]	[(0, 0), 0, (0, 0)]
Project #7	[(0.0036, 0.0040), 0.0049, (0.0052, 0.0052)]	[(0.0040, 0.0040), 0.0051, (0.0057, 0.0057)]	[(0.0024, 0.0024), 0.0031, (0.0034, 0.0034)]	[(0, 0), 0.0006, (0.0013, 0.0023)]	[(0.0021, 0.0021), 0.0027, (0.0030, 0.0030)]	[(0.0012, 0.0012), 0.0019, (0.0027, 0.0027)]	[(0, 0), 0, (0.0021, 0.0040)]	[(0, 0), 0, (0.0008, 0.0011)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]
Code	C3	C4	C5	D1	D2	D3	D4	D5	E	F
Weight	0.019	0.047	0.014	0.064	0.080	0.046	0.031	0.091	0.237	0.067
Sign	−	−	+	+	−	−	−	+	+	−
Ideal Alternative (X0)	[(0.0097, 0.0097), 0, (0, 0)]	[(0.0093, 0.0093), 0, (0, 0)]	[(0.0017, 0.0017), 0.0019, (0.0019, 0.0019)]	[(0.0078, 0.0078), 0.0086, (0.0086, 0.0086)]	[(0.0127, 0.0127), 0.0127, (0, 0)]	[(0.0068, 0.0068), 0.0068, (0, 0)]	[(0.0092, 0.0092), 0.0092, (0, 0)]	[(0.0134, 0.0134), 0.0149, (0.0149, 0.0149)]	[(0.0334, 0.0334), 0.0371, (0.0371, 0.0371)]	[(0.0198, 0.0198), 0.0198, (0, 0)]
Project #1	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.0013, 0.0015), 0.0018, (0.0019, 0.0019)]	[(0.0060, 0.0066), 0.0080, (0.0086, 0.0086)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.0105, 0.0114), 0.0139, (0.0149, 0.0149)]	[(0, 0), 0.0077, (0.0166, 0.0371)]	[(0.0188, 0.0188), 0, (0, 0)]
Project #2	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.0006, 0.0006), 0.0010, (0.0013, 0.0013)]	[(0.0060, 0.0066), 0.0080, (0.0086, 0.0086)]	[(0.0205, 0.0205), 0, (0, 0)]	[(0.0109, 0.0109), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.0134, 0.0134), 0.0149, (0.0149, 0.0149)]	[(0.0260, 0.0260), 0.0334, (0.0371, 0.0371)]	[(0, 0), 0, (0, 0)]
Project #3	[(0.0092, 0.0092), 0, (0, 0)]	[(0.0088, 0.0088), 0, (0, 0)]	[(0.0017, 0.0017), 0.0019, (0.0019, 0.0019)]	[(0, 0), 0.0025, (0.0046, 0.0060)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.0088, 0.0088), 0.0088, (0, 0)]	[(0, 0), 0.0015, (0.0045, 0.0045)]	[(0, 0), 0, (0.0053, 0.0111)]	[(0, 0), 0, (0, 0)]
Project #4	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.0013, 0.0013), 0.0017, (0.0019, 0.0019)]	[(0.0060, 0.0071), 0.0083, (0.0086, 0.0086)]	[(0.0141, 0.0141), 0, (0, 0)]	[(0.0075, 0.0075), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.0105, 0.0114), 0.0139, (0.0149, 0.0149)]	[(0, 0), 0.0132, (0.0220, 0.0371)]	[(0, 0), 0, (0, 0)]
Project #5	[(0, 0), 0, (0, 0)]	[(0.0154, 0.0154), 0, (0, 0)]	[(0.0013, 0.0013), 0.0017, (0.0019, 0.0019)]	[(0.0026, 0.0046), 0.0064, (0.0077, 0.0086)]	[(0, 0), 0, (0, 0)]	[(0.0112, 0.0112), 0.0112, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0.0015, 0.0015)]	[(0.0334, 0.0334), 0.0371, (0.0371, 0.0371)]	[(0, 0), 0, (0, 0)]
Project #6	[(0, 0), 0, (0, 0)]	[(0.0132, 0.0132), 0, (0, 0)]	[(0, 0), 0.0006, (0.0010, 0.0013)]	[(0, 0), 0, (0.0024, 0.0060)]	[(0.0181, 0.0181), 0.0181, (0, 0)]	[(0.0096, 0.0096), 0, (0, 0)]	[(0.0131, 0.0131), 0, (0, 0)]	[(0, 0), 0.0044, (0.0079, 0.0105)]	[(0, 0), 0.0080, (0.0166, 0.0371)]	[(0.0281, 0.0281), 0.0281, (0, 0)]
Project #7	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0.0013, 0.0013), 0.0017, (0.0019, 0.0019)]	[(0.0078, 0.0078), 0.0086, (0.0086, 0.0086)]	[(0.0149, 0.0149), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0, (0, 0)]	[(0, 0), 0.0053, (0.0089, 0.0149)]	[(0, 0), 0, (0.0037, 0.0037)]	[(0, 0), 0, (0, 0)]

.

Following the fourth step of the ARAS method and using Equation (44), the values of S, presented as interval-valued fuzzy numbers, were calculated. To perform the fifth step of the ARAS method and compute the Q values, we have to convert the interval-valued fuzzy numbers of S to Crisp numbers by using Equations (23)–(26). The conversion and calculations for each alternative are displayed for different values of λ in

Table 15. Final ARAS calculations with interval values and Alternative rankings.

Alternatives	S	λ = 0			λ = 0.5			λ = 1
		BNP	Q	Rank	BNP	Q	Rank	BNP	Q	Rank
Ideal Alternative	[(0.167, 0.169), 0.160, (0.096, 0.096)]	0.144	1	0	0.142	1	0	0.161	1	0
Project #1	[(0.062, 0.066),0.058, (0.072, 0.094)]	0.073	0.505	4	0.069	0.485	4	0.066	0.411	4
Project #2	[(0.118, 0.121), 0.091, (0.089, 0.090)]	0.101	0.704	1	0.010	0.705	1	0.094	0.581	1
Project #3	[(0.039, 0.041),0.034, (0.041, 0.050)]	0.043	0.2959	7	0.040	0.281	7	0.038	0.238	7
Project #4	[(0.056, 0.060), 0.064, (0.069, 0.093)]	0.070	0.483	5	0.068	0.477	5	0.066	0.409	5
Project #5	[(0.077, 0.081), 0.076, (0.074, 0.078)]	0.076	0.528	3	0.077	0.545	3	0.079	0.489	2
Project #6	[(0.106, 0.106), 0.079, (0.051, 0.080)]	0.080	0.553	2	0.083	0.590	2	0.068	0.420	3
Project #7	[(0.037, 0.038), 0.034, (0.047, 0.056)]	0.045	0.310	6	0.041	0.290	6	0.039	0.244	6

.

As seen, for different values of λ, Project #2 can be selected as the ideal alternative. The analytical discussion confirms its higher score for the criteria such as the quality of materials and goods used, scientific levels of drilling specialists working in the project, as well as the actual cost compliance percentage with planned cost.

5. Conclusions and Recommendations

The necessity of project performance evaluation is crucial and undeniable which helps projects identify weaknesses and strengths as well as recognize and improve inefficiencies. Therefore several project performance evaluation approach has been used and advanced over the years that MCDM models is one of them. As a result, due to the complexity of the projects, and in order to achieve a better imagination of environmental ambiguities and overcome the inherent uncertainty of the projects, in this study, an Interval-Valued Fuzzy Additive Ratio Assessment (ARAS) Method proposed as a novel MCDM approach for evaluating the projects. On the other hand, despite the importance and certain position of oil and gas well drilling projects as well as its impact on the economies of countries, no structured assessment methodology has been presented for these types of projects and research works show the lack of literature in this field. So, the oil and gas well drilling projects selected as a case study. Given the limited research on performance evaluation in the context of such operations, the initial list of criteria and sub-criteria of evaluation obtained from available literature, was improved using the Fuzzy Delphi method. In order to determine criteria weights, the importance value of each final criterion was calculated through SWARA method based on expert opinions. As the performance scores of projects were determined with respect to defined criteria, a set of seven Iranian oil and gas projects were ranked by using interval-valued fuzzy ARAS method. The results revealed six general assessment criteria for oil and gas well-drilling projects including: number of planned wells, number of drilled wells, materials & equipment, Human resource, quality and planning. However, the third to sixth criteria consist of several sub-criteria among which the number of drilled wells, actual cost compliance percentage with planned costs and percentage of waiting time to total well drilling time were defined as the most important sub-criteria. From the projects being studied, Project #2 was defined as the best alternative due to its distinguished performance in terms of sub-criteria of type of drilling rigs, number of operational experts working on the project, scientific levels of drilling specialists working in the project, type of drilled wells in terms of operational risk and actual cost compliance percentage with planned cost. Rather, Project #3 was considered as the weakest alternative. The managerial investigation of Project #2 showed that management stability, along with the use of new methods for employee assessment, as well as the implementation of knowledge management methods had significant role in the success of that project, while Project #3 presented frequently-changed management. The innovations of this study include the development of a comprehensive list of criteria and sub-criteria in performance evaluation of oil and gas well-drilling projects by using Fuzzy Delphi, SWARA and interval-valued fuzzy ARAS methods.

As mentioned before, there are unpredictable issues in the process of conducting research that are one of the sources of uncertainties having been emphasized in the literature. It seems that stochastic MADM methods which have been considered in the recent year’s literature can be used in future researches. On the other hand, performing drilling projects is time-consuming and the evaluation of options and the criteria weights may change over time due to changes in both the macro environment and the preferences and the priorities of the experts. Hence, the use of the prospective MADM method is recommended to better cover possible changes. Also, in future researches, we can use aggregate operators to integrate experts’ opinions and hesitant fuzzy sets to further investigate the sensitive analysis of decisions which are taken.

Finally, we assumed that identified criteria are independent from each other. Future research can examine the existence of this dependency and in case of confirmation of the relationship between criteria; they can use appropriate methods (Like ANP, DEMATEL and ISM).