A Prediction Method for the Damping Effect of Ring Dampers Applied to Thin-Walled Gears Based on Energy Method
Abstract
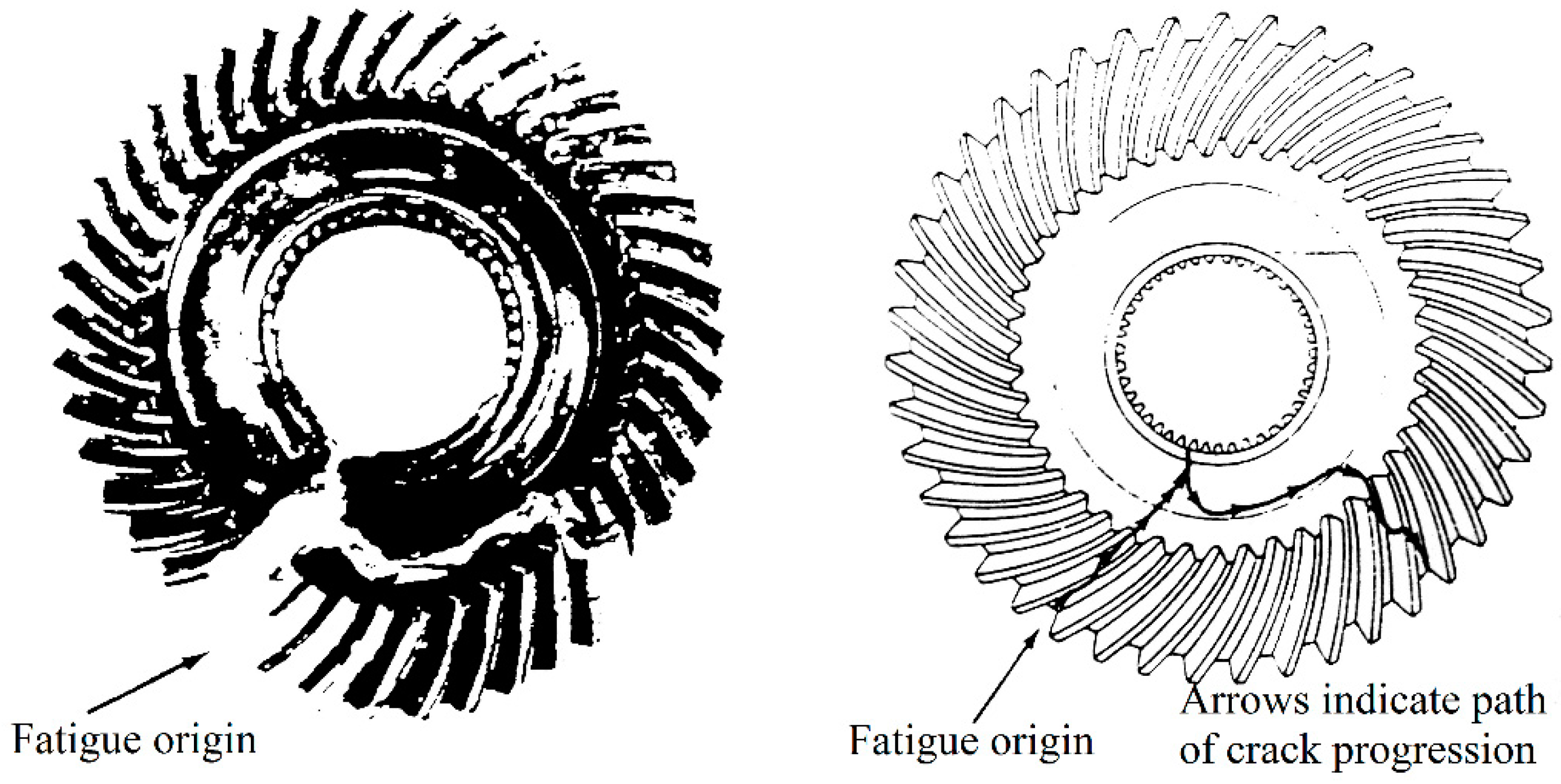
1. Introduction
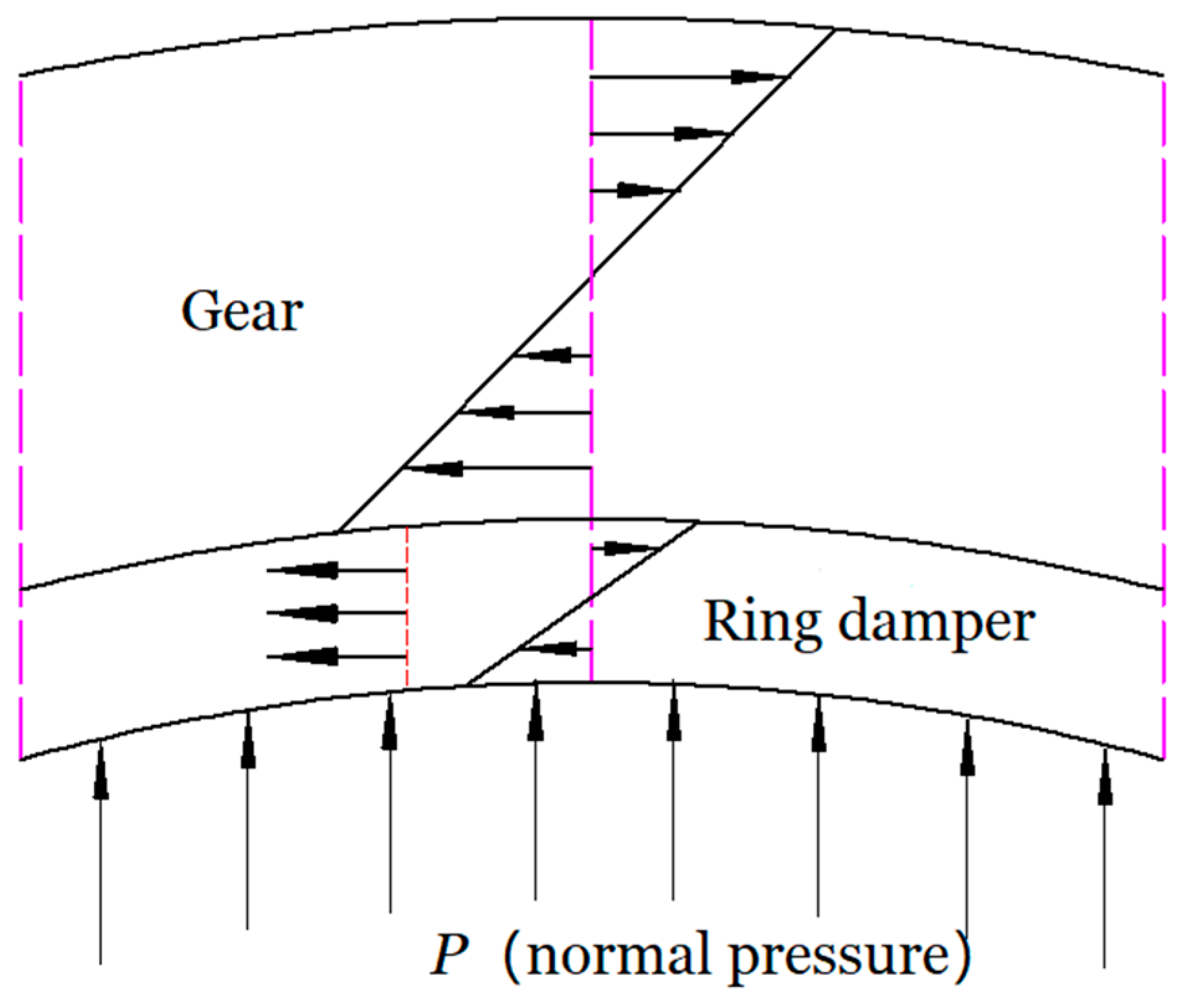
2. Vibration Analysis of The Gear-Ring Damper System
2.1. The Equations of Motion
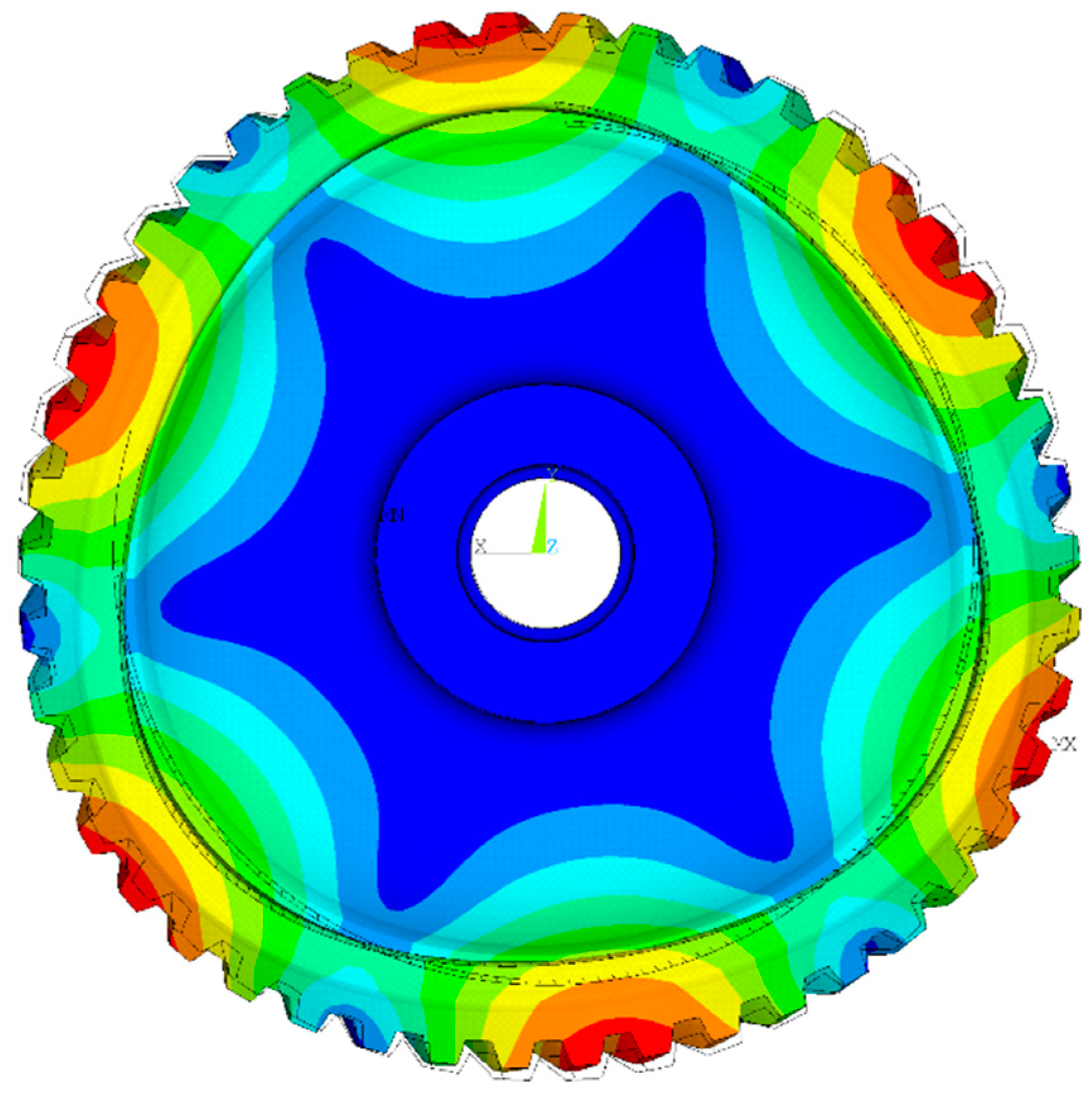
2.2. Modal Analysis
- The modal amplitude has an integer number of harmonic distributions along the circumferential direction.
- The nodal line passes through the center of rotation, and the vibration amplitude of the nodal line is zero.
- For thin-walled gears, the gear rim vibrates mainly in the radial direction.
3. Theoretical Model of Equivalent Damping Ratio of The Ring Damper
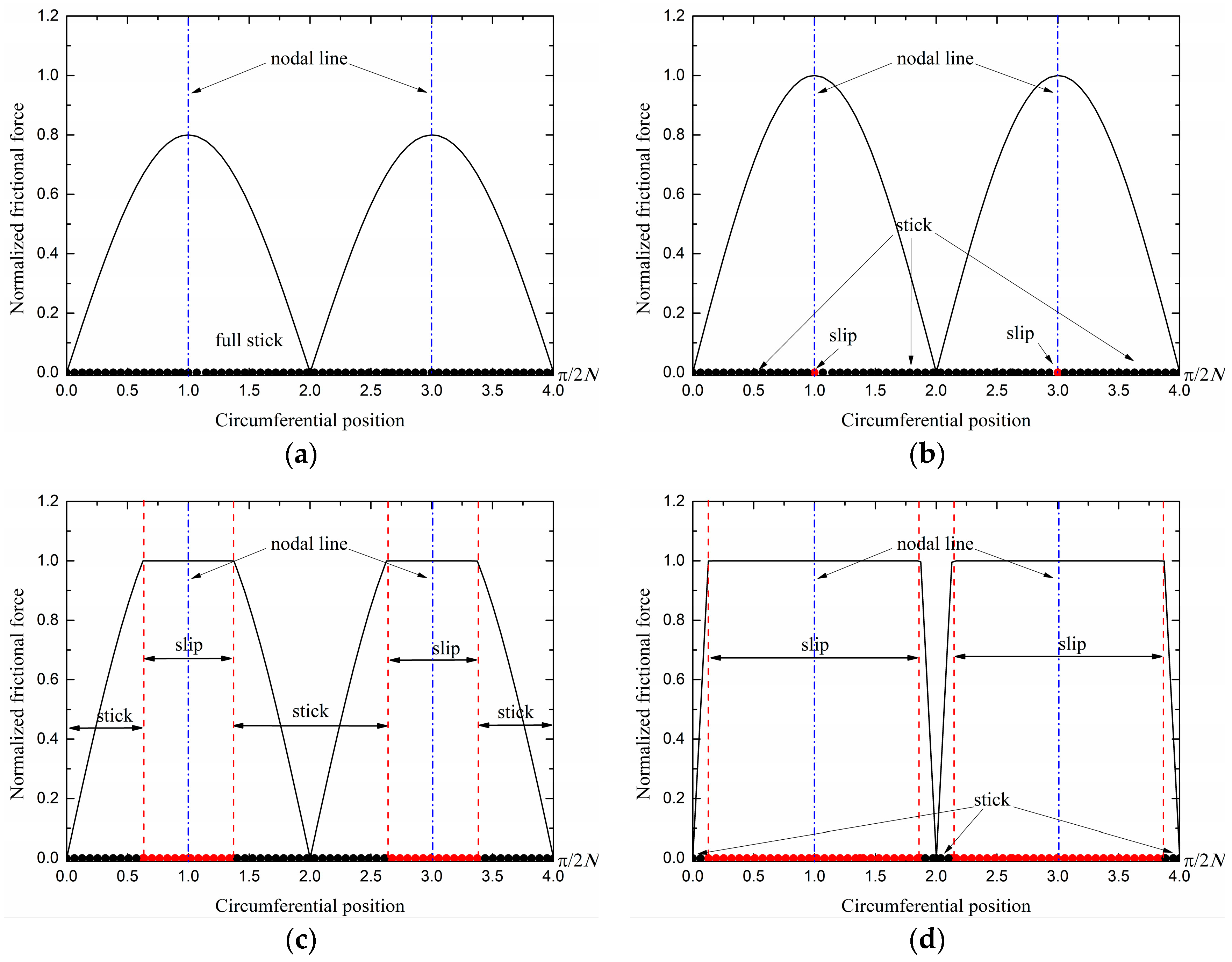
3.1. Energy Dissipated by Frictional Force
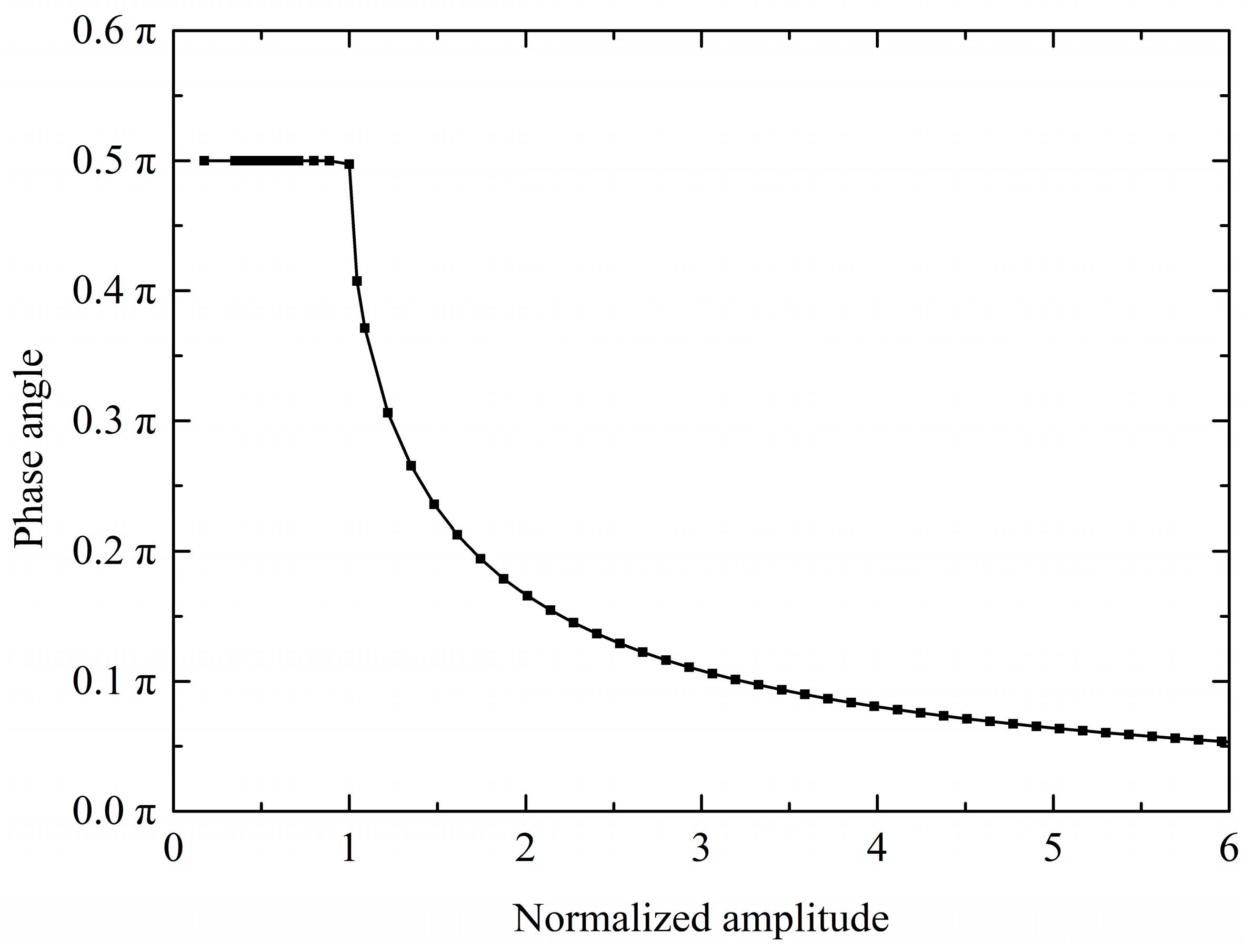
3.2. Equivalent Damping Ratio
4. Application and Discussion
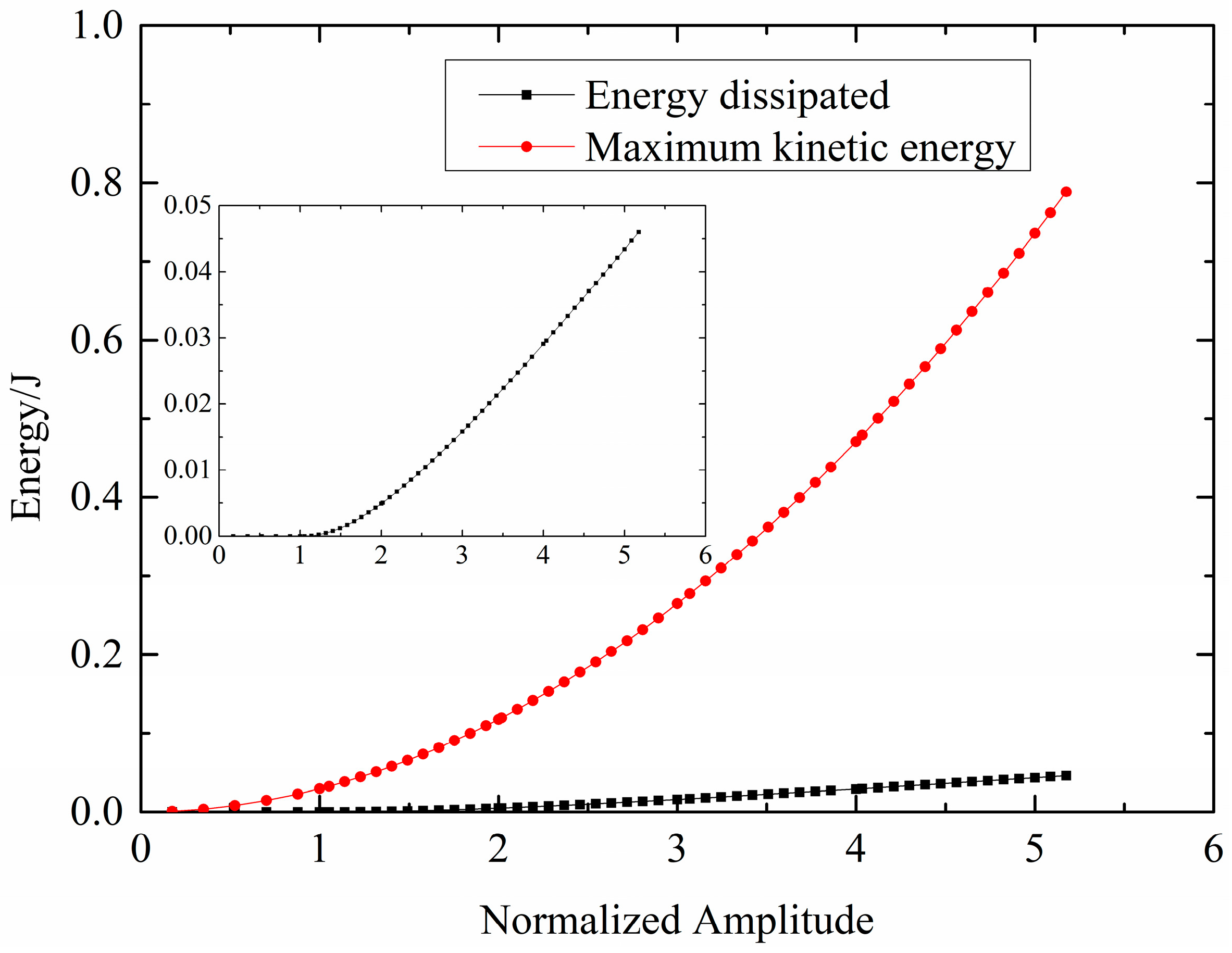
4.1. Method Validation
4.2. Effect of Ring Damper Parameters
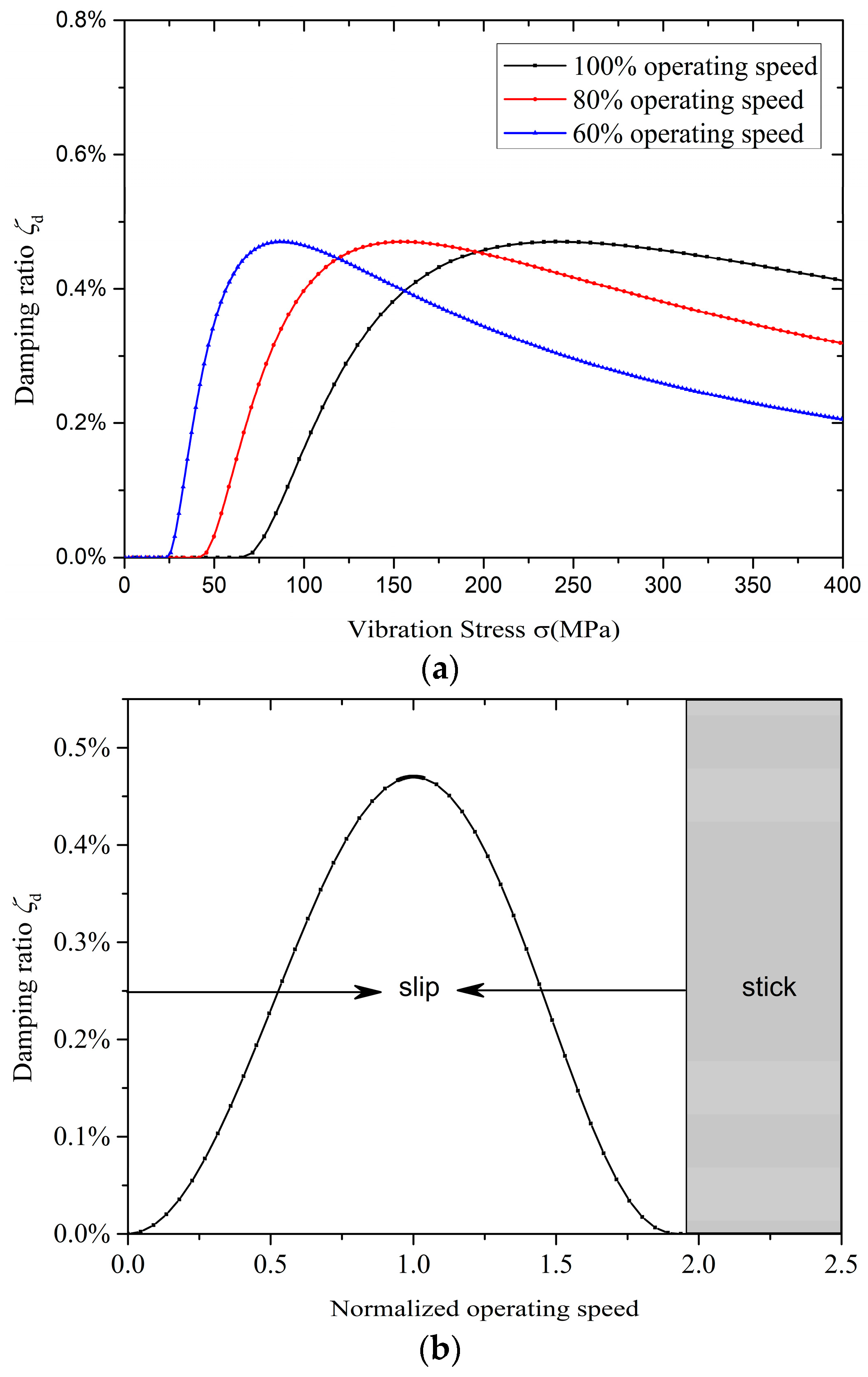
4.2.1. Effect of Rotating Speed or Normal Pressure
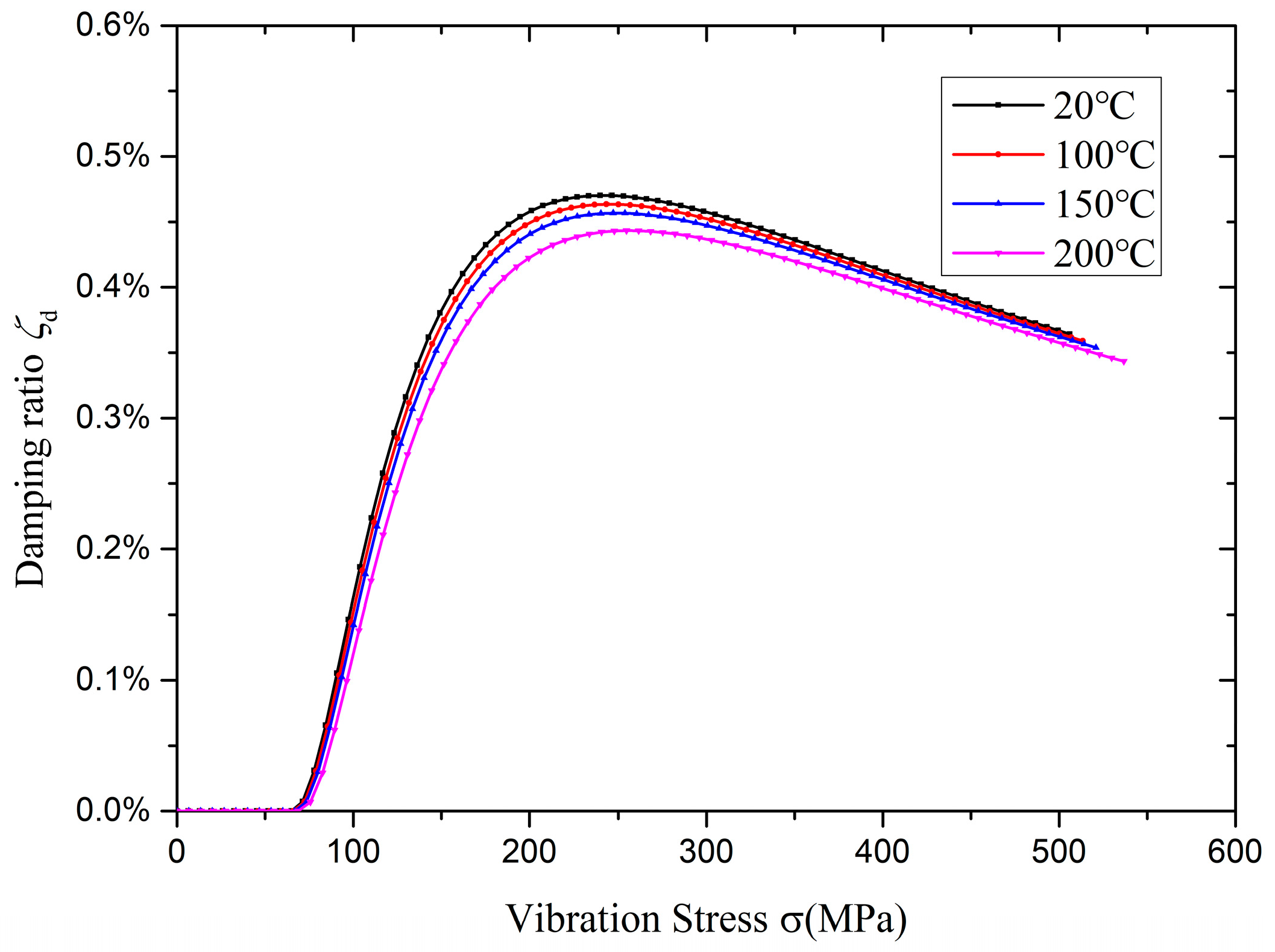
4.2.2. Effect of Temperature
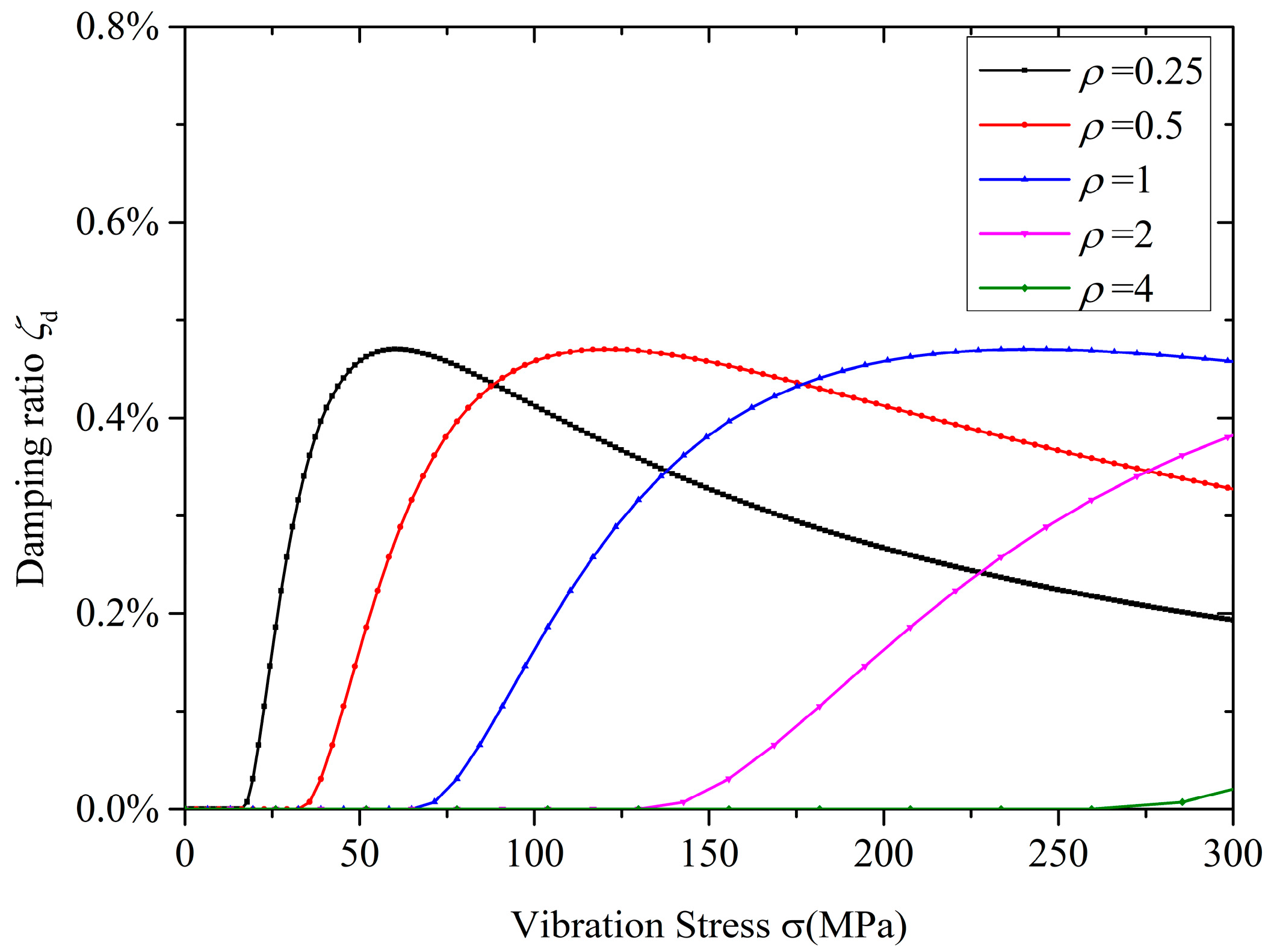
4.2.3. Effect of the Ring Damper Density
4.2.4. Effect of the Friction Coefficient
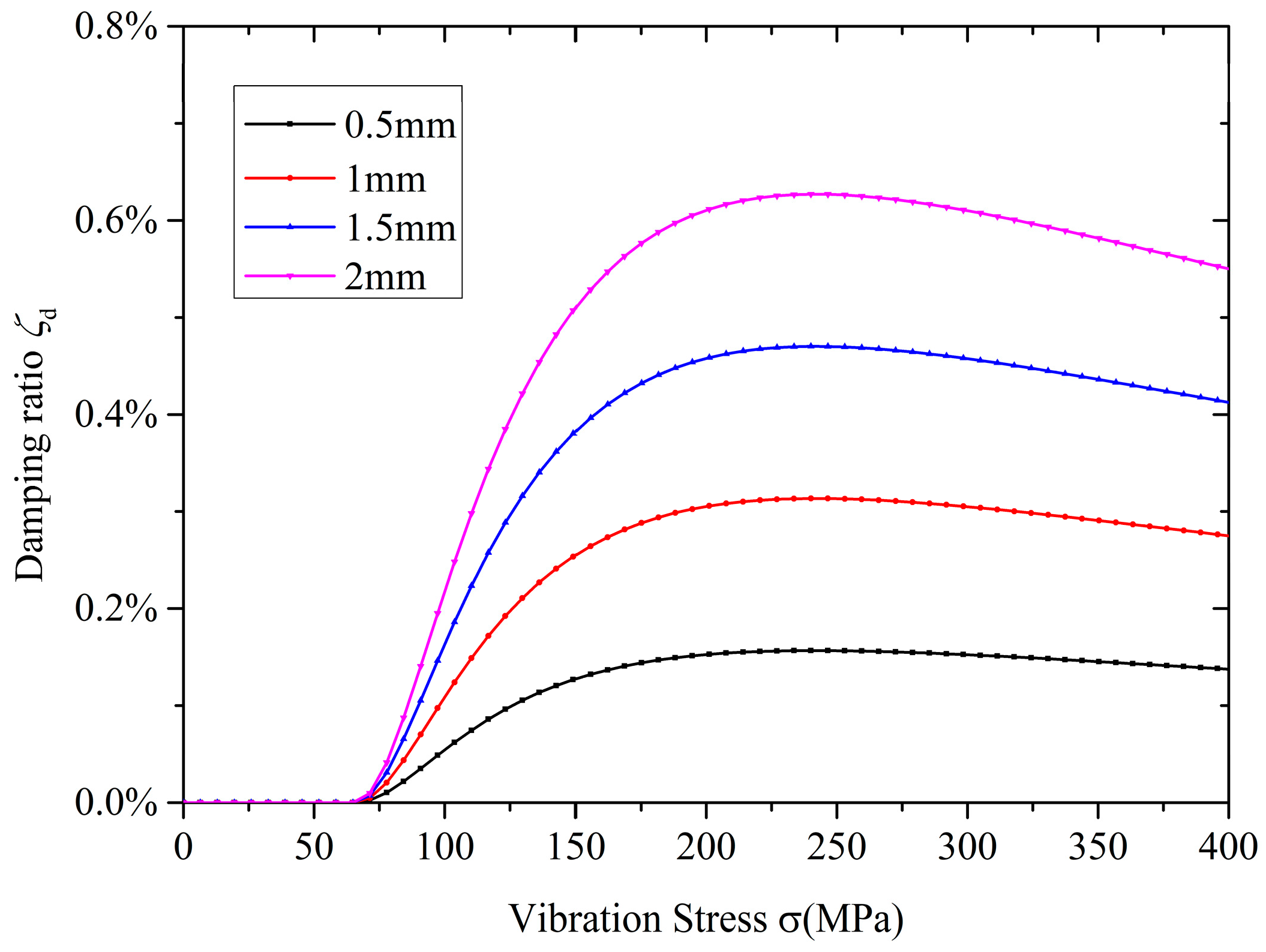
4.2.5. Effect of the Cross-Sectional Area of the Ring Damper
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Notation
| B | vibration amplitude | Subscript g | gear |
| Bc | critical vibration amplitude | Subscript d | ring damper |
| C | damping matrices of the gear | Subscript eq | equivalent |
| c | half-width of the gear rim or the ring damper | W | total energy of the system |
| E | Young’s modulus | w | radial displacement of the groove |
| F(t) | external periodic force | X | displacement vector |
| nonlinear frictional force | z | number of teeth of the gear | |
| Ff | frictional force per unit length | ε | strain |
| I | sectional moment of inertia | η | loss coefficient |
| K | stiffness matrices of the gear | κ | curvature |
| M | mass matrices of the gear | μ | friction coefficient |
| M | bending moment | θ | circumferential angle |
| N | number of nodal diameters | θ0 | critical slip angle |
| P | normal pressure | ρ | density of the ring damper |
| P’ | normalized normal pressure | ζ | damping ratio |
| R | radius | ζeq | equivalent damping ratio provided by the ring damper |
| s | relative displacement | ΔW | energy dissipated per cycle by the ring damper |
References
- Drago, R.J.; Brown, F.W. The analytical and experimental evaluation of resonant response in high-speed, lightweight, highly loaded gearing. J. Mech. Des. 1981, 103, 346–356. [Google Scholar] [CrossRef]
- Griffin, J.H. Friction damping of resonant stresses in gas turbine engine airfoils. J. Eng. Power 1980, 102, 329–333. [Google Scholar] [CrossRef]
- Cameron, T.M.; Griffin, J.H.; Kielb, R.E. An integrated approach for friction damper design. J. Vib. Acoust. 1990, 112, 175–182. [Google Scholar] [CrossRef]
- Ferri, A.A. Friction damping and isolation systems. J. Vib. Acoust. 1995, 117, 196–206. [Google Scholar] [CrossRef]
- Wang, Y.; Fu, Z.; Jiang, X.; Tian, A. Mistuning effects on aero-elastic stability of axial compressor rotor blades. J. Eng. Gas Turbines Power 2015, 137, 102504. [Google Scholar] [CrossRef]
- Fu, Z.; Wang, Y.; Jiang, X.; Wei, D. Tip Clearance effects on aero-elastic stability of axial compressor blades. J. Eng. Gas Turbines Power 2015, 137, 012501. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Y.; Xu, K. Mechanisms and key parameters for compressor blade stall flutter. J. Turbomach. 2013, 135, 024501. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Y.; Xu, K. Flutter prediction in turbomachinery with energy method. J. Aerosp. Eng. 2011, 225, 995–1002. [Google Scholar] [CrossRef]
- Koh, K.H.; Griffin, J.H.; Filippi, S.; Akay, A. Characterization of turbine blade friction dampers. J. Eng. Gas Turbines Power 2005, 127, 856–862. [Google Scholar] [CrossRef]
- Petrov, E.P.; Ewins, D.J. Advanced modeling of underplatform friction dampers for analysis of bladed disk vibration. J. Turbomach. 2007, 129, 143–150. [Google Scholar] [CrossRef]
- Botto, D.; Gastadi, C.; Gola, M.M.; Umer, M. An experimental investigation of the dynamics of a blade with two under-platform dampers. J. Eng. Gas Turbines Power 2017, 140, 032504. [Google Scholar] [CrossRef]
- Botto, D.; Umer, M. A novel test rig to investigate under-platform damper dynamics. Mech. Syst. Signal. Pr. 2018, 100. [Google Scholar] [CrossRef]
- Pesaresia, L.; Sallesa, L.; Jonesb, A.; Greenb, J.S.; Schwingshackla, C.W. Modelling the nonlinear behaviour of an underplatform damper test rig for turbine applications. Mech. Syst. Signal. Pr. 2017. [Google Scholar] [CrossRef]
- Kaneko, Y. Vibration response analysis of mistuned bladed disk with under-platform damper: Effect of variation of contact condition on vibration characteristics. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017. [Google Scholar]
- Lopez, I.; Busturia, J.M.; Nijmeijer, H. Energy dissipation of a friction damper. J. Sound Vib. 2004, 278, 539–561. [Google Scholar] [CrossRef]
- Lopez, I.; Nijmeijer, H. Prediction and validation of the energy dissipation of a friction damper. J. Sound Vib. 2009, 328, 396–410. [Google Scholar] [CrossRef]
- Laxalde, D.; Thouverez, F.; Lombard, J.P. Forced response analysis of integrally bladed disks with friction ring dampers. J. Vib. Acoust. 2010, 132, 011013. [Google Scholar] [CrossRef]
- Laxalde, D.; Salles, L.; Blanc, L.; Thouverez, F. Non-linear modal analysis for bladed disks with friction contact interfaces. In Proceedings of the ASME Turbo Expo 2008: Power for Land, Sea, and Air. American Society of Mechanical Engineers, Berlin, Germany, 9–13 June 2008. [Google Scholar]
- Zucca, S.; Firrone, C.M.; Facchini, M. A method for the design of ring dampers for gears in aeronautical applications. J. Mech. Des. 2012, 134, 091003. [Google Scholar] [CrossRef]
- Tang, W.; Epureanu, B.I. Geometric optimization of dry friction ring dampers. Int. J. Nonlin. Mech. 2018. [Google Scholar] [CrossRef]
- Tang, W.; Epureanu, B.I. Nonlinear dynamics of mistuned bladed disks with ring dampers, International. Int. J. Nonlin. Mech. 2017, 97, 30–40. [Google Scholar] [CrossRef]
- Firrone, C.M.; Zucca, S. Passive control of vibration of thin-walled gears: Advanced modelling of ring dampers. Nonlinear Dyn. 2014, 76, 263–280. [Google Scholar] [CrossRef]
- Buyukataman, K. A theoretical study on the vibration damping of aircraft gearbox gears. In Proceedings of the 27th Joint Propulsion Conference, Sacramento, CA, USA, 24–26 June 1991. [Google Scholar]
- Niemotka, M.A.; Ziegert, J.C. Optimal design of split ring dampers for gas turbine engines. J. Eng. Gas Turbines Power 1995, 117, 569–575. [Google Scholar] [CrossRef]
- Baek, S.; Epureanu, B. Reduced-order modeling of bladed disks with friction ring dampers. J. Vib. Acoust. 2017, 139, 061011. [Google Scholar] [CrossRef]
- Tangpong, X.W.; Wickert, J.A.; Akay, A. Finite element model for hysteretic friction damping of traveling wave vibration in axisymmetric structures. J. Vib. Acoust. 2008, 130, 011005. [Google Scholar] [CrossRef]
- Tangpong, X.W.; Wickert, J.A.; Akay, A. Distributed friction damping of travelling wave vibration in rods. Philos. Trans. R. Soc. A 2008, 366, 811–827. [Google Scholar] [CrossRef] [PubMed]
- Alford, J.S. Protection of labyrinth seals from flexural vibration. J. Eng. Power 1964, 86, 141–147. [Google Scholar] [CrossRef]
- Alford, J.S. Protecting turbomachinery from self-excited rotor whirl. J. Eng. Power 1965, 87, 333–343. [Google Scholar] [CrossRef]
- Alford, J.S. Protecting turbomachinery from unstable and oscillatory flows. J. Eng. Power 1967, 89, 513–527. [Google Scholar] [CrossRef]
- Alford, J.S. Nature, causes, and prevention of labyrinth air seal failures. J. Aircraft 1975, 12, 313–318. [Google Scholar] [CrossRef]
- Weaver, J.W.; Timoshenko, S.P.; Young, D.H. Vibration Problems in Engineering, 5th ed.; Wiley-Interscience: Hoboken, NJ, USA, 1990; ISBN 9780471632283. [Google Scholar]
- Rao, S.S. Mechanical Vibrations, 5th ed.; Prentice Hall: New York, NY, USA, 1995; ISBN 978-0-13-212819-3. [Google Scholar]
- Tang, W.; Baek, S.; Epureanu, B.I. Reduced-order models for blisks with small and large mistuning and friction dampers. J. Eng. Gas Turbines Power 2017, 139, 012507. [Google Scholar] [CrossRef]





© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Ye, H.; Jiang, X.; Tian, A. A Prediction Method for the Damping Effect of Ring Dampers Applied to Thin-Walled Gears Based on Energy Method. Symmetry 2018, 10, 677. https://doi.org/10.3390/sym10120677
Wang Y, Ye H, Jiang X, Tian A. A Prediction Method for the Damping Effect of Ring Dampers Applied to Thin-Walled Gears Based on Energy Method. Symmetry. 2018; 10(12):677. https://doi.org/10.3390/sym10120677
Chicago/Turabian StyleWang, Yanrong, Hang Ye, Xianghua Jiang, and Aimei Tian. 2018. "A Prediction Method for the Damping Effect of Ring Dampers Applied to Thin-Walled Gears Based on Energy Method" Symmetry 10, no. 12: 677. https://doi.org/10.3390/sym10120677
APA StyleWang, Y., Ye, H., Jiang, X., & Tian, A. (2018). A Prediction Method for the Damping Effect of Ring Dampers Applied to Thin-Walled Gears Based on Energy Method. Symmetry, 10(12), 677. https://doi.org/10.3390/sym10120677



