Investigating the Compound Influence of Tidal and River Floodplain Discharge Under Storm Events in the Brisbane River Estuary, Australia
Abstract
1. Introduction
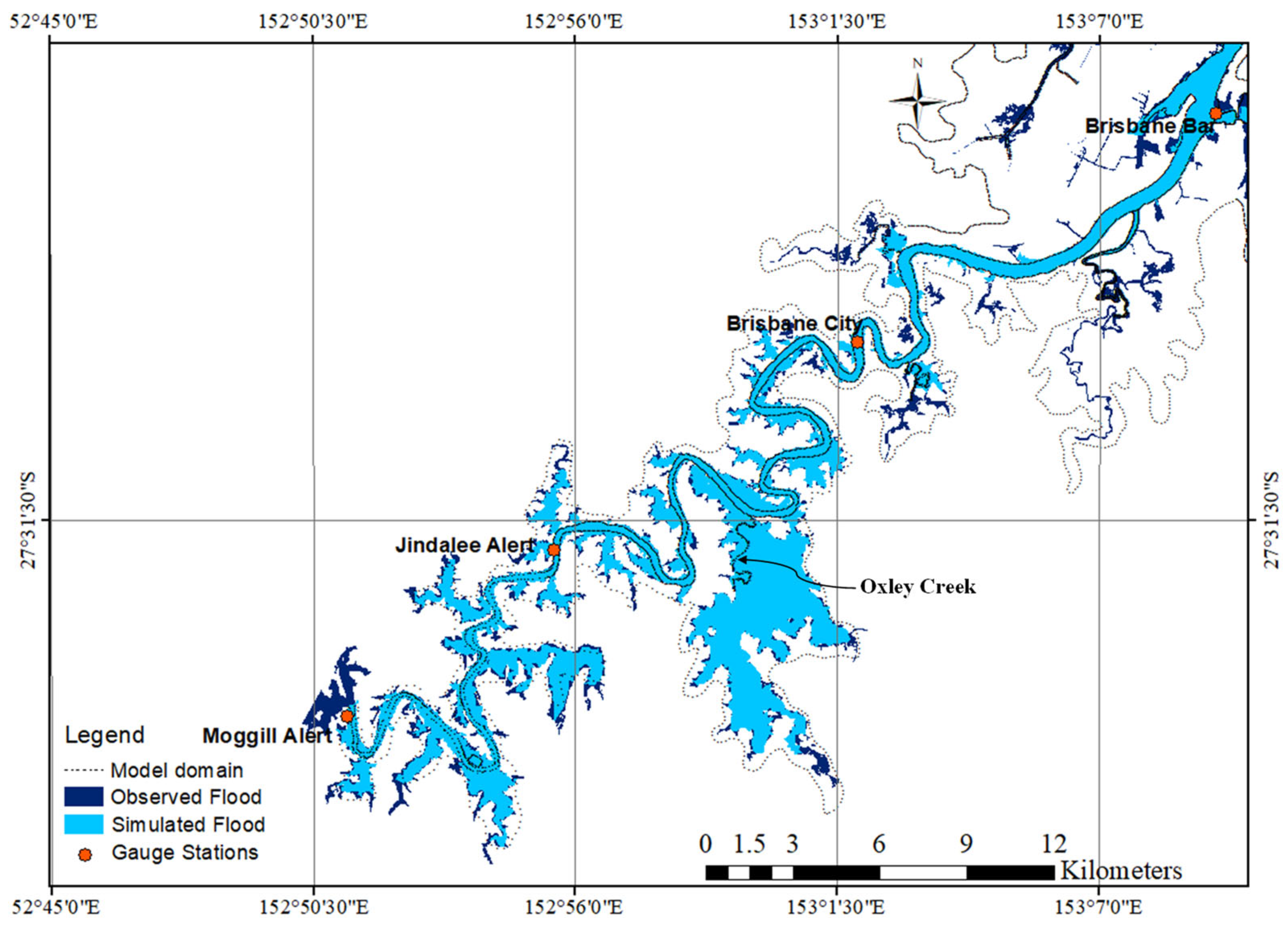
2. Study Area
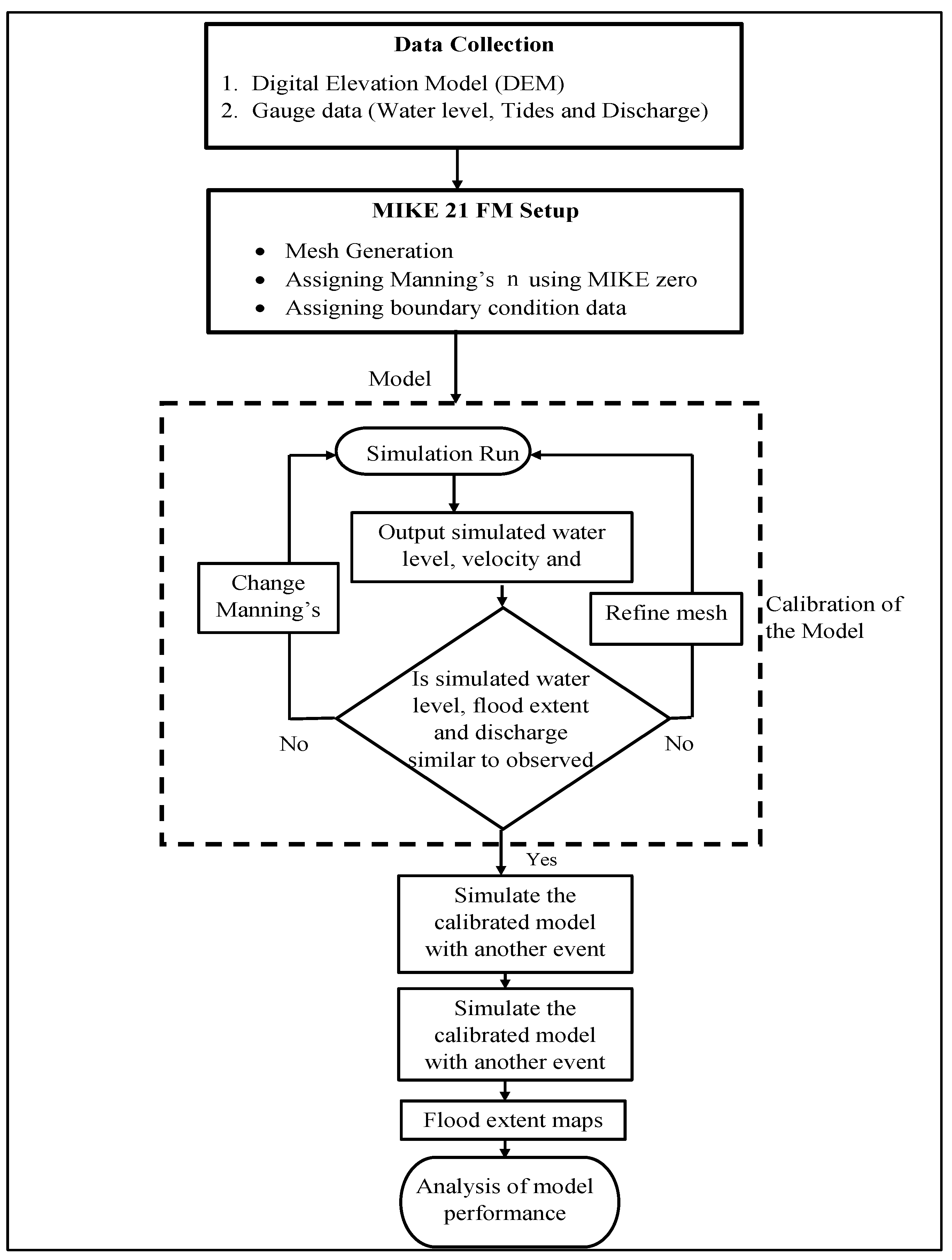
3. Materials and Methods
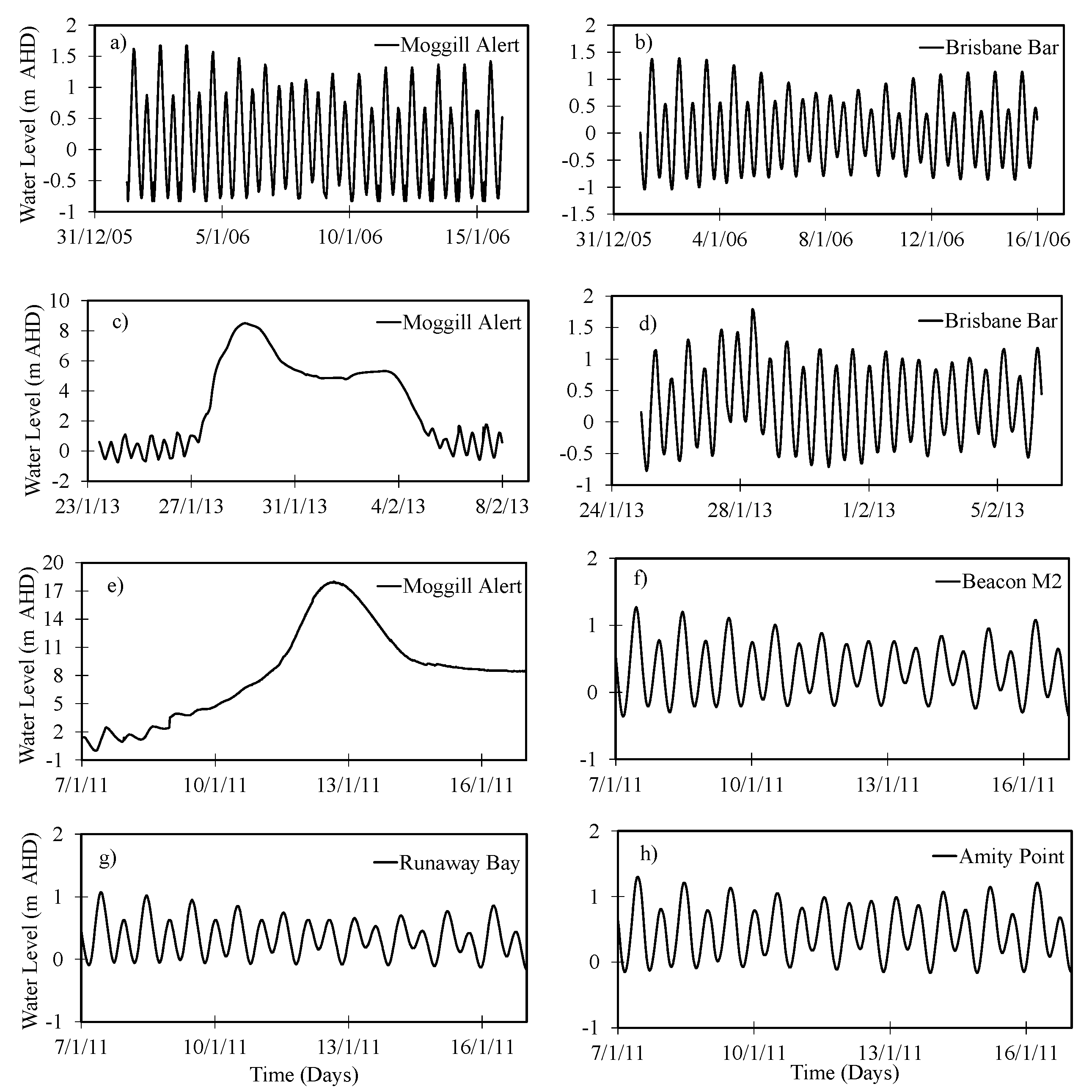
3.1. Data Collection
3.2. Hydrodynamic Model
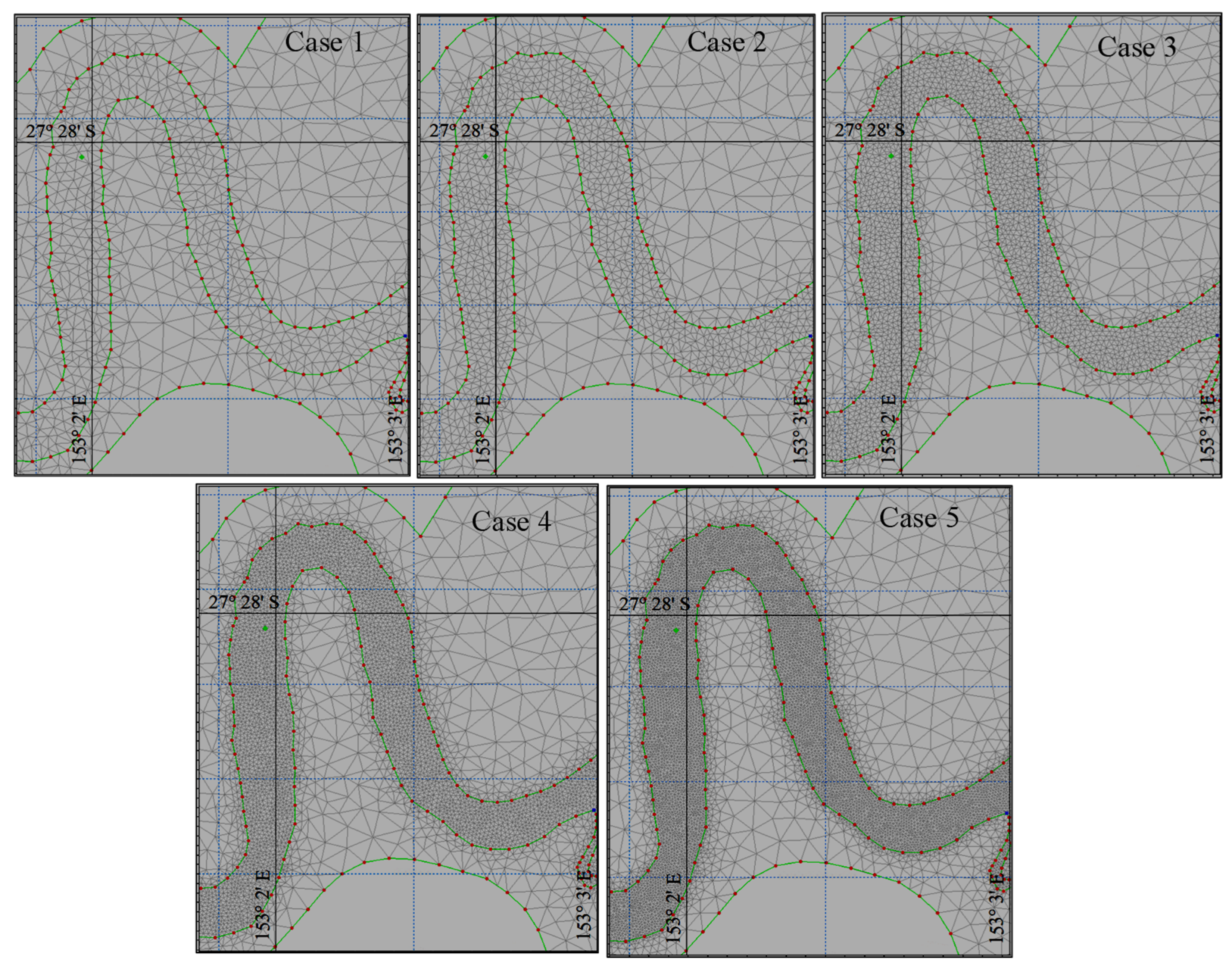
3.3. Mesh Generation and Cell Size Convergence
3.4. Future Scenarios of Storm Surge
3.5. Model Setup
3.6. Model Performance Evaluation Indices
4. Results and Discussion
4.1. Calibration and Validation of the Model
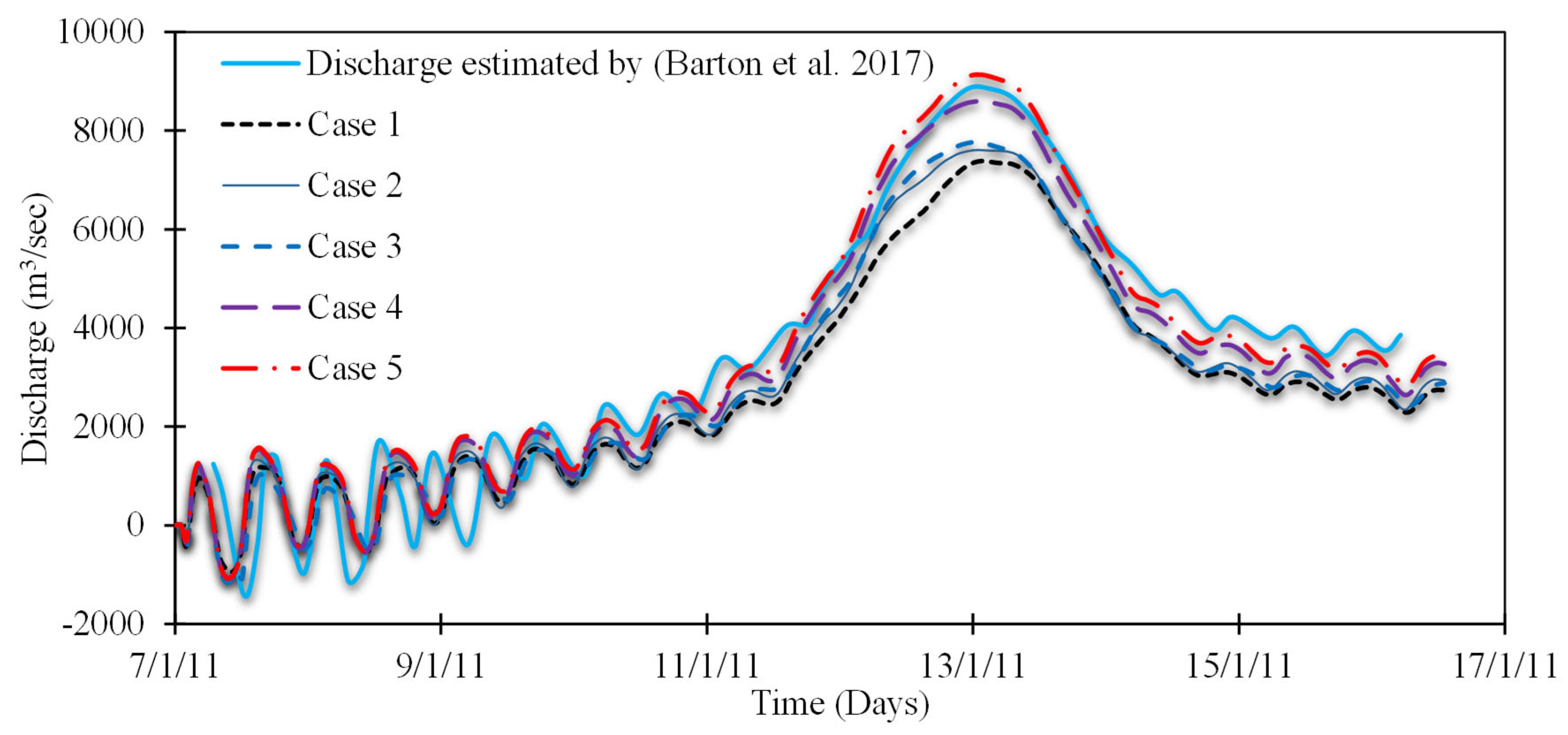
4.2. Mesh Resolution Effects on Discharge
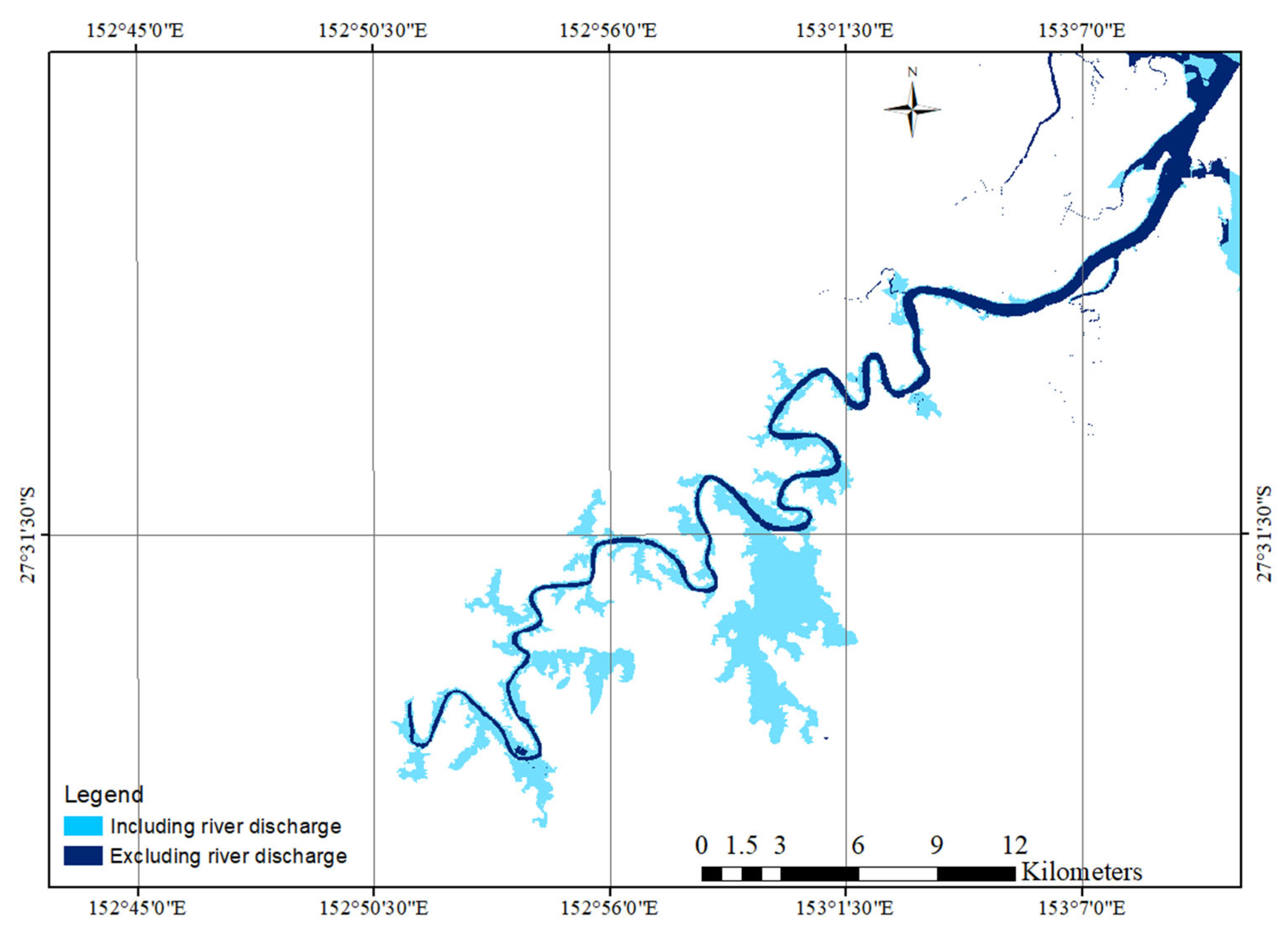
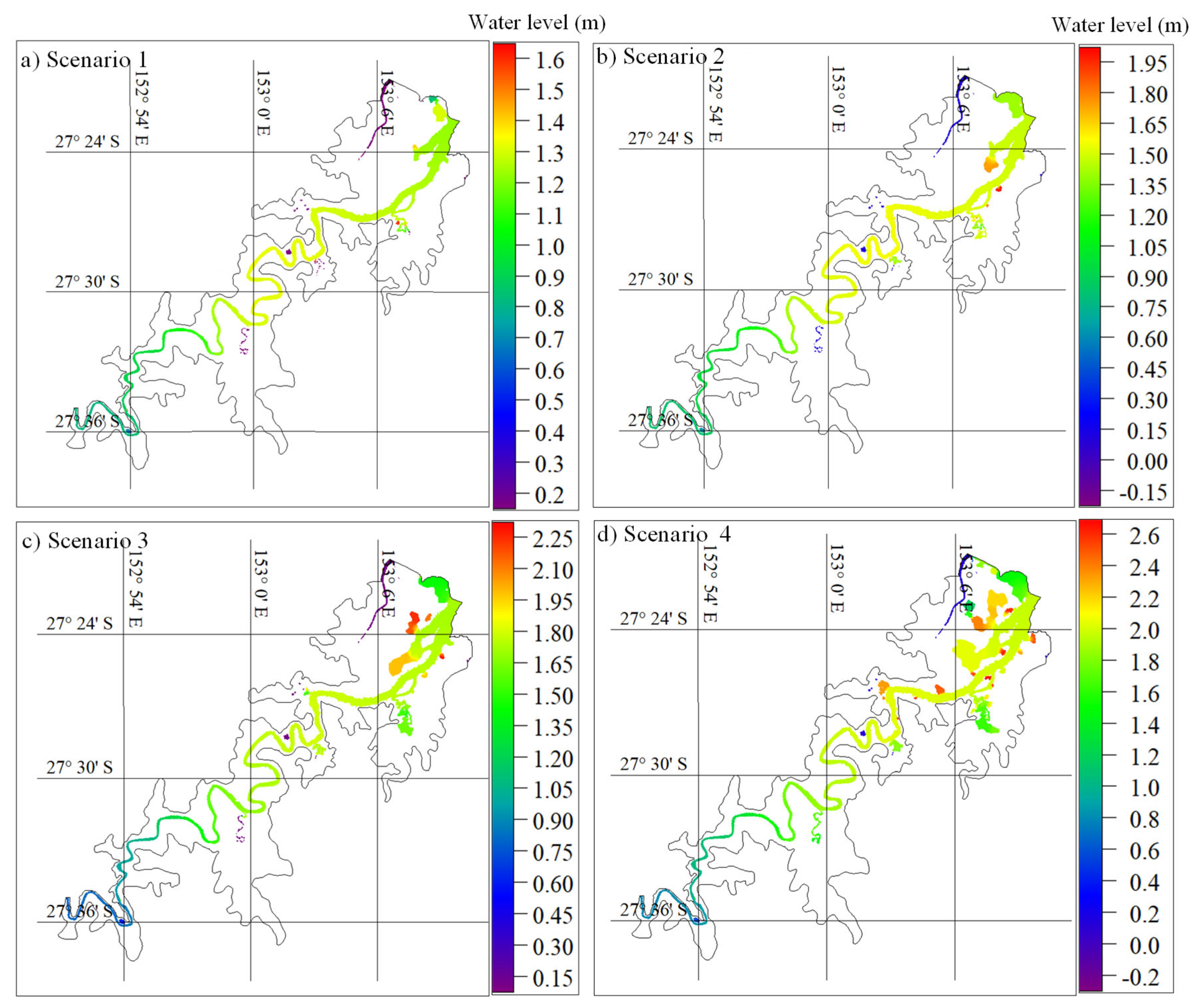
4.3. Modelled Water Levels and Flood Extents Under Varying Boundaries
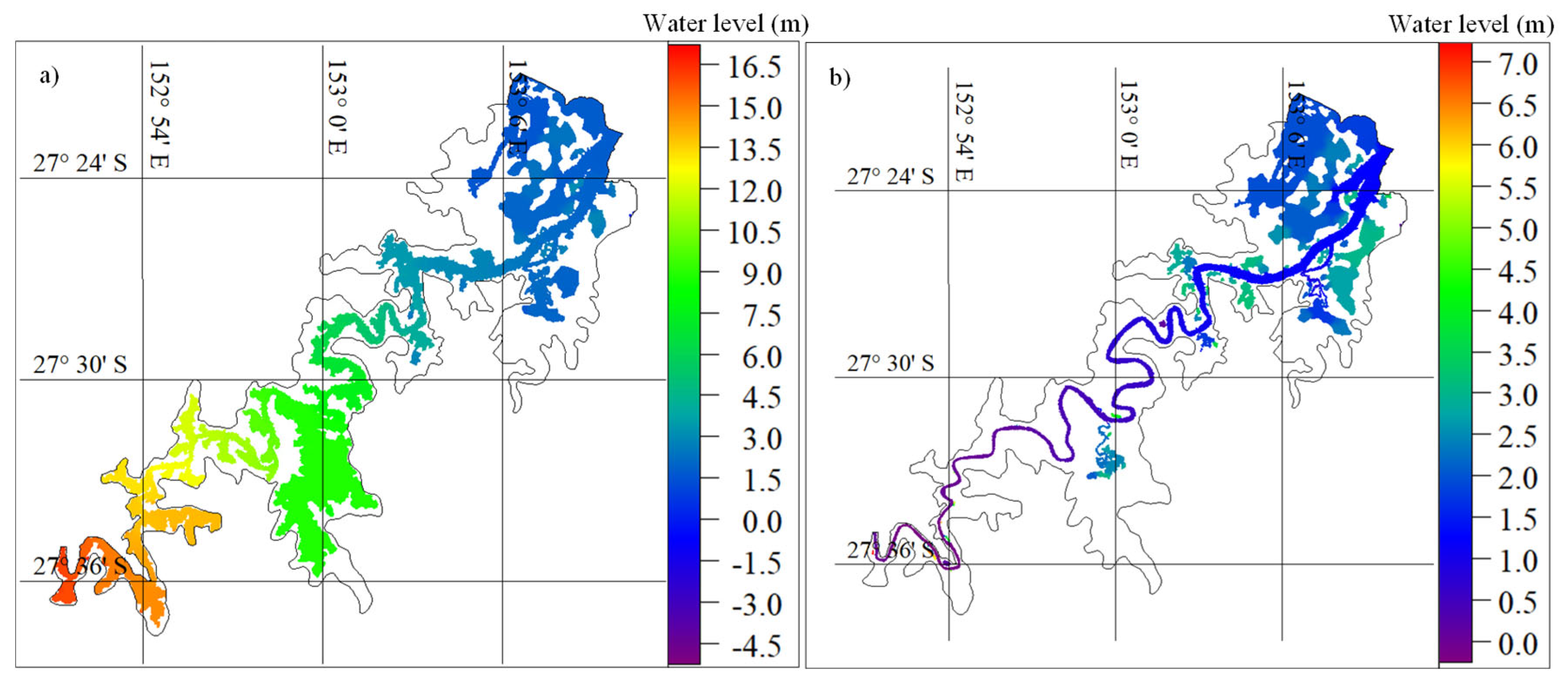
4.4. Modelled Flood Extents Under Future Storm Surge Cases
4.5. Discussion of Flood Extent Progression
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liu, X.; Lim, S. Flood Inundation Modelling for Mid-Lower Brisbane Estuary. River Res. Appl. 2017, 33, 415–426. [Google Scholar] [CrossRef]
- Khalil, U.; Khan, N.M. Floodplain Mapping for Indus River: Chashma–Taunsa Reach. Pak. J. Eng. Appl. Sci. 2017, 20, 30–48. [Google Scholar]
- Geravand, F.; Hosseini, S.M.; Ataie-Ashtiani, B. Influence of river cross-section data resolution on flood inundation modeling: Case study of Kashkan river basin in western Iran. J. Hydrol. 2020, 584, 124743. [Google Scholar] [CrossRef]
- Sadler, J.M.; Goodall, J.L.; Behl, M.; Bowes, B.D.; Morsy, M.M. Exploring real-time control of stormwater systems for mitigating flood risk due to sea level rise. J. Hydrol. 2020, 583, 124571. [Google Scholar] [CrossRef]
- Vitousek, S.; Barnard, P.L.; Fletcher, C.H.; Frazer, N.; Erikson, L.; Storlazzi, C.D. Doubling of coastal flooding frequency within decades due to sea-level rise. Sci. Rep. 2017, 7, 1399. [Google Scholar] [CrossRef]
- Pachauri, R.K.; Allen, M.R.; Barros, V.R.; Broome, J.; Cramer, W.; Christ, R.; Church, J.A.; Clarke, L.; Dahe, Q.; Dasgupta, P.; et al. Climate Change 2014: Synthesis Report. Contribution of Working Groups I, II and III to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; IPCC: Geneva, Switzerland, 2014.
- Van Coppenolle, R.; Temmerman, S. A global exploration of tidal wetland creation for nature-based flood risk mitigation in coastal cities. Estuarine. Coast. Shelf Sci. 2019, 226, 106262. [Google Scholar] [CrossRef]
- Hallegatte, S.; Green, C.; Nicholls, R.J.; Corfee-Morlot, J. Future flood losses in major coastal cities. Nat. Clim. Change 2013, 3, 802. [Google Scholar] [CrossRef]
- Tsoukala, V.K.; Chondros, M.; Kapelonis, Z.G.; Martzikos, N.; Lykou, A.; Belibassakis, K.; Makropoulos, C. An integrated wave modelling framework for extreme and rare events for climate change in coastal areas–the case of Rethymno, Crete. Oceanologia 2016, 58, 71–89. [Google Scholar] [CrossRef]
- McCallum, I.; Liu, W.; See, L.; Mechler, R.; Keating, A.; Hochrainer-Stigler, S.; Mochizuki, J.; Fritz, S.; Dugar, S.; Arestegui, M.; et al. Technologies to support community flood disaster risk reduction. Int. J. Disaster Risk Sci. 2016, 7, 198–204. [Google Scholar] [CrossRef]
- Sulis, A.; Frongia, S.; Liberatore, S.; Zucca, R.; Sechi, G.M. Combining water supply and flood control purposes in the Coghinas Basin (Sardinia, Italy). Int. J. River Basin Manag. 2018, 18, 13–22. [Google Scholar] [CrossRef]
- UNISDR. Global Assessment Report on Disaster Risk Reduction 2015: Making Development Sustainable: The Future of Disaster Risk Management; United Nations International Strategy for Disaster Reduction: Geneva, Switzerland, 2015. [Google Scholar]
- Lee, E.H.; Kim, J.H. Development of a flood-damage-based flood forecasting technique. J. Hydrol. 2018, 563, 181–194. [Google Scholar] [CrossRef]
- Syme, B. Executive Summary and Recommendations Brisbane River Strategic Floodplain Management Plan; BMT WBM: Brisbane, Australia, 2019. [Google Scholar]
- Barton, C.; Wallace, S.; Syme, B.; Wong, W.T.; Onta, P. Brisbane River catchment flood study: Comprehensive hydraulic assessment overview. In Proceedings of the Floodplain Management Association National Conference, Brisbane, Australia, 19–22 May 2015. [Google Scholar]
- van den Honert, R.C.; McAneney, J. The 2011 Brisbane floods: Causes, impacts and implications. Water 2011, 3, 1149–1173. [Google Scholar] [CrossRef]
- Rice, M.; Karoly, D.; Arndt, D.; Comer, J.; O’Rourke, S. Eye of the Storm: How Climate Pollution Fuels More Intense and Destructive Cyclones; Climate Council: London, UK, 20 March 2025. [Google Scholar]
- Neumann, J.E.; Price, J.; Chinowsky, P.; Wright, L.; Ludwig, L.; Streeter, R.; Jones, R.; Smith, J.B.; Perkins, W.; Jantarasami, L.; et al. Climate change risks to US infrastructure: Impacts on roads, bridges, coastal development, and urban drainage. Clim. Change 2014, 131, 97–109. [Google Scholar] [CrossRef]
- Karim, M.F.; Mimura, N. Impacts of climate change and sea-level rise on cyclonic storm surge floods in Bangladesh. Glob. Environ. Change 2008, 18, 490–500. [Google Scholar] [CrossRef]
- Zheng, F.; Westra, S.; Leonard, M.; Sisson, S.A. Modeling dependence between extreme rainfall and storm surge to estimate coastal flooding risk. Water Resour. Res. 2014, 50, 2050–2071. [Google Scholar] [CrossRef]
- Leonard, M.; Westra, S.; Phatak, A.; Lambert, M.; van den Hurk, B.; McInnes, K.; Risbey, J.; Schuster, S.; Jakob, D.; Stafford-Smith, M. A compound event framework for understanding extreme impacts. Wiley Interdiscip. Rev. Clim. Change 2014, 5, 113–128. [Google Scholar] [CrossRef]
- Wu, W.; Westra, S.; Leonard, M. Estimating the Probability of Compound Floods in Estuarine Regions. Hydrol. Earth Syst. Sci. Discuss. 2020, 25, 2821–2841. [Google Scholar] [CrossRef]
- Green, J.; Haigh, I.; Quinn, N.; Neal, J.; Wahl, T.; Wood, M.; Eilander, D.; de Ruiter, M.; Ward, P.; Camus, P. A comprehensive review of compound flooding literature with a focus on coastal and estuarine regions. EGUsphere 2024, 2024, 1–108. [Google Scholar] [CrossRef]
- Treloar, P.; Taylor, D.; Prenzler, P. Investigation of wave induced storm surge within a large coastal embayment-Moreton Bay (Australia). Coast. Eng. Proc. 2011, 1, 22. [Google Scholar] [CrossRef]
- Torres, J.M.; Bass, B.; Irza, N.; Fang, Z.; Proft, J.; Dawson, C.; Kiani, M.; Bedient, P. Characterizing the hydraulic interactions of hurricane storm surge and rainfall–runoff for the Houston–Galveston region. Coast. Eng. 2015, 106, 7–19. [Google Scholar] [CrossRef]
- Svensson, C.; Jones, D.A. Dependence between sea surge, river flow and precipitation in south and west Britain. Hydrol. Earth Syst. Sci. 2004, 8, 973–992. [Google Scholar] [CrossRef]
- Hawkes, P.; Svensson, C. Joint Probability: Dependence Mapping and Best Practice; T02-06-16; Defra/Environment Agency: London, UK, 2006.
- Zheng, F.; Westra, S.; Sisson, S.A. Quantifying the dependence between extreme rainfall and storm surge in the coastal zone. J. Hydrol. 2013, 505, 172–187. [Google Scholar] [CrossRef]
- Chen, A.S.; Evans, B.; Djordjević, S.; Savić, D.A. Multi-layered coarse grid modelling in 2D urban flood simulations. J. Hydrol. 2012, 470–471, 1–11. [Google Scholar] [CrossRef]
- Son, A.-L.; Kim, B.; Han, K.-Y. A Simple and Robust Method for Simultaneous Consideration of Overland and Underground Space in Urban Flood Modeling. Water 2016, 8, 494. [Google Scholar] [CrossRef]
- Bevacqua, E.; Vousdoukas, M.I.; Zappa, G.; Hodges, K.; Shepherd, T.G.; Maraun, D.; Mentaschi, L.; Feyen, L. More meteorological events that drive compound coastal flooding are projected under climate change. Commun. Earth Environ. 2020, 1, 47. [Google Scholar] [CrossRef]
- Kumbier, K.; Carvalho, R.C.; Vafeidis, A.T.; Woodroffe, C.D. Investigating compound flooding in an estuary using hydrodynamic modelling: A case study from the Shoalhaven River, Australia. Nat. Hazards Earth Syst. Sci. 2018, 18, 463–477. [Google Scholar] [CrossRef]
- Queensland Floods Commission Inquiry Report, Q. Queensland Floods Commission of Inquiry; Complete list of Final Report Recommendations; Royal Commissions: Brisbane, Australia, 2011.
- Yu, Y. Numerical Study of Hydrodynamic and Sediment Transport Within the Brisbane River Estuary and Moreton Bay, Australia. Ph.D. Thesis, Griffith University, Brisbane, Australia, 2017. [Google Scholar]
- Mani, P.; Chatterjee, C.; Kumar, R.J.N.H. Flood hazard assessment with multiparameter approach derived from coupled 1D and 2D hydrodynamic flow model. Nat. Hazards 2014, 70, 1553–1574. [Google Scholar]
- Barton, C.; Syme, B.; Ryan, P.; Rodgers, B.; Jensen, R. Milestone Report 2: Fast Model Development and Calibration Comprehensive Hydraulic Assessment; BMT WBM: Brisbane, Australia, 2017. [Google Scholar]
- Pellikka, H.; Leijala, U.; Johansson, M.M.; Leinonen, K.; Kahma, K.K. Future probabilities of coastal floods in Finland. Cont. Shelf Res. 2018, 157, 32–42. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, S.Q.; Jiang, C.; Sivakumar, M.; Enever, K.; Long, Y.; Deng, B.; Khalil, U.; Yin, L. Flood Mitigation Using an Innovative Flood Control Scheme in a Large Lake: Dongting Lake, China. Appl. Sci. 2019, 9, 2465. [Google Scholar] [CrossRef]
- Li, Y.; Yao, J. Estimation of transport trajectory and residence time in large river–lake systems: Application to Poyang Lake (China) using a combined model approach. Water 2015, 7, 5203–5223. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, Q.; Tan, Z.; Yao, J. On the hydrodynamic behavior of floodplain vegetation in a flood-pulse-influenced river-lake system (Poyang Lake, China). J. Hydrol. 2020, 585, 124852. [Google Scholar] [CrossRef]
- Khalil, U.; Yang, S.Q.; Sivakumar, M.; Enever, K.; Sajid, M.; Bin Riaz, M.Z. Investigating an Innovative Sea-Based Strategy to Mitigate Coastal City Flood Disasters and Its Feasibility Study for Brisbane, Australia. Water 2020, 12, 2744. [Google Scholar] [CrossRef]
- Haldar, R.; Khosa, R.; Gosain, A. Impact of Anthropogenic Interventions on the Vembanad Lake System. In Water Resources and Environmental Engineering I; Springer: Berlin/Heidelberg, Germany, 2019; pp. 9–29. [Google Scholar]
- Liu, J.; Sivakumar, M.; Yang, S.; Jones, B. Salinity Modelling and Management of the Lower Lakes of the Murray–Darling Basin, Australia. In Water Pollution XIV; University of Wollongong: Wollongong, Australia, 2018; pp. 257–268. [Google Scholar]
- Shrestha, A.; Bhattacharjee, L.; Baral, S.; Thakur, B.; Joshi, N.; Kalra, A.; Gupta, R. Understanding Suitability of MIKE 21 and HEC-RAS for 2D Floodplain Modeling; American Society of Civil Engineers: Reston, VA, USA, 2020; pp. 237–253. [Google Scholar]
- Eyre, B.; Hossain, S.; McKee, L. A suspended sediment budget for the modified subtropical Brisbane River estuary, Australia. Estuar. Coast. Shelf Sci. 1998, 47, 513–522. [Google Scholar] [CrossRef]
- Wolanski, E. Estuaries of Australia in 2050 and Beyond; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- DHI. MIKE 21 & MIKE 3 Flow Model FM, Hydrodynamic and Transport Module Scientific Documentation; DHI Group: Hørsholm, Denmark, 2017. [Google Scholar]
- DHI. MIKE 21 Hydrodynamic Module Step-by-Step Training Guide; DHI Group: Hørsholm, Denmark, 2014. [Google Scholar]
- DHI. MIKE ZERO, Mesh Generator, Step-by-Step Training Guide; DHI Group: Hørsholm, Denmark, 2012. [Google Scholar]
- IPCC. Fourth Assessment Report of the IPCC; IPCC: Geneva, Switzerland, 2007.
- Ayre, R. Brisbane River Catchment Flood Study: Comprehensive Hydrologic Assessment; Aurecon Australasia: Brisbane, Australia, 2017. [Google Scholar]
- DHI. MIKE 21 Flow Model FM, Hydrodynamic Module, User Guide; DHI Group: Hørsholm, Denmark, 2017. [Google Scholar]
- Teng, J.; Jakeman, A.; Vaze, J.; Croke, B.; Dutta, D.; Kim, S. Flood inundation modelling: A review of methods, recent advances and uncertainty analysis. Environ. Model. Softw. 2017, 90, 201–216. [Google Scholar] [CrossRef]





| Data | Description | Station No. | Sources |
|---|---|---|---|
| DEM data | Brisbane River and floodplains; 5 m × 5 m DEM | N/A | Queensland Government under the Brisbane River Catchment Flood Study (BRCFS) |
| Moreton Bay; 30 m × 30 m DEM | N/A | James Cook University (JCU) 3DGBR: GBR100 High-resolution | |
| River gauge data (instantaneous values) | Moggill Alert | 540200 | Bureau of Meteorology (BOM), Queensland |
| Jindalee | 540192 | ||
| Brisbane City Alert | 540198 | ||
| Flow data (daily) | Savage crossing | 143001 | Water Monitoring Information Portal, Queensland Government |
| Tidal data (10 minutes interval) | Beacon M2 Moreton Bay | 046206A | Maritime Safety Queensland |
| Runaway Bay | 045100B | ||
| Amity Point | 046211E | ||
| Brisbane Bar | 046046A | ||
| Whyte Island Alert | 540495 | ||
| Flood extent | Brisbane River flood extent | N/A | Queensland Government, Flood imagery and data |
| Case 1 | Case 2 | Case 3 | Case 4 | Case 5 | |
|---|---|---|---|---|---|
| Number of elements | 95,497 | 122,220 | 153,383 | 217,066 | 288,415 |
| Number of nodes | 48,215 | 61,605 | 77,352 | 11,3116 | 148,697 |
| Element area max. ×104 (m2) | 500 | 100 | 30 | 15 | 10 |
| Element area min. (m2) | 70 | 59.9 | 46.7 | 27.3 | 21.5 |
| CFLmax | 0.53 | 0.57 | 0.65 | 0.74 | 0.79 |
| Running time (Hours) | 11 | 13 | 18 | 28 | 36 |
| Scenario (S) | Years | Storm Tide Level |
|---|---|---|
| Base Scenario | Present | The 100-year storm surge level (currently 2.5 m) |
| Scenario 1 | 2030 | 2.59–2.71 m |
| Scenario 2 | 2050 | 2.67–2.88 m |
| Scenario 3 | 2070 | 2.69–3.10 m |
| Scenario 4 | 2100 | 2.76–3.32 m |
| Year | Gauging Stations | RMSE | Ens | R2 |
|---|---|---|---|---|
| 2006 | Brisbane City | 0.25 | 0.84 | 0.85 |
| 2013 | 0.2 | 0.87 | 0.88 | |
| 2011 | 0.3 | 0.94 | 0.95 | |
| Brisbane Bar | 0.1 | 0.95 | 0.96 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khalil, U.; Sajid, M.; Riaz, M.Z.B.; Iqbal, U.; Jnead, E.; Yang, S.-Q.; Sivakumar, M. Investigating the Compound Influence of Tidal and River Floodplain Discharge Under Storm Events in the Brisbane River Estuary, Australia. Water 2025, 17, 1554. https://doi.org/10.3390/w17101554
Khalil U, Sajid M, Riaz MZB, Iqbal U, Jnead E, Yang S-Q, Sivakumar M. Investigating the Compound Influence of Tidal and River Floodplain Discharge Under Storm Events in the Brisbane River Estuary, Australia. Water. 2025; 17(10):1554. https://doi.org/10.3390/w17101554
Chicago/Turabian StyleKhalil, Usman, Mariam Sajid, Muhammad Zain Bin Riaz, Umair Iqbal, Essam Jnead, Shu-Qing Yang, and Muttucumaru Sivakumar. 2025. "Investigating the Compound Influence of Tidal and River Floodplain Discharge Under Storm Events in the Brisbane River Estuary, Australia" Water 17, no. 10: 1554. https://doi.org/10.3390/w17101554
APA StyleKhalil, U., Sajid, M., Riaz, M. Z. B., Iqbal, U., Jnead, E., Yang, S.-Q., & Sivakumar, M. (2025). Investigating the Compound Influence of Tidal and River Floodplain Discharge Under Storm Events in the Brisbane River Estuary, Australia. Water, 17(10), 1554. https://doi.org/10.3390/w17101554







