1. Introduction
Stepped spillways are commonly constructed in dams to safely release overflow water and transfer high-energy water downstream of the dam. It plays an important role in improving energy dissipation, significantly reducing specific residual energy [
1,
2]. This type of spillway consists of steps that allow water to flow down from a higher elevation to a lower point, especially in mountainous regions. Effective spillway design and management are essential for minimizing the risks associated with extreme weather conditions and potential dam failures. A properly designed spillway ensures that the flow of water is safely released, reducing the risk of overflow and preventing structural damage to the dam [
3]. The concept of stepped spillways dates back to ancient times, but modern engineering has significantly refined their design and functionality. Stepped spillways are particularly popular for managing high-gravity flows and ensuring the safety of dam embankments through various engineering techniques [
3,
4]. The stepped design can be more cost-effective regarding construction material maintenance and enhanced energy dissipation capabilities [
5]. Stepped spillways can be built using various materials, including concrete, masonry, and even natural rock, depending on the site-specific requirements and the availability of resources [
6]. In addition, this type of spillway is considered effective in maintaining dam wall stability and integrity due to its high gravity base and inclination towards the dam side.
Despite their potential advantages, the design and implementation of stepped spillways offer several challenges. These challenges may include cavitation, high flow velocity, and pressure distribution in which the flow profile shifts into a skimming flow condition [
7,
8]. The geometric design of stepped spillways significantly influences the flow patterns and the proper alignment of water flow with the design, and the pressure parameters can result in nappe flow or skimming flow. The geometry of stepped spillways directly influences flow characteristics and reduces residual energy downstream, making them an effective natural method for dissipating flow energy. Investigation is crucial to improve and safeguard the local communities and dam infrastructure from potential flood damage downstream of the structure [
9]. Several researchers utilized various geometry models for stepped spillways to improve the accuracy and simplicity of flow stability and safety to minimize the risk of cavitation on the spillway surface and in the stilling basin [
10,
11,
12]. However, proper flow resistance is crucial for assessing frictional energy losses. In skimming flows, the flow resistance depends on several factors, including stepped chute geometry, flow depth, chute slope, flow conditions, step heights, and the degree of air entrainment. Because of the complexity and interplay of these factors, it can be challenging to account for all of them comprehensively.
Computational codes and hardware technology are being utilized to employ numerical solutions. The use of technological advancement enhances the efficiency and cost-effectiveness of flow analysis and assists in the design across various fields of study [
13,
14]. The numerical modeling approach is also extended to more complex multiphase flows and various 3D modeling. Such techniques numerically evaluate hydraulic performance, analyze erosion and sedimentation, assess structural integrity, study air entrainment and aeration, conduct environmental impact assessments, and optimize energy dissipation [
15,
16,
17]. Many researchers have widely employed numerical simulations in spillway design and analysis for various purposes. Several problems related to spillway fluid flow have been extensively investigated, including the dynamics of hydraulic jumps, the behavior of flow on chutes, and the effectiveness of various energy dissipation methods [
17,
18,
19].
In addition to the numerical method, many of these researchers have employed the volume of fluid (VOF) method and the realizable
k-ε turbulence model to study open channel flow. The realizable
k-ε turbulence model is often considered more efficient for simulating free surface flows. It is particularly useful for understanding various stepped spillway configurations’ hydraulic properties and performance. The realizable
k-ε model is especially effective in analyzing flow patterns, velocity distributions, and energy dissipation rates. Li et al. [
20] conducted a study using the realizable
k-ε turbulence model and the two-phase mixture model to investigate the effects of different step geometries, both rounded and trapezoidal, on air–water flows over a stepped spillway. Their findings revealed that the spillway with rounded steps exhibited higher air concentrations, whereas the trapezoidal steps resulted in higher flow velocities compared to the rounded step configuration. Ma et al. [
18] investigated the interval pooled stepped spillway using a VOF method with the realizable turbulence
k-ε model to evaluate energy dissipation. It was found that increasing the pool height had a limited effect on enhancing energy dissipation. Li et al. [
21] used Flow-3D to study a stepped spillway with pool heights of 2 cm, 4 cm, and 6 cm under varying flow rates. The results showed that, at each flow rate, turbulent energy at the midpoint of each step decreased with increasing pool height. The energy dissipation rate decreased for a constant pool height as the flow rate increased.
Ghaderi et al. [
1] tested four pool configurations and found that the notched pooled step configuration improved efficiency by approximately 5.8%. The interfacial velocities were higher in the pooled spillway than in the flat-stepped spillway. The pressure at the beginning of the steps was greater in the pooled configuration. However, the maximum pressure near the step-pool dropped for the notched pool, and the pool configuration had a minimal effect on the air entrainment site. Morovati et al. [
19] found that reducing the number of steps in pooled stepped spillways decreased flow velocity and increased relative energy dissipation at the end of the spillway. Furthermore, fewer steps result in higher values of turbulent kinetic energy. The findings of Saleh et al. indicate that flat-stepped spillways generally exhibit better energy dissipation performance compared to pooled configurations in certain condition [
22]. However, many studies show that pooled stepped spillways demonstrate improved aeration and energy dissipation rates under certain conditions. Studies by Morovati et al. [
19], Hantoosh et al. [
23], and Pandey et al. [
24] also reveal that factors such as pool height, step geometry, and discharge rates significantly influence the hydraulic behavior and energy dissipation characteristics of stepped spillways. These insights contribute to the optimization of stepped spillway designs for improved hydraulic performance and energy dissipation in dam structures.
Following previous research findings, growing interest in utilizing pooled stepped spillways has been increased due to their superior energy dissipation capabilities and improved aeration performance [
12,
25]. Studies have shown that stepped spillways with pooled designs are more effective in dissipating flow energy compared to traditional designs; findings indicate that increasing the number of steps in the spillway enhances the energy dissipation rate [
23]. The effects of steep slopes of 15°, 25°, and 45° with different inclined slopes and a maximum number of up to 50 are investigated [
26]. In their study, with a constant flow rate passing over a stepped spillway, energy dissipation increases as the spillway slope and the number of steps increase [
27,
28]. It was investigated that the energy dissipation performance of the interval pooled stepped spillway was generally better than that of the horizontal flat stepped spillway [
29]. Moreover, numerical simulations have demonstrated that the turbulent energy at the midpoint of each step decreases with an increase in pool height while the turbulent dissipation rate increases with the flow rate, but the energy dissipation rate decreases with a higher flow rate [
5,
12]. These spillway design and performance evaluation advancements contribute to the ongoing optimization of hydraulic structures for enhanced energy dissipation and aeration in water management systems [
30]. Considering previous findings, existing studies have primarily focused on traditional stepped spillways, and pooled stepped spillways receive relatively less recognition than conventional ones. Therefore, further research is required to enhance the understanding and performance of pooled stepped spillways.
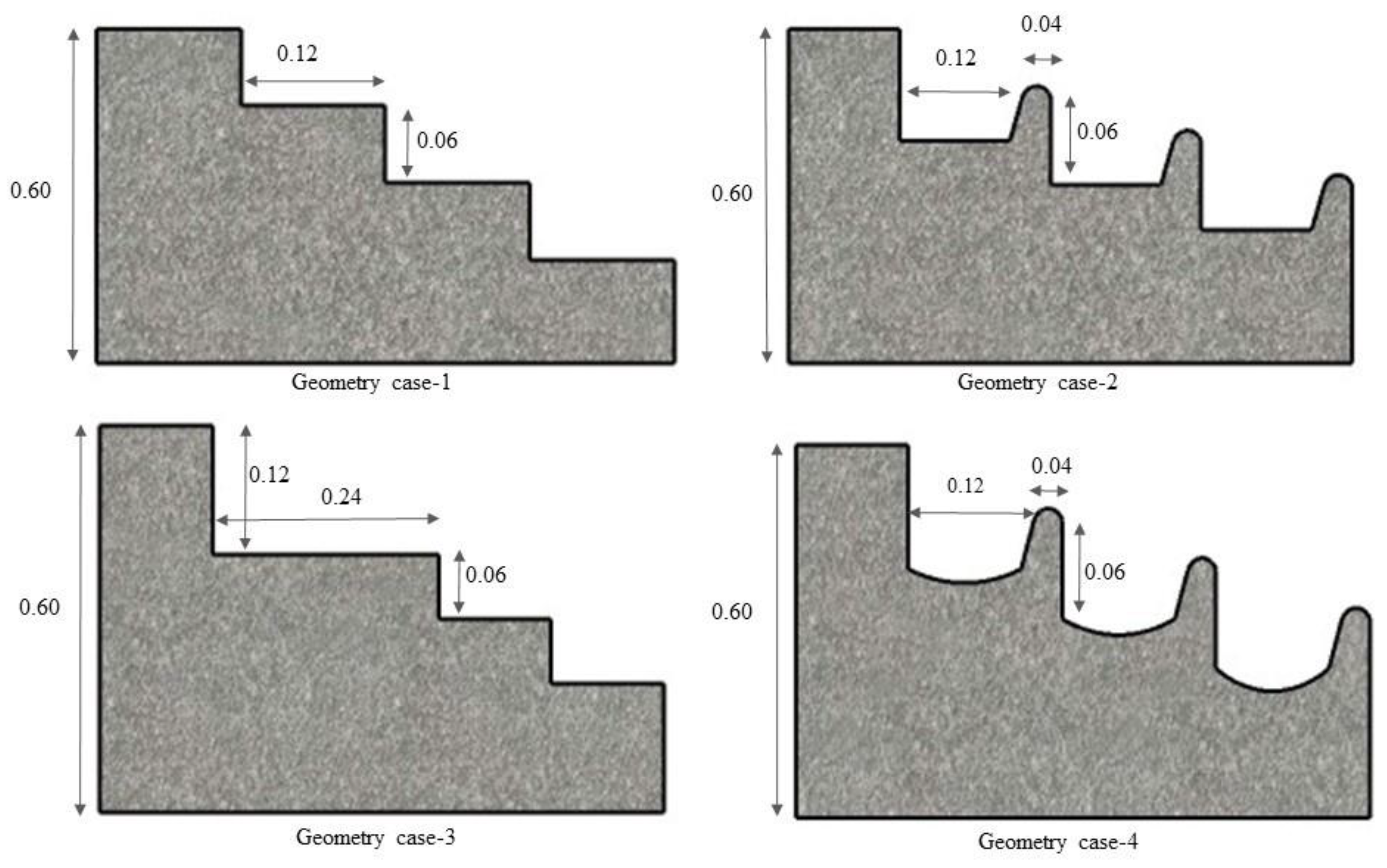
This study aims to evaluate the potential and efficiency of novel pooled stepped spillway designs compared to flat stepped spillways. It examines four geometric configurations with a chute slope of 26.6°, analyzing their effects on energy dissipation efficiency, flow patterns, velocity, pressure distributions, and Froude numbers. Additionally, the study seeks to determine which spillway design is most effective for ensuring safe operation across a range of flow conditions. It also aims to assess the reliability of the CFD technique by using the realizable k-ε turbulence model to validate its accuracy and robustness.
4. Conclusions
A numerical study was conducted to evaluate flat and pooled stepped spillways under four different configurations by using the realizable k-ε turbulence model with the VOF method. The experimental data of three different flow rates (45, 50, and 54 L/s) were collected from a physical model to validate the reliability of the numerical model. The numerical results showed a good resemblance with the experimental data, demonstrating that the realizable k-ε turbulence model could accurately predict various flow scenarios. Afterward, the study analyzed flow behaviors across four stepped spillway configurations and compared two flat and two pooled geometries, with velocities ranging from 0.45 to 0.70 m/s with 0.5 m/s intervals, to assess their impact on energy dissipation and flow dynamics.
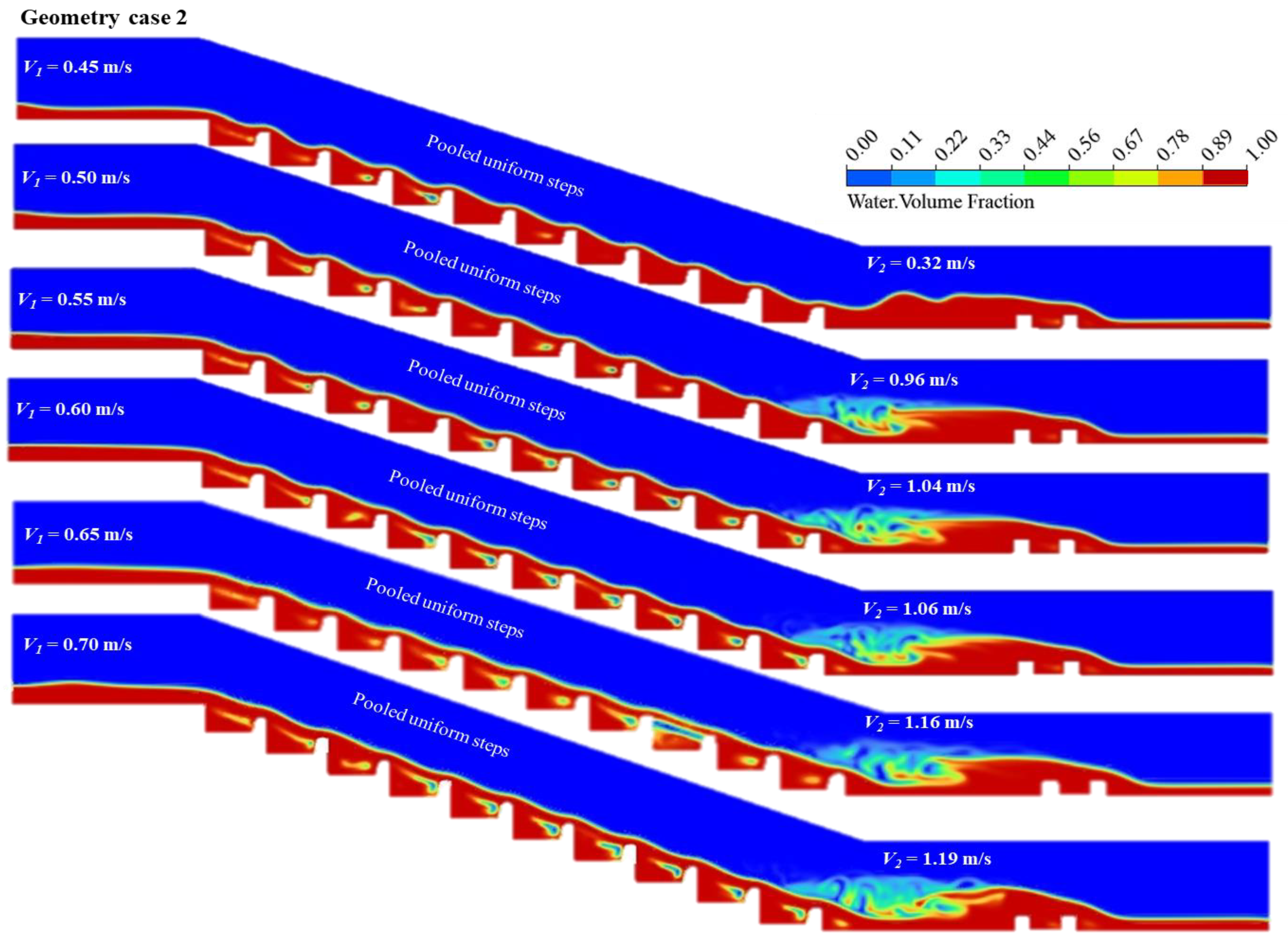
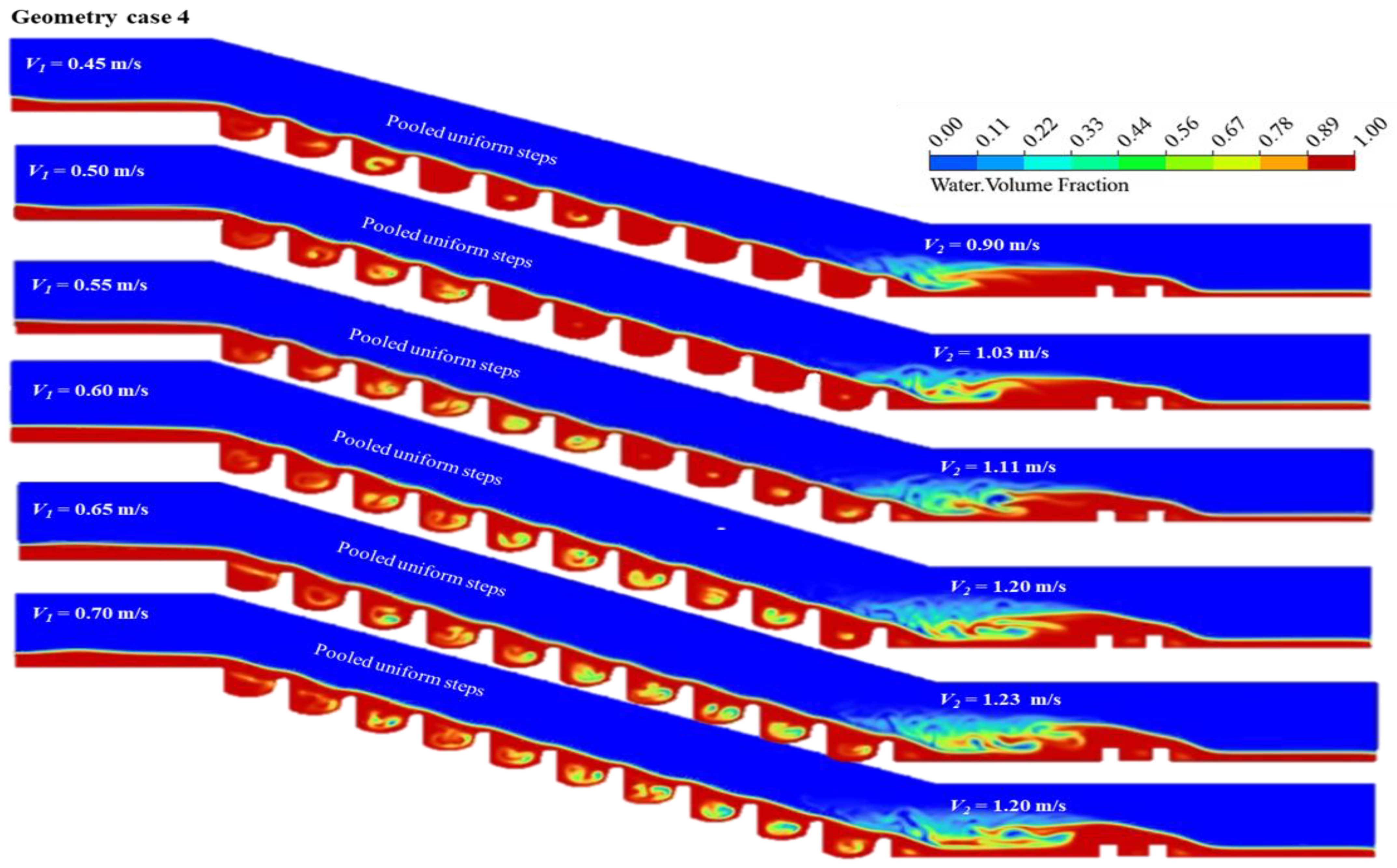
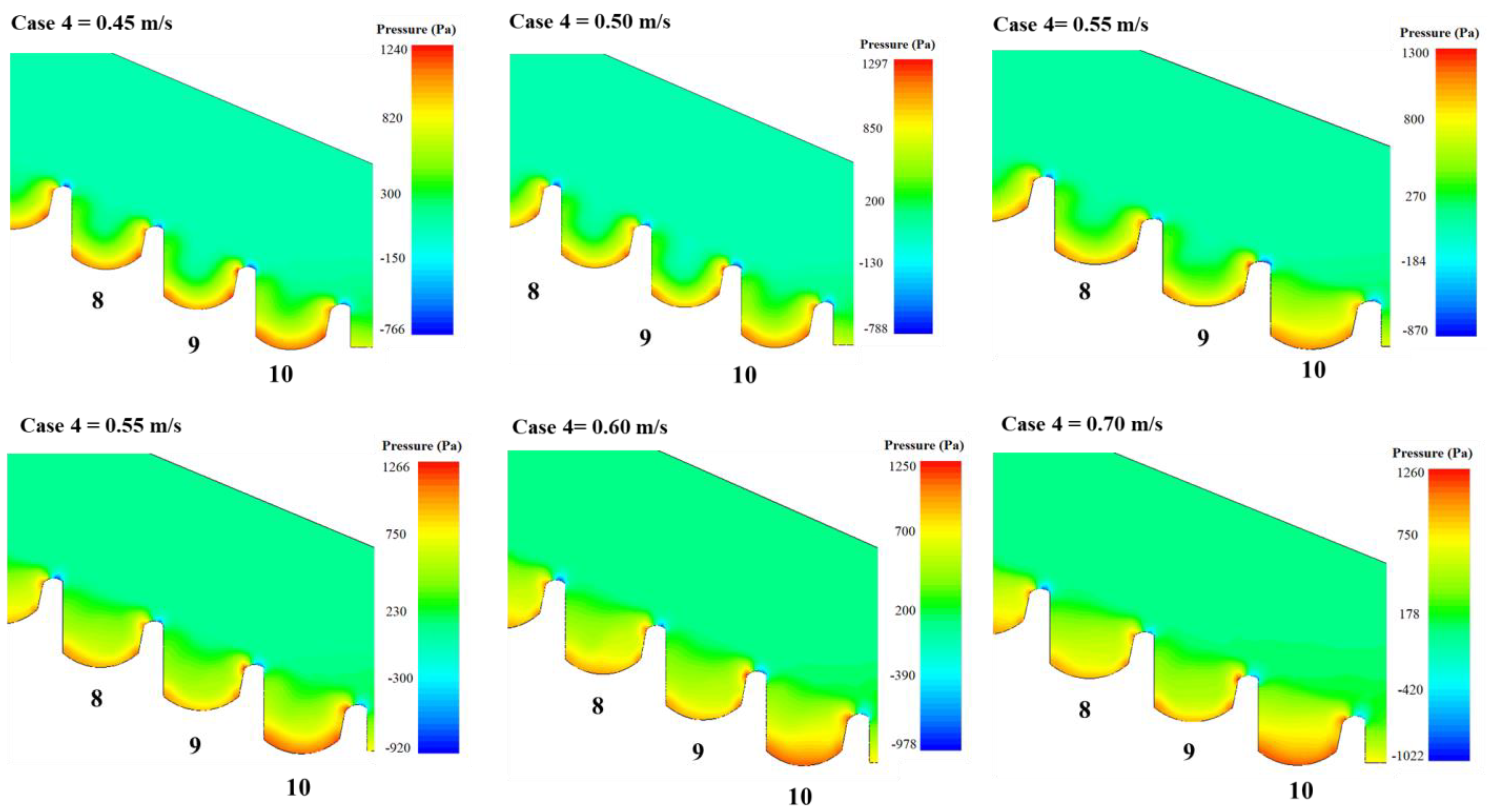
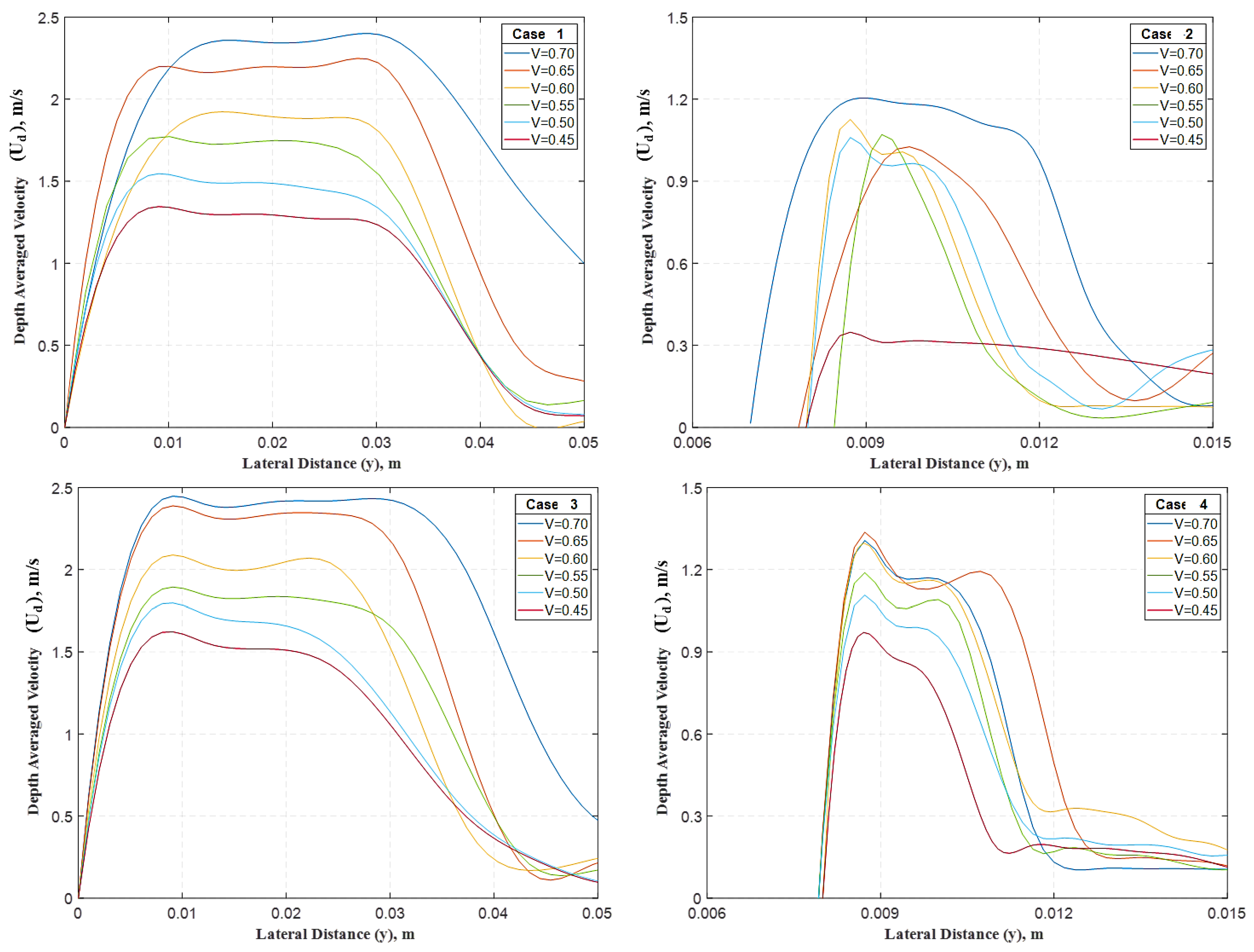
Based on the results, the findings show that the pooled stepped spillways case 2 and case 4 perform better than the flat stepped spillways case 1 and case 3 regarding energy dissipation. The efficiency of case 2 and case 4 at 0.70 m/s, compared to case-1, shows an increase in relative energy loss by 34.68% and 25.81%, respectively. Moreover, case 2 and case 4 show a substantial decrease in downstream velocity of 0.87 m/s and 1.30 m/s, respectively. Similarly, the downstream depth-averaged velocity also significantly decreased, as shown in
Figure 14. Additionally, the case 2 and case 4 reductions in hydraulic jump and low Froude numbers indicate improvements in energy dissipation due to the enhanced recirculation and breakdown of trapped water in pooled stepped spillways.
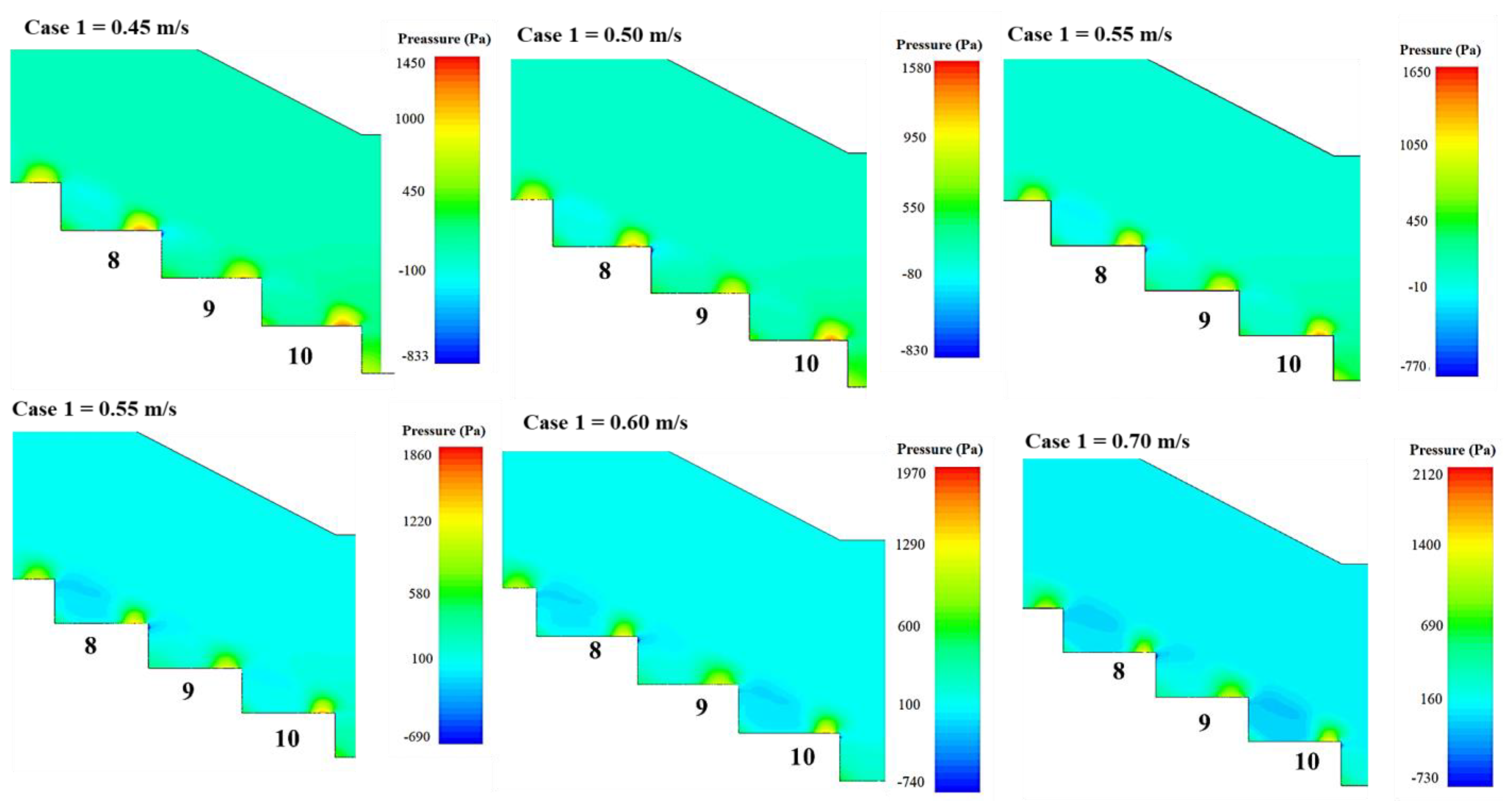
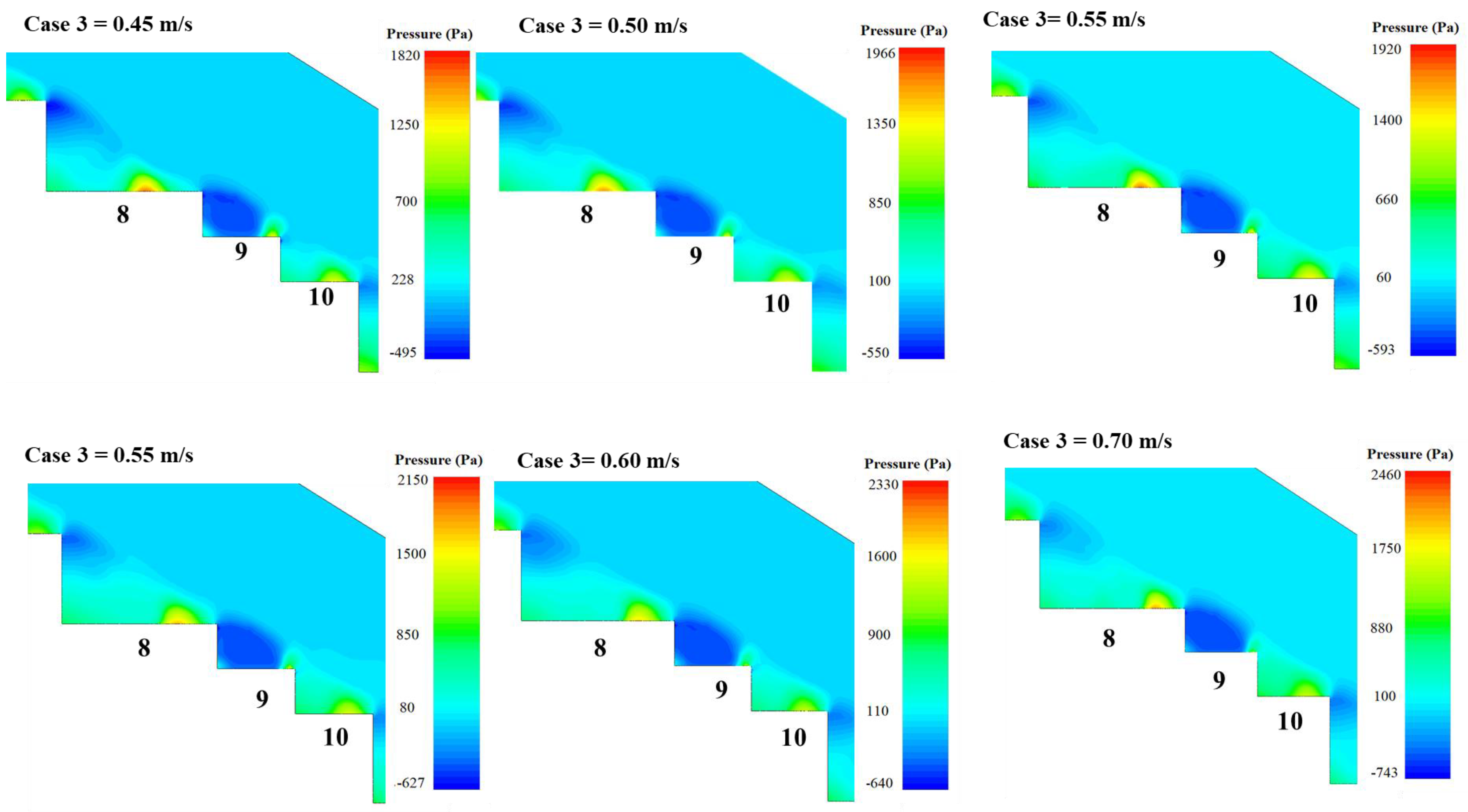
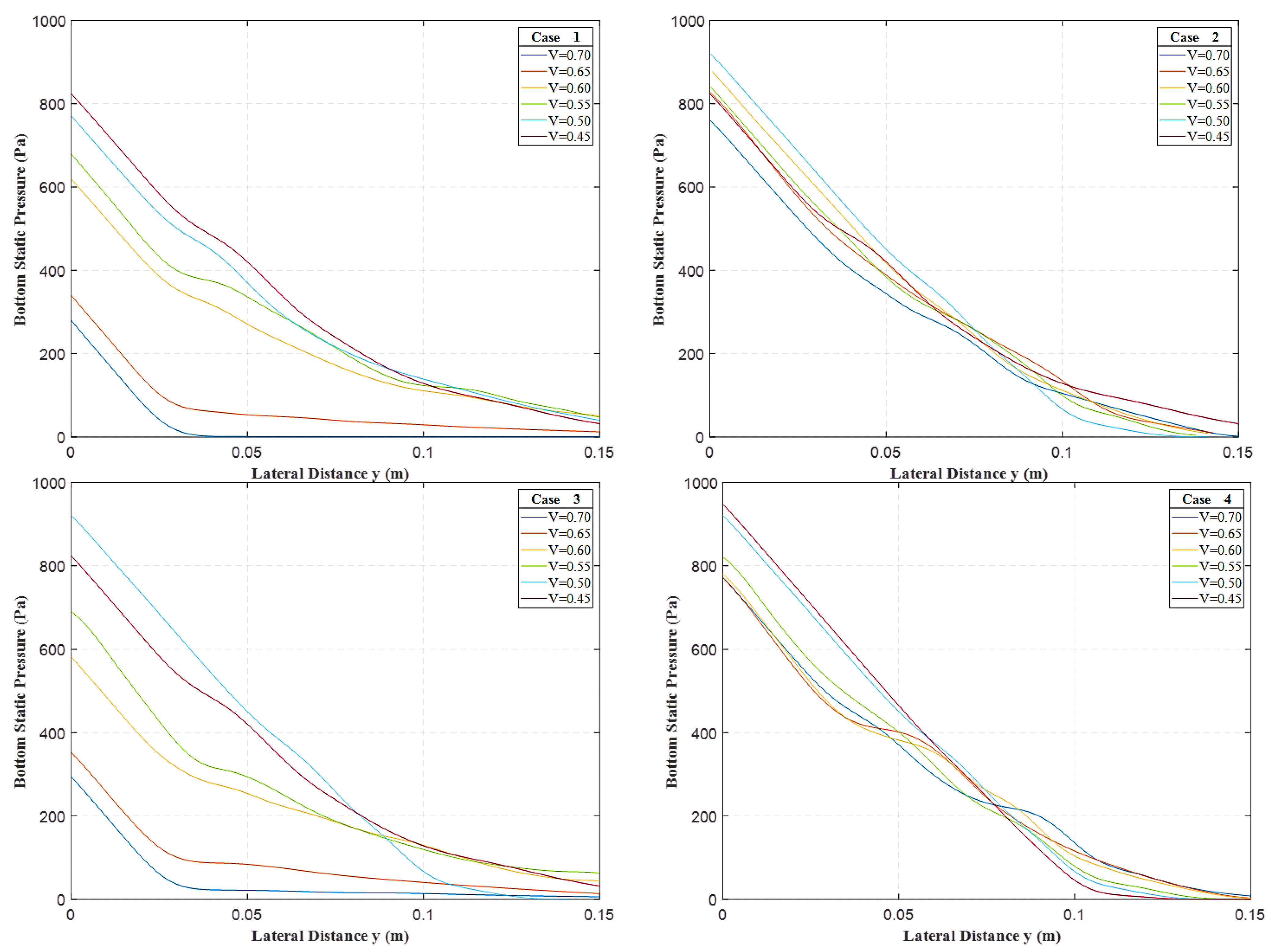
The pressure distribution on flat stepped spillways, especially case 3, exhibits high negative pressure values on the upper vertical faces of the steps and the horizontal steps. On the other hand, pooled stepped spillways show relatively lower negative pressure values. The turbulence kinetic energy in flat stepped case 1 and case 3 increased, while in pooled stepped spillways, it significantly decreased. Overall, pooled stepped spillways demonstrate superior performance in energy dissipation, particularly in the skimming flow regime, compared to flat stepped spillways. Further research on pooled stepped spillways is needed to address high discharge conditions, focusing on the effects of cavitation and aeration to improve spillway design and performance.