Occurrence of Micropollutants in Wastewater and Evaluation of Their Removal Efficiency in Treatment Trains: The Influence of the Adopted Sampling Mode
Abstract
1. Introduction
2. Materials and Methods
2.1. Definition of Representative Compounds
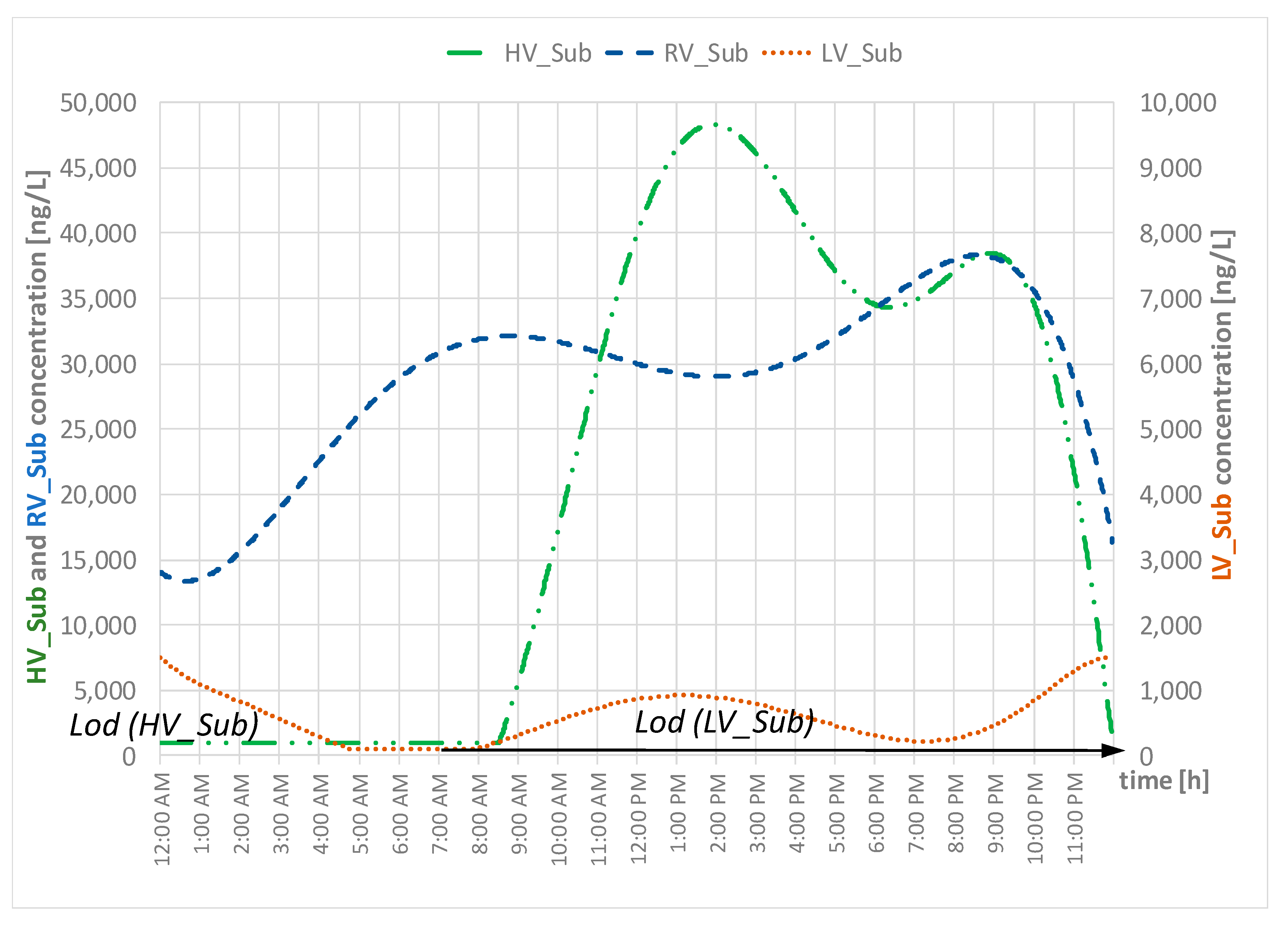
- a substance whose concentration in wastewater presents few but evident variations over the day, such as the diagnostic agents gadolinium and iopamidol [18], the cytostatic agent 5-fluoruracil [2] or the diuretic furosemide and the antibiotic sulphamethoxazole [13]. Such a substance is called a ‘high variability substance’, HV_Sub. During the night, its concentration decreases even lower than the corresponding limit of detection (Lod) for some hours;
- a substance whose concentration in wastewater presents a modest variation over the day, and is also detectable during the night, such as the anti-inflammatory ketoprofen [19], the antiseptic triclosan and the anticonvulsant agent phenytoin [13], and the antibiotic trimethoprim [13,23]. This is called the ‘low variability substance’, LV_Sub. It may happen that during the night its concentration decreases to values below its limit of detection, but only for very short periods;
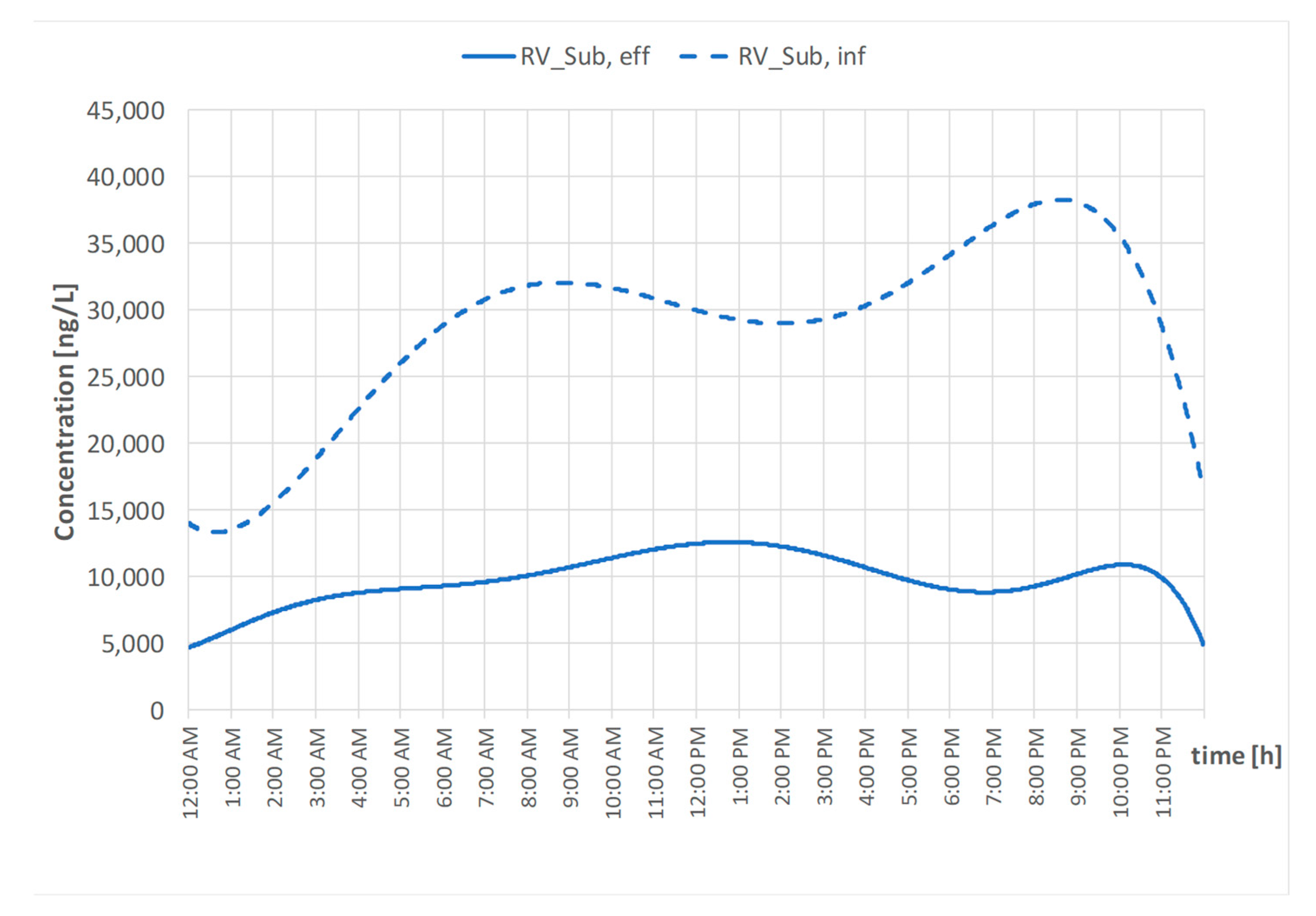
- a substance whose concentration “randomly” varies over the day, such as the antibiotics ciprofloxacin [12] lincomycin [23], the anti-inflammatories diclofenac [13], and 4-tert octylfenol (a degradation product of a surfactant). This substance is called a ‘random variability substance’, RV_Sub. Its profile pattern is not easily predictable.
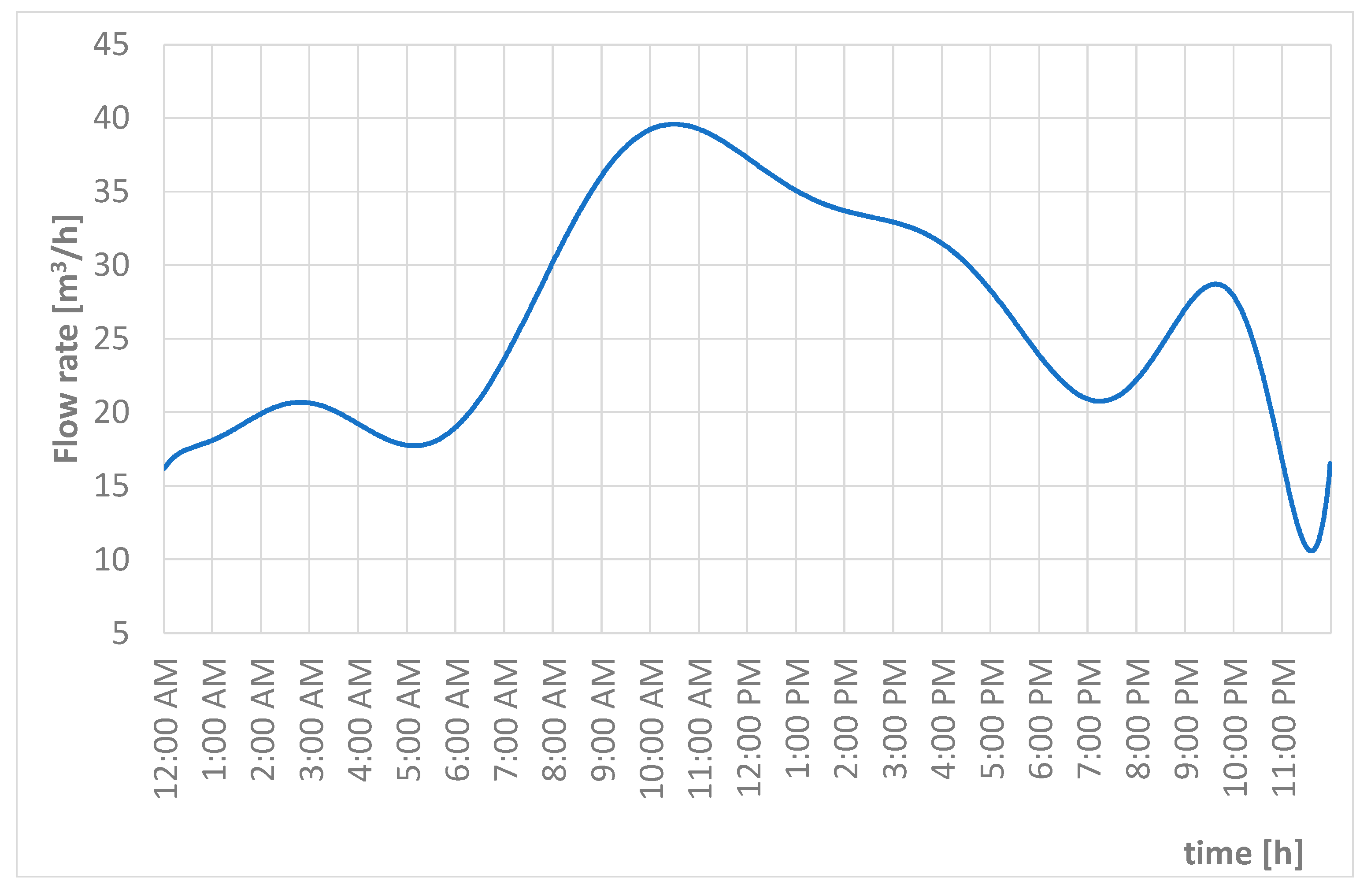
2.2. Flow Rate Curves Versus Time
2.3. The Sampling Modes Adopted and Compared
2.4. Daily Average Concentration Evaluation
- 1.
- definition of the sampling times according to Table 1;
- 2.
- calculation of the values of concentrations at each sampling time defined in the last column of Table 1 for the representative compound under study by the corresponding curve (Equations (1)–(3));
- 3.
- evaluation of the average daily concentration by applying the equation corresponding to the selected sampling mode (Equations (7)–(9)).
- 1.
- definition of the frequency of sampling (k samples), according to the last column of Table 1 and the wastewater volume Vvp (=Vdaily/k) which has to flow before collecting a water sample;
- 2.
- evaluation of the k sampling instants tn, by means of the V(t) curve (Equation (5)) posing V(tn) = n Vvp with n = 1, …, k;
- 3.
- calculation of the values of concentrations at each sampling time tn by the corresponding curve (Equations (1)–(3));
- 4.
- evaluation of the average daily concentration by applying Equation (10).
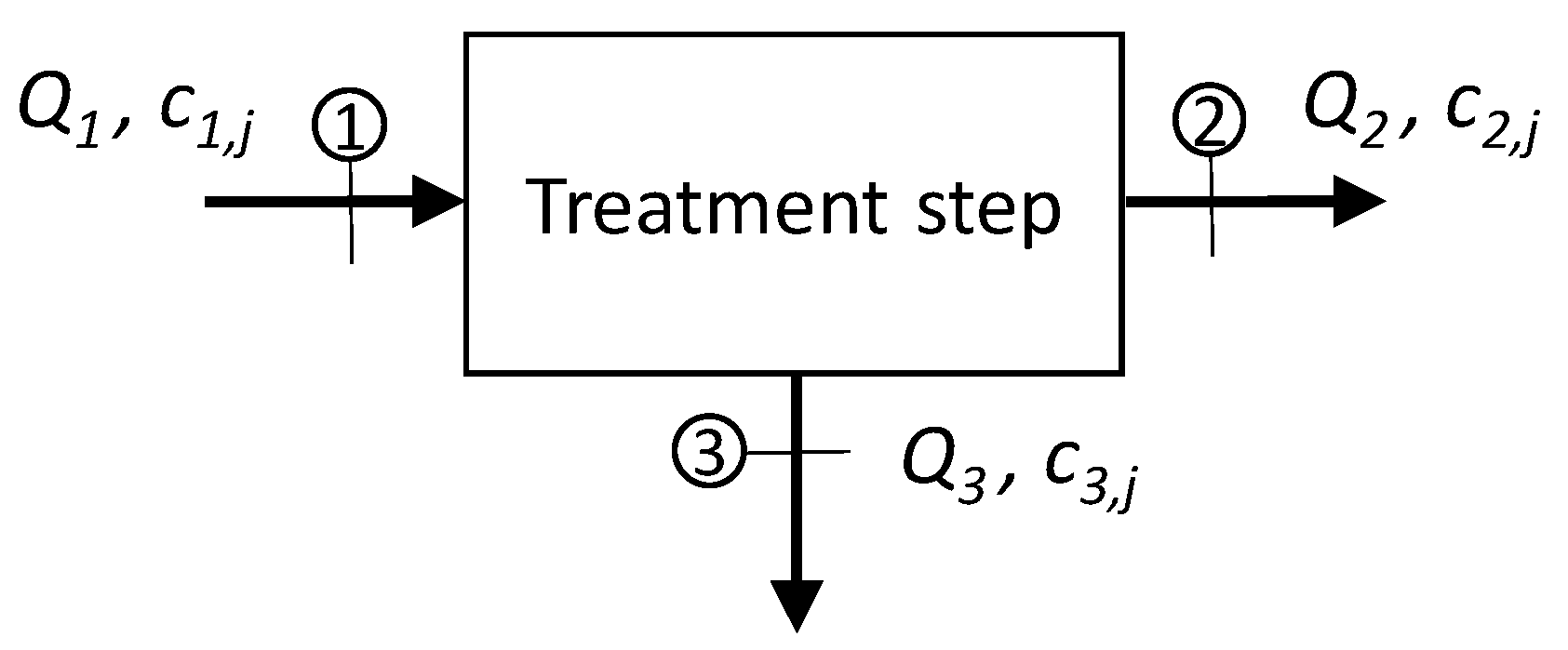
2.5. Mass Load Evaluation
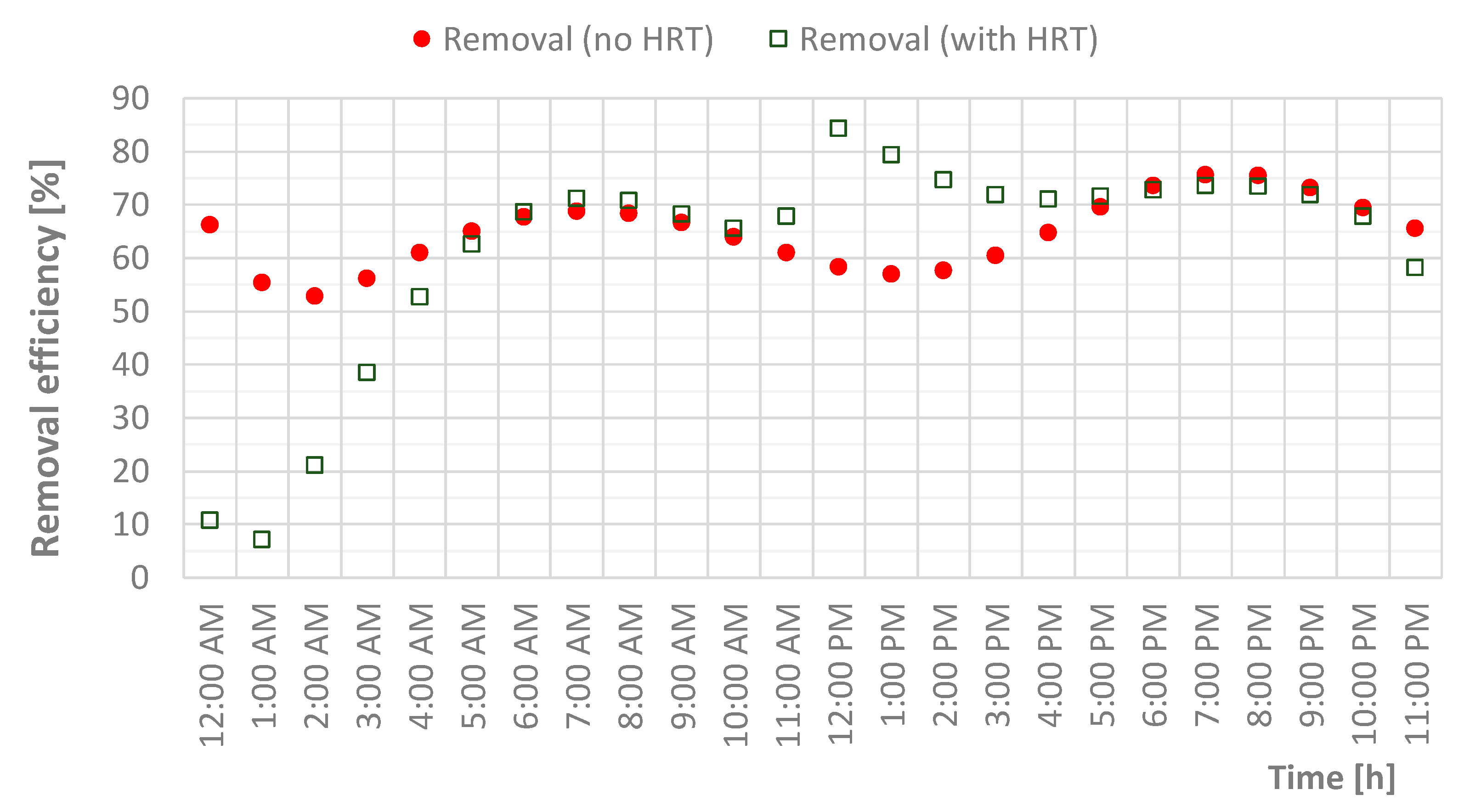
2.6. Removal Efficiency Evaluation of a Micropollutant: Considerations and Remarks
Case Study for the Evaluation of Removal Efficiency
3. Results
3.1. Average Concentration of the Key Compounds
3.2. Mass Load Evaluated for Each Substance
3.3. Average Removal Efficiency for RV-Sub
4. Discussion and Final Remarks
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Verlicchi, P.; Zambello, E. How Efficient are Constructed Wetlands in Removing Pharmaceuticals from Untreated and Treated Urban Wastewaters? A Review. Sci. Total Environ. 2014, 470, 1281–1306. [Google Scholar] [CrossRef] [PubMed]
- Weissbrodt, D.; Kovalova, L.; Ort, C.; Pazhepurackel, V.; Moser, R.; Hollender, J.; Siegrist, H.; Mcardell, C.S. Mass flows of X-ray contrast media and Cytostatics in hospital wastewater. Environ. Sci. Technol. 2009, 43, 4810–4817. [Google Scholar] [CrossRef] [PubMed]
- Verlicchi, P. Pharmaceutical Concentrations and Loads in Hospital Effluents: Is a Predictive Model or Direct Measurement the Most Accurate Approach? In Hospital Wastewater—Characteristics, Management, Treatment and Environmental Risks; Springer: Cham, Switzerland, 2018; pp. 101–134. [Google Scholar] [CrossRef]
- Kovalova, L.; Siegrist, H.; Singer, H.; Wittmer, A.; McArdell, C.S. Hospital wastewater treatment by membrane bioreactor: Performance and efficiency for organic micropollutant elimination. Environ. Sci. Technol. 2012, 46, 1536–1545. [Google Scholar] [CrossRef] [PubMed]
- Ort, C.; Lawrence, M.G.; Reungoat, J.; Mueller, J.F. Sampling for PPCPs in wastewater systems: Comparison of different sampling modes and optimization strategies. Environ. Sci. Technol. 2010, 44, 6289–6296. [Google Scholar] [CrossRef] [PubMed]
- Ort, C.; Lawrence, M.G.; Rieckermann, J.; Joss, A. Sampling of pharmaceuticals and personal care products (PPCPs) and illicit drugs in wastewater systems: Are your conclusions valid? A critical review. Environ. Sci. Technol. 2010, 44, 6024–6035. [Google Scholar] [CrossRef] [PubMed]
- Peña-Guzmán, C.; Ulloa-Sánchez, S.; Mora, K.; Helena-Bustos, R.; Lopez-Barrera, E.; Alvarez, J.; Rodriguez-Pinzón, M. Emerging pollutants in the urban water cycle in Latin America: A review of the current literature. J. Environ. Manag. 2019, 237, 408–423. [Google Scholar] [CrossRef] [PubMed]
- Fekadu, S.; Alemayehu, E.; Dewil, R.; Van der Bruggen, B. Pharmaceuticals in freshwater aquatic environments: A comparison of the African and European challenge. Sci. Total Environ. 2019, 654, 324–337. [Google Scholar] [CrossRef]
- Yap, H.C.; Pang, Y.L.; Lim, S.; Abdullah, A.Z.; Ong, H.C.; Wu, C. A comprehensive review on state-of-the-art photo-, sono-, and sonophotocatalytic treatments to degrade emerging contaminants. Int. J. Environ. Sci. Technol. 2019, 16, 601–628. [Google Scholar] [CrossRef]
- Cattaneo, S.; Marciano, F.; Masotti, L.; Vecchiato, G.; Verlicchi, P.; Zaffaroni, C. Improvement in the Removal of Micropollutants at Porto Marghera Industrial Wastewaters Treatment Plant by MBR Technology. Water Sci. Technol. 2008, 58, 1789–1796. [Google Scholar] [CrossRef][Green Version]
- Paíga, P.; Correia, M.; Fernandes, M.J.; Silva, A.; Carvalho, M.; Vieira, J.; Jorge, S.; Silva, J.G.; Freire, C.; Delerue-Matos, C. Assessment of 83 pharmaceuticals in WWTP influent and effluent samples by UHPLC-MS/MS: Hourly variation. Sci. Total. Environ. 2019, 648, 582–600. [Google Scholar] [CrossRef]
- Duong, H.; Pham, N.; Nguyen, H.; Hoang, T.; Pham, H.; CaPham, V.; Berg, M.; Giger, W.; Alder, A. Occurrence, fate and antibiotic resistance of fluoroquinolone antibacterials in hospital wastewaters in Hanoi, Vietnam. Chemosphere 2008, 72, 968–973. [Google Scholar] [CrossRef] [PubMed]
- Nelson, E.D.; Do, H.; Lewis, R.S.; Carr, S.A. Diurnal variability of pharmaceutical, personal care product, estrogen and alkylphenol concentrations in effluent from a tertiary wastewater treatment facility. Environ. Sci. Technol. 2011, 45, 1228–1234. [Google Scholar] [CrossRef] [PubMed]
- Burns, E.E.; Carter, L.J.; Kolpin, D.W.; Thomas-Oates, J.; Boxall, A.B.A. Temporal and spatial variation in pharmaceutical concentrations in an urban river system. Water Res. 2018, 137, 72–85. [Google Scholar] [CrossRef] [PubMed]
- Barbosa, M.O.; Ribeiro, A.R.; Ratola, N.; Hain, E.; Homem, V.; Pereira, M.F.R.; Blaney, L.; Silva, A.M.T. Spatial and seasonal occurrence of micropollutants in four Portuguese rivers and a case study for fluorescence excitation-emission matrices. Sci. Total Environ. 2018, 644, 1128–1140. [Google Scholar] [CrossRef] [PubMed]
- Ort, C.; Gujer, W. Sampling for representative micropollutant loads in sewer systems. Water Sci. Technol. 2006, 54, 169–176. [Google Scholar] [CrossRef] [PubMed]
- Ort, C.; Lawrence, M.G.; Reungoat, J.; Eaglesham, G.; Carter, S.; Keller, J. Determining the fraction of pharmaceutical residues in wastewater originating from a hospital. Water Res. 2010, 44, 605–615. [Google Scholar] [CrossRef] [PubMed]
- Kummerer, K.; Helmers, E. Hospital effluents as a source of gadolinium in the aquatic environment. Environ. Sci. Technol. 2000, 34, 573–577. [Google Scholar] [CrossRef]
- Khan, S.; Ongerth, J. Occurrence and removal of pharmaceuticals at an Australian sewage treatment plant. Water 2005, 32, 80–85. [Google Scholar]
- Verlicchi, P.; Galletti, A.; Al Aukidy, M. Hospital Wastewaters: Quali-quantitative Characterization and Strategies for Their Treatment and Disposal. In Wastewater Reuse and Management; Springer Science & Business Media: New York, NY, USA, 2013; pp. 225–251. [Google Scholar]
- Verlicchi, P.; Galletti, A.; Petrovic, M.; Barcelo, D. Hospital Effluents as a Source of Emerging Pollutants: An Overview of Micropollutants and Sustainable Treatment Options. J. Hydrol. 2010, 389, 416–428. [Google Scholar] [CrossRef]
- Boillot, C.; Bazin, C.; Tissot-Guerraz, F.; Droguet, J.; Perraud, M.; Cetre, J.C.; Trepo, D.; Perrodin, Y. Daily physicochemical, microbiological and ecotoxicological fluctuations of a hospital effluent according to technical and care activities. Sci. Total Environ. 2008, 403, 113–129. [Google Scholar] [CrossRef]
- Hong, Y.; Sharma, V.K.; Chiang, P-C.; Kim, H. Fast-target analysis and hourly variation of 60 pharmaceuticals in wastewater using UPLC-high resolution mass spectrometry. Arch. Environ. Contam. Toxicol. 2015, 69, 525–534. [Google Scholar] [CrossRef] [PubMed]
- Armbruster, D.A.; Pry, T. Limit of Blank, Limit of Detection and Limit of Quantitation. Clin. Biochem. Rev. 2008, 29, S49. [Google Scholar] [PubMed]
- Verlicchi, P.; Al Aukidy, M.; Zambello, E. Occurrence of Pharmaceutical Compounds in Urban Wastewater: Removal, Mass Load and Environmental Risk After a Secondary Treatment-A Review. Sci. Total Environ. 2012, 429, 123–155. [Google Scholar] [CrossRef] [PubMed]
- European Community (EC). Technical Guidance Document on Risk Assessment in support of Commission Directive 93/67/EEC on Risk Assessment for new notified substances, Commission Regulation (EC) No 1488/94 on Risk Assessment for Existing Substances, and Directive 98/8/EC of the European Parliament and of the Council concerning the placing of biocidal products on the market, Parts I. European Communities, EUR 20418 EN/1. 2003. Available online: https://echa.europa.eu/documents/10162/16960216/tgdpart1_2ed_en.pdf (accessed on 31 May 2019).
- European Community (EC). Technical Guidance Document on Risk Assessment in support of Commission Directive 93/67/EEC on Risk Assessment for new notified substances, Commission Regulation (EC) No 1488/94 on Risk Assessment for Existing Substances, and Directive 98/8/EC of the European Parliament and of the Council concerning the placing of biocidal products on the market, Parts II. European Communities, EUR 20418 EN/1. 2003. Available online: https://echa.europa.eu/documents/10162/16960216/tgdpart2_2ed_en.pdf (accessed on 31 May 2019).
- European Community (EC). Technical Guidance Document on Risk Assessment in support of Commission Directive 93/67/EEC on Risk Assessment for new notified substances, Commission Regulation (EC) No 1488/94 on Risk Assessment for Existing Substances, and Directive 98/8/EC of the European Parliament and of the Council concerning the placing of biocidal products on the market, Parts IV. European Communities, EUR 20418 EN/1. 2003. Available online: https://echa.europa.eu/documents/10162/16960216/tgdpart4_2ed_en.pdf (accessed on 31 May 2019).
- Jelic, A.; Fatone, F.; Di Fabio, S.; Petrovic, M.; Cecchi, F.; Barcelo, D. Tracing pharmaceuticals in a municipal plant for integrated wastewater and organic solid waste treatment. Sci. Total Environ. 2012, 433, 352–361. [Google Scholar] [CrossRef] [PubMed]
- Verlicchi, P.; Zambello, E. Predicted and measured concentrations of pharmaceuticals in hospital effluents. Examination of the strengths and weaknesses of the two approaches through the analysis of a case study. Sci. Total Environ. 2016, 565, 82–94. [Google Scholar] [CrossRef] [PubMed]
- Verlicchi, P.; Al Aukidy, M.; Jelic, A.; Petrović, M.; Barceló, D. Comparison of Measured and Predicted Concentrations of Selected Pharmaceuticals in Wastewater and Surface Water: A Case Study of a Catchment Area in the Po Valley (Italy). Sci. Total Environ. 2014, 470, 844–854. [Google Scholar] [CrossRef] [PubMed]
- Lai, F.Y.; Ort, C.; Gartner, C.; Carter, S.; Prichard, J.; Kirkbride, P.; Bruno, R.; Hall, W.; Eaglesham, G.; Mueller, J.F. Refining the estimation of illicit drug consumptions from wastewater analysis: Co-analysis of prescription pharmaceuticals and uncertainty assessment. Water Res. 2011, 45, 4437–4448. [Google Scholar] [CrossRef]
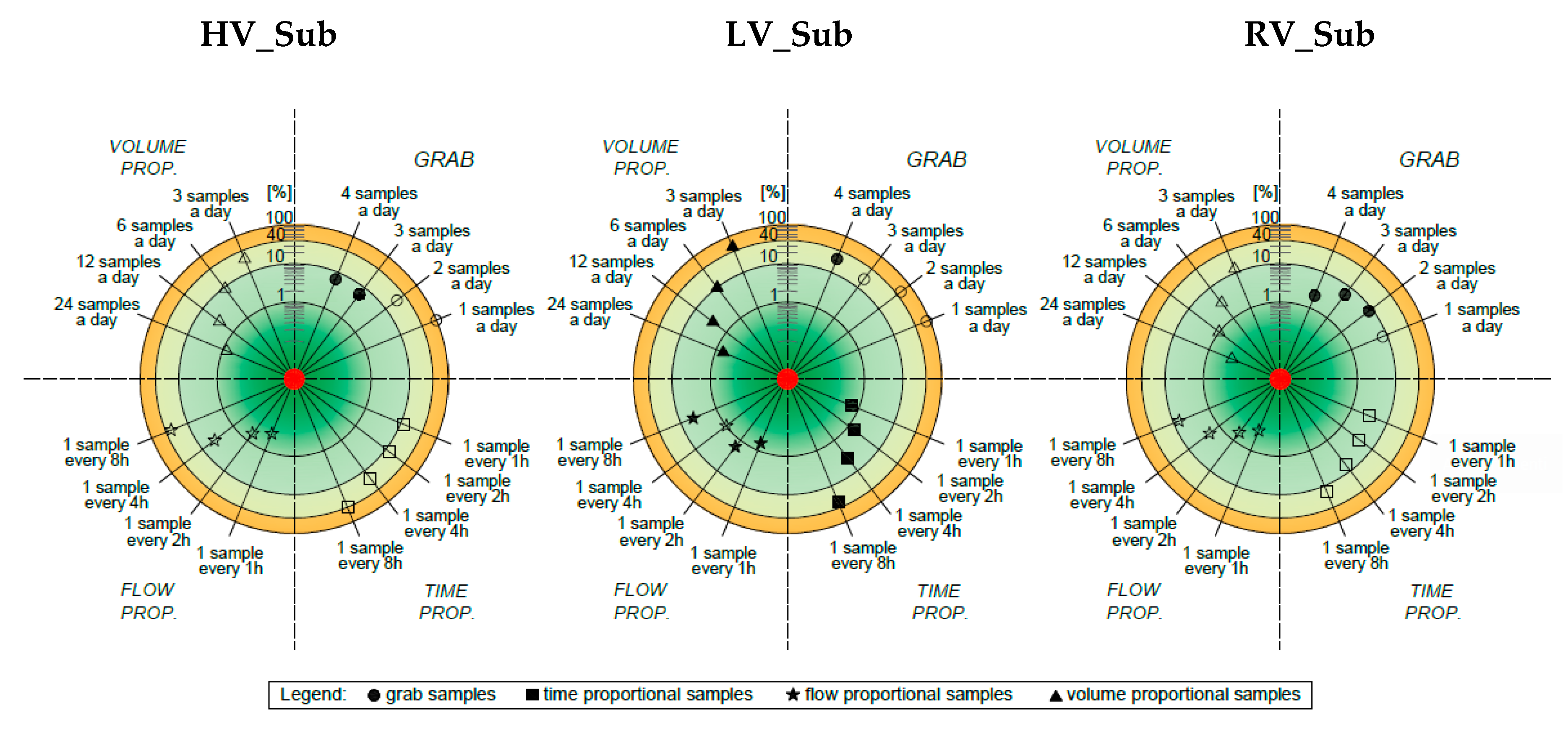

| Sampling | Description | Water Volume Sampled | Sampling Time, (Number of Samples) |
|---|---|---|---|
| Grab | The sampling consists of instantaneous (grab) wastewater withdrawal(s). The monitoring may include either one grab sample or a number of grab samples. The sampling time is defined by the investigation (monitoring protocol). | The requested wastewater volume for analysis | 8 a.m. (1) 8 a.m. + 5 p.m. (2) 8 a.m. + 12 p.m. + 5 p.m. (3) 8 a.m. + 12 p.m. + 4 p.m. + 11 p.m. (4) |
| 24-h time proportional composite | The sampling is performed at constant time intervals. It is the most common sampling mode. This is also called constant time, constant volume (CTCV) | A constant volume Vsample taken at each sampling instant | Every hour (24) Every 2 h (12) Every 4 h (6) Every 8 h (3) |
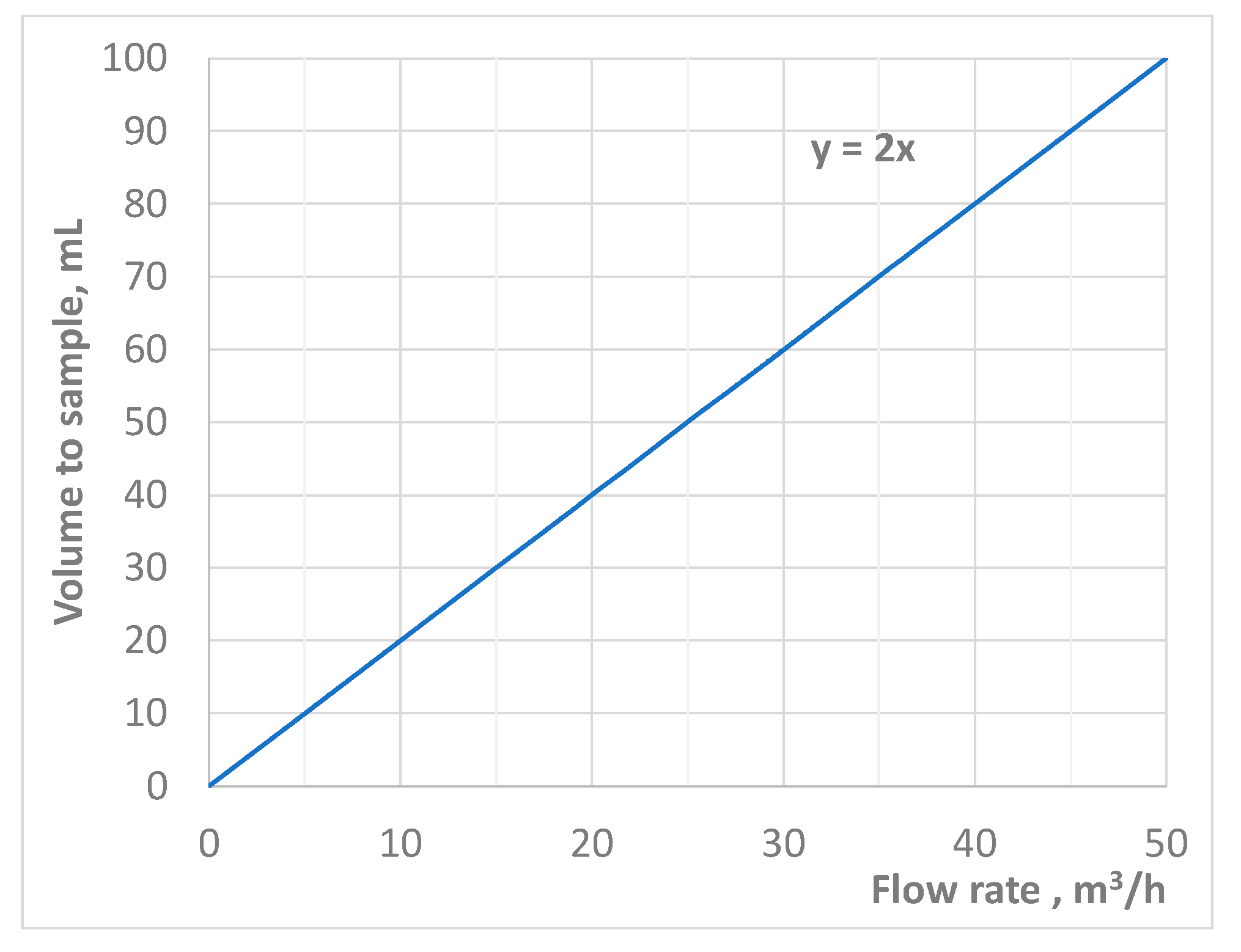
| 24-h flow proportional composite | The sampling is performed at constant time intervals. The volume of wastewater taken is proportional to the flow rate flowing at each instant of sampling. This is also called constant time, variable volume (CTVV) | A linear interpolation curve is defined between the minimum and maximum wastewater flow and wastewater sampled over the whole observed range of variability of the wastewater flow (see Figure 3) | Every hour (24) Every 2 h (12) Every 4 h (6) Every 8 h (3) |
| 24-h volume proportional composite | The sampling takes the same wastewater volume at variable time intervals, after a defined volume of wastewater has passed the sampling point. This is also called constant volume, variable time (CVVT) | A constant volume Vsample is taken at each defined sampling time | Frequency: Three times a day (3) Six times a day (6) Twelve times a day (12) Twenty-four times a day (24) |
| Sampling | Sampling Time for Influent and Effluent (Number of Samples) | Some Remarks and Number of Estimated Values of Removal Efficiencies in Brackets |
|---|---|---|
| Grab | Every hour (24), hydraulic retention time (HRT) not considered | Removal evaluated each hour (24 values) |
| Every hour (24), HRT considered | ||
| 8 a.m.; 5 p.m. (2) HRT not considered | Removal based on average values for influent and effluent (one value) | |
| 8 a.m.; 5 p.m. (2) HRT not considered | ||
| 8 a.m.; 12 p.m.; 5 p.m. (3) HRT not considered | ||
| 8 a.m.; 12 p.m.; 5 p.m. (3) HRT considered | ||
| 8 a.m.; 12 p.m.; 4 p.m.; 11 p.m. (4) HRT not considered | ||
| 8 a.m.; 12 p.m.; 4 p.m.; 11 p.m. (4) HRT considered | ||
| Time proportional | 24-h time proportional composite sample, time interval between two consecutive withdrawals equal to 1 h (1) | (One value) |
| Flow proportional | 24-h flow proportional composite sample, time interval between two consecutive withdrawals equal to 1 h (1) | (One value) |
| Volume proportional | 24-h volume proportional composite sample. Twenty-four samples a day mixed for the composite sample as reported in Table 1 (1) | (One value) |
| HV_Sub, ng/L | LV_Sub, ng/L | RV_Sub, ng/L |
|---|---|---|
| 24,561 ± 18,305 | 586 ± 377 | 29,609 ± 6674 |
| Number (#) of Grab Samples | HV_Sub, ng/L | LV_Sub, ng/L | RV_Sub, ng/L |
|---|---|---|---|
| 1 | 1000 | 112 | 31,852 |
| 2 | 19,041 | 287 | 31,954 |
| 3 | 26,014 | 478 | 31,301 |
| 4 | 26,117 | 724 | 30,263 |
| Interval (h), (#of Samples) | HV_Sub, ng/L | LV_Sub, ng/L | RV_Sub, ng/L |
|---|---|---|---|
| 1 (24) | 21,751 | 590 | 28,664 |
| 2 (12) | 21,518 | 595 | 28,472 |
| 4 (6) | 20,270 | 608 | 27,799 |
| 8 (3) | 14,535 | 750 | 25,409 |
| Interval (h), (#of Samples) | HV_Sub, ng/L | LV_Sub, ng/L | RV_Sub, ng/L |
|---|---|---|---|
| 1 (24) | 24,477 | 590 | 29,525 |
| 2 (12) | 24,412 | 596 | 29,443 |
| 4 (6) | 23,550 | 581 | 29,000 |
| 8 (3) | 17,406 | 612 | 27,543 |
| Frequency (#/d) | HV_Sub, ng/L | LV_Sub, ng/L | RV_Sub, ng/L |
|---|---|---|---|
| 3 | 18,848 | 888 | 25,948 |
| 6 | 22,359 | 644 | 28,702 |
| 12 | 23,867 | 602 | 29,314 |
| 24 | 24,365 | 590 | 29,541 |
| HV_Sub, g/d | LV_Sub, g/d | RV_Sub, g/d |
|---|---|---|
| 15.6 | 0.37 | 18.8 |
| Number of Grab Samples | Case 1: HRT not Considered | Case 2: HRT Considered |
|---|---|---|
| 2 | 68.9 | 71.2 |
| 3 | 65.9 | 75.3 |
| 4 | 64.3 | 71.1 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Verlicchi, P.; Ghirardini, A. Occurrence of Micropollutants in Wastewater and Evaluation of Their Removal Efficiency in Treatment Trains: The Influence of the Adopted Sampling Mode. Water 2019, 11, 1152. https://doi.org/10.3390/w11061152
Verlicchi P, Ghirardini A. Occurrence of Micropollutants in Wastewater and Evaluation of Their Removal Efficiency in Treatment Trains: The Influence of the Adopted Sampling Mode. Water. 2019; 11(6):1152. https://doi.org/10.3390/w11061152
Chicago/Turabian StyleVerlicchi, Paola, and Andrea Ghirardini. 2019. "Occurrence of Micropollutants in Wastewater and Evaluation of Their Removal Efficiency in Treatment Trains: The Influence of the Adopted Sampling Mode" Water 11, no. 6: 1152. https://doi.org/10.3390/w11061152
APA StyleVerlicchi, P., & Ghirardini, A. (2019). Occurrence of Micropollutants in Wastewater and Evaluation of Their Removal Efficiency in Treatment Trains: The Influence of the Adopted Sampling Mode. Water, 11(6), 1152. https://doi.org/10.3390/w11061152






