On the Roles of Advection and Solar Heating in Seasonal Variation of the Migrating Diurnal Tide in the Stratosphere, Mesosphere, and Lower Thermosphere
Abstract
1. Introduction
2. Model, Data and Method
2.1. Model
2.2. Thermodynamic Budget Equations
2.3. Three Latitudinal Structures of DW1 in Three Regions
3. Results
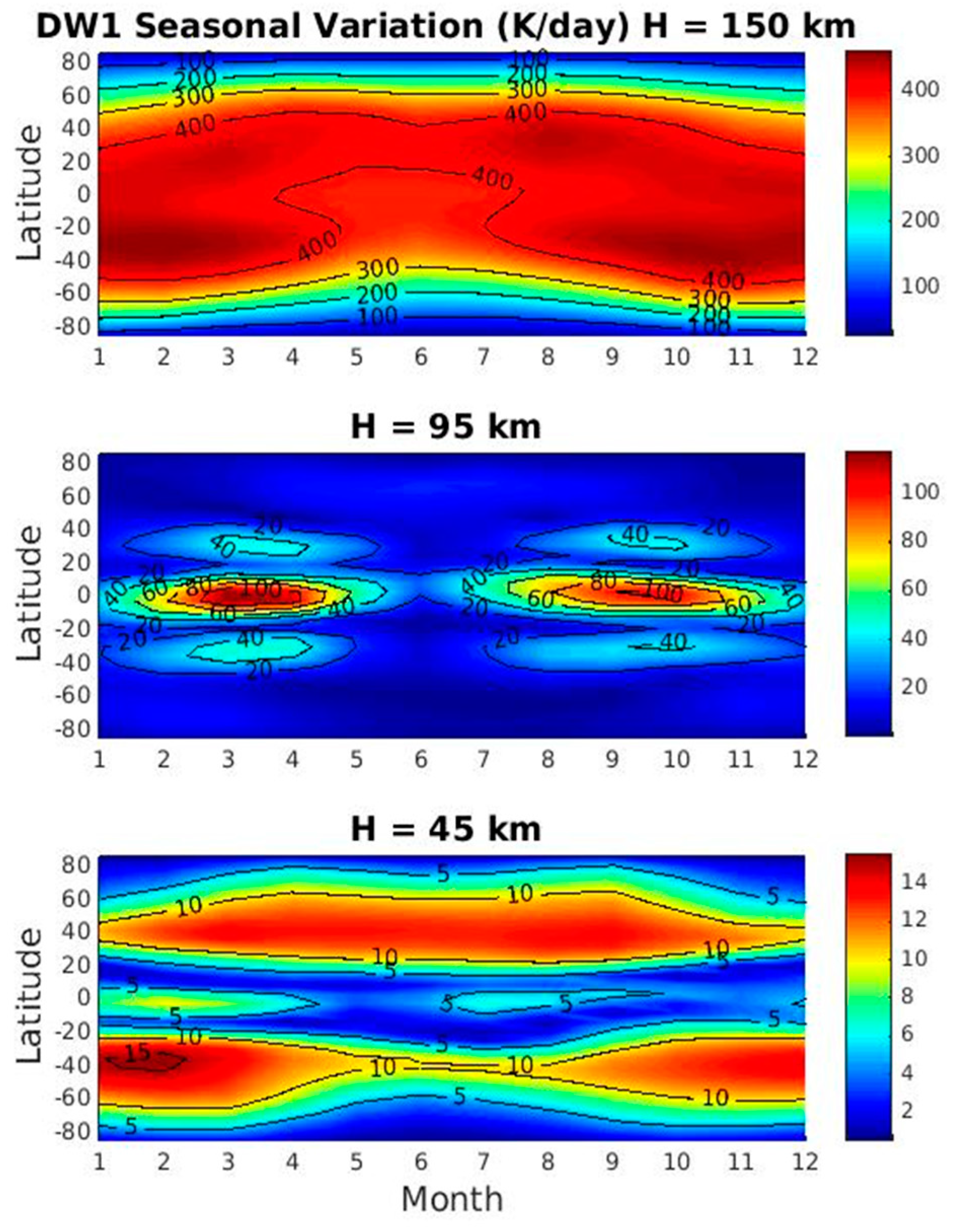
3.1. Seasonal Variations of DW1 in Three Regions
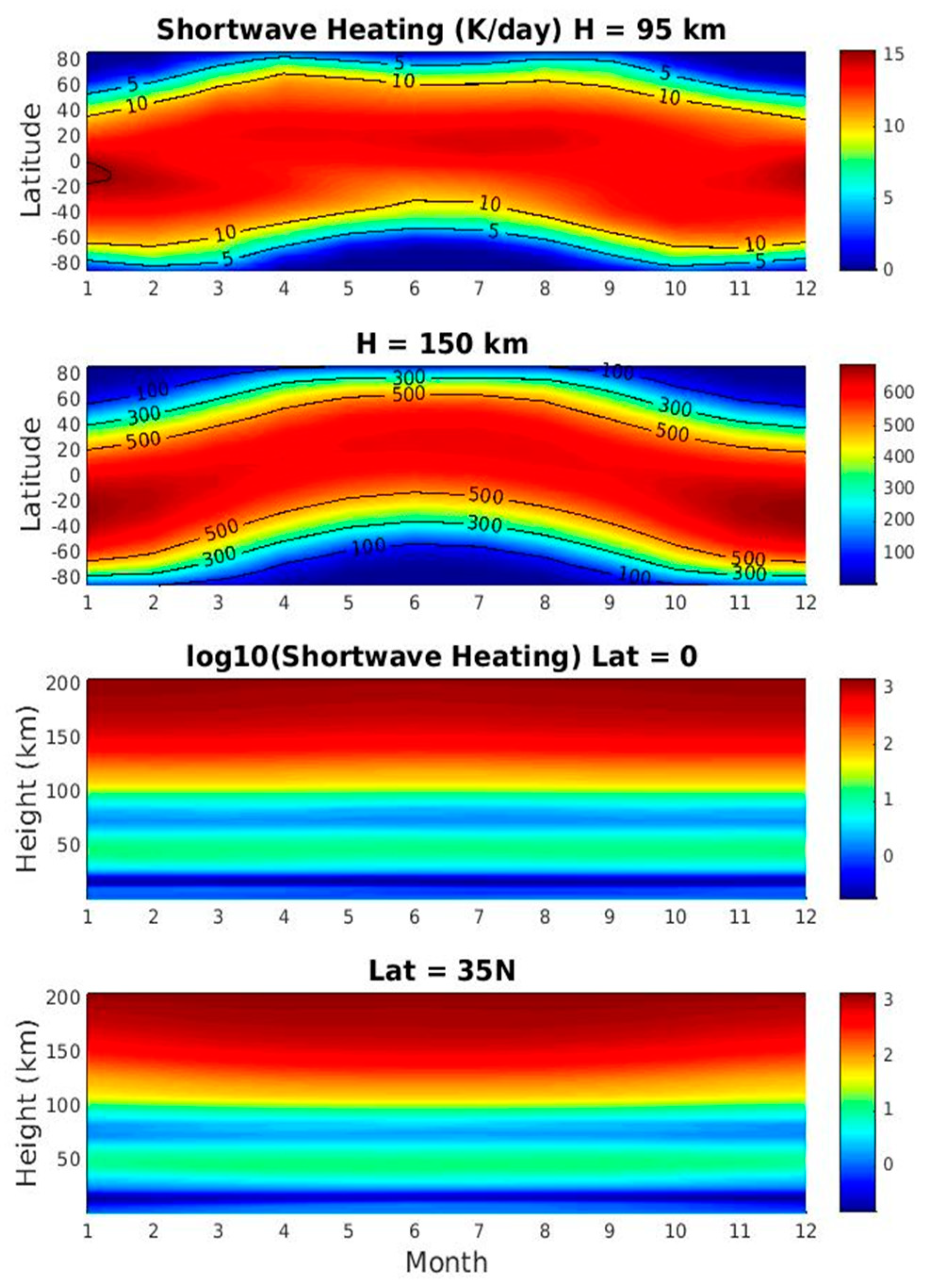
3.2. The Role of Solar Heating
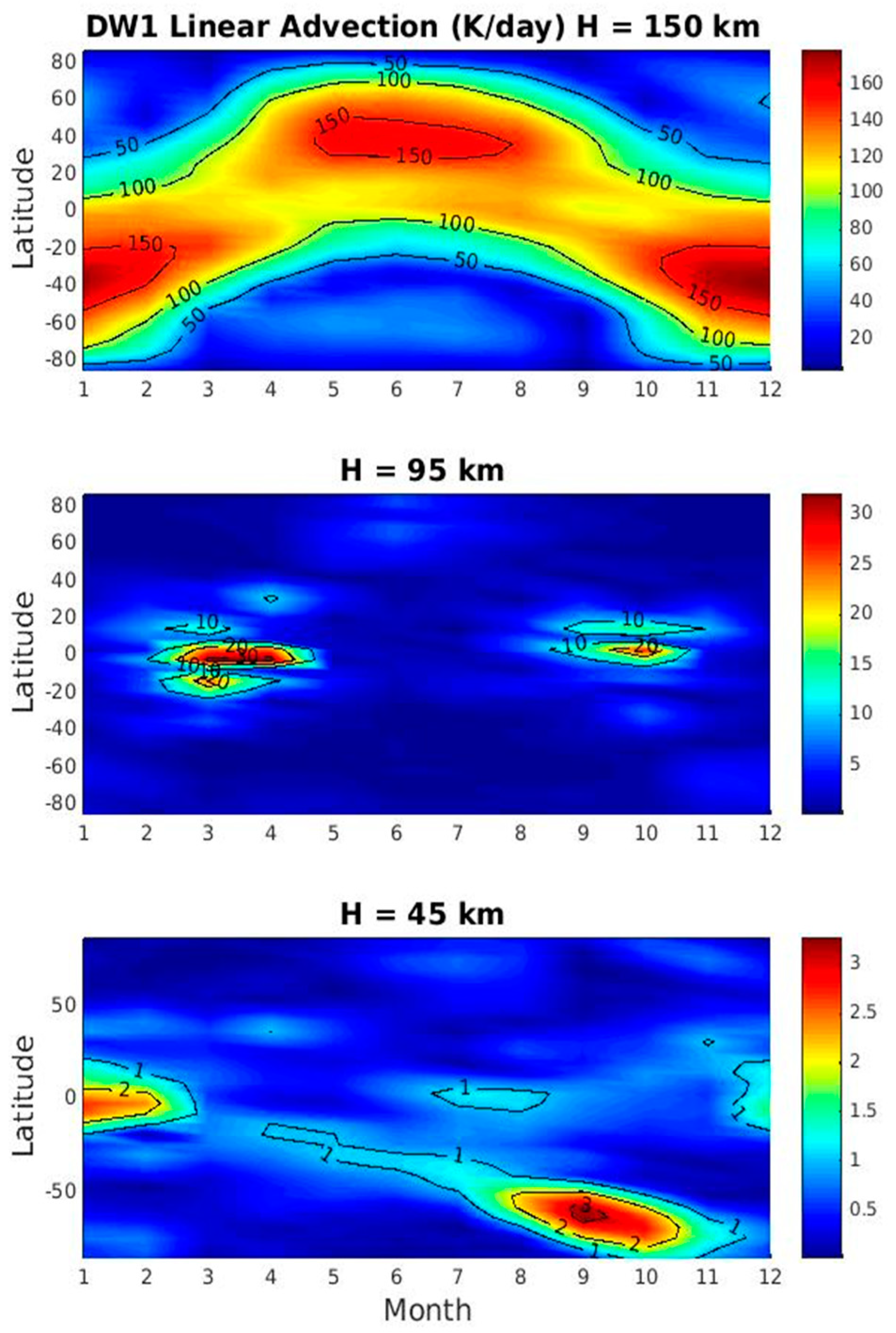
3.3. The Role of the Wave-Mean Flow Interactions (Linear Advection)
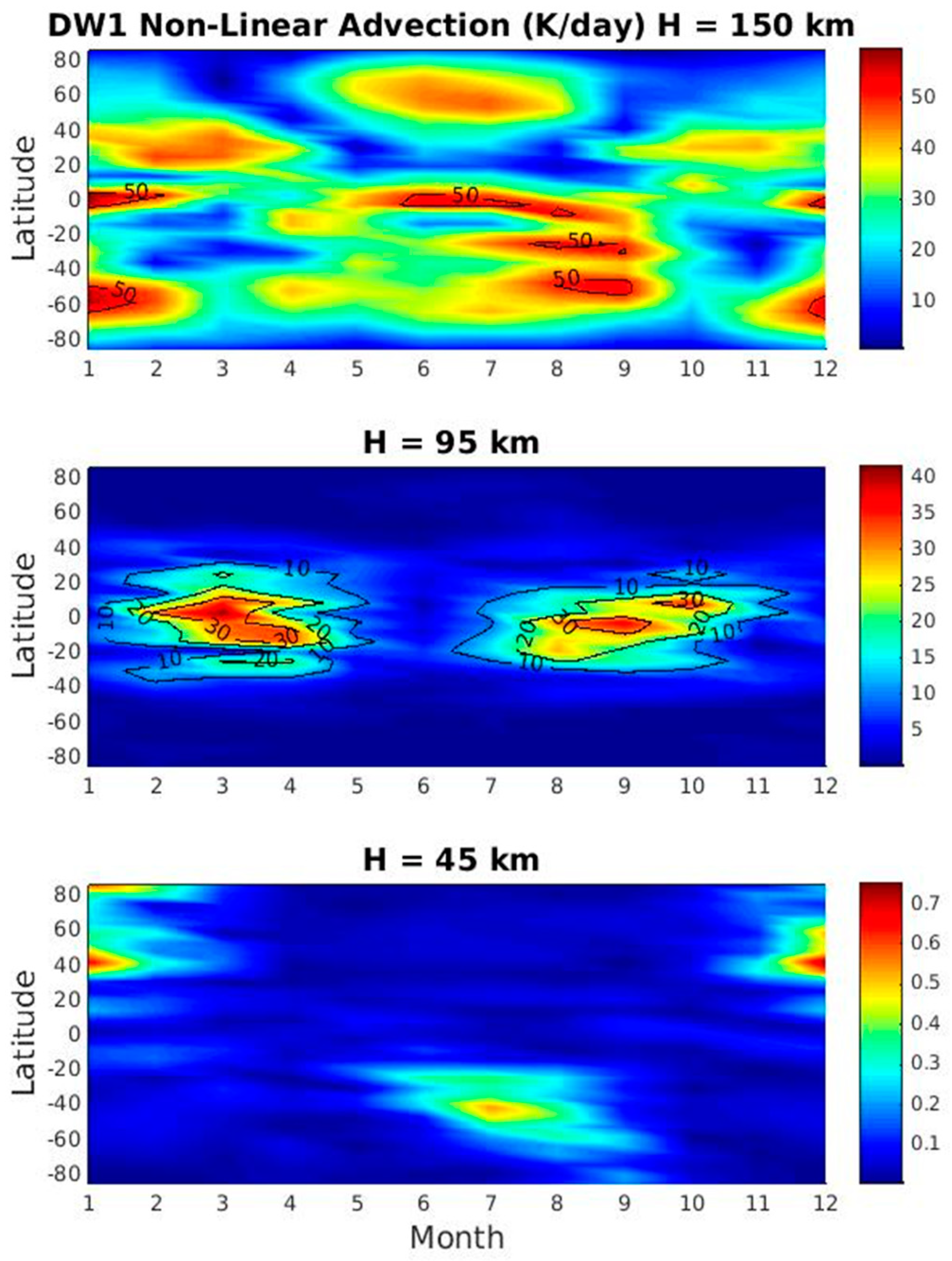
3.4. The Role of the Wave-Wave Interactions (Nonlinear Advection)
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Groves, G. Hough components of ozone heating. J. Atmos. Sol. Terr. Phys. 1982, 44, 111–121. [Google Scholar] [CrossRef]
- Groves, G. Hough components of water vapour heating. J. Atmos. Sol. Terr. Phys. 1982, 44, 281–290. [Google Scholar] [CrossRef]
- Forbes, J.M. Tidal and planetary waves, The upper mesosphere and lower thermosphere: A review of experiment and theory. Geophys. Monogr. Ser. 1995, 67–87. [Google Scholar] [CrossRef]
- Hagan, M.; Forbes, J.M. Migrating and nonmigrating diurnal tides in the middle and upper atmosphere excited by tropospheric latent heat release. J. Geophys. Res. 2002, 107, 4754. [Google Scholar] [CrossRef]
- Hagan, M.; Forbes, J.M. Migrating and nonmigrating semidiurnal tides in the upper atmosphere excited by tropospheric latent heat release. J. Geophys. Res. 2003, 108, 1062. [Google Scholar] [CrossRef]
- Teitelbaum, H.; Vial, F. On tidal variability induced by nonlinear interaction with planetary waves. J. Geophys. Res. 1991, 96, 14169–14178. [Google Scholar] [CrossRef]
- Beard, A.; Mitchell, N.; Williams, P.; Kunitake, M. Non-linear interactions between tides and planetary waves resulting in periodic tidal variability. J. Atmos. Sol. Terr. Phys. 1999, 61, 363–376. [Google Scholar] [CrossRef]
- Angelats i Coll, M.; Forbes, J.M. Nonlinear interactions in the upper atmosphere: The s = 1 and s = 3 nonmigrating semidiurnal tides. J. Geophys. Res. 2002, 107, 1157. [Google Scholar] [CrossRef]
- Hagan, M.; Roble, R.; Hackney, J. Migrating thermospheric tides. J. Geophys. Res. 2001, 106, 12739–12752. [Google Scholar] [CrossRef]
- Oberheide, J.; Hagan, M.E.; Richmond, A.D.; Forbes, J.M. Atmospheric Tides. In Encyclopedia of Atmospheric Sciences, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2015; Volume 2, pp. 287–297. ISBN 9780123822253. [Google Scholar]
- Williams, P.; Mitchell, N.; Beard, A.; Howells, V.S.C.; Muller, H. The coupling of planetary waves, tides and gravity waves in the mesosphere and lower thermosphere. Adv. Space Res. 1999, 24, 1571–1576. [Google Scholar] [CrossRef]
- Ortland, D.A.; Alexander, M.J. Gravity wave influence on the global structure of the diurnal tide in the mesosphere and lower thermosphere. J. Geophys. Res. 2006, 111, A10S10. [Google Scholar] [CrossRef]
- Palo, S.; Forbes, J.M.; Zhang, X.; Russell, J.; Mlynczak, M. An eastward propagating two-day wave: Evidence for nonlinear planetary wave and tidal coupling in the mesosphere and lower thermosphere. Geophys. Res. Lett. 2007, 34, L07807. [Google Scholar] [CrossRef]
- Agner, R.; Liu, A.Z. Local time variation of gravity wave momentum fluxes and their relationship with the tides derived from LIDAR measurements. J. Atmos. Sol. Terr. Phys. 2015, 135, 136–142. [Google Scholar] [CrossRef]
- Kazimirovsky, E.; Herraiz, M.; De la Morena, B. Effects on the ionosphere due to phenomena occurring below it. Surv. Geophys. 2003, 24, 139–184. [Google Scholar] [CrossRef]
- Immel, T.J.; Sagawa, E.; England, S.L.; Henderson, S.B.; Hagan, M.E.; Mende, S.B.; Frey, H.U.; Swenson, C.M.; Paxton, L.J. Control of equatorial ionospheric morphology by atmospheric tides. Geophys. Res. Lett. 2006, 33, L15108. [Google Scholar] [CrossRef]
- Yamazaki, Y.; Richmond, A.D. A theory of ionospheric response to upward-propagating tides: Electrodynamic effects and tidal mixing effects. J. Geophys. Res. 2013, 118, 5891–5905. [Google Scholar] [CrossRef]
- Negrea, C.; Zabotin, N.; Bullett, T.; Codrescu, M.; Fuller-Rowell, T. Ionospheric response to tidal waves measured by dynasonde techniques. J. Geophys. Res. 2016, 121, 602–611. [Google Scholar] [CrossRef]
- McLandress, C. The seasonal variation of the propagating diurnal tide in the mesosphere and lower thermosphere. Part I: The role of gravity waves and planetary waves. J. Atmos. Sci. 2002, 59, 893–906. [Google Scholar] [CrossRef]
- McLandress, C. The seasonal variation of the propagating diurnal tide in the mesosphere and lower thermosphere. Part II: The role of tidal heating and zonal mean winds. J. Atmos. Sci. 2002, 59, 907–922. [Google Scholar] [CrossRef]
- Hays, P.B.; Wu, D. HRDI Science Team, Observations of the diurnal tide from space. J. Atmos. Sci. 1994, 51, 3077–3093. [Google Scholar] [CrossRef]
- Zeng, Z.; Randel, W.; Sokolovskiy, S.; Deser, C.; Kuo, Y.-H.; Hagan, M.; Du, J.; Ward, W.E. Detection of migrating diurnal tide in the tropical upper troposphere and lower stratosphere using the Challenging Minisatellite Payload radio occultation data. J. Geophys. Res. 2008, 113, D03102. [Google Scholar] [CrossRef]
- Mukhtarov, P.; Pancheva, D.; Andonov, B. Global structure and seasonal and interannual variability of the migrating diurnal tide seen in the SABER/TIMED temperatures between 20 and 120 km. J. Geophys. Res. 2009, 114, A02309. [Google Scholar] [CrossRef]
- Sakazaki, T.; Fujiwara, M.; Zhang, X.; Hagan, M.; Forbes, J. Diurnal tides from the troposphere to the lower mesosphere as deduced from TIMED/SABER satellite data and six global reanalysis data sets. J. Geophys. Res. 2012, 117, D13108. [Google Scholar] [CrossRef]
- Smith, A.K. Global dynamics of the MLT. Surv. Geophys. 2012, 33, 1177–1230. [Google Scholar] [CrossRef]
- Oberheide, J.; Forbes, J.; Zhang, X.; Bruinsma, S. Climatology of upward propagating diurnal and semidiurnal tides in the thermosphere. J. Geophys. Res. 2011, 116, A11306. [Google Scholar] [CrossRef]
- Forbes, J.M.; Zhang, X.; Bruinsma, S.; Oberheide, J. Sun-synchronous thermal tides in exosphere temperature from CHAMP and GRACE accelerometer measurements. J. Geophys. Res. 2011, 116, A11309. [Google Scholar] [CrossRef]
- Jones, M., Jr.; Forbes, J.M.; Hagan, M.E.; Maute, A. Impacts of vertically propagating tides on the mean state of the ionosphere-thermosphere system. J. Geophys. Res. Space Phys. 2014, 119, 2197–2213. [Google Scholar] [CrossRef]
- Siskind, D.E.; Drob, D.P.; Dymond, K.F.; McCormack, J.P. Simulations of the effects of vertical transport on the thermosphere and ionosphere using two coupled models. J. Geophys. Res. Space Phys. 2014, 119, 1172–1185. [Google Scholar] [CrossRef]
- Lieberman, R.S.; Riggin, D.M.; Ortland, D.A.; Nesbitt, S.W.; Vincent, R.A. Variability of mesospheric diurnal tides and tropospheric diurnal heating during 1997–1998. J. Geophys. Res. 2007, 112, D20110. [Google Scholar] [CrossRef]
- Akmaev, R. Simulation of large-scale dynamics in the mesosphere and lower thermosphere with the Doppler-spread parameterization of gravity waves: 2. Eddy mixing and the diurnal tide. J. Geophys. Res. 2001, 106, 1205–1213. [Google Scholar] [CrossRef]
- Hagan, M.; Forbes, J.; Vial, F. On modeling migrating solar tides. Geophys. Res. Lett. 1995, 22, 893–896. [Google Scholar] [CrossRef]
- Hagan, M.; Burrage, M.D.; Forbes, J.; Hackney, J.; Randel, W.; Zhang, X. GSWM-98: Results for migrating solar tides. J. Geophys. Res. 1999, 104, 6813–6827. [Google Scholar] [CrossRef]
- McLandress, C. Seasonal variability of the diurnal tide: Results from the Canadian middle atmosphere general circulation model. J. Geophys. Res. 1997, 102, 29747–29764. [Google Scholar] [CrossRef]
- Lieberman, R.; Ortland, D.; Riggin, D.; Wu, Q.; Jacobi, C. Momentum budget of the migrating diurnal tide in the mesosphere and lower thermosphere. J. Geophys. Res. 2010, 115, D20105. [Google Scholar] [CrossRef]
- Lu, X.; Liu, H.L.; Liu, A.; Yue, J.; McInerney, J.; Li, Z. Momentum budget of the migrating diurnal tide in the Whole Atmosphere Community Climate Model at vernal equinox. J. Geophys. Res. 2012, 117, D07112. [Google Scholar] [CrossRef]
- Gan, Q.; Du, J.; Ward, W.E.; Beagley, S.R.; Fomichev, V.I.; Zhang, S.D. Climatology of the diurnal tides from eCMAM30 (1979 to 2010) and its comparison with SABER. Earth Planets Space 2014, 66, 103. [Google Scholar] [CrossRef]
- Hagan, M.E. Comparative effects of migrating solar sources on tidal signatures in the middle and upper atmosphere. J. Geophys. Res. 1996, 101, 21213–21222. [Google Scholar] [CrossRef]
- Liu, H.L.; Foster, B.T.; Hagan, M.E.; McInerney, J.M.; Maute, A.; Qian, L.; Richmond, A.D.; Roble, R.G.; Solomon, S.C.; Garcia, R.R.; et al. Thermosphere extension of the Whole Atmosphere Community Climate Model. J. Geophys. Res. 2010, 115, A12302. [Google Scholar] [CrossRef]
- García-Comas, M.; González-Galindo, F.; Funke, B.; Gardini, A.; Jurado-Navarro, A.; López-Puertas, M.; Ward, W.E. MIPAS observations of longitudinal oscillations in the mesosphere and the lower thermosphere: Climatology of odd-parity daily frequency modes. Atmos. Chem. Phys. 2016, 16, 11019–11041. [Google Scholar] [CrossRef]
- Beagley, S.R.; McLandress, C.; Fomichev, V.I.; Ward, W.E. Extended Canadian middle atmosphere model. Geophys. Res. Lett. 2000, 27, 2529–2532. [Google Scholar] [CrossRef]
- Fomichev, V.; Ward, W.E.; Beagley, S.; McLandress, C.; McConnell, J.; McFarlane, N.; Shepherd, T. Extended Canadian Middle Atmosphere Model: Zonal-mean climatology and physical parameterizations. J. Geophys. Res. 2002, 107, ACL 9-1–ACL 9-14. [Google Scholar] [CrossRef]
- McLandress, C.; Ward, W.E.; Fomichev, V.; Semeniuk, K.; Beagley, S.; McFarlane, N.; Shepherd, T. Large-scale dynamics of the mesosphere and lower thermosphere: An analysis using the extended Canadian Middle Atmosphere Model. J. Geophys. Res. 2006, 111, D17111. [Google Scholar] [CrossRef]
- Hines, C.O. Doppler-spread parameterization of gravity-wave momentum deposition in the middle atmosphere. 1. Basic formulation. J. Atmos. Sol. Terr. Phys. 1997, 59, 371–386. [Google Scholar] [CrossRef]
- Hines, C.O. Doppler-spread parameterization of gravity-wave momentum deposition in the middle atmosphere. 2. Broad and quasi monochromatic spectra, and implementation. J. Atmos. Sol. Terr. Phys. 1997, 59, 387–400. [Google Scholar] [CrossRef]
- Du, J.; Ward, W.E.; Oberheide, J.; Nakamura, T.; Tsuda, T. Semidiurnal tides from the extended Canadian Middle Atmosphere Model (CMAM) and comparisons with TIMED Doppler interferometer (TIDI) and meteor radar observations. J. Atmos. Sol. Terr. Phys. 2007, 69, 2159–2202. [Google Scholar] [CrossRef]
- Du, J.; Ward, W.E. Terdiurnal tide in the extended Canadian Middle Atmospheric Model (CMAM). J. Geophys. Res. 2010, 115, D24106. [Google Scholar] [CrossRef]
- Du, J.; Ward, W.E.; Cooper, F. The character of polar tidal signatures in the extended Canadian Middle Atmosphere Model. J. Geophys. Res. 2014, 119, 5928–5948. [Google Scholar] [CrossRef]
- de Grandpré, J.; Beagley, S.R.; Fomichev, V.I.; Griffioen, E.; McConnell, J.C.; Medvedev, A.S.; Shepherd, T.G. Ozone climatology using interactive chemistry: Results from the Canadian Middle Atmosphere Model. J. Geophys. Res. 2000, 105, 26475–26491. [Google Scholar] [CrossRef]
- Beagley, S.R.; Boone, C.D.; Fomichev, V.I.; Jin, J.J.; Semeniuk, K.; McConnell, J.C.; Bernath, P.F. First multi-year occultation observations of CO2 in the MLT by ACE satellite: Observations and analysis using the extended CMAM. Atmos. Chem. Phys. 2010, 10, 1133–1153. [Google Scholar] [CrossRef]
- Shepherd, M.G.; Beagley, S.R.; Fomichev, V.I. Stratospheric warming influence on the mesosphere/lower thermosphere as seen by the extended CMAM. Ann. Geophys. 2014, 32, 589–608. [Google Scholar] [CrossRef]
- Dee, D.; Uppala, S.; Simmons, A.; Berrisford, P.; Poli, P.; Kobayashi, S.; Andrae, U.; Balmaseda, M.; Balsamo, G.; Bauer, P. The ERA-Interim reanalysis: Configuration and performance of the data assimilation system. Q. J. R. Meteorol. Soc. 2011, 137, 553–597. [Google Scholar] [CrossRef]
- McLandress, C.; Scinocca, J.F.; Shepherd, T.G.; Reader, M.C.; Manney, G.L. Dynamical control of the mesosphere by orographic and nonorographic gravity wave drag during the extended northern winters of 2006 and 2009. J. Atmos. Sci. 2013, 70, 2152–2169. [Google Scholar] [CrossRef]
- Forster, P.M.; Fomichev, V.I.; Rozanov, E.; Cagnazzo, C.; Jonsson, A.I.; Langematz, U.; Fomin, B.; Iacono, M.J.; Mayer, B.; Mlawer, E.; et al. Evaluation of radiation scheme performance within chemistry climate models. J. Geophys. Res. 2011, 116. [Google Scholar] [CrossRef]
- Semeniuk, K.; Fomichev, V.I.; McConnell, J.C.; Fu, C.; Melo, S.M.L.; Usoskin, I.G. Middle atmosphere response to the solar cycle in irradiance and ionizing particle precipitation. Atmos. Chem. Phys. 2011, 11, 5045–5077. [Google Scholar] [CrossRef]
- Chapman, S.; Lindzen, R.S. Atmospheric Tides: Thermal and Gravitational; Gordon and Breach: New York, NY, USA, 1970; 200p. [Google Scholar]
- Hagan, M.E.; Häusler, K.; Lu, G.; Forbes, J.M.; Zhang, X. Upper thermospheric responses to forcing from above and below during 1–10 April 2010: Results from an ensemble of numerical simulations. J. Geophys. Res. Space Phys. 2015, 120, 3160–3174. [Google Scholar] [CrossRef]


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gu, H.; Du, J. On the Roles of Advection and Solar Heating in Seasonal Variation of the Migrating Diurnal Tide in the Stratosphere, Mesosphere, and Lower Thermosphere. Atmosphere 2018, 9, 440. https://doi.org/10.3390/atmos9110440
Gu H, Du J. On the Roles of Advection and Solar Heating in Seasonal Variation of the Migrating Diurnal Tide in the Stratosphere, Mesosphere, and Lower Thermosphere. Atmosphere. 2018; 9(11):440. https://doi.org/10.3390/atmos9110440
Chicago/Turabian StyleGu, Hongping, and Jian Du. 2018. "On the Roles of Advection and Solar Heating in Seasonal Variation of the Migrating Diurnal Tide in the Stratosphere, Mesosphere, and Lower Thermosphere" Atmosphere 9, no. 11: 440. https://doi.org/10.3390/atmos9110440
APA StyleGu, H., & Du, J. (2018). On the Roles of Advection and Solar Heating in Seasonal Variation of the Migrating Diurnal Tide in the Stratosphere, Mesosphere, and Lower Thermosphere. Atmosphere, 9(11), 440. https://doi.org/10.3390/atmos9110440



