A Detailed Limited-Area Atmospheric Energy Cycle for Climate and Weather Studies

Abstract

Lorenz’ seminal work on global atmospheric energetics improved our understanding of the general circulation. With the advent of Regional Climate Models (RCMs), it is important to have a limited-area energetic budget available that is applicable for both weather and climate, analogous to Lorenz’ global atmospheric energetics. A regional-scale energetic budget is obtained in this study by applying Reynolds decomposition rules to quadratic forms of the kinetic energy K and the available enthalpy A, to obtain time mean and time deviation contributions. According to the employed definition, the time mean energy contributions are decomposed in a component associated with the time-averaged atmospheric state and a component due to the time-averaged statistics of transient eddies; these contributions are suitable for the study of the climate over a region of interest. Energy fluctuations (the deviations of instantaneous energies from their climate value) that are appropriate for weather studies are split into quadratic and linear contributions. The sum of all the contributions returns exactly to the total primitive kinetic energy and available enthalpy equations.

1. Introduction

Atmospheric energetics identifies the different reservoirs of energy in the atmosphere and, according to conservation laws, describes the conversions or flow of energy from one reservoir to another. In this context, mechanisms responsible for those conversions are physical processes. Therefore, an atmospheric energy cycle identifies different physical processes responsible for the growth or the decay of the mechanical and thermal energies. Lorenz [1,2] provided the first modern picture of the energies and their conversions for the global atmosphere, which is called the Lorenz energy cycle. He formulated a global, dry atmosphere for the concept of Available Potential Energy (APE), which is the difference between the current energy state of the atmosphere and a reference state with minimum energy (e.g., [3]). Such an energy cycle can help diagnose the atmospheric dynamics and general circulation, which is widely used in studies of the atmosphere (e.g., [4]). Ref. [1] demonstrated that the general circulation is characterized by a conversion of zonal available potential energy, which is generated by net diabatic low-latitude heating and high-latitude cooling, to eddy available potential energy, to eddy kinetic energy, and to zonal kinetic energy. This energy cycle has also been applied to other Earth-like planets [5] and Mars [6]. Ref. [7] suggested a new definition of APE as having its source as the mean (uniform) heating rate, and its sink as the energy made available for conversion by the heating distribution, i.e., the APE source. These definitions lead to an expression of APE that is proportional to the global mean temperature variance, thus removing the Lorenz’ reference state concept. Ref. [8] quantitatively investigated the Lorenz energy cycle formalism for processes of energy transformations related to the dynamics of the atmosphere using contemporary high-resolution reanalysis data from the Japanese Meteorological Agency (JRA-55). They found significant trends in the conversion rates between zonal available potential and kinetic energy, consistent with an expansion of the Hadley cell, and a statistically significant trend in the eddy available potential energy, especially in the transient eddy available potential energy in the Southern Hemisphere. They also found that significant global and hemispheric energy fluctuations are caused by the El Niño–Southern Oscillation, the Arctic Oscillation, the Southern Annular Mode, and the meridional temperature gradient over the Southern Hemisphere. As defined by Lorenz, the energy cycle of the atmosphere comprises a methodology that is exploited in the diagnosis of atmospheric dynamics; however, because the available potential energy concept in the Lorenz energy cycle is defined over the entire atmosphere, the application of the energetics approach to local or regional scales presents a number of limitations [8]. Ref. [9] employed a modified Lorenz energy cycle (LEC) framework to understand the atmospheric circulation on tidally locked terrestrial planets. They found that both the baroclinic path (associated with strong baroclinic instabilities) and the barotropic path (associated with Hadley cells) are efficient. However, because of a large day–night surface temperature contrast and small rotation rate, the overturning circulation extends to the globe and the barotropic conversion dominates. Ref. [10] examined the formation and maintenance mechanisms of the sub-seasonal eastern Pacific pattern from an energetics perspective. Their results showed that both the baroclinic and the barotropic energy conversions act as major kinetic energy (KE) contributions.

Ref. [11] proposed the concept of atmospheric perturbation potential energy (PPE), reflecting the maximum amount of total potential energy that can be converted into kinetic energy at the regional scale. Their atmospheric PPE theory has been successfully applied to atmospheric energetics research into regional climate variability, such as the East Asia summer monsoon, South China Sea summer monsoon, Indian Ocean dipole and the El Niño–Southern Oscillation [12,13,14]. Ref. [15] re-examined the concept of available energy to define it locally in order to allow for studies of energy conversions within open domains (cyclones, baroclinic waves), with boundary fluxes being taken into account. He successfully derived a local energy cycle without approximation in an energetic balance, obtained when following the motion of an atmospheric parcel on pressure levels. Therefore, this energy cycle can be applied to any pressure level of any limited-area atmospheric domain. Inspired by Marquet, [16], noted “NL13” hereafter, established an approximate energy cycle that allows the study of energetics associated with the time fluctuations of inter-member spread (or internal variability) in an ensemble of simulations of a nested, limited-area model driven by a given set of lateral boundary conditions. This work was possible by establishing a close parallel between the energy conversions associated with time fluctuations of internal variability in ensemble simulations and the energy conversions taking place in weather systems. Following this formalism, Ref. [17] confirmed the current mid-latitude understanding that available enthalpy of the atmospheric time mean state is mainly generated by the covariance of diabatic heating and temperature, and that the most important conversions of energy are found to correspond to baroclinic processes that take place along the storm track, where perturbations of temperature and wind are important.

Local energy cycles are in principle suitable for climate studies, and some authors (e.g., [18]) have adapted them to weather studies. However, a specific energy cycle is required for weather studies. Ref. [17] attempted to establish a storm energy cycle, but their approach suffered a weakness in the treatment of transient eddies that did not vanish identically when averaged in the limit of long time as they should. The purpose of this paper is to establish both time-averaged and time variability energy cycles using a single approach that benefits from the quadratic form of main energy reservoirs. Local algebraic expression for a time-averaged energy cycle will be suitable for climate, climate change and internal variability studies, while a time variability energy cycle is suitable for storms, cyclones and baroclinic waves studies.

The paper is organized as follows: Section 2 will develop of quadratic forms of atmospheric energy reservoirs and introduce the decomposition into their time average and their time variability for climate and weather studies, respectively; Section 3 and Section 4 will develop the energy budgets for the climate and the weather, respectively, and verify that their sum returns the total energy. Section 5 shows an example of result obtained during the passage of an African Easterly Wave (AEW) over South Sudan in September 2006, and it announces forthcoming work applying this formalism to AEWs and the West African Monsoon (WAM). Section 6 concludes and summarizes the findings of this paper.

2. Quadratic Energy Reservoirs in the Atmosphere and Associated Decomposition Rules

The interpretation of the physical mechanisms responsible for the maintenance of the atmospheric general circulation and the life cycle of weather systems is facilitated by the decomposition of atmospheric variables and energies into their time mean and transient eddy components. Refs. [1,19] has shown that casting the thermodynamic energy equation in the form of a quadratic quantity such as Available Potential Energy (APE), has the advantage of focusing on the active component of thermodynamic energy available to produce storms. APE is a positive definite quantity that is much smaller in magnitude than total thermodynamic energy. The quadratic formulation of APE parallels that of kinetic energy, which facilitates the decomposition in time mean and transient eddy components. The APE approach has been widely used for global atmospheric energetic studies.

However, regional energetic studies require a somewhat different approach because “APE is a global concept defined for a system as a whole, not for a portion of it” (van Mieghem 1973, section 14.8). Refs. [15,20,21,22] proposed a locally defined approach based on Available Enthalpy, a positive definite quantity that can be split into contributions depending solely on temperature and pressure. For the moderate range of temperatures encountered in the atmosphere, a quadratic approximation to the temperature-dependent component of Available Enthalpy (henceforth noted as A) can be used:

$$A=\frac{C_p}{2T_r}{\left(T-T_r\right)}^2$$

(1)

where $T_r$ as the reference temperature and $C_p$ as the specific heat at constant pressure for dry air are constants [15]. The reference temperature $T_r$ does not have a physical interpretation; it simply arises as part of the process of splitting the Available Enthalpy between into contributions depending solely on temperature and pressure. With this approximation, the expression for available enthalpy parallels the quadratic form of the kinetic energy:

$$K=\frac{1}{2}\overrightarrow{V_h}\cdot \overrightarrow{V_h}$$

(2)

and it can be used advantageously for energetic studies over limited-area domains (where $\overrightarrow{V_h}$ is the horizontal wind).

The quadratic form of the kinetic energy $K$ and available enthalpy $A$ is very convenient for their decomposition in time mean and time deviation contributions. Consider a quadratic quantity $E={\psi }^2$ decomposed as $\psi =\langle \psi \rangle +{\psi }^{\prime }$ where < > denotes the time mean and ( )’ the departure thereof. Then, $E={\langle \psi \rangle }^2+{\psi }^{\prime 2}+2\langle \psi \rangle {\psi }^{\prime }$ and hence

$$E=E_C+E_X$$

(3)

where

$$E_C=\langle E\rangle ={\langle \psi \rangle }^2+\langle {\psi }^{\prime 2}\rangle$$

(4)

is the climatological (time mean) part and

$$E_X=E-E_C=\left(\psi {\prime }^2-\langle \psi {\prime }^2\rangle \right)+2\langle \psi \rangle {\psi }^{\prime }$$

(5)

reflects the departures thereof (energy fluctuations).

The climatological energy $E_C$ can further be split as follows:

$$E_C=E_M+E_E$$

(6)

where $E_M={\langle \psi \rangle }^2$ is the contribution associated with the time mean variables and $E_E=\langle {\psi }^{\prime 2}\rangle$ is the contribution associated with variance of time deviations (transient eddies); both contributions are relevant for climate studies.

We note that while $E_C$ is a positive definite quantity, $E_X$ varies in sign: it is positive/negative during episodes of more/less intense storminess, so that $\langle E_X\rangle =0$. Energy fluctuations can be further split as follows

$$E_X=E_{X1}+E_{X2}$$

(7)

where $E_{X1}={\psi }^{\prime 2}-\langle {\psi }^{\prime 2}\rangle$ and $E_{X2}=2\langle \psi \rangle {\psi }^{\prime }$ involve quadratic and linear contributions of transient eddies, respectively (see “Appendix A” for details).

We note that while time deviations of variables ${\psi }^{\prime }$ contribute to both $E_E$ and $E_X$, $E_E$ only contributes to the time mean energy because $\langle E_X\rangle =0$, as noted earlier, and only $E_X$ contributes to the time variation of $E$, since $d_t{E}_E=0$.

Hence to summarize

$$E=E_M+E_E+E_X$$

(8)

In an earlier study of the energetics of the life cycle of a storm, Ref. [17] considered the quantity $E_{TV}={\psi }^{\prime 2}$ to characterize the time variations of storm energetics. It appears in retrospect that $E_X$ is the more appropriate variable because it is the one contributing to the time variation of $E$ since $d_{t}E=d_t{E}_X$.

Using this approach, the kinetic energy $K$ and potential enthalpy $A$ can be decomposed as follows:

$$\begin{array}{lll}K& =& \frac{1}{2}\overrightarrow{V_h}\cdot \overrightarrow{V_h} \\ & =& K_M+K_E+K_X\end{array}$$

(9)

where

$$\begin{array}{l}{K}_M=\frac{1}{2}\langle \overrightarrow{V_h}\rangle \cdot \langle \overrightarrow{V_h}\rangle \\ K_E=\frac{1}{2}\left[\langle \overrightarrow{V_h}\cdot \overrightarrow{V_h}\rangle -\langle \overrightarrow{V_h}\rangle \cdot \langle \overrightarrow{V_h}\rangle \right]=\frac{1}{2}\langle \overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{V_h}^{\prime }}\rangle \\ K_X=\frac{1}{2}\left[\overrightarrow{V_h}\cdot \overrightarrow{V_h}-\langle \overrightarrow{V_h}\cdot \overrightarrow{V_h}\rangle \right]=\frac{1}{2}\left(\overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{V_h}^{\prime }}-\langle \overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{V_h}^{\prime }}\rangle \right)+\langle \overrightarrow{V_h}\rangle \cdot \overrightarrow{{V_h}^{\prime }}\end{array}$$

and

$$\begin{array}{lll}A& =& \frac{C_p}{2T_r}{\left(T-T_r\right)}^2\\ & =& A_M+A_E+A_X\end{array}$$

(10)

where

$$\begin{array}{l}{A}_M=\frac{C_p}{2T_r}{\langle T-T_r\rangle }^2\\ A_E=\frac{C_p}{2T_r}\left[\langle {\left(T-T_r\right)}^2\rangle -{\langle T-T_r\rangle }^2\right]=\frac{C_p}{2T_r}\langle T^{\prime 2}\rangle \\ A_X=\frac{C_p}{2T_r}\left[{\left(T-T_r\right)}^2-\langle {\left(T-T_r\right)}^2\rangle \right]=\frac{C_p}{2T_r}\left(T^{\prime 2}-\langle T^{\prime 2}\rangle \right)+\frac{C_p}{T_r}{T}^{\prime }\langle T-T_r\rangle \end{array}$$

In the following sections, we establish the relevant equations for the time mean climate ($E_C=E_M+E_E$) and for the time variations associated with weather systems ($E_X=E_{X1}+E_{X2}$).

3. Energy Budget Equations for Time Average or Climate Studies

Under hydrostatic equilibrium, the field equations can be expressed in a pressure coordinate as follows:

$$\frac{\partial \overrightarrow{V_h}}{\partial t}+\left(\overrightarrow{V}\cdot \overrightarrow{\nabla }\right)\cdot \overrightarrow{V_h}+f\overrightarrow{k}\times \overrightarrow{V_h}+\overrightarrow{{\nabla }_h}\varphi -\overrightarrow{F_h}=\overrightarrow{0}$$

(11)

$$\frac{\partial T}{\partial t}+\left(\overrightarrow{V}\cdot \overrightarrow{\nabla }\right)T-\frac{\omega {\alpha }_r}{C_p}-\frac{Q}{C_p} \simeq 0$$

(12)

$$\overrightarrow{\nabla }\cdot \overrightarrow{V}=0$$

(13)

$$\frac{\partial \varphi }{\partial p}+\alpha =0$$

(14)

$$\alpha -\frac{RT}{p}=0$$

(15)

Equation (11) is the horizontal momentum equation where $\overrightarrow{V_h}\left(u,v\right)$ is the horizontal wind vector, $\overrightarrow{V}\left(u,v,\omega \right)$ is the three dimensional wind vector with $\omega =dp/dt$ the vertical motion in pressure coordinate, $f$ is the Coriolis parameter, $\varphi$ is the geopotential and $\overrightarrow{F_h}$ is the external horizontal force. Equation (12) is an approximate form of the enthalpy equation, where $C_p$ is the specific heat at a constant pressure, $\alpha$ is the specific volume and $Q$ is the total diabatic heating rate. Equation (13) is the mass continuity equation, Equation (14) is the hydrostatic equilibrium equation and Equation (15) is the equation of state for an ideal gas. In these equations, $\overrightarrow{{\nabla }_h}=\left(\frac{\partial \hspace{0.17em}\hspace{0.17em}}{\partial x},\frac{\partial \hspace{0.17em}\hspace{0.17em}}{\partial y}\right)$ and $\overrightarrow{\nabla }=\left(\frac{\partial \hspace{0.17em}\hspace{0.17em}}{\partial x},\frac{\partial \hspace{0.17em}\hspace{0.17em}}{\partial y},\frac{\partial \hspace{0.17em}\hspace{0.17em}}{\partial p}\right)$ and $\frac{\partial \hspace{0.17em}\hspace{0.17em}}{\partial t}$ is the local tendency. In numerical weather prediction and climate models, these equations are completed by equations describing the evolution of water vapor and liquid and ice clouds and precipitations, the phase conversions of which contribute to the diabatic heating rate.

One should note the use of ${\alpha }_r$ instead of $\alpha$ in (12); this represents the approximate form of the thermodynamic equation that is consistent with the approximate quadratic form of the available enthalpy (NL13 [16]; Equation (65)). However, it differs in their equation due to the absence of the factor of order unity $\frac{T_r}{T}$ affecting the diabatic heating term; Equation (12) is a better approximation in the sense that no further approximation will have to be invoked in the ensuing algebraic manipulations (see “Supplementary Material S1” for details).

Equations of energy budget are derived from primitive atmospheric Equations (11)–(15). An example of how to obtain the kinetic energy tendency equation is shown in “Supplementary Material S2”. An equation for the climatological kinetic energy associated with the time mean wind, $K_M$, is obtained by taking the dot product of the time mean horizontal winds with the time mean horizontal momentum equation as follows:

$$\begin{array}{lll}\frac{\partial K_M}{\partial t}& =& \langle \overrightarrow{V_h}\rangle \cdot \frac{\partial \langle \overrightarrow{V_h}\rangle }{\partial t}\\ & =& -\left(F_{KM}+H_{KM}+C_K\right)+C_{MK}-D_M\end{array}$$

(16)

where

$$\begin{array}{l}{F}_{KM}=\overrightarrow{\nabla }\cdot \left(\langle \overrightarrow{V}\rangle K_M\right)\\ H_{KM}=\overrightarrow{\nabla }\cdot \langle \overrightarrow{V^{\prime }}\left(\overrightarrow{{V_h}^{\prime }}\cdot \langle \overrightarrow{V_h}\rangle \right)\rangle \\ C_K=-\langle \overrightarrow{{V_h}^{\prime }}\cdot \left(\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\cdot \langle \overrightarrow{V_h}\rangle \rangle \\ C_{MK}=-\langle \overrightarrow{V_h}\rangle \cdot \overrightarrow{{\nabla }_h}\langle \varphi \rangle \\ D_M=-\langle \overrightarrow{V_h}\rangle \cdot \langle \overrightarrow{F_h}\rangle \end{array}$$

(see “Supplementary Material S3” for details).

$F_{KM}$ and $H_{KM}$ are boundaries fluxes terms acting at the regional domain boundaries; $D_M$ is the physical sink term associated with the dissipation of kinetic energy, which is especially strong in the planetary boundary layer; $C_K$ is a conversion term that links $K_M$ and $K_E$; and $C_{MK}$ is the fraction of $A_M$ converted into $K_M$.

The easiest way to obtain the time mean kinetic energy associated with time variability of the wind is by subtracting the time mean kinetic energy equation and the kinetic energy equation associated with time mean wind as follows:

$$\begin{array}{lll}\frac{\partial K_E}{\partial t}& =& \langle \frac{\partial K}{\partial t}\rangle -\frac{\partial K_M}{\partial t}=\langle \overrightarrow{V_h}\cdot \frac{\partial \overrightarrow{V_h}}{\partial t}\rangle -\langle \overrightarrow{V_h}\rangle \cdot \frac{\partial \langle \overrightarrow{V_h}\rangle }{\partial t}\\ & =& -\left(F_{KE}+H_{KE}-C_K\right)+C_{EK}-D_E\end{array}$$

(17)

where

$$\begin{array}{l}{F}_{KE}=\overrightarrow{\nabla }\cdot \left(\langle \overrightarrow{V}\rangle K_E\right)\\ H_{KE}=\overrightarrow{\nabla }\cdot \langle \overrightarrow{V^{\prime }}\left(\frac{\overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{V_h}^{\prime }}}{2}\right)\rangle \\ C_K=-\langle \overrightarrow{{V_h}^{\prime }}\cdot \left(\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\cdot \langle \overrightarrow{V_h}\rangle \rangle \\ C_{EK}=\langle \overrightarrow{{V_H}^{\prime }}\cdot \overrightarrow{{\nabla }_h{\varphi }^{\prime }}\rangle \\ D_E=-\langle \overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{F_h}^{\prime }}\rangle \end{array}$$

(see “Supplementary Material S4” for details).

$F_{KE}$ and $H_{KE}$ are boundaries fluxes terms acting at the regional domain boundaries; $D_E$ is the physical sink term associated with the dissipation of kinetic energy that is especially strong in the planetary boundary layer; $C_K$ is a conversion term as it links $K_M$ and $K_E$, and appears in both tendency equations; and $C_{EK}$ is the fraction of $A_E$ converted into $K_E$.

An equation for the climatological mean available enthalpy associated with the time mean temperature, $A_M$, is obtained by multiplying with $\frac{C_p}{T_r}\langle T-T_r\rangle$ the time mean thermodynamics equation as follows:

$$\begin{array}{lll}\frac{\partial A_M}{\partial t}& =& \frac{C_p}{T_r}\langle T-T_r\rangle \langle \frac{\partial T}{\partial t}\rangle \\ & =& -\left(F_{AM}+H_{AM}+C_A\right)-\left(C_{MA}-I_{AB}\right)+G_M\end{array}$$

(18)

where

$$\begin{array}{l}{C}_A=-\frac{C_p}{T_r}\left(\langle T^{\prime } \overrightarrow{V^{\prime }}\rangle \cdot \overrightarrow{\nabla }\right)\langle T\rangle \\ F_{AM}=\overrightarrow{\nabla }\cdot \left(\langle \overrightarrow{V}\rangle A_M\right)\\ H_{AM}=\overrightarrow{\nabla }\cdot \langle \overrightarrow{V^{\prime }}\left(\frac{C_p}{T_r}\langle T-T_r\rangle T^{\prime }\right)\rangle \\ C_{MA}=-\langle \omega \rangle \langle \alpha \rangle \\ I_{AB}=-\langle \omega \rangle {\alpha }_r\\ G_M=\langle \left(\frac{T}{T_r}-1\right)\rangle \langle Q\rangle \end{array}$$

(see “Supplementary Material S5” for details).

$F_{AM}$ and $H_{AM}$ are boundary flux terms acting at the regional domain boundaries; $G_M$ is the physical source term associated with the generation of diabatic heating; $C_A$ is a conversion term that links $A_M$ and $A_E$, and appears in both tendency equations; $C_{MA}$ is the maximum time mean available enthalpy convertible into time mean kinetic energy; finally, $I_{AB}$ is a conversion term resulting from the splitting of the available enthalpy into temperature- and pressure-dependent components.

An equation for the time mean available enthalpy associated with time variability of the temperature, $A_E$, is obtained by subtracting the time mean available enthalpy equation by the time mean available enthalpy equation associated with time mean temperature as follows:

$$\begin{array}{lll}\frac{\partial A_E}{\partial t}& =& \langle \frac{\partial A}{\partial t}\rangle -\frac{\partial A_M}{\partial t}=\frac{C_p}{T_r}\left\{\langle \left(T-T_r\right)\frac{\partial T}{\partial t}\rangle -\langle T-T_r\rangle \langle \frac{\partial T}{\partial t}\rangle \right\}\\ & =& -\left(F_{AE}+H_{AE}-C_A\right)-C_{EA}+G_E\end{array}$$

(19)

where

$$\begin{array}{l}{C}_A=-\frac{C_p}{T_r}\left(\langle T^{\prime } \overrightarrow{V^{\prime }}\rangle \cdot \overrightarrow{\nabla }\right)\langle T\rangle \\ F_{AE}=\overrightarrow{\nabla }\cdot \left(\langle \overrightarrow{V}\rangle A_E\right)\\ H_{AE}=\overrightarrow{\nabla }\cdot \langle \overrightarrow{V^{\prime }} \left(\frac{C_p}{2T_r}{T}^{\prime 2}\right)\rangle \\ C_{EA}=-\langle {\omega }^{\prime }{\alpha }^{\prime }\rangle \\ G_E=\langle \frac{T^{\prime }{Q}^{\prime }}{T_r}\rangle \end{array}$$

(see “Supplementary Material S6” for details).

$F_{AE}$ and $H_{AE}$ are boundaries fluxes terms acting at the regional domain boundaries; $G_E$ is the physical term associated with covariances of diabatic heating and temperature; $C_A$ is a conversion term that links $A_M$ and $A_E$, and appears in both tendency equations; $C_{EA}$ is the maximum time variability available enthalpy convertible into eddy kinetic energy.

The equations for $K_M$ (16) and $A_M$ (18) can be linked by making use of the continuity Equation (13), hydrostatic Equation (14) and state law (15), to get

$$C_{MK}=C_{MA}-F_{\varphi M}$$

(20)

where

$$F_{\varphi M}=\overrightarrow{\nabla }\cdot \left(\langle \overrightarrow{V}\rangle \langle \varphi \rangle \right)$$

(see the last section of “Supplementary Material S3” for details).

And similarly for $K_E$ (17) and $A_E$ (19):

$$C_{EK}=C_{EA}-F_{\varphi E}$$

(21)

where

$$F_{\varphi E}=\overrightarrow{\nabla }\cdot \left(\overrightarrow{V^{\prime }} {\varphi }^{\prime }\right)$$

(see the last section of “Supplementary Material S4” for details).

We note in passing that $F_{\varphi M}$ and $F_{\varphi E}$ vanish when integrated over the entire atmosphere [1].

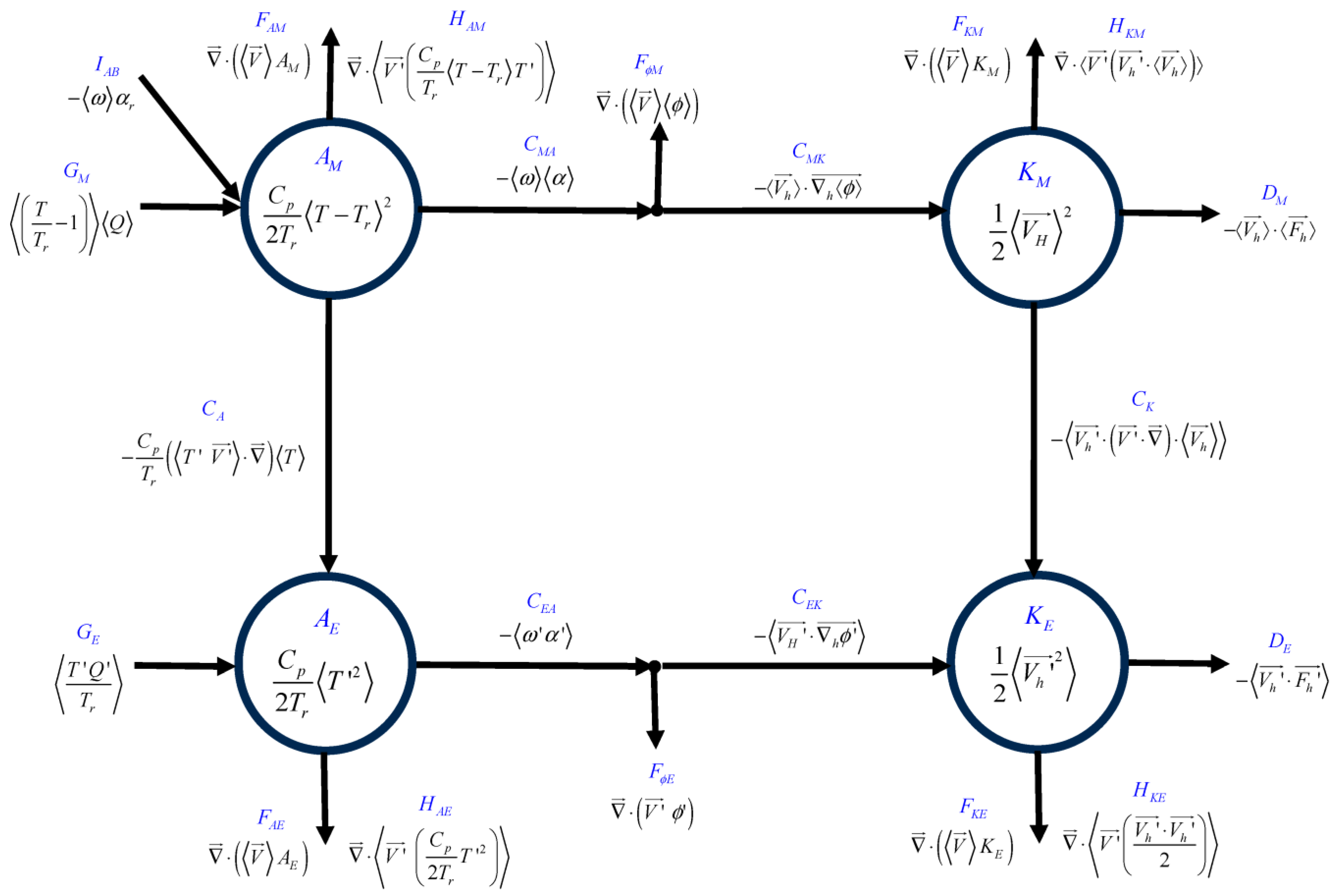
The set of atmospheric energetics equations suitable for climate studies is summarized in Figure 1. Boxes represents reservoirs and arrows indicate conversion terms and as sources/sinks of energy (or physical processes) acting on the corresponding reservoir. In addition to the four energy reservoirs, there are two zero-sum nodes corresponding to Equations (20) and (21). These nodes are important to understand that, contrary to the global atmospheric energetics, only a fraction of the available enthalpy is convertible into kinetic energy. Generally, $F_{\varphi M}$ constitutes a massive sink of energy due to the vertical stratification of the atmosphere, while $F_{\varphi E}$ can contribute positively or negatively depending on the domain boundaries. At this stage, the direction of the arrows is arbitrary and only reflects the choice of sign used in writing the Equations (16)–(19). According to these equations, positive (negative) terms are represented by inward (outward) pointing arrows. Terms that belong to two different equations are called conversion terms because they allow a direct energy exchange between reservoirs; this is the case of $C_A$ and $C_K$ connecting to $A_M$ to $A_E$ and $K_M$ to $K_E$, respectively. In the Lorenz global energy cycle, fluxes of geopotential vanish ($F_{\varphi M}=0$ and $F_{\varphi E}=0$) and terms $C_{MA}=C_{MK}$ and $C_{EA}=C_{EK}$ connect to $A_M$ to $K_M$ and $A_E$ to $K_E$, respectively, through baroclinic processes. However, in a limited-area domain, fluxes of geopotential ($F_{\varphi M}$ and $F_{\varphi E}$) act on the lateral boundaries, make inroads into conversion from available enthalpy to kinetic energy. Therefore, $C_{MK}\ne C_{MA}$ ($C_{EK}\ne C_{EA}$) represent the part of $A_M$ ($A_E$) converted into $K_M$($K_E$), the difference being lost or gained by lateral fluxes of geopotential $F_{\varphi M}\left(F_{\varphi E}\right)$. According to the dynamic and thermodynamic Equations (11) and (12), frictional terms dissipate kinetic energy and diabatic heating terms generate available enthalpy; therefore, $G_M$ and $G_E$ are physically responsible of the generation of available enthalpy, and $D_M$ and $D_E$ are responsible of the destruction of kinetic energy. Finally, $F$ and $H$ terms are boundary flux terms because of our open lateral boundaries; in the case of close atmospherics domain such as in Lorenz’ global energy cycle, they vanish.

We note a main difference with the formulation of NL13. Here, geopotential fluxes ($F_{\varphi M}$ and $F_{\varphi E}$) appear explicitly in the zero-sum nodes between kinetic energy and available enthalpy, while in NL13, these fluxes were combined with the kinetic energy tendency; as a result, $C_{MA}$ and $C_{EA}$ appeared to connect $A_M$ to $K_M$ and $A_E$ to $K_E$, respectively.

Finally, for simplicity purposes, it would be possible to combine in Figure 1 boundaries fluxes terms $F_{AM}$ and $H_{AM}$, $F_{AE}$ and $H_{AE}$, $F_{KM}$ and $H_{KM}$, $F_{KE}$ and $H_{KE}$, in a single term representing the total contribution of boundary flux divergence terms. As mentioned above, these terms will vanish when globally averaged.

4. Energy Budget Equations for Time Variability or Transient Eddies Studies

Unlike the earlier study by [17], who limited themselves to consider only one term contributing to the time evolution of storm energetics, here we develop the full transient eddy equations accounting for all conversions and sources and sinks of energy acting on storms. We reiterate that the advantage of this approach is that all terms appearing in the transient eddy energy budget vanish when averaged in time.

$$K_X=K_{X1}+K_{X2}$$

(22)

where

$$\begin{array}{l}{K}_{X1}=\frac{1}{2}\left(\overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{V_h}^{\prime }}-\langle \overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{V_h}^{\prime }}\rangle \right)\\ K_{X2}=\langle \overrightarrow{{V_h}^{\prime }}\rangle \cdot \overrightarrow{{V_h}^{\prime }}\end{array}$$

and

$$A_X=A_{X1}+A_{X2}$$

(23)

where

$$\begin{array}{l}{A}_{X1}=\frac{C_p}{2T_r}\left(T^{\prime 2}-\langle T^{\prime 2}\rangle \right)\\ A_{X2}=\frac{C_p}{T_r}{T}^{\prime }\langle T-T_r\rangle \end{array}$$

As shown in “Supplementary Material S7”, a prognostic equation for the instantaneous kinetic energy associated with quadratic contributions of wind fluctuations is obtained as follows:

$$\begin{array}{lll}\frac{\partial K_{X1}}{\partial t}& =& \overrightarrow{{V_h}^{\prime }}\cdot \frac{\partial \overrightarrow{{V_h}^{\prime }}}{\partial t}-\langle \overrightarrow{{V_h}^{\prime }}\cdot \frac{\partial \overrightarrow{{V_h}^{\prime }}}{\partial t}\rangle \\ & =& -\left(-c_{kx}+j_{kx}+f_{kx1}\right)+c_{x1k}-d_{x1}\end{array}$$

(24)

where

$$\begin{array}{l}{c}_{kx}=-\left\{\overrightarrow{{V_h}^{\prime }}\cdot \left(\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\cdot \langle \overrightarrow{V_h}\rangle -\langle \overrightarrow{{V_h}^{\prime }}\cdot \left(\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\cdot \langle \overrightarrow{V_h}\rangle \rangle \right\}\\ j_{kx}=-\overrightarrow{{V_h}^{\prime }}\cdot \langle \left(\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\cdot \overrightarrow{{V_h}^{\prime }}\rangle \\ f_{kx1}=\overrightarrow{\nabla }\cdot \left\{\overrightarrow{V} \left(\frac{1}{2}{V_h}^{\prime 2}\right)-\langle \overrightarrow{V} \left(\frac{1}{2}{V_h}^{\prime 2}\right)\rangle \right\}\\ c_{x1k}=-\left(\overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{\nabla }_h{\varphi }^{\prime }}-\langle \overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{\nabla }_h{\varphi }^{\prime }}\rangle \right)\\ d_{x1}=-\left\{\overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{F_h}^{\prime }}-\langle \overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{F_h}^{\prime }}\rangle \right\}\end{array}$$

$f_{kx1}$ is the boundary flux term acting at the regional domain boundaries; $d_{x1}$ is the physical term associated with the dissipation of $k_{x1}$, especially in the boundary layer; $c_{kx}$ is a conversion term as it links both reservoirs ($k_{X1}$ and $k_{X2}$) and appears in both tendency equations; $c_{kx}$ could convert $k_{X1}$ into $k_{X2}$, and vice versa; $c_{x1k}$ is the fraction of $k_{X1}$ converted into $k_{X2}$; $j_{kx}$ is also a conversion term but to a lesser extent due to it expression involving three time variability variables, and it should be weaker than $c_{x1k}$.

“Supplementary Material S8” shows that the prognostic equation of the instantaneous kinetic energy associated with linear contributions of wind fluctuations is obtained as follows:

$$\begin{array}{lll}\frac{\partial K_{X2}}{\partial t}& =& \overrightarrow{{V_h}^{\prime }}\cdot \frac{\partial \langle \overrightarrow{V_h}\rangle }{\partial t}+\langle \overrightarrow{V_h}\rangle \cdot \frac{\partial \overrightarrow{{V_h}^{\prime }}}{\partial t}\\ & =& -\left(f_{kx2}-j_{kx}+c_{kx}\right)+c_{x2k}-d_{x2}\end{array}$$

(25)

where

$$\begin{array}{l}{c}_{kx}=-\left\{\overrightarrow{{V_h}^{\prime }}\cdot \left(\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\cdot \langle \overrightarrow{V_h}\rangle -\langle \overrightarrow{{V_h}^{\prime }}\cdot \left(\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\cdot \langle \overrightarrow{V_h}\rangle \rangle \right\}\\ j_{kx}=-\overrightarrow{{V_h}^{\prime }}\cdot \langle \left(\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\cdot \overrightarrow{{V_h}^{\prime }}\rangle \\ f_{kx2}=\frac{1}{2}\overrightarrow{\nabla }\cdot \left\{\overrightarrow{V} \left(V_h{}^2-{V_h}^{\prime 2}\right)-\langle \overrightarrow{V} \left(V_h{}^2-{V_h}^{\prime 2}\right)\rangle \right\}\\ c_{x2k}=-\left(\overrightarrow{{V_h}^{\prime }}\cdot \overrightarrow{{\nabla }_h\langle {\varphi }^{\prime }\rangle }-\langle \overrightarrow{{V_h}^{\prime }}\rangle \cdot \overrightarrow{{\nabla }_h{\varphi }^{\prime }}\right)\\ d_{x2}=-\left\{\overrightarrow{{V_h}^{\prime }}\cdot \langle \overrightarrow{F_h}\rangle +\langle \overrightarrow{V_h}\rangle \cdot \overrightarrow{{F_H}^{\prime }}\right\}\end{array}$$

$f_{kx2}$ is the boundary flux term acting at the regional domain boundaries; $d_{x2}$ is the physical term associated with the dissipation of $k_{x2}$, especially in the boundary layer; $c_{kx}$ is a conversion term as it links both reservoirs ($k_{X1}$ and $k_{X2}$) and appears in both tendency equations; $c_{kx}$ is a conversion term that links $k_{X1}$ and $k_{X2}$, and $c_{x2k}$ is the fraction of $k_{X2}$ converted into $a_{X2}$; $j_{kx}$ is also a conversion term but to a lesser extent due to it expression involving three time variability variables, and it should be weaker than $c_{x2k}$.

“Supplementary Material S9” demonstrates that the prognostic equation for the instantaneous available enthalpy associated with the quadratic contribution of temperature fluctuations is obtained as follows:

$$\begin{array}{lll}\frac{\partial A_{X1}}{\partial t}& =& \frac{C_p}{T_r}\left(T^{\prime }\frac{\partial T^{\prime }}{\partial t}-\langle T^{\prime }\frac{\partial T^{\prime }}{\partial t}\rangle \right)\\ & =& -\left(f_{ax1}+j_{ax}-c_{ax}\right)-c_{x1a}+g_{x1}\end{array}$$

(26)

where

$$\begin{array}{l}{c}_{ax}=-\frac{C_p}{T_r}\left\{\left(T^{\prime }\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\langle T\rangle -\langle \left(T^{\prime }\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\langle T\rangle \rangle \right\}\\ f_{ax1}=\overrightarrow{\nabla }\cdot \left\{\overrightarrow{V} \left(\frac{C_p}{2T_r}{T}^{\prime 2}\right)-\langle \overrightarrow{V} \left(\frac{C_p}{2T_r}{T}^{\prime 2}\right)\rangle \right\}\\ j_{ax}=-\frac{C_p}{T_r}{T}^{\prime }\langle \left(\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)T^{\prime }\rangle \\ c_{x1a}=-\left\{{\omega }^{\prime }{\alpha }^{\prime }-\langle {\omega }^{\prime }{\alpha }^{\prime }\rangle \right\}\\ g_{x1}=\frac{1}{T_r}\left\{T^{\prime }{Q}^{\prime }-\langle T^{\prime }{Q}^{\prime }\rangle \right\}\end{array}$$

$f_{ax1}$ is the boundary flux term acting at the regional domain boundaries; $g_{x1}$ is the physical term associated with the anomaly of covariance of temperature and diabatic heating; $c_{ax}$ is a conversion term as it links both reservoirs $a_{X1}$ and $a_{X2}$, and appears in both tendency equations; $c_{ax}$ is a conversion term that links $a_{X1}$ and $a_{X2}$, and $c_{x1a}$ is the fraction of $a_{X1}$ converted into $k_{X1}$; $j_{ax}$ is also a conversion term but to a lesser extent due to it expression involving three time variability variables, and it should be weaker than $c_{x1a}$.

The prognostic equation for the instantaneous available enthalpy associated with the linear contribution of temperature fluctuations is obtained as follows (“Supplementary Material S10”):

$$\begin{array}{lll}\frac{\partial A_{X2}}{\partial t}& =& \frac{C_p}{T_r}\left(T^{\prime }\frac{\partial \langle T\rangle }{\partial t}+\langle T-T_r\rangle \frac{\partial T^{\prime }}{\partial t}\right)\\ & =& -\left(f_{ax2}-j_{ax}+c_{ax}\right)-\left(c_{x2a}-i_{ab}\right)+g_{x2}\end{array}$$

(27)

where

$$\begin{array}{l}{c}_{ax}=-\frac{C_p}{T_r}\left\{\left(T^{\prime }\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\langle T\rangle -\langle \left(T^{\prime }\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)\langle T\rangle \rangle \right\}\\ f_{ax2}=\frac{C_p}{2T_r}\overrightarrow{\nabla }\cdot \left\{\overrightarrow{V}\left(T^2-T^{\prime 2}\right)-\langle \overrightarrow{V}\left(T^2-T^{\prime 2}\right)\rangle \right\}\\ j_{ax}=-\frac{C_p}{T_r}{T}^{\prime }\langle \left(\overrightarrow{V^{\prime }}\cdot \overrightarrow{\nabla }\right)T^{\prime }\rangle \\ c_{x2a}=-\left\{\langle \omega \rangle {\alpha }^{\prime }+{\omega }^{\prime }\langle \alpha \rangle \right\}\\ i_{ab}=-{\omega }^{\prime }{\alpha }_r\\ g_{x2}=\frac{1}{T_r}\left\{T^{\prime }\langle Q\rangle +\langle T-T_r\rangle Q^{\prime }\right\}\end{array}$$

$f_{ax2}$ is the boundary flux term acting at the regional domain boundaries; $g_{x2 }$ is the physical term associated with the anomaly of covariance of temperature and diabatic heating; $c_{ax}$ is a conversion term as it links both reservoirs $a_{X1}$ and $a_{X2}$, and appears in both tendency equations; $c_{ax}$ is a conversion term that links $a_{X1}$ and $a_{X2}$, and $c_{x2a}$ is the fraction of $a_{X2}$ converted into $a_{X2}$; $j_{ax}$ is also a conversion term but to a lesser extent due to it expression involving three time variability variables, and it should be weaker than $c_{x1a}$.

The equations for $k_{X1}$ (24) and $k_{X1}$ (26) can be linked by making use of the continuity Equation (13), hydrostatic Equation (14) and state law (15), to get

$$f_{\varphi x1}-c_{x1a}=c_{x1k}$$

(28)

where

$$f_{\varphi x1}=\overrightarrow{\nabla }\cdot \left\{\overrightarrow{V^{\prime }} {\varphi }^{\prime }-\langle \overrightarrow{V^{\prime }} {\varphi }^{\prime }\rangle \right\}$$

(see the last section of “Supplementary Material S7” for details).

And similarly for $k_{X2}$ (25) and $k_{X2}$ (27)

$$f_{\varphi x2}-c_{x2a}=c_{x2k}$$

(29)

where

$$f_{\varphi x1}=\overrightarrow{\nabla }\cdot \left\{\overrightarrow{V^{\prime }} \langle {\varphi }^{\prime }\rangle -\langle \overrightarrow{V^{\prime }}\rangle {\varphi }^{\prime }\right\}$$

(see the last section of “Supplementary Material S8” for details).

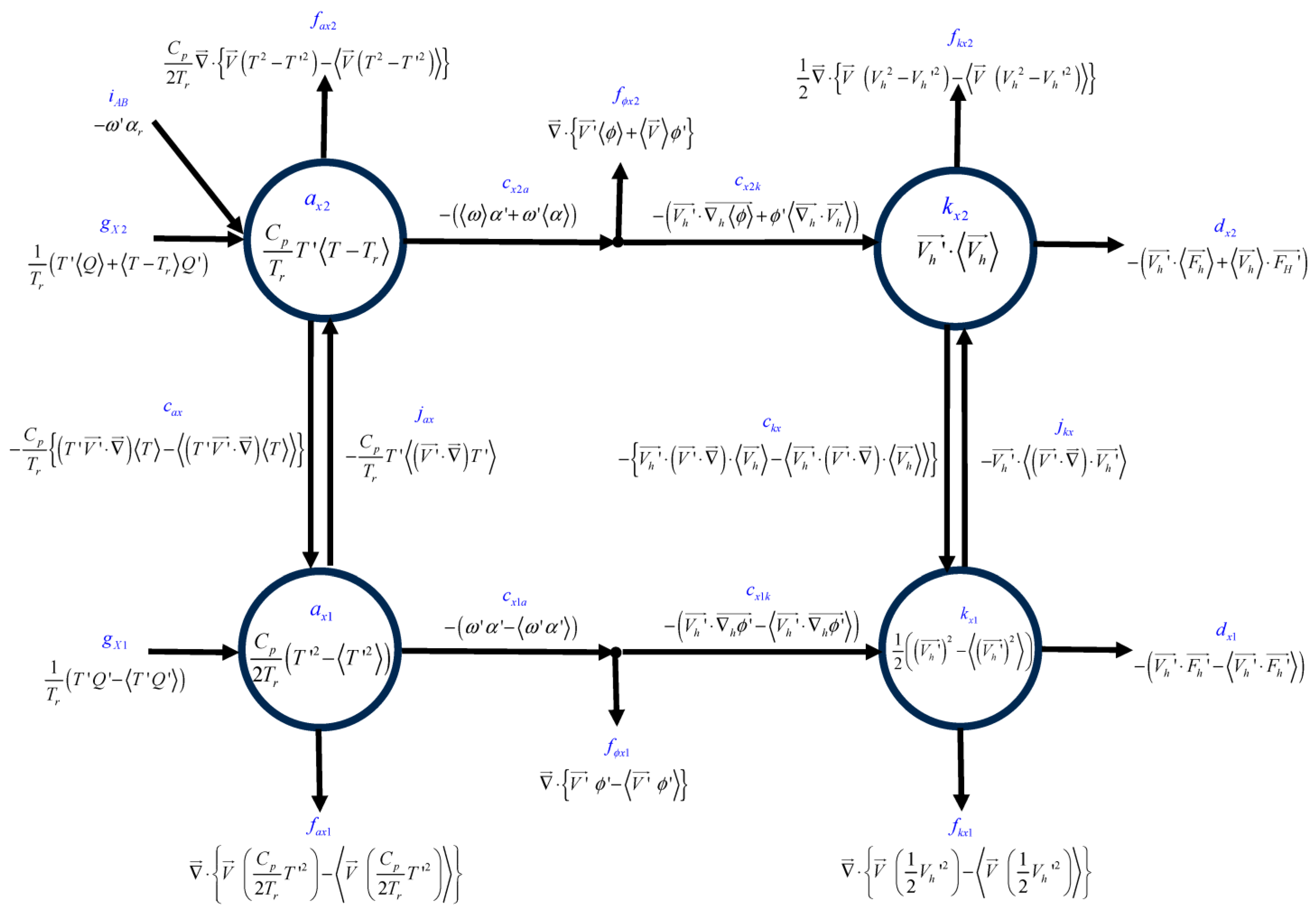
The set of atmospheric energetics equations suitable for storms studies is summarized in Figure 2. This diagram is a combination of Equations (24)–(27). Unlike the climate energy cycle (Figure 1), we note that in this transient eddy energy cycle, there are the third-order conversion terms $j_{ax}$ and $j_{kx}$, (made of products of three time variability variables), which are usually negligible in practice.

Finally, by summing time mean and time variability equations terms, the former total kinetic energy and available enthalpy equations are recovered (see Equations (3) and (8)), confirming that no piece of information has been lost. The algebraic proof is made in “Supplementary Material S11 and S12”.

5. Illustration of Storm Energetics during an African Easterly Wave

To illustrate the perspectives afforded with the storm energy cycle developed in Section 4 of this paper, we briefly present an example of the contributions to the time evolution of the transient eddy kinetic energy during the genesis of an African Easterly Wave (AEW) over South Sudan.

The contribution to the transient eddy kinetic energy equation (Equation (24)) was computed using data simulated by a developmental version of the sixth-generation Canadian Regional Climate Model (CRCM6/GEM5), derived from the version 5 of the ECCC Global Environmental Multiscale model. The simulation was driven by ERA5 reanalyses from May to October 2006, using a horizontal grid mesh of 0.11° and 71 terrain-following levels in the vertical, with a top level at 10 hPa. The computational domain comprised the whole of West Africa, from 37° W to 31° E in longitude and from 12° S to 25° N in latitude.

For diagnostic purposes, the simulated data were interpolated in the vertical to 37 pressure levels, using finer resolution near the surface. Contributions to the transient-eddy kinetic energy $k_{x1}$ budget (Equation (24) were vertically integrated in the layer 950–200 hPa and averaged over the region of South Sudan, using hourly archives of simulated data between the 5th and 9th September 2006. The AEW that developed over South Sudan was documented to later move across the continent and develop into a tropical depression when it reached the west coast, contributing to the genesis of Hurricane Helene [23,24] in the Atlantic Ocean. A 3–5 days bandpass filter was applied to the transient eddy kinetic energy contributions in order to focus on the AEW.

Figure 3a shows that the transient-eddy kinetic energy $k_{x1}$ reaches a maximum on 7 September and decays afterwards. Figure 3b shows that the various contributions to the time evolution of $k_{x1}$. The $c_{x1k}$ conversion term represents the covariance of ageostrophic wind perturbations acting on the geopotential gradient perturbations; it is the dominant contribution to $k_{x1}$ growth from the 5th to the 7th. In the absence of boundary-flux term $f_{\varphi x1}$ (Equation (28)) (for ex. over a global domain), $c_{x1k}$ would be equal to the baroclinic conversion $c_{x1a}$, which reflects covariance of vertical motion perturbations and density perturbations. Figure 3b shows that the barotropic conversion term $c_{kx}$ lags in time the $c_{x1k}$ term, becoming larger near the peak of transient eddy kinetic energy, but its importance remains secondary compared to the $c_{x1k}$ term. Finally, dissipation $d_{x1}$ and boundary-flux $f_{kx1}$ terms contribute to reducing the growth by baroclinic conversion, while the third-order term $j_{kx}$ remains negligible.

Figure 3b also shows the sum of the five contributions to the tendency of $k_{x1}$. One can see that the sum of the diagnostic contributions differs somewhat from the tendency of the simulated $k_{x1}$; this discrepancy is due to the several approximations used in computing the various contributions, such as vertical interpolations, finite differences, time sampling, etc. This result illustrates the challenges of obtaining quantitative estimates of the various contributions to the atmospheric energetics.

The modest results presented above hint to the importance of the baroclinic processes in the development of AEW. In a forthcoming paper, the detailed study of AEW energetics will be made using the storm energy cycle developed in this paper. In another paper in preparation, the regional climate atmospheric energetics formulation developed in Section 3 will be applied to study the WAM system, showing the physical mechanisms responsible for contrasting years of precipitation leading to drought and floods in the Sahel region.

6. Summary and Conclusions

In this study, we developed an atmospheric energetics framework suitable for both weather and climate studies over limited-area domains. This is accomplished by adapting the equations originally developed by NL3 for the study of inter-member variability to climate energetics, and by completely revising the framework proposed by [17] for the study of weather systems energetics. The main advantage of this approach is to allow for the retrieval of the total kinetic energy and enthalpy equations by summing climate and weather tendency equations. Our challenge was to generalize the equations originally developed by NL3 for the study of inter-member variability (and adaptable for climate energetics studies), to transient eddies. Moreover, the introduction of nodes between available enthalpy and kinetic energetics reservoirs facilitates the physical interpretation of the energy conversions between reservoirs. We succeeded in developing a unified approach applicable to both climate and weather energy cycles.

Energy reservoirs show the main reservoir of energy in the atmosphere (boxes) and physical processes such as conversions, diabatic contributions and boundary fluxes (arrows) acting to increase or decrease energy reservoirs. The energy content of reservoirs is obtained by horizontally and vertically integration over the domain of interest. According to their mathematical expression, climate energy reservoirs (boxes) are always positive, while weather energy reservoirs vary between positive and negative phases. Physical processes (arrows) could either be positive or negative. Positive (negative) inward (outward) arrows will suggest that the process is a source term, such as the time mean generation of diabatic heating $G_M$, while positive (negative) outward (inward) arrows indicate a sink of energy to the reservoir, such as frictional dissipative terms acting especially at the boundary layer. At this formal stage, it is not possible to know the sign of each term; therefore, the direction of arrows is intuitive and only reflects the choice of sign used in writing the equations. Only actual case studies could inform us of contributions from different processes on local energy reservoirs.

Before analyzing energy cycles, we must keep in mind that we made some approximations, such as using hydrostatic equations, which are relevant at large scales instead of fully elastic Euler equations. We also used an approximate form of the thermodynamic equation in order to obtain a quadratic form. Finally, the computation of the energy budget, in practice, involves several numerical (discretization, sampling) approximations that introduce errors in the computation of time tendencies, gradients, vertical interpolations and so on.