Are Near-Coastal Sea Levels Accelerating Faster Than Global during the Satellite Altimetry Era?
Abstract
:1. Introduction
2. Materials and Methods
2.1. Data
2.2. Methods
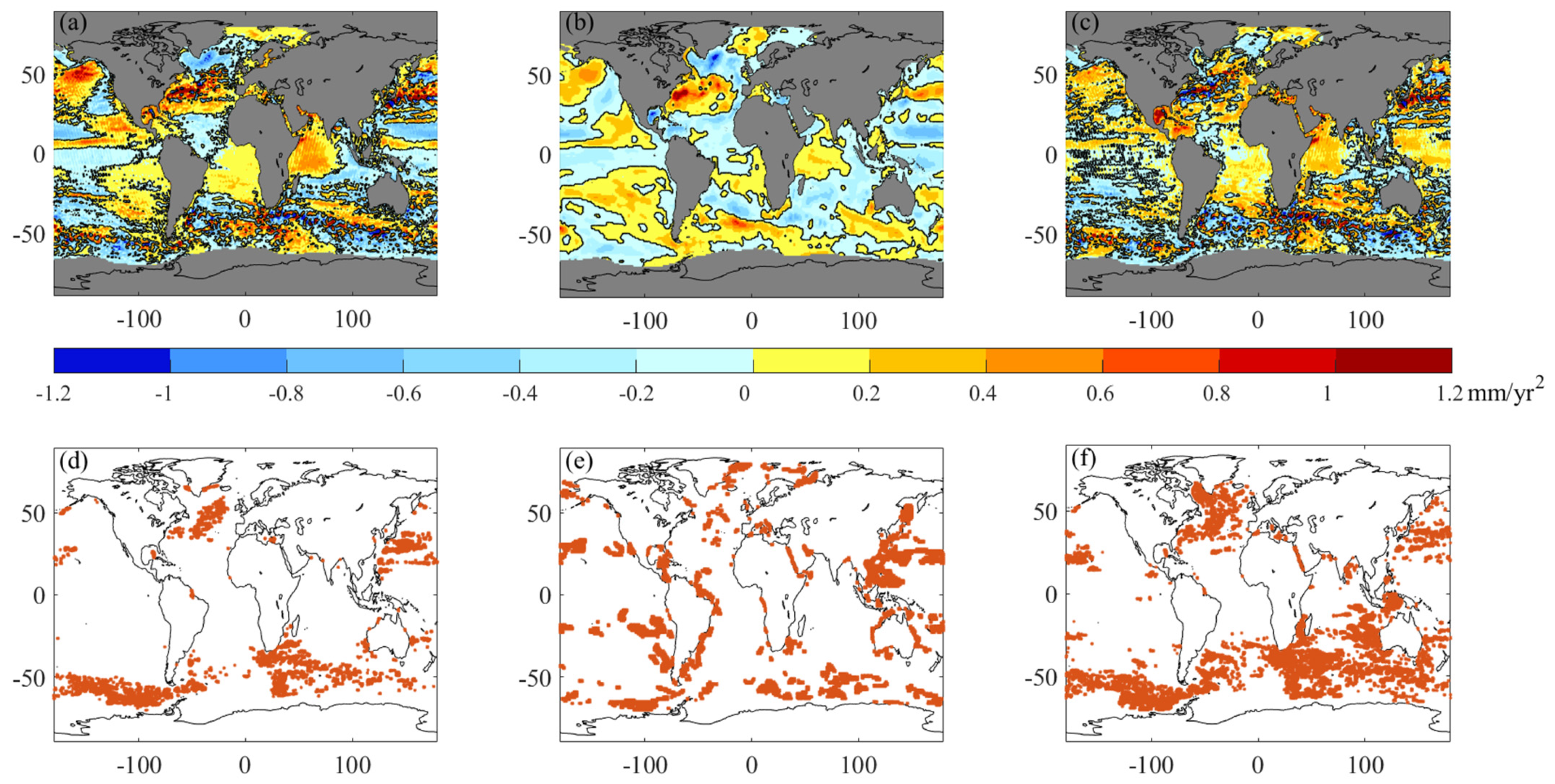
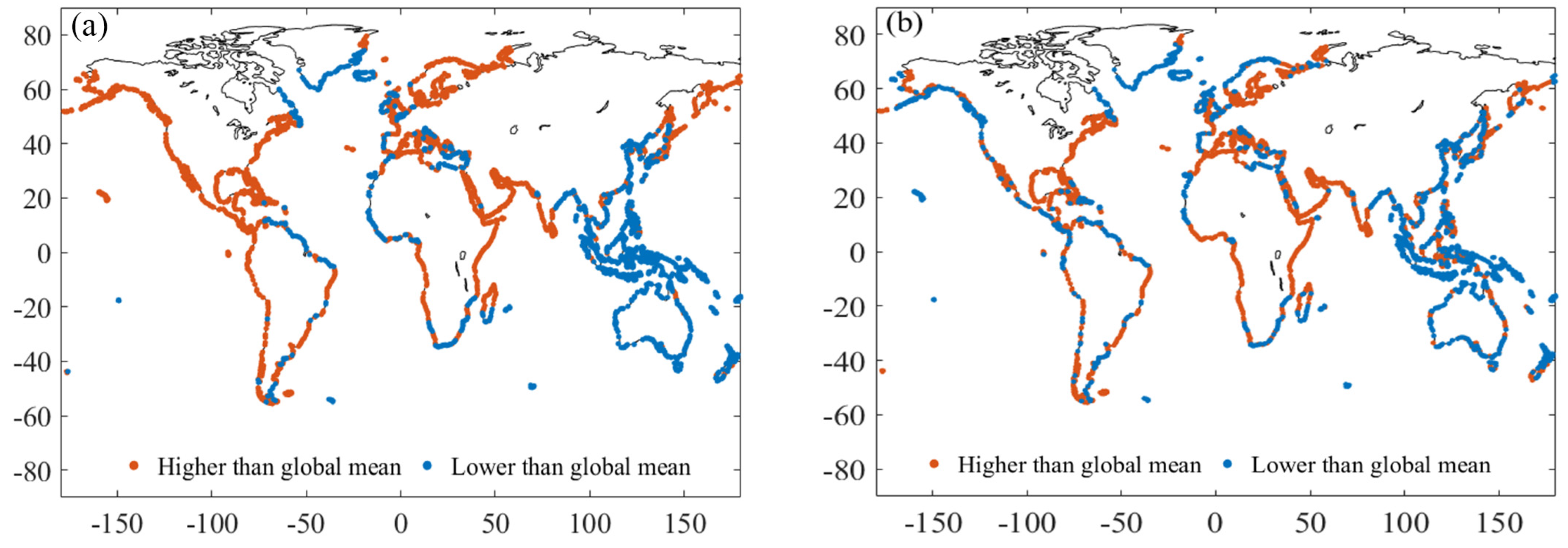
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Allan, R.P.; Hawkins, E.; Bellouin, N.; Collins, B. IPCC, 2021: Summary for Policymakers. In Climate Change 2021: The Physical Science Basis; Masson-Delmotte, V., Zhai, P., Pirani, A., Connors, S.L., Péan, C., Berger, S., Caud, N., Chen, Y., Goldfarb, L., Gomis, M.I., et al., Eds.; Contribution of Working Group I to the Sixth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2021; pp. 3–32. [Google Scholar] [CrossRef]
- Pörtner, H.O.; Roberts, D.C.; Poloczanska, E.S.; Mintenbeck, K.; Tignor, M.; Alegría, A.; Craig, M.; Langsdorf, S.; Löschke, S.; Möller, V.; et al. (Eds.) IPCC, 2022: Summary for Policymakers. In Climate Change 2022: Impacts, Adaptation and Vulnerability; Contribution of Working Group II to the Sixth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2022; pp. 3–33. [Google Scholar] [CrossRef]
- Jevrejeva, S.; Jackson, L.P.; Grinsted, A.; Lincke, D.; Marzeion, B. Flood damage costs under the sea level rise with warming of 1.5 °C and 2 °C. Environ. Res. Lett. 2018, 13, 074014. [Google Scholar] [CrossRef]
- Brown, S.; Jenkins, K.; Goodwin, P.; Lincke, D.; Vafeidis, A.T.; Tol, R.S.; Warren, R.; Nicholls, R.J.; Jevrejeva, S.; Sanchez Arcilla, A.; et al. Global costs of protecting against sea-level rise at 1.5 to 4.0 °C. Clim. Change 2021, 167, 4. [Google Scholar] [CrossRef]
- Abadie, L.M.; Jackson, L.P.; Murieta, E.S.; Jevrejeva, S.; Galarraga, I. Comparing urban coastal flood risk in 136 cities under two alternative sea-level projections: RCP 8.5 and an expert opinion-based high-end scenario. Ocean Coast. Manag. 2020, 193, 105249. [Google Scholar] [CrossRef]
- Nerem, R.S.; Beckley, B.D.; Fasullo, J.T.; Hamlington, B.D.; Masters, D.; Mitchum, G.T. Climate-changedriven accelerated sea-level rise detected in the altimeter era. Proc. Natl. Acad. Sci. USA 2018, 115, 2022–2025. [Google Scholar] [CrossRef] [PubMed]
- Moreira, L.; Cazenave, A.; Palanisamy, P. Influence of interannual variability in estimating the rate and acceleration of present-day global mean sea level. Glob. Planet. Change 2021, 199, 103450. [Google Scholar] [CrossRef]
- Hamlington, B.D.; Frederikse, T.; Nerem, R.S.; Fasullo, J.T.; Adhikari, S. Investigating the acceleration of regional sea level rise during the satellite altimeter era. Geophys. Res. Lett. 2020, 47, e2019GL086528. [Google Scholar] [CrossRef]
- Prandi, P.; Meyssignac, B.; Ablain, M.; Spada, G.; Ribes, A.; Benveniste, J. Local sea level trends, accelerations and uncertainties over 1993–2019. Sci. Data 2021, 8, 1. [Google Scholar] [CrossRef]
- Jevrejeva, S.; Moore, J.C.; Grinsted, A.; Matthews, A.P.; Spada, G. Trends and acceleration in global and regional sea levels since 1807. Glob. Planet. Change 2014, 113, 11–12. [Google Scholar] [CrossRef]
- Hay, C.C.; Morrow, E.; Kopp, R.E.; Mitrovica, J.X. Probabilistic reanalysis of twentieth-century sea-level rise. Nature 2015, 517, 481–484. [Google Scholar] [CrossRef]
- Frederikse, T.; Jevrejeva, S.; Riva, R.E.M.; Dangendorf, S. A Consistent Sea-Level Reconstruction and Its Budget on Basin and Global Scales over 1958–2014. J. Clim. 2018, 31, 1267–1280. [Google Scholar] [CrossRef]
- Johnson, G.C.; Lumpkin, R.L. (Eds.) State of the Climate in 2020. Global Oceans; American Meteorological Society: Boston, MA, USA, 2021. [Google Scholar] [CrossRef]
- Fox-Kemper, B.; Hewitt, H.T.; Xiao, C. Ocean, Cryosphere and Sea Level Change. In Climate Change 2021: The Physical Science Basis; Masson-Delmotte, V., Zhai, P., Pirani, A., Connors, S.L., Péan, C., Berger, S., Caud, N., Chen, Y., Goldfarb, L., Gomis, M.I., et al., Eds.; Contribution of Working Group I to the Sixth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2021; pp. 1211–1362. [Google Scholar] [CrossRef]
- Wouters, B.; van de Wal RS, W. Global sea level budget 1993-present. Earth Syst. Sci. Data 2018, 10, 1551–1590. [Google Scholar]
- Douglas, B.C. Global sea level acceleration. J. Geophys. Res. 1992, 97, 12699–12706. [Google Scholar] [CrossRef]
- Woodworth, P.L.; White, N.; Jevrejeva, S.; Holgate, S.; Church, J.A.; Gehrels, W.R. Evidence for the accelerations of sea level on multi-decade and century timescales. Int. J. Climatol. 2009, 29, 777–789. [Google Scholar] [CrossRef]
- Royston, S.; Watson, C.S.; Legrésy, B.; King, M.A.; Church, J.A.; Bos, M.S. Sea-level trend uncertainty with pacific climatic variability and temporally-correlated noise. J. Geophys. Res. Ocean. 2018, 123, 1978–1993. [Google Scholar] [CrossRef]
- Han, W.; Meehl, G.A.; Stammer, D.; Hu, A.; Hamlington, B.; Kenigson, J.; Palanisamy, H.; Thompson, P. Spatial patterns of sea level variability associated with natural internal climate modes. Surv. Geophys. 2017, 38, 217–250. [Google Scholar] [CrossRef]
- Han, W.; Stammer, D.; Thompson, P.; Ezer, T.; Palanisamy, H.; Zhang, X.; Domingues, C.; Zhang, L.; Yuan, D. Impacts of basin-scale climate modes on coastal sea level: A review. Surv. Geophys. 2019, 40, 1493–1541. [Google Scholar] [CrossRef]
- Wang, J.; Church, J.A.; Zhang, X.; Chen, X. Reconciling global mean and regional sea level change in projections and observations. Nat. Commun. 2021, 12, 990. [Google Scholar] [CrossRef]
- Fournier, S.; Willis, J.; Killett, E.; Qu, Z.; Zlotnicki, V. JPL MEaSUREs Gridded Sea Surface Height Anomalies Version 2205. 2022. Available online: https://deotb6e7tfubr.cloudfront.net/s3-edaf5da92e0ce48fb61175c28b67e95d/podaac-ops-cumulus-docs.s3.us-west-2.amazonaws.com/merged_alt/open/L4/docs/Documentation_SSH_Measures_V2205_Final.pdf?A-userid=None&Expires=1695387427&Signature=TiAoUOLUmRGqY9LeZwB5qk24b7140~gRe1S-LaQFLJtpoY-mOakPQzMmjnO0~GjaSdyd-micQyYpCJbM~8vbK6wR~ryeg1MnK-ldteUm2UXIAbWL3zDDbs-H8NauZSKTpsZkd~mB1mSNya1FmaWpFslrvKPESa~j4emQNB8WmDQiSLs38lk3GJH7OAgDdYu8g3ds2ATk28wBQ~tONwSe1P7QbHfNu0eEjFjGAHzCtAUNdkRPfwabq0LtnXtsFP~YvyrI1o-AyOH01I0QnWd2jPN0Idw5reHwWjB-vNjHWs~pzlCeyVZXaz9SZTOBvOb6ebUhJs9lm0jt1CDDrr2Mvw__&Key-Pair-Id=K2FIJB4PE30EQE (accessed on 21 September 2022).
- Cazenave, A.; Gouzenes, Y.; Birol, F.; Leger, F.; Passaro, M.; Calafat, F.M.; Shaw, A.; Nino, F.; Legeais, J.F.; Oelsmann, J.; et al. Sea level along the world’s coastlines can be measured by a network of virtual altimetry stations. Commun. Earth Environ. 2022, 3, 117. [Google Scholar] [CrossRef]
- Cheng, L.; Trenberth, K.E.; Fasullo, J.; Boyer, T.; Abraham, J.; Zhu, J. Improved estimates of ocean heat content from 1960 to 2015. Sci. Adv. 2017, 3, e1601545. [Google Scholar] [CrossRef]
- Zhang, L.; Delworth, T.L. Analysis of the characteristics and mechanisms of the Pacific decadal oscillation in a suite of coupled models from the Geophysical Fluid Dynamics Laboratory. J. Clim. 2015, 28, 7678–7701. [Google Scholar]
- Veng, T.; Andersen, O.B. Consolidating sea level acceleration estimates from satellite altimetry. Adv. Space Res. 2021, 68, 496–503. [Google Scholar] [CrossRef]
- Cipollini, P.; Benveniste, J.; Birol, F.; Fernandes, M.J.; Obligis, E.; Passaro, M.; Strub, P.T.; Valladeau, G.; Vignudelli, S.; Wilkin, J. Satellite altimetry in coastal regions. In Satellite Altimetry Over the Oceans and Land Surfaces; Stammer, D., Cazenave, A., Eds.; CRC Press: Boca Raton, FL, USA; London, UK; New York, NY, USA, 2018; pp. 343–373. [Google Scholar] [CrossRef]
- Durand, F.; Piecuch, C.G.; Becker, M.; Papa, F.; Raju, S.V.; Khan, J.U.; Ponte, R.M. Impact of continental freshwater runoff on coastal sea level. Surv. Geophys. 2019, 40, 1437–1466. [Google Scholar]
- Woodworth, P.L.; Melet, A.; Marcos, M.; Ray, R.D.; Wöppelmann, G.; Sasaki, Y.N.; Cirano, M.; Hibbert, A.; Huthnance, J.M.; Monserrat, S.; et al. Forcing Factors Causing Sea Level Changes at the Coast. Surv. Geophys. 2019, 40, 1351–1397. [Google Scholar] [CrossRef]
- The Climate Change Initiative Coastal Sea Level Team. Coastal sea level anomalies and associated trends from Jason satellite altimetry over 2002–2018. Sci. Data 2020, 7, 357. [Google Scholar] [CrossRef]
- Ostanciaux, É.; Husson, L.; Choblet, G.; Robin, C.; Pedoja, K. Present-day trends of vertical ground motion along the coast lines. Earth Sci. Rev. 2012, 110, 74–92. [Google Scholar] [CrossRef]
- Wöppelmann, G.; Marcos, M. Vertical land motion as a key to understanding sea level change and variability. Rev. Geophys. 2016, 54, 64–92. [Google Scholar] [CrossRef]
- Kleinherenbrink, M.; Riva, R.; Frederikse, T. A comparison of methods to estimate vertical land motion trends from GNSS and altimetry at tide gauge stations. Ocean Sci. 2018, 14, 187–204. [Google Scholar] [CrossRef]
- Volkov, D.L.; Lee, S.L.; Domingues, R.; Zhang, H.; Goes, M. Interannual Sea Level Variability Along the Southeastern Seaboard of the United States in Relation to the Gyre-Scale Heat Divergence in the North Atlantic. Geophys. Res. Lett. 2019, 46, 7481–7490. [Google Scholar] [CrossRef]
- Ghosh, S.; Mistri, B. Assessing coastal vulnerability to environmental hazards of Indian Sundarban delta using multi-criteria decision-making approaches. Ocean Coast. Manag. 2021, 209, 105641. [Google Scholar] [CrossRef]
- Hamlington, B.D.; Chambers, D.P.; Frederikse, T.; Dangendorf, S.; Fournier, S.; Buzzanga, B.; Nerem, R.S. Observation-based trajectory of future sea level for the coastal United States tracks near high-end model projections. Commun. Earth Environ. 2022, 3, 230. [Google Scholar] [CrossRef]
- Cayan, D.R.; Kammerdiener, S.A.; Dettinger, M.D.; Caprio, J.M.; Peterson, D.H. Changes in the onset of spring in the western United States. Bull. Amer. Meteor. Soc. 2001, 82, 399–415. [Google Scholar] [CrossRef]
- Deser, C.; Phillips, A.S.; Hurrell, J.W. Pacific interdecadal cli- mate variability: Linkages between the tropics and the North Pacific during boreal winter since 1900. J. Clim. 2004, 17, 3109–3124. [Google Scholar] [CrossRef]
- Cazenave, A.; Moreira, L. Contemporary sea-level changes from global to local scales: A review. Proc. R. Soc. A 2022, 478, 20220049. [Google Scholar] [CrossRef] [PubMed]
- Mohapatra, S.; Gnanaseelan, C.; Deepa, J.S. Multidecadal to decadal variability in the equatorial Indian Ocean subsurface temperature and the forcing mechanisms. Clim. Dyn. 2020, 54, 3475–3487. [Google Scholar] [CrossRef]
- Delworth, T.L.; Zeng, F. The impact of the North Atlantic Oscillation on climate through its influence on the Atlantic meridional overturning circulation. J. Clim. 2016, 29, 941–962. [Google Scholar] [CrossRef]
- Kim, W.M.; Yeager, S.; Danabasoglu, G. Atlantic multidecadal variability and associated climate impacts initiated by ocean thermohaline dynamics. J. Clim. 2020, 33, 1317–1334. [Google Scholar] [CrossRef]
- Borchert, L.F.; Müller, W.A.; Baehr, J. Atlantic ocean heat transport influences interannual-to-decadal surface temperature predictability in the North Atlantic region. J. Clim. 2018, 31, 6763–6782. [Google Scholar] [CrossRef]
- Menary, M.B.; Robson, J.; Allan, R.P.; Booth BB, B.; Cassou, C.; Gastineau, G.; Gregory, J.; Hodson, D.; Jones, C.; Mignot, J.; et al. Aerosol-forced AMOC changes in CMIP6 historical simulations. Geophys. Res. Lett. 2020, 47, e2020GL088166. [Google Scholar] [CrossRef]
- Zhang, R. Coherent surface-subsurface fingerprint of the Atlantic meridional overturning circulation. Geophys. Res. Lett. 2008, 35, L20705. [Google Scholar] [CrossRef]
- Caesar, L.; Rahmstorf, S.; Robinson, A.; Feulner, G.; Saba, V. Observed fngerprint of a weakening Atlantic Ocean overturning circulation. Nature 2018, 556, 191–196. [Google Scholar] [CrossRef]
- Sallenger, A.H.; Doran, K.S.; Howd, P.A. Hotspot of accelerated sea-level rise on the Atlantic coast of North America. Nat. Clim. Change 2012, 2, 884–888. [Google Scholar] [CrossRef]
- McCarthy, G.D.; Brown, P.J.; Flagg, C.N.; Goni, G.; Houpert, L.; Hughes, C.W.; Hummels, M.; Inall, M.; Jochumsen, K.; Larsen, K.M.H.; et al. Sustainable observations of the AMOC: Methodology and technology. Rev. Geophys. 2020, 58, e2019RG000654. [Google Scholar] [CrossRef]
- Kleinherenbrink, M.; Riva, R.; Scharroo, R. A revised acceleration rate from the altimetry-derived global mean sea level record. Sci. Rep. 2019, 9, 10908. [Google Scholar] [PubMed]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qu, Y.; Jevrejeva, S.; Palanisamy, H. Are Near-Coastal Sea Levels Accelerating Faster Than Global during the Satellite Altimetry Era? Atmosphere 2023, 14, 1573. https://doi.org/10.3390/atmos14101573
Qu Y, Jevrejeva S, Palanisamy H. Are Near-Coastal Sea Levels Accelerating Faster Than Global during the Satellite Altimetry Era? Atmosphere. 2023; 14(10):1573. https://doi.org/10.3390/atmos14101573
Chicago/Turabian StyleQu, Ying, Svetlana Jevrejeva, and Hindumathi Palanisamy. 2023. "Are Near-Coastal Sea Levels Accelerating Faster Than Global during the Satellite Altimetry Era?" Atmosphere 14, no. 10: 1573. https://doi.org/10.3390/atmos14101573
APA StyleQu, Y., Jevrejeva, S., & Palanisamy, H. (2023). Are Near-Coastal Sea Levels Accelerating Faster Than Global during the Satellite Altimetry Era? Atmosphere, 14(10), 1573. https://doi.org/10.3390/atmos14101573






