Numerical Modeling of the Radio Wave Over-the-Horizon Propagation in the Troposphere
Abstract
:1. Introduction
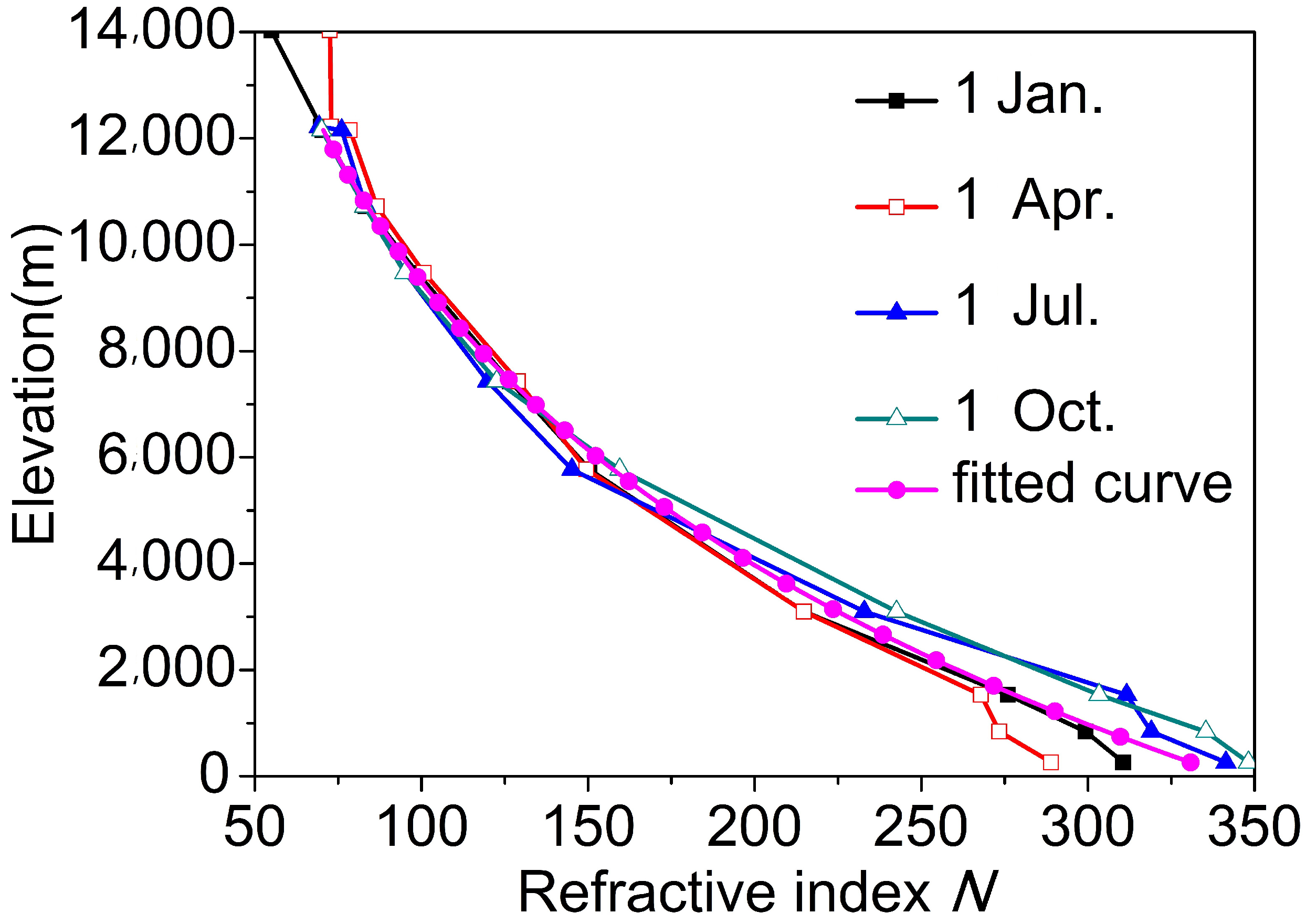
2. Refractive Index from Atmospheric Sounding Data
2.1. Refractive Index
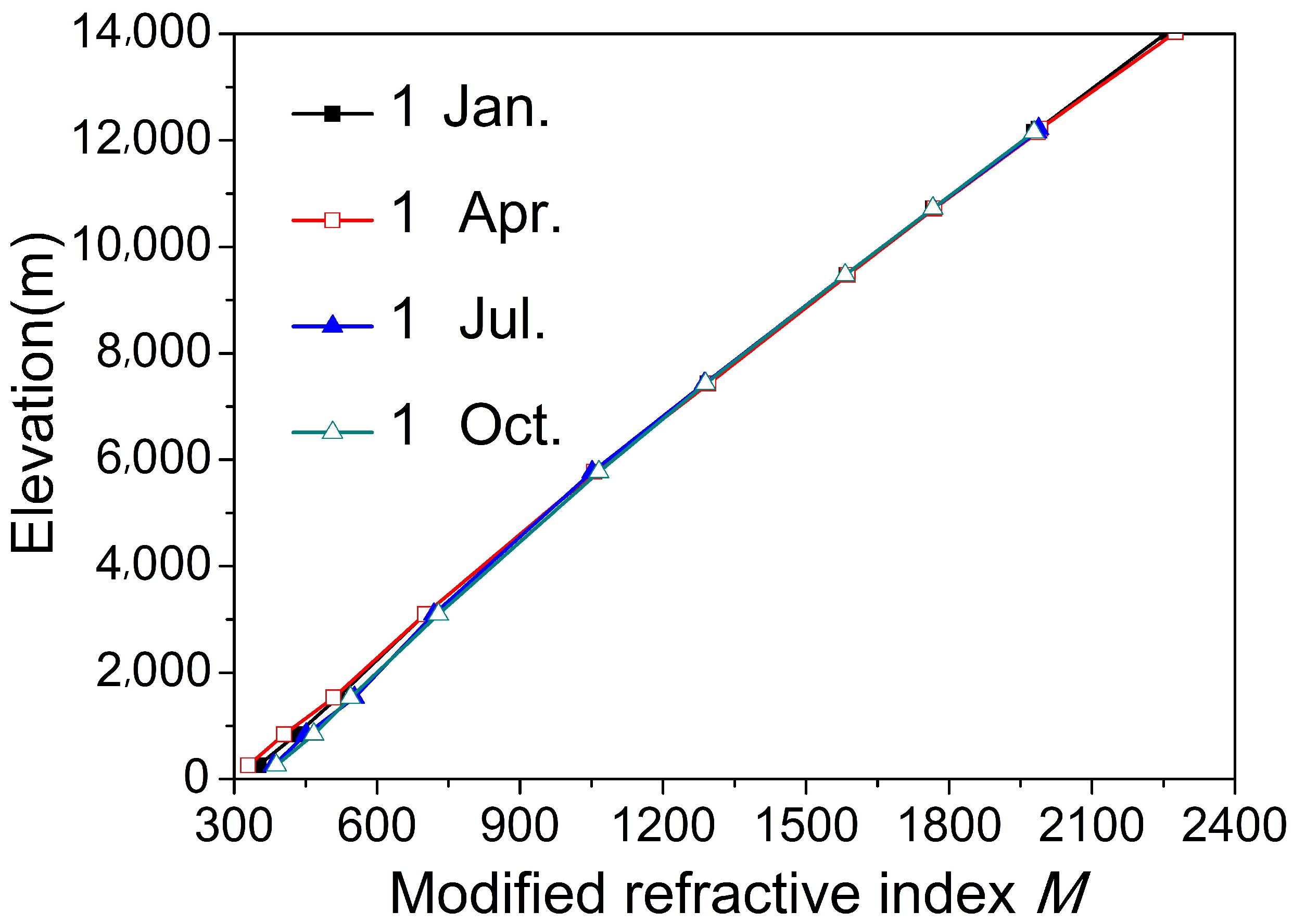
2.2. Modified by the Curvature of the Earth
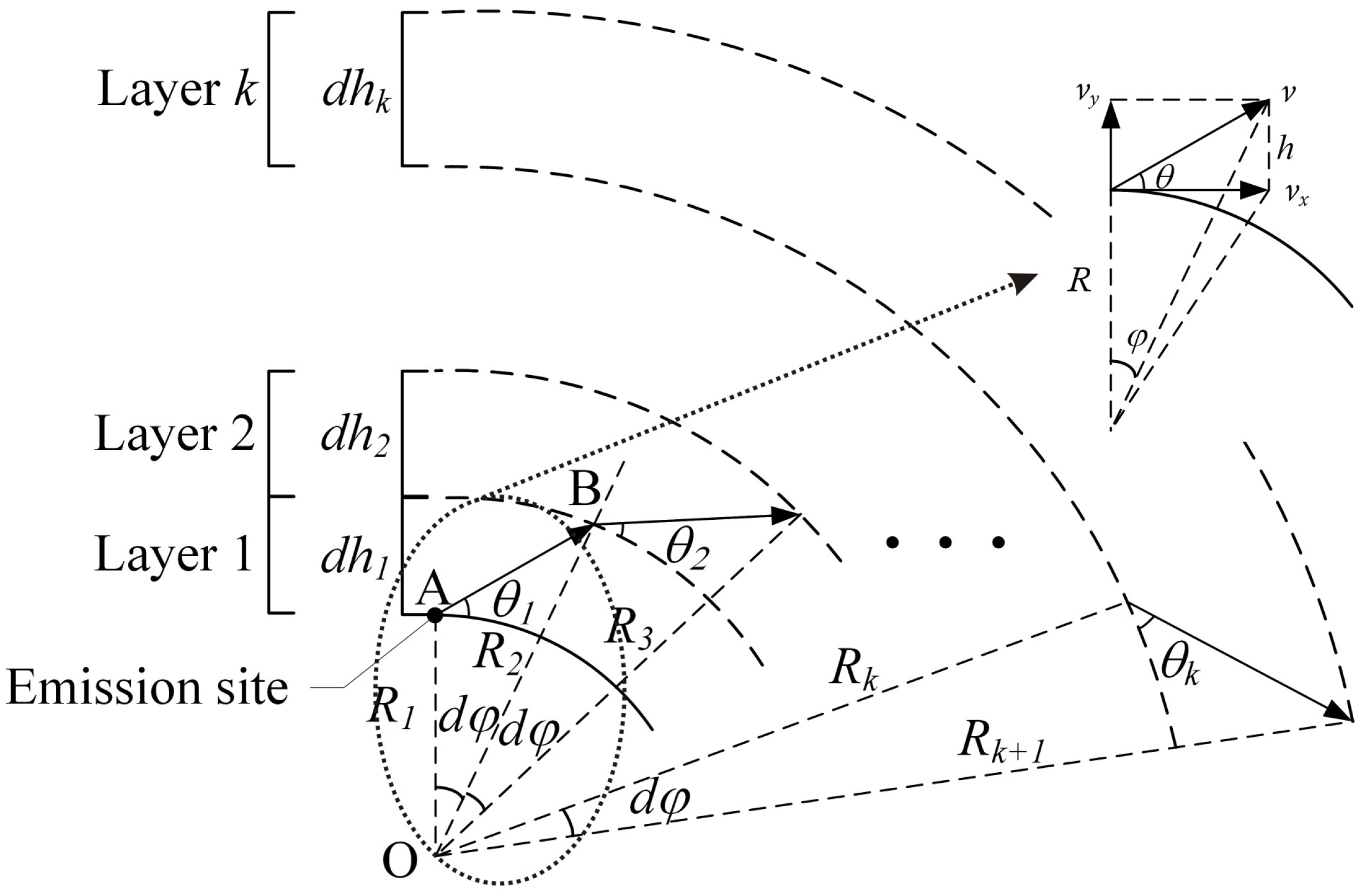
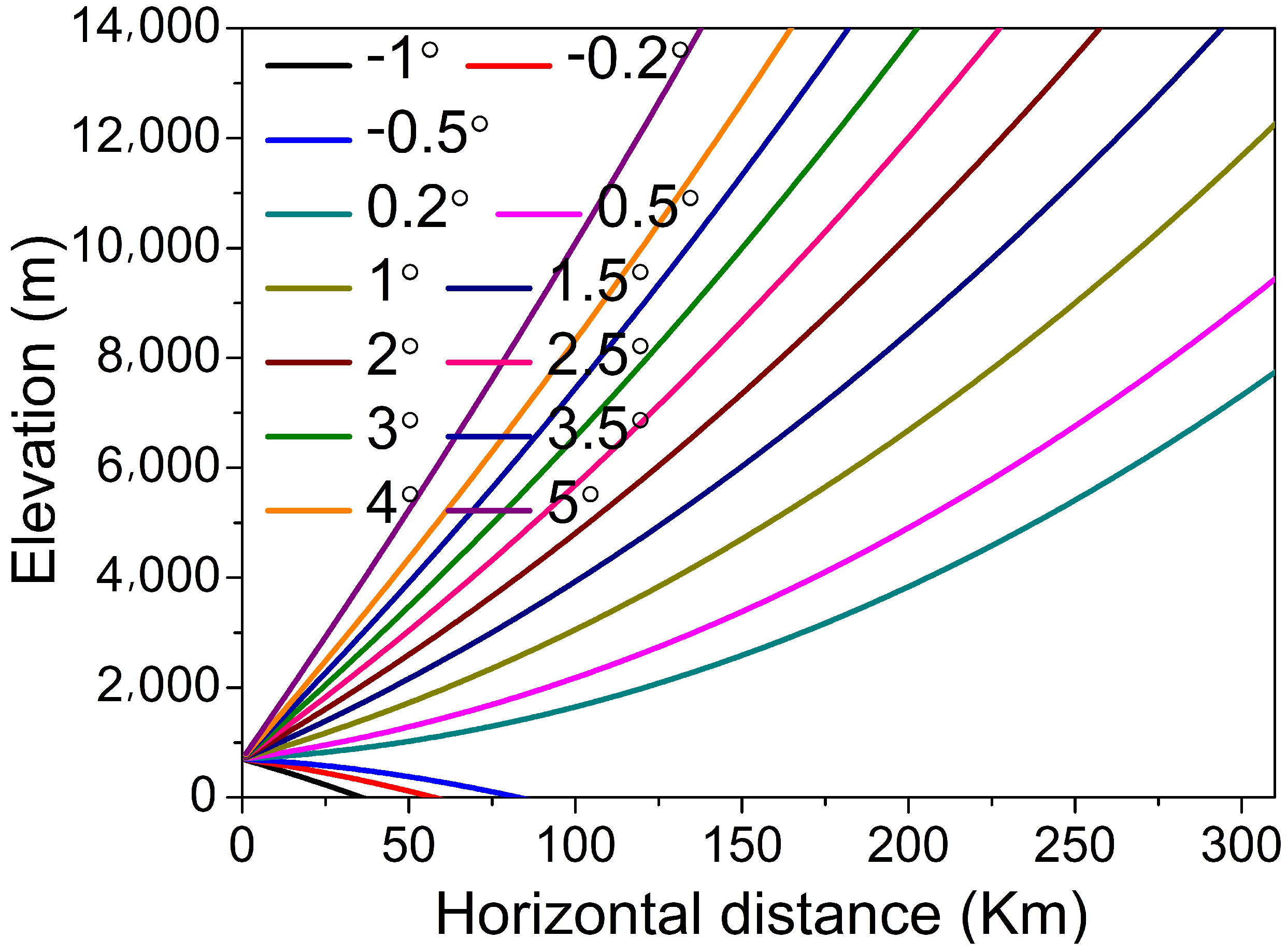
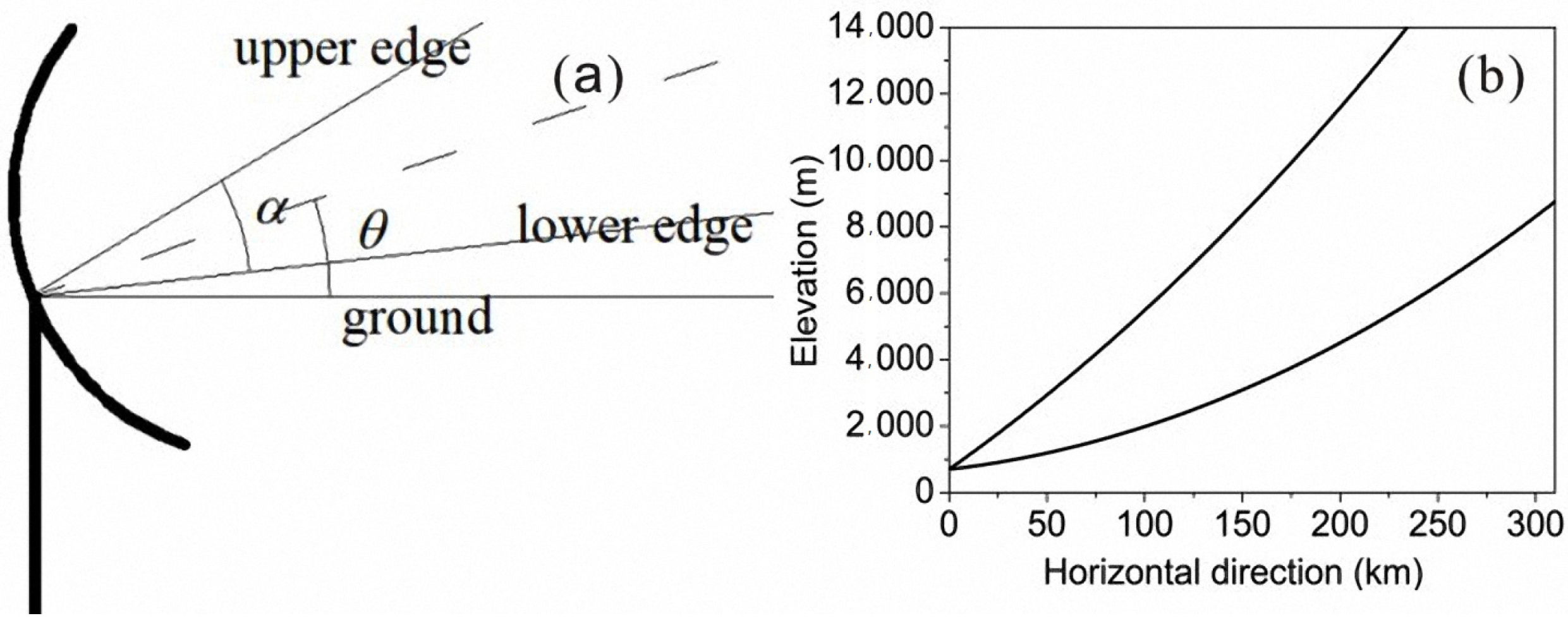
3. Propagation Path of the Radio Wave in Troposphere
4. PE with Actual Refractivity
4.1. Overview of the PE Method
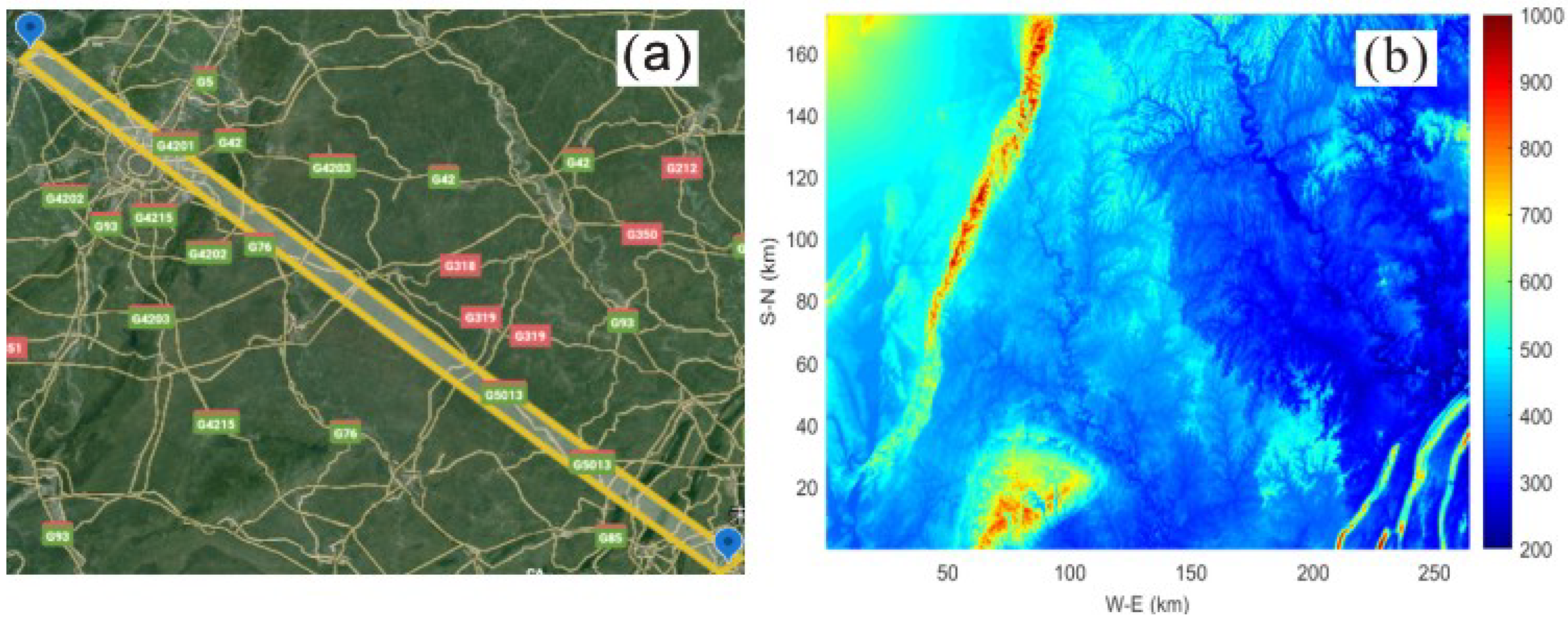
4.2. Calculation Conditions
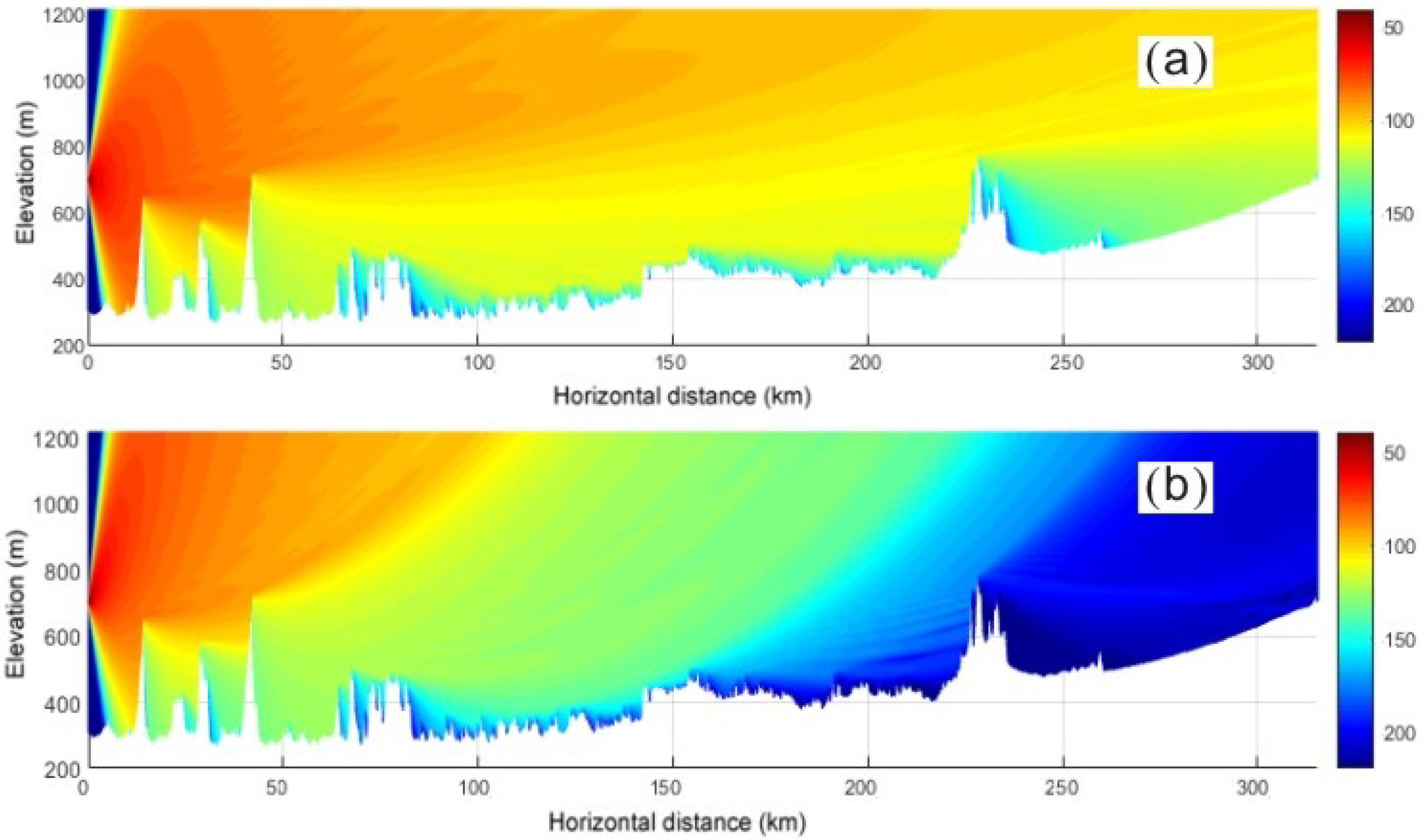
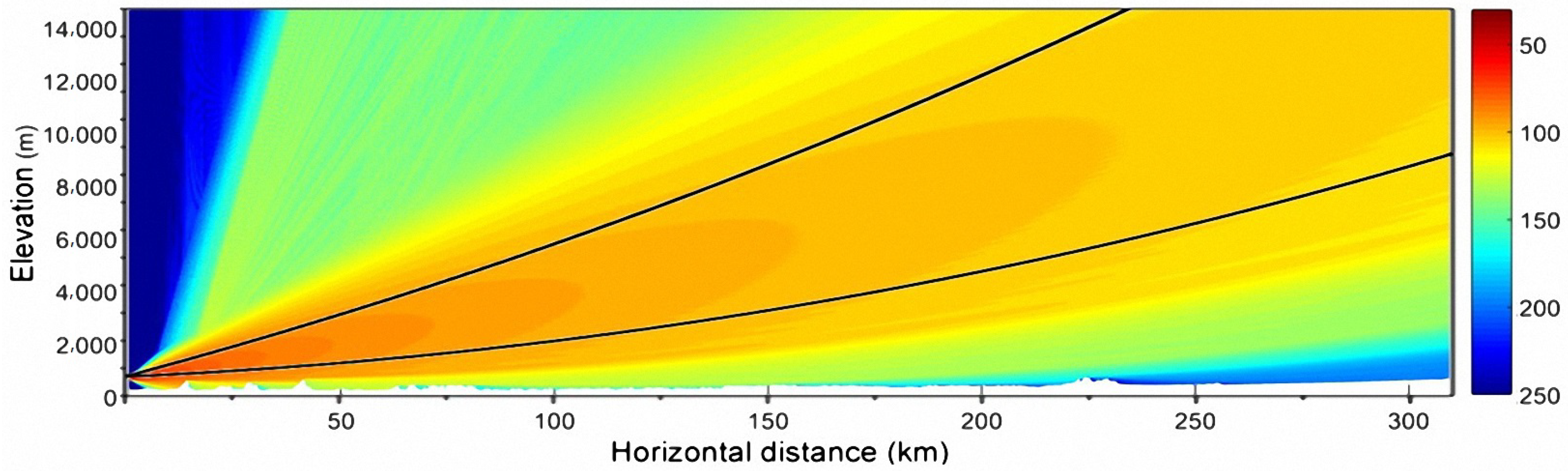
4.3. Calculation Results
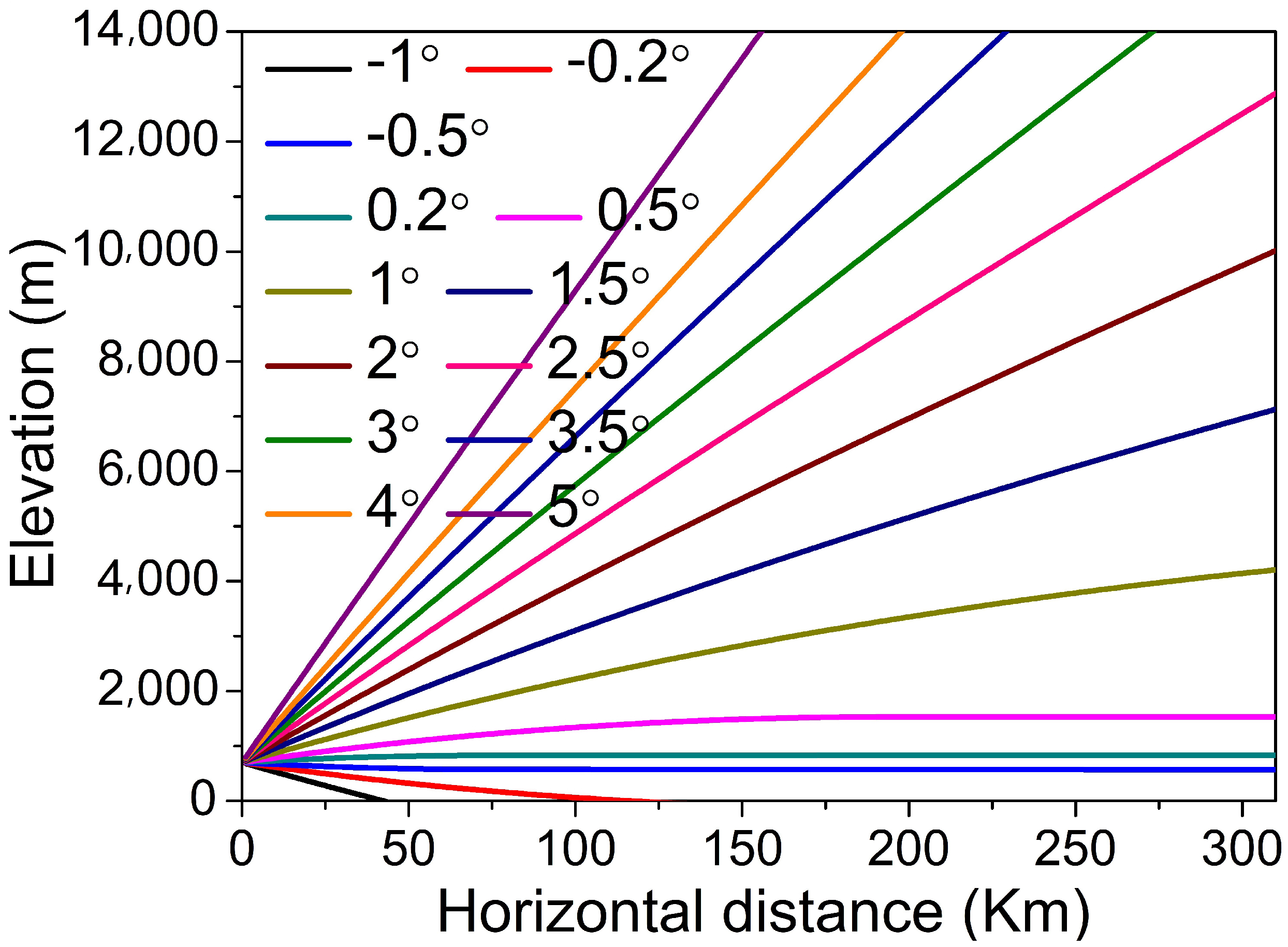
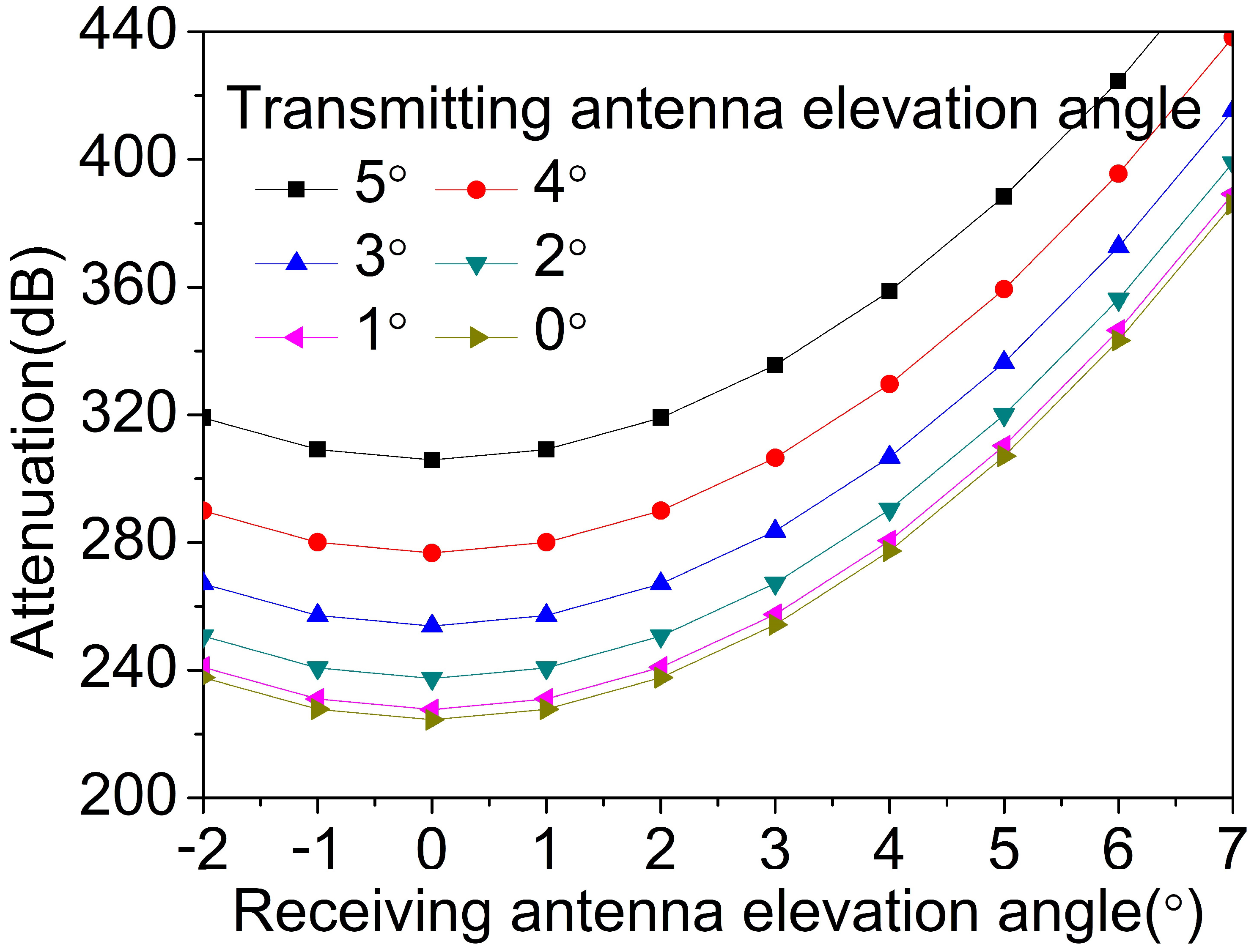
4.4. Effect of Antenna Elevation Angle
5. Comparison of Results
5.1. The Propagation Paths
5.2. The PE Method and the Measured Data
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yang, Y.; Long, Y. Modeling EM pulse propagation in the troposphere based on the TDPE method. IEEE Antennas Wirel. Propag. Lett. 2013, 12, 190–193. [Google Scholar] [CrossRef]
- Zelley, A.C.; Constantinou, C.C. A three-dimensional parabolic equation applied to VHF/UHF propagation over irregular terrain. IEEE Trans. Antennas Propag. 1999, 47, 1586–1596. [Google Scholar] [CrossRef]
- Holm, P.; Lundborg, B.; Waern, A. Parabolic Equation Technique in Vegetation and Urban Environments; FOI Report; Swedish Defence Research Agency: Linköping, Sweden, 2003. [Google Scholar]
- Awadallah, R.S.; Gehman, J.Z.; Kuttler, J.R.; Newkirk, M.H. Effects of lateral terrain variations on tropospheric radar propagation. IEEE Trans. Antennas Propag. 2005, 53, 420–434. [Google Scholar] [CrossRef]
- Ozgun, O. Recursive two-way parabolic equation approach for modeling terrain effects in tropospheric propagation. IEEE Trans. Antennas Propag. 2005, 57, 2706–2714. [Google Scholar] [CrossRef]
- Sizun, H.; de Fornel, P. Radio Wave Propagation for Telecommunication Applications; Springer: Paris, France, 2005; p. 171. [Google Scholar]
- Sofos, T.; Constantinou, P. Propagation model for vegetation effects in terrestrial and satellite mobile systems. IEEE Trans. Antennas Propag. 2004, 52, 1917–1920. [Google Scholar] [CrossRef]
- Arshad, K.; Katsriku, F.; Lasebae, A. Radiowave VHF Propagation modelling in forest using finite elements. In Proceedings of the 2006 2nd International Conference on Information & Communication Technologies, Damascus, Syria, 24–28 April 2006; Volume 2, pp. 2146–2149. [Google Scholar]
- Gay-Fernandez, J.A.; Cuinas, I. Peer to peer wireless propagation measurements and path-loss modeling in vegetated environments. IEEE Trans. Antennas Propag. 2013, 61, 3302–3311. [Google Scholar] [CrossRef]
- Bai, R.; Liao, C.; Sheng, N.; Zhang, Q. Prediction of wave propagation over digital terrain by parabolic equation model. In Proceedings of the 2013 5th IEEE International Symposium on Microwave, Antenna, Propagation and EMC Technologies for Wireless Communications, Chengdu, China, 29–31 October 2013; pp. 458–461. [Google Scholar]
- Guan, X.-W.; Guo, L.-X.; Wang, Y.-J.; Li, Q.-L. Parabolic Equation Modeling of Propagation over Terrain Using Digital Elevation Model. Int. J. Antennas Propag. 2018, 2018, 1–6. [Google Scholar] [CrossRef] [Green Version]
- Goudar, B.; Watson, R.J. Variability in propagation path delay for atmospheric remote sensing. In Proceedings of the 2014 IEEE Geoscience and Remote Sensing Symposium, Quebec City, QC, Canada, 13–18 July 2014; pp. 4127–4130. [Google Scholar]
- Levis, C.; Johnson, J.T.; Teixeira, F.L. Radiowave Propagation: Physics and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2010; pp. 120–134. [Google Scholar]
- Tao, T.; Guo, L.; Liu, L. Numerical modeling the propagation path of radio waves with atmospheric refractivity. Microw. Opt. Technol. Lett. 2020, 62, 1651–1655. [Google Scholar]
- Liu, Y.; Tang, T.; Liu, G.; Olaimat, M. Error Analysis of the Numerical Method for Correcting the Propagation of EM waves in the Troposphere. J. Microw. Optoelectron. Electromagn. Appl. 2020, 19, 407–414. [Google Scholar] [CrossRef]
- Arshad, K.; Katsriku, F.; Lasebae, A. Propagation modelling in troposphere using paraxial form of Helmholtz equation. In Proceedings of the 2005 Asia-Pacific Microwave Conference Proceedings, Suzhou, China, 4–7 December 2005; pp. 752–755. [Google Scholar]
- Fang, J.; Li, Q. Simulation method for wave propagation in the troposphere duct. In Proceedings of the 6th International Symposium on Antennas, Propagation and EM Theory, Beijing, China, 28 October–1 November 2003; pp. 524–527. [Google Scholar]
- Kang, S.; Wang, H. Analysis of microwave over-the-horizon propagation on the sea. In Proceedings of the 2009 Asia Pacific Microwave Conference, Singapore, 7–10 December 2009; pp. 1545–1548. [Google Scholar]
- Ozgun, Z.; Tanyer, S.G.; Erol, C.B. An examination of the Fourier split-step method of representing electromagnetic propagation in the troposphere. In Proceedings of the IEEE International Geoscience and Remote Sensing Symposium, Honolulu, HI, USA, 25–30 July 2010; Volume 6, pp. 3548–3550. [Google Scholar]
- Sirkova, I.; Mikhalev, M. Parabolic wave equation method applied to the tropospheric ducting propagation problem: A survey. Electromagnetics 2006, 26, 155–173. [Google Scholar] [CrossRef]
- Sirkova, I. Brief review on PE method application to propagation channel modeling in sea environment. Open Eng. 2011, 2, 19–38. [Google Scholar] [CrossRef]
- Karagianni, E.A.; Mitropoulos, A.P.; Latif, I.; Kavousanos-Kavousanakis, A.; Koukos, J.; Fafalios, M.E. Atmospheric effects on em propagation and weather effects on the performance of a dual band antenna for wlan communications. Nausivios Chora 2014 Part B Electr. Eng. Comput. Sci. 2014, 5, B-29. [Google Scholar]
- Zhao, X. On enhancement effect of scattering communication by acoustic wave interference in the troposphere. In Proceedings of the 2016 Progress in Electromagnetic Research Symposium, Shanghai, China, 8–11 August 2016; pp. 3634–3637. [Google Scholar]
- Abdullah, M.; Ullah, H.E.; Rasheed, H.; Mufti, N. An investigation of the radio refractive conditions in lowest portions of the troposphere near Margalla Hills, Pakistan. In Proceedings of the 2016 IEEE-APS Topical Conference on Antennas and Propagation in Wireless Communications, Cairns, QLD, Australia, 19–23 September 2016; pp. 225–228. [Google Scholar]
- Ning, W.; Li-xin, G.; Zhen-wei, Z.; Le-ke, L.; Zong-hua, D. Retrieving the troposphere duct by using ground-based radiometric profiler in South Coast in China. In Proceedings of the 2016 11th International Symposium on Antennas, Propagation and EM Theory, Guilin, China, 18–21 October 2016; pp. 216–219. [Google Scholar]
- University of Wyoming, Department of Atmospheric Science, Soundings. Available online: http://weather.uwyo.edu/upperair/sounding.html (accessed on 10 December 2021).
- Grabner, M.; Kvicera, V.A. Electromagnetic Waves; InTech: Rijeka, Croatia, 2011; pp. 139–156. [Google Scholar]
- Chao, Y.; Li-Xin, G.; Hong-Qiang, L. Modelling the Radio Wave Propagation in the Troposphere with Discrete Mixed Fourier Method. In Proceedings of the 2008 8th International Symposium on Antennas, Propagation and EM Theory, Kunming, China, 2–5 November2008; pp. 389–392. [Google Scholar]
- Zhang, D.; Liao, C.; Feng, J.; Deng, X. Pulse-Compression Signal Propagation and Parameter Estimation in the Troposphere With Parabolic Equation. IEEE Access 2019, 7, 99917–99927. [Google Scholar] [CrossRef]


| Item | Electrical Conductivity (S/m) | Relative Permittivity |
|---|---|---|
| wet soil | 0.02 | 20 |
| fresh water | 0.22 | 81 |
| concrete (buildings) | 0.015 | 7 |
| forest | 0.39 | leaf 26, branch 20 |
| grass | 0.85 | 40 |
| Time | 5 January 2020 | 2 April 2020 | 12 July 2020 | 9 October 2020 |
|---|---|---|---|---|
| Atn. | 230.65 | 236.24 | 240.32 | 238.47 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, M.; Olaimat, M.; Tang, T.; Ramahi, O.M.; Aldhaeebi, M.; Jin, Z.; Zhu, M. Numerical Modeling of the Radio Wave Over-the-Horizon Propagation in the Troposphere. Atmosphere 2022, 13, 1184. https://doi.org/10.3390/atmos13081184
Xu M, Olaimat M, Tang T, Ramahi OM, Aldhaeebi M, Jin Z, Zhu M. Numerical Modeling of the Radio Wave Over-the-Horizon Propagation in the Troposphere. Atmosphere. 2022; 13(8):1184. https://doi.org/10.3390/atmos13081184
Chicago/Turabian StyleXu, Min, Melad Olaimat, Tao Tang, Omar M. Ramahi, Maged Aldhaeebi, Zhu Jin, and Ming Zhu. 2022. "Numerical Modeling of the Radio Wave Over-the-Horizon Propagation in the Troposphere" Atmosphere 13, no. 8: 1184. https://doi.org/10.3390/atmos13081184
APA StyleXu, M., Olaimat, M., Tang, T., Ramahi, O. M., Aldhaeebi, M., Jin, Z., & Zhu, M. (2022). Numerical Modeling of the Radio Wave Over-the-Horizon Propagation in the Troposphere. Atmosphere, 13(8), 1184. https://doi.org/10.3390/atmos13081184








