Low-Latitude Ionospheric Responses and Coupling to the February 2014 Multiphase Geomagnetic Storm from GNSS, Magnetometers, and Space Weather Data
Abstract
:1. Introduction
- The Equatorial Ionization Anomaly (EIA) [21,22], where the E × B drift moves plasma vertically as a result of the east/west electric field. At high altitudes, the pressure gradients and gravity become important and cause plasma to diffuse along the lines of Earth’s magnetic field. As a result, two peaks of electron density at ±15° from the geomagnetic equator and a trough of electron density at the geomagnetic equator are created.
- Equatorial plasma bubbles (EPB) related to the pre-reversal enhancement (PRE) of the eastward electric field around sunset [25,26,27]. The electric field is eastward during the day and westward during night, and the PRE occurs as an eastward electric field increases before turning westward at sunset. The PRE associated with the sharp ionospheric depletion at sunset creates a Rayleigh Taylor instability at the origin of EPB.
- Transmission of electric fields related to the motion of particles in the magnetosphere, namely Prompt Penetration Electric Field (PPEF) [28].
- The electrojet in the auroral zone (ionospheric electric currents) dissipates energy through Joule heating and produces a thermal expansion of the atmosphere (variations in pressure, temperature, and motion) [29].
2. Data and Methods
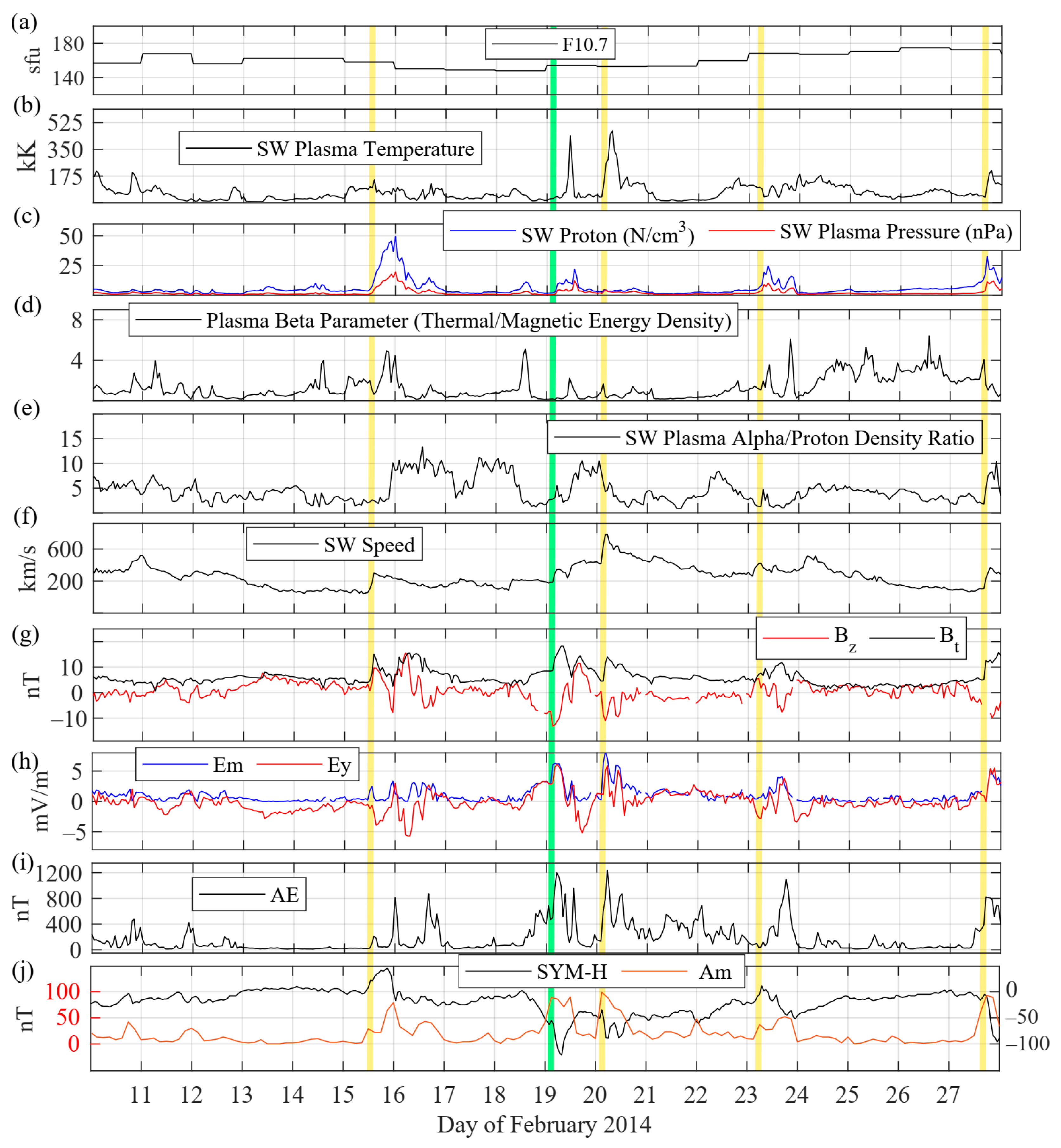
2.1. Solar Wind and Geomagnetic Indices
2.2. Vertical TEC from GNSS Stations in Africa
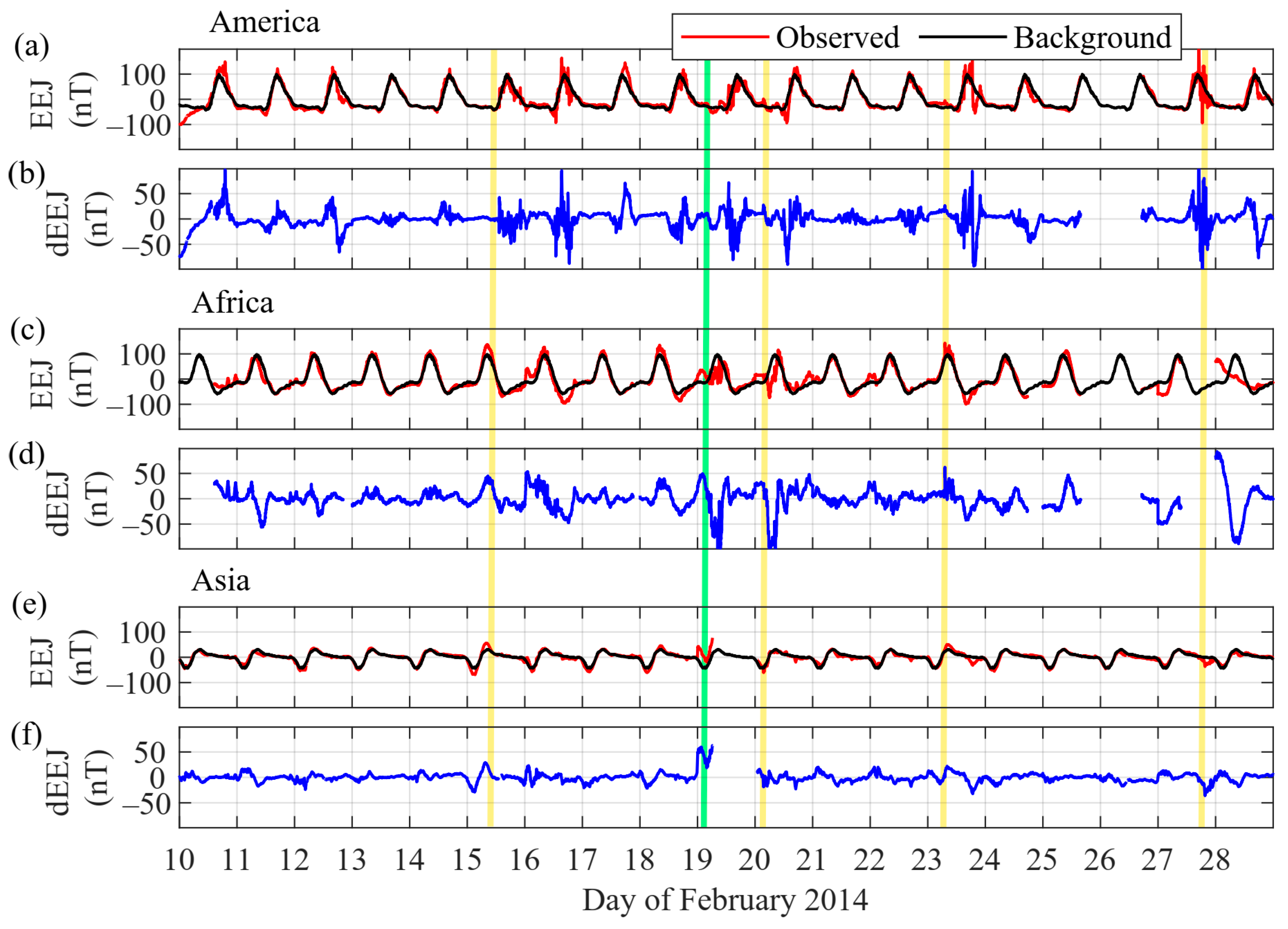
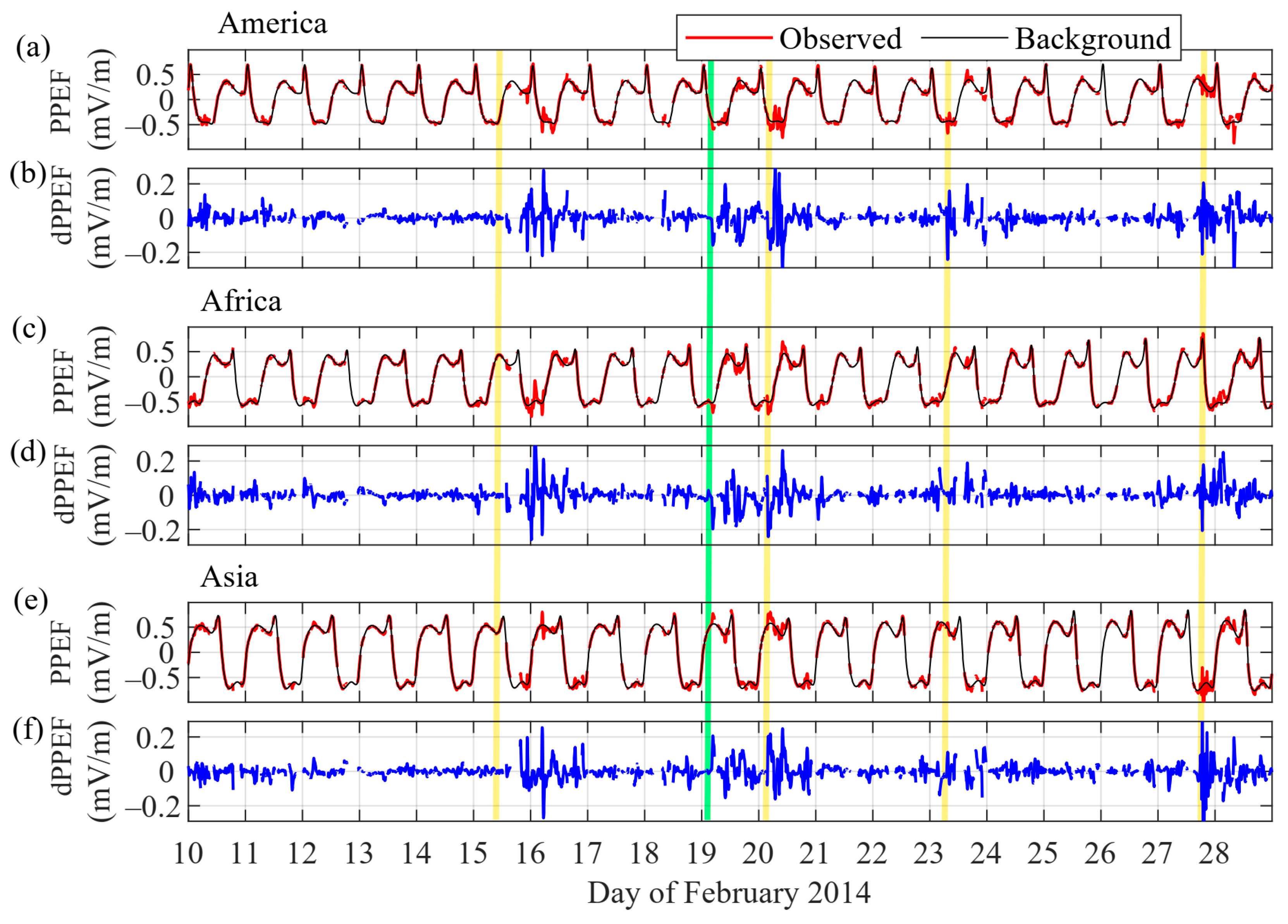
2.3. Penetration Electric Fields, Equatorial Electrojet, and Current Intensity
2.4. Vertical TEC from the International GNSS Service and Empirical Models
2.4.1. IGS Global Ionospheric Maps
2.4.2. The International Reference Ionosphere Model
2.4.3. A New TEC Model Based on Principal Component Analysis
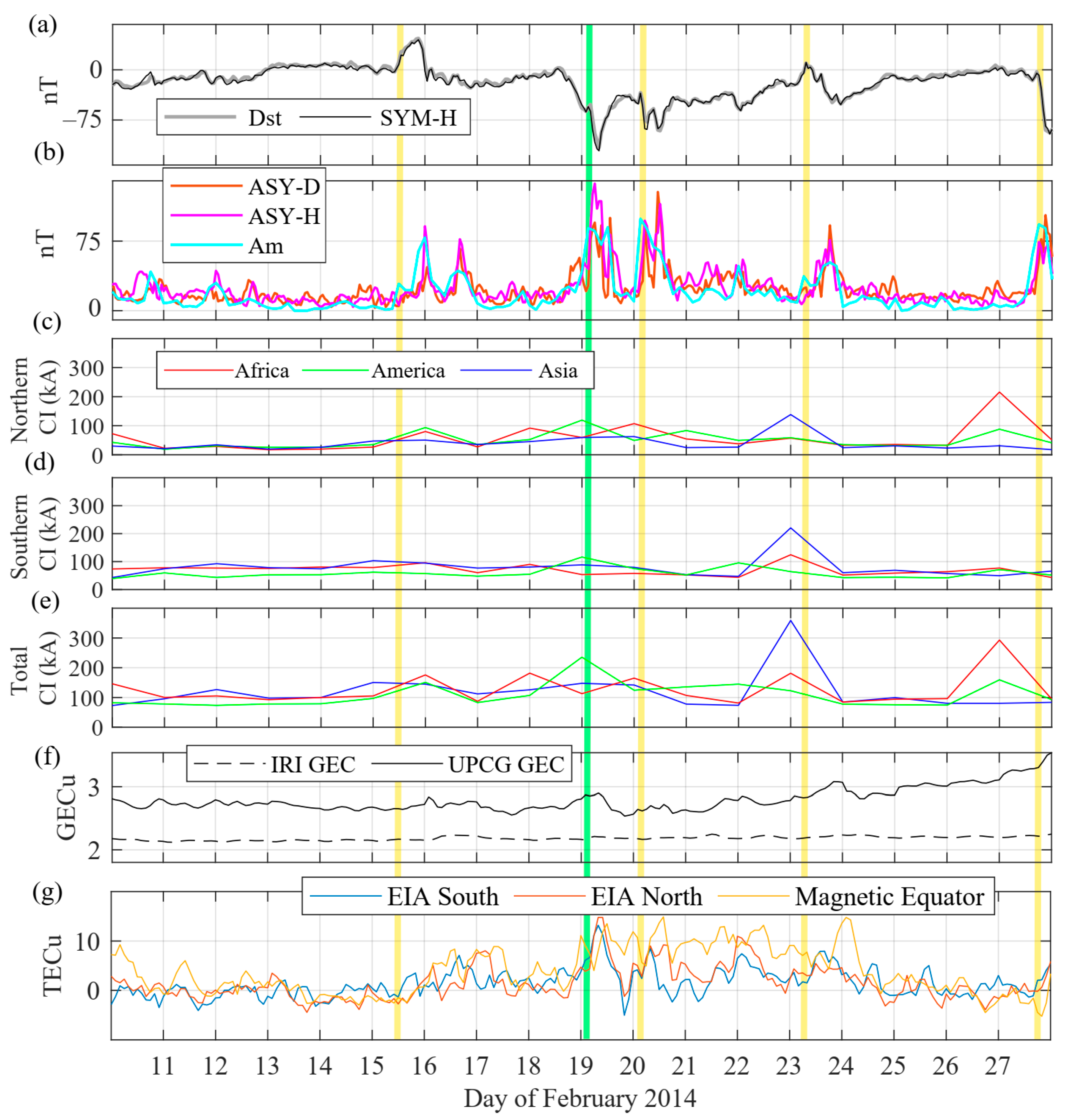
3. Results and Analyses
4. Discussion
5. Conclusions
- Clear TEC depletions/enhancements were observed at the GNSS stations used in this study, and TEC enhancements from the IGS GIMs are up to 20 TECU, lasting from 16 to 18 February 2014. A delay in TEC enhancements towards high-latitude stations was observed, which is attributed to the EIA expansion. TEC enhancements up to 20 TECU have been at low-latitudes with longer-lasting TEC enhancements at the dip equator (from 16 to 18 February) rather than in the EIA crests (only after the CMEs arrivals).
- Rapidly after the arrival of each CME, PPEF disturbances with amplitudes of 0.4 mV/m have been observed in the American, African, and Asian sectors. The EEJ variations exceeded amplitudes of 100 nT with the exception of the Asian region, which has shown minimal EEJ variability.
- The HSSW event on 19 February seems to have reenergized the existing storm conditions (CME arrival of 15 February) and subsequently caused a moderate G-2 geomagnetic storm, which was further enhanced by two more SSC events on 20 and 23 February. The prevalent magnetospheric convection caused by the preceding southward IMF on 19 February was the cause of no classical SSC. From the HSSW event, there has been the observation of a gradual increase in GEC up to 3.5 GECU at the end of the month, showing approximately 1 GECU of differential intensification. This gradient is not reflected by the IGS-derived TEC profiles, but clear rTEC enhancements over 60% during the same period at the RABT GNSS ground station were noticed, suggesting this enhanced TEC to be located at higher latitudes.
- The IRI-2012 model did not represent the actual TEC variability during the storms period, showing no distinction between events, and a clear bias of ~20 TECU to the actual quiet-time TEC. There was also a detection of a clear discrepancy in the distribution of TEC along the EIA, showing that IRI-2012 underestimates the northern crest at the African and Asian sectors and at both crests in the American sector. The median February average from the IGS GIM TEC shows enhanced values in the southern hemisphere, whereas the IRI-2012 does not show this asymmetry.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hernández-Pajares, M.; Juan, J.; Sanz, J. New approaches in global ionospheric determination using ground GPS data. J. Atmos. Sol. Terr. Phys. 1999, 61, 1237–1247. [Google Scholar] [CrossRef]
- Jin, S.G.; Su, K. PPP models and performances from single- to quad-frequency BDS observations. Satell. Nav. 2020, 1, 16. [Google Scholar] [CrossRef]
- Afraimovich, E.L.; Astafyeva, E.I.; Demyanov, V.V.; Edemskiy, I.K.; Gavrilyuk, N.S.; Ishin, A.B.; Kosogorov, E.A.; Leonovich, L.A.; Lesyuta, O.S.; Perevalova, N.P.; et al. Review of GPS/GLONASS studies of the ionospheric response to natural and anthropogenic processes and phenomena. J. Space Weather Space Clim. 2013, 3, A27. [Google Scholar] [CrossRef] [Green Version]
- Jin, S.; Jin, R.; Kutoglu, H. Positive and negative ionospheric responses to the March 2015 geomagnetic storm from BDS observations. J. Geod. 2017, 91, 613–626. [Google Scholar] [CrossRef]
- Gao, C.; Jin, S.; Yuan, L. Ionospheric responses to the June 2015 geomagnetic storm from ground and LEO GNSS observations. Remote Sens. 2020, 12, 2200. [Google Scholar] [CrossRef]
- Ratovsky, K.G.; Klimenko, M.V.; Dmitriev, A.V.; Medvedeva, I.V. Relation of Extreme Ionospheric Events with Geomagnetic and Meteorological Activity. Atmosphere 2022, 13, 146. [Google Scholar] [CrossRef]
- Klobuchar, J.A. Ionospheric time-delay algorithm for single-frequency GPS users. IEEE Trans. Aerosp. Electron. Syst. 1987, 23, 325–331. [Google Scholar] [CrossRef]
- Huba, J.D.; Joyce, G.; Fedder, J.A. Sami2 is Another Model of the Ionosphere (SAMI2): A new low-latitude ionosphere model. J. Geophys. Res. Atmos. 2000, 105, 23035–23053. [Google Scholar] [CrossRef]
- Hoque, M.M.; Jakowski, N.; Berdermann, J. Ionospheric correction using NTCM driven by GPS Klobuchar coefficients for GNSS applications. GPS Solut. 2017, 21, 1563–1572. [Google Scholar] [CrossRef]
- Radicella, S. The NeQuick model genesis, uses and evolution. Ann. Geophys. 2009, 52, 417–422. [Google Scholar] [CrossRef]
- Okoh, D.; Onwuneme, S.; Seemala, G.; Jin, S.G.; Rabiu, B.; Nava, B.; Uwamahoro, J. Assessment of the NeQuick-2 and IRI-Plas 2017 models using global and long-term GNSS measurements. J. Atmos. Sol. Terr. Phys. 2018, 170, 1–10. [Google Scholar] [CrossRef]
- Bilitza, D. IRI the International Standard for the Ionosphere. Adv. Radio Sci. 2018, 16, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Fuller Rowell, T.J. Stormtime response of the thermosphereionosphere system. In Aeronomy of the Earth’s Atmosphere and Ionosphere; Abdu, M.A., Pancheva, D., Bhattacharyya, A., Eds.; IAGA Spec. Sopron Book Series; Springer: Dordrecht, The Netherlands, 2011; Chapter 32; Volume 2, p. 419435. [Google Scholar] [CrossRef]
- Calabia, A.; Jin, S. Characterization of the Upper Atmosphere from Neutral and Electron Density Observations. In International Association of Geodesy Symposia; Springer: Berlin/Heidelberg, Germany, 2020. [Google Scholar] [CrossRef]
- Astafyeva, E.; Bagiya, M.S.; Förster, M.; Nishitani, N. Unprecedented Hemispheric Asymmetries During a Surprise Ionospheric Storm: A Game of Drivers. J. Geophys. Res. Space Phys. 2020, 125, e2019JA027261. [Google Scholar] [CrossRef]
- Shah, M.; Abbas, A.; Ehsan, M.; Calabia, A.; Adhikari, B.; Tariq, M.A.; Ahmed, J.; de Oliveira-Junior, J.F.; Yan, J.; Melgarejo-Morales, A.; et al. Ionospheric-Thermospheric Responses to the August 2018 Geomagnetic Storm over South America from Multiple observable. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2022, 15, 261–269. [Google Scholar] [CrossRef]
- Cai, X.; Burns, A.G.; Wang, W.; Qian, L.; Solomon, S.C.; Eastes, R.W.; McClintock, W.E.; Laskar, F.I. Isnvestigation of a neutral “tongue” observed by GOLD during the geomagnetic storm on 11 May 2019. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028817. [Google Scholar] [CrossRef]
- Jin, S.G.; Gao, C.; Yuan, L.; Guo, P.; Calabia, A.; Ruan, H.; Luo, P. Long-term variations of plasmaspheric total electron content from topside GPS observations on LEO satellites. Remote Sens. 2021, 13, 545. [Google Scholar] [CrossRef]
- Calabia, A.; Jin, S. Solar cycle, seasonal, and asymmetric dependencies of thermospheric mass density disturbances due to magnetospheric forcing. Ann. Geophys. 2019, 37, 989–1003. [Google Scholar] [CrossRef] [Green Version]
- Calabia, A.; Jin, S. Thermospheric mass density disturbances due to magnetospheric forcing from 2014–2020 CASSIOPE precise orbits. J. Geophys. Res. Space Phys. 2021, 126, e2021JA029540. [Google Scholar] [CrossRef]
- Namba, S.; Maeda, K.-I. Radio Wave Propagation; Corona Publishing: Tokyo, Japan, 1939; 86p. [Google Scholar]
- Appleton, E. Two anomalies in the ionosphere. Nature 1946, 693, 57–69. [Google Scholar] [CrossRef]
- Chapman, S. The equatorial electrojet as detected from the abnormal electric current distribution above Huancayo Peru, and elsewhere. Arch Meteorol. Geophys. U Bioklimatol. Ser. 1951, 4, 368–374. [Google Scholar] [CrossRef]
- Takeda, M. Contribution of wind, conductivity, and geomagnetic main field to the variation in the geomagnetic Sq field. J. Geophys. Res. Space Phys. 2013, 118, 4516–4522. [Google Scholar] [CrossRef]
- Basu, S.; Basu, S. Equatorial scintillations, a review. J. Atmos. Terr. Phys. 1981, 43, 473–478. [Google Scholar] [CrossRef]
- Kelley, M.C. The Earth Ionosphere; Elsevier Press: Amsterdam, The Netherlands; Academic Press: San Diego, CA, USA, 1989. [Google Scholar]
- Fejer, B.G.; de Paula, E.R.; González, S.A.; Woodman, R.F. Average vertical and zonal F region drifts over Jicamarca. J. Geophys. Res. Space Phys. 1991, 96, 13901–13906. [Google Scholar] [CrossRef] [Green Version]
- Vasyliunas, V.M. Mathematical models of the magnetospheric convection and its coupling to the ionosphere. In Particles and Fields in the Magnetosphere; McCormac, M., Ed.; Springer: New York, NY, USA, 1970. [Google Scholar] [CrossRef]
- Fuller-Rowell, T.J.; Codrescu, M.V.; Moffett, R.J.; Quegan, S. Response of the thermosphere and ionosphere to geomagnetic storms. J. Geophys. Res. Space Phys. 1994, 99, 3893–3914. [Google Scholar] [CrossRef]
- Blanc, M.; Richmond, A.D. The ionospheric disturbance dynamo. J. Geophys. Res. Space Phys. 1980, 85, 1669. [Google Scholar] [CrossRef]
- Fejer, B.G.; Larsen, M.F.; Farley, D.T. Equatorial disturbance dynamo electric fields. Geophys. Res. Lett. 1983, 10, 537–540. [Google Scholar] [CrossRef]
- Jacobsen, K.S.; Dähnn, M. Statistics of ionospheric disturbances and their correlation with GNSS positioning errors at high latitudes. J. Space Weather. Space Clim. 2014, 4, A27. [Google Scholar] [CrossRef] [Green Version]
- Jakowski, N.; Béniguel, Y.; de Franceschi, G.; Pajares, M.H.; Jacobsen, K.S.; Stanislawska, I.; Tomasik, L.; Warnant, R.; Wautelet, G. Monitoring, tracking and forecasting ionospheric perturbations using GNSS techniques. J. Space Weather. Space Clim. 2012, 2, A22. [Google Scholar] [CrossRef] [Green Version]
- Wang, W.; Lei, J.; Burns, A.G.; Solomon, S.C.; Wiltberger, M.; Xu, J.; Zhang, Y.; Paxton, L.; Coster, A. Ionospheric response to the initial phase of geomagnetic storms: Common features. J. Geophys. Res. Space Phys. 2010, 115, A07321. [Google Scholar] [CrossRef]
- Lei, J.; Wang, W.; Burns, A.G.; Solomon, S.C.; Richmond, A.D.; Wiltberger, M.; Goncharenko, L.P.; Coster, A.; Reinisch, B.W. Observations and simulations of the ionospheric and thermospheric response to the December 2006 geomagnetic storm: Initial phase. J. Geophys. Res. Space Phys. 2008, 113, A01314. [Google Scholar] [CrossRef]
- Sahai, Y.; Fagundes, P.R.; Becker-Guedes, F.; Bolzan, M.J.A.; Abalde, J.R.; Gil Pillat, V.; De Jesus, R.; Lima, W.L.C.; Crowley, G.; Shiokawa, K.; et al. Effects of the major geomagnetic storms of October 2003 on the equatorial and low latitude F region in two longitudinal sectors. J. Geophys. Res. Space Phys. 2005, 110, A12S91. [Google Scholar] [CrossRef]
- Kuai, J.; Liu, L.; Liu, J.; Zhao, B.; Chen, Y.; Le, H.; Wan, W. The long-duration positive storm effects in the equatorial ionosphere over Jicamarca. J. Geophys. Res. Space Phys. 2015, 120, 1311–1324. [Google Scholar] [CrossRef]
- Heelis, R.A. Electrodynamics in the low and middle latitude ionosphere: A tutorial. J. Atmos. Sol. Terr. Phys. 2004, 66, 825–838. [Google Scholar] [CrossRef]
- Pedatella, N.M.; Forbes, J.M.; Lei, J.; Thayer, J.P.; Larson, K.M. Changes in the longitudinal structure of the low latitude ionosphere during the July 2004 sequence of geomagnetic storms. J. Geophys. Res. Space Phys. 2008, 113, A11315. [Google Scholar] [CrossRef] [Green Version]
- Astafyeva, E.; Yasyukevich, Y.V.; Maletckii, B.; Oinats, A.; Vesnin, A.; Yasyukevich, A.S.; Syrovatskii, S.; Guendouz, N. Ionospheric Disturbances and Irregularities during the 25–26 August 2018 Geomagnetic Storm. J. Geophys. Res. Space Phys. 2022, 127, e2021JA029843. [Google Scholar] [CrossRef]
- Mansilla, G.A.; Zossi, M.M. Longitudinal Variation of the Ionospheric Response to the 26 August 2018 Geomagnetic Storm at Equatorial/Low Latitudes. Pure Appl. Geophys. 2020, 177, 5833–5844. [Google Scholar] [CrossRef]
- Kutiev, I.; Watanabe, S.; Otsuka, Y.; Saito, A. Total electron content behavior over Japan during geomagnetic storms. J. Geophys. Res. Space Phys. 2005, 110, A01308. [Google Scholar] [CrossRef] [Green Version]
- Ghamry, E.; Lethy, A.; Arafa-Hamed, T.; Elaal, E.A. A comprehensive analysis of the geomagnetic storms occurred during 18 February and 2 March 2014. NRIAG J. Astron. Geophys. 2016, 5, 263–268. [Google Scholar] [CrossRef] [Green Version]
- King, J.H.; Papitashvili, N.E. Solar wind spatial scales in and comparisons of hourly Wind and ACE plasma and magnetic field data. J. Geophys. Res. 2005, 110, A02209. [Google Scholar] [CrossRef]
- Mayaud, P.N. Derivation, Meaning, and Use of Geomagnetic Indices; Geophysical Monograph Series; AGU: Washington, DC, USA, 1980; Volume 22. [Google Scholar]
- Liu, R.; Lühr, H.; Doornbos, E.; Ma, S.-Y. Thermospheric mass density variations during geomagnetic storms and a prediction model based on the merging electric field. Ann. Geophys. 2010, 28, 1633–1645. [Google Scholar] [CrossRef] [Green Version]
- Kan, J.K.; Lee, L.C. Energy coupling function and solar wind-magnetosphere dynamo. Geophys. Res. Lett. 1979, 6, 577–580. [Google Scholar] [CrossRef]
- Gurtner, W.; Estey, L. RINEX: The Receiver Independent Exchange Format Version 3.00. 20. Available online: https://files.igs.org/pub/data/format/rinex300.pdf (accessed on 6 February 2022).
- Peymirat, C.; Richmond, A.D.; Kobea, A.T. Electrodynamic coupling of high and low latitudes: Simulations of shielding and overshielding effects. J. Geophys. Res. Space Phys. 2000, 105, 22991–23003. [Google Scholar] [CrossRef]
- Manoj, C.; Maus, S.; Lühr, H.; Alken, P. Long-period prompt-penetration electric fields derived from CHAMP satellite Magnetic Measurements. J. Geophys. Res. Space Phys. 2013, 118, 5919–5930. [Google Scholar] [CrossRef] [Green Version]
- Vasyliunas, V.M. The interrelationship of magnetospheric processes. In Earth’s Magnetosphere Processes; McCormac, M., Ed.; D. Reidel: Norwell, MA, USA, 1972; pp. 29–38. [Google Scholar]
- Kobéa, A.T.; Richmond, A.D.; Emery, B.A.; Peymirat, C.; Luhr, H.; Moretto, T.; Hairston, M.; Amory-Mazaudier, C. Electrodynamic Coupling of High and Low Latitudes Observations on 27 May 1993. J. Geophys. Res. 2000, 105, 22979–22989. [Google Scholar] [CrossRef] [Green Version]
- Rabiu, A.B.; Folarin, O.O.; Uozumi, T.; Yoshikawa, A. Simultaneity and asymmetry in the occurrence of counter equatorial electrojet along African longitudes. In Ionospheric Space Weather: Longitude and Hemispheric Dependences and Lower Atmosphere Forcing, 1st ed.; Fuller-Rowell, T., Yizengaw, E., Doherty, P.H., Basu, S., Eds.; Geophysical Monograph 220; American Geophysical Union, John Wiley & Sons, Inc.: Washington, DC, USA, 2017; pp. 21–31. [Google Scholar]
- Forbes, J.M. The equatorial electrojet. Rev. Geophys. 1981, 19, 469–504. [Google Scholar] [CrossRef]
- Yamazaki, Y.; Yumoto, K.; Cardinal, M.G.; Fraser, B.J.; Hattori, P.; Kakinami, Y.; Yoshikawa, A. An empirical model of the quiet daily geomagnetic field variation. J. Geophys. Res. Space Phys. 2011, 116, A10312. [Google Scholar] [CrossRef]
- Afraimovich, E.L.; Astafyeva, E.I.; Oinats, A.V.; Yasukevich, Y.V.; Zhivetiev, I.V. Global electron content: A new conception to track solar activity. Ann. Geophys. 2008, 26, 335–344. [Google Scholar] [CrossRef] [Green Version]
- Ratovsky, K.G.; Klimenko, M.V.; Yasyukevich, Y.V.; Klimenko, V.V. Statistical Analysis of Ionospheric Global Electron Content Response to Geomagnetic Storms. In Proceedings of the 2019 Russian Open Conference on Radio Wave Propagation (RWP), Kazan, Russia, 1–6 July 2019; pp. 183–186. [Google Scholar] [CrossRef]
- He, H.; Moses, M.; Volk, A.E.; Elezz, O.A.; Kassamba, A.A.; Bilitza, D. Assessment of IRI-2016 hmF2 model options with digisonde, COSMIC and ISR observations for low and high solar flux conditions. Adv. Space Res. 2021, 68, 2093–2103. [Google Scholar] [CrossRef]
- Liu, R.Y.; Smith, P.A.; King, J.W. A New Solar Index Which Leads to Improved foF2 Predictions Using the CCIR Atlas. Telecommun. J. 1983, 50, 408–414. [Google Scholar]
- Coisson, P.; Radicella, S.; Leitinger, R.; Nava, B. Topside electron density in IRI and NeQuick. Adv. Space Res. 2006, 37, 937–942. [Google Scholar] [CrossRef]
- Rush, C.; Fox, M.; Bilitza, D.; Davies, K.; McNamara, L.; Stewart, F.; PoKempner, M. Ionospheric Mapping: An Update of foF2 Coefficients. Telecomm. J. 1989, 56, 179–182. [Google Scholar]
- Calabia, A.; Jin, S. New modes and mechanisms of long-term ionospheric TEC variations from Global Ionosphere Maps. J. Geophys. Res. Space Phys. 2020, 125, e2019JA027703. [Google Scholar] [CrossRef]
- Calabia, A.; Jin, S. Supporting Information for “New modes and mechanisms of long-term ionospheric TEC variations from Global Ionosphere Maps”. Zenodo 2019. [Google Scholar] [CrossRef]
- Strickland, D.; Daniell, R.; Craven, J. Negative ionospheric storm coincident with DE 1-observed thermospheric disturbance on 14 October 1981. J. Geophys. Res. Space Phys. 2001, 106, 21049–21062. [Google Scholar] [CrossRef]
- Lu, G.; Huba, J.D.; Valladares, C. Modeling ionospheric super-fountain effect based on the coupled TIMEGCM-SAMI3. J. Geophys. Res. Space Phys. 2013, 118, 2527–2535. [Google Scholar] [CrossRef] [Green Version]
- Pignalberi, A.; Pezzopane, M.; Nava, B.; Coïsson, P. On the link between the topside ionospheric effective scale height and the plasma ambipolar diffusion, theory and preliminary results. Sci. Rep. 2020, 10, 17541. [Google Scholar] [CrossRef]
- Kikuchi, T.; Hashimoto, K.K. Transmission of the electric fields to the low latitude ionosphere in the magnetosphereionosphere current circuit. Geosci. Lett. 2016, 3, 111. [Google Scholar] [CrossRef] [Green Version]
- Oyedokun, O.J.; Akala, A.O.; Oyeyemi, E.O. Responses of the African equatorial ionization anomaly (EIA) to some selected intense geomagnetic storms during the maximum phase of solar cycle 24. Adv. Space Res. 2021, 67, 1222–1243. [Google Scholar] [CrossRef]
- Liu, H.; Lühr, H.; Henize, V.; Köhler, W. Global distribution of the thermospheric total mass density derived from CHAMP. J. Geophys. Res. 2005, 110, A04301. [Google Scholar] [CrossRef] [Green Version]
- Kassa, T.; Damtie, B.; Bires, A.; Yizengaw, E.; Cilliers, P. Storm-time characteristics of the equatorial ionization anomaly in the East African sector. Adv. Space Res. 2015, 56, 57–70. [Google Scholar] [CrossRef]
- Huang, C.-M. Disturbance dynamo electric fields in response to geomagnetic storms occurring at different universal times. J. Geophys. Res. Space Phys. 2013, 118, 496–501. [Google Scholar] [CrossRef]
- Tsurutani, B.T.; Echer, E.; Guarnieri, F.L.; Kozyra, J.U. CAWSES November 7–8, 2004, superstorm: Complex solar and interplanetary features in the post-solar maximum phase. Geophys. Res. Lett. 2008, 35, L06S05. [Google Scholar] [CrossRef] [Green Version]
- Doornbos, E.; van den IJssel, J.; Lühr, H.; Förster, M.; Koppen-wallner, G. Neutral density and cross-wind determination from arbitrarily oriented multiaxis accelerometers on satellites. J. Spacecr. Rocket. 2010, 47, 580–589. [Google Scholar] [CrossRef] [Green Version]
- Jin, S.; Calabia, A.; Yuan, L. Thermospheric sensing from GNSS and accelerometer on small satellites. Proc. IEEE 2017, 9, 1–12. [Google Scholar] [CrossRef]
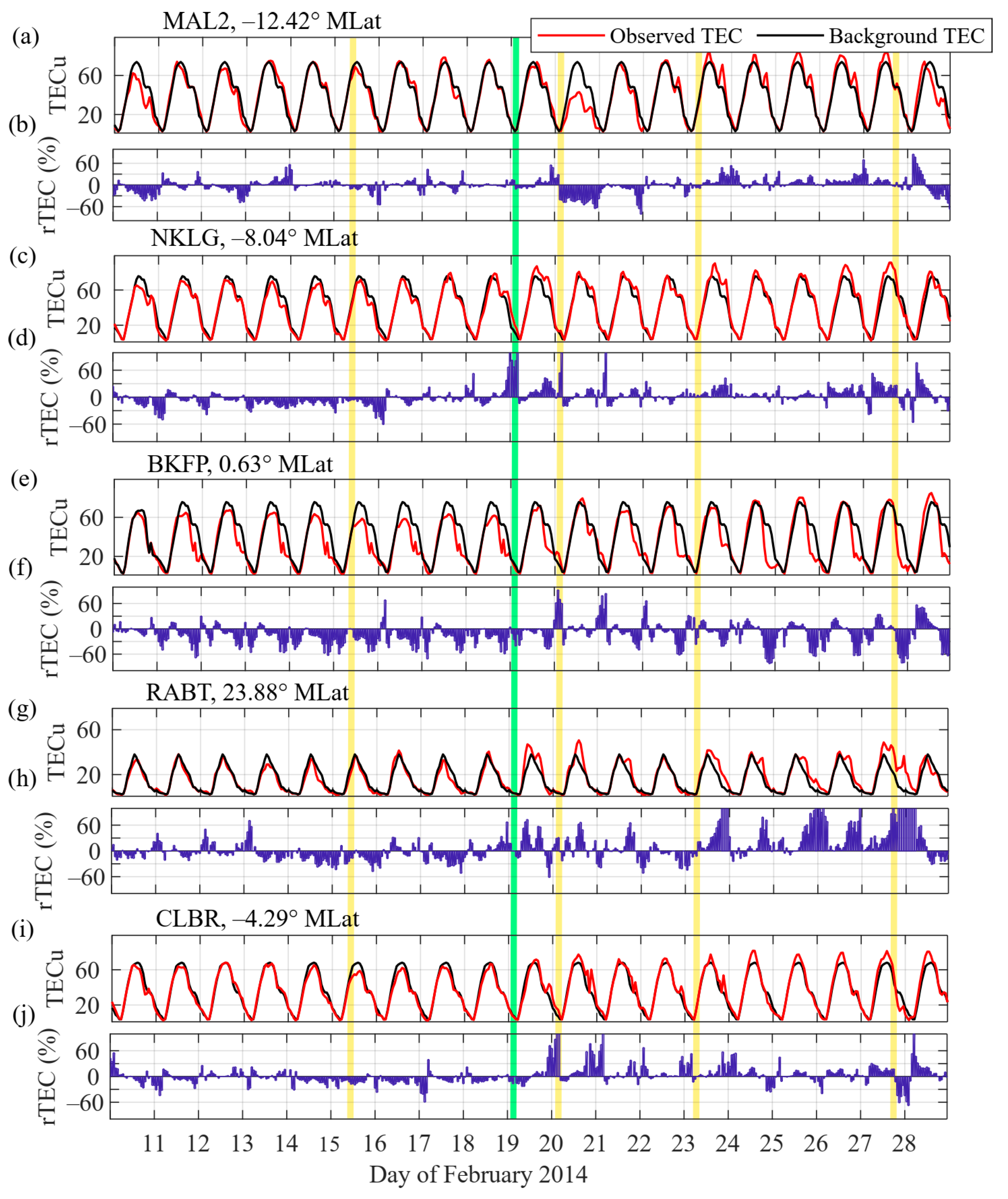
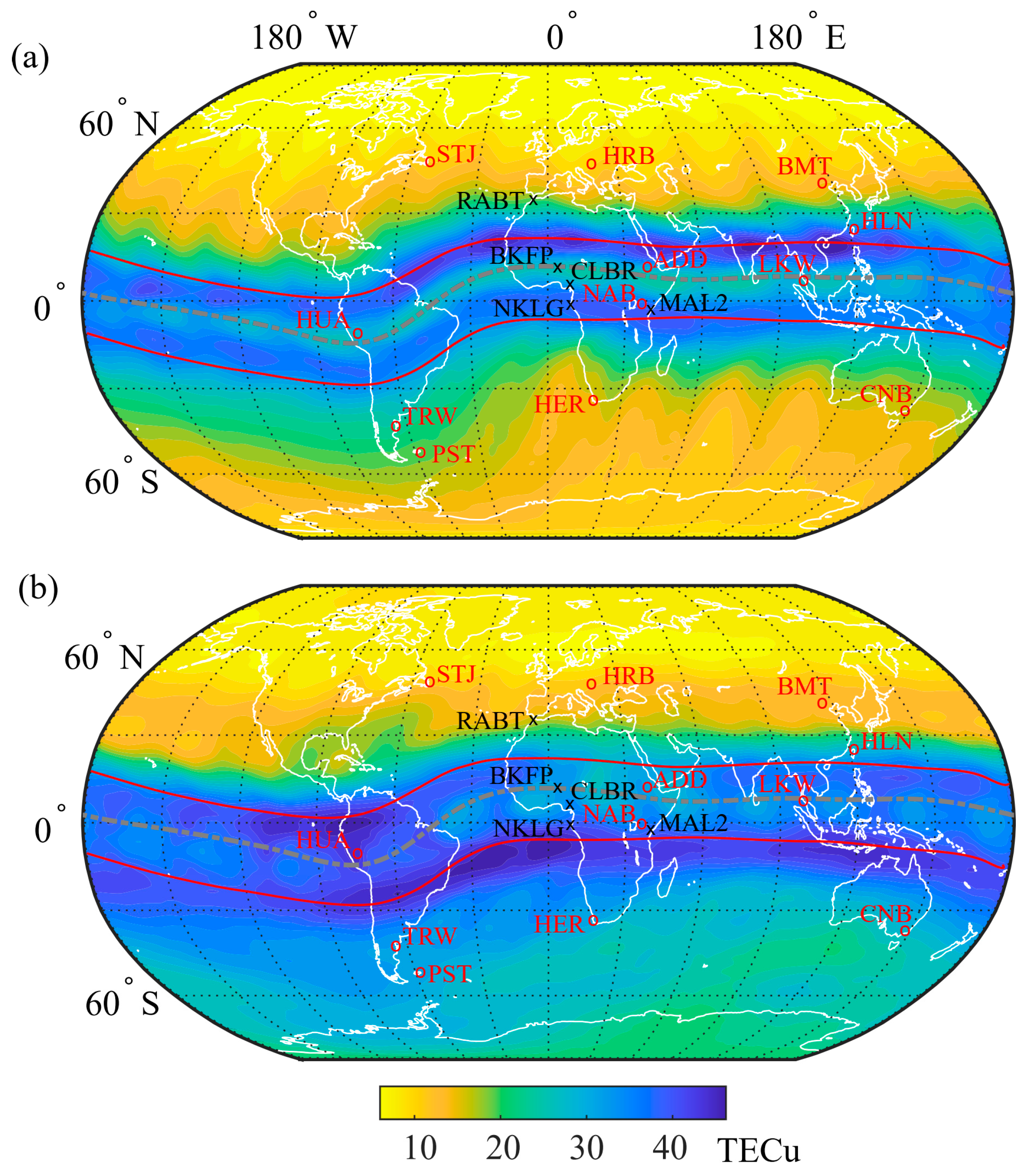
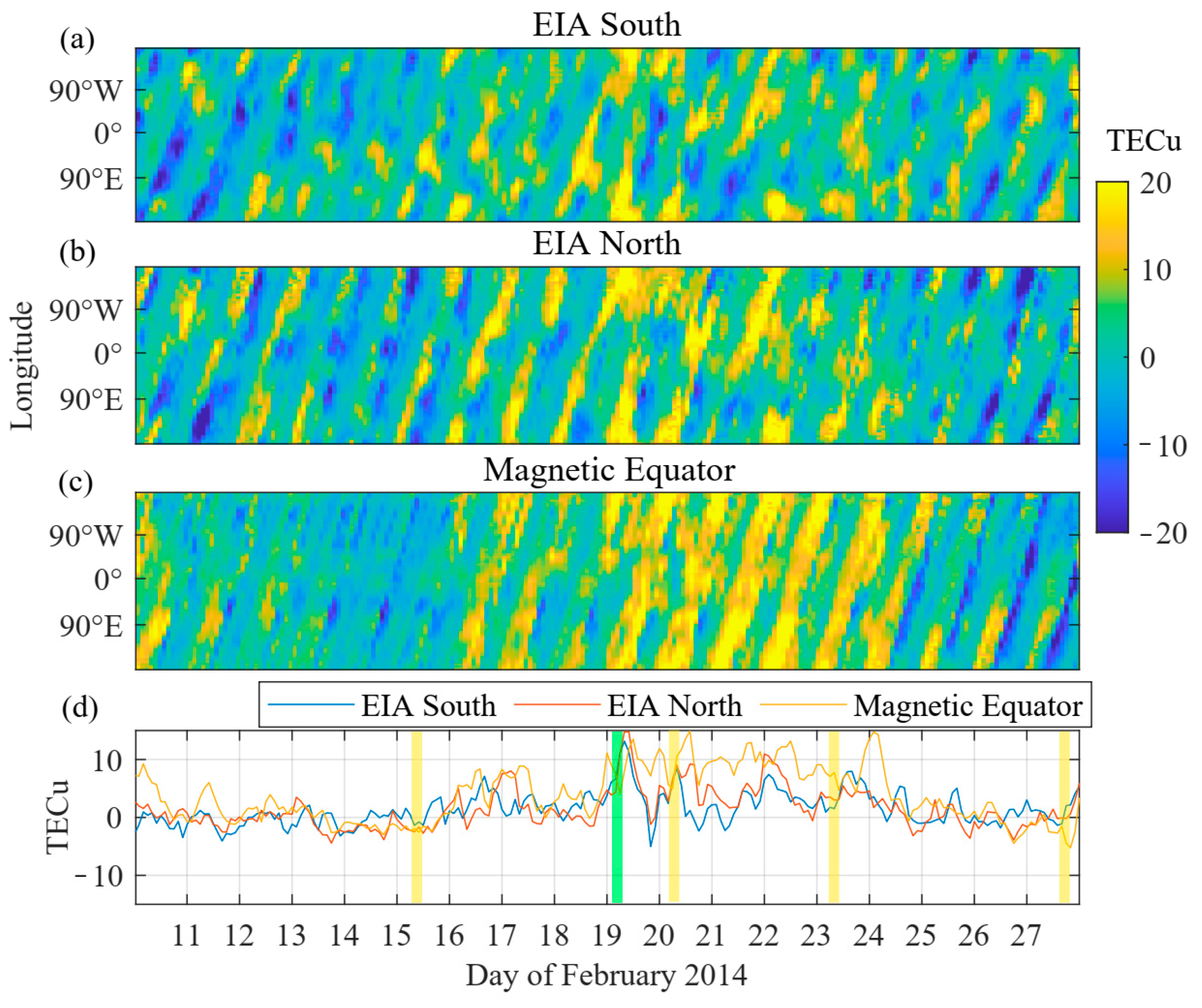
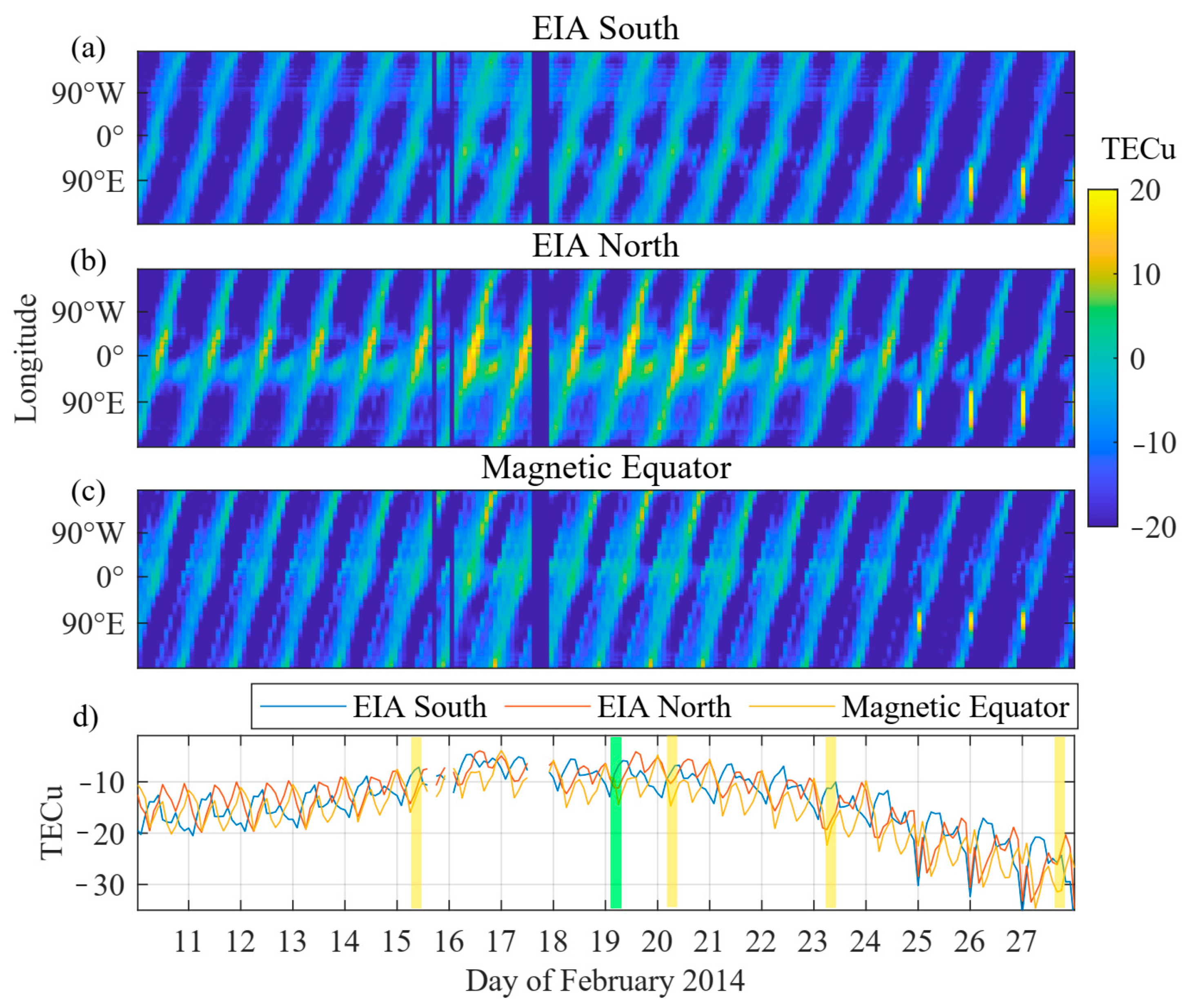
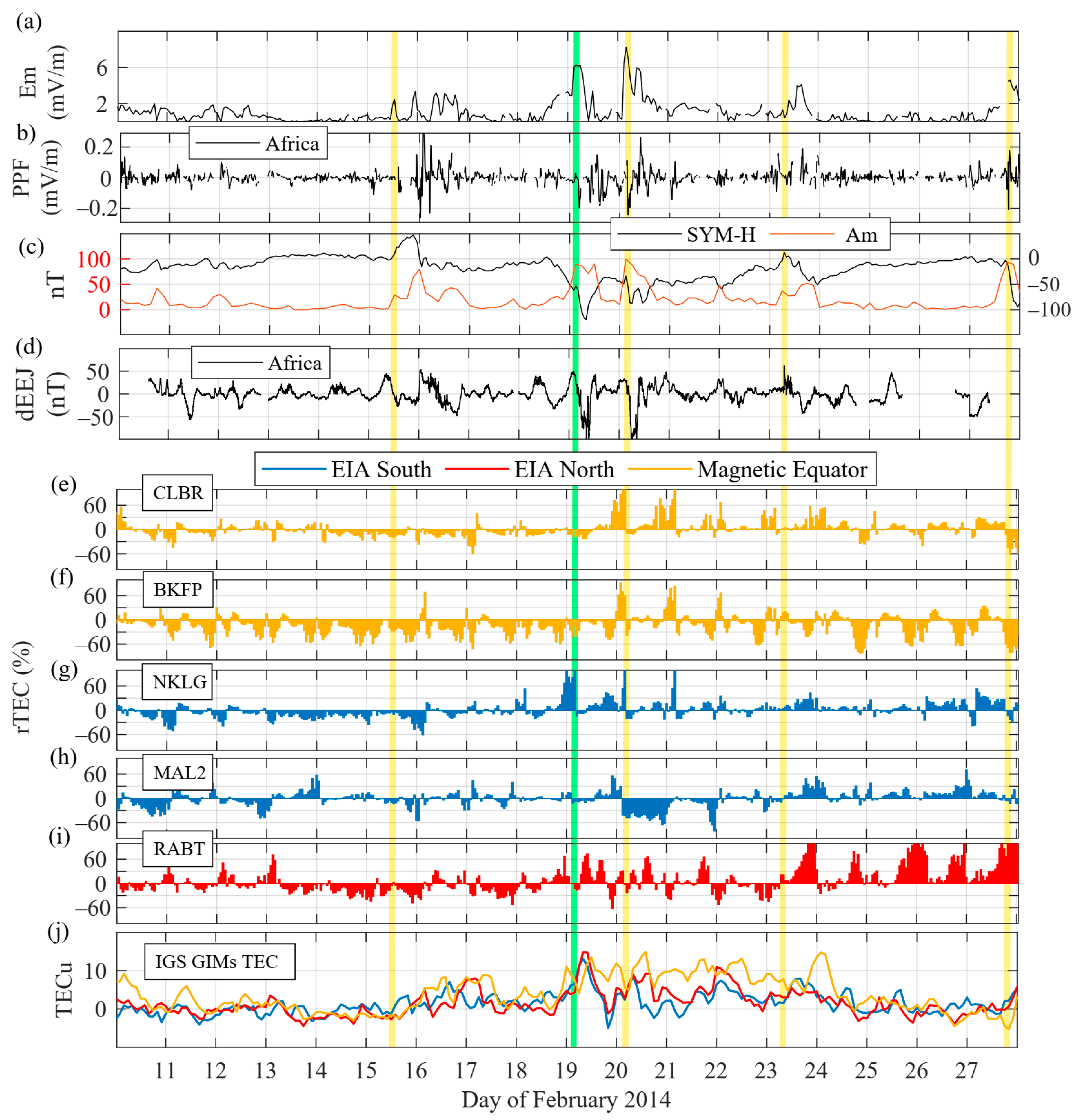
| Code | Name | Country | Latitude (°) | Longitude (°) | MLat (°) |
|---|---|---|---|---|---|
| NKLG | N’Koltang | Gabon | 0.35 | 9.67 | −8.04 |
| MAL2 | Malindi | Kenya | −2.99 | 40.19 | −12.42 |
| CLBR | Calabar | Nigeria | 4.95 | 8.35 | −4.29 |
| RABT | Rabat | Morocco | 33.99 | 353.14 | 23.88 |
| BKFP | Kebbi | Nigeria | 12.46 | 4.22 | 0.63 |
| Code | Name | Country | Latitude (°) | Longitude (°) | MLat (°) |
|---|---|---|---|---|---|
| HUA | Huancayo | Peru | −12.07 | −75.22 | −1.80 |
| TRW | Trelew | Argentina | −43.25 | −65.30 | −33.05 |
| AEE | Addis Ababa | Ethiopia | 9.04 | 38.77 | 0.18 |
| NAB | Nairobi | Kenya | −1.16 | 36.48 | −10.65 |
| LKW | Langkawi | Malaysia | 6.30 | 99.78 | −2.32 |
| HLN | Hualien | Taiwan | 23.9 | 121 | 16.86 |
| Code | Name | Country | Latitude (°) | Longitude (°) | MLat (°) |
|---|---|---|---|---|---|
| STJ | St John | Canada | 47.60 | −52.68 | 53.59 |
| PST | Port Stanly | Falkland Islands | −51.70 | −57.89 | −38.12 |
| HRB | Hurbanovo | Slovakia | 47.87 | 18.19 | −43.02 |
| HER | Hermanus | South Africa | −34.43 | 19.23 | −41.90 |
| BMT | Beijing Ming Tombs | China | 40.3 | 116.2 | 34.16 |
| CNB | Canberra | Australia | −35.32 | 149.36 | −45.24 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Calabia, A.; Anoruo, C.; Shah, M.; Amory-Mazaudier, C.; Yasyukevich, Y.; Owolabi, C.; Jin, S. Low-Latitude Ionospheric Responses and Coupling to the February 2014 Multiphase Geomagnetic Storm from GNSS, Magnetometers, and Space Weather Data. Atmosphere 2022, 13, 518. https://doi.org/10.3390/atmos13040518
Calabia A, Anoruo C, Shah M, Amory-Mazaudier C, Yasyukevich Y, Owolabi C, Jin S. Low-Latitude Ionospheric Responses and Coupling to the February 2014 Multiphase Geomagnetic Storm from GNSS, Magnetometers, and Space Weather Data. Atmosphere. 2022; 13(4):518. https://doi.org/10.3390/atmos13040518
Chicago/Turabian StyleCalabia, Andres, Chukwuma Anoruo, Munawar Shah, Christine Amory-Mazaudier, Yury Yasyukevich, Charles Owolabi, and Shuanggen Jin. 2022. "Low-Latitude Ionospheric Responses and Coupling to the February 2014 Multiphase Geomagnetic Storm from GNSS, Magnetometers, and Space Weather Data" Atmosphere 13, no. 4: 518. https://doi.org/10.3390/atmos13040518
APA StyleCalabia, A., Anoruo, C., Shah, M., Amory-Mazaudier, C., Yasyukevich, Y., Owolabi, C., & Jin, S. (2022). Low-Latitude Ionospheric Responses and Coupling to the February 2014 Multiphase Geomagnetic Storm from GNSS, Magnetometers, and Space Weather Data. Atmosphere, 13(4), 518. https://doi.org/10.3390/atmos13040518











