Australian Lidar Measurements of Aerosol Layers Associated with the 2015 Calbuco Eruption
Abstract
1. Introduction
2. Experiments
2.1. Buckland Park Rayleigh/Mie/Raman Lidar
2.2. Kingston Depolarisation Lidar
2.3. Scattering Ratio Profiles
2.4. Depolarisation Measurements
3. Results
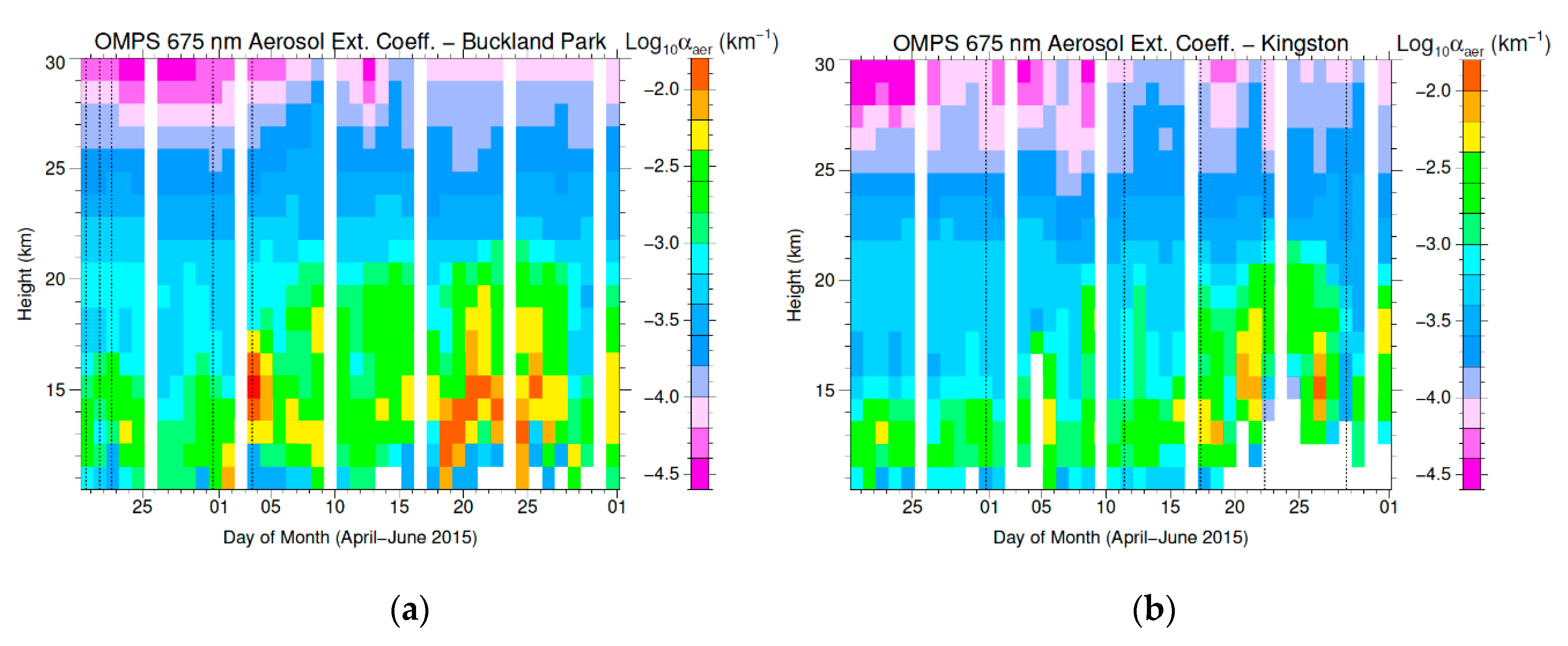
3.1. Buckland Park Scattering Ratio
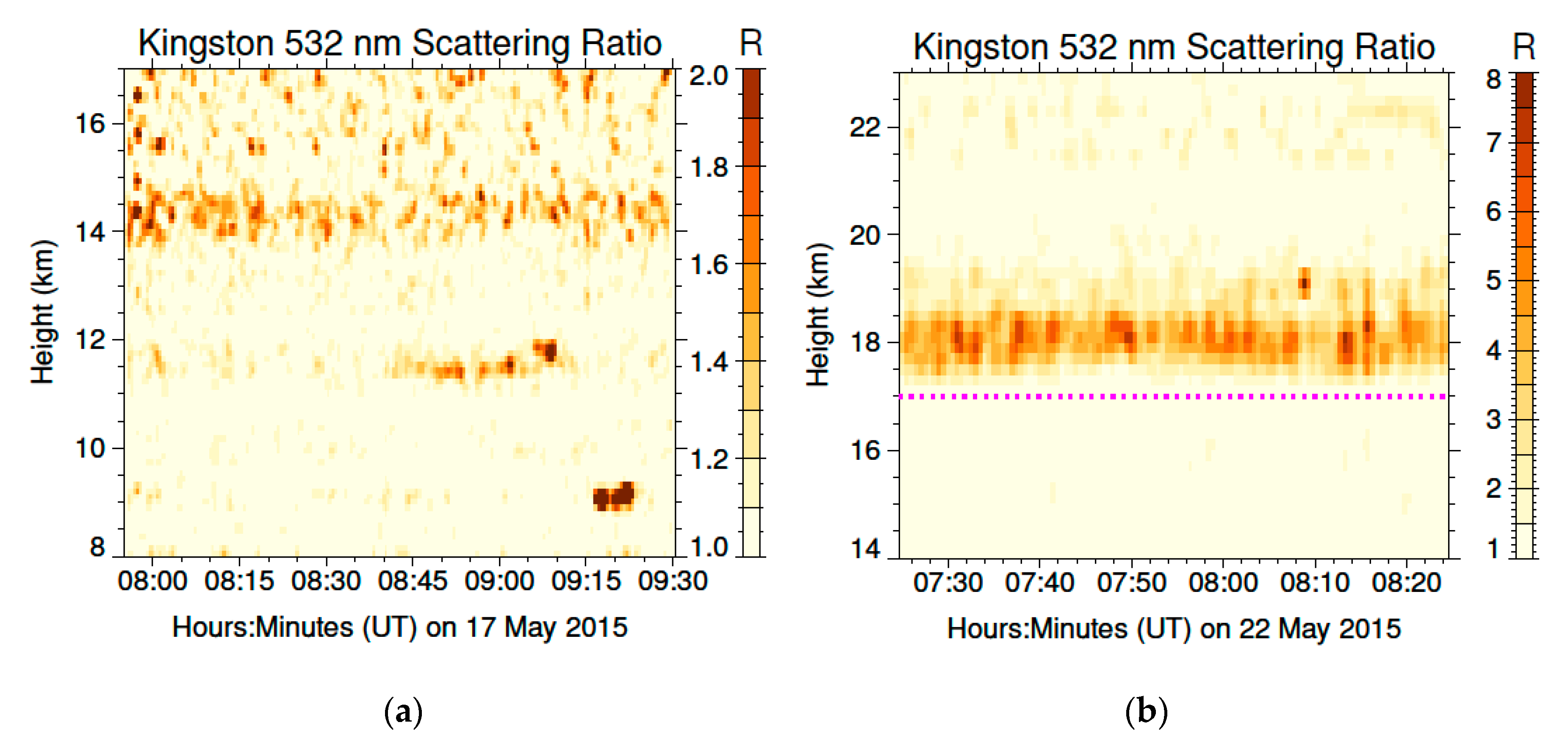
3.2. Kingston Scattering Ratio
3.3. Optical Depth and Lidar Ratio
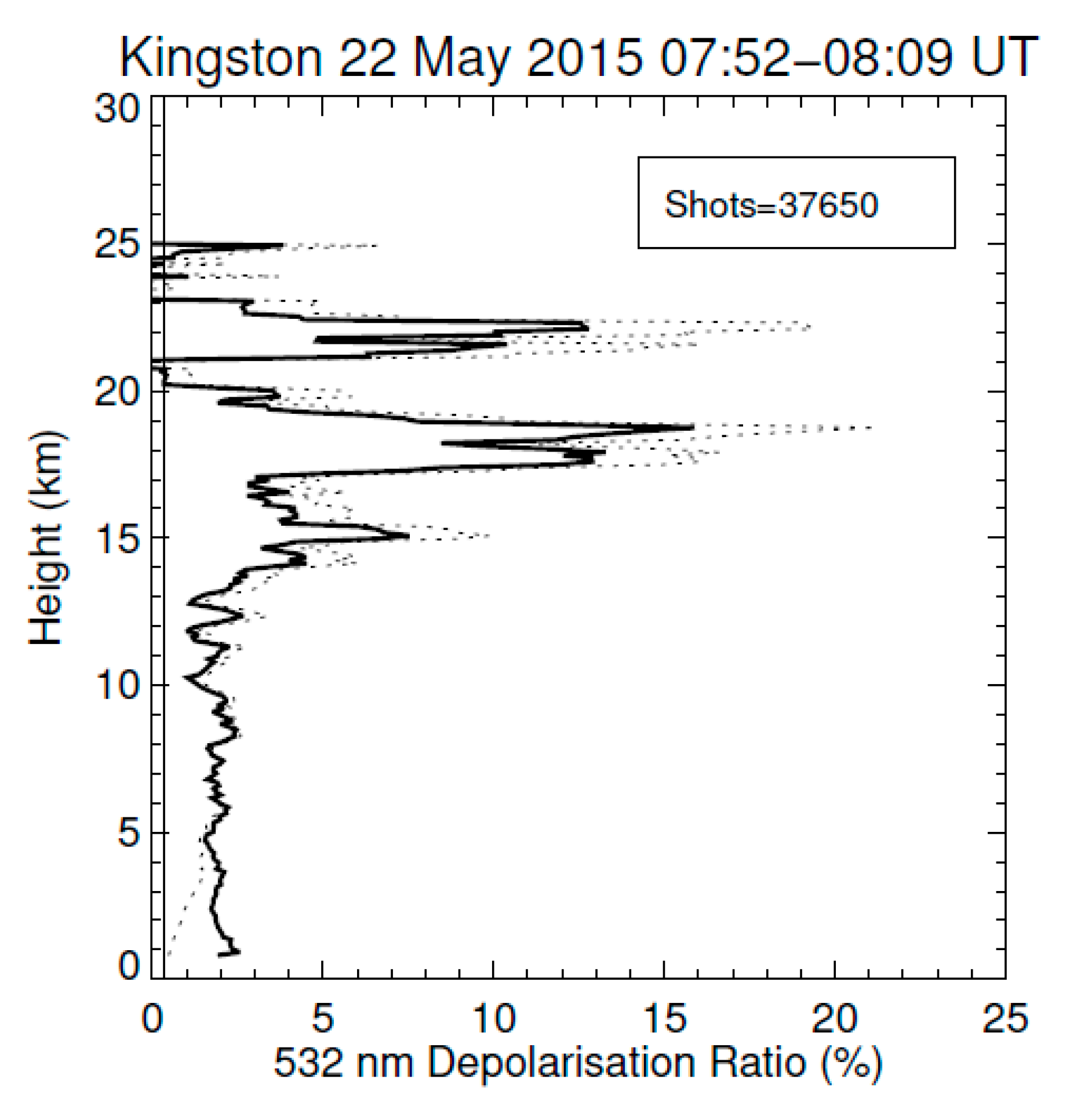
3.4. Depolarisation Analysis
4. Discussion
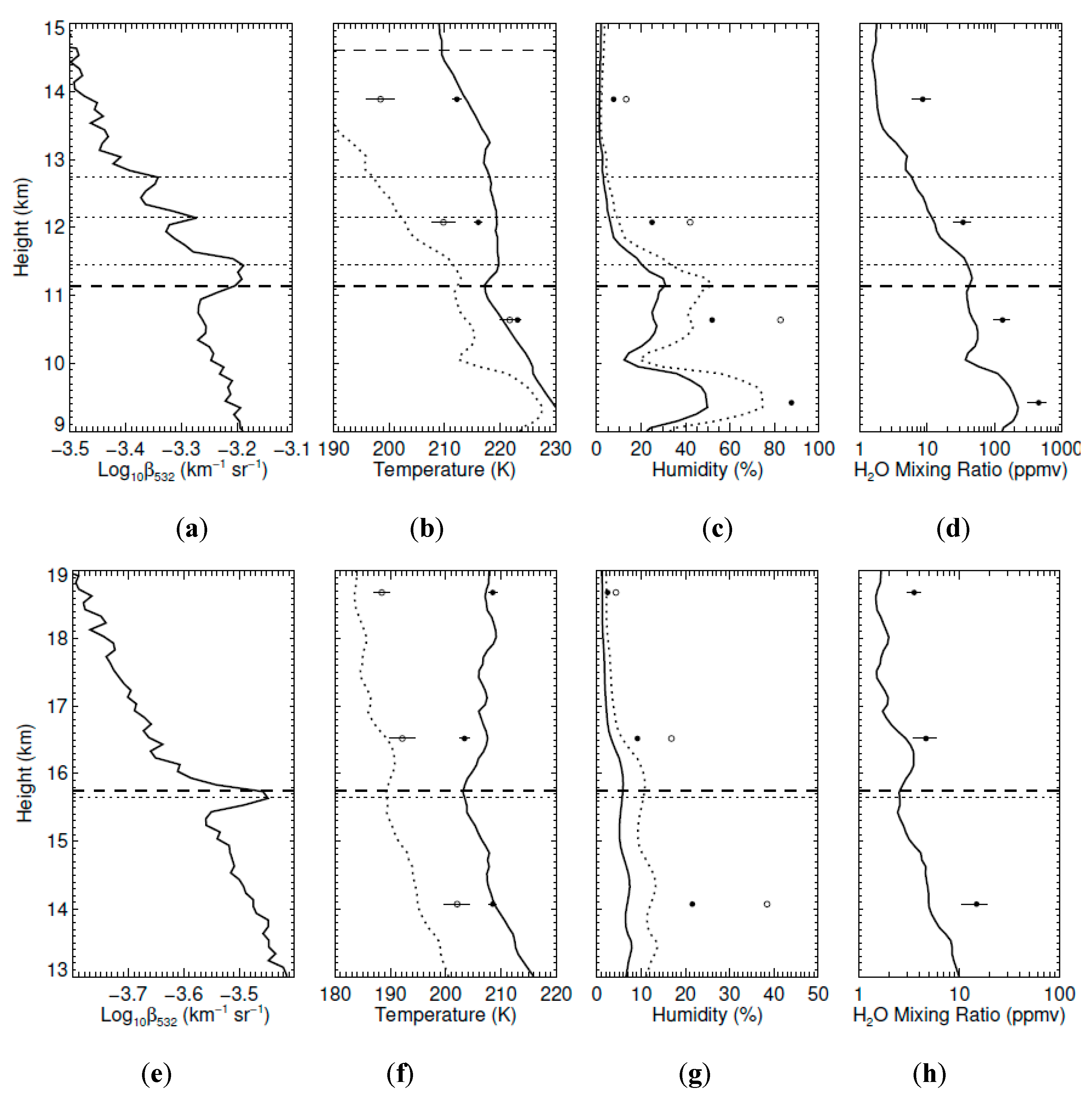
4.1. Synergy of Lidar and Radiosonde Measurements
4.2. Comparison with Other Volcanic Aerosol Measurements
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kremser, S.; Thomason, L.W.; Hobe, M.; Hermann, M.; Deshler, T.; Timmreck, C.; Toohey, M.; Stenke, A.; Schwarz, J.P.; Weigel, R.; et al. Stratospheric aerosol—Observations, processes, and impact on climate. Rev. Geophys. 2016, 54, 278–335. [Google Scholar] [CrossRef]
- Sofiev, M.; Sofieva, V.; Elperin, T.; Kleeorin, N.; Rogachevskii, I.; Zilitinkevich, S.S. Turbulent diffusion and turbulent thermal diffusion of aerosols in stratified atmospheric flows. J. Geophys. Res. 2009, 114, D18209. [Google Scholar] [CrossRef]
- Carazzo, G.; Jellinek, A.M. Particle sedimentation and diffusive convection in volcanic ash-clouds. J. Geophys. Res. Solid Earth 2013, 118, 1420–1437. [Google Scholar] [CrossRef]
- Romero, J.E.; Morgavi, D.; Arzilli, F.; Daga, R.; Caselli, A.; Reckziegel, F.; Viramonte, J.; Díaz-Alvarado, J.; Polacci, M.; Burton, M.; et al. Eruption dynamics of the 22–23 April 2015 Calbuco Volcano (Southern Chile): Analyses of tephra fall deposits. J. Volcanol. Geotherm. Res. 2016, 317, 15–29. [Google Scholar] [CrossRef]
- Reckziegel, F.; Bustos, E.; Mingari, L.; Báez, W.; Villarosa, G.; Folch, A.; Collini, E.; Viramonte, J.; Romero, J.; Osores, S. Forecasting volcanic ash dispersal and coeval resuspension during the April–May 2015 Calbuco eruption. J. Volcanol. Geotherm. Res. 2013, 321, 44–57. [Google Scholar] [CrossRef]
- Van Eaton, A.R.; Amigo, Á.; Bertin, D.; Mastin, L.G.; Giacosa, R.E.; González, J.; Valderrama, O.; Fontijn, K.; Behnke, A.A. Lightning and plume behavior reveal evolving hazards during the April 2015 eruption of Calbuco volcano, Chile. Geophys. Res. Lett. 2016, 43, 3563–3571. [Google Scholar] [CrossRef]
- Vidal, L.; Nesbitt, S.W.; Salio, P.; Farias, C.; Nicora, M.G.; Osores, M.S.; Mereu, L.; Marzano, F.S. C-band Dual-Polarization Radar Observations of a Massive Volcanic Eruption in South America. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2017, 10, 960–974. [Google Scholar] [CrossRef]
- Bègue, N.; Vignelles, D.; Berthet, G.; Portafaix, T.; Payen, G.; Jégou, F.; Benchérif, H.; Jumelet, J.; Vernier, J.-P.; Lurton, T.; et al. Long-range transport of stratospheric aerosols in the Southern Hemisphere following the 2015 Calbuco eruption. Atmos. Chem. Phys. 2017, 17, 15019–15036. [Google Scholar] [CrossRef]
- Carn, S.; Clarisse, L.; Prata, A.J. Multi-decadal satellite measurements of global volcanic degassing. J. Volcanol. Geotherm. Res. 2016, 311, 99–134. [Google Scholar] [CrossRef]
- Solomon, S.; Ivy, D.J.; Kinnison, D.; Mills, M.J.; Neely, R.R.; Schmidt, A. Emergence of healing in the Antarctic ozone layer. Science 2016, 353, 269–274. [Google Scholar] [CrossRef]
- Ivy, D.J.; Solomon, S.; Kinnison, D.; Mills, M.J.; Schmidt, A.; Neely, R.R. The influence of the Calbuco eruption on the 2015 Antarctic ozone hole in a fully coupled chemistry-climate model. Geophys. Res. Lett. 2017, 44, 2556–2561. [Google Scholar] [CrossRef]
- Stone, K.A.; Solomon, S.; Kinnison, D.E.; Pitts, M.C.; Poole, L.R.; Mills, M.J.; Schmidt, A.; Neely, R.R., III; Ivy, D.; Schwartz, M.J.; et al. Observing the impact of Calbuco volcanic aerosols on south polar ozone depletion in 2015. J. Geophys. Res. 2017, 122, 811–862. [Google Scholar] [CrossRef]
- Zhu, Y.; Toon, O.B.; Kinnison, D.; Harvey, L.V.; Mills, M.J.; Bardeen, C.G.; Pitts, M.; Bègue, N.; Renard, J.-B.; Berthet, G.; et al. Stratospheric aerosols, polar stratospheric clouds, and polar ozone depletion after the Mount Calbuco eruption in 2015. J. Geophys. Res. 2018, 123, 12–308. [Google Scholar] [CrossRef]
- Nakamae, K.; Uchino, O.; Morino, I.; Liley, B.; Sakai, T.; Nagai, T.; Yokota, T. Lidar observation of the 2011 Puyehue-Cordón Caulle volcanic aerosols at Lauder, New Zealand. Atmos. Chem. Phys. 2014, 14, 12099–12108. [Google Scholar] [CrossRef]
- Vernier, J.; Fairlie, T.D.; Murray, J.J.; Tupper, A.; Trepte, C.; Winker, D.; Pelon, J.; Garnier, A.; Jumelet, J.; Pavolonis, M.; et al. An advanced system to monitor the 3D structure of diffuse volcanic ash clouds. J. Appl. Meteorol. Climatol. 2013, 52, 2125–2138. [Google Scholar] [CrossRef]
- Bignami, C.; Corradini, S.; Merucci, L.; de Michele, M.; Raucoules, D.; de Astis, G.; Stramondo, S.; Piedra, J. Multisensor satellite monitoring of the 2011 Puyehue-Cordon Caulle eruption. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2014, 7, 2786–2796. [Google Scholar] [CrossRef]
- Reid, I.M.; Twigger, L.V.; Ottaway, D.O.; Lautenbach, J.; Klekociuk, A.R.; MacKinnon, A.D.; Spargo, A.J.; Yi, W.; Alexander, S.P.; Munch, J.; et al. High resolution observations of mesospheric dynamics using lidar and radar at Buckland Park, South Australia (34.6° S, 138.5° E). Atmosphere 2020. under review. [Google Scholar]
- Huang, Y.; Franklin, C.N.; Siems, S.T.; Manton, M.J.; Chubb, T.; Lock, A.; Alexander, S.; Klekociuk, A. Evaluation of boundary-layer cloud forecasts over the Southern Ocean in a limited-area numerical weather prediction system using in situ, space-borne and ground-based observations. Q. J. R. Meteorol. Soc. 2015, 141, 2259–2276. [Google Scholar] [CrossRef]
- Seldomridge, N.L.; Shaw, J.A.; Repasky, K.S. Dual-polarization lidar using a liquid crystal variable retarder. Opt. Eng. 2006, 45, 106202. [Google Scholar] [CrossRef]
- Atmospheric Infrared Sounder Version 6 Level 2 Data. Available online: https://docserver.gesdisc.eosdis.nasa.gov/repository/Mission/AIRS/3.3_ScienceDataProductDocumentation/3.3.4_ProductGenerationAlgorithms/V6_L2_Product_User_Guide.pdf (accessed on 23 October 2019).
- Klett, J.D. Lidar inversion with variable backscatter/extinction ratios. Appl. Opt. 1985, 24, 1638–1643. [Google Scholar] [CrossRef]
- Fernald, F.G. Analysis of atmospheric lidar observations: Some comments. Appl. Opt. 1984, 23, 652–653. [Google Scholar] [CrossRef] [PubMed]
- Sasano, Y.; Browell, E.V.; Ismail, S. Error caused by using a constant extinction/backscattering ratio in the lidar solution. Appl. Opt. 1995, 24, 3929–3932. [Google Scholar] [CrossRef] [PubMed]
- Ackermann, J. Two-wavelength lidar inversion algorithm for a two-component atmosphere with variable 865 extinction-to-backscatter ratios. Appl. Opt. 1984, 37, 3164–3171. [Google Scholar] [CrossRef] [PubMed]
- Kaskaoutis, D.G.; Kambezidis, H.D. Investigation into the wavelength dependence of the aerosol optical depth in the Athens area. Q. J. R. Meteorol. Soc. 2006, 132, 2217–2234. [Google Scholar] [CrossRef]
- Mortier, A.; Goloub, P.; Podvin, T.; Deroo, C.; Chaikovsky, A.; Ajtai, N.; Blarel, L.; Tanre, D.; Derimian, Y. Detection and characterization of volcanic ash plumes over Lille during the Eyjafjallajökull eruption. Atmos. Chem. Phys. 2013, 13, 3705–3720. [Google Scholar] [CrossRef]
- Kokkalis, P.; Papayannis, A.; Amiridis, V.; Mamouri, R.E.; Veselovskii, I.; Kolgotin, A.; Tsaknakis, G.; Kristiansen, N.I.; Stohl, A.; Mona, L. Optical, microphysical, mass and geometrical properties of aged volcanic particles observed over Athens, Greece, during the Eyjafjallajökull eruption in April 2010 through synergy of Raman lidar and sunphotometer measurements. Atmos. Chem. Phys. 2013, 13, 9303–9320. [Google Scholar] [CrossRef]
- Malinina, E.; Rozanov, A.; Rieger, L.; Bourassa, A.; Bovensmann, H.; Burrows, J.P.; Degenstein, D. Stratospheric aerosol characteristics from space-borne observations: Extinction coefficient and Ångström exponent. Atmos. Meas. Tech. 2019, 12, 3485–3502. [Google Scholar] [CrossRef]
- Argall, P.S.; Jacka, F. High-pulse-repetition-frequency lidar system using a single telescope for transmission and reception. Appl. Opt. 1996, 35, 2619–2629. [Google Scholar] [CrossRef]
- Donovan, D.P.; Whiteway, J.A.; Carswell, A.I. Correction for nonlinear photon-counting effects in lidar systems. Appl. Opt. 1993, 32, 6742–6753. [Google Scholar] [CrossRef]
- Belegante, L.; Bravo-Aranda, J.A.; Freudenthaler, V.; Nicolae, D.; Nemuc, A.; Ene, D.; Alados-Arboledas, L.; Amodeo, A.; Pappalardo, G.; D’Amico, G.; et al. Experimental techniques for the calibration of lidar depolarization channels in EARLINET. Atmos. Meas. Tech. 2018, 11, 1119–1141. [Google Scholar] [CrossRef]
- Dai, G.; Wu, S.-H.; Song, X. Depolarization ratio profiles calibration and observations of aerosol and cloud in the Tibetan Plateau based on polarization Raman lidar. Remote Sens. 2018, 10, 378. [Google Scholar] [CrossRef]
- Sakai, T.; Uchino, O.; Nagai, N.; Liley, B.; Morino, I.; Fujimoto, T. Long-term variation of stratospheric aerosols observed with lidars over Tsukuba, Japan, from 1982 and Lauder, New Zealand, from 1992 to 2015. J. Geophys. Res. Atmos. 2016, 121, 10283–10293. [Google Scholar] [CrossRef]
- Prata, A.T.; Young, S.A.; Siems, S.T.; Manton, M.J. Lidar ratios of stratospheric volcanic ash and sulfate aerosols retrieved from CALIOP measurements. Atmos. Chem. Phys. 2017, 17, 8599–8618. [Google Scholar] [CrossRef]
- Nagai, T.; Liley, B.; Sakai, T.; Shibata, T.; Uchino, O. Post-Pinatubo evolution and subsequent trend of the stratospheric aerosol layer observed by mid-latitude lidars in both hemispheres. SOLA 2010, 6, 69–72. [Google Scholar] [CrossRef][Green Version]
- CALIPSO Browse Images. Available online: https://www-calipso.larc.nasa.gov/products/lidar/browse_images/std_v4_index.php (accessed on 23 October 2019).
- Stein, A.F.; Draxler, R.R.; Rolph, G.D.; Stunder, B.J.B.; Cohen, M.D.; Ngan, F. NOAA’s HYSPLIT atmospheric transport and dispersion modeling system. Bull. Am. Meteorol. Soc. 2015, 96, 2059–2077. [Google Scholar] [CrossRef]
- Dee, D.P.; Uppala, S.M.; Simmons, A.J.; Berrisford, P.; Poli, P.; Kobayashi, S.; Andrae, U.; Balmaseda, M.A.; Balsamo, G.; Bauer, P.; et al. The ERA-Interim reanalysis: Configuration and performance of the data assimilation system. Q. J. R. Meteorol. Soc. 2011, 137, 553–597. [Google Scholar] [CrossRef]
- Ramon, J.; Lledó, L.; Torralba, V.; Soret, A.; Doblas-Reyes, F.J. What global reanalysis best represents near-surface winds? Q. J. R. Meteorol. Soc. 2019, 145, 3236–3251. [Google Scholar] [CrossRef]
- HYSPLIT Online Model. Available online: https://www.ready.noaa.gov/hypub-bin/trajtype.pl?runtype=archive (accessed on 23 October 2019).
- Rolph, G.; Stein, A.; Stunder, B. Real-time Environmental Applications and Display sYstem: READY. Environ. Model. Softw. 2017, 95, 210–228. [Google Scholar] [CrossRef]
- Schoeberl, M.; Ziemke, J.; Bojkov, B.; Livesey, N.; Duncan, B.; Strahan, S.; Froidevaux, L.; Kulawik, S.; Bhartia, P.; Chandra, S.; et al. A trajectory-based estimate of the tropospheric ozone column using the residual method. J. Geophys. Res. 2007, 112, D24S49. [Google Scholar] [CrossRef]
- Australian Bureau of Meteorology Synoptic Chart Archive. Available online: http://www.bom.gov.au/cgi-bin/charts/charts.browse.pl (accessed on 23 October 2019).
- Murphy, D.J.; Alexander, S.P.; Klekociuk, A.; Love, P.T.; Vincent, R.A. Radiosonde observations of gravity waves in the lower stratosphere over Davis, Antarctica. J. Geophys. Res. Atmos. 2014, 119, 11973–11996. [Google Scholar] [CrossRef]
- Bhartia, P.K.; Torres, O.O. OMPS-NPP L2 LP Aerosol Extinction Vertical Profile Swath Daily 3slit V1.5; Goddard Earth Sciences Data and Information Services Center: Greenbelt, MD, USA, 2019. [CrossRef]
- Uchino, O.; Tokunaga, M.; Seki, K.; Maeda, M.; Naito, K.; Takahashi, K. Lidar measurement of stratospheric transmission at a wavelength of 340 nm after the eruption of El Chichon. J. Atmos. Terr. Phys. 1983, 45, 849–850. [Google Scholar]
- Young, S.A. Analysis of lidar backscatter profiles in optically thin clouds. Appl. Opt. 1995, 34, 7019–7031. [Google Scholar] [CrossRef] [PubMed]
- Kovalev, V.A.; McElroy, J.L.; Eichinger, W.E. Lidar-inversion technique for monitoring and mapping localized aerosol plumes and thin clouds. In Application of Lidar to Current Atmospheric Topics; Society of Photo-Optical Instrumentation Engineers (SPIE): Bellingham, WA, USA, 1996; Volume 2833, pp. 251–258. [Google Scholar] [CrossRef]
- Winker, D.M.; Liu, Z.; Omar, A.; Tackett, J.; Fairlie, D. CALIOP observations of the transport of ash from the Eyjafjallajökull volcano in 20 April10. J. Geophys. Res. 2012, 117, D00U15. [Google Scholar] [CrossRef]
- Eloranta, E.W. Practical model for the calculation of multiply scattered lidar returns. Appl. Opt. 1996, 37, 2464–2472. [Google Scholar] [CrossRef] [PubMed]
- Freudenthaler, V.; Esselborn, M.; Wiegner, M.; Heese, B.; Tesche, M.; Ansmann, A.; Müller, D.; Althausen, D.; Wirth, M.; Fix, A.; et al. Depolarization ratio profiling at several wavelengths in pure Saharan dust during SAMUM 2006. Tellus B. 2009, 61, 165–179. [Google Scholar] [CrossRef]
- Behrendt, A.; Nakamura, T. Calculation of the calibration constant of polarization lidar and its dependency on atmospheric temperature. Opt. Express 2010, 10, 805–817. [Google Scholar] [CrossRef]
- Chen, W.-N.; Chiang, C.-W.; Nee, J.B. Lidar ratio and depolarization ratio for cirrus clouds. Appl. Opt. 2002, 41, 6470–6476. [Google Scholar] [CrossRef]
- Sassen, K.; Benson, S. A midlatitude cirrus cloud climatology from the Facility for Atmospheric Remote Sensing. Part II: Microphysical properties derived from lidar depolarization. J. Atmos. Sci. 2001, 58, 2103–2112. [Google Scholar] [CrossRef]
- Tesche, M.; Ansmann, A.; Müller, D.; Althausen, D.; Engelmann, R.; Freudenthaler, V.; Groß, S. Vertically resolved separation of dust and smoke over Cape Verde using multiwavelength Raman and polarization lidars during Saharan Mineral Dust Experiment 2008. J. Geophys. Res. 2009, 114, D13202. [Google Scholar] [CrossRef]
- Ansmann, A.; Tesche, M.; Seifert, P.; Groß, S.; Freudenthaler, V.; Apitule, A.; Wilson, K.M.; Serikov, I.; Linné, H.; Heinold, B.; et al. Ash and fine-mode particle mass profiles from EARLINET-AERONET observations over central Europe after the eruptions of the Eyjafjallajökull volcano in 2010. J. Geophys. Res. 2011, 116, D00U02. [Google Scholar] [CrossRef]
- Teitelbaum, H.; Basdevant, C.; Moustaoui, M. Explanations for simultaneous laminae in water vapor and aerosol profiles found during the SESAME experiment. Tellus A 2000, 52, 190–202. [Google Scholar] [CrossRef][Green Version]
- Chane Ming, F.; Vignelles, D.; Jegou, F.; Berthet, G.; Renard, J.-B.; Gheusi, F.; Kuleshov, Y. Gravity-wave effects on tracer gases and stratospheric aerosol concentrations during the 2013 ChArMEx campaign. Atmos. Chem. Phys. 2016, 16, 8023–8042. [Google Scholar] [CrossRef]
- Hu, Y.X.; Vaughan, M.; Liu, Z.Y.; Lin, B.; Yang, P.; Flittner, D.; Hunt, B.; Kuehn, R.; Huang, J.P.; Wu, D.; et al. The depolarization–attenuated backscatter relation: CALIPSO lidar measurements vs. theory. Opt. Express 2007, 15, 5327–5332. [Google Scholar] [CrossRef] [PubMed]
- CALIPSO Algorithm Theoretical Basis. Available online: https://www-calipso.larc.nasa.gov/resources/calipso_users_guide/data_summaries/profile_data.php#heading09 (accessed on 23 October 2019).
- CALIOP 532 nm Unattenuated Backscatter Coefficient Browse Images 22 May 2015 1600 UT. Available online: https://www-calipso.larc.nasa.gov/data/BROWSE/production/V4-10/2015-05-22/2015-05-22_15-32-54_V4.10_3_1.png (accessed on 23 October 2019).
- WMO (World Meteorological Organisation). Meteorology—A three dimensional science: Second session of the Commission for Aerology. WMO Bull. 1957, 4, 134–138. [Google Scholar]
- Miloshevich, L.M.; Vomel, H.; Whiteman, D.N.; Leblanc, T. Accuracy assessment and correction of VaisalaRS92 radiosonde water vapor measurements. J. Geophys. Res. 2009, 114, D11305. [Google Scholar] [CrossRef]
- Korolev, A.; Isaac, G.A. Relative humidity in liquid, mixed-phase, and ice clouds. J. Atmos. Sci. 2006, 63, 2865–2880. [Google Scholar] [CrossRef]
- Haag, W.; Kärcher, B. The impact of aerosols and gravity waves on cirrus clouds at midlatitudes. J. Geophys. Res. 2004, 109, D12202. [Google Scholar] [CrossRef]
- Sassen, K. Evidence for liquid-phase cirrus cloud formation from volcanic aerosols: Climatic implications. Science 1992, 257, 516–519. [Google Scholar] [CrossRef]
- Campbell, J.R.; Welton, E.J.; Krotkov, N.A.; Yang, K.; Stewart, S.A.; From, M.D. Likely seeding of cirrus clouds by stratospheric Kasatochi volcanic aerosol particles near a mid-latitude tropopause fold. Atmos. Environ. 2012, 46. [Google Scholar] [CrossRef]
- Shibata, T.; Hayashi, M.; Naganuma, A.; Hara, N.; Hara, K.; Hasebe, F.; Shimizu, K.; Komala, N.; Inai, Y.; Vömel, H.; et al. Cirrus cloud appearance in a volcanic aerosol layer around the tropical cold point tropopause over Biak, Indonesia, in January 2011. J. Geophys. Res. 2012, 117, D11209. [Google Scholar] [CrossRef]
- Friberg, J.; Martinsson, B.G.; Sporre, M.K.; Andersson, S.M.; Brenninkmeijer, C.A.M.; Hermann, M.; van Velthoven, P.F.J.; Zahn, A. Influence of volcanic eruptions on midlatitude upper tropospheric aerosol and consequences for cirrus clouds. Earth Space Sci. 2015, 2, 285–300. [Google Scholar] [CrossRef]
- Rolf, C.; Krämer, M.; Schiller, C.; Hildebrandt, M.; Riese, M. Lidar observation and model simulation of a volcanic-ash-induced cirrus cloud during the Eyjafjallajökull eruption. Atmos. Chem. Phys. 2012, 12, 10281–10294. [Google Scholar] [CrossRef]
- Seifert, P.; Ansmann, A.; Groß, S.; Freudenthaler, V.; Heinold, B.; Hiebsc, A.; Mattis, I.; Schmidt, J.; Schnell, F.; Tesche, M.; et al. Ice formation in ash-influenced clouds after the eruption of the Eyjafjallajökull volcano in April 2010. J. Geophys. Res. 2011, 116, D00U04. [Google Scholar] [CrossRef]
- Ansmann, A.; Tesche, M.; Althausen, D.; Mueller, D.; Seifert, P.; Freudenthaler, V.; Heese, B.; Wiegner, M.; Pisani, G.; Knippertz, P.; et al. Influence of Saharan dust on cloud glaciation in southern Morocco during the Saharan Mineral Dust Experiment. J. Geophys. Res. Atmos. 2008, 113, D04210. [Google Scholar] [CrossRef]
- Steinke, I.; Mohler, O.; Kiselev, A.; Niemand, M.; Saathoff, H.; Schnaiter, M.; Skrotzki, J.; Hoose, C.; Leisner, T. Ice nucleation properties of fine ash particles from the Eyjafjallajokull eruption in April 2010. Atmos. Chem. Phys. 2011, 11, 12945–12958. [Google Scholar] [CrossRef]
- DeMott, P.J.; Cziczo, D.J.; Prenni, A.J.; Murphy, D.M.; Kreidenweis, S.M.; Thomson, D.S.; Borys, R.; Rogers, D.C. Measurements of the concentration and composition of nuclei for cirrus formation. Proc. Natl. Acad. Sci. USA 2003, 100, 14655–14660. [Google Scholar] [CrossRef] [PubMed]
- Heymsfield, A.; Winker, D.; Avery, M.; Vaughan, M.; Diskin, G.; Deng, M.; Mitev, V.; Matthey, R. Relationships between Ice Water Content and Volume Extinction Coefficient from in situ observations for temperatures from 0° to −86 °C: Implications for spaceborne lidar retrievals. J. Appl. Meteorol. Climatol. 2014, 53, 479–505. [Google Scholar] [CrossRef]
- Schumann, U.; Mayer, B.; Gierens, K.; Unterstrasser, S.; Jessberger, P.; Petzold, A.; Voigt, C.; Gayet, J. Effective Radius of ice particles in cirrus and contrails. J. Atmos. Sci. 2011, 68, 300–321. [Google Scholar] [CrossRef]
- Ansmann, A.; Tesche, M.; Groß, S.; Freudenthaler, V.; Seifert, P.; Hiebsch, A.; Schmidt, J.; Wandinger, U.; Mattis, I.; Müller, D.; et al. The 16 April 2010 major volcanic ash plume over central Europe: EARLINET lidar and AERONET photometer observations at Leipzig and Munich, Germany. Geophys. Res. Lett. 2010, 37, L13810. [Google Scholar] [CrossRef]
- Gasteiger, J.; Groß, S.; Freudenthaler, V.; Wiegner, M. Volcanic ash from Iceland over Munich: Mass concentration retrieved from ground-based remote sensing measurements. Atmos. Chem. Phys. 2011, 11, 2209–2223. [Google Scholar] [CrossRef]
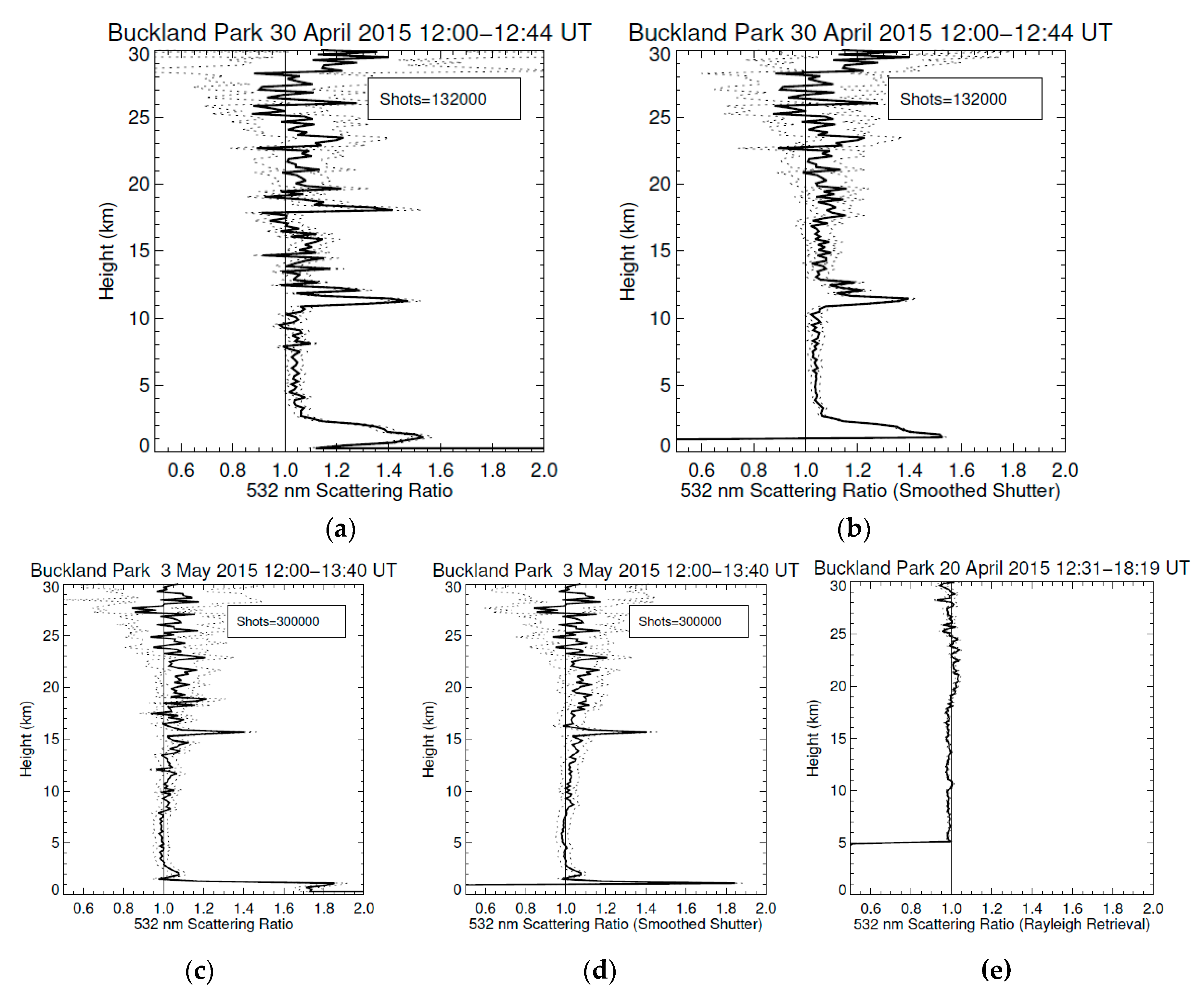
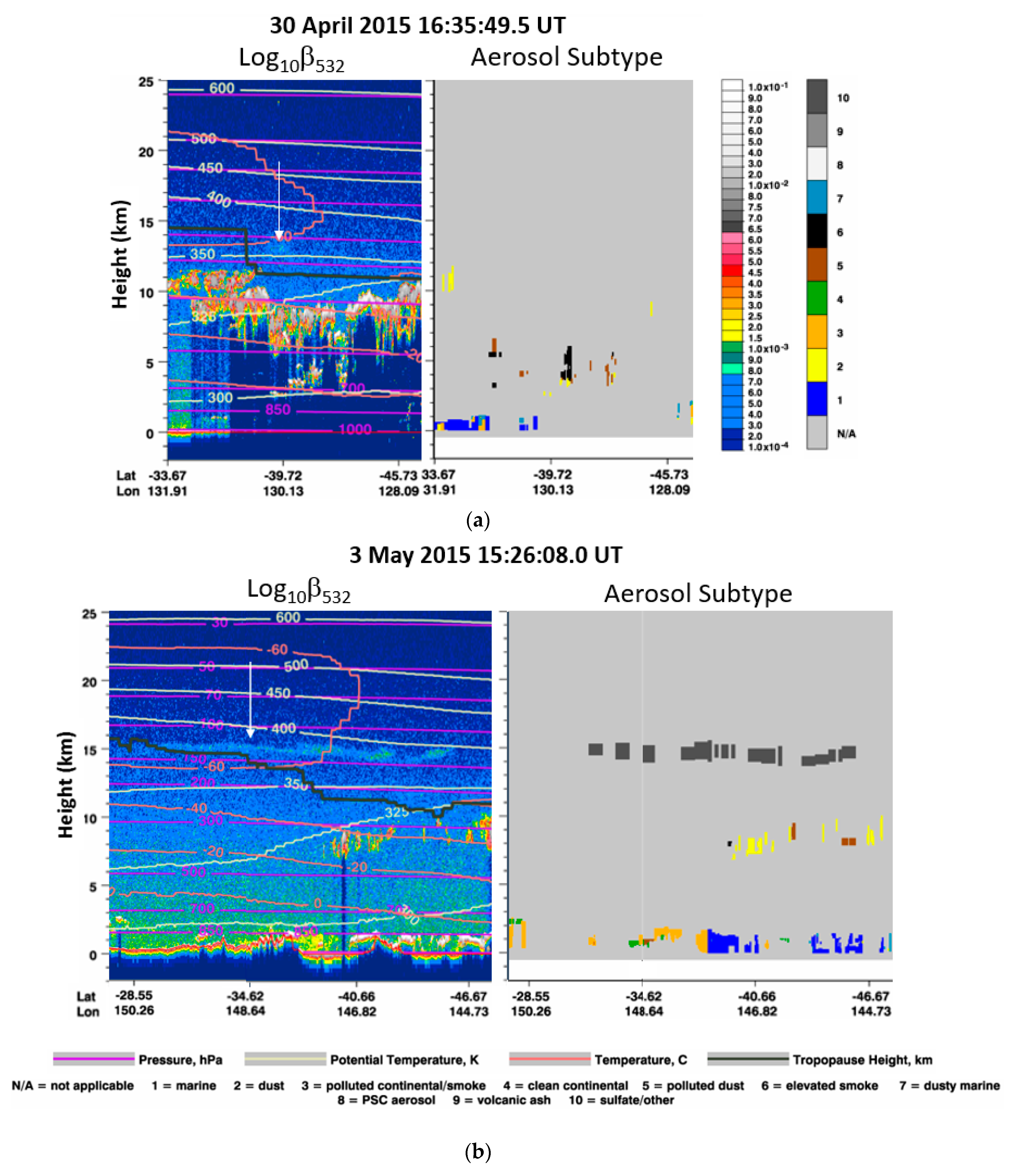

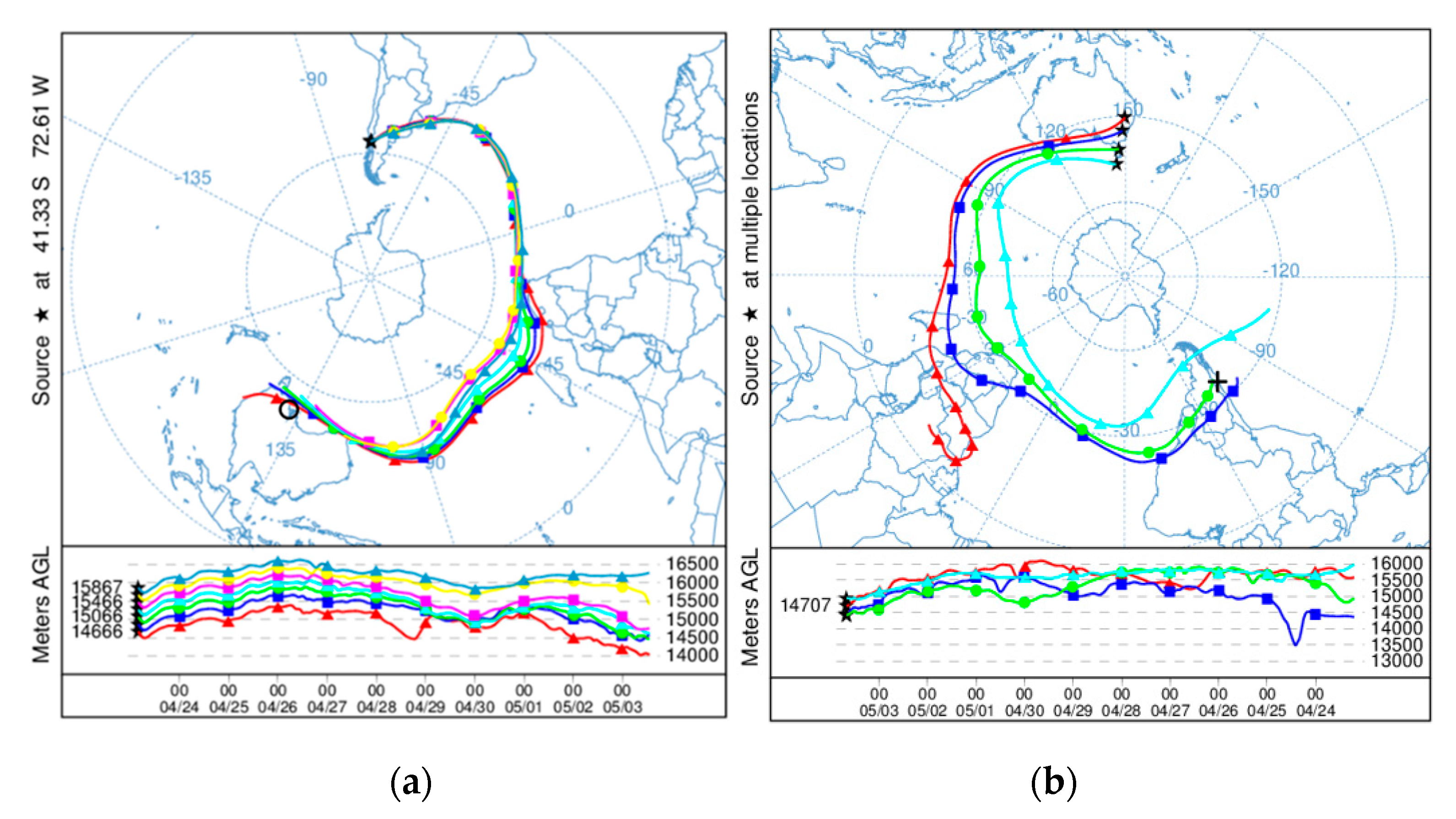
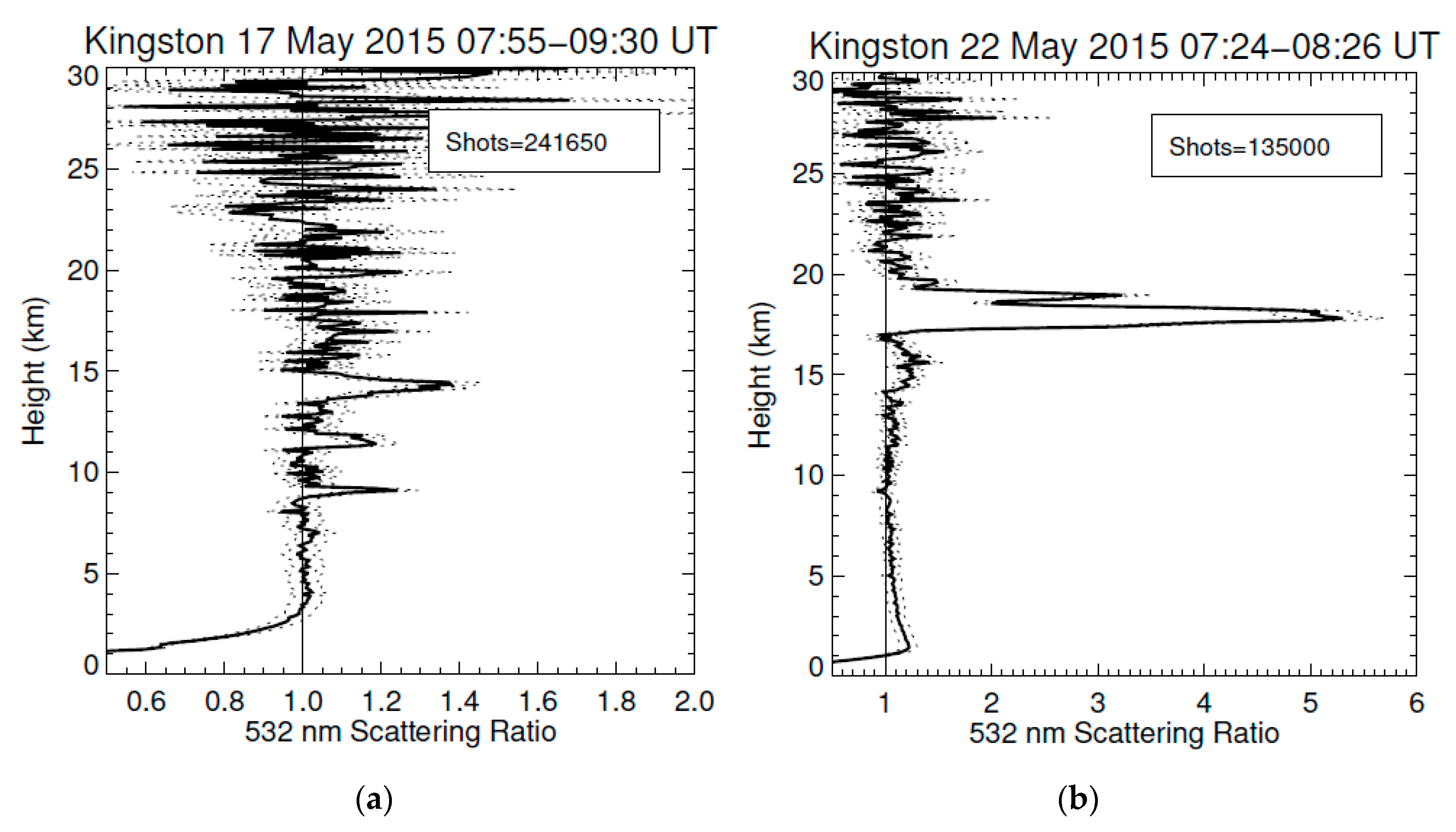
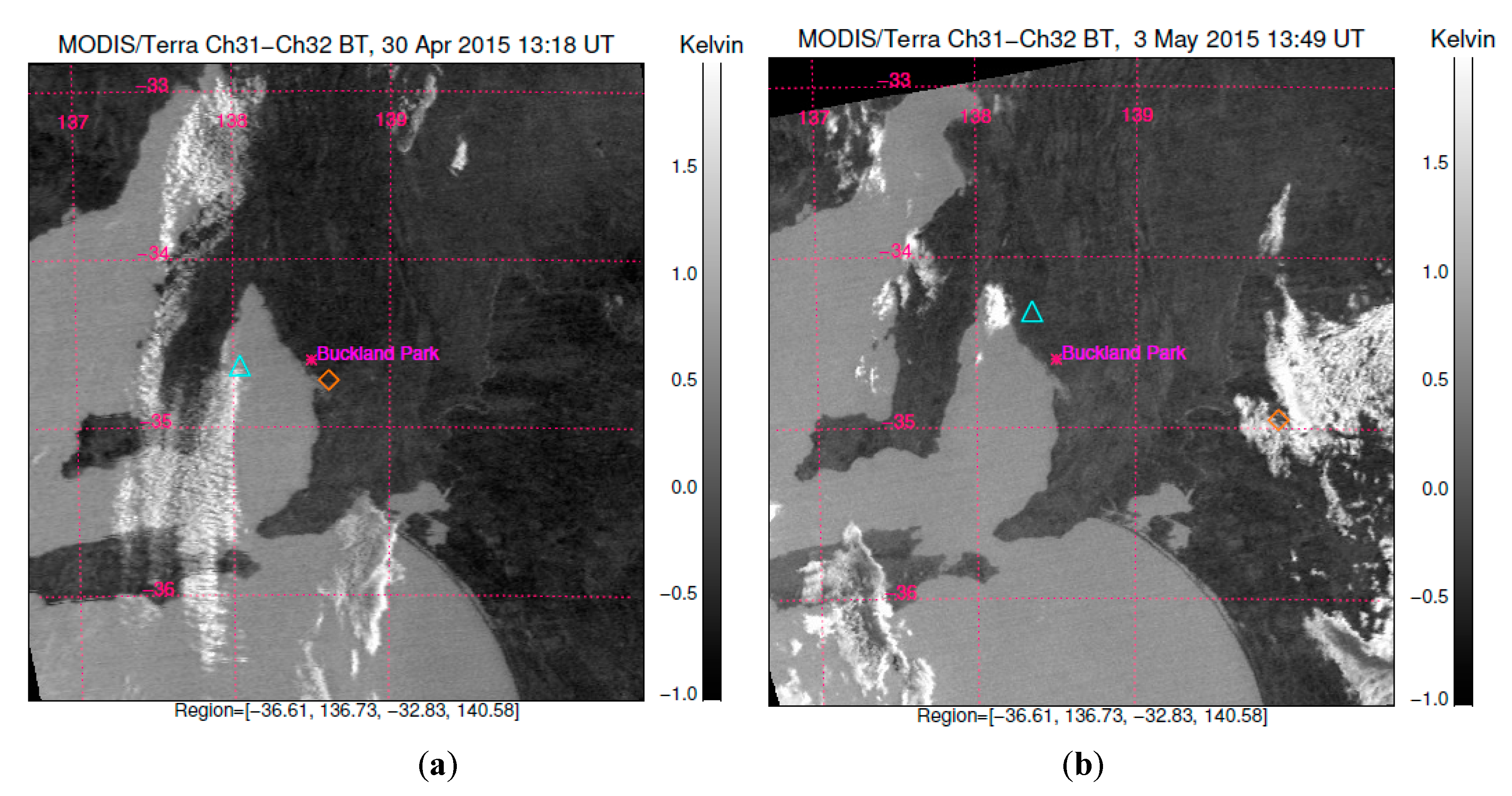
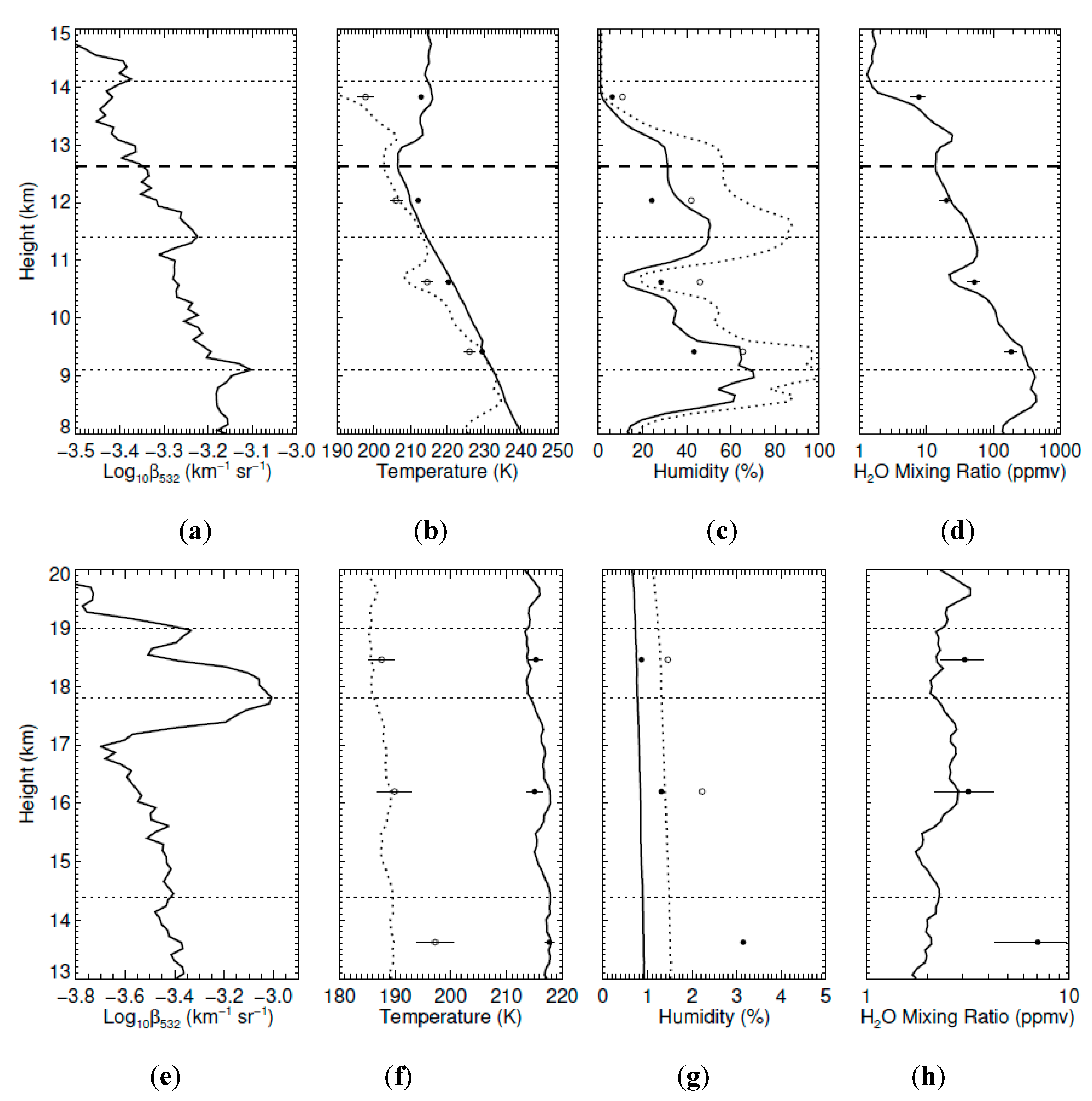
| Parameter | Buckland Park | Kingston |
|---|---|---|
| Location | 34.6° S, 138.5° E (~40 km NNW of Adelaide, South Australia) | 43.0° S, 147.3° E (~12 km S of Hobart, Tasmania) |
| Operating mode | Simultaneous Rayleigh/Mie (532 nm) and N2 Raman (608 nm) backscatter | Rayleigh/Mie (532 nm) backscatter; time-multiplexed co- and cross-polarised measurements were available for some periods |
| Laser energy per pulse and wavelength | 400 mJ, 532 nm | 75 mJ, 532 nm |
| Interference filter width (FWHM) | 0.3 nm (532 nm), 1.5 nm (608 nm, blocking >106 at 532 nm) | 0.1 nm |
| Laser repetition rate | 50 Hz | 50 Hz |
| Dwell per accumulation | 40 s (2000 shots) | 3 s (150 shots)—this was also the switching interval for depolarisation measurements |
| Range resolution | 50 m | 75 m |
| Receiver aperture | 1000 mm | 280 mm |
| Receiver configuration | coaxial full overlap above ~20 km range | bistatic, separation 160 mm full overlap above 900 m range |
| Receiver field of view (full angle) | 0.3 mrad | 0.5 mrad |
| Divergence of transmitter (full angle) | 0.1 mrad | 0.1 mrad |
| Date (2015) | Site | Observation Time Span (hour:minute (HH:MM) Universal Time (UT)) | CSR (km) | Time (HH:MM UT) of Radiosonde (Peak Height, km), AIRS Pass (Distance, km) 1 | Identified Aerosol Layers | |
|---|---|---|---|---|---|---|
| Altitude Range (km) | Peak 532 nm Scattering Ratio 2 (Height (km), θ (K) 3) | |||||
| 30 April | Kingston | 30 April 02:40–1 May 06:53 (depolarisation throughout) | (20,25) | 30 April 11:15 (20.6), 30 April 14:53 (23) | Nil 4 | <1.19 (0.07) over 10–20 km |
| Buckland Park | 12:00–13:00 | (30,35) | 11:16 (24.3), 16:29 (42) | 10.9–12.9 | 1.5 ± 0.1 (11.3, 336) 1.5 ± 0.1 (12.1, 349) 1.2 ± 0.1 (12.7, 356) | |
| 3 May | Buckland Park | 12:00–13:41 | (30,35) | 11:17 (24.5), 15:27 (34) | 15.3–16.2 | 1.5 ± 0.1 (15.7, 378) |
| 11 May | Kingston | 09:53–10:55 | (25,30) | 11:14 (21.6), 14:40 (37) | Nil 3,4 | <1.4 (0.2) over 10–20 km |
| 17 May | Kingston | 07:55–09:30 | (25,30) | 11:14 (23.7), 04:34 (26) | 11.0–15.2 17.2 | 1.2 ± 0.1 (11.4, 330) 1.4 ± 0.1 (14.5, 382) 1.1 ± 0.1 (17.2, 433) 6 |
| 22 May | Kingston | 07:24–08:26 (depolarisation 07:52–08:09) | (25,30) | 11:15 (22.9), 04:52 (29) | 14.0–17.0 17.2–18.5 18.7–19.1 | 1.4 ± 0.1 (15.6, 408) 5.2 ± 0.4 (17.8, 446) 3.2 ± 0.3 (19.0, 470) |
| 28 May | Kingston | 21:54–22:28 | (20,25) | 23:16 (29.1), 15:17 (29) | Nil 4,5 | <1.6 (0.4) over 10–20 km |
| Packet 1 May 21 23:15 UT | Packet 2 22 May 11:15 UT | Packet 3 21 May 23:15 UT | Packet 4 22 May 11:15 UT | |
|---|---|---|---|---|
| Extrapolated altitude (km) at mid-lidar observation | 19.4 | 19.2 | 20.4 | 20.6 |
| Ground-based period (h) | 0.7 | 1.1 | 0.6 | 1.4 |
| Vertical wavelength (km) | 0.5 | 0.6 | 1.8 | 2.1 |
| Vertical group speed (m h−1) | +38 | +40 | +199 | +63 |
| Peak–peak temperature perturbation (K) | 0.2 | 0.2 | 0.8 | 1.6 |
| Peak–peak zonal wind perturbation (m s−1) | 0.6 | 0.1 | 1.9 | 2.0 |
| Peak–peak meridional wind perturbation (m s−1) | 0.9 | 0.2 | 3.4 | 2.3 |
| Date (2015) and Time Range (UT) | Height Range (km) | Young Method Optical Depth 2 | Uchino et al. Method Optical Depth 2 | Kovalev et al. Method Optical Depth 2 | Layer- Mean Optical Depth 2 | OMPS Optical Depth for 532 nm 2 | Estimated Lidar Ratio Saer (sr) |
|---|---|---|---|---|---|---|---|
| 17 May 07:55–09:30 | 8.6–9.6 10.9–12.3 13.4–15.1 | 0.017 (18) 0.017 (12) 0.009 (11) | 0.029 (61) 0.018 (23) 0.009 (19) | 0.036 (27) 0.011 (14) NA 4 | 0.023 (40) 0.014 (17) 0.009 (15) | Nil 3 0.0004 (1) 0.0070 (3) (13–15 km) | 36 ± 61 71 ± 82 90 ± 153 |
| 22 May 07:24–08:26 | 14.0–20.5 | 0.078 (27) | 0.080 (39) | NA 4 | 0.079 (34) | 0.0093 (4) (14–20 km) | 86 ± 37 |
| Height Range (km) | Mean Particle Linear Depolarisation Ratio δp (%) | Maximum Particle Linear Depolarisation Ratio δp (%) | Estimated Ash Backscatter Fraction |
|---|---|---|---|
| 15.0–16.0 | 27 ± 9 | 0.80 ± 0.22 | |
| 17.2–18.5 | 13.9 ± 1.9 | 25 ± 29 (17.7 km) | 0.44 ± 0.27 |
| 18.5–19.1 | 26.0 ± 7.0 | 54 ± 72 (18.6 km) | 0.77 ± 0.18 |
| 17.2–19.1 | 18.0 ± 3.0 | 0.56 ± 0.20 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Klekociuk, A.R.; Ottaway, D.J.; MacKinnon, A.D.; Reid, I.M.; Twigger, L.V.; Alexander, S.P. Australian Lidar Measurements of Aerosol Layers Associated with the 2015 Calbuco Eruption. Atmosphere 2020, 11, 124. https://doi.org/10.3390/atmos11020124
Klekociuk AR, Ottaway DJ, MacKinnon AD, Reid IM, Twigger LV, Alexander SP. Australian Lidar Measurements of Aerosol Layers Associated with the 2015 Calbuco Eruption. Atmosphere. 2020; 11(2):124. https://doi.org/10.3390/atmos11020124
Chicago/Turabian StyleKlekociuk, Andrew R., David J. Ottaway, Andrew D. MacKinnon, Iain M. Reid, Liam V. Twigger, and Simon P. Alexander. 2020. "Australian Lidar Measurements of Aerosol Layers Associated with the 2015 Calbuco Eruption" Atmosphere 11, no. 2: 124. https://doi.org/10.3390/atmos11020124
APA StyleKlekociuk, A. R., Ottaway, D. J., MacKinnon, A. D., Reid, I. M., Twigger, L. V., & Alexander, S. P. (2020). Australian Lidar Measurements of Aerosol Layers Associated with the 2015 Calbuco Eruption. Atmosphere, 11(2), 124. https://doi.org/10.3390/atmos11020124






