Specific Adsorption of Heavy Metals in Soils: Individual and Competitive Experiments
Abstract
1. Introduction
2. Material and Methods
2.1. Soil Sampling and Soil Analysis
2.2. Single Adsorption and Desorption Experiments
2.3. Competitive Adsorption and Desorption Experiments
2.4. Data Analysis and Statistical Treatment
3. Results and Discussion
3.1. Soil Properties
3.2. Sorption and Desorption Experiments
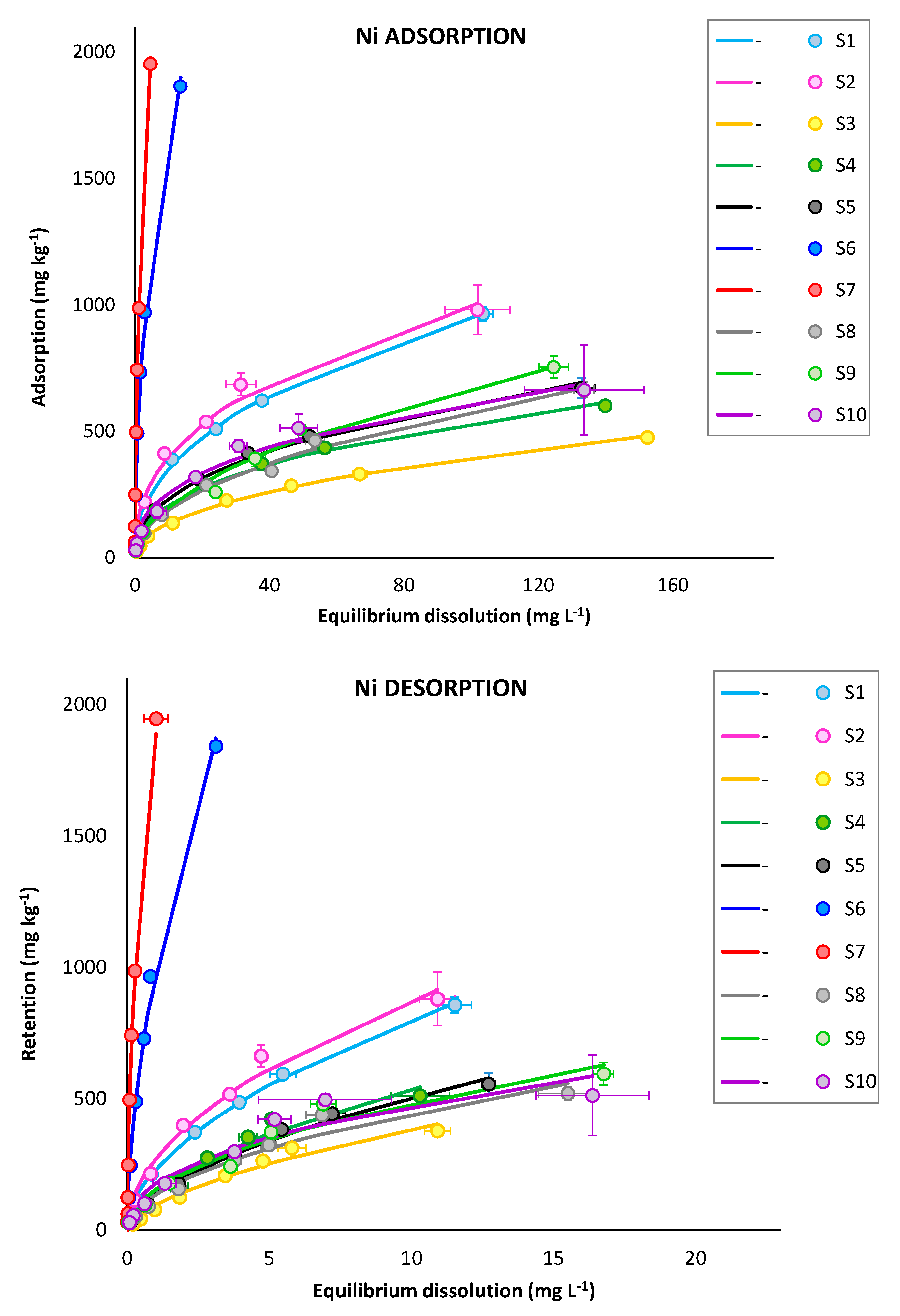
3.2.1. Adsorption and Retention Curves
3.2.2. Selectivity Sequences
3.2.3. Adsorption Versus Retention Concentrations
3.2.4. Pearson Correlation: Soil Properties vs. KF
3.2.5. Sorption Reversibility. Principal Component Analysis
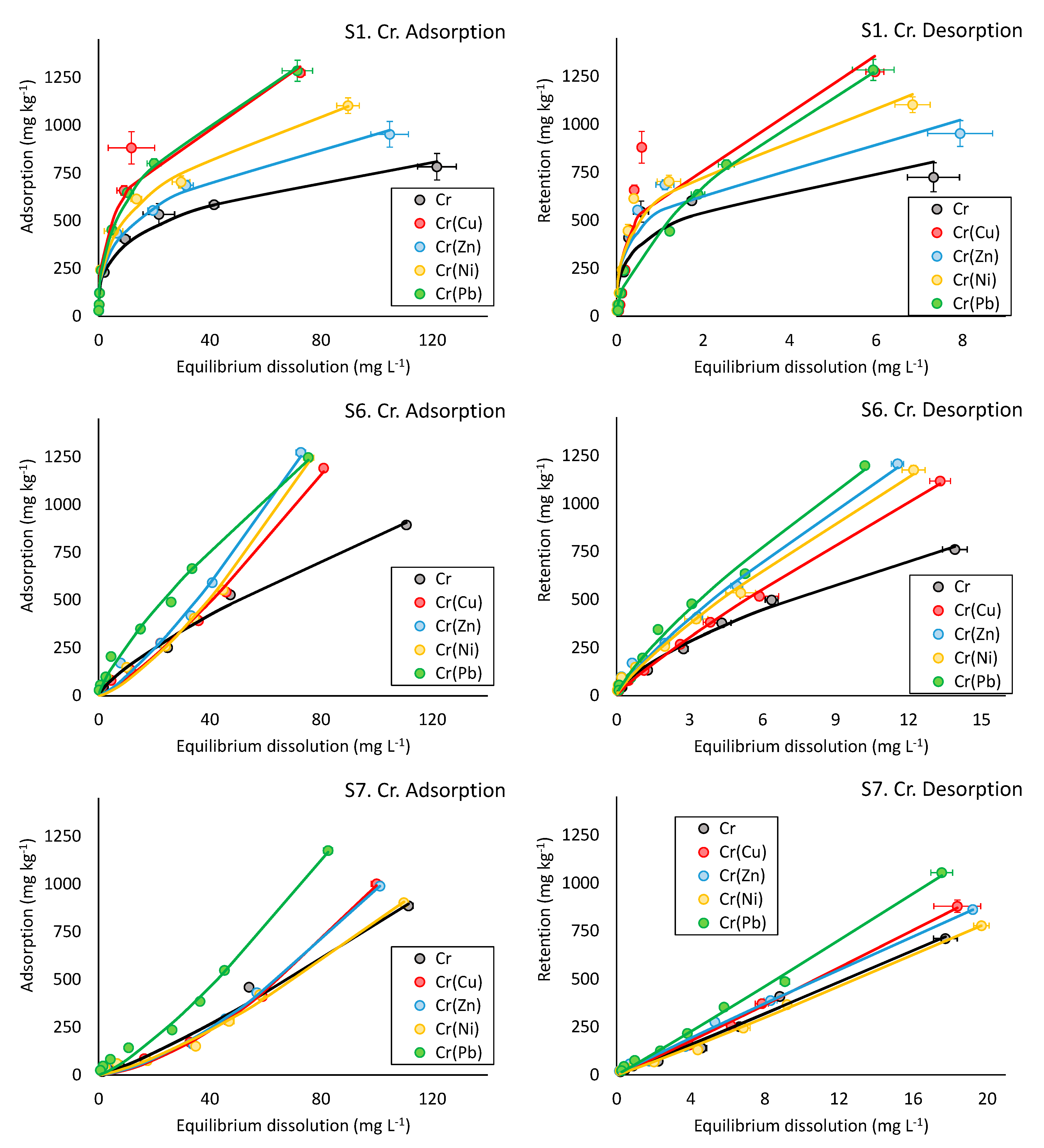
3.2.6. Competitive Adsorption and Desorption Experiments
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Vidal, M.; Santos, M.J.; Abrão, T.; Rodríguez, J.; Rigol, A. Modeling competitive metal sorption in a mineral soil. Geoderma 2009, 149, 189–198. [Google Scholar] [CrossRef]
- Elbana, T.A.; Selim, H.M. Modeling of cadmium and nickel release from different soils. Geoderma 2019, 338, 78–87. [Google Scholar] [CrossRef]
- Bradl, H.B. Adsorption of heavy metal ions on soils and soils constituents. J. Colloid Interface Sci. 2004, 277, 1–18. [Google Scholar] [CrossRef] [PubMed]
- Selim, H.M.; Zhang, H. Modeling approaches of competitive sorption and transport of trace metals and metalloids in soils: A review. J. Environ. Qual. 2013, 42, 640–653. [Google Scholar] [CrossRef] [PubMed]
- Ming, H.; Naidu, R.; Sarkar, B.; Lamb, D.T.; Liu, Y.; Megharaj, M.; Sparks, D. Competitive sorption of cadmium and zinc in contrasting soils. Geoderma 2016, 268, 60–68. [Google Scholar] [CrossRef]
- Wu, Z.; Chen, Y.; Han, Y.; Ke, T.; Liu, Y. Identifying the influencing factors controlling the spatial variation of heavy metals in suburban soil using spatial regression models. Sci. Total Environ. 2020, 717, 137212. [Google Scholar] [CrossRef]
- Huang, B.; Li, Z.; Huang, J.; Guo, L.; Nie, X.; Wang, Y.; Zhang, Y.; Zeng, G. Adsorption characteristics of Cu and Zn onto various size fractions of aggregates from red paddy soil. J. Hazard. Mater. 2014, 264, 176–183. [Google Scholar] [CrossRef]
- Fernández-Calviño, D.; Pérez-Novo, C.; Bermúdez-Couso, A.; López-Periago, E.; Arias-Estévez, M. Batch and stirred flow reactor experiments on Zn sorption in acid soils Cu competition. Geoderma 2010, 159, 417–424. [Google Scholar] [CrossRef]
- Violante, A.; Cozzolino, V.; Perelomov, L.; Caporale, A.G.; Pigna, M. Mobility and bioavailability of heavy metals and metalloids in soil environments. J. Soil Sci. Plant Nutr. 2010, 10, 268–292. [Google Scholar] [CrossRef]
- Dinić, Z.; Maksimović, J.; Stanojković-Sebić, A.; Pivić, R. Prediction Models for Bioavailability of Mn, Cu, Zn, Ni and Pb in Soils of Republic of Serbia. Agronomy 2019, 9, 856. [Google Scholar] [CrossRef]
- Huang, Y.; Fu, C.; Fang, F.; Ouyang, W.; Gou, J. Effect of dissolved organic matters on adsorption and desorption behavior of heavy metals in a water-level-fluctuation zone of the Three Gorges Reservoir, China. Ecotoxico. Environ. Saf. 2019, 185, 109695. [Google Scholar] [CrossRef]
- Abdin, Y.; Usman, A.; Ok, Y.S.; Tsnag, Y.F.; Al-Wabel, M. Competitive sorption and availability of coexisting heavy metals in mining-contaminated soil: Contrasting effects of mesquite and fishbone biochars. Environ. Res. 2020, 181, 108846. [Google Scholar] [CrossRef] [PubMed]
- Sparks, D.L. Environmental Soil Chemistry, 2nd ed.; Academic Press: San Diego, CA, USA, 2003; p. 352. [Google Scholar]
- Antoniadis, V.; Tsadilas, C.D. Sorption of cadmium, nickel, and zinc in mono- and multimetal systems. Appl. Geochem. 2007, 22, 2375–2380. [Google Scholar] [CrossRef]
- Silva-Yumi, J.; Escudey, M.; Gacitua, M.; Pizarro, C. Kinetics, adsorption and desorption of Cd(II) and Cu(II) on natural allophane: Effect of iron oxide coating. Geoderma 2018, 319, 70–79. [Google Scholar] [CrossRef]
- Sipos, P.; Tóth, A.; Kis, V.K.; Balázs, R.; Kovács, I.; Németh, T. Partition of Cd, Cu, Pb and Zn among mineral particles during their sorption in soils. J. Soils Sedim. 2019, 19, 1775–1787. [Google Scholar] [CrossRef]
- Shaheen, S.M.; Tsadilas, C.D.; Rupp, H.; Rinklebe, J.; Melssner, R. Distribution coefficients of cadmium and zinc in different soils in mono-metal and competitive sorption systems. J. Plant Nutr. Soil Sci. 2015, 178, 671–681. [Google Scholar] [CrossRef]
- Limousin, G.; Gaudet, J.P.; Charlet, L.; Szenknect, S.; Barthès, V.; Krimissa, M. Sorption isotherms: A review on physical bases, modelling and measurement. Appl. Geochem. 2007, 22, 249–275. [Google Scholar] [CrossRef]
- Caporale, A.G.; Violante, A. Chemical processes affecting the mobility of heavy metals and metalloids in soil environments. Curr. Pollut. Rep. 2016, 2, 15–27. [Google Scholar] [CrossRef]
- Elbana, T.A.; Selim, H.M.; Akrami, N.; Newman, A.; Shaheen, S.M.; Rinklebe, J. Freundlich sorption parameters for cadmium, copper, nickel, lead, and zinc for different soils: Influence of kinetics. Geoderma 2018, 324, 80–88. [Google Scholar] [CrossRef]
- Bogusz, A.; Oleszczuk, P.; Dobrowolski, R. Adsorption and desorption of heavy metals by the sewage sludge and biochar-amended soil. Environ. Geochem. Health 2019, 41, 1663–1674. [Google Scholar] [CrossRef]
- Liao, L.; Selim, H.M. Competitive sorption of nickel and cadmium in different soils. Soil Sci. 2009, 174, 549–555. [Google Scholar] [CrossRef]
- Guitián, F.; Carballas, T. Técnicas de Análisis de Suelos; Pico Sacro: Santiago de Compostela, Spain, 1976. [Google Scholar]
- Hoogsteen, M.J.J.; Lantinga, E.A.; Bakker, E.J.; Groot, J.C.J.; Tittonell, P.A. Estimating soil organic carbon through loss on ignition: Effects of ignition conditions and structural water loss. Eur. J. Soil Sci. 2015, 66, 320–328. [Google Scholar] [CrossRef]
- Sumner, M.E.; Miller, W.P. Cation exchange capacity and exchange coefficients. In Methods of Soil Analysis Part 3: Chemical Methods; Sparks, D.L., Page, A.L., Helmke, P.A., Loeppert, R.H., Soltanpour, P.N., Tabatabai, M.A., Johnston, C.T., Sumner, M.E., Eds.; Soil Science Society of America, Inc.: Madison, WI, USA, 1996. [Google Scholar]
- Bertsch, P.M.; Bloom, P.R. Aluminium. In Methods of Soil Analysis Part 3: Chemical Methods; Sparks, D.L., Page, A.L., Helmke, P.A., Loeppert, R.H., Soltanpour, P.N., Tabatabai, M.A., Johnston, C.T., Sumner, M.E., Eds.; Soil Science Society of America, Inc.: Madison, WI, USA, 1996. [Google Scholar]
- Harter, R.D.; Naidu, R. An assessment of environmental and solution parameter impact on trace-metal sorption by soils. Soil Sci. Soc. Am. J. 2001, 65, 597–612. [Google Scholar] [CrossRef]
- Murali, V.; Aylmore, A.G. Competitive adsorption during solute transport in soils: 1. Mathematical models. Soil Sci. 1983, 135, 143–150. [Google Scholar] [CrossRef]
- Macías-Vázquez, F.; Calvo de Anta, R. Niveles genéricos de referencia de metales pesados y otros elementos traza en suelos de Galicia. In Consellería de Medio Ambiente e Desenvolvemento Sostible; Xunta de Galicia: Santiago, Spain, 2009. [Google Scholar]
- Komonweeraket, K.; Cetin, B.; Aydilek, A.H.; Benson, C.H.; Edil, T.B. 2015. Effects of pH on the leaching mechanisms of elements from fly ashmixed soils. Fuel 1984, 140, 788–802. [Google Scholar] [CrossRef]
- Otero, M.; Cutillas-Barreiro, L.; Nóvoa-Muñoz, J.C.; Arias-Estévez, M.; Fernández-Sanjurjo, M.J.; Álvarez-Rodríguez, E.; Núñez-Delgado, A. Cr(VI) sorption/desorption on untreated and mussel-shell-treated soil materials: Fractionation and effects of pH and chromium concentration. Solid Earth 2015, 6, 373–382. [Google Scholar] [CrossRef]
- Choppala, G.; Kunhikrishnan, A.; Seshadri, B.; Park, J.H.; Bush, R.; Bolan, N. Comparative sorption of chromium species as influenced by pH, surface charge and organic matter content in contaminated soils. J. Geochem. Explor. 2018, 184, 255–260. [Google Scholar] [CrossRef]
- Yang, Z.; Liang, J.; Tang, L.; Zeng, G.; Yu, M.; Li, X.; Li, X.; Qian, Y.; Wu, H.; Luo, Y.; et al. Sorption-desorption behaviors of heavy metals by biochar-compost amendment with different ratios in contaminated wetland soil. J. Soils Sedim. 2018, 18, 1530–1539. [Google Scholar] [CrossRef]
- Basta, N.T.; Tabatabai, M.A. Effect of cropping systems on adsorption of metals by soils: III. Competitive adsorption. Soil Sci. 1992, 153, 331–337. [Google Scholar] [CrossRef]
- Gomes, P.C.; Fontes, M.P.F.; da Silva, A.G.; Mendonça, E.d.S.; Netto, A.R. Selectivity sequence and competitive adsorption of heavy metals by Brazilian soils. Soil Sci. Soc. Am. J. 2001, 65, 1115–1121. [Google Scholar] [CrossRef]
- Conde-Cid, M.; Fernández-Calviño, D.; Nóvoa-Muñoz, J.C.; Núñez-Delgado, A.; Fernández-Sanjurjo, M.J.; Arias-Estévez, M.; Álvarez-Rodríguez, E. Experimental data and model prediction of tetracycline adsorption and desorption in agricultural soils. Environ. Res. 2019, 177, 108607. [Google Scholar] [CrossRef]
- Gustafsson, J.P. Visual Minteq 3.1; KTH Royal Institute of Technology: Stockholm, Sweden, 2013. [Google Scholar]
- Echeverría, J.C.; Morera, M.T.; Mazkiarán, C.; Garrido, J.J. Competitive sorption of heavy metal by soils. Isotherms and fractional factorial experiments. Environ. Pollut. 1998, 101, 275–284. [Google Scholar] [CrossRef]
- Arias, M.; Pérez-Novo, C.; Osorio, F.; López, E.; Soto, B. Adsorption and desorption of copper and zinc in the surface layer of acid soils. J. Colloid Interface Sci. 2005, 288, 21–29. [Google Scholar] [CrossRef] [PubMed]
- Tiller, K.G.; Hodgson, J.F. The specific sorption of cobalt and zinc by layer silicates. In Clays and Clay Minerals; Swineford, A., Ed.; Pergamon Press: New York, NY, USA, 1962. [Google Scholar]
- Martínez-Cortizas, A.M.; Muñiz, I.R.; Taboada, T.; Toro, M.; Granados, I.; Giralt, S.; Pla-Rebés, S. Factors controlling the geochemical composition of Limnopolar Lake sediments (Byers Peninsula, Livingston Island, South Shetland Island, Antarctica) during the last ca. 1600 years. Solid Earth 2014, 5, 651–663. [Google Scholar] [CrossRef]
- Rosen, V.; Chen, Y. Effects of compost application on soil vulnerability to heavy metal pollution. Environ. Sci. Pollut. Res. 2018, 25, 35221–35231. [Google Scholar] [CrossRef] [PubMed]
- Kabata-Pendias, A.; Pendias, H. Trace Elements in Soils and Plants; CRC Press Inc.: Boca Raton, FL, USA, 1984; p. 315. [Google Scholar]
- Veli, S.; Alyüz, B. Adsorption of copper and zinc from aqueous solutions by using natural clay. J. Hazard. Mater. 2007, 149, 226–233. [Google Scholar] [CrossRef] [PubMed]
- Arias, M.; Pérez-Novo, C.; López, E.; Soto, B. Competitive adsorption and desorption of copper and zinc in acid soils. Geoderma 2006, 133, 151–159. [Google Scholar]
- Hossain, M.A.; Furumai, M.A.; Nakajima, F. Competitive adsorption of heavy metals in soil underlying an infiltration facility installed in an urban area. Water Sci. Technol. 2019, 59, 303–310. [Google Scholar] [CrossRef]
- Garrido-Rodriguez, B.; Cutillas-Barreiro, L.; Fernández-Calviño, D.; Arias-Estévez, M.; Fernández-Sanjurjo, M.J.; Álvarez-Rodríguez, E.; Núñez-Delgado, A. Competitive adsorption and transport of Cd, Cu, Ni and Zn in a mine soil amended with mussel Shell. Chemosphere 2014, 107, 379–385. [Google Scholar] [CrossRef]
- Pérez-Novo, C.; Bermúdez-Couso, A.; López-Periago, E.; Fernández-Calviño, D.; Arias-Estévez, M. The effect of phosphate on the sorption of copper by acid soils. Geoderma 2009, 150, 166–170. [Google Scholar] [CrossRef]
- Pérez-Novo, C.; Fernández-Calviño, D.; Bermúdez-Couso, A.; López-Periago, J.E.; Arias-Estévez, M. Phosphorus effect on Zn adsorption-desorption kinetics in acid soils. Chemosphere 2011, 83, 1028–1034. [Google Scholar] [CrossRef] [PubMed]


| S1 | S2 | S3 | S4 | S5 | S6 | S7 | S8 | S9 | S10 | ||
|---|---|---|---|---|---|---|---|---|---|---|---|
| pHW | 4.0 | 4.6 | 4.8 | 4.9 | 4.9 | 6.4 | 7.5 | 5.0 | 4.7 | 4.9 | |
| pHKCl | 3.0 | 3.8 | 4.2 | 4.5 | 4.2 | 5.8 | 6.9 | 4.5 | 4.3 | 4.4 | |
| OM | % | 14 | 14 | 12 | 12 | 11 | 14 | 15 | 10 | 20 | 29 |
| TC | 7.0 | 6.7 | 8.23 | 3.7 | 5.0 | 6.6 | 5.5 | 6.7 | 10.3 | 14.3 | |
| TN | 0.5 | 0.4 | 0.6 | 0.3 | 0.5 | 0.5 | 0.4 | 0.4 | 0.8 | 1.0 | |
| DOC | mg kg−1 | 123.7 | 139.6 | 65.2 | 27.9 | 59.1 | 111.7 | 106.1 | 53.9 | 50.6 | 88.5 |
| eCEC | cmolc kg−1 | 8.0 | 9.1 | 5.8 | 3.1 | 1.9 | 23.4 | 29.1 | 4.1 | 6.8 | 6.9 |
| Cae | 1.9 | 3.4 | 1.9 | 1.7 | 0.4 | 21.4 | 27.8 | 2.1 | 3.3 | 3.7 | |
| Mge | 1.0 | 1.2 | 0.4 | 0.3 | 0.1 | 1.2 | 1.1 | 0.4 | 0.4 | 0.4 | |
| Ke | 0.1 | 0.2 | 0.2 | 0.1 | 0.1 | 0.7 | 0.1 | 0.3 | 0.2 | 0.4 | |
| Nae | 0.2 | 1.6 | 0.2 | 0.2 | 0.1 | 0.2 | 0.1 | 0.2 | 0.3 | 0.5 | |
| Ale | 4.8 | 2.7 | 3.2 | 0.9 | 1.2 | <0.2 | <0.2 | 1.1 | 2.6 | 1.9 | |
| Sand | % | 71 | 62 | 50 | 47 | 68 | 19 | 20 | 32 | 46 | 31 |
| Silt | 13 | 19 | 25 | 35 | 16 | 67 | 52 | 37 | 35 | 45 | |
| Clay | 16 | 19 | 25 | 17 | 16 | 14 | 28 | 32 | 19 | 24 |
| ADSORPTION | DESORPTION-RETENTION | HISTERESIS | ||||||
|---|---|---|---|---|---|---|---|---|
| KF | n | R2 | KF | n | R2 | KFDES/KFADS | ||
| Cu | S1 | 403.6 ± 12.5 | 0.34 ± 0.01 | 0.99 | 749.9 ± 22.6 | 0.45 ± 0.02 | 0.97 | 1.86 |
| S2 | 425.2 ± 21.7 | 0.35 ± 0.02 | 0.97 | 763.4 ± 27.6 | 0.47 ± 0.03 | 0.96 | 1.80 | |
| S3 | 204.6 ± 12.0 | 0.36 ± 0.02 | 0.99 | 455.3 ± 08.7 | 0.43 ± 0.01 | 0.99 | 2.23 | |
| S4 | 240.6 ± 08.3 | 0.37 ± 0.01 | 0.99 | 542.2 ± 00.4 | 0.43 ± 0.02 | 0.98 | 2.25 | |
| S5 | 343.0 ± 07.1 | 0.33 ± 0.01 | 1.00 | 599.8 ± 11.5 | 0.36 ± 0.11 | 0.99 | 1.75 | |
| S6 | 2539.8 ± 86.8 | 0.91 ± 0.04 | 0.98 | 9244.0 ± 872 | 1.22 ± 0.06 | 0.97 | 3.64 | |
| S7 | 8407.2 ± 654 | 1.28 ± 0.06 | 0.98 | 19665 ± 3638 | 1.23 ± 0.09 | 0.95 | 2.34 | |
| S8 | 347.0 ± 09.5 | 0.37 ± 0.01 | 1.00 | 638.5 ± 09.6 | 0.39 ± 0.01 | 1.00 | 1.84 | |
| S9 | 461.9 ± 08.5 | 0.33 ± 0.01 | 1.00 | 783.5 ± 18.1 | 0.38 ± 0.01 | 0.98 | 1.70 | |
| S10 | 491.4 ± 35.2 | 0.29 ± 0.02 | 0.93 | 800.5 ± 19.7 | 0.41 ± 0.02 | 0.98 | 1.63 | |
| Zn | S1 | 115.9 ± 12.7 | 0.38 ± 0.03 | 0.94 | 18.9 ± 07.2 | 0.64 ± 0.11 | 0.78 | 0.16 |
| S2 | 148.3 ± 15.4 | 0.37 ± 0.03 | 0.94 | 13.1 ± 03.7 | 0.81 ± 0.08 | 0.92 | 0.09 | |
| S3 | 60.8 ± 09.7 | 0.39 ± 0.04 | 0.90 | 22.1 ± 08.2 | 0.65 ± 0.13 | 0.70 | 0.36 | |
| S4 | 79.6 ± 10.2 | 0.36 ± 0.03 | 0.92 | 9.6 ± 04.5 | 0.88 ± 0.15 | 0.79 | 0.12 | |
| S5 | 115.8 ± 14.0 | 0.33 ± 0.03 | 0.92 | 20.8 ± 07.9 | 0.69 ± 0.12 | 0.78 | 0.18 | |
| S6 | 700.4 ± 24.2 | 0.44 ± 0.02 | 0.98 | 876.3 ± 46.0 | 0.37 ± 0.03 | 0.92 | 1.25 | |
| S7 | 1332.3 ± 35.5 | 0.44 ± 0.02 | 0.97 | 2416.0 ± 96.5 | 0.59 ± 0.03 | 0.97 | 1.81 | |
| S8 | 68.3 ± 08.3 | 0.42 ± 0.03 | 0.95 | 115.2 ± 13.6 | 0.44 ± 0.05 | 0.86 | 1.69 | |
| S9 | 80.0 ± 08.8 | 0.39 ± 0.03 | 0.95 | 26.5 ± 07.7 | 0.61 ± 0.10 | 0.80 | 0.33 | |
| S10 | 112.4 ± 10.8 | 0.37 ± 0.02 | 0.96 | 35.6 ± 10.2 | 0.60 ± 0.09 | 0.81 | 0.32 | |
| Ni | S1 | 128.4 ± 05.3 | 0.44 ± 0.01 | 0.99 | 229.6 ± 05.6 | 0.54 ± 0.01 | 1.00 | 1.79 |
| S2 | 157.4 ± 14.1 | 0.40 ± 0.02 | 0.97 | 268.2 ± 14.9 | 0.51 ± 0.03 | 0.97 | 1.70 | |
| S3 | 46.6 ± 02.8 | 0.47 ± 0.01 | 0.99 | 95.2 ± 06.9 | 0.60 ± 0.04 | 0.96 | 2.05 | |
| S4 | 79.8 ± 03.8 | 0.41 ± 0.01 | 0.99 | 150.7 ± 09.0 | 0.55 ± 0.03 | 0.97 | 1.89 | |
| S5 | 89.2 ± 05.4 | 0.42 ± 0.02 | 0.99 | 134.8 ± 06.9 | 0.57 ± 0.03 | 0.98 | 1.51 | |
| S6 | 537.1 ± 17.0 | 0.48 ± 0.01 | 0.99 | 976.0 ± 17.4 | 0.57 ± 0.02 | 0.99 | 1.82 | |
| S7 | 867.1 ± 16.4 | 0.54 ± 0.01 | 0.99 | 1866.7 ± 92.5 | 0.53 ± 0.04 | 0.92 | 2.15 | |
| S8 | 61.3 ± 04.8 | 0.49 ± 0.02 | 0.99 | 134.1 ± 09.9 | 0.52 ± 0.03 | 0.96 | 2.19 | |
| S9 | 62.0 ± 06.7 | 0.52 ± 0.03 | 0.98 | 152.2 ± 11.5 | 0.50 ± 0.03 | 0.95 | 2.45 | |
| S10 | 106.2 ± 18.1 | 0.38 ± 0.04 | 0.89 | 174.7 ± 18.4 | 0.43 ± 0.05 | 0.88 | 1.65 | |
| Pb | S1 | 1239.8 ± 41.1 | 0.47 ± 0.03 | 0.96 | 3427.3 ± 176.1 | 0.91 ± 0.05 | 0.97 | 2.76 |
| S2 | 2100.9 ± 70.0 | 0.74 ± 0.04 | 0.97 | 5082.4 ± 375.4 | 1.21 ± 0.07 | 0.97 | 2.42 | |
| S3 | 673.6 ± 12.9 | 0.35 ± 0.01 | 0.99 | 1100.7 ± 17.9 | 0.40 ± 0.01 | 0.99 | 1.63 | |
| S4 | 779.7 ± 39.2 | 0.33 ± 0.02 | 0.94 | 1646.7 ± 59.8 | 0.53 ± 0.03 | 0.95 | 2.11 | |
| S5 | 1186.2 ± 41.8 | 0.33 ± 0.02 | 0.95 | 2084.6 ± 103.2 | 0.41 ± 0.03 | 0.93 | 1.76 | |
| S6 | 4957.0 ± 552.1 | 0.62 ± 0.05 | 0.92 | NA | ||||
| S7 | NA | |||||||
| S8 | 924.1 ± 49.7 | 0.42 ± 0.03 | 0.92 | 1890.2 ± 50.1 | 0.43 ± 0.02 | 0.98 | 2.05 | |
| S9 | 945.1 ± 49.2 | 0.43 ± 0.03 | 0.93 | 2021.3 ± 52.7 | 0.43 ± 0.02 | 0.98 | 2.14 | |
| S10 | 1337.5 ± 82.8 | 0.45 ± 0.04 | 0.85 | 2599.9 ± 128 | 0.41 ± 0.03 | 0.96 | 1.94 | |
| Cr | S1 | 186.2 ± 14.8 | 0.31 ± 0.02 | 0.96 | 442.5 ± 29.1 | 0.30 ± 0.04 | 0.78 | 2.38 |
| S2 | 135.8 ± 19.4 | 0.34 ± 0.04 | 0.90 | 352.8 ± 25.4 | 0.30 ± 0.04 | 0.79 | 2.60 | |
| S3 | 61.3 ± 04.4 | 0.43 ± 0.02 | 0.98 | 86.3 ± 8.8 | 0.48 ± 0.04 | 0.92 | 1.41 | |
| S4 | 41.4 ± 04.9 | 0.45 ± 0.03 | 0.96 | 147.3 ± 7.6 | 0.36 ± 0.03 | 0.93 | 3.56 | |
| S5 | 91.2 ± 04.4 | 0.44 ± 0.01 | 0.99 | 120.0 ± 6.2 | 0.52 ± 0.02 | 0.99 | 1.32 | |
| S6 | 25.8 ± 02.7 | 0.76 ± 0.02 | 0.99 | 135.8 ± 6.8 | 0.66 ± 0.02 | 0.99 | 5.27 | |
| S7 | 3.3 ± 00.8 | 1.19 ± 0.05 | 0.98 | 38.3 ± 4.4 | 1.02 ± 0.04 | 0.98 | 11.75 | |
| S8 | 85.4 ± 03.5 | 0.45 ± 0.01 | 1.00 | 178.0 ± 6.6 | 0.39 ± 0.02 | 0.99 | 2.09 | |
| S9 | 68.0 ± 03.1 | 0.47 ± 0.01 | 1.00 | 128.0 ± 6.0 | 0.45 ± 0.02 | 0.98 | 1.88 | |
| S10 | 109.1 ± 06.1 | 0.44 ± 0.01 | 0.99 | 208.5 ± 9.1 | 0.39 ± 0.02 | 0.98 | 1.91 | |
| pH | pHKCl | OM | DOC | eCEC | Ca | Mg | Na | Al | Sand | Silt | Clay | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Cu | All soils | ADS | 0.913 ** | 0.858 ** | 0.890 ** | 0.913 ** | ||||||||
| DES | 0.948 ** | 0.891 ** | 0.943 ** | 0.967 ** | −0.635 * | |||||||||
| No. S6&S7 | ADS | 0.709 * | ||||||||||||
| DES | ||||||||||||||
| Zn | All soils | ADS | 0.944 ** | 0.881 ** | 0.953 ** | 0.972 ** | ||||||||
| DES | 0.939 ** | 0.886 ** | 0.912 ** | 0.940 ** | ||||||||||
| No S6&S7 | ADS | 0.786 * | ||||||||||||
| DES | 0.846 ** | |||||||||||||
| Ni | All soils | ADS | 0.931 ** | 0.861 ** | 0.970 ** | 0.982 ** | 0.643 * | |||||||
| DES | 0.933 ** | 0.868 ** | 0.957 ** | 0.971 ** | ||||||||||
| No. S6&S7 | ADS | 0.857 ** | 0.819 * | 0.722 * | ||||||||||
| DES | 0.847 ** | 0.709 * | 0.897 ** | 0.748 * | ||||||||||
| Pb | All soils | ADS | 0.828 * | 0.754 * | 0.875 ** | |||||||||
| DES | 0.776 ** | 0.672 * | 0.651 * | 0.896 ** | 0.863 ** | 0.761 * | ||||||||
| No. S6&S7 | ADS | 0.828 * | 0.754 * | 0.875 ** | ||||||||||
| DES | 0.887 ** | 0.892 ** | 0.845 ** | |||||||||||
| Cr | All soils | ADS | −0.790 ** | −0.869 ** | −0.639 * | 0.813 ** | 0.722 * | −0.724 * | ||||||
| DES | −0.734 * | 0.698 * | ||||||||||||
| No. S6&S7 | ADS | −0.766 * | −0.866 ** | 0.887 ** | 0.800 * | |||||||||
| DES | −0.781 * | −0.856 ** | 0.858 ** | 0.900 ** |
| Loadings | PC1-Pb | PC2-Cu | PC3-Zn | PC4-Cr | PC5-Ni | Communality |
|---|---|---|---|---|---|---|
| Mge | 0.852 | 0.015 | 0.000 | 0.050 | 0.036 | 0.954 |
| Ale | 0.768 | 0.000 | 0.053 | 0.083 | 0.062 | 0.966 |
| DOC | 0.732 | 0.053 | 0.001 | 0.002 | 0.169 | 0.957 |
| pH | 0.719 | 0.040 | 0.152 | 0.001 | 0.002 | 0.914 |
| eCEC | 0.673 | 0.258 | 0.001 | 0.003 | 0.019 | 0.954 |
| His-Pb | 0.648 | 0.000 | 0.007 | 0.194 | 0.004 | 0.853 |
| OM | 0.002 | 0.887 | 0.047 | 0.004 | 0.001 | 0.941 |
| Cae | 0.083 | 0.761 | 0.016 | 0.057 | 0.050 | 0.966 |
| Ke | 0.000 | 0.667 | 0.255 | 0.047 | 0.001 | 0.969 |
| His-Cu | 0.027 | 0.393 | 0.012 | 0.097 | 0.160 | 0.689 |
| His-Zn | 0.032 | 0.006 | 0.901 | 0.002 | 0.013 | 0.954 |
| Clay | 0.032 | 0.031 | 0.803 | 0.011 | 0.050 | 0.927 |
| His-Cr | 0.010 | 0.010 | 0.016 | 0.949 | 0.002 | 0.987 |
| His-Ni | 0.002 | 0.003 | 0.115 | 0.000 | 0.783 | 0.902 |
| % of variance explained | 32.72 | 22.32 | 17.00 | 10.72 | 9.64 |
| Scores | PC1-Pb | PC2-Cu | PC3-Zn | PC4-Cr | PC5-Ni |
|---|---|---|---|---|---|
| S1 | 1.84 | −0.63 | −0.46 | −0.14 | −0.06 |
| S2 | 1.13 | 0.38 | 0.07 | 0.83 | −0.85 |
| S3 | −0.08 | −0.61 | 0.02 | −1.10 | 1.10 |
| S4 | −1.03 | −0.73 | −0.78 | 1.89 | 0.35 |
| S5 | −0.96 | −0.87 | −0.67 | −1.16 | −1.42 |
| S8 | −0.32 | −0.39 | 2.35 | 0.19 | 0.01 |
| S9 | −0.10 | 0.86 | −0.47 | −0.33 | 1.57 |
| S10 | −0.47 | 1.98 | −0.07 | −0.18 | −0.69 |
| Metal 1 | Metal 2 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Competitive Adsorption Freundlich Metal 1 (Metal 2) | Murali-Aylmore Metal 1 (Metal 2) | Competitive Adsorption Freundlich Metal 2 (Metal 1) | Murali-Aylmore Metal 2 (Metal 1) | |||||||
| KF-m1(m2) | n | R2 | am1(m2) | R2 | KF-m2(m1) | n | R2 | am2(m1) | R2 | |
| Cu (Zn) | Zn (Cu) | |||||||||
| S1 | 339.27 ± 13.38 | 0.36 ± 0.01 | 0.99 | 0.02 ± 0.01 | 0.98 | 84.71 ± 10.23 | 0.23 ± 0.03 | 0.82 | 9.24 ± 0.86 | 0.93 |
| S6 | 1705.76 ± 55.13 | 0.78 ± 0.04 | 0.96 | 0.02 ± 0.00 | 0.96 | 508.51 ± 21.45 | 0.36 ± 0.01 | 0.98 | 14.50 ± 5.43 | 0.73 |
| S7 | 3014.59 ± 100.76 | 0.91 ± 0.03 | 0.98 | 0.25 ± 0.02 | 0.98 | 1126.38 ± 34.50 | 0.45 ± 0.02 | 0.97 | 0.37 ± 0.20 | 0.95 |
| Cu (Ni) | Ni (Cu) | |||||||||
| S1 | 299.93 ± 10.17 | 0.38 ± 0.01 | 0.99 | 0.04 ± 0.01 | 0.99 | 97.03 ± 11.21 | 0.27 ± 0.03 | 0.88 | 7.50 ± 0.58 | 0.95 |
| S6 | 1624.25 ± 48.58 | 0.79 ± 0.04 | 0.96 | 0.02 ± 0.00 | 0.97 | 397.88 ± 26.36 | 0.40 ± 0.02 | 0.96 | 16.99 ± 6.20 | 0.74 |
| S7 | 4343.61 ± 263.52 | 1.30 ± 0.07 | 0.98 | 0.08 ± 0.01 | 0.98 | 635.11 ± 29.85 | 0.45 ± 0.02 | 0.97 | 9.11 ± 3.45 | 0.78 |
| Cu (Pb) | Pb (Cu) | |||||||||
| S1 | 334.83 ± 15.67 | 0.31 ± 0.01 | 0.98 | 0.96 ± 0.16 | 0.98 | 540.61 ± 12.74 | 0.36 ± 0.01 | 0.99 | NA | NA |
| S6 | 2263.07 ± 89.36 | 0.90 ± 0.05 | 0.96 | nd | nd | nd | nd | |||
| S7 | 4622.01 ± 249.33 | 1.07 ± 0.05 | 0.98 | nd | nd | nd | nd | |||
| Cu (Cr) | Cr (Cu) | |||||||||
| S1 | 533.81 ± 37.96 | 0.34 ± 0.02 | 0.93 | −0.06 ± 0.01 | 0.94 | 281.84 ± 32.80 | 0.36 ± 0.04 | 0.88 | −1.03 ± 0.16 | 0.85 |
| S6 | 2697.76 ± 65.99 | 1.18 ± 0.04 | 0.99 | 0.00 ± 0.00 | 0.95 | 5.00 ± 0.98 | 1.24 ± 0.05 | 0.99 | −25.82 ± 6.95 | 0.90 |
| S7 | 2715.07 ± 215.35 | 0.99 ± 0.09 | 0.90 | 0.01 ± 0.00 | 0.97 | 0.67 ± 0.15 | 1.59 ± 0.05 | 0.99 | −26.44 ± 5.32 | 0.96 |
| Zn (Ni) | Ni (Zn) | |||||||||
| S1 | 65.72 ± 04.24 | 0.40 ± 0.02 | 0.99 | 0.65 ± 0.05 | 0.99 | 100.64 ± 07.77 | 0.33 ± 0.02 | 0.97 | 1.00 ± 0.16 | 0.87 |
| S6 | 546.25 ± 19.35 | 0.36 ± 0.01 | 0.98 | 0.42 ± 0.09 | 0.94 | 438.05 ± 16.97 | 0.39 ± 0.01 | 0.99 | 0.80 ± 0.02 | 0.98 |
| S7 | 1175.36 ± 17.80 | 0.41 ± 0.01 | 0.99 | 0.04 ± 0.01 | 0.99 | 585.18 ± 36.89 | 0.48 ± 0.03 | 0.95 | 2.32 ± 0.57 | 0.89 |
| Zn (Pb) | Pb (Zn) | |||||||||
| S1 | 97.49 ± 08.23 | 0.37 ± 0.02 | 0.97 | 5.24 ± 1.47 | 0.95 | 816.52 ± 12.19 | 0.38 ± 0.01 | 1.00 | 0.04 ± 0.01 | 0.81 |
| S6 | 700.48 ± 25.97 | 0.42 ± 0.02 | 0.98 | nd | nd | nd | nd | |||
| S7 | 1277.42 ± 52.45 | 0.46 ± 0.03 | 0.94 | nd | nd | nd | nd | |||
| Zn (Cr) | Cr (Zn) | |||||||||
| S1 | 157.54 ± 18.70 | 0.39 ± 0.03 | 0.93 | −0.32 ± 0.03 | 0.97 | 209.98 ± 14.64 | 0.33 ± 0.02 | 0.97 | −0.08 ± 0.04 | 0.94 |
| S6 | 803.83 ± 22.08 | 0.47 ± 0.02 | 0.99 | −0.00 ± 0.00 | 0.96 | 5.48 ± 1.36 | 1.27 ± 0.06 | 0.98 | −5.11 ± 0.19 | 0.99 |
| S7 | 1441.87 ± 25.43 | 0.40 ± 0.01 | 0.99 | 0.00 ± 0.00 | 0.98 | 0.94 ± 0.19 | 1.51 ± 0.04 | 0.99 | −8.34 ± 1.24 | 0.99 |
| Ni (Pb) | Pb (Ni) | |||||||||
| S1 | 138.03 ± 21.84 | 0.30 ± 0.04 | 0.83 | 13.18 ± 1.10 | 0.98 | 772.02 ± 15.88 | 0.39 ± 0.01 | 0.99 | 0.05 ± 0.01 | 0.84 |
| S6 | 444.12 ± 28.35 | 0.51 ± 0.03 | 0.96 | nd | nd | nd | nd | |||
| S7 | 737.65 ± 36.46 | 0.52 ± 0.03 | 0.96 | nd | nd | nd | nd | |||
| Ni (Cr) | Cr (Ni) | |||||||||
| S1 | 203.11 ± 21.09 | 0.35 ± 0.03 | 0.94 | −0.14 ± 0.07 | 0.92 | 228.67 ± 18.09 | 0.35 ± 0.02 | 0.96 | −0.14 ± 0.04 | 0.89 |
| S6 | 550.03 ± 22.18 | 0.48 ± 0.02 | 0.98 | −0.00 ± 0.00 | 0.99 | 3.26 ± 0.92 | 1.37 ± 0.07 | 0.98 | −2.58 ± 0.10 | 0.992 |
| S7 | 768.96 ± 14.08 | 0.49 ± 0.01 | 0.99 | 0.01 ± 0.00 | 0.95 | 1.62 ± 0.36 | 1.35 ± 0.05 | 0.99 | −0.17 ± 0.73 | 0.98 |
| Pb (Cr) | Cr (Pb) | |||||||||
| S1 | 0.00 ± 0.00 | 0.76 | 238.33 ± 13.31 | 0.40 ± 0.02 | 0.98 | NA | NA | |||
| S6 | nd | nd | 44.14 ± 4.16 | 0.77 ± 0.02 | 0.99 | nd | nd | |||
| S7 | nd | nd | 4.05 ± 0.79 | 1.28 ± 0.05 | 0.99 | nd | nd | |||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Campillo-Cora, C.; Conde-Cid, M.; Arias-Estévez, M.; Fernández-Calviño, D.; Alonso-Vega, F. Specific Adsorption of Heavy Metals in Soils: Individual and Competitive Experiments. Agronomy 2020, 10, 1113. https://doi.org/10.3390/agronomy10081113
Campillo-Cora C, Conde-Cid M, Arias-Estévez M, Fernández-Calviño D, Alonso-Vega F. Specific Adsorption of Heavy Metals in Soils: Individual and Competitive Experiments. Agronomy. 2020; 10(8):1113. https://doi.org/10.3390/agronomy10081113
Chicago/Turabian StyleCampillo-Cora, Claudia, Manuel Conde-Cid, Manuel Arias-Estévez, David Fernández-Calviño, and Flora Alonso-Vega. 2020. "Specific Adsorption of Heavy Metals in Soils: Individual and Competitive Experiments" Agronomy 10, no. 8: 1113. https://doi.org/10.3390/agronomy10081113
APA StyleCampillo-Cora, C., Conde-Cid, M., Arias-Estévez, M., Fernández-Calviño, D., & Alonso-Vega, F. (2020). Specific Adsorption of Heavy Metals in Soils: Individual and Competitive Experiments. Agronomy, 10(8), 1113. https://doi.org/10.3390/agronomy10081113








