Compliance Modeling and Kinetostatic Analysis of a Generalized 3-PSS Compliant Parallel Micro-Motion Platform
Abstract
1. Introduction
2. Structure of Micro-Motion Platform
3. The Compliance and Kinetostatic Model of the Micro-Motion Platform
3.1. Compliance Modeling
3.2. Kinetostatic Modeling
4. Finite Element Verification and Analysis
4.1. Compliance Verification
4.2. Kinetostatic Verification
4.3. Performance Analysis of Kinetostatic
5. Conclusions
- The compliance model of the micro-motion platform was established using the coordinate transformation method. The compliance model was verified through finite element simulation with an example. The results showed the relative error between theoretical calculation results and the simulation values on the main diagonal elements was less than 8%, indicating the correctness of the compliance model.
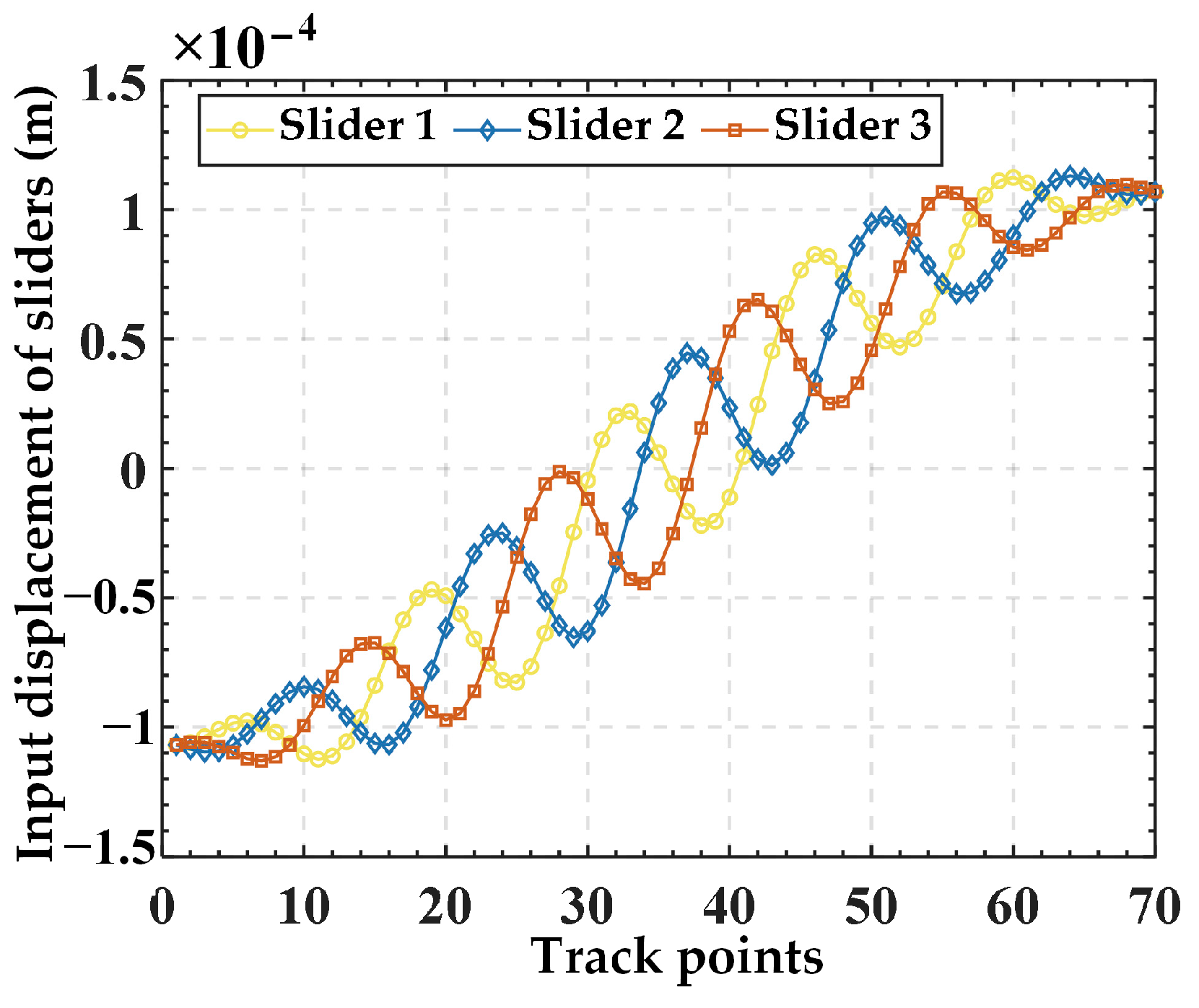
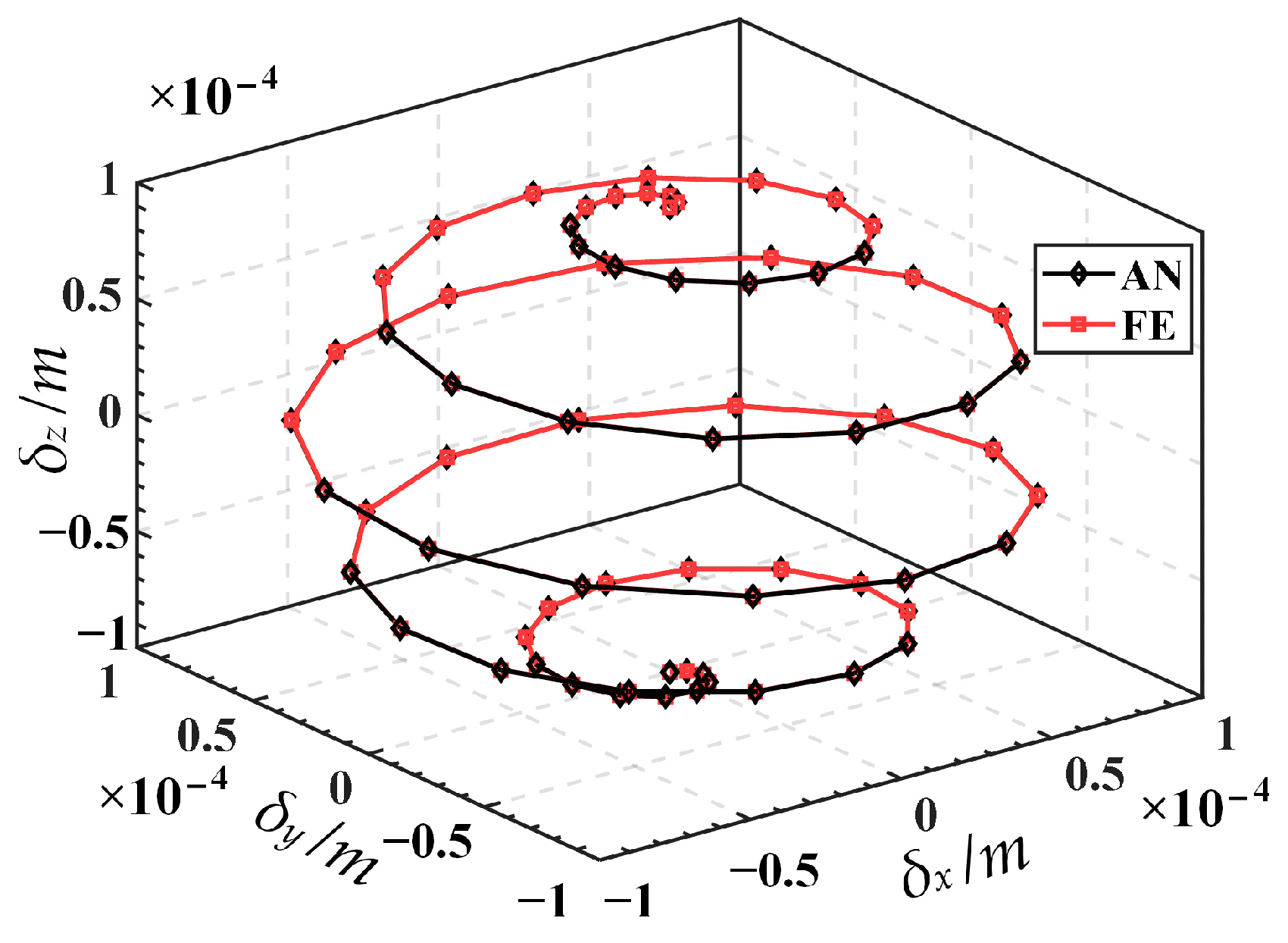
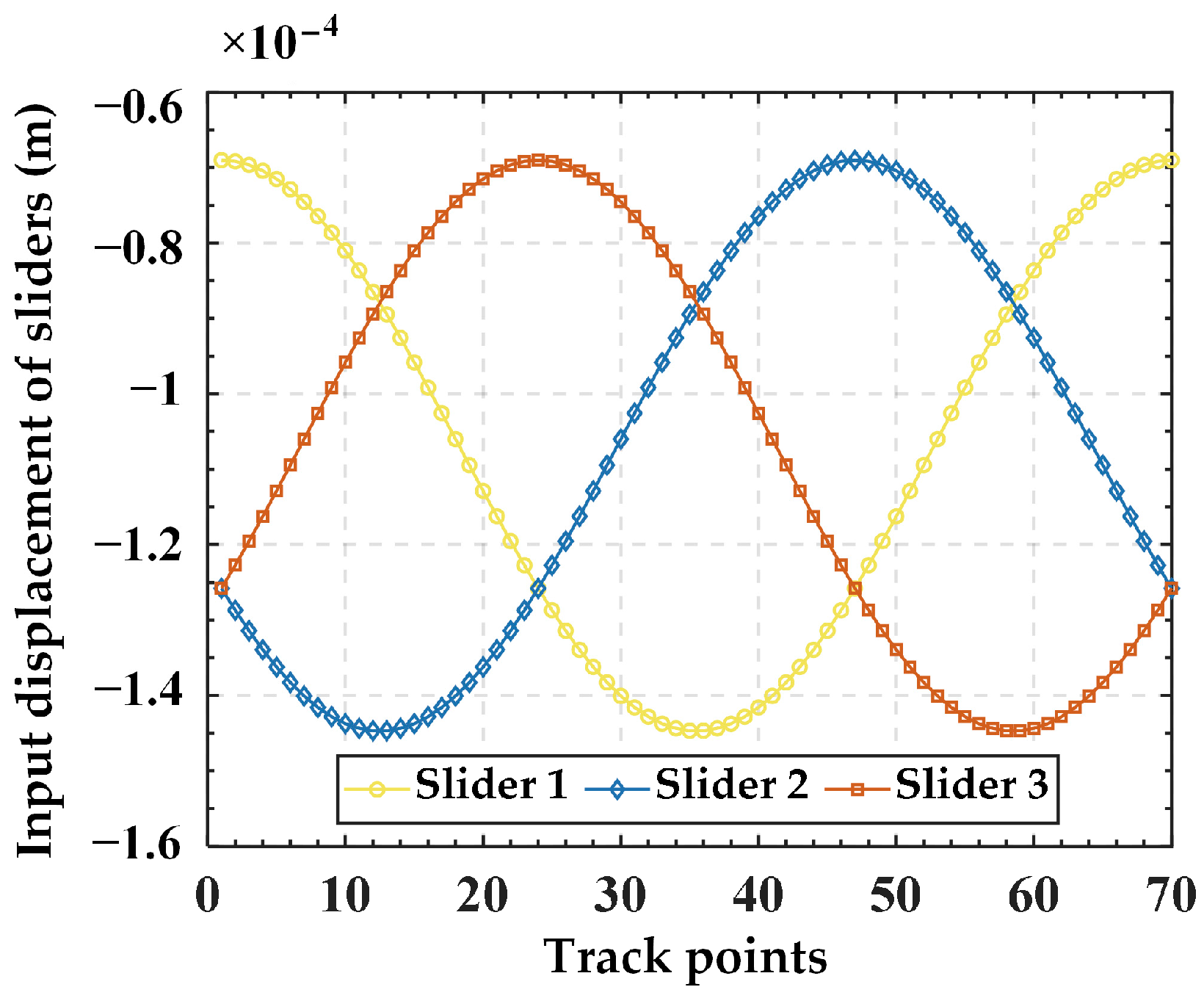
- The governing equation of the equivalent spring system for the micro-motion platform was established based on Hooke’s Law. Then, the kinetostatic model of the generalized 3-PSS compliant parallel micro-motion platform was established using the compliance matrix method. Based on this model, the mapping relationship between the input and output displacements of the micro-motion platform was further derived. Finite element simulation results demonstrated that the relative errors of displacement in the x and y directions were within 0.16%, while the relative error in the z direction was merely about 0.0004%. This strong consistency validated the correctness of the kinetostatic model of the micro-motion platform.
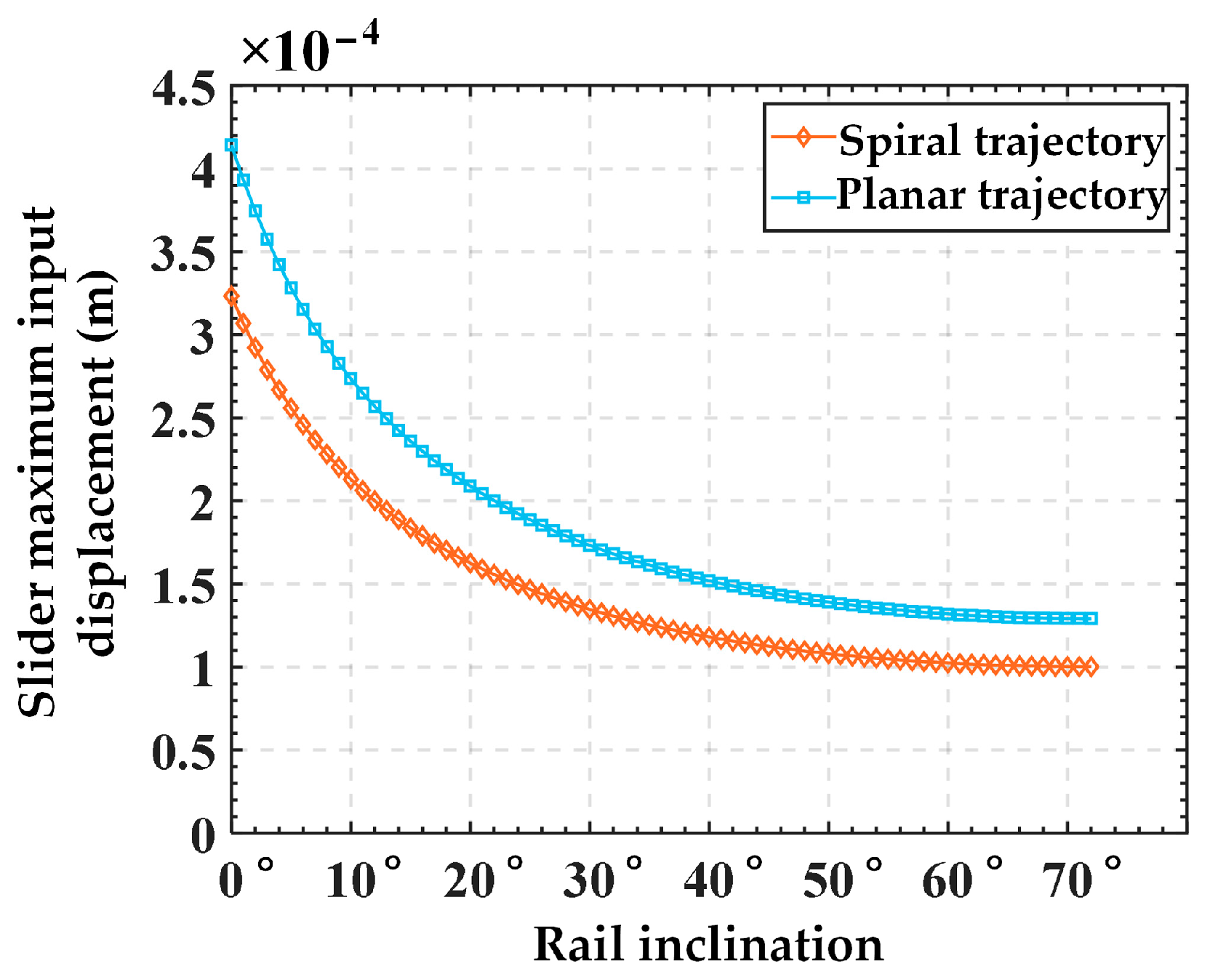
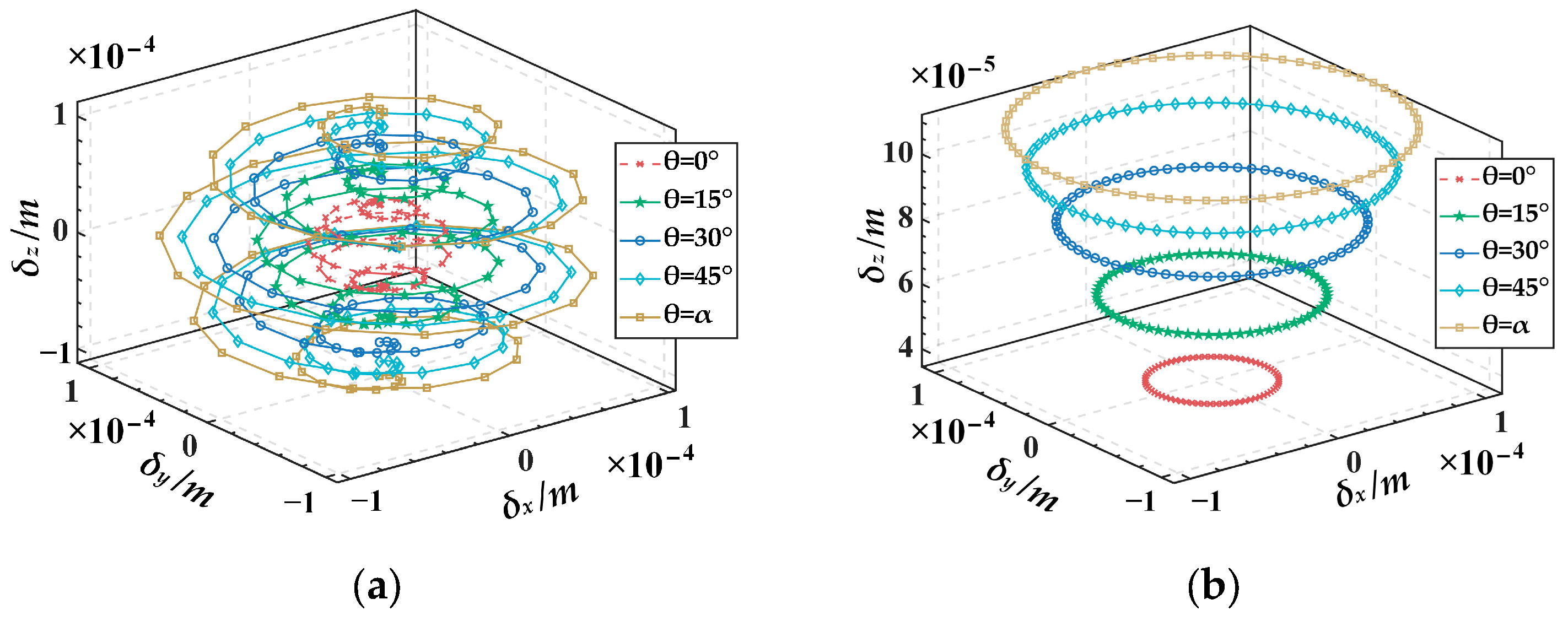
- The results on the influence of guide rail inclination angel variation on the kinetostatic performance of the micro-motion platform indicate that (1) for the same output trajectory, as the guide rail inclination angel θ increases from 0° to α (the angle between the PSS link and the fixed platform), the required maximum input displacement for the sliders gradually decreases. It can be inferred that when meeting the same platform output displacement requirements, designing a larger guide rail inclination angle allows for the use of a piezoelectric stage with a smaller stroke range (corresponding to smaller dimensions and lower costs) to fulfill the specifications. (2) Under the same input displacement, as the guide rail inclination angle gradually increases from 0° to α, the output displacements of the mobile platform in the x, y, and z directions all increase. Therefore, it can be inferred that, under the same input displacement, increasing the guide rail inclination angle can expand the workspace of the mechanism. However, this comes at the cost of sacrificing some output motion precision. This analysis provides guidance for the rational design of such micro-motion platforms.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Liu, T.; Hao, G. Design of a Cylindrical Compliant Linear Guide with Decoupling Parallelogram Mechanisms. Micromachines 2022, 13, 1275. [Google Scholar] [CrossRef] [PubMed]
- Geng, R.-R.; Mills, J.K.; Yao, Z.-Y. Design and analysis of a novel 3-DOF spatial parallel microgripper driven by LUMs. Robot. Comput.-Integr. Manuf. 2016, 42, 147–155. [Google Scholar] [CrossRef]
- Huang, G.; Zhang, D.; Zou, Q.; Ye, W.; Kong, L. Analysis and design method of a class of reconfigurable parallel mechanisms by using reconfigurable platform. Mech. Mach. Theory 2023, 181, 105215. [Google Scholar] [CrossRef]
- Plitea, N.; Lese, D.; Pisla, D.; Vaida, C. Structural design and kinematics of a new parallel reconfigurable robot. Robot. Comput.-Integr. Manuf. 2013, 29, 219–235. [Google Scholar] [CrossRef]
- Qi, Z.; Dan, Z.; Xueling, L. Type synthesis and application of a class of single DOF parallel mechanisms with one constraint couple. In Proceedings of the 2019 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Hong Kong, China, 8–12 July 2019. [Google Scholar] [CrossRef]
- Ye, W.; Chai, X.; Zhang, K. Kinematic modeling and optimization of a new reconfigurable parallel mechanism. Mech. Mach. Theory 2020, 149, 103850. [Google Scholar] [CrossRef]
- Arredondo-Soto, M.; Cuan-Urquizo, E.; Gómez-Espinosa, A.; Roman-Flores, A.; Coronado, P.D.U.; Jimenez-Martinez, M. The compliant version of the 3-RRR spherical parallel mechanism known as “Agile-Eye”: Kinetostatic analysis and parasitic displacement evaluation. Mech. Mach. Theory 2023, 180, 105160. [Google Scholar] [CrossRef]
- Lu, Q.; Chen, X.; Zheng, L.; Xu, N. A Novel Parallel Precision Stage with Large Working Range Based on Structural Parameters of Flexible Hinges. Int. J. Precis. Eng. Manuf. 2019, 21, 483–490. [Google Scholar] [CrossRef]
- Pinskier, J.; Shirinzadeh, B.; Clark, L.; Qin, Y. Development of a 4-DOF haptic micromanipulator utilizing a hybrid parallel-serial flexure mechanism. Mechatronics 2018, 50, 55–68. [Google Scholar] [CrossRef]
- Valizadeh, A.; Shariatee, M.; Sbarufatti, C. PZT Actuators’ Effect on Vibration Control of the PRRRP 2-DOF Flexible Parallel Manipulator. Shock. Vib. 2021, 2021, 6985661. [Google Scholar] [CrossRef]
- Xing, Q.; Ge, Y. Parametric study of a novel asymmetric micro-gripper mechanism. J. Adv. Mech. Des. Syst. Manuf. 2015, 9, JAMDSM0075. [Google Scholar] [CrossRef][Green Version]
- Al-Jodah, A.; Shirinzadeh, B.; Ghafarian, M.; Das, T.K.; Pinskier, J. Design, modeling, and control of a large range 3-DOF micropositioning stage. Mech. Mach. Theory 2021, 156, 104159. [Google Scholar] [CrossRef]
- Gao, J.; Han, X.; Hao, L.; Chen, L. Design and analysis of a novel large-range 3-DOF compliant parallel micromanipulator. Adv. Mech. Eng. 2021, 13. [Google Scholar] [CrossRef]
- Wang, G.; Wang, Y.; Lv, B.; Ma, R.; Liu, L. Research on a New Type of Rigid-Flexible Coupling 3-DOF Micro-Positioning Platform. Micromachines 2020, 11, 1015. [Google Scholar] [CrossRef] [PubMed]
- Chen, N.; Tian, C. Design, modeling and testing of a 3-DOF flexible piezoelectric thin sheet nanopositioner. Sens. Actuators A Phys. 2021, 323, 112660. [Google Scholar] [CrossRef]
- Ren, J.; Cao, Q. Dynamic Modeling and Frequency Characteristic Analysis of a Novel 3-PSS Flexible Parallel Micro-Manipulator. Micromachines 2021, 12, 678. [Google Scholar] [CrossRef] [PubMed]
- Ling, M.; Howell, L.L.; Cao, J.; Chen, G. Kinetostatic and Dynamic Modeling of Flexure-Based Compliant Mechanisms: A Survey. Appl. Mech. Rev. 2020, 72, 030802. [Google Scholar] [CrossRef]
- Midha, A.; Howell, L.L.; Norton, T.W. Limit positions of compliant mechanisms using the pseudo-rigid-body model concept. Mech. Mach. Theory 2000, 35, 99–115. [Google Scholar] [CrossRef]
- Lobontiu, N. Compliant Mechanisms: Design of Flexure Hinges; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar]
- Koseki, Y.; Tanikawa, T.; Koyachi, N.; Arai, T. Kinematic analysis of translational 3-DOF micro parallel mechanism using matrix method. In Proceedings of the 2000 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS 2000) (cat. No. 00CH37113), Takamatsu, Japan, 31 October–5 November 2000; pp. 786–792. [Google Scholar]
- Venkiteswaran, V.K.; Su, H.-J. A Versatile 3R Pseudo-Rigid-Body Model for Initially Curved and Straight Compliant Beams of Uniform Cross Section. J. Mech. Des. 2018, 140, 092305. [Google Scholar] [CrossRef]
- Chen, G.; Ma, F.; Bai, R.; Zhu, W.; Magleby, S.P.; Howell, L.L. An Energy-Based Framework for Nonlinear Kinetostatic Modeling of Compliant Mechanisms Utilizing Beam Flexures. J. Comput. Inf. Sci. Eng. 2021, 21, 064501. [Google Scholar] [CrossRef]
- Ren, J.; Wu, H. Kinematic Modeling of a Novel 3-PSS/S Flexible Parallel Micro-turntable: Accuracy Comparison Between Pseudo-Rigid-Body Model-Based and Compliance Matrix Method-Based Models. Iran. J. Sci. Technol. Trans. Mech. Eng. 2023, 47, 2071–2088. [Google Scholar] [CrossRef]
- Ren, J.; Li, Q. Analysis of Compliance and Kinetostatic of a Novel Class of n-4R Compliant Parallel Micro Pointing Mechanism. Micromachines 2022, 13, 1014. [Google Scholar] [CrossRef] [PubMed]
- Arredondo-Soto, M.; Cuan-Urquizo, E.; Gómez-Espinosa, A. The compliance matrix method for the kinetostatic analysis of flexure-based compliant parallel mechanisms: Conventions and general force–displacement cases. Mech. Mach. Theory 2022, 168, 104583. [Google Scholar] [CrossRef]
- Yao, J.; Li, L.; Yang, W. Analytical calculation of compliance matrix for right-circular flexure spherical hinge. Opt. Precis. Eng. 2014, 22, 1857. [Google Scholar] [CrossRef]

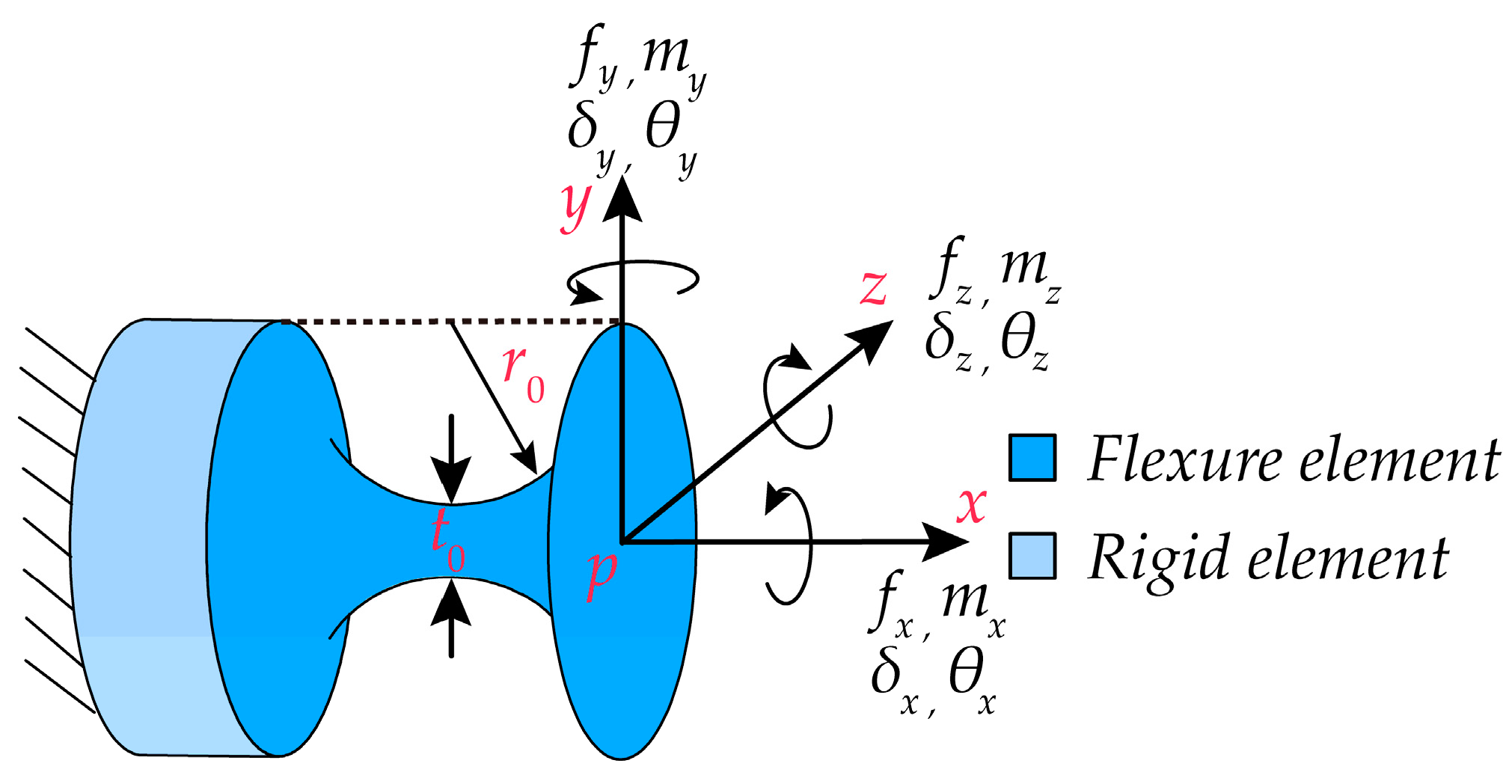
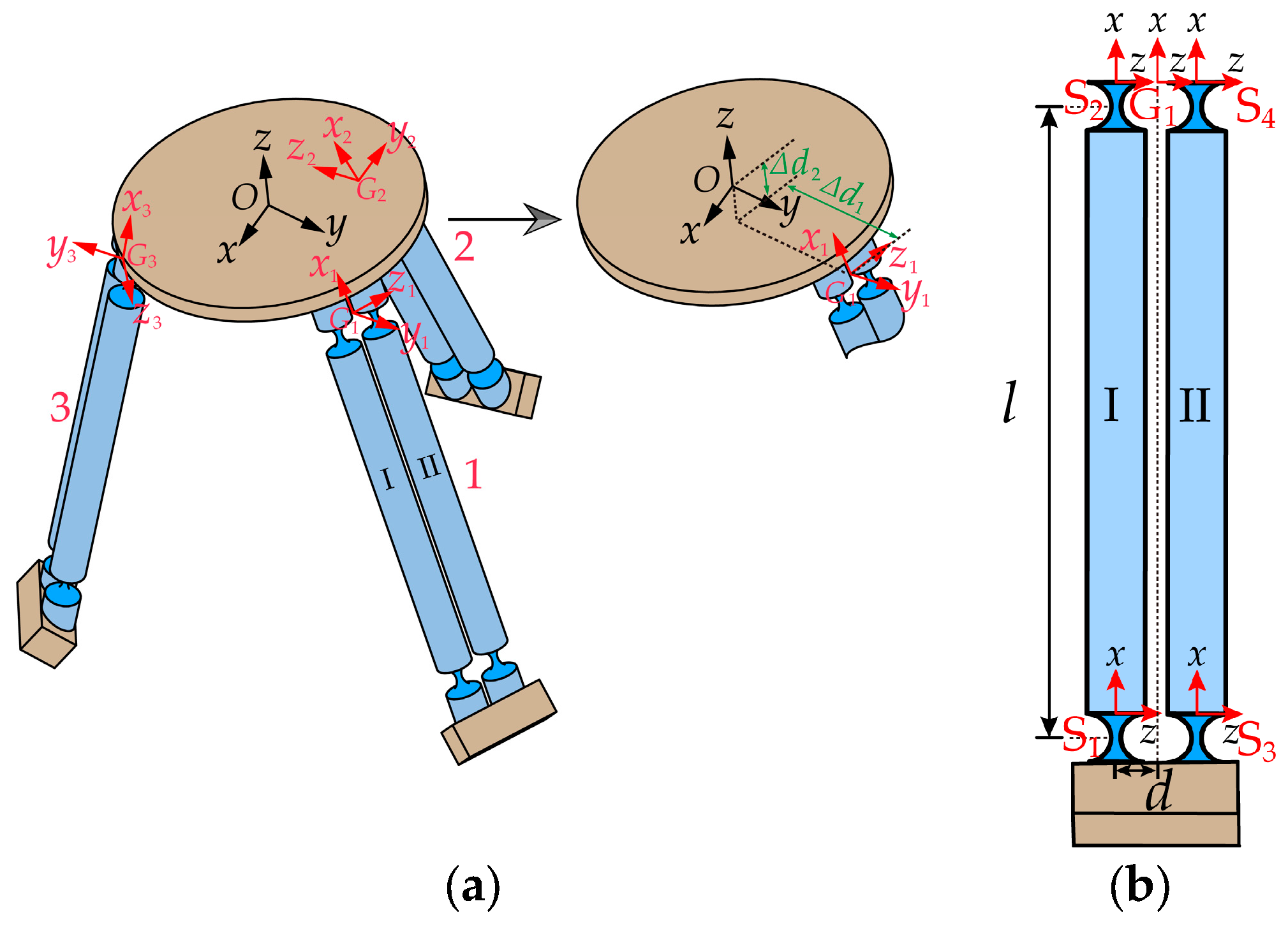

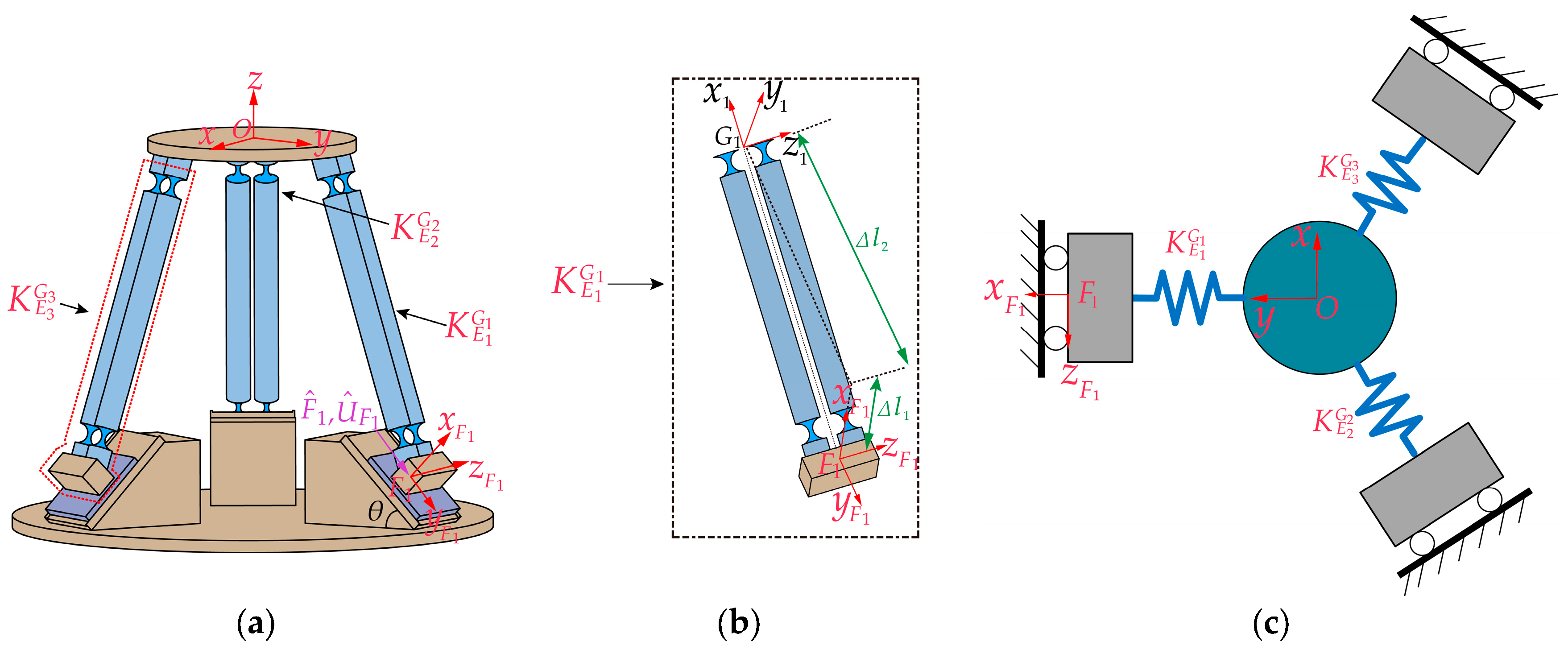
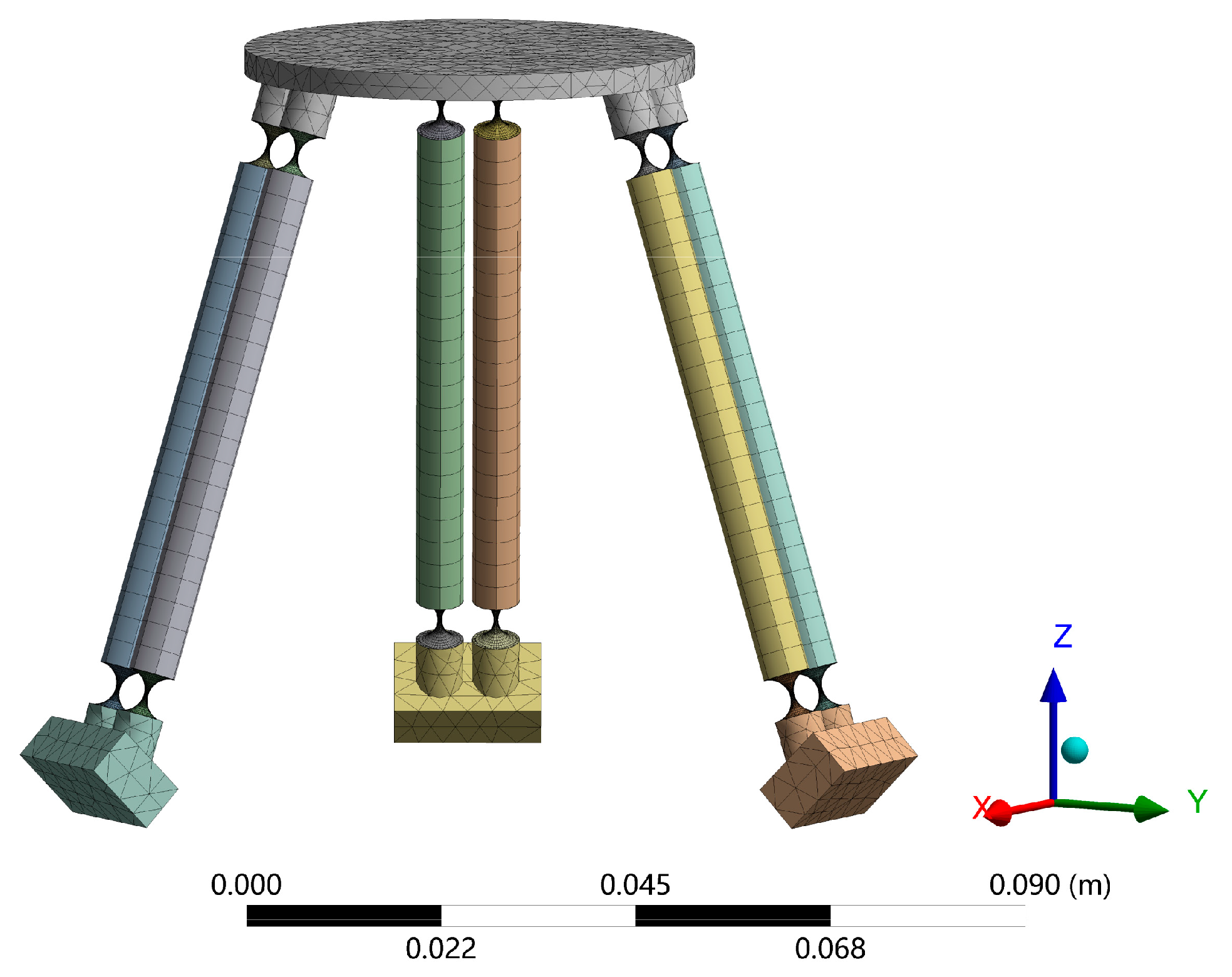

| Parameter | Value (mm) | Parameter | Value (mm) |
|---|---|---|---|
| 25 | 65 | ||
| 45 | 3.5 |
| Density ρ/(kg/m3) | Elastic Modulus E/(Gpa) | Poisson’s Ratio ν | Minimum Thickness (mm) | Cutting Radius (mm) |
|---|---|---|---|---|
| 8000 | 128 | 0.3 | 1 | 2.5 |
| Compliance | AN | FE | Relative Error (%) |
|---|---|---|---|
| (rad/(N·m)) | 1.0912 × 10−3 | 1.1747 × 10−3 | 7.108 |
| (rad/(N·m)) | 1.0912 × 10−3 | 1.1747 × 10−3 | 7.108 |
| (rad/(N·m)) | 5.4606 × 10−3 | 5.8785 × 10−3 | 7.109 |
| (m/N) | 4.9958 × 10−6 | 5.3785 × 10−6 | 7.115 |
| (m/N) | 4.9958 × 10−6 | 5.3785 × 10−6 | 7.115 |
| (m/N) | 8.0793 × 10−9 | 8.7215 × 10−9 | 7.363 |
| Parameter | Value (mm) | Parameter | Value (mm) |
|---|---|---|---|
| 24.23 | −8.12 | ||
| 37.55 | −69.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, J.; Lan, A. Compliance Modeling and Kinetostatic Analysis of a Generalized 3-PSS Compliant Parallel Micro-Motion Platform. Micromachines 2024, 15, 354. https://doi.org/10.3390/mi15030354
Ren J, Lan A. Compliance Modeling and Kinetostatic Analysis of a Generalized 3-PSS Compliant Parallel Micro-Motion Platform. Micromachines. 2024; 15(3):354. https://doi.org/10.3390/mi15030354
Chicago/Turabian StyleRen, Jun, and Aojie Lan. 2024. "Compliance Modeling and Kinetostatic Analysis of a Generalized 3-PSS Compliant Parallel Micro-Motion Platform" Micromachines 15, no. 3: 354. https://doi.org/10.3390/mi15030354
APA StyleRen, J., & Lan, A. (2024). Compliance Modeling and Kinetostatic Analysis of a Generalized 3-PSS Compliant Parallel Micro-Motion Platform. Micromachines, 15(3), 354. https://doi.org/10.3390/mi15030354





