Analytical Approach for a Heat Transfer Process through Nanofluid over an Irregular Porous Radially Moving Sheet by Employing KKL Correlation with Magnetic and Radiation Effects: Applications to Thermal System
Abstract
:1. Introduction
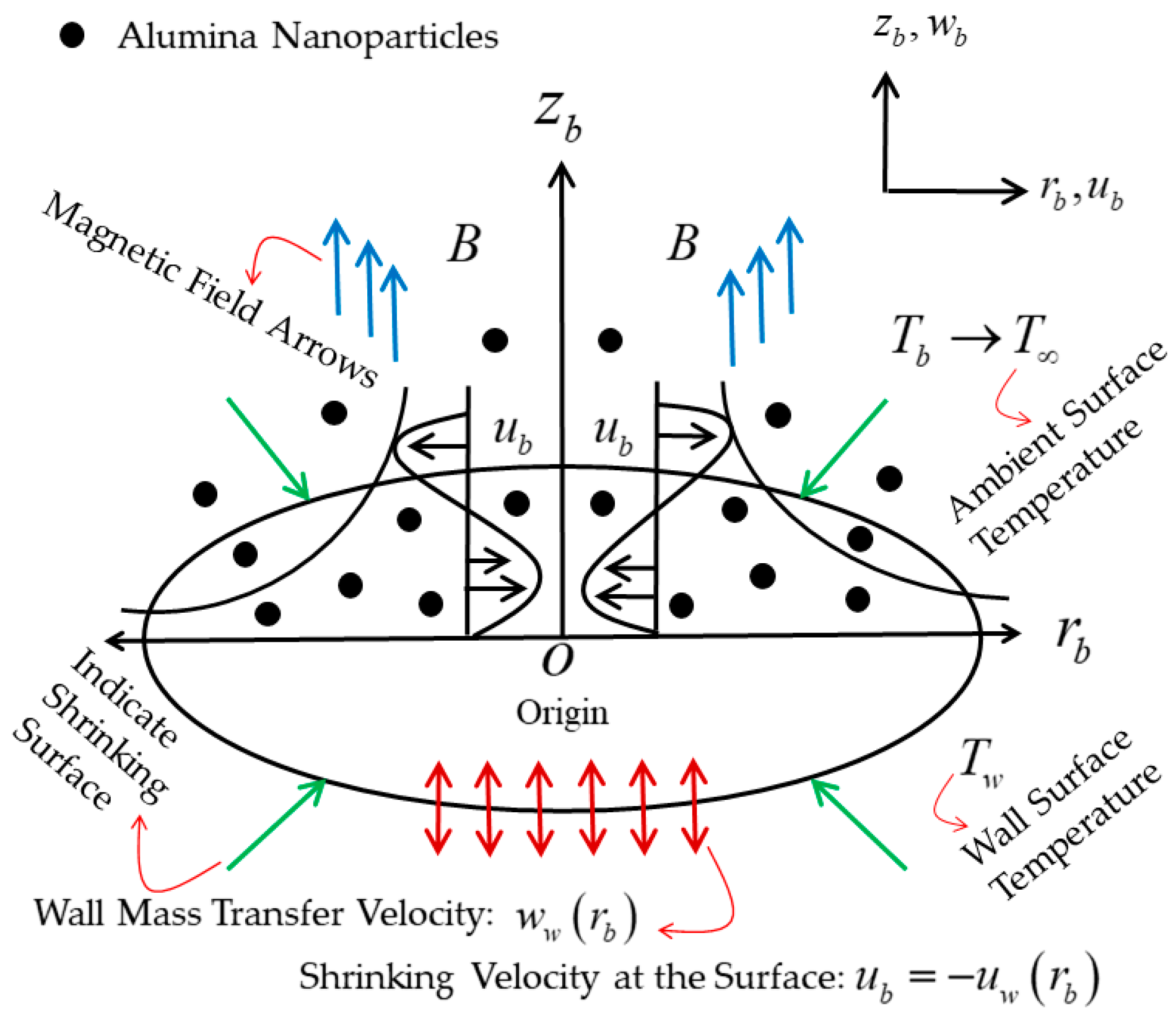
2. Mathematical Modeling
2.1. Constitutive Equation
2.2. Basic Equations
2.3. Non-Dimensional Equations
2.4. Gradients
3. Analytic Solutions Methodology
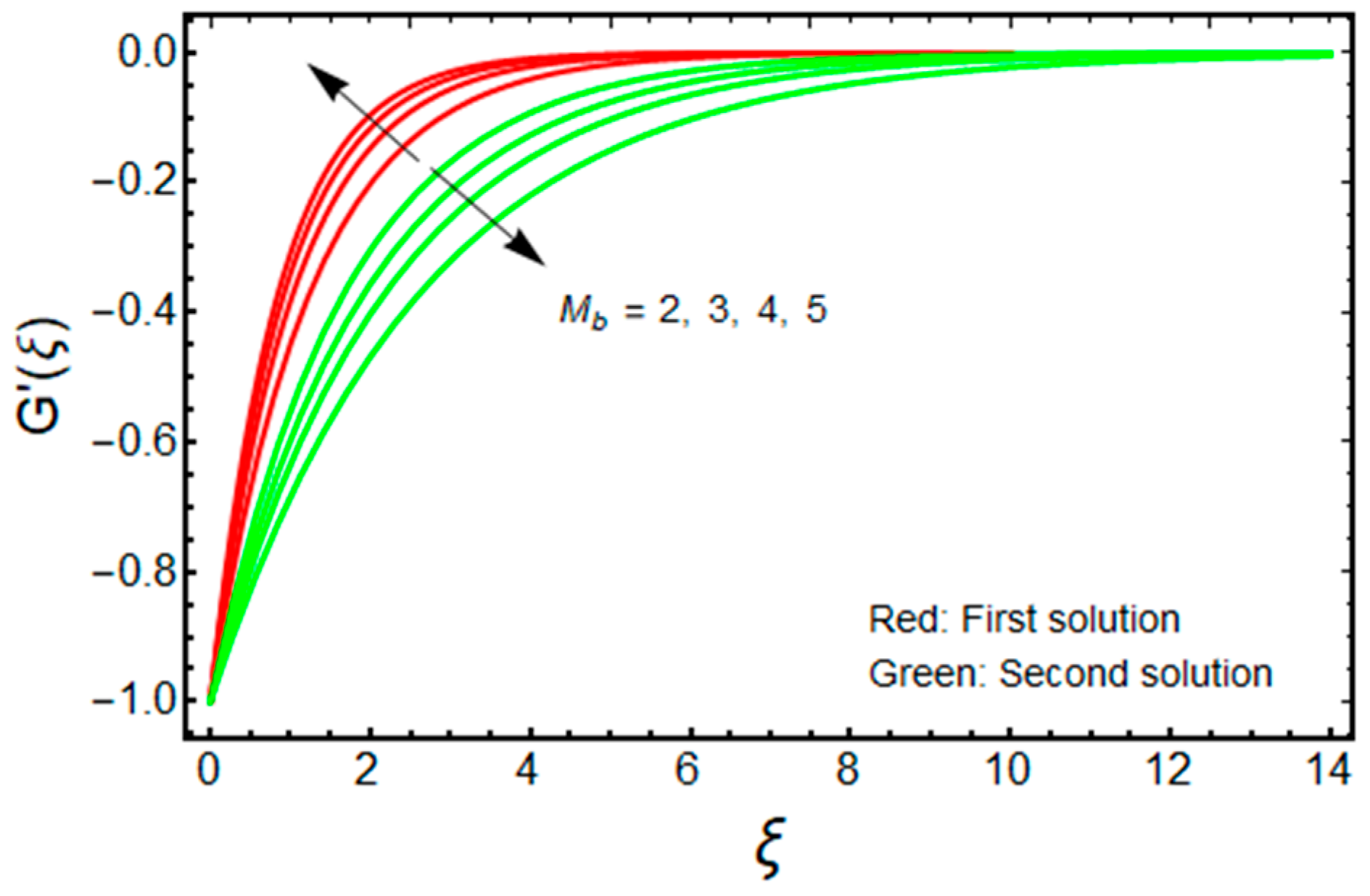
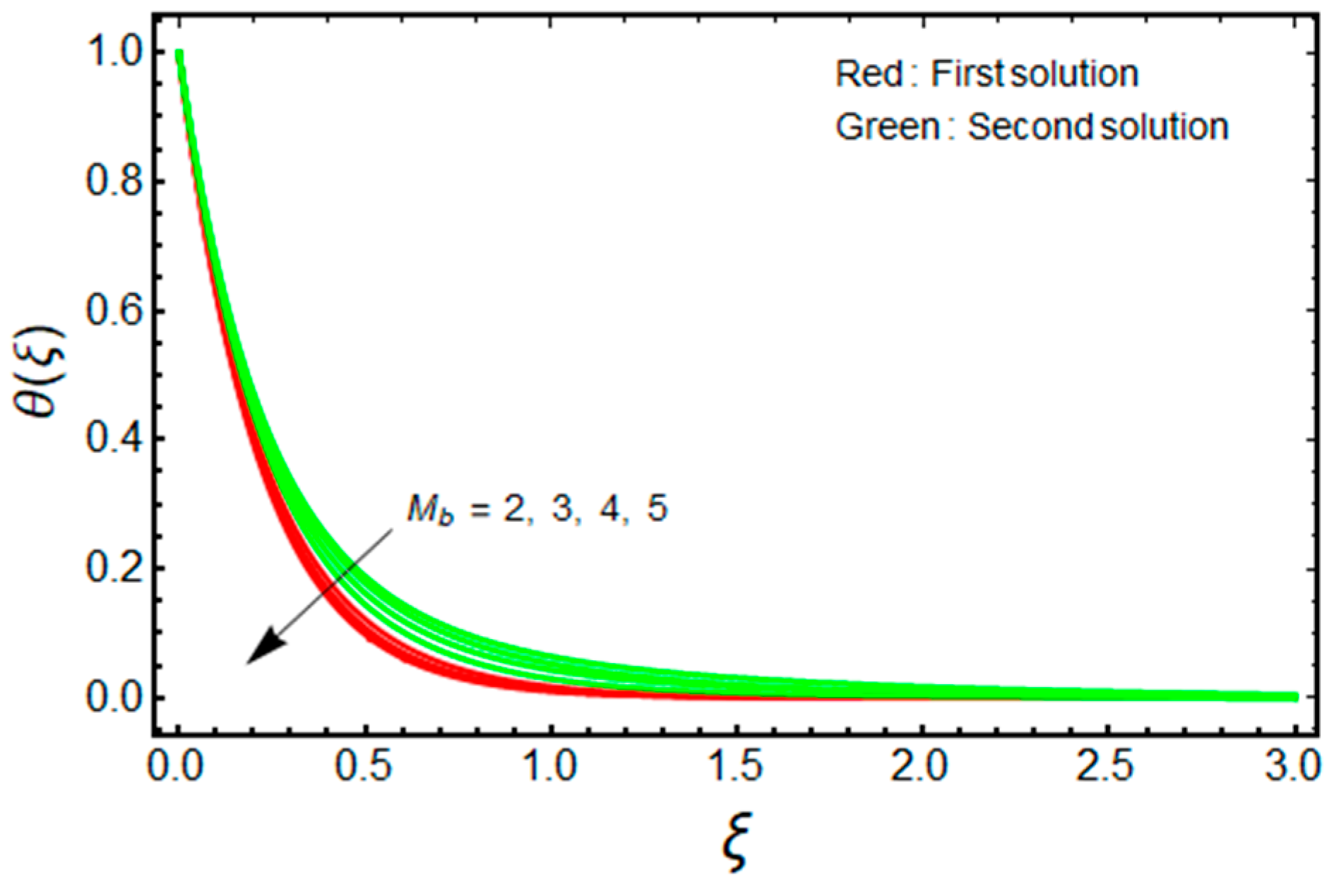
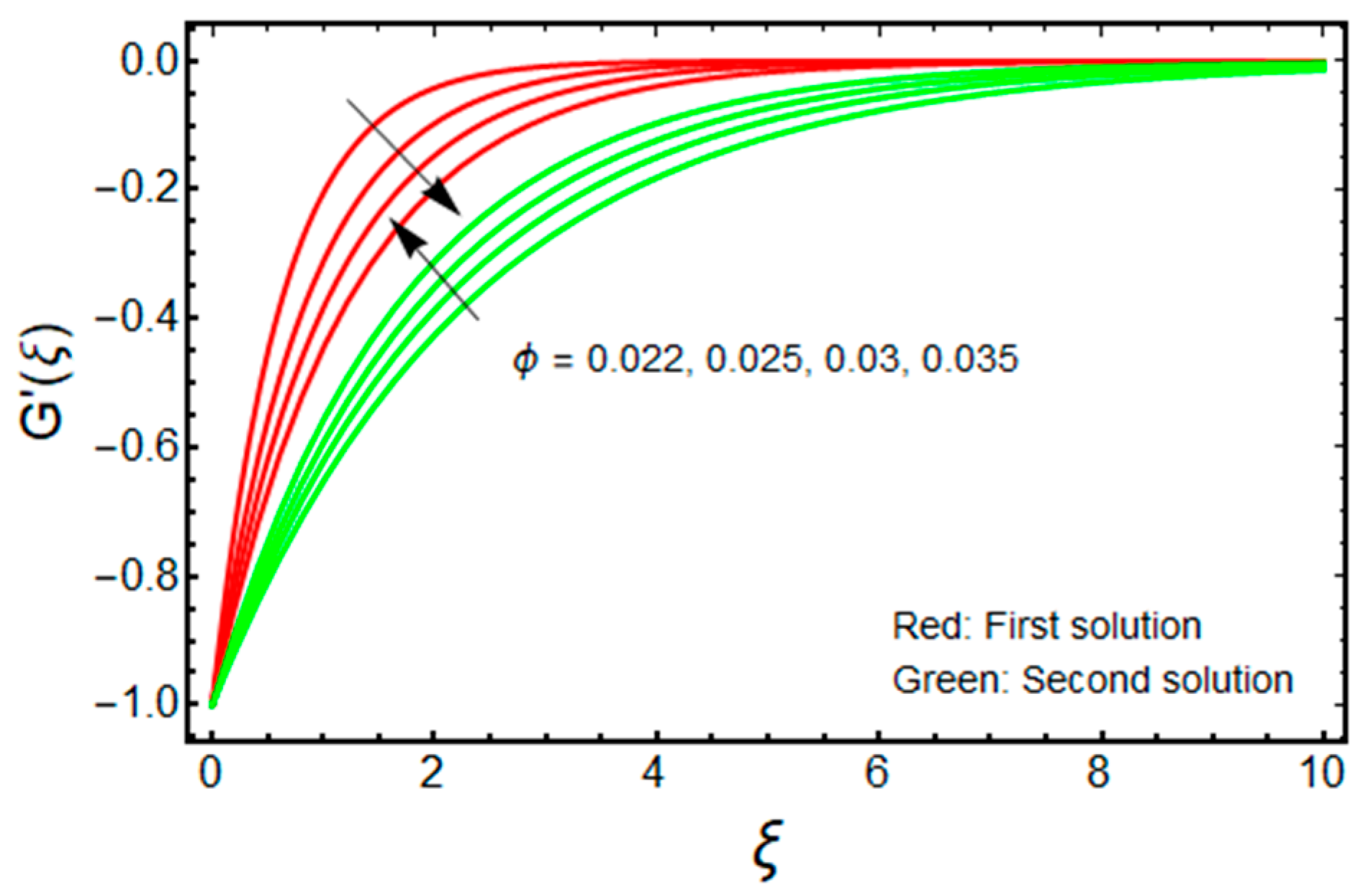
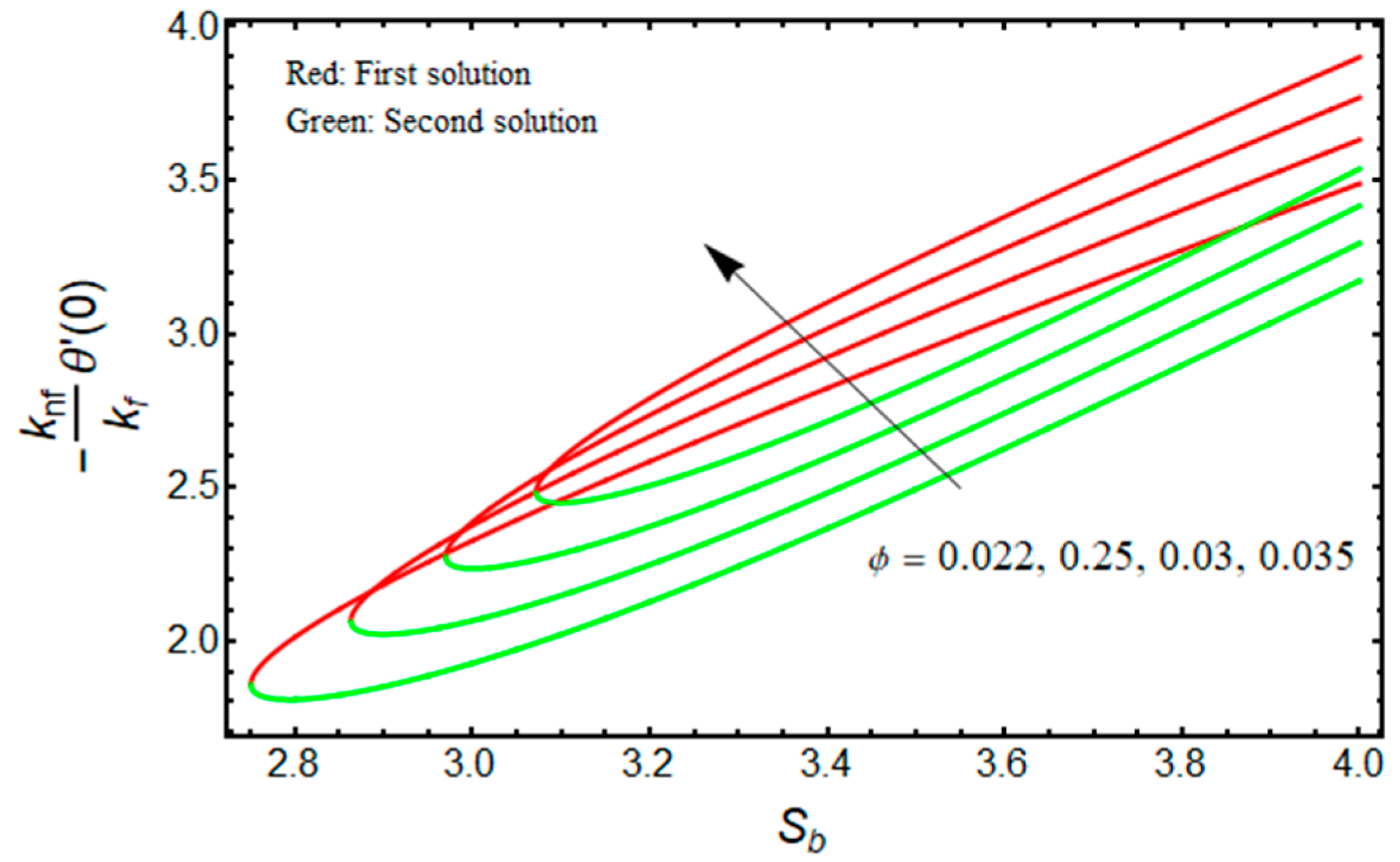
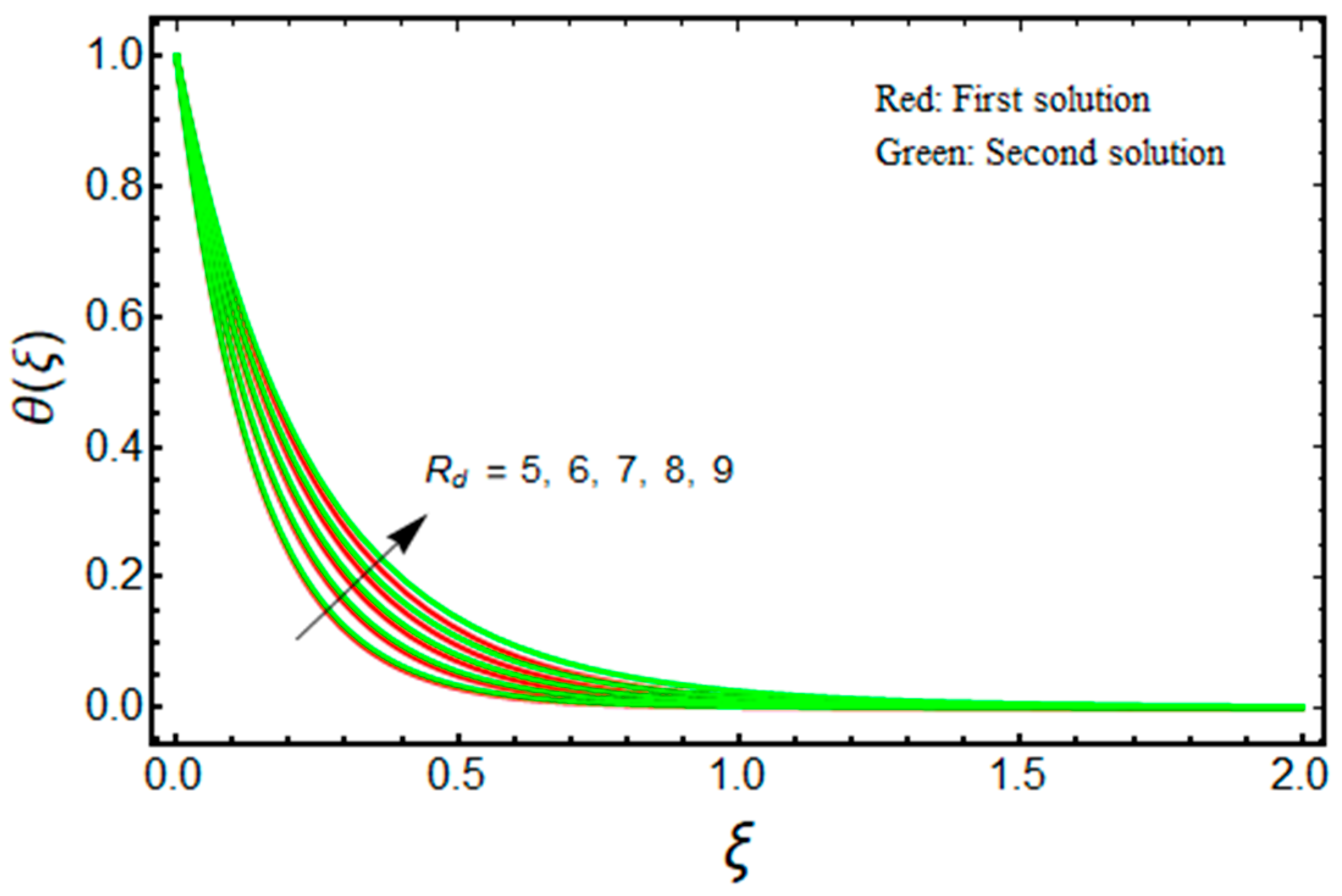
4. Results and Discussion
5. Conclusions
- The suction and magnetic parameters accelerate the velocity in the first branch solution and decelerate in the second branch solution, whilst declining the temperature distribution in both branch solutions.
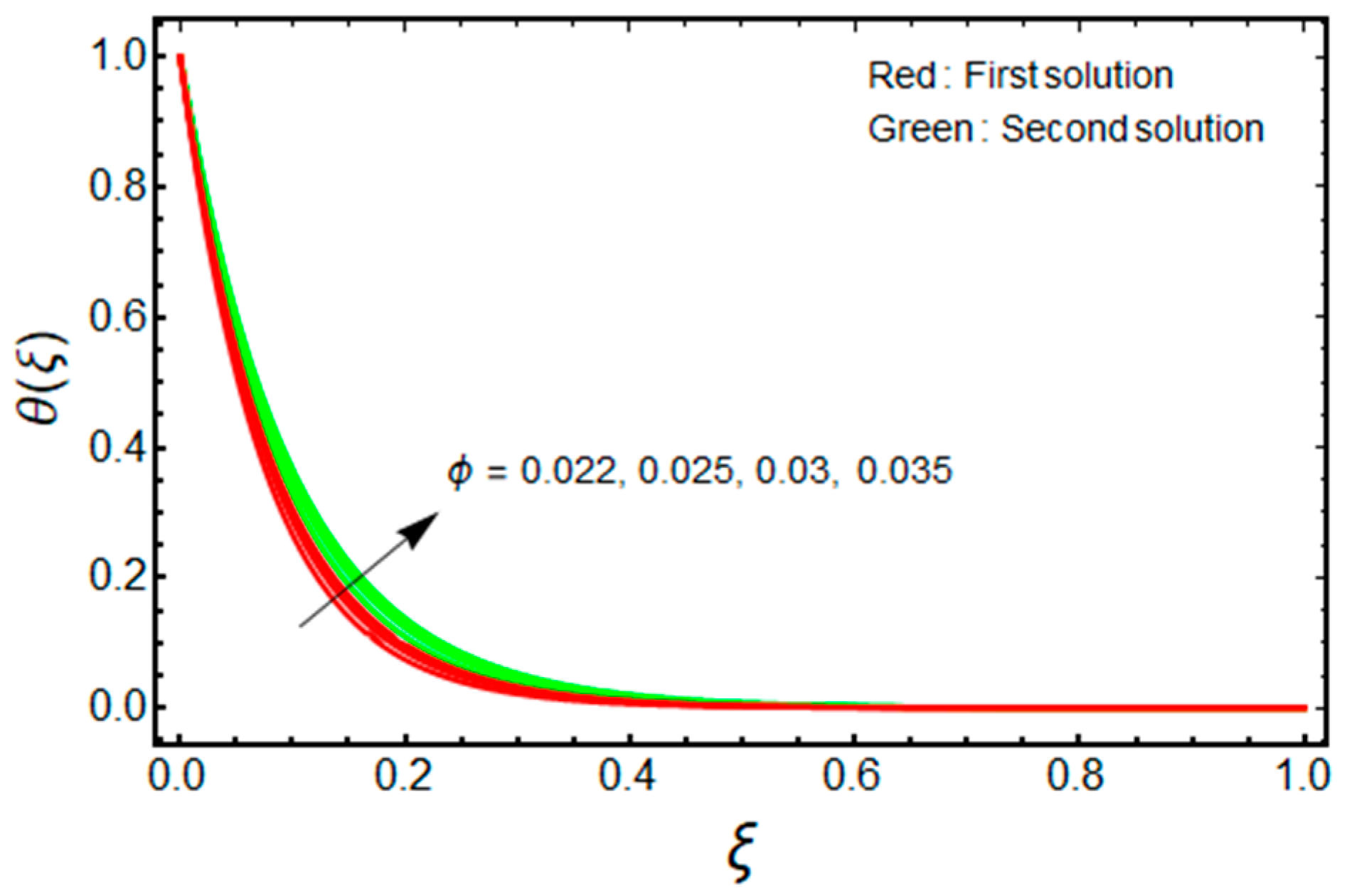
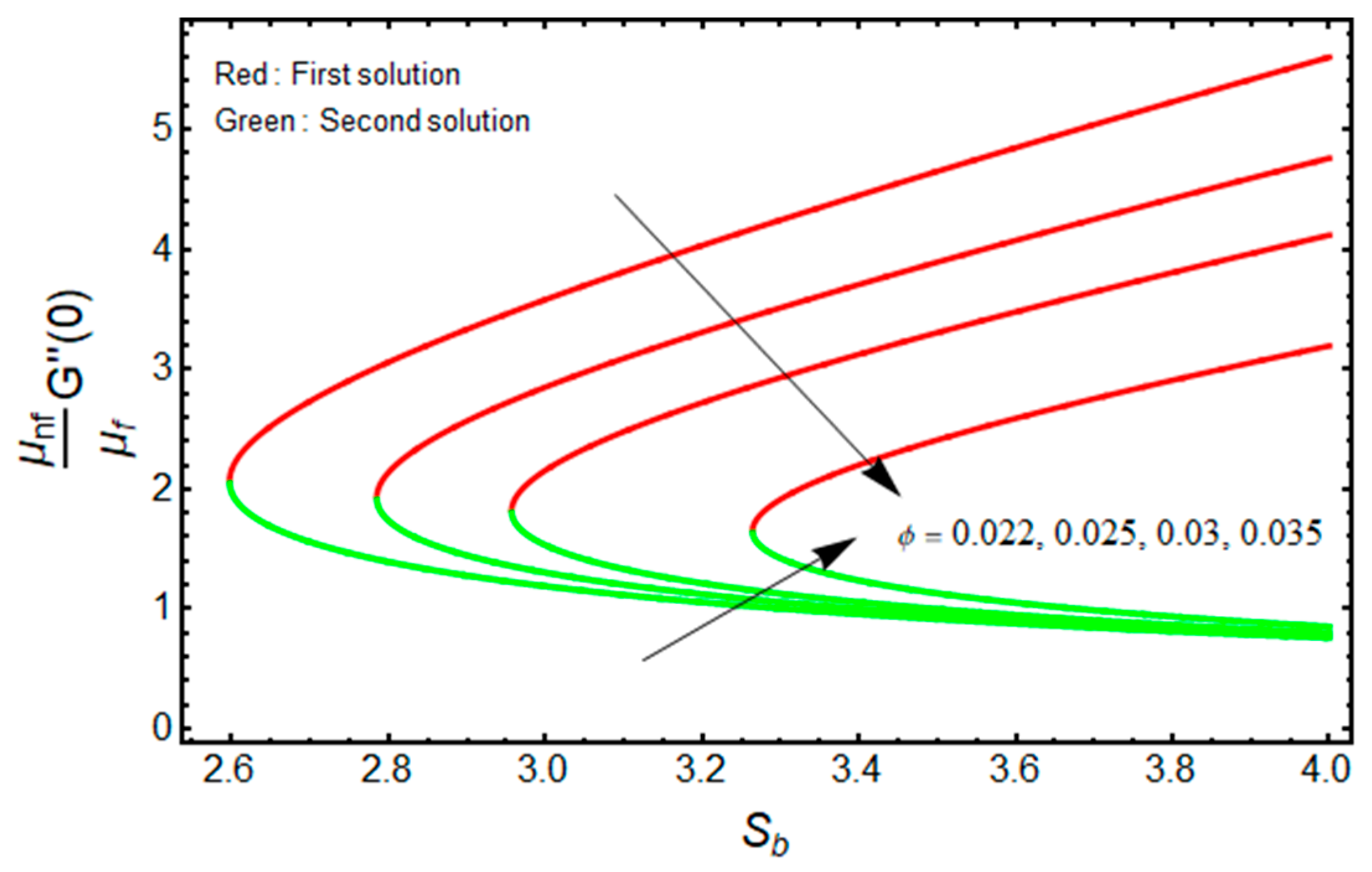
- The temperature profile uplifts due to the solid nanoparticle volume fraction in both solutions, while the velocity increases and decreases due to the solid nanoparticle volume fraction in the first and second branch solutions, respectively.
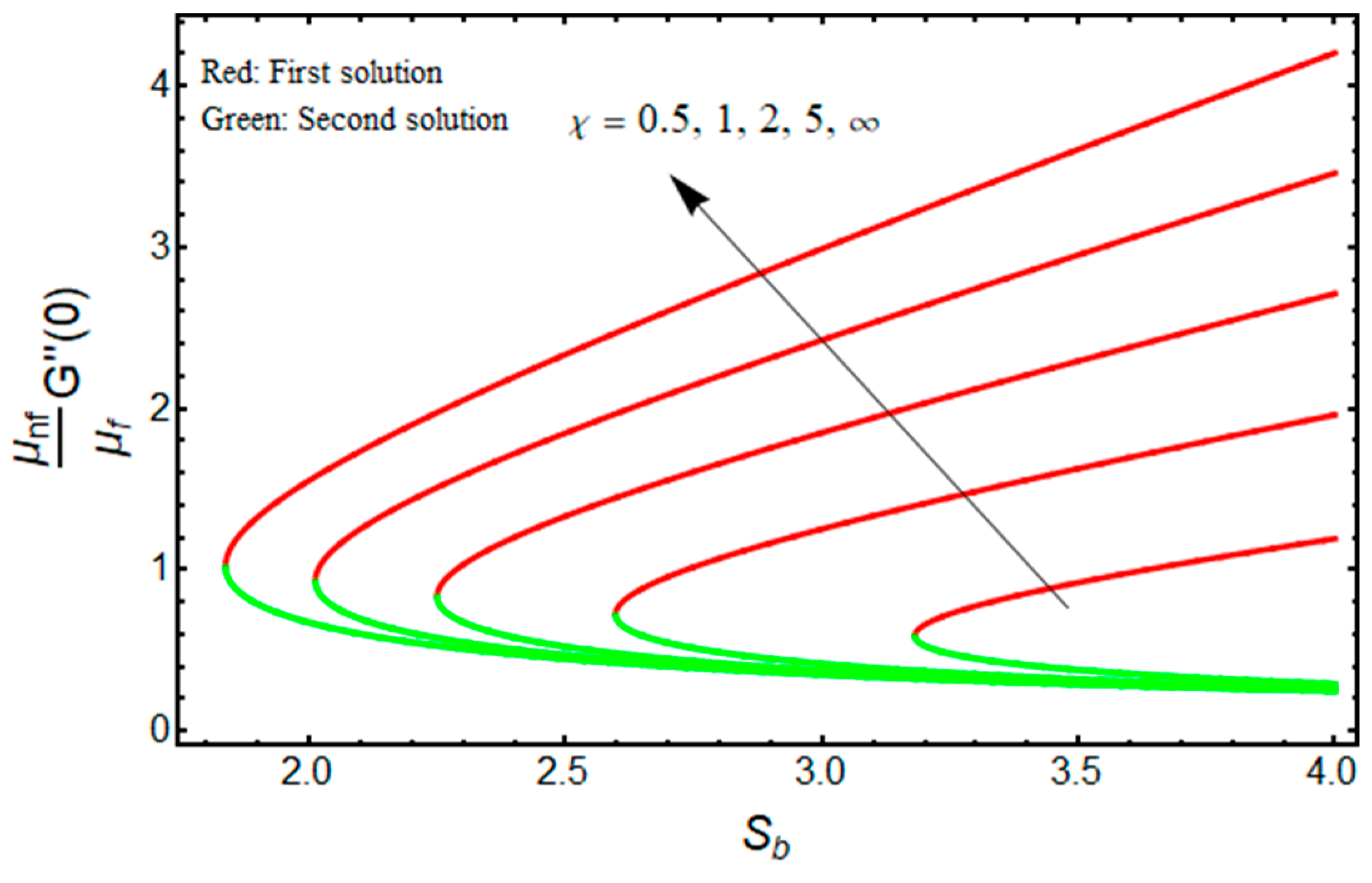
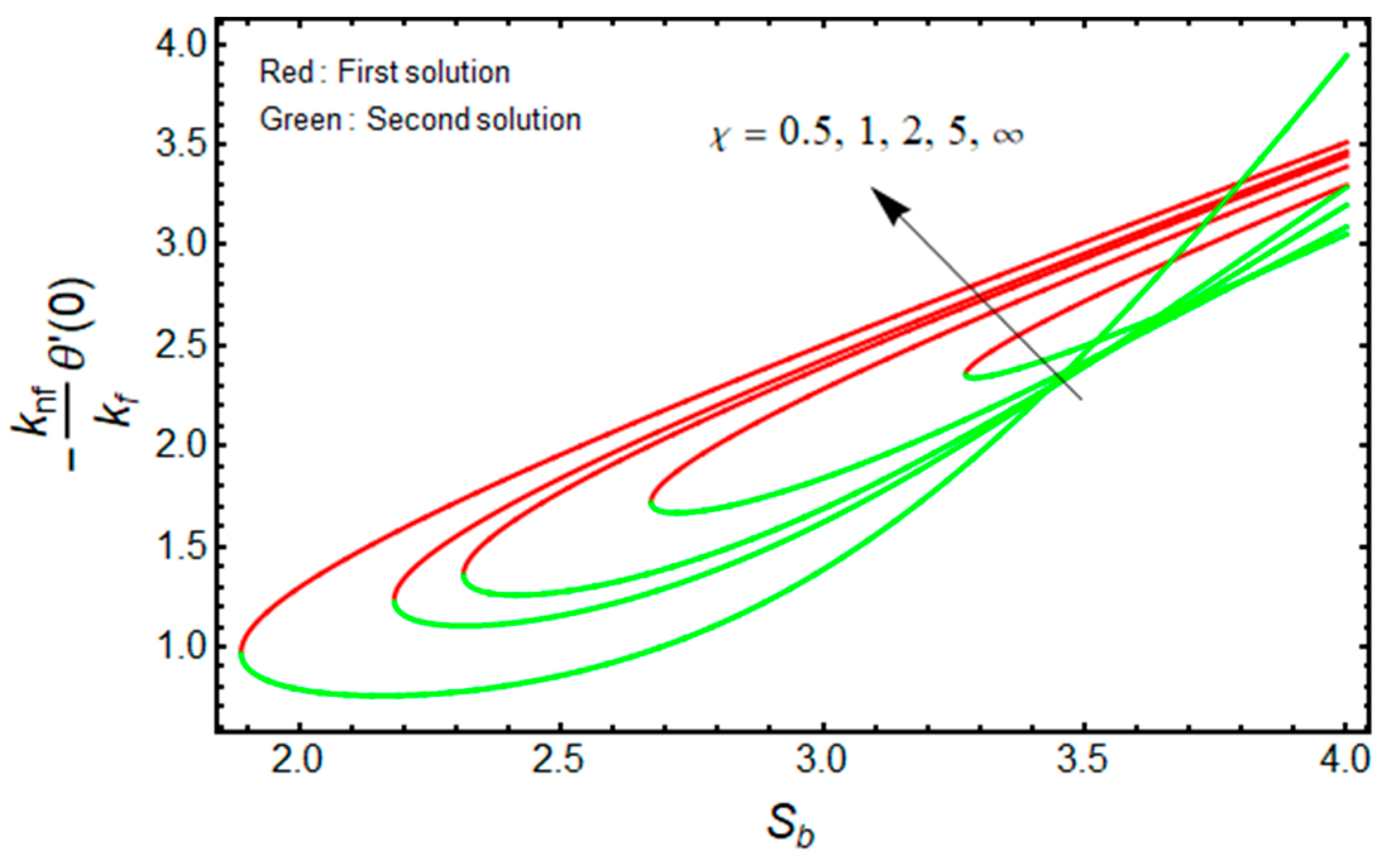
- The heat transfer and the friction factor increase due to the Casson parameter.
- The nanoparticle volume fraction augments the heat transfer and declines the friction factor.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Velocity of the shrinking disk (m/s) | |
| Variable magnetic field strength (Tesla) | |
| Constant magnetic field strength (Tesla) | |
| Wall mass transfer velocity (m/s) | |
| Wall temperature (K) | |
| Arbitrary constants | |
| Ambient temperature (K) | |
| Fluid temperature (K) | |
| Radiative heat flux | |
| Constant reference temperature (K) | |
| Dimensionless velocity | |
| Magnetic parameter | |
| Prandtl number | |
| Mass transfer factor | |
| Heat transfer | |
| Skin friction coefficient | |
| Reynolds number | |
| Denotes the roots of the equation | |
| Dimensionless velocity | |
| Radiation parameter | |
| Relative factor of the power-law index | |
| Mean absorption coefficient (1/m) | |
| Thermal conductivity (W/(m·K)) | |
| Velocity components along the rb- and zb-axes (m/s) | |
| Cylindrical coordinates (m) | |
| Specific heat at constant pressure (J/Kg·K) | |
| and | Arbitrary constants |
Greek Symbols
| Kinematic viscosity (m2/s) | |
| and | Empirical functions |
| Dimensionless temperature | |
| Non-Newtonian or Casson fluid parameter | |
| Electrical conductivity (Ω−1m−1) | |
| Dynamic viscosity (N·s/m2) | |
| Pseudo-similarity variable | |
| Density (kg/m3) | |
| Stefan-Boltzmann constant (W/(m2·K4)) | |
| Stream function | |
| New similarity variable | |
| Solid nanoparticle volume fraction |
Acronyms
| KKL | Koo–Kleinstreuer–Li |
| MHD | Magnetohydrodynamics |
| EOF | Electro-osmotic forces |
| Al2O3 | Alumina |
| 2D, 3D | Two and three-dimensional |
| BCs | Boundary conditions |
| BLT | Boundary layer thickness |
| BLF | Boundary layer flow |
| VD | Viscous dissipation |
| TIR | Thermal interfacial resistance |
Subscripts
| Nanoparticles | |
| Nanofluid | |
| Regular based-fluid | |
| Wall boundary condition | |
| Far-field condition |
Superscript
| ‘ | Derivative with respect to ξ |
Appendix A
References
- Choi, S.U.S. Enhancing thermal conductivity of fluids with nanoparticles. ASME Int. Mech. Eng. 1995, 66, 99–105. [Google Scholar]
- Hamad, M.A.; Pop, I. Scaling transformations for boundary layer flow near the stagnation-point on a heated permeable stretching surface in a porous medium saturated with a nanofluid and heat generation/absorption effects. Transp. Porous Med. 2010, 87, 25–39. [Google Scholar] [CrossRef]
- Makinde, O.D.; Aziz, A. Boundary layer flow of a nanofluid past a stretching sheet with a convective boundary condition. Int. J. Therm. Sci. 2011, 50, 1326–1332. [Google Scholar] [CrossRef]
- Rana, P.; Bhargava, R. Flow and heat transfer of a nanofluid over a nonlinearly stretching sheet: A numerical study. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 212–226. [Google Scholar] [CrossRef]
- Pal, D.; Mandal, G. Mixed convection-radiation on stagnation-point flow of nanofluids over a stretching/shrinking sheet in a porous medium with heat generation and viscous dissipation. J. Petrol. Sci. Eng. 2015, 126, 16–25. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Farooq, M.; Yasmeen, T.; Alsaedi, A. Water-carbon nanofluid flow with variable heat flux by a thin needle. J. Mol. Liq. 2016, 224, 786–791. [Google Scholar] [CrossRef]
- Eid, M.R. Chemical reaction effect on MHD boundary layer flow of two-phase nanofluid model over an exponentially stretching sheet with a heat generation. J. Mol. Liq. 2016, 220, 718–725. [Google Scholar] [CrossRef]
- Zaib, A.; Rashidi, M.M.; Chamkha, A.J. Flow of nanofluid containing gyrotactic microorganisms over static wedge in Darcy–brinkman porous medium with convective boundary condition. J. Porous Med. 2018, 21, 911–928. [Google Scholar] [CrossRef]
- Soomro, F.A.; Zaib, A.; Haq, R.U.; Sheikholeslami, M. Dual nature solution of water functionalized copper nanoparticles along a permeable shrinking cylinder: FDM approach. Int. J. Heat Mass Transf. 2019, 129, 1242–1249. [Google Scholar] [CrossRef]
- Khan, U.; Shafiq, A.; Zaib, A.; Baleanu, D. Hybrid nanofluid on mixed convective radiative flow from an irregular variably thick moving surface with convex and concave effects. Case Stud. Therm. Eng. 2020, 21, 100660. [Google Scholar] [CrossRef]
- Zaher, A.Z.; Ali, K.K.; Mekheimer, K.S. Electroosmosis forces EOF driven boundary layer flow for a non-Newtonian fluid with planktonic microorganism: Darcy Forchheimer model. Int. J. Num. Meth. Heat Fluid Flow 2021, 31, 2534–2559. [Google Scholar] [CrossRef]
- Gowda, R.J.P.; Kumar, R.N.; Prasannakumara, B.C.; Nagaraja, B.; Gireesha, B.J. Exploring magnetic dipole contribution on ferromagnetic nanofluid flow over a stretching sheet: An application of Stefan blowing. J. Mol. Liq. 2021, 335, 116215. [Google Scholar] [CrossRef]
- Shahid, A.; Bhatti, M.M.; Ellahi, R.; Mekheimer, K.S. Numerical experiment to examine activation energy and bi-convection Carreau nanofluid flow on an upper paraboloid porous surface: Application in solar energy. Sustain. Energy Technol. Assess. 2022, 52, 102029. [Google Scholar] [CrossRef]
- Mukhopaday, S. Casson fluid flow and heat transfer over a nonlinearly stretching surface. Chin. Phys. B 2013, 22, 074701. [Google Scholar] [CrossRef]
- Nadeem, S.; Haq, R.U.; Lee, C. MHD flow of a Casson fluid over an exponentially shrinking sheet. Sci. Iran. 2012, 19, 1550–1553. [Google Scholar] [CrossRef] [Green Version]
- Pramanik, S. Casson fluid flow and heat transfer past an exponentially porous stretching surface in presence of thermal radiation. Ain Shams Eng. J. 2013, 1, 205–212. [Google Scholar] [CrossRef] [Green Version]
- Haq, R.U.; Nadeem, S.; Khan, Z.H.; Okedayo, T.G. Convective heat transfer and MHD effects on Casson nanofluid flow over a shrinking sheet. Cent. Eur. J. Phys. 2014, 12, 862–871. [Google Scholar] [CrossRef]
- Makinde, O.D.; Sandeep, N.; Ajayi, T.M.; Animasaun, I.L. Numerical exploration of heat transfer and Lorentz force effects on the flow of MHD Casson fluid over an upper horizontal surface of a thermally stratified melting surface of a paraboloid of revolution. Int. J. Nonlinear Sci. Numer. Simul. 2018, 19, 93–106. [Google Scholar] [CrossRef]
- Faraz, F.; Imran, S.M.; Ali, B.; Haider, S. Thermo-Diffusion and multi-slip effect on anaxisymmetric Casson flow over a unsteady radially stretching sheet in the presence of chemical reaction. Processes 2019, 7, 851. [Google Scholar] [CrossRef] [Green Version]
- Khan, U.; Bilal, S.; Zaib, A.; Makinde, O.D.; Wakif, A. Numerical simulation of a nonlinear coupled differential system describing a convective flow of Casson gold–blood nanofluid through a stretched rotating rigid disk in the presence of Lorentz forces and nonlinear thermal radiation. Numer. Methods Partial Diff. Eq. 2022, 38, 308–328. [Google Scholar] [CrossRef]
- Zaib, A.; Haq, R.U.; Chamkha, A.J. Mixed convective flow of a Casson fluid over a vertical plate in Darcy–Brinkman porous medium with slips. J. Porous Med. 2021, 24, 1–12. [Google Scholar] [CrossRef]
- Jyothi, A.M.; Kumar, R.N.; Gowda, R.J.P.; Veeranna, Y.; Prasannakumara, B.C. Impact of activation energy and gyrotactic microorganisms on flow of Casson hybrid nanofluid over a rotating moving disk. Heat Transf. 2021, 96, 075210. [Google Scholar] [CrossRef]
- Hossain, M.A.; Takhar, H.S. Radiation effect on mixed convection along a vertical plate with uniform surface temperature. Int. J. Heat Mass Transf. 1996, 31, 243–248. [Google Scholar] [CrossRef]
- Takhar, H.S.; Gorla, R.S.R.; Soundalgekar, V.M. Radiation effects on MHD free convection flow of a gas past a semi-infinite vertical plate. Int. J. Numer. Methods Heat Fluid Flow 1996, 6, 77–83. [Google Scholar] [CrossRef]
- Hossain, M.A.; Alim, M.A.; Rees, D.A. The effect of radiation on free convection from a porous vertical plate. Int. J. Heat Mass Transf. 1999, 42, 181–191. [Google Scholar] [CrossRef]
- Hayat, T.; Qasim, M. Radiation and magnetic field effects on the unsteady mixed convection flow of a second grade fluid over a vertical stretching sheet. Int. J. Numer. Methods Fluids 2011, 66, 820–832. [Google Scholar] [CrossRef]
- Mukhopadhyay, S.; Bhattacharyya, K.; Layek, G.C. Steady boundary layer flow and heat transfer over a porous moving plate in presence of thermal radiation. Int. J. Heat Mass Transf. 2011, 54, 2751–2757. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Shah, F. Thermal radiation on unsteady electrical MHD flow of nanofluid over stretching sheet with chemical reaction. J. King Saud Univ. Sci. 2019, 31, 804–812. [Google Scholar] [CrossRef]
- Mekheimer, K.S.; Ramadan, S.F. New Insight into gyrotactic microorganisms for Bio-thermal convection of Prandtl nanofluid past a stretching/shrinking permeable sheet. SN Appl. Sci. 2020, 2, 450. [Google Scholar] [CrossRef] [Green Version]
- Ramana, R.M.; Kumar, J.G.; Raju, K.V. Melting and radiation effects on MHD heat and mass transfer of Casson fluid flow past a permeable stretching sheet in the presence of chemical reaction. AIP Proced. 2020, 2246, 020021. [Google Scholar]
- Abbas, W.; Mekheimer, K.S.; Ghazy, M.M.; Moawad, A.M.A. Thermal radiation effects on oscillatory squeeze flow with a particle-fluid suspension. Heat Transf. 2021, 50, 2129–2149. [Google Scholar] [CrossRef]
- Khan, U.; Zaib, A.; Khan, I.; Nisar, K.S. Insight into the dynamics of transient blood conveying gold nanoparticles when entropy generation and Lorentz force are significant. Int. Commun. Heat Mass Transf. 2021, 127, 105415. [Google Scholar] [CrossRef]
- Ariel, P.D. Axisymmetric flow due to a stretching sheet with partial slip. Comput. Math. Appl. 2007, 54, 1169–1183. [Google Scholar] [CrossRef] [Green Version]
- Mirgolbabaei, H.; Ganji, D.D.; Etghani, M.M.E.; Sobati, A. Adapted variational iteration method and axisymmetric flow over a stretching sheet. Wolrd J. Model. Simul. 2009, 5, 307–314. [Google Scholar]
- Ariel, P.D. Axisymmetric flow of a second grade fluid past a stretching sheet. Int. J. Eng. Sci. 2001, 39, 529–553. [Google Scholar] [CrossRef]
- Sahoo, B. Effects of partial slip on axisymmetric flow of an electrically conducting viscoelastic fluid past a stretching sheet. Central Eur. J. Phys. 2010, 8, 498–508. [Google Scholar] [CrossRef]
- Shahzad, A.; Ali, R.; Kamran, M.; Khan, S.U.D.; Khan, S.U.; Farooq, A. Axisymmetric flow with heat transfer over exponentiallystretching sheet: A computational approach. Phys. A 2020, 554, 124242. [Google Scholar] [CrossRef]
- Mukhopadhyay, S. Effects of thermal radiation on Casson fluid flow and heat transfer over an unsteady stretching surface subjected to suction/blowing. Chin. Phys. B 2013, 22, 114702. [Google Scholar] [CrossRef]
- Mondal, S.; Sibanda, P. Unsteady Casson nanofluid flow over a stretching sheet with thermal radiation, convective and slip boundary conditions. Alex. Eng. J. Eng. 2016, 55, 1025–1035. [Google Scholar]
- Azeem, S.; Ramzan, A.; Masood, K. On the exact solution for axisymmetric flow and heat transfer over a nonlinear radially stretching sheet. Chin. Phys. Lett. 2012, 29, 084705. [Google Scholar]
- Khan, U.; Zaib, A.; Khan, I.; Nisar, K.S. Activation energy on MHD flow of titanium alloy (Ti6Al4V) nanoparticle along with a cross flow and streamwise direction with binary chemical reaction and non-linear radiation: Dual Solutions. J. Mater. Res. Technol. 2020, 9, 188–199. [Google Scholar] [CrossRef]
- Alghamdi, M.; Wakif, A.; Thumma, T.; Khan, U.; Baleanu, D.; Rasool, G. Significance of variability in magnetic field strength and heat source on the radiative-convective motion of sodium alginate-based nanofluid within a Darcy–Brinkman porous structure bounded vertically by an irregular slender surface. Case Stud. Therm. Eng. 2021, 28, 101428. [Google Scholar] [CrossRef]
- Koo, J.; Kleinstreuer, C. Viscous dissipation effects in microtubes and microchannels. Int. J. Heat Mass Transf. 2004, 47, 3159–3169. [Google Scholar] [CrossRef]
- Koo, J. Computational Nanofluid Flow and Heat Transfer Analyses Applied to Micro-Systems. Ph.D. Thesis, NC State University, Raleigh, NC, USA, 2005. [Google Scholar]
- Jang, S.P.; Choi, S.U. Role of Brownian motion in the enhanced thermal conductivity of nanofluids. Appl. Phys. Lett. 2004, 84, 4316–4318. [Google Scholar] [CrossRef]
- Prasher, R.; Bhattacharya, P.; Phelan, P.E. Thermal conductivity of nanoscale colloidal solutions (nanofluids). Phys. Rev. Lett. 2005, 94, 025901. [Google Scholar] [CrossRef]
- Li, J. Computational Analysis of Nanofluid Flow in Microchannels with Applications to Micro-Heat Sinks and Bio-MEMS. Ph.D. Thesis, University Libraries, North Carolina State University, Raleigh, NC, USA, 2008. [Google Scholar]
- Fang, T.; Yao, S.; Zhang, J.; Aziz, A. Viscous flow over a shrinking sheet with a second order slip flow model. Commun. Nonlinear Sci. Num. Simul. 2010, 15, 1831–1842. [Google Scholar] [CrossRef]
- Nandeppanavar, M.M.; Vajravelu, K.; Abel, M.S.; Siddalingappa, M.N. Second order slip flow and heat transfer over astretching sheet with non-linear Navier boundary condition. Int. J. Therm. Sci. 2012, 58, 143–150. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Heat and mass transfer of MHD second order slip flow. Comp. Fluids 2013, 71, 426–434. [Google Scholar] [CrossRef]
- Hakeem, A.K.A.; Ganesh, N.V.; Ganga, B. Magnetic field effect on second order slip flow of nanofluid over a stretching/shrinking sheet with thermal radiation effect. J. Magn. Magn. Mat. 2015, 381, 243–257. [Google Scholar] [CrossRef]



| Physical Properties | Water | Al2O3 |
|---|---|---|
| (Wm−1K−1) | 0.613 | 25 |
| (J kg−1 K−1) | 4179 | 765 |
| (kg m−3) | 997.1 | 3970 |
| 0.05 | 1 × 10−10 | |
| (nm) | - | 47 |
| Pr | 6.2 | - |
| Coefficient Values | Water-Al2O3 |
|---|---|
| 52.813 | |
| 6.115 | |
| 0.695 | |
| 4.1 × 10−2 | |
| 0.176 | |
| −2.98.198 | |
| −34.532 | |
| −3.922 | |
| −0.235 | |
| −0.999 |
| 3.5 | 4 | 4.5 | 5 | ||||
|---|---|---|---|---|---|---|---|
| 0.5 | 0.5 | 0.025 | First solution | 2.58605 | 3.40613 | 4.09306 | 4.73029 |
| Second solution | 1.17470 | 0.89187 | 0.74219 | 0.64220 | |||
| 1 | 0.5 | 0.025 | First solution | 4.66415 | 5.63891 | 6.55804 | 7.44685 |
| Second solution | 0.97697 | 0.80809 | 0.69483 | 0.61190 | |||
| 2 | 0.5 | 0.025 | First solution | 6.60110 | 7.81896 | 8.99505 | 10.14620 |
| Second solution | 0.92040 | 0.77704 | 0.67544 | 0.59881 | |||
| 5 | 0.5 | 0.025 | First solution | 8.50938 | 9.98435 | 11.42330 | 12.83980 |
| Second solution | 0.89249 | 0.76064 | 0.66483 | 0.59149 | |||
| ∞ | 0.5 | 0.025 | First solution | 10.4065 | 12.1435 | 13.8436 | 15.5307 |
| Second solution | 0.87575 | 0.75048 | 0.65812 | 0.58680 |
| 3.5 | 4 | 4.5 | 5 | ||||||
|---|---|---|---|---|---|---|---|---|---|
| 0.5 | 0.5 | 0.025 | 5 | 1 | First solution | 2.82016 | 3.52056 | 4.10507 | 4.66170 |
| Second solution | 2.61293 | 3.32299 | 3.99296 | 4.68492 | |||||
| 1 | 0.5 | 0.025 | 5 | 1 | First solution | 3.04693 | 3.63183 | 4.18821 | 4.72967 |
| Second solution | 2.66077 | 3.44115 | 4.28216 | 5.18029 | |||||
| 2 | 0.5 | 0.025 | 5 | 1 | First solution | 3.13405 | 3.69660 | 4.24086 | 4.77445 |
| Second solution | 2.70750 | 3.72440 | 4.87577 | 6.24120 | |||||
| 5 | 0.5 | 0.025 | 5 | 1 | First solution | 3.19054 | 3.74125 | 4.27820 | 4.80674 |
| Second solution | 2.85264 | 4.31988 | 6.24757 | 9.17801 | |||||
| ∞ | 0.5 | 0.025 | 5 | 1 | First solution | 3.23132 | 3.77438 | 4.30633 | 4.83129 |
| Second solution | 3.18356 | 5.90969 | 11.6249 | 39.6040 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, U.; Zaib, A.; Ishak, A.; Waini, I.; Raizah, Z.; Galal, A.M. Analytical Approach for a Heat Transfer Process through Nanofluid over an Irregular Porous Radially Moving Sheet by Employing KKL Correlation with Magnetic and Radiation Effects: Applications to Thermal System. Micromachines 2022, 13, 1109. https://doi.org/10.3390/mi13071109
Khan U, Zaib A, Ishak A, Waini I, Raizah Z, Galal AM. Analytical Approach for a Heat Transfer Process through Nanofluid over an Irregular Porous Radially Moving Sheet by Employing KKL Correlation with Magnetic and Radiation Effects: Applications to Thermal System. Micromachines. 2022; 13(7):1109. https://doi.org/10.3390/mi13071109
Chicago/Turabian StyleKhan, Umair, Aurang Zaib, Anuar Ishak, Iskandar Waini, Zehba Raizah, and Ahmed M. Galal. 2022. "Analytical Approach for a Heat Transfer Process through Nanofluid over an Irregular Porous Radially Moving Sheet by Employing KKL Correlation with Magnetic and Radiation Effects: Applications to Thermal System" Micromachines 13, no. 7: 1109. https://doi.org/10.3390/mi13071109
APA StyleKhan, U., Zaib, A., Ishak, A., Waini, I., Raizah, Z., & Galal, A. M. (2022). Analytical Approach for a Heat Transfer Process through Nanofluid over an Irregular Porous Radially Moving Sheet by Employing KKL Correlation with Magnetic and Radiation Effects: Applications to Thermal System. Micromachines, 13(7), 1109. https://doi.org/10.3390/mi13071109









