A Cross-Sectional Validation Study of Camry EH101 versus JAMAR Plus Handheld Dynamometers in Colorectal Cancer Patients and Their Correlations with Bioelectrical Impedance and Nutritional Status
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Design
2.2. Data Collection
2.2.1. Clinical Variables and Cancer Staging
2.2.2. Handgrip Strength Protocol
2.2.3. Operative Definitions of Dynapenia, Malnutrition, and Sarcopenia
2.2.4. Bioelectrical Impedance Analysis Protocol
2.2.5. Basic Anthropometry Protocol
2.2.6. Data Quality
2.3. Data Analysis
3. Results
3.1. General Characteristics of the Sample
3.2. Handgrip Strength Determined with Handheld Dynamometers
3.2.1. Correlation and Precision
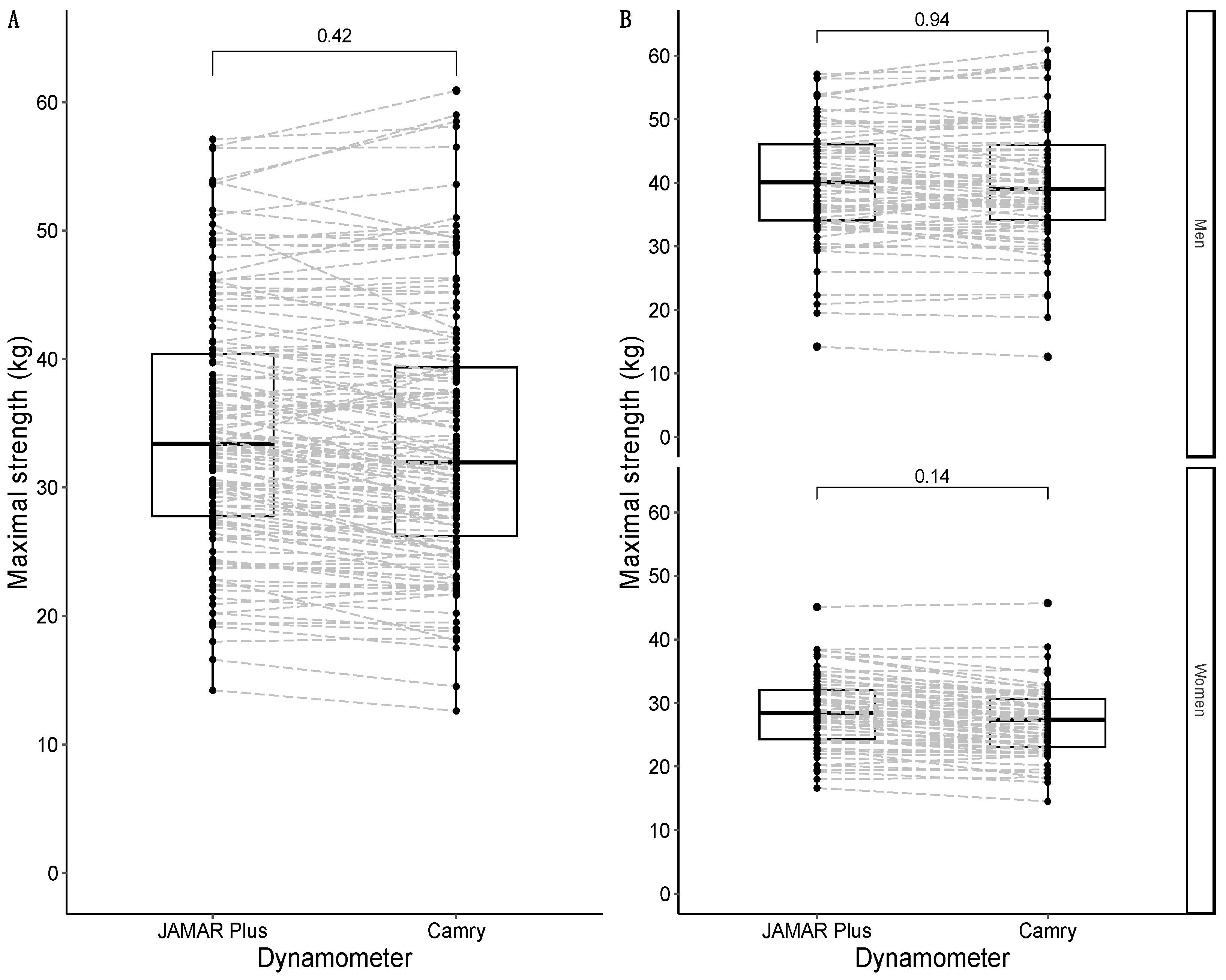
3.2.2. Comparison of Maximal Handgrip Strength between Devices
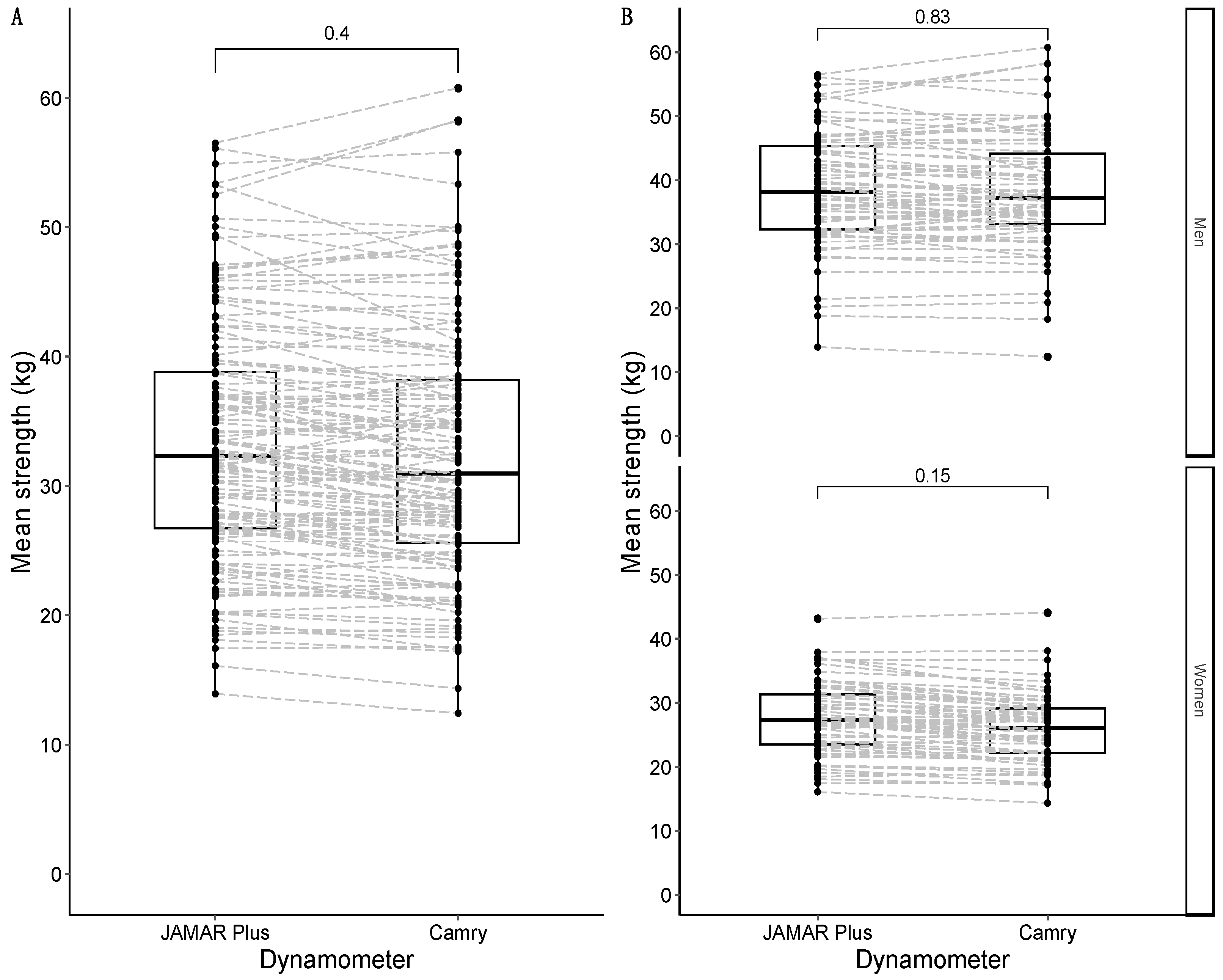
3.2.3. Comparison of Average Handgrip Strength between Devices
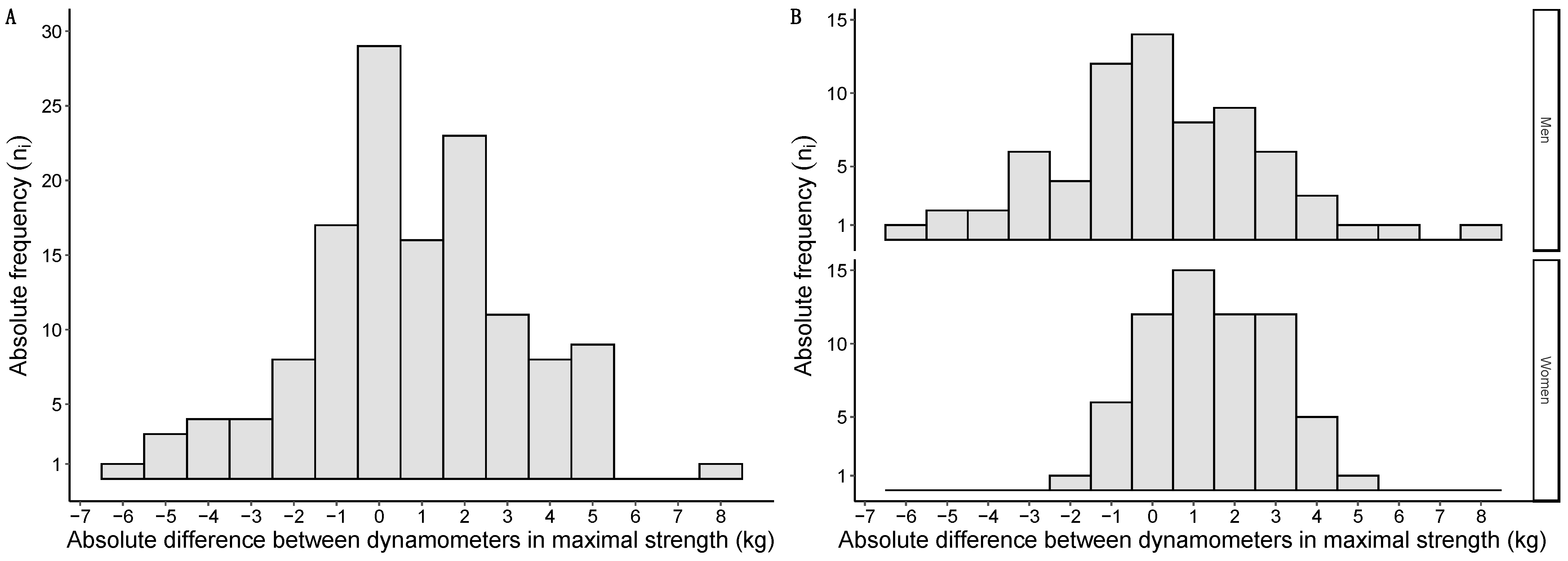
3.2.4. Distribution of Differences between Devices
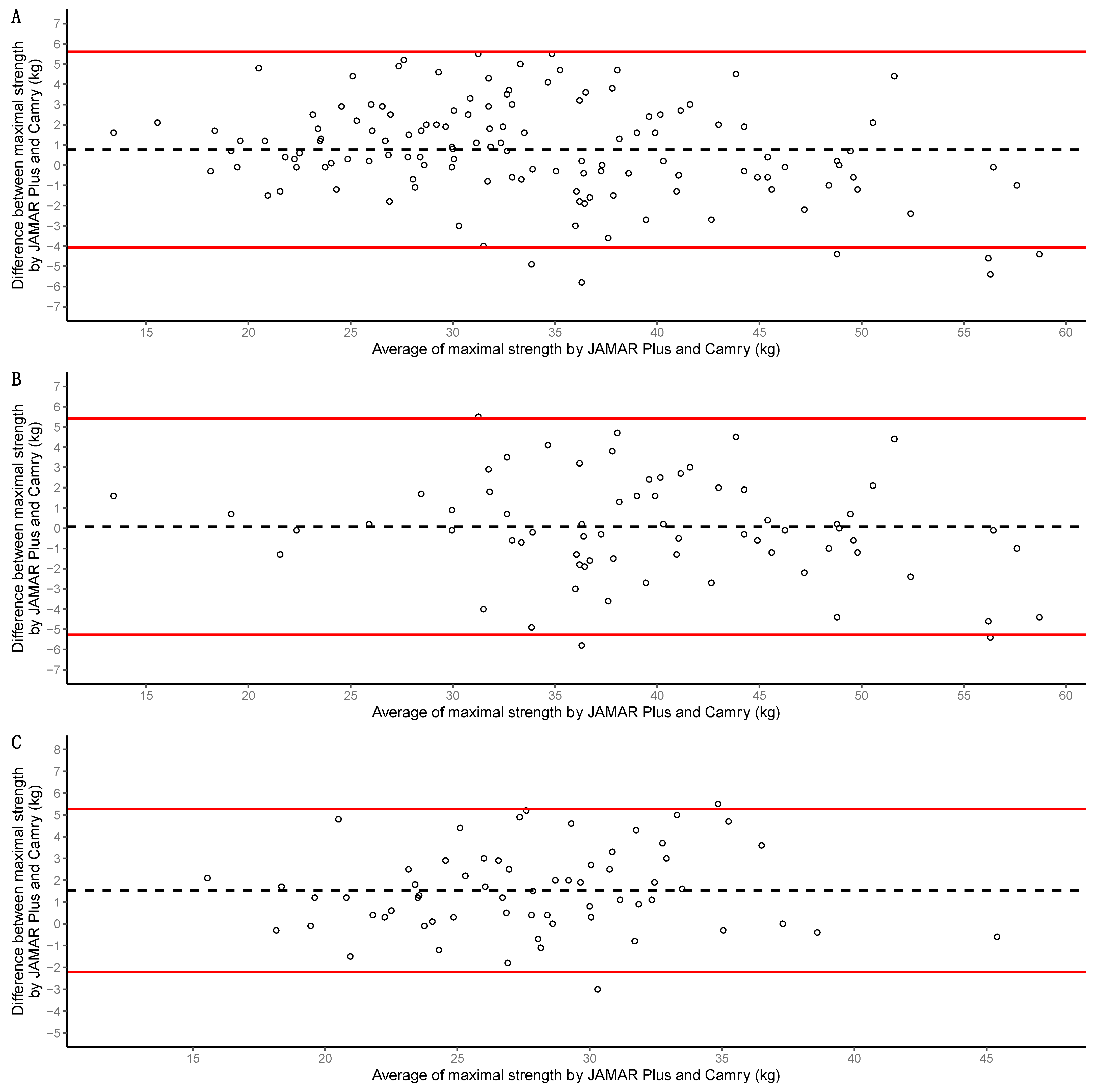
3.2.5. Bland–Altman Analysis
3.2.6. Differential Classification Bias in Dynapenia
3.3. Nutritional Status Assessment
3.3.1. Nutritional Diagnosis and Prevalence of Malnutrition
3.3.2. Body Composition Analysis with BIA
3.3.3. Correlation between Maximal Strength and BIA-Estimated Muscle Mass
3.3.4. Relations between Dynapenia, Muscle Atrophy, and Phase Angle
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Prado, C.M.; Purcell, S.A.; Laviano, A. Nutrition Interventions to Treat Low Muscle Mass in Cancer. J. Cachexia Sarcopenia Muscle 2020, 11, 366–380. [Google Scholar] [CrossRef] [PubMed]
- Meyer, H.-J.; Strobel, A.; Wienke, A.; Surov, A. Prognostic Role of Low-Skeletal Muscle Mass on Staging Computed Tomography in Metastasized Colorectal Cancer: A Systematic Review and Meta-Analysis. Clin. Colorectal Cancer 2022, 21, e213–e225. [Google Scholar] [CrossRef] [PubMed]
- van Baar, H.; Winkels, R.M.; Brouwer, J.G.M.; Posthuma, L.; Bours, M.J.L.; Weijenberg, M.P.; Boshuizen, H.C.; van Zutphen, M.; van Duijnhoven, F.J.B.; Kok, D.E.; et al. Associations of Abdominal Skeletal Muscle Mass, Fat Mass, and Mortality among Men and Women with Stage I-III Colorectal Cancer. Cancer Epidemiol. Biomark. Prev. 2020, 29, 956–965. [Google Scholar] [CrossRef] [PubMed]
- Shirdel, M.; Andersson, F.; Myte, R.; Axelsson, J.; Rutegård, M.; Blomqvist, L.; Riklund, K.; van Guelpen, B.; Palmqvist, R.; Gylling, B. Body Composition Measured by Computed Tomography Is Associated with Colorectal Cancer Survival, Also in Early-Stage Disease. Acta Oncol. 2020, 59, 799–808. [Google Scholar] [CrossRef] [PubMed]
- Derksen, J.W.G.; Kurk, S.A.; Peeters, P.H.M.; Dorresteijn, B.; Jourdan, M.; van der Velden, A.M.T.; Nieboer, P.; de Jong, R.S.; Honkoop, A.H.; Punt, C.J.A.; et al. The Association between Changes in Muscle Mass and Quality of Life in Patients with Metastatic Colorectal Cancer. J. Cachexia Sarcopenia Muscle 2020, 11, 919–928. [Google Scholar] [CrossRef] [PubMed]
- Barbalho, E.R.; Gonzalez, M.C.; Bielemann, R.M.; da Rocha, I.M.G.; de Sousa, I.M.; Bezerra, R.A.; de Medeiros, G.O.C.; Fayh, A.P.T. Is Skeletal Muscle Radiodensity Able to Indicate Physical Function Impairment in Older Adults with Gastrointestinal Cancer? Exp. Gerontol. 2019, 125, 110688. [Google Scholar] [CrossRef] [PubMed]
- Hanna, L.; Nguo, K.; Furness, K.; Porter, J.; Huggins, C.E. Association between Skeletal Muscle Mass and Quality of Life in Adults with Cancer: A Systematic Review and Meta-Analysis. J. Cachexia Sarcopenia Muscle 2022, 13, 839–857. [Google Scholar] [CrossRef] [PubMed]
- Xiao, J.; Caan, B.J.; Cespedes Feliciano, E.M.; Meyerhardt, J.A.; Peng, P.D.; Baracos, V.E.; Lee, V.S.; Ely, S.; Gologorsky, R.C.; Weltzien, E.; et al. Association of Low Muscle Mass and Low Muscle Radiodensity With Morbidity and Mortality for Colon Cancer Surgery. JAMA Surg. 2020, 155, 942–949. [Google Scholar] [CrossRef] [PubMed]
- Martin, L.; Hopkins, J.; Malietzis, G.; Jenkins, J.T.; Sawyer, M.B.; Brisebois, R.; MacLean, A.; Nelson, G.; Gramlich, L.; Baracos, V.E. Assessment of Computed Tomography (CT)-Defined Muscle and Adipose Tissue Features in Relation to Short-Term Outcomes after Elective Surgery for Colorectal Cancer: A Multicenter Approach. Ann. Surg. Oncol. 2018, 25, 2669–2680. [Google Scholar] [CrossRef] [PubMed]
- Kurk, S.; Peeters, P.; Stellato, R.; Dorresteijn, B.; De Jong, P.; Jourdan, M.; Creemers, G.; Erdkamp, F.; De Jongh, F.; Kint, P.; et al. Skeletal Muscle Mass Loss and Dose-limiting Toxicities in Metastatic Colorectal Cancer Patients. J. Cachexia Sarcopenia Muscle 2019, 10, 803–813. [Google Scholar] [CrossRef] [PubMed]
- Aleixo, G.F.P.; Shachar, S.S.; Nyrop, K.A.; Muss, H.B.; Battaglini, C.L.; Williams, G.R. Bioelectrical Impedance Analysis for the Assessment of Sarcopenia in Patients with Cancer: A Systematic Review. Oncologist 2020, 25, 170–182. [Google Scholar] [CrossRef] [PubMed]
- Moreau, J.; Ordan, M.; Barbe, C.; Mazza, C.; Perrier, M.; Botsen, D.; Brasseur, M.; Portefaix, C.; Renard, Y.; Tallière, B.; et al. Correlation between Muscle Mass and Handgrip Strength in Digestive Cancer Patients Undergoing Chemotherapy. Cancer Med. 2019, 8, 3677–3684. [Google Scholar] [CrossRef] [PubMed]
- Piccoli, A.; Rossi, B.; Pillon, L.; Bucciante, G. A New Method for Monitoring Body Fluid Variation by Bioimpedance Analysis: The RXc Graph. Kidney Int. 1994, 46, 534–539. [Google Scholar] [CrossRef] [PubMed]
- Souza, N.C.; Avesani, C.M.; Prado, C.M.; Martucci, R.B.; Rodrigues, V.D.; de Pinho, N.B.; Heymsfield, S.B.; Gonzalez, M.C. Phase Angle as a Marker for Muscle Abnormalities and Function in Patients with Colorectal Cancer. Clin. Nutr. 2021, 40, 4799–4806. [Google Scholar] [CrossRef] [PubMed]
- Arab, A.; Karimi, E.; Vingrys, K.; Shirani, F. Is Phase Angle a Valuable Prognostic Tool in Cancer Patients’ Survival? A Systematic Review and Meta-Analysis of Available Literature. Clin. Nutr. 2021, 40, 3182–3190. [Google Scholar] [CrossRef] [PubMed]
- Norman, K.; Stobäus, N.; Zocher, D.; Bosy-Westphal, A.; Szramek, A.; Scheufele, R.; Smoliner, C.; Pirlich, M. Cutoff Percentiles of Bioelectrical Phase Angle Predict Functionality, Quality of Life, and Mortality in Patients with Cancer. Am. J. Clin. Nutr. 2010, 92, 612–619. [Google Scholar] [CrossRef] [PubMed]
- Beaudart, C.; Bruyère, O.; Geerinck, A.; Hajaoui, M.; Scafoglieri, A.; Perkisas, S.; Bautmans, I.; Gielen, E.; Reginster, J.-Y.; Buckinx, F. Equation Models Developed with Bioelectric Impedance Analysis Tools to Assess Muscle Mass: A Systematic Review. Clin. Nutr. ESPEN 2020, 35, 47–62. [Google Scholar] [CrossRef] [PubMed]
- Gort-van Dijk, D.; Weerink, L.B.M.; Milovanovic, M.; Haveman, J.-W.; Hemmer, P.H.J.; Dijkstra, G.; Lindeboom, R.; Campmans-Kuijpers, M.J.E. Bioelectrical Impedance Analysis and Mid-Upper Arm Muscle Circumference Can Be Used to Detect Low Muscle Mass in Clinical Practice. Nutrients 2021, 13, 2350. [Google Scholar] [CrossRef] [PubMed]
- Mueller, T.C.; Reik, L.; Prokopchuk, O.; Friess, H.; Martignoni, M.E. Measurement of Body Mass by Bioelectrical Impedance Analysis and Computed Tomography in Cancer Patients with Malnutrition—A Cross-Sectional Observational Study. Medicine 2020, 99, e23642. [Google Scholar] [CrossRef] [PubMed]
- Souza, N.C.; Gonzalez, M.C.; Martucci, R.B.; Rodrigues, V.D.; de Pinho, N.B.; Qureshi, A.R.; Avesani, C.M. Comparative Analysis Between Computed Tomography and Surrogate Methods to Detect Low Muscle Mass Among Colorectal Cancer Patients. JPEN J. Parenter. Enter. Nutr. 2020, 44, 1328–1337. [Google Scholar] [CrossRef] [PubMed]
- Cederholm, T.; Jensen, G.L.; Correia, M.I.T.D.; Gonzalez, M.C.; Fukushima, R.; Higashiguchi, T.; Baptista, G.; Barazzoni, R.; Blaauw, R.; Coats, A.; et al. GLIM Criteria for the Diagnosis of Malnutrition—A Consensus Report from the Global Clinical Nutrition Community. Clin. Nutr. 2019, 38, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Barbosa, M.V.; Dos Santos, M.P.; Leite, J.A.; Rodrigues, V.D.; de Pinho, N.B.; Martucci, R.B. Association between Functional Aspects and Health-Related Quality of Life in Patients with Colorectal Cancer: Can Handgrip Strength Be the Measure of Choice in Clinical Practice? Support. Care Cancer 2023, 31, 144. [Google Scholar] [CrossRef] [PubMed]
- Zhang, F.-M.; Zhang, X.-Z.; Shi, H.-P.; Zhang, Z.; Wang, S.-L.; Shen, Z.-L.; Chen, X.-L.; Shen, X.; Yu, Z.; Zhuang, C.-L. Comparisons and Impacts of the Basic Components of Sarcopenia Definition and Their Pairwise Combinations in Gastric Cancer: A Large-Scale Study in a Chinese Population. Front. Nutr. 2021, 8, 709211. [Google Scholar] [CrossRef] [PubMed]
- Zhuang, C.-L.; Zhang, F.-M.; Li, W.; Wang, K.-H.; Xu, H.-X.; Song, C.-H.; Guo, Z.-Q.; Shi, H.-P. Associations of Low Handgrip Strength with Cancer Mortality: A Multicentre Observational Study. J. Cachexia Sarcopenia Muscle 2020, 11, 1476–1486. [Google Scholar] [CrossRef] [PubMed]
- Contreras-Bolívar, V.; Sánchez-Torralvo, F.J.; Ruiz-Vico, M.; González-Almendros, I.; Barrios, M.; Padín, S.; Alba, E.; Olveira, G. GLIM Criteria Using Hand Grip Strength Adequately Predict Six-Month Mortality in Cancer Inpatients. Nutrients 2019, 11, E2043. [Google Scholar] [CrossRef] [PubMed]
- Ordan, M.-A.; Mazza, C.; Barbe, C.; Perrier, M.; Botsen, D.; Renard, Y.; Moreau, J.; Brasseur, M.; Taillière, B.; Bertin, É.; et al. Feasibility of Systematic Handgrip Strength Testing in Digestive Cancer Patients Treated with Chemotherapy: The FIGHTDIGO Study. Cancer 2018, 124, 1501–1506. [Google Scholar] [CrossRef] [PubMed]
- Scherbov, S.; Spitzer, S.; Steiber, N. Thresholds for Clinical Practice That Directly Link Handgrip Strength to Remaining Years of Life: Estimates Based on Longitudinal Observational Data. BMJ Open 2022, 12, e058489. [Google Scholar] [CrossRef] [PubMed]
- Steiber, N. Strong or Weak Handgrip? Normative Reference Values for the German Population across the Life Course Stratified by Sex, Age, and Body Height. PLoS ONE 2016, 11, e0163917. [Google Scholar] [CrossRef] [PubMed]
- Cooper, R.; Lessof, C.; Wong, A.; Hardy, R. The Impact of Variation in the Device Used to Measure Grip Strength on the Identification of Low Muscle Strength: Findings from a Randomised Cross-over Study. J. Frailty Sarcopenia Falls 2021, 6, 225–230. [Google Scholar] [CrossRef] [PubMed]
- Benton, M.J.; Spicher, J.M.; Silva-Smith, A.L. Validity and Reliability of Handgrip Dynamometry in Older Adults: A Comparison of Two Widely Used Dynamometers. PLoS ONE 2022, 17, e0270132. [Google Scholar] [CrossRef]
- Díaz Muñoz, G.A.; Calvera Millán, S.J. Comparing the Camry Dynamometer to the Jamar Dynamometer for Use in Healthy Colombian Adults. Rev. Salud Bosque 2019, 9, 21–29. [Google Scholar] [CrossRef]
- Abe, T.; Loenneke, J.P.; Thiebaud, R.S.; Loftin, M. The Bigger the Hand, the Bigger the Difference? Implications for Testing Strength With 2 Popular Handgrip Dynamometers. J. Sport. Rehabil. 2019, 28, 278–282. [Google Scholar] [CrossRef] [PubMed]
- Huang, L.; Liu, Y.; Lin, T.; Hou, L.; Song, Q.; Ge, N.; Yue, J. Reliability and Validity of Two Hand Dynamometers When Used by Community-Dwelling Adults Aged over 50 Years. BMC Geriatr. 2022, 22, 580. [Google Scholar] [CrossRef] [PubMed]
- Lupton-Smith, A.; Fourie, K.; Mazinyo, A.; Mokone, M.; Nxaba, S.; Morrow, B. Measurement of Hand Grip Strength: A Cross-Sectional Study of Two Dynamometry Devices. S. Afr. J. Physiother. 2022, 78, 1768. [Google Scholar] [CrossRef]
- Andrade, M.S.; Honorato, M.P.; Vargas, J.P.; de los Angeles Galvez, M.; Rojas, M.R. Comparison of Two Handgrip Dynamometers in Older Adults before Elective Surgery. Perioper. Med. 2023, 12, 46. [Google Scholar] [CrossRef]
- Lu, M.-J.; Zhong, W.-H.; Liu, Y.-X.; Miao, H.-Z.; Li, Y.-C.; Ji, M.-H. Sample Size for Assessing Agreement between Two Methods of Measurement by Bland-Altman Method. Int. J. Biostat. 2016, 12, 20150039. [Google Scholar] [CrossRef] [PubMed]
- Oken, M.M.; Creech, R.H.; Tormey, D.C.; Horton, J.; Davis, T.E.; McFadden, E.T.; Carbone, P.P. Toxicity and Response Criteria of the Eastern Cooperative Oncology Group. Am. J. Clin. Oncol. 1982, 5, 649–655. [Google Scholar] [CrossRef] [PubMed]
- Amin, M.B.; Greene, F.L.; Edge, S.B.; Compton, C.C.; Gershenwald, J.E.; Brookland, R.K.; Meyer, L.; Gress, D.M.; Byrd, D.R.; Winchester, D.P. The Eighth Edition AJCC Cancer Staging Manual: Continuing to Build a Bridge from a Population-Based to a More “Personalized” Approach to Cancer Staging. CA Cancer J. Clin. 2017, 67, 93–99. [Google Scholar] [CrossRef] [PubMed]
- Cervantes, A.; Adam, R.; Roselló, S.; Arnold, D.; Normanno, N.; Taïeb, J.; Seligmann, J.; Baere, T.D.; Osterlund, P.; Yoshino, T.; et al. Metastatic Colorectal Cancer: ESMO Clinical Practice Guideline for Diagnosis, Treatment and Follow-Up. Ann. Oncol. 2023, 34, 10–32. [Google Scholar] [CrossRef] [PubMed]
- Trampisch, U.S.; Franke, J.; Jedamzik, N.; Hinrichs, T.; Platen, P. Optimal Jamar Dynamometer Handle Position to Assess Maximal Isometric Hand Grip Strength in Epidemiological Studies. J. Hand Surg. 2012, 37, 2368–2373. [Google Scholar] [CrossRef] [PubMed]
- Ruiz-Ruiz, J.; Mesa, J.L.M.; Gutiérrez, A.; Castillo, M.J. Hand Size Influences Optimal Grip Span in Women but Not in Men. J. Hand Surg. Am. 2002, 27, 897–901. [Google Scholar] [CrossRef] [PubMed]
- Roberts, H.C.; Denison, H.J.; Martin, H.J.; Patel, H.P.; Syddall, H.; Cooper, C.; Sayer, A.A. A Review of the Measurement of Grip Strength in Clinical and Epidemiological Studies: Towards a Standardised Approach. Age Ageing 2011, 40, 423–429. [Google Scholar] [CrossRef] [PubMed]
- Mehmet, H.; Yang, A.W.H.; Robinson, S.R. Measurement of Hand Grip Strength in the Elderly: A Scoping Review with Recommendations. J. Bodyw. Mov. Ther. 2020, 24, 235–243. [Google Scholar] [CrossRef] [PubMed]
- Savas, S.; Kilavuz, A.; Kayhan Koçak, F.Ö.; Cavdar, S. Comparison of Grip Strength Measurements by Widely Used Three Dynamometers in Outpatients Aged 60 Years and Over. J. Clin. Med. 2023, 12, 4260. [Google Scholar] [CrossRef] [PubMed]
- Dodds, R.M.; Syddall, H.E.; Cooper, R.; Benzeval, M.; Deary, I.J.; Dennison, E.M.; Der, G.; Gale, C.R.; Inskip, H.M.; Jagger, C.; et al. Grip Strength across the Life Course: Normative Data from Twelve British Studies. PLoS ONE 2014, 9, e113637. [Google Scholar] [CrossRef] [PubMed]
- Cruz-Jentoft, A.J.; Bahat, G.; Bauer, J.; Boirie, Y.; Bruyère, O.; Cederholm, T.; Cooper, C.; Landi, F.; Rolland, Y.; Sayer, A.A.; et al. Sarcopenia: Revised European Consensus on Definition and Diagnosis. Age Ageing 2019, 48, 16–31. [Google Scholar] [CrossRef] [PubMed]
- Huemer, M.-T.; Kluttig, A.; Fischer, B.; Ahrens, W.; Castell, S.; Ebert, N.; Gastell, S.; Jöckel, K.-H.; Kaaks, R.; Karch, A.; et al. Grip Strength Values and Cut-off Points Based on over 200,000 Adults of the German National Cohort—A Comparison to the EWGSOP2 Cut-off Points. Age Ageing 2023, 52, afac324. [Google Scholar] [CrossRef] [PubMed]
- Correia, M.I.T.D.; Tappenden, K.A.; Malone, A.; Prado, C.M.; Evans, D.C.; Sauer, A.C.; Hegazi, R.; Gramlich, L. Utilization and Validation of the Global Leadership Initiative on Malnutrition (GLIM): A Scoping Review. Clin. Nutr. 2022, 41, 687–697. [Google Scholar] [CrossRef] [PubMed]
- Masanes Toran, F.; Culla, A.; Navarro-Gonzalez, M.; Navarro-Lopez, M.; Sacanella, E.; Torres, B.; Lopez-Soto, A. Prevalence of Sarcopenia in Healthy Community-Dwelling Elderly in an Urban Area of Barcelona (Spain). J. Nutr. Health Aging 2012, 16, 184–187. [Google Scholar] [CrossRef] [PubMed]
- Barazzoni, R.; Jensen, G.L.; Correia, M.I.T.D.; Gonzalez, M.C.; Higashiguchi, T.; Shi, H.P.; Bischoff, S.C.; Boirie, Y.; Carrasco, F.; Cruz-Jentoft, A.; et al. Guidance for Assessment of the Muscle Mass Phenotypic Criterion for the Global Leadership Initiative on Malnutrition (GLIM) Diagnosis of Malnutrition. Clin. Nutr. 2022, 41, 1425–1433. [Google Scholar] [CrossRef] [PubMed]
- Earthman, C.P. Body Composition Tools for Assessment of Adult Malnutrition at the Bedside: A Tutorial on Research Considerations and Clinical Applications. J. Parenter. Enter. Nutr. 2015, 39, 787–822. [Google Scholar] [CrossRef] [PubMed]
- Janssen, I.; Heymsfield, S.B.; Baumgartner, R.N.; Ross, R. Estimation of Skeletal Muscle Mass by Bioelectrical Impedance Analysis. J. Appl. Physiol. 1985 2000, 89, 465–471. [Google Scholar] [CrossRef] [PubMed]
- Bosy-Westphal, A.; Danielzik, S.; Dörhöfer, R.-P.; Later, W.; Wiese, S.; Müller, M.J. Phase Angle from Bioelectrical Impedance Analysis: Population Reference Values by Age, Sex, and Body Mass Index. JPEN J. Parenter. Enter. Nutr. 2006, 30, 309–316. [Google Scholar] [CrossRef] [PubMed]
- Jiang, N.; Zhang, J.; Cheng, S.; Liang, B. The Role of Standardized Phase Angle in the Assessment of Nutritional Status and Clinical Outcomes in Cancer Patients: A Systematic Review of the Literature. Nutrients 2022, 15, 50. [Google Scholar] [CrossRef] [PubMed]
- Whiting, P.F.; Rutjes, A.W.S.; Westwood, M.E.; Mallett, S.; Deeks, J.J.; Reitsma, J.B.; Leeflang, M.M.G.; Sterne, J.A.C.; Bossuyt, P.M.M.; QUADAS-2 Group. QUADAS-2: A Revised Tool for the Quality Assessment of Diagnostic Accuracy Studies. Ann. Intern. Med. 2011, 155, 529–536. [Google Scholar] [CrossRef] [PubMed]
- Bland, J.M.; Altman, D.G. Statistical Methods for Assessing Agreement between Two Methods of Clinical Measurement. Lancet 1986, 1, 307–310. [Google Scholar] [CrossRef] [PubMed]
- Koo, T.K.; Li, M.Y. A Guideline of Selecting and Reporting Intraclass Correlation Coefficients for Reliability Research. J. Chiropr. Med. 2016, 15, 155–163. [Google Scholar] [CrossRef] [PubMed]
- Shrout, P.E.; Fleiss, J.L. Intraclass Correlations: Uses in Assessing Rater Reliability. Psychol. Bull. 1979, 86, 420–428. [Google Scholar] [CrossRef] [PubMed]
- Lessof, C.; Cooper, R.; Wong, A.; Bendayan, R.; Caleyachetty, R.; Cheshire, H.; Cosco, T.; Elhakeem, A.; Hansell, A.L.; Kaushal, A.; et al. Comparison of Devices Used to Measure Blood Pressure, Grip Strength and Lung Function: A Randomised Cross-over Study. PLoS ONE 2023, 18, e0289052. [Google Scholar] [CrossRef] [PubMed]


| Parameter | Results |
|---|---|
| Sample size (ni) | n = 134 |
| Age (years) | Me = 65.7 years IQR = 13.4 years |
| Older than 65 (ni) | n = 71 (52.98%) |
| Female (ni) | n = 64 (47.76%) |
| Type of neoplasm (ni) | Right colon, n = 32 Rectum, n = 36 Sigma, n = 27 Left colon, n = 15 Transverse colon, n = 11 Rectosigmoid, n = 10 Krukenberg’s tumor (colon as the primary site), n = 1 |
| Stage (TNM) at diagnosis | IIA (n = 24); IIB (n = 3); IIC (n = 4) IIIA (n = 12); IIIB (n = 43); IIIC (n = 13) IVA (n = 14); IVB (n = 15); IVC (n = 6) |
| Previous surgery | Yes, n = 123 No, n = 11 |
| Active chemotherapy (ni) | No, n = 102 Yes, n = 32 |
| ECOG (ni) | 0, n = 86 1, n = 42 2, n = 5 3, n = 1 |
| Parameter | Total Sample | Men | Women | p-Value |
|---|---|---|---|---|
| Measuring side (ni) | Right, n = 125 Left, n = 9 | Right, n = 65 Left, n = 5 | Right, n = 60 Left, n = 4 | <2.2 × 10−16 |
| Hand size (cm) | Me = 20.0 IQR = 2.0 | Me = 21.0 IQR = 2.0 | Me = 19.0 IQR = 2.0 | 7.827 × 10−11 |
| First dynamometer (ni) | JAMAR, n = 63 Camry, n = 71 | JAMAR, n = 38 Camry, n = 32 | JAMAR, n = 25 Camry, n = 39 | 0.299 |
| Maximal strength JAMAR (kg) | Me = 33.4 IQR = 12.6 | Me = 40.0 IQR = 12.0 | Me = 28.4 IQR = 7.8 | 4.387 × 10−13 |
| Average strength JAMAR (kg) | Me = 32.3 IQR = 12.1 | Me = 32.0 IQR = 14.1 | Me = 32.5 IQR = 10.2 | 1.205 × 10−12 |
| ICC JAMAR | 0.968 | 0.961 | 0.931 | NC |
| SD differences JAMAR (kg) | 2.3 | 2.3 | 2.3 | NC |
| SEM JAMAR (kg) | 0.4 | 0.4 | 0.6 | NC |
| MDC JAMAR (kg) | 1.1 | 1.2 | 1.6 | NC |
| Maximal strength Camry (kg) | Me = 32.0 IQR = 13.1 | Me = 39.0 IQR = 11.8 | Me = 27.4 IQR = 7.6 | 9.127 × 10−15 |
| Average strength Camry (kg) | Me = 31.0 IQR = 12.6 | Me = 37.3 IQR = 11.0 | Me = 26.2 IQR = 6.98 | 8.215 × 10−15 |
| ICC Camry | 0.971 | 0.958 | 0.938 | NC |
| SD differences Camry (kg) | 2.4 | 2.4 | 2.4 | NC |
| SEM Camry (kg) | 0.4 | 0.5 | 0.6 | NC |
| MDC Camry (kg) | 1.1 | 1.3 | 1.6 | NC |
| Difference in maximal strength between devices (kg) | Me = 0.6 IQR = 2.9 | Me = −0.1 IQR = 3.1 | Me = 1.2 IQR = 2.5 | 0.377 × 10−3 |
| Cutoff Point | JAMAR Plus | Camry |
|---|---|---|
| Dodds et al. (p10) [45] | 5 (3.67%) | 7 (5.14%) |
| Dodds et al. (dichotomous) [45] | 5 (3.67%) | 6 (4.41%) |
| Parameter | Results |
|---|---|
| Weight (kg) | Me = 73.0 IQR = 18.2 |
| Height (m) | Mean = 1.64 SD = 0.09 |
| BMI (kg/m2) | Me = 27.3 IQR = 5.6 |
| BMI by group (ni) | Underweight, n = 5 Normal weight, n = 44 Overweight, n = 50 Grade 1 obesity, n = 22 Grade 2 obesity, n = 8 Grade 3 obesity, n = 5 |
| Percentage of weight loss (%) | Men: Me = −4.23; IQR = 12.3 Women: Me = −0.17; IQR = 3.37 |
| GLIM malnutrition (ni) | No malnutrition, n = 94 Moderate malnutrition, n = 38 Severe malnutrition, n = 2 |
| Parameter | Men | Women | p-Value |
|---|---|---|---|
| R (Ω) | Mean = 503.0 SD = 67.9 | Mean = 602.0 SD = 78.7 | 2.315 × 10−12 |
| Xc (Ω) | Mean = 45.4 SD = 8.5 | Mean = 50.6 SD = 9.4 | 0.105 × 10−2 |
| Z (Ω) | Mean = 504.76 SD = 68.08 | Mean = 604.4 SD = 79.0 | 5.336 × 10−11 |
| θ (°) | Me = 5.3 IQR = 0.8 | Me = 4.9 IQR = 0.7 | 0.874 × 10−3 |
| Standardized θ (Z-score) | Me = −0.9 IQR = 1.3 | Me = −0.8 IQR = 0.9 | 0.692 |
| MM (kg) | Me = 26.6 IQR = 3.40 | Me = 17.4 IQR = 3.93 | <2.2 × 10−16 |
| SMI (kg/m2) | Me = 9.60 IQR = 1.46 | Me = 6.80 IQR = 1.19 | <2.2 × 10−16 |
| Muscle atrophy (ni) | Atrophy, n = 7 No atrophy, n = 62 | Atrophy, n = 28 No atrophy, n = 36 | 0.890 × 10−5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiménez-Sánchez, A.; Pereira-Cunill, J.L.; Limón-Mirón, M.L.; López-Ladrón, A.; Salvador-Bofill, F.J.; García-Luna, P.P. A Cross-Sectional Validation Study of Camry EH101 versus JAMAR Plus Handheld Dynamometers in Colorectal Cancer Patients and Their Correlations with Bioelectrical Impedance and Nutritional Status. Nutrients 2024, 16, 1824. https://doi.org/10.3390/nu16121824
Jiménez-Sánchez A, Pereira-Cunill JL, Limón-Mirón ML, López-Ladrón A, Salvador-Bofill FJ, García-Luna PP. A Cross-Sectional Validation Study of Camry EH101 versus JAMAR Plus Handheld Dynamometers in Colorectal Cancer Patients and Their Correlations with Bioelectrical Impedance and Nutritional Status. Nutrients. 2024; 16(12):1824. https://doi.org/10.3390/nu16121824
Chicago/Turabian StyleJiménez-Sánchez, Andrés, José Luis Pereira-Cunill, María Luisa Limón-Mirón, Amelia López-Ladrón, Francisco Javier Salvador-Bofill, and Pedro Pablo García-Luna. 2024. "A Cross-Sectional Validation Study of Camry EH101 versus JAMAR Plus Handheld Dynamometers in Colorectal Cancer Patients and Their Correlations with Bioelectrical Impedance and Nutritional Status" Nutrients 16, no. 12: 1824. https://doi.org/10.3390/nu16121824
APA StyleJiménez-Sánchez, A., Pereira-Cunill, J. L., Limón-Mirón, M. L., López-Ladrón, A., Salvador-Bofill, F. J., & García-Luna, P. P. (2024). A Cross-Sectional Validation Study of Camry EH101 versus JAMAR Plus Handheld Dynamometers in Colorectal Cancer Patients and Their Correlations with Bioelectrical Impedance and Nutritional Status. Nutrients, 16(12), 1824. https://doi.org/10.3390/nu16121824







