Early Post-Seismic Deformation Revealed After the Wushi (China) Earthquake (Mw = 7.1) Occurred on 22 January 2024
Abstract
:1. Introduction
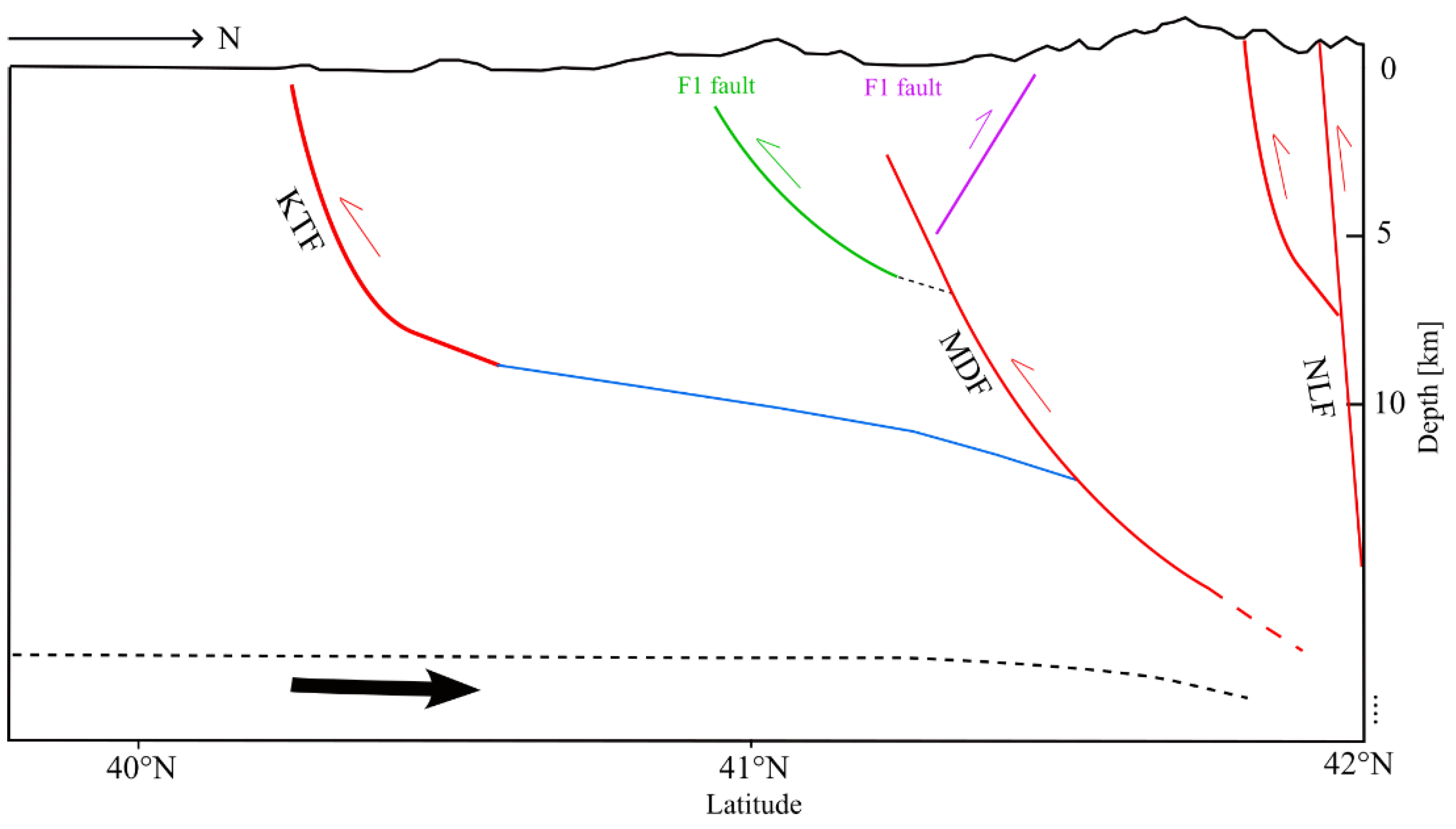
2. Geologic Background
3. InSAR Data and Processing
4. Modeling Approach
5. Results
5.1. InSAR Post-Seismic Deformation
5.2. Afterslip
6. Discussion
6.1. Early Afterslip of Wushi Earthquake
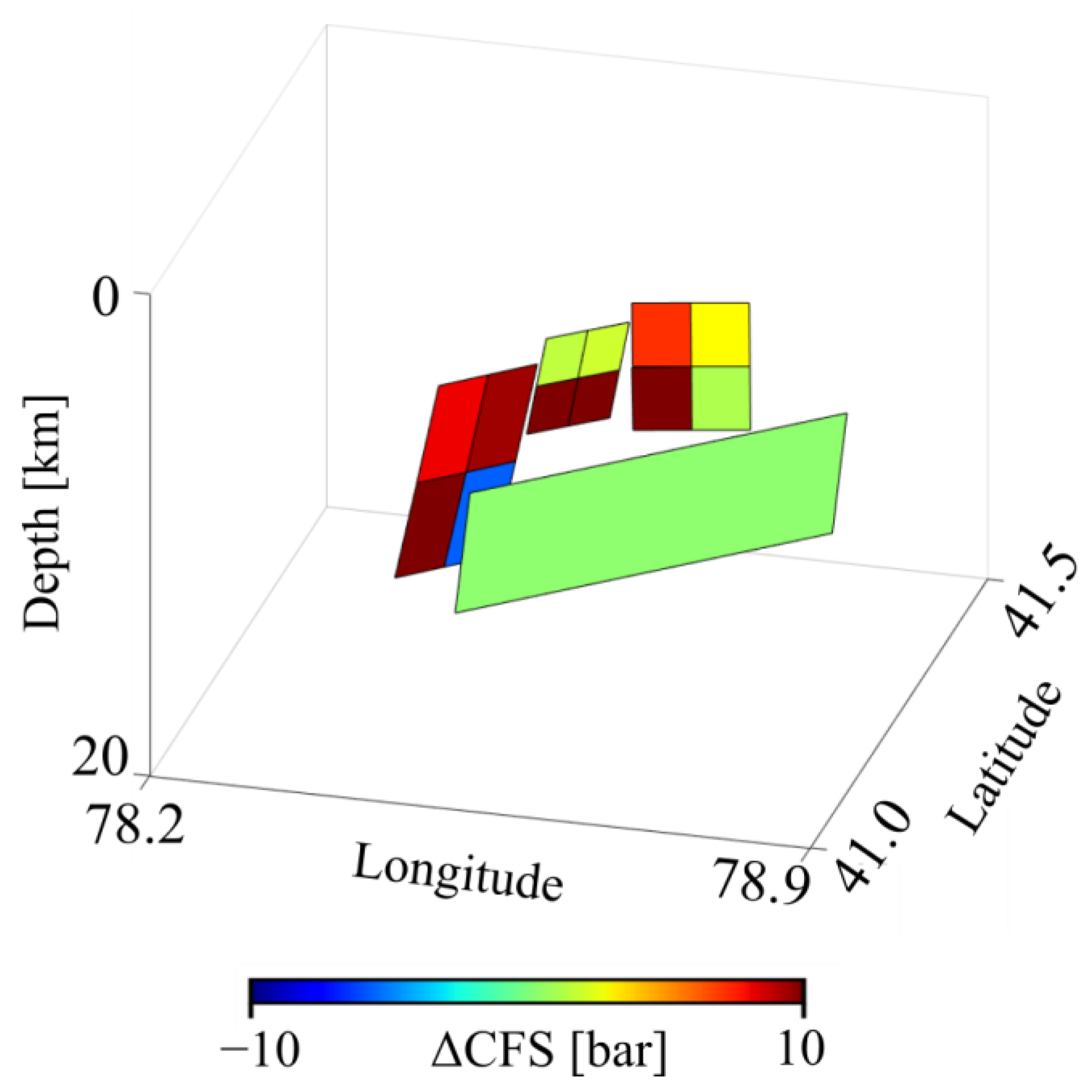
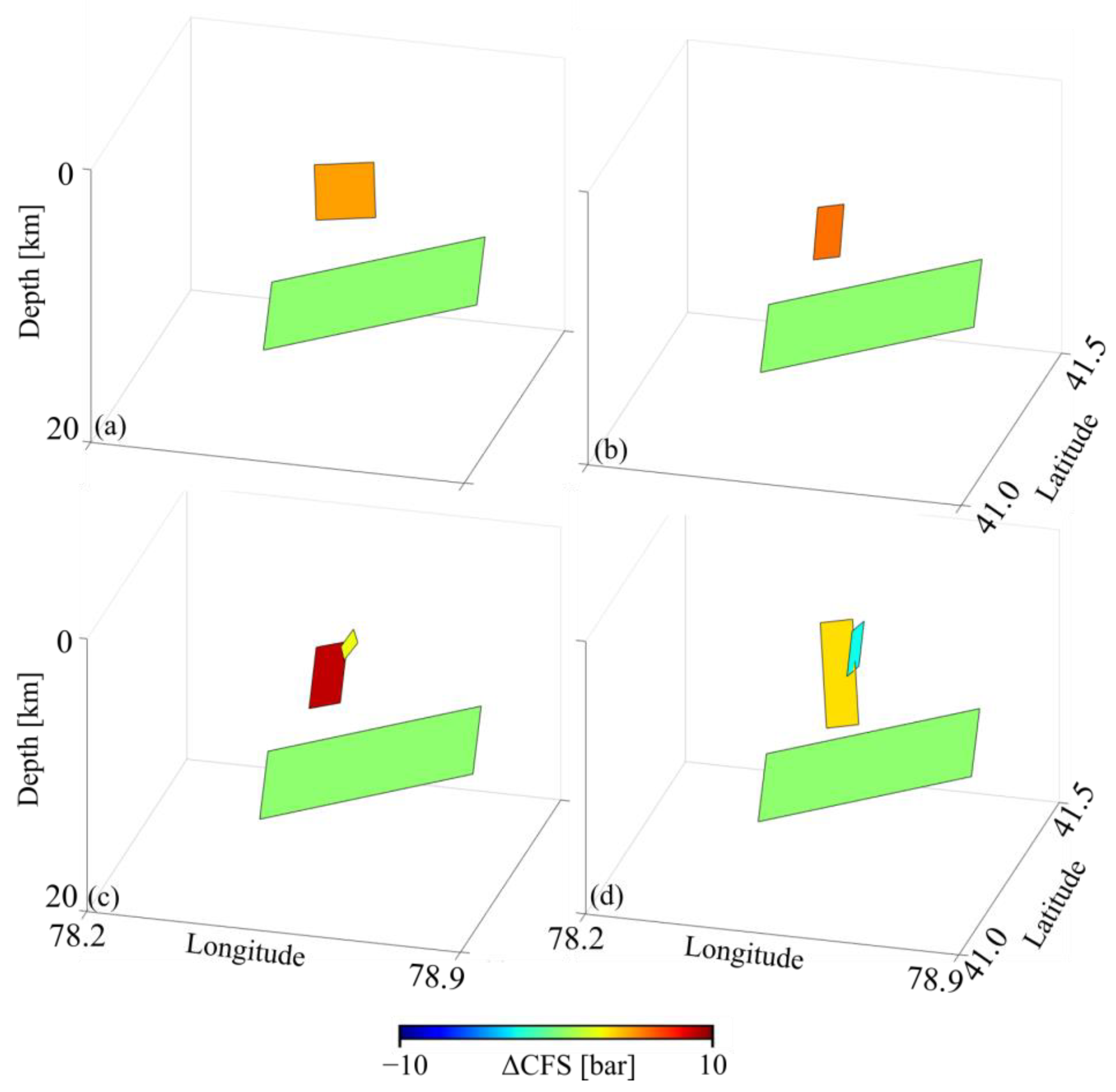
6.2. Coulomb Stress Triggering Relationship
6.3. Aftershock on 29 January 2024
7. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yu, S.; Li, Z.; Zhao, P.; Luo, J.; Yang, Y. Source Parameters and Seismogenic Fault Model of the 2024 Mw 7.0 Wushi (Xinjiang, China) Earthquake Revealed by InSAR Observations. Pure Appl. Geophys. 2024. [Google Scholar] [CrossRef]
- Zhang, B.; Qian, L.; Li, T.; Chen, J.; Xu, J.; Yao, Y.; Fang, L.; Xie, C.; Chen, J.; Liu, G.; et al. Geological disasters and surface ruptures of January 23, 2024 MS7.1 Wushi earthquake, Xinjiang, China. Seismol. Geol. 2024, 46, 220–234. [Google Scholar]
- Avouac, J.P. From geodetic imaging of seismic and aseismic fault slip to dynamic modeling of the seismic cycle. Annu. Rev. Earth Planet. Sci. 2015, 43, 233–271. [Google Scholar] [CrossRef]
- Lv, X.; Amelung, F.; Shao, Y.; Ye, S.; Liu, M.; Xie, C. Rheology of the Zagros Lithosphere from Post-Seismic Deformation of the 2017 Mw7.3 Kermanshah, Iraq, Earthquake. Remote Sens. 2020, 12, 2032. [Google Scholar] [CrossRef]
- Lv, X.; Amelung, F.; Shao, Y. Widespread Aseismic Slip Along the Makran Megathrust Triggered by the 2013 Mw 7.7 Balochistan Earthquake. Geophys. Res. Lett. 2022, 49, e2021GL097411. [Google Scholar] [CrossRef]
- Wiseman, K.; Bürgmann, R.; Freed, A.M.; Banerjee, P. Viscoelastic relaxation in a heterogeneous Earth following the 2004 Sumatra–Andaman earthquake. Earth Planet. Sci. Lett. 2015, 431, 308–317. [Google Scholar] [CrossRef]
- Pollitz, F.F. Lithosphere and shallow asthenosphere rheology from observations of post-earthquake relaxation. Phys. Earth Planet. Inter. 2019, 293, 106271. [Google Scholar] [CrossRef]
- Hughes, K.L.H.; Masterlark, T.; Mooney, W.D. Poroelastic stress-triggering of the 2005 M8.7 Nias earthquake by the 2004 M9.2 Sumatra–Andaman earthquake. Earth Planet. Sci. Lett. 2010, 293, 289–299. [Google Scholar] [CrossRef]
- Hu, Y.; Bürgmann, R.; Freymueller, J.T.; Banerjee, P.; Wang, K. Contributions of poroelastic rebound and a weak volcanic arc to the postseismic deformation of the 2011 Tohoku earthquake. Earth Planets Space 2014, 66, 106. [Google Scholar] [CrossRef]
- Qiu, J.; Sun, J.; Ji, L. The 2024 Mw 7.1 Wushi Earthquake: A Thrust and Strike-Slip Event Unveiling the Seismic Mechanisms of the South Tian Shan’s Thick-Skin Tectonics. Remote Sens. 2024, 16, 2937. [Google Scholar] [CrossRef]
- Cetin, E.; Meghraoui, M.; Cakir, Z.; Akoglu, A.M.; Mimouni, O.; Chebbah, M. Seven years of postseismic deformation following the 2003 Mw = 6.8 Zemmouri earthquake (Algeria) from InSAR time series. Geophys. Res. Lett. 2012, 39. [Google Scholar] [CrossRef]
- Jin, X.; Wang, S.; Jiang, X.; Zhang, L. Coseismic deformation and slip distribution of the MW 6.9 Menyuan, Qinghai earthquake revealed by Sentinel-1A SAR imagery. Prog. Geophys. 2022, 37, 2267–2274. [Google Scholar]
- Lv, X.; Fang, H.; Luo, G.; Zheng, L. Land subsidence and transportation routes deformation monitoring in Sanhe City based on joint PS/DS InSAR. Sci. Surv. Mapp. 2024, 49, 195–208. [Google Scholar]
- Li, J.; Jiang, H.; Wang, Q. Crustal attenuation structure of the Tianshan tectonic belt and its spatiotemporal variations. Front. Earth Sci. 2023, 11, 1094151. [Google Scholar] [CrossRef]
- Li, J.; Gao, Y.; Wang, Q. Anisotropic zoning in the upper crust of the Tianshan Tectonic Belt. Sci. China Earth Sci. 2021, 64, 651–666. [Google Scholar] [CrossRef]
- Li, Y.; Liu, Q.; Chen, J.; Li, S.; Guo, B.; Lai, Y. Shear wave velocity structure of the crust and upper mantle underneath the tianshan orogenic belt. Sci. China Ser. D Earth Sci. 2007, 50, 321–330. [Google Scholar] [CrossRef]
- Deng, Q. Research on Active Fault; Seismic Publishing House: Beijing, China, 1991. [Google Scholar]
- Wang, X.; Xu, C.; Wen, Y.; Zhao, X.; Wang, S.; Xu, G. Distribution of Interseismic Coupling Along the Maidan Fault in Tianshan Before the 2024 Mw 7.0 Wushi Earthquake. Geophys. Res. Lett. 2024, 51, e2024GL111472. [Google Scholar] [CrossRef]
- Wu, C.; Zheng, W.; Zhang, Z.; Jia, Q.; Yang, H. Large-earthquake rupturing and slipping behavior along the range-front Maidan fault in the southern Tian Shan, northwestern China. J. Asian Earth Sci. 2020, 190, 104193. [Google Scholar] [CrossRef]
- Xia, B.; Zhang, L.; Xia, Y.; Bader, T. The tectonic evolution of the Tianshan Orogenic Belt: Evidence from U–Pb dating of detrital zircons from the Chinese southwestern Tianshan accretionary mélange. Gondwana Res. 2014, 25, 1627–1643. [Google Scholar] [CrossRef]
- Wang, Q.; Ding, G.; Qiao, X.; Wang, X.; You, X. Recent rapid shortening of crust across the Tianshan Mts. and relative motion of tectonic blocks in the north and south. Chin. Sci. Bull. 2000, 45, 1995–1999. [Google Scholar] [CrossRef]
- Sun, M.; Tan, K.; Lu, X.; Zhang, C.; Li, Q.; Liu, Z. Three-dimensional Numerical Simulation of the Present Crustal Deformation and the Tectonic Stress Field in the Tianshan Region. J. Seismol. Res. 2022, 45, 535–542. [Google Scholar]
- Wu, C.; Zheng, W.; Zhang, P.; Zhang, Z.; Jia, Q.; Yu, J.; Zhang, H.; Yao, Y.; Liu, J.; Han, G.; et al. Oblique Thrust of the Maidan Fault and Late Quaternary Tectonic Deformation in the Southwestern Tian Shan, Northwestern China. Tectonics 2019, 38, 2625–2645. [Google Scholar] [CrossRef]
- Li, J.; Yao, Y.; Li, R.; Yusan, S.; Li, G.; Freymueller, J.T.; Wang, Q. Present-Day Strike-Slip Faulting and Thrusting of the Kepingtage Fold-and-Thrust Belt in Southern Tianshan: Constraints From GPS Observations. Geophys. Res. Lett. 2022, 49, e2022GL099105. [Google Scholar] [CrossRef]
- Wang, X. A Study on the Spatiotemporal Symmetrical Structure and Related Mechanisms of Earthquake Disasters in Active Fault Blocks in Western China. Master’s Thesis, Shanxi Normal University, Xi’an, China, 2021. [Google Scholar]
- Pang, Y.; Wu, Y.; Su, G.; Chen, C.; Yang, S.; Li, H. The stress evolution surrounding the Maidan fault in Southwest Tianshan and Implications for the future seismic hazard. Acta Petrol. Sin. 2024, 40, 4020–4029. [Google Scholar]
- Gulizinati, Y.; Gao, C.; Hairensha, S.; Zulipiya, B. Static coulomb stress triggering and its impact on surrounding faults of Jiashi MS6.4 earthquake on January 19, 2020. Inland Eartquake 2023, 37, 381–391. [Google Scholar]
- Guo, N.; Wu, Y.; Zhu, S.; Chen, C. Coseismic deformation and interseismic strain accumulation of the 2024 MS 7.1 Wushi earthquake in Xinjiang, China. Adv. Space Res. 2024, 74, 1586–1594. [Google Scholar] [CrossRef]
- Zheng, R.; Zou, R.; Dong, R.; Fang, Z.; Wang, Q. The 2024 Mw 7.0 Wushi Earthquake in Southern Tianshan Convergent Zone: Finite-Fault Model for the Coseismic Rupture and Aftershock. Seismol. Res. Lett. 2024, 96, 816–827. [Google Scholar] [CrossRef]
- Famiglietti, N.A.; Cheloni, D.; Caputo, R.; Vicari, A. Geodetic model of the 2024 January 22 Mw 7.0 Wushi (northwestern China) earthquake and Mw 5.7 aftershock from inversion of InSAR data. Geophys. J. Int. 2025, 241, 941–953. [Google Scholar] [CrossRef]
- Zhao, L.; Chen, Z.D.; Xie, L.; Zhu, Z.H.; Xu, W.B. Coseismic deformation and slip model of the 2024 MW7.0 Wushi earthquake obtained from InSAR observation. Rev. Geophys. Planet. Phys. 2024, 55, 453–460. (In Chinese) [Google Scholar]
- Rosen, P.A.; Gurrola, E.; Sacco, G.F.; Zebker, H. (Eds.) The InSAR scientific computing environment. In Proceedings of the EUSAR 2012—9th European Conference on Synthetic Aperture Radar, Nuremberg, Germany, 23–26 April 2012. [Google Scholar]
- Lv, X.; Shao, Y. Rheology of the Northern Tibetan Plateau Lithosphere Inferred from the Post-Seismic Deformation Resulting from the 2001 Mw 7.8 Kokoxili Earthquake. Remote Sens. 2022, 14, 1207. [Google Scholar] [CrossRef]
- Farr, T.G.; Rosen, P.A.; Caro, E.; Crippen, R.; Duren, R.; Hensley, S.; Kobrick, M.; Paller, M.; Rodriguez, E.; Roth, L.; et al. The Shuttle Radar Topography Mission. Rev. Geophys. 2007, 45. [Google Scholar] [CrossRef]
- Chen, C.W.; Zebker, H.A. Two-dimensional phase unwrapping with use of statistical models for cost functions in nonlinear optimization. J. Opt. Soc. Am. A 2001, 18, 338–351. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Fattahi, H.; Amelung, F. Small baseline InSAR time series analysis: Unwrapping error correction and noise reduction. Comput. Geosci. 2019, 133, 104331. [Google Scholar]
- Laske, G.; Masters, G.; Ma, Z.; Pasyanos, M.E. (Eds.) Update on CRUST1.0—A 1-degree Global Model of Earth’s Crust 2013. In Proceedings of the European Geosciences Union General Assembly 2013, Vienna, Austria, 7–12 April 2013. [Google Scholar]
- Wang, R.; Lorenzo-Martín, F.; Roth, F. PSGRN/PSCMP—A new code for calculating co- and post-seismic deformation, geoid and gravity changes based on the viscoelastic-gravitational dislocation theory. Comput Geosci. 2006, 32, 527–541. [Google Scholar] [CrossRef]
- Vasyura-Bathke, H.; Dettmer, J.; Steinberg, A.; Heimann, S.; Isken, M.P.; Zielke, O.; Mai, P.M.; Sudhaus, H.; Jónsson, S. The Bayesian Earthquake Analysis Tool. Seism. Res. Lett. 2020, 91, 1003–1018. [Google Scholar] [CrossRef]
- Vasyura-Bathke, H.; Dettmer, J.; Steinberg, A.; Heimann, S.; Isken, M.P.; Zielke, O.; Mai, P.M.; Sudhaus, H.; Jónsson, S. BEAT—Bayesian Earthquake Analysis Tool, V. 1.0; GFZ Data Services: Potsdam, Germany, 2019. [Google Scholar]
- Jouanne, F.; Awan, A.; Madji, A.A.; Pêcher, A.; Latif, M.; Kausar, A.; Mugnier, J.L.; Khan, I.; Khan, N.A. Postseismic deformation in Pakistan after the 8 October 2005 earthquake: Evidence of afterslip along a flat north of the Balakot-Bagh thrust. J. Geophys. Res. Solid Earth 2011, 116. [Google Scholar] [CrossRef]
- Jiang, G.; Wang, Y.; Wen, Y.; Liu, Y.; Xu, C.; Xu, C. Afterslip evolution on the crustal ramp of the Main Himalayan Thrust fault following the 2015 Mw 7.8 Gorkha (Nepal) earthquake. Tectonophysics 2019, 758, 29–43. [Google Scholar] [CrossRef]
- Tao, W.; Shen, Z. Heat flow distribution in Chinese continent and its adjacent areas. Prog. Nat. Sci. 2008, 18, 843–849. [Google Scholar] [CrossRef]
- Cheng, S.; Xiao, X.; Wu, J.; Wang, W.; Sun, L.; Wang, X.; Wen, L. Crustal thickness and Vp/Vs variation beneath continental China revealed by receiver function analysis. Geophys. J. Int. 2021, 228, 1731–1749. [Google Scholar] [CrossRef]
- Song, S.; Jia, K.; Hou, Y.; Hao, M.; Wang, Q.; Wu, J.; Zhou, S. Physics-based earthquake simulations of the Anninghe-Zemuhe-Daliangshan-Xiaojiang fault system in Southwestern China. Tectonophysics 2023, 859, 229854. [Google Scholar] [CrossRef]
- Toda, S.; Stein, R.S.; Sevilgen, V.; Lin, J. Coulomb 3.3 Graphic-Rich Deformation and Stress-Change Software for Earthquake, Tectonic, and Volcano Research and Teaching—User Guide; U.S. Geological Survey Open-File Report 2011–1060; 2011; 63p. Available online: https://pubs.usgs.gov/of/2011/1060/ (accessed on 6 April 2025).
- Doin, M.P.; Lasserre, C.; Peltzer, G.; Cavalié, O.; Doubre, C. Corrections of stratified tropospheric delays in SAR interferometry: Validation with global atmospheric models. J. Appl. Geophys. 2009, 69, 35–50. [Google Scholar] [CrossRef]
- Jolivet, R.; Agram, P.S.; Lin, N.Y.; Simons, M.; Doin, M.P.; Peltzer, G.; Li, Z. Improving InSAR geodesy using Global Atmospheric Models. J. Geophys. Res. Solid Earth 2014, 119, 2324–2341. [Google Scholar] [CrossRef]
- Jolivet, R.; Grandin, R.; Lasserre, C.; Doin, M.P.; Peltzer, G. Systematic InSAR atmospheric phase delay corrections from global meteorological reanalysis data. Geophys. Res. Lett. 2011, 38, L1731110. [Google Scholar] [CrossRef]
- Barbot, S.; Fialko, Y. Fourier-domain Green’s function for an elastic semi-infinite solid under gravity, with applications to earthquake and volcano deformation. Geophys. J. Int. 2010, 182, 568–582. [Google Scholar] [CrossRef]

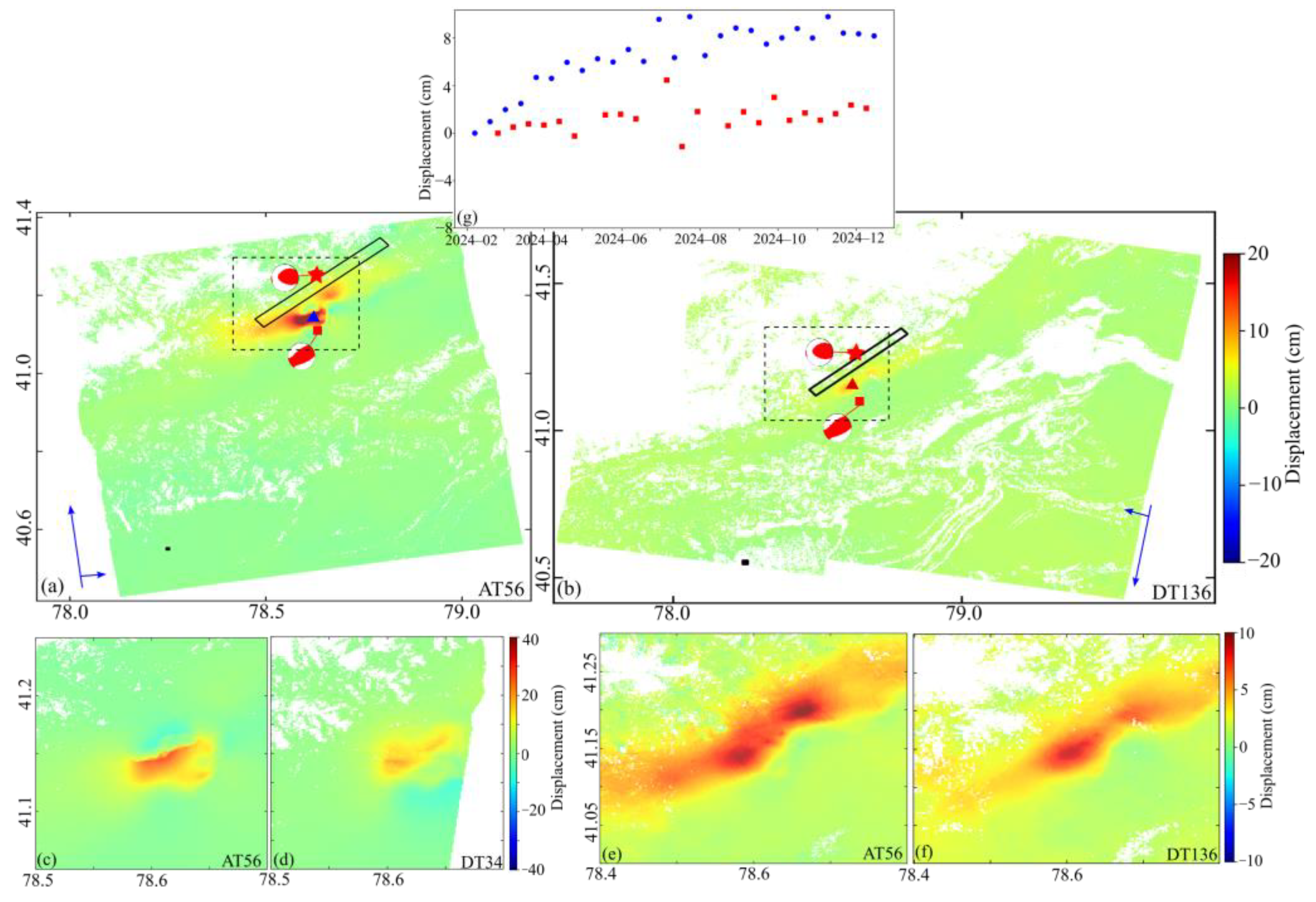



| Track | Time Period | Usage |
|---|---|---|
| AT56 | 26 January 2024–7 February 2024 | Aftershock |
| 7 February 2024–15 December 2024 | Afterslip | |
| DT136 | 25 February 2024–9 December 2024 | Afterslip |
| DT34 | 25 January 2024–6 February 2024 | Aftershock |
| Length (km) | Width (km) | Depth (km) | Dip (°) | Strike (°) | Rake (°) | Slip (m) | Lat * (°) | Lon * (°) | Mw | RMSE (cm) | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Segment1 (South segment) | 6.20 | 0.57 | |||||||||
| Min | 1 | 1 | 0 | 0 | 180 | 0 | 0 | 41.133 | 78.561 | ||
| Max | 15 | 15 | 5 | 90 | 270 | 180 | 1 | ||||
| Optimum | 9.38 | 12.81 | 4.33 | 48.8 | 228.3 | 41.1 | 0.18 | ||||
| 94% HDI | 3.52–14.99 | 9.56–15.00 | 3.18–5.00 | 40.0–57.7 | 211.5–247.6 | 19.3–63.9 | 0.06–0.35 | ||||
| Segment2 (Middle segment) | |||||||||||
| Min | 1 | 1 | 0 | 0 | 180 | 0 | 0 | 41.161 | 78.648 | ||
| Max | 15 | 15 | 5 | 90 | 270 | 180 | 1 | ||||
| Optimum | 7.73 | 6.32 | 2.85 | 48.7 | 230.6 | 75.6 | 0.30 | ||||
| 94% HDI | 6.63–8.99 | 3.64–8.86 | 2.33–3.43 | 41.9–55.2 | 224.9–236.5 | 63.6–88.3 | 0.21–0.41 | ||||
| Segment3 (North segment) | |||||||||||
| Min | 1 | 1 | 0 | 0 | 180 | 0 | 0 | 41.201 | 78.76 | ||
| Max | 15 | 15 | 5 | 90 | 270 | 180 | 1 | ||||
| Optimum | 10.02 | 7.74 | 2.46 | 53.7 | 255.7 | 68.3 | 0.21 | ||||
| 94% HDI | 4.90–12.51 | 4.25–10.89 | 1.80–3.20 | 46.3–59.9 | 248.7–262.9 | 56.4–80.5 | 0.12–0.35 | ||||
| Length (km) | Width (km) | Depth (km) | Dip (°) | Strike (°) | Rake (°) | Slip (m) | Lat * (°) | Lon * (°) | RMSE (cm) | |
|---|---|---|---|---|---|---|---|---|---|---|
| SW-dipping one-fault model | ||||||||||
| Optimum | 9 | 5 | 1.3 | 41 | 70 | 90 | 0.43 | 41.144 | 78.565 | 4.82 |
| NE-dipping one-fault model | ||||||||||
| Optimum | 4.09 | 5.53 | 2.19 | 61.35 | 240.83 | 116.08 | 1.16 | 41.140 | 78.628 | 4.91 |
| NE-dipping two-fault model | ||||||||||
| Optimum | 5.06 | 5.80 | 1.58 | 60.3 | 232.3 | 107.5 | 0.67 | 41.145 | 78.634 | 4.83 |
| 6.36 | 1.14 | 1.22 | 58.3 | 2.2 | 42.8 | 0.68 | 41.112 | 78.634 | ||
| SW-dipping two-fault model | ||||||||||
| Optimum | 5 | 8 | 0.12 | 60 | 61 | 108 | 6.5 | 41.140 | 78.588 | 6.11 |
| 4 | 3.5 | 0.11 | 76 | 191 | 178 | 0.38 | 41.160 | 78.662 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lv, X.; Luo, G.; Zheng, L.; Zhang, B.; Zhang, C. Early Post-Seismic Deformation Revealed After the Wushi (China) Earthquake (Mw = 7.1) Occurred on 22 January 2024. Remote Sens. 2025, 17, 1340. https://doi.org/10.3390/rs17081340
Lv X, Luo G, Zheng L, Zhang B, Zhang C. Early Post-Seismic Deformation Revealed After the Wushi (China) Earthquake (Mw = 7.1) Occurred on 22 January 2024. Remote Sensing. 2025; 17(8):1340. https://doi.org/10.3390/rs17081340
Chicago/Turabian StyleLv, Xiaoran, Guichun Luo, Lifu Zheng, Bozhi Zhang, and Chen Zhang. 2025. "Early Post-Seismic Deformation Revealed After the Wushi (China) Earthquake (Mw = 7.1) Occurred on 22 January 2024" Remote Sensing 17, no. 8: 1340. https://doi.org/10.3390/rs17081340
APA StyleLv, X., Luo, G., Zheng, L., Zhang, B., & Zhang, C. (2025). Early Post-Seismic Deformation Revealed After the Wushi (China) Earthquake (Mw = 7.1) Occurred on 22 January 2024. Remote Sensing, 17(8), 1340. https://doi.org/10.3390/rs17081340






