Linear Frequency Modulation and Orthogonal Code Modulation for Co-Located Multiple-Input Multiple-Output High-Frequency Surface Wave Radar
Abstract
:1. Introduction
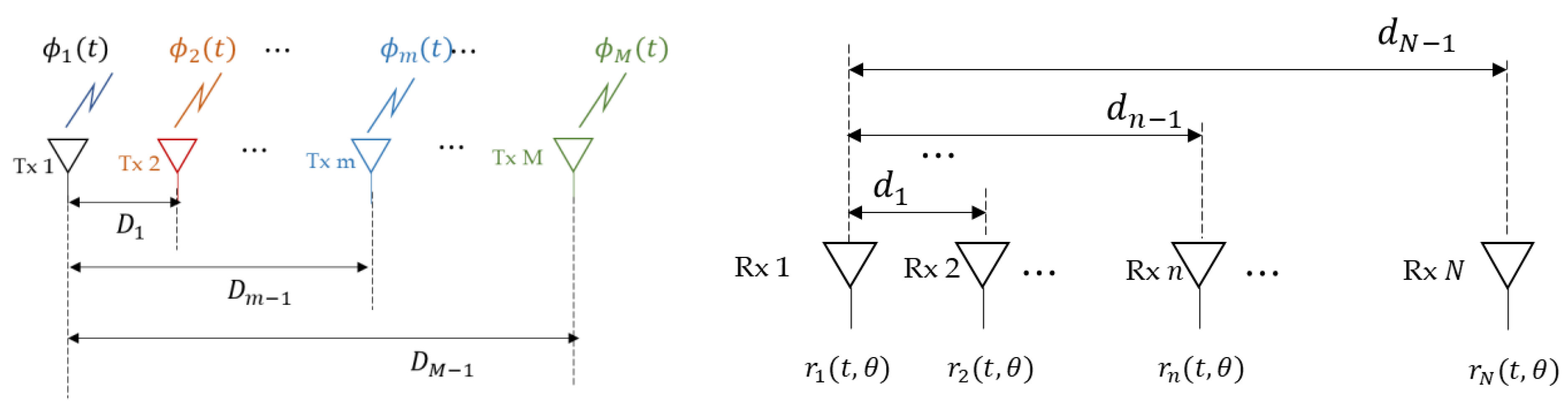
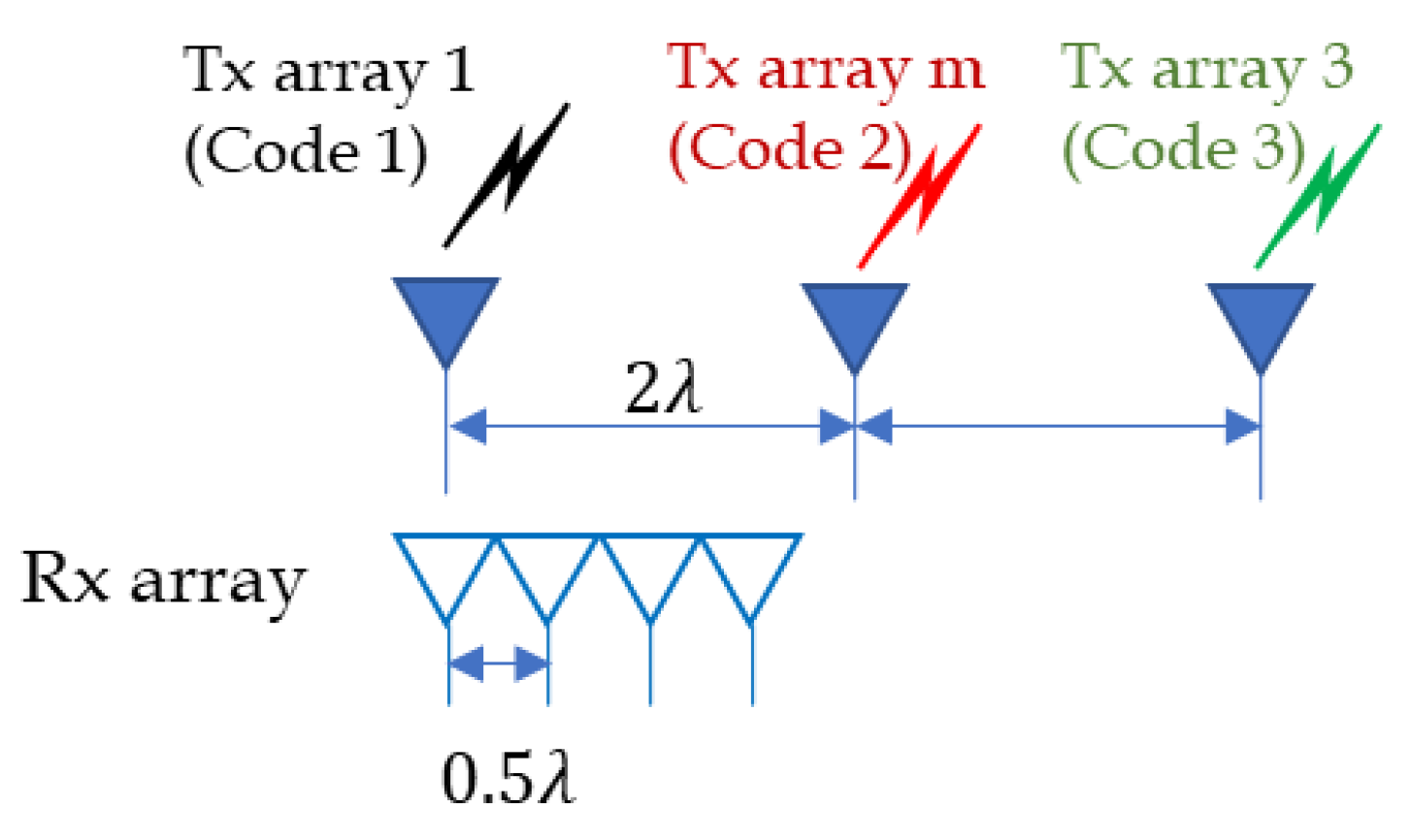
2. Summary of the Co-Located MIMO System
2.1. MIMO Virtual Antenna
2.2. Orthogonal Polyphase Codes
- If the p-th row vector of H is written as
3. HFSWR and the Proposed Method
3.1. HFSWR System
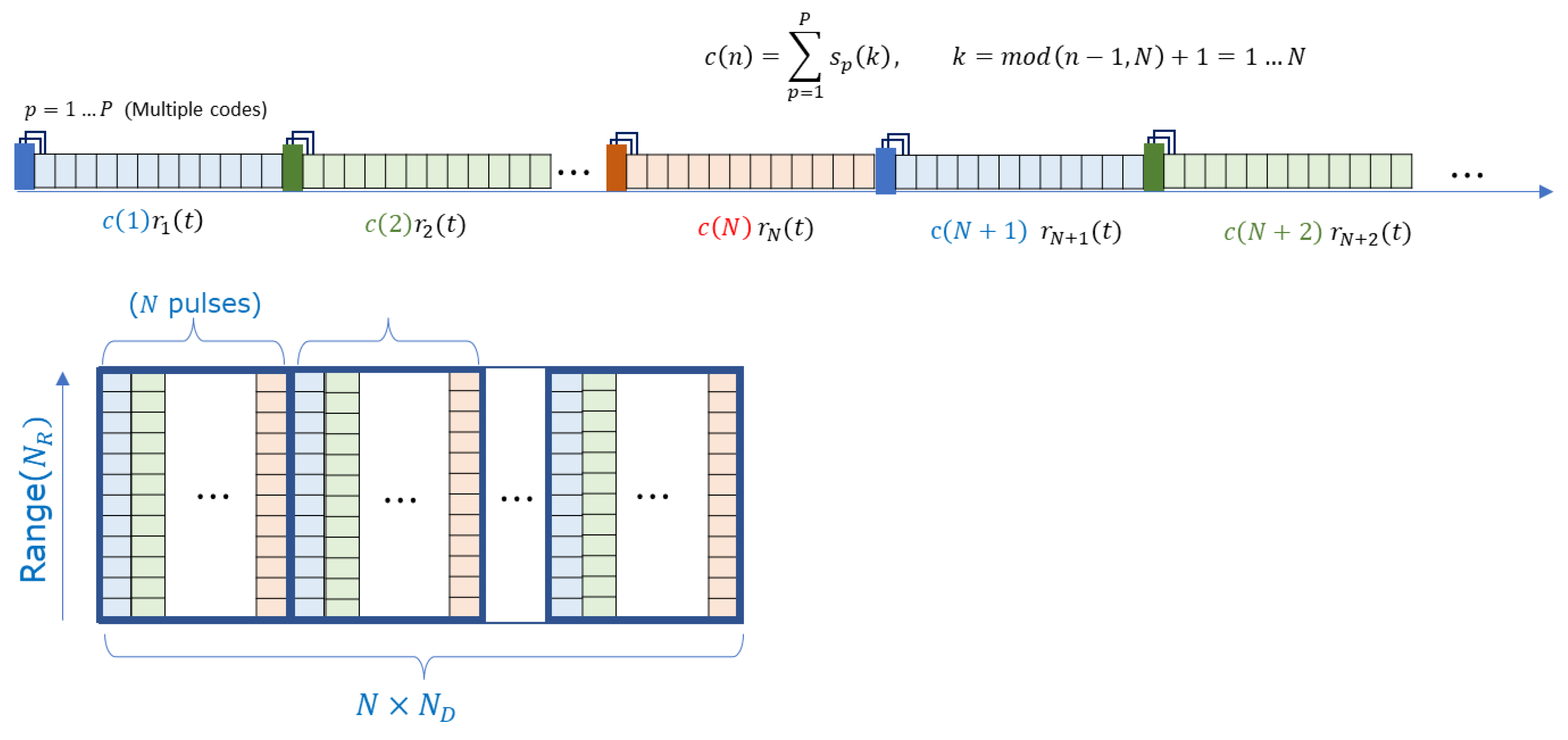
3.2. LFM and Orthogonal Code Modulation
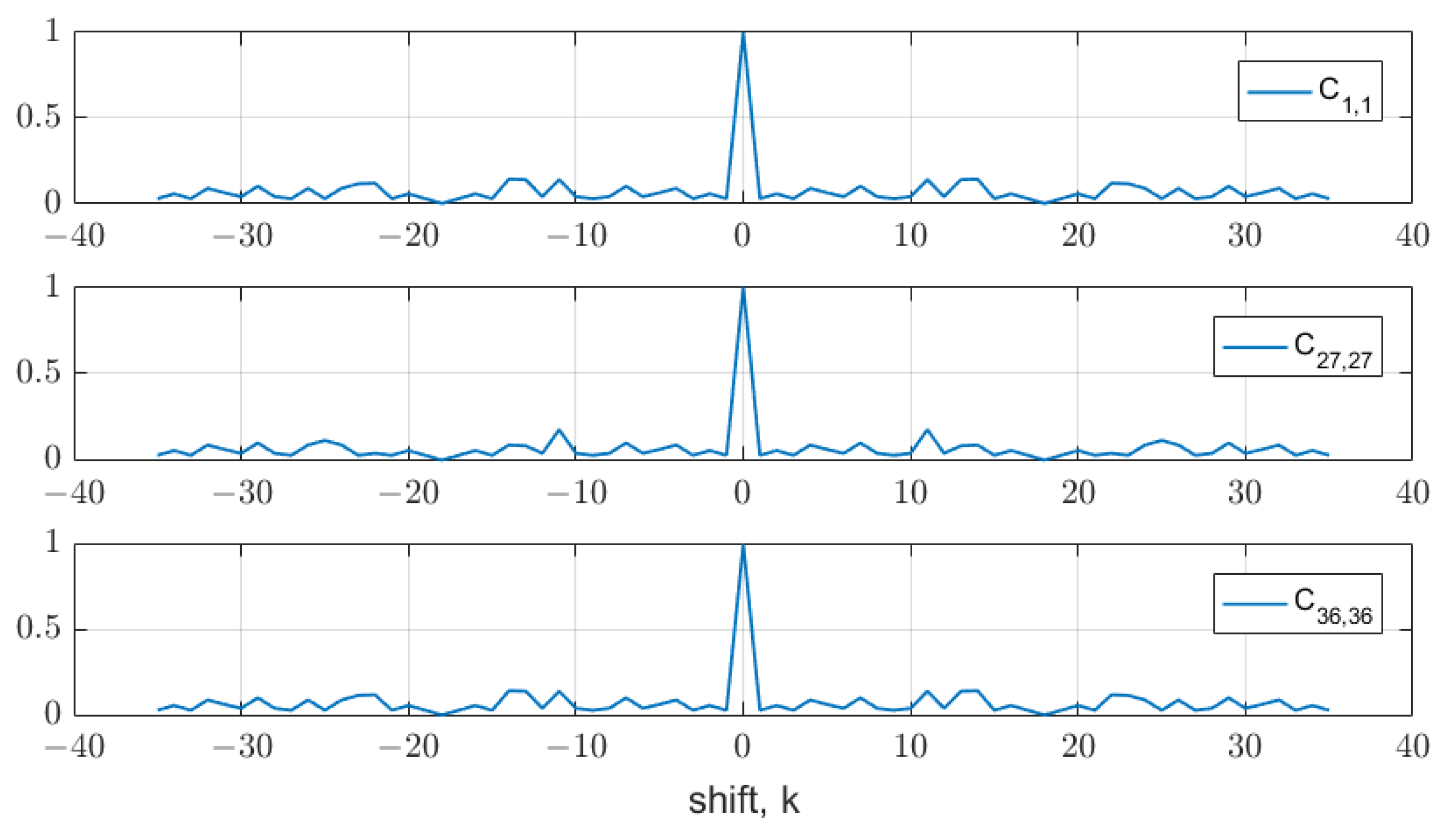
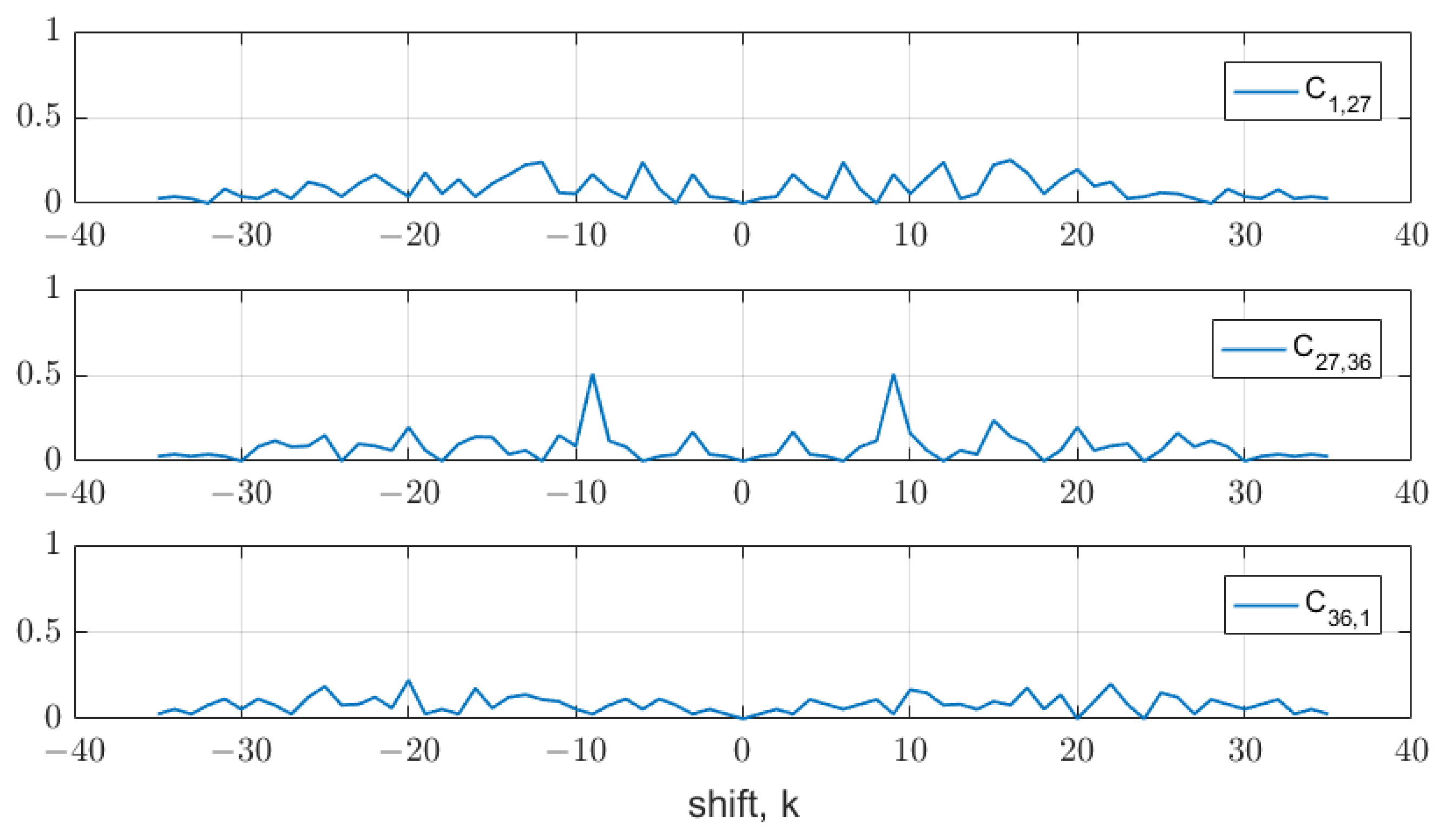
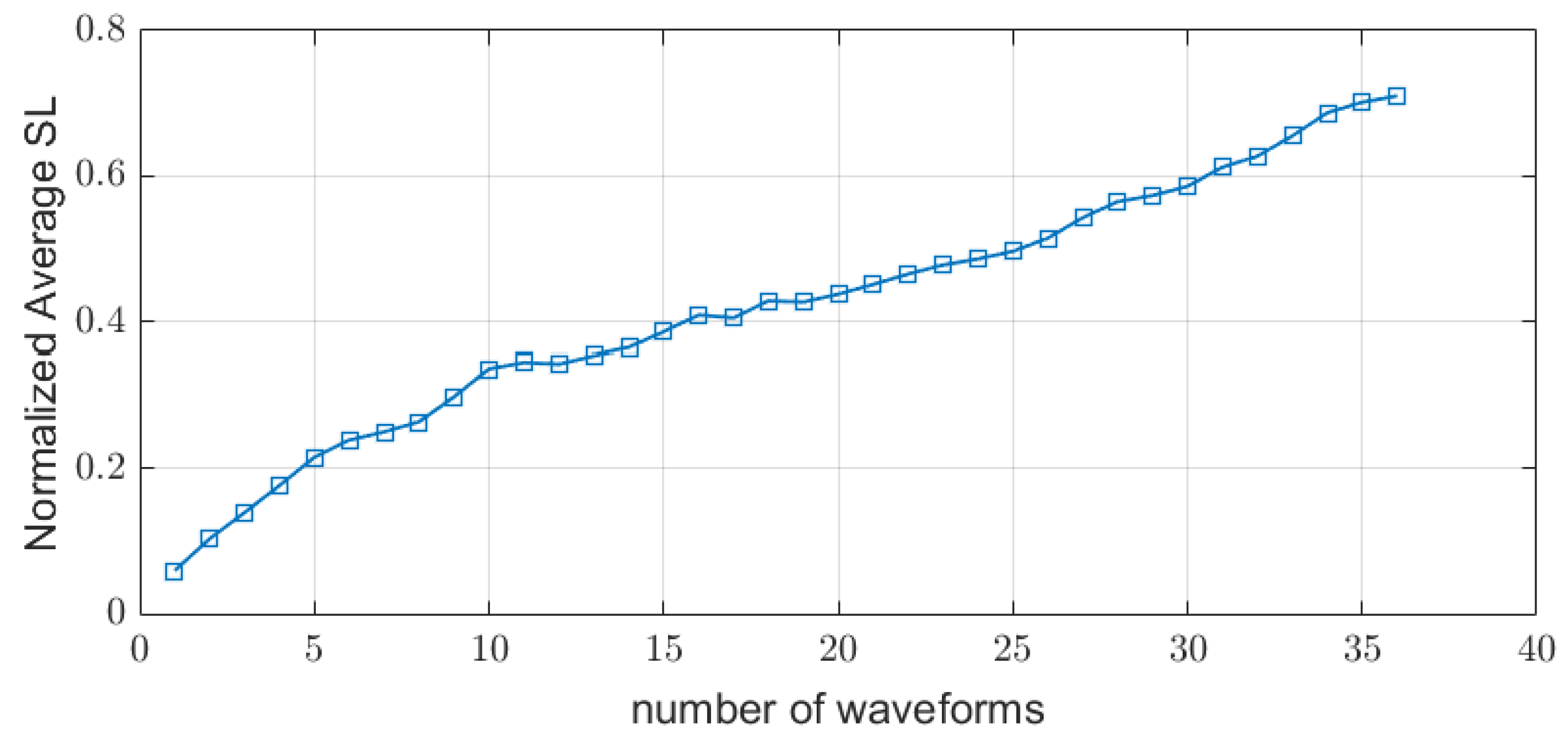
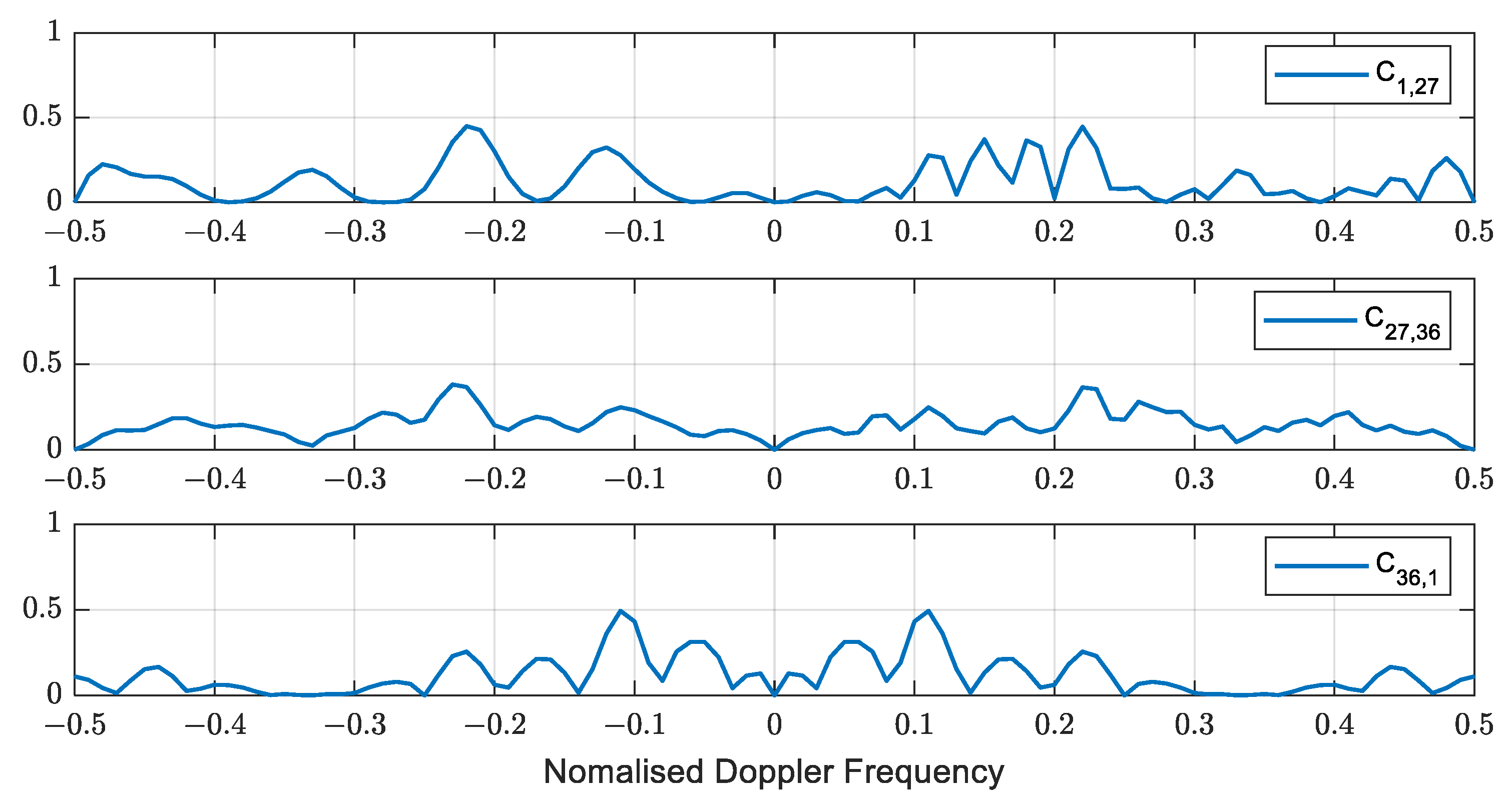
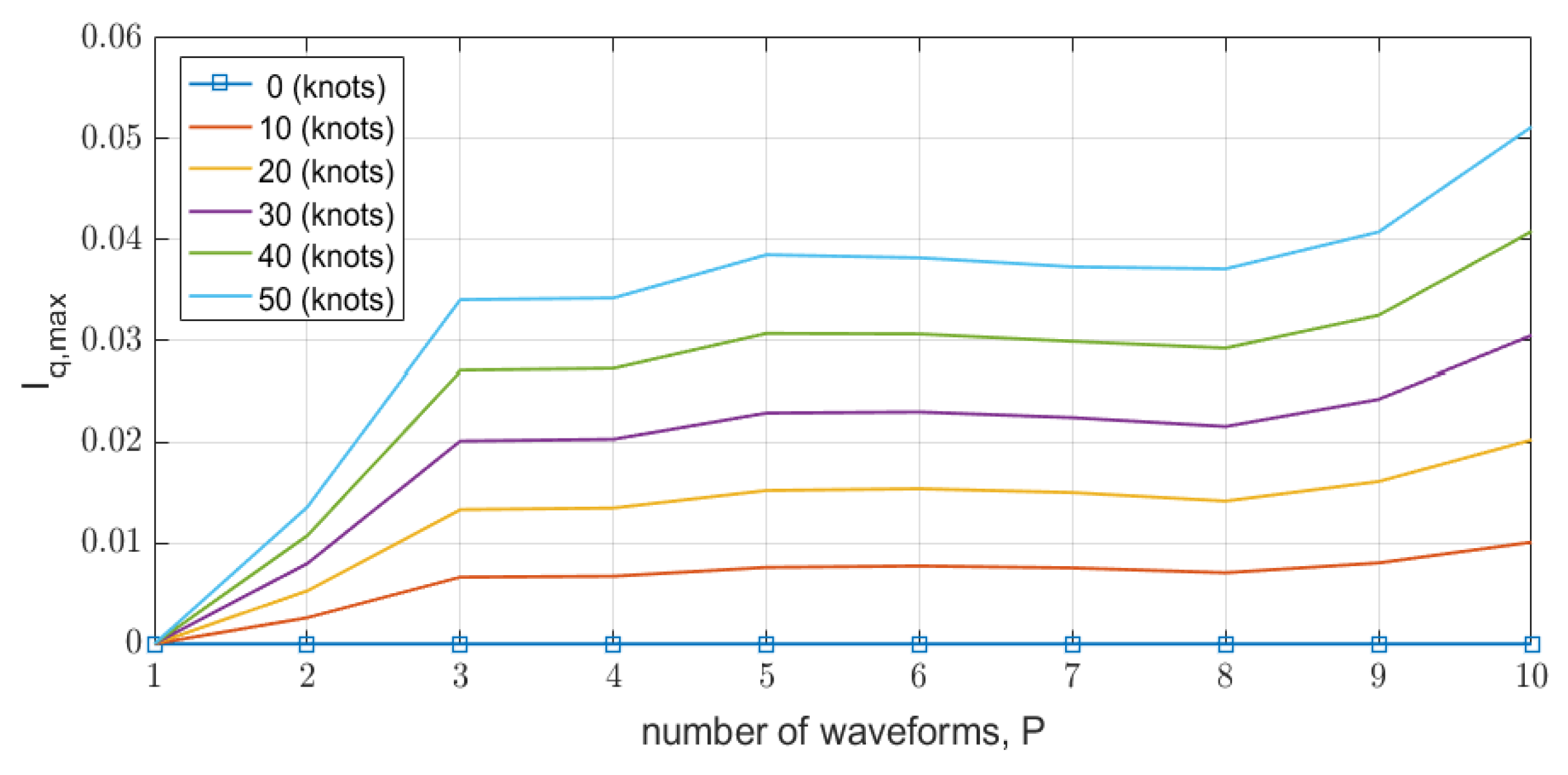
3.3. Doppler Insensitive Code Selection
4. Simulation
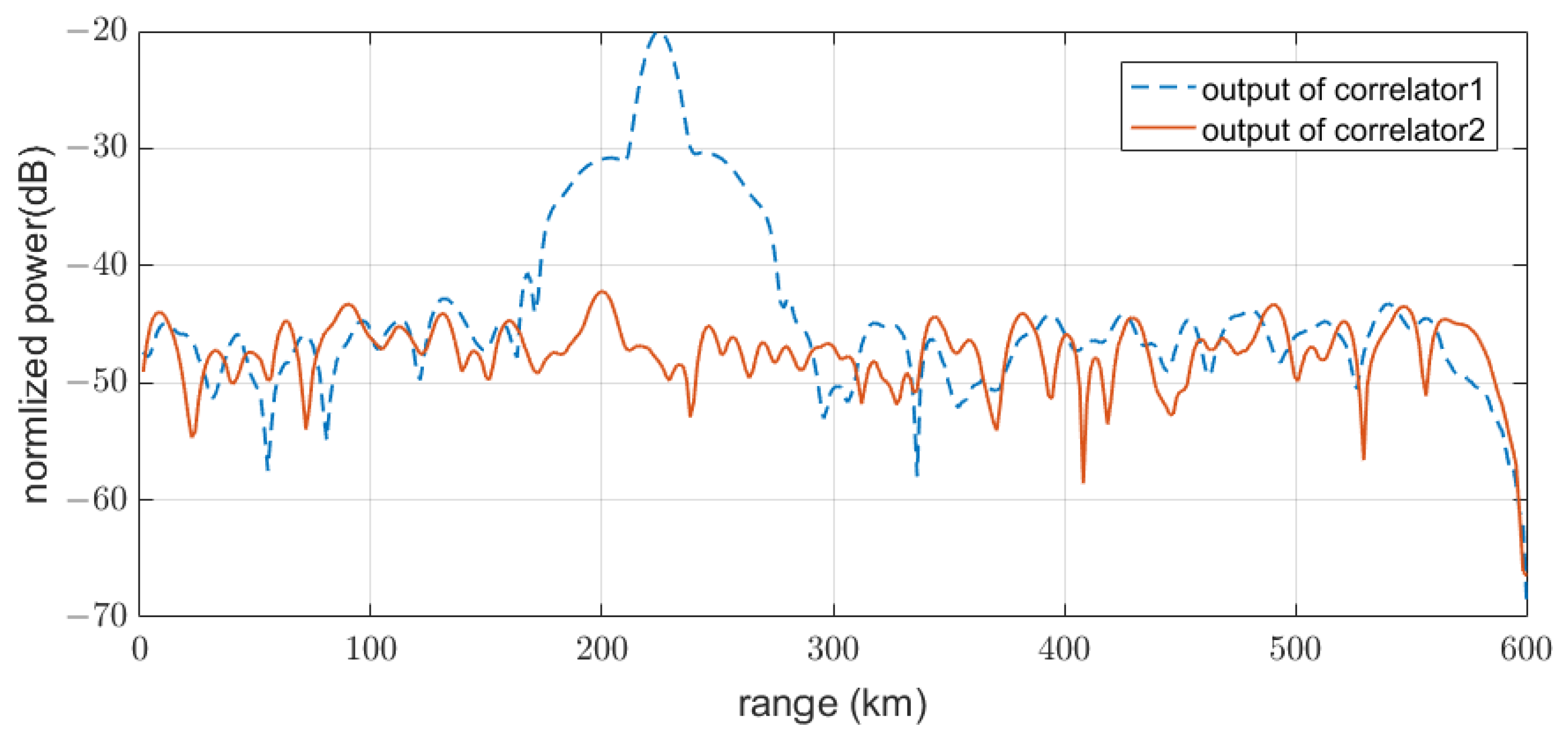
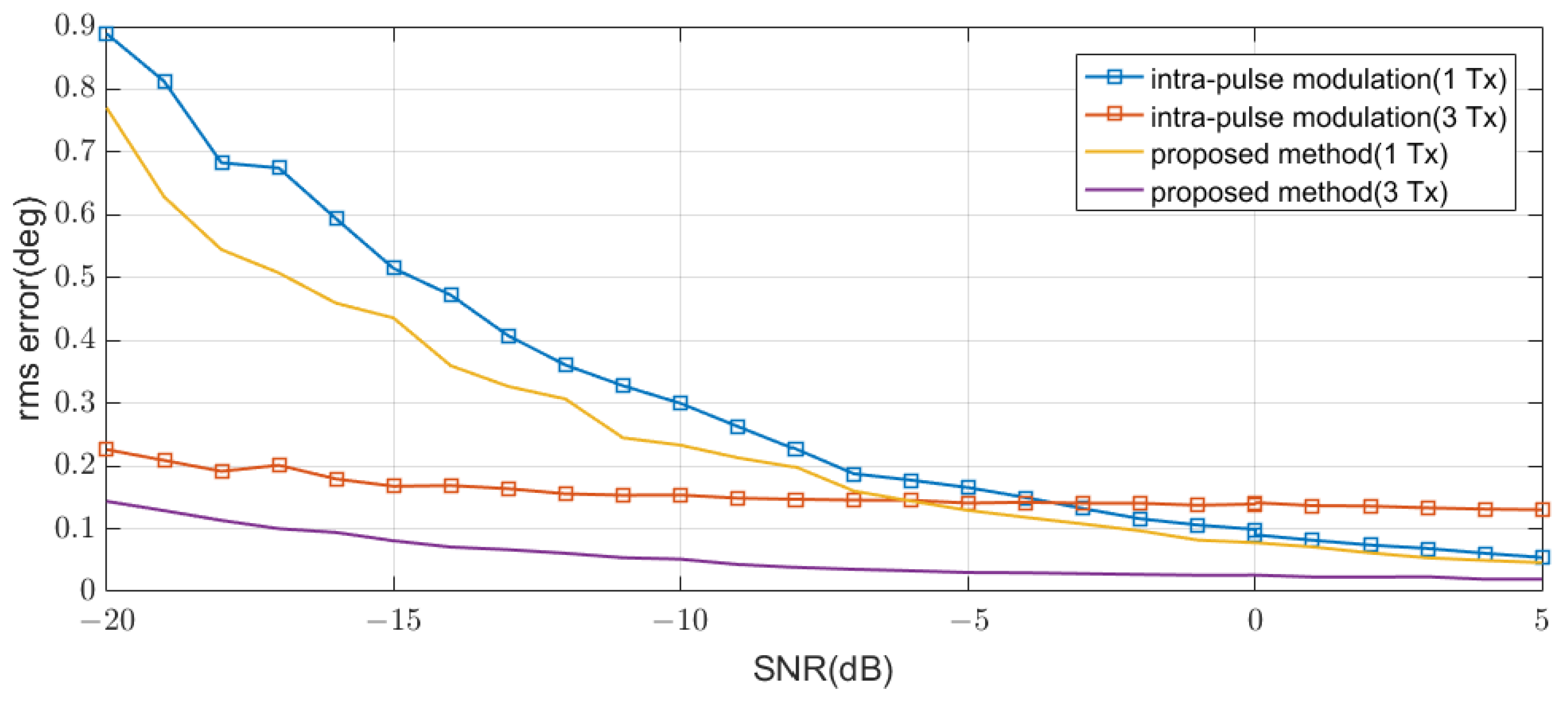
4.1. Comparison with Intra-Pulse Modulation
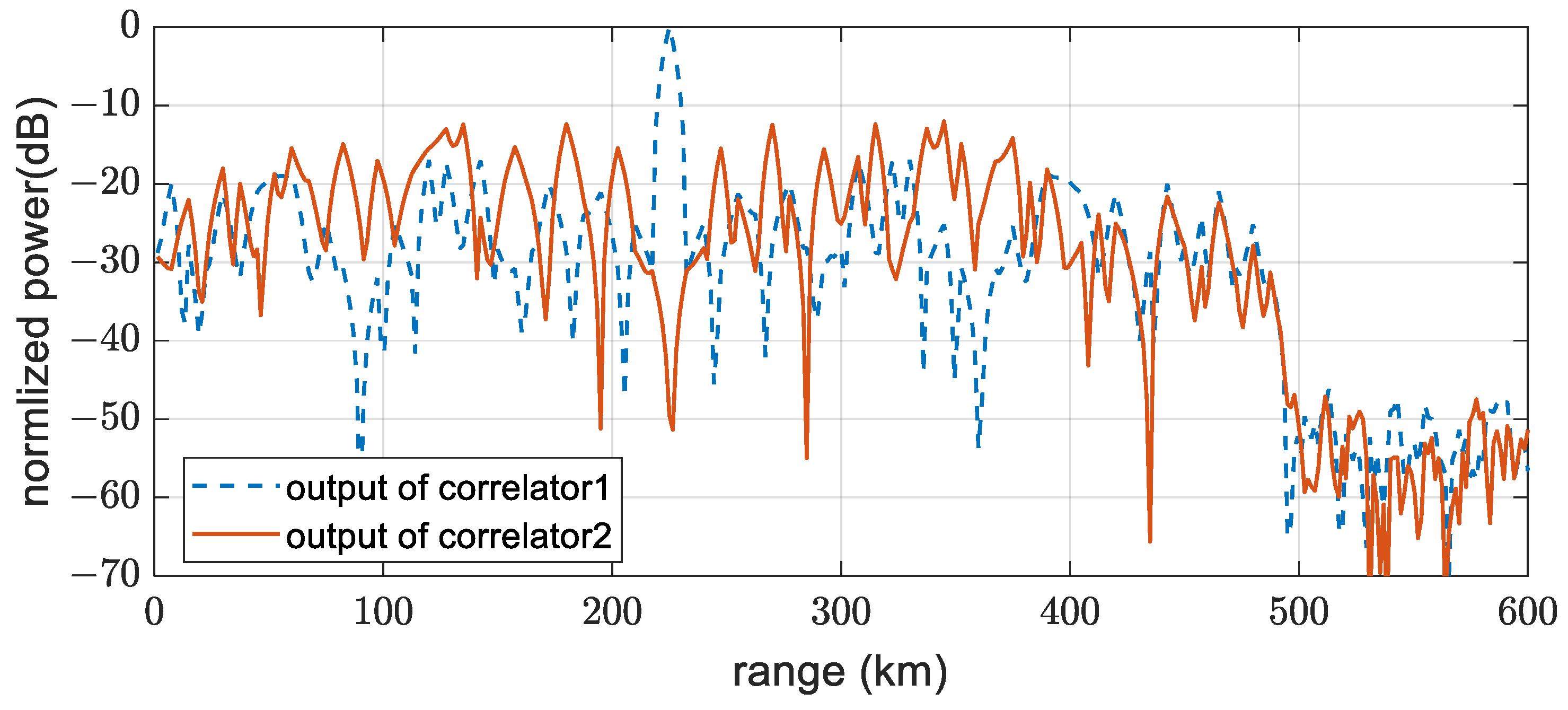
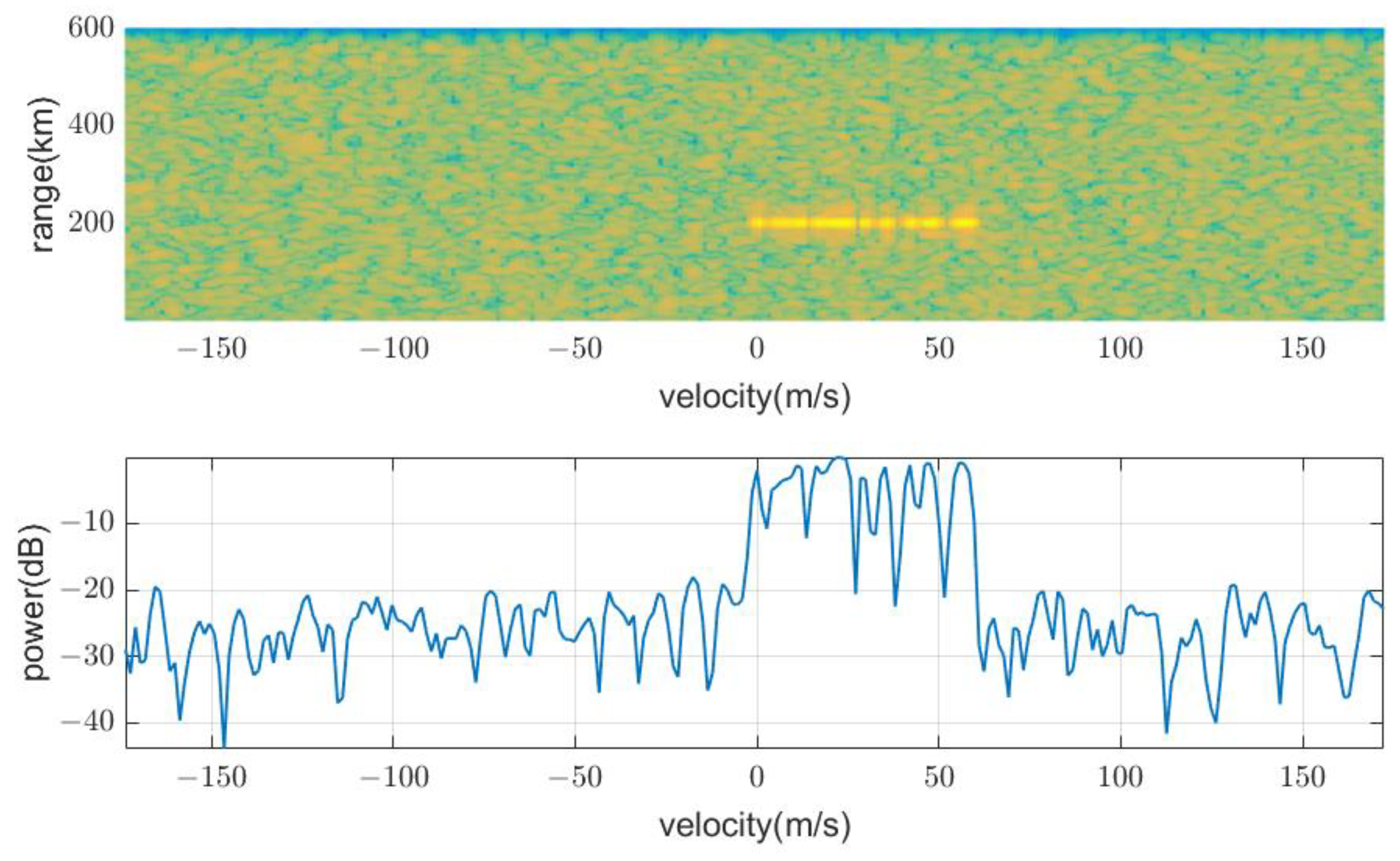
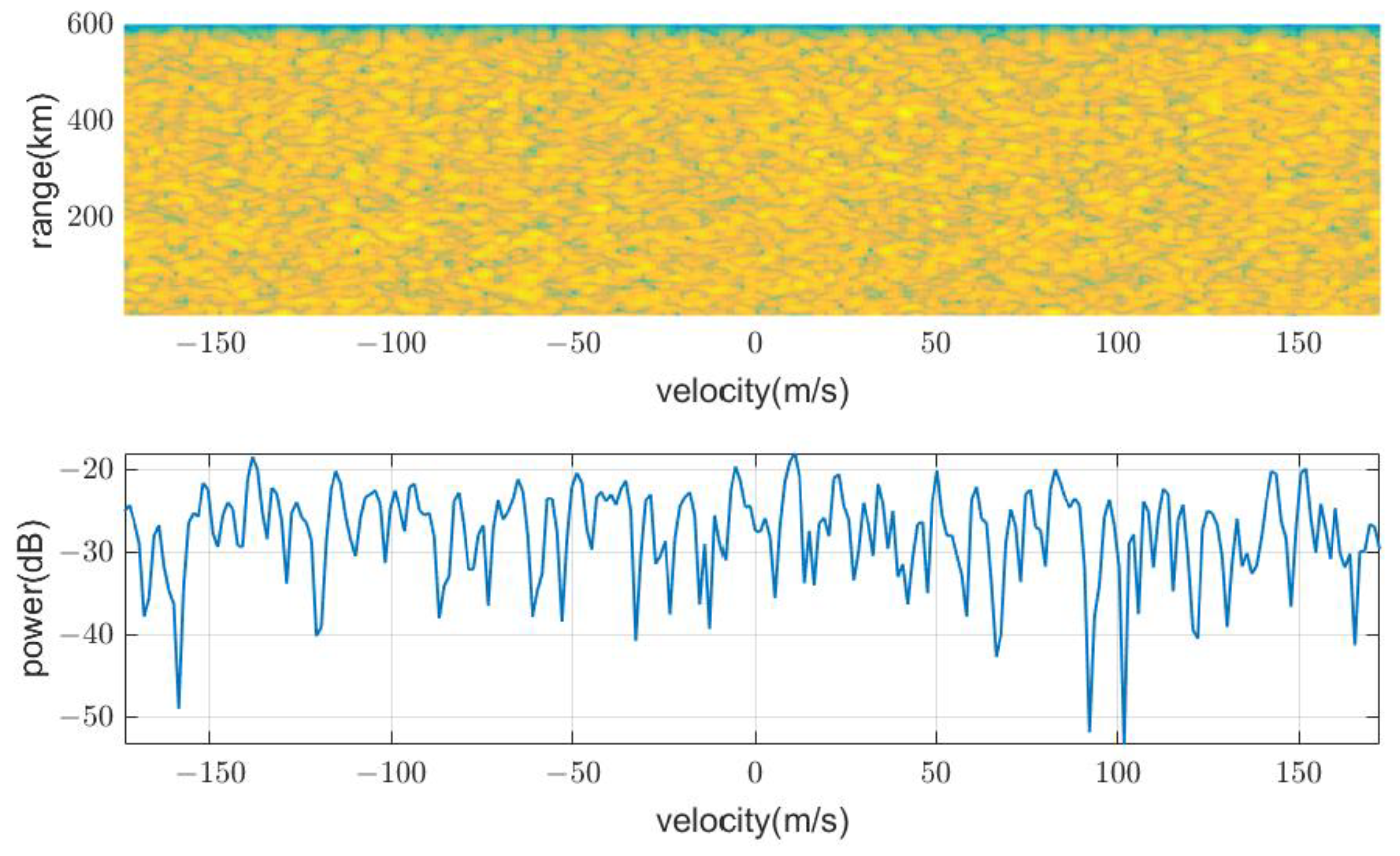
4.2. Effect of Removing Second-Time-around Echo
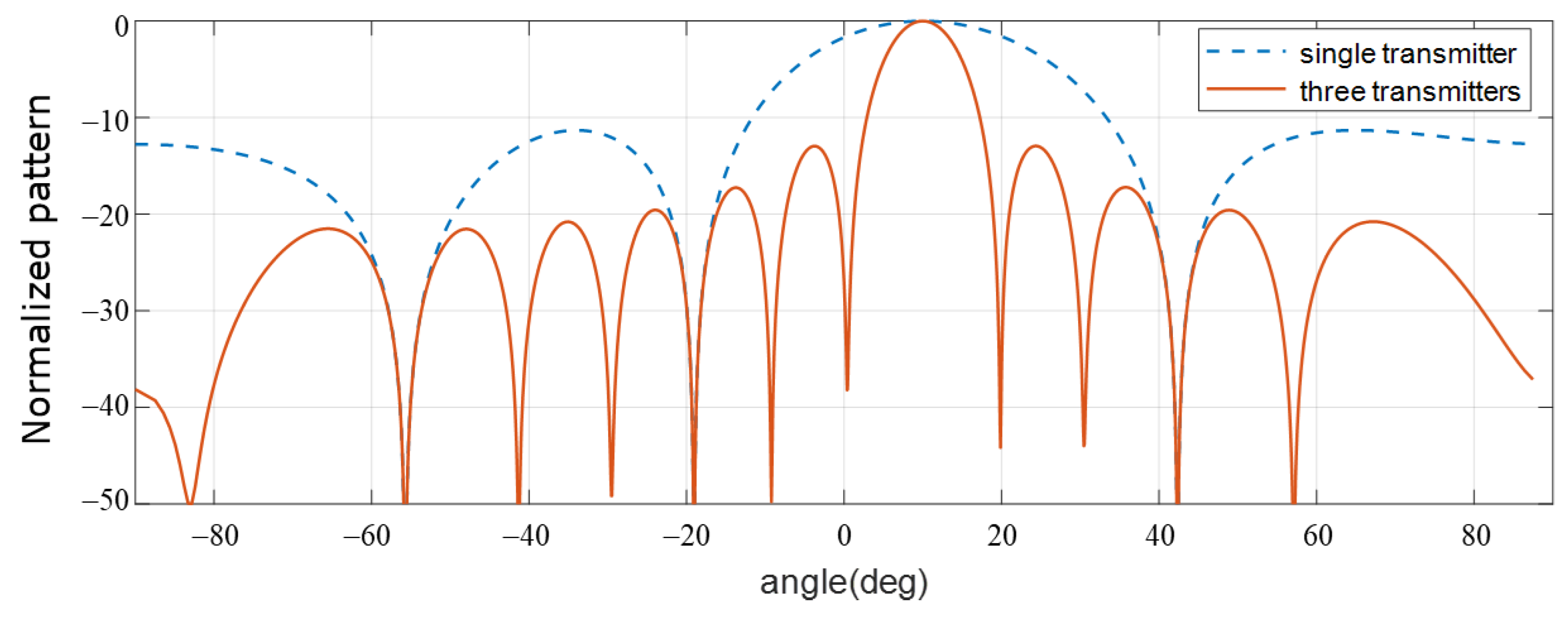
4.3. Improvement of Angle Resolution via Virtual Array Antenna
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sun, W.; Ji, M.; Huang, W.; Ji, Y.; Dai, Y. Vessel tracking using bistatic compact HFSWR. Remote Sens. 2020, 12, 1266. [Google Scholar] [CrossRef]
- Ponsford, T.; Wang, J. A review of high frequency surface wave radar for detection and tracking of ships. Turk. J. Electr. Eng. Comput. Sci. 2010, 18, 409–428. [Google Scholar] [CrossRef]
- Ponsford, A.M.; Dizaji, R.M.; Mckerracher, R. HF surface wave radar operation in adverse conditions. In Proceedings of the International Conference on Radar (IEEE Cat. No. 03EX695), Adelaide, SA, Australia, 3–5 September 2003; IEEE: Piscataway, NJ, USA, 2003; pp. 593–598. [Google Scholar]
- Headrick, J.M.; Stuart, J.A.; Merrill, S. HF over-the-horizon radar. In Radar Handbook; McGraw Hill: New York, NY, USA, 2008; Volume 20. [Google Scholar]
- Zhang, L.; Shi, C.; Niu, J.; Ji, Y.; Wu, Q.J. DOA estimation for HFSWR target based on PSO-ELM. IEEE Geosci. Remote Sens. Lett. 2021, 19, 3504205. [Google Scholar] [CrossRef]
- Chen, Z.; He, C.; Zhao, C.; Xie, F. Enhanced target detection for HFSWR by 2-D MUSIC based on sparse recovery. IEEE Geosci. Remote Sens. Lett. 2017, 14, 1983–1987. [Google Scholar] [CrossRef]
- Greiff, C.; Giovanneschi, F.; Gonzalez-Huici, M.A. Matrix pencil method for DoA estimation with interpolated arrays. In Proceedings of the 2020 IEEE International Radar Conference (RADAR), Washington, DC, USA, 28–30 April 2020; pp. 566–571. [Google Scholar]
- Zheng, G.; Chen, C.; Song, Y. Height Measurement for Meter Wave MIMO Radar based on Matrix Pencil Under Complex Terrain. IEEE Trans. Veh. Technol. 2023, 72, 11844–11854. [Google Scholar] [CrossRef]
- Yilmazer, Y.; Sarkar, T.K. Efficient computation of the azimuth and elevation angles of the sources by using unitary matrix pencil method (2-d ump). In Proceedings of the 2006 IEEE Antennas and Propagation Society International Symposium, Albuquerque, NM, USA, 9–14 July 2006; pp. 1145–1148. [Google Scholar]
- Xiao, J.J.; Nehorai, A. Optimal polarized beampattern synthesis using a vector antenna array. IEEE Trans. Signal Process. 2008, 57, 576–587. [Google Scholar] [CrossRef]
- Chintagunta, S. Joint 2D-DOA estimation of coherent targets using EV sensors in MIMO radar. Signal Process. 2022, 201, 108715. [Google Scholar] [CrossRef]
- Wen, F.; Shi, J.; Gui, G.; Gacanin, H.; Dobre, O.A. 3-D positioning method for anonymous UAV based on bistatic polarized MIMO radar. IEEE Internet Things J. 2022, 10, 815–827. [Google Scholar] [CrossRef]
- Liu, A.; Zhang, X.; Yang, Q.; Deng, W. DOA estimation with extended sparse and parametric approach in multi-carrier MIMO HFSWR. J. Eng. 2019, 21, 7810–7814. [Google Scholar] [CrossRef]
- Donnet, B.J.; Longstaff, I.D. MIMO radar, techniques and opportunities. In Proceedings of the 2006 European Radar Conference, Manchester, UK, 13–15 September 2006; IEEE: Piscataway, NJ, USA, 2006; pp. 112–115. [Google Scholar]
- Frazer, G.J.; Abramovich, Y.I.; Johnson, B.A.; Robey, F.C. Recent results in MIMO over-the-horizon radar. In Proceedings of the 2008 IEEE Radar Conference, Rome, Italy, 26–30 May 2008; pp. 1–6. [Google Scholar]
- Lesturgie, M. Some relevant applications of MIMO to radar. In Proceedings of the 2011 12th International Radar Symposium (IRS), Leipzig, Germany, 7–9 September 2011; IEEE: Piscataway, NJ, USA, 2011; pp. 714–721. [Google Scholar]
- Frazer, G.J.; Abramovich, Y.I.; Johnson, B.A. Spatially waveform diverse radar: Perspectives for high frequency OTHR. In Proceedings of the 2007 IEEE Radar Conference, Waltham, MA, USA, 17–20 April 2007; IEEE: Piscataway, NJ, USA, 2007; pp. 385–390. [Google Scholar]
- Willis, N.J. Bistatic Radar; SciTech Publishing: Raleigh, NC, USA, 2005. [Google Scholar]
- Riddolls, R.J. A Canadian Perspective on High-Frequency Over-the-Horizon Radar; Technical Report. DREO TR 285; Defense Research Development Canada: Ottawa, ON, USA, 2006.
- Daum, F.; Huang, J. MIMO radar: Snake oil or good idea? IEEE Aerosp. Electron. Syst. Mag. 2009, 24, 8–12. [Google Scholar] [CrossRef]
- Kim, E.H.; Kim, K.H. Random phase code for automotive MIMO radars using combined frequency shift keying-linear FMCW waveform. IET Radar Sonar Navig. 2018, 12, 1090–1095. [Google Scholar] [CrossRef]
- Blunt, S.D.; Mokole, E.L. Overview of radar waveform diversity. IEEE Aerosp. Electron. Syst. Mag. 2016, 31, 2–42. [Google Scholar] [CrossRef]
- Li, J.; Stoica, P. MIMO radar with colocated antennas. IEEE Signal Process. Mag. 2007, 24, 106–114. [Google Scholar] [CrossRef]
- Deng, H.; Geng, Z.; Himed, B. MIMO Radar Waveform Design for Transmit Beamforming and Orthogonality. IEEE Trans. Aerosp. Electron. Syst. 2016, 52, 1421–1433. [Google Scholar] [CrossRef]
- Liu, B. Orthogonal discrete frequency-coding waveform set design with minimized autocorrelation sidelobes. IEEE Trans. Aerosp. Electron. Syst. 2009, 45, 1650–1657. [Google Scholar] [CrossRef]
- Yu, C.; Chang, G.; Ji, Y.; Wang, Y.; Liu, A. DFCW-LFM analysis for MIMO HFSWR. In Proceedings of the 2016 CIE International Conference on Radar (RADAR), Guangzhou, China, 10–13 October 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 1–5. [Google Scholar]
- Khan, H.A.; Edwards, D.J. Doppler problems in orthogonal MIMO radars. In Proceedings of the 2006 IEEE Conference on Radar, Verona, NY, USA, 24-27 April 2006; pp. 244–247. [Google Scholar]
- Kim, E. MIMO FMCW Radar with Doppler-Insensitive Polyphase Codes. Remote Sens. 2022, 14, 2595. [Google Scholar] [CrossRef]
- Linwei, W.; Bo, L.; Changjun, Y.; Zhe, L. LFM-CCC orthogonal waveform design for MIMO-HFSWR. In Proceedings of the IET International Radar Conference (IET IRC 2020), Online, 4–6 November 2020; pp. 481–487. [Google Scholar]
- Chang, G.; Liu, A.; Yu, C.; Ji, Y.; Wang, Y.; Zhang, J. Orthogonal waveform with multiple diversities for MIMO radar. IEEE Sens. J. 2018, 18, 4462–4476. [Google Scholar] [CrossRef]
- Bergin, J.; Guerci, J.R. MIMO Radar: Theory and Application; Artech House: Norwood, MA, USA, 2018. [Google Scholar]
- Velazquez-Gutierrez, J.M.; Vargas-Rosales, C. Sequence sets in wireless communication systems: A survey. IEEE Commun. Surv. Tutor. 2016, 19, 1225–1248. [Google Scholar] [CrossRef]
- Frank, R.L. Polyphase Complementary-Codes. IEEE Trans. Inf. Theory 1980, 26, 641–647. [Google Scholar] [CrossRef]
- Ji, X.; Li, J.; Yang, Q. Annual Characteristic Analysis of Ionosphere Reflection from Middle-Latitude HF Over-the-Horizon Radar in the Northern Hemisphere. IEEE Trans. Geosci. Remote Sensing. 2023, 61, 5104117. [Google Scholar] [CrossRef]
- Yang, X.; Lie, A.; Yu, C.; Wang, L. Ionospheric Clutter model for HF Sky-wave path propagation with an FMCW source. Int. J. Antennas Propag. 2019, 2019, 1782942. [Google Scholar] [CrossRef]







| P | Row Number | P | Row Number |
|---|---|---|---|
| 1 | 1 | 6 | 7, 20, 24,27, 30, 32 |
| 2 | 19, 28 | 7 | 7, 9, 13, 15, 22, 31, 36 |
| 3 | 12, 26, 33 | 8 | 4, 5, 7, 8, 11, 13, 15, 26 |
| 4 | 2, 21, 27, 29 | 9 | 2, 5, 7, 9, 11, 13, 14, 16, 24 |
| 5 | 7, 12, 14, 16, 20 | 10 | 8, 13, 15, 22, 24, 25, 26, 29, 33, 35 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, E.; Sohn, S.; Moon, H.; Choi, J.H.; Lee, K. Linear Frequency Modulation and Orthogonal Code Modulation for Co-Located Multiple-Input Multiple-Output High-Frequency Surface Wave Radar. Remote Sens. 2024, 16, 104. https://doi.org/10.3390/rs16010104
Kim E, Sohn S, Moon H, Choi JH, Lee K. Linear Frequency Modulation and Orthogonal Code Modulation for Co-Located Multiple-Input Multiple-Output High-Frequency Surface Wave Radar. Remote Sensing. 2024; 16(1):104. https://doi.org/10.3390/rs16010104
Chicago/Turabian StyleKim, Eunhee, Sunghwan Sohn, Hyunwook Moon, Jun Hyeok Choi, and Kiwon Lee. 2024. "Linear Frequency Modulation and Orthogonal Code Modulation for Co-Located Multiple-Input Multiple-Output High-Frequency Surface Wave Radar" Remote Sensing 16, no. 1: 104. https://doi.org/10.3390/rs16010104
APA StyleKim, E., Sohn, S., Moon, H., Choi, J. H., & Lee, K. (2024). Linear Frequency Modulation and Orthogonal Code Modulation for Co-Located Multiple-Input Multiple-Output High-Frequency Surface Wave Radar. Remote Sensing, 16(1), 104. https://doi.org/10.3390/rs16010104






