Enhanced Doppler Resolution and Sidelobe Suppression Performance for Golay Complementary Waveforms
Abstract
1. Introduction
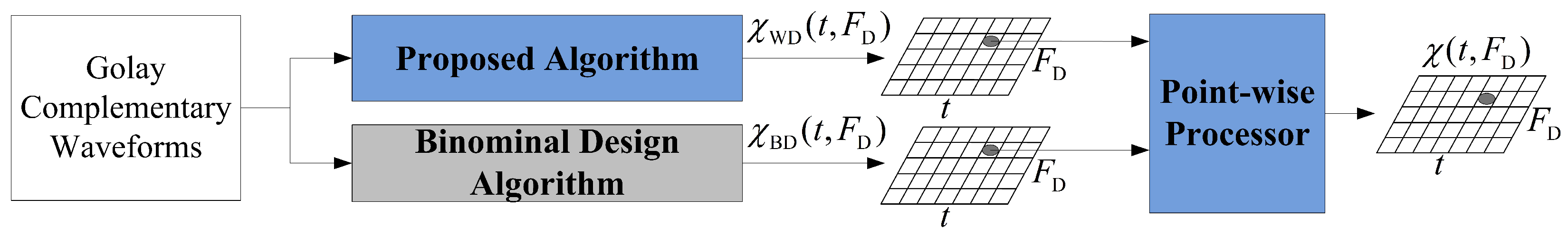
2. Golay Complementary Waveforms and Pointwise Processors
2.1. Golay Pairs
2.2. Pointwise Minimization Procedure
2.3. Pointwise Thresholding Procedure
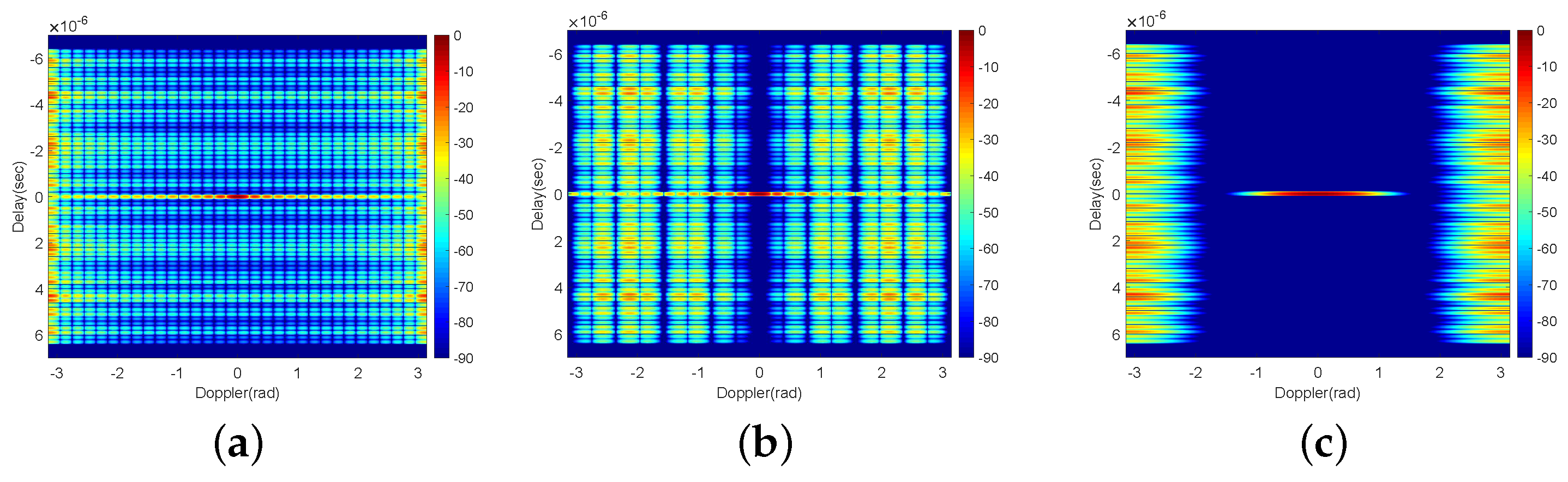
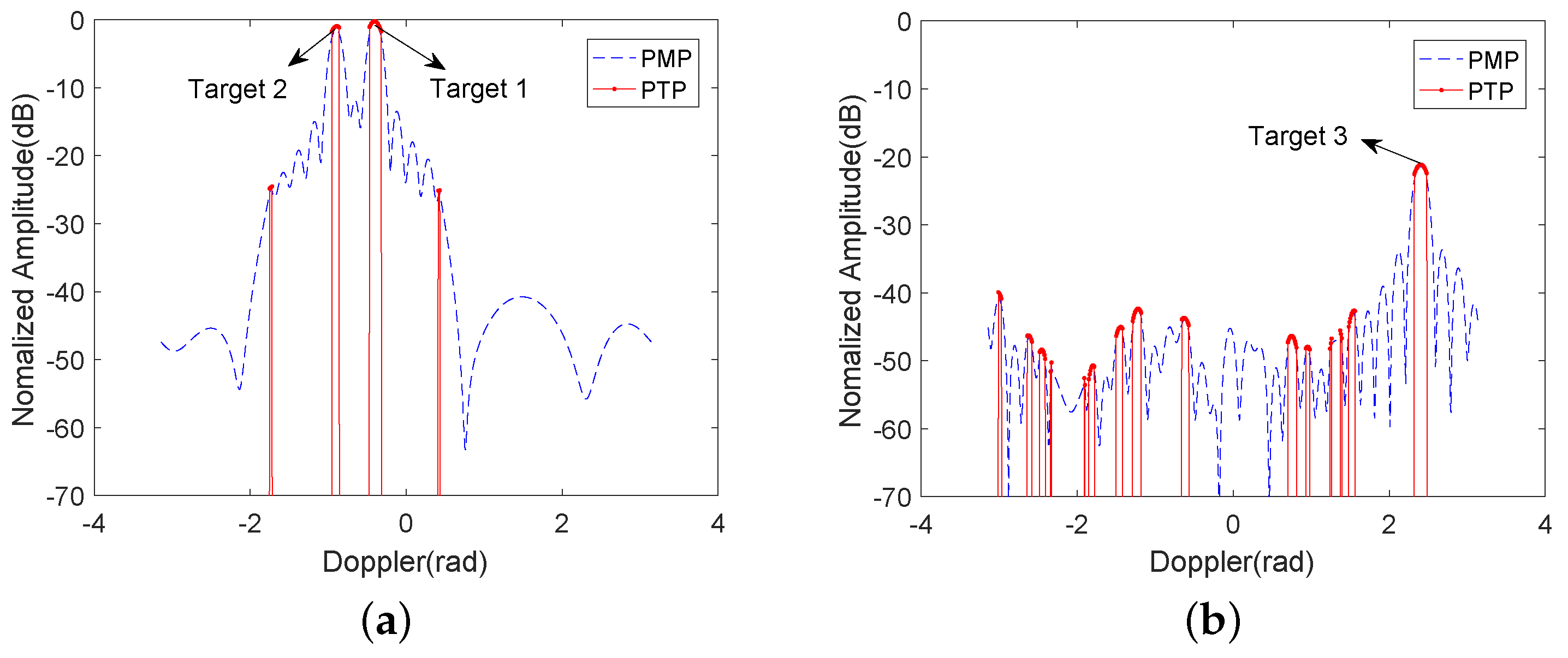
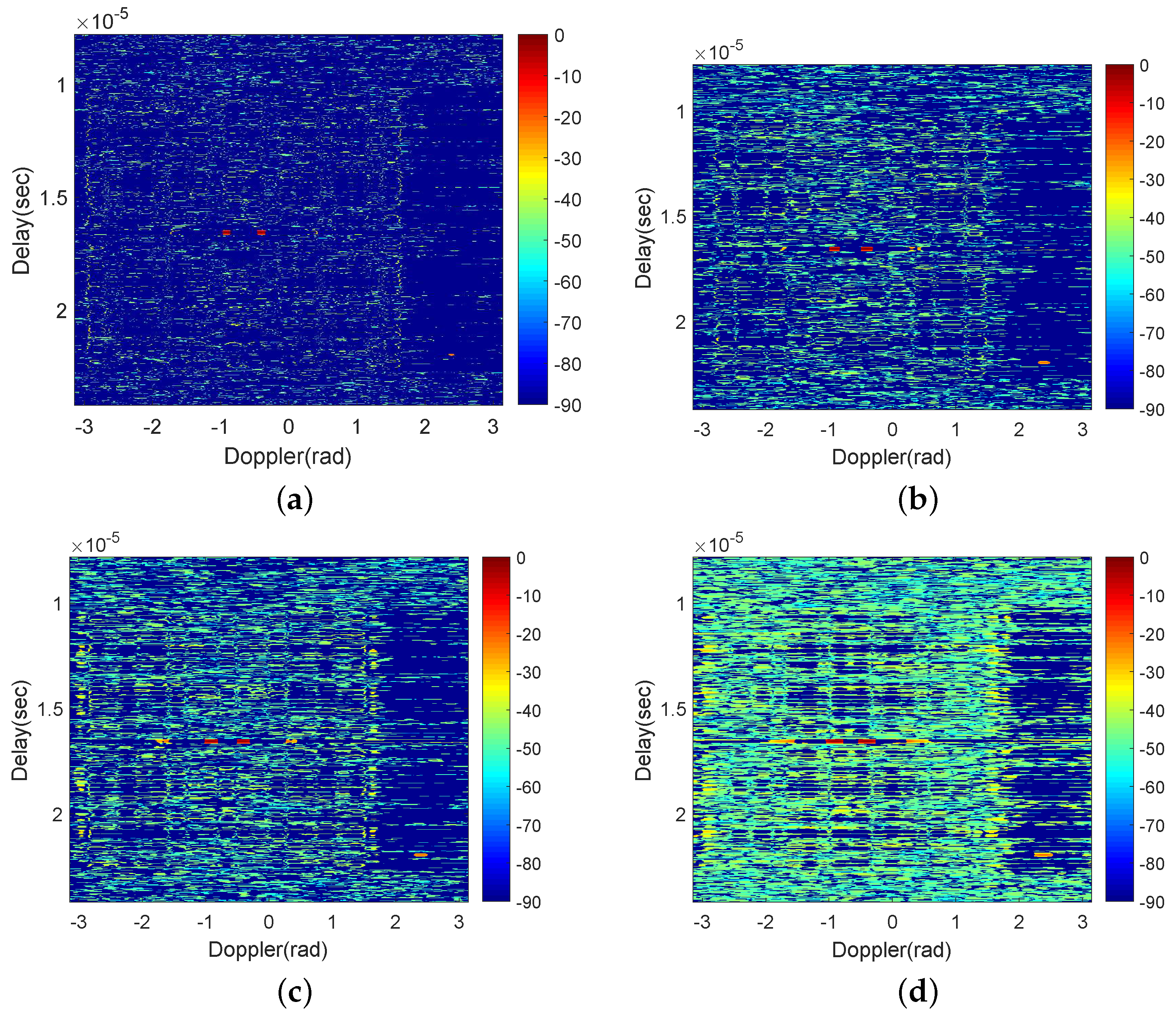
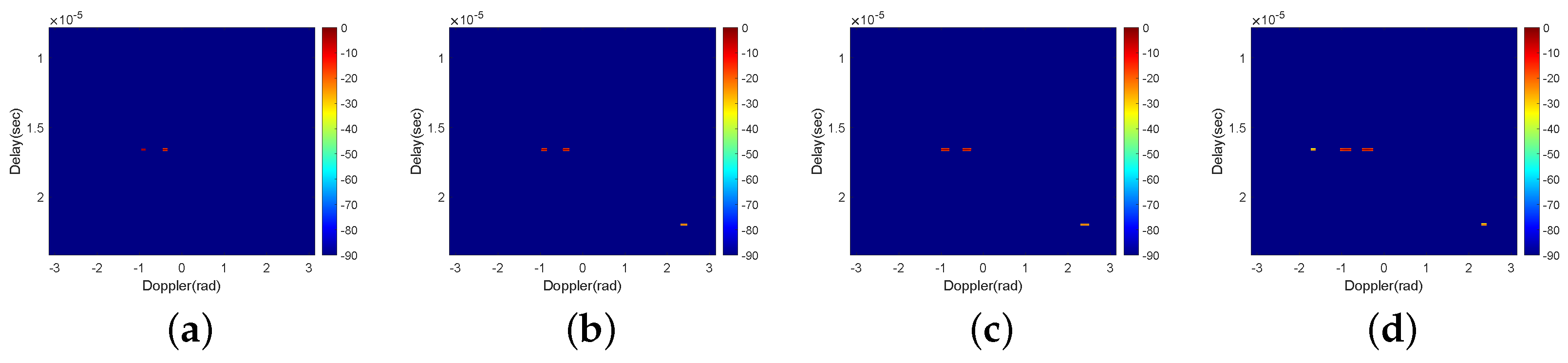
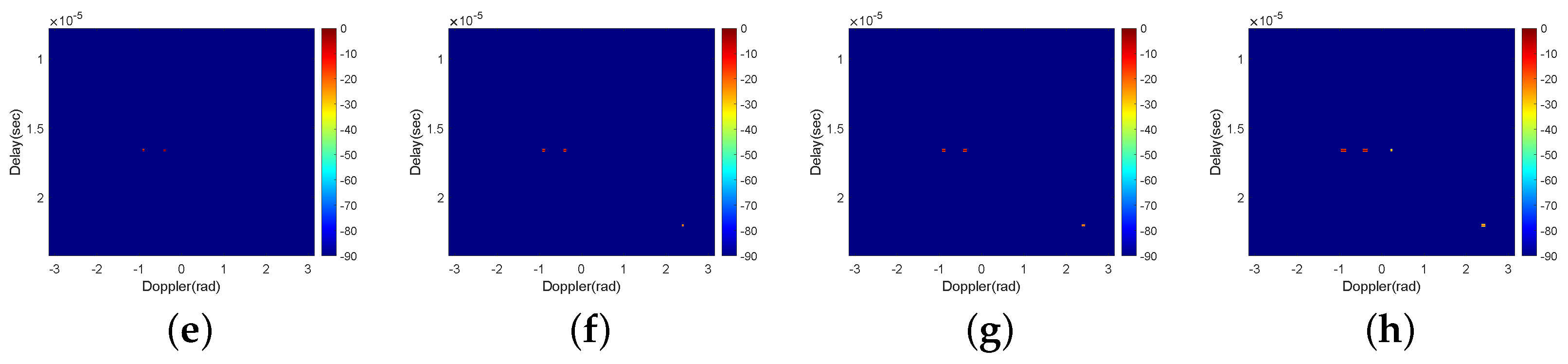
3. Simulation and Further Discussion
- , carrier frequency: 1 GHz;
- B, bandwidth: 50 MHz;
- , time sampling rate: 2 B;
- , Doppler sampling rate: 0.01 rad;
- T, PRI: 50 μs;
- N, pulse number: 32;
- L, chip number of Golay pair: 64;
- , chip interval: 0.1 μs;
- E∼, complex Gaussian zero-mean white noise: −10 dB (i.e., = 10 dB).
3.1. Fixed Scenario
3.2. Further Filtering for the PTP
3.3. Randomized Scenario
- (1)
- Target number: 2 (one strong and one weak);
- (2)
- Target number: 3 (one strong and two weak);
- (3)
- Target number: 4 (two strong and two weak);
- (4)
- Target number: 5 (three strong and two weak).
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pezeshki, A.; Calderbank, A.R.; Moran, W.; Howard, S.D. Doppler resilient Golay complementary waveforms. IEEE Trans. Inform. Theory 2008, 54, 4254–4266. [Google Scholar] [CrossRef]
- Calderbank, R.; Howard, S.; Moran, B. Waveform diversity in radar signal processing. IEEE Signal Process. Mag. 2009, 26, 32–41. [Google Scholar] [CrossRef]
- Suvorova, S.; Howard, S.; Moran, B.; Calderbank, R.; Pezeshki, A. Doppler resilience, Reed-Muller codes and complementary waveforms. In Proceedings of the 2007 Conference Record of the Forty-First Asilomar Conference on Signals, Systems and Computers, Pacific Grove, CA, USA, 4–7 November 2007; pp. 1839–1843. [Google Scholar]
- Dang, W.; Pezeshki, A.; Howard, S.; Moran, W.; Calderbank, R. Coordinating complementary waveforms for sidelobe suppression. In Proceedings of the 2011 Conference Record of the Forty Fifth Asilomar Conference on Signals, Systems and Computers (ASILOMAR), Pacific Grove, CA, USA, 6–9 November 2011; pp. 2096–2100. [Google Scholar]
- Wu, Z.; Wang, C.; Jiang, P.; Zhuo, Z. Range-Doppler sidelobe suppression for pulsed radar based on Golay complementary codes. IEEE Signal Process. Lett. 2020, 27, 1205–1209. [Google Scholar] [CrossRef]
- Wu, Z.; Wang, C.; Jiang, P.; Zhuo, Z. Doppler resilient complementary waveform design for active sensing. IEEE Sen. J. 2020, 20, 9963–9976. [Google Scholar] [CrossRef]
- Zhu, J.; Song, Y.; Fan, C.; Huang, X. Nonlinear processing for enhanced delay-Doppler resolution of multiple targets based on an improved radar waveform. Signal Process. 2017, 130, 355–364. [Google Scholar] [CrossRef]
- Richards, M.A.; Scheer, J.A.; Holm, W.A. Principles of Modern Radar Volume I-Basic Principles; Scitech Publishing: Raleigh, NC, USA, 2010. [Google Scholar]
- Ciuonzo, D. On time-reversal imaging by statistical testing. IEEE Signal Process. Lett. 2017, 24, 1024–1028. [Google Scholar] [CrossRef]
- Zhu, J.; Wang, X.; Huang, X.; Suvorova, S.; Moran, B. Range sidelobe suppression for using Golay complementary waveforms in multiple moving target detection. Signal Process. 2017, 141, 28–31. [Google Scholar] [CrossRef]
- Golay, M. Complementary series. IRE Trans. Inform. Theory 1961, 7, 82–87. [Google Scholar] [CrossRef]
- Richards, M.A. Fundamentals of Radar Signal Processing; McGraw-Hill Education: New York, NY, USA, 2005. [Google Scholar]
- Zhu, J.; Wang, X.; Huang, X.; Suvorova, S.; Moran, B. Golay Complementary Waveforms in Reed-Müller Sequences for Radar Detection of Nonzero Doppler Targets. Sensors 2018, 18, 192. [Google Scholar] [CrossRef] [PubMed]
- Dang, W. Signal Design for Active Sensing. Ph.D. Dissertation, Colorado State University, Fort Collins, CO, USA, 2014. [Google Scholar]
- Rasool, S.B.; Bell, M.R. Biologically inspired processing of radar waveforms for enhanced delay-Doppler resolution. IEEE Trans. Signal Process. 2011, 59, 2698–2709. [Google Scholar] [CrossRef]
- Xie, Z.; Fan, C.; Xu, Z.; Zhu, J.; Huang, X. Robust joint code-filter design under uncertain target interpulse fluctuation. Signal Process. 2022, 201, 108687. [Google Scholar] [CrossRef]
- Xie, Z.; Xu, Z.; Fan, C.; Han, S.; Huang, X. Robust radar waveform optimization under target interpulse fluctuation and practical constraints via sequential lagrange dual approximation. IEEE Trans. Aero. Electron. Syst. 2023; accepted for publication. [Google Scholar] [CrossRef]
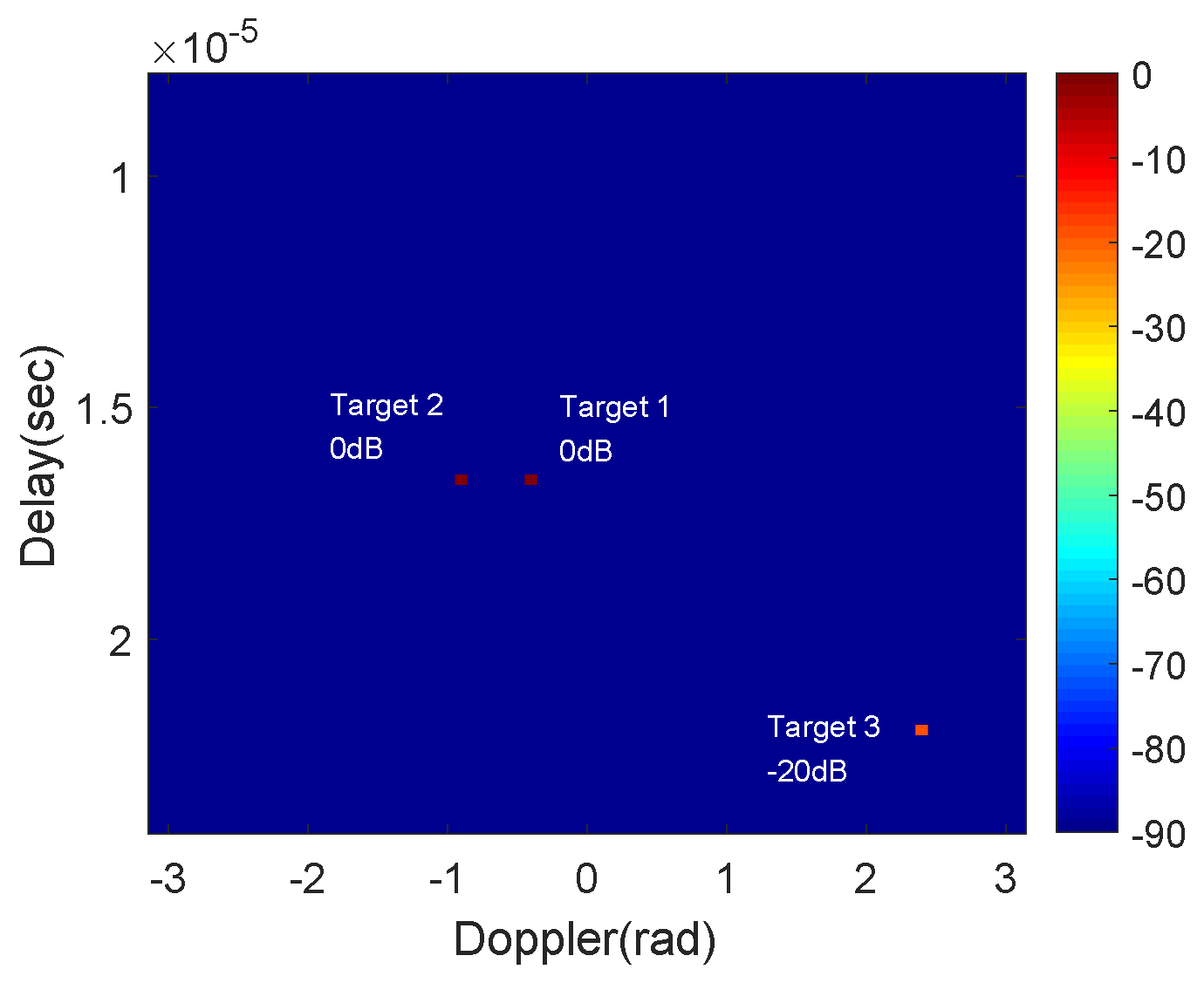

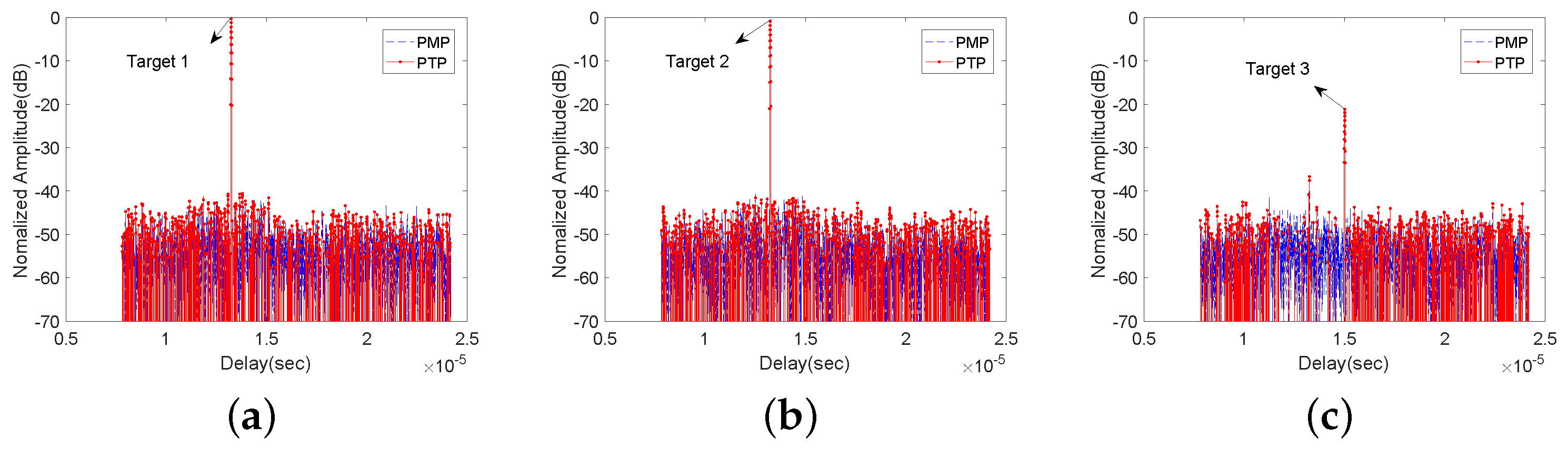

| Target | Delay | Doppler | Magnitude |
|---|---|---|---|
| Target No. 1 | 0 dB | ||
| Target No. 2 | 0 dB | ||
| Target No. 3 | −20 dB |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, J.; Song, Y.; Jiang, N.; Xie, Z.; Fan, C.; Huang, X. Enhanced Doppler Resolution and Sidelobe Suppression Performance for Golay Complementary Waveforms. Remote Sens. 2023, 15, 2452. https://doi.org/10.3390/rs15092452
Zhu J, Song Y, Jiang N, Xie Z, Fan C, Huang X. Enhanced Doppler Resolution and Sidelobe Suppression Performance for Golay Complementary Waveforms. Remote Sensing. 2023; 15(9):2452. https://doi.org/10.3390/rs15092452
Chicago/Turabian StyleZhu, Jiahua, Yongping Song, Nan Jiang, Zhuang Xie, Chongyi Fan, and Xiaotao Huang. 2023. "Enhanced Doppler Resolution and Sidelobe Suppression Performance for Golay Complementary Waveforms" Remote Sensing 15, no. 9: 2452. https://doi.org/10.3390/rs15092452
APA StyleZhu, J., Song, Y., Jiang, N., Xie, Z., Fan, C., & Huang, X. (2023). Enhanced Doppler Resolution and Sidelobe Suppression Performance for Golay Complementary Waveforms. Remote Sensing, 15(9), 2452. https://doi.org/10.3390/rs15092452







