A Study of Tropospheric and Ionospheric Propagation Conditions during Differential Interferometric SAR Measurements Applied on Zagreb 22 March 2020 Earthquake
Abstract
1. Introduction
2. Materials and Methods
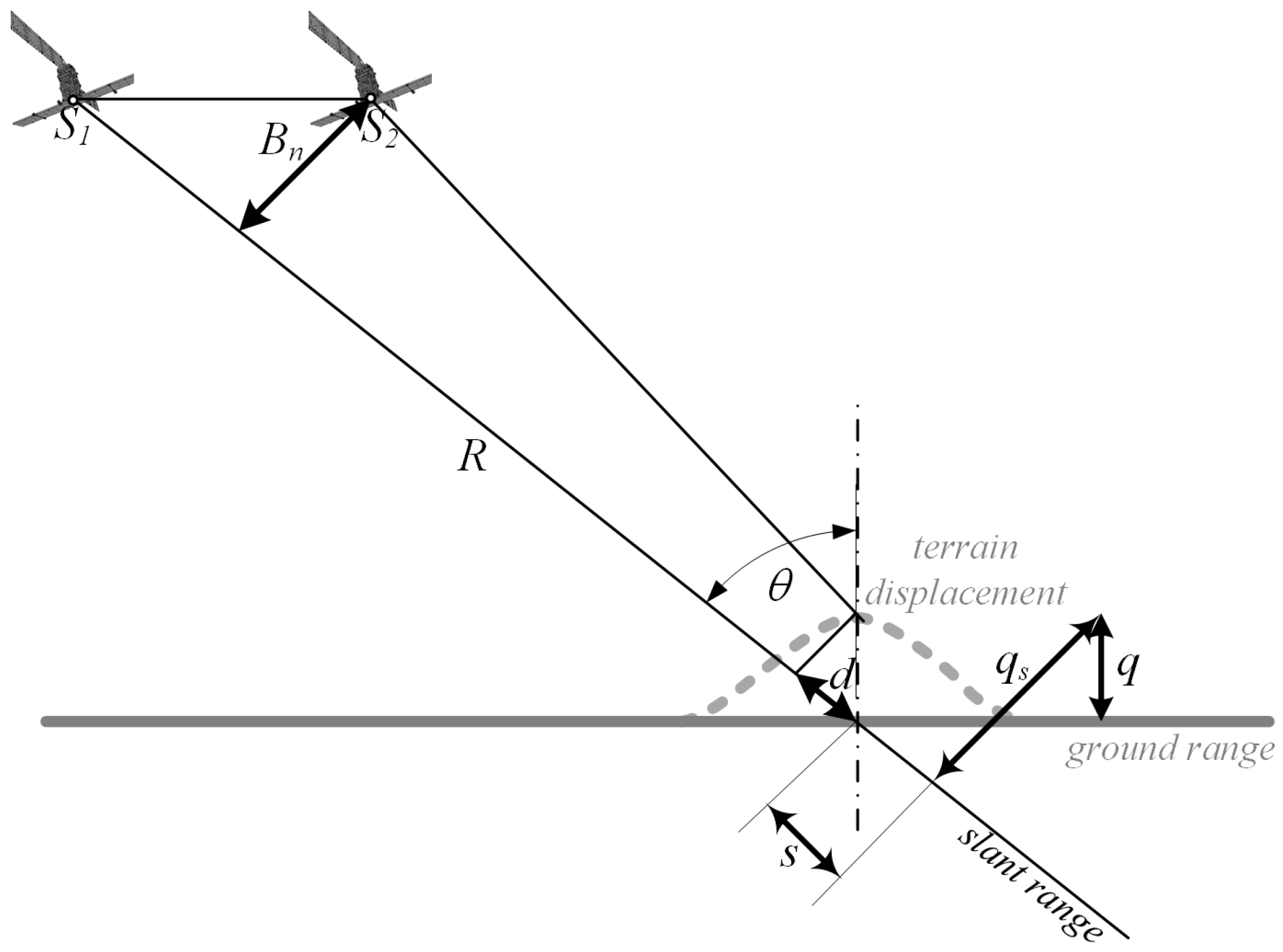
2.1. Error Sources in Differential Interferometry SAR
2.2. Atmospheric Refractive Conditions
2.2.1. Determination of the Tropospheric Refractive Conditions
2.2.2. Determination of Ionospheric Refractive Conditions
3. Results
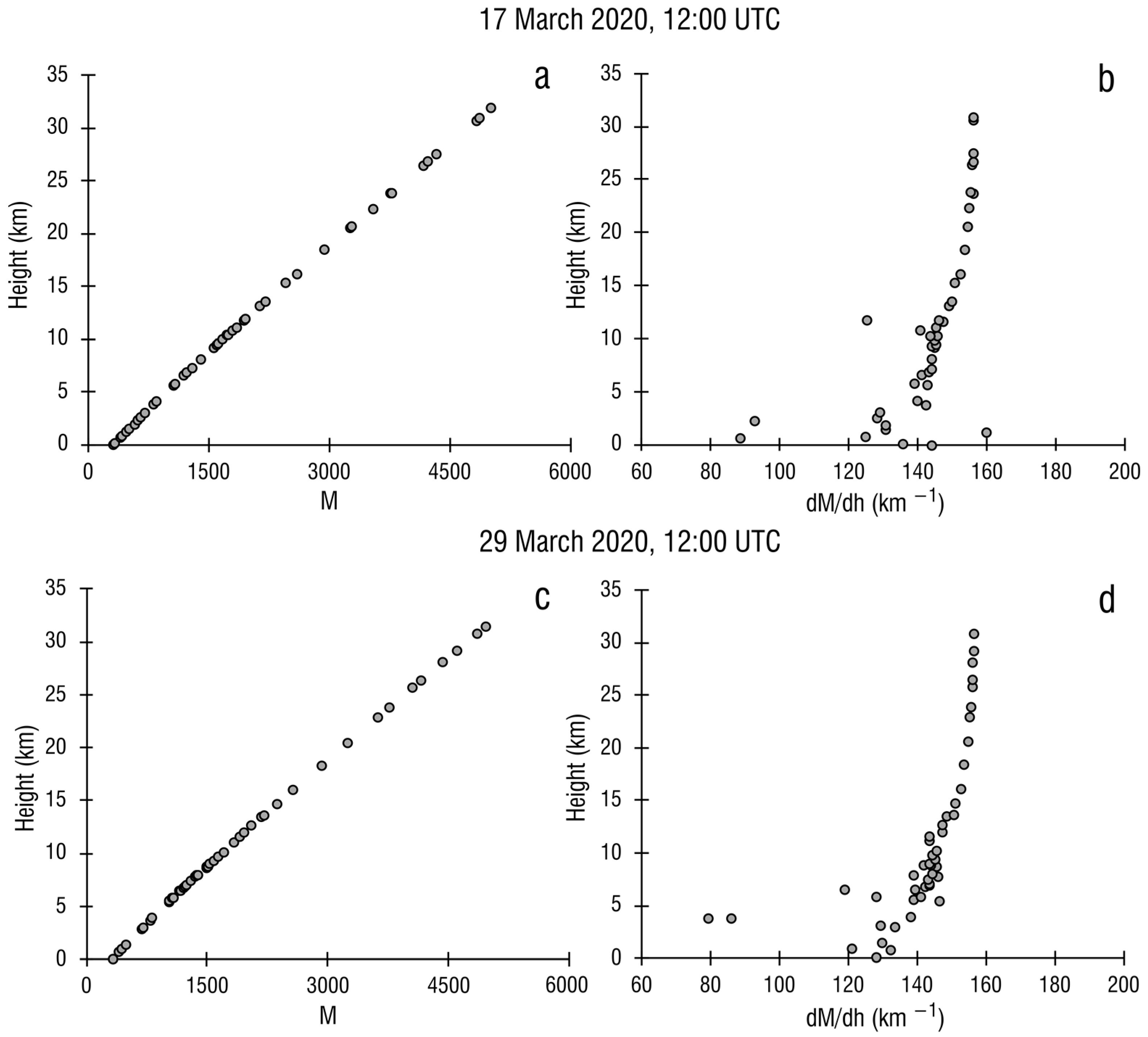
3.1. Tropospheric Refractive Conditions
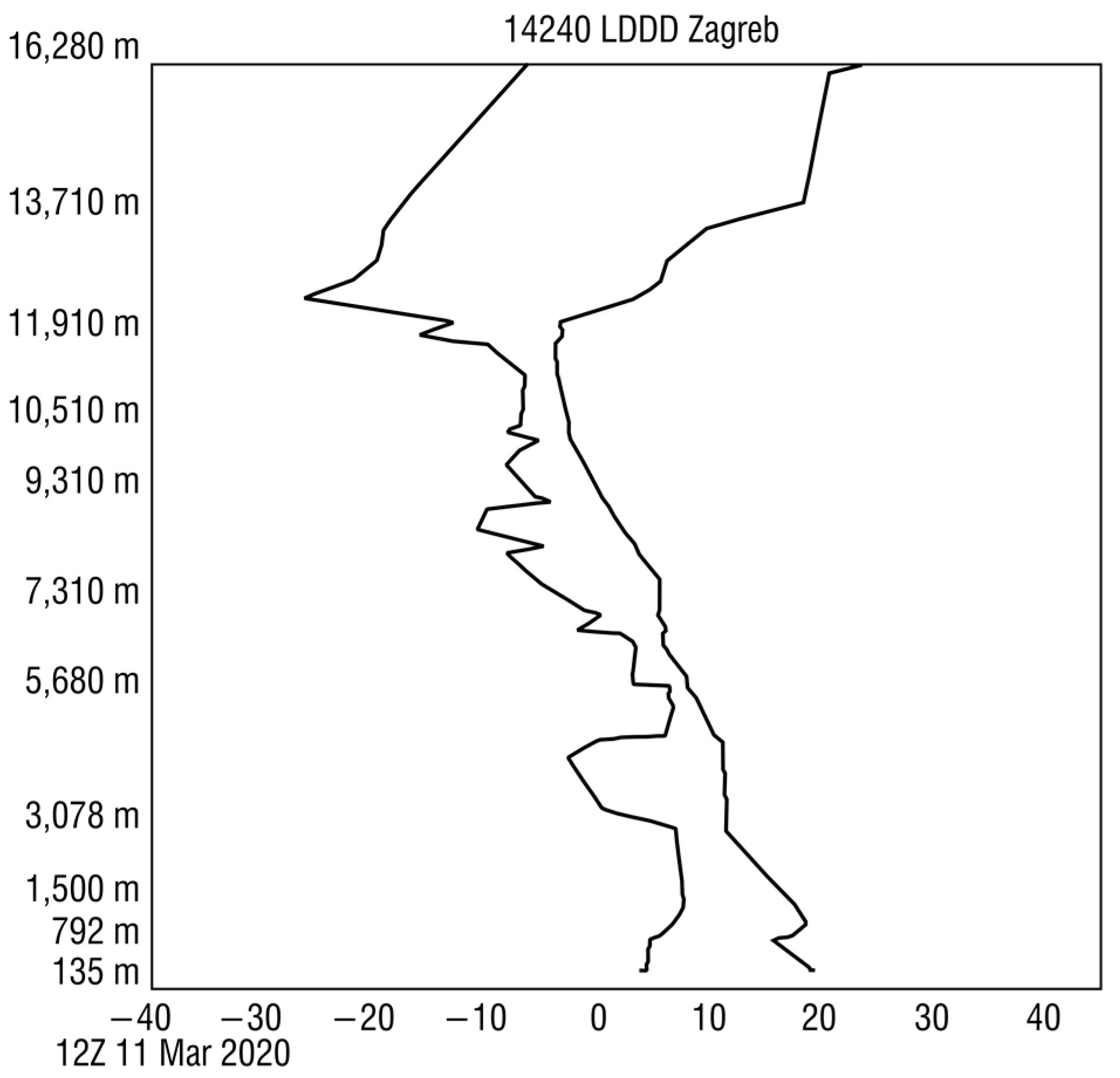
3.1.1. Tropospheric Refractive Conditions for the Sentinel-1A Interferometric Pair
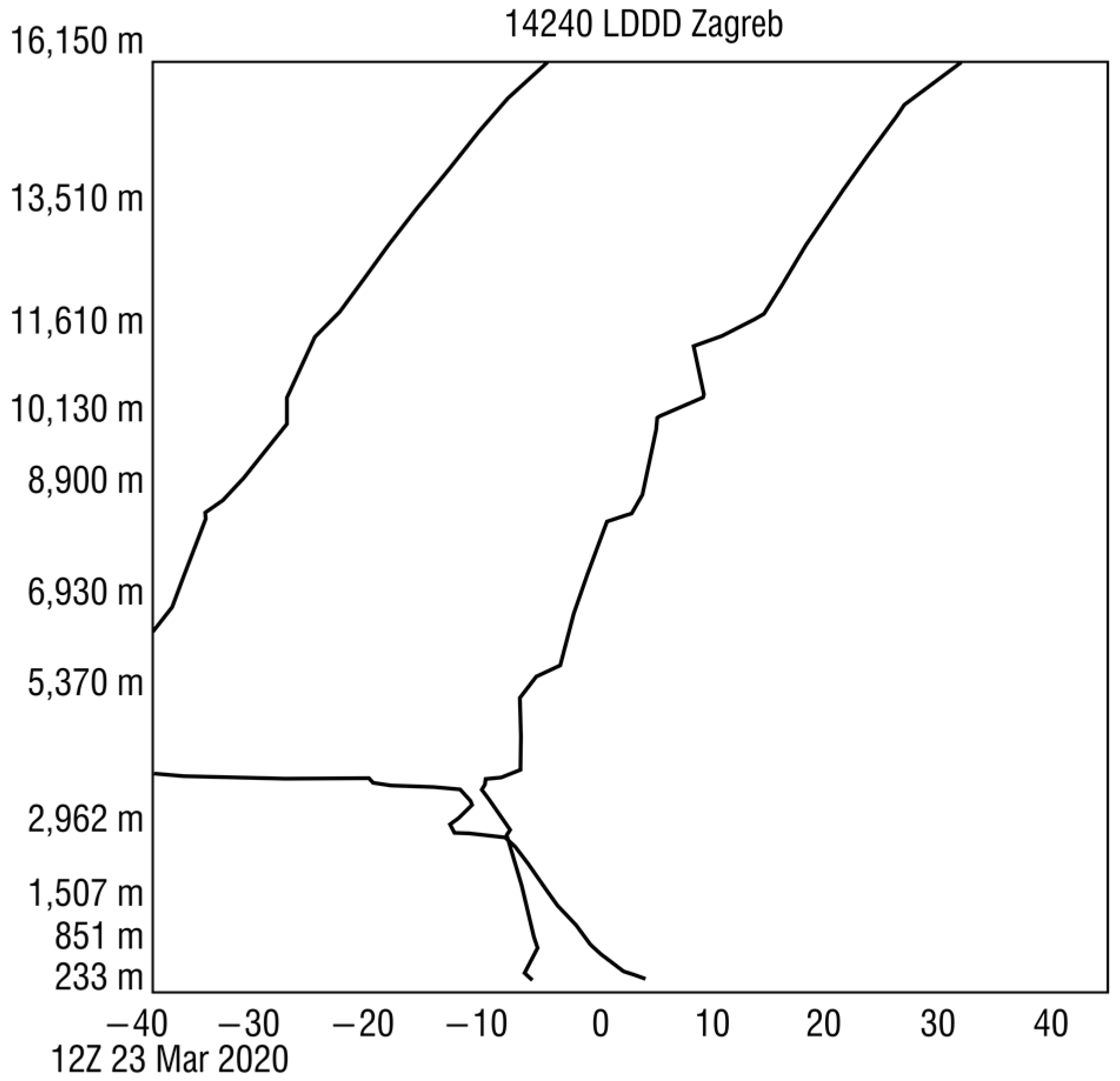
3.1.2. Tropospheric Refractive Conditions for the Sentinel-1B Interferometric Pair
3.2. Ionospheric Refractive Conditions
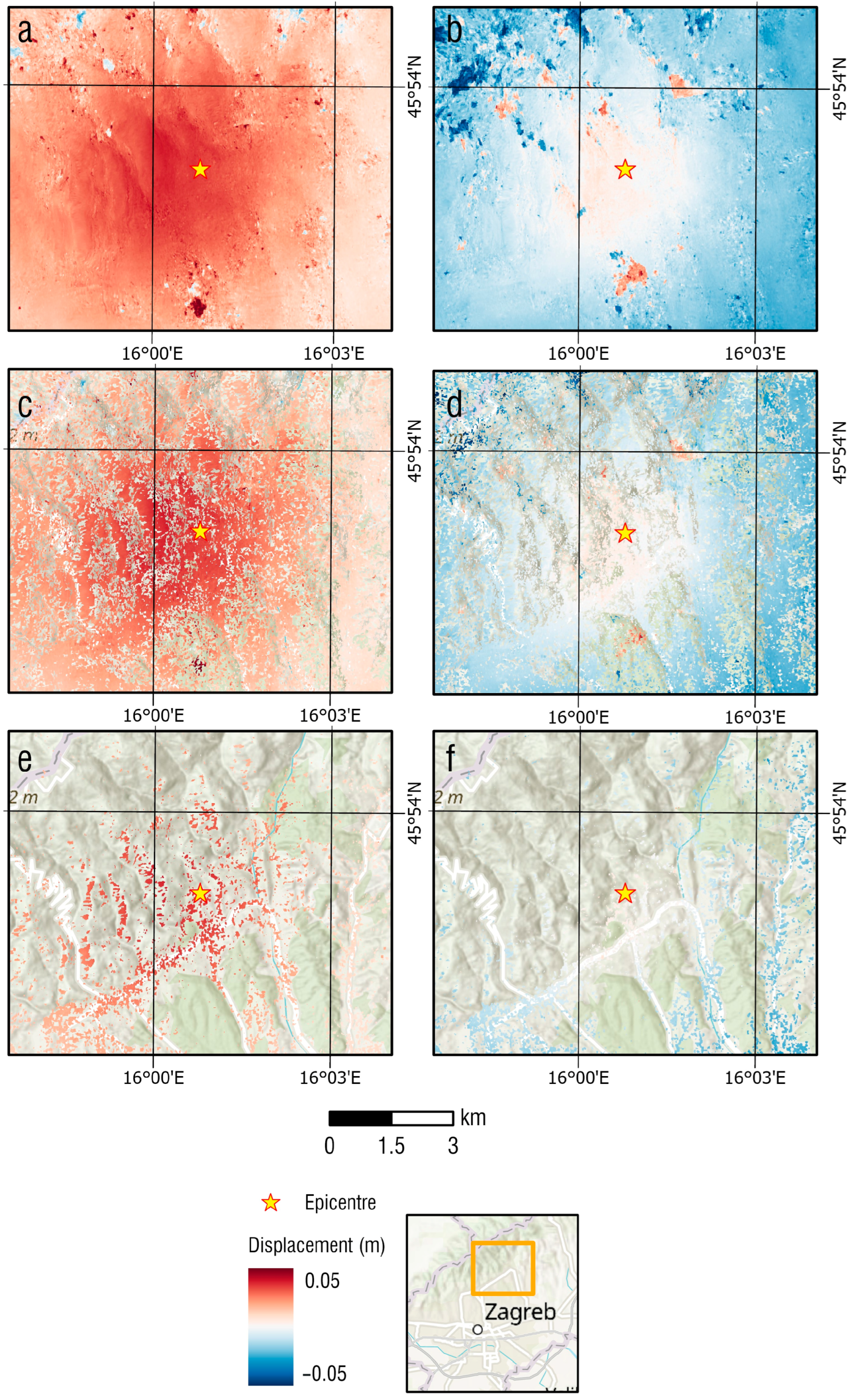
3.3. DInSAR Displacements during Observed Atmospheric Conditions
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
- Height over the ground;
- Modified refraction index M (dimensionless) computed by Equation (9);
- Refraction index n (dimensionless) computed by Equation (4);
- Water vapor pressure e;
- Total air pressure p;
- Air temperature T;
- Dew point Td;
- Wind speed Ws;
- Wind direction Wd (in geographic degrees);
- The vertical gradient of the modified refraction index dM/dh (dimensions km−1);
- The type of the refraction is determined in accordance with Table 1 (norm—normal refraction conditions, subrf—subrefractions and SUPER—for superrefractive conditions in the layer).
| Height [m] | M | n | e [hPa] | P [hPa] | t [°C] | td [°C] | Ws [ms−1] | Wd [°] | dM/dh [km−1] | Refraction |
|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 313.63 | 1.000313630 | 5.2 | 1014.0 | 16.8 | −2.2 | 1 | 100 | 143.97 | norm |
| 118 | 330.62 | 1.000312094 | 5.2 | 1000.0 | 14.8 | −2.2 | 2 | 210 | 135.87 | norm |
| 643 | 401.96 | 1.000301009 | 5.2 | 939.0 | 9.8 | −2.2 | 88.51 | norm | ||
| 770 | 413.20 | 1.000292310 | 4.2 | 925.0 | 9.8 | −5.2 | 4 | 20 | 124.83 | norm |
| 1171 | 463.25 | 1.000279403 | 3.5 | 881.0 | 7.6 | −7.4 | 160.08 | subrf | ||
| 1467 | 510.63 | 1.000280311 | 5.0 | 850.0 | 5.4 | −2.6 | 9 | 25 | 130.91 | norm |
| 1897 | 566.93 | 1.000269101 | 4.6 | 806.0 | 2.2 | −3.8 | 130.78 | norm | ||
| 2243 | 612.17 | 1.000260019 | 4.4 | 772.0 | 0.6 | −4.4 | 92.63 | norm | ||
| 2538 | 639.50 | 1.000241034 | 2.0 | 744.0 | −0.5 | −14.5 | 128.35 | norm | ||
| 3026 | 702.14 | 1.000227058 | 1.2 | 700.0 | −4.1 | −21.1 | 5 | 345 | 129.19 | norm |
| 3810 | 803.42 | 1.000205250 | 0.1 | 633.0 | −8.3 | −45.3 | 142.5 | norm | ||
| 4121 | 847.74 | 1.000200743 | 0.4 | 608.0 | −9.9 | −31.9 | 139.8 | norm | ||
| 5597 | 1054.08 | 1.000175351 | 0.3 | 500.0 | −21.7 | −34.7 | 10 | 310 | 143.03 | norm |
| 5745 | 1075.25 | 1.000173285 | 0.4 | 490.0 | −22.7 | −32.7 | 139.1 | norm | ||
| 6540 | 1185.83 | 1.000159050 | 0.1 | 439.0 | −28.3 | −43.3 | 141.21 | norm | ||
| 6804 | 1223.11 | 1.000154882 | 0.1 | 423.0 | −30.5 | −47.5 | 143.18 | norm | ||
| 7207 | 1280.82 | 1.000149321 | 0.1 | 400.0 | −33.9 | −47.9 | 12 | 305 | 143.95 | norm |
| 8066 | 1404.47 | 1.000138108 | 0.1 | 353.0 | −41.9 | −46.6 | 144 | norm | ||
| 9147 | 1560.13 | 1.000124051 | 0.0 | 300.0 | −51.3 | −59.3 | 11 | 325 | 145.12 | norm |
| 9366 | 1591.91 | 1.000121448 | 0.0 | 290.0 | −53.3 | −60.3 | 144.15 | norm | ||
| 9500 | 1611.23 | 1.000119730 | 0.0 | 284.0 | −54.3 | −64.3 | 145.23 | norm | ||
| 9915 | 1671.50 | 1.000114845 | 0.0 | 266.0 | −57.7 | −62.2 | 144.88 | norm | ||
| 10,297 | 1726.84 | 1.000110211 | 0.0 | 250.0 | −60.5 | −65.5 | 17 | 320 | 145.71 | norm |
| 10,322 | 1730.49 | 1.000109936 | 0.0 | 249.0 | −60.7 | −65.7 | 143.78 | norm | ||
| 10,786 | 1797.20 | 1.000103798 | 0.0 | 231.0 | −61.9 | −67.9 | 14 | 310 | 140.67 | norm |
| 11,092 | 1840.25 | 1.000098806 | 0.0 | 220.0 | −59.5 | −75.5 | 145.31 | norm | ||
| 11,687 | 1926.71 | 1.000091851 | 0.0 | 200.0 | −60.5 | −79.5 | 7 | 300 | 147.35 | norm |
| 11,749 | 1935.84 | 1.000091247 | 0.0 | 198.0 | −60.9 | −80.9 | 125.32 | norm | ||
| 11,812 | 1943.74 | 1.000089256 | 0.0 | 196.0 | −57.1 | −82.1 | 146.01 | norm | ||
| 13,107 | 2132.82 | 1.000075021 | 0.0 | 160.0 | −52.3 | −85.3 | 149.12 | norm | ||
| 13,537 | 2196.94 | 1.000071631 | 0.0 | 150.0 | −52.9 | −85.9 | 10 | 305 | 150.01 | norm |
| 15,235 | 2451.65 | 1.000059755 | 0.0 | 115.0 | −55.7 | −87.7 | 150.67 | norm | ||
| 16,127 | 2586.05 | 1.000054111 | 0.0 | 100.0 | −54.1 | −88.1 | 2 | 230 | 152.42 | norm |
| 18,397 | 2932.04 | 1.000043711 | 0.0 | 70.0 | −56.9 | −88.9 | 5 | 310 | 153.61 | norm |
| 20,527 | 3259.24 | 1.000036501 | 0.0 | 50.0 | −57.5 | −89.5 | 2 | 90 | 154.41 | norm |
| 20,641 | 3276.84 | 1.000036203 | 0.0 | 49.1 | −57.9 | −89.9 | 154.35 | norm | ||
| 22,303 | 3533.37 | 1.000031799 | 0.0 | 37.8 | −53.5 | −89.5 | 155.13 | norm | ||
| 23,759 | 3759.24 | 1.000029077 | 0.0 | 30.1 | −54.7 | −89.7 | 156.03 | norm | ||
| 23,797 | 3765.17 | 1.000029041 | 0.0 | 30.0 | −54.7 | −89.7 | 3 | 30 | 155.56 | norm |
| 26,407 | 4171.18 | 1.000025281 | 0.0 | 20.0 | −51.5 | −88.5 | 5 | 80 | 155.85 | norm |
| 26,739 | 4222.92 | 1.000024897 | 0.0 | 19.0 | −50.9 | −88.9 | 156.07 | norm | ||
| 27,457 | 4334.98 | 1.000024231 | 0.0 | 17.0 | −53.1 | −89.1 | 156.22 | norm | ||
| 30,618 | 4828.80 | 1.000021774 | 0.0 | 10.4 | −52.7 | −89.7 | 156.39 | norm | ||
| 30,897 | 4872.44 | 1.000021611 | 0.0 | 10.0 | −51.5 | −88.5 | 4 | 175 | 156.42 | norm |
| 31,803 | 5014.16 | 1.000021089 | 0.0 | 8.7 | −49.1 | −87.1 | ----- | ----- |
| Height [m] | M | n | e [hPa] | P [hPa] | t [°C] | td [°C] | Ws [ms−1] | Wd [°] | dM/dh [km−1] | Refraction |
|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 323.26 | 1.000323260 | 8.0 | 999.0 | 15.8 | 3.8 | 1 | 170 | 128.01 | norm |
| 642 | 405.44 | 1.000304646 | 6.7 | 925.0 | 9.2 | 1.2 | 3 | 235 | 132.35 | norm |
| 903 | 439.98 | 1.000298209 | 6.4 | 896.0 | 6.6 | 0.6 | 121.11 | norm | ||
| 1335 | 492.30 | 1.000282705 | 5.2 | 850.0 | 3.8 | −2.2 | 3 | 355 | 129.53 | norm |
| 2874 | 691.65 | 1.000240432 | 2.9 | 700.0 | −9.5 | −10.1 | 5 | 340 | 133.57 | norm |
| 3018 | 710.88 | 1.000237054 | 2.8 | 687.0 | −10.3 | −10.3 | 129.43 | norm | ||
| 3653 | 793.07 | 1.000219549 | 1.9 | 632.0 | −14.9 | −15.2 | 79.03 | norm | ||
| 3677 | 794.96 | 1.000217671 | 1.6 | 630.0 | −15.3 | −17.2 | 85.95 | norm | ||
| 3847 | 809.57 | 1.000205591 | 0.3 | 616.0 | −14.3 | −35.3 | 138.03 | norm | ||
| 5397 | 1023.51 | 1.000176181 | 0.2 | 500.0 | −24.1 | −39.1 | 8 | 350 | 146.29 | norm |
| 5455 | 1032.00 | 1.000175565 | 0.3 | 496.0 | −24.5 | −36.5 | 139.04 | norm | ||
| 5737 | 1071.21 | 1.000170501 | 0.2 | 477.0 | −26.9 | −40.9 | 127.94 | norm | ||
| 5798 | 1079.01 | 1.000168724 | 0.1 | 473.0 | −27.1 | −49.1 | 140.91 | norm | ||
| 6380 | 1161.02 | 1.000159360 | 0.2 | 436.0 | −30.1 | −41.1 | 118.82 | norm | ||
| 6413 | 1164.94 | 1.000158099 | 0.1 | 434.0 | −30.1 | −49.1 | 139.45 | norm | ||
| 6662 | 1199.66 | 1.000153726 | 0.0 | 419.0 | −31.3 | −55.3 | 142.19 | norm | ||
| 6815 | 1221.42 | 1.000151465 | 0.1 | 410.0 | −32.1 | −50.1 | 143.58 | norm | ||
| 6997 | 1247.55 | 1.000149021 | 0.1 | 400.0 | −33.5 | −49.5 | 11 | 310 | 143.47 | norm |
| 7372 | 1301.35 | 1.000143946 | 0.1 | 379.0 | −36.5 | −46.5 | 143.01 | norm | ||
| 7725 | 1351.83 | 1.000139005 | 0.1 | 360.0 | −39.7 | −52.7 | 145.87 | norm | ||
| 7878 | 1374.15 | 1.000137304 | 0.1 | 352.0 | −41.3 | −49.3 | 138.69 | norm | ||
| 7975 | 1387.60 | 1.000135525 | 0.0 | 347.0 | −41.9 | −63.9 | 144.19 | norm | ||
| 8642 | 1483.78 | 1.000126986 | 0.0 | 314.0 | −47.3 | −61.3 | 145.33 | norm | ||
| 8726 | 1495.99 | 1.000126008 | 0.0 | 310.0 | −47.9 | −55.9 | 141.75 | norm | ||
| 8790 | 1505.06 | 1.000125030 | 0.0 | 307.0 | −48.3 | −62.3 | 143.99 | norm | ||
| 8947 | 1527.67 | 1.000122991 | 0.0 | 300.0 | −48.9 | −56.9 | 14 | 330 | 143.35 | norm |
| 9327 | 1582.14 | 1.000117801 | 0.0 | 283.0 | −50.9 | −69.9 | 145.04 | norm | ||
| 9655 | 1629.71 | 1.000113875 | 0.0 | 269.0 | −53.1 | −65.1 | 144.25 | norm | ||
| 10,127 | 1697.80 | 1.000107861 | 0.0 | 250.0 | −54.9 | −67.9 | 15 | 320 | 145.43 | norm |
| 11,079 | 1836.25 | 1.000096847 | 0.0 | 215.0 | −59.1 | −74.1 | 14 | 315 | 143.58 | norm |
| 11,537 | 1902.01 | 1.000090701 | 0.0 | 200.0 | −57.1 | −82.1 | 7 | 295 | 143.47 | norm |
| 11,965 | 1963.42 | 1.000084915 | 0.0 | 187.0 | −53.5 | −85.5 | 147.31 | norm | ||
| 12,576 | 2053.42 | 1.000078988 | 0.0 | 170.0 | −53.9 | −85.9 | 147.17 | norm | ||
| 13,387 | 2172.78 | 1.000071021 | 0.0 | 150.0 | −50.3 | −86.3 | 8 | 300 | 148.27 | norm |
| 13,563 | 2198.88 | 1.000069489 | 0.0 | 146.0 | −49.7 | −86.7 | 150.33 | norm | ||
| 14,674 | 2365.89 | 1.000062072 | 0.0 | 123.0 | −52.9 | −87.9 | 150.83 | norm | ||
| 16,017 | 2568.46 | 1.000053791 | 0.0 | 100.0 | −52.1 | −88.1 | 4 | 335 | 152.48 | norm |
| 18,317 | 2919.16 | 1.000043391 | 0.0 | 70.0 | −54.1 | −89.1 | 7 | 300 | 153.64 | norm |
| 20,467 | 3249.49 | 1.000036171 | 0.0 | 50.0 | −53.5 | −90.5 | 7 | 240 | 154.70 | norm |
| 22,798 | 3610.09 | 1.000030804 | 0.0 | 34.7 | −55.7 | −91.7 | 155.13 | norm | ||
| 23,737 | 3755.76 | 1.000029051 | 0.0 | 30.0 | −54.9 | −90.9 | 5 | 290 | 155.48 | norm |
| 25,667 | 4055.84 | 1.000026121 | 0.0 | 22.2 | −52.5 | −89.5 | 155.85 | norm | ||
| 26,347 | 4161.81 | 1.000025331 | 0.0 | 20.0 | −53.1 | −90.1 | 3 | 265 | 156.04 | norm |
| 28,013 | 4421.77 | 1.000023729 | 0.0 | 15.4 | −56.5 | −92.5 | 156.10 | norm | ||
| 29,144 | 4598.32 | 1.000022712 | 0.0 | 12.9 | −52.7 | −89.7 | 156.35 | norm | ||
| 30,777 | 4853.63 | 1.000021641 | 0.0 | 10.0 | −53.7 | −90.7 | 11 | 230 | 156.45 | norm |
| 31,451 | 4959.08 | 1.000021273 | 0.0 | 9.0 | −54.3 | −90.3 | ----- | ----- |
| Height [m] | M | n | e [hPa] | P [hPa] | t [°C] | td [°C] | Ws [ms−1] | Wd [°] | dM/dh [km−1] | Refraction |
|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 318.33 | 1.000318330 | 7.2 | 1001.0 | 17.2 | 2.2 | 3 | 210 | 171.68 | subrf |
| 12 | 320.39 | 1.000318506 | 7.4 | 1000.0 | 17.6 | 2.6 | 2 | 225 | 129.18 | norm |
| 658 | 403.84 | 1.000300534 | 6.2 | 926.0 | 11.2 | 0.2 | 132.15 | norm | ||
| 669 | 405.29 | 1.000300257 | 6.2 | 925.0 | 11.2 | 0.2 | 5 | 300 | 115.57 | norm |
| 732 | 412.57 | 1.000297646 | 6.4 | 918.0 | 12.6 | 0.6 | 129.30 | norm | ||
| 972 | 443.60 | 1.000290996 | 6.6 | 892.0 | 13.0 | 1.0 | 131.80 | norm | ||
| 1377 | 496.98 | 1.000280791 | 6.2 | 850.0 | 10.2 | 0.2 | 11 | 330 | 129.95 | norm |
| 2955 | 702.05 | 1.000238115 | 3.5 | 700.0 | −2.9 | −7.4 | 15 | 310 | 143.40 | norm |
| 2966 | 703.63 | 1.000237968 | 3.5 | 699.0 | −2.9 | −7.3 | 112.07 | norm | ||
| 3335 | 744.99 | 1.000221395 | 1.9 | 667.0 | −4.5 | −15.5 | 132.50 | norm | ||
| 4381 | 883.58 | 1.000195763 | 0.9 | 583.0 | −9.5 | −23.5 | 139.81 | norm | ||
| 4622 | 917.27 | 1.000191616 | 1.0 | 565.0 | −10.7 | −22.7 | 140.42 | norm | ||
| 4745 | 934.55 | 1.000189585 | 1.0 | 556.0 | −11.5 | −22.5 | 193.13 | subrf | ||
| 4814 | 947.87 | 1.000192072 | 1.7 | 551.0 | −12.3 | −16.8 | 138.35 | norm | ||
| 5354 | 1022.58 | 1.000182002 | 1.4 | 513.0 | −16.1 | −18.5 | 137.61 | norm | ||
| 5557 | 1050.52 | 1.000178071 | 1.3 | 500.0 | −17.5 | −19.9 | 25 | 340 | 139.80 | norm |
| 5753 | 1077.92 | 1.000174699 | 1.2 | 487.0 | −19.1 | −20.6 | 98.99 | norm | ||
| 5799 | 1082.47 | 1.000172027 | 0.9 | 484.0 | −19.3 | −24.3 | 137.94 | norm | ||
| 5922 | 1099.44 | 1.000169686 | 0.8 | 476.0 | −19.9 | −24.9 | 141.00 | norm | ||
| 6515 | 1183.05 | 1.000160195 | 0.7 | 439.0 | −24.9 | −27.4 | 137.71 | norm | ||
| 6749 | 1215.28 | 1.000155687 | 0.5 | 425.0 | −26.1 | −29.9 | 118.52 | norm | ||
| 6800 | 1221.32 | 1.000153720 | 0.4 | 422.0 | −26.1 | −34.1 | 143.48 | norm | ||
| 7078 | 1261.21 | 1.000149964 | 0.4 | 406.0 | −28.1 | −33.1 | 137.05 | norm | ||
| 7187 | 1276.15 | 1.000147791 | 0.3 | 400.0 | −28.5 | −35.5 | 29 | 330 | 140.48 | norm |
| 7703 | 1348.63 | 1.000139259 | 0.2 | 372.0 | −31.1 | −42.1 | 143.75 | norm | ||
| 8210 | 1421.52 | 1.000132550 | 0.1 | 346.0 | −35.5 | −47.5 | 146.84 | norm | ||
| 8311 | 1436.35 | 1.000131523 | 0.1 | 341.0 | −36.3 | −44.3 | 143.71 | norm | ||
| 8620 | 1480.76 | 1.000127420 | 0.1 | 326.0 | −39.1 | −52.1 | 145.04 | norm | ||
| 8984 | 1533.55 | 1.000123062 | 0.1 | 309.0 | −42.1 | −53.1 | 148.01 | norm | ||
| 9094 | 1549.83 | 1.000122072 | 0.1 | 304.0 | −43.1 | −47.9 | 145.07 | norm | ||
| 9187 | 1563.32 | 1.000120961 | 0.1 | 300.0 | −43.9 | −49.9 | 34 | 325 | 145.32 | norm |
| 9716 | 1640.20 | 1.000114788 | 0.0 | 277.0 | −48.3 | −55.3 | 146.04 | norm | ||
| 9981 | 1678.90 | 1.000111883 | 0.0 | 266.0 | −50.5 | −55.5 | 146.41 | norm | ||
| 10,129 | 1700.57 | 1.000110317 | 0.0 | 260.0 | −51.7 | −54.3 | 144.64 | norm | ||
| 10,254 | 1718.65 | 1.000108772 | 0.0 | 255.0 | −52.5 | −58.5 | 146.30 | norm | ||
| 10,387 | 1738.10 | 1.000107341 | 0.0 | 250.0 | −53.3 | −57.6 | 33 | 330 | 146.29 | norm |
| 11,169 | 1852.50 | 1.000098967 | 0.0 | 221.0 | −58.7 | −61.6 | 146.58 | norm | ||
| 11,667 | 1925.50 | 1.000093781 | 0.0 | 204.0 | −61.7 | −67.7 | 145.03 | norm | ||
| 11,787 | 1942.90 | 1.000092341 | 0.0 | 200.0 | −61.9 | −73.9 | 34 | 330 | 145.00 | norm |
| 11,818 | 1947.40 | 1.000091974 | 0.0 | 199.0 | −61.9 | −74.9 | 147.45 | norm | ||
| 12,006 | 1975.12 | 1.000090178 | 0.0 | 193.0 | −63.1 | −72.1 | 32 | 330 | 142.41 | norm |
| 12,407 | 2032.22 | 1.000084321 | 0.0 | 181.0 | −58.7 | −88.7 | 145.58 | norm | ||
| 12,655 | 2068.32 | 1.000081485 | 0.0 | 174.0 | −57.7 | −85.7 | 148.40 | norm | ||
| 12,988 | 2117.74 | 1.000078624 | 0.0 | 165.0 | −59.1 | −85.1 | 147.41 | norm | ||
| 13,503 | 2193.65 | 1.000073679 | 0.0 | 152.0 | −58.3 | −87.3 | 145.97 | norm | ||
| 13,587 | 2205.92 | 1.000072761 | 0.0 | 150.0 | −57.5 | −87.5 | 26 | 350 | 145.13 | norm |
| 13,936 | 2256.57 | 1.000068618 | 0.0 | 142.0 | −52.1 | −88.1 | 151.00 | norm | ||
| 16,026 | 2572.16 | 1.000056078 | 0.0 | 102.0 | −61.5 | −89.5 | 148.48 | norm | ||
| 16,157 | 2591.62 | 1.000054971 | 0.0 | 100.0 | −59.3 | −89.3 | 17 | 340 | 152.01 | norm |
| 18,407 | 2933.63 | 1.000043731 | 0.0 | 70.0 | −57.1 | −89.1 | 3 | 330 | 153.07 | norm |
| 18,846 | 3000.83 | 1.000042008 | 0.0 | 65.3 | −56.9 | −89.9 | 153.60 | norm | ||
| 19,538 | 3107.12 | 1.000039654 | 0.0 | 58.5 | −58.1 | −90.1 | 153.04 | norm | ||
| 19,916 | 3164.97 | 1.000038158 | 0.0 | 55.1 | −55.3 | −89.3 | 154.17 | norm | ||
| 20,537 | 3260.71 | 1.000036401 | 0.0 | 50.0 | −56.3 | −90.3 | 2 | 200 | 154.43 | norm |
| 21,563 | 3419.15 | 1.000033759 | 0.0 | 42.5 | −57.5 | −90.5 | 154.91 | norm | ||
| 23,777 | 3762.12 | 1.000029131 | 0.0 | 30.0 | −56.5 | −90.5 | 4 | 125 | 155.43 | norm |
| 24,057 | 3805.64 | 1.000028691 | 0.0 | 28.7 | −57.3 | −90.3 | 155.39 | norm | ||
| 25,467 | 4024.73 | 1.000026411 | 0.0 | 23.0 | −52.7 | −89.7 | 155.82 | norm | ||
| 26,367 | 4164.97 | 1.000025351 | 0.0 | 20.0 | −53.5 | −89.5 | 4 | 20 | 156.05 | norm |
| 28,738 | 4534.97 | 1.000023104 | 0.0 | 13.8 | −55.1 | −90.1 | 156.16 | norm | ||
| 29,803 | 4701.28 | 1.000022209 | 0.0 | 11.7 | −49.5 | −87.5 | 156.38 | norm | ||
| 30,837 | 4862.98 | 1.000021571 | 0.0 | 10.0 | −49.1 | −87.1 | 6 | 80 | 156.48 | norm |
| 31,381 | 4948.11 | 1.000021293 | 0.0 | 9.2 | −50.3 | −88.3 | ----- | ----- |
| Height [m] | M | n | e [hPa] | P [hPa] | t [°C] | td [°C] | Ws [ms−1] | Wd [°] | dM/dh [km−1] | Refraction |
|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 321.85 | 1.000321850 | 3.4 | 1014.0 | 2.2 | −7.8 | 6 | 10 | 130.66 | norm |
| 110 | 336.22 | 1.000318950 | 3.2 | 1000.0 | 0.4 | −8.6 | 8 | 55 | 130.70 | norm |
| 617 | 402.49 | 1.000305621 | 2.9 | 938.0 | −4.7 | −9.7 | 122.70 | norm | ||
| 728 | 416.11 | 1.000301814 | 2.8 | 925.0 | −5.5 | −10.5 | 10 | 15 | 127.33 | norm |
| 1384 | 499.63 | 1.000282342 | 2.1 | 850.0 | −11.3 | −14.1 | 13 | 25 | 129.07 | norm |
| 2649 | 662.91 | 1.000247017 | 1.1 | 718.0 | −21.7 | −21.9 | 123.46 | norm | ||
| 2773 | 678.22 | 1.000242859 | 0.9 | 706.0 | −22.5 | −24.3 | 109.79 | norm | ||
| 2839 | 685.47 | 1.000239747 | 0.7 | 700.0 | −22.5 | −27.5 | 20 | 45 | 131.61 | norm |
| 2944 | 699.28 | 1.000237072 | 0.6 | 690.0 | −23.3 | −28.3 | 135.47 | norm | ||
| 3321 | 750.36 | 1.000228963 | 0.6 | 655.0 | −26.5 | −28.1 | 132.38 | norm | ||
| 3600 | 787.29 | 1.000222090 | 0.5 | 630.0 | −28.7 | −30.8 | 117.87 | norm | ||
| 3714 | 800.73 | 1.000217632 | 0.2 | 620.0 | −29.1 | −39.1 | 132.25 | norm | ||
| 3806 | 812.90 | 1.000215358 | 0.2 | 612.0 | −29.5 | −39.5 | 63.18 | SUPER | ||
| 3841 | 815.11 | 1.000212073 | 0.0 | 609.0 | −27.9 | −55.9 | 122.09 | norm | ||
| 3948 | 828.17 | 1.000208334 | 0.0 | 600.0 | −26.9 | −61.9 | 135.88 | norm | ||
| 5200 | 998.29 | 1.000181890 | 0.0 | 503.0 | −33.3 | −68.3 | 139.16 | norm | ||
| 5247 | 1004.83 | 1.000181051 | 0.0 | 500.0 | −33.5 | −68.5 | 17 | 15 | 135.11 | norm |
| 5606 | 1053.34 | 1.000173198 | 0.0 | 475.0 | −33.9 | −69.9 | 132.72 | norm | ||
| 5846 | 1085.19 | 1.000167368 | 0.0 | 459.0 | −32.9 | −71.9 | 139.17 | norm | ||
| 6807 | 1218.93 | 1.000150231 | 0.0 | 400.0 | −36.5 | −72.5 | 8 | 360 | 141.24 | norm |
| 8354 | 1437.44 | 1.000125862 | 0.0 | 319.0 | −41.5 | −77.5 | 136.49 | norm | ||
| 8483 | 1455.04 | 1.000123209 | 0.0 | 313.0 | −40.1 | −78.1 | 143.12 | norm | ||
| 8777 | 1497.12 | 1.000119131 | 0.0 | 300.0 | −40.7 | −77.7 | 13 | 350 | 144.88 | norm |
| 10,007 | 1675.32 | 1.000104221 | 0.0 | 250.0 | −45.7 | −78.7 | 13 | 340 | 146.27 | norm |
| 10,114 | 1690.97 | 1.000103072 | 0.0 | 246.0 | −46.3 | −79.3 | 142.51 | norm | ||
| 10,475 | 1742.42 | 1.000097845 | 0.0 | 233.0 | −44.1 | −81.1 | 147.73 | norm | ||
| 11,321 | 1867.40 | 1.000090003 | 0.0 | 205.0 | −49.5 | −83.5 | 10 | 290 | 143.91 | norm |
| 11,487 | 1891.29 | 1.000087831 | 0.0 | 200.0 | −48.1 | −84.1 | 13 | 305 | 145.25 | norm |
| 11,860 | 1945.47 | 1.000083450 | 0.0 | 189.0 | −46.1 | −84.1 | 148.68 | norm | ||
| 13,387 | 2172.50 | 1.000070741 | 0.0 | 150.0 | −49.1 | −86.1 | 9 | 305 | 150.31 | norm |
| 15,286 | 2457.93 | 1.000058028 | 0.0 | 112.0 | −52.1 | −88.1 | 151.02 | norm | ||
| 16,027 | 2569.84 | 1.000053601 | 0.0 | 100.0 | −50.9 | −87.9 | 6 | 285 | 152.51 | norm |
| 18,337 | 2922.14 | 1.000043231 | 0.0 | 70.0 | −52.7 | −89.7 | 4 | 280 | 153.73 | norm |
| 20,497 | 3254.20 | 1.000036171 | 0.0 | 50.0 | −53.5 | −90.5 | 3 | 350 | 154.52 | norm |
| 21,581 | 3421.70 | 1.000033483 | 0.0 | 42.2 | −55.1 | −90.1 | 154.93 | norm | ||
| 23,777 | 3761.93 | 1.000028941 | 0.0 | 30.0 | −52.7 | −89.7 | 7 | 55 | 155.62 | norm |
| 26,397 | 4169.64 | 1.000025311 | 0.0 | 20.0 | −52.5 | −89.5 | 7 | 175 | 155.80 | norm |
| 26,594 | 4200.34 | 1.000025082 | 0.0 | 19.4 | −51.9 | −88.9 | 156.02 | norm | ||
| 27,521 | 4344.96 | 1.000024163 | 0.0 | 16.8 | −53.3 | −90.3 | 155.95 | norm | ||
| 28,084 | 4432.76 | 1.000023572 | 0.0 | 15.4 | −50.1 | −88.1 | 156.28 | norm | ||
| 30,844 | 4864.10 | 1.000021592 | 0.0 | 10.1 | −48.1 | −87.1 | 156.54 | norm | ||
| 30,917 | 4875.53 | 1.000021561 | 0.0 | 10.0 | −48.3 | −86.3 | 16 | 125 | 156.49 | norm |
| 32,549 | 5130.92 | 1.000020727 | 0.0 | 7.8 | −48.1 | −87.1 | ----- | ----- |
References
- Chen, K.-S. Principles of Synthetic Aperture Radar Imaging: A System Simulation Approach; CRC Press: Boca Raton, FL, USA, 2016; Volume 2. [Google Scholar]
- Curlander, J.C.; McDonough, R.N. Synthetic Aperture Radar: Systems and Signal Processing; Wiley: New York, NY, USA, 1991. [Google Scholar]
- Ferretti, A.; Monti-Guarnieri, A.; Prati, C.; Rocca, F.; Massonnet, D. Insar Principles: Guidelines for SAR Interferometry Processing and Interpretation; ESA Publications TM-19: Frascati, Italy, 2007. [Google Scholar]
- Hanssen, R.F. Radar Interferometry: Data Interpretation and Error Analysis; Springer: Dordrecht, The Netherlands, 2001. [Google Scholar] [CrossRef]
- Massonnet, D.; Feigl, K.L. Radar interferometry and its application to changes in the Earth’s surface. Rev. Geophys. 1998, 36, 441–500. [Google Scholar] [CrossRef]
- Zebker, H.A.; Rosen, P.A.; Hensley, S. Atmospheric effects in interferometric synthetic aperture radar surface deformation and topographic maps. J. Geophys. Res. Solid Earth 1997, 102, 7547–7563. [Google Scholar] [CrossRef]
- Goldstein, R. Atmospheric limitations to repeat-track radar interferometry. Geophys. Res. Lett. 1995, 22, 2517–2520. [Google Scholar] [CrossRef]
- American Institute of Aeronautics and Astronautics. Guide to Reference and Standard Atmosphere Models (G-003B-2004); American Institute of Aeronautics and Astronautics: Reston, Virginia, USA, 2004. [Google Scholar] [CrossRef]
- National Oceanic and Atmospheric Administration. National Aeronautic and Space Administration; United States Air Force. U.S. Standard Atmosphere; NOAA: Washington, DC, USA, 1976; p. 227. [Google Scholar]
- Viher, M.; Prtenjak, M.T. A multi-year study of radio-wave refractivity profiles above the Adriatic Sea up to an altitude of 40 km. Meteorol. Z. 2012, 21, 365–370. [Google Scholar] [CrossRef] [PubMed]
- Zolesi, B.; Cander, L.R. Ionospheric Prediction and Forecasting; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar] [CrossRef]
- International Telecommunication Union. Recommendation ITU-R P.453-14 (08/19) The radio refractive index: Its formula and refractivity data, P Series, Radiowave Propagation; International Telecommunication Union: Geneva, Switzerland, 2019. [Google Scholar]
- Villarini, G.; Krajewski, W.F. Review of the Different Sources of Uncertainty in Single Polarization Radar-Based Estimates of Rainfall. Surv. Geophys. 2010, 31, 107–129. [Google Scholar] [CrossRef]
- Darvishi, M.; Cuozzo, G.; Bruzzone, L.; Nilfouroushan, F. Performance Evaluation of Phase and Weather-Based Models in Atmospheric Correction with Sentinel-1Data: Corvara Landslide in the Alps. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2020, 13, 1332–1346. [Google Scholar] [CrossRef]
- Gomba, G.; Cong, X.Y.; Eineder, M. Correction of Ionospheric and Tropospheric Path Delay For L-Band Interferograms. In Proceedings of the IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Milan, Italy, 26–31 July 2015; pp. 310–313. [Google Scholar]
- Hu, Z.B.; Mallorqui, J.J. An Accurate Method to Correct Atmospheric Phase Delay for InSAR with the ERA5 Global Atmospheric Model. Remote Sens. 2019, 11, 1969. [Google Scholar] [CrossRef]
- Mathew, J.; Majumdar, R.; Kumar, K.V. Estimating the Atmospheric Phase Delay for Quantifying Co-Seismic Deformation Using Repeat Pass Differential Sar Interferometry: Observations From 20th April 2013 Lushan (China) Earthquake. In Proceedings of the ISPRS Technical Commission VIII Symposium, Hyderabad, India, 9–12 December 2014; pp. 57–64. [Google Scholar]
- Milczarek, W.; Kopec, A.; Glabicki, D. Estimation of Tropospheric and Ionospheric Delay in DInSAR Calculations: Case Study of Areas Showing (Natural and Induced) Seismic Activity. Remote Sens. 2019, 11, 621. [Google Scholar] [CrossRef]
- Qian, F.M.; Chen, G.; Lu, J.; Chen, X.M.; Lou, L.S.; Jiang, T.; Liu, W.; Wang, S. Correcting method of slant-range error for the TH-2 satellites. Remote Sens. Lett. 2021, 12, 194–201. [Google Scholar] [CrossRef]
- Zhu, M.; Zeng, Q.M.; Jiao, J. Quantitative assessment to the impact of InSAR ionospheric and tropospheric corrections on source parameter modelling: Application to the 4th nuclear test, North Korea. Geophys. J. Int. 2021, 224, 86–99. [Google Scholar] [CrossRef]
- Liu, Q.; Zeng, Q.; Zhang, Z. Evaluation of InSAR Tropospheric Correction by Using Efficient WRF Simulation with ERA5 for Initialization. Remote Sens. 2023, 15, 273. [Google Scholar] [CrossRef]
- Murray, K.D.; Bekaert, D.P.S.; Lohman, R.B. Tropospheric corrections for InSAR: Statistical assessments and applications to the Central United States and Mexico. Remote Sens. Environ. 2019, 232, 111326. [Google Scholar] [CrossRef]
- Bekaert, D.P.S.; Hooper, A.; Wright, T.J. A spatially variable power law tropospheric correction technique for InSAR data. J. Geophys. Res. Solid Earth 2015, 120, 1345–1356. [Google Scholar] [CrossRef]
- Doin, M.P.; Lasserre, C.; Peltzer, G.; Cavalié, O.; Doubre, C. Corrections of stratified tropospheric delays in SAR interferometry: Validation with global atmospheric models. J. Appl. Geophys. 2009, 69, 35–50. [Google Scholar] [CrossRef]
- Fattahi, H.; Amelung, F. InSAR bias and uncertainty due to the systematic and stochastic tropospheric delay. J. Geophys. Res. Solid Earth 2015, 120, 8758–8773. [Google Scholar] [CrossRef]
- Parker, A.L.; Biggs, J.; Walters, R.J.; Ebmeier, S.K.; Wright, T.J.; Teanby, N.A.; Lu, Z. Systematic assessment of atmospheric uncertainties for InSAR data at volcanic arcs using large-scale atmospheric models: Application to the Cascade volcanoes, United States. Remote Sens. Environ. 2015, 170, 102–114. [Google Scholar] [CrossRef]
- Kim, J.-R.; Lin, S.-Y.; Yun, H.-W.; Tsai, Y.-L.; Seo, H.-J.; Hong, S.; Choi, Y. Investigation of Potential Volcanic Risk from Mt. Baekdu by DInSAR Time Series Analysis and Atmospheric Correction. Remote Sens. 2017, 9, 138. [Google Scholar]
- Brcic, R.; Parizzi, A.; Eineder, M.; Bamler, R.; Meyer, F. Estimation and compensation of ionospheric delay for SAR interferometry. In Proceedings of the International Geoscience and Remote Sensing Symposium (IGARSS), Honolulu, HI, USA, 25–30 July 2010; pp. 2908–2911. [Google Scholar]
- Gomba, G.; Parizzi, A.; De Zan, F.; Eineder, M.; Bamler, R. Toward Operational Compensation of Ionospheric Effects in SAR Interferograms: The Split-Spectrum Method. IEEE Trans. Geosci. Remote Sens. 2016, 54, 1446–1461. [Google Scholar] [CrossRef]
- Rosen, P.A.; Hensley, S.; Chen, C. Measurement and mitigation of the ionosphere in L-band Interferometric SAR data. In Proceedings of the IEEE National Radar Conference–Proceedings, Arlington, VA, USA, 10–14 May 2010; pp. 1459–1463. [Google Scholar]
- Xu, X.; Sandwell, D.T.; Tymofyeyeva, E.; Gonzalez-Ortega, A.; Tong, X. Tectonic and anthropogenic deformation at the cerro prieto geothermal step-over revealed by sentinel-1A insar. IEEE Trans. Geosci. Remote Sens. 2017, 55, 5284–5292. [Google Scholar] [CrossRef]
- Bekaert, D.P.S.; Walters, R.J.; Wright, T.J.; Hooper, A.J.; Parker, D.J. Statistical comparison of InSAR tropospheric correction techniques. Remote Sens. Environ. 2015, 170, 40–47. [Google Scholar] [CrossRef]
- Lin, Y.N.N.; Simons, M.; Hetland, E.A.; Muse, P.; Dicaprio, C. A multiscale approach to estimating topographically correlated propagation delays in radar interferograms. Geochem. Geophys. Geosystems 2010, 11, Q09002. [Google Scholar] [CrossRef]
- Tymofyeyeva, E.; Fialko, Y. Mitigation of atmospheric phase delays in InSAR data, with application to the eastern California shear zone. J. Geophys. Res. Solid Earth 2015, 120, 5952–5963. [Google Scholar] [CrossRef]
- Löfgren, J.S.; Björndahl, F.; Moore, A.W.; Webb, F.H.; Fielding, E.J.; Fishbein, E.F. Tropospheric correction for InSAR using interpolated ECMWF data and GPS zenith total delay from the Southern California integrated GPS network. In Proceedings of the International Geoscience and Remote Sensing Symposium (IGARSS), Honolulu, HI, USA, 25–30 July 2010; pp. 4503–4506. [Google Scholar]
- Gong, W.; Meyer, F.; Webley, P.W.; Morton, D.; Liu, S. Performance analysis of atmospheric correction in InSAR data based on the Weather Research and Forecasting Model (WRF). In Proceedings of the International Geoscience and Remote Sensing Symposium (IGARSS), Honolulu, HI, USA, 25–30 July 2010; pp. 2900–2903. [Google Scholar]
- Jolivet, R.; Grandin, R.; Lasserre, C.; Doin, M.P.; Peltzer, G. Systematic InSAR tropospheric phase delay corrections from global meteorological reanalysis data. Geophys. Res. Lett. 2011, 38, L17311. [Google Scholar] [CrossRef]
- Li, Z.; Fielding, E.J.; Cross, P.; Preusker, R. Advanced InSAR atmospheric correction: MERIS/MODIS combination and stacked water vapour models. Int. J. Remote Sens. 2009, 30, 3343–3363. [Google Scholar] [CrossRef]
- Li, Z.; Muller, J.P.; Cross, P.; Albert, P.; Fischer, J.; Bennartz, R. Assessment of the potential of MERIS near-infrared water vapour products to correct ASAR interferometric measurements. Int. J. Remote Sens. 2006, 27, 349–365. [Google Scholar] [CrossRef]
- Cheng, S.; Perissin, D.; Lin, H.; Chen, F. Atmospheric delay analysis from GPS meteorology and InSAR APS. J. Atmos. Sol.-Terr. Phys. 2012, 86, 71–82. [Google Scholar] [CrossRef]
- Zhu, W.; Ding, X.L.; Jung, H.S.; Zhang, Q.; Zhang, B.C.; Qu, W. Investigation of ionospheric effects on SAR Interferometry (InSAR): A case study of Hong Kong. Adv. Space Res. 2016, 58, 564–576. [Google Scholar] [CrossRef]
- Markušić, S.; Stanko, D.; Korbar, T.; Belić, N.; Penava, D.; Kordić, B. The Zagreb (Croatia) M5.5 Earthquake on 22 March 2020. Geosciences 2020, 10, 252. [Google Scholar] [CrossRef]
- Braun, A.; Veci, L. TOPS Interferometry Tutorial; SkyWatch Space Applications Inc.: Paris, France, 2020. [Google Scholar]
- Crosetto, M.; Monserrat, O.; Cuevas, M.; Crippa, B. Spaceborne Differential SAR Interferometry: Data Analysis Tools for Deformation Measurement. Remote Sens. 2011, 3, 305–318. [Google Scholar] [CrossRef]
- Zhu, C.H.; Wang, C.S.; Zhang, B.C.; Qin, X.Q.; Shan, X.J. Differential Interferometric Synthetic Aperture Radar data for more accurate earthquake catalogs. Remote Sens. Environ. 2021, 266, 11. [Google Scholar] [CrossRef]
- Zebker, H.A.; Rosen, P.A.; Goldstein, R.M.; Gabriel, A.; Werner, C.L. On The Derivation of Coseismic Displacement-Fields Using Differential Radar Interferometry–The Landers Earthquake. J. Geophys. Res. Solid Earth 1994, 99, 19617–19634. [Google Scholar] [CrossRef]
- Dalla Via, G.; Crosetto, M.; Crippa, B. Resolving vertical and east-west horizontal motion from differential interferometric synthetic aperture radar: The L’Aquila earthquake. J. Geophys. Res. Solid Earth 2012, 117, 14. [Google Scholar] [CrossRef]
- Ferretti, A. Satellite InSAR Data: Reservoir Monitoring from Space; Earthdoc: London, UK, 2014. [Google Scholar]
- Viher, M. A study of the modified refraction indices over the Alpine and sub-Alpine region. Meteorol. Z. 2006, 15, 625–630. [Google Scholar] [CrossRef]
- Susaki, J.; Maeda, N.; Akatsuka, S. Estimation of phase delay due to precipitable water for DInSAR-based land deformation monitoring. ISPRS Ann. Photogramm. Remote Sens. Spat. Inf. Sci. 2017, IV-2/W4, 477–484. [Google Scholar] [CrossRef]
- International Radio Consultative Committee. Recommendations and reports of the CCIR, 1986; International Radio Consultative Committee: Dubrovnik, Croatia, 1986; p. 19. [Google Scholar]
- Patterson, W.; Hattan, C.; Hitney, H.; Paulus, R.; Lindem, G. Engineer’s Refractive Effects Prediction System (EREPS) Version 3.0; Naval Command Control and Ocean Surveillance Center Rdt and E Division: San Diego, CA, USA, 1994. [Google Scholar]
- Bech, J.; Codina, B.; Lorente, J. Forecasting weather radar propagation conditions. Meteorol. Atmos. Phys. 2007, 96, 229–243. [Google Scholar] [CrossRef]
- Klobuchar, J.A.; Kunches, J.M. Comparative range delay and variability of the earth’s troposphere and the ionosphere. GPS Solut. 2003, 7, 55–58. [Google Scholar] [CrossRef]
- Vuković, J.; Kos, T. Locally adapted NeQuick 2 model performance in European middle latitude ionosphere under different solar, geomagnetic and seasonal conditions. Adv. Space Res. 2017, 60, 1739–1750. [Google Scholar] [CrossRef]
- RTCA. DO-229C Minimum Operational Performance Standards for Global Positioning System/Wide Area Augmentation System Airborne Equipment; RTCA: Washington, DC, USA, 2001. [Google Scholar]
- Liao, W.T.; Tseng, K.H.; Lee, I.T.; Liibusk, A.; Lee, J.C.; Liu, J.Y.; Chang, C.P.; Lin, Y.C. Sentinel-1 interferometry with ionospheric correction from global and local TEC maps for land displacement detection in Taiwan. Adv. Space Res. 2020, 65, 1447–1465. [Google Scholar] [CrossRef]
- Bartels, J. The standardized index, Ks, and the planetary index, Kp. IATME Bull. 1949, 97, 0001. [Google Scholar]
- Mandic, I.; Vujic, E.; Heilig, B.; Pelajic, I.; Herak, D. Recent Efforts Toward the Establishment of the Lonjsko Polje Geomagnetic Observatory. Acta Geophys. 2016, 64, 1311–1339. [Google Scholar] [CrossRef]
- Sugiura, M. Hourly Values of Equatorial Dst for the IGY; Pergamon Press: Oxford, UK, 1964; pp. 7–45. [Google Scholar]
- Nose, M.; Sugiura, M.; Kamei, T.; Iyemori, T.; Koyama, Y. Dst Index. WDC for Geomagnetism, Kyoto: 2015. Available online: https://doi.org/10.17593/14515-74000 (accessed on 11 October 2022).
- Gurtner, W.; Estey, L. RINEX: The Receiver Independent Exchange Format Version 2.11; Astronomical Institute, University of Bern: Bern, Switzerland, 2007. [Google Scholar]
- Ciraolo, L.; Azpilicueta, F.; Brunini, C.; Meza, A.; Radicella, S.M. Calibration errors on experimental slant total electron content (TEC) determined with GPS. J. Geod. 2007, 81, 111–120. [Google Scholar] [CrossRef]
- Prtenjak, M.T.; Horvat, I.; Tomazic, I.; Kvakic, M.; Viher, M.; Grisogono, B. Impact of mesoscale meteorological processes on anomalous radar propagation conditions over the northern Adriatic area. J. Geophys. Res. Atmos. 2015, 120, 8759–8782. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Viher, M.; Vuković, J.; Racetin, I. A Study of Tropospheric and Ionospheric Propagation Conditions during Differential Interferometric SAR Measurements Applied on Zagreb 22 March 2020 Earthquake. Remote Sens. 2023, 15, 701. https://doi.org/10.3390/rs15030701
Viher M, Vuković J, Racetin I. A Study of Tropospheric and Ionospheric Propagation Conditions during Differential Interferometric SAR Measurements Applied on Zagreb 22 March 2020 Earthquake. Remote Sensing. 2023; 15(3):701. https://doi.org/10.3390/rs15030701
Chicago/Turabian StyleViher, Mladen, Josip Vuković, and Ivan Racetin. 2023. "A Study of Tropospheric and Ionospheric Propagation Conditions during Differential Interferometric SAR Measurements Applied on Zagreb 22 March 2020 Earthquake" Remote Sensing 15, no. 3: 701. https://doi.org/10.3390/rs15030701
APA StyleViher, M., Vuković, J., & Racetin, I. (2023). A Study of Tropospheric and Ionospheric Propagation Conditions during Differential Interferometric SAR Measurements Applied on Zagreb 22 March 2020 Earthquake. Remote Sensing, 15(3), 701. https://doi.org/10.3390/rs15030701






