Development of GNSS Buoy for Sea Surface Elevation Observation of Offshore Wind Farm
Abstract
:1. Introduction
- Philip J. Knight and colleagues developed a small, cost-effective GNSS buoy for tide and wave measurements in the intertidal zone. The buoy features a central platform surrounded by drag-reducing floats, employing a single-frequency GNSS receiver. It can operate for up to 4 days, and RTKLIB is used for data processing. While tidal measurements from the buoy align well with nearby coastal tide gauge stations, it experiences challenges during strong tidal currents, such as high tide, leading to discontinuities in the GNSS signal lock [9].
- Clemence Chupin and team installed GNSS devices on a floating carpet and an unmanned surface vehicle for sea level elevation observations in the nearshore area. Their tests demonstrated a measurement accuracy similar to tide gauge stations under stationary conditions, with minimal measurement errors in dynamic conditions [10].
- Yen-Pin Lin et al. developed a circular GNSS buoy for real-time tide and wave measurements using virtual base station and real-time kinematic GNSS-positioning technology. This 2.5 m diameter buoy, positioned approximately 2.1 km offshore from the coast of Taiwan, yielded tidal measurements with a root mean square error (RMSE) within 10 cm when compared to coastal tide gauge stations [11].
- Kato et al. utilized a single-frequency real-time kinematic GPS receiver on a cylindrical buoy measuring 3.4 m in radius and 15 m in height, and weighing 15 tons for tsunami monitoring in waters 20 km offshore [12].
2. Methods
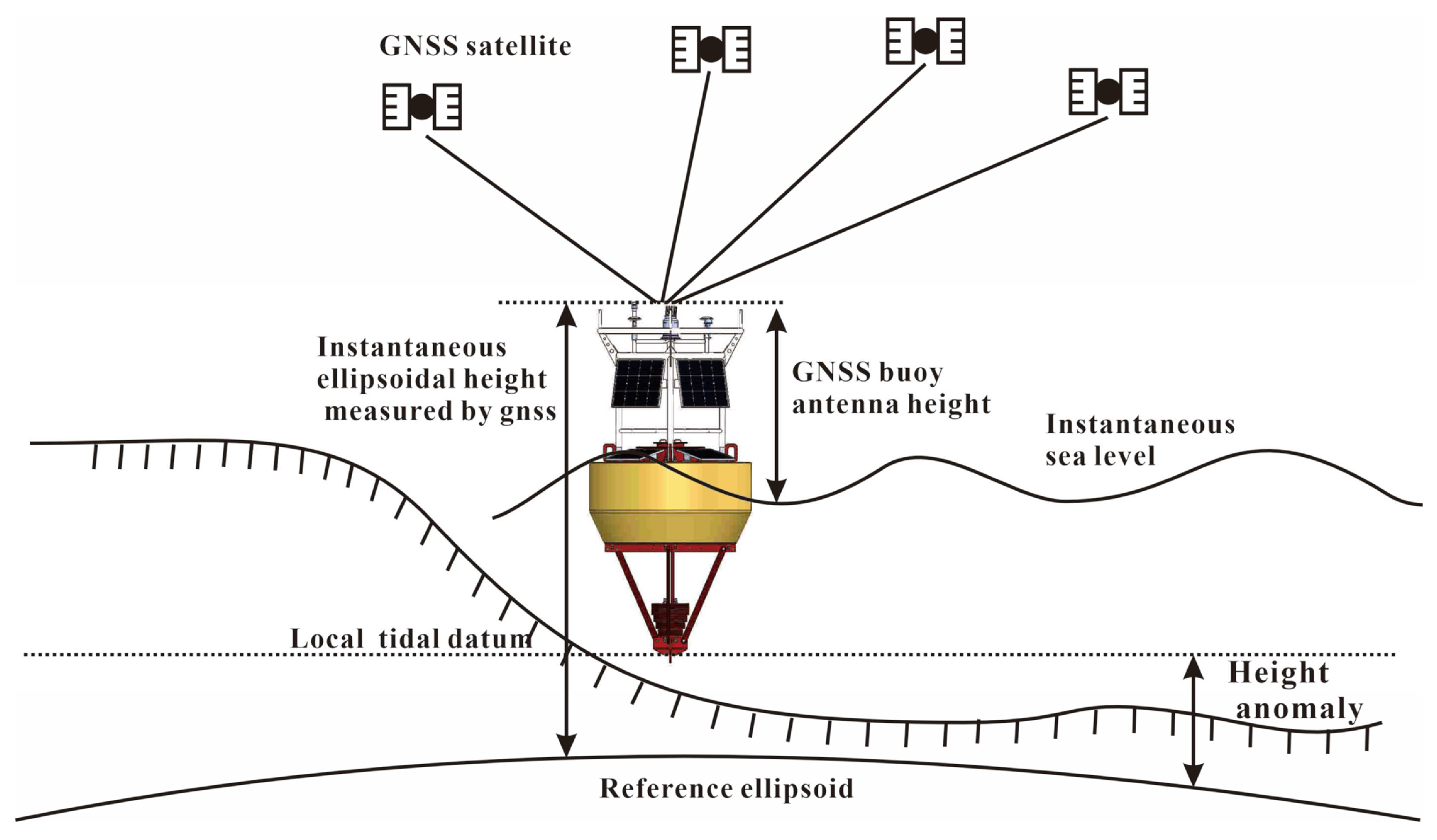
2.1. Principles of GNSS Buoy Tide-Level Measurement
2.2. Procedure of Data Processing
- Using the PPP technique to calculate the GNSS antenna phase center height involves the following steps: (a) combining the precise ephemeris and clock data provided by the International GNSS Service (IGS) with the GNSS observation data collected from the buoy’s antenna in Receiver Independent Exchange Format (RENIX) format; and (b) Employing Inertial Explorer software (Version 8.90) developed by NovAtel (Calgary, Canada) for data processing. Through this process, the precise three-dimensional co-ordinates of the observation station are obtained.
- During this data processing, some observed data may exhibit poor quality, causing difficulties in fixing the integer ambiguities in the carrier phase observations. This can result in gross errors in the final outputs, particularly in the elevation component. To address the issue, LARISA criteria are employed to diminish the errors of elevation data. Specifically, we first calculate the average elevation value and the standard deviation (σ) of the elevation data for each 1 min interval. If the value, , satisfies the condition , then it is considered a gross error and will be discarded.
- To better mitigate the multipath effects on GNSS receiver antennae, GNSS antennae are typically installed at a certain distance above the sea surface. Therefore, the GNSS receiver antenna’s phase height needs to be calibrated using attitude data to obtain the instantaneous sea surface elevation in the area. This measurement is achieved by the sea surface elevation measurement equipment. The formula is shown as follows:
- 4.
- The obtained instantaneous sea surface elevation is then subjected to a Kalman filter to remove high-frequency signals (i.e., wave motion and noise) [19]. The Kalman algorithm is a filtering technique based on a state-space model. It models the signal as a state variable and continuously adjusts the estimated value of the state variable to improve the accuracy of observations. The Kalman filter parameters are defined as follows: state transition matrix: ; observation matrix: ; state transition noise covariance matrix: ; and observation noise variance: .
- 5.
- The sea surface elevation obtained from the GNSS buoy measurements corresponds to geodetic height. It takes ellipsoidal surface as the elevation reference. Currently, sea surface elevation observations are typically referenced against the China National Height Datum 1985 (CNHD 1985). Sea surface elevation can be calculated by subtracting the observed geodetic height from the elevation anomaly of the observed sea area. The elevation anomaly of the observed sea area can be determined by using the EGM2008 model [20]. The EGM2008 is a spherical harmonic model of the Earth’s gravitational potential, developed by the National Geospatial-Intelligence Agency of the United States (Springfield, the United States). It has been developed over years by building upon previous experiences and theoretical foundations in constructing Earth’s gravity field models. The model utilizes the PGM2007B as a reference model and integrates gravity data collected from the GRACE (Gravity Recovery and Climate Experiment) satellites, along with global 5′ × 5′ gravity anomaly data.
2.3. Buoy Body Design
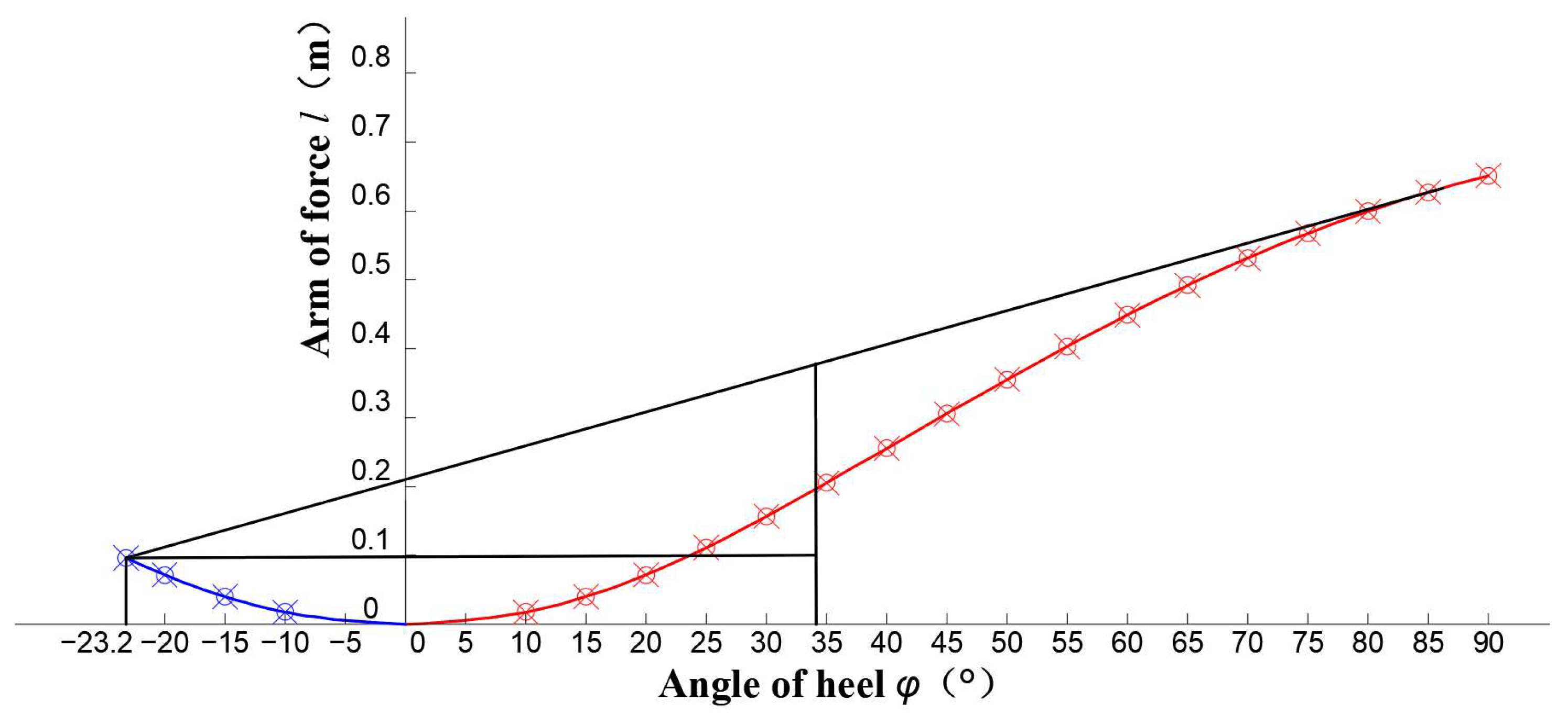
2.4. Buoy Stability Calculation
- Initial metacentric height: 1.056 m;
- Metacentric radius: 1.033 m;
- Natural roll period (free): 5.2 s;
- Maximum free roll angle: 15.3°;
- Maximum restoring arm: 0.58 m;
- Ultimate roll angle: 41°;
- Stability lost angle: >90°;
- Minimum capsizing arm: 0.27 m;
- Stability criterion number: 1.26.
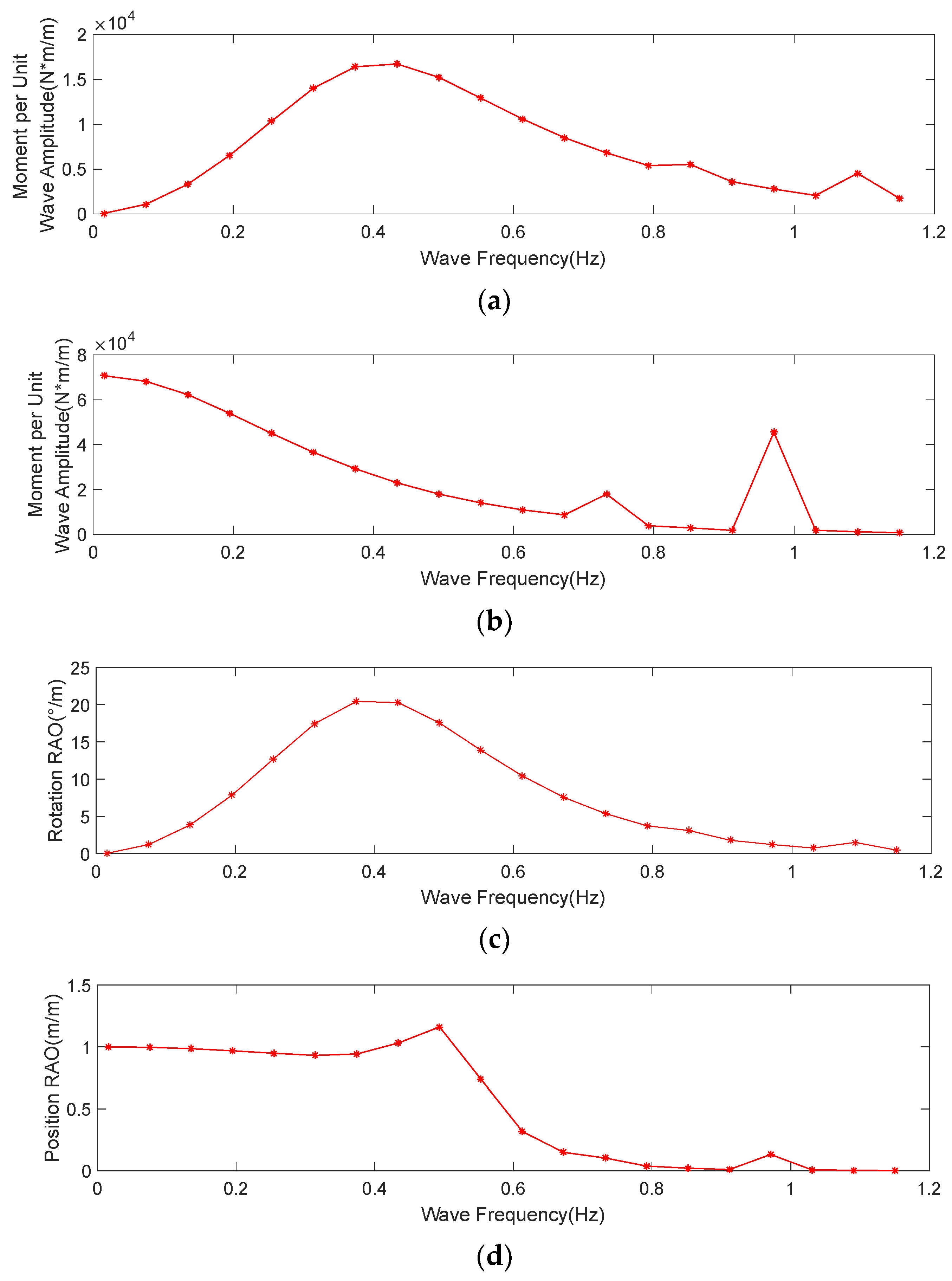
2.5. Buoy Hydrodynamic Analysis
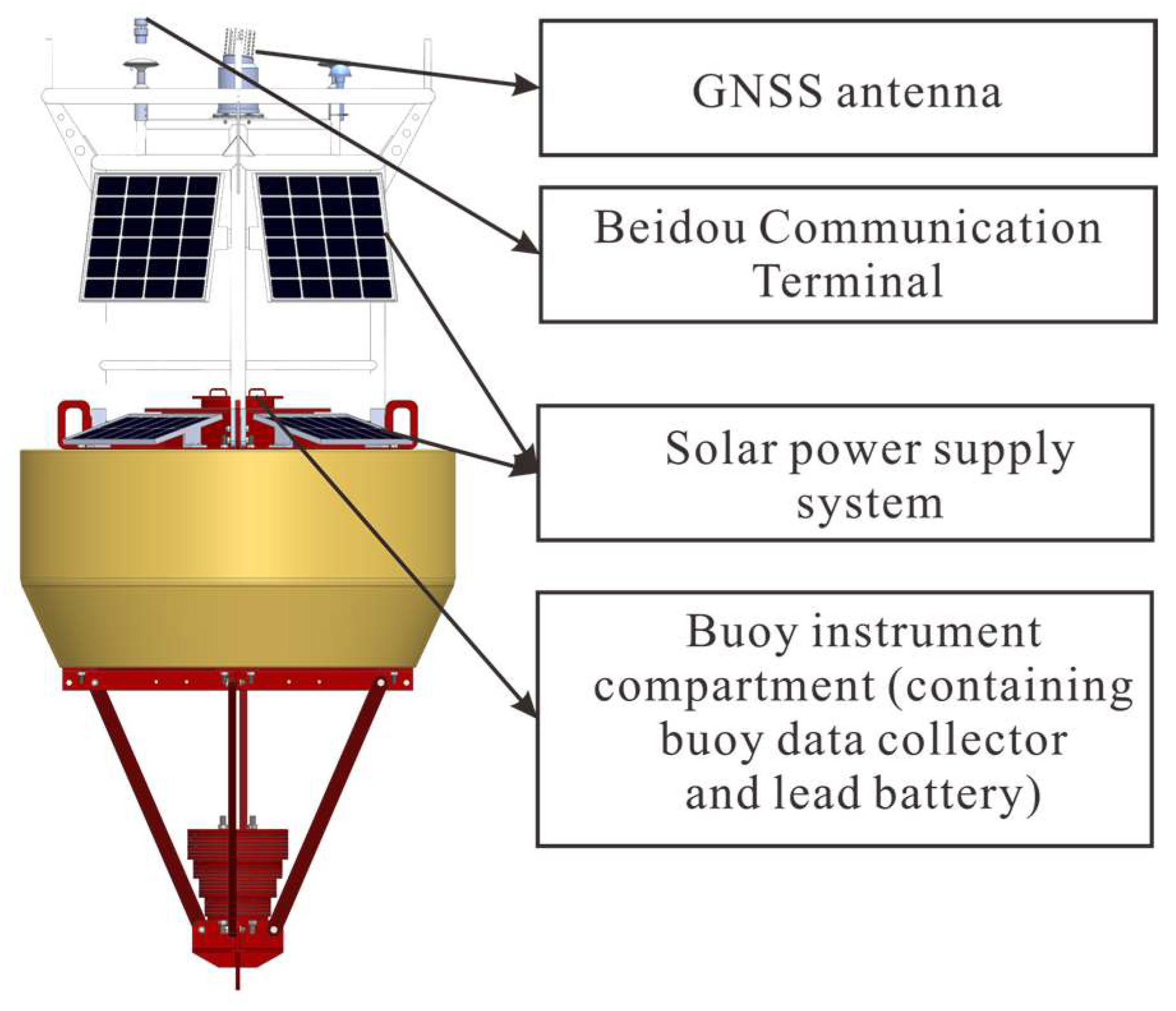
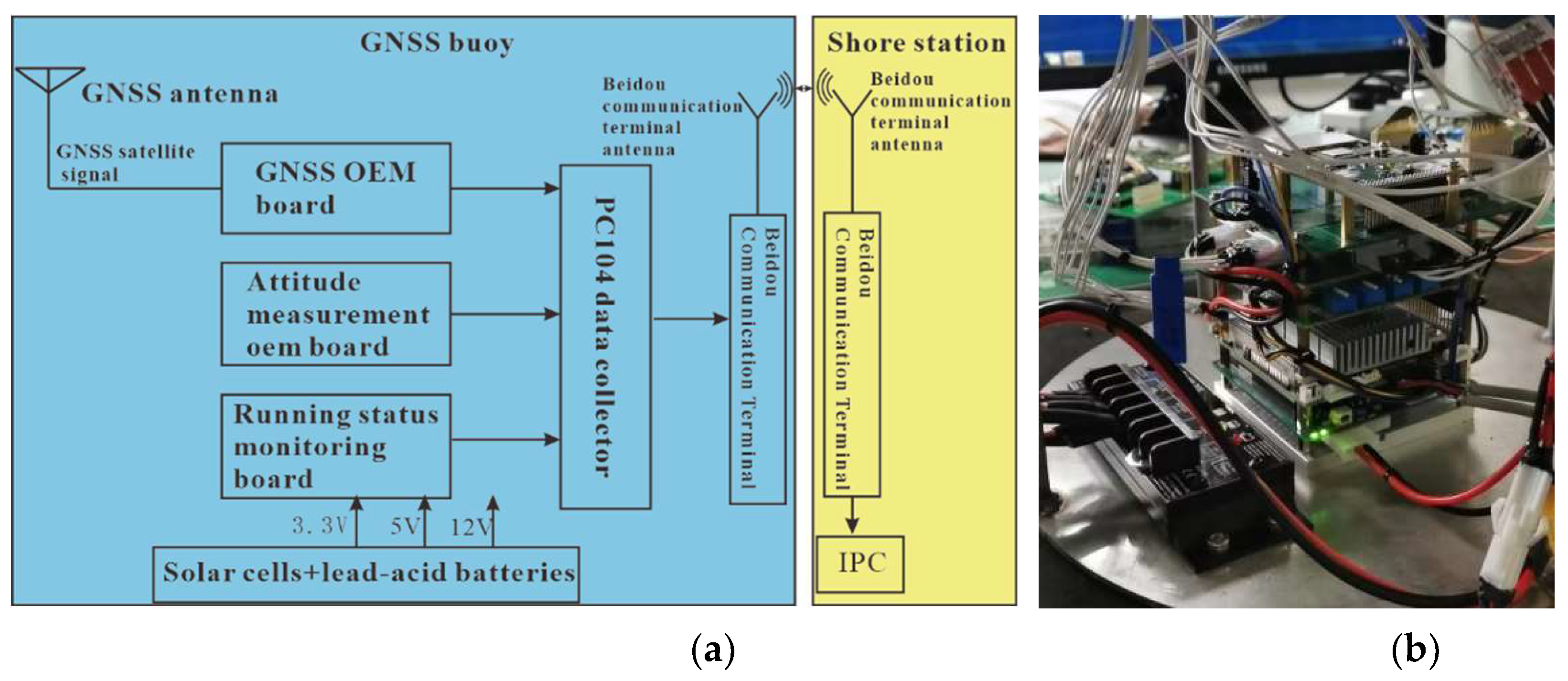
2.6. Development of Buoy Data Acquisition Unit
3. Results
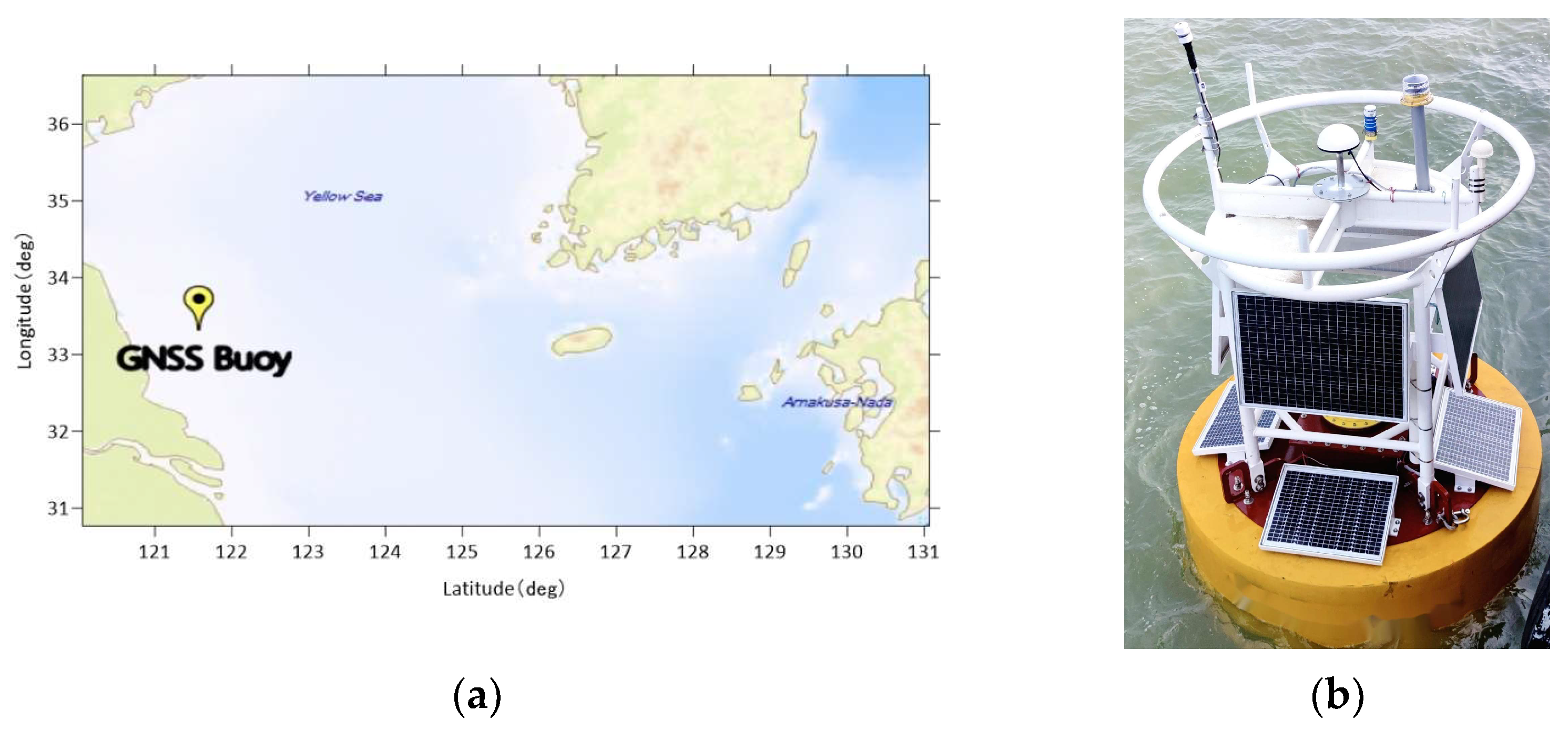
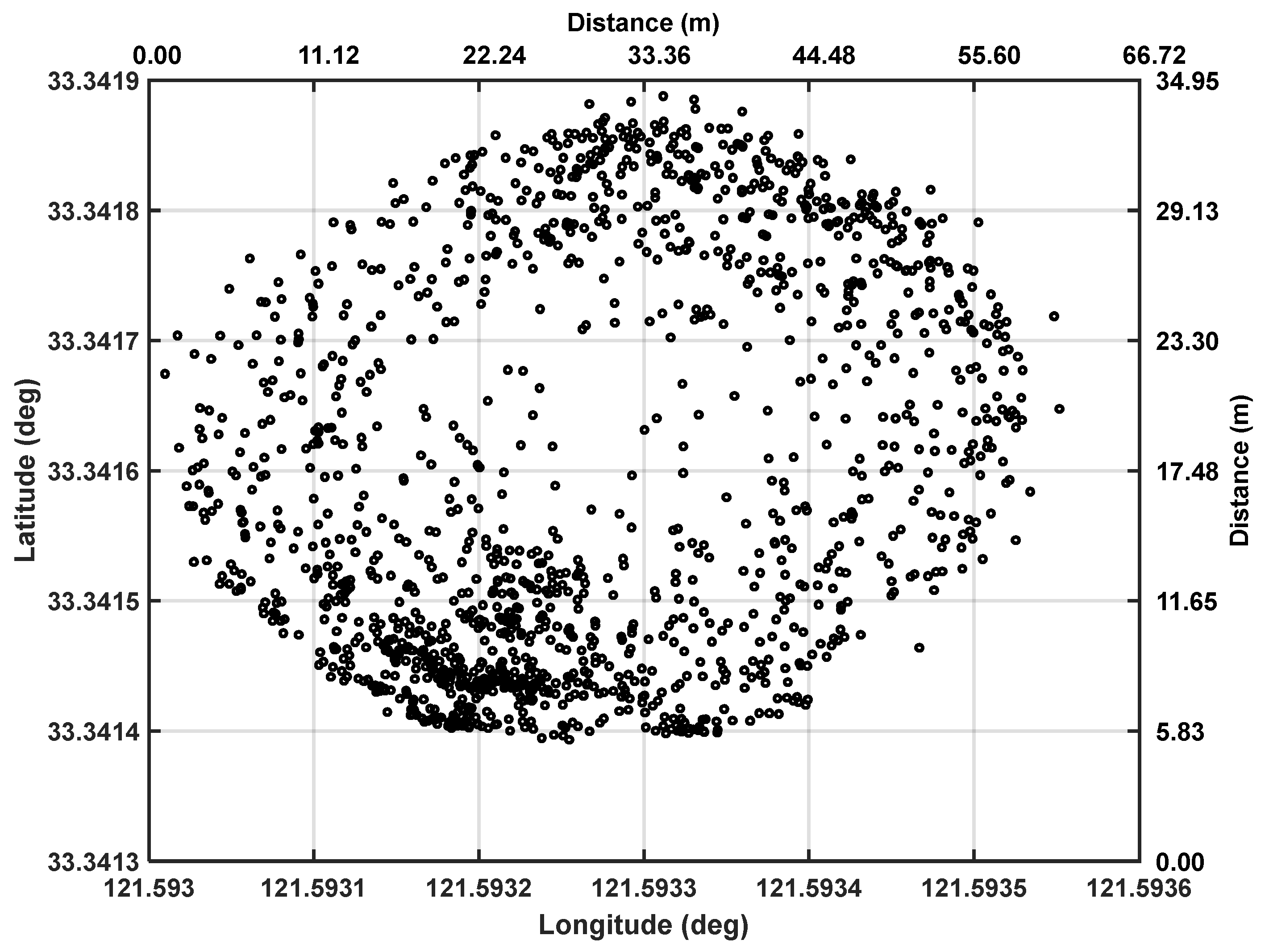
3.1. Performance Evaluation on Sea Trial
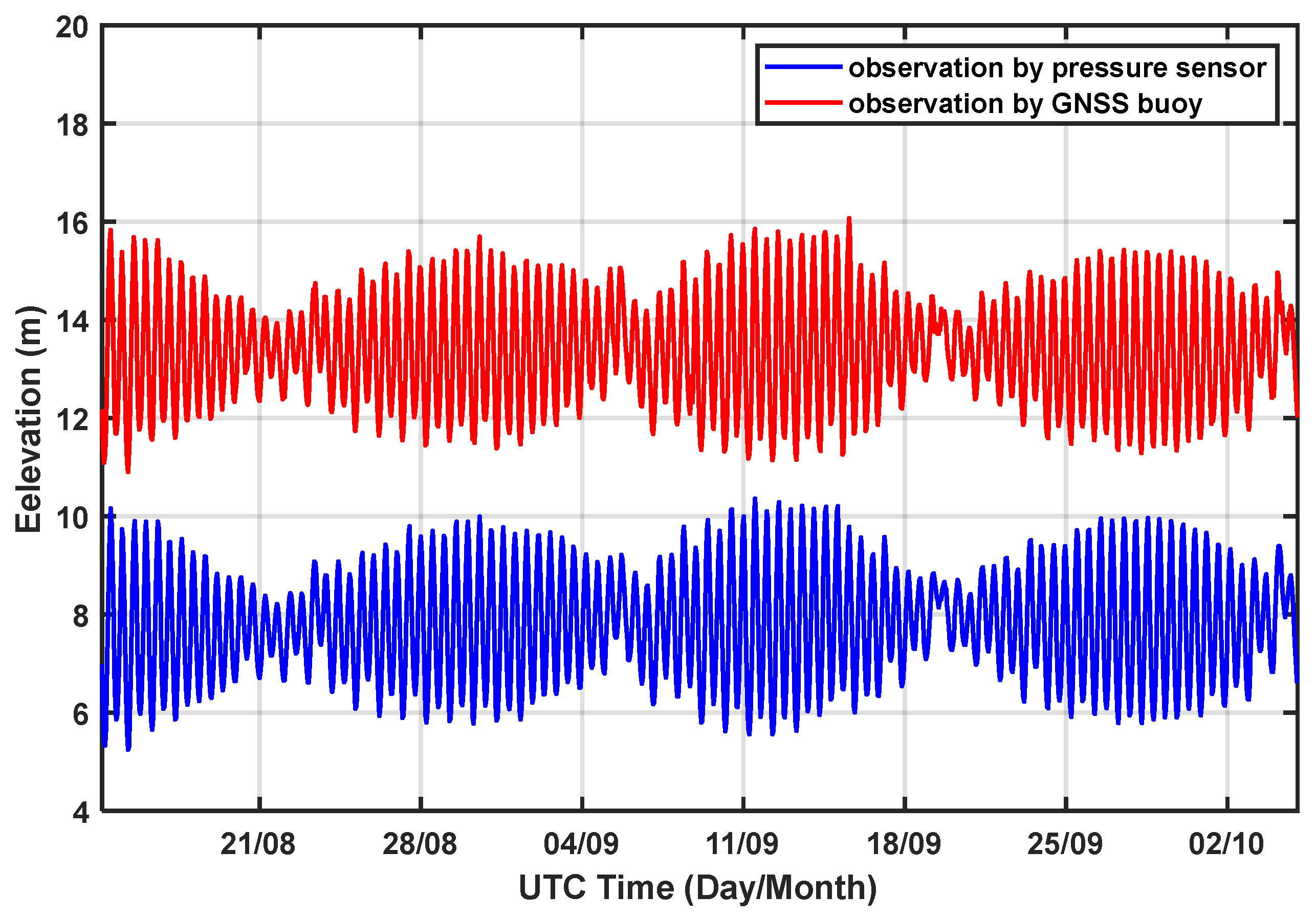
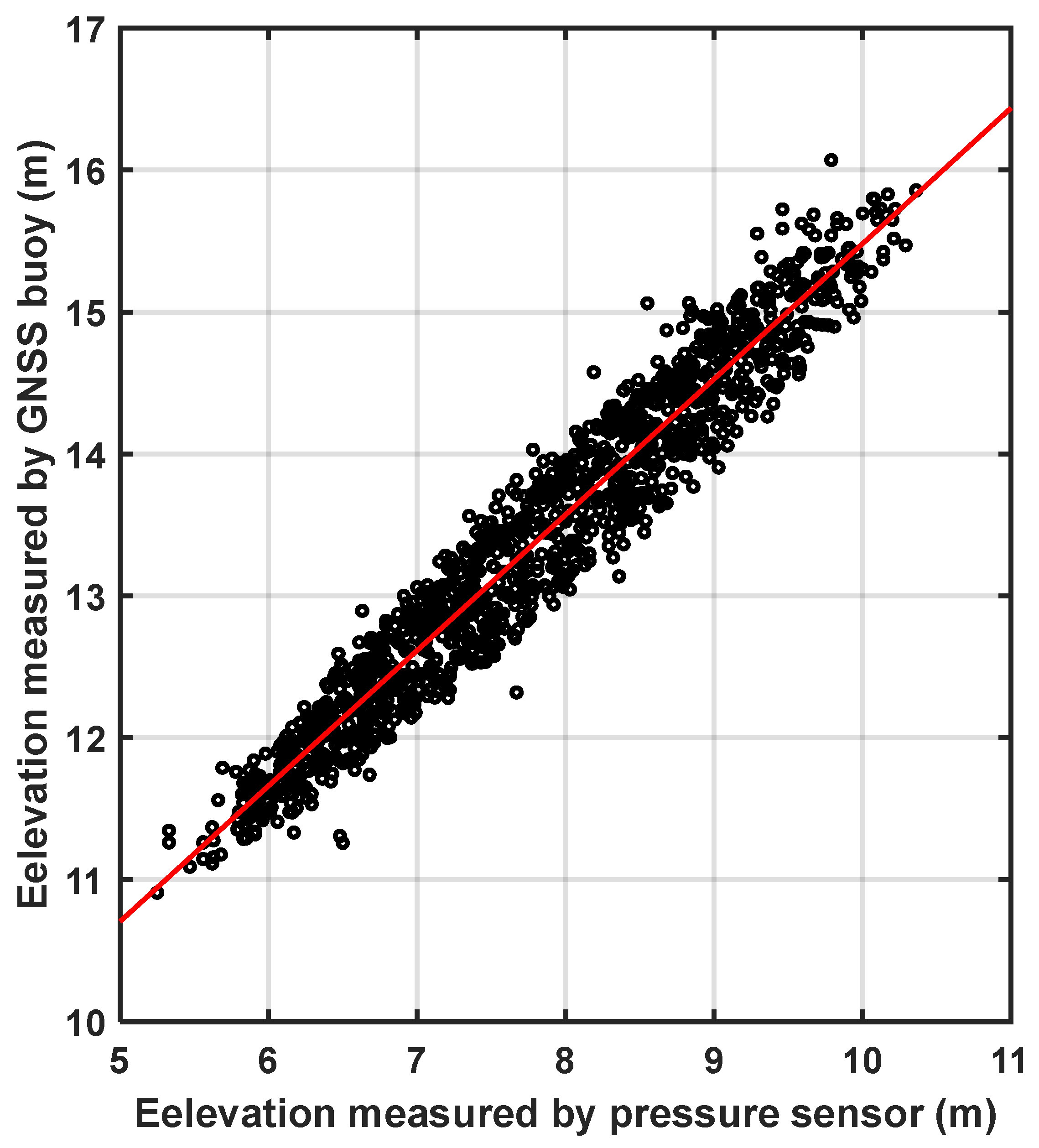
3.2. Marine Test Results
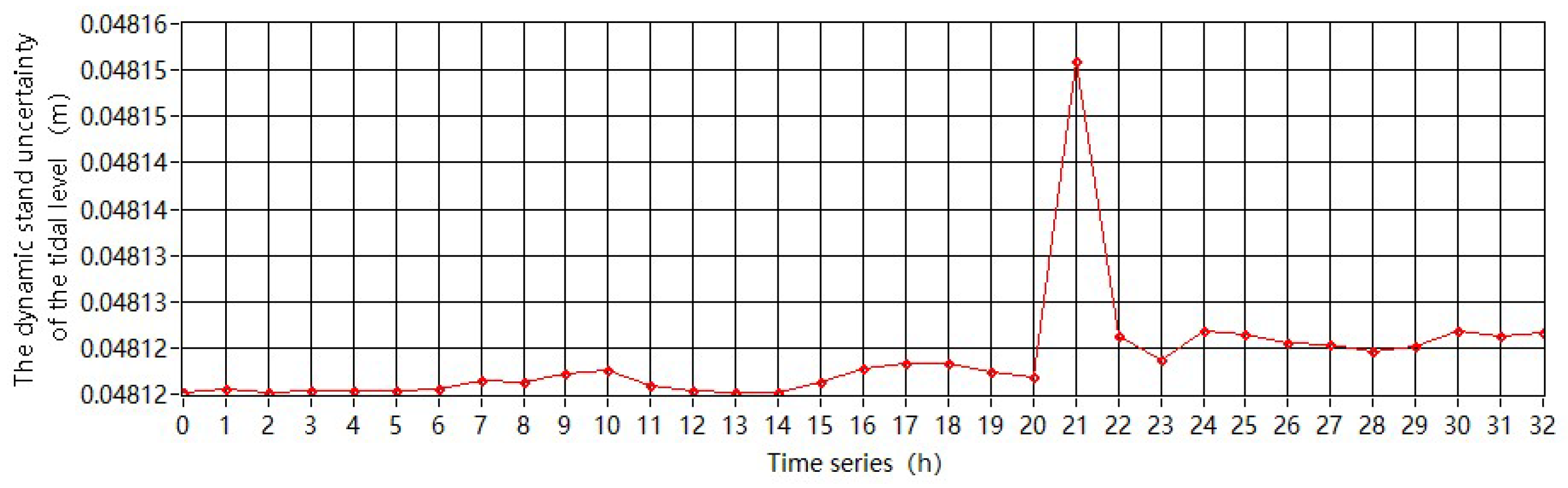
3.3. Uncertainty Evaluation of Sea Surface Elevation
4. Discussion
4.1. The Influence of GNSS Buoy Data Collection Approach on Measurement Results
4.2. The Impact of Float Design and Simulation on Technological Applications
4.3. Real-Time Sea Surface Elevation Measurements by GNSS Buoy
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ko, D.H.; Jeong, S.T.; Cho, H.-Y.; Kang, K.-S. Analysis on the estimation errors of the lowest and highest astronomical tides for the southwestern 2.5 GW offshore wind farm, Korea. Int. J. Nav. Archit. Ocean Eng. 2018, 10, 85–94. [Google Scholar] [CrossRef]
- Christie, E.; Li, M.; Moulinec, C. Comparison of 2D and 3D large scale morphological modeling of offshore wind farms using HPC. Coast. Eng. Proc. 2012, 1, 42–48. [Google Scholar] [CrossRef]
- Loughney, S.; Wang, J.; Bashir, M.; Armin, M.; Yang, Y. Development and application of a multiple-attribute decision-analysis methodology for site selection of floating offshore wind farms on the UK Continental Shelf. Sustain. Energy Technol. Assess. 2021, 47, 101440. [Google Scholar] [CrossRef]
- Yu, Y.; Mi, Z.Q.; Liu, X.J.; Sun, L. Modeling method of large-scale wind farm based on operating data. Acta Energiae Solaris Sin. 2011, 32, 1543–1548. [Google Scholar]
- Díaz, H.; Soares, C.G. An integrated GIS approach for site selection of floating offshore wind farms in the Atlantic continental European coastline. Renew. Sustain. Energy Rev. 2020, 134, 110328. [Google Scholar] [CrossRef]
- Giardina, M.F.; Earle, M.D.; Cranford, J.C.; Osiecki, D.A. Development of a Low-Cost Tide Gauge. J. Atmos. Ocean. Technol. 2000, 17, 575–583. [Google Scholar] [CrossRef]
- Míguez, B.M.; Testut, L.; Wöppelmann, G. Performance of modern tide gauges: Towards mm-level accuracy. Sci. Mar. 2012, 76, 221–228. [Google Scholar] [CrossRef]
- Dawidowicz, K. Sea level changes monitoring using GNSS technology—A review of recent efforts. Acta Adriat. 2014, 55, 145–161. [Google Scholar]
- Knight, P.J.; Bird, C.O.; Sinclair, A.; Higham, J.; Plater, A.J. Beach Deployment of a Low-Cost GNSS Buoy for Determining Sea-Level and Wave Characteristics. Geosciences 2021, 11, 494. [Google Scholar] [CrossRef]
- Chupin, C.; Ballu, V.; Testut, L.; Tranchant, Y.-T.; Calzas, M.; Poirier, E.; Coulombier, T.; Laurain, O.; Bonnefond, P.; Team FOAM Project. Mapping Sea Surface Height Using New Concepts of Kinematic GNSS Instruments. Remote Sens. 2020, 12, 2656. [Google Scholar] [CrossRef]
- Lin, Y.-P.; Huang, C.-J.; Chen, S.-H.; Doong, D.-J.; Kao, C.C. Development of a GNSS Buoy for Monitoring Water Surface Elevations in Estuaries and Coastal Areas. Sensors 2017, 17, 172. [Google Scholar] [CrossRef] [PubMed]
- Kato, T.; Terada, Y.; Tadokoro, K.; Futamura, A. Developments of GNSS buoy for a synthetic geohazard monitoring system. Proc. Jpn. Acad. Ser. B 2022, 98, 49–71. [Google Scholar] [CrossRef] [PubMed]
- Herbers, T.H.C.; Jessen, P.F.; Janssen, T.T.; Colbert, D.B.; MacMahan, J.H. Observing Ocean Surface Waves with GPS-Tracked Buoys. J. Atmos. Ocean. Technol. 2012, 29, 944–959. [Google Scholar] [CrossRef]
- Chung-Yen, K.; Kuan-Wei, C.; Kai-Wei, C.; Kai-Chien, C.; Li-Ching, L.; Hong-Zeng, T.; Hsiang-Tseng, L. High-frequency sea level variations observed by GPS buoys using precise point positioning technique. TAO Terr. Atmos. Ocean. Sci. 2012, 23, 209. [Google Scholar]
- Ma, H.; Zhao, Q.; Verhagen, S.; Psychas, D.; Liu, X. Assessing the Performance of Multi-GNSS PPP-RTK in the Local Area. Remote Sens. 2020, 12, 3343. [Google Scholar] [CrossRef]
- Yigit, C.O.; Gurlek, E. Experimental testing of high-rate GNSS precise point positioning (PPP) method for detecting dynamic vertical displacement response of engineering structures. Geomat. Nat. Hazards Risk 2017, 8, 893–904. [Google Scholar] [CrossRef]
- Hohensinn, R.; Stauffer, R.; Glaner, M.F.; Pinzón, I.D.H.; Vuadens, E.; Rossi, Y.; Clinton, J.; Rothacher, M. Low-Cost GNSS and Real-Time PPP: Assessing the Precision of the u-blox ZED-F9P for Kinematic Monitoring Applications. Remote Sens. 2022, 14, 5100. [Google Scholar] [CrossRef]
- Jiang, Z.H.; Zhang, P.; Bei, J.Z.; Li, Z.C. Characteristics of the non-linear movement of CORS network in China based on the CGCS2000 frame. Chin. J. Geophys. 2012, 55, 841–850. [Google Scholar]
- Slobbe, D.C.; Sumihar, J.; Frederikse, T.; Verlaan, M.; Klees, R.; Zijl, F.; Farahani, H.H.; Broekman, R. A Kalman Filter Approach to Realize the Lowest Astronomical Tide Surface. Mar. Geodesy 2017, 41, 44–67. [Google Scholar] [CrossRef]
- Pavlis, N.K.; Holmes, S.A.; Kenyon, S.C.; Factor, J.K. The development and evaluation of the Earth Gravitational Model 2008 (EGM2008). J. Geophys. Res. Solid Earth 2012, 117, 8916. [Google Scholar] [CrossRef]
- National Marine Standardization Technical Committee. Small Mooring Buoy System for Ocean Observing: HYT 143-2011; State Oceanic Administration: Beijing, China, 2011; pp. 6–8. [Google Scholar]
- Wang, Y.-L. Design of a cylindrical buoy for a wave energy converter. Ocean Eng. 2015, 108, 350–355. [Google Scholar] [CrossRef]
- Shi, H.; Han, Z.; Zhao, C. Numerical study on the optimization design of the conical bottom heaving buoy convertor. Ocean Eng. 2019, 173, 235–243. [Google Scholar] [CrossRef]
- Zhang, S.; Yang, W.; Xin, Y.; Wang, R.; Li, C.; Cai, D.; Gao, H. Prototype System Design of Mooring Buoy for Seafloor Observation and Construction of Its Communication Link. J. Coast. Res. 2018, 83, 41–49. [Google Scholar] [CrossRef]
- Arany, L.; Bhattacharya, S. Simplified load estimation and sizing of suction anchors for spar buoy type floating offshore wind turbines. Ocean Eng. 2018, 159, 348–357. [Google Scholar] [CrossRef]
- Maritime Safety Administration of the People’s Republic of China. Technical Rules for Statutory Inspection of Domestic Navigating Marine Vessels; China Communication Press: Beijing, China, 2011; pp. 212–216. [Google Scholar]
- Ghafari, H.; Dardel, M. Parametric study of catenary mooring system on the dynamic response of the semi-submersible plaform. Ocean Eng. 2018, 153, 319–332. [Google Scholar] [CrossRef]
- Bódai, T.; Srinil, N. Performance analysis and optimization of a box-hull wave energy converter concept. Renew. Energy 2015, 81, 551–565. [Google Scholar] [CrossRef]
- Amaechi, C.V.; Wang, F.; Hou, X.; Ye, J. Strength of submarine hoses in Chinese-lantern configuration from hydrodynamic loads on CALM buoy. Ocean Eng. 2018, 171, 429–442. [Google Scholar] [CrossRef]
- Liu, K.; Sun, J.; Guo, C.; Yang, Y.; Yu, W.; Wei, Z. Seasonal and Spatial Variations of the M 2 Internal Tide in the Yellow Sea. J. Geophys. Res. Oceans 2019, 124, 1115–1138. [Google Scholar] [CrossRef]
- Li, S.; Liu, L.; Cai, S.; Wang, G. Tidal harmonic analysis and prediction with least-squares estimation and inaction method. Estuar. Coast. Shelf Sci. 2019, 220, 196–208. [Google Scholar] [CrossRef]
- Mauder, M.; Cuntz, M.; Drüe, C.; Graf, A.; Rebmann, C.; Schmid, H.P.; Schmidt, M.; Steinbrecher, R. A strategy for quality and uncertainty assessment of long-term eddy-covariance measurements. Agric. For. Meteorol. 2013, 169, 122–135. [Google Scholar] [CrossRef]


| Parameter | Value |
|---|---|
| Design diameter | 2.30 m |
| Design mass | kg |
| Design height | 5.11 m |
| Reserved buoyancy | kg |
| Center of gravity height | 1.23 m |
| Buoyant center height | 1.26 m |
| Draft height | 1.55 m |
| Buoy projected area normal to the wind | 3.29 m2 |
| Buoy projected area normal to the flow | 2.53 m2 |
| x-axis moment of inertia (CG) | kg∙m2 |
| y-axis moment of inertia (CG) | kg∙m2 |
| z-axis moment of inertia (CG) | kg∙m2 |
| Parameter | Value |
|---|---|
| Wind speed | 60.0 m/s |
| Wave period | 12.0 s |
| Wavelength | 224 m |
| Significant wave height | 15.0 m |
| Maximum wave height | 20.0 m |
| Surface current speed | 3.50 m/s |
| Parameter | Value |
|---|---|
| Buoy mass | kg |
| Antenna height | 2.21 m |
| Operating temperature | −20.0~80.0 °C |
| GNSS signals | GPS: L1, L2, L5, GPS: L1 C/A, L1C, L2C, L2P, L5 BeiDou: B1, B2, B3 GLONASS: L1 C/A, L2C, L2P, L3, L5 |
| Attitude accuracy | Roll/Pitch: 0.200° |
| GNSS data update rate | 5.00 Hz |
| Attitude data update rate | 5.00 Hz |
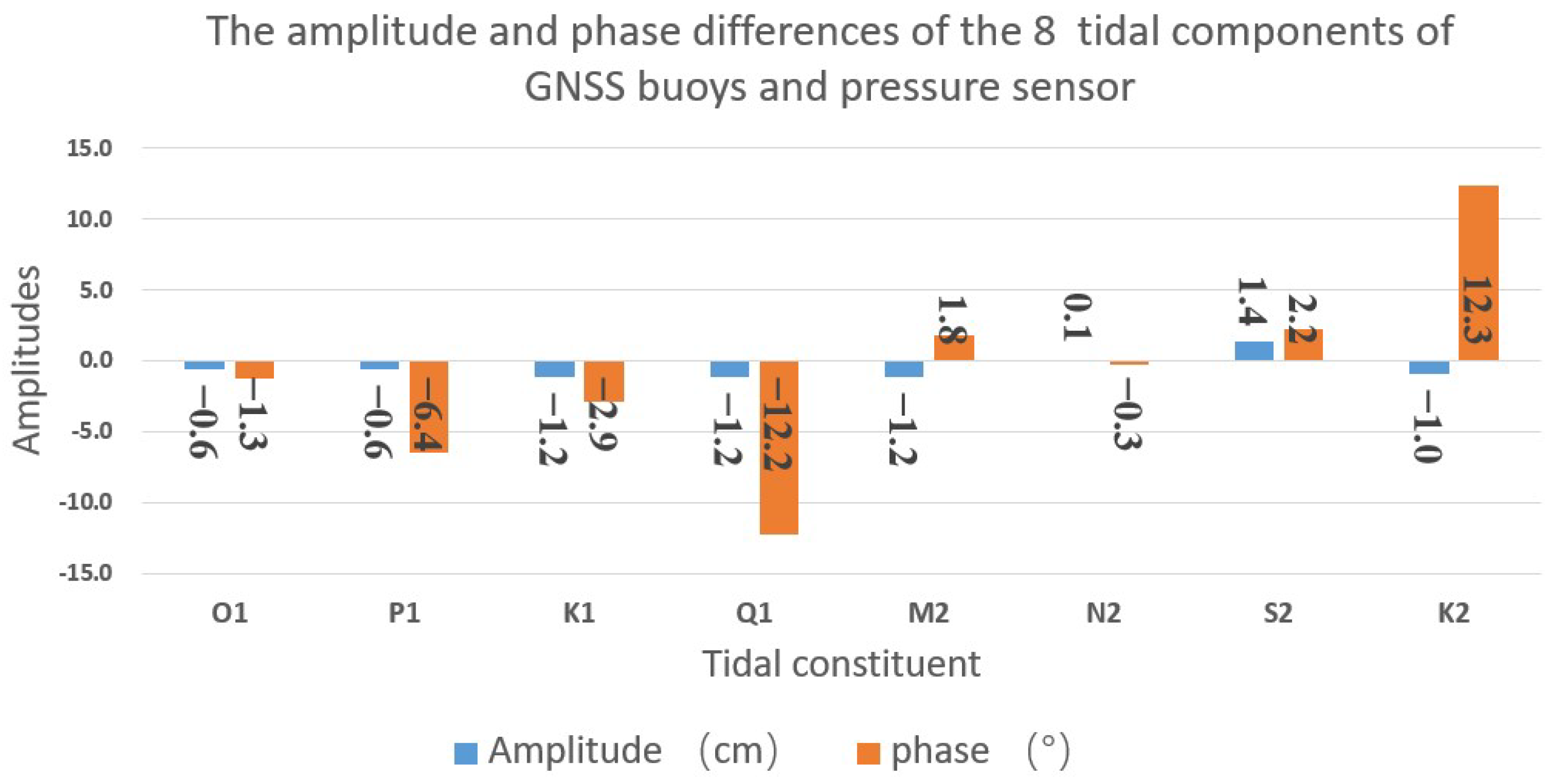
| O1 | P1 | K1 | Q1 | M2 | N2 | S2 | K2 | ||
|---|---|---|---|---|---|---|---|---|---|
| Amplitude (cm) | GNSS buoy | 16.1 | 6.1 | 20.4 | 2.8 | 137.7 | 20.8 | 58.8 | 21.2 |
| Pressure sensor | 16.7 | 6.7 | 21.6 | 3.9 | 138.9 | 20.7 | 57.5 | 22.1 | |
| Difference | −0.6 | −0.6 | −1.2 | −1.2 | −1.2 | 0.1 | 1.4 | −1.0 | |
| Phase (°) | GNSS buoy | 257.0 | 293.6 | 326.4 | 168.1 | 96.9 | 63.2 | 130.1 | 155.7 |
| Pressure sensor | 258.3 | 300.0 | 329.3 | 180.3 | 95.1 | 63.5 | 127.9 | 143.4 | |
| Difference | −1.3 | −6.4 | −2.9 | −12.2 | 1.8 | −0.3 | 2.2 | 12.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, G.; Li, S.; Bao, K.; Wang, G.; Teng, F.; Zhang, F.; Wang, Y.; Guan, S.; Wei, Z. Development of GNSS Buoy for Sea Surface Elevation Observation of Offshore Wind Farm. Remote Sens. 2023, 15, 5323. https://doi.org/10.3390/rs15225323
Liang G, Li S, Bao K, Wang G, Teng F, Zhang F, Wang Y, Guan S, Wei Z. Development of GNSS Buoy for Sea Surface Elevation Observation of Offshore Wind Farm. Remote Sensing. 2023; 15(22):5323. https://doi.org/10.3390/rs15225323
Chicago/Turabian StyleLiang, Guanhui, Shujiang Li, Ke Bao, Guanlin Wang, Fei Teng, Fengye Zhang, Yanfeng Wang, Sheng Guan, and Zexun Wei. 2023. "Development of GNSS Buoy for Sea Surface Elevation Observation of Offshore Wind Farm" Remote Sensing 15, no. 22: 5323. https://doi.org/10.3390/rs15225323
APA StyleLiang, G., Li, S., Bao, K., Wang, G., Teng, F., Zhang, F., Wang, Y., Guan, S., & Wei, Z. (2023). Development of GNSS Buoy for Sea Surface Elevation Observation of Offshore Wind Farm. Remote Sensing, 15(22), 5323. https://doi.org/10.3390/rs15225323





