Two-Step Correction Based on In-Situ Sound Speed Measurements for USBL Precise Real-Time Positioning
Abstract
:1. Introduction
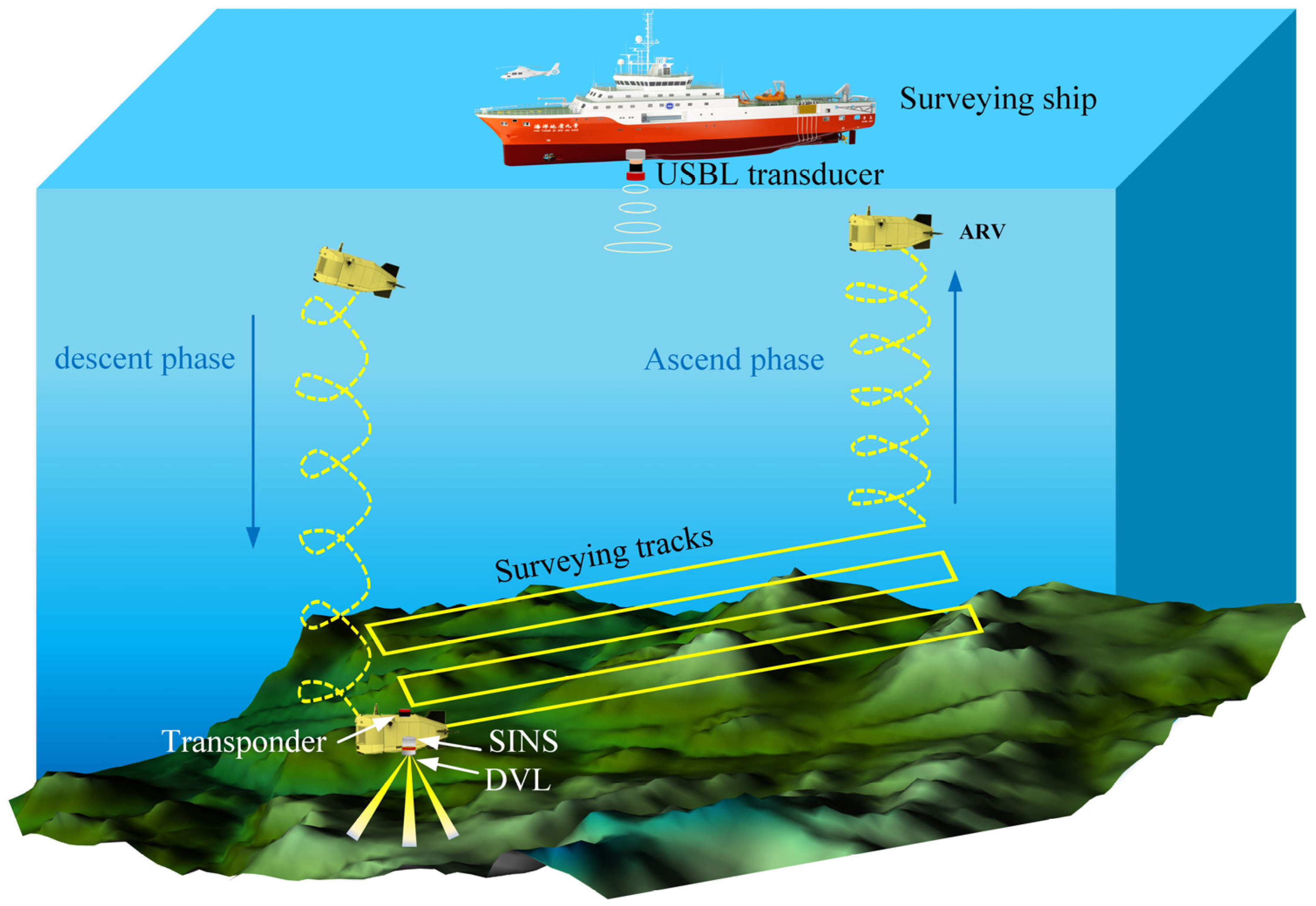
2. Materials and Methods
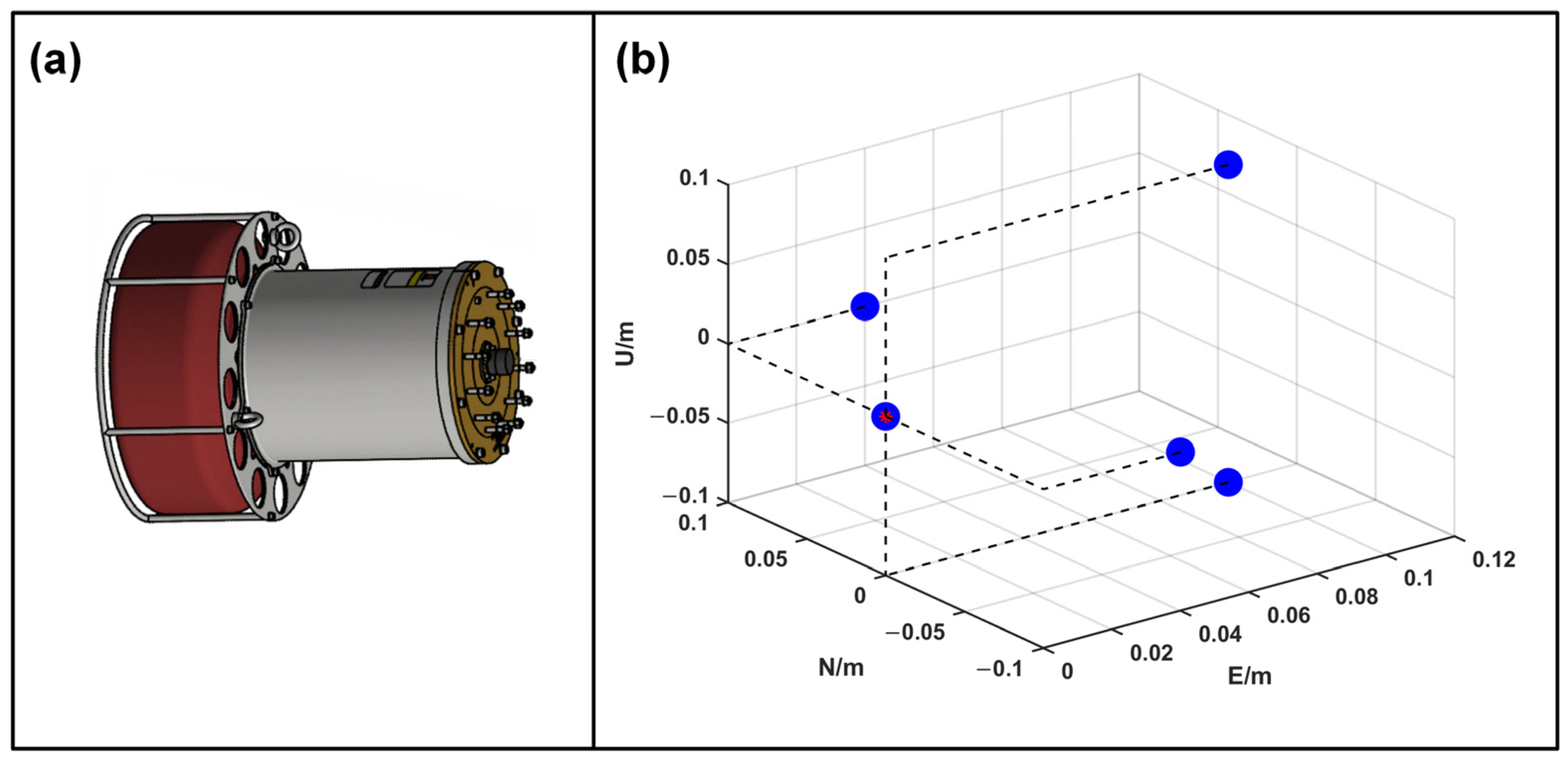
2.1. USBL Positioning Algorithm for ARV
2.2. Acoustic Ray Bending Correction for USBL Positioning
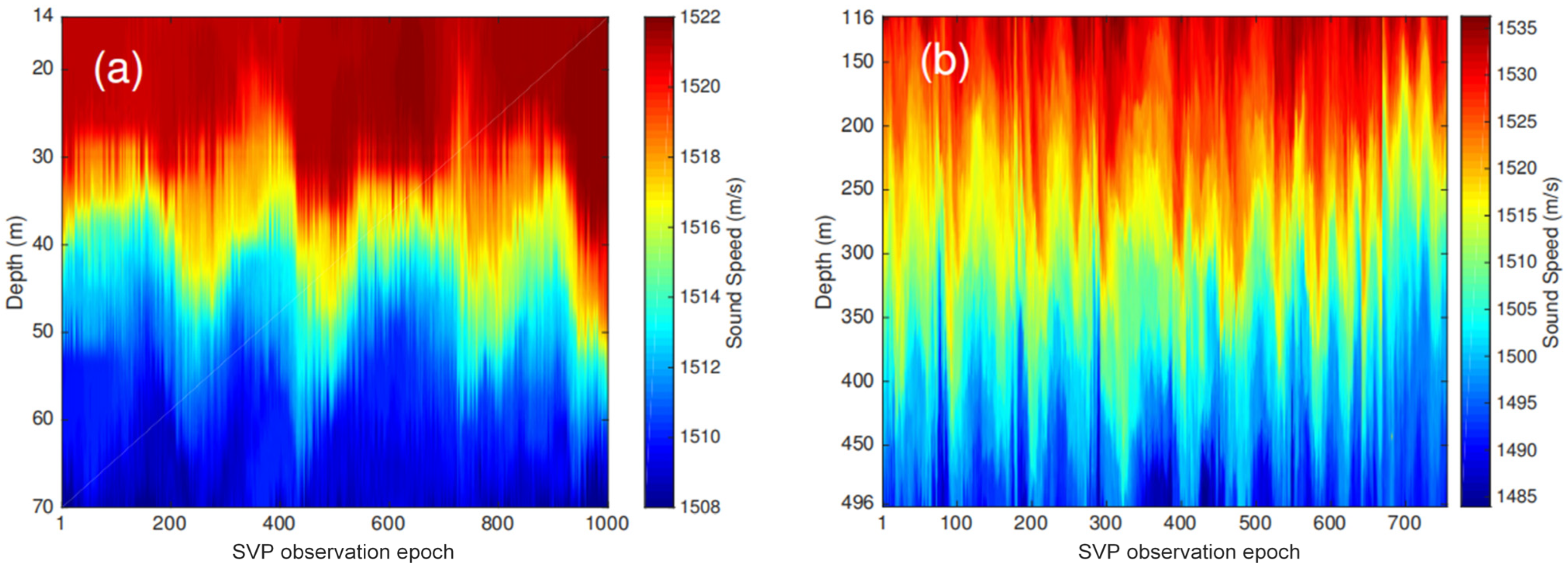
2.3. Temporal Sound Speed Correction for USBL Positioning
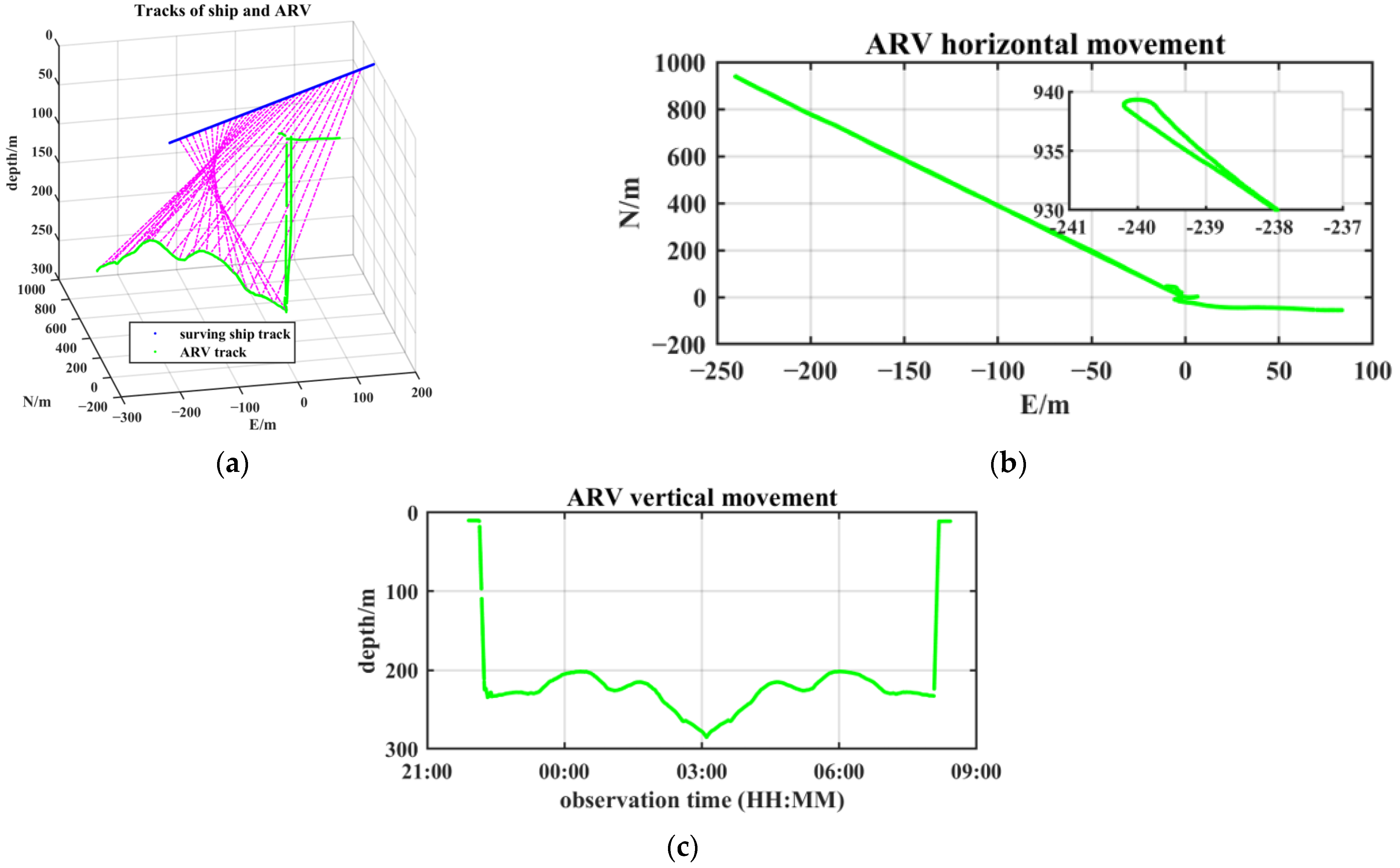
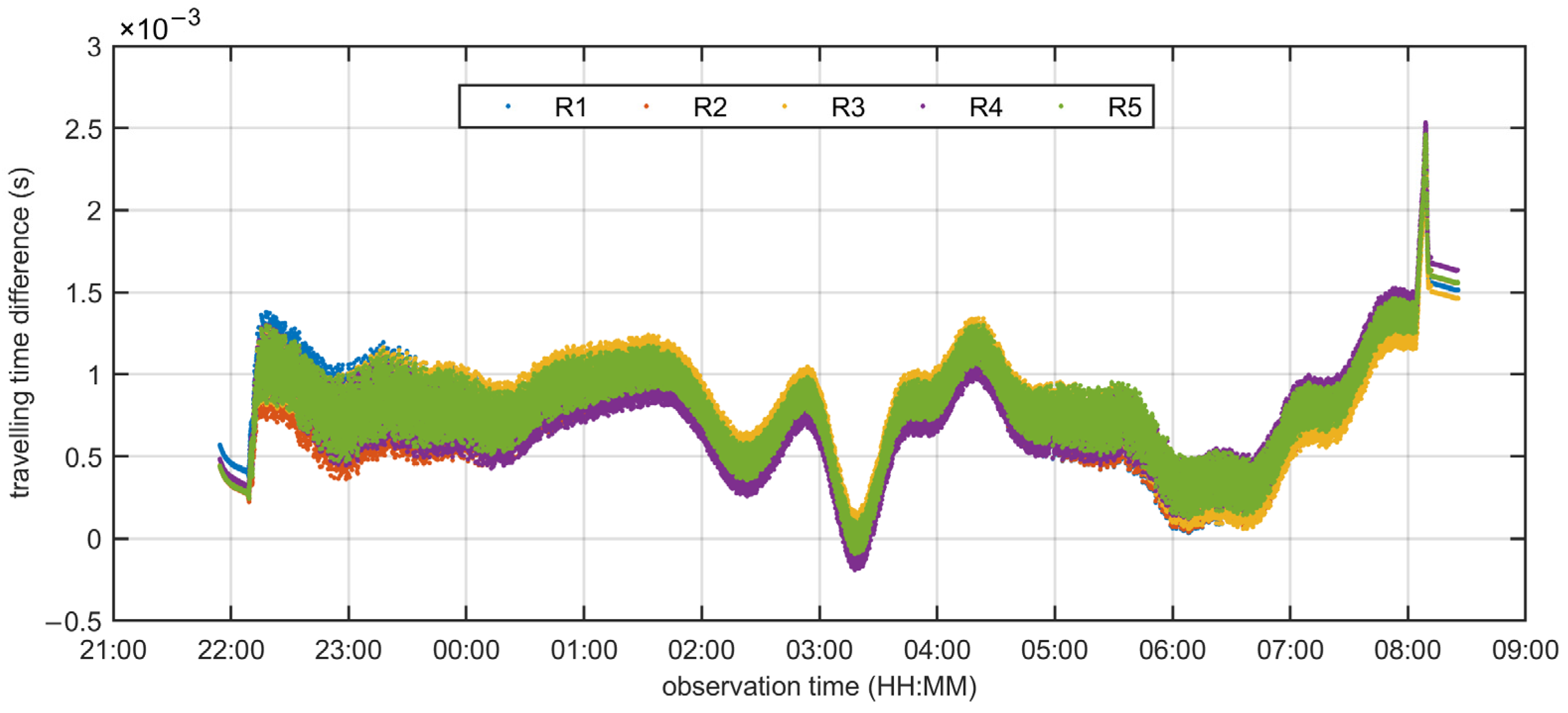
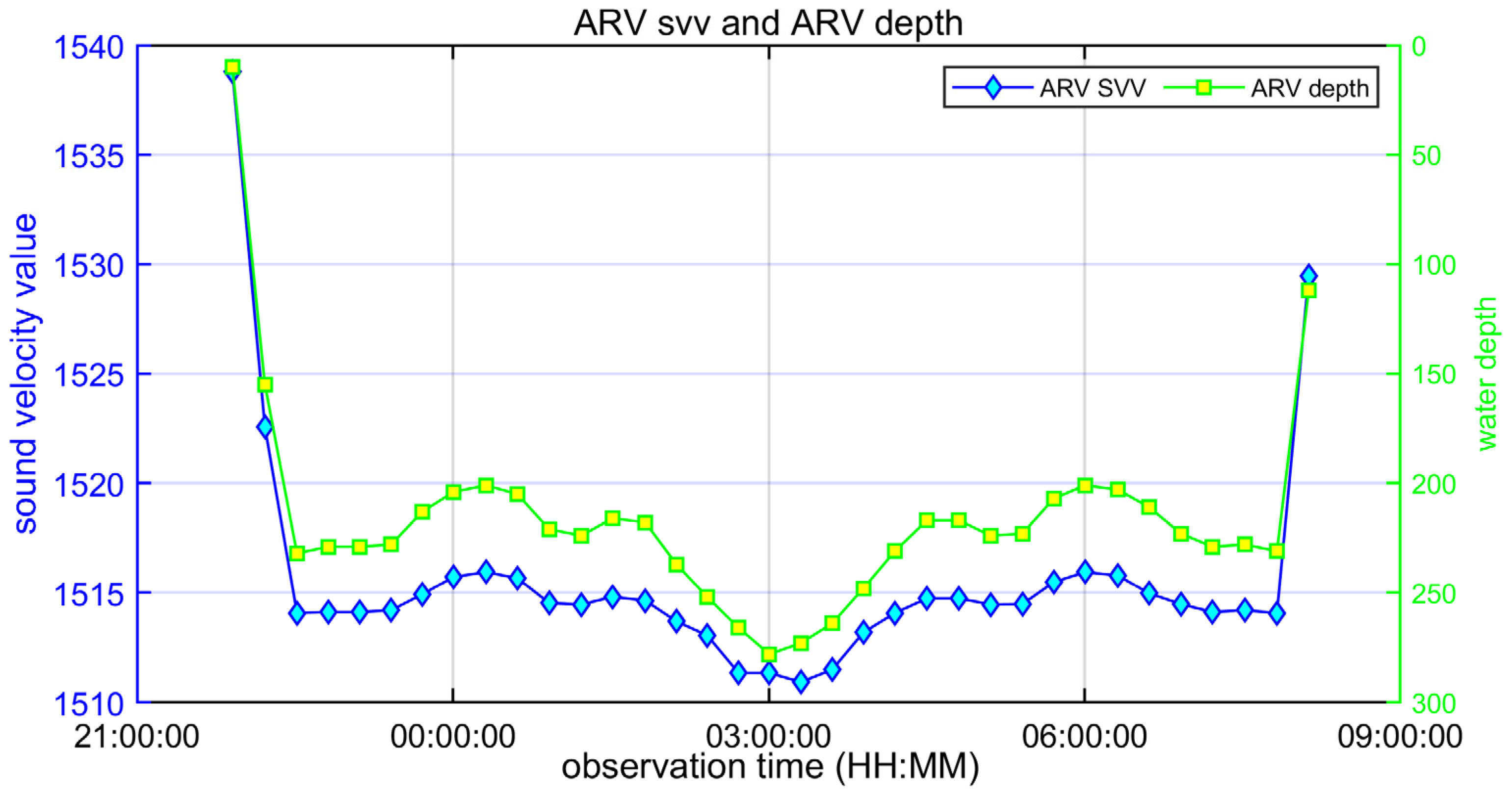
3. Results
- M1—the ARV’s position was calculated by using the constant sound speed value method.
- M2—the ARV’s position was calculated by using the typical correction method based on fixed SVP.
- M3—the ARV’s position was calculated by using the proposed two-step sound speed correction method.
4. Conclusions
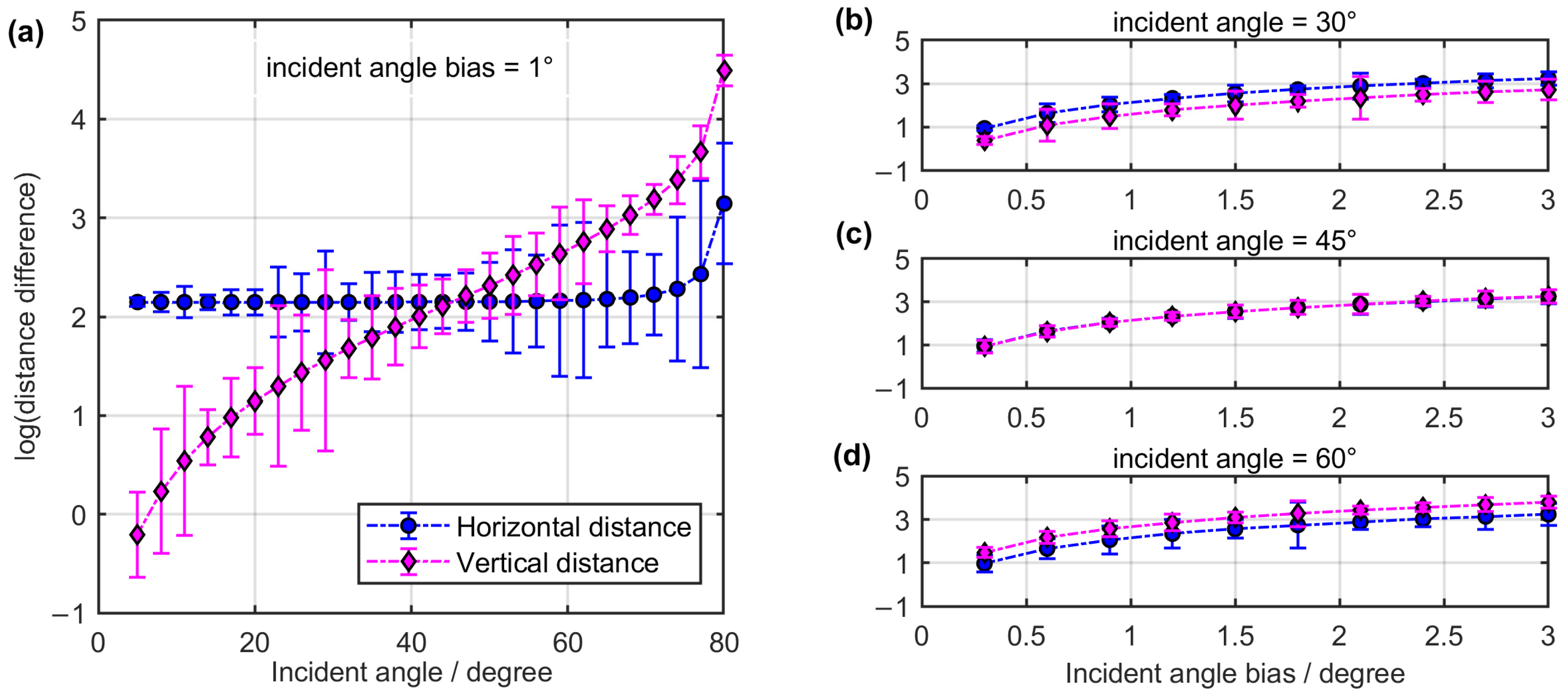
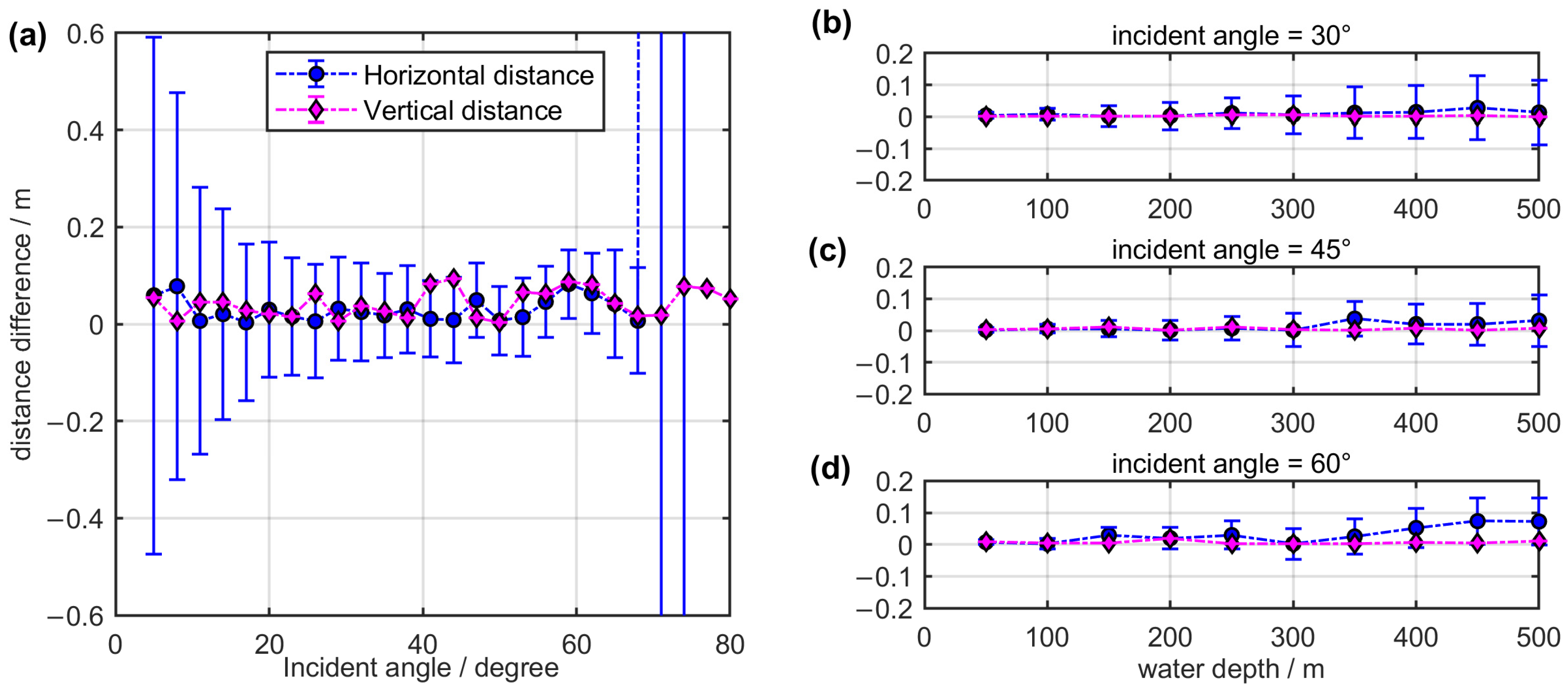
- The difference in horizontal distance between the geometric path and the curvature route is more susceptible to the incident angle bias than to the depth bias when using the direct ray-tracing method for acoustic ray bending correction. Therefore, it is highly recommended to employ the depth-based direct ray-tracing strategy;
- SVPs corresponding to the main layers of the depth profile exhibit relatively stable temporal variations. The in-situ sound velocity measurements, which contain the temporal variation information, allow for dynamic adjustments to the fixed SVP;
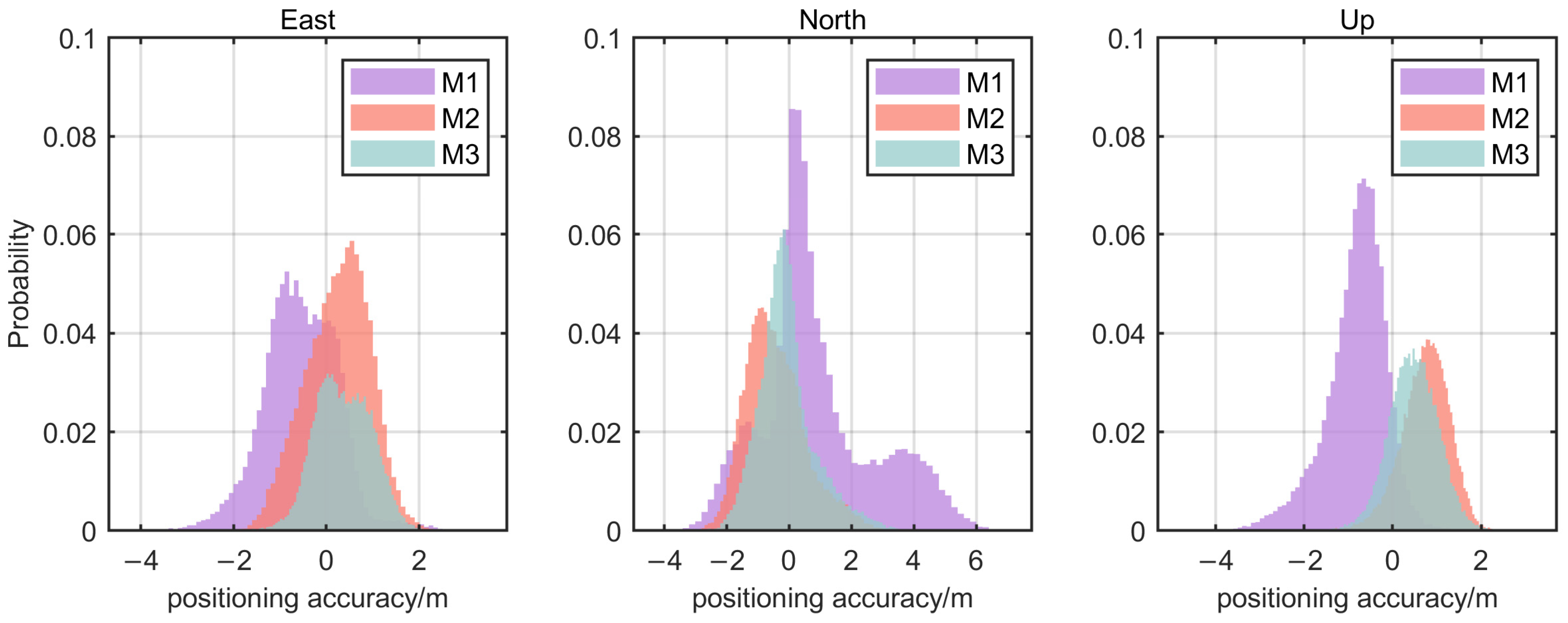
- When compared with the fixed-SVV method, the fixed-SVP method, which adopts the depth-based ray-tracing policy and significantly corrects horizontal deviation caused by the acoustic ray bending effect, leads to improved averaged positioning accuracy in the east, north, and up directions by 22%, 40%, and 7%, respectively;
- Additionally, the two-step resilient-SVP method, which further corrects SVP timing-variant errors, demonstrates improved averaged positioning accuracy in the east, north, and up directions by 8%, 21%, and 26%, respectively, when compared to the fixed-SVP method; this indicates that the two-step resilient-SVP method enhances the adaptability of sound speed observations and shows better performance in real-time USBL positioning.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Urick, R.J. Principles of Underwater Sound, 3rd ed.; Peninsula Pub: Baileys Harbor, WI, USA, 1996. [Google Scholar]
- Kussat, N.H.; Chadwell, C.D.; Zimmerman, R. Absolute positioning of an autonomous underwater vehicle using GPS and acoustic measurements. IEEE J. Ocean. Eng. 2005, 30, 153–164. [Google Scholar] [CrossRef]
- Maki, T.; Matsuda, T.; Sakamaki, T.; Ura, T.; Kojima, J. Navigation method for underwater vehicles based on mutual acoustical positioning with a single seafloor station. IEEE J. Ocean. Eng. 2013, 38, 167–177. [Google Scholar] [CrossRef]
- Chen, H.; Wang, C. Accuracy assessment of GPS/Acoustic positioning using a Seafloor Acoustic Transponder System. Ocean Eng. 2011, 38, 1472–1479. [Google Scholar] [CrossRef]
- Reis, J.; Morgado, M.; Batista, P.; Oliveira, P.; Silvestre, C. Design and experimental validation of a USBL underwater acoustic positioning system. Sensors 2016, 16, 1491. [Google Scholar] [CrossRef]
- Graça, P.A.A. Relative Acoustic Localization with USBL (Ultra-Short Baseline). Master’s Thesis, University of Porto, Porto, Portugal, 2020. [Google Scholar]
- Zhang, J.; Han, Y.; Zheng, C.; Sun, D. Underwater target localization using long baseline positioning system. Appl. Acoust. 2016, 111, 129–134. [Google Scholar] [CrossRef]
- Luo, Q.; Yan, X.; Wang, C.; Shao, Y.; Zhou, Z.; Li, J.; Hu, C.; Wang, C.; Ding, J. A SINS/DVL/USBL integrated navigation and positioning IoT system with multiple sources fusion and federated Kalman filter. J. Cloud Comput. 2022, 11, 18. [Google Scholar] [CrossRef]
- Liu, H.; Wang, Z.; Shan, R.; He, K.; Zhao, S. Research into the integrated navigation of a deep-sea towed vehicle with USBL/DVL and pressure gauge. Appl. Acoust. 2020, 159, 107052. [Google Scholar] [CrossRef]
- Vickery, K. Acoustic positioning systems: A practical overview of current systems. In Proceedings of the 1998 Workshop on Autonomous Underwater Vehicles, Cambidge, MA, USA, 21 August 1998; IEEE: Piscataway, NJ, USA, 1998. [Google Scholar]
- Tong, J.; Xu, X.; Zhang, T.; Li, Y.; Yao, Y.; Weng, C.; Hou, L.; Zhang, L. A misalignment angle error calibration method of underwater acoustic array in strapdown inertial navigation system/ultrashort baseline integrated navigation system based on single transponder mode. Rev. Sci. Instrum. 2019, 90, 085001. [Google Scholar] [CrossRef]
- Chen, H.-H. The estimation of angular misalignments for ultra short baseline navigation systems. Part II: Experimental results. J. Navig. 2013, 66, 773–787. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhang, T.; Xu, S.; Shin, H.-S.; Li, P.; Jin, B.; Zhang, L.; Weng, C.; Li, Y. A calibration method of USBL installation error based on attitude determination. IEEE Trans. Veh. Technol. 2020, 69, 8317–8328. [Google Scholar] [CrossRef]
- Zhao, S.; Wang, Z.; Nie, Z.; He, K.; Ding, N. Investigation on total adjustment of the transducer and seafloor transponder for GNSS/Acoustic precise underwater point positioning. Ocean Eng. 2021, 221, 108533. [Google Scholar] [CrossRef]
- Van de Voort, N. Inversion Methods of the Sound Velocity Profile for Improving the Accuracy of the USBL Positioning System. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 2022. [Google Scholar]
- Zhao, S.; Wang, Z.; He, K.; Ding, N. Investigation on underwater positioning stochastic model based on acoustic ray incidence angle. Appl. Ocean Res. 2018, 77, 69–77. [Google Scholar] [CrossRef]
- Sun, D.; Li, H.; Zheng, C.; Li, X. Sound velocity correction based on effective sound velocity for underwater acoustic positioning systems. Appl. Acoust. 2019, 151, 55–62. [Google Scholar] [CrossRef]
- Sakic, P.; Ballu, V.; Crawford, W.; Wöppelmann, G. Acoustic ray tracing comparisons in the context of geodetic precise off-shore positioning experiments. Mar. Geod. 2018, 41, 315–330. [Google Scholar] [CrossRef]
- Chadwell, C.; Sweeney, A. Acoustic Ray-Trace Equations for Seafloor Geodesy. Mar. Geod. 2010, 33, 164–186. [Google Scholar] [CrossRef]
- He, C.; Wang, Y.; Yu, W.; Song, L. Underwater target localization and synchronization for a distributed SIMO Sonar with an isogradient SSP and uncertainties in receiver locations. Sensors 2019, 19, 1976. [Google Scholar] [CrossRef]
- Ameer, P.M.; Jacob, L. Localization using ray tracing for underwater acoustic sensor networks. IEEE Commun. Lett. 2010, 14, 930–932. [Google Scholar] [CrossRef]
- Zhao, J.; Liu, J. Multibeam Bathymetry and Image Data Processing; Wuhan University Press: Wuhan, China, 2008. [Google Scholar]
- Rousselet, L.; Doglioli, A.M.; de Verneil, A.; Pietri, A.; Della Penna, A.; Berline, L.; Marrec, P.; Grégori, G.; Thyssen, M.; Carlotti, F.; et al. Vertical motions and their effects on a biogeochemical tracer in a cyclonic structure finely observed in the Ligurian Sea. J. Geophys. Res. Ocean. 2019, 124, 3561–3574. [Google Scholar] [CrossRef]
- Church, I.W. Multibeam sonar ray-tracing uncertainty evaluation from a hydrodynamic model in a highly stratified estuary. Mar. Geod. 2020, 43, 359–375. [Google Scholar] [CrossRef]
- Shao, M.; Ortiz-Suslow, D.G.; Haus, B.K.; Lund, B.; Williams, N.J.; Özgökmen, T.M.; Laxague, N.J.; Horstmann, J.; Klymak, J.M.J.J.O.G.R.O. The variability of winds and fluxes observed near submesoscale fronts. J. Geophys. Res. Ocean. 2019, 124, 7756–7780. [Google Scholar] [CrossRef]
- Watanabe, S.; Ishikawa, T.; Yokota, Y.; Nakamura, Y. GARPOS: Analysis software for the GNSS-A seafloor positioning with simultaneous estimation of sound speed structure. Front. Earth Sci. 2020, 8, 597532. [Google Scholar] [CrossRef]
- Yokota, Y.; Ishikawa, T.; Watanabe, S. Gradient field of undersea sound speed structure extracted from the GNSS-A oceanography. Mar. Geophys. Res. 2019, 40, 493–504. [Google Scholar] [CrossRef]
- Honsho, C.; Kido, M.; Tomita, F.; Uchida, N. Offshore postseismic deformation of the 2011 Tohoku earthquake revisited: Application of an improved GPS-acoustic positioning method considering horizontal gradient of sound speed structure. J. Geophys. Res. Solid Earth 2019, 124, 5990–6009. [Google Scholar] [CrossRef]
- Yang, Y.; Qin, X. Resilient observation models for seafloor geodetic positioning. J. Geod. 2021, 95, 79. [Google Scholar] [CrossRef]
- Xu, P.; Ando, M.; Tadokoro, K. Precise, three-dimensional seafloor geodetic deformation measurements using difference techniques. Earth Planets Space 2005, 57, 795–808. [Google Scholar] [CrossRef]
- Xue, S.; Yang, Y.; Yang, W. Single-differenced models for GNSS-acoustic seafloor point positioning. J. Geod. 2022, 96, 38. [Google Scholar] [CrossRef]
- Wang, Z.; Zhao, S.; Ji, S.; He, K.; Nie, Z.; Liu, H.; Shan, R. Real-time stochastic model for precise underwater positioning. Appl. Acoust. 2019, 150, 36–43. [Google Scholar] [CrossRef]
- Zhao, S.; Wang, Z.; He, K.; Nie, Z.; Liu, H.; Ding, N. Investigation on stochastic model refinement for precise underwater positioning. IEEE J. Ocean. Eng. 2020, 45, 1482–1496. [Google Scholar] [CrossRef]
- Yokota, Y.; Kaneda, M.; Hashimoto, T.; Yamaura, S.; Kouno, K.; Hirakawa, Y. Experimental verification of seafloor crustal deformation observations by UAV-based GNSS-A. Sci. Rep. 2023, 13, 4105. [Google Scholar] [CrossRef]
- Tadokoro, K.; Kinugasa, N.; Kato, T.; Terada, Y.; Matsuhiro, K. A Marine-buoy-mounted system for continuous and real-time measurment of seafloor crustal deformation. Front. Earth Sci. 2020, 8, 123. [Google Scholar] [CrossRef]
- Iinuma, T.; Kido, M.; Ohta, Y.; Fukuda, T.; Tomita, F.; Ueki, I. GNSS-Acoustic observations of seafloor crustal deformation using a wave glider. Front. Earth Sci. 2021, 9, 87. [Google Scholar] [CrossRef]
- Yli-Hietanen, J.; Kalliojarvi, K.; Astola, J. Low-complexity angle of arrival estimation of wideband signals using small arrays. In Proceedings of the 8th Workshop on Statistical Signal and Array Processing, Corfu, Greece, 24–26 June 1996; IEEE: Piscataway, NJ, USA, 1996. [Google Scholar]
- iXblue Corp. Homepage. Available online: https://www.ixblue.com/ (accessed on 17 October 2023).
- Konsberg Corp. Homepage. Available online: https://www.kongsberg.com/ (accessed on 17 October 2023).
- Sakic, P.; Chupin, C.; Ballu, V.; Coulombier, T.; Morvan, P.-Y.; Urvoas, P.; Beauverger, M.; Royer, J.-Y. Geodetic seafloor positioning using an unmanned surface vehicle—Contribution of direction-of-arrival observations. Front. Earth Sci. 2021, 9, 636156. [Google Scholar] [CrossRef]
- Liu, H. Research on the Underwater Vehicle Integrated Navigation Based on Bayesian Filter[D]; Chinese University of Petroleum: Beijing, China, 2020. [Google Scholar]
- Tong, J.; Xu, X.; Zhao, T.; Zhang, T.; Zhang, L.; Li, Y. Study on installation error analysis and calibration of acoustic transceiver array based on SINS/USBL integrated system. IEEE Access 2018, 6, 66923–66939. [Google Scholar]
- Bianco, M.; Gerstoft, P. Dictionary learning of sound speed profiles. J. Acoust. Soc. Am. 2017, 141, 1749–1758. [Google Scholar] [CrossRef]
- Yokota, Y.; Ishikawa, T.; Watanabe, S. Seafloor crustal deformation data along the subduction zones around Japan obtained by GNSS-A observations. Sci. Data 2018, 5, 180182. [Google Scholar] [CrossRef]



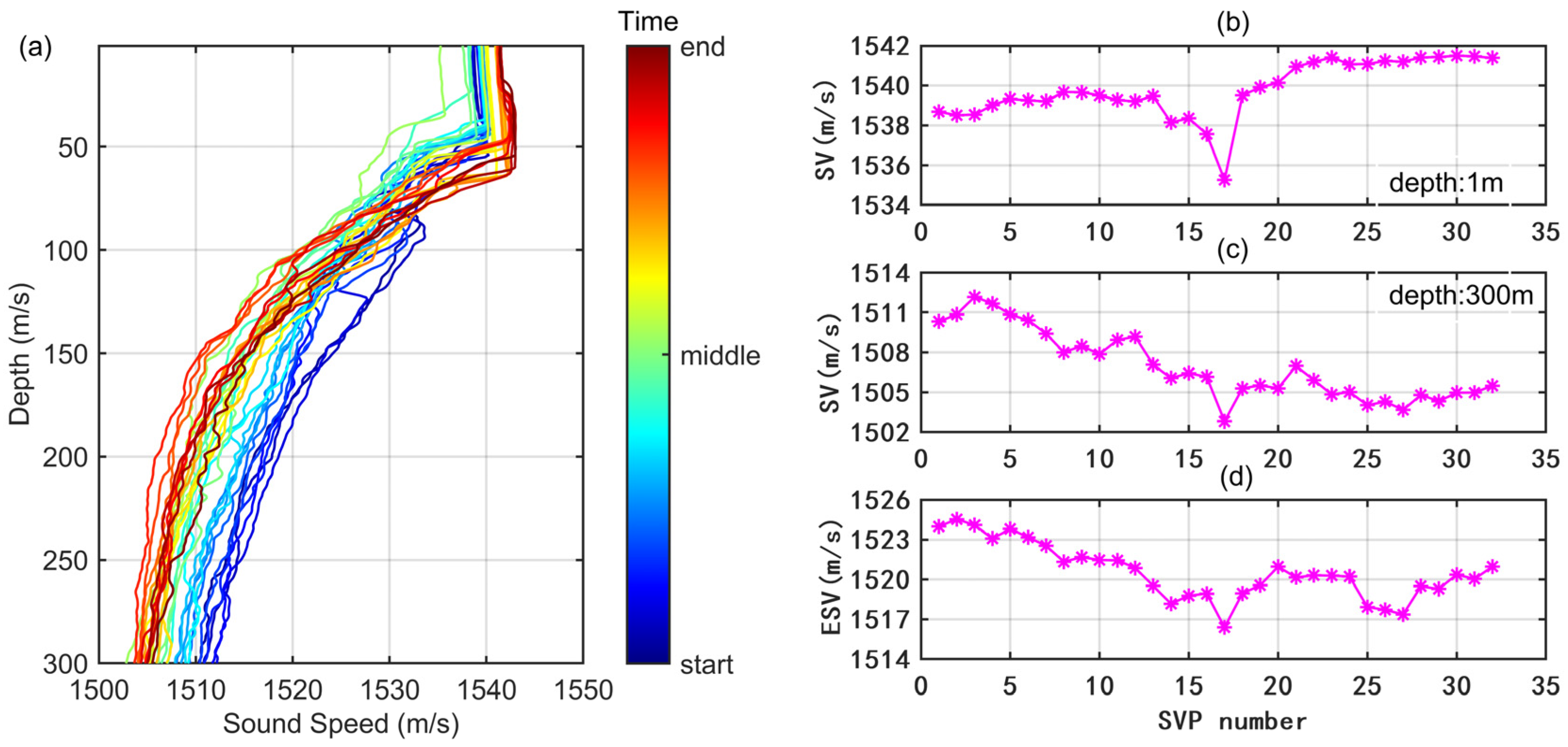
| No. | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|---|
| depth (m) | 5 | 10 | 20 | 50 | 100 | 150 | 200 | 250 | 300 |
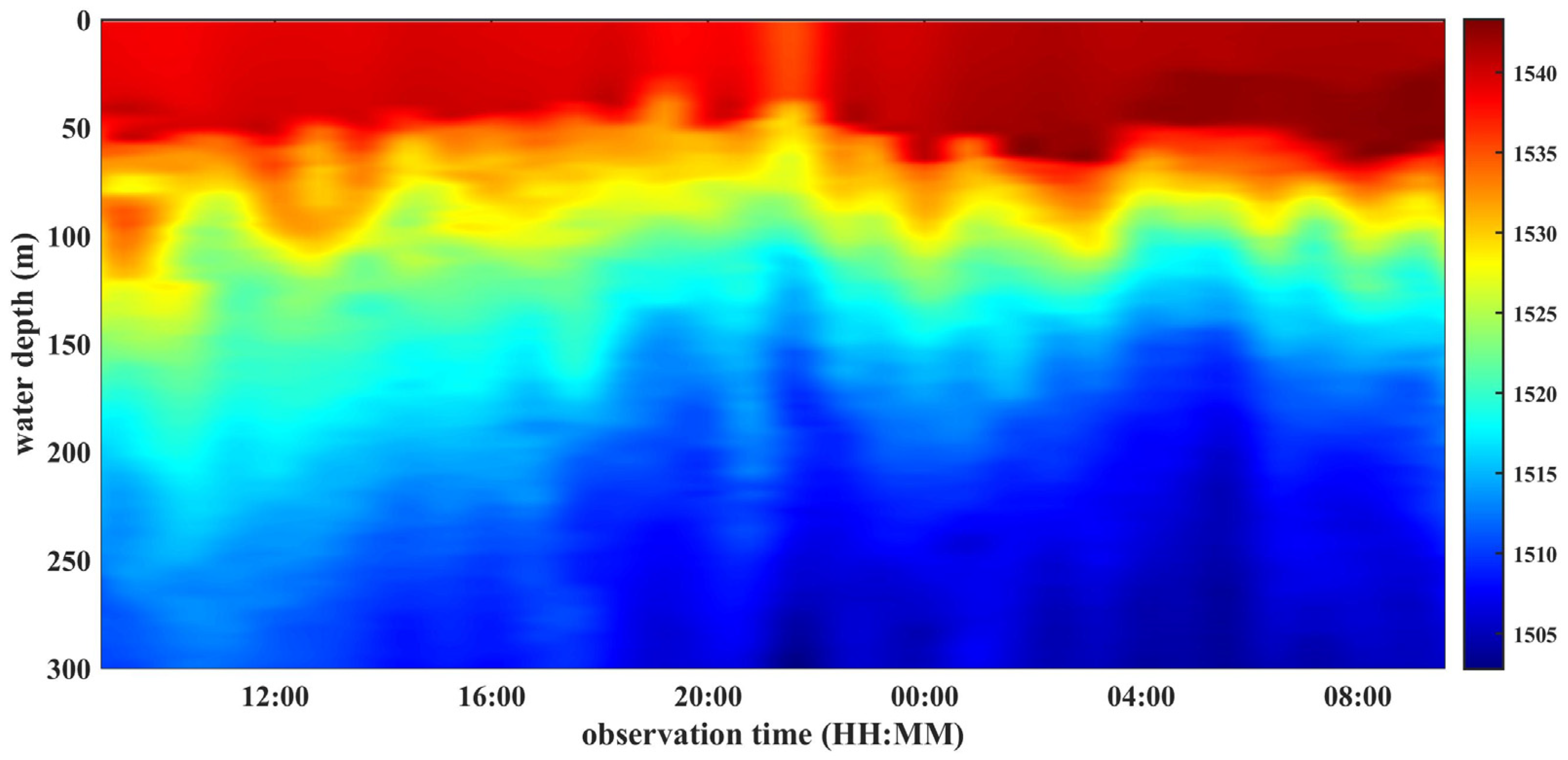
| correlation | 0.9998 | 0.9999 | 0.9971 | 0.8665 | 0.4235 | 0.5382 | 0.6864 | 0.8401 | 0.8939 |
| Methods | Average | STD | ||||
|---|---|---|---|---|---|---|
| E | N | U | E | N | U | |
| M1 | 0.779 | 1.467 | 0.866 | 0.806 | 1.829 | 0.689 |
| M2 | 0.606 | 0.879 | 0.803 | 0.689 | 0.974 | 0.547 |
| M3 | 0.559 | 0.691 | 0.594 | 0.600 | 0.913 | 0.558 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, S.; Liu, H.; Xue, S.; Wang, Z.; Xiao, Z. Two-Step Correction Based on In-Situ Sound Speed Measurements for USBL Precise Real-Time Positioning. Remote Sens. 2023, 15, 5046. https://doi.org/10.3390/rs15205046
Zhao S, Liu H, Xue S, Wang Z, Xiao Z. Two-Step Correction Based on In-Situ Sound Speed Measurements for USBL Precise Real-Time Positioning. Remote Sensing. 2023; 15(20):5046. https://doi.org/10.3390/rs15205046
Chicago/Turabian StyleZhao, Shuang, Huimin Liu, Shuqiang Xue, Zhenjie Wang, and Zhen Xiao. 2023. "Two-Step Correction Based on In-Situ Sound Speed Measurements for USBL Precise Real-Time Positioning" Remote Sensing 15, no. 20: 5046. https://doi.org/10.3390/rs15205046
APA StyleZhao, S., Liu, H., Xue, S., Wang, Z., & Xiao, Z. (2023). Two-Step Correction Based on In-Situ Sound Speed Measurements for USBL Precise Real-Time Positioning. Remote Sensing, 15(20), 5046. https://doi.org/10.3390/rs15205046






