PolSAR Image Classification by Introducing POA and HA Variances
Abstract
:1. Introduction
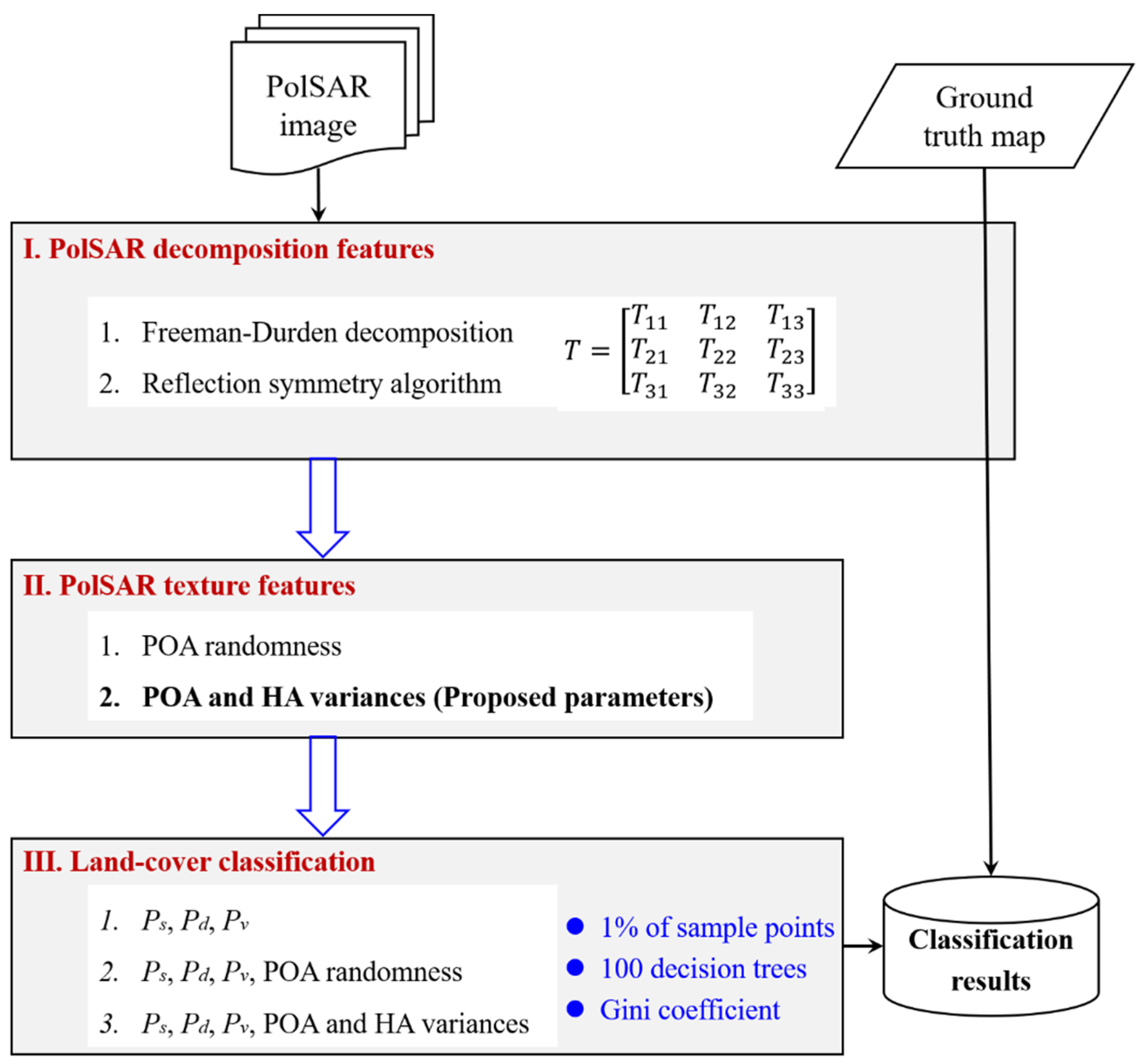
2. Methodology
2.1. The Freeman–Durden Decomposition and Reflection Symmetry Approximation Methods
2.2. POA Variance and HA Variance
- (1)
- Compute the POA and HA for the entire PolSAR image:where and denote real and imagenary parts of . Then, labels are assigned from 1 to 10 based on the values of the POA and HA, as illustrated in Figure 1a. In this study, the decision to divide the orientation angles into 10 parts is driven by the objective of preventing targets with similar orientations from being classified into different categories.
- (2)
- To calculate the POA variance and HA variance for each pixel, the following formula can be used:where and represent the label of the POA and the label of the HA, respectively. and are the labels of the central pixel. denotes the number of window sizes used to calculate the variance, as illustrated by Figure 1b.
2.3. Classification Method
3. Study Areas and Datasets
4. Experimental Results and Analysis
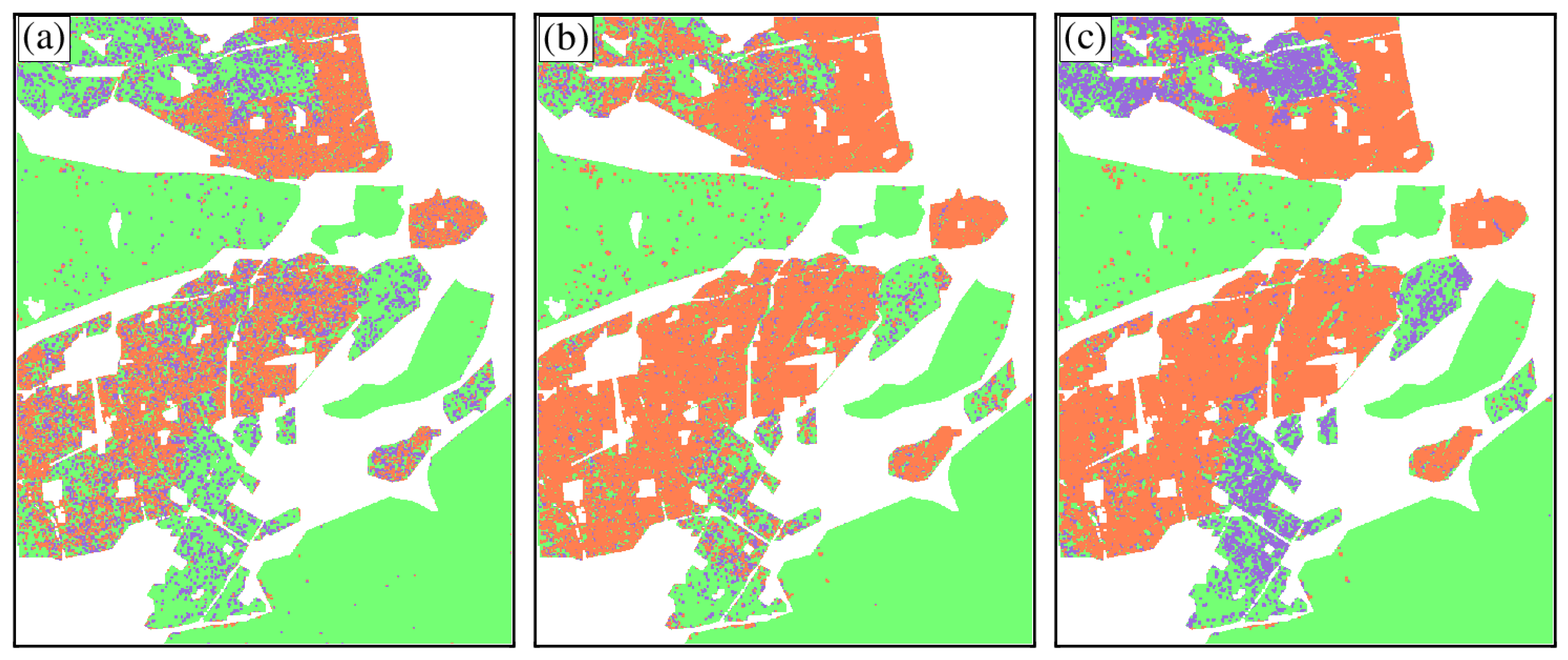
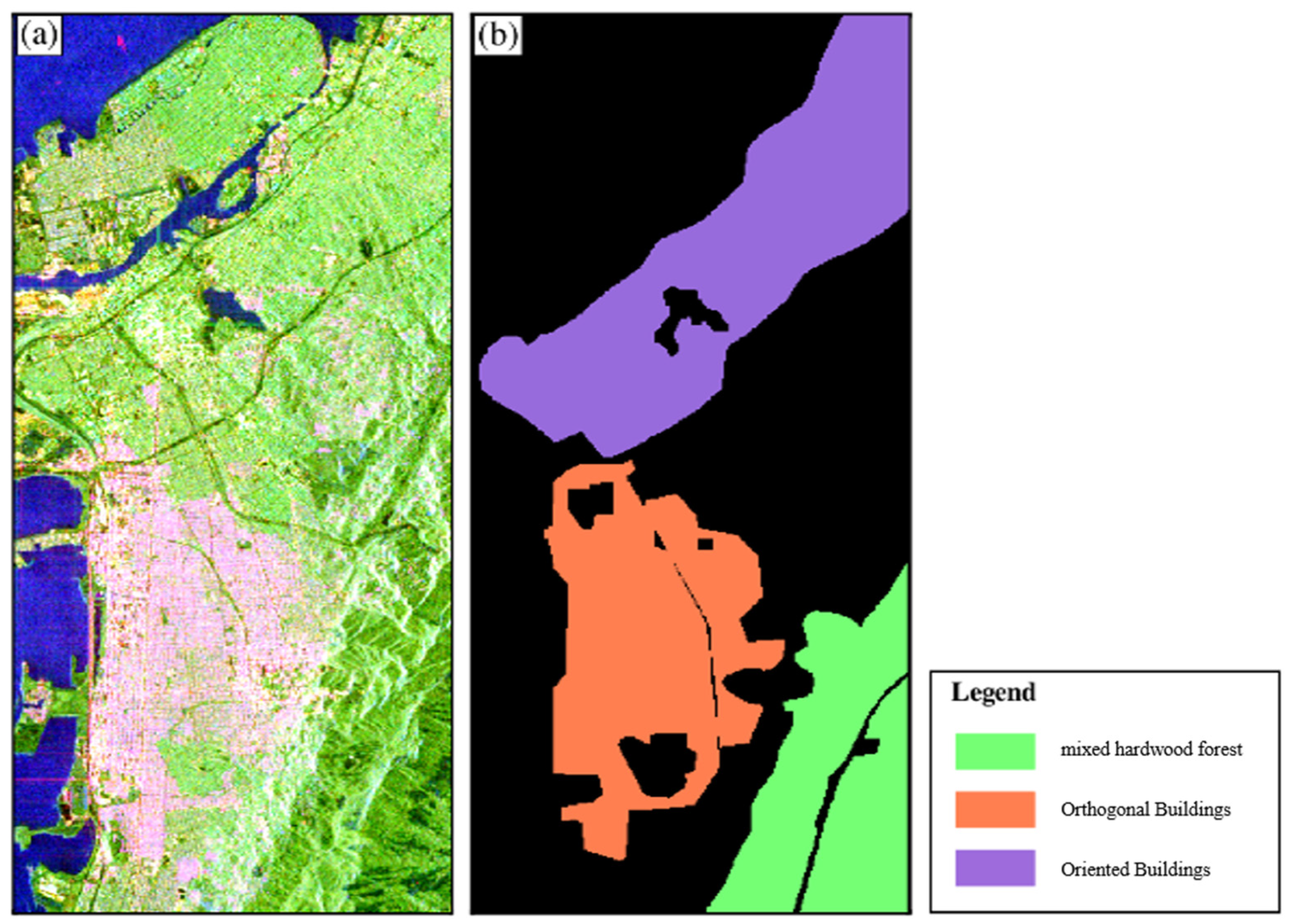
4.1. San Fernando Valley Test Site
4.2. Oakland Test Site
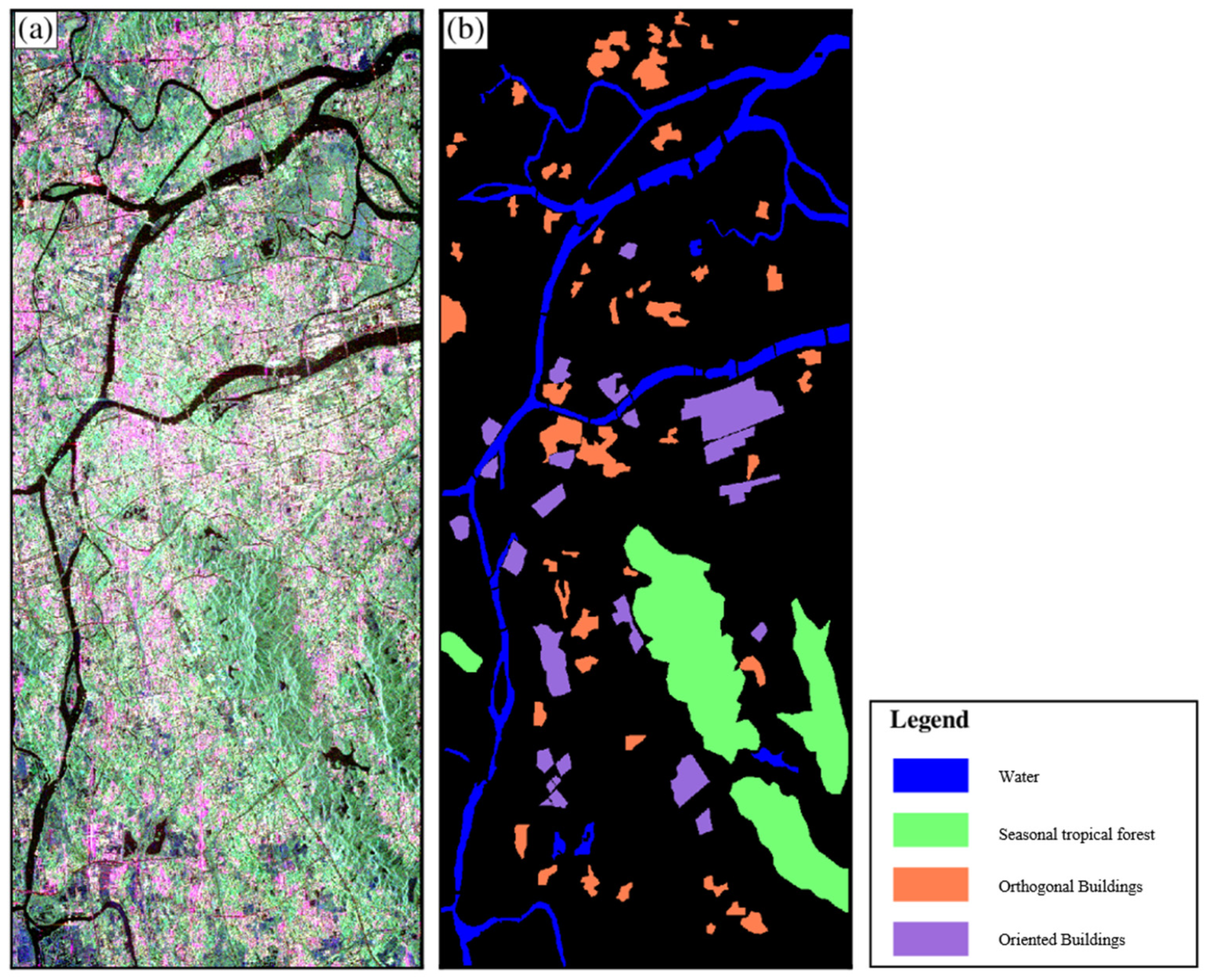
4.3. Guangzhou Test Site
5. Discussion
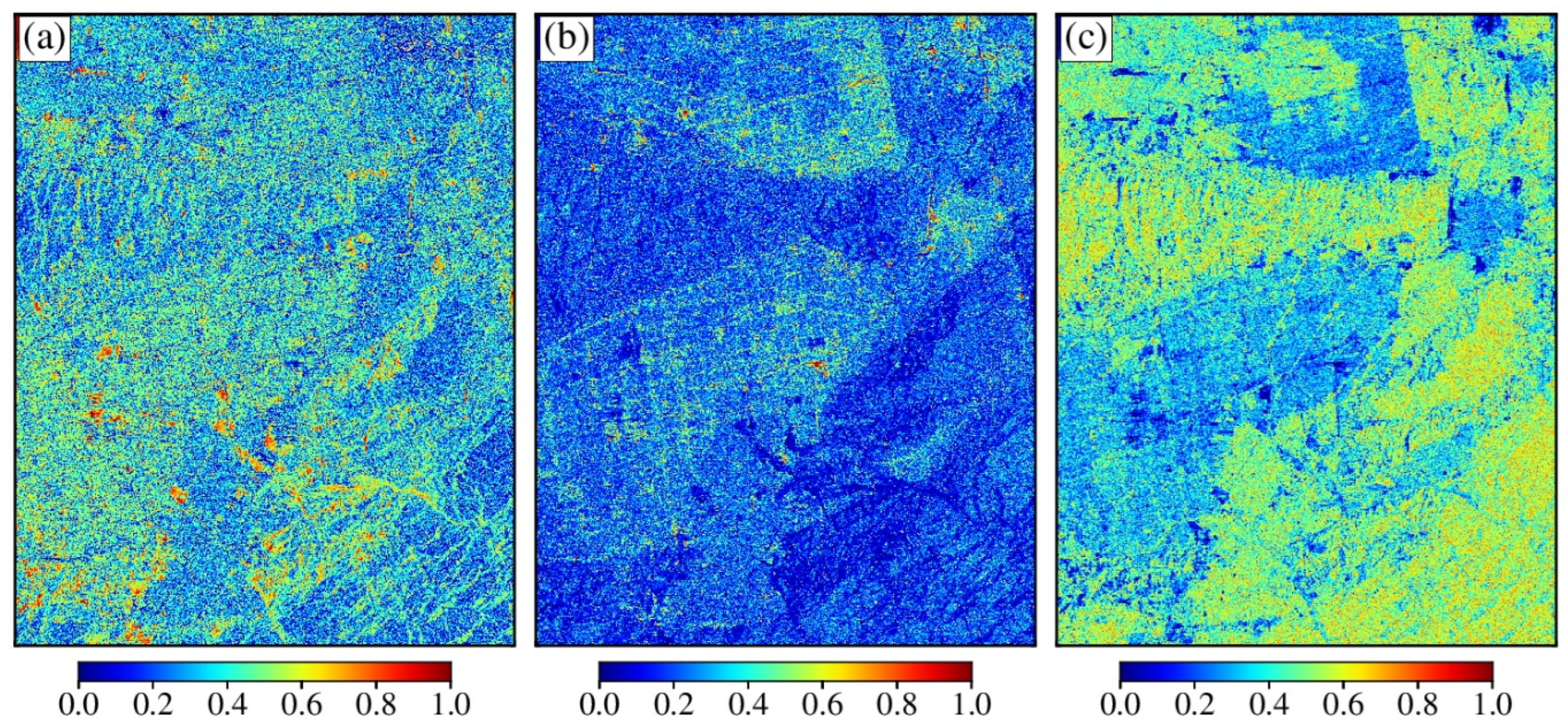
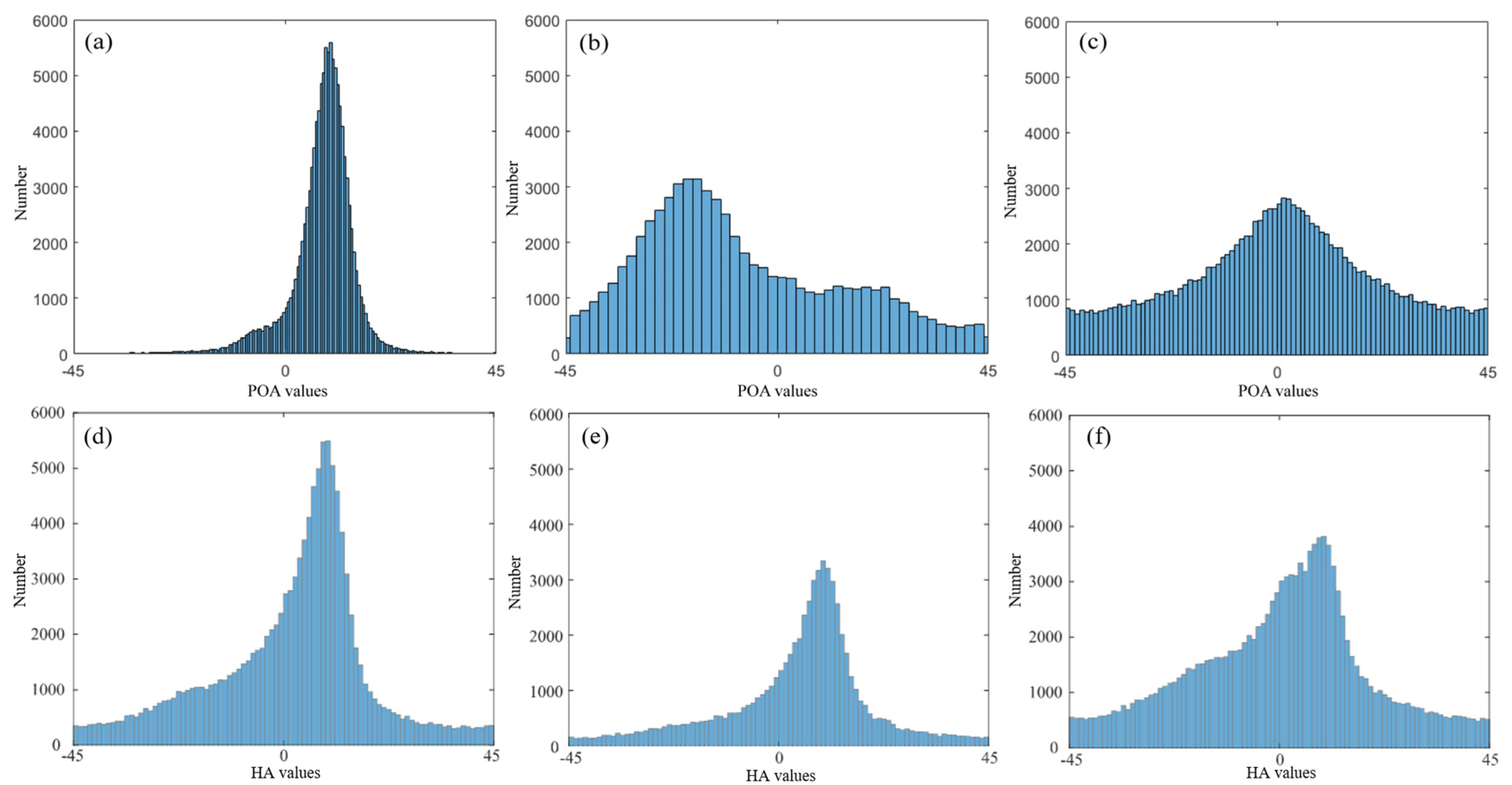
5.1. The POA and HA Characteristics of Ground Targets
5.2. The Effect of Window Size on Classification Results
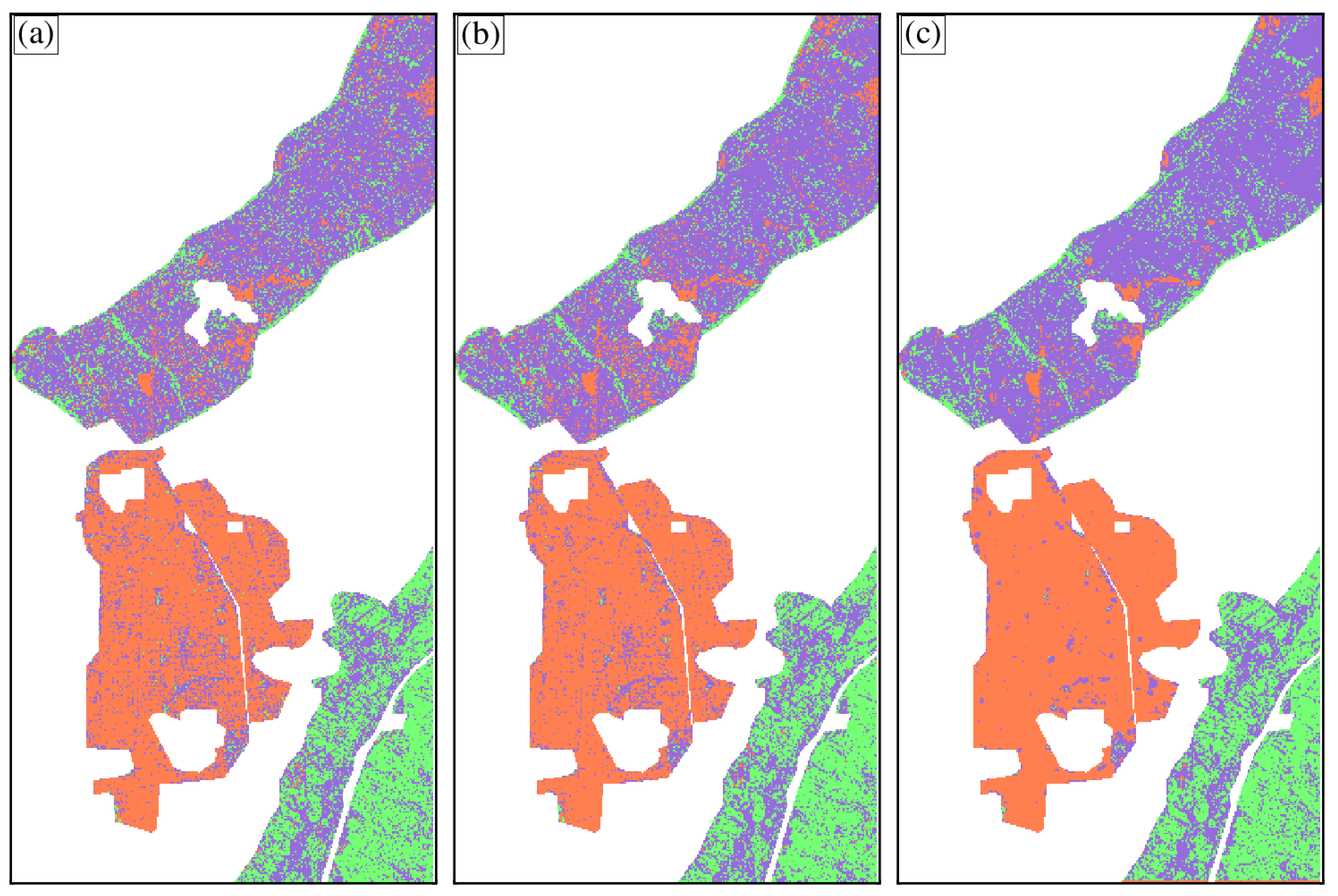
5.3. Complementary Properties of Scattering Power and Proposed Parameters
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Turkar, V.; Checker, J.; De, S.; Singh, G. Impact of G4U and 7-component Target Decomposition on PolSAR Image Semantic Segmentation. Adv. Space. Res. 2022, 70, 3798–3810. [Google Scholar]
- De, S.; Bruzzone, L.; Bhattacharya, A.; Bovolo, F.; Chaudhuri, S. A novel technique based on deep learning and a synthetic target database for classification of urban areas in PolSAR data. IEEE J. Sel. Topics Appl. Earth Observ. Remote Sens. 2018, 11, 154–170. [Google Scholar] [CrossRef]
- Fan, H.; Quan, S.; Dai, D.; Wang, X.; Xiao, S. Refined model-based and feature-driven extraction of buildings from PolSAR images. Remote Sens. 2019, 11, 1379. [Google Scholar] [CrossRef]
- Chen, S.W.; Wang, X.S.; Xiao, S.P. Urban Damage Level Mapping Based on Co-Polarization Coherence Pattern Using Multitemporal Polarimetric SAR Data. IEEE J. Sel. Topics Appl. Earth Observ. Remote Sens. 2018, 11, 2657–2667. [Google Scholar] [CrossRef]
- Wang, H.; Yang, H.; Huang, Y.; Wu, L.; Guo, Z.; Li, N. Classification of Land Cover in Complex Terrain Using Gaofen-3 SAR Ascending and Descending Orbit Data. Remote Sens. 2023, 15, 2177. [Google Scholar] [CrossRef]
- van Zyl, J.J. Application of Cloude’s target decomposition theorem to polarimetric imaging radar data. Proc. SPIE Int. Soc. Opt. Eng. 1993, 1748, 184–192. [Google Scholar]
- Cloude, S.R.; Pottier, E. A review of target decomposition theorems in radar polarimetry. IEEE Trans. Geosci. Remote Sens. 1996, 34, 498–518. [Google Scholar] [CrossRef]
- Qi, Z.; Yeh, A.G.-O.; Li, X.; Lin, Z. A novel algorithm for land use and land cover classification using RADARSAT-2 polarimetric SAR data. Remote Sens. Environ. 2012, 118, 21–39. [Google Scholar] [CrossRef]
- Quan, S.; Xiong, B.; Xiang, D.; Kuang, G. Derivation of the Orientation Parameters in Built-Up Areas: With Application to Model-Based Decomposition. IEEE Trans. Geosci. Remote Sens. 2018, 56, 4714–4730. [Google Scholar] [CrossRef]
- Liu, W.; Yang, J.; Li, P.; Han, Y.; Zhao, J.; Shi, H. A Novel Object-Based Supervised Classification Method with Active Learning and Random Forest for PolSAR Imagery. Remote Sens. 2018, 10, 1092. [Google Scholar] [CrossRef]
- De Borba, A.A.; Muhuri, A.; Marengoni, M.; Frery, A.C. Feature Selection for Edge Detection in PolSAR Images. Remote Sens. 2023, 15, 2479. [Google Scholar] [CrossRef]
- Bouhlel, N.; Akbari, V.; Méric, S. Change Detection in Multilook Polarimetric SAR Imagery with Determinant Ratio Test Statistic. IEEE Trans. Geosci. Remote Sens. 2022, 60, 1–15. [Google Scholar] [CrossRef]
- Xiang, D.L.; Ban, Y.; Su, Y. Model-based decomposition with cross scattering for polarimetric SAR urban areas. IEEE Geosci. Remote Sens. Lett. 2015, 12, 2496–2500. [Google Scholar] [CrossRef]
- Quan, S.; Zhang, T.; Wang, W.; Kuang, G.; Wang, X.; Zeng, B. Exploring Fine Polarimetric Decomposition Technique for Built-up Area Monitoring. IEEE Trans. Geosci. Remote Sens. 2023, 61, 5204719. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, L.; Zou, B.; Gu, G. Polarimetric SAR Decomposition Method Based on Modified Rotational Dihedral Model. Remote Sens. 2023, 15, 101. [Google Scholar] [CrossRef]
- Chen, S.; Li, Y.; Wang, X.; Xiao, S.; Sato, M. Modeling and interpretation of scattering mechanisms in polarimetric synthetic aperture radar: Advances and perspectives. IEEE Signal Process. Mag. 2014, 31, 79–89. [Google Scholar] [CrossRef]
- Krogager, E. New decomposition of the radar target scattering matrix. Electron. Lett. 1990, 26, 1525–1527. [Google Scholar] [CrossRef]
- Cameron, W.L.; Youssef, N.N.; Leung, L.K. Simulated polarimetric signatures of primitive geometrical shapes. IEEE Trans. Geosci. Remote Sens. 1996, 34, 793–803. [Google Scholar] [CrossRef]
- Touzi, R. Target scattering decomposition in terms of roll-invariant target parameters. IEEE Trans. Geosci. Remote Sens. 2007, 45, 73–84. [Google Scholar] [CrossRef]
- Freeman, A.; Durden, S.L. A three-component scattering model for polarimetric SAR data. IEEE Trans. Geosci. Remote Sens. 1998, 36, 963–973. [Google Scholar] [CrossRef]
- Zhang, L.M.; Zou, B.; Cai, H.J. Multiple-component scattering model for polarimetric SAR image decomposition. IEEE Geosci. Remote Sens. Lett. 2008, 5, 603–607. [Google Scholar] [CrossRef]
- Chen, S.; Wang, X.S.; Xiao, S.P.; Sato, M. General Polarimetric Model-Based Decomposition for Coherency Matrix. IEEE Trans. Geosci. Remote Sens. 2014, 52, 1843–1855. [Google Scholar] [CrossRef]
- Sato, A.; Yamaguchi, Y.; Singh, G.; Park, S.E. Four-component scattering power decomposition with extended volume scattering model. IEEE Geosci. Remote Sens. Lett. 2012, 9, 166–170. [Google Scholar] [CrossRef]
- Lee, J.S.; Pottier, E. Polarimetric Radar Imaging: From Basics to Applications; CRC Press: Boca Raton, FL, USA, 2009. [Google Scholar]
- Li, D.; Zhang, Y. Adaptive Model-Based Classification of PolSAR Data. IEEE Trans. Geosci. Remote Sens. 2018, 56, 6940–6955. [Google Scholar] [CrossRef]
- Xiang, D.; Tang, T.; Ban, Y.; Su, Y.; Kuang, G. Unsupervised polarimetric SAR urban area classification based on model-based decomposition with cross scattering. ISPRS J. Photogramm. Remote Sens. 2016, 116, 86–100. [Google Scholar] [CrossRef]
- Han, W.; Fu, H.; Zhu, J.; Zhang, S.; Xie, Q.; Hu, J. A Polarimetric Projection-Based Scattering Characteristics Extraction Tool and Its Application to PolSAR Image Classification. ISPRS J. Photogramm. Remote Sens. 2023, 20, 314–333. [Google Scholar] [CrossRef]
- Lee, J.S.; Grunes, M.R.; Ainsworth, T.L.; Du, L.J.; Schuler, D.L.; Cloude, S.R. Unsupervised classification using polarimetric decomposition and the complex wishart classifier. IEEE Trans. Geosci. Remote Sens. 2002, 37, 2249–2258. [Google Scholar]
- Xie, Q.; Wang, J.; Liao, C.; Shang, J.; Lopez-Sanchez, J.M.; Fu, H.; Liu, X. On the Use of Neumann Decomposition for Crop Classification Using Multi-Temporal RADARSAT-2 Polarimetric SAR Data. Remote Sens. 2019, 11, 776. [Google Scholar] [CrossRef]
- Hariharan, S.; Mandal, D.; Tirodkar, S.; Kumar, V.; Bhattacharya, A.; Lopez-Sanchez, J.M. A Novel Phenology Based Feature Subset Selection Technique Using Random Forest for Multitemporal PolSAR Crop Classification. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2018, 11, 4244–4258. [Google Scholar] [CrossRef]
- Zhou, Y.; Wang, H.; Xu, F.; Jin, Y.Q. Polarimetric SAR Image Classification Using Deep Convolutional Neural Networks. IEEE Geosci. Remote Sens. Lett. 2016, 13, 1935–1939. [Google Scholar] [CrossRef]
- Yamaguchi, Y.; Moriyama, T.; Ishido, M.; Yamada, H. Four-component scattering model for polarimetric SAR image decomposition. IEEE Trans. Geosci. Remote Sens. 2005, 43, 1699–1706. [Google Scholar] [CrossRef]
- Singh, G.; Yamaguchi, Y. Model-based six-component scattering matrix power decomposition. IEEE Trans. Geosci. Remote Sens. 2018, 56, 5687–5704. [Google Scholar] [CrossRef]
- Singh, G.; Malik, R.; Mohanty, S. Seven-component scattering power decomposition of POLSAR coherency matrix. IEEE Trans. Geosci. Remote Sens. 2019, 57, 8371–8382. [Google Scholar] [CrossRef]
- An, W.T.; Lin, M. A reflection symmetry approximation of multilook polarimetric SAR data and its application to Freeman–Durden decomposition. IEEE Trans. Geosci. Remote Sens. 2019, 57, 3649–3660. [Google Scholar] [CrossRef]
- Yamaguchi, Y.; Sato, A.; Boerner, W.M. Four-component scattering power decomposition with rotation of coherency matrix. IEEE Trans. Geosci. Remote Sens. 2011, 49, 2251–2258. [Google Scholar] [CrossRef]
- Lee, J.S.; Ainsworth, T.L. The effect of orientation angle compensation on coherency matrix and polarimetric target decompositions. IEEE Trans. Geosci. Remote Sens. 2010, 49, 53–64. [Google Scholar] [CrossRef]
- Singh, G.; Yamaguchi, Y.; Park, S.E. General four-component scattering power decomposition with unitary transformation of coherency matrix. IEEE Trans. Geosci. Remote Sens. 2013, 51, 3014–3022. [Google Scholar] [CrossRef]
- Quan, S.N.; Xiong, B.L.; Xiang, D.L.; Zhao, L.J.; Zhang, S.Q.; Kuang, G.Y. Eigenvalue-based urban area extraction using polarimetric SAR data. IEEE J. Sel. Topics Appl. Earth Observ. Remote Sens. 2018, 11, 458–471. [Google Scholar] [CrossRef]
- Kajimoto, M.; Susaki, J. Urban-Area Extraction from Polarimetric SAR Images Using Polarization Orientation Angle. IEEE Geoscie. Remote Sens. Lett. 2013, 10, 337–341. [Google Scholar] [CrossRef]
- Pal, M. Random forest classifier for remote sensing classification. Int. J. Remote Sens. 2005, 26, 217–222. [Google Scholar] [CrossRef]
- Sonobe, R.; Tani, H.; Wang, X.; Kobayashi, N.; Shimamura, H. Random forest classification of crop type using multi-temporal TerraSAR-X dual-polarimetric data. Remote Sens. Lett. 2014, 5, 157–164. [Google Scholar] [CrossRef]
- Collins, M.J.; Allan, J.M. Modeling and simulation of SAR image texture. IEEE Trans. Geosci. Remote Sens. 2009, 47, 3530–3546. [Google Scholar] [CrossRef]
- Yu, H.; Jiang, H.; Liu, Z.; Zhou, S.; Yin, X. EDTRS: A Superpixel Generation Method for SAR Images Segmentation Based on Edge Detection and Texture Region Selection. Remote Sens. 2022, 14, 5589. [Google Scholar] [CrossRef]

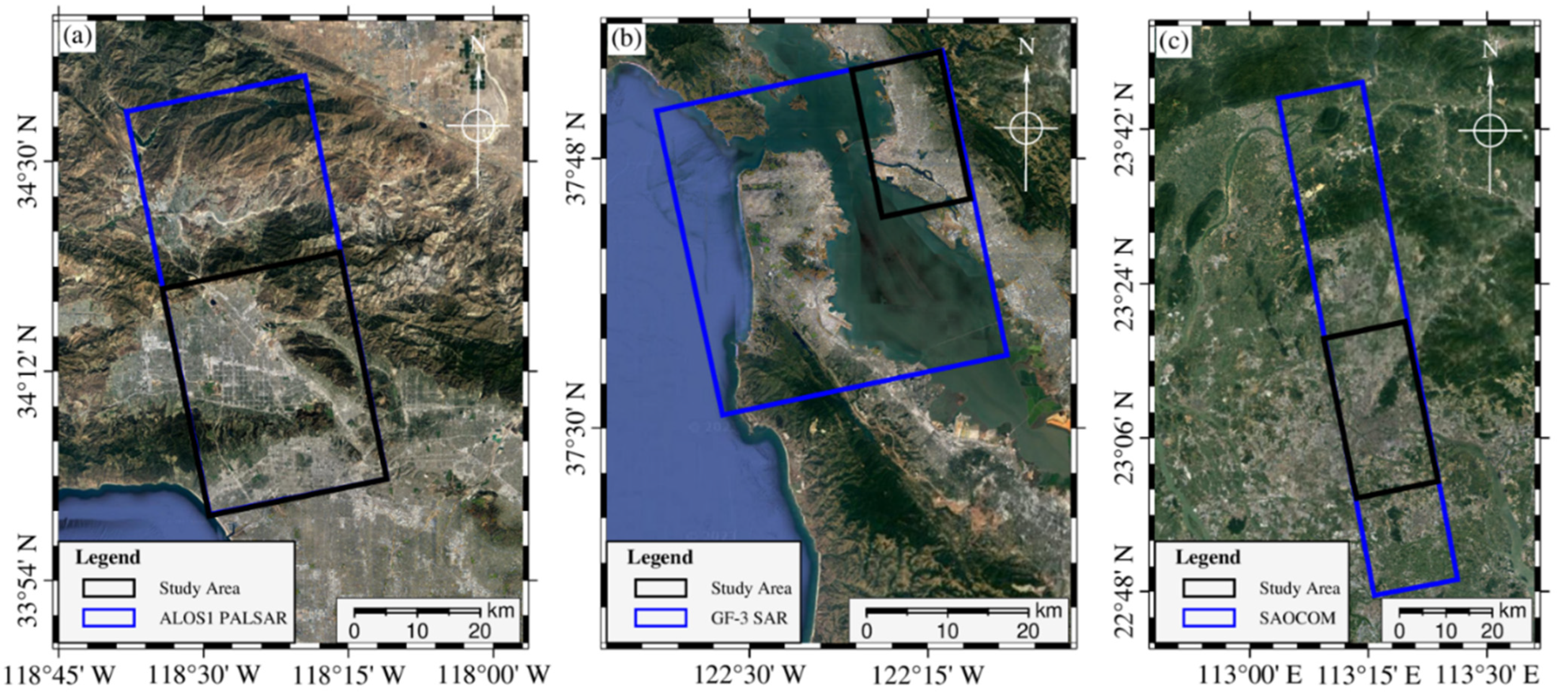







| Study Area | Dataset | Acquisition Date | Incidence Angle | Band | Pixel Spacing (Range × Azimuth) (m) | Image Size (km) |
|---|---|---|---|---|---|---|
| San Fernando Valley | ALOS1 | 8 June 2006 | 23.85° | L- | 9.37 × 3.55 | 42 × 23 |
| Oakland | GF-3 | 15 September 2017 | 21.22° | C- | 2.25 × 5.37 | 17 × 10 |
| Guangzhou | SAOCOM | 12 November 2022 | 22.35° | L- | 4.80 × 6.00 | 35 × 17 |
| Classification | Orthogonal Buildings | Oriented Buildings | Chaparral |
|---|---|---|---|
| Orthogonal buildings | 58.91% | 4.92% | 0.99% |
| Oriented buildings | 20.04% | 29.23% | 1.20% |
| Chaparral | 21.05% | 65.85% | 97.81% |
| Overall accuracy | 68.67% | Kappa coefficient | 0.50 |
| Classification | Orthogonal Buildings | Oriented Buildings | Chaparral |
|---|---|---|---|
| Orthogonal buildings | 83.88% | 6.57% | 0.88% |
| Oriented buildings | 10.62% | 38.88% | 1.30% |
| Chaparral | 5.50% | 54.55% | 97.82% |
| Overall accuracy | 80.20% | Kappa coefficient | 0.68 |
| Classification | Orthogonal Buildings | Oriented Buildings | Chaparral |
|---|---|---|---|
| Orthogonal buildings | 89.39% | 4.76% | 1.37% |
| Oriented buildings | 4.37% | 51.39% | 0.59% |
| Chaparral | 6.24% | 43.85% | 98.04% |
| Overall accuracy | 85.00% | Kappa coefficient | 0.76 |
| Classification | Orthogonal Buildings | Oriented Buildings | Mixed Hardwood Forest | Overall Accuracy | Kappa Coefficient |
|---|---|---|---|---|---|
| {Ps, Pd, and Pv} | 98.44% | 67.03% | 84.76% | 73.37% | 0.50 |
| {Ps, Pd, Pv, and POA randomness} | 98.60% | 69.29% | 85.00% | 75.78% | 0.55 |
| {Ps, Pd, Pv, POA, and HA variances} | 96.47% | 75.15% | 86.87% | 81.30% | 0.66 |
| Classification | Water | Orthogonal Buildings | Oriented Buildings | Seasonal Tropical Forest | Overall Accuracy | Kappa Coefficient |
|---|---|---|---|---|---|---|
| {Ps, Pd, and Pv} | 91.42% | 69.12% | 32.90% | 84.03% | 74.62% | 0.64 |
| {Ps, Pd, Pv, and POA randomness} | 91.65% | 81.50% | 25.88% | 87.36% | 76.94% | 0.68 |
| {Ps, Pd, Pv, POA, and HA variances} | 95.19% | 88.07% | 48.07% | 91.10% | 84.30% | 0.78 |
| Classification | Orthogonal Buildings | Oriented Buildings | Chaparral |
|---|---|---|---|
| Orthogonal buildings | 92.04% | 6.19% | 1.02% |
| Oriented buildings | 6.06% | 72.65% | 8.02% |
| Chaparral | 1.90% | 21.16% | 90.95% |
| Overall accuracy | 87.53% | Kappa coefficient | 0.81 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lan, Z.; Liu, Y.; He, J.; Hu, X. PolSAR Image Classification by Introducing POA and HA Variances. Remote Sens. 2023, 15, 4464. https://doi.org/10.3390/rs15184464
Lan Z, Liu Y, He J, Hu X. PolSAR Image Classification by Introducing POA and HA Variances. Remote Sensing. 2023; 15(18):4464. https://doi.org/10.3390/rs15184464
Chicago/Turabian StyleLan, Zeying, Yang Liu, Jianhua He, and Xin Hu. 2023. "PolSAR Image Classification by Introducing POA and HA Variances" Remote Sensing 15, no. 18: 4464. https://doi.org/10.3390/rs15184464
APA StyleLan, Z., Liu, Y., He, J., & Hu, X. (2023). PolSAR Image Classification by Introducing POA and HA Variances. Remote Sensing, 15(18), 4464. https://doi.org/10.3390/rs15184464






